Curve Fitting for Damage Evolution through Regression Analysis for the Kachanov–Rabotnov Model to the Norton–Bailey Creep Law of SS-316 Material
Abstract
:1. Introduction
2. Theoretical Framework
2.1. Norton Bailey Model
2.2. Omega Model
2.3. Kachanov–Rabotnov Model
3. Methodology
3.1. Analytical Creep Strain
3.2. Finite Element Simulation—The Regression Model
3.3. Development of Model in Finite Element Analysis
3.4. Sensitivity Analysis Using RSM and ANOVA
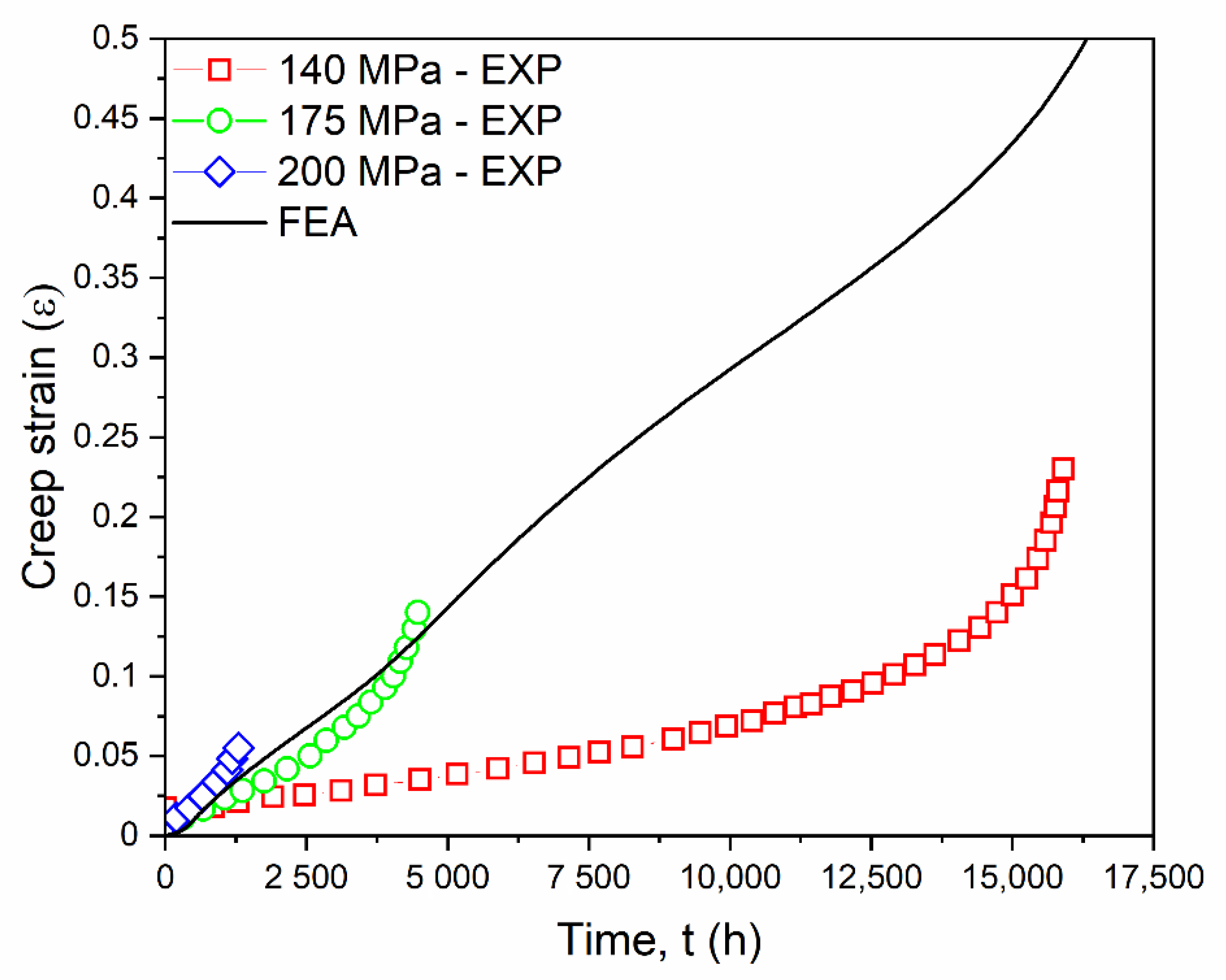
3.5. Model Validation
4. Results
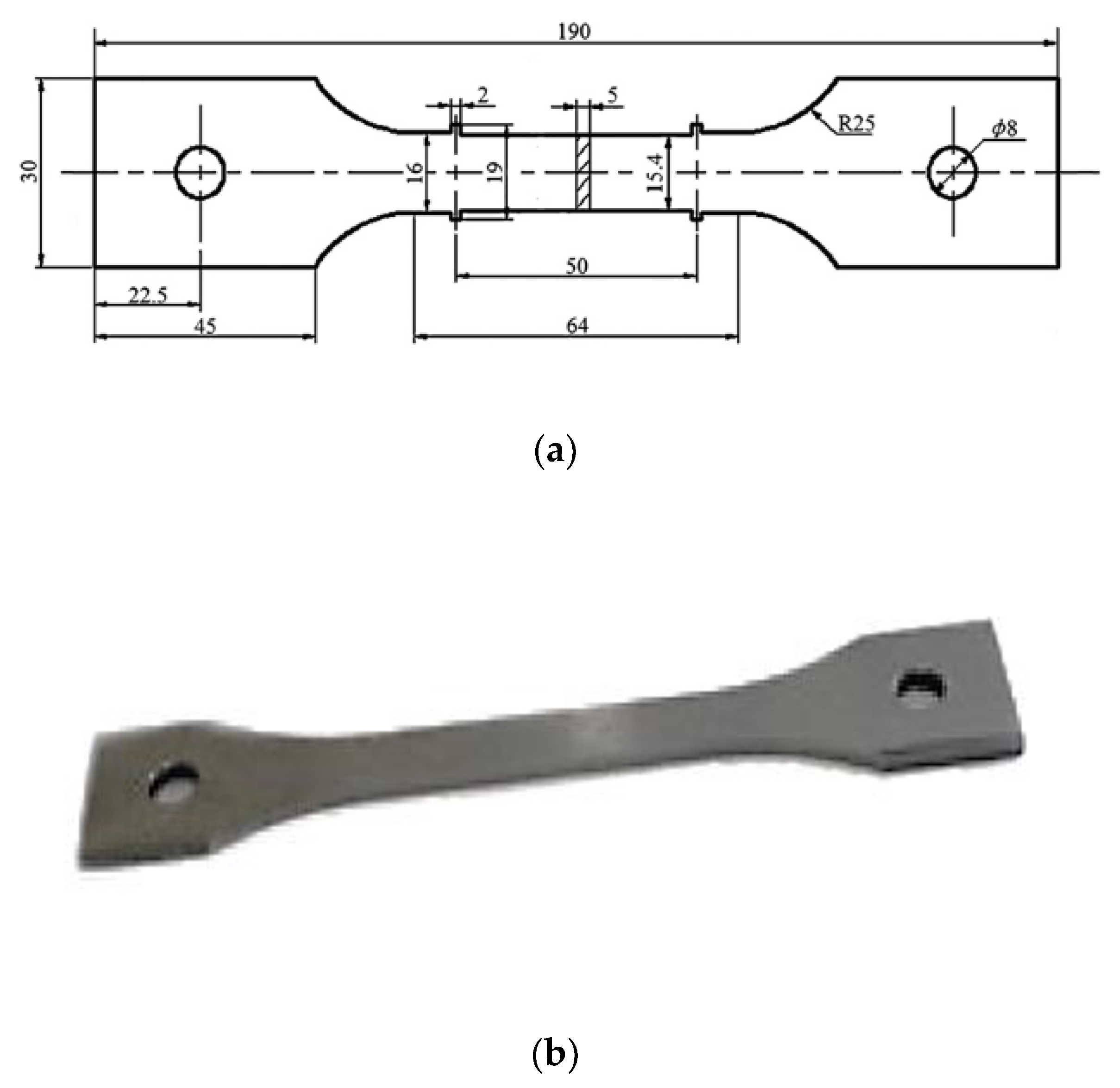
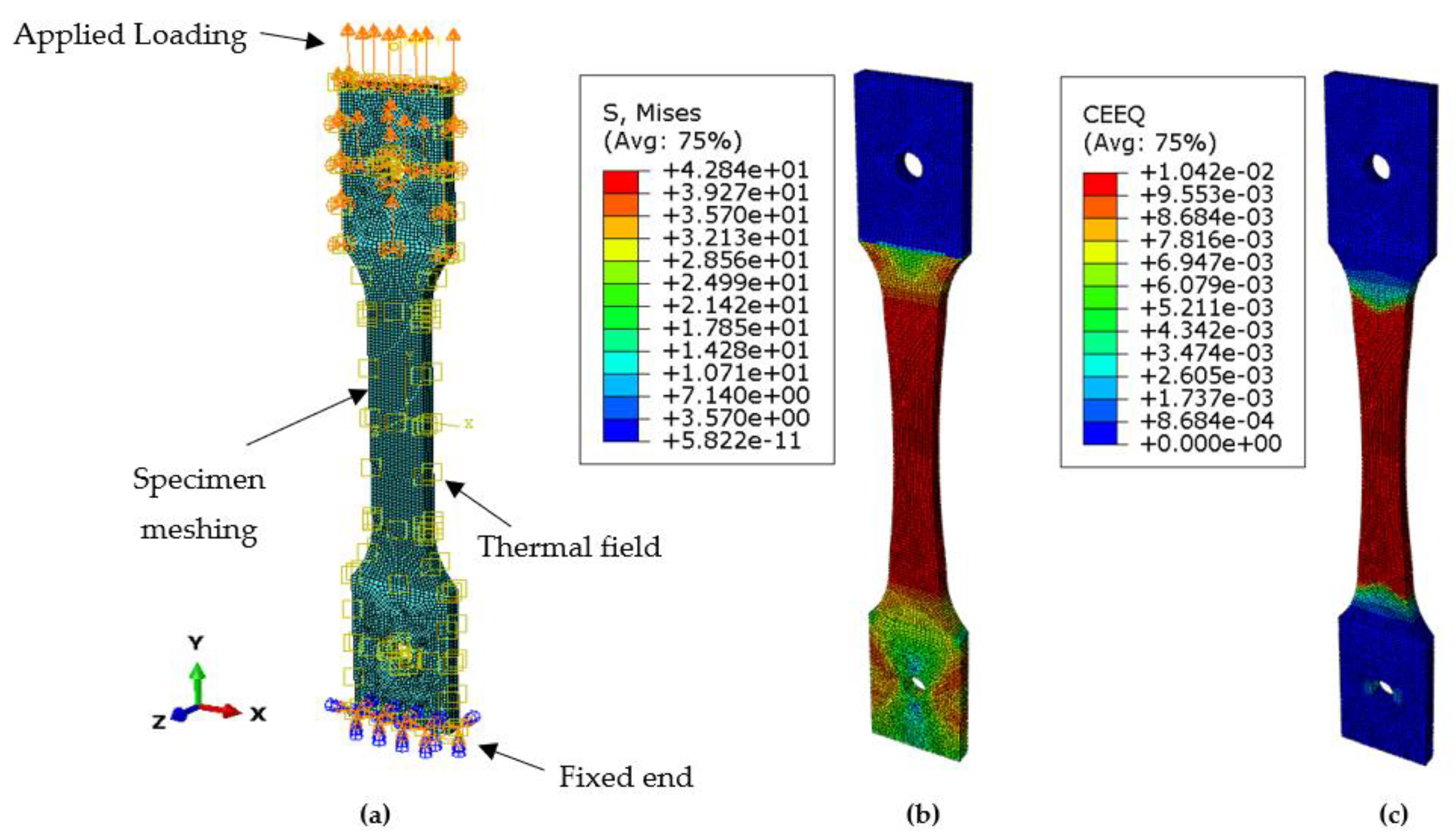
4.1. Dog Bone Specimen Simulation
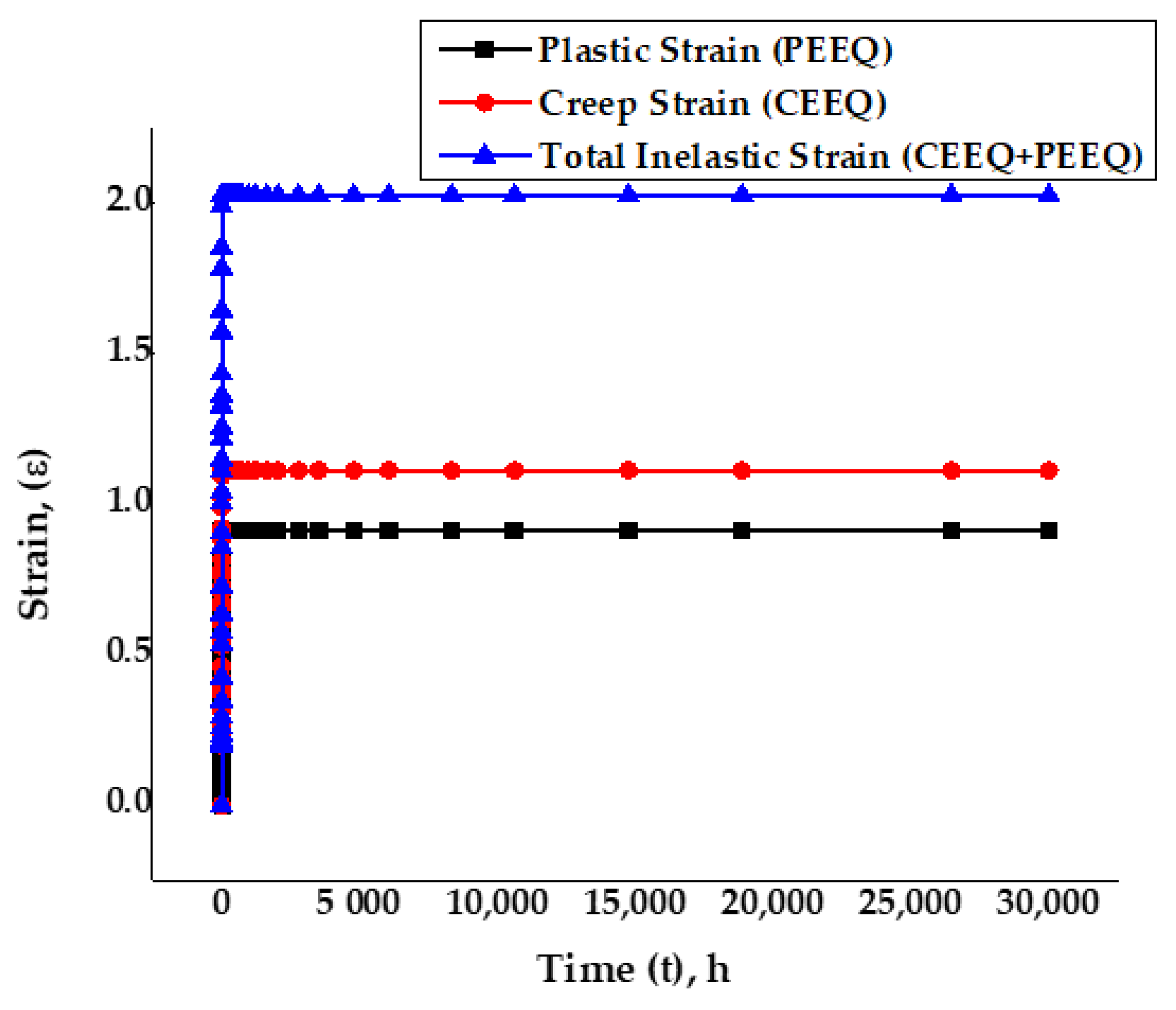
4.2. Creep and Plastic Strain Initiation and Propagation
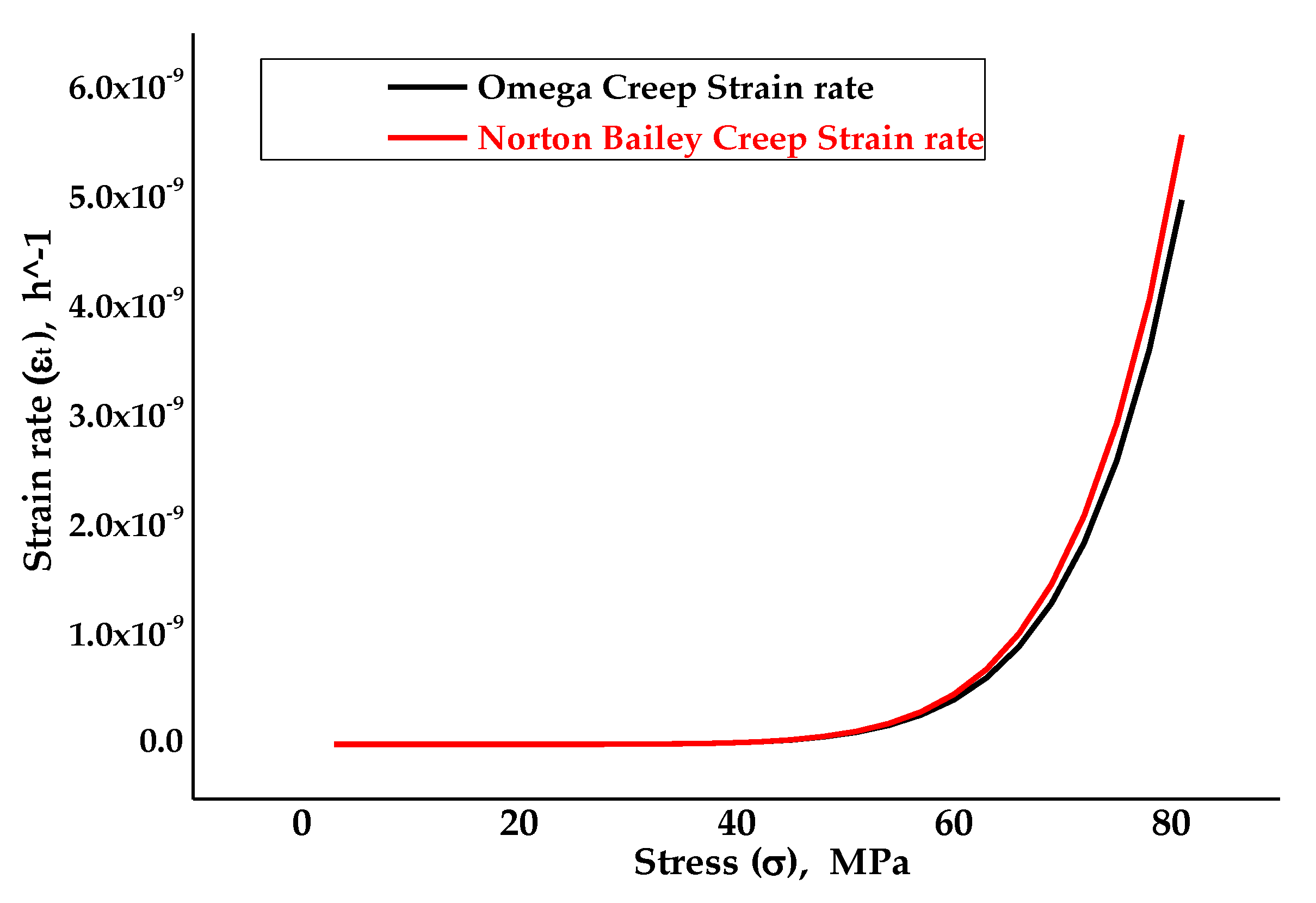
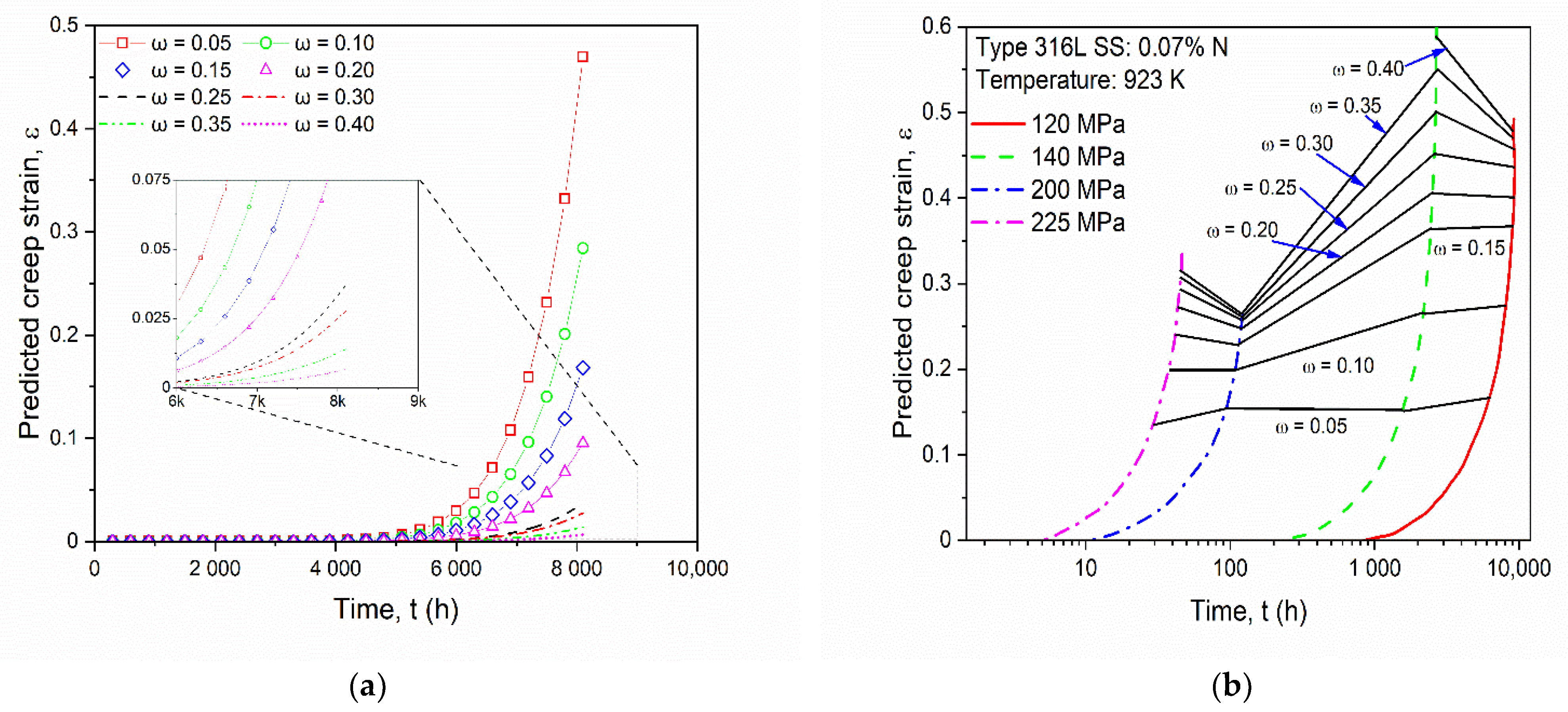
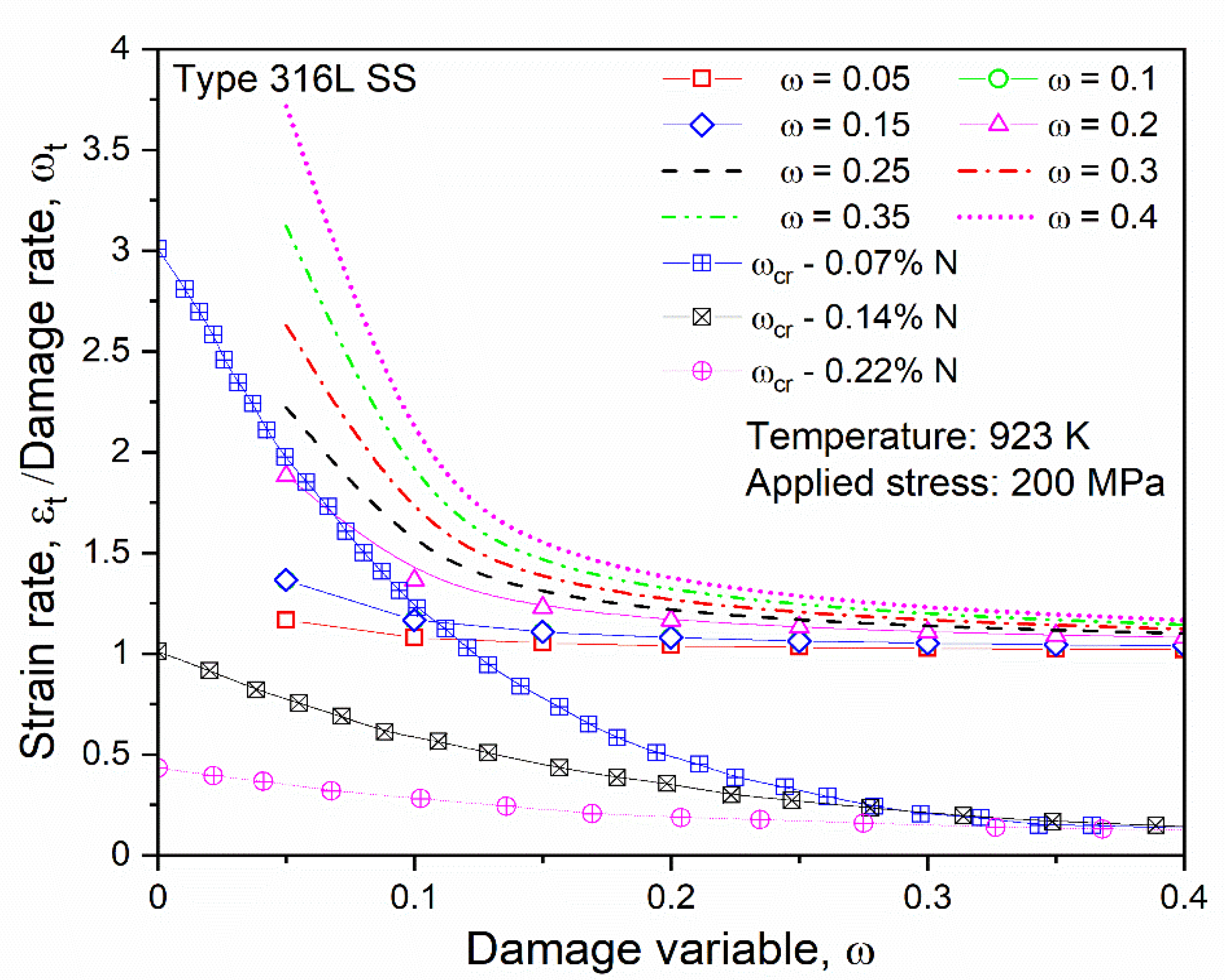
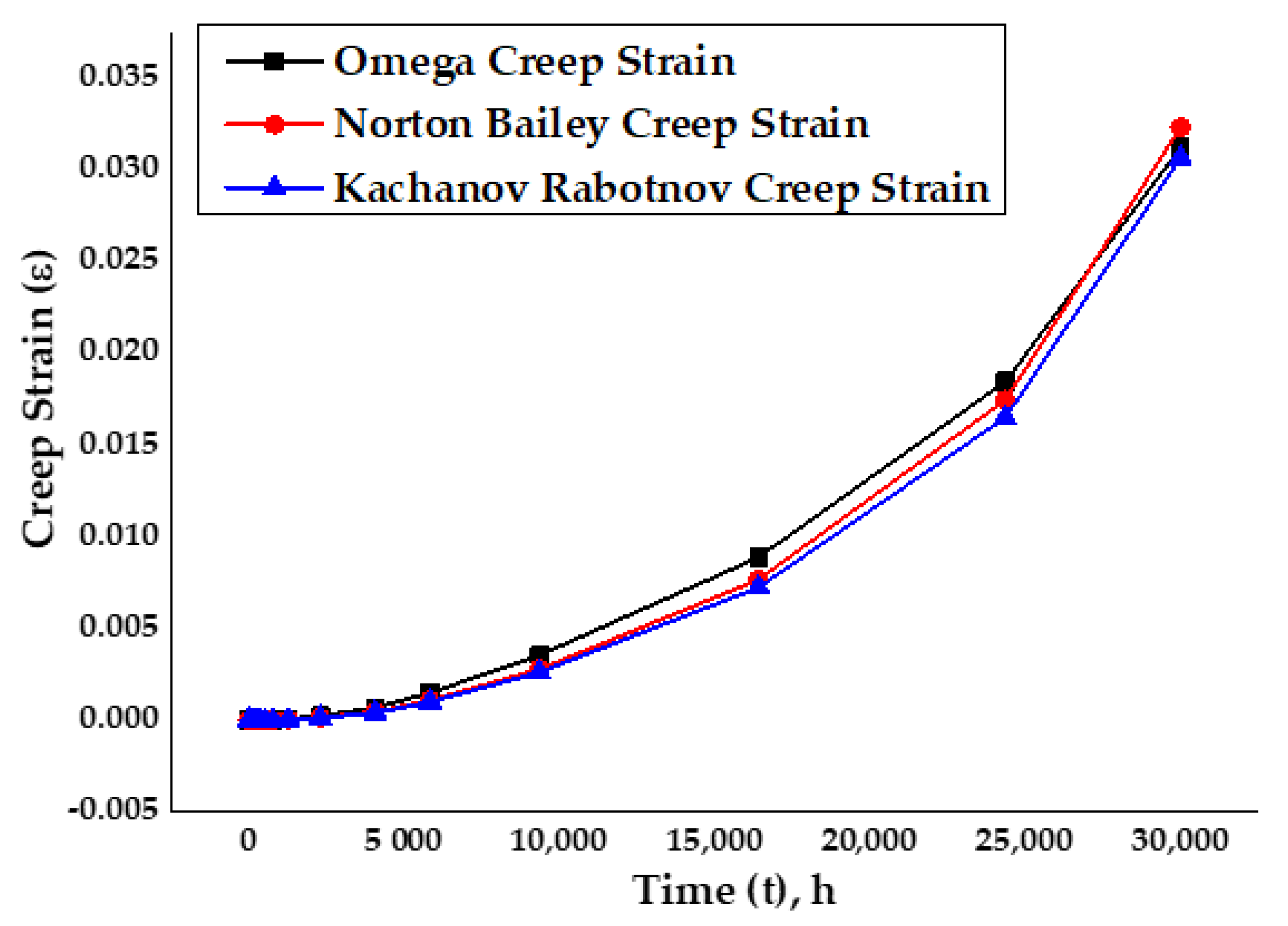
4.3. Omega, Norton–Bailey, and Kachanov–Rabotnov Models Comparison
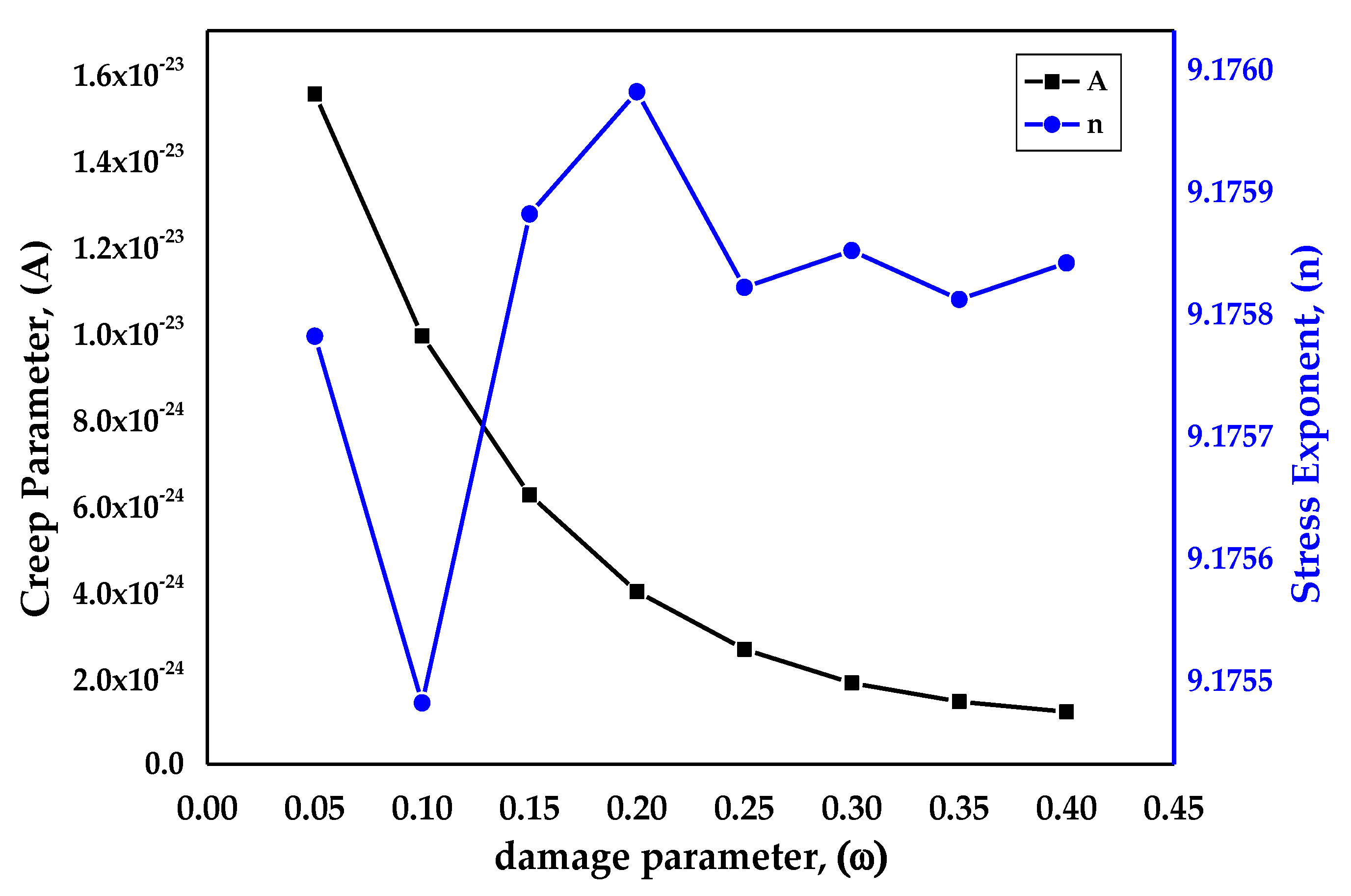
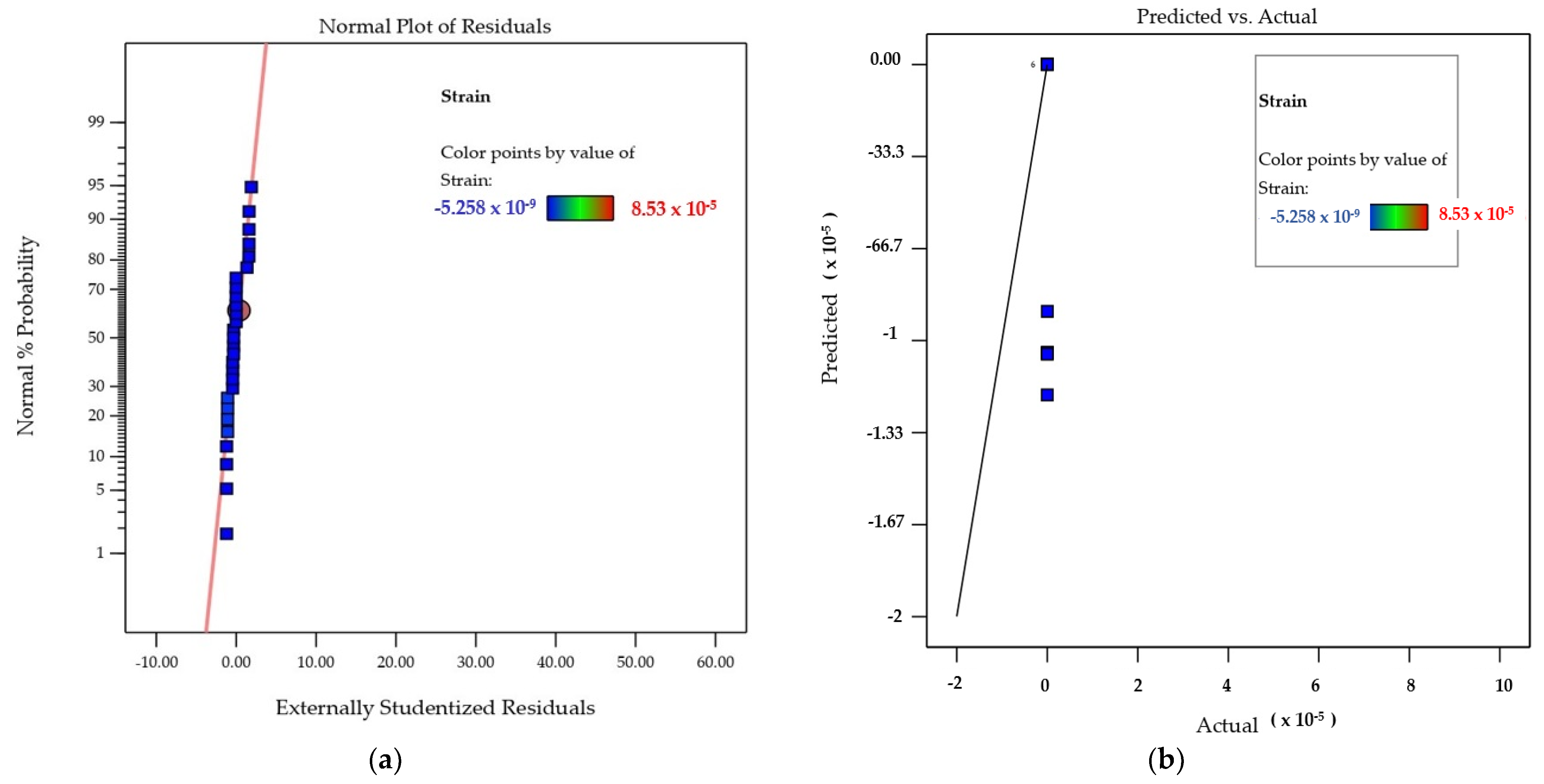
4.4. Data Optimization by Statistical Modelling
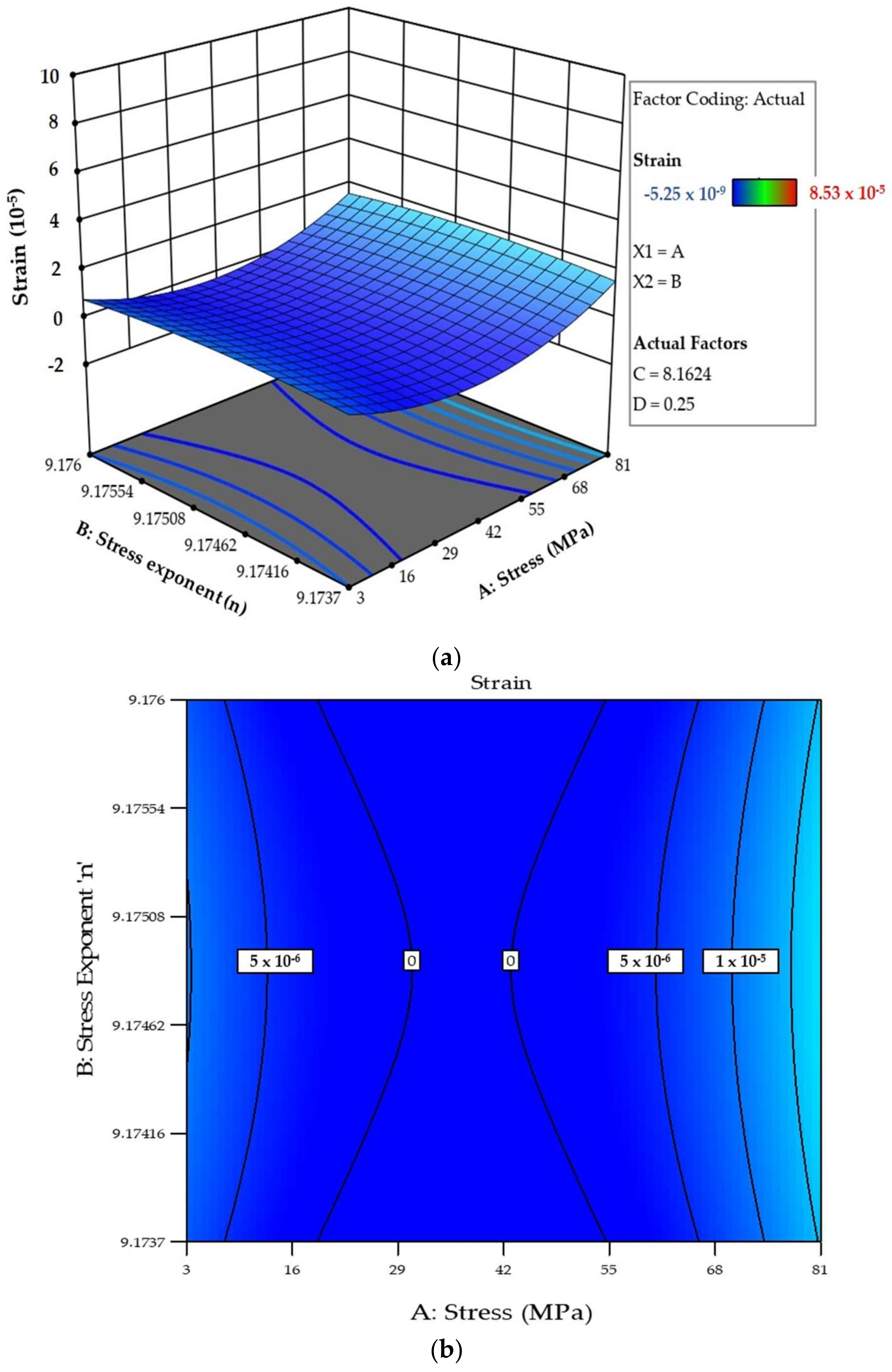
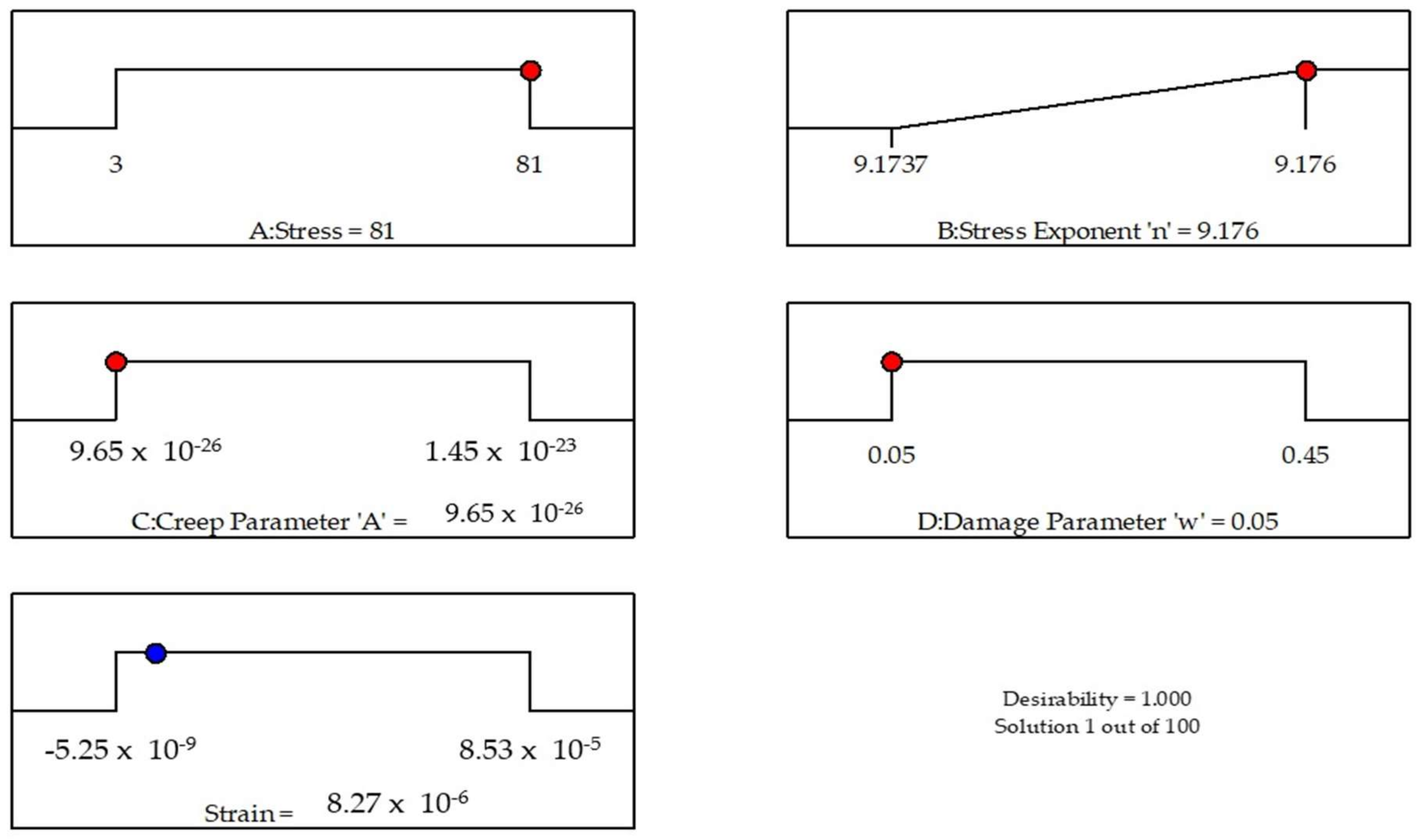
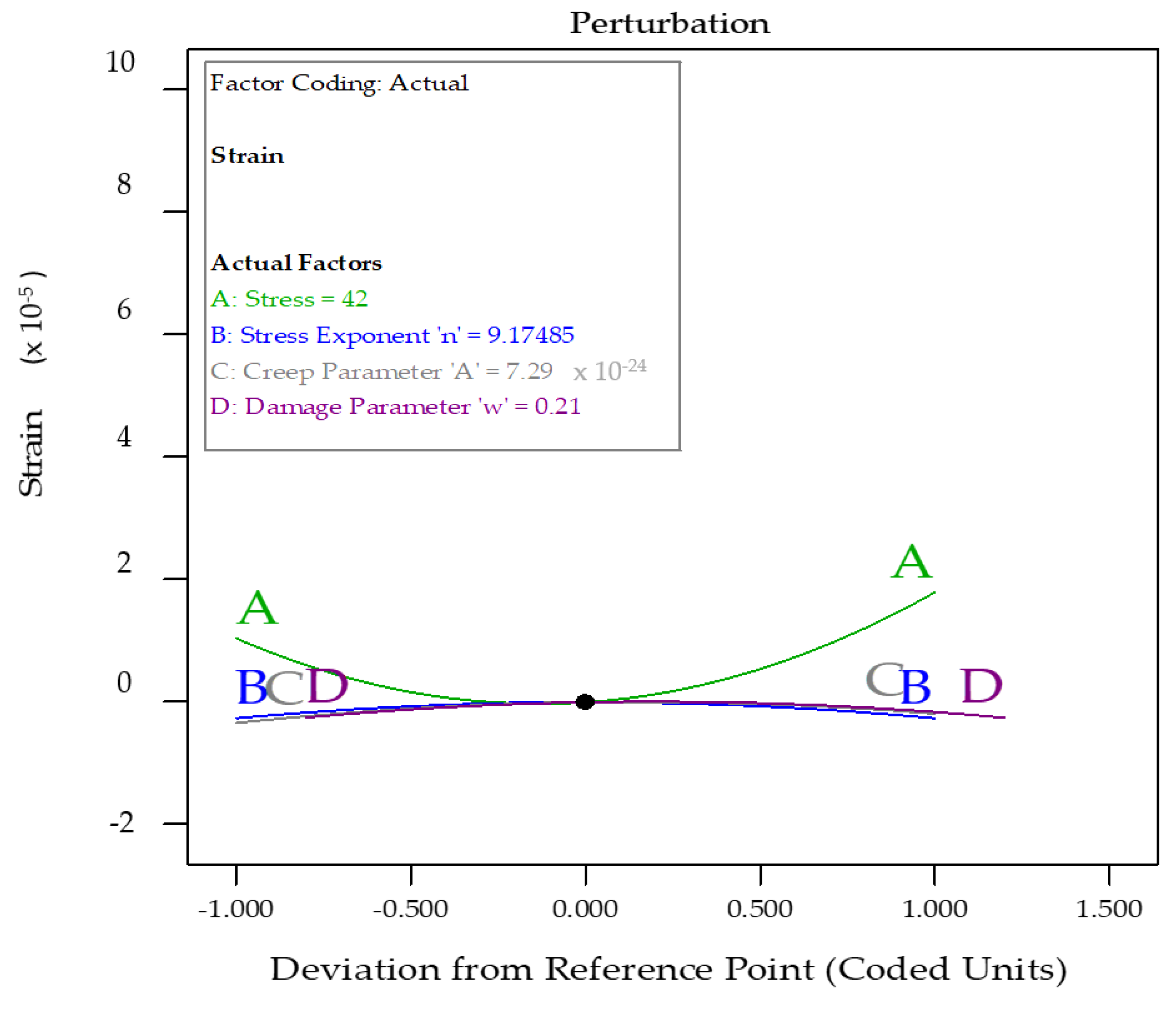
4.5. Discrete Effects of Factors on the Response
5. Conclusions
- (1)
- The method formulated in this article can be applied to curve-fit tertiary creep damage evolution parameters and to run creep analysis by finite element methods for any material. By deriving a damage evolution constant in the equation, a material’s behavior in the tertiary stage can be identified and predicted. A complete creep curve can be obtained covering all three stages by applying this technique.
- (2)
- From the results it is clear that the Omega model can work as a tool, because creep strain analytical data can be extracted from ASME FFS-1/API-579 standards and applied to embedded Norton–Bailey and Kachanov–Rabotnov models by regression analysis in Abaqus for any material. Obtained creep parameters work as inputs along with other parameters in the FEA package for damage evolution. Comparative assessment for creep strain was made among the Omega, Kachanov–Rabotnov, and Norton–Bailey models based on the proposed curve-fitting technique.
- (3)
- The fit statistics for the quadratic model of creep strain points revealed that the anticipated and simulated/actual values were more closely aligned. This proved that the quadratic model could navigate the design space effectively. Furthermore, as evidenced by their p-values, the interaction terms of mixing conditions had a substantial influence on the variables and the response.
- (4)
- Detailed statistical analysis and successive geometric optimization were performed using the response surface modelling approach and the ANOVA technique. The resulting 3D surface plot was analysed to comprehend the combined effect of the design factors: stress, the stress exponent, the creep parameter, and the damage evolution parameter on the relevant response: strain. The impact on the strain response was analysed and investigated with the help of contour creep deformation maps.
- (5)
- The FEA model was validated with the published experimental creep test, and the results showed good agreement between simulated and experimental results. Hence, the model was validated and applied. The combined effects in uncertainties can be removed by increasing the sample size of the creep data and for further extrapolation for creep prediction.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Norton’s power law constant |
| R | Universal gas constant |
| T | Temperature |
| tr | rupture time |
| Q | activation energy |
| Qc | Norton’s activation energy |
| σ1, σ2 & σ3 | principal stresses |
| S1 | Stress parameter |
| δΩ | Omega parameter |
| α | Triaxiality parameter |
| ε0 | Initial creep strain |
| n | Norton’s power law constant |
| ω | Omega damage parameter |
| εt | Creep strain rate |
| Ω | Omega material damage constant |
| σe | Effective stress |
| Ωm | Omega multiaxial damage parameter |
| Ωt | Omega material damage constant with respect to time |
| Ωn | Omega uniaxial damage parameter |
| ∆cd | Adjustment factor for creep ductility |
| εΩ | Accumulated creep strain |
| A0 | Stress coefficient |
| AΩ | Stress coefficient |
| QΩ | Temperature dependence of Ω |
| Creep rupture life | |
| nBN | Norton–Bailey coefficient |
| Trefa | Reference temperature |
| βΩ | Omega parameter to 0.33 |
| FEA | Finite element analysis |
| FFS | Fitness for service |
| API | American Petroleum Institute |
| MPC | Material Properties Council |
| UTS | Ultimate tensile strength |
| BPVC | Boiler and pressure vessel codes |
| ASME | American Society for Mechanical Engineers |
| ASTM | American Standards for Testing of Materials |
| UTS | Ultimate tensile strength |
| SS | Stainless steel |
| CDM | Continuum damage mechanics |
References
- Oxide, A. Multiaxial Creep Models. In High Temperature Deformation and Fracture of Materials, 3rd ed.; Zhang, J.-S., Ed.; Woodhead Publishing: Cambridge, UK, 2010; pp. 182–187. [Google Scholar]
- Dyson, B. Use of CDM in Materials Modelling and Component Creep Life Prediction. J. Press. Vessel Technol. Trans. ASME 2000, 122, 281–296. [Google Scholar] [CrossRef]
- Chaboche, J.-L. Continuum Damage Mechanics. Part II. J. Appl. Mech. 1988, 55, 59–72. [Google Scholar] [CrossRef]
- Murakami, S. Continuum Damage Mechanics. In Solid Mechanics and Its Applications; Springer: New York, NY, USA, 2012; Volume 185, ISBN 9789400726659. [Google Scholar]
- Betten, J. Creep Mechanics, 3rd ed.; Springer: Berlin, Germany, 2008; Volume 53, ISBN 9788578110796. [Google Scholar]
- Kachanov, L.M. Rupture Time under Creep Conditions. Int. J. Fract. 1999, 97, 11–18. [Google Scholar] [CrossRef]
- Skrzypek, A.G.J. Modeling of Material Damage and Failure of Structures; Springer: New York, NY, USA, 1998. [Google Scholar]
- Stewart, C.M.; Gordon, A.P. Methods to Determine the Critical Damage Criterion of the Kachanov-Rabotnov Law. ASME Int. Mech. Eng. Congr. Expo. Proc. 2012, 3, 663–670. [Google Scholar] [CrossRef]
- Hayhurst, D.R. Use of Continuum Damage Mechanics in Creep Analysis for Design. J. Strain Anal. Eng. Des. 1994, 29, 233–241. [Google Scholar] [CrossRef]
- Yao, H.T.; Xuan, F.Z.; Wang, Z.; Tu, S.T. A Review of Creep Analysis and Design under Multi-Axial Stress States. Nucl. Eng. Des. 2007, 237, 1969–1986. [Google Scholar] [CrossRef]
- Esposito, L.; Bonora, N. Time-Independent Formulation for Creep Damage Modeling in Metals based on Void and Crack Evolution. Mater. Sci. Eng. A 2009, 510–511, 207–213. [Google Scholar] [CrossRef]
- Norton, F. The Creep of Steels at High Temperatures; Mc Graw Hill: New York, NY, USA, 1929; Volume 1, p. 90. [Google Scholar]
- Prager, M. Development of the MPC Omega Method for Life Assessment in the Creep Range. J. Press. Vessel Technol. Trans. ASME 1995, 117, 95–103. [Google Scholar] [CrossRef]
- Yeom, J.T.; Kim, J.Y.; Na, Y.S.; Park, N.K. Creep Strain and Creep-Life Prediction for Alloy 718 Using the Omega Method. J. Met. Mater. Int. 2003, 9, 555–560. [Google Scholar] [CrossRef]
- Prager, M. The Omega Method—An Engineering Approach to Life Assessment. J. Press. Vessel. Technol. 2000, 122, 273–280. [Google Scholar] [CrossRef]
- Stewart, C.M.; Gordon, A.P. Strain and Damage-based Analytical Methods to Determine the Kachanov-Rabotnov Tertiary Creep-Damage Constants. Int. J. Damage Mech. 2012, 21, 1186–1201. [Google Scholar] [CrossRef]
- Evans, R.W.; Parker, J.D.; Wilshire, B. The θ Projection Concept-A Model-Based Approach to Design and Life Extension of Engineering Plant. Int. J. Press. Vessel. Pip. 1992, 50, 147–160. [Google Scholar] [CrossRef]
- Alipour, R.; Nejad, A.F. Creep Behaviour Characterisation of a Ferritic Steel Alloy based on the Modified Theta-Projection Data at an Elevated Temperature. Int. J. Mater. Res. 2016, 107, 406–412. [Google Scholar] [CrossRef]
- Stewart, C.M. A Hybrid Constitutive Model for Creep, Fatigue, and Creep-Fatigue Damage; University of Central Florida: Orlando, FL, USA, 2013. [Google Scholar]
- Alipour, R.; Farokhi Nejad, A.; Nilsaz Dezfouli, H. Steady State Creep Characteristics of a Ferritic Steel at Elevated Temperature: An Experimental and Numerical Study. ADMT J. 2018, 11, 115–129. [Google Scholar]
- Benallal, A.; Billardon, R.; Lemaitre, J. Continuum Damage Mechanics and Local Approach to Fracture: Numerical Procedures. Comput. Methods Appl. Mech. Eng. 1991, 92, 141–155. [Google Scholar] [CrossRef]
- Batsoulas, N.D. Mathematical Description of the Mechanical Behaviour of Metallic Materials under Creep Conditions. J. Mater. Sci. 1997, 32, 2511–2527. [Google Scholar] [CrossRef]
- Le May, I.; Furtado, H.C. Creep Damage Assessment and Remaining Life Evaluation. Int. J. Fract. 1999, 97, 125–135. [Google Scholar] [CrossRef]
- Maruyama, K.; Nonaka, I.; Sawada, K.; Sato, H.; Koike, J.I.; Umaki, H. Improvement of Omega Method for Creep Life Prediction. ISIJ Int. 1997, 37, 419–423. [Google Scholar] [CrossRef] [Green Version]
- Chen, H.; Zhu, G.R.; Gong, J.M. Creep Life Prediction for P91/12Cr1MoV Dissimilar Joint Based on the Omega Method. Procedia Eng. 2015, 130, 1143–1147. [Google Scholar] [CrossRef] [Green Version]
- Penny, R.K.; Weber, M.A. Robust Methods of Life Assessment During Creep. Int. J. Press. Vessel. Pip. 1992, 50, 109–131. [Google Scholar] [CrossRef]
- Haque, M.S.; Stewart, C.M. Comparative Analysis of the Sin-Hyperbolic and Kachanov–Rabotnov Creep-Damage Models. Int. J. Press. Vessel. Pip. 2019, 171, 1–9. [Google Scholar] [CrossRef]
- Haque, M.S.; Stewart, C.M. The Disparate Data Problem: The Calibration of Creep Laws across Test type and Stress, Temperature, and Time Scales. Theor. Appl. Fract. Mech. 2019, 100, 251–268. [Google Scholar] [CrossRef]
- Stewart, C.M.; Gordon, A.P. Analytical Method to Determine the Tertiary Creep Damage Constants of the Kachanov-Rabotnov Constitutive Model. In Proceedings of the ASME, International Mechanical Engineering Congress & Exposition IMECE2010, Vancouver, BC, Canada, 12–18 November 2010; pp. 1–8. [Google Scholar]
- Engels, F. A sensitivity Study on Creep Crack growth in Pipes. In Proceedings of the ASME 2002, Pressure Vessels & Piping Conference, Vancouver, BC, Canada, 5–9 August 2002; pp. 1–9. [Google Scholar]
- Sui, F.; Sandström, R. Basic Modelling of Tertiary Creep of Copper. J. Mater. Sci. 2018, 53, 6850–6863. [Google Scholar] [CrossRef] [Green Version]
- Brown, S.G.R.; Evans, R.W.; Wilshire, B. A Comparison of Extrapolation Techniques for Long-term Creep Strain and Creep Life Prediction based on Equations Designed to represent Creep Curve Shape. Int. J. Press. Vessel. Pip. 1986, 24, 251–268. [Google Scholar] [CrossRef]
- Bolton, J. A Visual Perspective of Creep Rupture Extrapolation. Mater. High Temp. 2013, 30, 87–98. [Google Scholar] [CrossRef]
- Wafles, K.F.A.; Graham, A. On the Extrapolation and Scatter of Creep Data; Her Majesty’s Stationery Office: London, UK, 1963.
- Potirniche, G. Prediction and Monitoring Systems of Creep-Fracture Behavior of 9Cr-1Mo Steels for Reactor Pressure Vessels; US Department of Energy: Washington, DC, USA, 2013.
- Kucuk, U.; Eyuboglu, M.; Kucuk, H.O.; Degirmencioglu, G. Importance of using Proper Post HOC Test with ANOVA. Int. J. Cardiol. 2016, 209, 346. [Google Scholar] [CrossRef]
- Mahmood, T. Integrity Assessment of Pressure Components Operating with Creep Regime; The University of New South Whales: Sydney, Australia, 2014. [Google Scholar]
- Abdallah, Z.; Gray, V.; Whittaker, M.; Perkins, K. A Critical Analysis of the Conventionally Employed Creep Lifing Methods. Materials 2014, 7, 3371–3398. [Google Scholar] [CrossRef] [Green Version]
- May, D.L.; Gordon, A.P.; Segletes, D.S. The Application of the Norton-Bailey Law for Creep Prediction through Power Law Regression. In Proceedings of the ASME Turbo Expo, San Antonio, TX, USA, 3–7 June 2013; Volume 7A, pp. 1–8. [Google Scholar]
- Manu, C.C.; Birk, A.M.; Kim, I.Y. Uniaxial High-Temperature Creep Property Predictions made by CDM and MPC Omega Techniques for ASME SA 455 Steel. Eng. Fail. Anal. 2009, 16, 1303–1313. [Google Scholar] [CrossRef]
- Kwon, O.; Thomas, C.; Knowles, D.; Saunders-Tack, A. Remnant Life Assessment of Platformer Heater T9 Tubes using API 579 Omega Method. In Volume 9: Eighth International Conference on Creep and Fatigue at Elevated Temperatures, Proceedings of the ASME 2007 Pressure Vessels and Piping Conference, San Antonio, TX, USA, 22–26 July 2007; ASME: New York, NY, USA, 2007; pp. 389–397. [Google Scholar]
- Stewart, C.M.; Gordon, A.P.; Hogan, E.A.; Saxena, A. Characterization of the Creep Deformation and Rupture Behavior of DS GTD-111 using the Kachanov-Rabotnov Constitutive Model. J. Eng. Mater. Technol. Trans. ASME 2011, 133. [Google Scholar] [CrossRef]
- ASME. American Petroleum Institute API-579, Fitness for Service, 3rd ed.; API: Washington, DC, USA, 2016; 1320p. [Google Scholar]
- ASME Boiler and Pressure Vessel Code. ASME Boiler and Pressure Vessel Code an International Code—Section II Part A; American Society of Mechanical Engineers: New York, NY, USA, 1998. [Google Scholar] [CrossRef]
- Christopher, J.; Praveen, C.; Ganesan, V.; Reddy, G.P.; Albert, S.K. Influence of Varying Nitrogen on Creep Deformation and Damage Behaviour of Type 316L in the Framework of Continuum Damage Mechanics Approach. Int. J. Damage Mech. 2021, 30, 3–24. [Google Scholar] [CrossRef]
- Holmstrom, S.; Auerkari, P. Robust Prediction of Full Creep Curves from Minimal Data and Time to Rupture Model. Energy Mater. 2006, 1, 249–255. [Google Scholar] [CrossRef]
- Hu, M.; Li, K.; Li, S.; Cai, Z.; Pan, J. Analytical Model to Compare and select Creep Constitutive Equation for Stress Relief Investigation during Heat Treatment in Ferritic Welded Structure. Metals 2020, 10, 688. [Google Scholar] [CrossRef]
- Sattar, M.; Othman, A.R.; Othman, M.F.; Musa, F. Regression Analysis of Omega Model to Norton-Bailey Law for Creep Prediction in Fitness for Service Assessment of Steel Material. Solid State Technol. 2020, 63, 1228–1239. [Google Scholar]
- Eno, D.R.; Young, G.A.; Sham, T.L. A Unified View of Engineering Creep Parameters. In Proceedings of the ASME 2008 Pressure Vessels and Piping Conference, Chicago, IL, USA , 27–31 July 2008; 6, pp. 777–792. [Google Scholar] [CrossRef]
- Booker, M.K. Use of Generalized Regression Models for the Analysis of Stress-Rupture Data. In Proceedings of the ASME/CSME Pressure Vessels and Piping Conference, Montreal, QC, Canada, 25 June 1978; pp. 459–499. [Google Scholar]
- Al-Bakri, A.A.; Sajuri, Z.; Ariffin, A.K.; Razzaq, M.A.; Fafmin, M.S. Tensile and Fracture Behaviour of very thin 304 Stainless Steel Sheet. J. Teknol. 2016, 78, 45–50. [Google Scholar] [CrossRef] [Green Version]
- Xiao, B.; Xu, L.; Zhao, L.; Jing, H.; Han, Y.; Zhang, Y. Creep Properties, Creep Deformation Behavior, and Microstructural Evolution of 9Cr-3W-3Co-1CuVNbB Martensite Ferritic Steel. Mater. Sci. Eng. A 2018, 711, 434–447. [Google Scholar] [CrossRef]
- Alemayehu, D.B.; Huang, S.J.; Koricho, E.G. Experimental and FEM Analysis of three Carbon steel Characterization under Quasi-Static Strain Rate for Bumper Beam Application. In Proceedings of the 2017 The 2nd International Conference on Precision Machinery and Manufacturing Technology, Kenting, Taiwan, 19–21 May 2017; Volume 123. [Google Scholar] [CrossRef] [Green Version]
- Alam, M.A.; Ya, H.H.; Azeem, M.; Bin Hussain, P.; bin Salit, M.S.; Khan, R.; Arif, S.; Ansari, A.H. Modelling and Optimisation of Hardness Behaviour of Sintered Al/SiC Composites using RSM and ANN: A Comparative Study. J. Mater. Res. Technol. 2020, 9, 14036–14050. [Google Scholar] [CrossRef]
- LeRoy, A.F.; Belva, J.C. An Experiential Approach to Integrating ANOVA Concepts. J. Stat. Educ. 2002, 10. [Google Scholar] [CrossRef] [Green Version]
- Memon, A.M.; Hartadi Sutanto, M.; Napiah, M.; Khan, M.I.; Rafiq, W. Modeling and Optimization of Mixing Conditions for Petroleum Sludge Modified Bitumen using Response Surface Methodology. Constr. Build. Mater. 2020, 264, 120701. [Google Scholar] [CrossRef]
- Fraiman, D.; Fraiman, R. An ANOVA Approach for Statistical Comparisons of Brain Networks. Sci. Rep. 2018, 8, 4746. [Google Scholar] [CrossRef]
- Said, K.A.M.; Yakub, I.; Amin, M.A.M. Overview of Response Surface Methodology (RSM) in Extraction Process. J. Appl. Sci. Process Eng. 2015, 2, 279–287. [Google Scholar] [CrossRef]
- Lai, J.; Wang, H.; Wang, D.; Fang, F.; Wang, F.; Wu, T. Ultrasonic Extraction of Antioxidants from Chinese Sumac (Rhus typhina L.) Fruit using Response Surface Methodology and their Characterization. Molecules 2014, 19, 9019–9032. [Google Scholar] [CrossRef]
- Alam, M.A.; Ya, H.H.; Yusuf, M.; Sivraj, R.; Mamat, O.B.; Sapuan, M.S.; Masood, F.; Parveez, B.; Sattar, M. Modeling, Optimization and Performance Evaluation of Response Surface Methodology. Materials 2021, 14, 4703. [Google Scholar] [CrossRef]
- Kumari, M.; Gupta, S.K. Response Surface Methodological (RSM) Approach for Optimizing the Removal of Trihalomethanes (THMs) and its Precursor’s by Surfactant Modified Magnetic Nanoadsorbents (sMNP)—An Endeavor to diminish Probable Cancer Risk. Sci. Rep. 2019, 9, 18339. [Google Scholar] [CrossRef]
- Morero, B.; Groppelli, E.S.; Campanella, E.A. Evaluation of Biogas Upgrading Technologies using a Response Surface Methodology for Process Simulation. J. Clean. Prod. 2017, 141, 978–988. [Google Scholar] [CrossRef]





| Omega Parameter—Ω | |||
|---|---|---|---|
| A0 | −18.9 | B0 | −4.163 |
| A1 | 41,230.11 | B1 | 16,793.192 |
| A2 | −12,446.783 | B2 | −10,221.744 |
| A3 | 1299.221 | B3 | 1634.960 |
| A4 | 111.222 | B4 | 222.222 |
| Young’s Modulus (MPa) | Poisson’s Ratio | Temperature (°C) |
|---|---|---|
| 134,000 | 0.31 | −25 |
| 128,000 | 0.31 | 65 |
| 120,000 | 0.31 | 100 |
| 115,000 | 0.31 | 125 |
| 111,000 | 0.31 | 150 |
| 104,000 | 0.31 | 200 |
| 97,600 | 0.31 | 250 |
| 93,100 | 0.31 | 300 |
| 90,700 | 0.31 | 325 |
| 88,400 | 0.31 | 350 |
| 86,600 | 0.31 | 375 |
| 84,700 | 0.31 | 400 |
| 83,500 | 0.31 | 425 |
| 82,300 | 0.31 | 450 |
| 80,500 | 0.31 | 475 |
| 79,100 | 0.31 | 500 |
| 77,800 | 0.31 | 525 |
| 76,800 | 0.31 | 550 |
| 74,700 | 0.31 | 575 |
| 70,000 | 0.31 | 600 |
| 55,300 | 0.31 | 625 |
| 42,900 | 0.31 | 650 |
| Creep Parameter A | Stress Exponent n | Temperature °C |
|---|---|---|
| 1.71460 × | 9.37430 | 630 |
| 2.96370 × | 9.32270 | 635 |
| 5.09125 × | 9.27170 | 640 |
| 8.70190 × | 9.22120 | 645 |
| 1.47830 × | 9.17100 | 650 |
| 2.48800 × | 9.12180 | 655 |
| 4.19040 × | 9.07300 | 660 |
| 7.00796 × | 9.02400 | 665 |
| 1.1612 × | 8.97670 | 670 |
| 1.9187 × | 8.92940 | 675 |
| Std | Run | Factor 1 A: Stress, σ (MPa) | Factor 2 B: Stress Exponent, n | Factor 3 C: Creep Parameter, A (MPa−n h−1) | Factor 4 D: Damage Parameter (ω) | Response: Strain (ε) |
|---|---|---|---|---|---|---|
| 29 | 1 | 42 | 9.17485 | 7.29825 × 10−24 | 0.25 | 5.40131 × 10−9 |
| 3 | 2 | 3 | 9.176 | 9.65 × 10−26 | 0.05 | 1.97521 × 10−21 |
| 26 | 3 | 42 | 9.17485 | 7.29825 × 10−24 | 0.25 | 5.40131 × 10−9 |
| 21 | 4 | 42 | 9.17485 | 2.17017 × 10−23 | 0.25 | 1.6061 × 10−8 |
| 5 | 5 | 3 | 9.1737 | 1.45 × 10−23 | 0.05 | 2.96056 × 10−19 |
| 23 | 6 | 42 | 9.17485 | 7.29825 × 10−24 | 0.65 | 4.94469 × 10−9 |
| 15 | 7 | 3 | 9.176 | 1.45 × 10−23 | 0.45 | 7.79436 × 10−20 |
| 25 | 8 | 42 | 9.17485 | 7.29825 × 10−24 | 0.25 | 5.40131 × 10−9 |
| 17 | 9 | 120 | 9.17485 | 7.29825 × 10−24 | 0.25 | 8.53267 × 10−5 |
| 14 | 10 | 81 | 9.1737 | 1.45 × 10−23 | 0.45 | 4.43651 × 10−6 |
| 12 | 11 | 81 | 9.176 | 9.65 × 10−26 | 0.45 | 2.98253 × 10−8 |
| 24 | 12 | 42 | 9.17485 | 7.29825 × 10−24 | 0.25 | 5.40131 × 10−9 |
| 9 | 13 | 3 | 9.1737 | 9.65 × 10−26 | 0.45 | 5.17613 × 10−22 |
| 10 | 14 | 81 | 9.1737 | 9.65 × 10−26 | 0.45 | 2.95257 × 10−8 |
| 13 | 15 | 3 | 9.1737 | 1.45 × 10−23 | 0.45 | 7.7776 × 10−20 |
| 18 | 16 | 42 | 9.17255 | 7.29825 × 10−24 | 0.25 | 5.35515 × 10−9 |
| 19 | 17 | 42 | 9.17715 | 7.29825 × 10−24 | 0.25 | 5.44787 × 10−9 |
| 28 | 18 | 42 | 9.17485 | 7.29825 × 10−24 | 0.25 | 5.44787 × 10−9 |
| 27 | 19 | 42 | 9.17485 | 7.29825 × 10−24 | 0.25 | 5.44787 × 10−9 |
| 4 | 20 | 81 | 9.176 | 9.65 × 10−26 | 0.05 | 3.12123 × 10−8 |
| 1 | 21 | 3 | 9.1737 | 9.65 × 10−26 | 0.05 | 1.97031 × 10−21 |
| 6 | 22 | 81 | 9.1737 | 1.45 × 10−23 | 0.05 | 4.64277 × 10−6 |
| 11 | 23 | 3 | 9.176 | 9.65 × 10−26 | 0.45 | 5.18728 × 10−22 |
| 22 | 24 | 42 | 9.17485 | 7.29825 × 10−24 | −0.15 | 5.89512 × 10−9 |
| 2 | 25 | 81 | 9.1737 | 9.65 × 10−26 | 0.05 | 3.08984 × 10−8 |
| 16 | 26 | 81 | 9.176 | 1.45 × 10−23 | 0.45 | 4.48152 × 10−6 |
| 7 | 27 | 3 | 9.176 | 1.45 × 10−23 | 0.05 | 2.96794 × 10−19 |
| 8 | 28 | 81 | 9.176 | 1.45 × 10−23 | 0.05 | 4.68992 × 10−6 |
| Statistical Parameters | Values | Remarks |
|---|---|---|
| R2 | 0.7643 | The quadratic model is significant to search the design space |
| Adjusted R2 | 0.5286 | |
| Predicted R2 | −0.9414 | |
| Adequate Precision | 9.7876 |
| Source | Sum of Squares | df | Mean Square | F-Value | p-Value | |
|---|---|---|---|---|---|---|
| Mean vs. Total | 3.713 × 10−10 | 1 | 3.713 × 10−10 | Suggested Aliased | ||
| Linear vs. Mean | 1.679 × 10−9 | 4 | 4.198 × 10−10 | 1.90 | 0.1439 | |
| 2FI vs. Linear | 2.056 × 10−11 | 6 | 3.427 × 10−12 | 0.0117 | 1.0000 | |
| Quadratic vs. 2FI | 3.654 × 10−9 | 4 | 9.112 × 10−10 | 7.74 | 0.0017 | |
| Cubic vs. Quadratic | 1.648 × 10−9 | 8 | 2.060 × 10−10 | 4.352 × 10−9 | <0.0001 | |
| Residual | 2.84 × 10−19 | 6 | 4.734 × 10−20 | |||
| Total | 7.364 × 10−9 | 29 | 2.539 × 10−10 |
| Source | Sum of Squares | df | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|
| Model | 5.345 × 10−9 | 14 | 3.818 × 10−10 | 3.24 | 0.0176 |
| A-Stress | 2.428 × 10−10 | 1 | 2.428 × 10−10 | 2.06 | 0.1729 |
| B-Stress Exponent n | 3.601 × 10−16 | 1 | 3.601 × 10−16 | 3.059 × 10−6 | 0.9986 |
| C-Creep Parameter A | 1.376 × 10−11 | 1 | 1.376 × 10−11 | 0.1169 | 0.7375 |
| D-Damage Parameter ω | 7.326 × 10−15 | 1 | 7.326 × 10−15 | 0.0001 | 0.9938 |
| AB | 5.380 × 10−16 | 1 | 5.380 × 10−16 | 4.570 × 10−6 | 0.9983 |
| AC | 2.054 × 10−11 | 1 | 2.054 × 10−11 | 0.1745 | 0.6825 |
| AD | 1.089 × 10−14 | 1 | 1.089 × 10−14 | 0.0001 | 0.9925 |
| BC | 5.239 × 10−16 | 1 | 5.239 × 10−16 | 4.450 × 10−6 | 0.9983 |
| BD | 2.917 × 10−19 | 1 | 2.917 × 10−19 | 2.478 × 10−9 | 1.0000 |
| CD | 1.060 × 10−14 | 1 | 1.060 × 10−14 | 0.0001 | 0.9926 |
| A2 | 3.235 × 10−9 | 1 | 3.235 × 10−9 | 27.48 | 0.0001 |
| B2 | 1.824 × 10−10 | 1 | 1.824 × 10−10 | 1.55 | 0.2337 |
| C2 | 1.824 × 10−10 | 1 | 1.824 × 10−10 | 1.55 | 0.2337 |
| D2 | 1.824 × 10−10 | 1 | 1.824 × 10−10 | 1.55 | 0.2337 |
| Lack of fit (LOF) | 1.648 × 10−9 | 9 | 1.831 × 10−10 | Insignificant LOF shows a good fit for the model | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sattar, M.; Othman, A.R.; Akhtar, M.; Kamaruddin, S.; Khan, R.; Masood, F.; Alam, M.A.; Azeem, M.; Mohsin, S. Curve Fitting for Damage Evolution through Regression Analysis for the Kachanov–Rabotnov Model to the Norton–Bailey Creep Law of SS-316 Material. Materials 2021, 14, 5518. https://doi.org/10.3390/ma14195518
Sattar M, Othman AR, Akhtar M, Kamaruddin S, Khan R, Masood F, Alam MA, Azeem M, Mohsin S. Curve Fitting for Damage Evolution through Regression Analysis for the Kachanov–Rabotnov Model to the Norton–Bailey Creep Law of SS-316 Material. Materials. 2021; 14(19):5518. https://doi.org/10.3390/ma14195518
Chicago/Turabian StyleSattar, Mohsin, Abdul Rahim Othman, Maaz Akhtar, Shahrul Kamaruddin, Rashid Khan, Faisal Masood, Mohammad Azad Alam, Mohammad Azeem, and Sumiya Mohsin. 2021. "Curve Fitting for Damage Evolution through Regression Analysis for the Kachanov–Rabotnov Model to the Norton–Bailey Creep Law of SS-316 Material" Materials 14, no. 19: 5518. https://doi.org/10.3390/ma14195518
APA StyleSattar, M., Othman, A. R., Akhtar, M., Kamaruddin, S., Khan, R., Masood, F., Alam, M. A., Azeem, M., & Mohsin, S. (2021). Curve Fitting for Damage Evolution through Regression Analysis for the Kachanov–Rabotnov Model to the Norton–Bailey Creep Law of SS-316 Material. Materials, 14(19), 5518. https://doi.org/10.3390/ma14195518







