Load-Deflection Behavior of Over- and Under-Reinforced Concrete Beams with Hybrid FRP-Steel Reinforcements
Abstract
:1. Introduction
- Accurate deflection and cracking moment calculations strongly depend on the accurate determination of the material properties of concrete and reinforcement. In none of the studies in the literature were all needed mechanical properties of concrete and reinforcement encountered. The authors determined the compressive strength of concrete from cylinder tests and the modulus of rupture from the prism tests. Furthermore, the yielding stress of steel, the rupture strength, and elastic modulus of FRP were determined from axial tension tests. The FRP bar samples were tested according to a special procedure to reach reliable tensile strength and elastic modulus values. With the help of all these material tests, the authors could reach more reliable conclusions on the proposed and available analytical methods. Due to the lack of all these measured material properties, the authors could not rely on test results of the existing over-reinforced beams in the literature.
- Unlike the previous studies, the authors are of the opinion that RC beams with hybrid FRP-steel reinforcement can be designed under-reinforced in terms of FRP reinforcement. The required ductility of the beam is achieved with the help of the yielding of steel reinforcement, even if the FRP bar ruptures before the concrete crushing. The previous researchers preferred concrete crushing to precede FRP rupture, as concrete crushing is more ductile than FRP rupture. However, they ignored the fact that the required ductile behavior of a hybrid FRP-steel RC beam is already reached with the yielding of steel before the concrete crushing or FRP rupture. Hence, the authors also tested under-reinforced beams in this study.
2. Existing Effective Moment of Inertia Expressions
3. Experimental Study
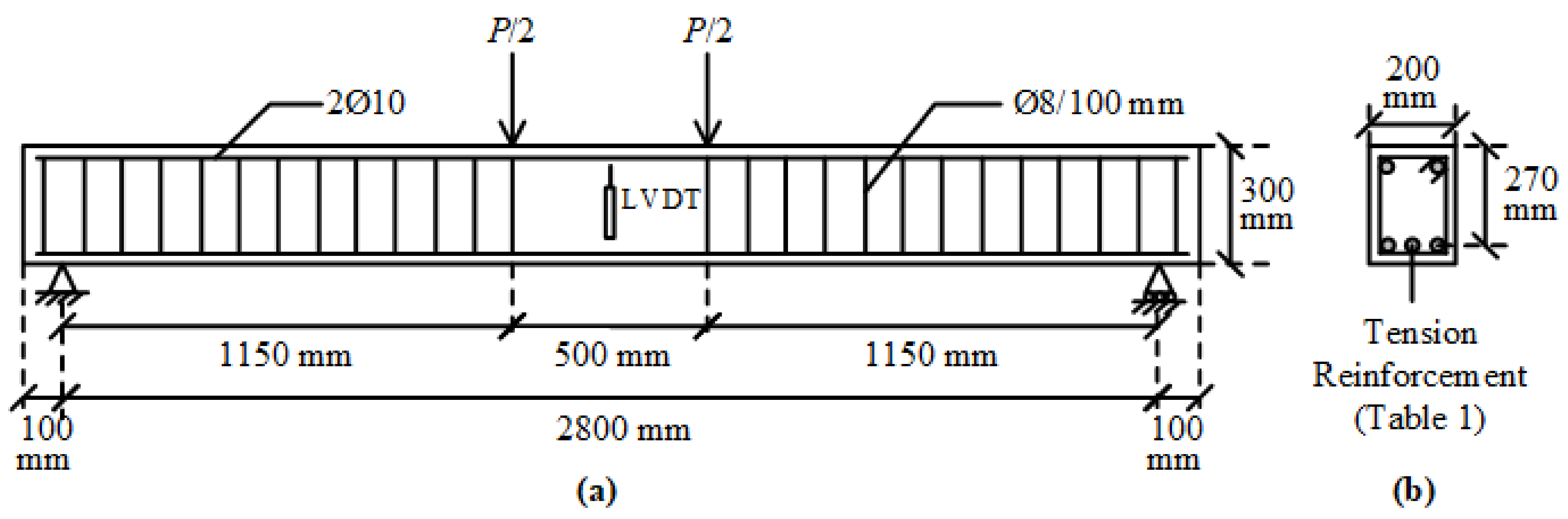
3.1. Test Specimens and Material Properties
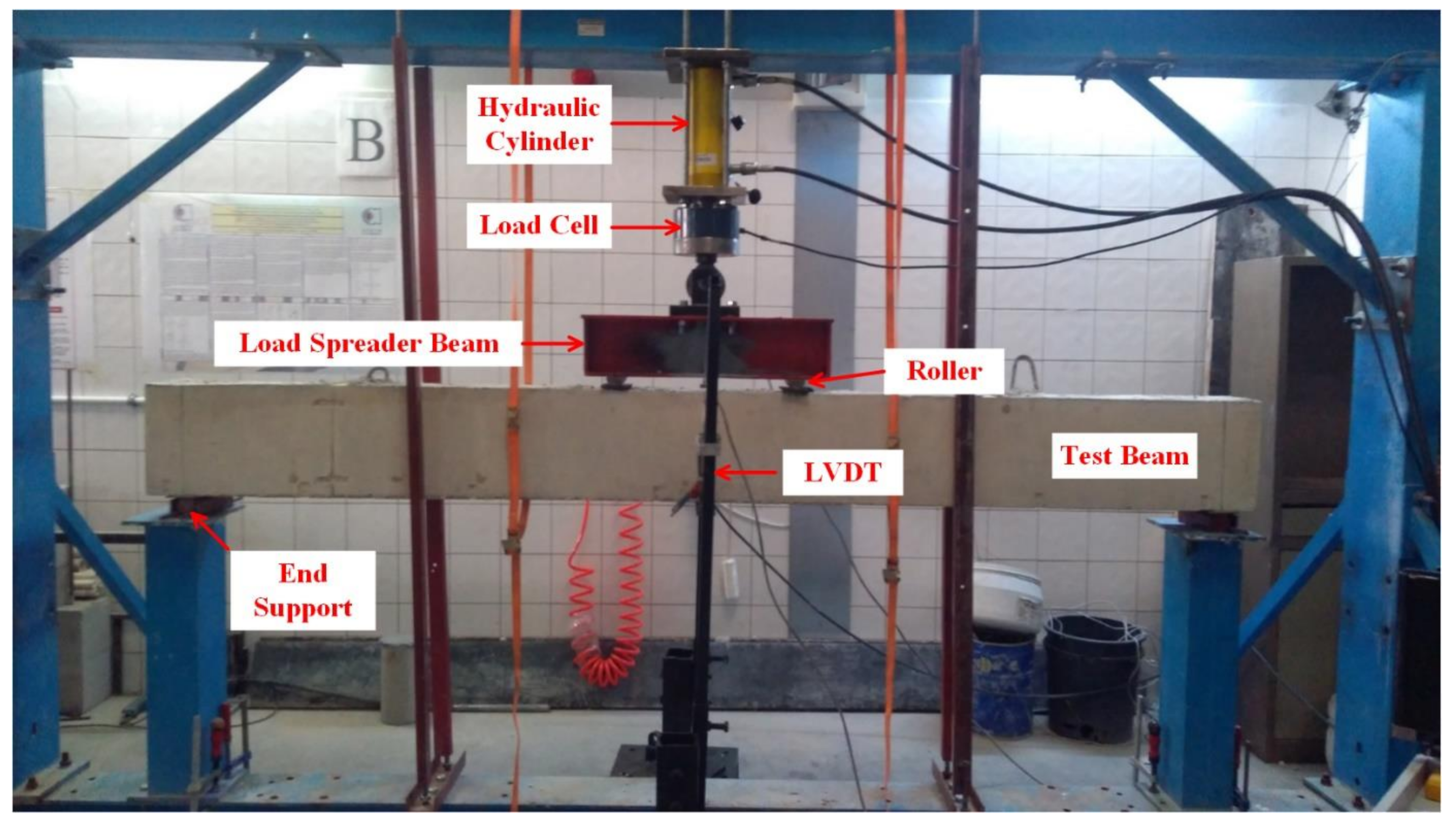
3.2. Experimental Setup
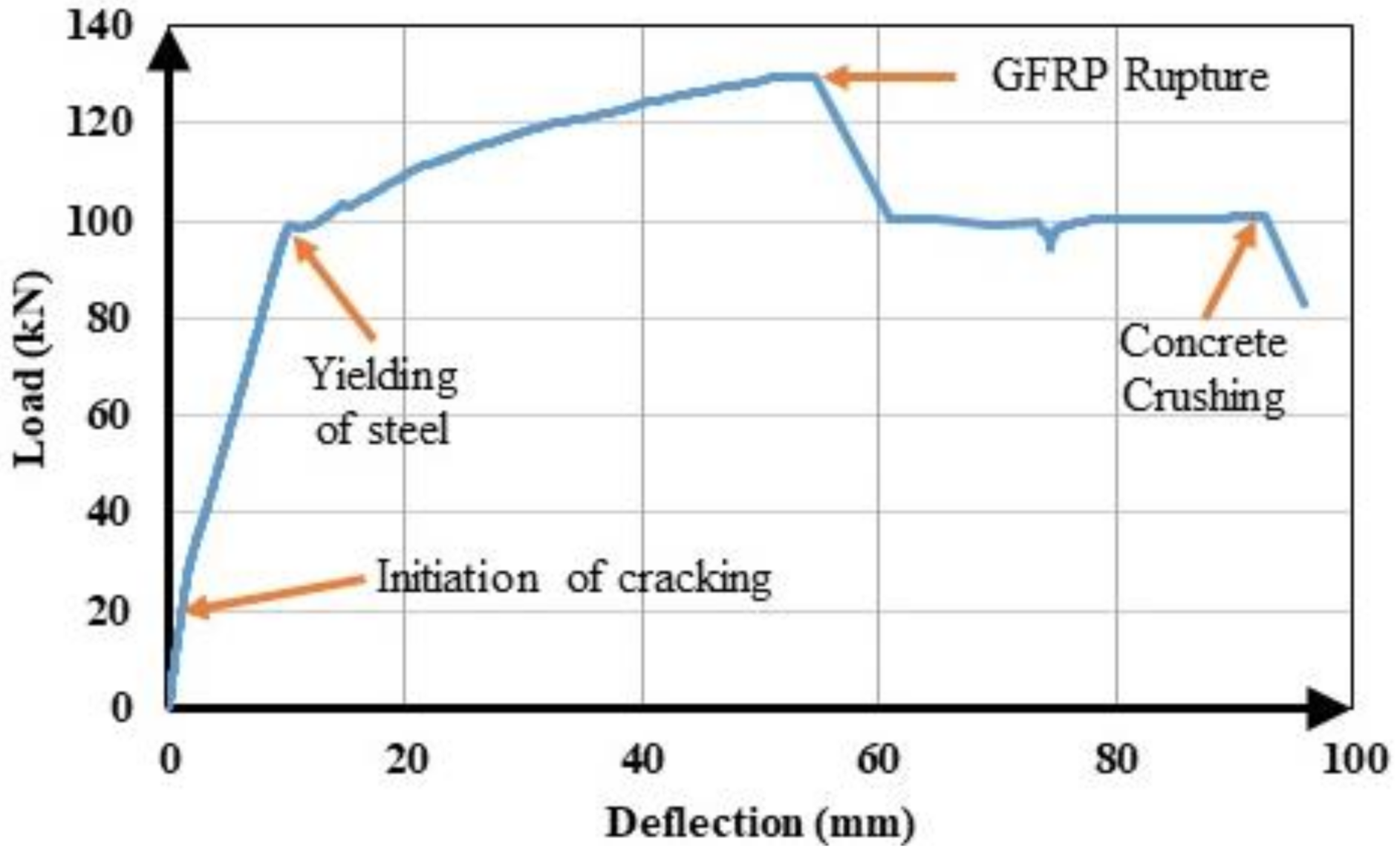
4. Experimental Results and Discussions
5. First-Cracking Moment Calculations
5.1. Moment of Inertia
5.2. Modulus of Rupture
5.3. Comparison of the Analytical and Experimental Cracking Moment Values
6. Deflection Calculations
6.1. Moment of Inertia
6.2. Modulus of Elasticity of Concrete
6.2.1. ACI 318M-19 Modulus of Elasticity Expression
6.2.2. Secant Modulus of Elasticity
6.3. Analytical Calculations
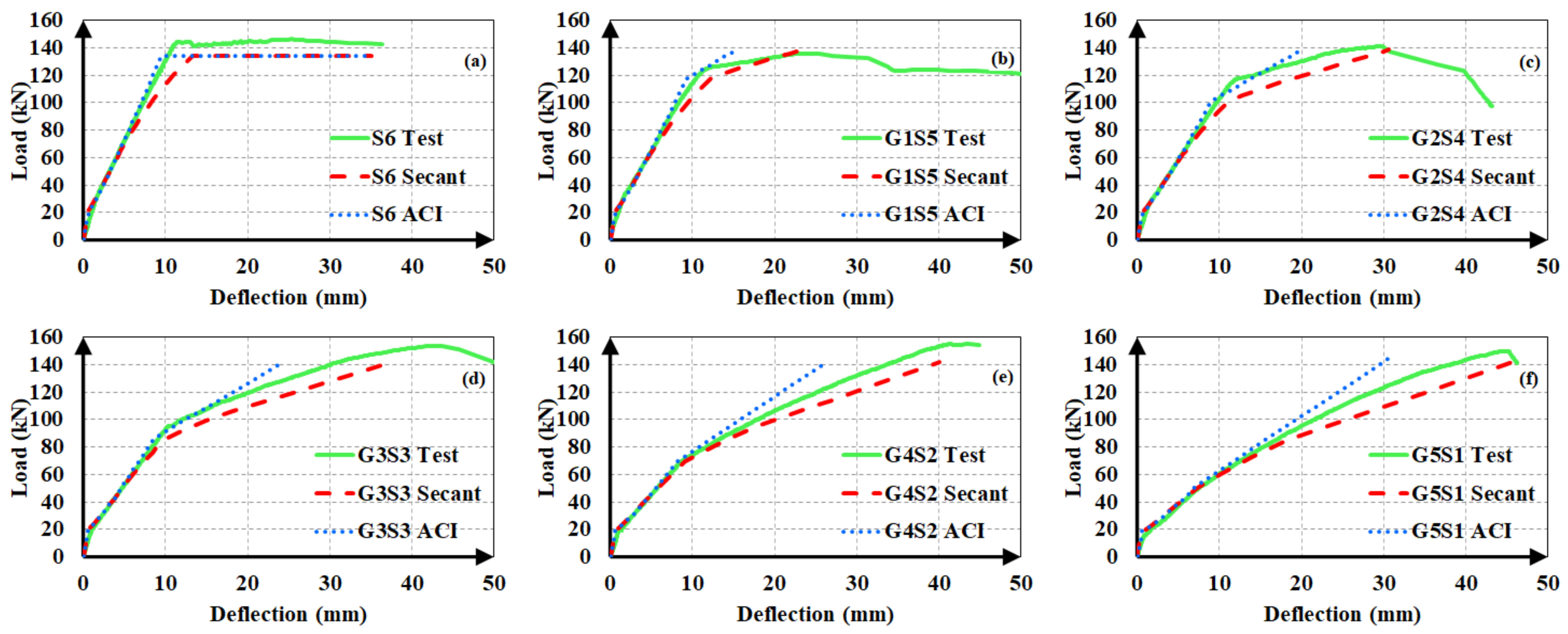
6.4. Comparison of the Analytical and Experimental Load-Deflection Curves
6.4.1. Hybrid FRP-Steel and Steel RC Beams
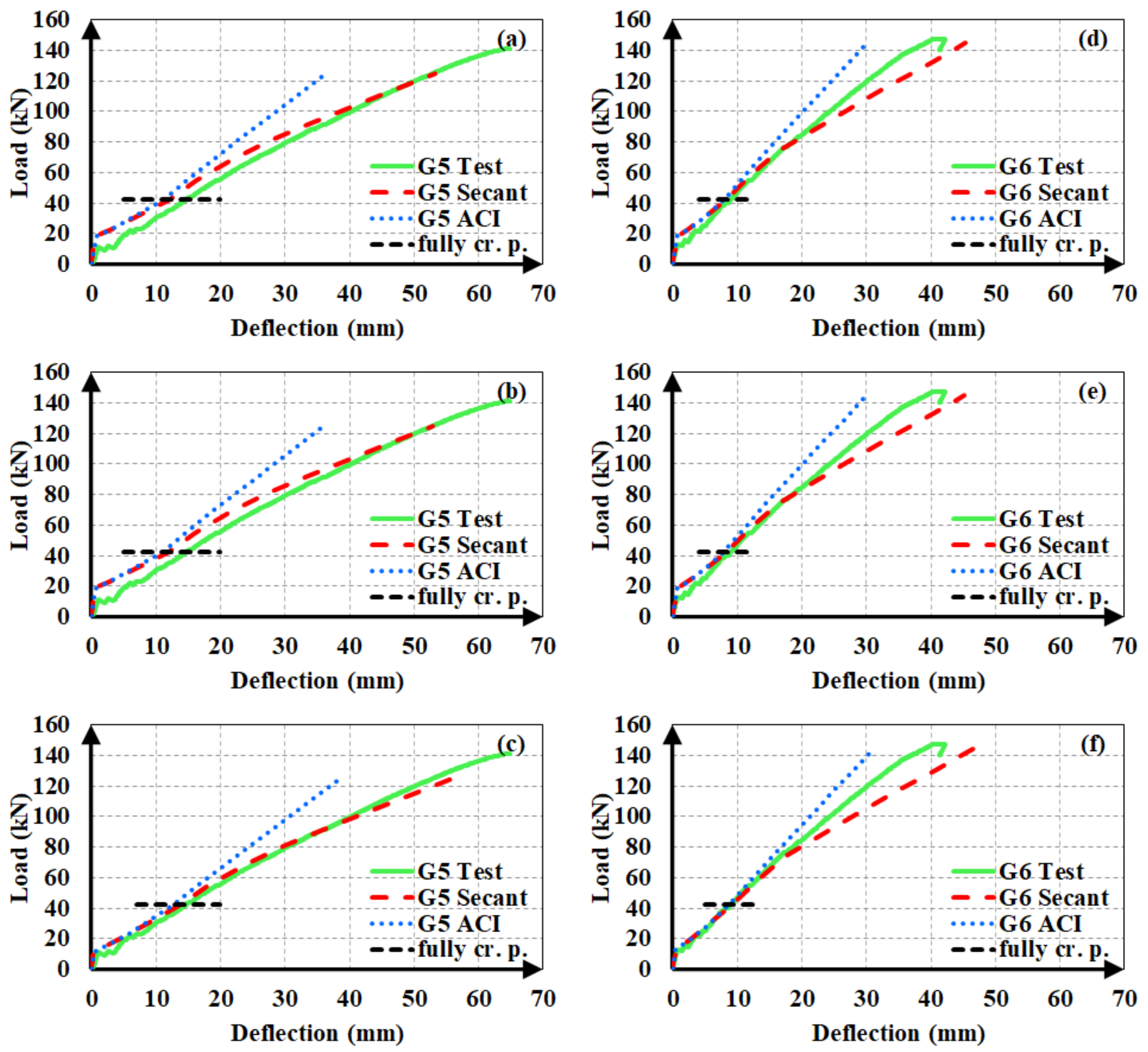
6.4.2. FRP RC Beams
7. Conclusions
- The load-deflection curve of a hybrid FRP-steel RC beam can be approximated into three linear segments, separated by the initiation of flexural cracking and initiation of yielding. The slope of the curve undergoes sudden reductions as soon as the flexural cracking initiates at the first-cracking moment and the tension steel starts yielding at the yielding moment. As the steel proportion within the tension reinforcement increases, tension steel yields under greater loads and the beam undergoes smaller deflections at the same load levels. The deformation capacity of a hybrid RC beam increases with increasing FRP content in the tension zone.
- The sole presence of FRP in the tension zone results in fluctuations along the load-deflection curve. These fluctuations correspond to minor losses of load capacity in the FRP reinforcing bars, caused by the slip of fibers inside the matrix. The fluctuations are not encountered in hybrid FRP-steel RC beams. In other words, the fiber slip does not affect the beam behavior when steel bars and FPR bars are used in the tension zone.
- Among different beam groups, the analytical cracking moment values were in closest agreement with the experimental values in over-reinforced hybrid RC beams. The least agreement between the experimental and analytical cracking moment values, however, corresponds to the beams with only FRP reinforcement. The variations in the mechanical properties of FRP bars, due to the variations in the material composition and the undesired but unavoidable misalignment of fibers inside the matrix, complicate the estimation of the cracking moments of FRP RC beams. These uncertainties decrease the accuracy of the estimates.
- The use of the uncracked transformed moment of inertia increases the accuracy of the analytical cracking moment estimates by about 20% in steel RC beams as compared to the use of the gross moment of inertia. Accordingly, the contribution of longitudinal steel to the response of the beam in the uncracked stage should not be ignored when the beam is reinforced solely with steel bars. In beams with only FRP reinforcement, however, ignoring or considering the contribution of longitudinal reinforcement, i.e., using the uncracked transformed or gross moment of inertia, has little influence on the accuracy of the analytical estimates. This insignificant influence is related to the low modulus of elasticity of FRP, as compared to steel.
- In both under-reinforced and over-reinforced hybrid RC beams, the cracking moments can be quite closely estimated when the Eurocode 2 [31] modulus of rupture expression is used, alongside when the gross moment of inertia or the ACI 318M-19 [32] modulus of rupture expression is used in combination with the uncracked transformed moment of inertia.
- The cracking moments of FRP RC beams are significantly overestimated by the analytical formulations, while the cracking moments of steel RC beams are markedly underestimated by these formulations even when the experimental modulus of rupture is used in calculations. The accuracy of the analytical estimates in FRP RC beams can be increased by using the ACI 318M-19 [32] modulus of rupture expression together with the gross moment of inertia.
- The secant modulus of elasticity corresponding to the extreme compression fiber strain provides closer and safer deflection estimates in hybrid FRP-steel RC beams, particularly after the initiation of yielding in the tension steel. The deflection estimates based on the ACI 318M-19 [32] modulus of elasticity expression are generally significantly lower than the experimental values. In other words, the decrease in the material stiffness in the course of loading cannot be reflected in the calculations when the ACI 318M-19 [32] modulus definition is used.
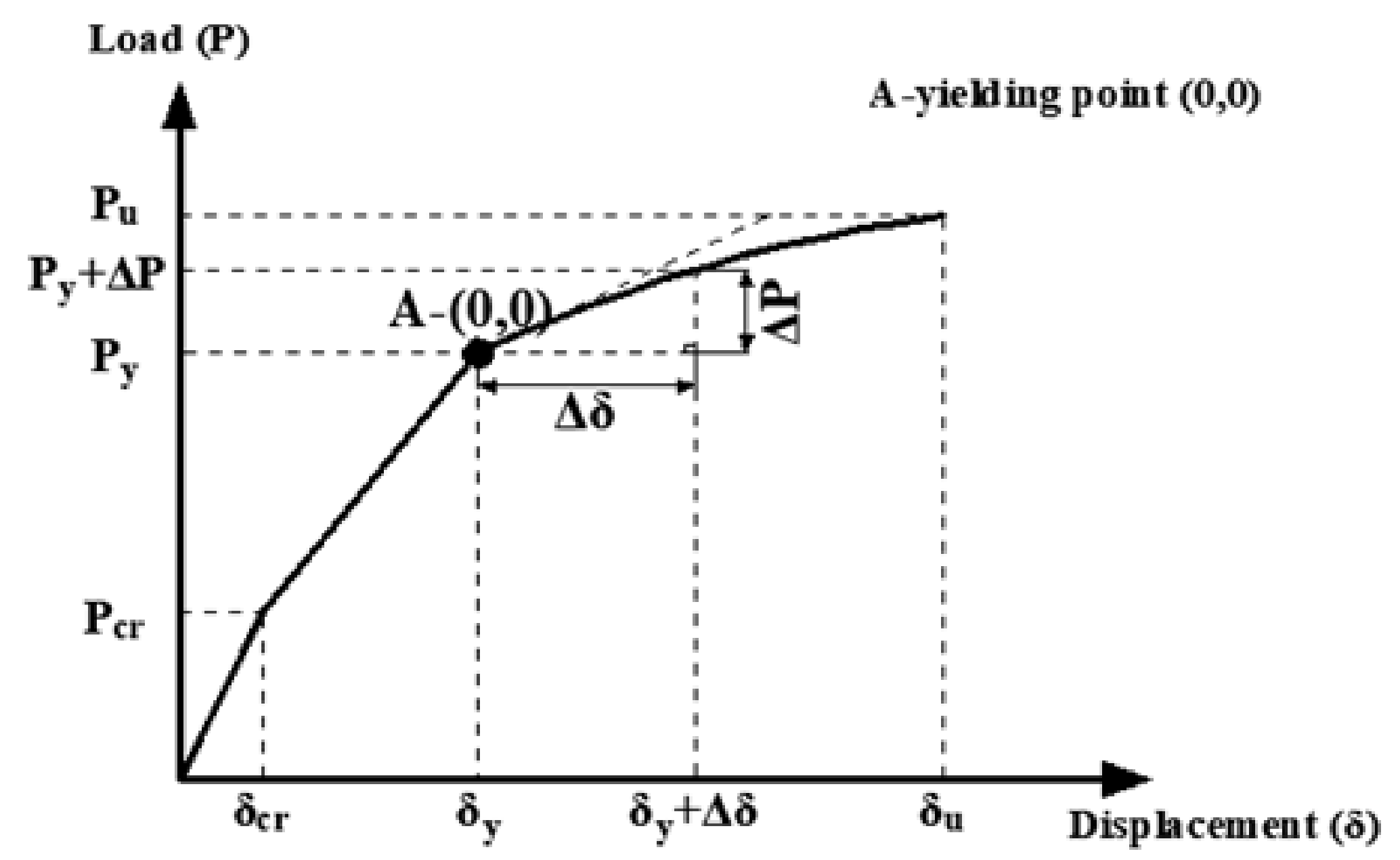
- The deflections in hybrid RC and FRP RC beams along the entire load-deflection curve should not be calculated directly from the elastic curve equation. Instead, these beams should be assumed to undergo a new loading phase (reloading) at the initiation of yielding or once the beam reaches the fully cracked stage. In other words, the yielding or full-cracking points should be assumed to be the origin for a loading phase of hybrid and FRP RC beams, respectively. In this new loading phase, deflection increments are calculated from load increments and the total deflections are obtained from the summation of both these deflection increments and the deflection at the start of the new loading phase. In this way, the load-deflection curves of the hybrid FRP-steel and FRP RC beams were shown to be estimated more closely.
- The success of the analytical deflection calculations depends on the accurate estimation of the cracking moment of a beam. The accuracy of the estimates can be greatly increased by using the experimental cracking moment of a beam. The present tests indicated that the cracking moment from the uncracked transformed section yields closer deflection estimates in comparison with the estimates from the gross cross-section.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations and Notations
| FRP | fiber-reinforced polymer |
| AFRP | aramid fiber-reinforced polymer |
| BFRP | basalt fiber-reinforced polymer |
| CFRP | carbon fiber-reinforced polymer |
| GFRP | glass fiber-reinforced polymer |
| Asc | cross-sectional area of the compression reinforcement |
| Afrp | cross-sectional area of the FRP tension reinforcement |
| Ast | cross-sectional area of the steel tension reinforcement |
| ccr | neutral axis depth of the section at fully cracked state |
| cy | neutral axis depth of the section at the initiation of yielding of steel tension reinforcement |
| Cc | internal force in the rectangular concrete compression block |
| Cs | internal force in the compression steel |
| d | effective depth |
| d′ | depth of the compression reinforcement from the compression face |
| E | modulus of elasticity |
| Ec | modulus of elasticity of concrete |
| Ef | modulus of elasticity of FRP |
| Es | modulus of elasticity of steel |
| Esec | secant modulus of elasticity of concrete |
| Esh | slope of the strain-hardening portion of the stress-strain curve of steel |
| fc′ | specified compressive strength of concrete |
| fc″ | maximum compressive strength of concrete in the Todeschini et al. [34] stress-strain model |
| fc(εc) | stress in concrete corresponding to the extreme compression fiber strain |
| fck | concrete characteristic compressive strength |
| fctm | mean direct tensile strength of concrete |
| fctm,fl | mean flexural tensile strength of concrete |
| ffrp(εst) | stress in tension FRP corresponding to the extreme compression fiber strain |
| ffu | rupture stress of FRP |
| fr | modulus of rupture of concrete |
| fs(εst) | stress in tension steel corresponding to the extreme compression fiber strain |
| fsc(εsc) | stress in compression steel corresponding to the extreme compression fiber strain |
| h | total beam height in mm |
| I | second moment of area (moment of inertia) |
| Icr | cracked moment of inertia (tension steel not yielded) |
| Icr2 | second cracked moment of inertia (tension steel yielded) |
| Ie | effective moment of inertia |
| Ig | gross moment of inertia |
| Im | modified moment of inertia |
| Iucr | uncracked transformed moment of inertia |
| Iy | yielding moment of inertia (right before yielding of tension steel) |
| Iy2 | second yielding moment of inertia (immediately after yielding of tension steel) |
| k2(εc) | coefficient in the Todeschini et al. [34] stress-strain model for the depth of the centroid of concrete compression blocks from compression faces |
| L | length of the beam span |
| Lss | shear span length |
| m | power term in the effective moment of inertia expression |
| Ma | applied moment |
| Mcr | first-cracking moment |
| Mcc | moment contribution of concrete block |
| Mfcr | full-cracking moment |
| Msc | moment contribution of compression reinforcement |
| Mt | moment contribution of tension reinforcement |
| n | modular ratio of reinforcement to concrete |
| nf | modular ratio of FRP to concrete |
| P | applied load |
| Pcr | cracking load |
| Pcr1 | analytical cracking load estimate for the experimental modulus of rupture and uncracked transformed moment of inertia |
| Pcr2 | analytical cracking load estimate for the experimental modulus of rupture and gross moment of inertia |
| Pcr1A | analytical cracking load estimate for the ACI318m-11 [32] modulus of rupture and uncracked transformed moment of inertia |
| Pcr2A | analytical cracking load estimate for the ACI318m-11 [32] modulus of rupture and gross transformed moment of inertia |
| Pcr1E | analytical cracking load estimate for the Eurocode 2 [31] modulus of rupture and uncracked transformed moment of inertia |
| Pcr2E | analytical cracking load estimate for the Eurocode 2 [31] modulus of rupture and gross transformed moment of inertia |
| Pcrt | experimental cracking load |
| Pfcr | full-cracking load |
| Pult | ultimate load |
| Py | yielding load |
| Tf | internal force in the tension FRP |
| Ts | internal force in the tension steel |
| α | reduction factor in the effective moment of inertia expression |
| αb | reduction factor in the effective moment of inertia depending on the reinforcement ratio |
| β | reduction factor in the effective moment of inertia expression |
| βd | reduction factor in the effective moment of inertia reflecting the modulus of elasticity and bonding properties of FRP on beam deflections |
| β1(εc) | coefficient in the Todeschini et al. [34] stress-strain model for the width of the equivalent concrete compression block |
| δ | deflection |
| Δδ | incremental deflection |
| ΔP | incremental load |
| εc | extreme compression fiber strain |
| εcu | crushing strain of concrete |
| εo | concrete strain at ultimate stress |
| εfu | rupture strain of FRP |
| εsc | strain of the compression reinforcement |
| εsh | strain of steel at the initiation of strain-hardening |
| εst | strain of the tension reinforcement |
| εy | yielding strain of steel |
| εu | ultimate (rupture) strain of steel |
| ρ | total tension reinforcement ratio |
| ρf | ratio of FRP tension reinforcement |
| ρfb | balanced FRP tension reinforcement ratio |
References
- Rabinovitch, O.; Frostig, Y. Experiments and analytical comparison of RC beams strengthened with CFRP composites. Compos. Part B 2003, 34, 133–140. [Google Scholar] [CrossRef]
- Odess, I.; Rabinovitch, O.; Frostig, Y. High-order crack propagation in compressed sandwich panels. J. Sandw. Struct. Mater. 2019, 21, 42. [Google Scholar] [CrossRef]
- Funari, M.F.; Spadea, S.; Fabbrocino, F.; Luciano, R. A Moving interface finite element formulation to predict dynamic edge debonding in FRP-strengthened concrete beams in service conditions. Fibers 2020, 8, 42. [Google Scholar] [CrossRef]
- ACI Committee 440. ACI 440.1R-15: Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars; American Concrete Institute: Farmington Hills, MI, USA, 2015. [Google Scholar]
- Aiello, M.A.; Ombres, L. Structural performances of concrete beams with hybrid (Fiber-reinforced polymer-steel) reinforcements. J. Compos. Constr. 2002, 6, 133–140. [Google Scholar] [CrossRef]
- Leung, H.Y.; Balendran, R.V. Flexural behaviour of concrete beams internally reinforced with GFRP rods and steel rebars. Struct. Surv. 2003, 21, 146–157. [Google Scholar] [CrossRef]
- Qu, W.; Zhang, X.; Huang, H. Flexural behavior of concrete beams reinforced with hybrid (GFRP and steel) bars. J. Compos. Constr. 2009, 13, 350–359. [Google Scholar] [CrossRef]
- Lau, D.; Pam, H.J. Experimental study of hybrid FRP reinforced concrete beams. Eng. Struct. 2010, 32, 3857–3865. [Google Scholar] [CrossRef]
- Yinghao, L.; Yong, Y. Arrangement of hybrid rebars on flexural behavior of HSC beams. Compos. Part B 2013, 45, 22–31. [Google Scholar] [CrossRef]
- Ge, W.; Zhang, J.; Cao, D.; Tu, Y. Flexural behaviors of hybrid concrete beams reinforced with BFRP bars and steel bars. Constr. Build. Mater. 2015, 87, 28–37. [Google Scholar] [CrossRef]
- Kara, L.F.; Ashour, A.F.; Köroğlu, M.A. Flexural behavior of hybrid FRP/steel reinforced concrete beams. Compos. Struct. 2015, 129, 111–121. [Google Scholar] [CrossRef]
- El Refai, A.; Abed, F.; Al-Rahmani, A. Structural performance and serviceability of concrete beams reinforced with hybrid (GFRP and steel) bars. Constr. Build. Mater. 2015, 96, 518–529. [Google Scholar] [CrossRef]
- Bencardino, F.; Condello, A.; Ombres, L. Numerical and analytical modeling of concrete beams with steel, FRP and hybrid FRP-steel reinforcements. Compos. Struct. 2016, 140, 53–65. [Google Scholar] [CrossRef]
- Safan, M.A. Flexural behavior and design of steel-GFRP reinforced concrete beams. ACI Mater. J. 2013, 110, 677–685. [Google Scholar]
- ISIS Canada Research Network. Reinforcing Concrete Structures with FiberReinforced Polymers (Design Manual No. 3, Version 2); The Canadian Network of Centers of Excellence on Intelligent Sensing for Innovative Structures: Winnipeg, MB, Canada, 2006. [Google Scholar]
- Branson, B. Instantaneous and Time-Dependent Deflections of Simple and Continuous Reinforced Concrete Beams; State of Alabama, Highway Department, Bureau of Research and Development: Auburn, AL, USA, 1965. [Google Scholar]
- Bischoff, P.H. Reevaluation of deflection prediction for concrete beams reinforced with steel and fiber reinforced polymer bars. ASCE J. Struct. Eng. 2005, 131, 752–767. [Google Scholar] [CrossRef]
- ACI Institute Committee 440. ACI 440.1R-96: Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars; American Concrete Institute: Farmington Hills, MI, USA, 1996. [Google Scholar]
- Faza, S.S.; GangaRao, H.V.S. Pre-and post-cracking deflection behaviour of concrete beams reinforced with fibre-reinforced plastic rebars. In Proceedings of the 1st International Conference on Advanced Composite Materials in Bridges and Structures (ACMBS 1), Sherbrooke, QB, Canada, 7–9 October 1992. [Google Scholar]
- Toutanji, H.A.; Saafi, M. Flexural behavior of concrete beams reinforced with glass fiber-reinforced polymer (GFRP) bars. ACI Struct. J. 2000, 97, 712–719. [Google Scholar]
- Alsayed, S.H.; Al-Salloum, Y.A.; Almusallam, T.H. Performance of glass fiber reinforced plastic bars as a reinforcing material for concrete structures. Compos. Part B 2000, 31, 555–567. [Google Scholar] [CrossRef]
- Benmokrane, B.; Chaallal, O.; Masmoudi, R. Flexural response of concrete beams reinforced with FRP reinforcing bars. ACI Struct. J. 1996, 93, 46–55. [Google Scholar]
- Gao, D.; Benmokrane, B.; Masmoudi, R.A. Calculating Method of Flexural Properties of FRP-Reinforced Concrete Beam: Part 1: Crack Width and Deflection; Department of Civil Engineering, University of Sherbrooke: Sherbrooke, QC, Canada, 1998. [Google Scholar]
- ACI Committee 440. ACI 440.1R-01: Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars; American Concrete Institute: Farmington Hills, MI, USA, 2001. [Google Scholar]
- ACI Committee 440. ACI 440.1R-03: Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars; American Concrete Institute: Farmington Hills, MI, USA, 2003. [Google Scholar]
- Yost, J.R.; Gross, S.P.; Dinehart, D.W. Effective moment of inertia for glass fiber-reinforced polymer-reinforced concrete beams. ACI Struct. J. 2003, 100, 732–739. [Google Scholar]
- ACI Committee 440. ACI 440.1R-06: Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars; American Concrete Institute: Farmington Hills, MI, USA, 2006. [Google Scholar]
- Kalkan, İ. Deflection prediction for reinforced concrete beams through different effective moment of inertia expressions. Int. J. Eng. Res. Dev. 2013, 5, 1. [Google Scholar]
- ASTM International. ASTM D7205/D7205M-06: Standard Test Method for Tensile Properties of Fiber Reinforced Polymer Matrix Composite Bars; American Society for Testing and Materials: West Conshohocken, PA, USA, 2016. [Google Scholar]
- ASTM International. ASTM C78/C78M-18: Standard Test method for Flexural Strength of Concrete (Using Simple Beam with Third-Point Loading); American Society for Testing and Materials: West Conshohocken, PA, USA, 2018. [Google Scholar]
- European Committee for Standardization. EN 1992-1-1: Design of Concrete Structures (Eurocode 2)—Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization (CEN): Brussels, Belgium, 2004. [Google Scholar]
- ACI Committee 318. ACI 318M-19: Building Code Requirements for Structural Concrete; American Concrete Institute: Farmington Hills, MI, USA, 2019. [Google Scholar]
- Bischoff, P.H. Comparison of existing approaches for computing deflection of reinforced concrete. ACI Struct. J. 2020, 117, 231–240. [Google Scholar] [CrossRef]
- Todeschini, C.E.; Bianchini, A.C.; Kesler, C.E. Behavior of concrete columns reinforced with high strength steels. ACI J. Proc. 1964, 61, 701–716. [Google Scholar]
- Yoon, Y.S.; Yang, J.M.; Min, K.H.; Shin, H.O. Flexural strength and deflection characteristics of high strength concrete beams with hybrid FRP and steel bar reinforcement. In Proceedings of the 10th International Symposium on Fiber-Reinforced Polymer Reinforcement for Concrete Structures (FRPRCS-10), Tampa, FL, USA, 2–4 April 2011; Sen, R., Seracino, R., Shield, C., Gold, W., Eds.; ACI Special Publication 275. American Concrete Institute: Farminton Hills, MI, USA, 2011. [Google Scholar]











| Group | Specimen | Sectional Dimensions, mm | Tension Reinforcement | Concrete Strength, MPa | FRP Modulus of Elasticity, GPa Mean (Standard Deviation) | FRP Tensile Strength, MPa Mean (Standard Deviation) |
|---|---|---|---|---|---|---|
| First | S5 | 200 × 300 | 5Ø12 steel | 31.28 | - | - |
| B1S4 | 200 × 300 | 1Ø8.68 BFRP + 4Ø12 steel | 31.28 | 43 (1.0) | 1034 (33) | |
| B2S3 | 200 × 300 | 2Ø8.68 BFRP + 3Ø12 steel | 31.28 | 43 (1.0) | 1034 (33) | |
| B3S2 | 200 × 300 | 3Ø8.68 BFRP + 2Ø12 steel | 31.28 | 43 (1.0) | 1034 (33) | |
| B4S1 | 200 × 300 | 4Ø8.68 BFRP + 1Ø12 steel | 31.28 | 43 (1.0) | 1034 (33) | |
| B5 | 200 × 300 | 5Ø8.68 BFRP | 31.28 | 43 (1.0) | 1034 (33) | |
| G1S4 | 200 × 300 | 1Ø12.86 GFRP + 4Ø12 steel | 31.28 | 35 (3.1) | 449 (61) | |
| G2S3 | 200 × 300 | 2Ø12.86 GFRP + 3Ø12 steel | 31.28 | 35 (3.1) | 449 (61) | |
| G3S2 | 200 × 300 | 3Ø12.86 GFRP + 2Ø12 steel | 31.28 | 35 (3.1) | 449 (61) | |
| G4S1 | 200 × 300 | 4Ø12.86 GFRP + 1Ø12 steel | 31.28 | 35 (3.1) | 449 (61) | |
| G5 | 200 × 300 | 5Ø12.86 GFRP | 31.28 | 35 (3.1) | 449 (61) | |
| Second | S6 | 199.8 × 303.29 | 6Ø12 steel | 30.49 | - | - |
| G1S5 | 200.8 × 301.86 | 1Ø12.23 GFRP + 5Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G2S4 | 199.8 × 301.14 | 2Ø12.23 GFRP + 4Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G3S3 | 200.6 × 304.43 | 3Ø12.23 GFRP +3Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G4S2 | 198.6 × 304.57 | 4Ø12.23 GFRP + 2Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G5S1 | 200.6 × 306.00 | 5Ø12.23 GFRP + 1Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G6 | 200.0 × 307.00 | 6Ø12.23 GFRP | 30.49 | 46 (2.7) | 580 (55) | |
| Third | S3 | 200.8 × 304.71 | 3Ø12 steel | 30.49 | - | - |
| B1S2 | 199.8 × 308.00 | 1Ø8.68 BFRP + 2Ø12 steel | 30.49 | 43 | 1034 (33) | |
| B2S1 | 199.2 × 301.71 | 2Ø8.68 BFRP + 1Ø12 steel | 30.49 | 43 | 1034 (33) | |
| B5 | 199.0 × 302.00 | 5Ø5.3 BFRP | 30.49 | - | - | |
| G1S2 | 198.6 × 304.86 | 1Ø12.23 GFRP + 2Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G2S1 | 202.0 × 301.57 | 2Ø12.23 GFRP + 1Ø12 steel | 30.49 | 46 (2.7) | 580 (55) | |
| G3 | 198.8 × 308.71 | 3Ø12.23 GFRP | 30.49 | 46 (2.7) | 580 (55) |
| Group | Beam | Pcrta (kN) | Pcr1b (kN) | Pcr2c (kN) | Pcrt/Pcr1 | Pcrt/Pcr2 | Pcr1Ed (kN) | Pcr2Ee (kN) | Pcrt/Pcr1E | Pcrt/Pcr2E | Pcr1Af (kN) | Pcr2Ag (kN) | Pcre/Pcr1A | Pcre/Pcr2A |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Only Steel | S5 | 25.70 | 22.29 | 18.52 | 1.15 | 1.39 | 24.30 | 20.19 | 1.06 | 1.27 | 21.85 | 18.16 | 1.18 | 1.42 |
| S6 | 27.74 | 21.05 | 17.30 | 1.32 | 1.60 | 24.68 | 20.28 | 1.12 | 1.37 | 22.28 | 18.31 | 1.24 | 1.52 | |
| S3 | 23.13 | 19.45 | 17.55 | 1.19 | 1.32 | 22.8 | 20.57 | 1.01 | 1.12 | 20.59 | 18.57 | 1.12 | 1.25 | |
| Mean (COV%) | 1.22 (7.29) | 1.44 (10.14) | Mean (COV %) | 1.06 (5.19) | 1.25 (10.05) | Mean (COV %) | 1.18 (5.09) | 1.40 (9.76) | ||||||
| Only FRP | B5 | 14.53 | 19.08 | 18.52 | 0.76 | 0.78 | 20.80 | 20.19 | 0.70 | 0.72 | 18.71 | 18.16 | 0.78 | 0.80 |
| G5 | 11.08 | 19.11 | 18.52 | 0.58 | 0.60 | 20.83 | 20.19 | 0.53 | 0.55 | 18.73 | 18.16 | 0.59 | 0.61 | |
| G6 | 12.45 | 18.20 | 17.74 | 0.68 | 0.70 | 21.34 | 20.80 | 0.58 | 0.60 | 19.27 | 18.78 | 0.65 | 0.66 | |
| G3 | 15.93 | 18.06 | 17.83 | 0.88 | 0.89 | 21.18 | 20.90 | 0.75 | 0.76 | 19.12 | 18.87 | 0.83 | 0.84 | |
| Mean (COV %) | 0.73 (17.50) | 0.74 (16.55) | Mean (COV %) | 0.64 (15.99) | 0.66 (15.02) | Mean (COV %) | 0.71 (15.65) | 0.73 (15.12) | ||||||
| Group | Beam | Pcrta (kN) | Pcr1b (kN) | Pcr2c (kN) | Pcrt/Pcr1 | Pcrt/Pcr2 | Pcr1Ed (kN) | Pcr2Ee (kN) | Pcrt/Pcr1E | Pcrt/Pcr2E | Pcr1Af (kN) | Pcr2Ag (kN) | Pcre/Pcr1A | Pcre/Pcr2A |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Hybrid Over-reinforced | B2S3 | 23.00 | 21.02 | 18.52 | 1.09 | 1.24 | 22.91 | 20.19 | 1.00 | 1.14 | 20.60 | 18.16 | 1.12 | 1.27 |
| B3S2 | 20.09 | 20.37 | 18.52 | 0.99 | 1.08 | 22.21 | 20.19 | 0.90 | 1.00 | 19.97 | 18.16 | 1.01 | 1.11 | |
| B4S1 | 18.02 | 19.73 | 18.52 | 0.91 | 0.97 | 21.51 | 20.19 | 0.84 | 0.89 | 19.34 | 18.16 | 0.93 | 0.99 | |
| G2S3 | 20.00 | 21.03 | 18.52 | 0.95 | 1.08 | 22.92 | 20.19 | 0.87 | 0.99 | 20.61 | 18.16 | 0.97 | 1.10 | |
| G3S2 | 17.62 | 20.39 | 18.52 | 0.86 | 0.95 | 22.23 | 20.19 | 0.79 | 0.87 | 19.99 | 18.16 | 0.88 | 0.97 | |
| G4S1 | 18.32 | 19.75 | 18.52 | 0.93 | 0.99 | 21.53 | 20.19 | 0.85 | 0.91 | 19.36 | 18.16 | 0.95 | 1.01 | |
| G1S5 | 23.09 | 20.41 | 17.22 | 1.13 | 1.34 | 23.93 | 20.19 | 0.97 | 1.14 | 21.60 | 18.23 | 1.07 | 1.27 | |
| G2S4 | 22.01 | 19.69 | 17.05 | 1.12 | 1.29 | 23.08 | 19.99 | 0.95 | 1.10 | 20.84 | 18.05 | 1.06 | 1.22 | |
| G3S3 | 18.85 | 19.62 | 17.50 | 0.96 | 1.08 | 23.00 | 20.51 | 0.82 | 0.92 | 20.77 | 18.52 | 0.91 | 1.02 | |
| G4S2 | 19.14 | 18.91 | 17.34 | 1.01 | 1.10 | 22.17 | 20.33 | 0.86 | 0.94 | 20.02 | 18.35 | 0.96 | 1.04 | |
| G5S1 | 14.17 | 18.70 | 17.68 | 0.76 | 0.80 | 21.92 | 20.72 | 0.65 | 0.68 | 19.79 | 18.71 | 0.72 | 0.76 | |
| B1S2 | 20.55 | 19.16 | 17.84 | 1.07 | 1.15 | 22.46 | 20.91 | 0.91 | 0.98 | 20.28 | 18.88 | 1.01 | 1.09 | |
| Mean (COV %) | 0.98 (11.28) | 1.09 (14.04) | Mean (COV %) | 0.87 (10.68) | 0.96 (13.39) | Mean (COV %) | 0.97 (10.81) | 1.07 (13.35) | ||||||
| Hybrid Under-reinforced | B1S4 | 24.39 | 21.66 | 18.52 | 1.13 | 1.32 | 23.61 | 20.19 | 1.03 | 1.21 | 21.23 | 18.16 | 1.15 | 1.34 |
| G1S4 | 22.89 | 21.66 | 18.52 | 1.06 | 1.24 | 23.61 | 20.19 | 0.97 | 1.13 | 21.23 | 18.16 | 1.08 | 1.26 | |
| B2S1 | 18.84 | 17.76 | 17.07 | 1.06 | 1.10 | 20.82 | 20.01 | 0.90 | 0.94 | 18.80 | 18.06 | 1.00 | 1.04 | |
| G1S2 | 19.83 | 18.72 | 17.37 | 1.06 | 1.14 | 21.94 | 20.37 | 0.90 | 0.97 | 19.81 | 18.39 | 1.00 | 1.08 | |
| G2S1 | 17.32 | 18.07 | 17.29 | 0.96 | 1.00 | 21.18 | 20.27 | 0.82 | 0.85 | 19.12 | 18.30 | 0.91 | 0.95 | |
| Mean (COV %) | 1.05 (5.76) | 1.16 (10.70) | Mean (COV %) | 0.92 (8.61) | 1.02 (14.38) | Mean (COV %) | 1.03 (8.85) | 1.13 (14.21) | ||||||
| Parameter | Load Range | ||
|---|---|---|---|
| 0–Pcr | Pcr–Py | Py–Pult | |
| Moment of inertia | Ig or Iucr | Bischoff [17] expression from Ig to Iy or from Iucr to Iy | Bischoff [17] expression from Iy2 to Icr2 |
| Modulus of elasticity | ACI 318M-19 [32] definition or secant modulus | ACI 318M-19 [32] definition or secant modulus | ACI 318M-19 [32] definition or secant modulus |
| Deflection from the elastic curve equation | Total deflection (δ) | Total deflection (δ) | Incremental deflection (Δδ) |
| Parameter | Load Range | ||
|---|---|---|---|
| 0–Pcr | Pcr–Pfcr | Pfcr–Pult | |
| Moment of inertia | Ig or Iucr | Bischoff [17] expression from Ig to Icr or from Iucr to Icr | Bischoff [17] expression from Ig to Icr or from Iucr to Icr |
| Modulus of elasticity | ACI 318M-19 [32] definition or secant modulus | ACI 318M-19 [32] definition or secant modulus | ACI 318M-19 [32] definition or secant modulus |
| Deflection from the elastic curve equation | Total deflection (δ) | Total deflection (δ) | Incremental deflection (Δδ) |
| Parameter | Load Range | |
|---|---|---|
| 0–Pcr | Pcr–Pult | |
| Moment of inertia | Ig or Iucr | Bischoff [17] expression from Ig to Icr or from Iucr to Icr |
| Modulus of elasticity | ACI 318M-19 [32] definition or secant modulus | ACI 318M-19 [32] definition or secant modulus |
| Deflection from the elastic curve equation | Total deflection (δ) | Total deflection (δ) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kartal, S.; Kalkan, I.; Beycioglu, A.; Dobiszewska, M. Load-Deflection Behavior of Over- and Under-Reinforced Concrete Beams with Hybrid FRP-Steel Reinforcements. Materials 2021, 14, 5341. https://doi.org/10.3390/ma14185341
Kartal S, Kalkan I, Beycioglu A, Dobiszewska M. Load-Deflection Behavior of Over- and Under-Reinforced Concrete Beams with Hybrid FRP-Steel Reinforcements. Materials. 2021; 14(18):5341. https://doi.org/10.3390/ma14185341
Chicago/Turabian StyleKartal, Saruhan, Ilker Kalkan, Ahmet Beycioglu, and Magdalena Dobiszewska. 2021. "Load-Deflection Behavior of Over- and Under-Reinforced Concrete Beams with Hybrid FRP-Steel Reinforcements" Materials 14, no. 18: 5341. https://doi.org/10.3390/ma14185341
APA StyleKartal, S., Kalkan, I., Beycioglu, A., & Dobiszewska, M. (2021). Load-Deflection Behavior of Over- and Under-Reinforced Concrete Beams with Hybrid FRP-Steel Reinforcements. Materials, 14(18), 5341. https://doi.org/10.3390/ma14185341





