Investigation of Anisotropic Subsequent Yield Behavior for 45 Steel by the Distortional Yield Surface Constitutive Model
Abstract
1. Introduction
2. Theoretical Model
2.1. The Distortional Yield Function
2.2. The Anisotropic Distortional Function
2.3. The Anisotropic Distortional Factor
2.4. The Flow Directions of the Anisotropic Model
3. Calibration of Model Parameters and FEM Model
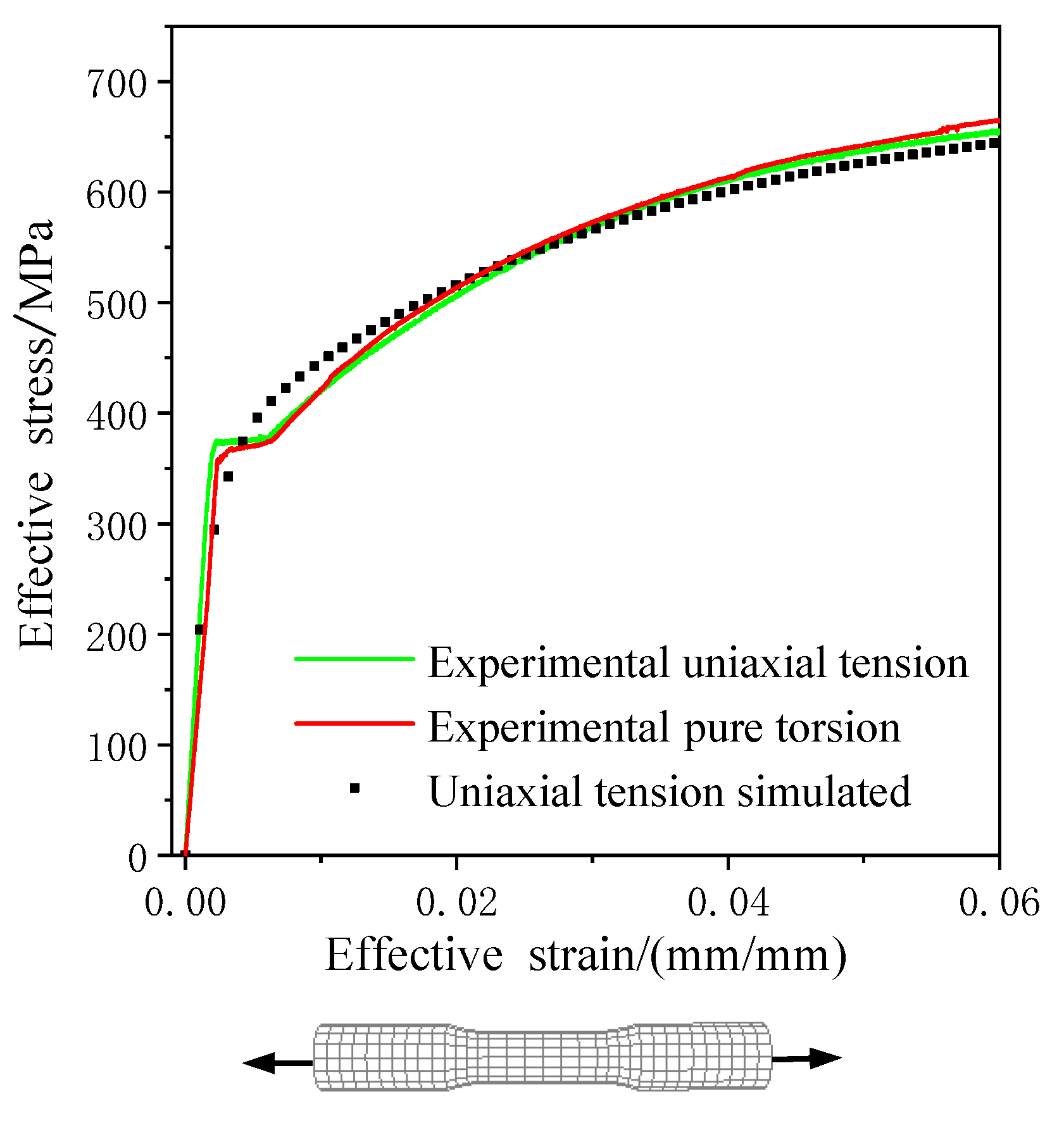
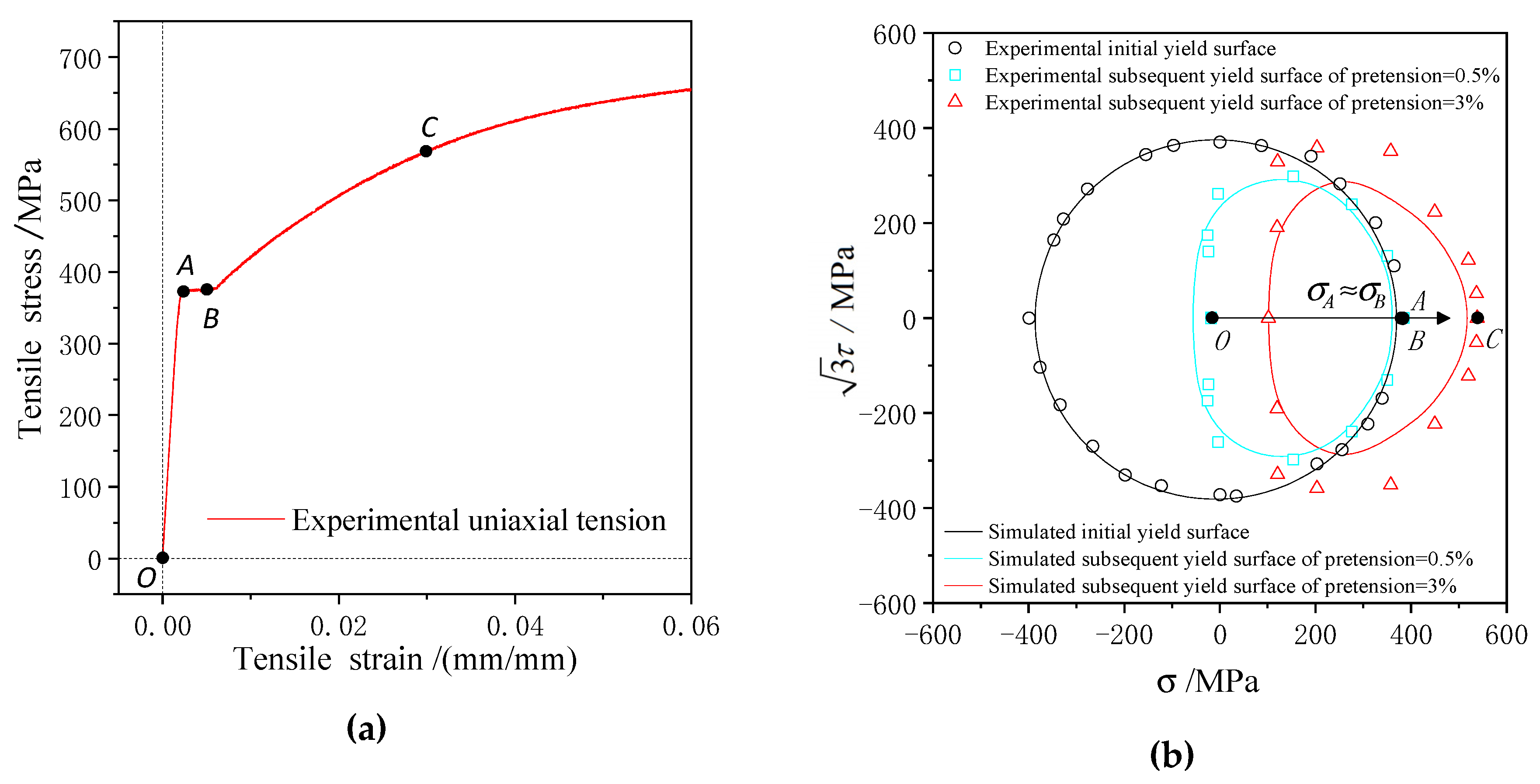
3.1. Calibration of Model
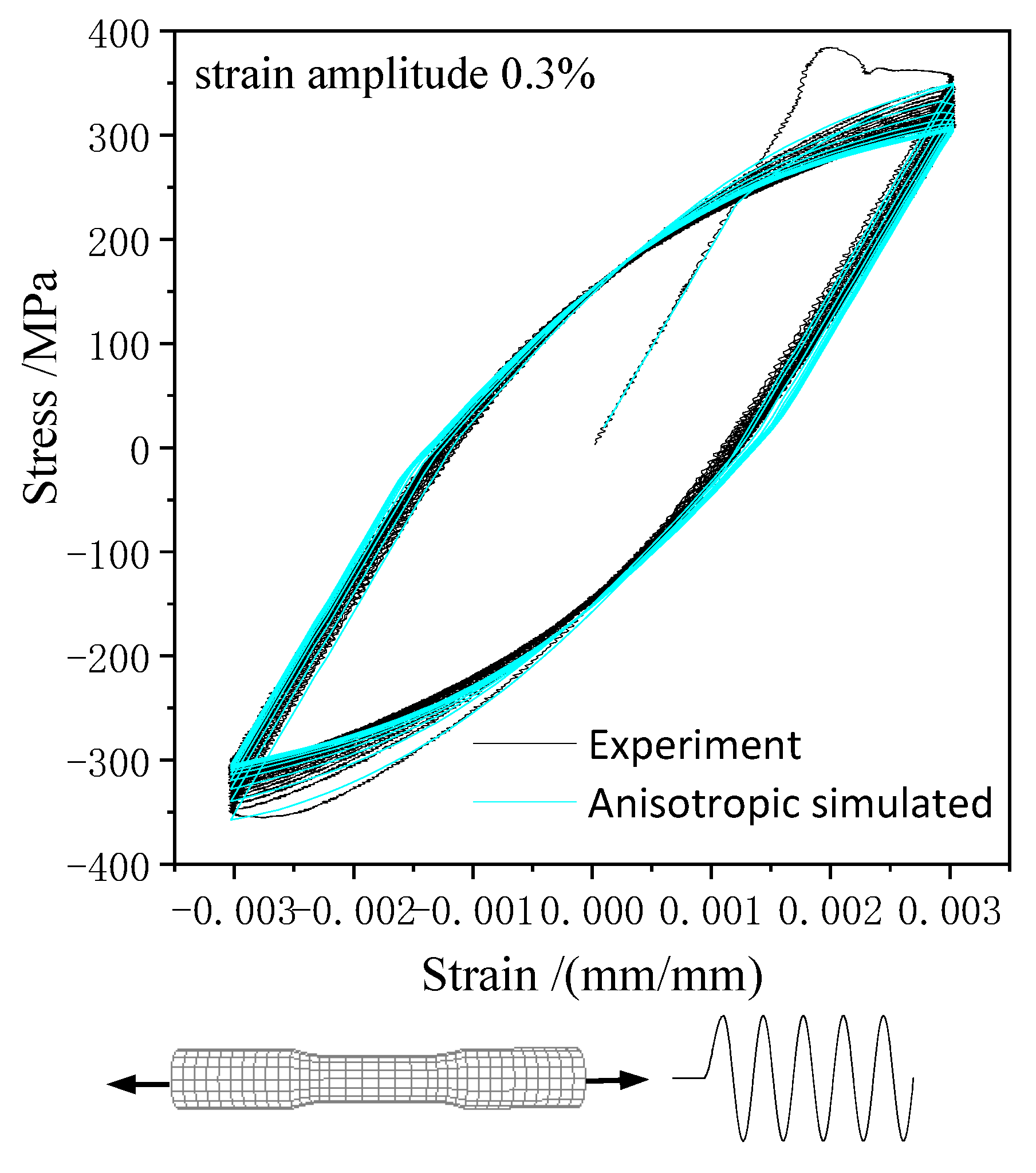
3.1.1. Calibration of Anisotropic Parameters
3.1.2. Calibration of Hardening Parameters
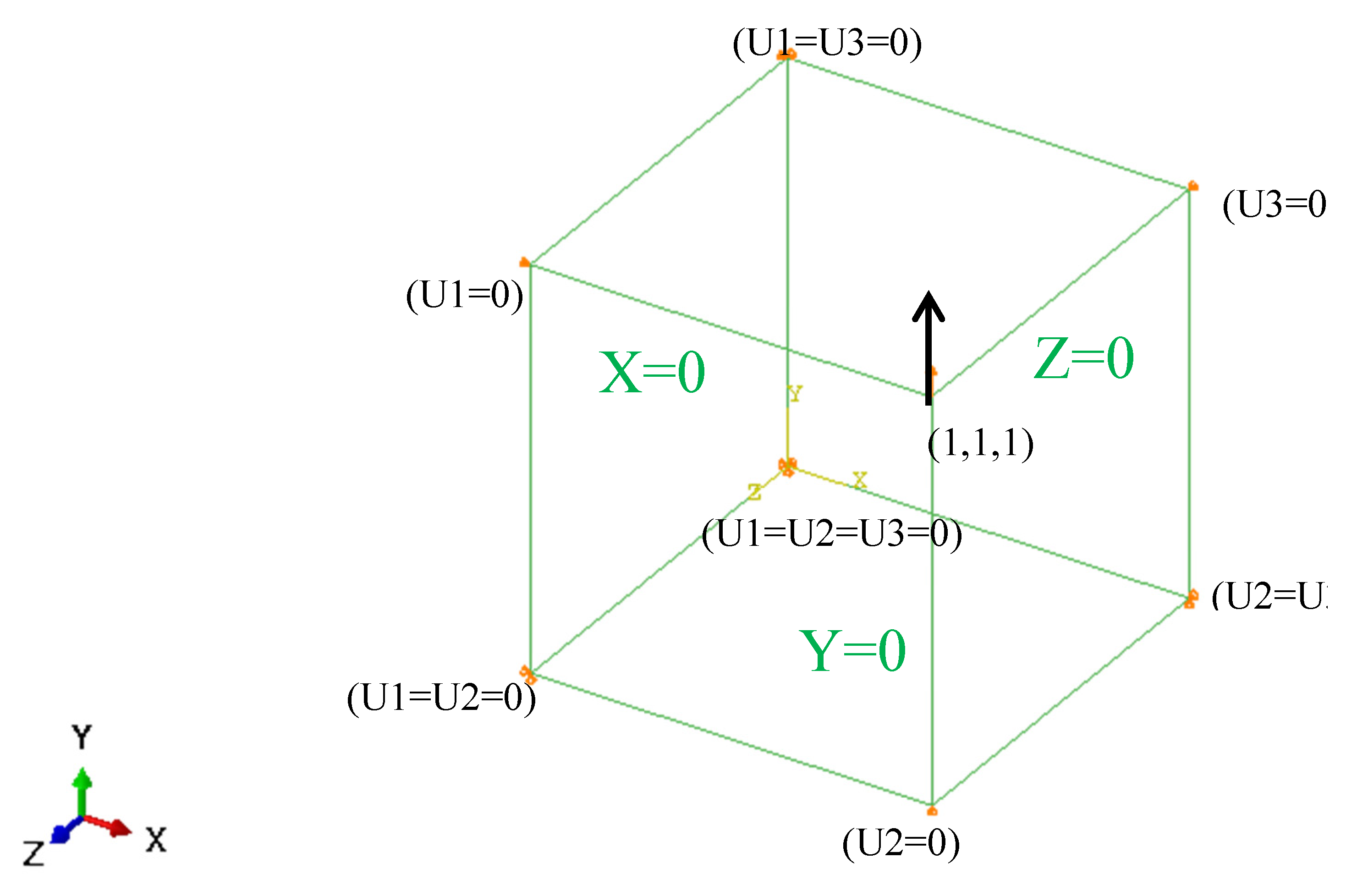
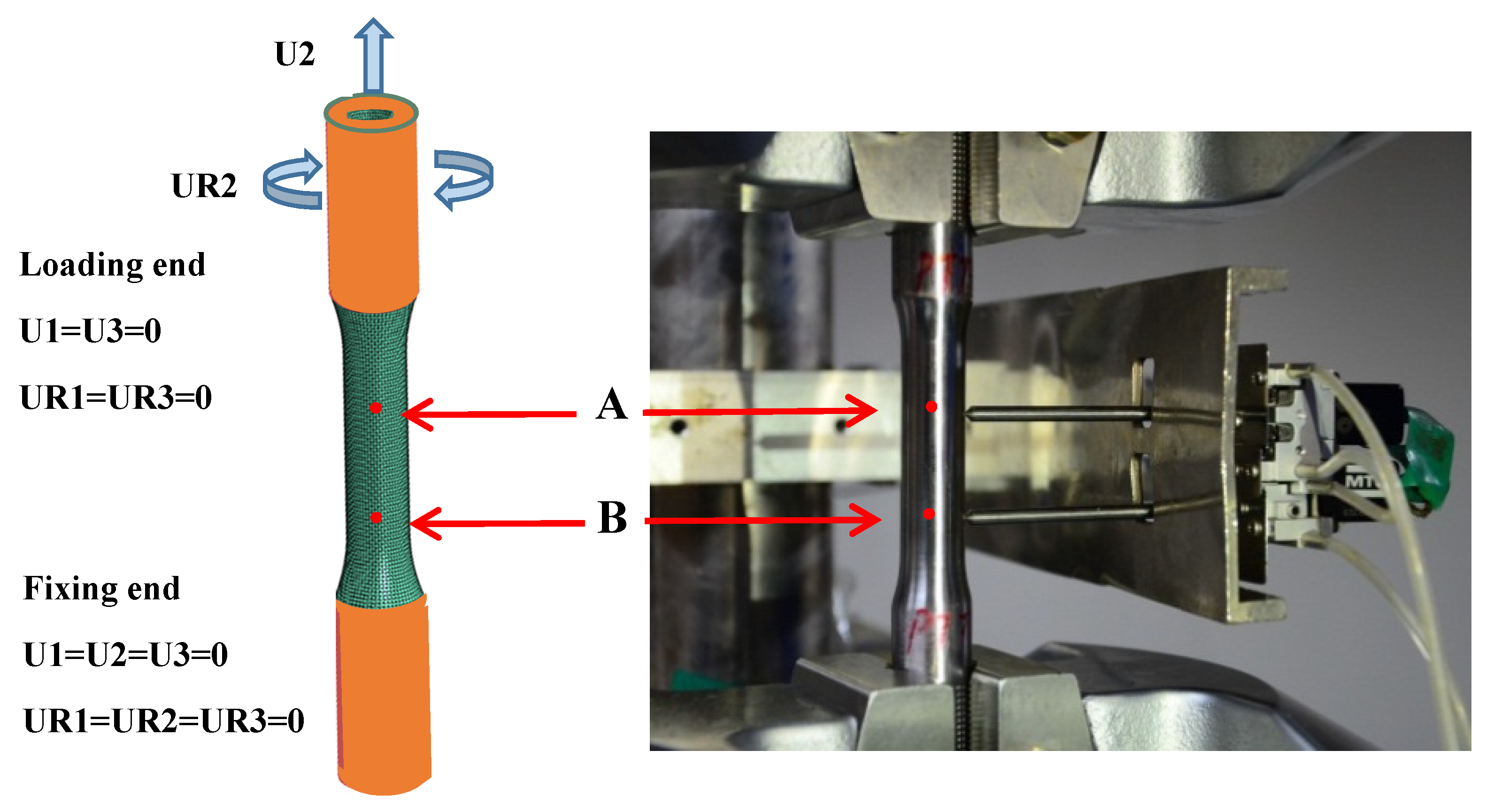
3.2. Finite Element Model
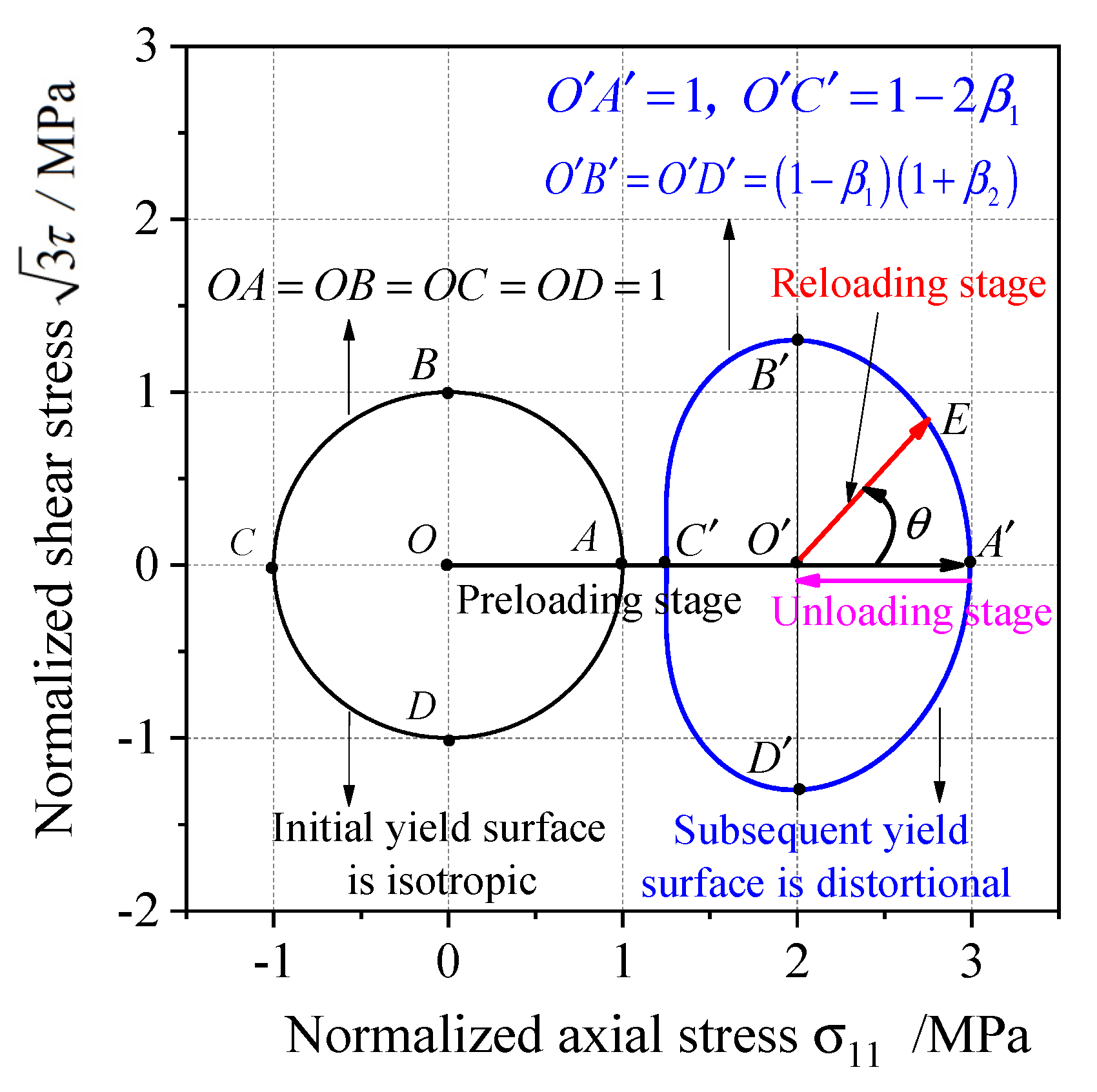
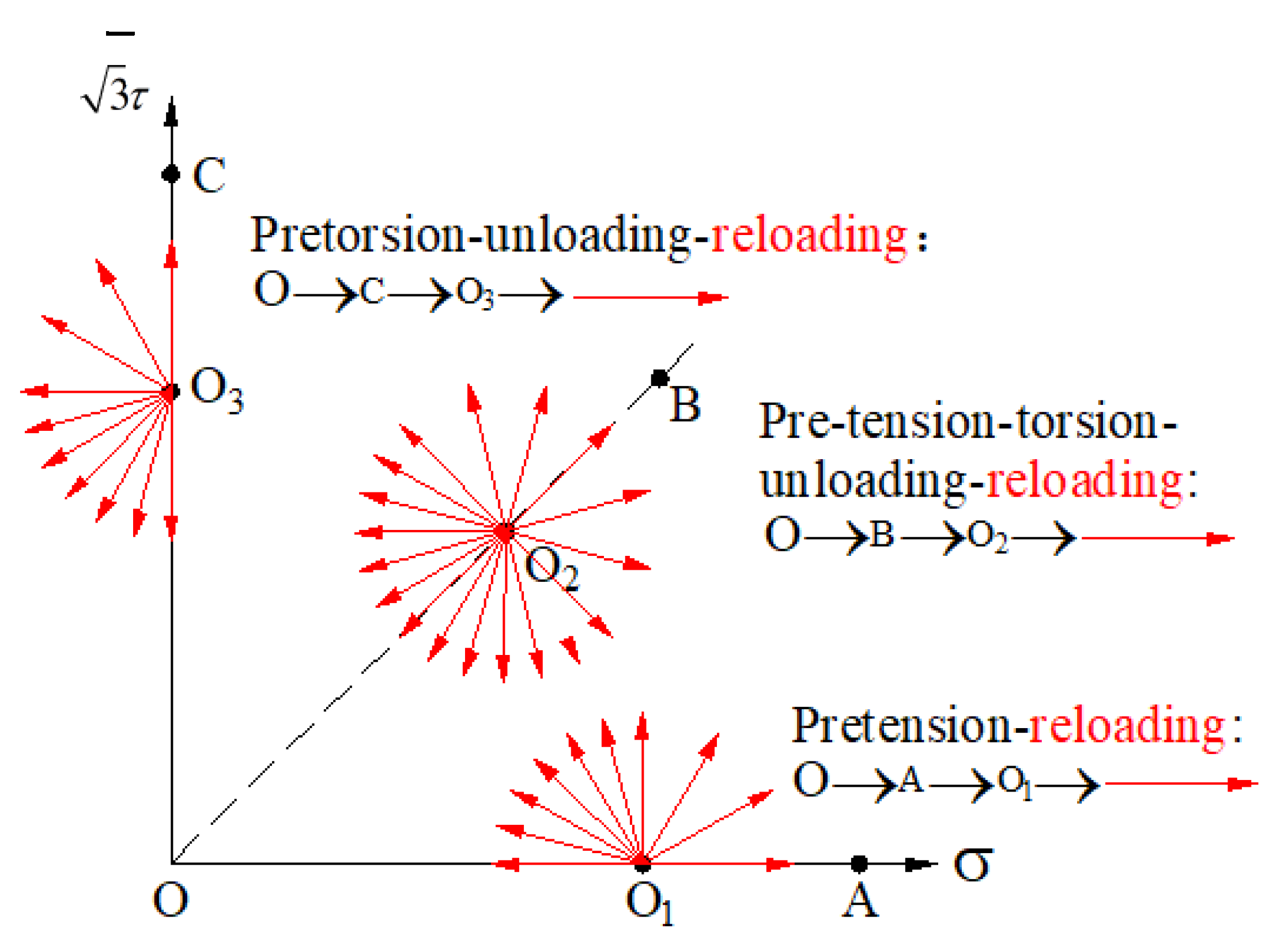
4. Determination Method for the Subsequent Yield Surfaces
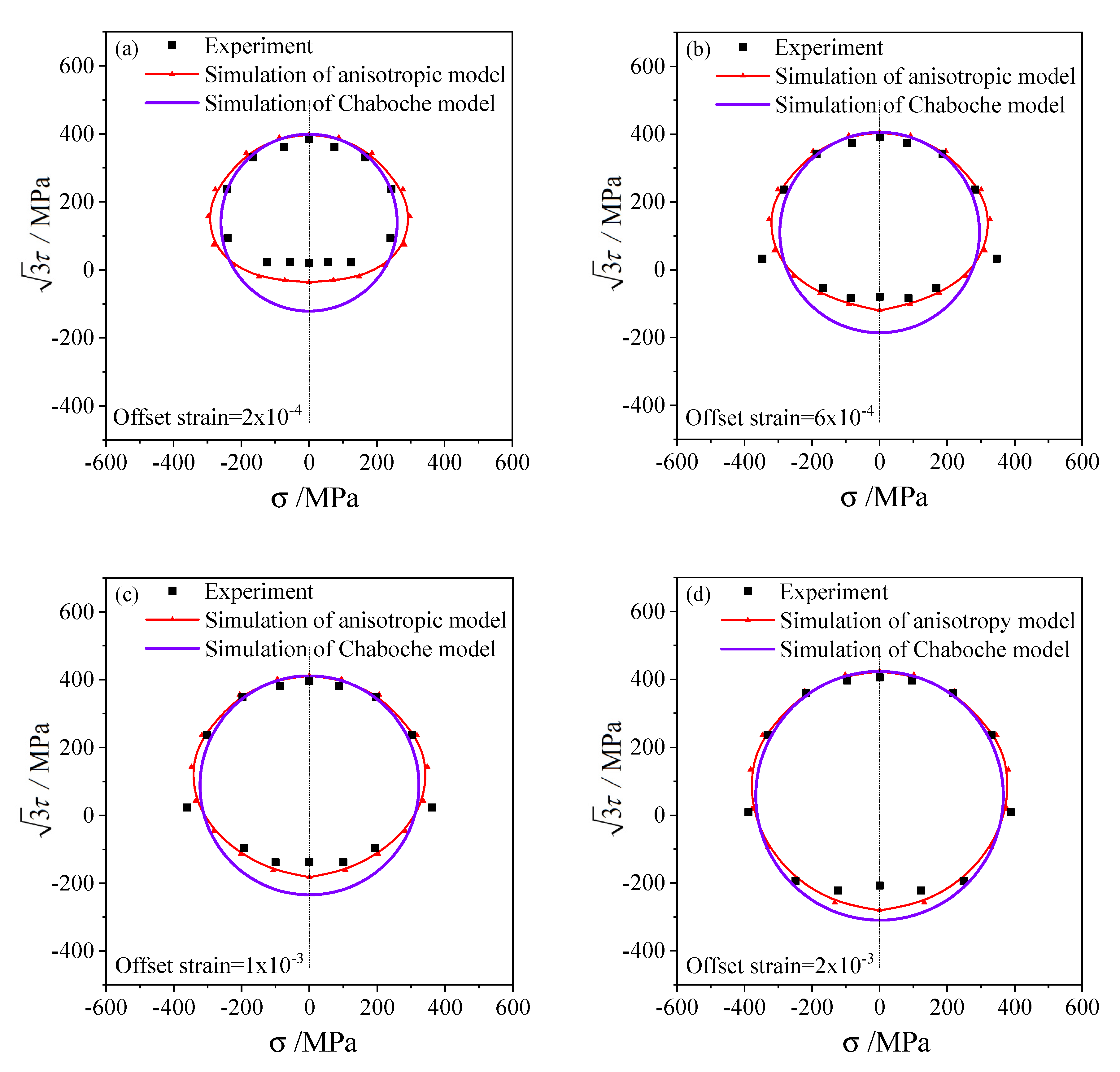
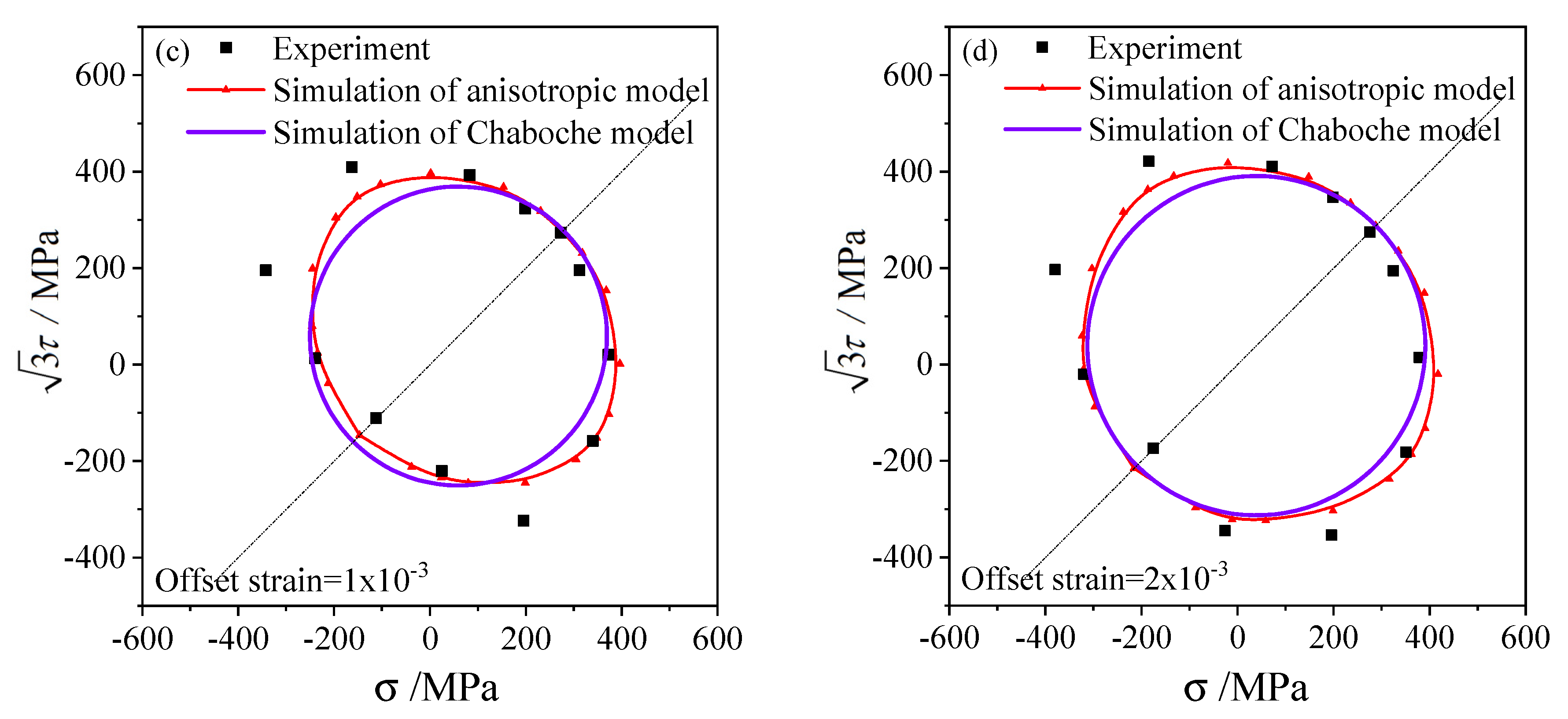
5. The Simulation Results for the Yield Surfaces
6. Discussions and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Prager, W. Recent developments in the mathematical theory of plasticity. J. Appl. Phys. 1949, 20, 235–241. [Google Scholar] [CrossRef]
- Ziegler, H. A modification of Prager’s hardening rule. Q. Appl. Math. 1959, 17, 55–65. [Google Scholar] [CrossRef]
- Armstrong, P.J.; Frederick, C.O. A mathematical representation of the multiaxial Bauschinger effect. High Temp. Technol. 2007, 24, 1–26. [Google Scholar]
- Chaboche, J.L.; Jung, O. Application of a kinematic hardening viscoplasticity model with thresholds to the residual stress relaxation. Int. J. Plast. 1997, 13, 785–807. [Google Scholar] [CrossRef]
- Chaboche, J.L. On some modifications of kinematic hardening to improve the description of ratchetting effects. Int. J. Plast. 1991, 7, 661–678. [Google Scholar] [CrossRef]
- Phillips, A.; Lu, W.L. An experimental investigation yield surfaces and loading of surfaces of pure aluminum with stress-controlled and strain-controlled paths of loading. J. Eng. Mater. Technol. 1984, 106, 349–354. [Google Scholar] [CrossRef]
- Wu, H.C.; Yeh, W.C. On the experimental determination of yield surfaces and some results of annealed 304 stainless steel. Int. J. Plast. 1991, 7, 803–826. [Google Scholar] [CrossRef]
- Zhang, K.S.; Huang, S.H.; Liu, G.J.; Lu, D.M. Measuring subsequent yield surface of pure copper by crystal plasticity simulation. Chin. J. Theor. Appl. Mech. 2017, 49, 870–879. [Google Scholar]
- Khan, A.S.; Pandey, A.; Stoughton, T. Evolution of subsequent yield surfaces and elastic constants with finite plastic deformation. Part II: A very high work hardening aluminum alloy (annealed 1100 Al). Int. J. Plast. 2010, 26, 1421–1431. [Google Scholar] [CrossRef]
- Khan, A.S.; Pandey, A.; Stoughton, T. Evolution of subsequent yield surfaces and elastic constants with finite plastic deformation. Part III: Yield surface in tension–tension stress space (Al 6061–T 6511 and annealed 1100 Al). Int. J. Plast. 2010, 26, 1432–1441. [Google Scholar] [CrossRef]
- Khan, A.S.; Kazmi, R.; Pandey, A.; Stoughton, T. Evolution of subsequent yield surfaces and elastic constants with finite plastic deformation. Part-I: A very low work hardening aluminum alloy (Al6061-T6511). Int. J. Plast. 2009, 25, 1611–1625. [Google Scholar] [CrossRef]
- Francois, M. A plasticity model with yield surface distortion for non proportional loading. Int. J. Plast. 2010, 17, 703–717. [Google Scholar] [CrossRef]
- Feigenbaum, H.P.; Dugdale, J.; Dafalias, Y.F.; Kourousis, K.I.; Plesek, J. Multiaxial ratcheting with advanced kinematic and directional distortional hardening rules. Int. J. Solids Struct. 2012, 49, 3063–3076. [Google Scholar] [CrossRef]
- Feigenbaum, H.P.; Dafalias, Y.F. Directional distortional hardening in metal plasticity within thermodynamics. Int. J. Solids Struct. 2007, 44, 7526–7542. [Google Scholar] [CrossRef]
- Vincent, L.; Calloch, S.; Marquis, D. A general cyclic plasticity model taking into account yield surface distortion for multiaxial ratchetting. Int. J. Plast. 2004, 20, 1817–1850. [Google Scholar] [CrossRef]
- Shi, B.D.; Yan, P.; Chong, Y.; Pan, F.S.; Cheng, R.J.; Peng, Q.M. Loading path dependent distortional hardening of Mg alloys: Experimental investigation and constitutive modeling. Int. J. Plast. 2017, 90, 76–95. [Google Scholar] [CrossRef]
- Chen, J.Y.; Zhang, K.S.; Kuang, Z.; Hu, G.J.; Song, Q.; Chang, Y.J. The anisotropic distortional yield surface constitutive model based on the Chaboche cyclic plastic model. Materials 2019, 12, 543. [Google Scholar] [CrossRef]
- Shi, Y.K.; Zhang, K.S.; Hu, G.J. Subsequent yield and plastic flow analysis of polycrystalline copper under biaxial loading. Acta Metall. Sin. 2009, 45, 1370–1377. [Google Scholar]
- Liu, G.L.; Huang, S.H.; Shi, C.S.; Zeng, B.; Zhang, K.S.; Zhong, X.C. Experimental investigations on subsequent yield surface of pure copper by single-sample and multi-sample methods under various pre-deformation. Materials 2018, 11, 277. [Google Scholar] [CrossRef]
- Hu, G.J.; Zhang, K.S.; Huang, S.H.; Ju, J.W. Yield surfaces and plastic flow of 45 steel under tension-torsion loading paths. Acta Mech. Solida Sin. 2012, 25, 348–360. [Google Scholar] [CrossRef]
- Chen, J.Y. Subsequent Yield Behavior and Anisotropic Yield Surface Model of HRB400E Steel under Combined Pull-Twist Loading. Ph.D. Thesis, Guangxi University, Nanning, China, 2019. [Google Scholar]
- Hu, G.J.; Zhang, K.S.; Mo, Z.L. Numerical analysis of subsequent yield surfaces and plastic flow direction of 45 steel Chaboche model. Chin. J. Guangxi Univ. 2014, 39, 171–179. [Google Scholar]


| Elastic Constants | Isotropic Hardening Parameters | Kinematic Hardening Parameters | Anisotropic Hardening Parameters | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Unit | GPa | - | - | MPa | MPa | MPa | - | MPa | - | MPa | - | - | - | - |
| anisotropic model | 193 | 0.33 | 1.05 | −25 | 160 | 80 | 200 | 90 | 800 | 210 | 35 | 0.3 | 0.8 | 2 |
| Chaboche model | 193 | 0.33 | 1.05 | −25 | 160 | 80 | 200 | 90 | 800 | 210 | 35 | 0 | 0 | 0 |
| Error (%) (Standard Deviation) (%) | Offset Strain | Mean (%) | ||||
|---|---|---|---|---|---|---|
| 2 × 10−4 | 6 × 10−4 | 1 × 10−3 | 2 × 10−3 | |||
| Simulation by the Chaboche model | Pre-tension loading | 20.24 (10.20) | 14.75 (7.27) | 13.18 (4.78) | 8.27 (5.99) | 14.82 (9.74) |
| Pre-torsion loading | 25.11 (25.65) | 15.21 (10.56) | 12.44 (7.59) | 8.63 (6.92) | ||
| Pre-tension–torsion loading | 19.15 (13.76) | 14.90 (8.17) | 13.74 (8.57) | 12.20 (7.43) | ||
| Simulation by the anisotropic model | Pre-tension loading | 8.07 (6.57) | 10.49 (5.77) | 9.20 (6.93) | 7.04 (6.14) | 9.49 (6.56) |
| Pre-torsion loading | 15.93 (6.81) | 7.56 (4.77) | 6.40 (4.54) | 6.26 (5.20) | ||
| Pre-tension–torsion loading | 12.01 (9.68) | 9.38 (7.27) | 10.38 (7.58) | 11.11 (7.41) | ||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, Y.; Kuang, Z.; Tang, R.; Chen, J.; Song, Q. Investigation of Anisotropic Subsequent Yield Behavior for 45 Steel by the Distortional Yield Surface Constitutive Model. Materials 2020, 13, 1196. https://doi.org/10.3390/ma13051196
Chang Y, Kuang Z, Tang R, Chen J, Song Q. Investigation of Anisotropic Subsequent Yield Behavior for 45 Steel by the Distortional Yield Surface Constitutive Model. Materials. 2020; 13(5):1196. https://doi.org/10.3390/ma13051196
Chicago/Turabian StyleChang, Yanjun, Zheng Kuang, Runsen Tang, Jianyun Chen, and Qiao Song. 2020. "Investigation of Anisotropic Subsequent Yield Behavior for 45 Steel by the Distortional Yield Surface Constitutive Model" Materials 13, no. 5: 1196. https://doi.org/10.3390/ma13051196
APA StyleChang, Y., Kuang, Z., Tang, R., Chen, J., & Song, Q. (2020). Investigation of Anisotropic Subsequent Yield Behavior for 45 Steel by the Distortional Yield Surface Constitutive Model. Materials, 13(5), 1196. https://doi.org/10.3390/ma13051196





