A Novel Multi-Scale Particle Morphology Descriptor with the Application of SPHERICAL Harmonics
Abstract
1. Introduction
2. Morphological Data Acquisition and SH-Based Particle Surface Decomposition
2.1. Data Acquisition by X-ray μCT
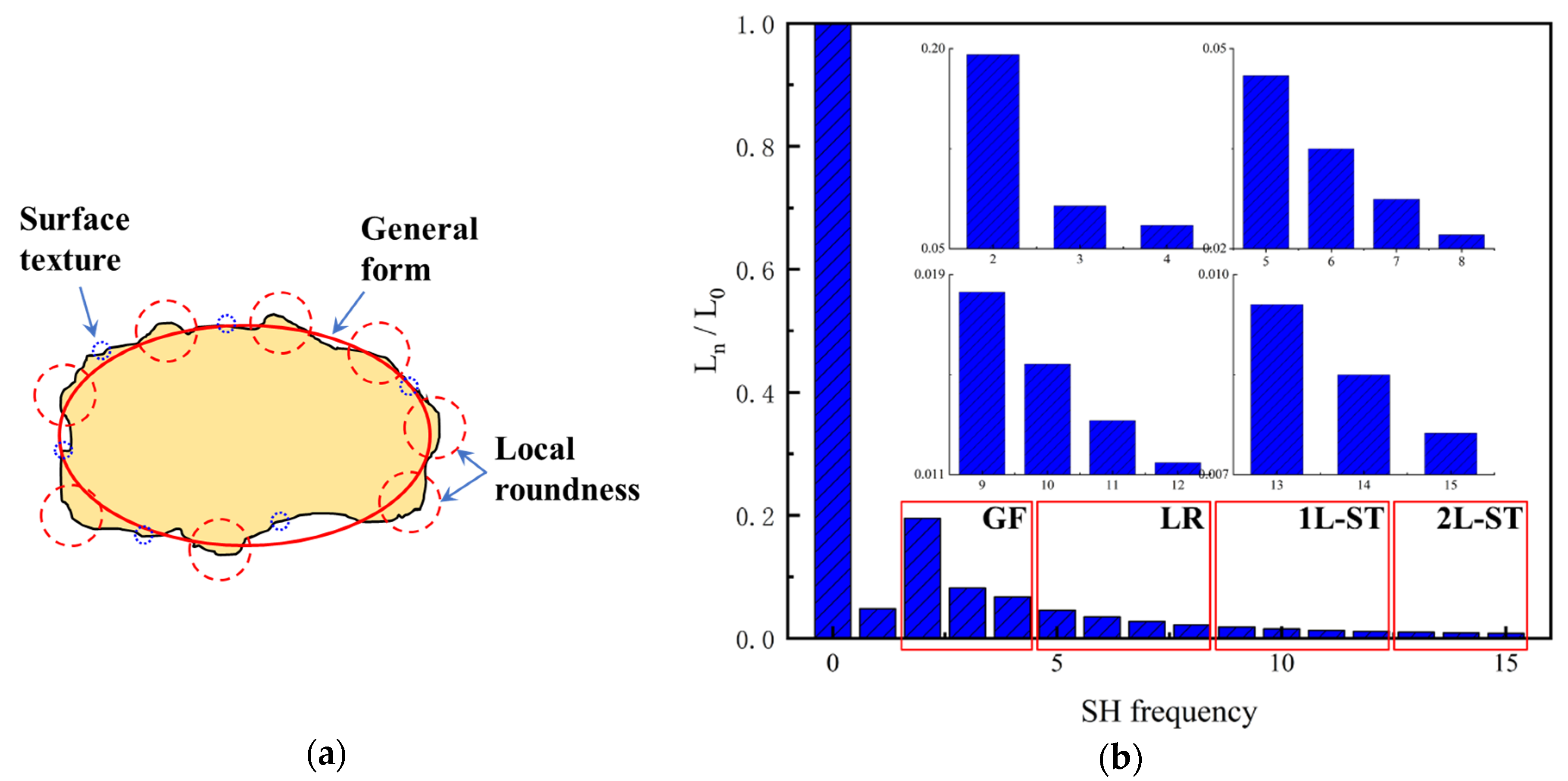
2.2. Spherical Harmonic-Based Particle Surface Decomposition
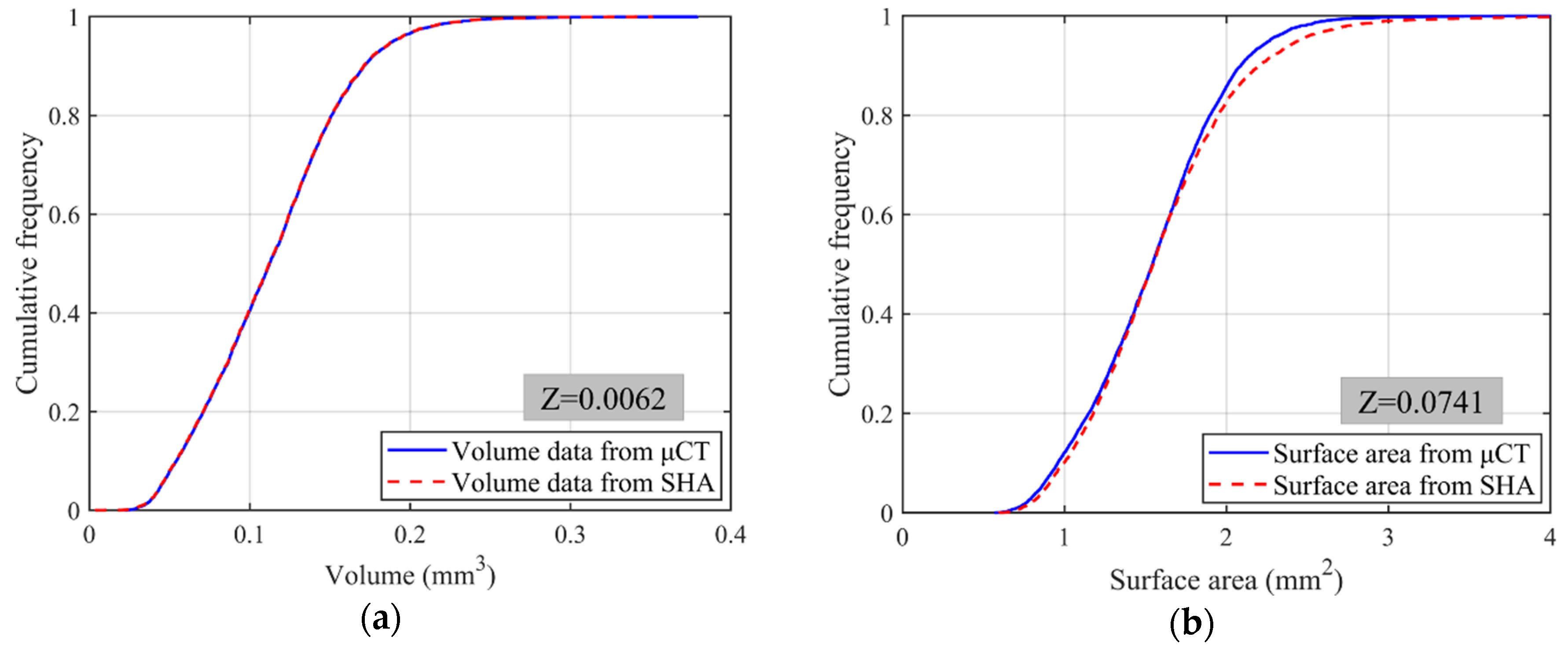
2.3. Verification
3. Inter-Scale Effect of Traditional Morphology Descriptors
4. A Novel Multi-Scale Morphology Descriptor
4.1. Definition
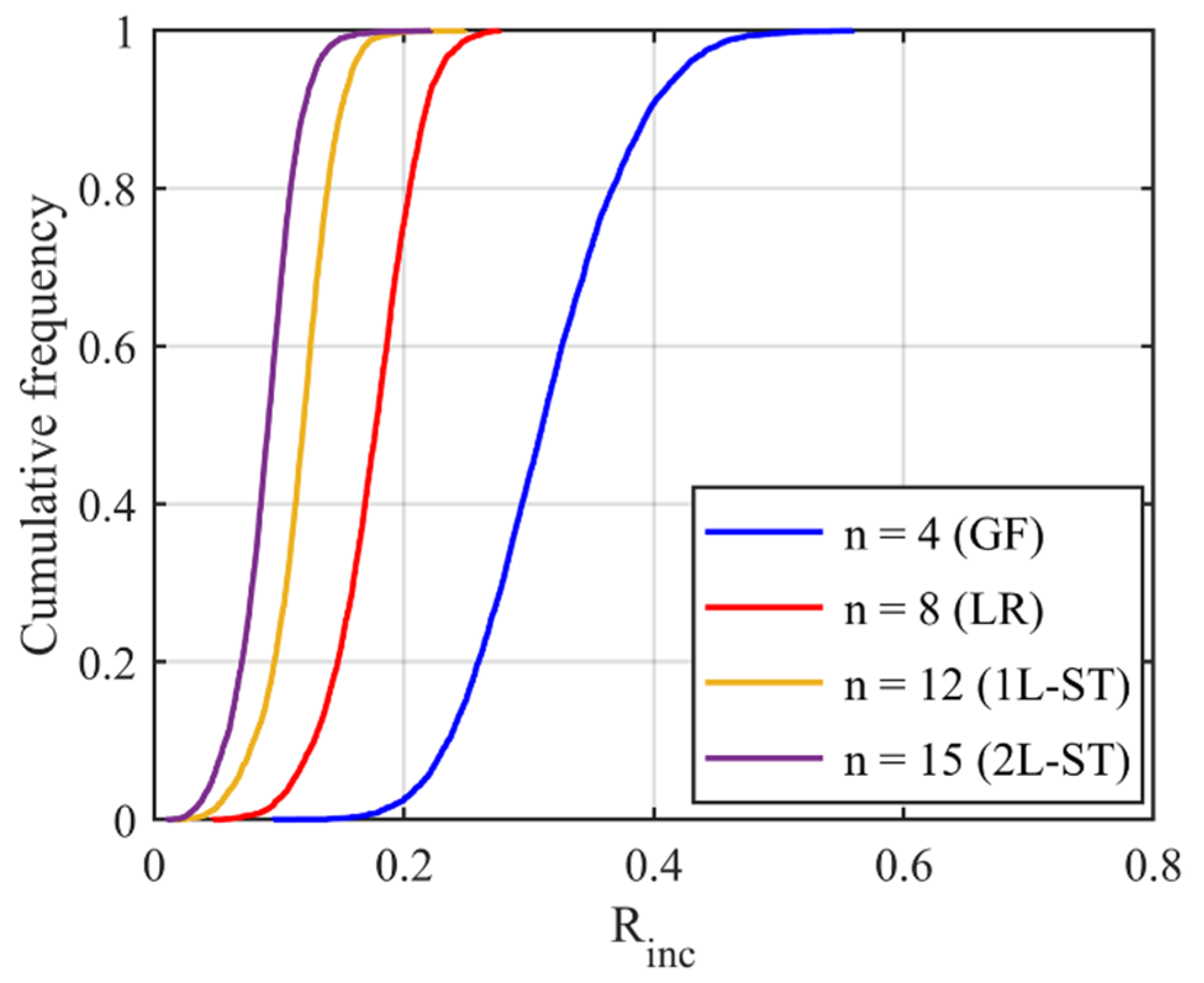
4.2. Variation of Rinc with SH Decomposition
4.3. Estimating Rinc Using Artificial Neural Network (ANN)
4.4. Variation of Rinc against Incremental Surface Area
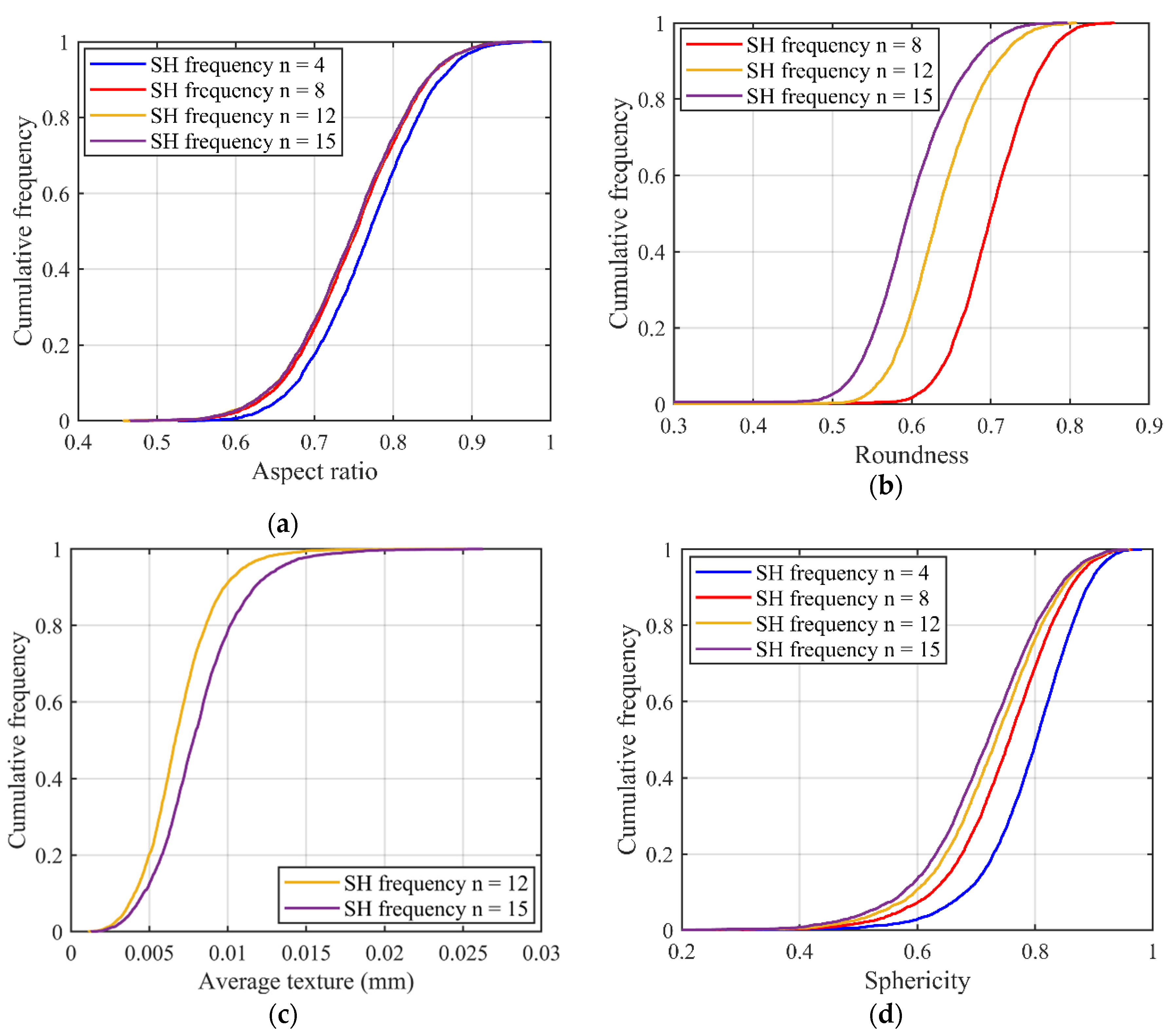
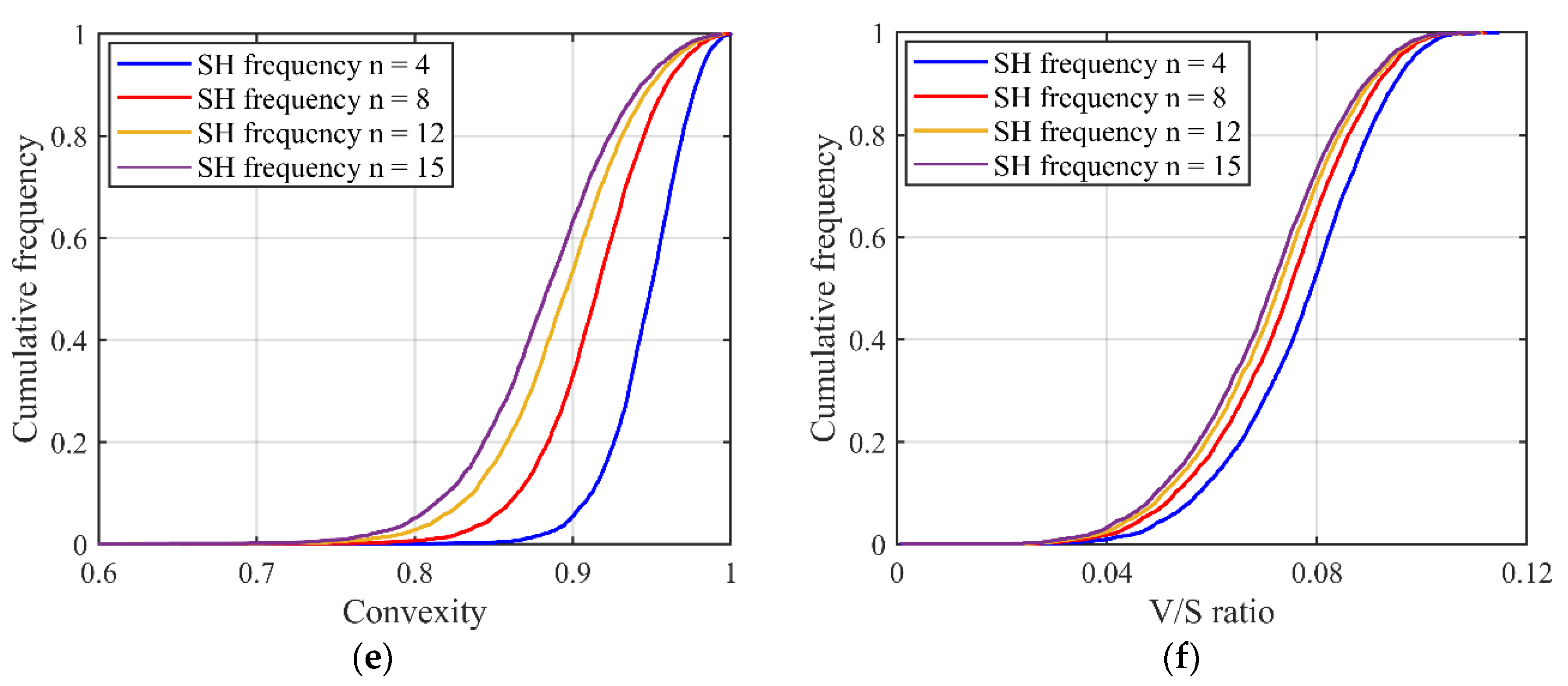
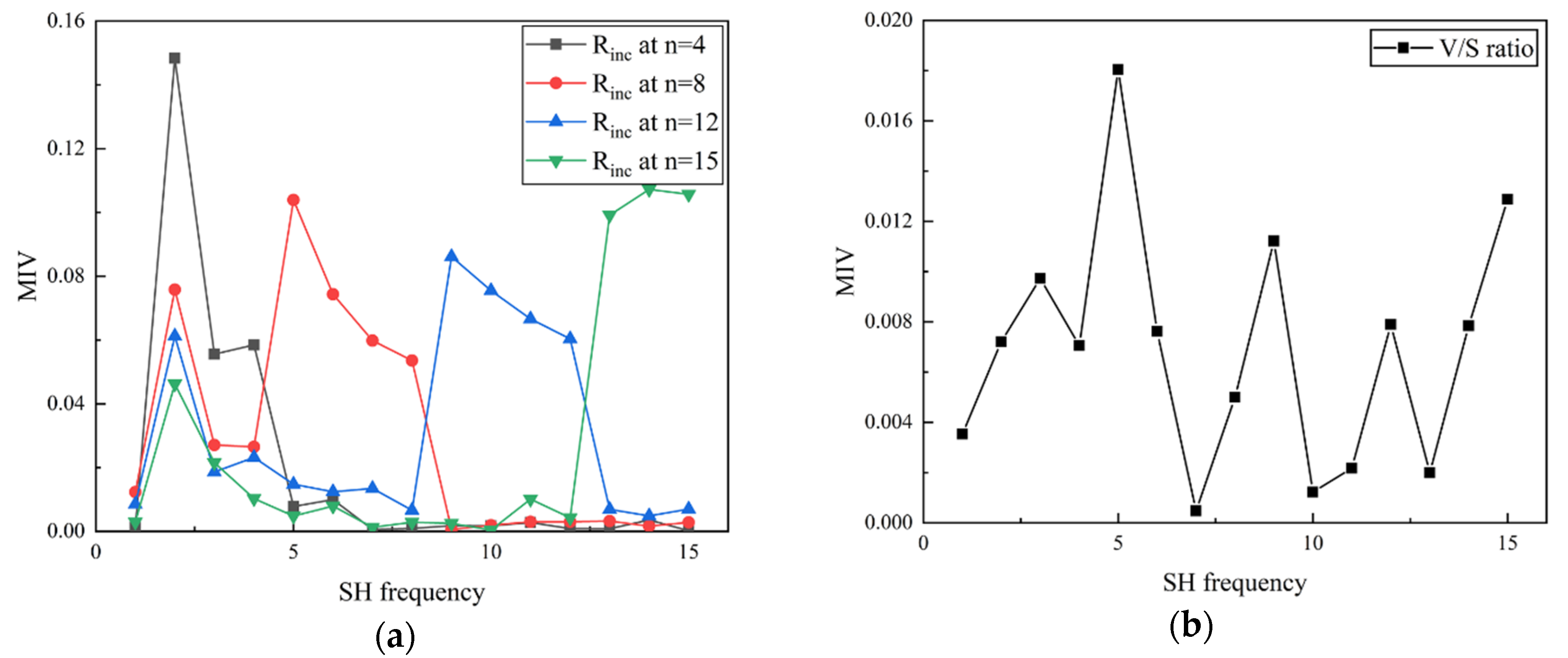
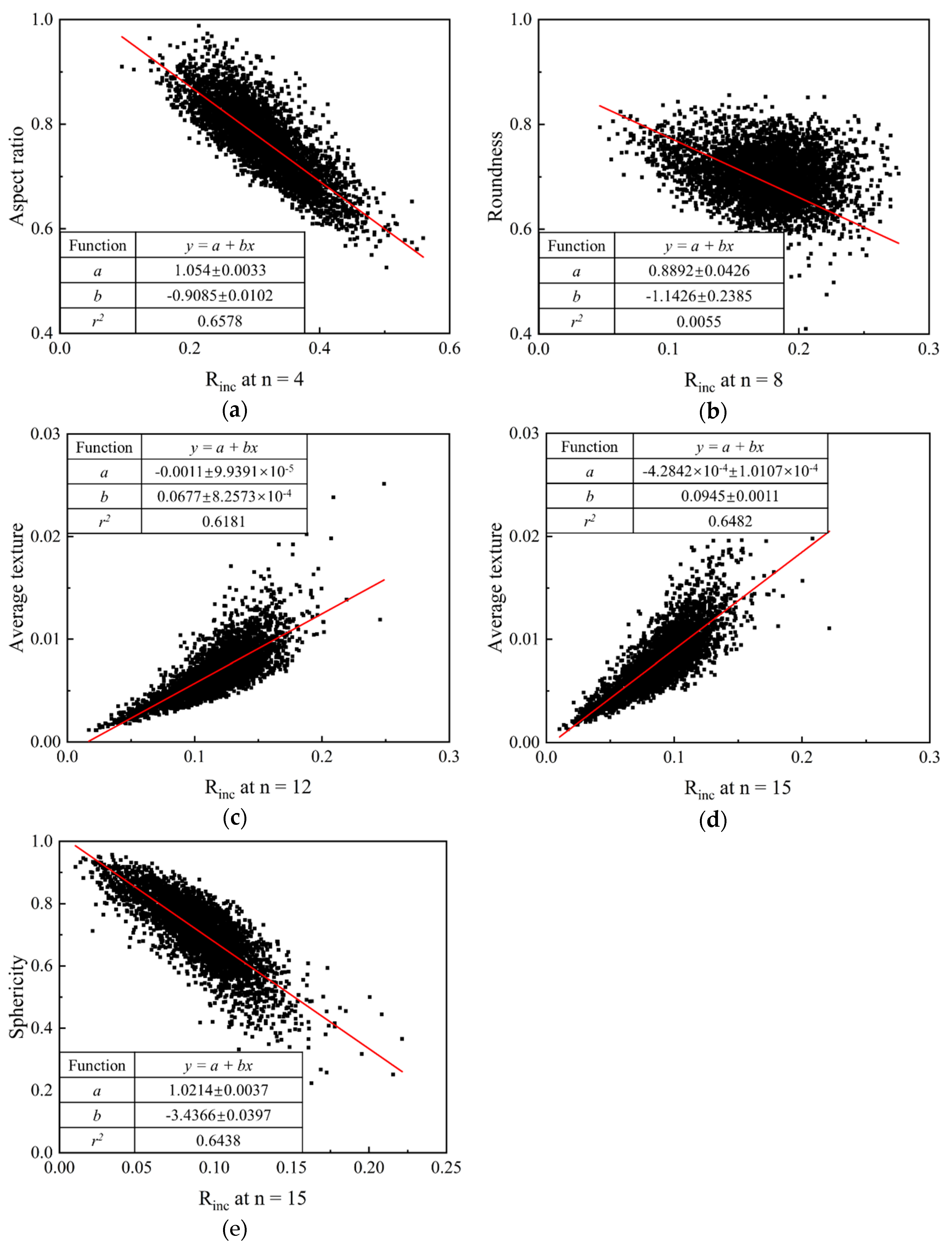
4.5. Variation of Rinc against Traditional Descriptors at Target RLS
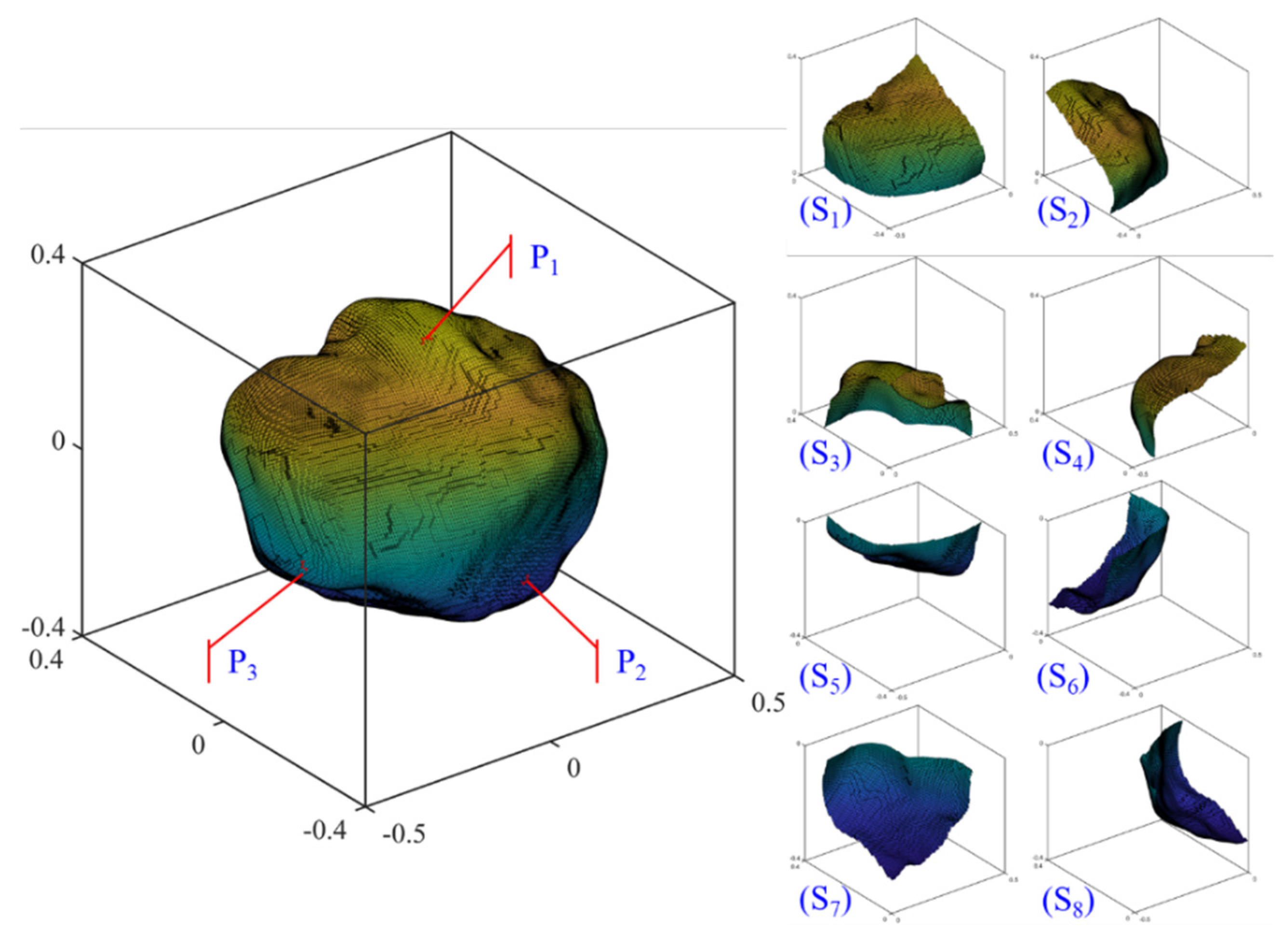
4.6. Surface Roughness Heterogeneity by Rinc
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| ANN | Artificial neural network |
| CT | Computed tomography |
| GF | General form |
| IMV | Incremental morphology variation |
| LBS | Leighton Buzzard Sand |
| LOESS | locally weighted regression smoothing |
| LR | Local roundness |
| MIV | Mean impact value |
| RLS | Relative length scale |
| SH | Spherical harmonics |
| SHA | Spherical harmonic analysis |
| SSRF | Shanghai Synchrotron Radiation Facility |
| ST | Surface texture |
| V/S ratio | Ratio of volume to surface area |
| μCT | Micro-tomography |
| 1L-ST | First-level surface texture |
| 2L-ST | Second-level surface texture |
| 3D | Three-dimensional |
References
- Santamarina, C.; Cascante, G. Effect of surface roughness on wave propagation parameters. Geotechnique 1998, 48, 129–136. [Google Scholar] [CrossRef]
- Iwashita, K.; Oda, M. Micro-deformation mechanism of shear banding process based on modified distinct element method. Powder Technol. 2000, 109, 192–205. [Google Scholar] [CrossRef]
- Cho, G.-C.; Dodds, J.; Santamarina, J.C. Particle shape effects on packing density, stiffness, and strength: Natural and crushed sands. J. Geotech. Geoenviron. Eng. 2006, 132, 591–602. [Google Scholar] [CrossRef]
- Wang, J.; Yan, H. On the role of particle breakage in the shear failure behavior of granular soils by DEM. Int. J. Numer. Anal. Methods Geomech. 2013, 37, 832–854. [Google Scholar] [CrossRef]
- Zhao, S.; Zhang, N.; Zhou, X.; Zhang, L. Particle shape effects on fabric of granular random packing. Powder Technol. 2017, 310, 175–186. [Google Scholar] [CrossRef]
- Zhao, S.; Evans, T.M.; Zhou, X. Shear-induced anisotropy of granular materials with rolling resistance and particle shape effects. Int. J. Solids Struct. 2018, 150, 268–281. [Google Scholar] [CrossRef]
- Wu, M.; Wang, J.; Russell, A.; Cheng, Z. DEM modelling of mini-triaxial test based on one-to-one mapping of sand particles. Géotechnique 2020, 1–49. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, J.; Cheng, Y. Quantified evaluation of particle shape effects from micro-to-macro scales for non-convex grains. Particuology 2016, 25, 23–35. [Google Scholar] [CrossRef]
- Krumbein, W.C.; Sloss, L.L. Stratigraphy and Sedimentation; LWW: Philadelphia, PA, USA, 1951; Volume 71. [Google Scholar]
- Altuhafi, F.; COOP, M.R. Changes to particle characteristics associated with the compression of sands. Géotechnique 2011, 61, 459–471. [Google Scholar] [CrossRef]
- Zheng, J.; Hryciw, R. Soil particle size and shape distributions by stereophotography and image analysis. Geotech. Test. J. 2017, 40, 317–328. [Google Scholar] [CrossRef]
- Zhao, B.; Wang, J. 3D quantitative shape analysis on form, roundness, and compactness with μCT. Powder Technol. 2016, 291, 262–275. [Google Scholar] [CrossRef]
- Bowman, E.T.; Soga, K.; Drummond, W. Particle shape characterisation using Fourier descriptor analysis. Geotechnique 2001, 51, 545–554. [Google Scholar] [CrossRef]
- Mollon, G.; Zhao, J. Generating realistic 3D sand particles using Fourier descriptors. Granul. Matter 2013, 15, 95–108. [Google Scholar] [CrossRef]
- Orford, J.; Whalley, W. The use of the fractal dimension to quantify the morphology of irregular-shaped particles. Sedimentology 1983, 30, 655–668. [Google Scholar] [CrossRef]
- Yang, H.; Baudet, B.A.; Yao, T. Characterization of the surface roughness of sand particles using an advanced fractal approach. Proc. R. Soc. A 2016, 472, 20160524. [Google Scholar] [CrossRef]
- Mallat, S.G. A theory for multiresolution signal decomposition: The wavelet representation. IEEE Trans. Pattern Anal. Mach. Intell. 1989, 11, 674–693. [Google Scholar] [CrossRef]
- Shen, L.; Farid, H.; McPeek, M.A. Modeling three-dimensional morphological structures using spherical harmonics. Evolution 2009, 63, 1003–1016. [Google Scholar] [CrossRef]
- Zhao, B.; Wang, J.; Coop, M.; Viggiani, G.; Jiang, M. An investigation of single sand particle fracture using X-ray micro-tomography. Géotechnique 2015, 65, 625–641. [Google Scholar] [CrossRef]
- Zhou, B.; Wang, J.; Wang, H. Three-dimensional sphericity, roundness and fractal dimension of sand particles. Géotechnique 2018, 68, 18–30. [Google Scholar] [CrossRef]
- Blott, S.J.; Pye, K. Particle shape: A review and new methods of characterization and classification. Sedimentology 2008, 55, 31–63. [Google Scholar] [CrossRef]
- Zhou, B.; Wang, J.; Zhao, B. Micromorphology characterization and reconstruction of sand particles using micro X-ray tomography and spherical harmonics. Eng. Geol. 2015, 184, 126–137. [Google Scholar] [CrossRef]
- Zheng, J.; Hryciw, R.D. Traditional soil particle sphericity, roundness and surface roughness by computational geometry. Géotechnique 2015, 65, 494–506. [Google Scholar] [CrossRef]
- Santamarina, J.; Cho, G.-C. Soil behaviour: The role of particle shape. In Proceedings of the Advances in geotechnical engineering: The Skempton conference: Proceedings of a three day conference on advances in geotechnical engineering, organised by the Institution of Civil Engineers and held at the Royal Geographical Society, London, UK, 29–31 March 2004; pp. 604–617. [Google Scholar]
- Fonseca, J.; O’sullivan, C.; Coop, M.R.; Lee, P. Non-invasive characterization of particle morphology of natural sands. Soils Found. 2012, 52, 712–722. [Google Scholar] [CrossRef]
- Hall, S.A.; Bornert, M.; Desrues, J.; Pannier, Y.; Lenoir, N.; Viggiani, G.; Bésuelle, P. Discrete and continuum analysis of localised deformation in sand using X-ray μCT and volumetric digital image correlation. Géotechnique 2010, 60, 315–322. [Google Scholar] [CrossRef]
- Hasan, A.; Alshibli, K. Experimental assessment of 3D particle-to-particle interaction within sheared sand using synchrotron microtomography. Géotechnique 2010, 60, 369–379. [Google Scholar] [CrossRef]
- Cheng, Z.; Wang, J. A particle-tracking method for experimental investigation of kinematics of sand particles under triaxial compression. Powder Technol. 2018, 328, 436–451. [Google Scholar] [CrossRef]
- Cheng, Z.; Wang, J.; Coop, M.R.; Ye, G. A miniature triaxial apparatus for investigating the micromechanics of granular soils with in situ X-ray micro-tomography scanning. Front. Struct. Civ. Eng. 2020, 14, 267–279. [Google Scholar] [CrossRef]
- Fonseca, J.; O'SULLIVAN, C.; COOP, M.R.; Lee, P. Quantifying the evolution of soil fabric during shearing using directional parameters. Géotechnique 2013, 63, 487–499. [Google Scholar] [CrossRef]
- Cheng, Z.; Wang, J. Experimental investigation of inter-particle contact evolution of sheared granular materials using X-ray micro-tomography. Soils Found. 2018, 58, 1492–1510. [Google Scholar] [CrossRef]
- Chen, R.-C.; Dreossi, D.; Mancini, L.; Menk, R.; Rigon, L.; Xiao, T.-Q.; Longo, R. PITRE: Software for phase-sensitive X-ray image processing and tomography reconstruction. J. Synchrotron Radiat. 2012, 19, 836–845. [Google Scholar] [CrossRef]
- Cheng, Z.; Wang, J. Visualization of Failure and the Associated Grain-Scale Mechanical Behavior of Granular Soils under Shear using Synchrotron X-Ray Micro-Tomography. JoVE 2019, e60322. [Google Scholar] [CrossRef]
- Perona, P.; Malik, J. Scale-space and edge detection using anisotropic diffusion. IEEE Trans. Pattern Anal. Mach. Intell. 1990, 12, 629–639. [Google Scholar] [CrossRef]
- Otsu, N. A threshold selection method from gray-level histograms. IEEE Trans. Syst. Man Cybern. 1979, 9, 62–66. [Google Scholar] [CrossRef]
- Nie, J.-Y.; Li, D.-Q.; Cao, Z.-J.; Zhou, B.; Zhang, A.-J. Probabilistic characterization and simulation of realistic particle shape based on sphere harmonic representation and Nataf transformation. Powder Technol. 2020, 360, 209–220. [Google Scholar] [CrossRef]
- Zhou, B.; Wang, J. Random generation of natural sand assembly using micro x-ray tomography and spherical harmonics. Géotechnique Lett. 2015, 5, 6–11. [Google Scholar] [CrossRef]
- Zhou, B.; Wang, J. Generation of a realistic 3D sand assembly using X-ray micro-computed tomography and spherical harmonic-based principal component analysis. Int. J. Numer. Anal. Methods Geomech. 2017, 41, 93–109. [Google Scholar] [CrossRef]
- Cheng, Z.; Zhou, B.; Wang, J. Tracking particles in sands based on particle shape parameters. Adv. Powder Technol. 2020, 31, 2005–2019. [Google Scholar] [CrossRef]
- Zhou, B.; Wang, J.; Wang, H. A novel particle tracking method for granular sands based on spherical harmonic rotational invariants. Géotechnique 2018, 68, 1116–1123. [Google Scholar] [CrossRef]
- Mitchell, J.K.; Soga, K. Fundamentals of Soil Behavior; John Wiley & Sons: New York, NY, USA, 2005. [Google Scholar]
- Sprinthall, R.C.; Fisk, S.T. Basic Statistical Analysis; Prentice Hall: Englewood Cliffs, NJ, USA, 1990. [Google Scholar]
- Sun, Q.; Zheng, J. Realistic soil particle generation based on limited morphological information by probability-based spherical harmonics. Comput. Part. Mech. 2020, 1–21. [Google Scholar] [CrossRef]
- Lüttig, G. Eine neue, einfache geröllmorphometrische Methode. E G Quat. Sci. J. 1956, 7, 13–20. [Google Scholar] [CrossRef]
- Dobkins, J.E.; Folk, R.L. Shape development on Tahiti-nui. J. Sediment. Res. 1970, 40, 1167–1203. [Google Scholar]
- Alshibli, K.A.; Druckrey, A.M.; Al-Raoush, R.I.; Weiskittel, T.; Lavrik, N.V. Quantifying morphology of sands using 3D imaging. J. Mater. Civ. Eng. 2015, 27, 04014275. [Google Scholar] [CrossRef]
- Wadell, H. Volume, shape, and roundness of quartz particles. J. Geol. 1935, 43, 250–280. [Google Scholar] [CrossRef]
- Tahmasebi, P. Packing of discrete and irregular particles. Comput. Geotech. 2018, 100, 52–61. [Google Scholar] [CrossRef]
- Su, D.; Yan, W. 3D characterization of general-shape sand particles using microfocus X-ray computed tomography and spherical harmonic functions, and particle regeneration using multivariate random vector. Powder Technol. 2018, 323, 8–23. [Google Scholar] [CrossRef]
- Dombi, G.W.; Nandi, P.; Saxe, J.M.; Ledgerwood, A.M.; Lucas, C.E. Prediction of rib fracture injury outcome by an artificial neural network. J. Trauma Acute Care Surg. 1995, 39, 915–921. [Google Scholar] [CrossRef]
- Lourakis, M.I. A brief description of the Levenberg-Marquardt algorithm implemented by levmar. Found. Res. Technol. 2005, 4, 1–6. [Google Scholar]
- Rabbani, A.; Babaei, M. Hybrid pore-network and lattice-Boltzmann permeability modelling accelerated by machine learning. Adv. Water Resour. 2019, 126, 116–128. [Google Scholar] [CrossRef]
- Hryciw, R.D.; Zheng, J.; Shetler, K. Particle roundness and sphericity from images of assemblies by chart estimates and computer methods. J. Geotech. Geoenviron. Eng. 2016, 142, 04016038. [Google Scholar] [CrossRef]
- Zheng, J.; Hryciw, R.D. Roundness and sphericity of soil particles in assemblies by computational geometry. J. Comput. Civ. Eng. 2016, 30, 04016021. [Google Scholar] [CrossRef]



| Group | Descriptor | Formula | Definition | References |
|---|---|---|---|---|
| General form | Elongation | Ratio of the second principal dimension (I) over the first principal dimension (L) | [21,44] | |
| Flatness | Ratio of the third principal dimension (S) over the second principal dimension | |||
| Aspect ratio | The mean value of elongation and flatness | |||
| Local roundness | Roundness | Ratio of all corner curvature radii () to the largest inscribed sphere radius (Rinsc) | [20,45] | |
| Surface texture | Average texture | The arithmetic average of the target surface departure from the mean surface | [46] | |
| Overall shape parameter | Sphericity | Ratio of the surface area (SA) of a sphere with the same volume (V) as the given particle to surface area of this particle | [20,47] | |
| Ratio of volume to surface area | Ratio of particle volume to particle surface area | [48] | ||
| Convexity | Ratio of particle volume over its convex hull volume (VCH) | [49] |
| Particle | n = 4 | n = 8 | n = 12 | n = 15 |
|---|---|---|---|---|
| Sphere |  Rinc = 0 |  Rinc = 0 |  Rinc = 0 |  Rinc = 0 |
| Cube | 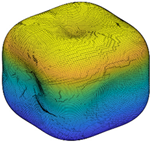 Rinc = 0.2877 | 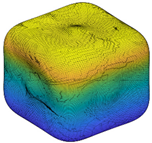 Rinc = 0.1608 |  Rinc = 0.1308 | 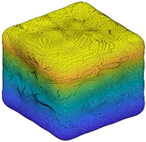 Rinc = 0.0738 |
| Particle 0006 | 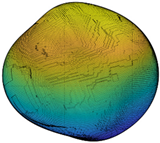 Rinc = 0.2003 |  Rinc = 0.1303 | 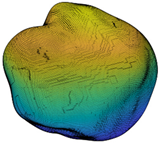 Rinc = 0.1051 | 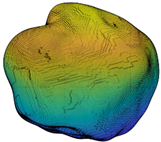 Rinc = 0.0658 |
| Particle 0036 | 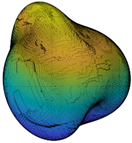 Rinc = 0.2839 | 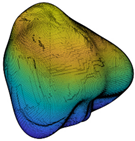 Rinc = 0.1500 | 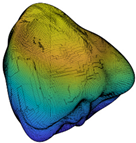 Rinc = 0.1076 |  Rinc = 0.0748 |
| Data Set | Samples | MSE (×10−4) | R |
|---|---|---|---|
| Training | 3323 | 5.0937 | 0.9715 |
| Validation | 416 | 4.6397 | 0.9746 |
| Testing | 416 | 5.2094 | 0.9709 |
| Rinc n = 4 (GF) | Rinc n = 8 (LR) | Rinc n = 12 (1L-ST) | Rinc n = 15 (2L-ST) | ||
|---|---|---|---|---|---|
| Whole Particle | 0.2003 | 0.1303 | 0.1051 | 0.0658 | |
| Local points | P1 | 0.1522 | 0.0878 | 0.1425 | 0.1251 |
| P2 | 0.1683 | 0.1852 | 0.1444 | 0.1093 | |
| P3 | 0.3550 | 0 | 0.0526 | 0.1033 | |
| Local surfaces | S1 | 0.2051 | 0.1163 | 0.0964 | 0.0806 |
| S2 | 0.1803 | 0.1079 | 0.1063 | 0.0692 | |
| S3 | 0.1713 | 0.1203 | 0.1580 | 0.0726 | |
| S4 | 0.1786 | 0.1235 | 0.0725 | 0.0625 | |
| S5 | 0.2988 | 0.1336 | 0.0821 | 0.0675 | |
| S6 | 0.1954 | 0.1171 | 0.0945 | 0.0583 | |
| S7 | 0.1427 | 0.1683 | 0.1451 | 0.0686 | |
| S8 | 0.2416 | 0.1567 | 0.0838 | 0.0477 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, W.; Wang, J.; Cheng, Z. A Novel Multi-Scale Particle Morphology Descriptor with the Application of SPHERICAL Harmonics. Materials 2020, 13, 3286. https://doi.org/10.3390/ma13153286
Xiong W, Wang J, Cheng Z. A Novel Multi-Scale Particle Morphology Descriptor with the Application of SPHERICAL Harmonics. Materials. 2020; 13(15):3286. https://doi.org/10.3390/ma13153286
Chicago/Turabian StyleXiong, Wei, Jianfeng Wang, and Zhuang Cheng. 2020. "A Novel Multi-Scale Particle Morphology Descriptor with the Application of SPHERICAL Harmonics" Materials 13, no. 15: 3286. https://doi.org/10.3390/ma13153286
APA StyleXiong, W., Wang, J., & Cheng, Z. (2020). A Novel Multi-Scale Particle Morphology Descriptor with the Application of SPHERICAL Harmonics. Materials, 13(15), 3286. https://doi.org/10.3390/ma13153286







