Next Article in Journal
Investigation of the Condition of the Gold Electrodes Surface in a Plasma Reactor
Next Article in Special Issue
Comparing Fly Ash Samples from Different Types of Incinerators for Their Potential as Storage Materials for Thermochemical Energy and CO2
Previous Article in Journal
Hydrogen/Deuterium Dynamics in Hydroxyl Salts Co2(OH)3Br/Co2(OD)3Br Revealed by Muon Spin Relaxation
Previous Article in Special Issue
LiFePO4-Graphene Composites as High-Performance Cathodes for Lithium-Ion Batteries: The Impact of Size and Morphology of Graphene
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Materials
Volume 12
Issue 13
10.3390/ma12132136
materials-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Lithium Attachment to C60 and Nitrogen- and Boron-Doped C60: A Mechanistic Study

by
Yingqian Chen
1,
Chae-Ryong Cho
2 and
Sergei Manzhos
3,*
1
Department of Mechanical Engineering, National University of Singapore, Singapore 117576, Singapore
2
Department of Nanoenergy Engineering, Pusan National University, Busan 46241, Korea
3
Centre Énergie Matériaux Télécommunications, Institut National de la Recherche Scientifique, 1650 boulevard Lionel-Boulet, Varennes, QC J3X1S2, Canada
*
Author to whom correspondence should be addressed.
Materials 2019, 12(13), 2136; https://doi.org/10.3390/ma12132136
Submission received: 14 June 2019 / Revised: 28 June 2019 / Accepted: 30 June 2019 / Published: 3 July 2019
(This article belongs to the Special Issue Sustainable Energy Storage Materials)
Browse Figures
Versions Notes

Abstract

:
Fullerene-based materials including C60 and doped C60 have previously been proposed as anodes for lithium ion batteries. It was also shown earlier that n- and p-doping of small molecules can substantially increase voltages and specific capacities. Here, we study ab initio the attachment of multiple lithium atoms to C60, nitrogen-doped C60 (n-type), and boron doped C60 (p-type). We relate the observed attachment energies (which determine the voltage) to changes in the electronic structure induced by Li attachment and by doping. We compare results with a GGA (generalized gradient approximation) functional and a hybrid functional and show that while they agree semi-quantitatively with respect to the expected voltages, there are qualitative differences in the electronic structure. We show that, contrary to small molecules, single atom n- and p-doping will not lead to practically useful modulation of the voltage–capacity curve beyond the initial stages of lithiation.

1. Introduction

Fullerene-based materials have been explored for use as active electrode materials for Li and Na ion batteries in several works [1,2,3,4,5]. Cyclic voltammetry of C60 in solution identified well-resolved peaks at −1.07, −1.43, and −1.92 V vs. Fc/Fc+ in [6] (about 2.4, 2.1, and 1.6 V, respectively, vs. Li/Li+). In [7], reduction peaks for thin film C60 were reported around −0.5, −1.0, −1.7, and −2.2 V vs. Fc/Fc+. In [8], an expanded measurement window was used and reduction potentials (E1/2 values) relative to Fc/Fc+ were measured in solution at −0.98, −1.37, −1.87, −2.35, −2.85, and -3.26 V (about 2.4, 2.1, 1.6, 1.1, 0.6, and 0.2 V, respectively, vs. Li/Li+). When C60 is used as an active electrode material, voltammograms recorded during lithiation show a large sloping plateau in the 0.7 V area and short plateaus around 1.5, 1.9 and 2.3 V vs. Li/Li+ [2]. Experiments indicate a maximum specific capacity reaching 400 mAh/g, corresponding to insertion of 10 to 12 Li atoms per molecule [2]. The broad plateau in the 0.7 V area extends from Li3C60 to Li10C60 (corresponding to a specific capacity of 372 mAh/g) and Li12C60 is reached at about 0.1 V [2]. Formation of Li10C60 corresponds to C60 accepting 10 valence electrons from the Li atoms into its lowest unoccupied molecular orbitals. In this configuration, similar to other known complexes like K10C60 [9], all 10 attached Li should be fully ionized.
The voltages observed with C60 imply its possible use as an anode. In the anode application, one desires either a voltage close to 0 vs. Li/Li+ (while remaining sufficiently positive to avoid plating) or a voltage just above about 1.3 V which would avoid reduction of common carbonate based electrolytes and thus enable high-rate operation unhampered by the SEI (solid-electrolyte interphase) and plating/dendrites [10,11,12]. The main plateau of a C60 electrode at around 0.7 V does not ideally achieve either of these purposes for a Li ion battery. It is possible to modulate the voltage–capacity curve by chemical modification including functionalization and doping. Functionalized and doped C60 based materials have been explored in experimental and modelling works for use in Li ion batteries. Examples are Ag-doped/functionalized fullerenol [13] or substitutional nitrogen-doped C59N and functionalized C59N [14].
Doping of the active electrode material is a powerful technique to modulate the interaction energy of a semiconductor host with the active cation [15]. In inorganic materials such as monoelemental semiconductors, the initial stage of lithiation, sodiation, magnesiation etc. involves donation of the valence electron(s) to the conduction band of the host [16,17,18,19,20,21,22,23,24,25]; in oxides, the valence electron can occupy a state in the conduction band [26,27,28,29,30,31]. These are relatively high-energy states; p-doping can be used to create unoccupied states near the top of the valence band which can be occupied by the valence electron of the alkali atom and thereby strengthen the binding via the bandstructure part of the total energy, which can either increase the voltage or induce electrochemical activity in materials which are inactive in undoped state [15,16]. n-Doping, on the other hand, is not expected to improve the voltage in inorganic solids [15]. This is ultimately related to small reorganization energies of such materials. Many organic electrode materials [32] are semiconductors, and the strategy of p-doping is fully applicable to them; in [33], we computed that p-doping can lead to strengthening of the binding energy on the order of 2 eV (corresponding to a voltage increase on the order of 2 V) when using typical organic small molecule building blocks. With organic molecules, in contrast to inorganic solid semiconductors, n-doping was also shown to lead to stronger binding. This was related in [33] to strain effects. Here, we will show that in molecules, stronger binding (higher voltages) induced by n-doping can also be understood from the bandstructure perspective (in the following, we will liberally use “bandstructure” as set language even when talking about molecules). Substitutions not amounting to doping (where the number of valence electrons does not change) can also be used to strengthen interaction with the active cation; for example, we showed that replacing a -CH- group with N in disodium terephthalate [34,35] to result in disodium pyridine dicarboxylate leads to increased voltage for first Na attachment per formula unit, in agreement with experiment [36,37]. This was related to a bond formation between Na and N.
In small-molecule systems, the theoretical capacity is typically reached with a small number of Li or Na attached per formula unit. Specifically in materials operating by the insertion and reduction mechanism, the specific capacity is typically reached with two Li or Na per molecule, corresponding to full occupancy of the molecular LUMO (lowest unoccupied molecular orbital), for example in dicarboxylates and tetracyanides [10,36,38]. In this case, an increase of the voltage for first Li, Na attachment is practically relevant, as it significantly modifies the voltage–capacity curve, at least up to a half of the theoretical capacity. For example, a voltage–capacity curve with two plateaus was obtained in [36] which decreased segregation into the fully sodiated state and improved cycle rate and life. In inorganic hosts, to induce practically relevant improvement in the voltage–capacity curve, a high dopant concentration is needed, to the tune of several at%, which is experimentally feasible for some materials [17,39]. One may therefore question whether a single dopant can substantially change the voltage–capacity curve of C60 [14] considering that the final state of charge involves at least 10 Li atoms per molecule.
Density functional theory (DFT) models are able to describe experimental voltage–capacity curves with semi-quantitative accuracy [40]. Good match with experiment can be achieved when the voltage–capacity curve is due to the active material itself rather than morphological effects, impurities, etc. Typically, this requires periodic solid-state calculations, which limits the range of practically applicable approximations. Specifically, it is more difficult and CPU-costly to use hybrid functionals, and GGA (generalized gradient approximation) functionals remain the most widely used in this application. This, even though the charge transfer nature of the Li–host material interactions makes desirable the inclusion of exact exchange. Molecular or oligomeric models can be used for organic materials [32]. For materials operating by oxidation, molecular/oligomeric models are sometimes able to predict the voltage capacity curve [41]. For materials operating by insertion/reduction, we observed in a series of studies that the qualitative features of the measured voltage–capacity curve, including its shape and any effects of doping are well reproduced in a molecular model, while the absolute magnitude of the voltage is underestimated on the order of 1 V due to the neglect of aggregate state effects [10,34,35,36,37,38,42]. Another disadvantage of a molecular model is artificial persistence of the voltage–capacity curve beyond the theoretical capacity [34,37,42]; in the present work, we are, however, able to circumvent this issue (see Section 2). A significant advantage of a molecular model is ease of application of a hybrid functional (and of wavefunction based methods, as needed) and of comparison between different computational approaches [41,42,43,44]. This is also the route taken in this work. A recent DFT based work on N-doped C60 that suggested voltage increase by N doping also used a molecular model with a hybrid functional [14].
In this work, we perform an ab initio study of Li attachment to C60, N-doped C60, and B-doped C60, the last two chosen as examples of substitutional n- and p-doping, respectively. We study whether doping can be used to modulate in a practically significant way the voltage–capacity curve of C60. We focus on the mechanistic understanding of Lin–C60 interaction, derived from the electronic structure; we therefore work with a molecular model which is expected to underestimate the magnitude of the voltage but preserve the shape of the voltage–capacity curve [36,37] and correctly predict the effects due to doping [15,32]. This model allows us to compare the results obtained with a GGA functional and a hybrid functional and thereby assess whether a GGA functional—still most practical for solid state modelling—correctly describes properties of this system such as bandstructure changes during Li attachment and the voltage–capacity curve. In contrast to the model of [14] which computed the reduction potential and therefore provided a preview into the effects of doping and functionalization on the open-circuit voltage, i.e., the initial part of the voltage–capacity curve (which, from what is known about its shape for C60 [2], is not much practically relevant), we consider the effects of doping on the entire curve and show that, contrary to small molecules, single atom n- and p-doping will not lead to practically relevant modulation of the voltage–capacity curve beyond the initial stages of lithiation.

2. Methods

Density functional theory calculations were performed in Gaussian 09 [45] using the B3LYP [46] and PBE (Perdew–Burke–Ernzerhof) [47] exchange correlation functionals. As mentioned in Section 1, a comparison between the two is useful, as GGA functionals, and specifically PBE, continue to be most widely used in solid state modeling, including modeling of battery electrode materials [40]. PBE is an ab initio functional that expresses the exchange-correlation energy as a functional of the electron density and its gradient. While practical and widely used, it famously underestimates the bandgap and suffers from delocalization errors. B3LYP is a hybrid functional widely used in molecular simulations which includes an empirically determined fraction of exact exchange as well as empirical weights for other components of the energy. It much more accurately reproduces bandstructures and electronic state localization but is also much more CPU costly, especially in solid state calculations. That is why it is important to compare these two types of functionals for a system where both are feasible, as is done here in a molecular model, to assess if PBE is apt to reproduce voltages and mechanism of Li attachment.
The 6–31+g(d,p) basis set was used. Spin polarization was used for systems with odd numbers of electrons. Tight convergence criteria were used for structure optimization. Initial C59N, C59B structures were obtained by replacing one C atom with an N or B atom, respectively. Bader charges were computed with the Bader analysis program [48]. Partial densities of states (PDOS) were produced with the GaussSum program [49], and visualizations with VESTA [50]. Bond formation was identified by using charge density differences, Δ ρ = ρ L i − s y s − ( ρ L i + ρ s y s ) , where sys is one of C60/C59N/C59B, and electron densities ρ for Li and sys are computed at the geometries of Li–sys. Formation energies Ef of complexes Lin–C60/C59N/C59B were computed as:
E f = E n L i − s y s − n E L i b c c − E s y s ,
where E n L i − s y s is the energy of the complex Lin–C60/C59N/C59B and E L i b c c is an estimate of the energy of one Li atom in a bcc (body-centered cubic) structure, which is computed as E L i b c c = Eatom – Ecoh, where Eatom is the energy of a Li atom computed in Gaussian 09 and Ecoh is the cohesive energy of bcc Li taken as 1.63 eV/atom [51,52]. Voltage–capacity curves were computed from piece-wise voltages V between Li fractions n1 and n2 as [40]:
V = − E n 2 L i − s y s − E n 1 L i − s y s − ( n 2 − n 1 ) E L i b c c n 2 − n 1 ,
where n1 and n2 correspond to inflection points on the convex hull built from the dependence Ef(n). Multiple Li configurations (attachment sites) were tried, and the lowest formation energies were used to build the convex hull and to compute V.
As discussed in Section 1, the absence of aggregate state effects in a molecular model not only causes a shift of the voltage–capacity curve as a whole but also makes it less obvious at what state of charge the maximum capacity would be reached. We used the extent of Li ionization—more specifically an abrupt change of its degree—as an indicator of the maximum capacity expected in an experiment. Indeed, we noted in our previous comparative studies of molecular and solid materials that while in molecular calculations the capacity persists beyond that computed in solid state (and that observed experimentally), those states of charge correspond to a markedly lower degree of Li ionization and to occupancy of Li-centred states [10,38,42].

3. Results

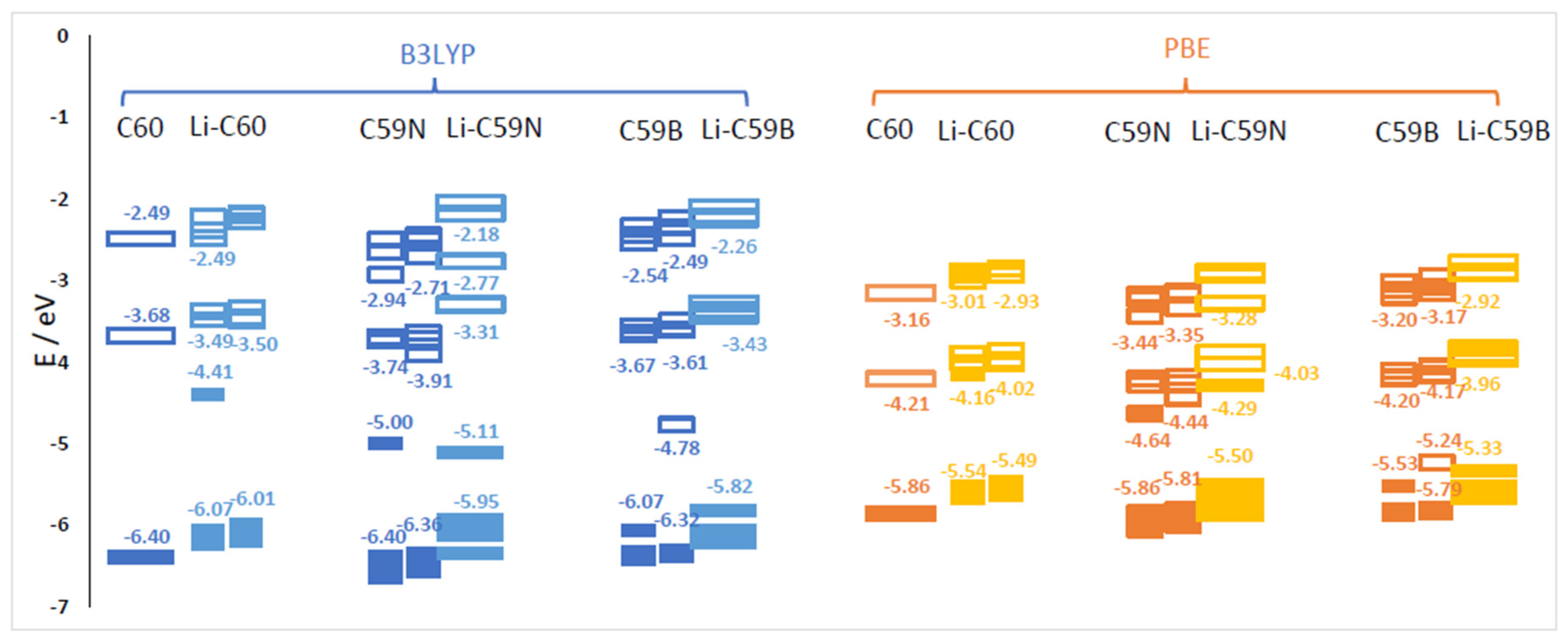
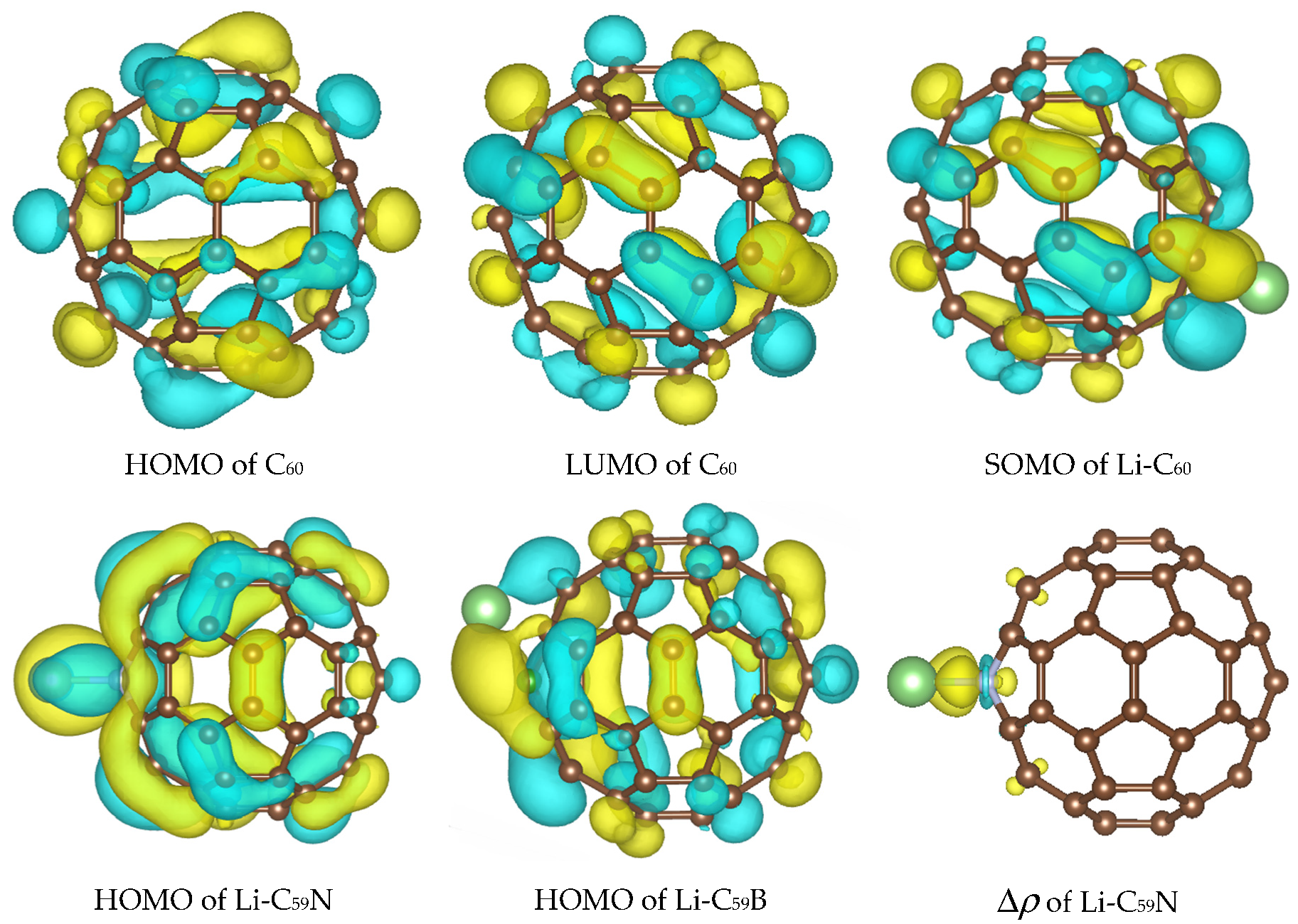
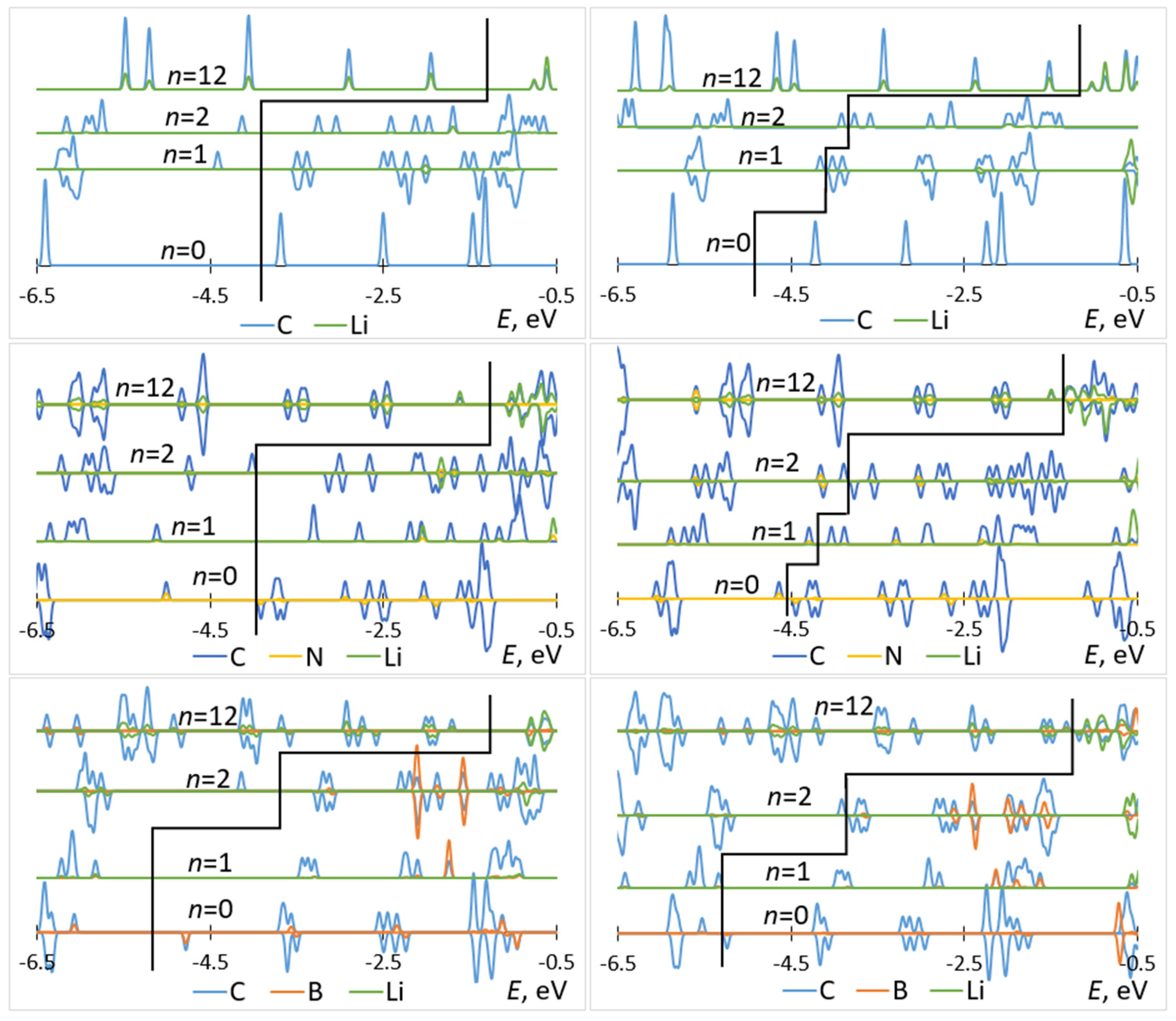
3.1. Bandstructure of Li–C60/C59N/C59B Complexes

Energies of relevant molecular orbitals of C60, C59N, C59B and their complexes with Li are shown in Figure 1. We noted that the orbital corresponding to the valence electron of a free Li atom is E(Li) = −3.65 eV with B3LYP and –3.22 with PBE, i.e., higher than the LUMO of C60 by 0.03 eV with B3LYP and by 0.99 eV with PBE. For spin-polarized systems, both spin channels are shown. Li donates its valence electron to the LUMO of C60 (see Figure 2). While PBE results in an artificially contracted HOMO (highest occupied molecule orbital)–LUMO gap, this phenomenon of Li ionization is qualitatively similar with both functionals. Upon charge donation, the resulting SOMO (single occupied molecular orbital) is stabilized vs. the original LUMO by 0.73 eV with B3LYP but is destabilized by 0.05 eV with PBE; as a result the band energy of this electron is different by only 0.25 eV between the functionals, while with both functionals, the C60’s HOMO is destabilized by a similar amount (about 0.35 eV). The stabilization of the band energy of Li valence electron upon donation also differs by only 0.2 eV between the functionals (and is stronger with PBE). This helps explain the relatively small (given major quantitative and qualitative differences in bandstructure) difference in voltages computed with the two functionals (on the order of 0.2 V, vide infra).
The effect of the substitutional N dopant is to introduce an occupied state (SOMO) in the gap in one spin channel. The SUMO (single unoccupied molecular orbital) of the other spin channel is occupied by the Li valence electron upon Li attachment and stabilized by 1.2 eV, from –3.91 eV to –5.11 eV (forming the HOMO of the complex shown in Figure 2) with B3LYP, but is destabilized by 0.15 eV, from −4.44 eV to −4.29 eV, with PBE. The bandstructure energy of the valence electron of Li is therefore stabilized by 1.46 and 1.07 eV upon attachment to C60 with B3LYP and PBE, respectively. The destabilization of the molecular HOMO (HOMO-1 of the complex) is of similar magnitude with both functionals. The bandstructure argument implies a stronger effect of the doping on the open-circuit voltage with B3LYP; this is indeed confirmed by the computed voltage–capacity curve below, although to a smaller degree than suggested by the bandstructure. However, in this case the bandstructure argument is less directly applicable than in the case of pristine C60; the bond Li–C59N is less ionic, and we could observe covalent bond formation between Li and N, as shown in Figure 2. This is similar to Na–N covalent bond formation previously observed in disodium pyridine dicarboxylate [36,37]. A critical difference between B3LYP and PBE in this case is due to the fact that Li attachment in this model corresponds to going from a state with two unpaired electrons on each component (well-separated Li and C59N) with negligible exchange energy to a singlet state of C59N stabilized by contributions from exact exchange in the case of B3LYP but not PBE. We therefore expected a stronger open-circuit voltage increase with B3LYP than with PBE, which is indeed what we compute below with Equation (2).
In the case of boron doping, substitutional B leads to the appearance of an unoccupied state in the gap and of a SOMO derived from a half-occupied C60 HOMO. Upon Li attachment, this state is strongly stabilized forming a doubly occupied C60 HOMO-like orbital. Valence electron of Li thus occupies a state by 2.17 eV lower vs. isolated atom with B3LYP and by 2.11 eV lower with PBE. The effects of B doping on the open-circuit voltage are expected to be strong and of similar magnitude with both functionals, by the valence bandstructure argument. We indeed find below that they were strong and similar albeit smaller than 2 V due to electron correlation and other effects. The different effect of the hybrid vs. GGA functional here is explained by the fact that with n-doping by nitrogen, the energy of the orbital occupied by the Li valence electron is in the gap and much above the C60 HOMO and could be stabilized by exact exchange contributions, while with p-doping by boron, it is near C60 HOMO with a relatively high density of states (HOMO to HOMO-4 of C60 have similar energies shown as one merged bar in Figure 1) and likely cannot be stabilized further than the top of the valence band due to electron correlation effects.

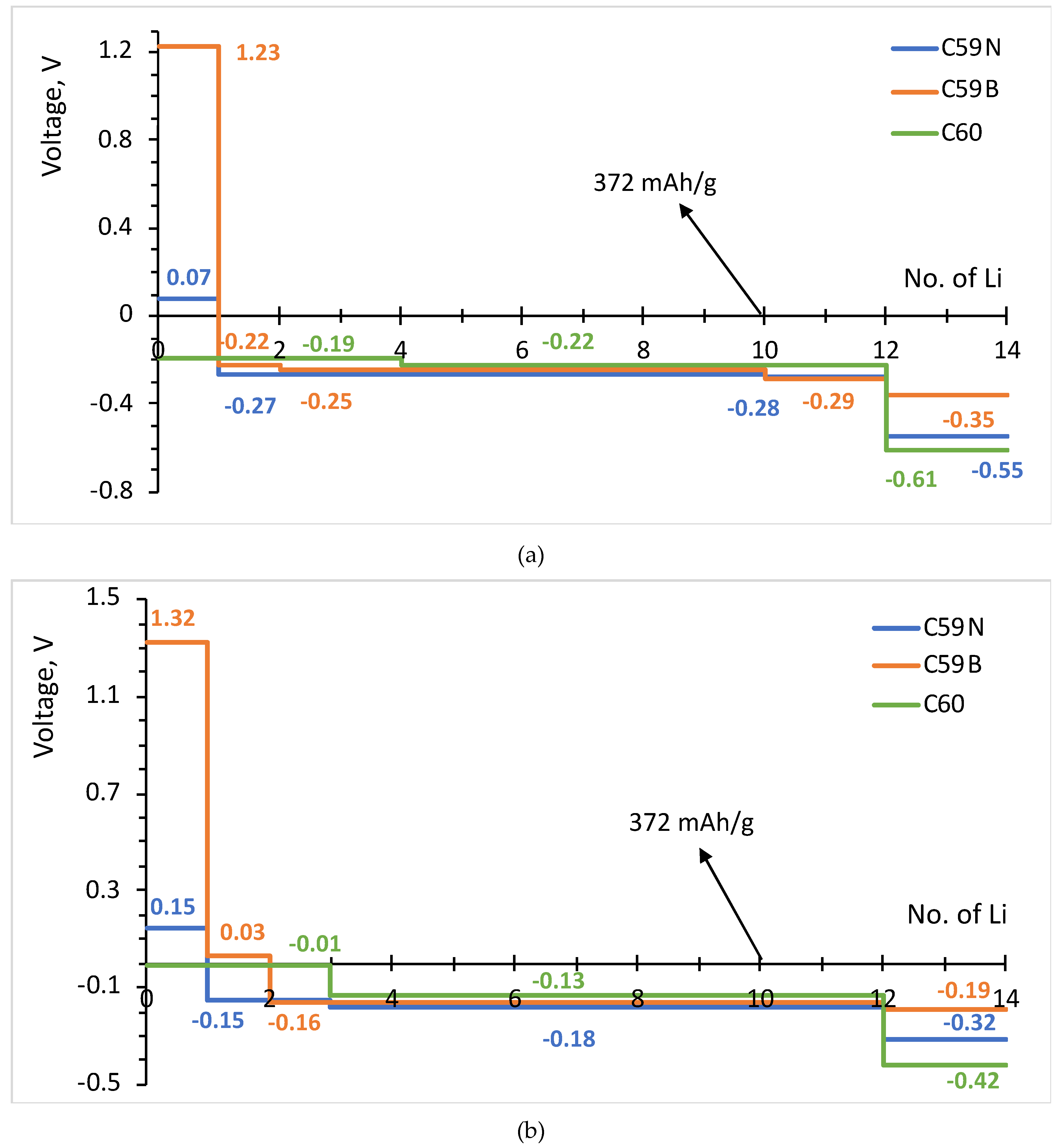
3.2. Formation Energies and Voltage–Capacity Curves

The formation energies of the lowest energy Lin–C60/C59N/C59B complexes are shown in Figure 3. The corresponding voltage–capacity curves are shown in Figure 4. The curves are down-shifted vs. experiment [2] on the order of 1 V due to the neglect of effects due to molecular packing in a solid [10,36,37,38,42]. Of course, any measured voltage plateaus and CV peaks may also be sloped and smeared due to the effect of other cell components, such as resistance. We focus on the comparison of doped and undoped cases. For undoped C60, B3LYP predicts an almost flat curve (plateaus at −0.19 V up to Li4C60 and −0.22 V thereafter) at around −0.2 V up to Li12C60. PBE predicts a plateau near 0 up to Li3C60 followed by a plateau at −0.13 V up to Li12C60, suggesting a theoretical capacity of 446 mAh/g. The two functionals, in spite of resulting in significantly different electronic structures, end up predicting voltage–capacity curves differing only on the order of 0.1 V. This is rationalized in the proceeding section.
The final state of charge with n = 12 is in decent agreement with the experiments of [2]. The computed curve for C60 shows a drastic drop after that point which corresponds to a sudden change in the degree of ionization of Li. We use the degree of ionization to call the final state of charge expected in an experiment. Table 1 lists Bader charges on Li in Lin–C60/C59N/C59B. For attachment up to a dozen Li per C60, all Li atoms are practically fully ionized, with Bader charges on the order of 0.9 |e| for all Li. Beyond n = 12, some Li atoms show a markedly lower degree of ionization, this point correspond to a down-step in the voltage–capacity curve in Figure 4. This behavior is similar with B3LYP and PBE. This is therefore the computed final state of charge. With N doping, the drop in the degree of ionization starts a little earlier and with B doping a little later, as is expected with an electron rich n-doped system and an electron-deficient p-doped system, respectively. Equation (2) predicts a similar theoretical capacity for C59N as for C60 but a slightly larger capacity for C59B (Figure 4).
The attachment of multiple Li atoms to C60/C59N/C59B corresponds to occupation of a number of unoccupied molecular states equal to the number of Li atoms, as can be seen from the PDOS plots in Figure 5. While for attachment of first Li atoms, there are no significant contributions from Li to the occupied states, for attachment of multiple Li atoms, such contributions are notable, indicating a significant degree of hybridization. Most importantly, any effect of doping is limited to the initial stage of lithiation and had negligible effect on the rest of the voltage–capacity curve responsible for most of the useful specific capacity. There is a strong increase of the voltage for first Li attachment, on the order of 1.5 V for boron doping, with both functionals, as expected from the bandstructure. A smaller increase, on the order of 0.3 V with both functionals, is computed for nitrogen doping. There is a minor effect for the second attached lithium, and no significant effect on the bulk of the plateau extending to n = 12.

4. Conclusions

We performed a DFT analysis of storage of multiple Li atoms at C60 and n- and p-doped C60 (C59N and C59B, respectively). We used a molecular model which allowed us to compare the mechanism of Li storage, i.e., electronic structure changes induced by lithiation, between a hybrid (B3LYP) and a GGA (GGA) functional and among C60, C59N, and C59B. While the measured voltage–capacity curve for undoped C60 is available in the literature [2], we predict the overall shape of the voltage capacity curve expected in an experiment with C59N and C59B. We predict that a moderate increase in the voltage with C59N and a strong (>1 V) increase with C59B vs. undoped C60 will be observed for the initial part of the voltage–capacity curve only. We predict that no significant changes will be observed for the bulk of the voltage–capacity curve responsible for most of the reversible capacity. We predict a slight increase of theoretical capacity (on the order of 15%) with B doping. That is, contrary to small molecules, single atom n- and p-doping of C60 will not lead to practically useful modulation of the voltage–capacity curve.
We observed differences in the electronic structure changes due to Li attachment between B3LYP and PBE functionals, and these changes were also different between n- and p-doping. Specifically, the exact exchange contribution much stabilizes the gap state formed by Li s electron donation to the SUMO of C59N, while with PBE this state is slightly destabilized, leading to a stronger bump to the open-circuit voltage with B3LYP. In contrast, with p-doping, the orbital at the top of the valence band occupied by the Li electron does not benefit from the extra stabilization, leading to a similar in magnitude bump to the open-circuit voltage with both functionals.
B3LYP and PBE functionals, in spite of resulting in significantly different electronic structures, end up predicting voltage–capacity curves differing only on the order of 0.1 V. This was rationalized based on bandstructure changes induced by lithiation. On one hand, this result is good news for solid-state modeling where PBE functionals remain the most practical solution, even though the charge transfer nature of the Li–host material interactions makes desirable the inclusion of exact exchange; on the other hand, our results also show that this apparent similarity in voltages hides significant (quantitative and qualitative) differences in electronic structure changes induced by Li attachment. Important mechanistic details could therefore be missed even with a quantitatively accurate voltage curve.
The presence of the dopant atoms is expected to significantly increase the voltage only up to states of charge where the number of attached Li is just sufficient to fill the empty states created by the dopants [1]. We therefore expect that even with fullerenes with a higher heteroatom content such as C57N3 [53] only a small part of the voltage–capacity curve will be modulated. The present model considered that C60 and doped C60 molecules would largely preserve their structure and electronic properties in solid state, which is true for vdW (van der Waals) crystals of molecular fullerenes [54,55]. In the future, however, electrochemically induced dimerization of C60 and dimerization of C59N, which are known to occur, should also be explored [56,57].

Author Contributions

Conceptualization, S.M. and C.-R.C.; Calculations and Data Analysis, Y.C.; Writing, Review, and Editing, S.M.

Funding

This research received no external funding.

Acknowledgments

We thank Il Jeon of the University of Tokyo for discussions. We thank Compute Canada and National University of Singapore for access to computational resources.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Kawabe, S.; Kawai, T.; Sugimoto, R.; Yagasaki, E.; Yoshino, K. Electrochemical properties of fullerene derivative polymers as electrode materials. Jpn. J. Appl. Phys. 1997, 36, L1055–L1058. [Google Scholar] [CrossRef]
  2. Chabre, Y.; Djurado, D.; Armand, M.; Romanow, W.R.; Coustel, N.; McCauley, J.P., Jr.; Fischer, J.E.; Smith, A.B., III. Electrochemical intercalation of lithium into solid C60. J. Am. Chem. Soc. 1992, 114, 764–766. [Google Scholar] [CrossRef]
  3. Lemont, S.; Ghanbaja, J.; Billaud, D. Electrochemical intercalation of sodium ions into fullerene. Mater. Res. Bull. 1994, 29, 465–472. [Google Scholar] [CrossRef]
  4. Yildirim, T.; Zhou, O.; Fischer, J.E.; Bykovetz, N.; Strongin, R.A.; Cichy, M.A.; Smith III, A.B.; Lin, C.L.; Jelinek, R. Intercalation of sodium heteroclusters into the C60 lattice. Nature 1992, 360, 568–571. [Google Scholar] [CrossRef]
  5. Scaravonati, S.; Magnani, G.; Gaboardi, M.; Allodi, G.; Ricco, M.; Pontiroli, D. Electrochemical intercalation of fullerene and hydrofullerene with sodium. Carbon 2018, 130, 11–18. [Google Scholar] [CrossRef]
  6. Jehoulet, C.; Bard, A.J.; Wudl, F. Electrochemical reduction and oxidation of C60 films. J. Am. Chem. Soc. 1991, 113, 5456–5457. [Google Scholar] [CrossRef]
  7. Jehoulet, C.; Obeng, Y.S.; Kim, Y.T.; Zhou, F.; Bard, A.J. Electrochemistry and Langmuir trough studies of fullerene C60 and C70 films. J. Am. Chem. Soc. 1992, 114, 4237–4247. [Google Scholar] [CrossRef]
  8. Xie, Q.; Perez-Cordero, E.; Echegoyen, L. Electrochemical detection of C606- and C706-: Enhanced stability of fullerides in solution. J. Am. Chem. Soc. 1992, 114, 3978–3980. [Google Scholar] [CrossRef]
  9. Tycko, R.; Dabbagh, G.; Rosseinsky, M.J.; Murphy, D.W.; Fleming, R.M.; Ramirez, A.P.; Tully, J.C. 13C NMR spectroscopy of KxC60: Phase separation, molecular dynamics, and metallic properties. Science 1991, 253, 884–886. [Google Scholar] [CrossRef]
  10. Chen, Y.; Manzhos, S. Lithium and sodium storage on tetracyanoethylene (TCNE) and TCNE-(doped)-graphene complexes: a computational study. Mater. Chem. Phys. 2015, 156, 180–187. [Google Scholar] [CrossRef]
  11. Madian, M.; Eychmüller, A.; Giebeler, L. Current advances in TiO2-based nanostructure electrodes for high performance lithium ion batteries. Batteries 2018, 4, 7. [Google Scholar] [CrossRef]
  12. Saravanan, K.; Ananthanarayanan, K.; Balaya, P. Mesoporous TiO2 with high packing density for superior lithium storage. Energy Environ. Sci. 2010, 3, 939–948. [Google Scholar] [CrossRef]
  13. Wang, F.F.; Wang, C.; Liu, R.Q.; Tian, D.; Li, N. Experimental study on the preparation of Ag nanoparticle doped fullerenol for lithium ion battery application. J. Phys. Chem. C 2012, 116, 10461–10467. [Google Scholar] [CrossRef]
  14. Sood, P.; Kim, K.C.; Jang, S.S. Electrochemical and electronic properties of nitrogen doped fullerene and its derivatives for lithium-ion battery applications. J. Energy Chem. 2018, 27, 528–534. [Google Scholar] [CrossRef]
  15. Lueder, J.; Legrain, F.; Chen, Y.; Manzhos, S. Doping of active electrode materials for electrochemical batteries: an electronic structure perspective. MRS Commun. 2017, 7, 523–540. [Google Scholar] [CrossRef]
  16. Legrain, F.; Manzhos, S. A first-principles comparative study of lithium, sodium, and magnesium storage in pure and gallium-doped germanium: competition between interstitial and substitutional sites. J. Chem. Phys. 2017, 146, 034706. [Google Scholar] [CrossRef] [PubMed]
  17. Legrain, F.; Manzhos, S. Aluminum doping improves the energetics of lithium, sodium, and magnesium storage in silicon: a first-principles study. J. Power Sources 2015, 274, 65–70. [Google Scholar] [CrossRef]
  18. Legrain, F.; Malyi, O.I.; Manzhos, S. Comparative computational study of the energetics of Li, Na, and Mg storage in amorphous and crystalline silicon. Comput. Mater. Sci. 2014, 94, 214–217. [Google Scholar] [CrossRef] [Green Version]
  19. Legrain, F.; Malyi, O.I.; Manzhos, S. Comparative computational study of the diffusion of Li, Na, and Mg in silicon including the effect of vibrations. Solid State Ionics 2013, 253, 157–163. [Google Scholar] [CrossRef]
  20. Legrain, F.; Malyi, O.I.; Tan, T.L.; Manzhos, S. Computational study of Mg insertion into amorphous silicon: advantageous energetics over crystalline silicon for Mg storage. MRS Proc. 2013, 1540, mrss13-1540-e03-06. [Google Scholar] [CrossRef]
  21. Tan, T.L.; Malyi, O.I.; Legrain, F.; Manzhos, S. Role of inter-dopant interactions on the diffusion of Li and Na atoms in bulk Si anodes. MRS Proc. 2013, 1541, mrss13-1541-f06-13. [Google Scholar] [CrossRef]
  22. Malyi, O.I.; Tan, T.L.; Manzhos, S. A computational study of the insertion of Li, Na, and Mg atoms into Si(111) nanosheets. Nano Energy 2013, 2, 1149–1157. [Google Scholar] [CrossRef]
  23. Malyi, O.I.; Tan, T.L.; Manzhos, S. A comparative computational study of structures, diffusion, and dopant interactions between Li and Na insertion into Si. Appl. Phys. Express 2013, 6, 027301. [Google Scholar] [CrossRef]
  24. Malyi, O.I.; Tan, T.L.; Manzhos, S. In search of high performance anode materials for Mg batteries: computational studies of Mg in Ge, Si, and Sn. J. Power Sources 2013, 233, 341–345. [Google Scholar] [CrossRef]
  25. Kulish, V.; Malyi, O.I.; Ng, M.F.; Chen, Z.; Manzhos, S.; Wu, P. Controlling Na diffusion by rational design of Si-based layered architectures. Phys. Chem. Chem. Phys. 2014, 16, 4260–4267. [Google Scholar] [CrossRef] [PubMed]
  26. Kulish, V.; Koch, D.; Manzhos, S. Insertion of mono- vs. bi- vs. trivalent atoms in prospective active electrode materials for electrochemical batteries: an ab initio perspective. Energies 2017, 10, 2061. [Google Scholar] [CrossRef]
  27. Legrain, F.; Malyi, O.I.; Manzhos, S. Insertion energetics of lithium, sodium, and magnesium in crystalline and amorphous titanium dioxide: a comparative first-principles study. J. Power Sources 2015, 278, 197–202. [Google Scholar] [CrossRef]
  28. Koch, D.; Kulish, V.; Manzhos, S. A first-principles study of the potassium insertion in crystalline vanadium oxide phases as possible potassium-ion battery cathode materials. MRS Commun. 2017, 7, 819–825. [Google Scholar] [CrossRef]
  29. Kulish, V.; Manzhos, S. Comparison of Li, Na, Mg and Al-ion insertion in vanadium pentoxides and vanadium dioxides. RSC Adv. 2017, 7, 18643. [Google Scholar] [CrossRef]
  30. Koch, D.; Manzhos, S. A comparative first-principles study of lithium, sodium and magnesium insertion energetics in brookite titanium dioxide. MRS Adv. 2019, 4, 837–842. [Google Scholar] [CrossRef]
  31. Koch, D.; Manzhos, S. First-principles study of the calcium insertion in layered and non-layered phases of vanadia. MRS Adv. 2018, 3, 3507–3512. [Google Scholar] [CrossRef]
  32. Manzhos, S. Organic electrode materials for lithium and post-lithium batteries: an ab initio perspective on design. Curr. Opin. Green Sustain. Chem. 2019, 17, 8–14. [Google Scholar] [CrossRef]
  33. Lueder, J.; Cheow, M.H.; Manzhos, S. Understanding doping strategies in the design of organic electrode materials for Li and Na ion batteries: an electronic structure perspective. Phys. Chem. Chem. Phys. 2017, 19, 13195–13209. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  34. Sk, M.A.; Manzhos, S. Sodium interaction with disodium terephthalate molecule: an ab initio study. MRS Adv. 2016, 1, 3579–3584. [Google Scholar] [CrossRef]
  35. Sk, M.A.; Manzhos, S. Exploring the sodium storage mechanism in disodium terephthalate as anode for organic battery using density-functional theory calculations. J. Power Sources 2016, 324, 572–581. [Google Scholar] [CrossRef]
  36. Padhy, H.; Chen, Y.; Lüder, J.; Gajella, S.R.; Manzhos, S.; Balaya, P. Charge and discharge processes and sodium storage in disodium pyridine-2,5-dicarboxylate anode - insights from experiments and theory. Adv. Energy Mater. 2018, 8, 1701572. [Google Scholar] [CrossRef]
  37. Chen, Y.; Lueder, J.; Manzhos, S. Disodium pyridine dicarboxylate vs disodium terephthalate as anode materials for organic Na ion batteries: effect of molecular structure on voltage from the molecular modeling perspective. MRS Adv. 2017, 2, 3231–3235. [Google Scholar] [CrossRef]
  38. Chen, Y.; Manzhos, S. Comparative computational study of lithium and sodium insertion in van der Waals and covalent tetracyanoethylene (TCNE) -based crystals as promising materials for organic lithium and sodium ion batteries. Phys. Chem. Chem. Phys. 2016, 18, 8874–8880. [Google Scholar] [CrossRef] [PubMed]
  39. Moutanabbir, O.; Isheim, D.; Blumtritt, H.; Senz, S.; Pippel, E.; Seidman, D.N. Colossal injection of catalyst atoms into silicon nanowires. Nature 2013, 496, 78–82. [Google Scholar] [CrossRef]
  40. Urban, A.; Seo, D.H.; Ceder, G. Computational understanding of Li-ion batteries. npj Comput. Mater. 2016, 2, 16002. [Google Scholar] [CrossRef]
  41. Chen, Y.; Manzhos, S. Voltage and capacity control of polyaniline based organic cathodes: An ab initio study. J. Power Sources 2016, 336, 126–131. [Google Scholar] [CrossRef]
  42. Chen, Y.; Manzhos, S. A computational study of lithium interaction with tetracyanoethylene (TCNE) and tetracyaniquinodimethane (TCNQ) molecules. Phys. Chem. Chem. Phys. 2016, 18, 1470–1477. [Google Scholar] [CrossRef] [PubMed]
  43. Sk, M.A.; Chen, Y.; Manzhos, S. Orbital order switching in molecular calculations using GGA functionals: qualitative errors in materials modeling for electrochemical power sources and how to fix them. Chem. Phys. Lett. 2016, 659, 270–276. [Google Scholar] [CrossRef]
  44. Chen, Y.; Lueder, J.; Ng, M.F.; Sullivan, M.; Manzhos, S. Polyaniline and CN-functionalized polyaniline as organic cathodes for lithium and sodium ion batteries: a combined molecular dynamics and Density Functional Tight Binding Study in solid state. Phys. Chem. Chem. Phys. 2018, 20, 232–237. [Google Scholar] [CrossRef] [PubMed]
  45. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 09, Revision D.3; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
  46. Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
  47. Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
  48. Sanville, E.; Kenny, S.D.; Smith, R.; Henkelman, G. An improved grid-based algorithm for Bader charge allocation. J. Comp. Chem. 2007, 28, 899–908. [Google Scholar] [CrossRef]
  49. O’Boyle, N.M.; Tenderholt, A.L.; Langner, K.M. CCLIB: a library for package-independent computational chemistry algorithms. J. Comp. Chem. 2008, 29, 839–845. [Google Scholar] [CrossRef]
  50. Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
  51. Kittel, C. Introduction to Solid State Physics, 8th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
  52. Kaxiras, E. Atomic and Electronic Structure of Solids; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
  53. Otero, G.; Biddau, G.; Sanchez-Sanchez, C.; Caillard, R.; Lopez, M.F.; Rogero, C.; Palomares, F.J.; Cabello, N.; Basanta, M.A.; Ortega, J.; et al. Fullerenes from aromatic precursors by surface-catalysed cyclodehydrogenation. Nature 2018, 454, 865–869. [Google Scholar] [CrossRef]
  54. Arabnejad, S.; Pal, A.; Yamashita, K.; Manzhos, S. Effect of nuclear motion on charge transport in fullerenes: a combined Density Functional Tight Binding – Density Functional Theory investigation. Front. Energy Res. 2019, 7, 3. [Google Scholar] [CrossRef]
  55. Pal, A.; Lai, K.W.; Chia, Y.J.; Jeon, I.; Matsuo, Y.; Manzhos, S. Comparative Density Functional Theory – Density Functional Tight Binding Study of fullerene derivatives: effects due to fullerene size, addends, and crystallinity on band structure, charge transport and optical properties. Phys. Chem. Chem. Phys. 2017, 19, 28330–28343. [Google Scholar] [CrossRef] [PubMed]
  56. Konarev, D.V.; Lyubovskaya, R.N.; Khasanov, S.S.; Otsuka, A.; Saito, G. Formation and properties of (C60−)2 dimers of fullerenes bonded by one and two σ-bonds in ionic complexes. Mol. Cryst. Liq. Cryst. 2007, 468, 227–579. [Google Scholar] [CrossRef]
  57. Hummelen, J.C.; Knight, B.; Pavlovich, J.; González, R.; Wudl, F. Isolation of the heterofullerene C59N as its dimer (C59N)2. Science 1995, 269, 1554–1556. [Google Scholar] [CrossRef] [PubMed]
Materials 12 02136 g001 550
Figure 1. Energies of selected molecular orbitals (MOs) of C60, C59N, C59B and corresponding molecules with one Li attachment computed with B3LYP and PBE functionals. The filled symbols denote occupied MOs and the empty ones unoccupied MOs. For open-shell cases, both alpha (left) and beta (right) spin channel MOs are shown.
Figure 1. Energies of selected molecular orbitals (MOs) of C60, C59N, C59B and corresponding molecules with one Li attachment computed with B3LYP and PBE functionals. The filled symbols denote occupied MOs and the empty ones unoccupied MOs. For open-shell cases, both alpha (left) and beta (right) spin channel MOs are shown.
Materials 12 02136 g001
Materials 12 02136 g002 550
Figure 2. Highest occupied molecule orbital (HOMO), lowest unoccupied molecular orbital (LUMO) of C60 and the orbital occupied by the Li valence electron in Li–C60, Li–C59N, and Li–C59B, as well as charge density difference map Δρ showing bond formation between Li and N dopant. Results of B3LYP calculations are shown, those with PBE are visually similar. Isosurface values are 0.02 e1/2/Å3 for orbitals and 0.005 e1/2/Å3 for the charge density difference map. The atomic color scheme is C—brown, N—grey, B—green, Li—light green.
Figure 2. Highest occupied molecule orbital (HOMO), lowest unoccupied molecular orbital (LUMO) of C60 and the orbital occupied by the Li valence electron in Li–C60, Li–C59N, and Li–C59B, as well as charge density difference map Δρ showing bond formation between Li and N dopant. Results of B3LYP calculations are shown, those with PBE are visually similar. Isosurface values are 0.02 e1/2/Å3 for orbitals and 0.005 e1/2/Å3 for the charge density difference map. The atomic color scheme is C—brown, N—grey, B—green, Li—light green.
Materials 12 02136 g002
Materials 12 02136 g003 550
Figure 3. Formation energies (Ef) vs. the number of Li atoms attached to the C60, C59N, and C59B molecules computed with B3LYP (top panels) and PBE (bottom panels) functionals. The most stable Ef for each concentration are plotted. The line connects the stable phases (filled points) during Li attachment, forming a convex hull.
Figure 3. Formation energies (Ef) vs. the number of Li atoms attached to the C60, C59N, and C59B molecules computed with B3LYP (top panels) and PBE (bottom panels) functionals. The most stable Ef for each concentration are plotted. The line connects the stable phases (filled points) during Li attachment, forming a convex hull.
Materials 12 02136 g003
Materials 12 02136 g004 550
Figure 4. Voltage–capacity curves estimated for lithium attachment to molecular C60, C59N and C59B computed with B3LYP (a) and PBE (b) functionals.
Figure 4. Voltage–capacity curves estimated for lithium attachment to molecular C60, C59N and C59B computed with B3LYP (a) and PBE (b) functionals.
Materials 12 02136 g004
Materials 12 02136 g005 550
Figure 5. Partial densities of states of Lin–C60 (top panels), Lin–C59N (middle panels), and Lin–C59B (bottom panels) computed with B3LYP (left panels) and PBE (right panels) for selected values of n. The black elbow line is used to mark off occupied (left) and unoccupied (right) states.
Figure 5. Partial densities of states of Lin–C60 (top panels), Lin–C59N (middle panels), and Lin–C59B (bottom panels) computed with B3LYP (left panels) and PBE (right panels) for selected values of n. The black elbow line is used to mark off occupied (left) and unoccupied (right) states.
Materials 12 02136 g005
Table
Table 1. Bader charges on Li atoms for attachment of different numbers of Li atoms to C60, C59N, and C59B computed with B3LYP and PBE functionals. Average charge per Li is given when the charges are similar among Li atoms, and the charge of the last Li attached is shown after “/” when it is significantly different from the average of charges of all other Li atoms.
Table 1. Bader charges on Li atoms for attachment of different numbers of Li atoms to C60, C59N, and C59B computed with B3LYP and PBE functionals. Average charge per Li is given when the charges are similar among Li atoms, and the charge of the last Li attached is shown after “/” when it is significantly different from the average of charges of all other Li atoms.
Bader Charge, |e|B3LYPPBE
C60C59NC59BC60C59NC59B
1 Li0.910.900.900.900.900.90
2 Li0.900.900.900.900.900.89
3 Li0.900.890.890.900.900.89
.....................
12 Li0.860.85/0.340.860.860.84/0.450.86
14 Li0.84/0.59 0.85/0.740.84/0.620.85/0.74

Share and Cite

MDPI and ACS Style

Chen, Y.; Cho, C.-R.; Manzhos, S. Lithium Attachment to C60 and Nitrogen- and Boron-Doped C60: A Mechanistic Study. Materials 2019, 12, 2136. https://doi.org/10.3390/ma12132136

AMA Style

Chen Y, Cho C-R, Manzhos S. Lithium Attachment to C60 and Nitrogen- and Boron-Doped C60: A Mechanistic Study. Materials. 2019; 12(13):2136. https://doi.org/10.3390/ma12132136

Chicago/Turabian Style

Chen, Yingqian, Chae-Ryong Cho, and Sergei Manzhos. 2019. "Lithium Attachment to C60 and Nitrogen- and Boron-Doped C60: A Mechanistic Study" Materials 12, no. 13: 2136. https://doi.org/10.3390/ma12132136

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop