Comprehensive Comparison of Lightning Properties of Insulating Liquids in Relation to Mineral Oil Under Positive Lightning Impulse
Abstract
1. Introduction
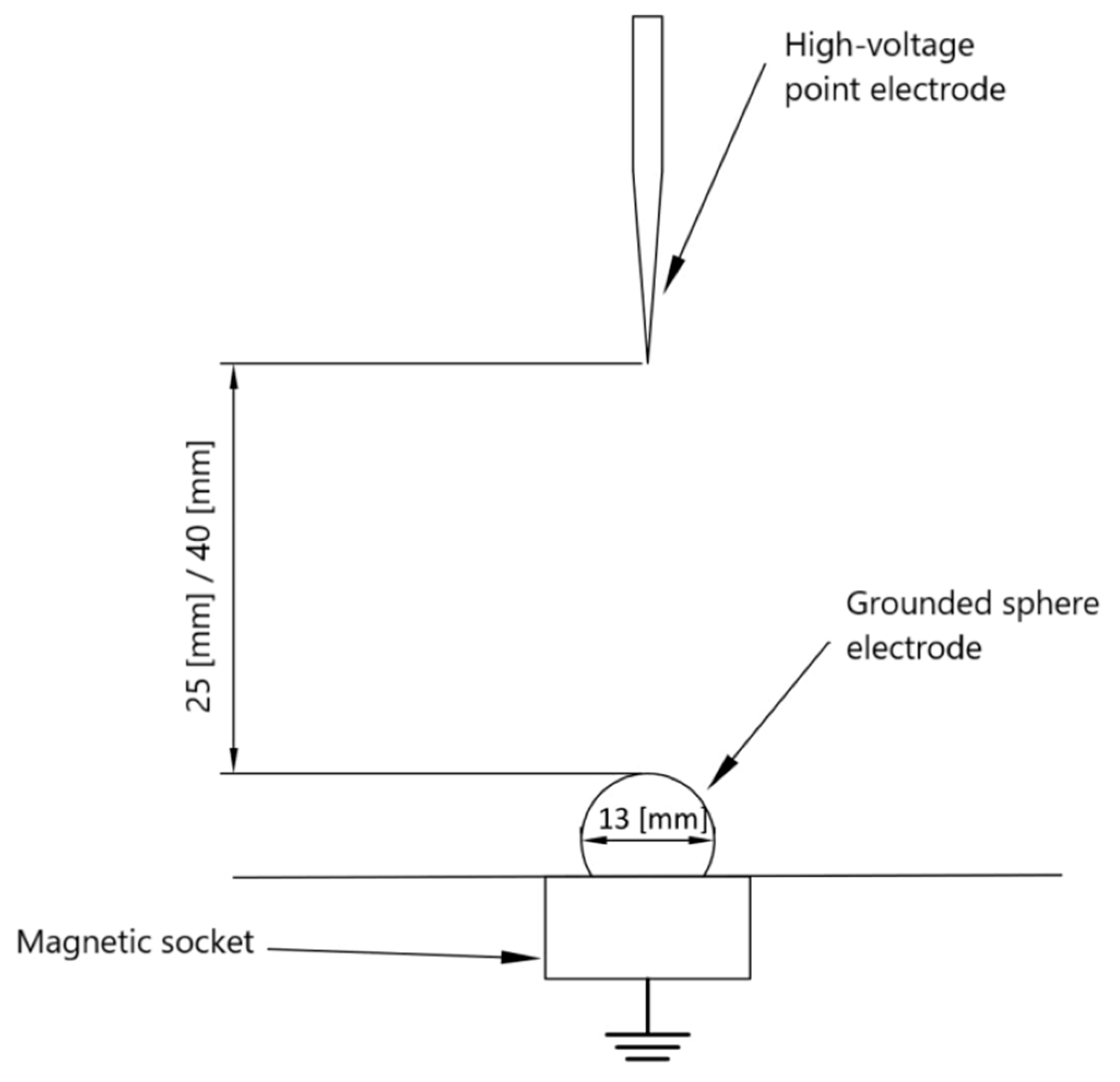
2. Measurement Setup and Methodology
- -
- naphthenic uninhibited mineral oil (UMO);
- -
- inhibited mineral oil (IMO);
- -
- soybean-based natural ester (NE);
- -
- pentaerythritol-based synthetic ester (SE);
- -
- bio-based hydrocarbon (BIO);
- -
- dielectric liquid made using Gas-to-Liquids technology (GTL).
3. Measurement Results and Discussion
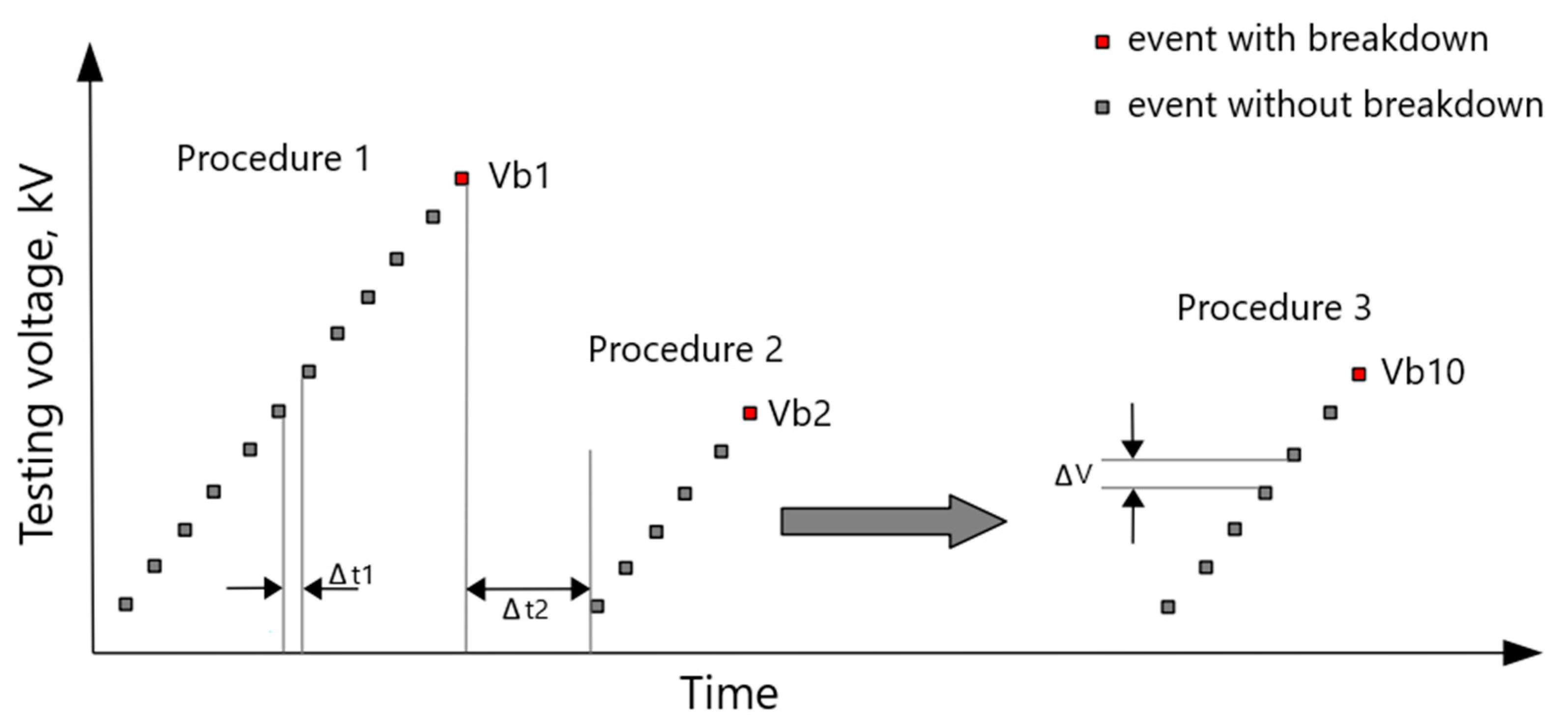
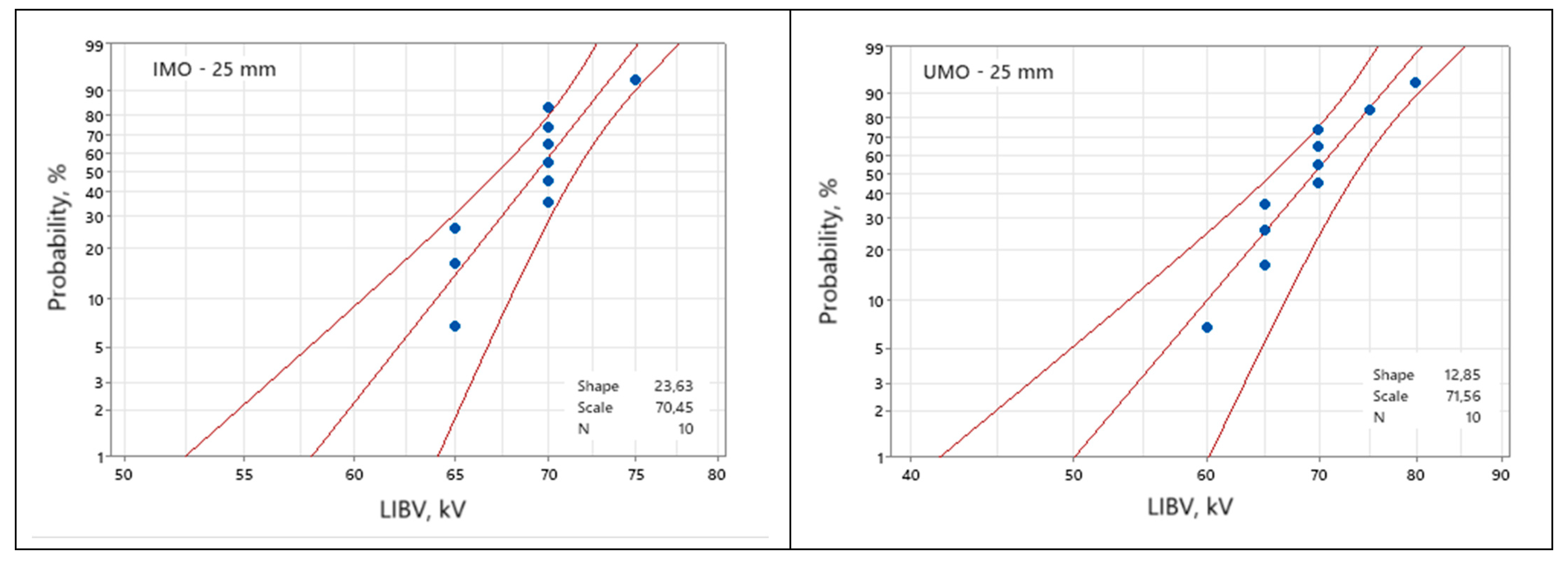
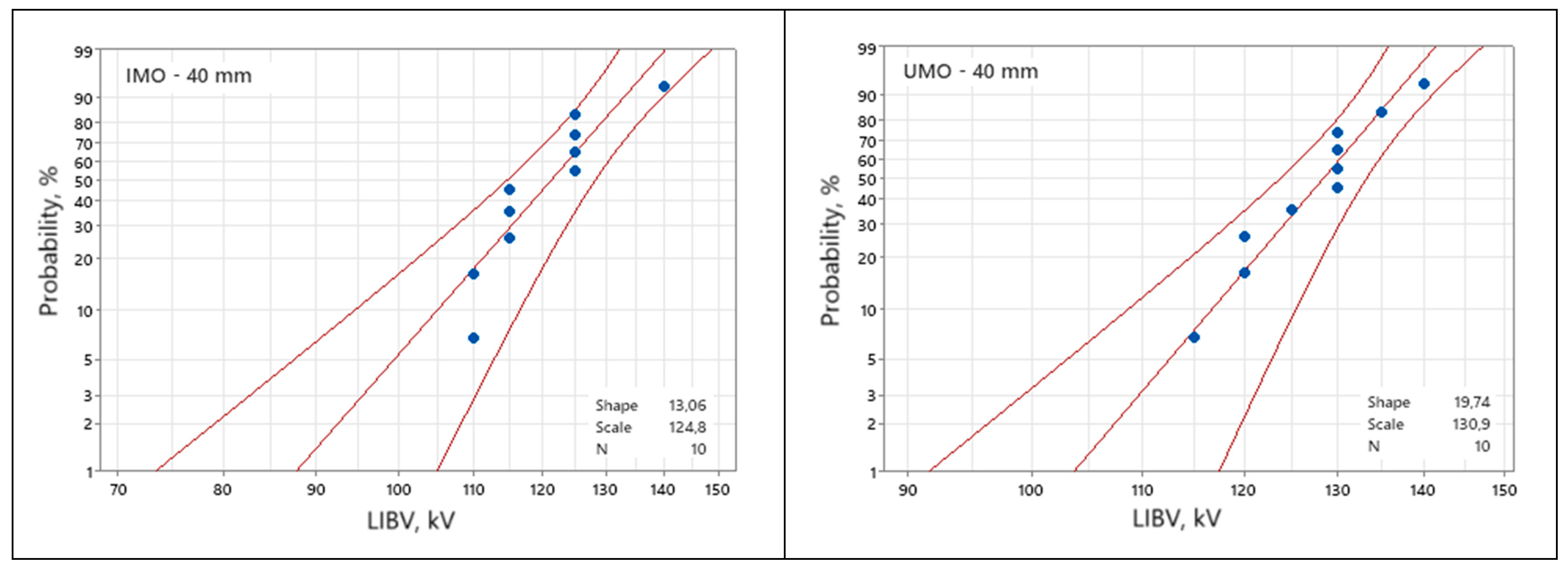
3.1. LIBV
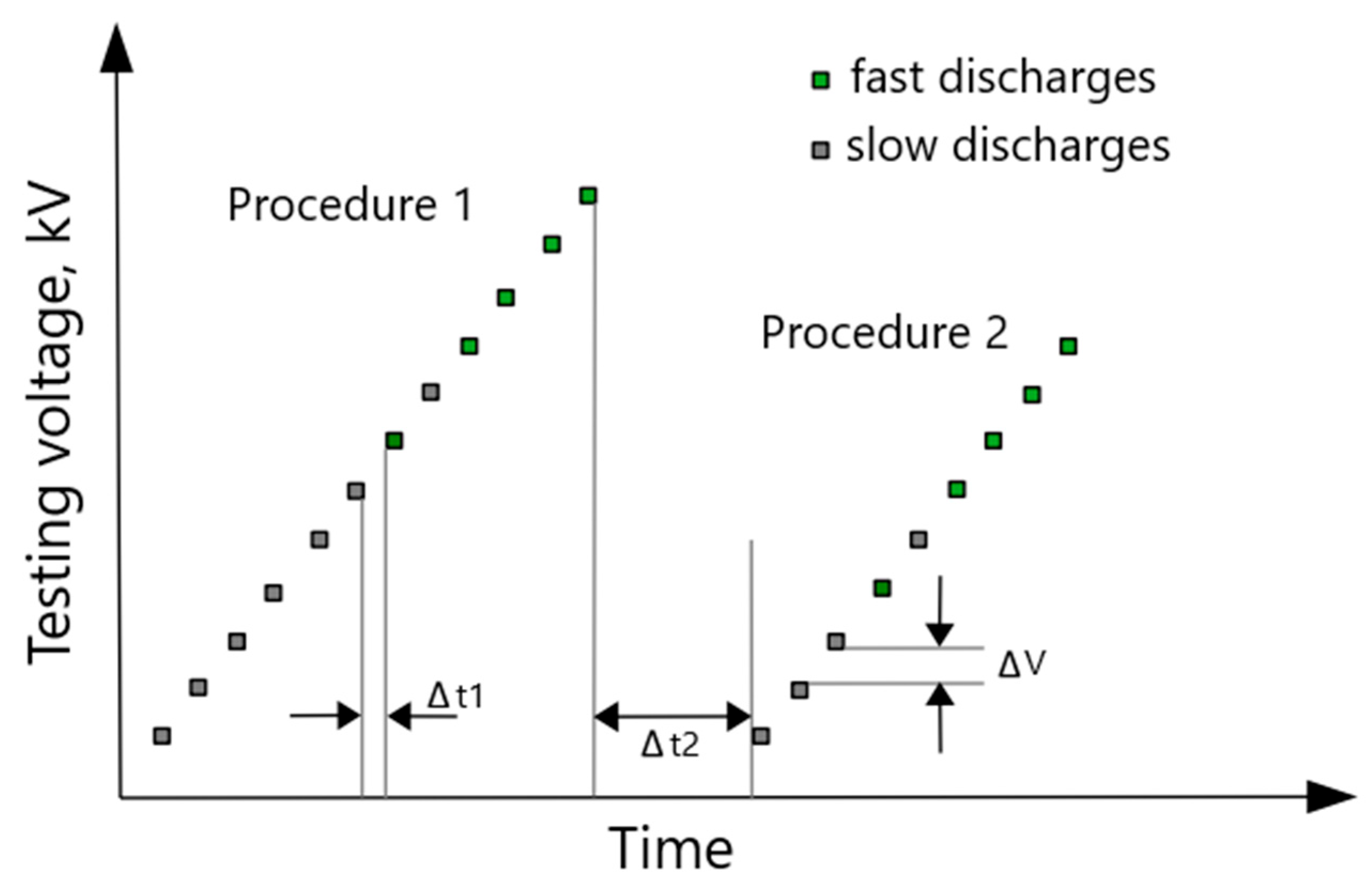
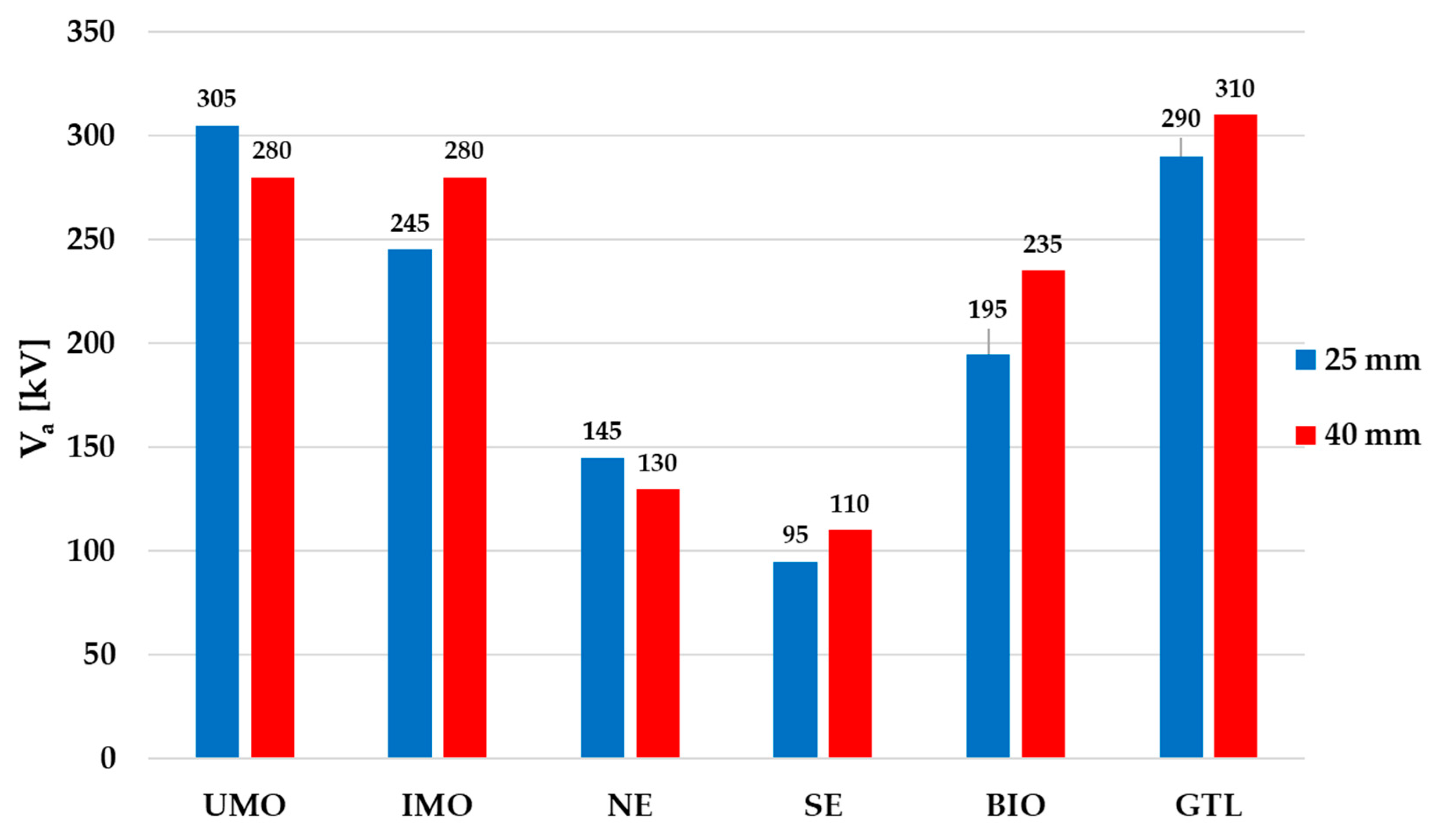
3.2. Acceleration Voltage
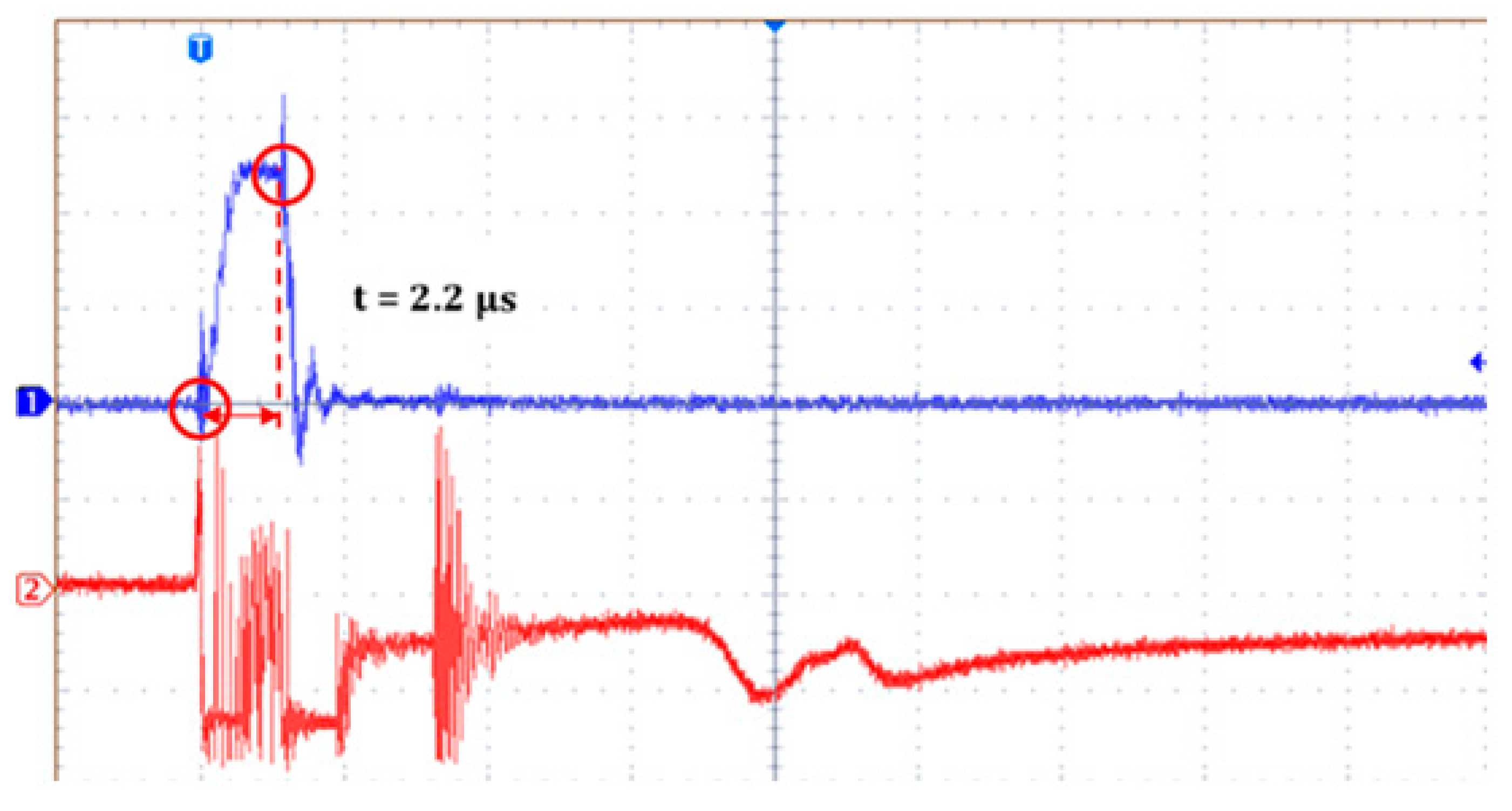
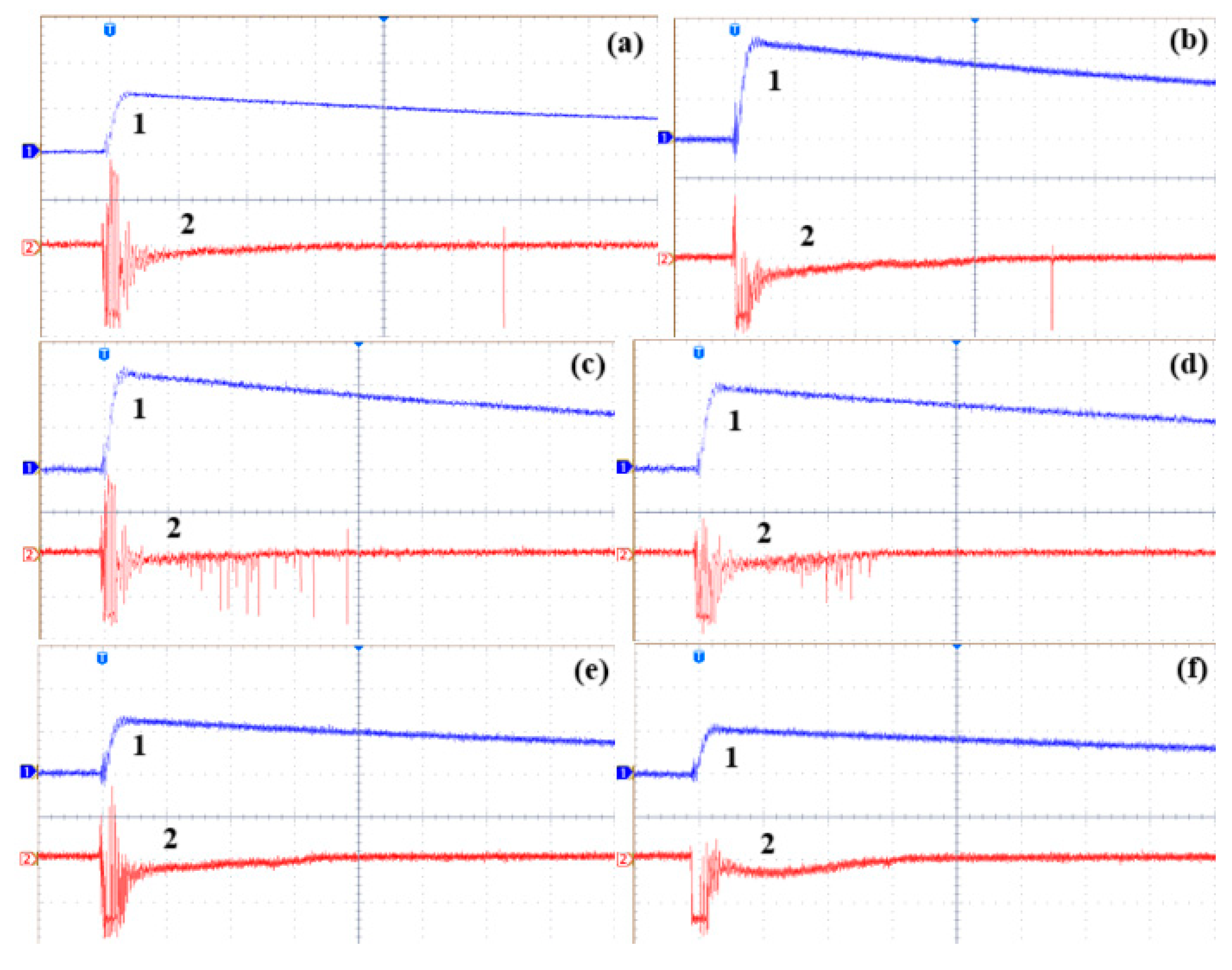
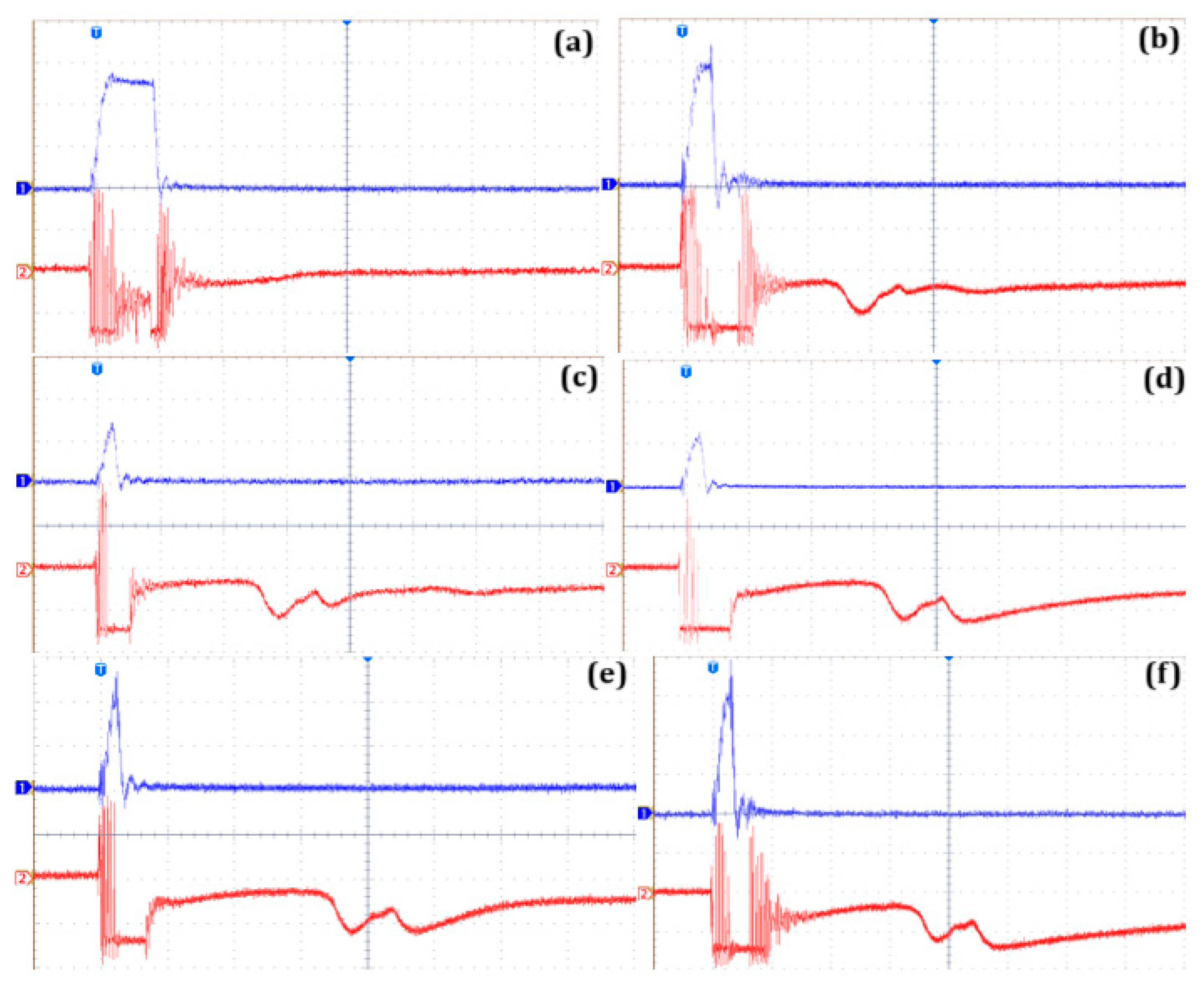
3.3. Light Emission
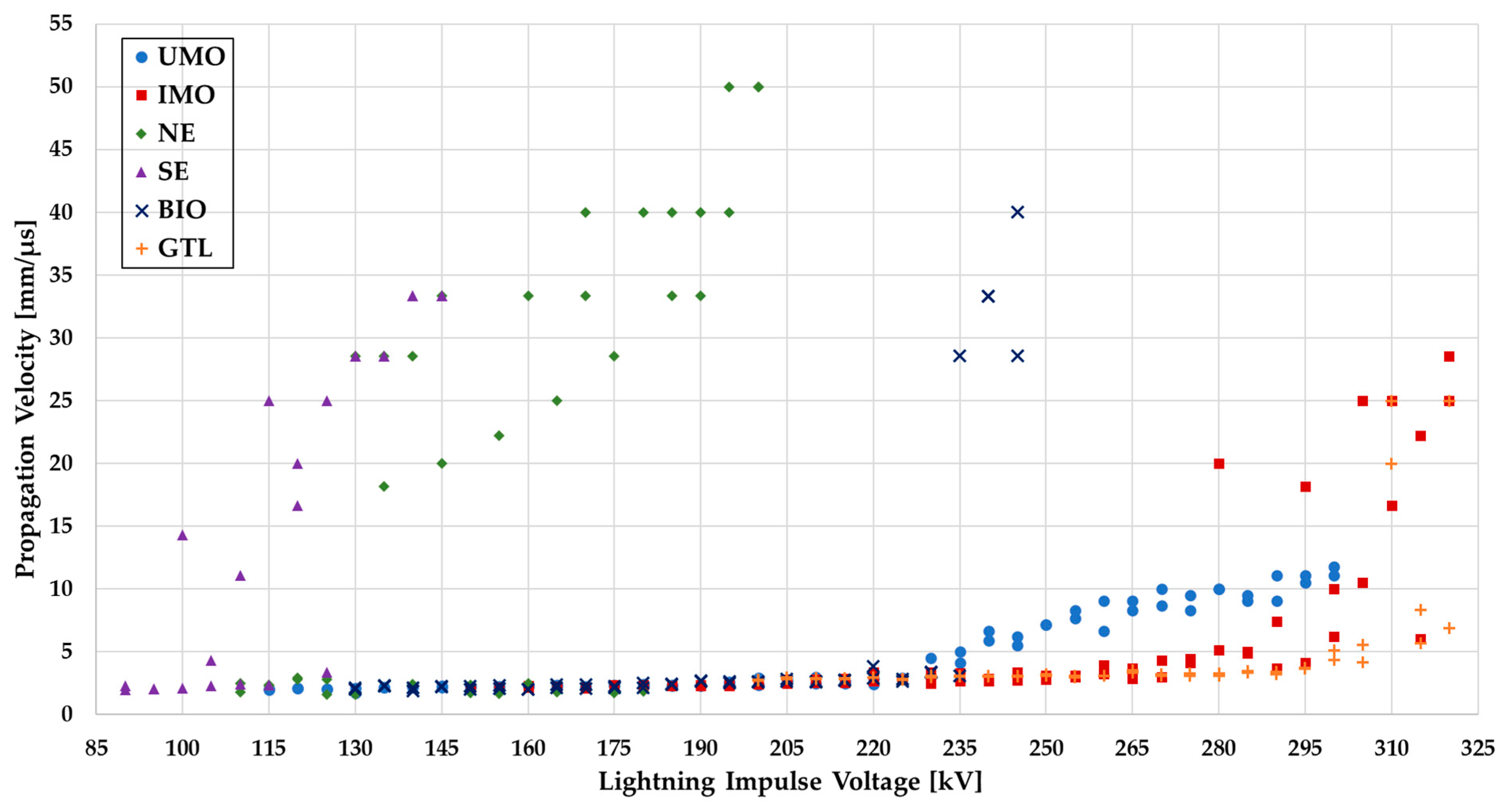
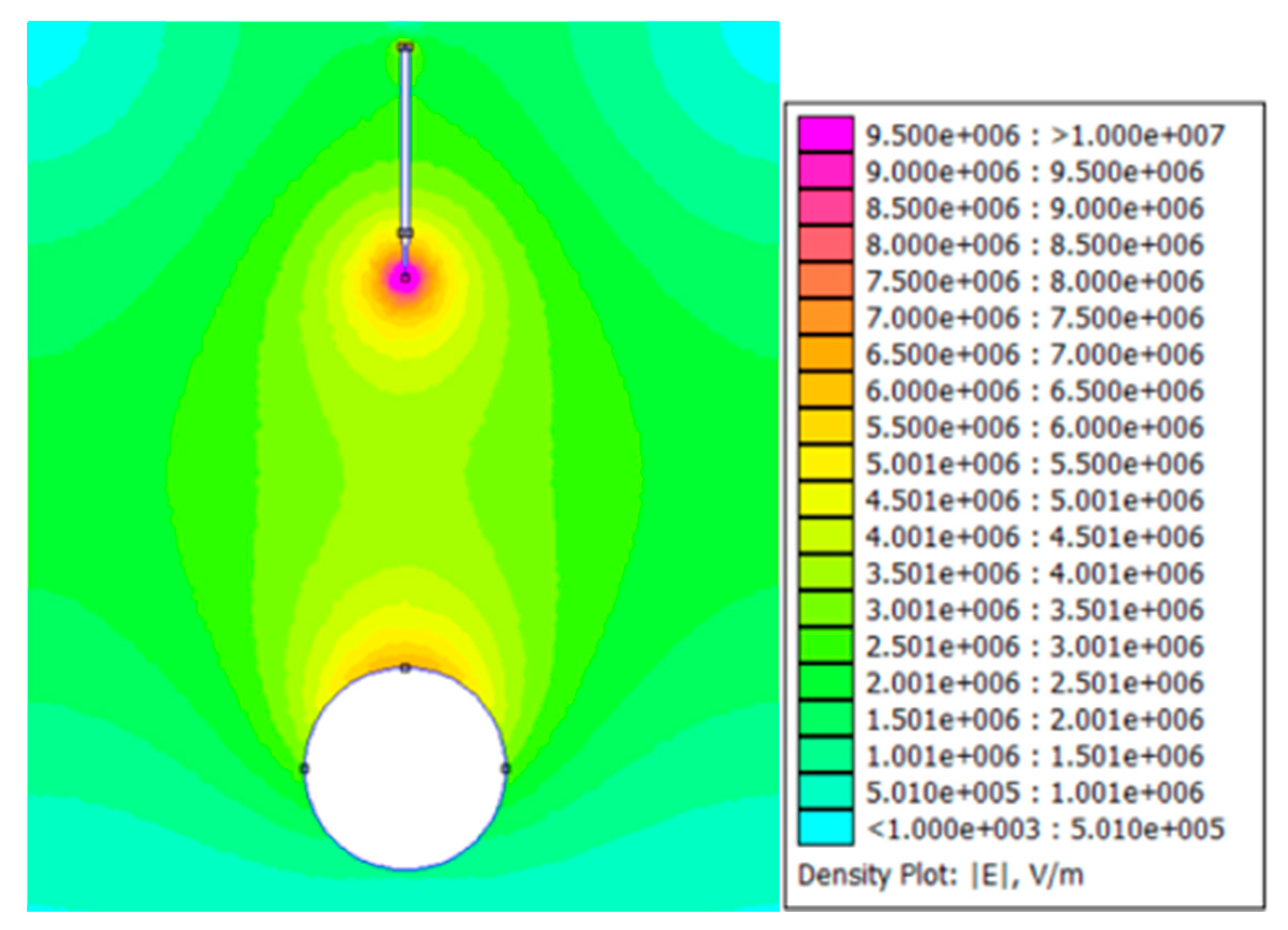
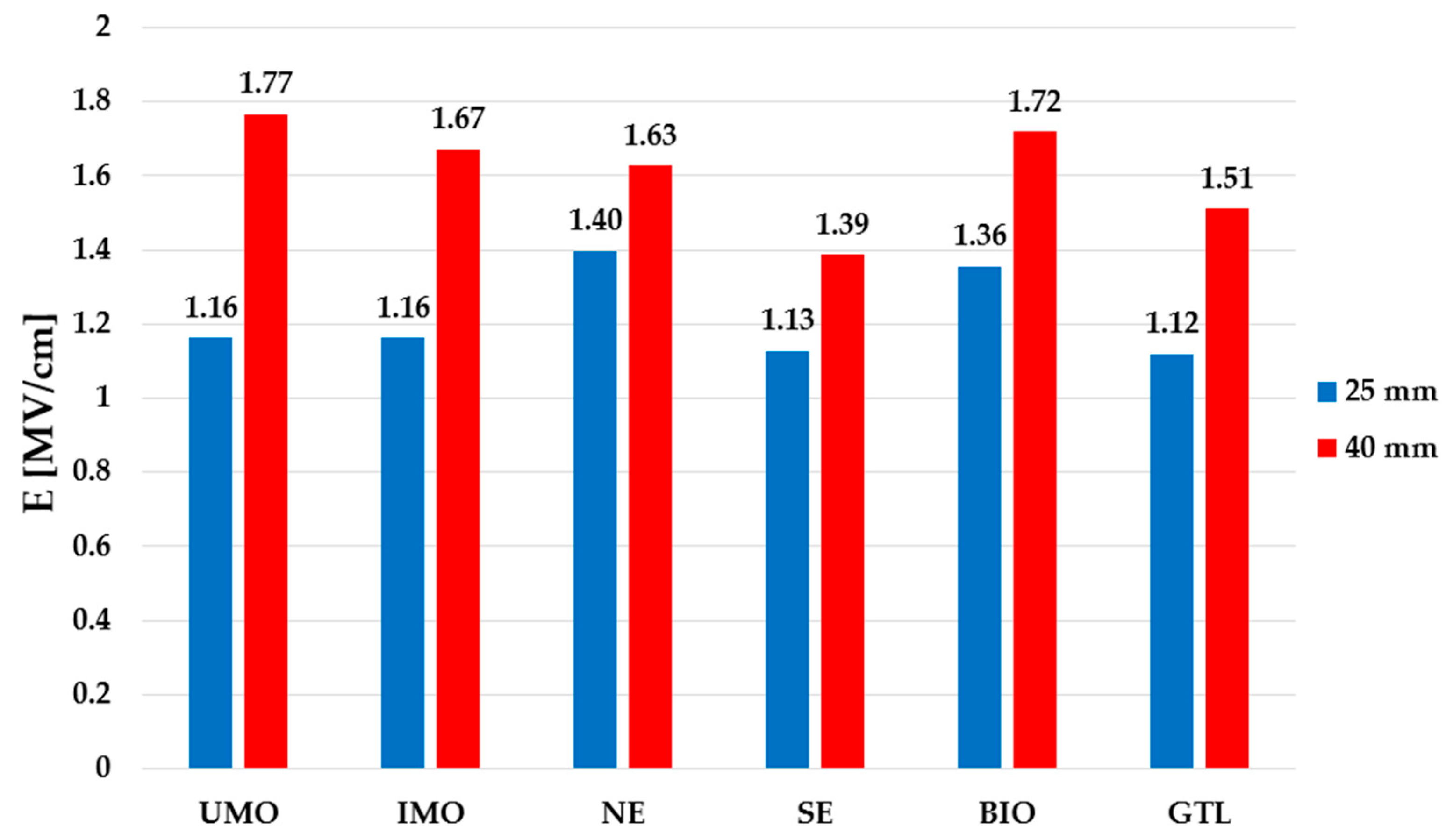
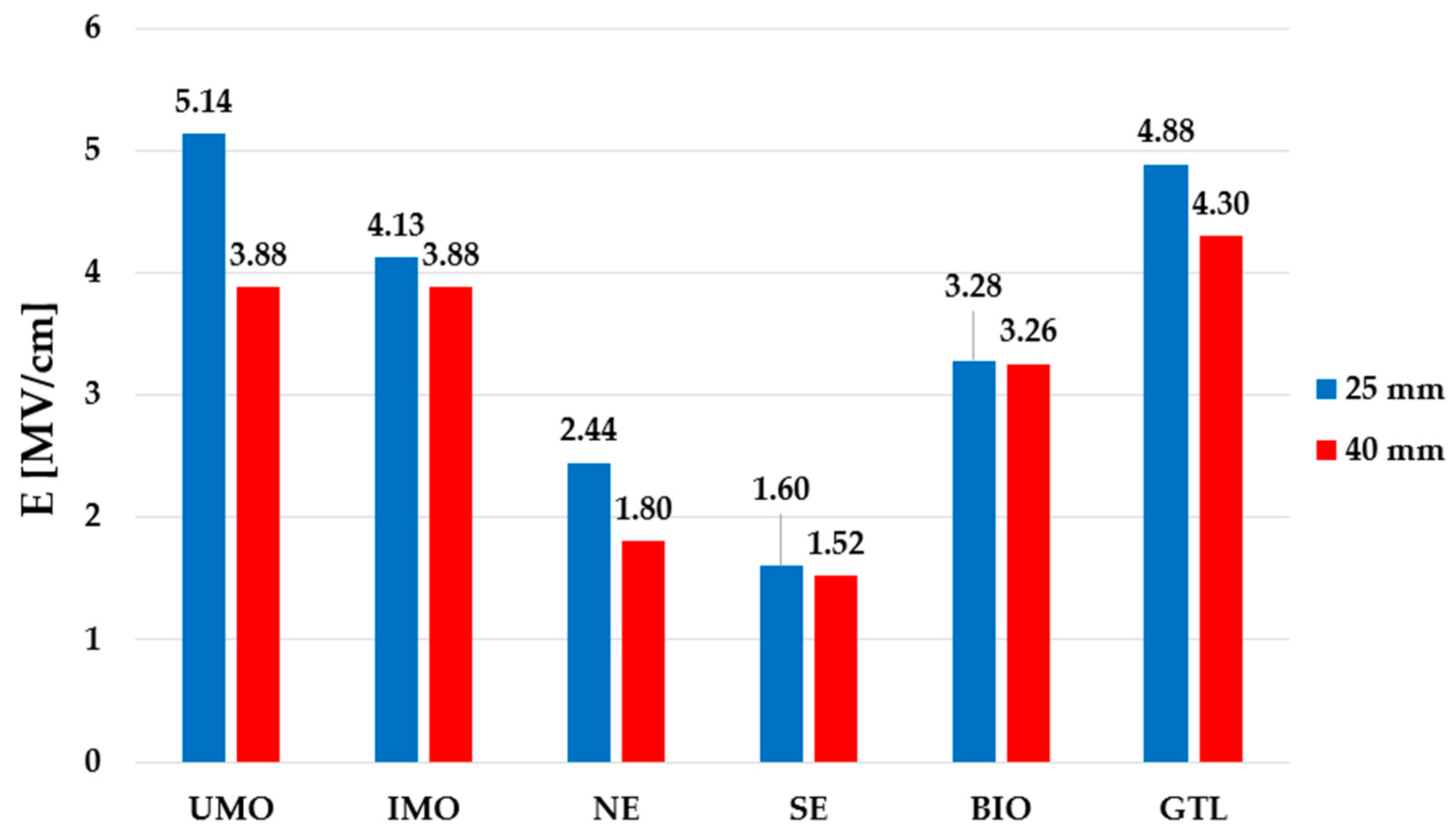
3.4. Electric Field Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fofana, I. 50 years in the development of insulating liquids. IEEE Electr. Insul. Mag. 2013, 29, 13–25. [Google Scholar] [CrossRef]
- Rozga, P.; Rao, U.M.; Fofana, I.; Beroual, A.; Calcara, L.; Pompili, M.; Wang, F.; Casserly, E.; Martin, R.; Malde, J. Next-Generation Ester Dielectric Liquids: Some Key Findings and Perspectives. IEEE Electr. Insul. Mag. 2024, 40, 23–35. [Google Scholar] [CrossRef]
- Working Group A2.35. Experiences in Service with New Insulating Liquids; CIGRE Brochure 436; CIGRE: Paris, France, 2011. [Google Scholar]
- Rozga, P.; Beroual, A.; Przybylek, P.; Jaroszewski, M.; Strzelecki, K. A Review on Synthetic Ester Liquids for Transformer Applications. Energies 2020, 13, 6429. [Google Scholar] [CrossRef]
- Rafiq, M.; Shafique, M.; Ateeq, M.; Zink, M.; Targitay, D. Natural esters as sustainable alternating dielectric liquids for transformer insulation system: Analyzing the state of the art. Clean Technol. Environ. Policy 2024, 26, 623–659. [Google Scholar] [CrossRef]
- Jayasree, T.; Rao, U.M.; Fofana, I.; Brettschneider, S.; Celis, E.M.R.; Picher, P. Pre-breakdown Phenomena and Influence of Aging Byproducts in Thermally Aged Low Pour Point Ester Fluids Under AC Stress. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 1563–1570. [Google Scholar] [CrossRef]
- Oparanti, S.O.; Rao, U.M.; Fofana, I. Natural Esters for Green Transformers: Challenges and Keys for Improved Serviceabil-ity. Energies 2023, 16, 61. [Google Scholar] [CrossRef]
- IEC 60897; Methods for the Determination of the Lightning Impulse Breakdown Voltage of Insulating Liquids. International Electrotechnical Commission (IEC): Geneva, Switzerland, 1987.
- IEC 60156; Insulating Liquids—Determination of the Breakdown Voltage at Power Frequency—Test Method. International Electrotechnical Commission (IEC): Geneva, Switzerland, 2018.
- Dang, V.-H.; Beroual, A.; Perrier, C. Comparative study of statistical breakdown in mineral, synthetic and natural ester oils under AC voltage. IEEE Trans. Dielectr. Electr. Insul. 2012, 19, 1508–1513. [Google Scholar] [CrossRef]
- Denat, A.; Lesaint, O.; Mc Cluskey, F. Breakdown of liquids in long gaps: Influence of distance, impulse shape, liquid nature, and interpretation of measurements. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 2581–2591. [Google Scholar] [CrossRef]
- Rao, U.M.; Fofana, I.; Beroual, A.; Rozga, P.; Pompili, M.; Calcara, L.; Rapp, K.J. A review on pre-breakdown phenomena in ester fluids: Prepared by the international study group of IEEE DEIS liquid dielectrics technical committee. IEEE Trans. Dielectr. Electr. Insul. 2020, 27, 1546–1560. [Google Scholar] [CrossRef]
- Working Group A2.35. Dielectric Performance of Insulating Liquids for Transformers; CIGRE Brochure 856; CIGRE: Paris, France, 2021. [Google Scholar]
- Lesaint, O.; Massala, G. Positive streamer propagation in large oil gaps: Experimental characterization of propagation modes. IEEE Trans. Dielectr. Electr. Insul. 1998, 5, 360–370. [Google Scholar] [CrossRef]
- Liu, Q.; Wang, Z.D. Streamer characteristic and breakdown in synthetic and natural ester transformer liquids under standard lightning impulse voltage. IEEE Trans. Dielectr. Electr. Insul. 2011, 18, 285–294. [Google Scholar] [CrossRef]
- Lesaint, O. Prebreakdown phenomena in liquids: Propagation ‘modes’ and basic physical properties. J. Phys. D Appl. Phys. 2016, 49, 14401–14422. [Google Scholar] [CrossRef]
- Linhjell, D.; Lundgaard, L.; Unge, M.; Hjortstam, O. Prebreakdown phenomena in hydrocarbon liquids in a point-plane gap under step voltage. Part 1: Behaviour at positive polarity. J. Phys. Commun. 2020, 4, 045012. [Google Scholar] [CrossRef]
- Lundgaard, L.E.; Liu, Q.; Lesaint, O.; Madshaven, I. Dielectric Performance of Transformer Liquids–Summary of a CIGRE Study. IEEE Electr. Insul. Mag. 2023, 39, 7–16. [Google Scholar] [CrossRef]
- Stuchała, F.; Małaczek, M.; Puchała, K.; Jakubowski, J.; Binek, M. Breakdown and acceleration voltage of biodegradable liquid made in GTL technology at positive lightning impulse voltage. Przeglad Elektrotechniczny 2024, 11, 182–185. [Google Scholar] [CrossRef]
- Stuchala, F.; Rozga, P.; Malaczek, M.; Staniewski, J. Comparative Analysis of Selected Insulating Liquids Including Bio-Based Hydrocarbon and GTL in Terms of Breakdown and Acceleration Voltage at Negative Lightning Impulse. IEEE Trans. Dielectr. Electr. Insul. 2024, 31, 2443–2450. [Google Scholar] [CrossRef]
- Rozga, P.; Stuchala, F.; Ungarala, M.R.; Sree, T.J. Assessing the lightning performance of sustainable GTL type dielectric liquids versus mineral oils and synthetic ester in a non-uniform electric field. Measurement 2025, 242, 116089. [Google Scholar] [CrossRef]
- Tobazcon, R. Prebreakdown phenomena in dielectric liquids. IEEE Trans. Dielectr. Electr. Insul. 1994, 1, 1132–1147. [Google Scholar] [CrossRef]
- Beroual, A.; Zahn, M.; Badent, A.; Kist, K.; Schwabe, A.; Yamashita, H.; Yamazawa, K.; Danikas, M.; Chadband, W.; Torshin, Y. Propagation and structure of streamers in liquid dielectrics. IEEE Electr. Insul. Mag. 1998, 14, 6–17. [Google Scholar] [CrossRef]
- ISO 12185; Crude Petroleum, Petroleum Products and Related Products—Determination of Density—Laboratory Density Meter with an Oscillating U-Tube Sensor. International Organization for Standardization (ISO): Geneva, Switzerland, 2024.
- ISO 3104; Petroleum Products—Transparent and Opaque Liquids—Determination of Kinematic Viscosity and Calculation of Dynamic Viscosity. International Organization for Standardization (ISO): Geneva, Switzerland, 2023.
- ISO 2719; Determination of Flash Point—Pensky-Martens Closed Cup Method. International Organization for Standardization (ISO): Geneva, Switzerland, 2016.
- ISO 3016; Petroleum and Related Products from Natural or Synthetic Sources—Determination of Pour Point. International Organization for Standardization (ISO): Geneva, Switzerland, 2019.
- OECD Guideline for Testing Chemicals, Test No. 301: Ready Biodegradability; Organisation for Economic Co-operation and Development (OECD): Paris, France, 1992.
- ASTM D6866-22; Standard Test Methods for Determining the Biobased Content of Solid, Liquid, and Gaseous Samples Using Radiocarbon Analysis. American Society for Testing and Materials (ASTM): West Conshohocken, PA, USA, 2024.
- IEC 60247; Insulating Liquids—Measurement of Relative Permittivity, Dielectric Dissipation Factor (tan d) and d.c. Resistivity. International Electrotechnical Commission (IEC): Geneva, Switzerland, 2004.
- Sunesson, A.; Barmann, P.; Kroll, S.; Walfridsson, L. Laser triggering of electric breakdown in liquids. IEEE Trans. Dielectr. Electr. Insul. 1994, 1, 680–691. [Google Scholar] [CrossRef]
- Bårmann, P.; Kröll, S.; Sunesson, A. Spectroscopic measurements of streamer filaments in electric breakdown in a dielectric liquid. J. Phys. D Appl. Phys. 1996, 29, 1188–1196. [Google Scholar] [CrossRef]
- Rozga, P.; Tabaka, P. Comparative analysis of breakdown spectra registered using optical spectrometry technique in biodegradable ester liquids and mineral oil. IET Sci. Meas. Technol. 2018, 12, 684–690. [Google Scholar] [CrossRef]
- Rozga, P.; Stuchala, F.; Pasternak, B.; Binek, M. Comparison of negative streamer development in synthetic ester and mineral oil in a point-sphere gap divided by a pressboard barrier. J. Electrost. 2023, 125, 103839. [Google Scholar] [CrossRef]
- Yu, H.; Liu, Q.; Wang, Z.; Krause, C.; Wilkinson, M. Breakdown Characteristics of Transformer Liquids with Winding Conductor Segments under Lightning Impulse. IEEE Trans. Dielectr. Electr. Insul. 2025; early access. [Google Scholar] [CrossRef]
- Trinh, N.G.; Vincent, C. Statistical Significance of Test Methods for Low Probability Breakdown and Withstand Voltages. IEEE Trans. Power Appar. Syst. 1980, PAS-99, 711–719. [Google Scholar] [CrossRef]
- Liu, Q.; Wang, Z.D.; Perrot, F. Impulse Breakdown Voltages of Ester-based Transformer Oils Determined by Using Different Test Methods. In Proceedings of the 2009 IEEE Conference on Electrical Insulation and Dielectric Phenomena, Virginia Beach, VA, USA, 18–21 October 2009; pp. 608–612. [Google Scholar]
- Haegele, S.; Vahidi, F.; Tenbohlen, S.; Rapp, K.J.; Sbravati, A. Lightning Impulse Withstand of Natural Ester Liquid. Energies 2018, 11, 1964. [Google Scholar] [CrossRef]
- Rozga, P.; Stuchala, F.; Wolmarans, C.; Milone, M. Discharge Inception and Breakdown of the Oil-Wedge Type Electrode Model Insulated with GTL based Die-lectric Liquids. In Proceedings of the 2025 IEEE 23rd International Conference on Dielectric Liquids (ICDL), Lodz, Poland, 18–22 May 2025. [Google Scholar]
- Chen, Q.; Beroual, A.; Sima, W.; Sun, P. AC and Lightning Impulse Breakdown Voltage Comparative Study of Mineral Oil-Based Fe3O4, Al2O3, and TiO2 Nanofluids. IEEE Trans. Dielectr. Electr. Insul. 2024, 31, 278–287. [Google Scholar] [CrossRef]
- Guo, R.; Becerra, M.; Li, J. Macroscopic Simulation of Streamer Development in Mineral Oil. In Proceedings of the 2022 IEEE 21st International Conference on Dielectric Liquids (ICDL), Sevilla, Spain, 29 May–2 June 2022; pp. 1–4. [Google Scholar]
- Li, S.; Wang, F.; Wang, Q.; Ouyang, L.; Chen, X.; Li, J. Numerical Modeling of Branching-Streamer Propagation in Ester-Based Insulating Oil Under Positive Lightning Impulse Voltage: Effects from Needle Curvature Radius. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 139–147. [Google Scholar] [CrossRef]
- Li, C.-H.; Liang, X.; Liu, T.; Wang, F.; Huang, Z.; Li, J. Three-Dimensional Features of Streamers in Natural Ester Insulating Oil Under Lightning Impulse Voltage. IEEE Trans. Dielectr. Electr. Insul. 2024, 31, 95–101. [Google Scholar] [CrossRef]






| Chemical Family | UMO—Complex Mixture of Hydrocarbons | IMO—Complex Mixture of Hydrocarbons | NE—Natural Ester (Triglycerides, Soybean) | SE—Synthetic Ester (Pentaerythritol Based) | BIO— Hydrocarbon (Iso-Alkane) | GTL— Hydrocarbon (Iso-Paraffinic) |
|---|---|---|---|---|---|---|
| Trade name | Diala S2 | Nytro 10XN | Envirotemp FR3 | Envirotemp 360 | Nytro BIO 300X | Diala S4 ZX-I |
| Density at 20 °C [kg/dm3] [24] | 0.88 | 0.87 | 0.92 | 0.97 | 0.78 | 0.8 |
| Viscosity at 40 °C [mm2/s] [25] | 11 | 7.8 | 32 | 29 | 3.7 | 9.7 |
| Flash point [°C] [26] | 146 | 146 | 320 | 260 | 145 | 191 |
| Pour point [°C] [27] | −50 | −63 | −23 | −56 | −63 | −41 |
| Biodegradability [28] | Inherently biodegradable | Inherently biodegradable | Readily biodegradable | Readily biodegradable | Readily biodegradable | Readily Biodegradable |
| Bio-based carbon content [%] [29] | 0 | 0 | ≈97 | ≈>50 | 99 | 0 |
| Relative permittivity at 90 °C [-] [30] | 2.2 | 2.2 | 3.1 | 3.2 | 1.99 | 2.03 |
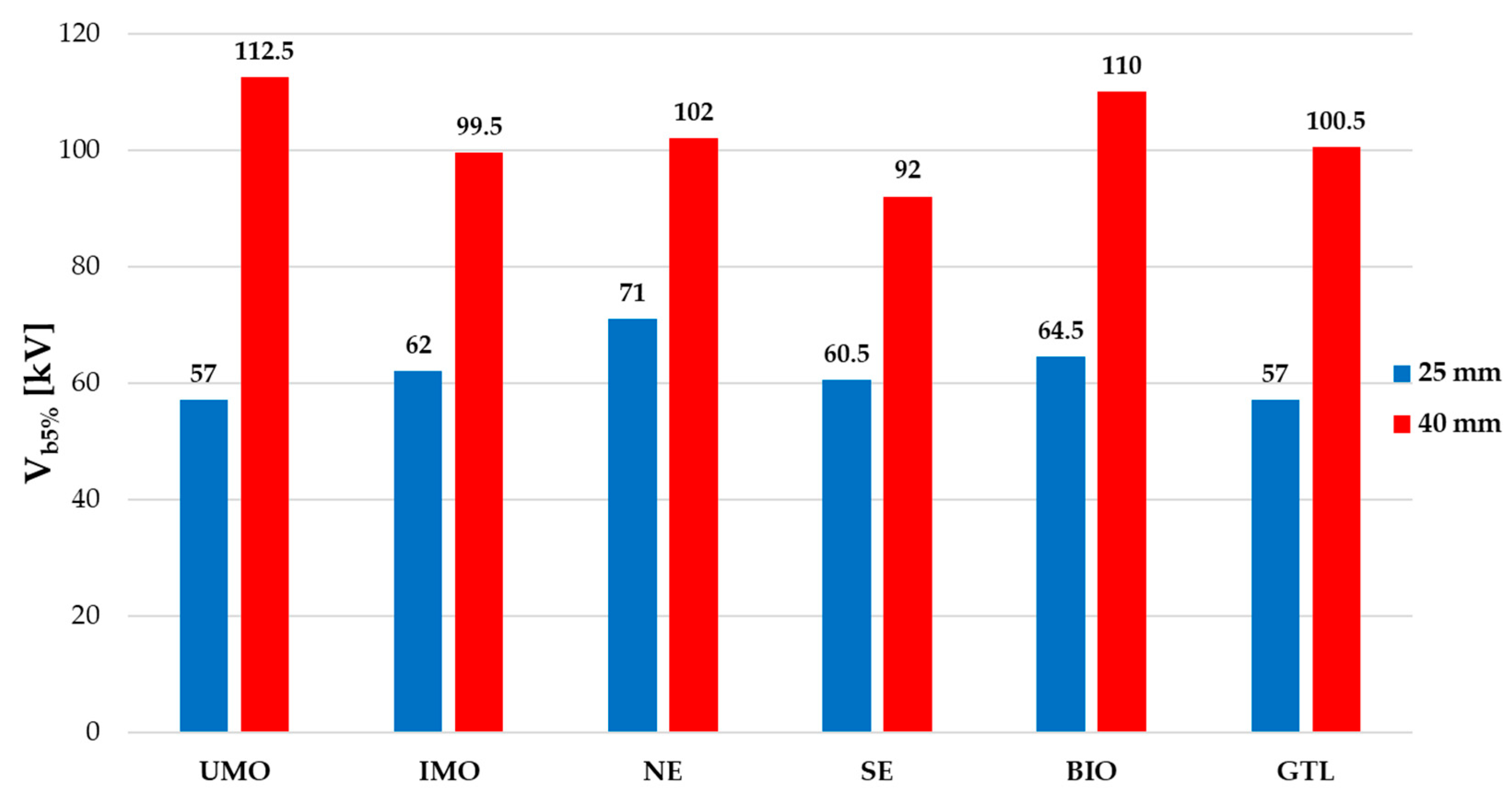
| Breakdown Probability | UMO | IMO | NE | SE | BIO | GTL |
|---|---|---|---|---|---|---|
| 25 mm | ||||||
| Vb [kV] | 69 | 69 | 83 | 67 | 80.5 | 66.5 |
| Vb5% [kV] | 57 | 62 | 71 | 60.5 | 64.5 | 57 |
| Vb50% [kV] | 69.5 | 69.5 | 84 | 68 | 81 | 67 |
| SD [kV] | 5.68 | 3.16 | 6.32 | 5.37 | 7.25 | 4.12 |
| Median [kV] | 70 | 55 | 80 | 70 | 77.5 | 65 |
| 40 mm | ||||||
| Vb [kV] | 127.5 | 120.5 | 117.5 | 100 | 124 | 109 |
| Vb5% [kV] | 112.5 | 99.5 | 102 | 92 | 110 | 100.5 |
| Vb50% [kV] | 128.5 | 121.5 | 118.5 | 100.5 | 125.5 | 110 |
| SD [kV] | 7.55 | 9.26 | 7.17 | 4.08 | 8.43 | 5.16 |
| Median [kV] | 130 | 120 | 115 | 100 | 125 | 110 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stuchala, F.; Rozga, P. Comprehensive Comparison of Lightning Properties of Insulating Liquids in Relation to Mineral Oil Under Positive Lightning Impulse. Energies 2025, 18, 2381. https://doi.org/10.3390/en18092381
Stuchala F, Rozga P. Comprehensive Comparison of Lightning Properties of Insulating Liquids in Relation to Mineral Oil Under Positive Lightning Impulse. Energies. 2025; 18(9):2381. https://doi.org/10.3390/en18092381
Chicago/Turabian StyleStuchala, Filip, and Pawel Rozga. 2025. "Comprehensive Comparison of Lightning Properties of Insulating Liquids in Relation to Mineral Oil Under Positive Lightning Impulse" Energies 18, no. 9: 2381. https://doi.org/10.3390/en18092381
APA StyleStuchala, F., & Rozga, P. (2025). Comprehensive Comparison of Lightning Properties of Insulating Liquids in Relation to Mineral Oil Under Positive Lightning Impulse. Energies, 18(9), 2381. https://doi.org/10.3390/en18092381







