Energy Adaptability Analysis Based on the Stall Fault of Solar Array Drive Assembly for Medium Earth Orbit Satellite
Abstract
1. Introduction
2. Methodology
3. Results
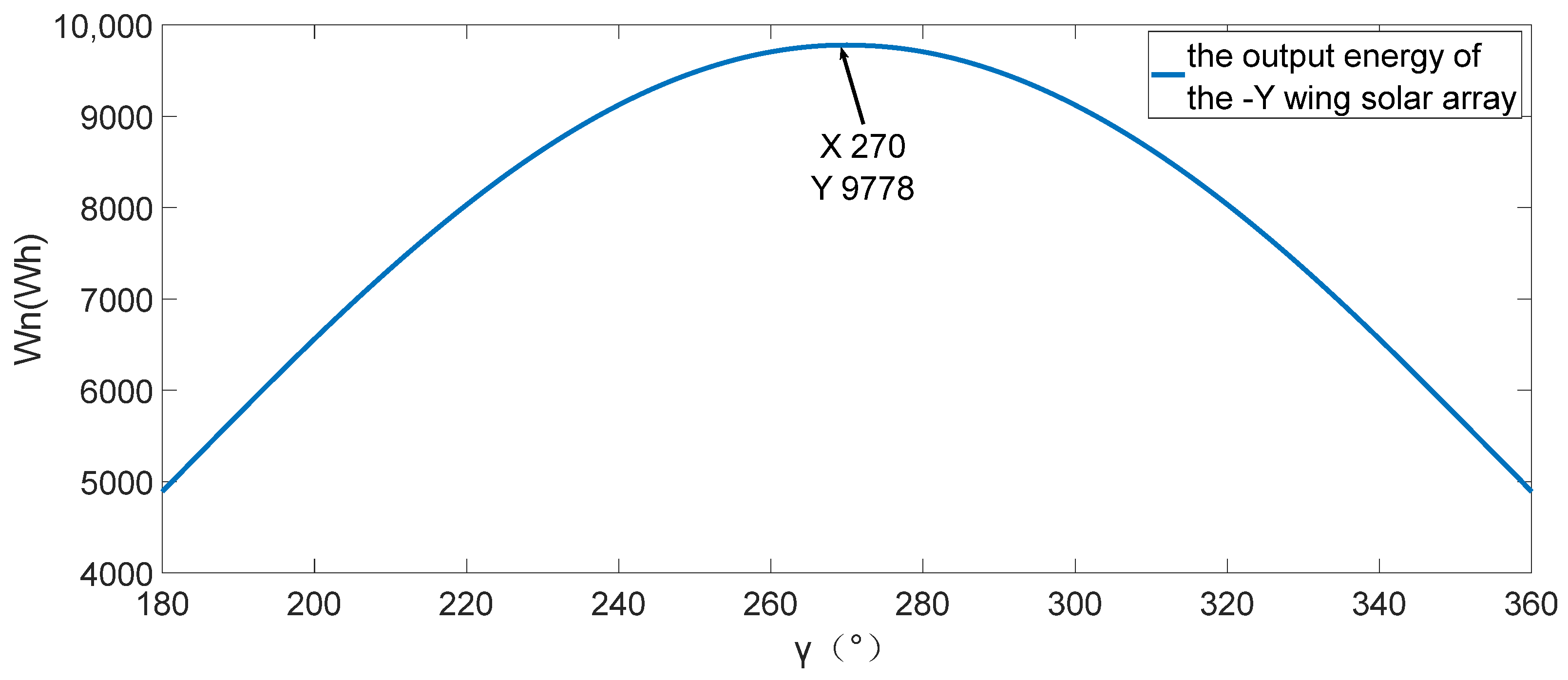
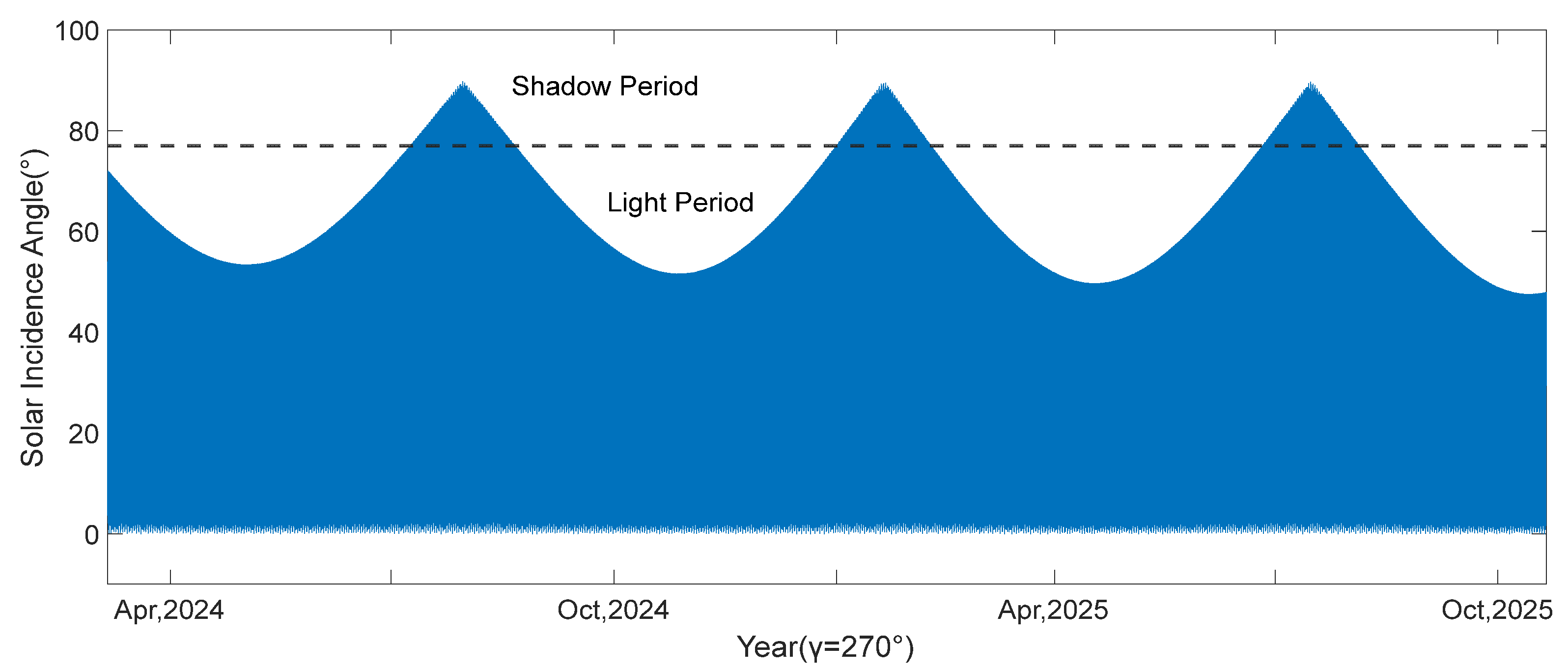
3.1. Analysis of the Optimal Stalling Angle of the Y-Wing Solar Panel for Energy
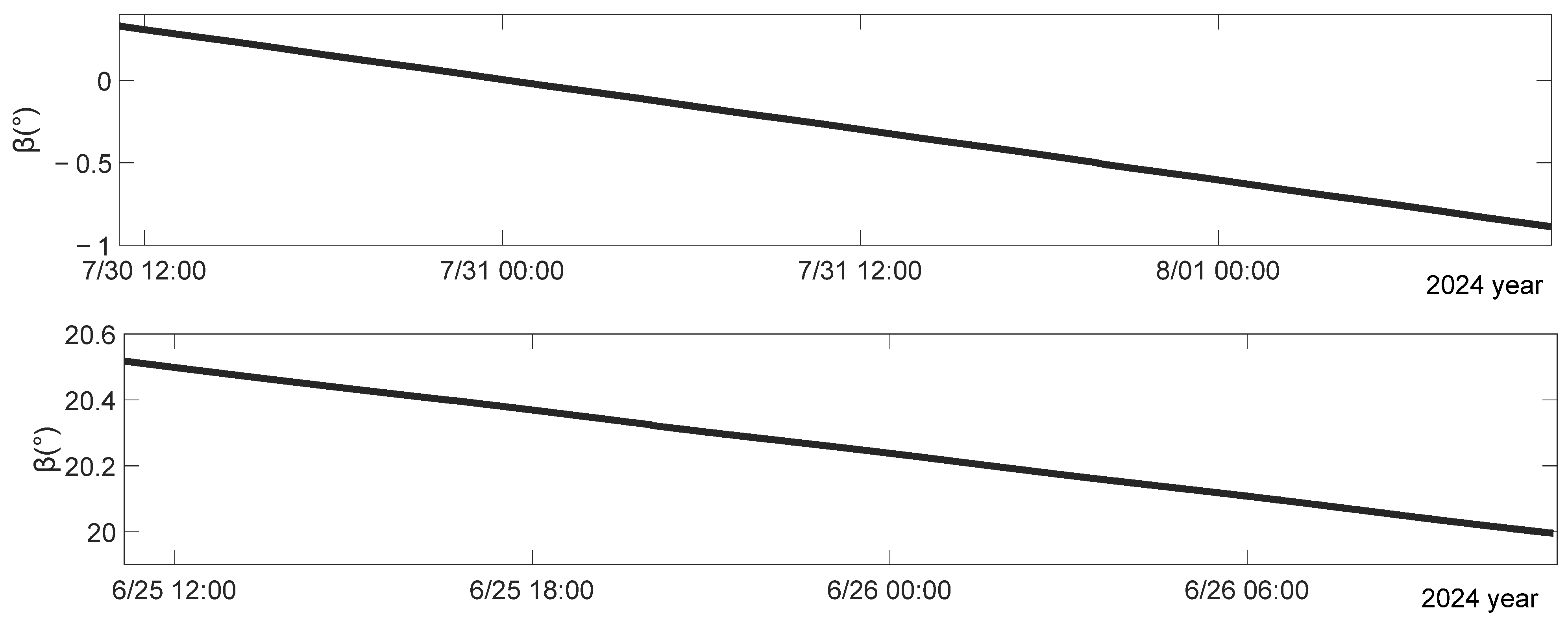
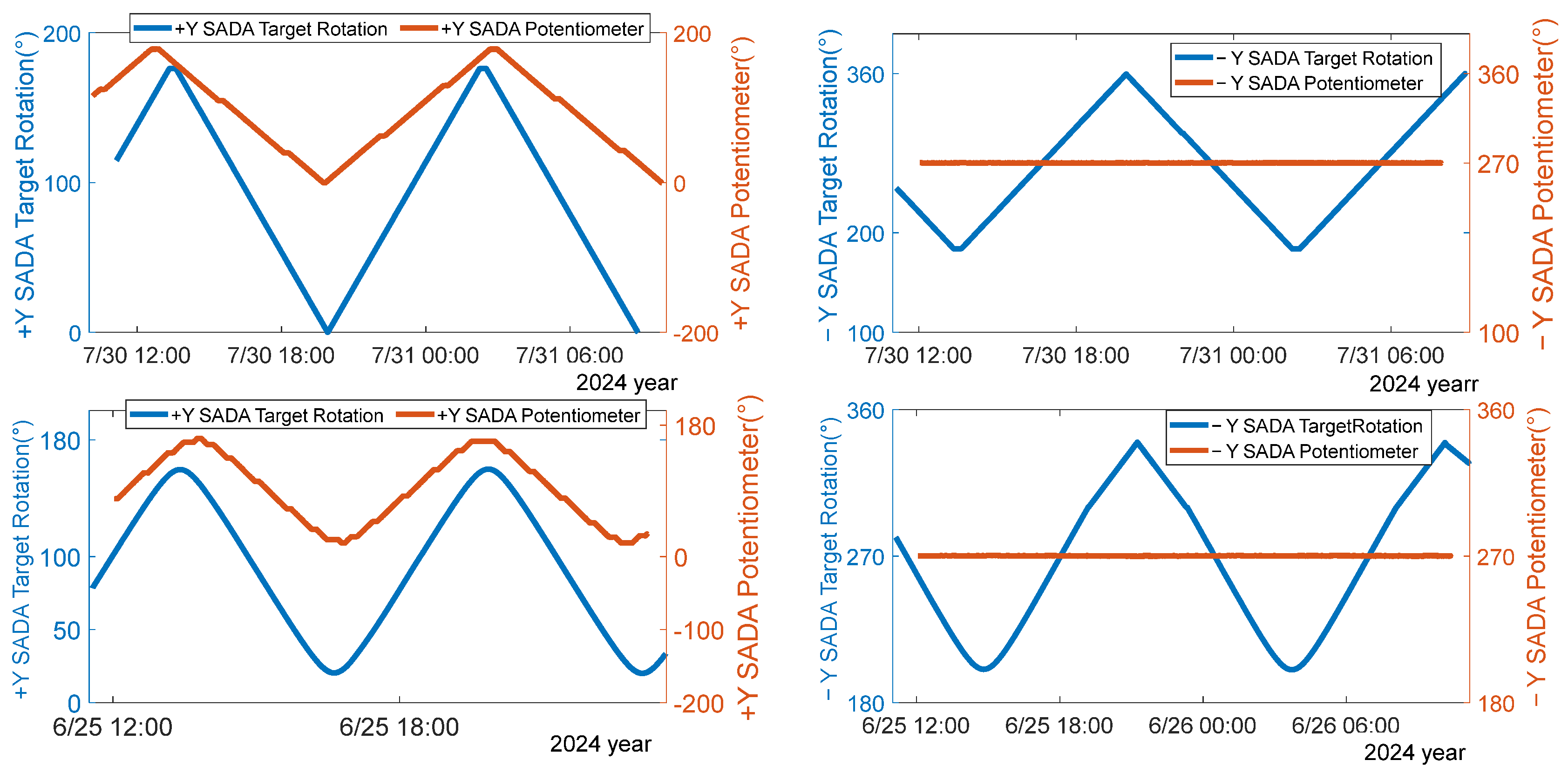
3.2. In-Orbit Control Strategy Validation and Realization
4. Discussion
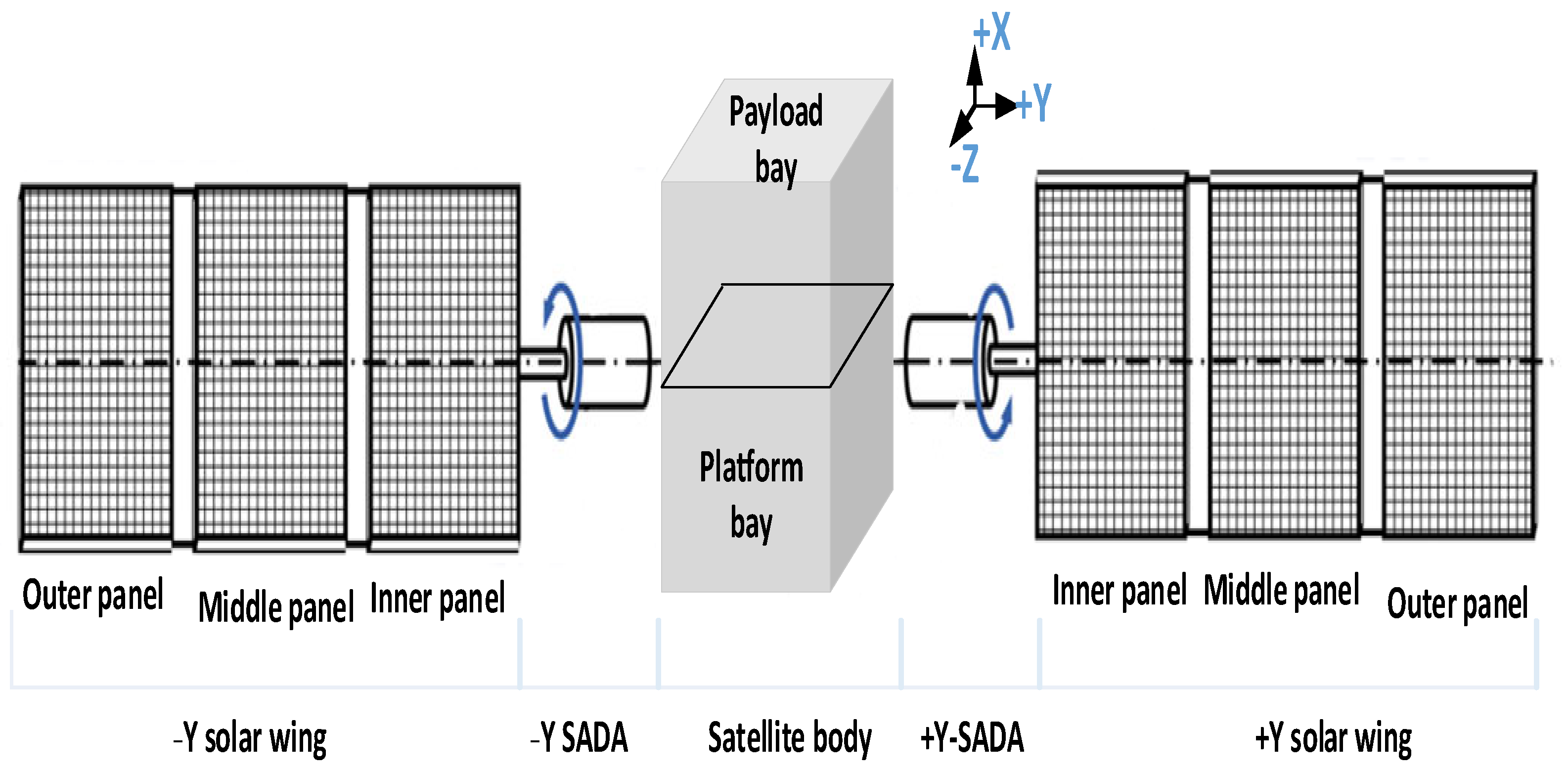
4.1. Overview of the Satellite Status
4.2. Satellite Energy Adaptability Algorithm
5. Conclusions
- The energy of −Y-wing SADA stalls at different angles of the satellite during shadow and light period is verified by simulation.
- The satellite’s energy is in the optimal state, when the SADA of the −Y wing is stalled at 270°. Similarly, it can be concluded that, when the SADA of the +Y wing is stalled at 90°, the energy is also in the optimal state.
- When the −Y-wing SADA is stalled at 270°and the +Y-wing SADA is functioning normally, the satellite energy is balanced. During the shadow period, the battery discharges one more time with a depth of approximately 3% compared to the normal situation within one orbital cycle.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| SADA | The solar array drive assembly |
| SADM_A | +Y-wing solar array drive mechanism |
| SADM_B | −Y-wing solar array drive mechanism |
| SADE | SADA generally consists of the controller circuit box |
| MEO | Medium Earth Orbit |
| Orbital solar angle |
References
- De Asís Delgado, F.; Hernández, P.; Toso, M. MicroSADA-18 Development of One Axis Solar Array Drive Mechanism for Small Satellites. In Proceedings of the 2023 13th European Space Power Conference (ESPC), Elche, Spain, 2–6 October 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Zhu, X.; Li, L.; Mo, Y.; Dong, Y.; Shen, X.; Chen, X.; Qin, S.J. Data-driven approaches for satellite SADA system health monitoring with limited data. In Proceedings of the 2024 IEEE 20th International Conference on Automation Science and Engineering (CASE), Bari, Italy, 28 August–1 September 2024; pp. 3225–3230. [Google Scholar]
- Liang, J.; Zhu, X.; Tong, L.; Zhang, M.; Yu, G. Experimental Research on The Influence of Two-dimensional Deployment of Solar Array on SADA Drive Circuit. In Proceedings of the 2023 IEEE 18th Conference on Industrial Electronics and Applications (ICIEA), Ningbo, China, 18–22 August 2023; pp. 452–457. [Google Scholar] [CrossRef]
- Minow, J.I. Spacecraft Anomalies and Failures Workshop 2023: NASA Introductory Comments. In Spacecraft Anomalies and Failures 2023 Workshop, Langley Research Center, Hampton, Virginia, United States; National Aeronautics and Space Administration and National Reconnaissance Office: Washington, DC, USA, 2023. [Google Scholar]
- Smith, J. Cygnus Solar Array Fails to Deploy (N). SpaceNews Daily, 2022-11-09. Available online: https://www.spacenews.com/cygnus-solar-array-fails-to-deploy/ (accessed on 24 April 2025).
- Leach, R. Spacecraft system failures and anomalies attributed to the natural space environment. In Proceedings of the 1995 Space Programs and Technologies Conference, Huntsville, AL, USA, 26–28 September 1995; pp. 1–17. [Google Scholar]
- Jang, S.S.; Choi, J. Energy balance analysis of small satellite in Low Earth Orbit (LEO). In Proceedings of the 2008 IEEE 2nd International Power and Energy Conference, Johor Bahru, Malaysia, 1–3 December 2008; pp. 967–971. [Google Scholar]
- Yuan, L.; Wang, W.; Qiu, Q.; Xun, W.; Li, C.; Jiang, S. An Accurate Power Balance Analysis and Improved Design Method of the High Orbit Communication Satellites. In Proceedings of the 2023 IEEE 7th Conference on Energy Internet and Energy System Integration (EI2), Hangzhou, China, 15–18 December 2023; pp. 2355–2358. [Google Scholar]
- Gao, Y.; Su, P.; Dong, T.; Liu, X.; Chen, M. Damage Analysis and Energy Evaluation of the Solar Array of the Biaxial Drive Solar Panel. Electron. Meas. Technol. 2021, 44, 68–72. [Google Scholar] [CrossRef]
- Lin, B.J. Functional Chain-Based Engineering of Navigation Satellite Systems; Science Press: Beijing, China, 2021. [Google Scholar]
- Xiong, X.; Zhang, M.; Zhao, H.; Jin, D.; Jia, M. Solar-Array Attenuation Analysis Method for Solar Synchronous Orbit Satellites. In Proceedings of the 2023 14th International Conference on Reliability, Maintainability and Safety (ICRMS), Urumuqi, China, 26–29 August 2023; pp. 232–237. [Google Scholar]
- Fikhtengol’ts, G.M.; Xu, X.; Leng, S.; Liang, W. A Course of Mathematical Analysis; Higher Education Press: Beijing, China, 2006. [Google Scholar]
- Liu, W.; Li, X.; Luo, G. Simulation and Design of Satellite Power Conditioning Unit Based on S4R Technology. In Proceedings of the 2024 6th International Conference on Internet of Things, Automation and Artificial Intelligence (IoTAAI), Guangzhou, China, 26–28 July 2024; pp. 391–395. [Google Scholar] [CrossRef]
- Hou, X.; Zhang, X.; Wang, C.; Wang, M.; Li, Y.; Jiang, S.; Zhang, X.; Li, D.; Hou, R. A High Voltage and High Power PCDU for Space. In Proceedings of the 2023 13th European Space Power Conference (ESPC), Elche, Spain, 2–6 October 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Farahani, G.; Taherbaneh, M. Extracting best reliable scheme for Electrical Power Subsystem (EPS) of satellite. In Proceedings of the 5th International Conference on Recent Advances in Space Technologies—RAST2011, Istanbul, Turkey, 9–11 June 2011; pp. 532–537. [Google Scholar] [CrossRef]
- Pequet, E.; Delporte, P.; Fayt, P.; Gak, M.; Canon, T. ESA qualified EPC for telecommunication satellites TWTA. In Proceedings of the International Vacuum Electronics Conference 2000 (Cat. No.00EX392), Monterey, CA, USA, 2–4 May 2000; p. 2. [Google Scholar] [CrossRef]
- Adnan, S.M.; Bhutto, M.S. Modular & COTS based power system for small LEO satellite. In Proceedings of the 2013 International Conference on Aerospace Science & Engineering (ICASE), Islamabad, Pakistan, 21–23 August 2013; pp. 1–3. [Google Scholar] [CrossRef]
- İnce, B.; Yıldırım, D.; Karagöz, F.E.; Çetin, E.; Şahin, Y. High Power Density Sequential Switching Shunt Regulator Module. In Proceedings of the 2023 13th European Space Power Conference (ESPC), Elche, Spain, 2–6 October 2023; pp. 1–7. [Google Scholar] [CrossRef]
- Şahin, Y.; Çetin, E.; Karagöz, F.E.; İnce, B.; Yıldırım, D. Battery Discharge Regulator Based on Weinberg Topology for High Power Communication Satellites. In Proceedings of the 2023 13th European Space Power Conference (ESPC), Elche, Spain, 2–6 October 2023; pp. 1–7. [Google Scholar] [CrossRef]
- Lin, Z.; Xie, W.; Dong, M.; Lan, J. Research of S3MPR Engineering Application on Space Power Sources. In Proceedings of the 2020 IEEE 4th Information Technology, Networking, Electronic and Automation Control Conference (ITNEC), Chongqing, China, 12–14 June 2020; pp. 817–823. [Google Scholar] [CrossRef]
- Macellari, M.; Palmerini, G.B.; Schirone, L. On the Power System of the AMALIA moon rover. In Proceedings of the 2015 IEEE Aerospace Conference, Big Sky, MT, USA, 7–14 March 2015; pp. 1–8. [Google Scholar] [CrossRef]
- Mohapatra, M.K.; Madhusudhana, C.S.; Biradar, R.C. Solar Array Bus Voltage Regulator for Small Satellite Using FS3R (Fixed Switching String Shunt Regulator) Technique. In Proceedings of the 2024 International Conference on Electronics, Computing, Communication and Control Technology (ICECCC), Bengaluru, India, 2–3 May 2024; pp. 1–6. [Google Scholar] [CrossRef]
- Chen, Q.; Yu, H.; Huang, X.; Lu, Y.; Qiu, P.; Tong, K.; Xuan, J.; Xu, F.; Xuan, X.; Huang, W.; et al. Modelling and analysis of avionics battery discharge regulator. J. Power Electron. 2016, 16, 1218–1225. [Google Scholar] [CrossRef]
- Maset, E.; Ferreres, A.; Ejea, J.; Sanchis-Kilders, E.; Jordan, J.; Esteve, V. 5 kW Weinberg converter for battery discharging in high power communications satellite. In Proceedings of the IEEE 36th Power Electronics Specialists Conference, Dresden, Germany, 16 June 2005; pp. 69–75. [Google Scholar]
- Xi, C.; Liu, Y.; Lin, B.; Wang, X.; Jiang, G.; Kong, C. Radiation-resistant design of triple-junction GaAs solar arrays for high-orbit satellites. J. Sol. Energy 2022, 43, 9–13. [Google Scholar]
- Hoang, B.; Beyene, S.; Keys, C.C.; Watkins, B.; Coughlin, C.; Rotlisberger, L. Solar array performance of a geostationary mission with full electric orbit raising. In Proceedings of the 2020 47th IEEE Photovoltaic Specialists Conference (PVSC), Calgary, ON, Canada, 15 June–21 August 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 0056–0060. [Google Scholar]
- Rehman, A.; Lee, S.H.; Lee, S.H. Silicon space solar cells: Progression and radiation-resistance analysis. J. Korean Phys. Soc. 2016, 68, 593–598. [Google Scholar] [CrossRef]
- Zhang, X.; Zuo, Z. Analysis on Solar Cell Degradation Characteristic of MEO Satellite. Spacecr. Eng. 2014, 23, 75–80. [Google Scholar]
- Tu, J.; Liang, G.; Xie, D. Influence on power output of solar cells with small area of shadow. Chin. J. Power Sour. 2014, 38, 474–477. [Google Scholar]
- Hui, Y.; Yanhui, P.; Xiaoying, L.; Wenming, G. Degradation assessment of solar cell array of low orbit satellite in orbit. In Proceedings of the 2022 IEEE International Conference on Electrical Engineering, Big Data and Algorithms (EEBDA), Changchun, China, 25–27 February 2022; pp. 21–23. [Google Scholar] [CrossRef]
- Haining, M.A.; Xiang, Z.; Wenhe, L. Calculation of average power generated by solar array of CubeSat. China Sci. 2018, 13, 2669–2703. [Google Scholar]
- Piszczor, M.F.; Benson, S.W.; Scheiman, D.A.; Snyder, D.B.; Fincannon, H.J.; Oleson, S.R.; Landis, G.A. Advanced solar cell and array technology for NASA deep space missions. In Proceedings of the 2008 33rd IEEE Photovoltaic Specialists Conference, San Diego, CA, USA, 11–16 May 2008; IEEE: Piscataway, NJ, USA, 2008. [Google Scholar]



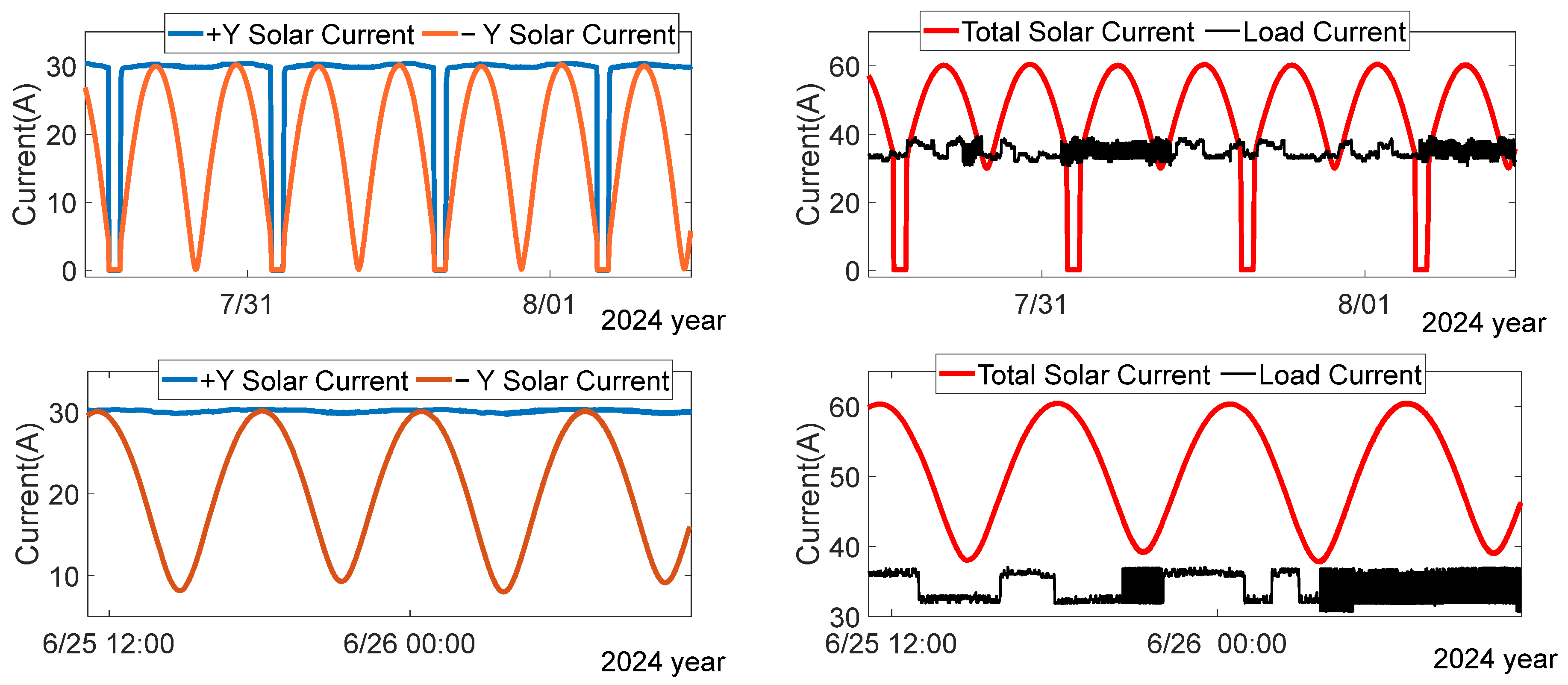
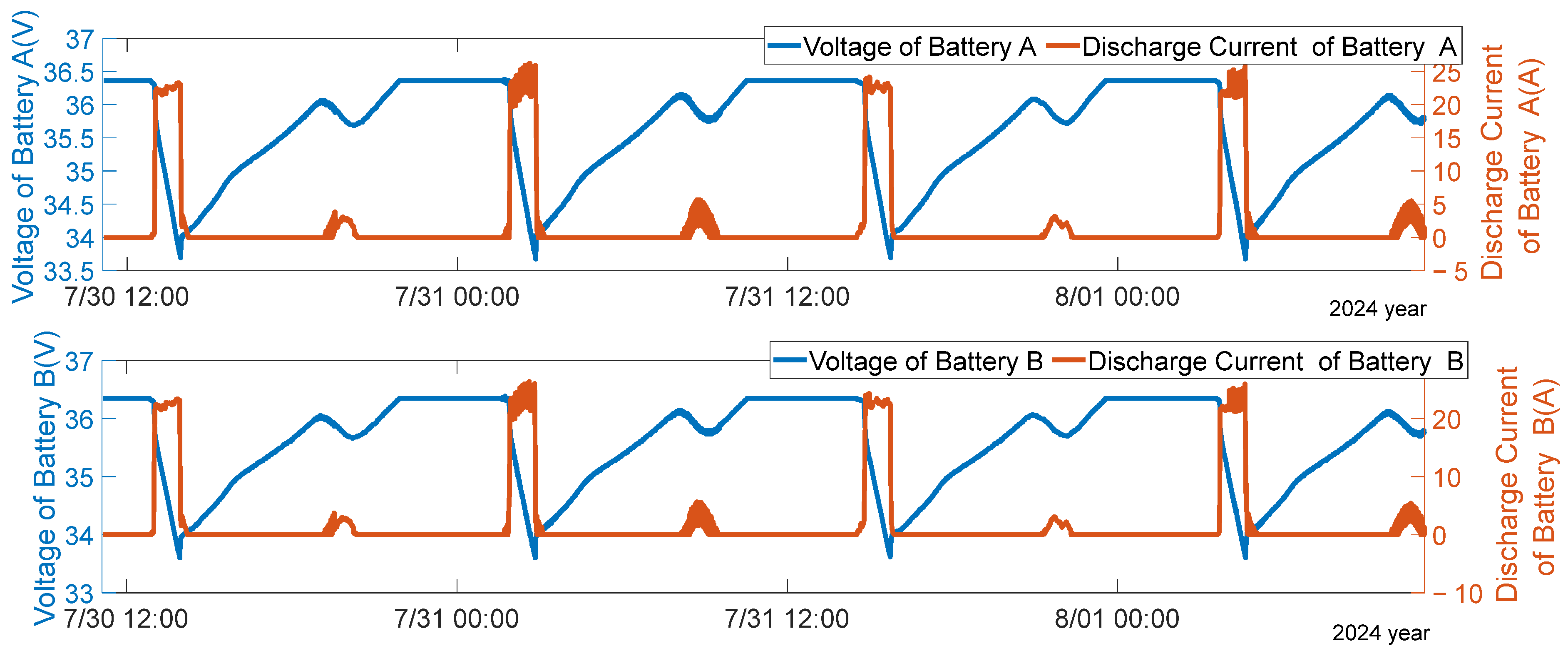
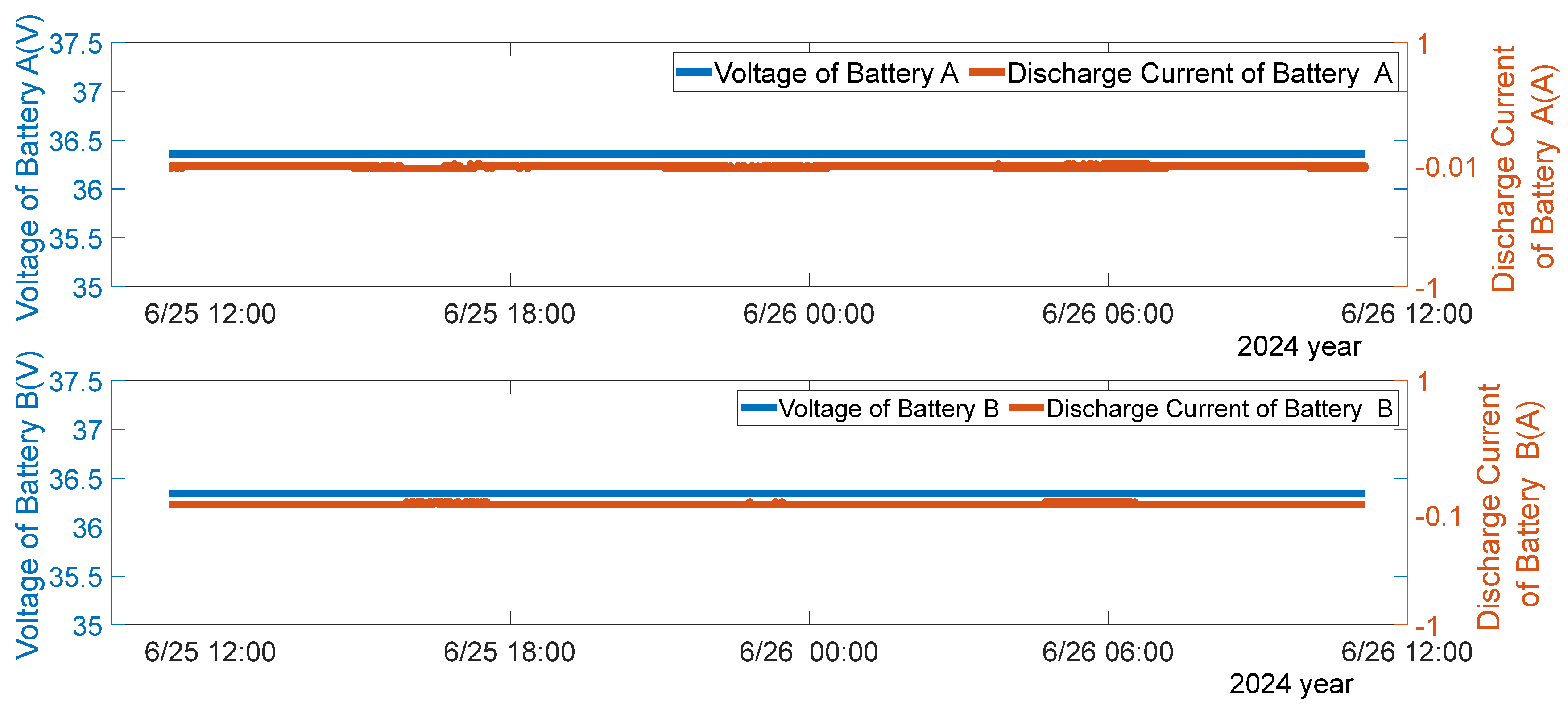





| Working Condition | (Wh) | (Wh) | (Wh) | In Area1 (Wh) | In Area2 (Wh) | The Depth of Discharge in Area1 | The Depth of Discharge in Area2 | Energy Balance | Battery Discharge |
|---|---|---|---|---|---|---|---|---|---|
| Simulation (Shadow Period) | 6224 | 4843 | 1729 | 1577 | 152 | 28% | 3% | Yes | Yes |
| on-orbit results (Shadow Period) | 7668 | 5825 | 1580 | 1500 | 80 | 26.6% | 1.6% | Yes | Yes |
| Simulation (Light Period) | / | / | / | / | / | / | / | Yes | No |
| on-orbit results (Light Period) | / | / | / | / | / | / | / | Yes | N0 |
| Satellite Working Mode | SADA Target Rotation Angle | Solar Wing Control Flow |
|---|---|---|
| Uncontrolled, solar capture, earth capture, and minimum safety modes | +Y solar wing: 0° −Y solar wing: 0° | SADA operating mode is auto-zero. Entering no control when the solar wing is not deployed, autonomously deploying the solar wing |
| Orbit-controlling mode | +Y solar wing: 90° −Y solar wing: 270° | SADA operating mode is incremental, pointing the solar wing normal to the satellite + X-axis. |
| Stabilized ground, yaw maneuver mode | Calculation of the solar wing target rotation angle from the current orbital system sun vector | Calculate the solar wing rotation angle deviation based on the target angle. Greater than 5° in incremental mode, up to 3° in cruise mode. |
| Isc | Note | |
|---|---|---|
| 50 | Iorthophoto | For data located between two corners, the calculation is based on the linearity of the difference between the two corners. |
| 55 | Iorthophoto | |
| 60 | Iorthophoto | |
| 65 | Iorthophoto | |
| 70 | Iorthophoto | |
| 75 | Iorthophoto | |
| 80 | Iorthophoto |
| (°) | (Wh) | (Wh) | (Wh) | In Area1 (Wh) | In Area2 (Wh) | The Depth of Discharge of the Battery in Area1 | The Depth of Discharge of the Battery in Area2 | Energy Balance |
|---|---|---|---|---|---|---|---|---|
| 270 | 6224 | 4843 | 1729 | 1577 | 152 | 28% | 3% | Yes |
| 330 | 4886 | 3663 | 2830 | / | / | / | / | Yes |
| 345 | 4033 | 3036 | 3193 | / | / | / | / | No |
| 360 | 3095 | 2410 | 3546 | / | / | / | / | No |
| (°) | (Wh) | (Wh) | Energy Balance | Battery Discharge |
|---|---|---|---|---|
| 270 | / | / | Yes | No |
| 330 | 4076 | 1505 | Yes | Yes |
| 345 | 3237 | 1910 | Yes | Yes |
| 360 | 2448 | 2311 | Yes | Yes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, C.; Liu, H.; Lin, B.; Wang, X.; Zhang, Q.; Wang, Y. Energy Adaptability Analysis Based on the Stall Fault of Solar Array Drive Assembly for Medium Earth Orbit Satellite. Energies 2025, 18, 2315. https://doi.org/10.3390/en18092315
Kong C, Liu H, Lin B, Wang X, Zhang Q, Wang Y. Energy Adaptability Analysis Based on the Stall Fault of Solar Array Drive Assembly for Medium Earth Orbit Satellite. Energies. 2025; 18(9):2315. https://doi.org/10.3390/en18092315
Chicago/Turabian StyleKong, Chenjie, Huan Liu, Baojun Lin, Xueliang Wang, Qiang Zhang, and Yabin Wang. 2025. "Energy Adaptability Analysis Based on the Stall Fault of Solar Array Drive Assembly for Medium Earth Orbit Satellite" Energies 18, no. 9: 2315. https://doi.org/10.3390/en18092315
APA StyleKong, C., Liu, H., Lin, B., Wang, X., Zhang, Q., & Wang, Y. (2025). Energy Adaptability Analysis Based on the Stall Fault of Solar Array Drive Assembly for Medium Earth Orbit Satellite. Energies, 18(9), 2315. https://doi.org/10.3390/en18092315





