Galloping Performance of Transmission Line System Aeroelastic Model with Rime Through Wind-Tunnel Tests

Abstract

This study presents an experimental investigation for the galloping performance of the transmission line system with rime under wind excitation. A full aeroelastic model wind-tunnel test is conducted to investigate the dynamic response of a two-bundled transmission line system with rime under different conditions. The time histories of the displacement of the conductor and the acceleration of the tower are measured in detail to analyze the characteristic of the wind-induced response. A comprehensive parametric experiment is performed to explore the effects of wind speed, wind direction, the number of conductor spans and the coupling between the conductor and the tower on the galloping performance of the transmission line system with rime. The results showed that the wind speed, wind direction and the number of conductor spans have significant influence on the galloping performance of conductor. The zero-degree wind direction is the most dangerous direction for the conductor. The multi-span conductor has different galloping initiation wind speed and vibration characteristics compared to the single-span conductor. The coupling effect between the conductor and the tower has trivial influence on the response of tower. This study uses 3D-printing models to simulate the aerodynamic shape of ice-covered wires with different thicknesses for wind-tunnel tests and obtains the influence of a series of parameters on the galloping vibration of transmission tower line systems.

1. Introduction

With the increasing application of ultra-high voltage transmission lines, multi bundle conductors have been applied in practice because they can reduce line inductance and corona discharge (Song et al. [1]). The collapse or the trips of lines is common under wind loads, resulting in considerable economic losses. Therefore, evaluating the wind-induced response of transmission line systems is crucial. When the cross-section shape of the iced or rimed conductor becomes non-circular under climate conditions such as snow, storm and freezing rain, the nonlinear aerodynamic excitation introduces a galloping vibration of the conductor. About 679 power outages due to severe weather were reported during 2003 to 2012 (Azzi et al. [2]). Assessing the performance of wind-excited transmission line system is very complicated due to the nonlinear aerodynamic character of the galloping of multi-bundled conductors with rime under strong wind.

Until now, many researchers have examined the aerodynamic properties of iced conductors. Blevins and Iwan [3] developed the theoretical model of coupled in-plane and torsional galloping and conducted a comprehensive analysis of the galloping performance under resonant and non-resonant conditions. They made significant contributions to the understanding of galloping in iced conductors by developing a theoretical model that couples in-plane and torsional vibrations. Their work was pivotal in analyzing the galloping behavior of iced conductors, particularly under both resonant and non-resonant conditions. Jones [4] proposed a mathematical model of the in-plane galloping of iced conductors by the Hamilton principle. Liu et al. [5] developed three types of theoretical models of different coupled galloping by the Hamilton principle to describe the nonlinear interactions between vibrations in different directions. Liu et al. [6] proposed a theoretical model of the coupled in-plane and out-of-plane galloping, which shows the significant influence of the out-of-plane motion on the galloping. Wu and Chen [7] proposed an analytical framework to investigate the three-dimensional coupled galloping for multi-span four-bundled conductors. Numerical simulation is also an important method to study the complex nonlinear characteristics of galloping of conductors. Desai et al. [8] developed a perturbation-based finite element method to investigate the galloping vibration of the iced conductor, and Yan et al. [9] further investigated the galloping performance of the iced quad bundled conductors using the method. Braun and Awruch [10] investigated the wind flow over multi-bundled conductors and calculated the corresponding wind loads. Multi-bundled conductors are often used in high-voltage transmission lines to improve efficiency and reduce electromagnetic interference. However, their complex geometry makes them more susceptible to aerodynamic effects, such as wind-induced vibrations and galloping. Hu et al. [11] and Zhou et al. [12] investigated the galloping of iced conductor by the ABAQUS software and proposed a customer-defined cable element to better simulate the galloping of iced eight-bundled conductors.

The wind-tunnel experiment is also a very important method to investigate the wind-induced performance of the transmission line system. Loredo and Davenport [13] proposed a modeling approach for conductors and examined the effect of turbulence on the response of conductors. Xie et al. [14] and Xu et al. [15] measured the aerodynamic force coefficients of multi-bundled conductors under different conditions. Lou et al. [16] explored the galloping response of iced conductors in wind-tunnel test and examined an aerodynamic anti-galloping technique. These studies on multi-bundled conductors by a wind-tunnel test employed rigid models of conductors from which the aerodynamic force coefficients can be obtained to investigate the stability of galloping and establish the self-excited force model for theoretical study. However, the aeroelastic effect usually cannot be considered in the rigid models. The aerodynamic force of conductors is the key factor for the occurrence of galloping vibration of the conductor. Therefore, the aeroelastic model wind tunnel is more desirable to obtain more wind performance of transmission line system. Huo et al. [17] examined the galloping response of a single-span iced conductor to validate the theoretical analysis. Gao et al. [18] measured the displacements of a single-span iced conductor in different directions through an aeroelastic model wind-tunnel test. However, the transmission tower-line system usually covers for several kilometers with many spans. Liang et al. [19] studied the influence of the coupling effect between the tower and the line on the wind-induced vibration of the tower through aeroelastic model experiments. The performance of wind-excited multi-span conductors could be different from that of single-span conductors (Song et al. [1]). Lou et al. [20] investigated the wind-induced responses of an eight-span transmission line system through the finite element model. It is stated that the wind-induced response of multi-span conductors cannot be accurately estimated by the single-span conductor. Song et al. [1] investigated the buffeting response of the wind-excited multi-span multi-bundled transmission lines without ice through an aeroelastic wind model test. Azzi et al. [21] examined the buffeting response of the transmission tower with lines by the wind-tunnel test. Cui et al. [22] investigated the galloping performance of iced bundled conductors without a tower through the aeroelastic model wind-tunnel test. It was found that the aeroelastic model wind-tunnel test for the transmission tower and conductor simultaneously is difficult to conduct.

This study investigates the galloping performance of wind-excited single-span and multi-span, multi-bundled transmission line system with rime. A full aeroelastic model of the two-bundled transmission line system with rime is fabricated according to the similarity criteria. The design of the line model adopts the transmission line aeroelastic model equivalent design method. The time histories of displacement of the conductor and the acceleration of the tower are measured to characterize the wind-induced response of the transmission line system under wind load. A comprehensive parametric experiment is performed to explore the effects of wind speed, wind direction, the number of conductor spans and the coupling between the conductor and the tower on the galloping performance of the transmission line system with rime. The results of this study help in developing improved understanding of the galloping performance of the transmission line system with rime through a full aeroelastic model wind-tunnel test.

2. Aeroelastic Model and Wind-Tunnel Test

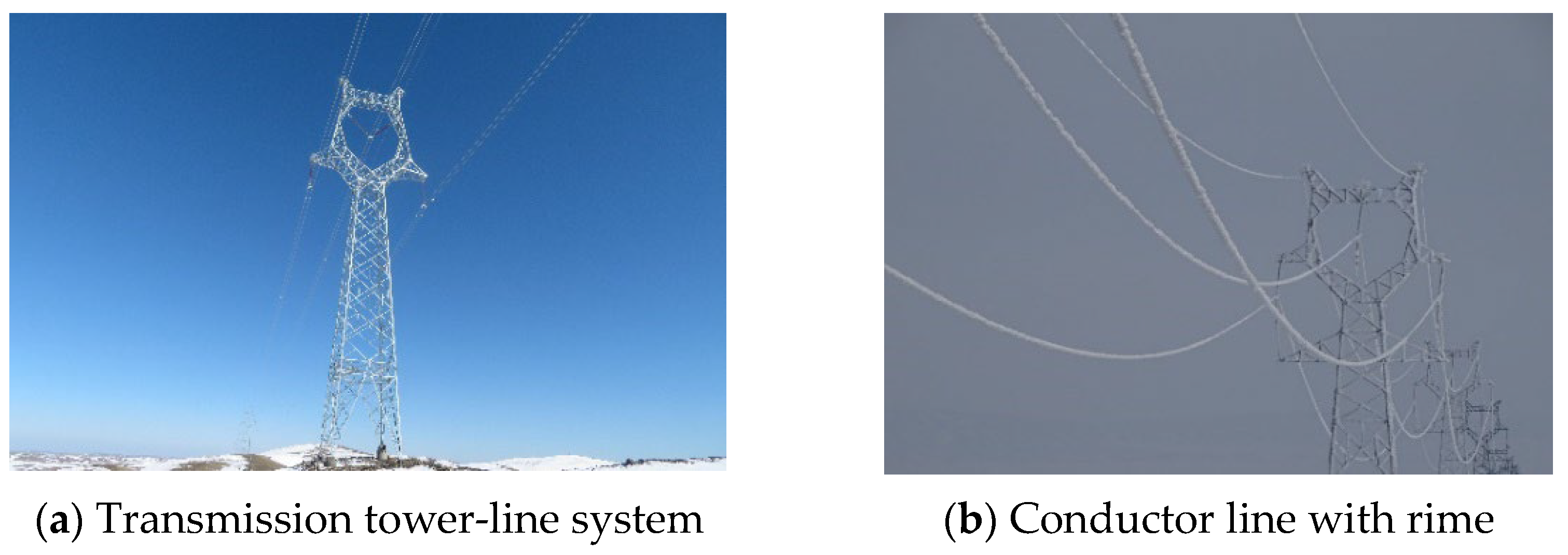
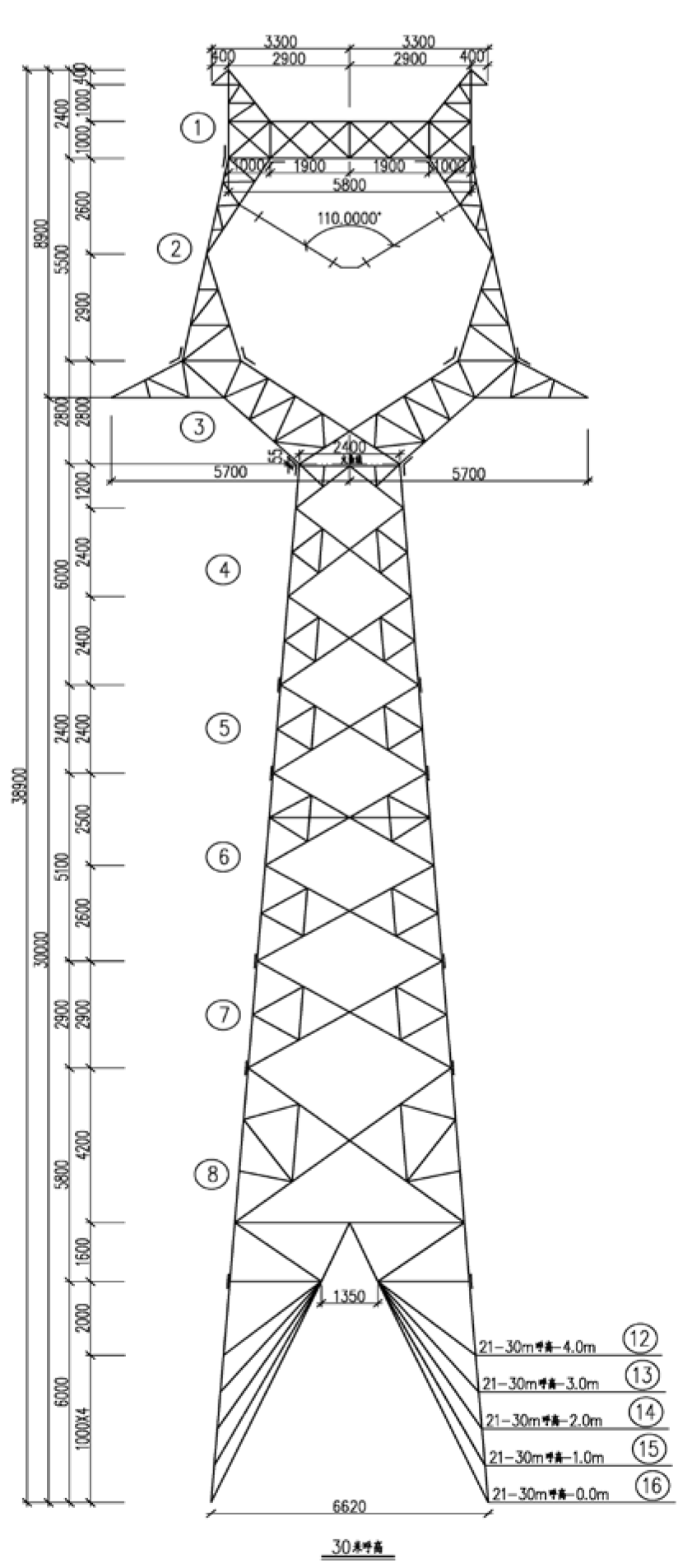
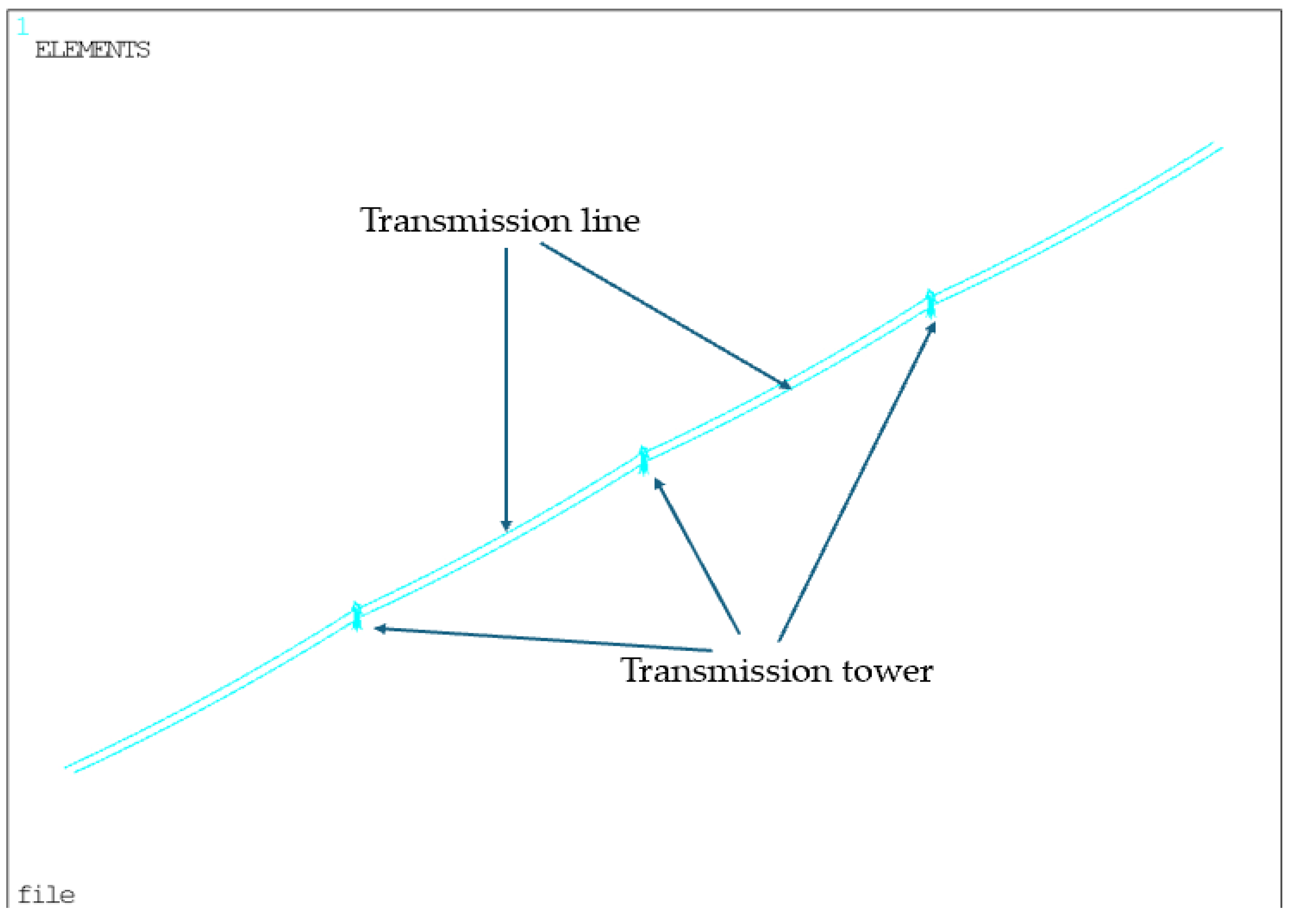
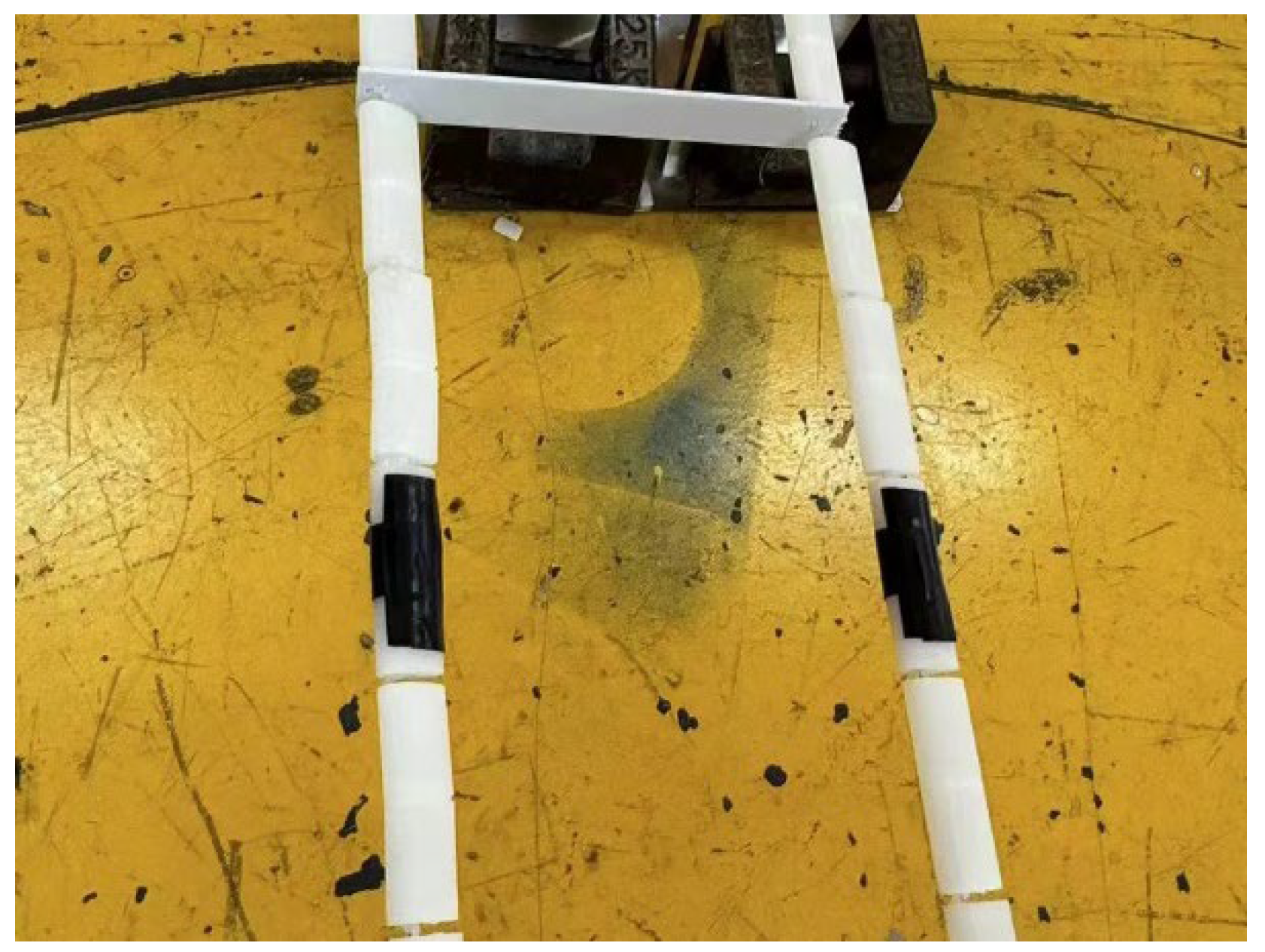
2.1. Aeroelastic Model

The prototype of the experimental model adopts the transmission tower line system of the 200 kV transmission line in Xinjiang, as shown in Figure 1. The transmission tower is a lattice style cat head tower, with a height of 29.9 m and a nominal height of 21 m, as shown in Figure 2. The horizontal span is 400 m, and the conductor sag is 9.68 m. The wire is divided into three phases, with the phase lines on both sides arranged horizontally and suspended on the cantilever of the tower through insulators. The intermediate phase line is suspended in the middle of the locomotive through insulators. Each phase is equipped with 2XJL/G1A-400/35 wires, with a split spacing of 200 mm and I-shaped gaskets. According to the similarity theory, an aeroelastic model was created, as shown in Figure 3. In the model design of tower line systems, not only must the structure and all components strictly meet geometric similarity, but the most important parameters, such as Strouhal number, Froude number, elastic parameters (Cauchy number), inertial parameters (mass parameters), damping ratio, etc., must also strictly meet similarity. The similarity of Reynolds numbers is clearly unsatisfactory.

However, since all components of the transmission tower are made of angle steel, the separation points of the surrounding flow field are not affected by the difference in Reynolds numbers, and relaxing the requirement for Reynolds number similarity will not result in significant errors in the test results.

The Wind Engineering and Wind Environment Research Center of Changsha University of Science and Technology has an advanced large-scale boundary layer wind-tunnel laboratory in China, covering an area of 4000 square meters. The aerodynamic profile of the wind tunnel is 75 m long and 31 m wide. The main dimensions of the test section are 10.0 m × 3.0 m × 21.0 m, with a wind speed range of 1.0~18.0 m/s, suitable for large-span aeroelastic model testing.

The wind-tunnel test was conducted in wind tunnel of Changsha University of Science and Technology with 10 m width, 3 m height and 21 m length. According to the geometric size of the wind tunnel, the 1:20 geometric scale of the model was selected so that a three tower two-span full aeroelastic model of tower-line system was established according to the similarity theory. For the space-lattice-type tower, its overall stiffness, mass and damping mainly depend on the materials and dimensions of the spatial bars, node plates and tower base constraints. In order to reflect the dynamic characteristics of the tower, the stiffness and mass distribution of the aeroelastic model tower should be similar to that of the prototype tower. The similarity of the aeroelastic models of transmission towers is shown in

Table 1. Similarity ratios of aeroelastic model of transmission tower.

Parameter	Symbol	Unit	Similarity Ratio
Length	$L$	m	λL = 1:20
Wind speed	$U$	m/s	λU = 1:2.3
Frequency	$f$	Hz	λf = λU/λL = 8.696:1
Mass per unit length	$m$	kg/m	λm = (λL)2 = 1:202
Stiffness	$EA$	N	λEA = (λL)2(λU)2 = 1:2116
Damping ratio	$\zeta$	/	λζ = 1

.

Compared to transmission lines and transmission towers, insulators are smaller, but their stiffness is higher. The insulators are considered as the two-force members with both ends being hinged. The red copper tube is adopted as the mandrel, and the umbrella-shaped ABS plastic plate with 10 mm intervals is adopted as the wave-shaped appearance of the insulator.

The roughness of the ice surface will significantly alter the boundary layer characteristics of the airflow on the wire surface, affecting the distribution of aerodynamic forces. The roughness of ice surface may cause irregular fluctuations in lift coefficient, increasing the complexity and uncertainty of aerodynamic response. Pneumatic torque variation: The roughness of the ice surface may alter the aerodynamic torque of the wire cross-section, affecting the torsional response of the wire. The roughness of the ice surface may alter the aerodynamic damping characteristics of the wire, increasing the risk of galloping. The roughness of the ice surface may affect the dancing characteristics of the conductor, increase the amplitude and frequency of vibration and lead to fatigue damage of the conductor. The roughness of the ice surface has a significant impact on the aerodynamic response and vibration of iced conductors.

The rime on the conductor lines is modeled as the crescent-shaped section with the angle of attack of 0 degree and shown in Figure 4. The models are made of resin by 3D-printing technology and are placed evenly every meter along the conductors. The model of the rime shape is to determine the aerodynamic wind load on the conductors with rime, and the model parameters are shown in

Table 2. The design parameter of conductors with rime (20 mm).

Rime Area	Rime Mass	Mass of Line with Rime	Mass
			Standard Model	Equivalent Model
0.000421 ${\mathrm{m}}^2$	378.8761 g	1726.376 g	4.316 g	28.773 g

.

In order to test the frequencies and the damping ratio of the model, before the wind-tunnel test, the dynamic characteristics of the aeroelastic model of the tower and lines are calculated by ANSYS 19.1 (Figure 5). As a highly flexible structure, the guide (ground) wire mainly bears tensile force, and its compressive and bending bearing capacity can be basically ignored. This article uses the three-dimensional pole element LINK10 to simulate transmission lines, which can achieve structural nonlinearity, stress stiffening, large deformation and other characteristics. By using keyopt (3) = 0 to set the unit to only be in tension, wire relaxation can be simulated when the wire loses tension. When conducting finite element modeling, in order to obtain sufficient accuracy and ensure model calculation efficiency, it is necessary to divide the suspension cable into a sufficient number of elements and concentrate the load on the nodes at both ends of the elements. Therefore, it is chosen to set up a unit every 10 m when dividing the transmission line units. The frequencies of the model are shown in

Table 3. The frequency of the tower (Hz).

Mode		Aeroelastic Model	Theoretical Value	Error
Longitudinal direction	First mode	22.78	23.01	−1.00%
	Second mode	58.12	64.56	−9.98%
Transverse direction	First mode	24.79	23.26	6.58%
	Second mode	59.98	66.33	−9.58%

and

Table 4. Frequency of conductors (Hz).

Mode	Aeroelastic Model	Theoretical Value	Error
First mode (out-of-plane)	1.45	1.54	−5.8%
First mode (in-plane)	1.94	1.85	4.86%

.

2.2. Wind-Tunnel Test

The displacements for single- and two-span conductors under different wind speeds and wind directions were tested. The accelerations of tower with and without lines are also tested. The angle of attack of the rime is 0° for all cases. The in-plane and out-of-plane accelerations of the tower model in the wind-tunnel test is obtained by the micro-acceleration sensor. The displacements of the tower and conductors are obtained by the non-contact video displacement meter. The arrangement of the acceleration sensors is shown in Figure 6. The target of the non-contact video displacement meter is shown in Figure 7. There are a total of nine target papers for conductors in one span. In the experiment, the in-plane and out-of-plane displacements of the conductor were measured directly by the non-contact video displacement meter to delineate the characteristics of galloping. Through further data processing, the dynamic responses of the tower and the conductor in the in-plane and out-of-plane direction at different positions and different wind speeds could be visualized, including the time history response, spectral response and the trajectory response. The wind direction α is defined in Figure 8.

3. Experiment Results of the Conductors

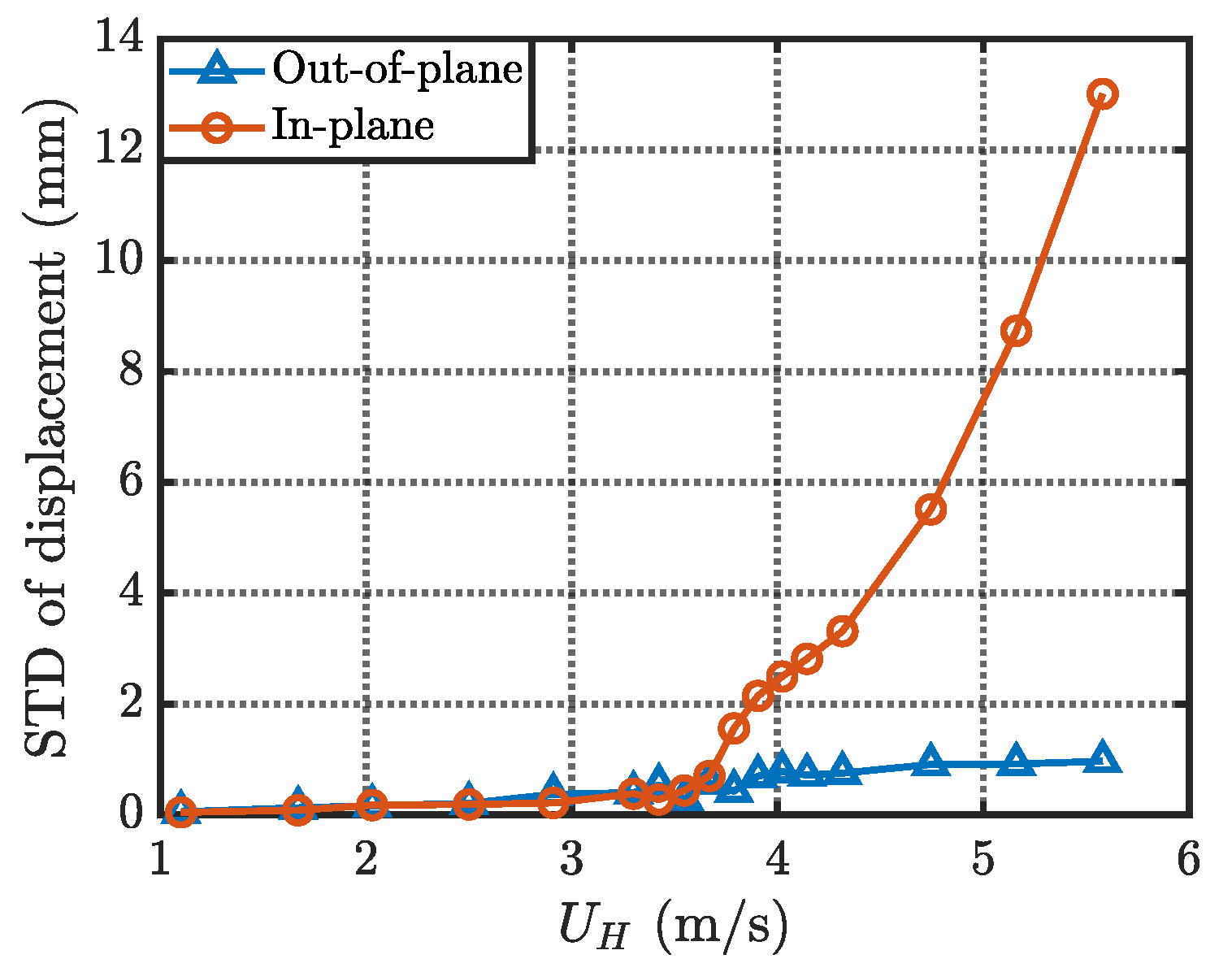
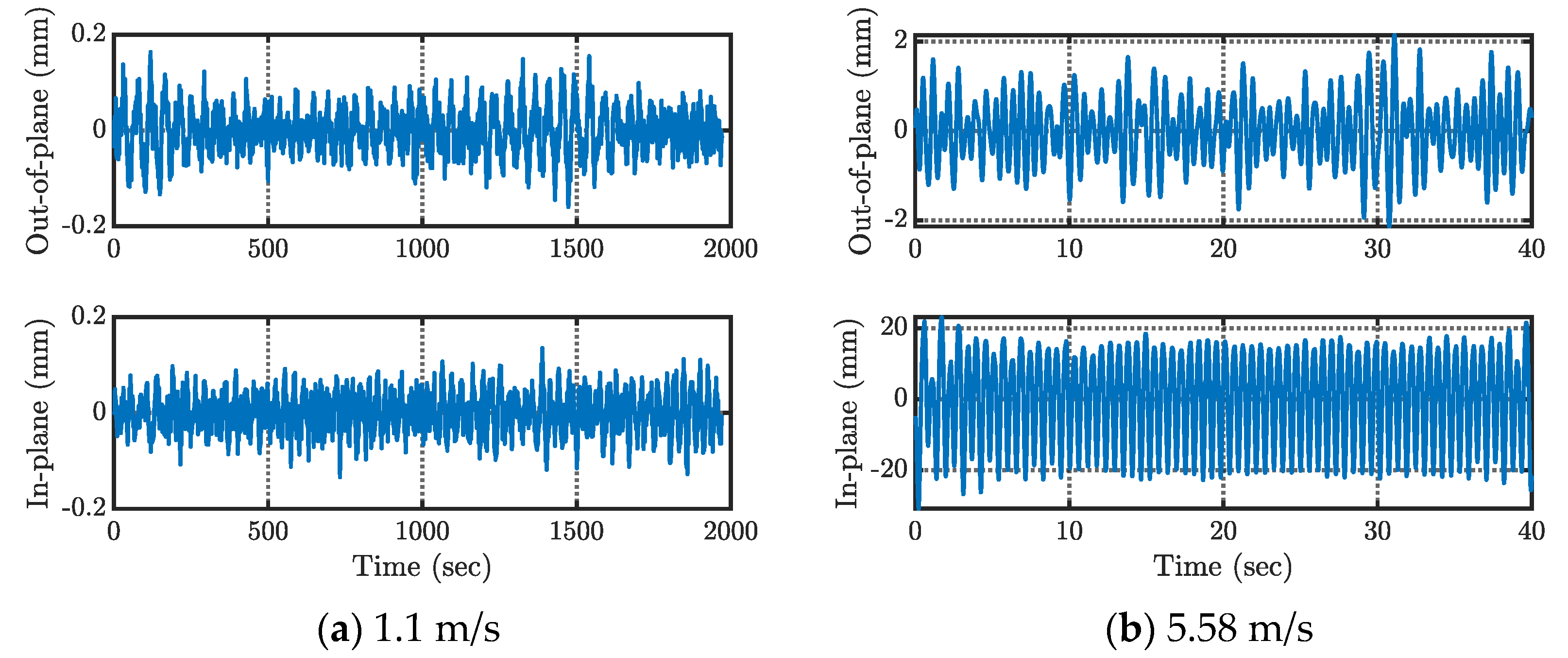
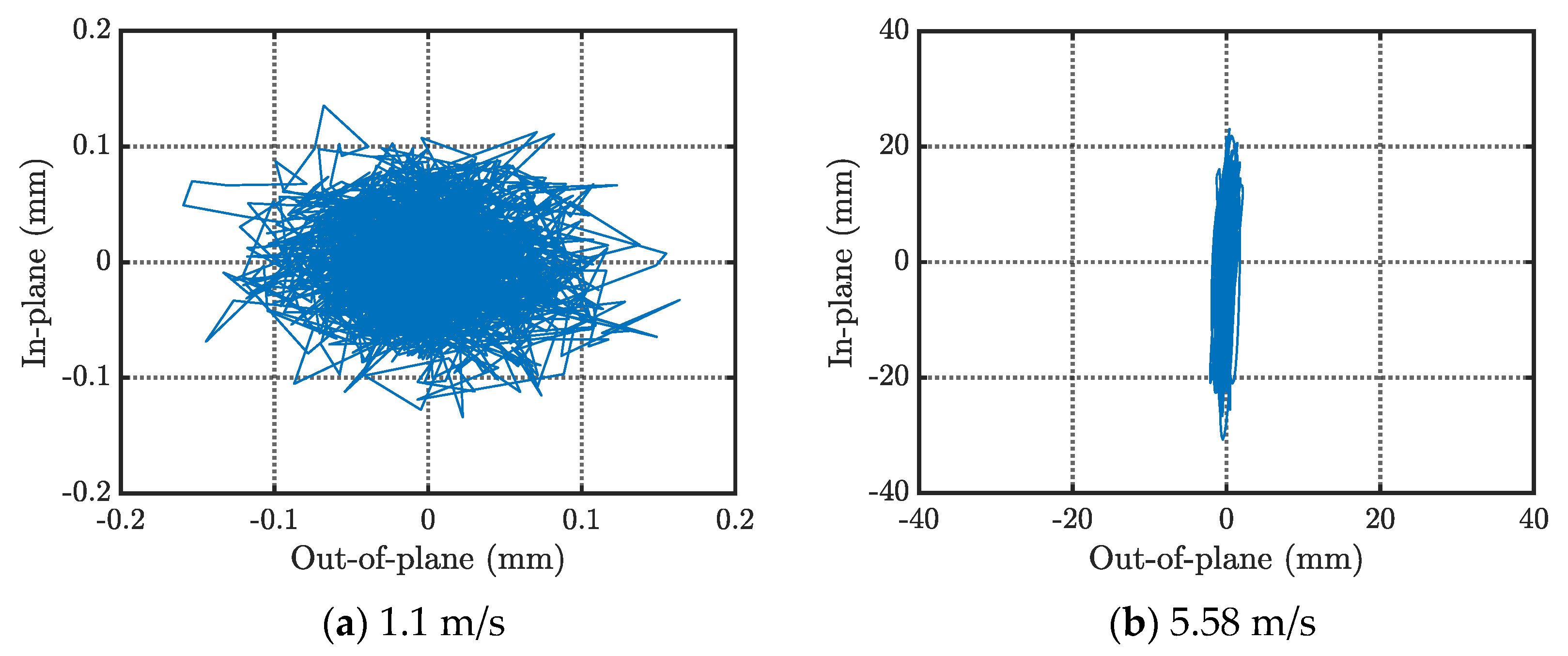
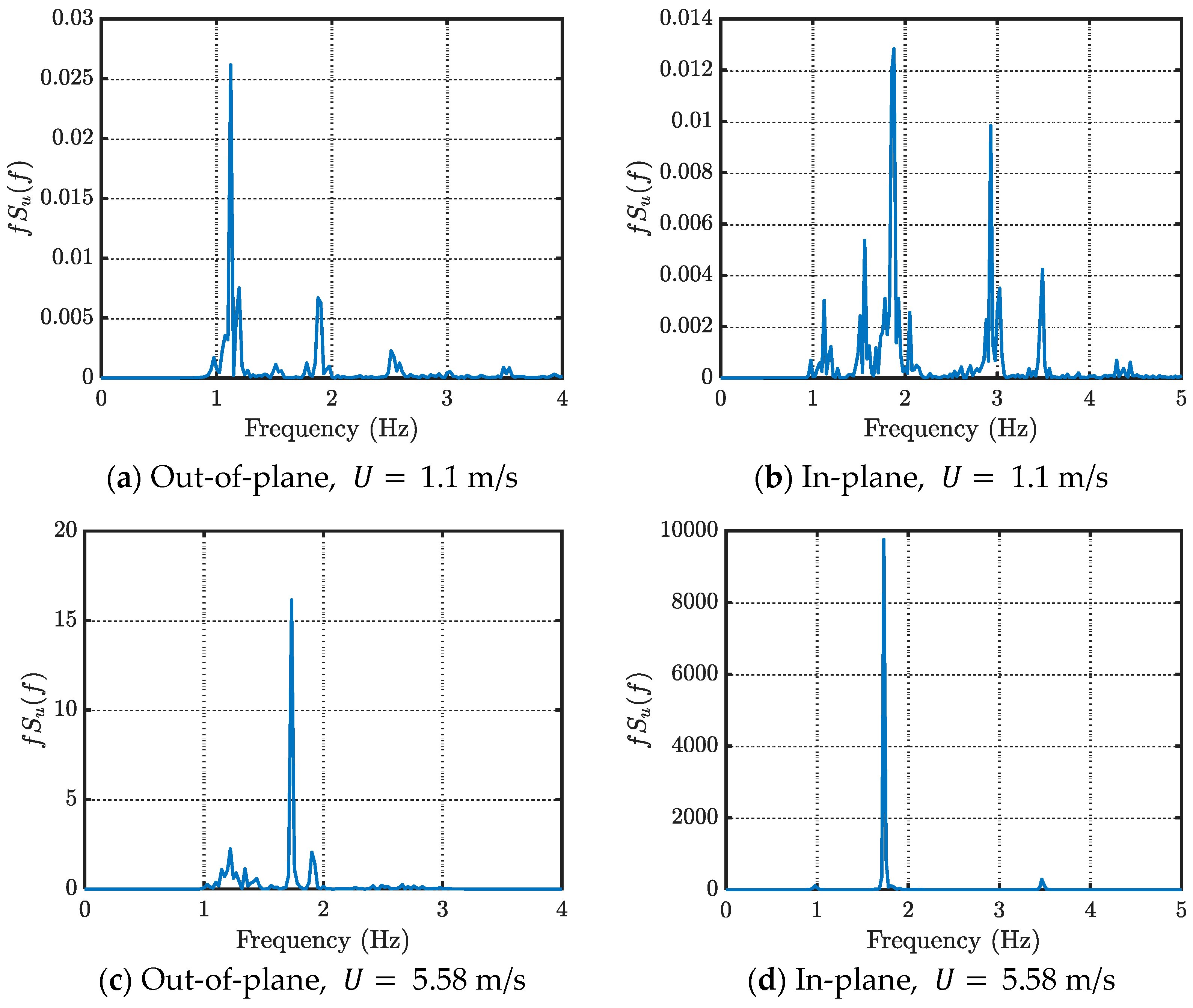
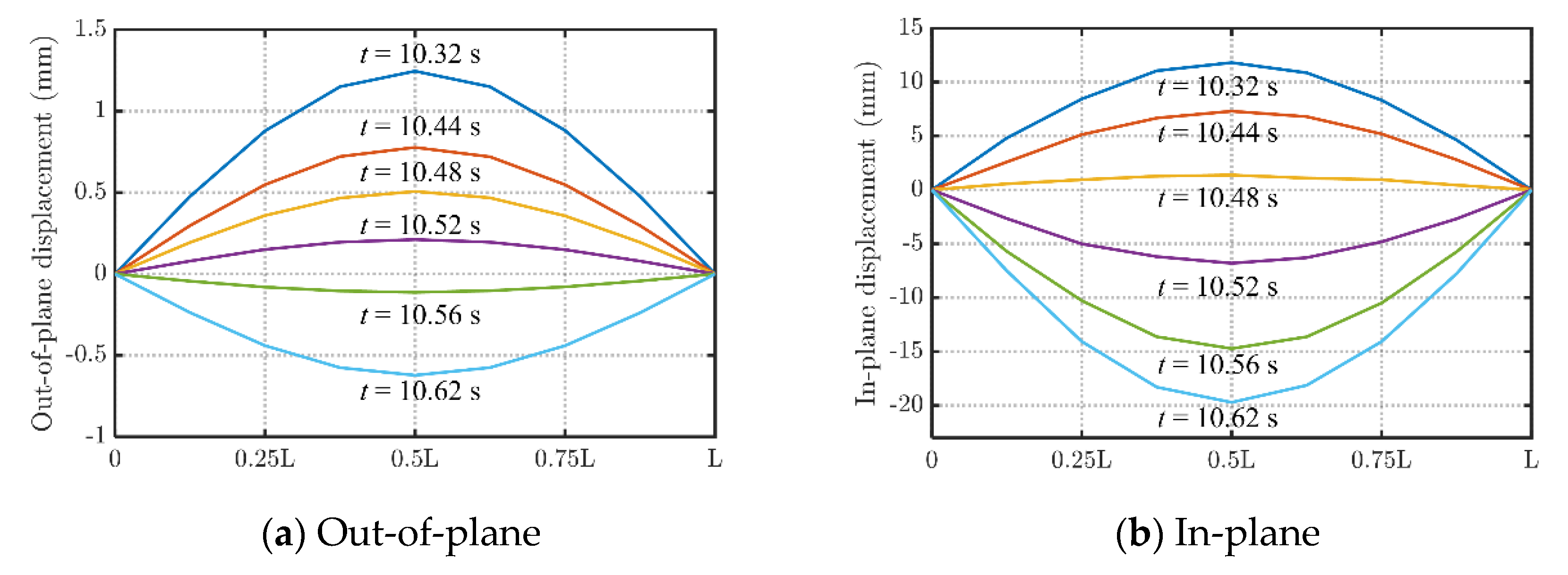
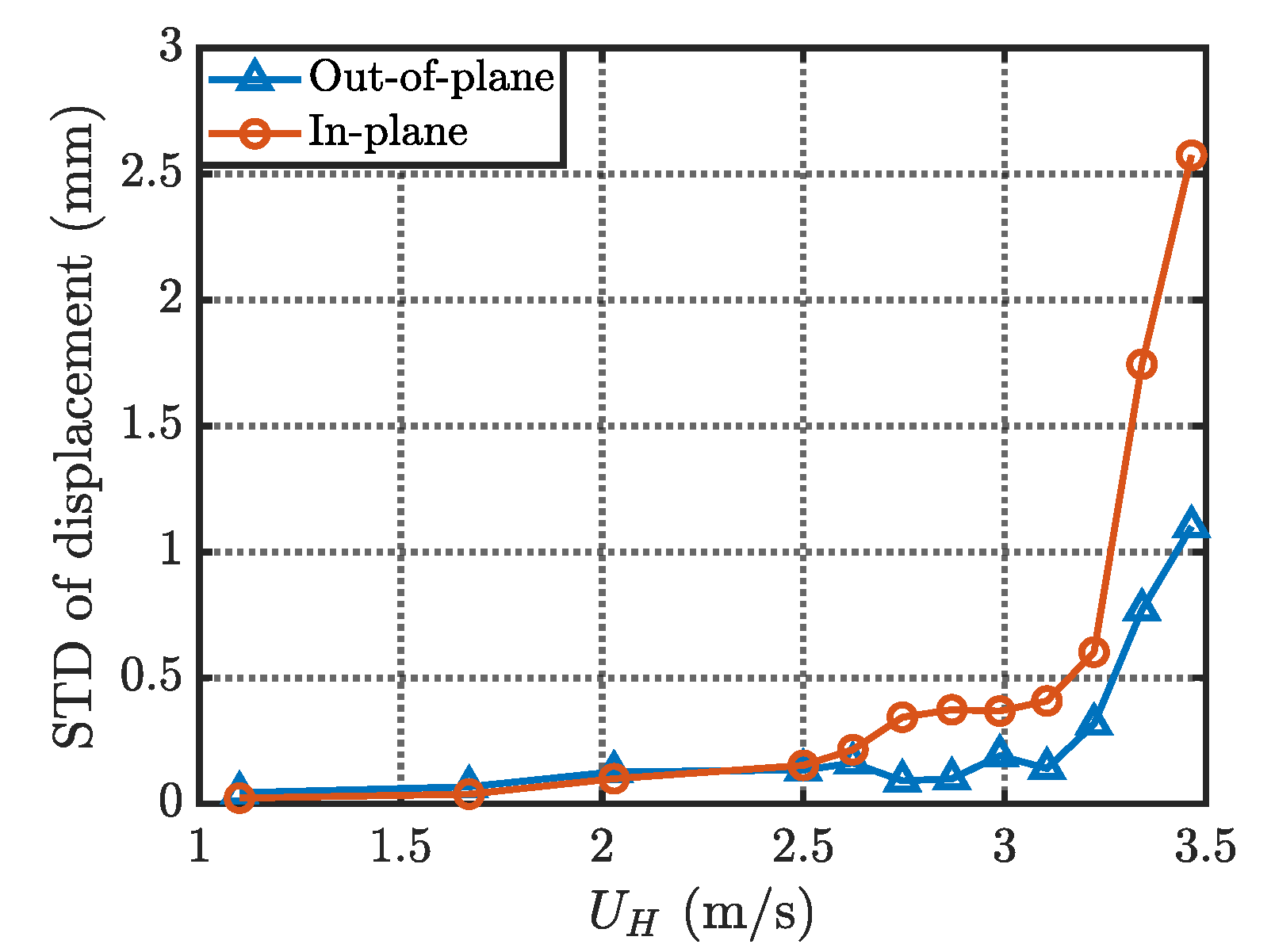
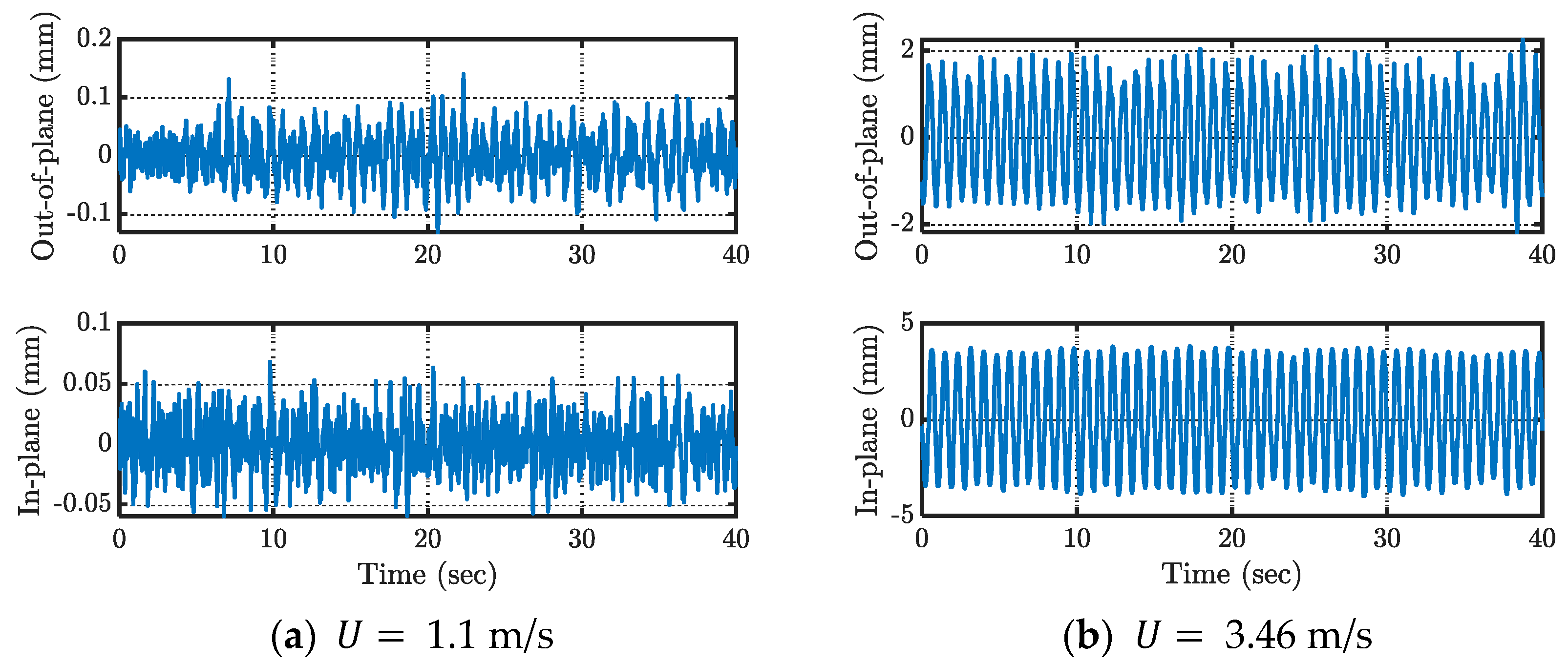
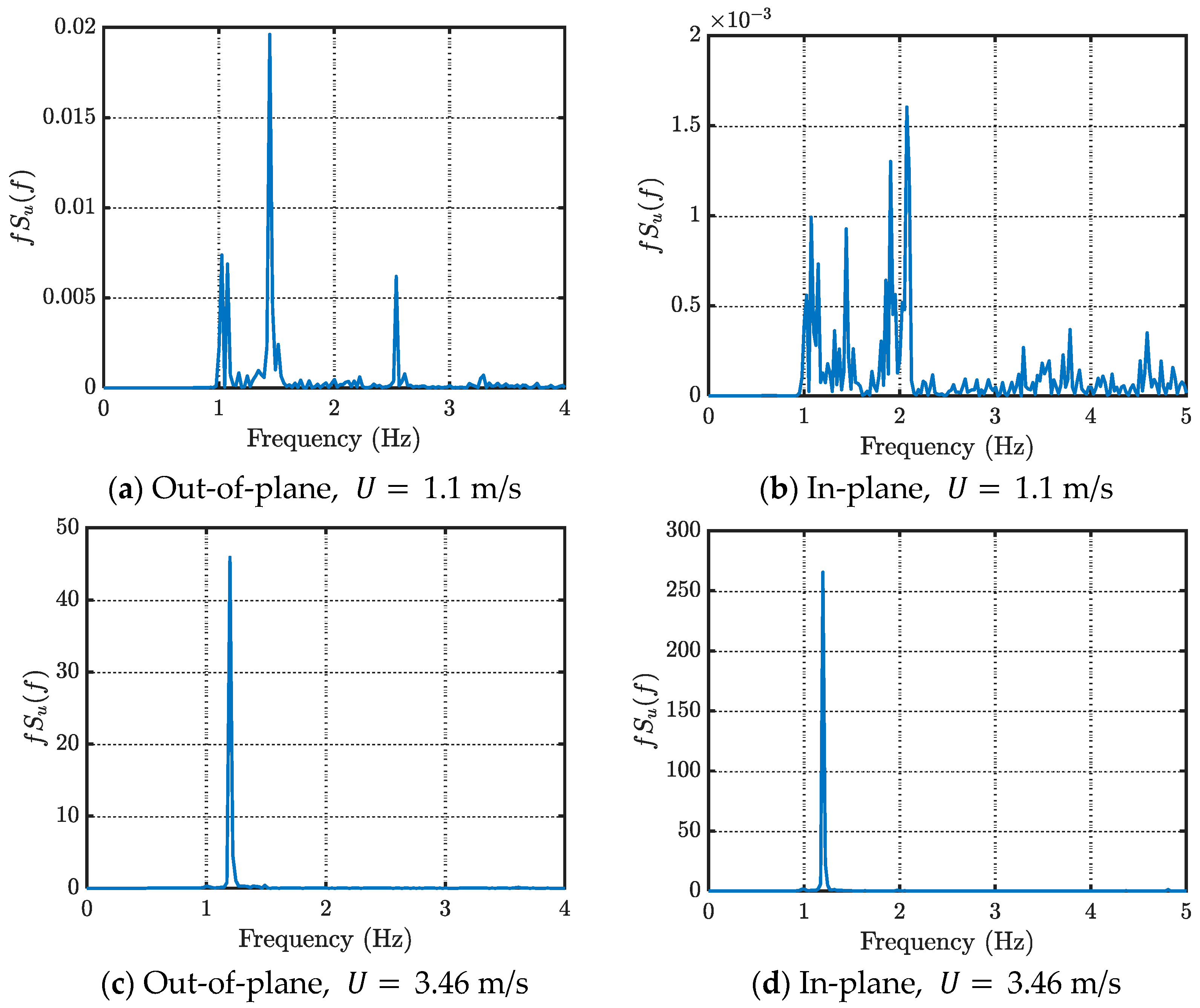
3.1. Response of Single-Span Conductors

Figure 9 shows the variation of the STDs of the midspan displacements of the conductor lines with wind speed at wind direction α = 0°. It is shown that the galloping initiation wind speed is 3.8 m/s, and the vibration is dominated by the in-plane galloping. Figure 10 shows the displacement time histories of the midspan of conductor. At a wind speed of 1.1 m/s, the vibration of conductor is stochastic and is dominated by buffeting vibration in both in-plane and out-of-plane directions. At a wind speed of 5.58 m/s, the galloping vibration occurs, and the displacements show obvious narrow-band characteristics, especially in in-plane direction. It should be noted that the static displacement caused by wind has been eliminated in the response.

Figure 11 shows the trajectory of the midspan of conductor. At a wind speed of 1.1 m/s, the in-plane and out-of-plane displacements are comparable and show little correlation between them. At a wind speed of 5.58 m/s, the galloping vibration occurs and is dominated in the in-plane direction, thus, the shape of the trajectory is a very flattened ellipse.

Figure 12 shows the PSD of the midspan of the conductor. The peak frequency is 1.73 Hz for both in-plane and out-of-plane displacements. When the wind speed is low, the responses are dominated by the buffeting vibration, and the values of the PSDs are very small. When the wind speed is 5.58 m/s, the displacements have one peak frequency of 1.73 Hz, especially in the out-of-plane direction. Figure 13 shows the variation mode of conductor line at a wind speed of 5.58 m/s. It is shown that the conductor line vibration dominated by the first mode. When the galloping happens, the response of the wire is narrowband vibration, and the response trajectory becomes a clear ellipse.

3.2. Response of Multi-Span Conductors

The responses of the two-span conductors with the same span length as single-span conductor under the same wind direction and angle of attack are shown below. Figure 14 shows the variation of STDs of the midspan displacements of one of the conductor lines with wind speed. It is shown that galloping initiation wind speed is 3.1 m/s, which is lower than that of single-span conductor, and is dominated by the coupled in-plane and out-of-plane galloping.

Figure 15 and Figure 16 show the times histories and trajectories of the in-plane and out-of-plane midspan displacements of one of the two-span conductors. The response of another one of the two-span conductors is similar. It is shown that at 1.1 m/s, the in-plane and out-of-plane displacements are stochastic and uncorrelated, similar as the response for single-span conductors. At 3.46 m/s, the in-plane and out-of-plane displacements show more obvious narrow-band character, and the trajectory is a larger ellipse as compared to those for single-span conductor, which shows apparent correlation.

Figure 17 shows the corresponding PSDs of the midspan displacements. At a wind speed of 1.1 m/s, the values of PSDs are small, and the PSDs have many peaks since the displacements are very stochastic. At a wind speed of 3.46 m/s, the PSDs of the in-plane and out-of-plane displacements show a very clear one-peak frequency of 1.2 Hz since the displacements are dominated by galloping vibration. Figure 18 shows the vibration mode of the two-span conductor line. It is shown that the vibration mode is antisymmetric for the galloping vibration. Since the vibration mode for the galloping of the two-span conductor changes to an antisymmetric shape, its peak frequency is smaller than that for the single-span conductor by 17%. When the mode shape is antisymmetric, there is tension compensation between the conductors at two adjective spans; thus, the first mode frequency and the equivalent stiffness decrease, which leads to a smaller galloping initiation wind speed.

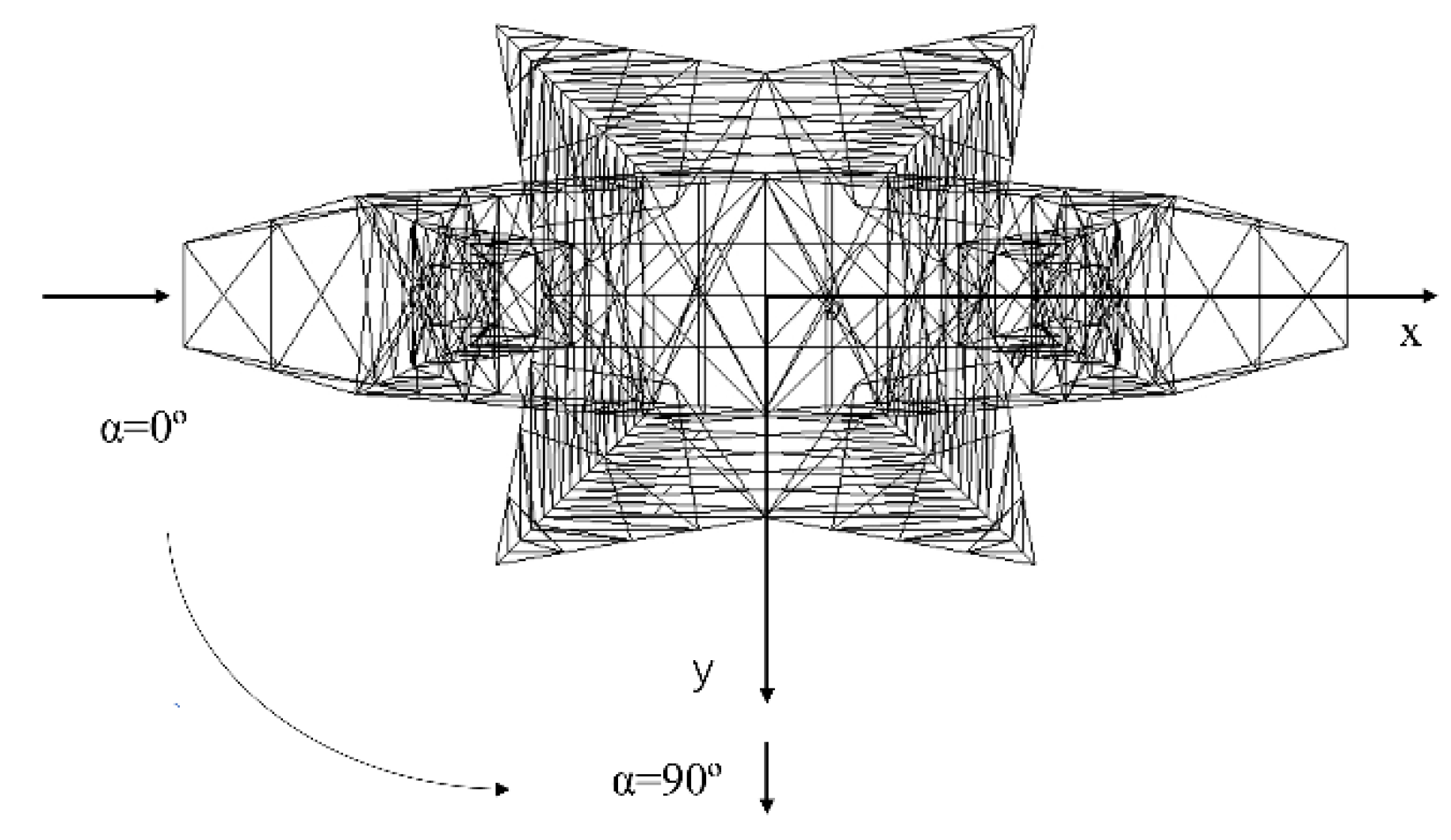
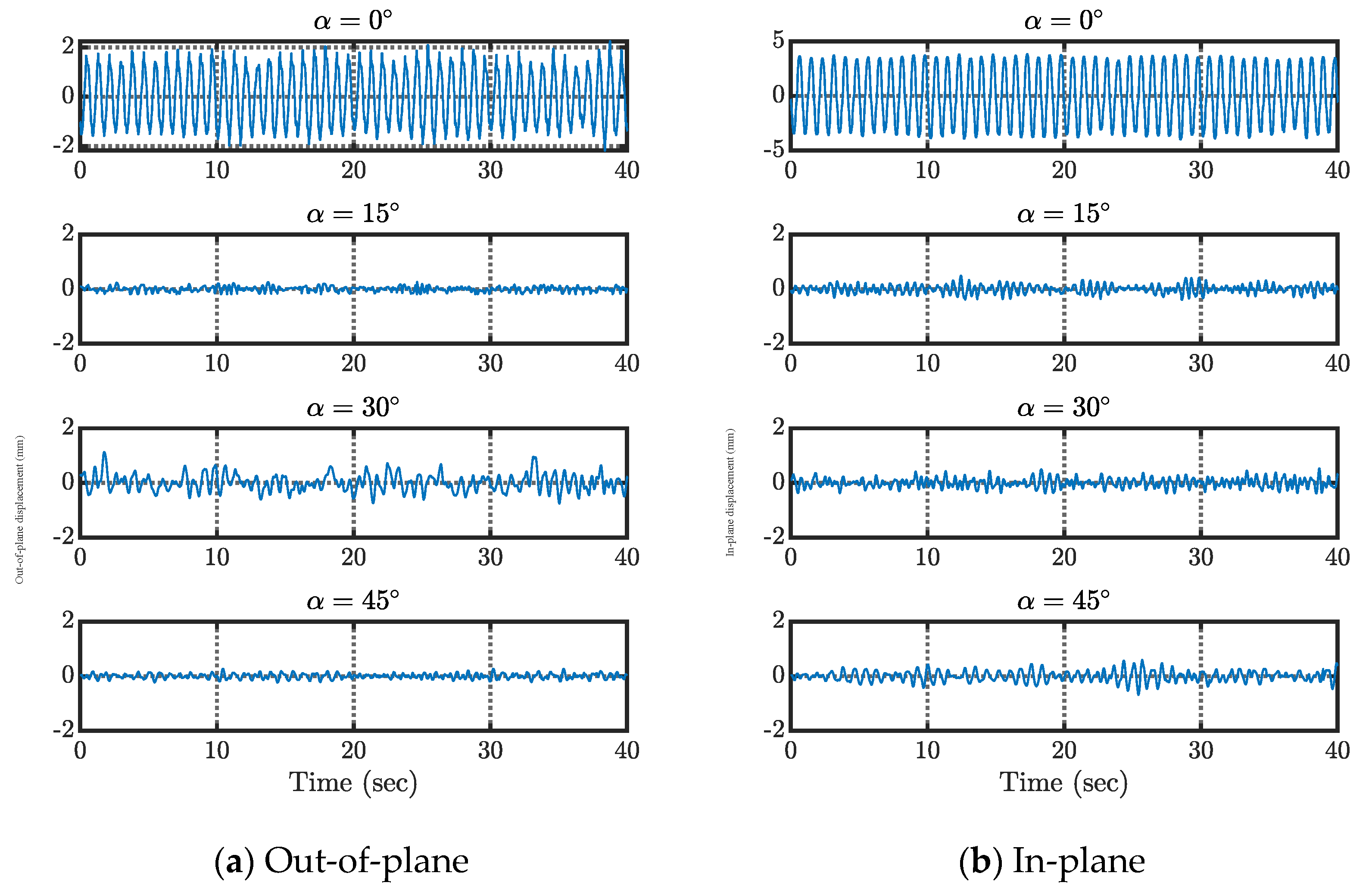
3.3. Influence of Wind Direction on Donfuctor

Due to the symmetry of the transmission tower-line system, only the influence of the wind direction from 0°~45° is investigated. Figure 19 shows the time histories of the midspan displacements of one of the two-span conductors at a wind speed of 3.65 m/s in different wind directions. It is shown that when the conductor has a galloping vibration at wind direction α = 0°, the response of the conductor at other directions is small and stochastic in both in-plane and out-of-plane directions. Figure 20 shows the corresponding STDs of the midspan displacement at different wind directions and wind speeds. It is shown that at wind direction α = 0°, the conductor has a galloping initiation wind speed after which the response of the conductor increases rapidly, while at other directions, the galloping phenomena does not happen. These results conform to the current design code in China (DL/T 5154-2012) [23] that the wind direction α = 0° is most prone to galloping vibration.

4. The Response of Transmission Tower

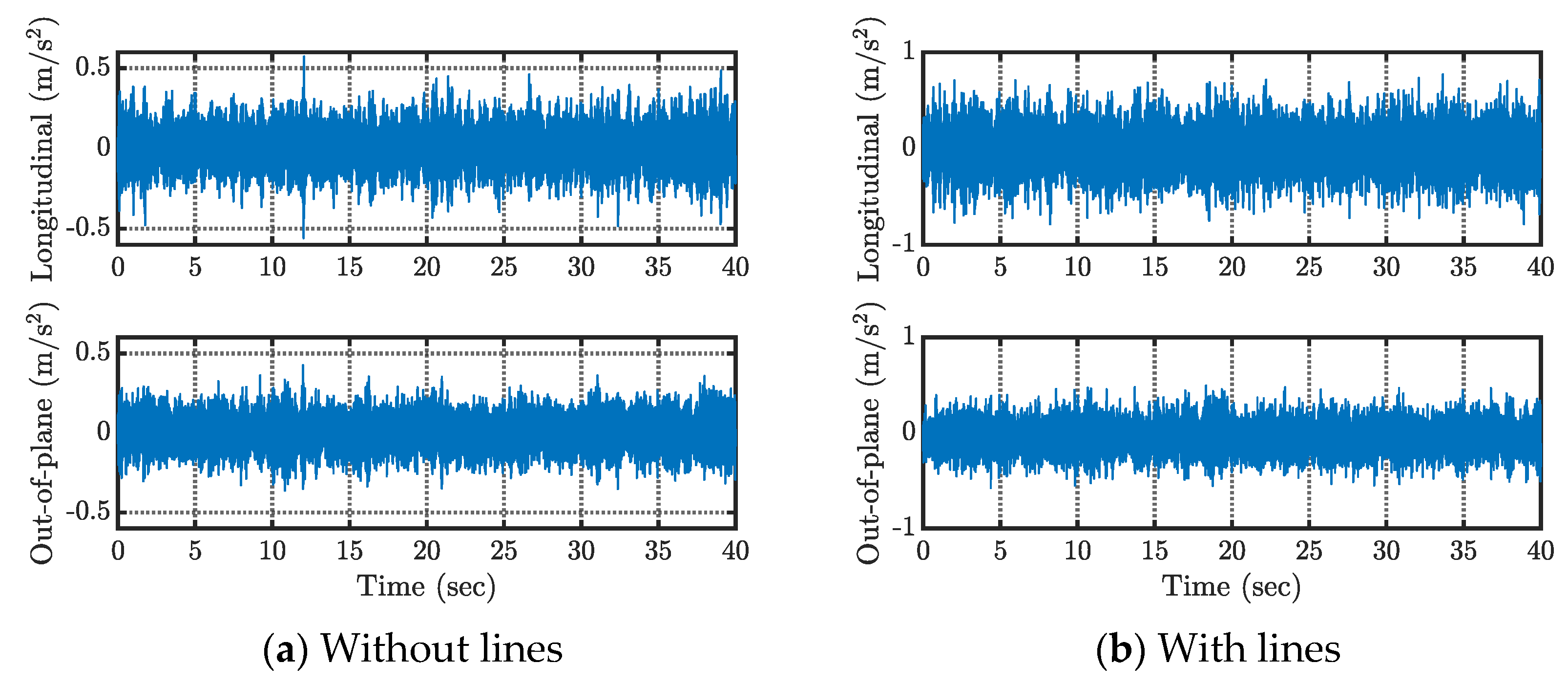
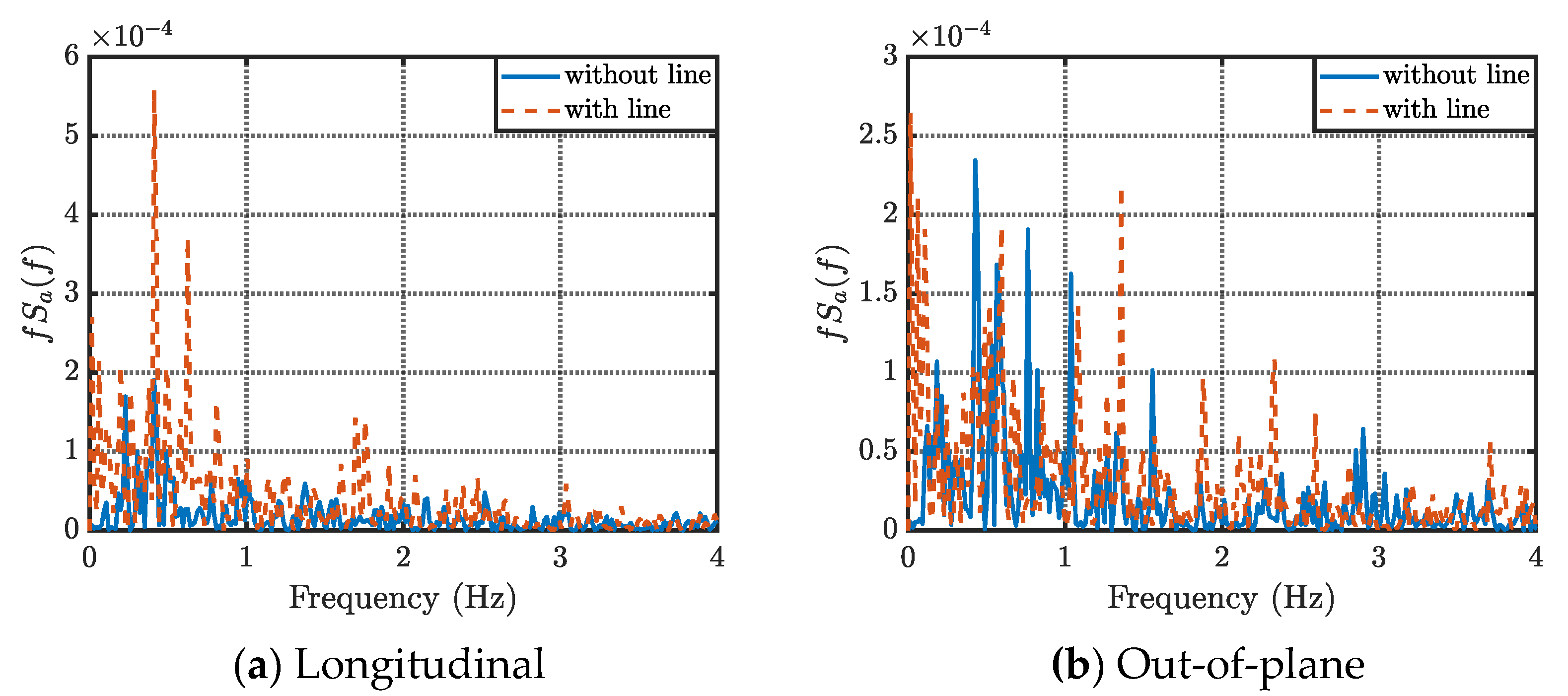
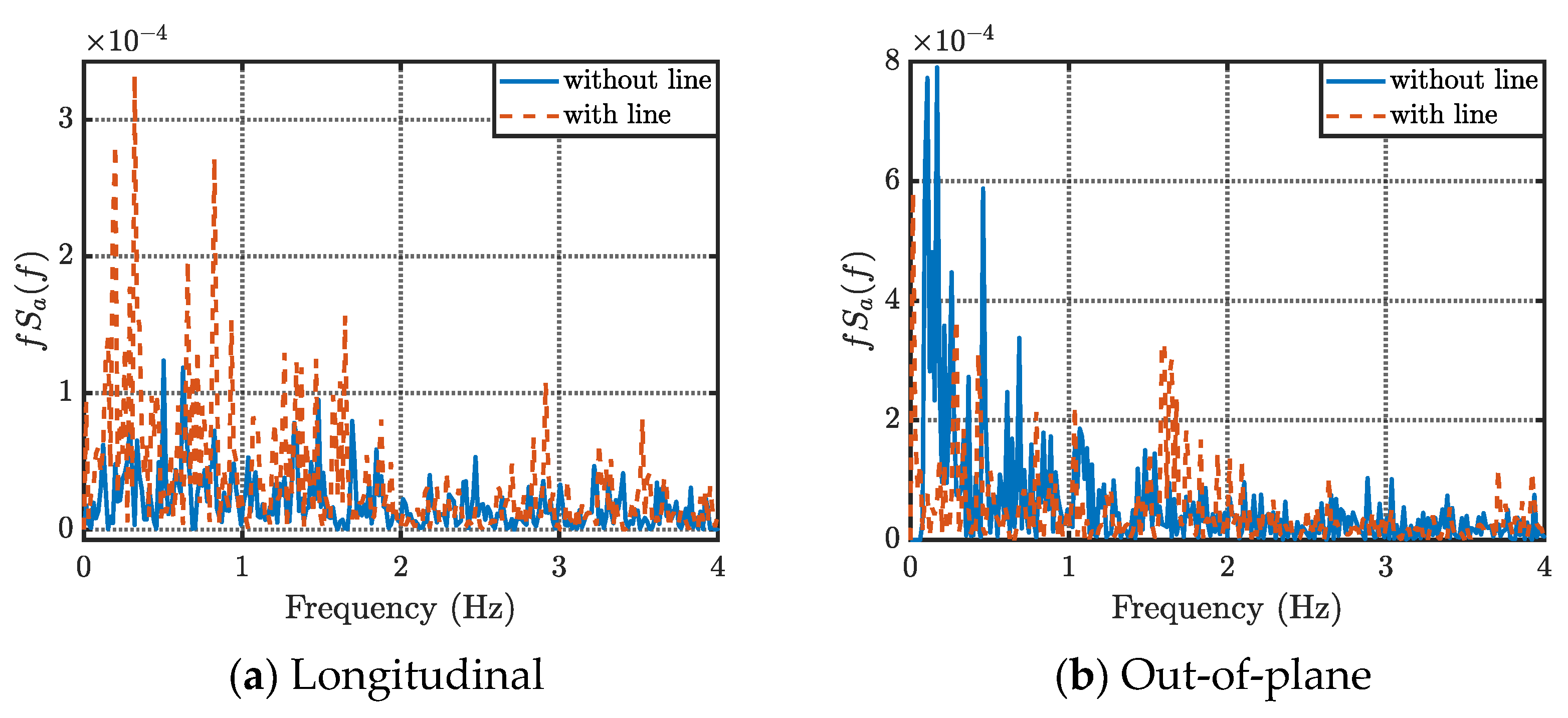
4.1. Influence of Coupled Effect

Figure 21 and Figure 22 show the time histories of the accelerations of the tower with and without conductor lines in longitudinal and out-of-plane directions at different wind speeds. Figure 23 and Figure 24 show the corresponding PSDs of the accelerations of the tower. It is noted that the acceleration response time histories of the tower are stochastic vibration, the values of the corresponding PSDs are small, and no narrow-band feature is shown in the PSD. The tower without lines has a buffeting response. The tower with lines also has a buffeting response at low wind speed and at wind speed when the conductor lines have a galloping vibration. Figure 25 shows the STDs of the accelerations of the tower with and without lines. The STDs of the accelerations of the tower are very low and are slightly increased when connected to the conductors. The influence of the conductor lines on the response of tower is trivial.

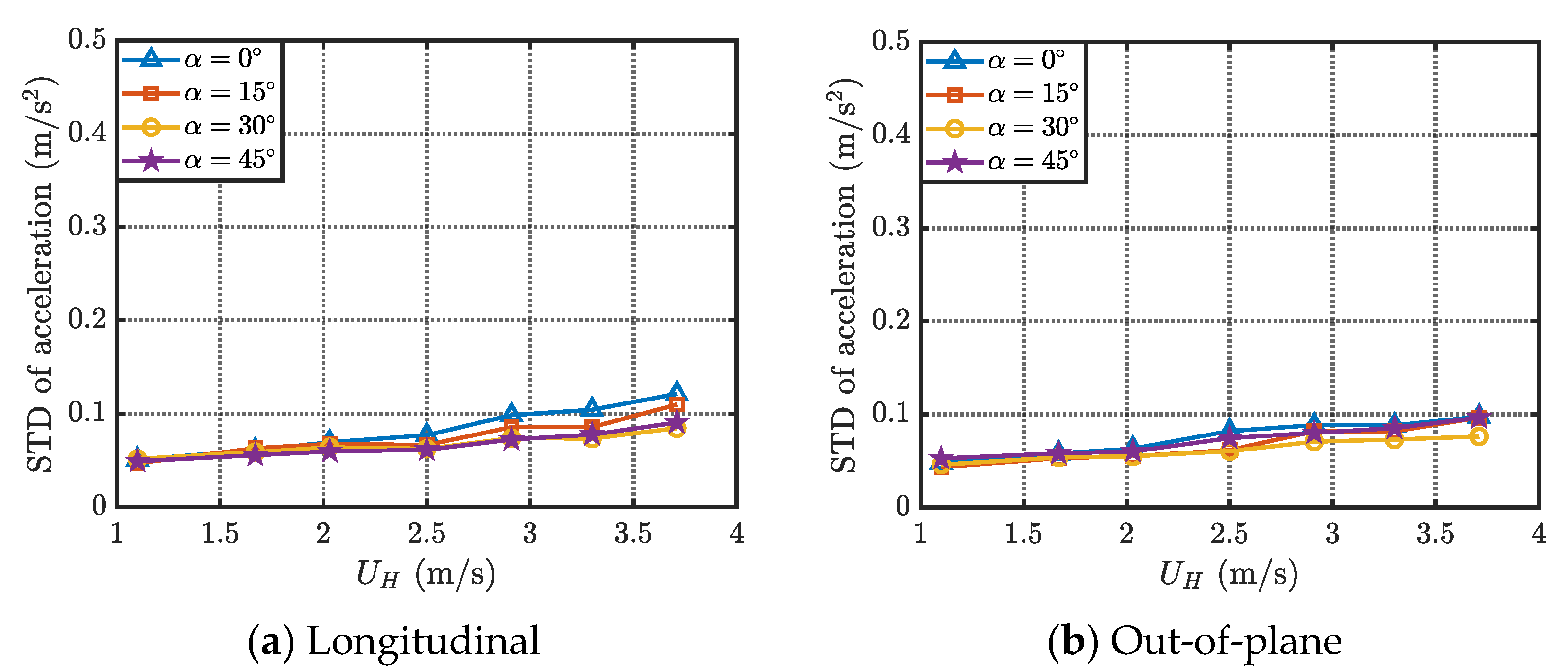
4.2. Influence of Wind Direction on Tower

Figure 26 and Figure 27 show the influence of wind direction on the response of the tower with and without lines. Since the tower is a square tower, the wind direction has little influence on the response of the tower without lines. When the tower is connected with a line, and the conductor line has a galloping vibration at a wind direction of 0 degree, which has a larger dynamic tension force, the response of the conductor also has little influence on the response of tower. At other wind directions, the conductor has a lower buffeting vibration, which has a slight effect on the response of tower.

5. Findings and In-Depth Discussion

To investigate the galloping phenomenon of the transmission line system with rime, an aeroelastic models of transmission line system of single-span and two-span two-bundled transmission conductors with rime have been fabricated according to the similarity criteria. The wind-tunnel test for the full aeroelastic model has been conducted for different wind speeds and directions. The effects of the multiple spans, wind speed and wind direction on the wind-induced response and the galloping performance of the transmission line system have been investigated from the results of the wind-tunnel test. The conclusions are summarized below.

• The single- and two-span conductors with rime have the galloping phenomenon at a relatively low wind speed, and the vibration amplitude increases to a steady-state value. Before the galloping happens, the response of the conductor is very stochastic, which is a buffeting vibration. When the galloping happens, the response of the conductor is a narrow-band vibration, and the trajectory of the response becomes an obvious ellipse.
• The characteristics of the galloping responses of single-span and two-span conductors are different under the same angle of attack. In the wind-tunnel test, it is shown that the vibration frequencies and vibration modes are different for the galloping responses of single- and two-span conductors. The galloping response of the two-span conductor is more complicated because the antisymmetric mode may happen, and the galloping initiation wind speed decreases from 3.8 m/s for the single-span conductor to 3.1 m/s. The vibration amplitude for two-span conductor is larger than that of single-span conductor under the same wind speed. The trajectories of the displacements of single- and two-span conductors are also different due to the different phases.
• The wind direction has influence on the response of the conductor. It is found in the wind-tunnel test that the 90° between the wind and conductor (α = 0°) is the most dangerous direction, which is more prone to galloping. This phenomenon conforms to the current design specifications.
• The coupling effect between the transmission tower and the conductor has little effect on the response of tower; however, the response of the tower without lines is very small. Even when the conductor has a galloping vibration, the effect of the conductor has little influence on the response of the tower. The wind direction has no influence on the responses of the towers with and without lines.

Due to the change in the cross-sectional shape of the wire after icing, the ice coating has various shapes and is subjected to complex aerodynamic forces. The mechanism of wire galloping caused by icing is still unclear and lacks a unified theory. This article conducts experiments on the effect of different parameters on the galloping vibration of iced conductors and draws some conclusions. How to establish a unified galloping model for ice-covered conductors is still a major challenge.