Building a Novel Electromechanical-Thermal Model for Semi-Solid-State Batteries
Abstract
1. Introduction
2. Research Methodology
2.1. Construction of the Electrochemical Model
2.1.1. Introduction and Assumptions of the P2D Model
2.1.2. Electrochemical Model Governing Equations
- (1)
- Solid-phase charge conservation:
- (1)
- Liquid-phase charge conservation:
- (2)
- Solid-phase mass conservation:
- (3)
- Liquid-phase mass conservation:
- (4)
- Electrochemical kinetics equation:
2.2. Construction of the Thermal Model
Thermal Model Governing Equations
2.3. Construction of the Mechanical Model
Mechanical Model Governing Equations
2.4. Semi-Solid-State Charge-Discharge Cycling Experiment
2.4.1. Basic Parameters of the Experimental Battery
2.4.2. Experimental Equipment
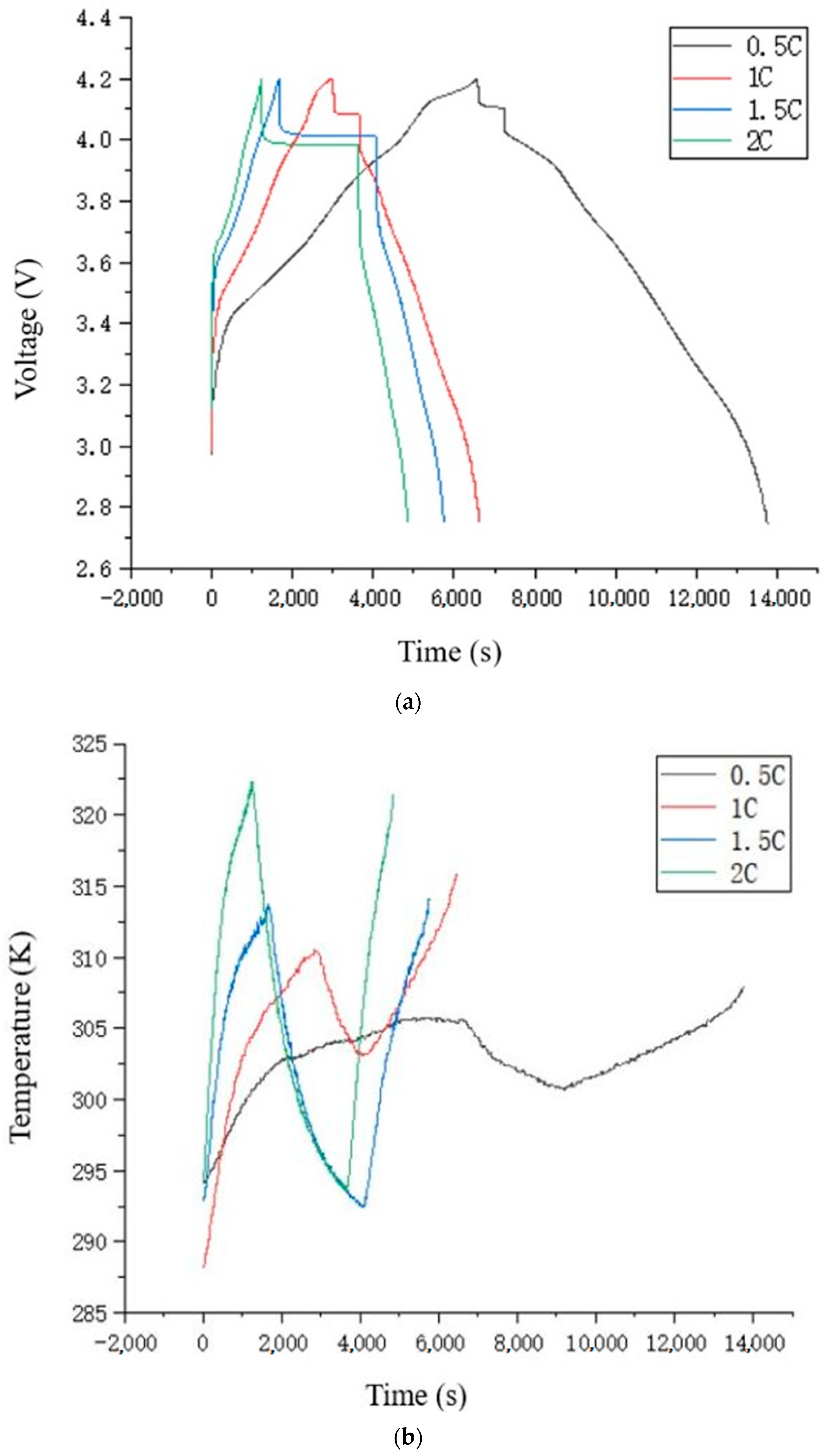
2.4.3. Experimental Data
- (1)
- Battery voltage curve:
- (2)
- Battery temperature curve:
3. Results and Discussion
3.1. Electrochemical Model Verification and Analysis
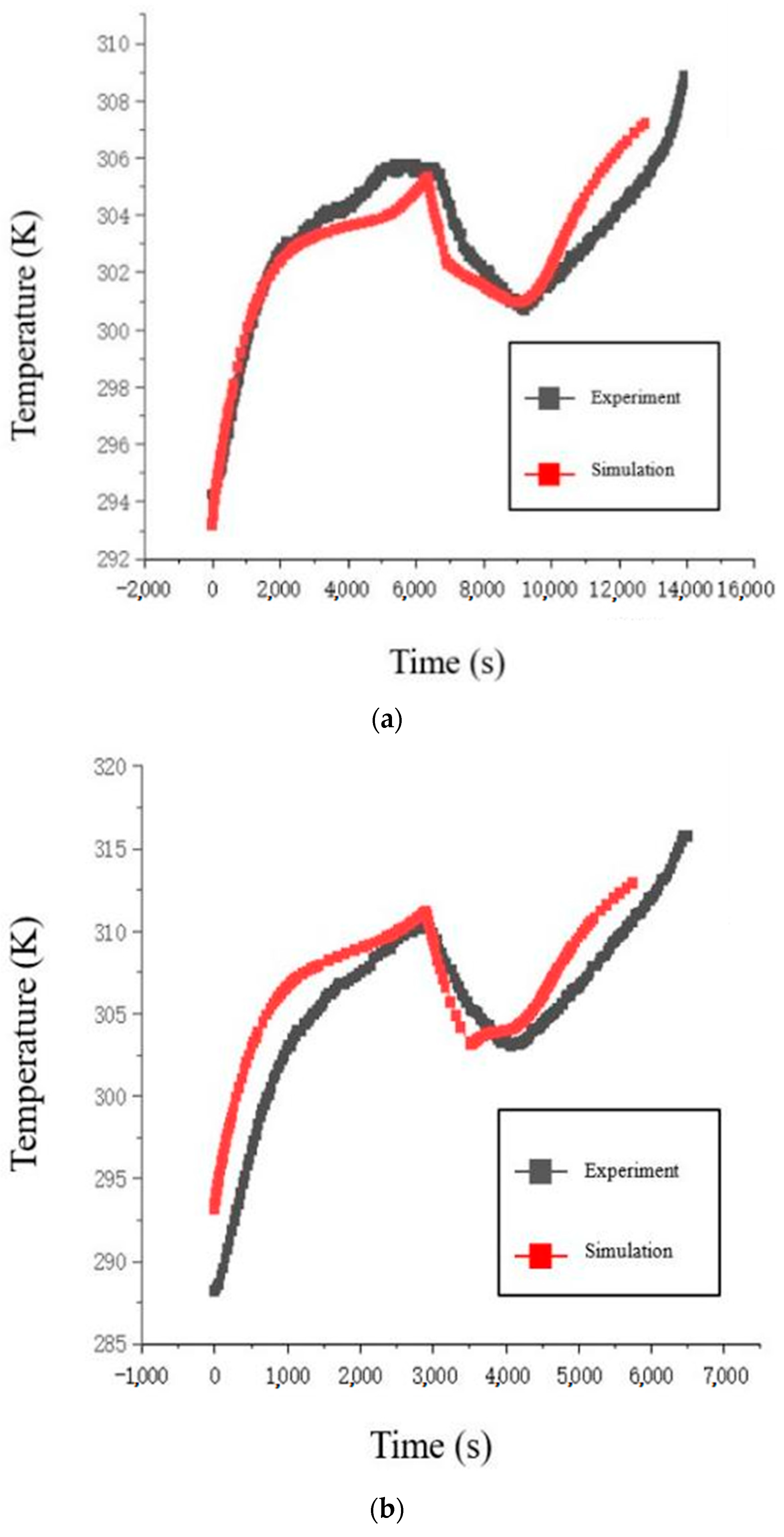
3.2. Thermal Model Validation and Analysis
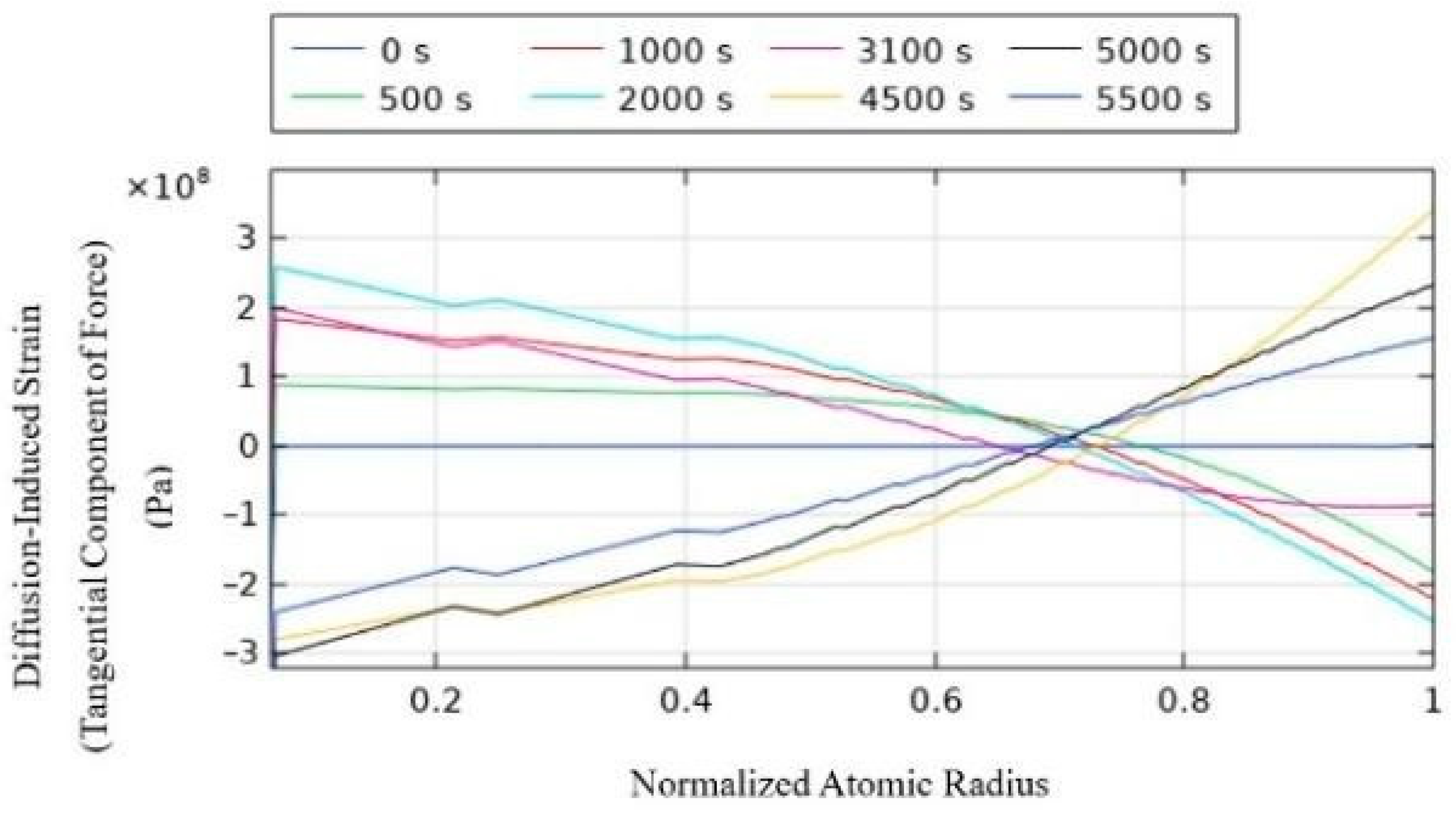
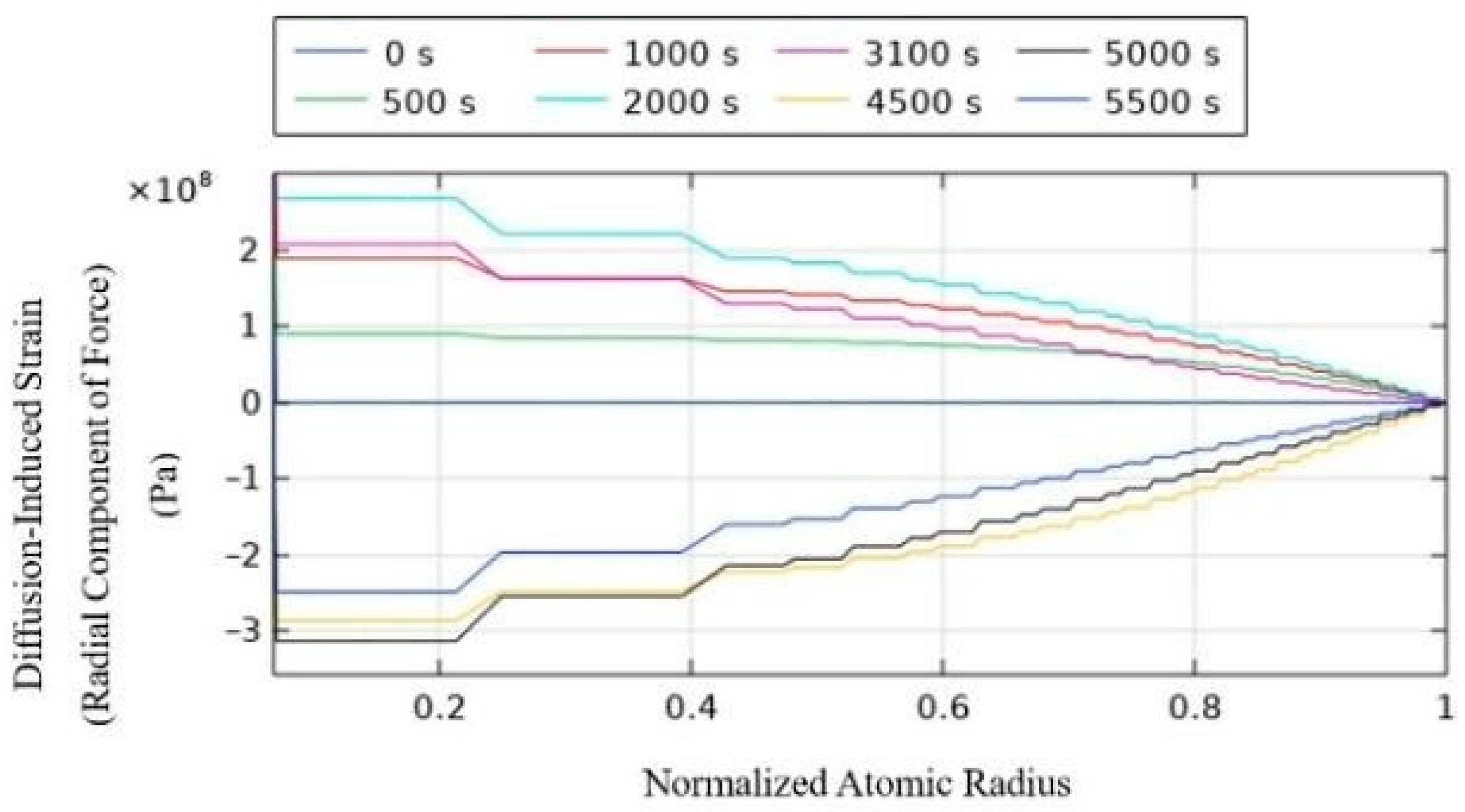
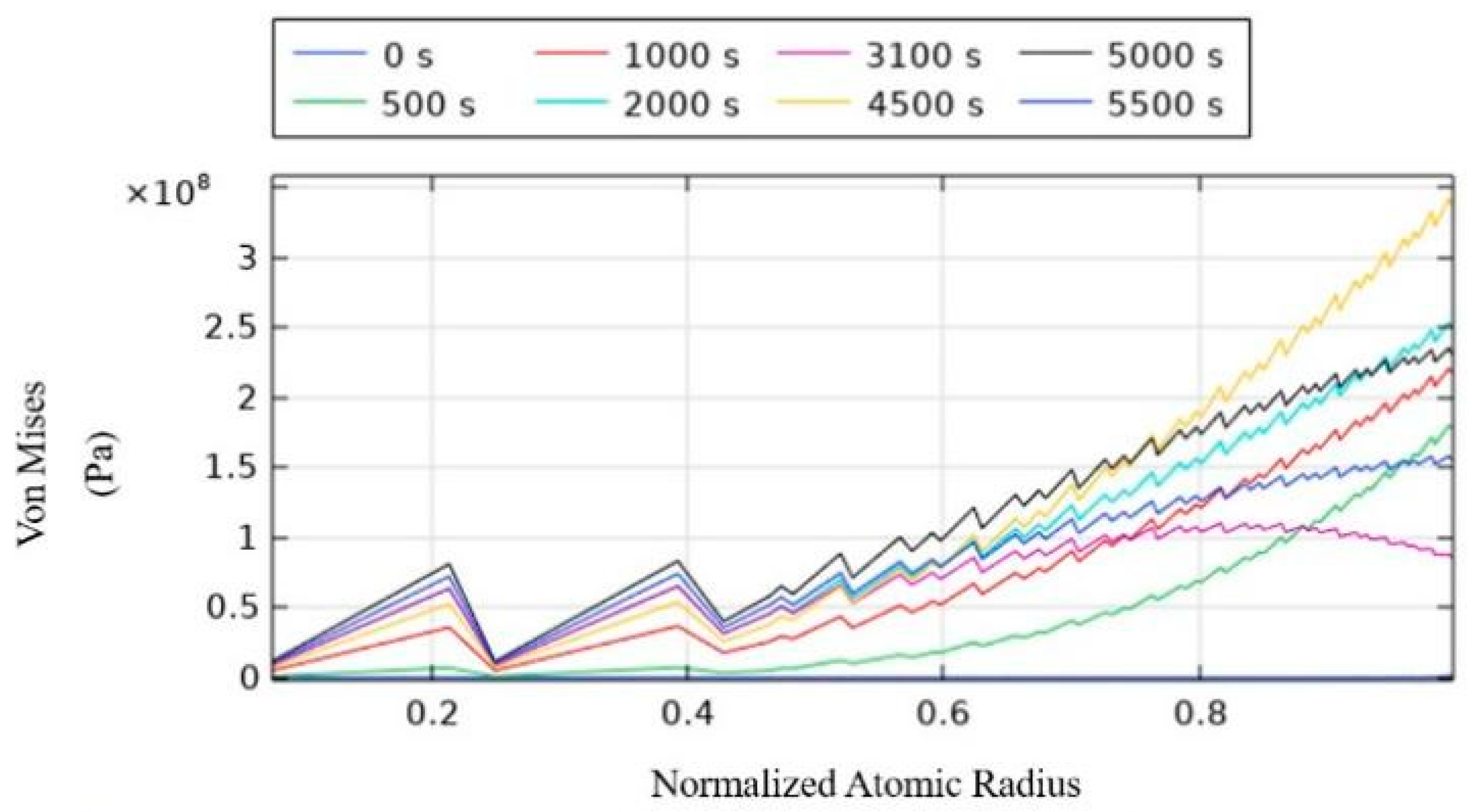
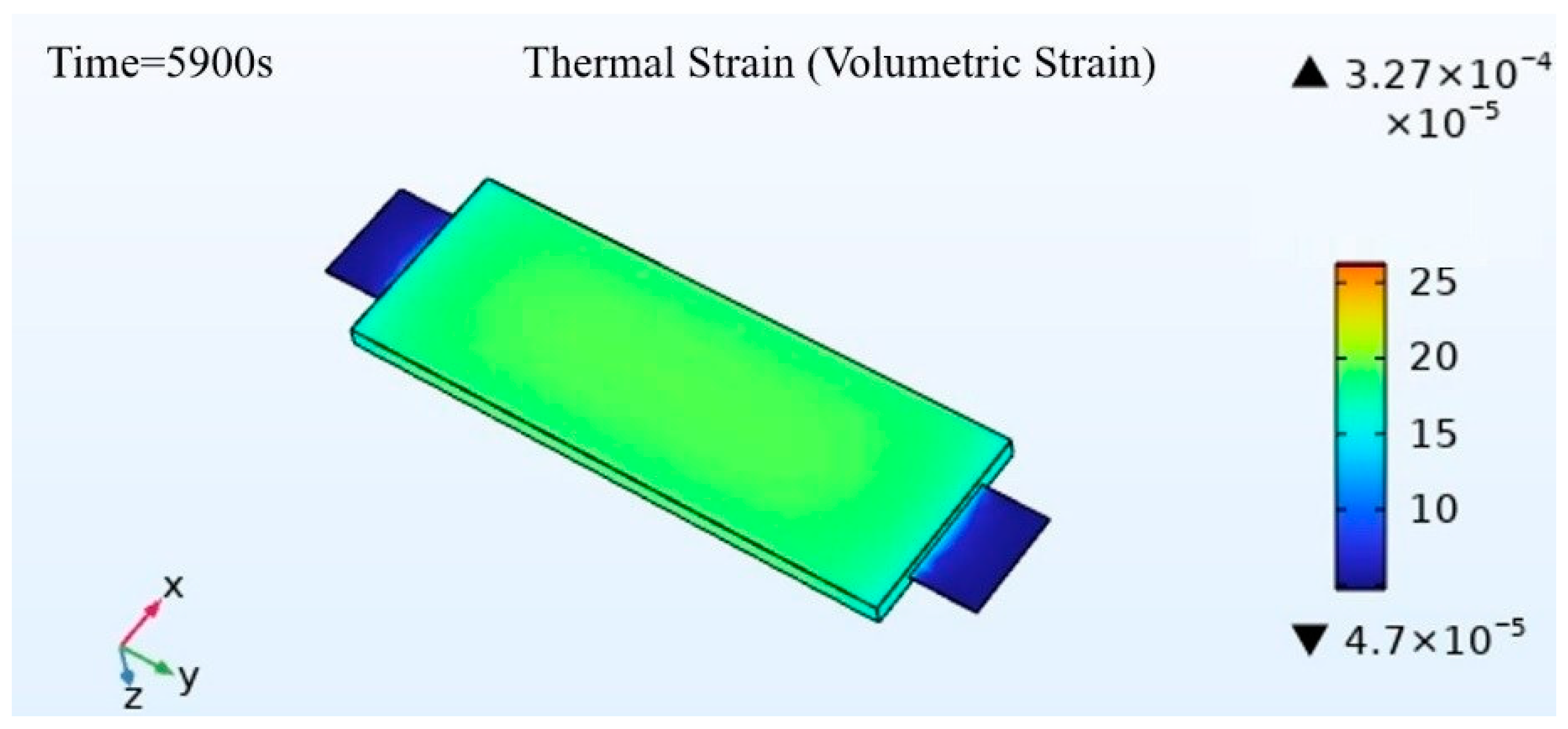
3.3. Force Model Analysis and Thermal Expansion Behavior
4. Conclusions
- The electromechanical-thermal model developed was based on a P2D model, which was integrated with the solid heat transfer module and the solid mechanics module. The model was validated through constant current charge/discharge cycling experiments, and the simulation results demonstrated good agreement with the experimental data at low charge/discharge rates. Therefore, the model was found to enable a comprehensive evaluation of the battery’s electrochemical, thermal, and mechanical performance.
- The electromechanical-thermal model developed in this study serves as an effective tool for simulating semi-solid-state lithium-ion batteries. The model accurately predicts the battery’s performance under various operating conditions, providing valuable guidance for battery design optimization and performance improvement. Future research could further explore the coupling effects of multiple physical fields within the battery and the impact of different material systems on battery performance to achieve more accurate model development and more comprehensive performance evaluation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Feng, Z.; Qiu, X.; Zhang, T.; Dai, Z.; Guo, X. Progress in the study of thermal safety of all-solid-state lithium batteries. Fine Chem. 2024, 5, 960–970. [Google Scholar]
- Yang, X.; Liu, S.; Zhou, W.; Huang, Q.; Zu, W.; Qu, S.; Li, J. Research on modeling techniques for lithium-ion batteries. Distrib. Energy 2021, 6, 77–82. [Google Scholar]
- Zhang, X.; Wang, Y. Modeling and simulation of the thermal characteristics of lithium-ion batteries. In Proceedings of the 23rd Chinese Symposium on System Simulation Technology and Its Applications (CCSSTA 23rd), Hefei, China, October 2022. [Google Scholar]
- Feng, Y.; Zheng, L.; Dai, Z.; Zhang, Z.; Du, G.; Wang, D. Progress in simulation models of lithium-ion batteries. Energy Storage Sci. Technol. 2019, 8 (Suppl. S1), 18–22. [Google Scholar]
- Sun, Z.; He, Y.; Chen, S.; Nie, L.; Huang, Y.; Liu, W. Electrochemical process simulation of all-solid-state lithium metal batteries under multi-physics coupling. J. Chin. Chem. Soc. 2021, 42, 1598–1609. [Google Scholar]
- Dai, W. Analysis of Diffusion Polarization and Diffusion Stress in lithium-Ion Batteries Based on Electrochemical-Mechanical Coupling Model. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2020. [Google Scholar]
- Zhang, K. Thermal Characteristics Simulation of Lithium-Ion Batteries Based on Multilayer Structure and Multi-Physics Coupling. Master’s Thesis, Hunan University, Changsha, China, 2021. [Google Scholar]
- Mei, W.; Wang, Q.; Sun, J. Stress simulation of graphite particles during the charging process of lithium-ion batteries based on electrochemical-mechanical coupling model. Eng. Mech. 2020, 37 (Suppl. S1), 352–357. [Google Scholar]
- Doyle, M.; Fuller, T.F.; Newman, J. Modeling of galvanostatic charge and discharge of the lithium/polymer/insertion Cell. J. Electrochem. Soc. 1993, 140, 1526–1533. [Google Scholar] [CrossRef]
- Hong, Q. Simulation Study of Stress and Dendrite Growth Based on Solid Electrolyte Battery Model. Master’s Thesis, Huazhong University of Science and Technology, Wuhan, China, 2022. [Google Scholar]
- Reniers, J.M.; Mulder, G.; Howey, D.A. Review and Performance Comparison of Mechanical-Chemical Degradation Models for Lithium-Ion Batteries. J. Electrochem. Soc. 2019, 166, A3189–A3200. [Google Scholar] [CrossRef]
- Chen, Z.; Danilov, D.L.; Zhang, Q.; Jiang, M.; Zhou, J.; Eichel, R.A.; Notten, P. Modeling NCA/C6-Si battery ageing. Electrochim. Acta 2022, 430, 141077. [Google Scholar] [CrossRef]
- Chen, Y. Multiscale Force-Electrochemical Simulation Study of Silicon-Based Anode Lithium-Ion Batteries. Ph.D. Thesis, University of Chinese Academy of Sciences (Institute of Physics, Chinese Academy of Sciences), Beijing, China, 2022. [Google Scholar]
- Sethuraman, V.A.; Chon, M.J.; Shimshak, M.; Srinivasan, V.; Guduru, P.R. In situ measurements of stress evolution in silicon thin films during electrochemical lithiation and delithiation. J. Power Sources 2010, 195, 5062–5066. [Google Scholar] [CrossRef]




| Parameters | Unit | Negative Electrode | Separator | Positive Electrode |
|---|---|---|---|---|
| Electrode thickness L | um | 52 | 15 | 64.5 |
| Active particle radius R | um | 10 (C) 6.5 (Si) | 3.9 | |
| Active material volume fraction εs | 0.5896 (C) 0.1383 (Si) | 0.7635 | ||
| Electrolyte volume fraction εɭ | 0.2721 | 0.5 | 0.2365 | |
| Solid phase conductivity σs | S/m | 1000 | 50 | |
| Electrolyte conductivity σɭ | S/m | 7 | ||
| Battery minimum state of charge SOCmin | 0.0269 | 0.1709 | ||
| Battery maximum state of charge SOCmax | 0.98 | 0.88 | ||
| Maximum lithium insertion concentration cs,max | mol/m3 | 30,778 (C) 165,177 (Si) | 58,945 | |
| Initial lithium concentration c0 | mol/m3 | 2401 (C) 14,866 (Si) | 50,987 | |
| Initial electrolyte salt concentration cɭ0 | mol/m3 | 1700 | 1700 | 1700 |
| Electrode electrolyte reference concentration cɭ,ref | mol/m3 | 1000 | 1000 | |
| Solid phase diffusion coefficient Ds | m2/s | Equation (1) (C) 3 × 10−14 (Si) | Equation (2) | |
| Liquid phase diffusion coefficient Dɭ | m2/s | 5.34 × 10−10 | ||
| Exchange current reaction constant K | m/s | 2 × 10−10 | 2 × 10−10 | |
| Gas constant Rg | J/mol/K | 8.314 | ||
| Faraday constant F | C/mol | 96,486 | ||
| Lithium-ion transfer coefficient t+ | 0.363 | |||
| Anode charge transfer coefficient αɑ | 0.5 | 0.5 | ||
| Cathode charge transfer coefficient αc | 0.5 | 0.5 | ||
| Reference temperature Tref | K | 293.15 |
| Parameters | Unit | Cathode Current Collector | Cathode Current Collector |
|---|---|---|---|
| Current collector thickness L | um | 6 | 12 |
| Conductivity σ | S/m | 5.998 × 107 | 3.774 × 107 |
| Parameter | Unit | Value |
|---|---|---|
| Average heat capacity at constant pressure Cp | J/kg/K | 1600 |
| Average density ρ | kg/m3 | 2600 |
| Thermal conductivity along the X-axis Kx | W/m/K | 7.82 |
| Thermal conductivity along the Y-axis Ky | W/m/K | 1.64 |
| Thermal conductivity along the Z-axis Kz | W/m/K | 7.82 |
| Parameter | Unit | Value |
| Convective heat transfer coefficient h | W/m2/K | 23.5 |
| Ambient temperature Teir | K | 298.15 |
| Parameters | Unit | Si | C |
|---|---|---|---|
| Poisson’s ratio | 0.28 | 0.3 | |
| Young’s modulus E | Gpa | 90 | 15 |
| Partial molar volume Ω | m3/mol | 9 × 10−6 | 4.9 × 10−6 |
| Parameters | Unit | Battery Overall |
|---|---|---|
| Poisson’s ratio | 0.27 | |
| Young’s modulus E | Gpa | 150 |
| Coefficient of thermal expansion | 1/K | 3.2 × 10−6 |
| Parameters (Unit) | Value |
|---|---|
| Charging Cut-off Voltage (V) | 4.2 |
| Discharging Cut-off Voltage (V) | 2.75 |
| Nominal Battery Capacity (Ah) | 70 Ah (20 °C) |
| Battery Geometric Dimensions (mm) (excluding tabs) | 267.5 × 94.5 × 9.7 |
| Tab Geometric Dimensions (mm) | 43 × 52 × 0.12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.W.; Zhi, S.T.; Xu, X.T.; Liu, X.Y.; Zhen, H.S. Building a Novel Electromechanical-Thermal Model for Semi-Solid-State Batteries. Energies 2025, 18, 844. https://doi.org/10.3390/en18040844
Wang WW, Zhi ST, Xu XT, Liu XY, Zhen HS. Building a Novel Electromechanical-Thermal Model for Semi-Solid-State Batteries. Energies. 2025; 18(4):844. https://doi.org/10.3390/en18040844
Chicago/Turabian StyleWang, W. W., S. T. Zhi, X. T. Xu, X. Y. Liu, and H. S. Zhen. 2025. "Building a Novel Electromechanical-Thermal Model for Semi-Solid-State Batteries" Energies 18, no. 4: 844. https://doi.org/10.3390/en18040844
APA StyleWang, W. W., Zhi, S. T., Xu, X. T., Liu, X. Y., & Zhen, H. S. (2025). Building a Novel Electromechanical-Thermal Model for Semi-Solid-State Batteries. Energies, 18(4), 844. https://doi.org/10.3390/en18040844





