The Study of Multi-Terminal DC Systems in an Offshore Wind Environment: A Focus on Cable Ripple Analysis
Abstract
1. Introduction
- The total harmonic distortion of terminal voltages was analyzed across different wind conditions and system configurations, consistently maintaining voltages below 2%. This performance surpasses expectations, especially notable in multi-connected cable terminals in which a high current ripple and superimposed currents might induce significant voltage variations;
- Research into cable AC losses examined variations across diverse wind conditions and system configurations, distinguishing their characteristics in both multi-connected submarine cables and those in 100 km;
- The system’s dynamic performance under extreme wind conditions reveals its resilience, notably in swiftly recovering from brief deviations below or above the cut-in and cut-out wind speeds. Such resilience is attributed to the infrequent occurrence of unexpected wind fluctuations, which typically affect only a few turbines within large wind farm systems for short periods;
- The performance of various ISIPOS converter structures is compared, and corresponding application environments are suggested.
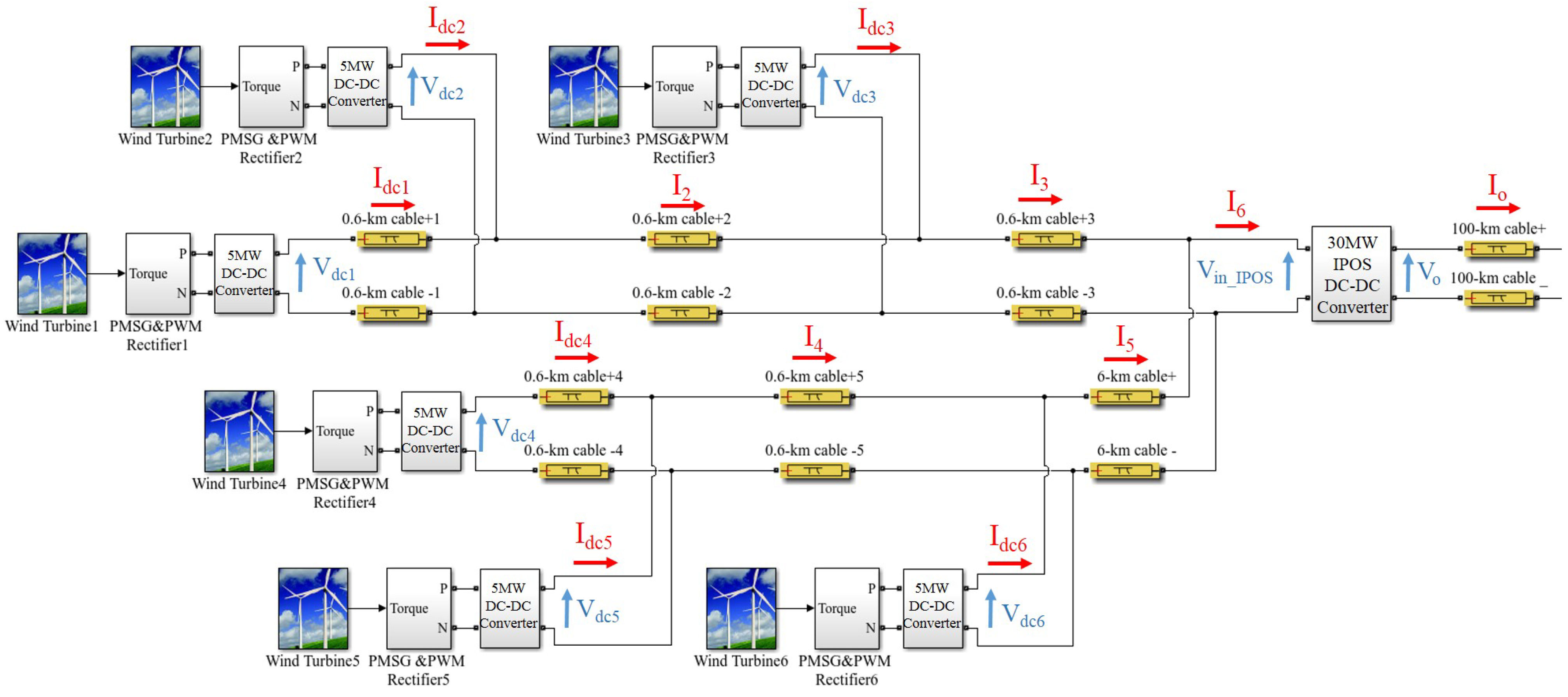
2. Overview of the Simulation Model
2.1. Circuit Diagram and Specification of the Simulation Model
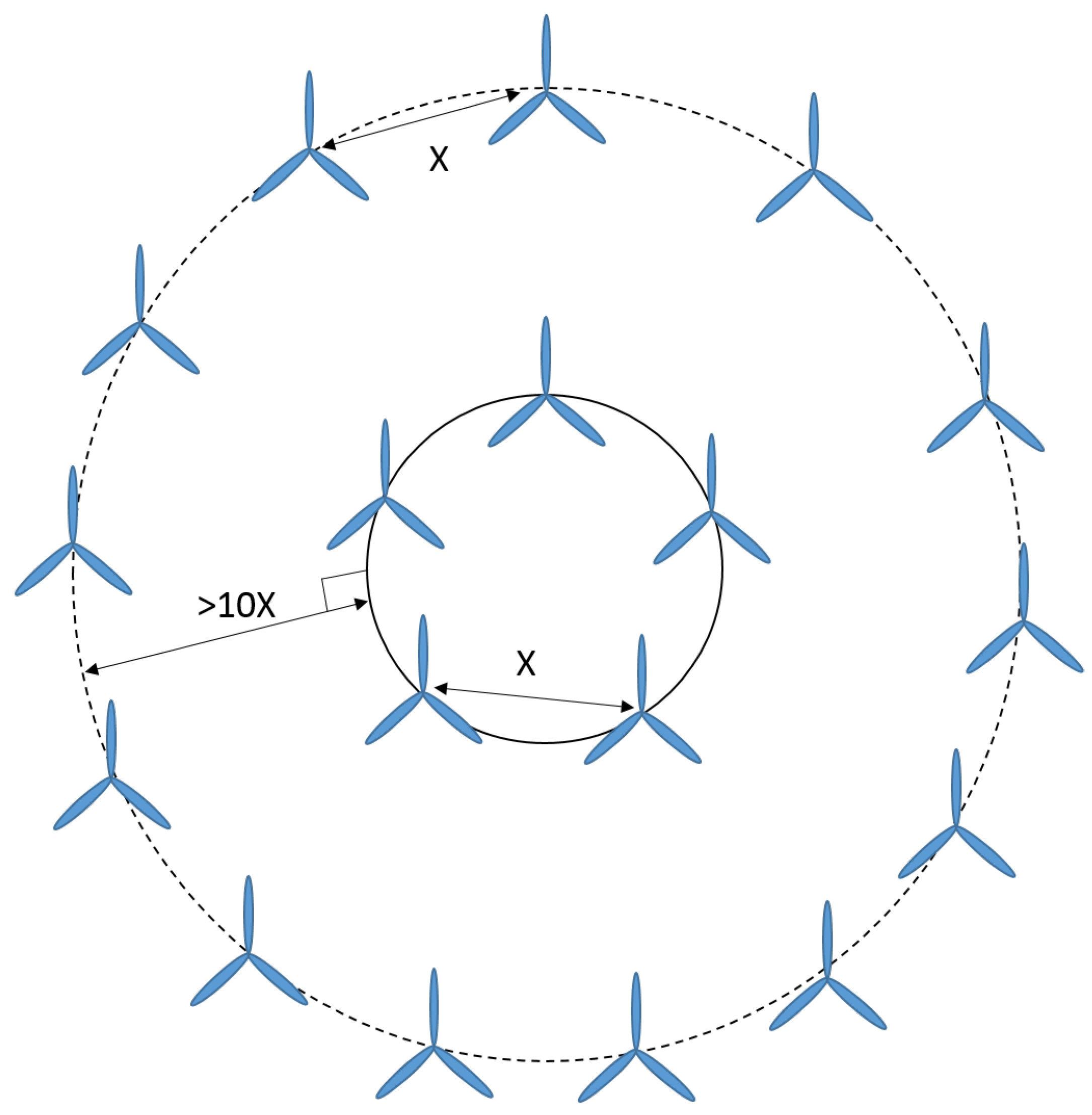
2.2. Circular Wind Farm Structure
2.3. Selection of the Phase Angle of Different DC–DC Converters
- All the switches of these six multi-connected 5 MW converters are controlled via the same signal;
- There are 60-degree phase shifts between the switches’ control signals of these 5 MW, multi-connected SAB converters.
3. Simulation Study of the System under Different Wind Conditions
3.1. Simulation Results of the System with Identical Three-Phase and Identical SAB IPOS Converter Structures under Wind Condition 1
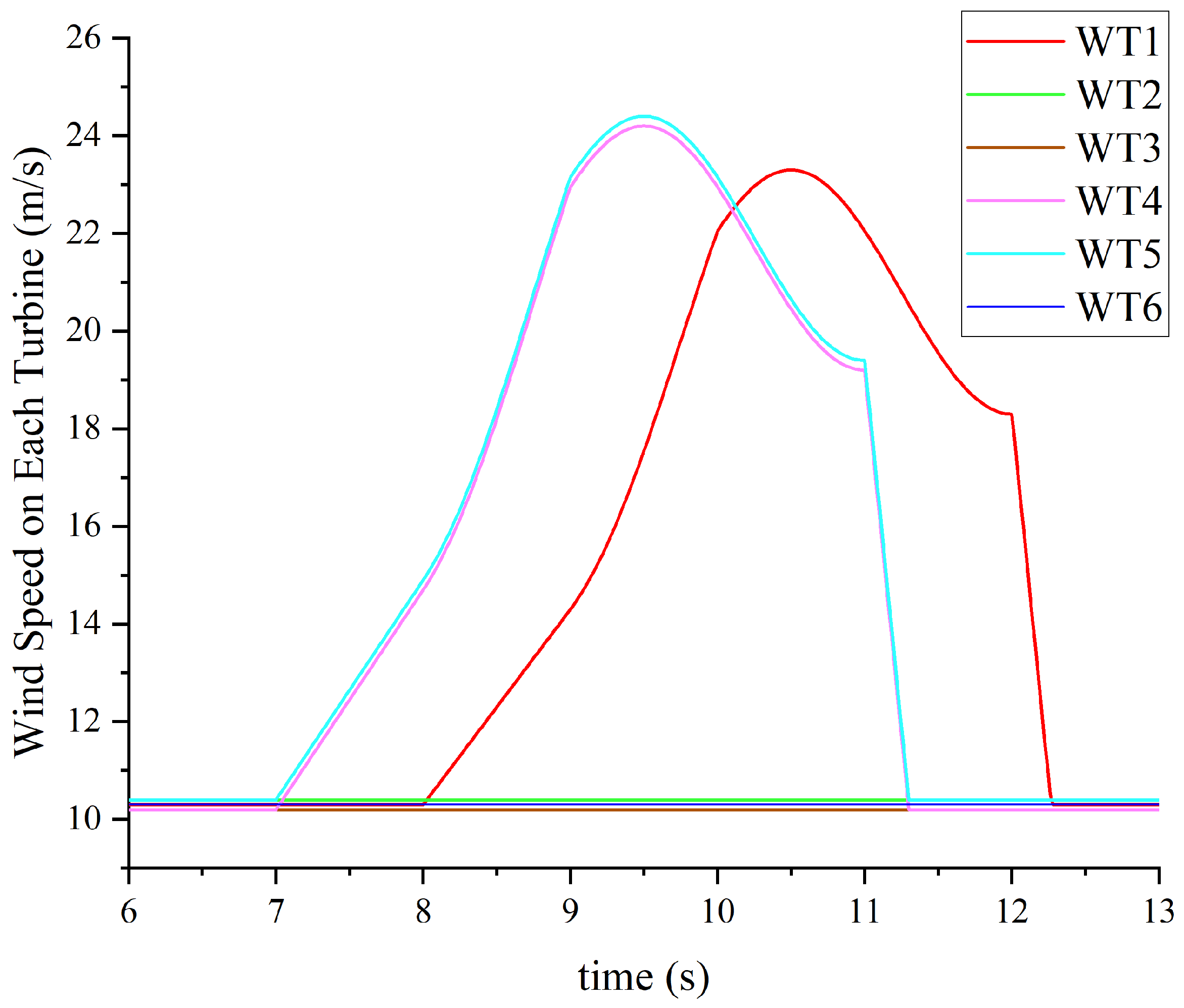
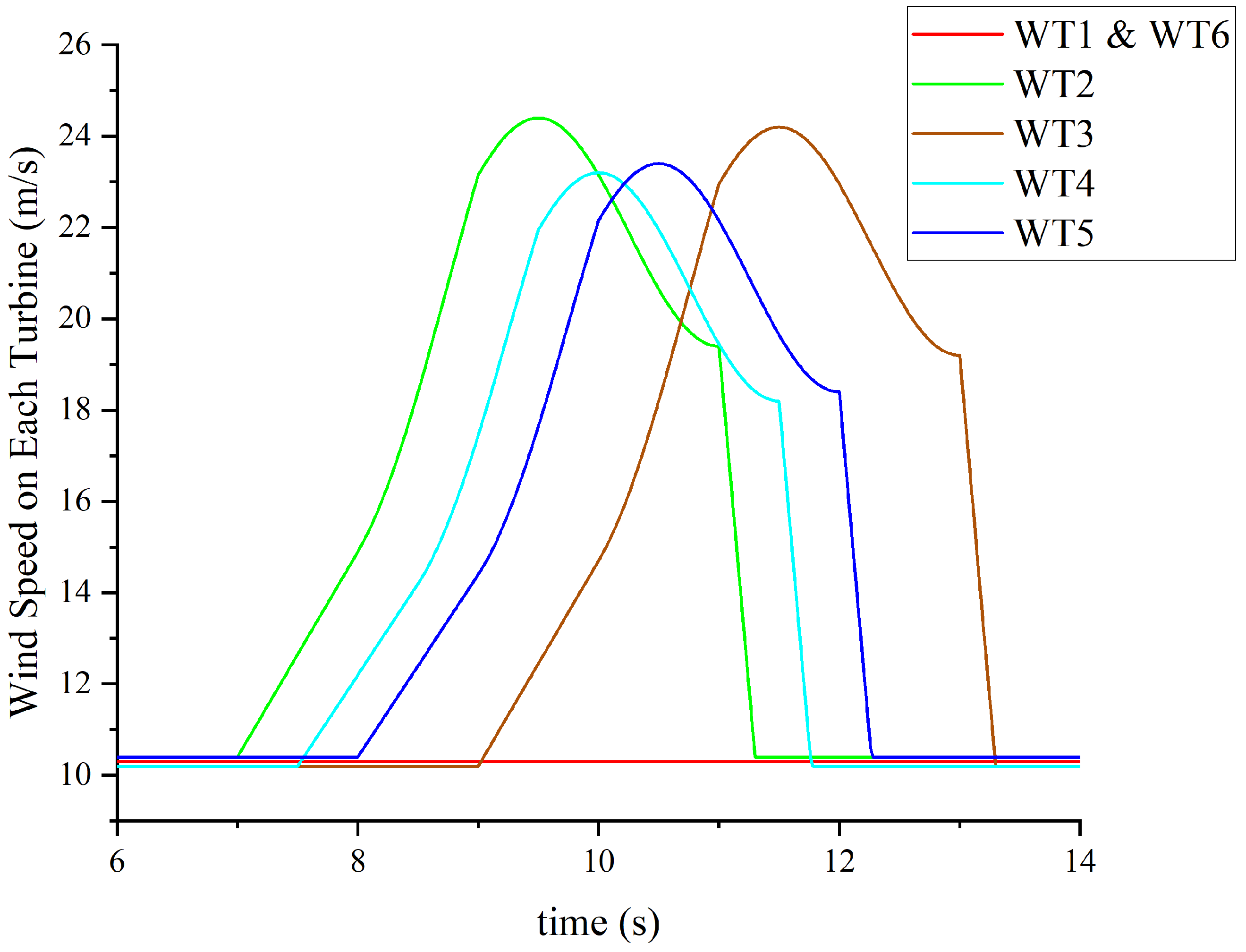
3.1.1. Wind Condition 1
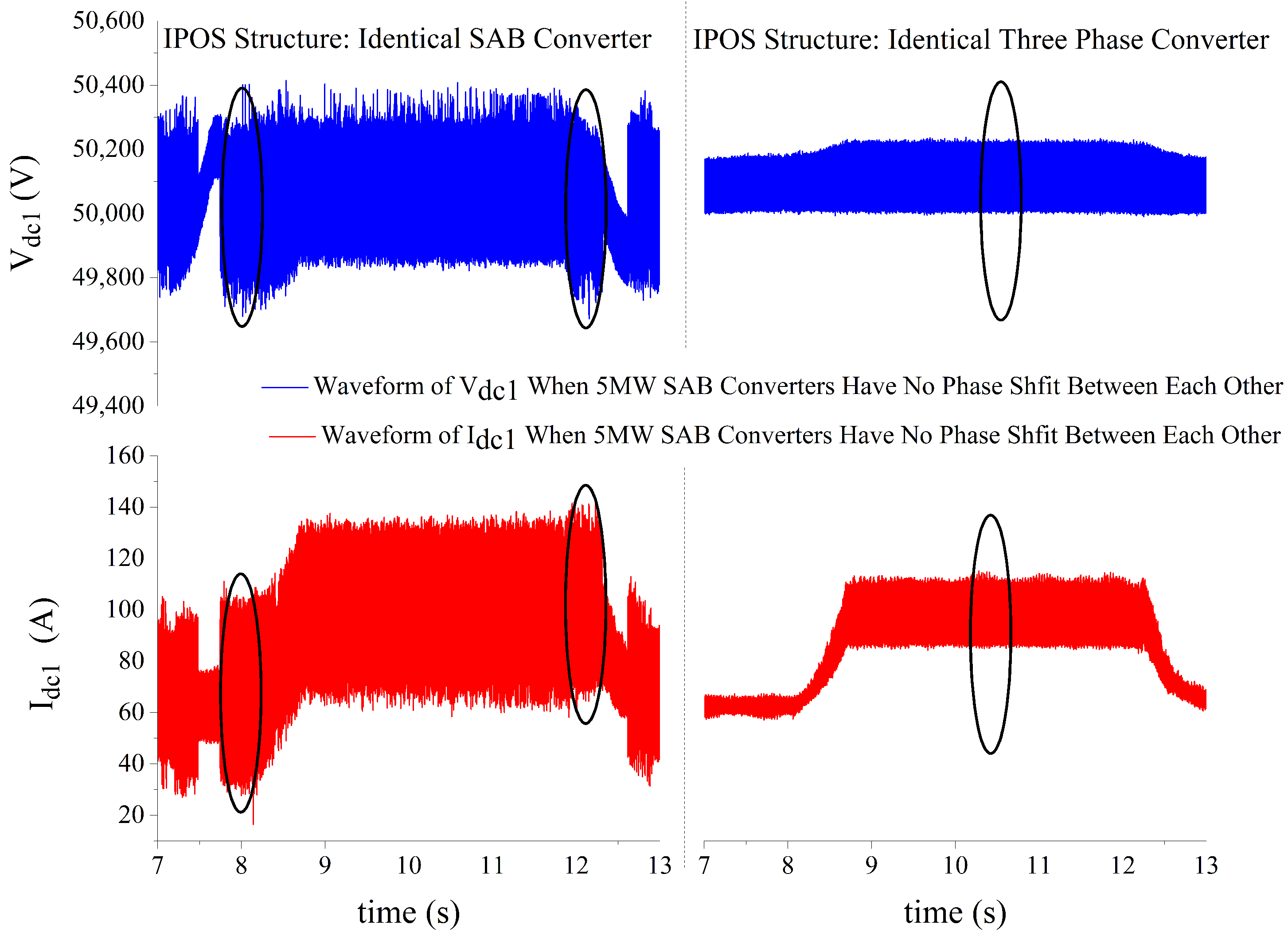
3.1.2. Vdc1 and Idc1 When the Identical SAB IPOS Structure Is Applied in the System and There Is No Phase Difference between 5 MW SAB DC–DC Converters
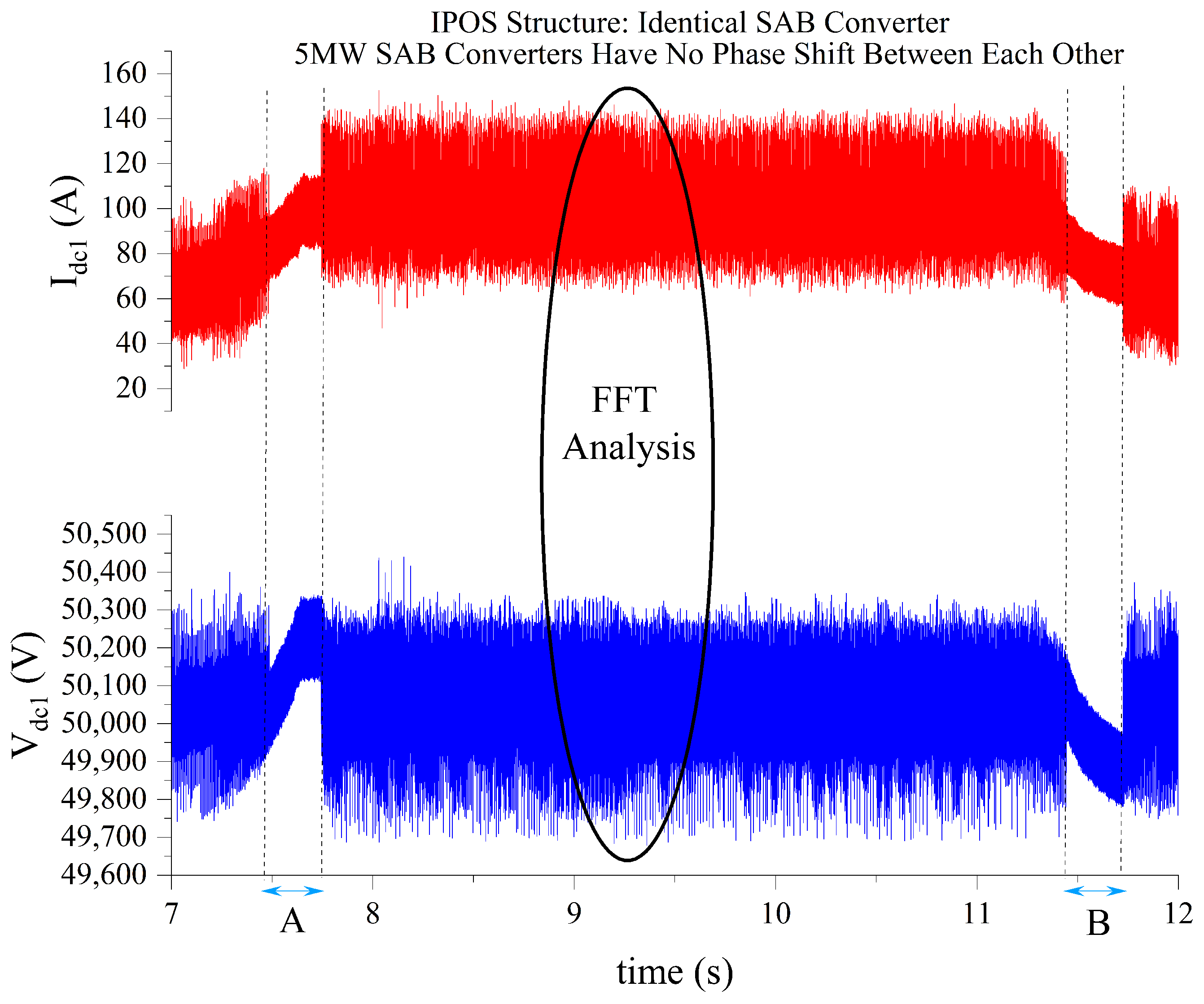

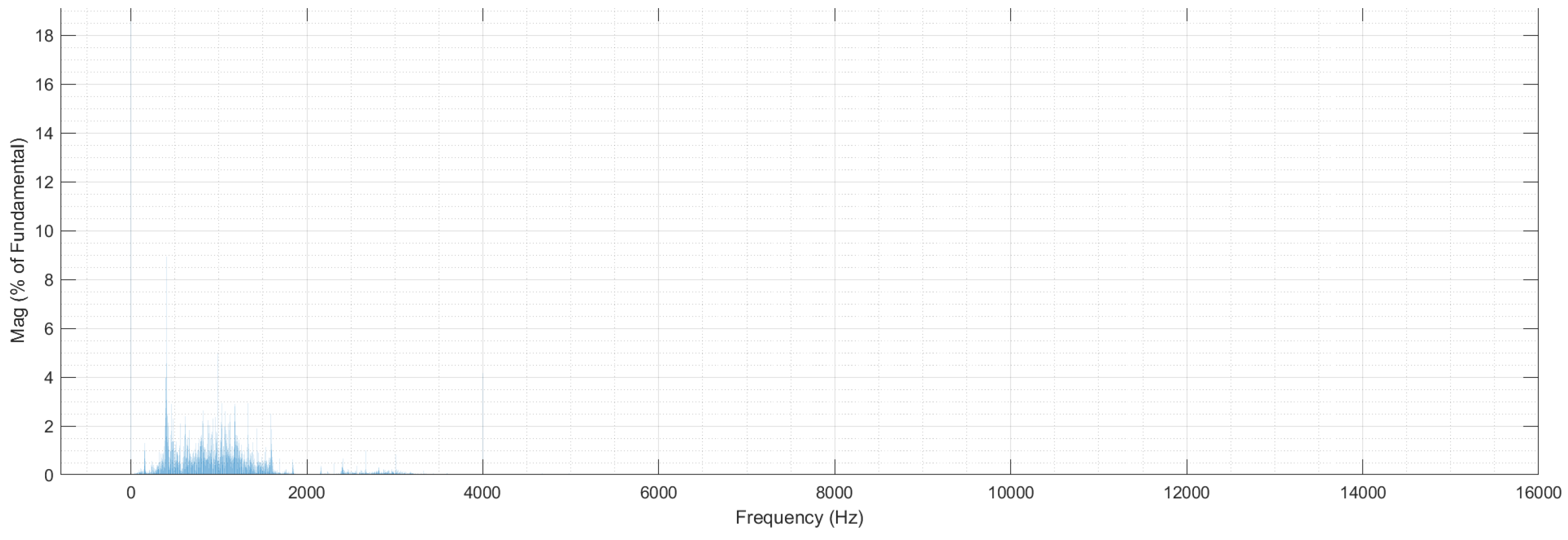
3.1.3. Waveforms of Terminal Voltages and Cable Currents Based on the Average Values under Wind Condition 1
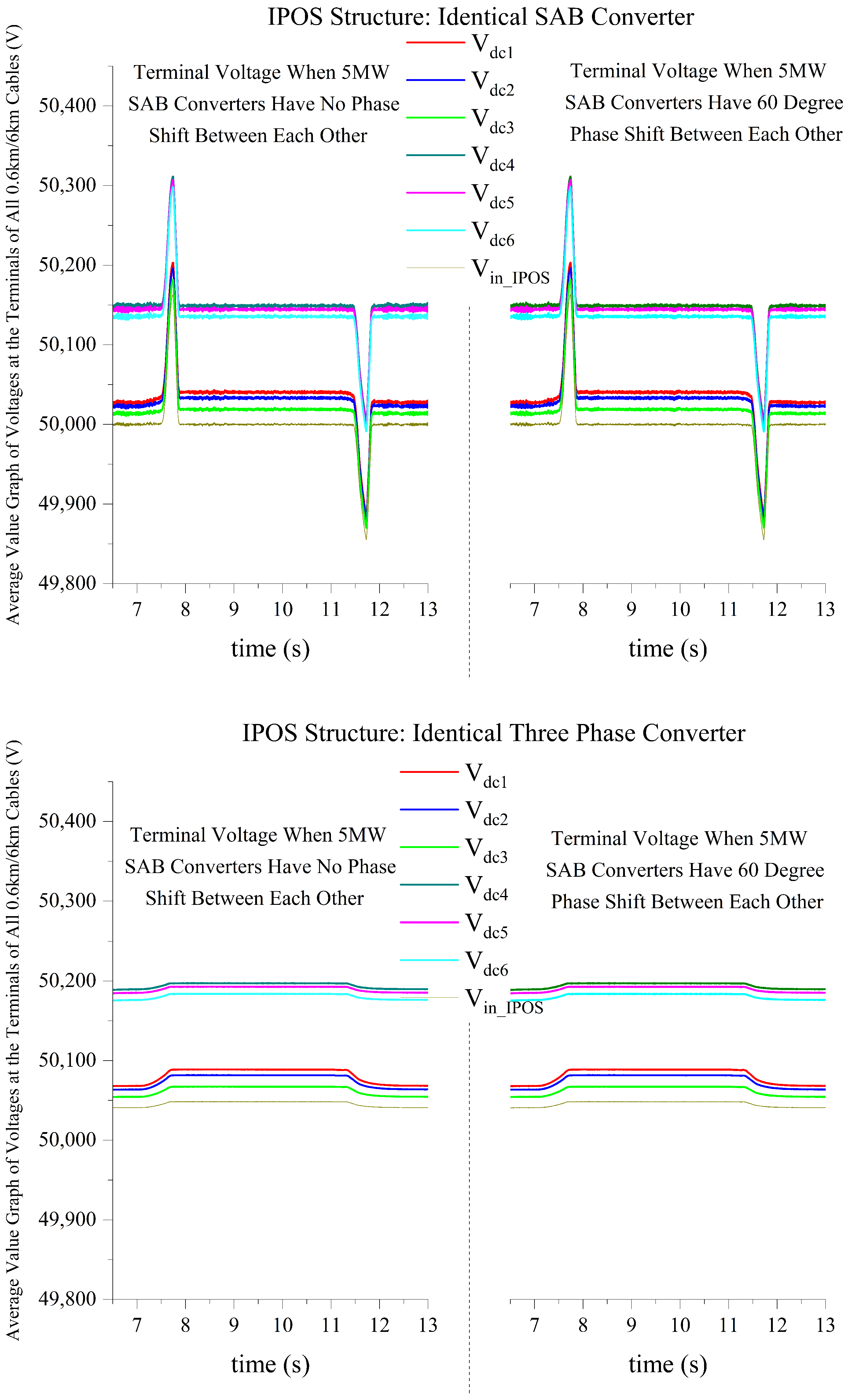
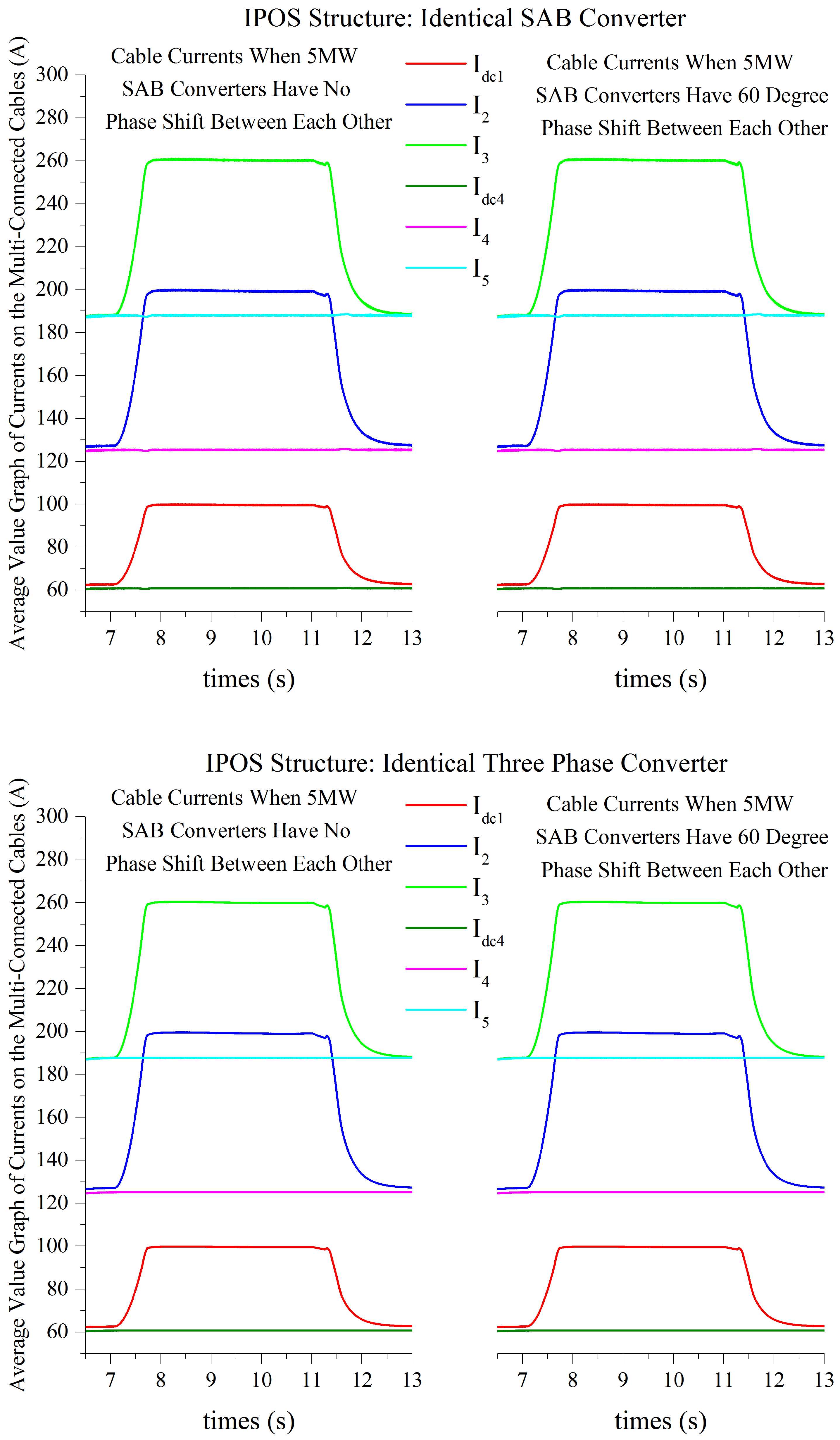
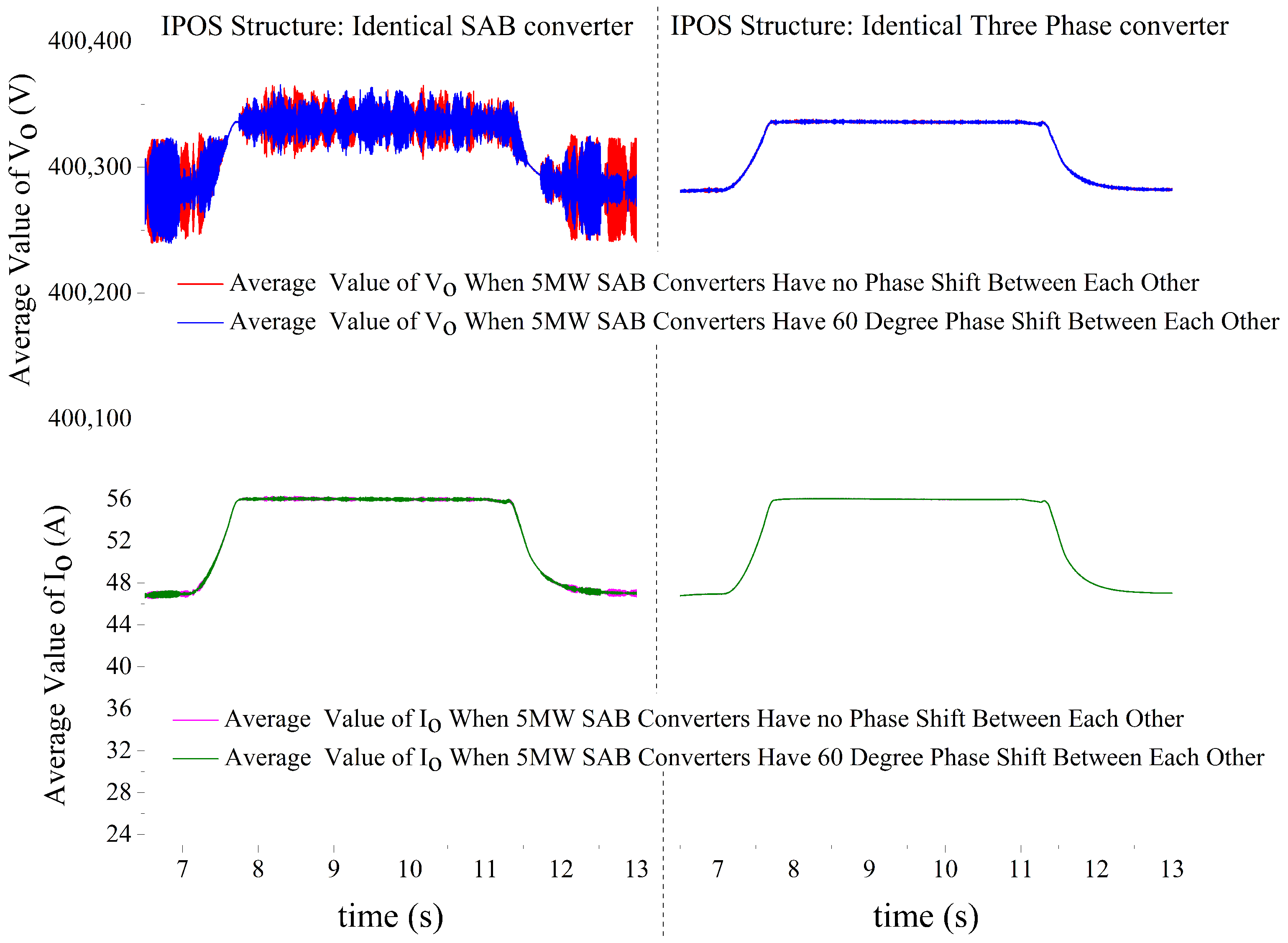
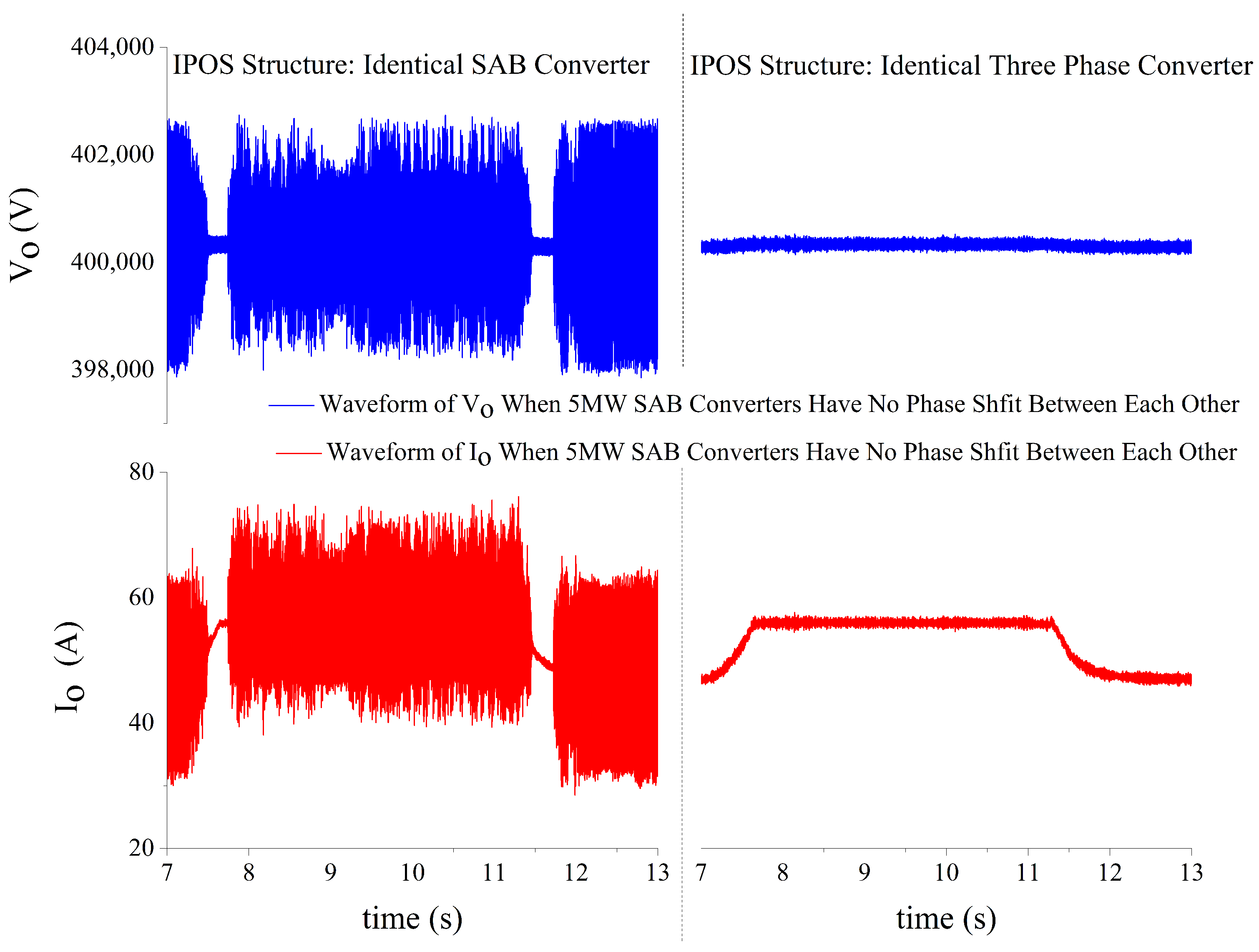
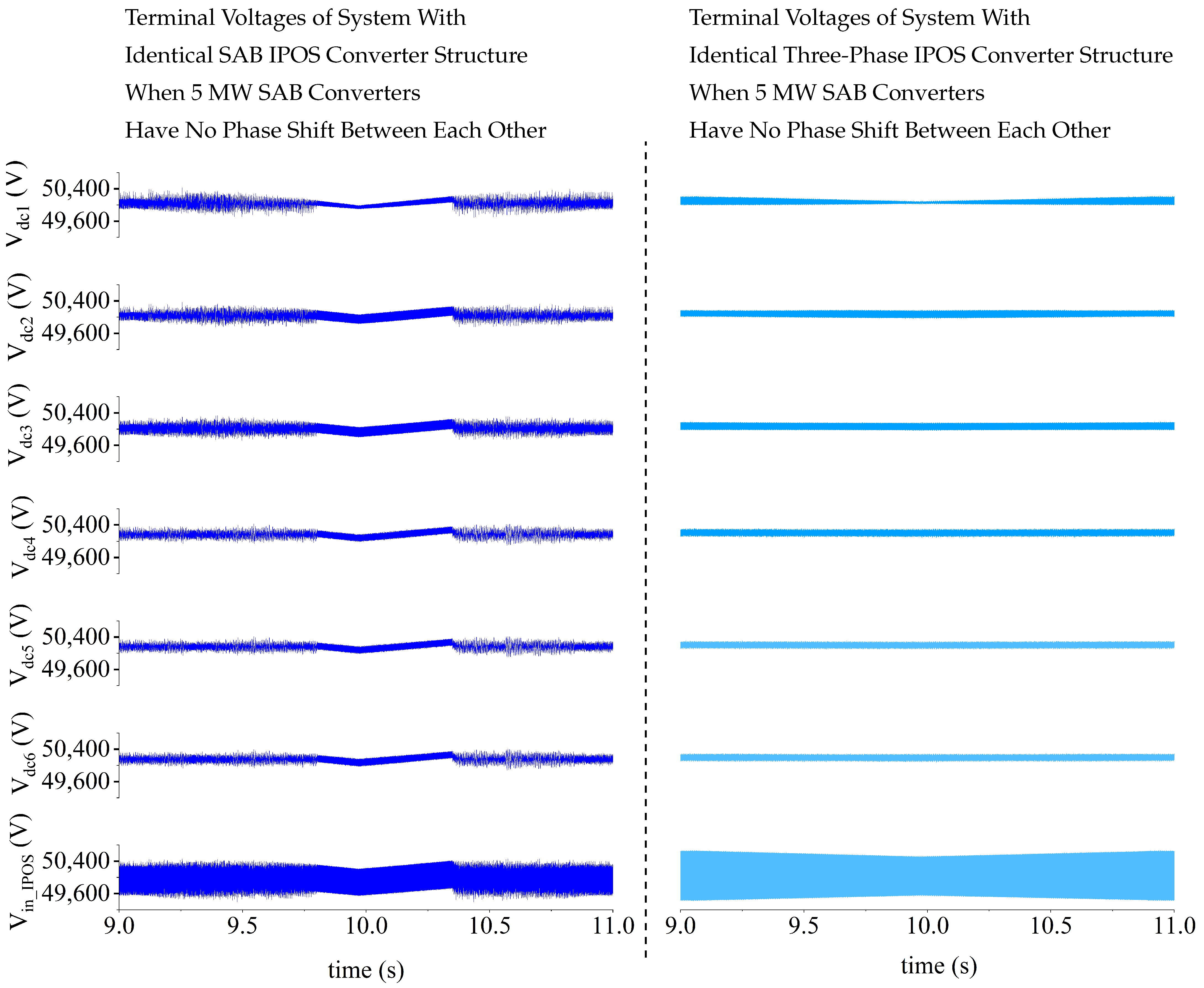
- In Figure 8, for a system with the Identical SAB IPOS structure, the DC value of Vin_IPOS is controlled well at 50 kV, while that of the system with the Identical Three-Phase IPOS structure is mostly dependent on the DC voltage value at the receiving end of the system and the current flow through the transmission cables. It can be noticed that the value of Vin_IPOS in the system with the Identical Three-Phase IPOS structure increases with increasing wind power; this is due to an increase in the DC component/average value of Io when more power is fed into the system, leading to a higher voltage drop on the 100-km transmission cables;
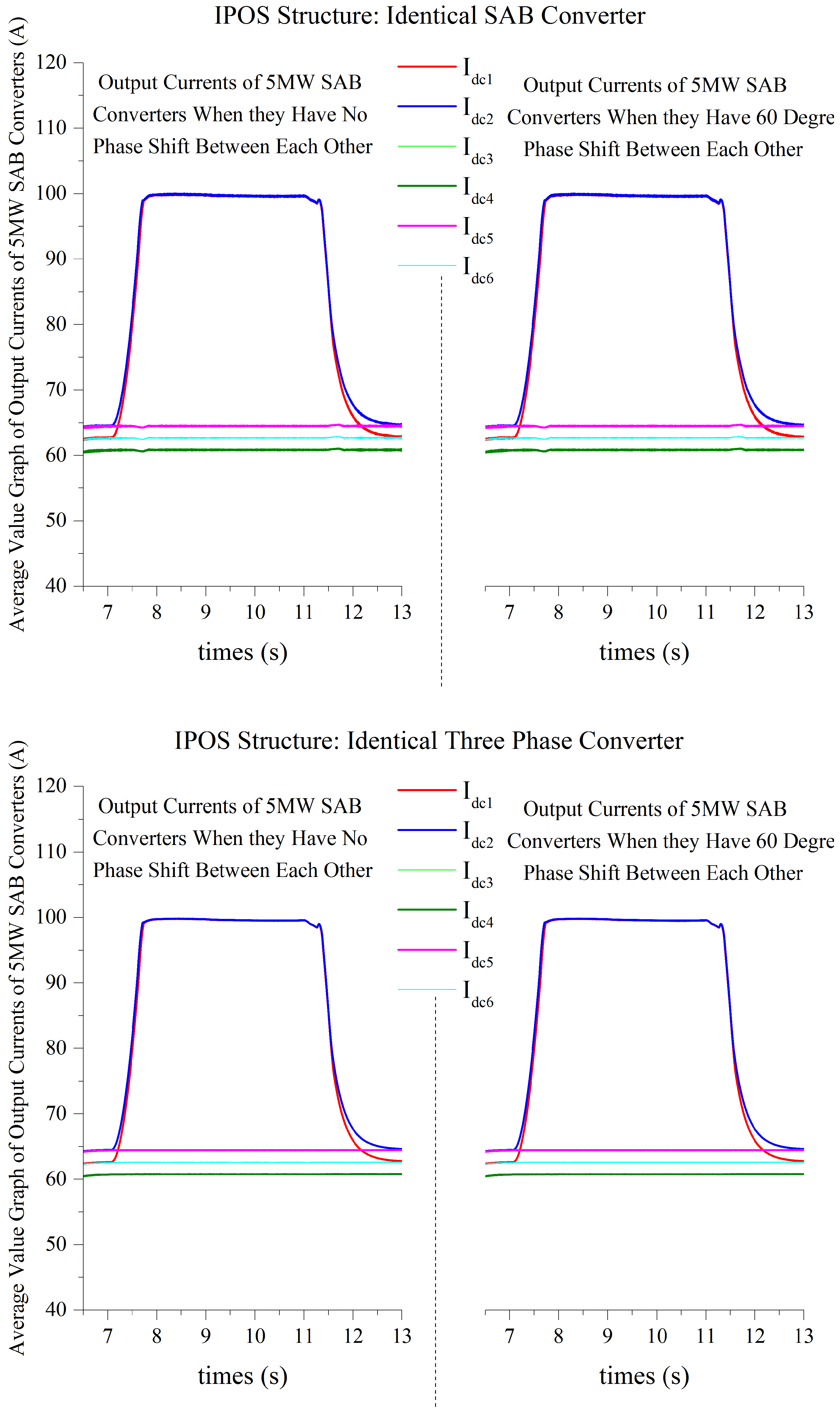
- The current superposition along the multi-connected cables towards the receiving end of the system is shown in Figure 9 and Figure 10. These cable currents result in a voltage drop on the multi-connected cables, which can be seen in Figure 8. It should be noted that the 6-km cable with I5 flowing through it undergoes a higher voltage drop in the system, as its equivalent resistance is ten times higher than that of the 0.6-km cables;
- Figure 13 shows the waveform of Vdc1 and Idc1 when the Identical Three-Phase IPOS structure is used in the system. When comparing Figure 5 with Figure 13, it is clear that, during the time when the captured wind power is increasing or decreasing, the terminal voltages and currents have lower ripple content but a higher or lower DC value in the system with the closed loop-controlled SAB IPOS structure, which also explains the ”average voltage spike” in Figure 8. The appearance of the reduced ripple content with the controllable Identical SAB IPOS converter is because of the variation in the duty ratio of the IPOS converter, which can lead to phase changes in the ripple content;
- As expected, in Figure 11 and Figure 12, the average power transferred to the onshore power system is similar in systems with different IPOS converter structures under the same wind condition. Nonetheless, the advantage of reduced cable ripple content in the system employing the Identical Three-Phase IPOS structure is distinctly evident. A more detailed FFT analysis of the system under this wind condition is presented in Section 3.4.
3.1.4. FFT Analysis of DC Cable Voltage and Current AC Ripples
3.2. Simulation Results of the System with Identical Three-Phase and Identical SAB IPOS Converter Structures under Wind Condition 2
3.2.1. Wind Condition 2
3.2.2. Simulation Results of Vdc1 and Idc1 under Wind Condition 2
3.3. Simulation Results of the System with Identical Three-Phase and Identical SAB IPOS Converter Structures under Wind Condition 3
3.3.1. Wind Condition 3
3.3.2. Simulation Results of Vdc1 and Idc1 under Wind Condition 3
3.4. Comparison of the Simulation Results of the Double Branch System under Wind Condition 1–3
- The THD of all terminal voltages is well below 2% even though the FFT analyses were based on the region with a relatively high ripple.
- The Total Relative AC Losses on multi-section cables increased with the increase in the total power transferred; however, the efficiency relative to the AC losses on the multi-section cables is independent of the total power transferred.
- The Total Relative AC Losses on the 100-km cable did not follow the variation in the total power transferred in the system but remained approximately constant. Therefore, the efficiency of the system can be enhanced if more power is extracted from the wind.
- Referring to Equation (A3) in Appendix D, the referred value of the Total Relative AC Losses for the 100-km cable is listed in Table 8 as 100 . It is shown that the 100-km cable’s relative AC losses in the system with the Identical SAB IPOS converter structure were about twenty times those of the Identical Three-Phase IPOS converter or about 50 kW higher in the system with six turbines. However, for a system with an Identical SAB IPOS converter structure, the main losses occur in the 100-km submarine cables, and they are much greater than the losses for the multi-connected cables. As the losses for the 100-km cable do not increase with an increasing power transfer, the superiority of the system with the Identical Three-Phase IPOS converter structure will reduce with an increase in the number of turbines, branches, or transferred power, but it will remain superior.
3.5. Simulation Results of the System with Identical Three-Phase and Identical SAB IPOS Converter Structures under Wind Condition 4: Cut-Off
3.5.1. Wind Condition 4
3.5.2. Simulation Results of All Terminal Voltages and Cable Currents under Wind Condition 4 with Cut-Off
4. Conclusions
- The total harmonic distortion of all the terminal voltages can be limited to well below 2% in any type of wind condition, with different IPOS converter structures when proper filter capacitors are applied in the system. This result is much better than the expectation, especially when looking at the terminal voltages at the multi-connected cables of the system, as relatively high current ripple content and the superposition of the current may cause high voltage ripple content.
- At all times, a higher power transfer amounts to little more than higher losses in the system. However, in this study, it was found that the AC losses for the 100-km submarine cable did not increase with an increase in the total power transferred, and they remained stable under all conditions.
- The AC losses for the multi-connected submarine cables were in direct proportion to the total power transferred, which is the normal situation.
- Gust winds and interactions between the wind turbines in a wind farm do not cause significant ripple content or AC losses in cables. Before this study, it was opined that the variation in wind speed may produce higher cable ripple content. However, the results lead to the opposite conclusion, and hence, variable wind does not need to be included in the study of the ripple content of cables in future work. The variation in wind speed and the interaction between wind turbines can still influence the power that can be absorbed by the turbines, and it must be considered when looking at the power transfer ability of the system.
- The system has relatively good recoverability when the wind speed is below the cut-in speed or above the cut-out speed for a short period of time, based on the fact that unexpected high or low wind occurs only occasionally and will only impact a few turbines at a large wind farm system during a short period. During the cut-off time, no significant high ripple content or AC losses occur, ruling out the concern before the study.
- Compared with the closed loop controlled SAB IPOS converter structure, the open loop duty ratio controlled Three-Phase IPOS converter can help reduce the value and size of passive filters, leading to the lowest cable ripple content and AC losses, and it can have a high power carrying ability. Against this background, if the onshore power system at the receiving end is robust enough or can always provide a relatively constant voltage value, the Identical Three-Phase ISIPOS converter should be used in the system. However, in a system whose receiving end is likely to fluctuate, the closed loop input voltage-controlled SAB converter should be applied in the IPOS converter structure of the system.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Possible Wind Conditions at Each Wind Turbine under Different Natural Wind Situations

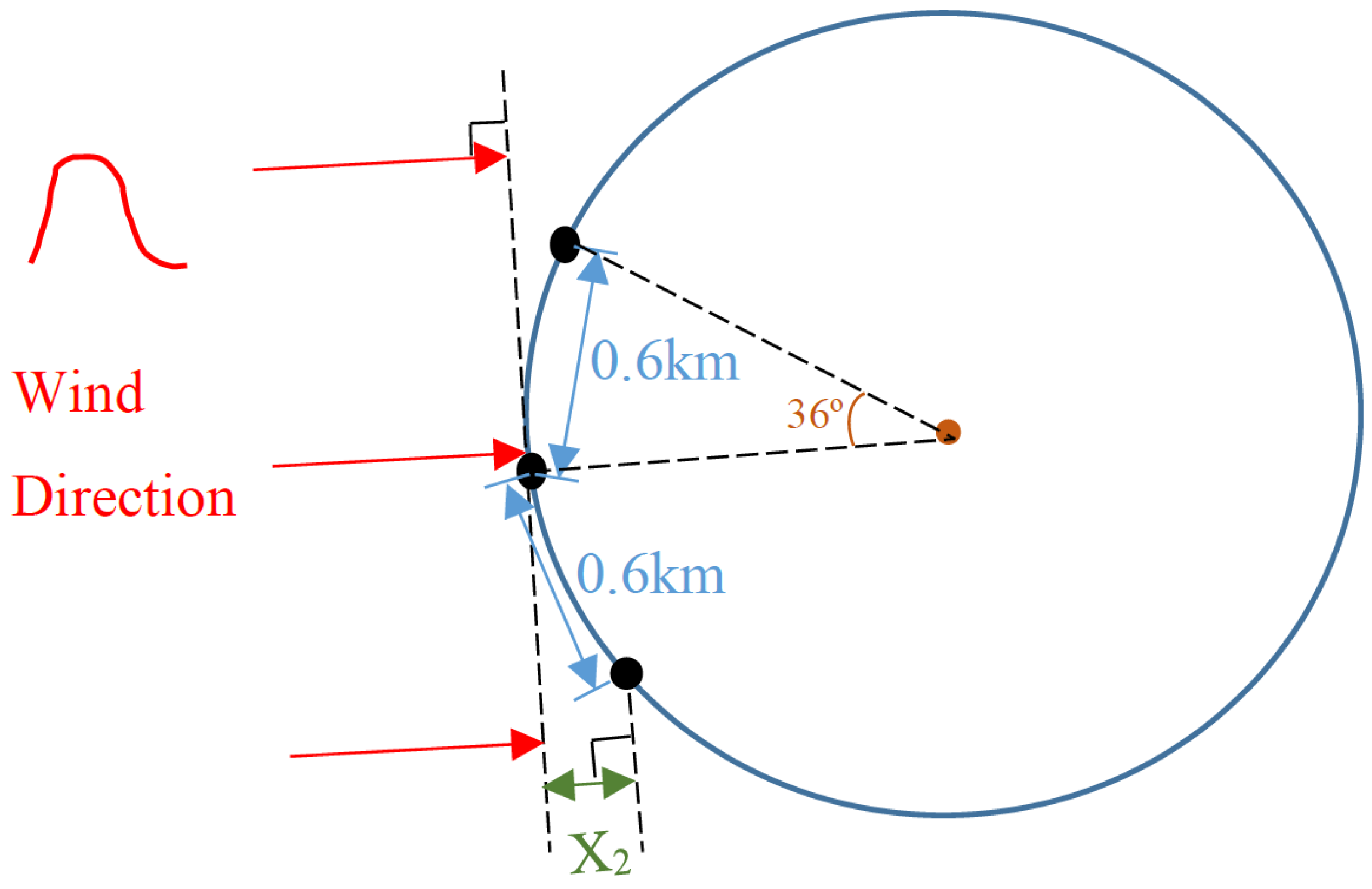
- In Figure 1, turbine 1 and turbine 2 are affected by the bluster simultaneously, while all other turbines are working under a steady free stream;
- In Figure 1, turbine 4 and turbine 5 are affected by the bluster simultaneously, and turbine 1 is moved by the bluster with a few seconds’ delay, while all the other turbines are working under a steady free stream;
- In Figure 1, turbine 2, turbine 3, turbine 4, and turbine 5 are influenced by an asynchronous bluster, while all the other turbines are working under a steady free stream;
- In Figure 1, turbine 1 is affected by the bluster, which surpasses the cut-out speed, while all the other turbines are working under a steady free stream.
Appendix B. Natural Wind Example
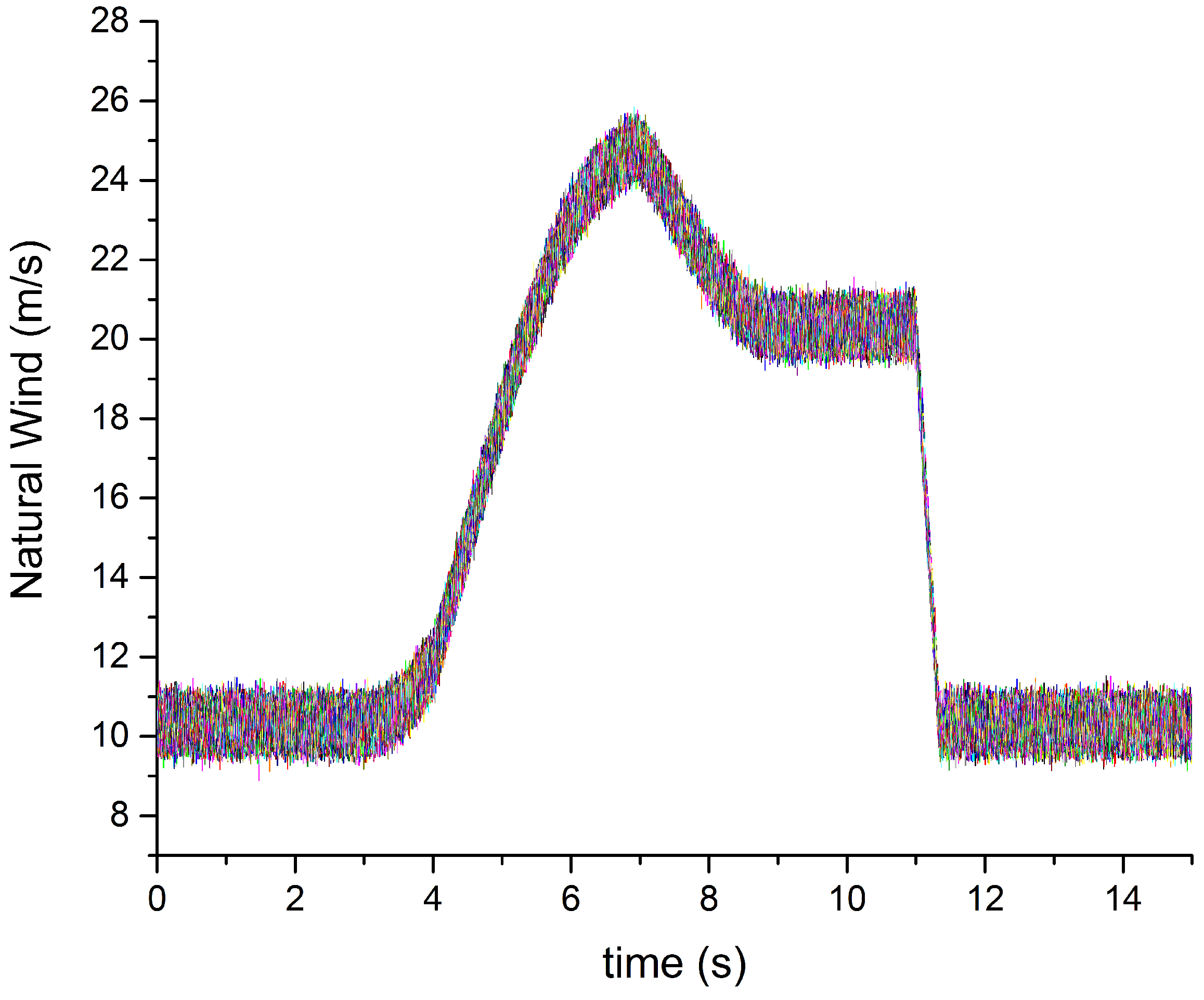
Appendix C. FFT Analysis under WC 1 with Identical SAB IPOS Converter Structure
| Converter Type: Balanced (Identical SAB) IPOS Converter Base Value: Vdc = 50 kV Swb = 10.31 m/s Power = 3.2 MW Idc = 63 A Non: 5 MW SAB No Phase Shift 60: 5 MW SAB 60 degree Phase Shift 5 MW SAB Output Capacitor Value: 40 F 30 MW IPOS Converter Input Capacitor Value: 4 F | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Frequency | 4 kHz | 8 kHz | 12 kHz | 16 kHz | ||||||
| HP * % | Non | 60 | Non | 60 | Non | 60 | Non | 60 | ||
| V/I | ||||||||||
| Vdc1 | 0.14 | 0.13 | 0.03 | 0.03 | 0.01 | 0.01 | 0.01 | 0.01 | ||
| Vdc2 | 0.18 | 0.18 | 0.04 | 0.04 | 0.01 | 0.01 | 0.01 | 0.01 | ||
| Vdc3 | 0.16 | 0.12 | 0.03 | 0.03 | 0.01 | 0.01 | 0.01 | 0.01 | ||
| Vdc4 | 0.13 | 0.14 | 0.03 | 0.03 | 0.01 | 0.01 | 0.01 | 0.01 | ||
| Vdc5 | 0.13 | 0.16 | 0.03 | 0.03 | 0.01 | 0.01 | 0.01 | 0.01 | ||
| Vdc6 | 0.13 | 0.14 | 0.03 | 0.03 | 0.01 | 0.01 | 0.01 | 0.01 | ||
| Vin_IPOS | 0.2 | 0.15 | 0.01 | 0.01 | 0.4 | 0.4 | 0 | 0.01 | ||
| Idc1 | 4.2 | 15.5 | 0.2 | 2.69 | 0.05 | 0.83 | 0.02 | 0.29 | ||
| I2 | 21.26 | 6.7 | 2.6 | 0.63 | 0.8 | 0.01 | 0.13 | 0.15 | ||
| I3 | 30.38 | 21.5 | 1.75 | 1.09 | 11.8 | 11.83 | 0.08 | 0.22 | ||
| Idc4 | 1.78 | 15.62 | 0.34 | 2.28 | 0.14 | 0.6 | 0.07 | 0.18 | ||
| I4 | 1.21 | 13.76 | 0.18 | 2.08 | 0.12 | 0 | 0.04 | 0.24 | ||
| I5 | 2.75 | 1.59 | 0.13 | 0.28 | 6.08 | 0 | 0.02 | 0.07 | ||
Appendix D. Normalization of the Relative Losses
References
- Wu, C.; Zhang, X.; Sterling, M. Wind power generation variations and aggregations. CSEE J. Power Energy Syst. 2022, 10, 17–38. [Google Scholar]
- Bashir, M.; Bashir, A. Principle Parameters and Environmental Impacts That Affect the Performance of Wind Turbine: An Overview. Arab. J. Sci. Eng. 2022, 47, 7891–7909. [Google Scholar] [CrossRef] [PubMed]
- Saidur, R.; Rahim, N.A.; Islam, M.R.; Solangi, K.H. Environmental impact of wind energy. Renew. Sustain. Energy Rev.L 2011, 15, 2423–2430. [Google Scholar] [CrossRef]
- Díaz, H.; Soares, C.G. Review of the current status, technology and future trends of offshore wind farms. Ocean. Eng. 2020, 209, 10783. [Google Scholar] [CrossRef]
- Hu, M.; Xie, S.; Zhang, J.; Ma, Z. Desing selection of DC & AC submarine power cable for offshore wind mill. In Proceedings of the 2014 China International Conference on Electricity Distribution (CICED), Shenzhen, China, 22 December 2014. [Google Scholar]
- Ryndzionek, R.; Sienkiewicz, T. Evolution of the HVDC Link Connecting Offshore Wind Farms to Onshore Power Systems. Energies 2020, 13, 1914. [Google Scholar] [CrossRef]
- Muljadi, E.; Singh, M.; Gevorgian, V. Doubly Fed Induction Generator in an Offshore Wind Power Plant Operated at Rated V/Hz. IEEE Trans. Ind. Appl. 2013, 49, 2197–2205. [Google Scholar] [CrossRef]
- Rong, X.; Shek, J.K.H.; Macpherson, D.E.; Mawby, P. The Effects of Filter Capacitors on Cable Ripple at Different Sections of the Wind Farm Based Multi-Terminal DC System. Energies 2021, 14, 7000. [Google Scholar] [CrossRef]
- Ashrafi, N.S.H.; Hosseini, S.M.; Abdoos, A.A. Fault detection of HVDC cable in multi-terminal offshore wind farms using transient sheath voltage. IET Renew. Power Gener. 2017, 11, 1707–1713. [Google Scholar] [CrossRef]
- Sun, J.; Debnath, S.; Bloch, M.; Saeedifard, M. A Hybrid DC Fault Primary Protection Algorithm for Multi-Terminal HVdc Systems. IEEE Trans. Power Deliv. 2022, 37, 1285–1294. [Google Scholar] [CrossRef]
- Panahi, H.; Sanaye-Pasand, M.; Niaki, S.H.A.; Zamani, R. Fast Low Frequency Fault Location and Section Identification Scheme for VSC-Based Multi-Terminal HVDC Systems. IEEE Trans. Power Deliv. 2022, 37, 2220–2229. [Google Scholar] [CrossRef]
- Pourmirasghariyan, M.; Zarei, S.F.; Hamzeh, M.; Blaabjerg, F. A Power Routing-Based Fault Detection Strategy for Multi-Terminal VSC-HVDC Grids. IEEE Trans. Power Deliv. 2023, 38, 528–540. [Google Scholar] [CrossRef]
- Vasconcelos, L.A.; Passos Filho, J.A.; Marcato, A.L.M.; Junqueira, G.S. A Full-Newton AC-DC Power Flow Methodology for HVDC Multi-Terminal Systems and Generic DC Network Representation. Energies 2021, 14, 1658. [Google Scholar] [CrossRef]
- Wang, P.; Liu, P.; Gu, T.; Jiang, N.; Zhang, X. Small-Signal Stability of DC Current Flow Controller Integrated Meshed Multi-Terminal HVDC System. IEEE Trans. Power Syst. 2023, 38, 188–203. [Google Scholar] [CrossRef]
- Liao, Y.; Wu, H.; Wang, X.; Ndreko, M.; Dimitrovski, R.; Winter, W. Stability and Sensitivity Analysis of Multi-Vendor, Multi-Terminal HVDC Systems. IEEE Open J. Power Electron. 2023, 4, 52–66. [Google Scholar] [CrossRef]
- Li, J.; Li, Y.; Xiong, L.; Jia, K.; Song, G. DC Fault Analysis and Transient Average Current Based Fault Detection for Radial MTDC System. IEEE Trans. Power Deliv. 2020, 35, 1310–1320. [Google Scholar] [CrossRef]
- Deng, W.; Pei, W.; Zhuang, Y.; Zhuang, X. Interaction Behavior and Stability Analysis of Low-Voltage Multi-Terminal DC System. IEEE Trans. Power Deliv. 2022, 37, 3555–3566. [Google Scholar] [CrossRef]
- Dey, S.; Bhattacharya, T.; Samajdar, D. A Modular DC-DC Converter as a Hybrid Interlink between Monopolar VSC and Bipolar LCC-Based HVDC Links and Fault Management. IEEE Trans. Ind. Appl. 2023, 59, 4342–4352. [Google Scholar] [CrossRef]
- Ye, S.; Huang, R.; Xie, J.; Ou, J.J. A Power Flow Calculation Method for Multi-Voltage Level DC Power Grid Considering the Control Modes and DC/DC Converter. IEEE Access 2023, 11, 98182–98190. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, X.; Mehrabankhomartash, M.; Saeedifard, M.; Meng, Y.; Wang, X. Harmonic Stability Assessment of Multiterminal DC (MTDC) Systems Based on the Hybrid AC/DC Admittance Model and Determinant-Based GNC. IEEE Trans. Power Electron. 2021, 37, 1653–1665. [Google Scholar]
- Guo, H.; Guo, Q.; Guo, T.; Huang, L.; Deng, L.; Lu, Y.; Zhuo, F. Mechanism analysis and suppression method of high frequency harmonic resonance in VSC-HVDC. Elect. Power Energy Syst. 2022, 143, 108486. [Google Scholar] [CrossRef]
- Zhu, B.; Chen, X.; Luo, X. VSC control strategy for HVDC compensating harmonic components. In Proceedings of the 3rd International Conference on Power Engineering (ICPE 2022), Hainan, China, 9–11 December 2022. [Google Scholar]
- Ni, Q.; Luo, L.; Fan, J.; Jin, Z. Harmonic loss analysis of converter transformer in LCL-HVDC system. In Proceedings of the 7th International Conference on Power and Energy Systems Engineering (CPESE 2020), Fukuoka, Japan, 26–29 September 2020. [Google Scholar]
- Zheng, Y.; Li, X.; Huang, H. Harmonic Characteristics of HVDC Transmission System and Its Suppression Method System. J. Phys. Conf. Ser. 2022, 2401, 12045. [Google Scholar] [CrossRef]
- Liu, D.; Li, X.; Cai, Z. Analysis of the Harmonic Transmission Characteristics of HVDC Transmission Based on a Unified Port Theory Model. IEEE Access 2020, 8, 8922–8934. [Google Scholar] [CrossRef]
- Li, P.; Wang, Y.; Li, X.; Yue, B.; Li, R.; Feng, B.; Yin, T. DC Impedance Modeling and Design-Oriented Harmonic Stability Analysis of MMC-PCCF-Based HVDC System. IEEE Trans. Power Electron. 2022, 37, 4301–4319. [Google Scholar] [CrossRef]
- Ji, K.; Pang, H.; Yang, J.; Tang, G. DC Side Harmonic Resonance Analysis of MMC-HVDC Considering Wind Farm Integration. IEEE Trans. Power Deliv. 2021, 36, 254–266. [Google Scholar] [CrossRef]
- Wei, Y.; Han, S.; Shi, S. The Modelling and Simulation of the Combined Wind Speed in the Wind Power System. Renew. Energy Resour. 2010, 28, 3. [Google Scholar]
- Rong, X. The Study of the Offshore Wind Farm Based Multi-Terminal DC System and the Application of High Power DC-DC Converters. Ph.D. Thesis, University of Edinburgh, Edinburgh, UK, 2019. [Google Scholar]
- Whittington, H.W.; Flynn, B.W.; Macpherson, D.E. Introduction and Basic Circuits, 2nd ed.; Switched Mode Power Supplies: UK, 1997; pp. 7–57. [Google Scholar]
- Park, K.; Chen, Z. Control and dynamic analysis of a parallel-connected single active bridge DC–DC converter for DC-grid wind farm application. IET Power Electron. 2015, 8, 665–671. [Google Scholar] [CrossRef]
- Rong, X.; Shek, J.K.H.; Macpherson, D.E. The study of different unidirectional input parallel output series connected DC-DC converters for wind farm based multi-connected DC system. Int. Trans. Electr. Energy Syst. 2021, 5, e12855. [Google Scholar] [CrossRef]
- 4C-Offshore. Hywind Scotland Pilot Park Offshore Wind Farm. Available online: http://www.4coffshore.com/windfarms/hywind-scotland-pilot-park-united-kingdom-uk76.html (accessed on 6 January 2022).
- Veerachary, M.; Saxena, A.R. Optimized Power Stage Design of Low Source Current Ripple Fourth-Order Boost DC–DC Converter: A PSO Approach. IEEE Trans. Ind. Electron. 2014, 62, 1491–1502. [Google Scholar] [CrossRef]
- Wang, B.; Kanamarlapudi, V.R.K.; Xian, L.; Peng, X.; Tan, K.T.; So, P.L. Model Predictive Voltage Control for Single-Inductor Multiple-Output DC–DC Converter With Reduced Cross Regulation. IEEE Trans. Ind. Electron. 2016, 35, 4187–4197. [Google Scholar] [CrossRef]
- Woodmead-Energy-Service. Wind Turbine Planning Application. Available online: https://woodmeadenergyservices.com/renewable-energy/wind-turbine-planning-application/ (accessed on 8 January 2023).






| Wind Turbine (WT) | |||||
|---|---|---|---|---|---|
| Type | Power Rating | Cut-In Wind Speed | Cut-Out Wind Speed | Rated Wind Speed | Nominal Rotation Speed |
| AD5135 | 5 MW | 5 m/s | 25 m/s | 12 m/s | 12 rpm |
| Pulse Width Modulation Rectifier (PWM Rectifier) 1 | |||
|---|---|---|---|
| Vline_rms | Iline_rms | Power Rating | Output DC Voltage |
| 2.6 kV | 1.1 kA | 5 MW | 5 kV |
| 5 MW DC–DC Converter 1 | ||||
|---|---|---|---|---|
| Converter Type | Input Voltage | Output Voltage | Power Rating | Switching Frequency |
| Single Active Bridge (SAB) | 5 kV | 50 kV | 5 MW | 2 kHz |
| Input Series Input Parallel Output Series (ISIPOS) DC–DC Converter * | ||||
|---|---|---|---|---|
| Converter Type | Input Voltage | Output Voltage | Power Rating | Switching Frequency |
| Identical Three-Phase ISIPOS converter OR Identical SAB ISIPOS converter OR SAB+Three-Phase ISIPOS converter | 50 kV | 400 kV | 200∼1000 MW (reduced to 30 MW in this simulation work) | SAB converter: 2 kHz Three-Phase converter: 667 Hz |
| ±25 kV DC Cables 1 | ||
|---|---|---|
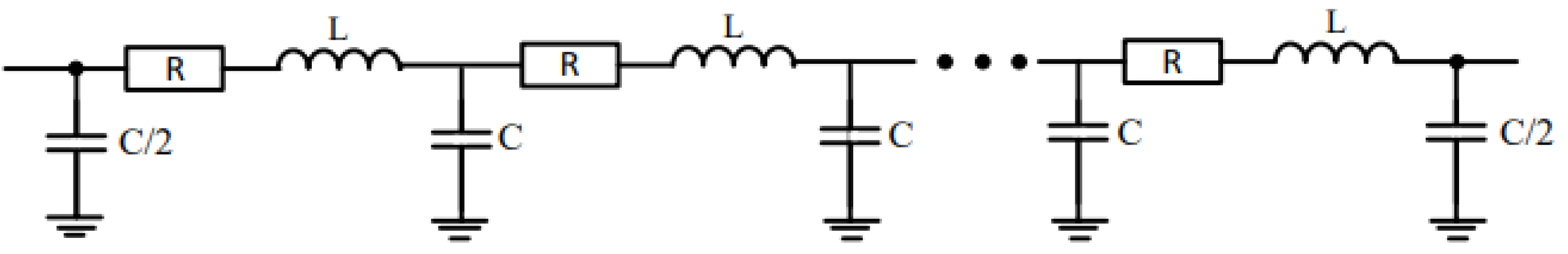
| Cable Length of Each Branch | Power Rating of the Cable of Each Branch | Cable RLC Parameters |
| 0.6x km (x is the number of turbines in each branch | 5x MW | R = 60 m/km L = 0.3 mH/km C = 0.2 F/km |
| ±200 kV DC Cables 1 | ||
|---|---|---|
| Cable Length | Power Rating of the Cable | Cable RLC Parameters |
| 100 km | 5y MW (y is the number of turbines in the whole offshore wind farm) | R = 30 m/km L = 0.8 mH/km C = 0.13 F/km |
| Base Value: Vdc = 50 kV Power = 3.2 MW Idc = 63 A 5 MW SAB Output Capacitor Value: 40 F IPOS Converter Input Capacitor Value: 0.4 F (TP) or 4 F (SAB) | ||||||
|---|---|---|---|---|---|---|
| WC 1 | WC 2 | WC 3 | ||||
| SAB | TP | SAB | TP | SAB | TP | |
| THD Vdc1 (%) | 0.3 | 0.15 | 0.32 | 0.17 | 0.26 | 0.14 |
| THD Vdc2 (%) | 0.3 | 0.18 | 0.31 | 0.13 | 0.26 | 0.19 |
| THD Vdc3 (%) | 0.27 | 0.15 | 0.29 | 0.16 | 0.26 | 0.2 |
| THD Vdc4 (%) | 0.19 | 0.15 | 0.23 | 0.16 | 0.18 | 0.17 |
| THD Vdc5 (%) | 0.19 | 0.15 | 0.27 | 0.16 | 0.19 | 0.16 |
| THD Vdc6 (%) | 0.18 | 0.15 | 0.26 | 0.15 | 0.17 | 0.16 |
| THD Vin_IPOS (%) | 0.51 | 0.03 | 0.51 | 0.03 | 0.52 | 0.03 |
| TRL-MS | 7.5 k | 0.8 k | 8.9 k | 0.9 k | 7.8 k | 1.8 k |
| Base Value: Vdc = 400 kV Power = 18.9 MW Idc = 47 A 5 MW SAB Output Capacitor Value: 40 F IPOS Converter Input Capacitor Value: 0.4 F (TP) or 4 F (SAB) | ||||||
|---|---|---|---|---|---|---|
| WC 1 | WC 2 | WC 3 | ||||
| SAB | TP | SAB | TP | SAB | TP | |
| THD Vo (%) | 0.28 | 0.2 | 0.38 | 0.02 | 0.32 | 0.01 |
| 100 kmTL | 90 | 0.32 | 92 | 0.9 | 92 | 1 |
| 100 | 4.2 k | 15 | 4.3 k | 42 | 4.3 k | 46 |
| Io(DC)/Ibase (%) * | 119 | 119 | 123 | 129 | 140 | 139 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rong, X.; Shek, J.K.H.; Macpherson, D.E.; Mawby, P. The Study of Multi-Terminal DC Systems in an Offshore Wind Environment: A Focus on Cable Ripple Analysis. Energies 2024, 17, 1978. https://doi.org/10.3390/en17081978
Rong X, Shek JKH, Macpherson DE, Mawby P. The Study of Multi-Terminal DC Systems in an Offshore Wind Environment: A Focus on Cable Ripple Analysis. Energies. 2024; 17(8):1978. https://doi.org/10.3390/en17081978
Chicago/Turabian StyleRong, Xiaoyun, Jonathan K. H. Shek, D. Ewen Macpherson, and Phil Mawby. 2024. "The Study of Multi-Terminal DC Systems in an Offshore Wind Environment: A Focus on Cable Ripple Analysis" Energies 17, no. 8: 1978. https://doi.org/10.3390/en17081978
APA StyleRong, X., Shek, J. K. H., Macpherson, D. E., & Mawby, P. (2024). The Study of Multi-Terminal DC Systems in an Offshore Wind Environment: A Focus on Cable Ripple Analysis. Energies, 17(8), 1978. https://doi.org/10.3390/en17081978







