Study on Primary Frequency Regulation Reserve Configuration of High Wind Power System Considering Governor Limiting Links
Abstract
1. Introduction
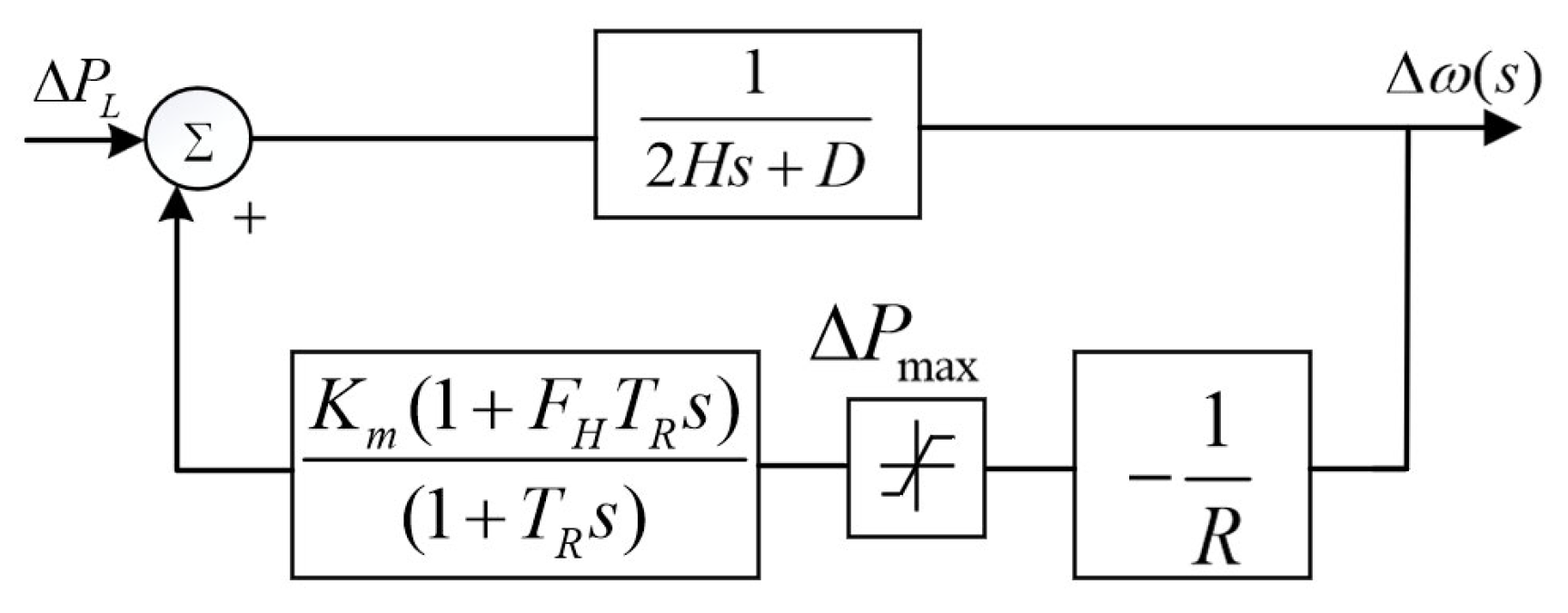
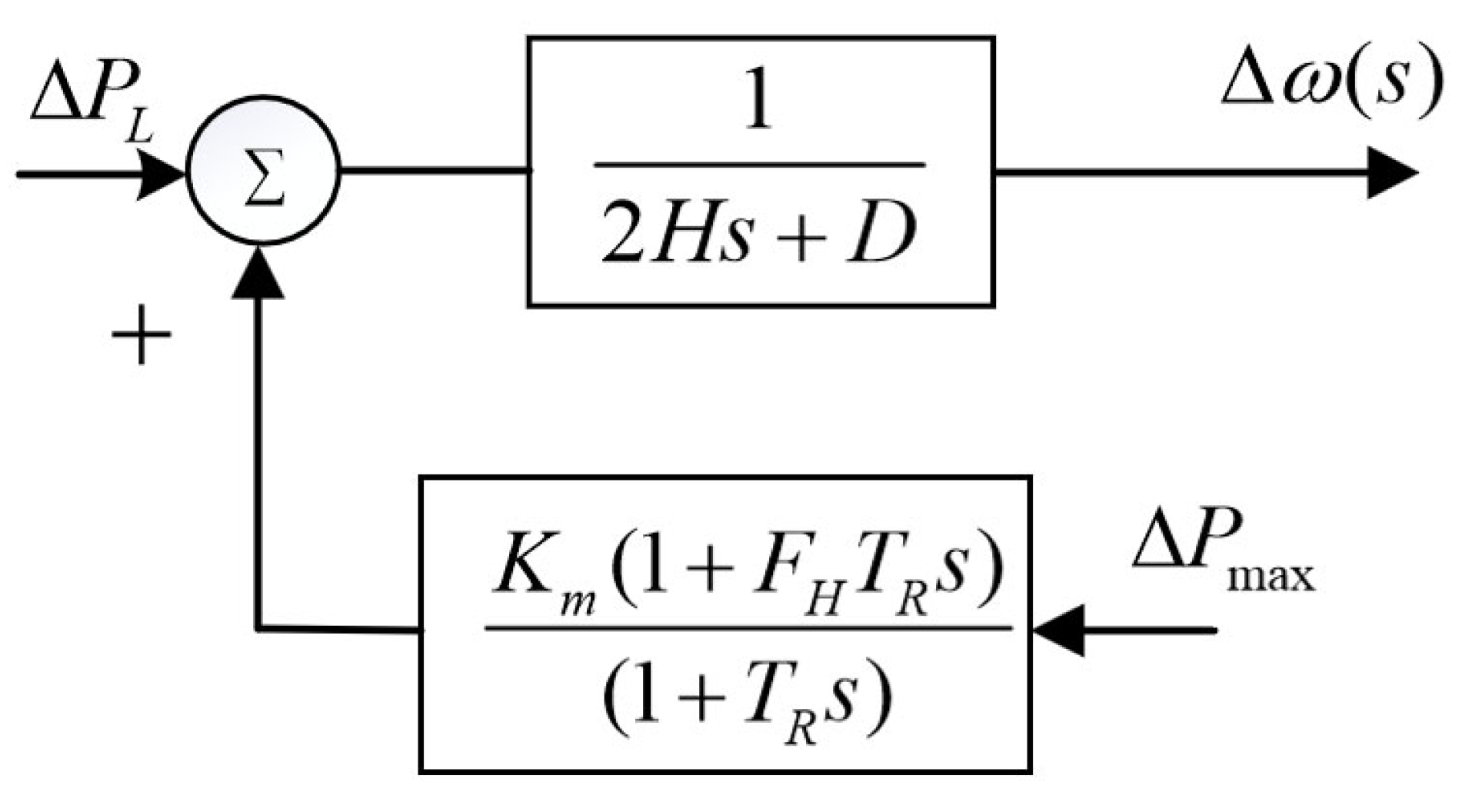
2. The SFR Model of the Power System, Including Wind Power, Taking into Account Primary Frequency Regulation Limiting
2.1. SFR Frequency Domain (S-Domain) Model of a System Containing a High Proportion of Wind Power
2.2. System Single Speed Regulator SFR Frequency Domain (S-Domain) Model Based on the Multi-Machine Aggregation Method
2.3. Single Speed Regulator SFR Frequency Domain (S-Domain) Model of the System Entering the Limiting State
2.4. Time Domain Analytical Model Considering the System Frequency of the Limiting Link
3. Optimization of Primary Frequency Regulation Backup Configuration of New Energy Power Systems: Considering the Limiting Link
3.1. Optimization Model of Primary Frequency Regulation Backup Configuration of Power System
3.1.1. Objective Function
3.1.2. General Constraints
3.2. Construction of Frequency Safety Constraints Based on the SFR Time Domain Model
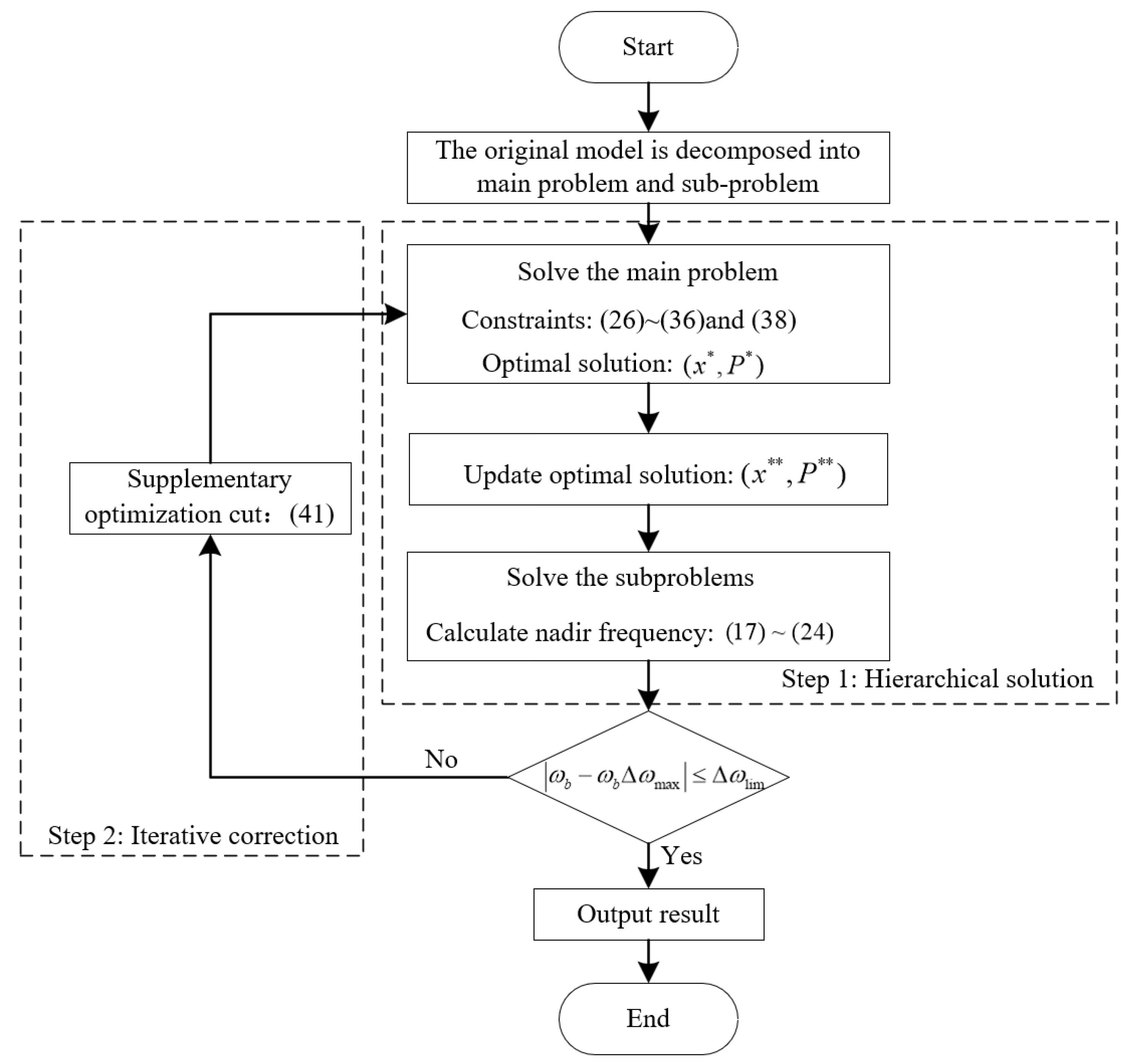
4. Two-Stage Model Solution Method Based on the L-Shape Algorithm
- (1)
- Optimize and solve the main problem. Based on the objective function (26) and constraints (26)–(36) and (38), the start-up and shutdown plan of the unit as well as the unit output and primary frequency regulation reserve capacity can be determined for each period of the day, that is, the optimal solution (), so the system equivalent speed regulator limit in each period can be obtained as:
- (2)
- Solve the sub-problem. Calculate the frequency nadir of each period and the time to reach the lowest frequency according to Equations (17)~(24). For periods that cannot meet the lowest point frequency constraint condition (39), additional optimization cuts are required:where represents the optimal cut after update generation.
- (3)
- Use the generated optimal cut to return to the main problem and re-optimize the solution to obtain a new feasible solution (). When the sum of the output and reserve of all units in the system does not reach the maximum output of the unit, as shown in Equation (42), it means that the frequency response capability of the system can be improved at this time, and the main problem continues to increase the equivalent speed regulation of the system. The direction of the limiter is optimized until the period meets the minimum limit of the dynamic frequency.
- (4)
- Use the newly generated optimal cut to continuously iterate in the direction of increasing the system equivalent speed regulator limit, and repeat the above process until all periods meet the dynamic frequency constraints or the sum of the output and reserve of all units reaches the unit stop at maximum output.
5. Example Analysis
5.1. Introduction to Simulation Examples
5.2. Analysis of Results under Different Scenarios
5.2.1. Primary Frequency Modulation Backup Optimization Economic Indicators
- (1)
- Case 1: This model allocates a primary frequency regulation reserve to each power supply in the powered-on state. Although cost factors are taken into consideration when configuring the reserve, and the frequency modulation reserve is allocated to units with lower reserve costs, the adequacy of the reserve is not considered and is of little reference.
- (2)
- Case 2: Considering the frequency change rate constraint, the number of systems turned on will inevitably increase, and the power generation cost of the system will inevitably change. This scheme combines the lowest frequency constraint obtained by the authors of [11], but requires the speed regulator to work in a linear state and still reserves a proportional frequency modulation for backup. For this reason, the frequency modulation reserve left by each power supply is still relatively large. Compared with option 1, the cost of one frequency modulation reserve is reduced by 0.39%, and the total operating cost is reduced by 0.38%.
- (3)
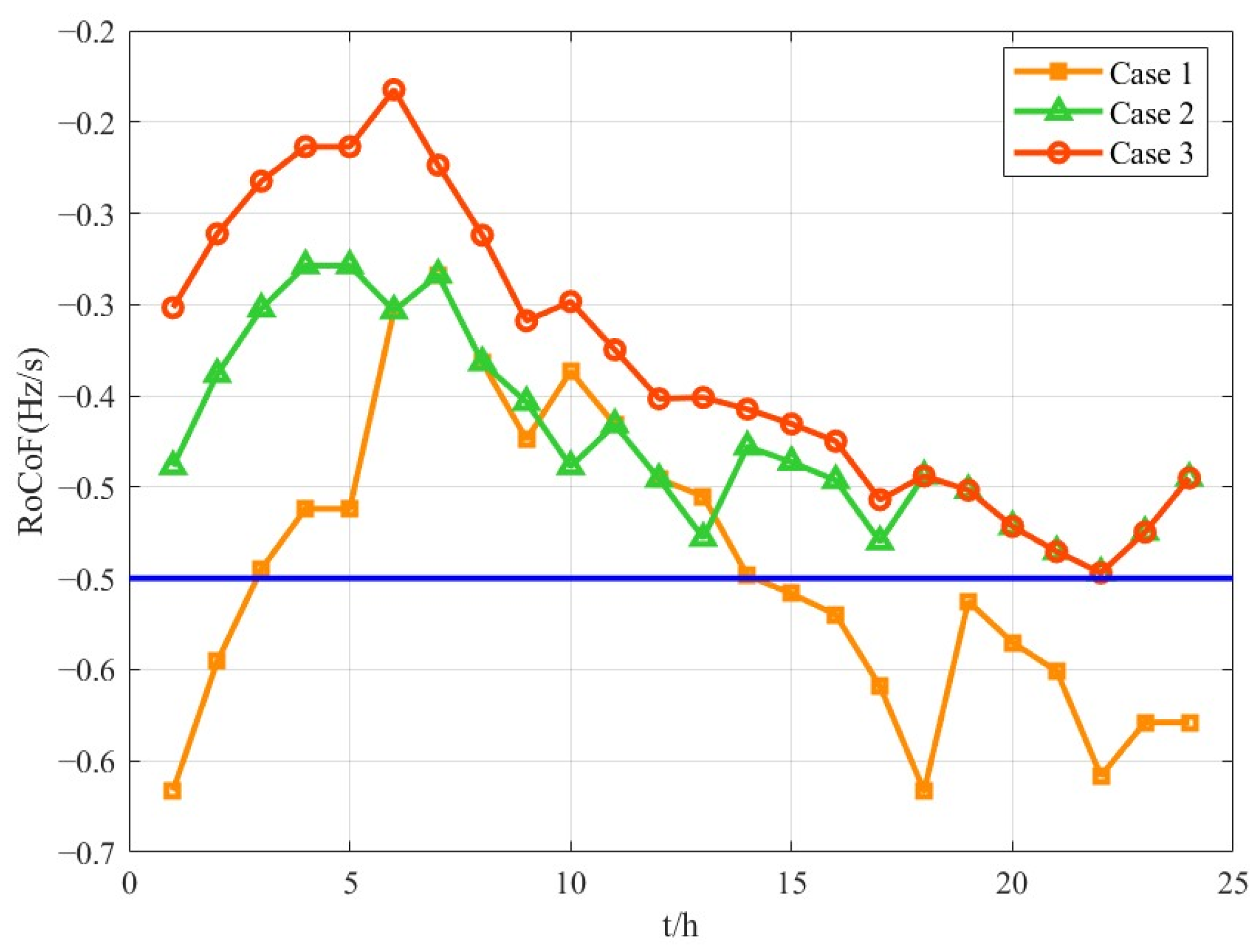
- Case 3: The model considers the frequency stability constraints and the limiting link of each power supply speed regulator. After aggregating the parameters, combined with the judgment that the lowest frequency point will inevitably appear in the speed regulator limiting stage, the primary frequency modulation is realized. Optimization of spare capacity configuration. Since the frequency limit constraint is more stringent, the primary frequency modulation reserve required by the system is bound to increase. Compared with option 1, the cost of the primary frequency modulation reserve increases by 15.15%, and the total operating cost increases by 22.53%. Compared to Case 2, the cost of the primary frequency modulation reserve increased by 15.6%, and the total operating cost increased by 23%. Since the backup is distributed among the units already turned on, after accounting for the limiting link, if the cost of the backup configuration is greater than the operating cost of turning on another unit in the system, the choice is made to turn on the new unit. Comparing Figure 8, Figure 9 and Figure 10, it can be seen that Case 3 has a high demand for frequency modulation backup, which leads to the need to switch on new units in addition to the backup provided by the wind turbines. For example, at 9–12, Unit 8 in Case 3 is operational and provides a percentage of the standby.
5.2.2. System Frequency Stability Verification under Large Disturbance Conditions
6. Conclusions
- (1)
- The constraints established take into account the impact of governor limiting on frequency response, making the estimation of the frequency modulation capability of the system more accurate. This further ensures the effectiveness of the obtained unit combination and primary frequency regulation capacity configuration plan, thereby effectively ensuring the frequency stability of the new energy power system.
- (2)
- Due to the existence of high-order nonlinear terms in the system frequency and extreme point constraints after disturbance, a two-stage iterative solution method based on L-shape is proposed to decompose the nonlinear optimization model into main sub-problems. This method effectively reduces the solution cost of the primary frequency modulation backup configuration model.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Z.Y.; Zhang, N.; Du, E.S.; Kang, C.Q.; Wang, Z.D. Review and Countermeasures on Frequency Security Issues of Power Systems with High Shares of Renewables and Power Electronics. Proc. CSEE 2022, 42, 1–25. [Google Scholar]
- Li, Z.W.; Wu, X.L.; Zhuang, K.Q.; Wang, L.; Mu, Y.C.; Li, B.J. Analysis and Reflection on Frequency Characteristics of East China Grid After Bipolar Locking of “9·19” Jinping-Sunan DC Transmission Line. Autom. Electr. Power Syst. 2017, 41, 149–155. [Google Scholar]
- Sun, H.D.; Xu, T.; Guo, Q.; Li, Y.L.; Lin, W.F.; Yi, J.; Li, W.F. Analysis on Blackout in Great Britain Power Grid on August 9th, 2019 and Its Enlightenment to Power Grid in China. Proc. CSEE 2019, 39, 6183–6191. [Google Scholar]
- GB/T 40595-2021; Guide for Technology and Test on Primary Frequency Control of Grid-Connected Power Resource. State Administration for Maket Regulation: Beijing, China, 2021.
- Wang, M.J.; Guo, J.B.; Ma, S.C.; Wang, T.Z.; Zhang, X.; Luo, K.; Wang, G.Z. Review of Transient Frequency Stability Analysis and Frequency Regulation Control Methods for Renewable Power Systems. Proc. CSEE 2023, 43, 1672–1693. [Google Scholar]
- Wen, Y.; Chung, C.Y.; Liu, X.; Che, L. Microgrid Dispatch with Frequency-Aware Islanding Constraints. IEEE Trans. Power Syst. 2019, 34, 2465–2468. [Google Scholar] [CrossRef]
- Javadi, M.; Gong, Y.; Chung, C.Y. Frequency Stability Constrained Microgrid Scheduling Considering Seamless Islanding. IEEE Trans. Power Syst. 2022, 37, 306–316. [Google Scholar] [CrossRef]
- Anderson, P.M.; Mirheydar, M. A low-order system frequency response model. IEEE Trans. Power Syst. 1990, 5, 720–729. [Google Scholar] [CrossRef]
- Chan, M.L.; Dunlop, R.D.; Schweppe, F. Dynamic Equivalents for Average System Frequency Behavior Following Major Distribances. IEEE Trans. Power App. Syst. 1972, 91, 1637–1642. [Google Scholar] [CrossRef]
- Shintai, T.; Miura, Y.; Ise, T. Oscillation Damping of a Distributed Generator Using a Virtual Synchronous Generator. IEEE Trans. Power Deliv. 2014, 29, 668–676. [Google Scholar] [CrossRef]
- Zhang, Z.; Du, E.; Teng, F.; Zhang, N.; Kang, C. Modeling Frequency Dynamics in Unit Commitment with a High Share of Renewable Energy. IEEE Trans. Power Syst. 2020, 35, 4383–4395. [Google Scholar] [CrossRef]
- Shen, J.K.; Li, W.D.; Li, Z.W.; Zeng, H.; Zheng, H.C. Unit Commitment of Power System with High Proportion of Wind Power Considering the Deadband and Limiter of Primary Frequency Response. Power Syst. Technol. 2022, 46, 1326–1334. [Google Scholar]
- Ahmadi, H.; Ghasemi, H. Security-Constrained Unit Commitment with Linearized System Frequency Limit Constraints. IEEE Trans. Power Syst. 2014, 29, 1536–1545. [Google Scholar] [CrossRef]
- Paturet, M.; Markovic, U.; Delikaraoglou, S.; Vrettos, E.; Aristidou, P.; Hug, G. Stochastic Unit Commitment in Low-Inertia Grids. IEEE Trans. Power Syst. 2020, 35, 3448–3458. [Google Scholar] [CrossRef]
- Li, J.M.; Qiao, Y.; Lu, Z.X.; Ma, W.; Cao, X.; Sun, R.F. Integrated Frequency-constrained Scheduling Considering Coordination of Frequency Regulation Capabilities from Multi-source Converters. J. Mod. Power Syst. Clean Energy 2024, 12, 261–274. [Google Scholar] [CrossRef]
- Lagos, D.T.; Hatziargyriou, N.D. Data-Driven Frequency Dynamic Unit Commitment for Island Systems with High RES Penetration. IEEE Trans. Power Syst. 2021, 36, 4699–4711. [Google Scholar] [CrossRef]
- Zhang, Y.; Cui, H.; Liu, J.; Qiu, F.; Hong, T.; Yao, R.; Li, F. Encoding Frequency Constraints in Preventive Unit Commitment Using Deep Learning with Region-of-Interest Active Sampling. IEEE Trans. Power Syst. 2022, 37, 1942–1955. [Google Scholar] [CrossRef]
- Shen, Y.K.; Wu, W.C.; Wang, B.; Yang, Y.; Lin, Y. Data-driven Convexification for Frequency Nadir Constraint of Unit Commitment. J. Mod. Power Syst. Clean. Energy 2023, 11, 1711–1717. [Google Scholar] [CrossRef]
- Liu, L.K.; Hu, Z.C.; Wen, Y.L.; Ma, Y.X. Modeling of Frequency Security Constraints and Quantification of Frequency Control Reserve Capacities for Unit Commitment. IEEE Trans. Power Syst. 2024, 39, 2080–2092. [Google Scholar] [CrossRef]
- Ge, X.L.; Liu, Y.; Fu, Y.; Jia, F. Distributed Robust Unit Commitment Considering the Whole Process of Inertia Support and Frequency Regulations. Proc. CSEE 2021, 41, 4043–4058. [Google Scholar]
- Wang, X.; Ying, L.M.; Lu, S.P. Joint Optimization Model for Primary and Secondary Frequency Regulation Considering Dynamic Frequency Constraint. Power Syst. Technol. 2020, 44, 2858–2867. [Google Scholar]
- Zhou, X.Y.; Liu, R.; Bao, F.Z.; Zhang, M.Z.; Wang, H.X.; Li, W.D. Joint Optimization Model for Hundred-megawatt-level Energy Storage Participating in Dual Ancillary Services Dispatch of Power Grid. Autom. Electr. Power Syst. 2021, 45, 60–69. [Google Scholar]
- Ye, J.; Lin, T.; Zhang, L.; Bi, R.Y.; Xu, X.L. Isolated Grid Unit Commitment with Dynamic Frequency Constraint Considering Photovoltaic Power Plants Participating in Frequency Regulation. Trans. China Electrotech. Soc. 2017, 32, 194–202. [Google Scholar]
- Yang, M.Q.; Wang, C.; Ge, C.Y.; Bi, T.S.; Wang, X.G.; Zhou, Z. Primary Frequency Regulation Reserve Procurement of Renewable Energy Power System Considering Multiple Damping States and Governor Limiters. Proc. CSEE. 2024, 44, 147–160. [Google Scholar]
- Ni, Y.X.; Chen, S.S.; Zhang, B.L. Theory and Analysis of Dynamic Power Systems, 1st ed.; Tsinghua University Press: Beijing, China, 2002; pp. 76–78. [Google Scholar]
- Shi, Q.; Li, F.; Cui, H. Analytical Method to Aggregate Multi-Machine SFR Model with Applications in Power System Dynamic Studies. IEEE Trans. Power Syst. 2018, 33, 6355–6367. [Google Scholar] [CrossRef]
- Lee, Y.Y.; Baldick, R. A Frequency-Constrained Stochastic Economic Dispatch Model. IEEE Trans. Power Syst. 2013, 28, 2301–2312. [Google Scholar] [CrossRef]
- Golshani, A.; Sun, W.; Zhou, Q.; Zheng, Q.P.; Hou, Y. Incorporating Wind Energy in Power System Restoration Planning. IEEE Trans. Smart Grid 2019, 10, 16–28. [Google Scholar] [CrossRef]




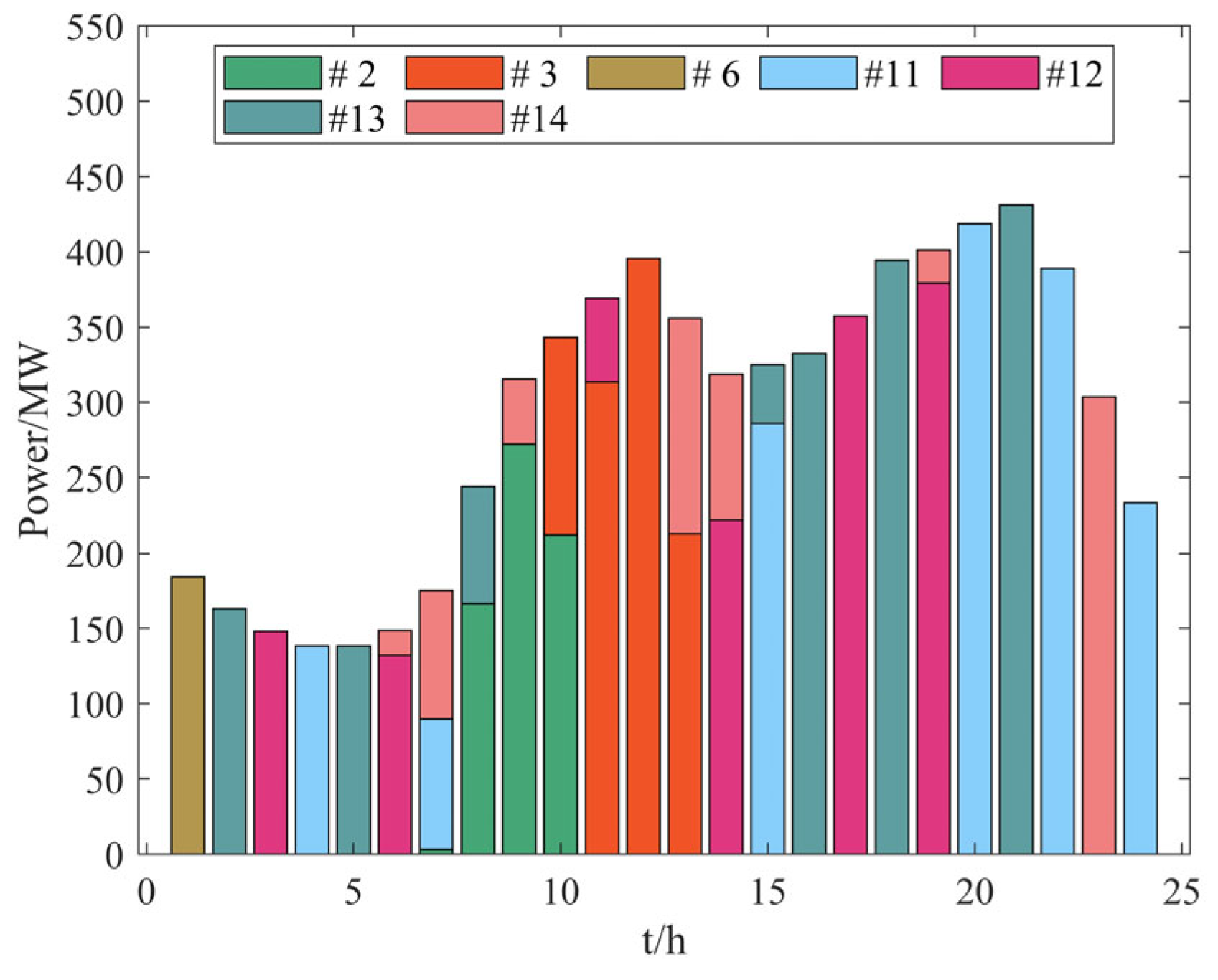




| Unit | Capacity (MW) | Minimum Output (MW) | Uphill Climb Rate (MW/h) | Downhill Climb Rate (MW/h) | Generation Costs (Ұ/MWh) |
|---|---|---|---|---|---|
| 1 | 300 | 100 | 100 | 100 | 25 |
| 2 | 630 | 100 | 300 | 300 | 16 |
| 3 | 750 | 100 | 300 | 300 | 23 |
| 4 | 740 | 100 | 300 | 300 | 17 |
| 5 | 620 | 100 | 300 | 300 | 16 |
| 6 | 720 | 100 | 300 | 300 | 15 |
| 7 | 650 | 100 | 300 | 300 | 23 |
| 8 | 600 | 100 | 300 | 300 | 24 |
| 9 | 1000 | 100 | 300 | 300 | 26 |
| 10 | 1100 | 100 | 300 | 300 | 25 |
| 11 | 500 | \ | \ | \ | 10 |
| 12 | 500 | \ | \ | \ | 10 |
| 13 | 500 | \ | \ | \ | 10 |
| 14 | 500 | \ | \ | \ | 10 |
| Unit | H (s) | R (pu) | (pu) | (s) |
|---|---|---|---|---|
| 1 | 4.78 | 0.04 | 0.25 | 6 |
| 2 | 4.81 | 0.05 | 0.3 | 8 |
| 3 | 4.77 | 0.04 | 0.3 | 8 |
| 4 | 3.86 | 0.05 | 0.3 | 8 |
| 5 | 4.19 | 0.05 | 0.3 | 8 |
| 6 | 4.83 | 0.06 | 0.2 | 8 |
| 7 | 4.06 | 0.04 | 0.3 | 8 |
| 8 | 4.05 | 0.04 | 0.3 | 8 |
| 9 | 3.45 | 0.03 | 0.35 | 10 |
| 10 | 4.31 | 0.03 | 0.35 | 10 |
| 11 | 2.00 | 0.02 | 0 | 8 |
| 12 | 2.00 | 0.02 | 0 | 8 |
| 13 | 2.00 | 0.02 | 0 | 8 |
| 14 | 2.00 | 0.02 | 0 | 8 |
| Case | Power Generation Cost (Yuan) | PR Backup Cost (Yuan) | Total Costs (Yuan) |
|---|---|---|---|
| Case 1 | 12,277,829 | 1,289,952 | 13,567,781 |
| Case 2 | 12,230,830 | 1,284,871 | 13,515,701 |
| Case 3 | 15,199,350 | 1,485,335 | 16,624,685 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; Xu, C. Study on Primary Frequency Regulation Reserve Configuration of High Wind Power System Considering Governor Limiting Links. Energies 2024, 17, 1805. https://doi.org/10.3390/en17081805
Wang M, Xu C. Study on Primary Frequency Regulation Reserve Configuration of High Wind Power System Considering Governor Limiting Links. Energies. 2024; 17(8):1805. https://doi.org/10.3390/en17081805
Chicago/Turabian StyleWang, Min, and Chunxing Xu. 2024. "Study on Primary Frequency Regulation Reserve Configuration of High Wind Power System Considering Governor Limiting Links" Energies 17, no. 8: 1805. https://doi.org/10.3390/en17081805
APA StyleWang, M., & Xu, C. (2024). Study on Primary Frequency Regulation Reserve Configuration of High Wind Power System Considering Governor Limiting Links. Energies, 17(8), 1805. https://doi.org/10.3390/en17081805







