Influence of DC/DC Converter Control on Source Current Ripple of the Switched Reluctance Motor
Abstract
1. Introduction
2. Problem of Source Current Ripple
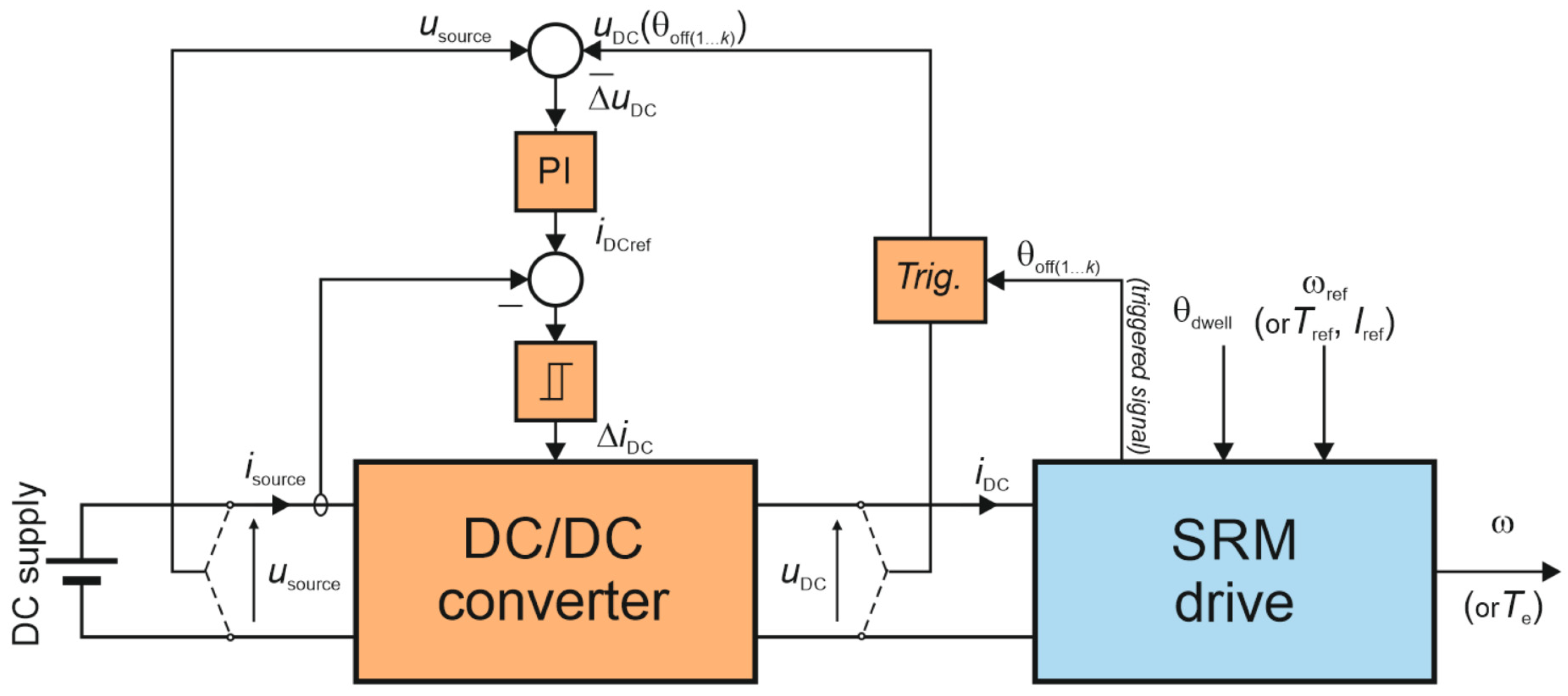
3. Reduction in Source Current Ripple by the Proposed Method
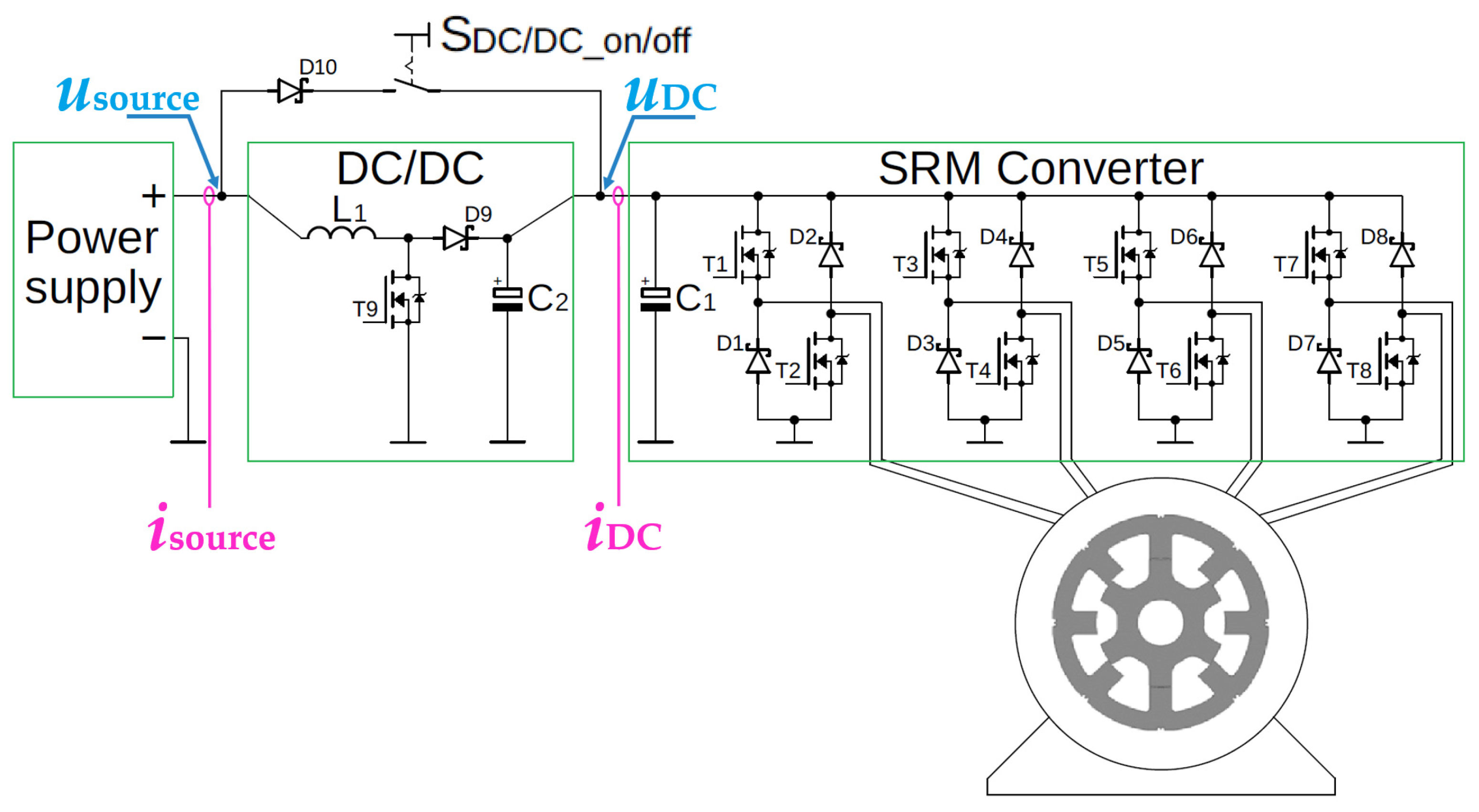
3.1. Schematic of an SRM Drive with a DC/DC Converter
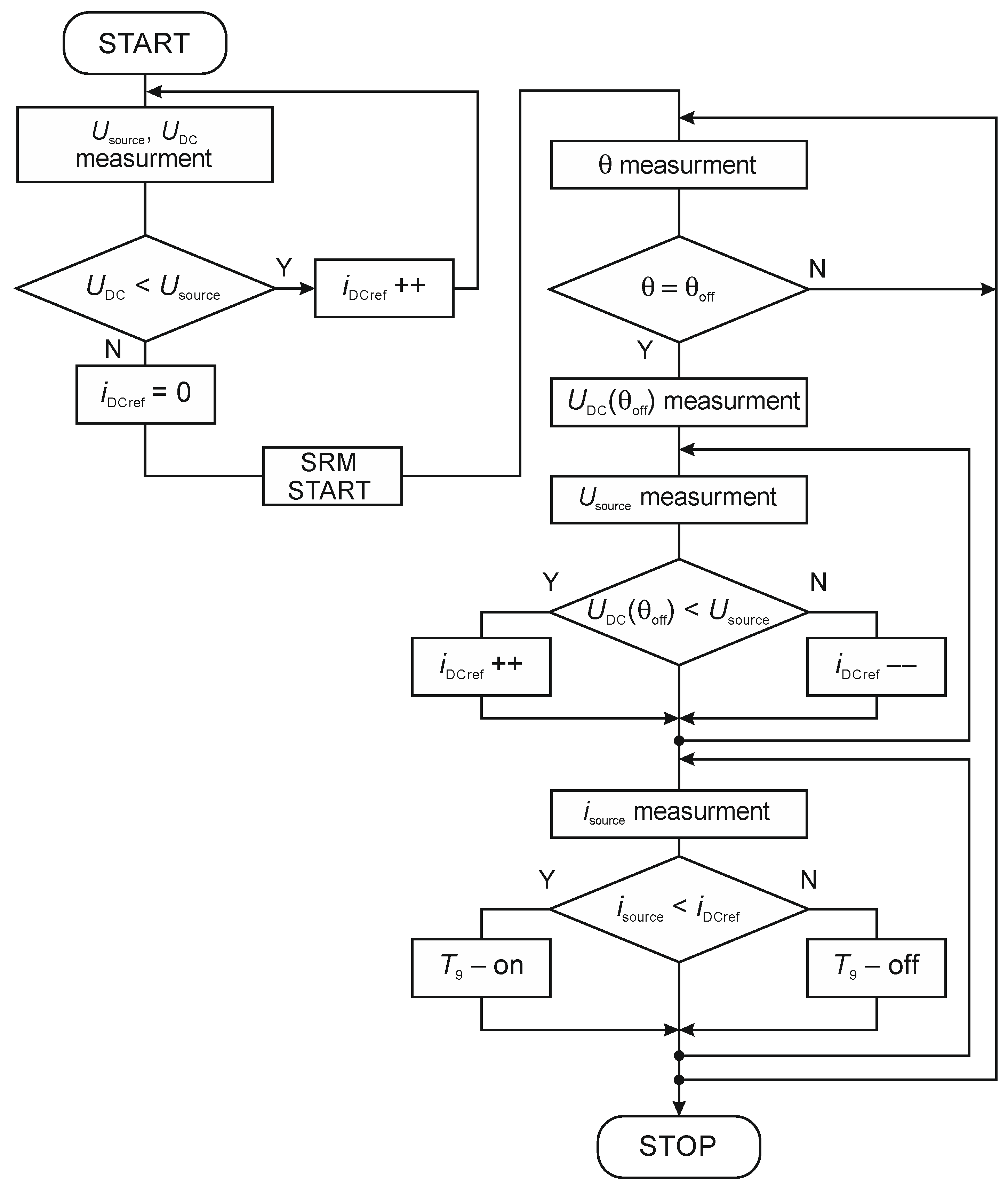
3.2. Description of the Proposed Control Method
4. Study of SRM Drive
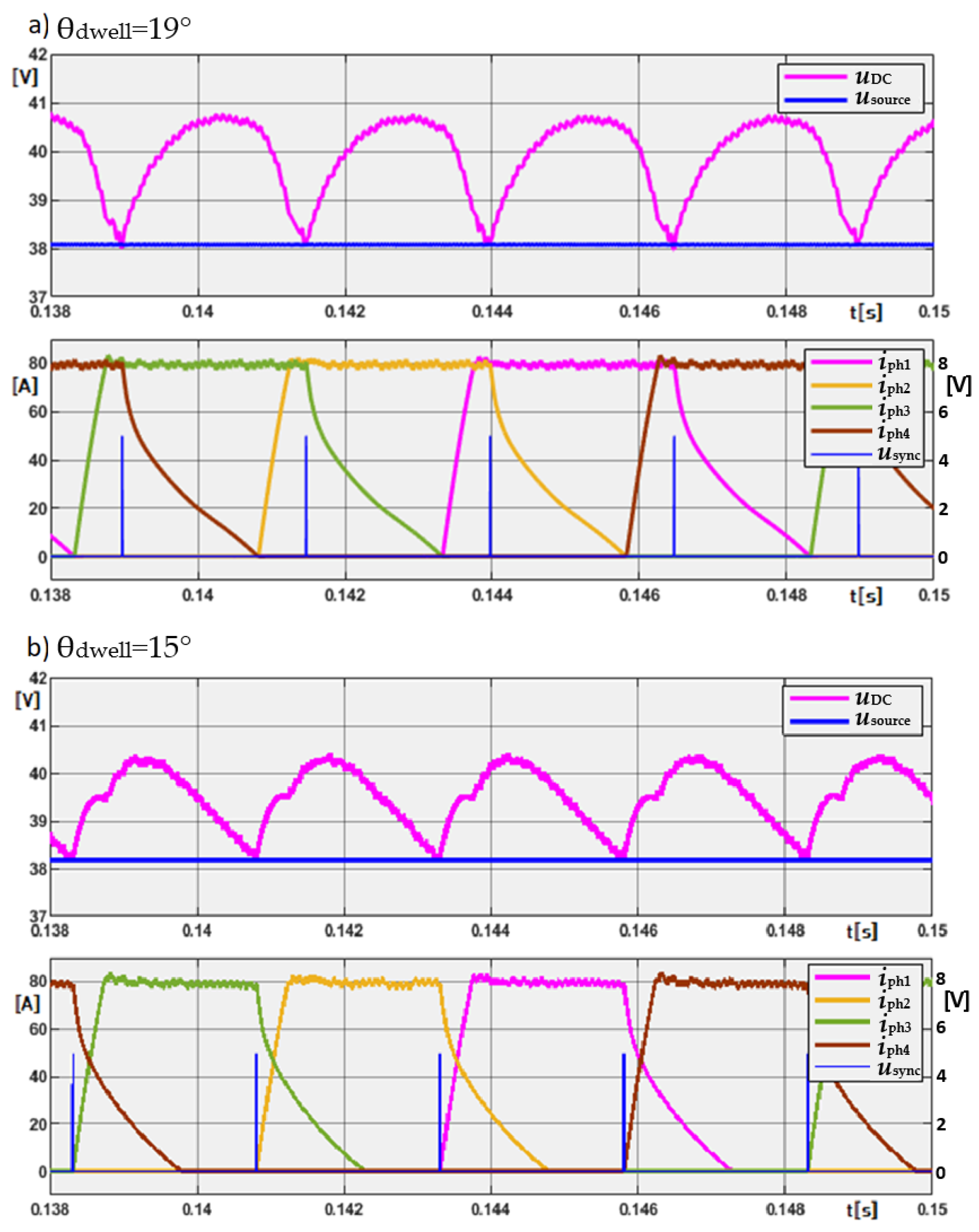
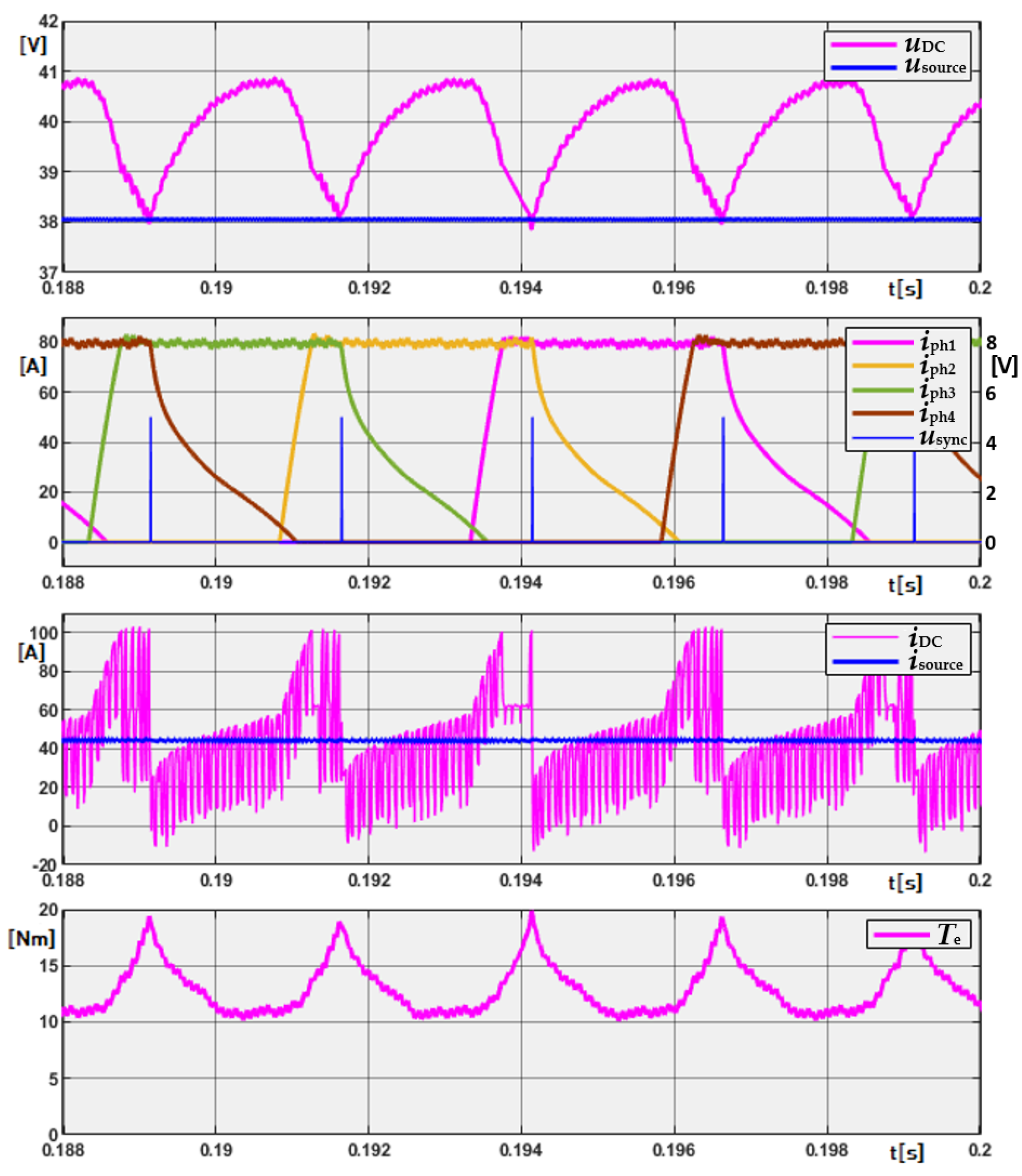
4.1. Simulation Results
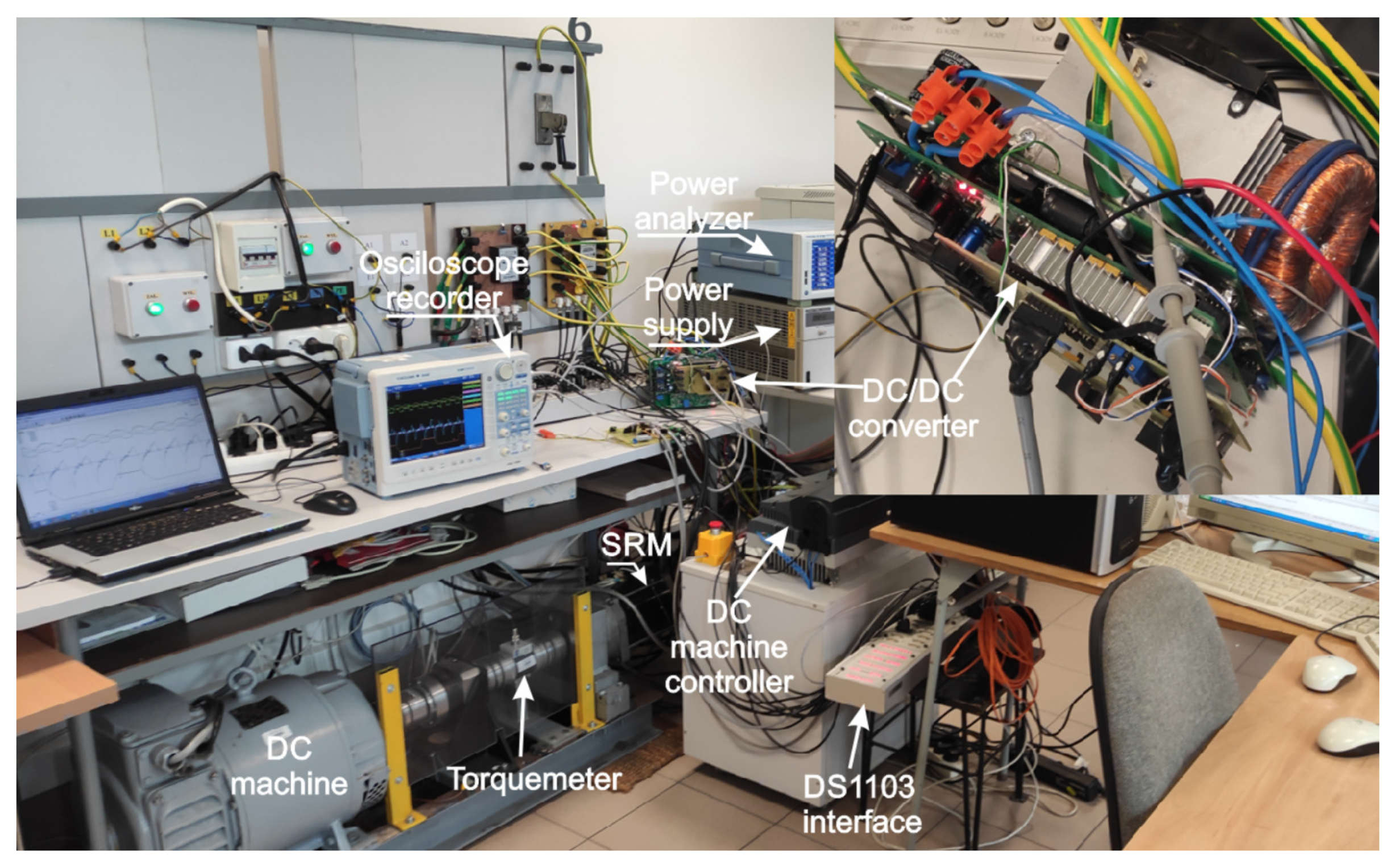
4.2. Test Stand
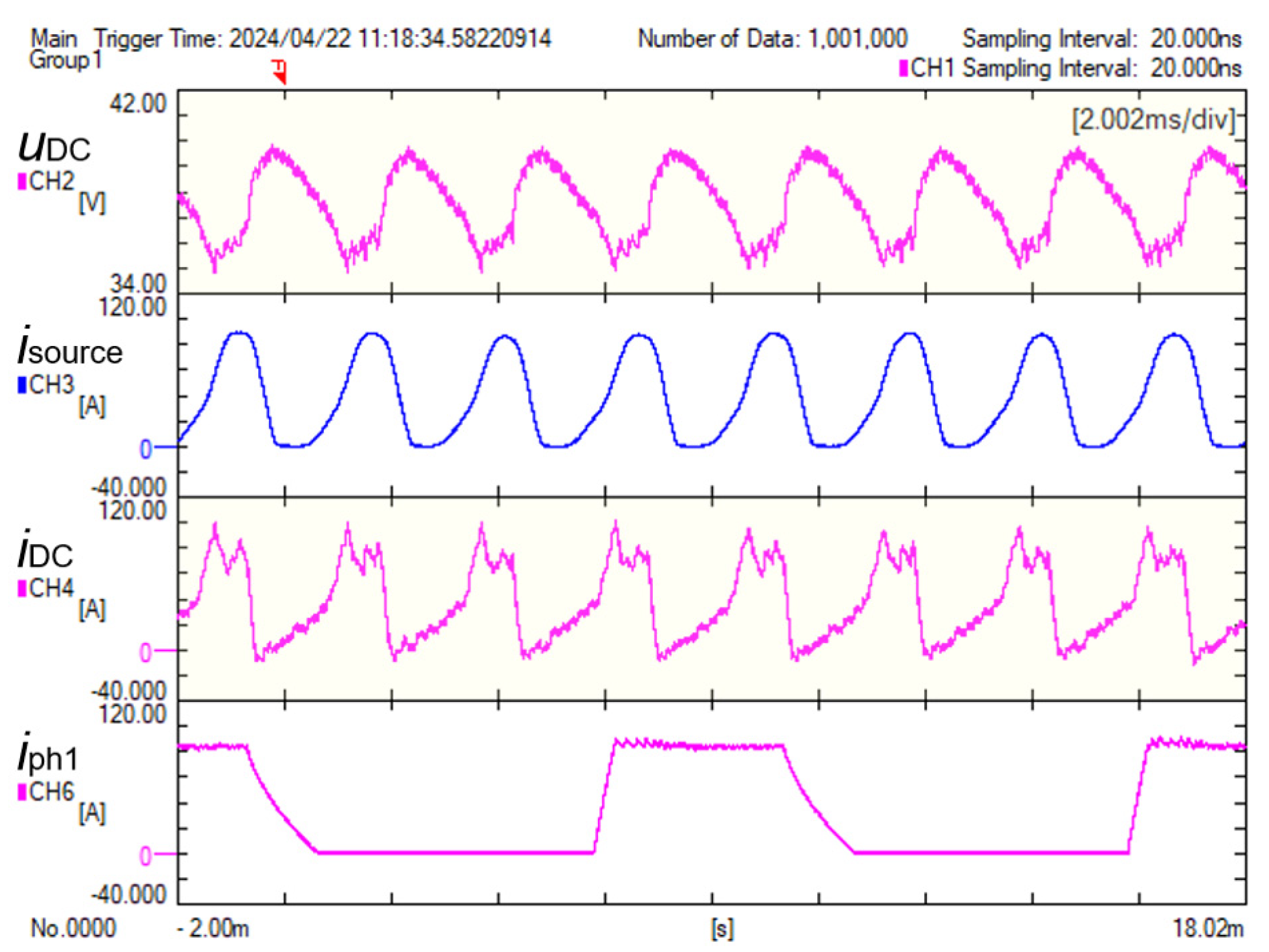
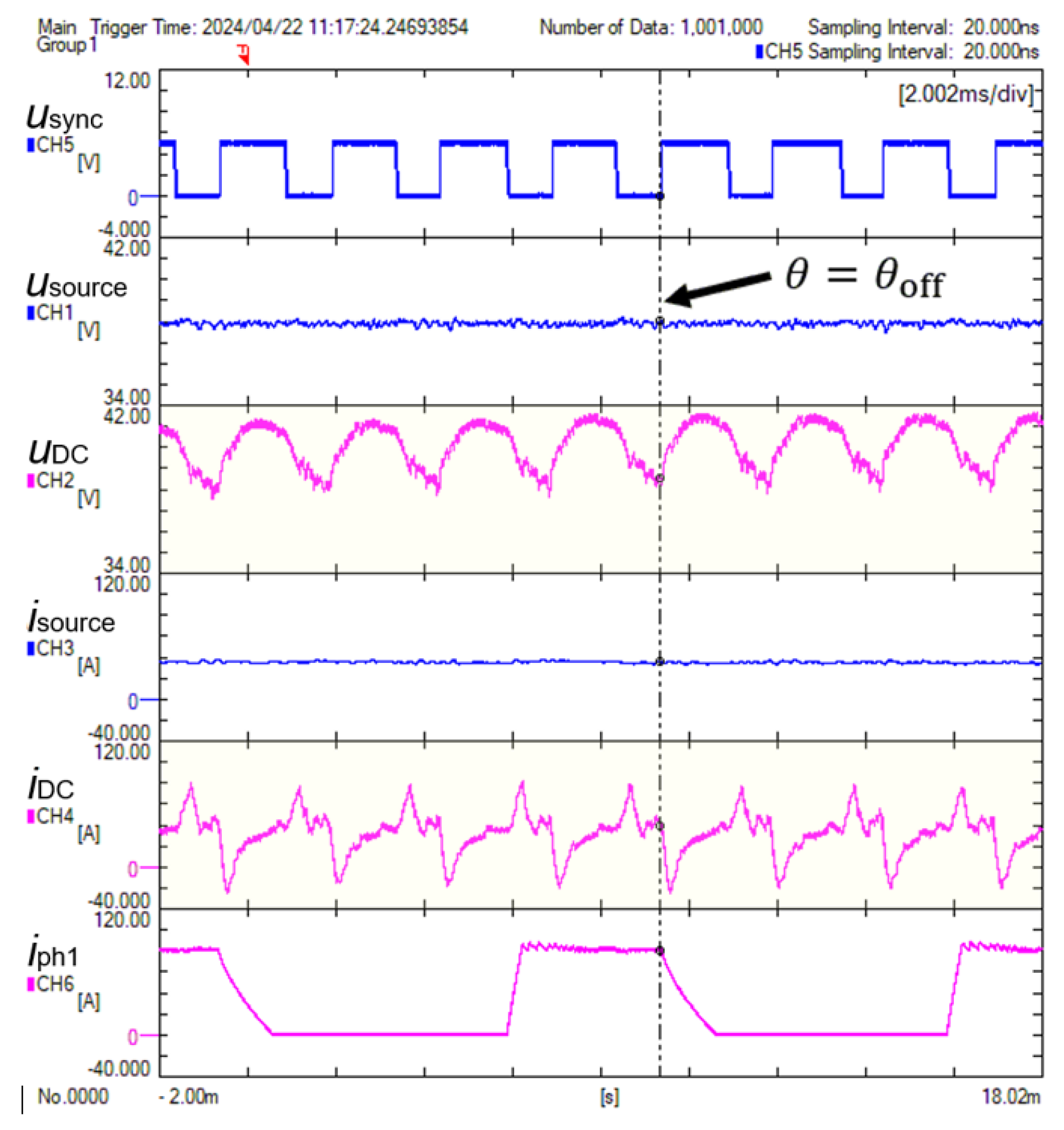
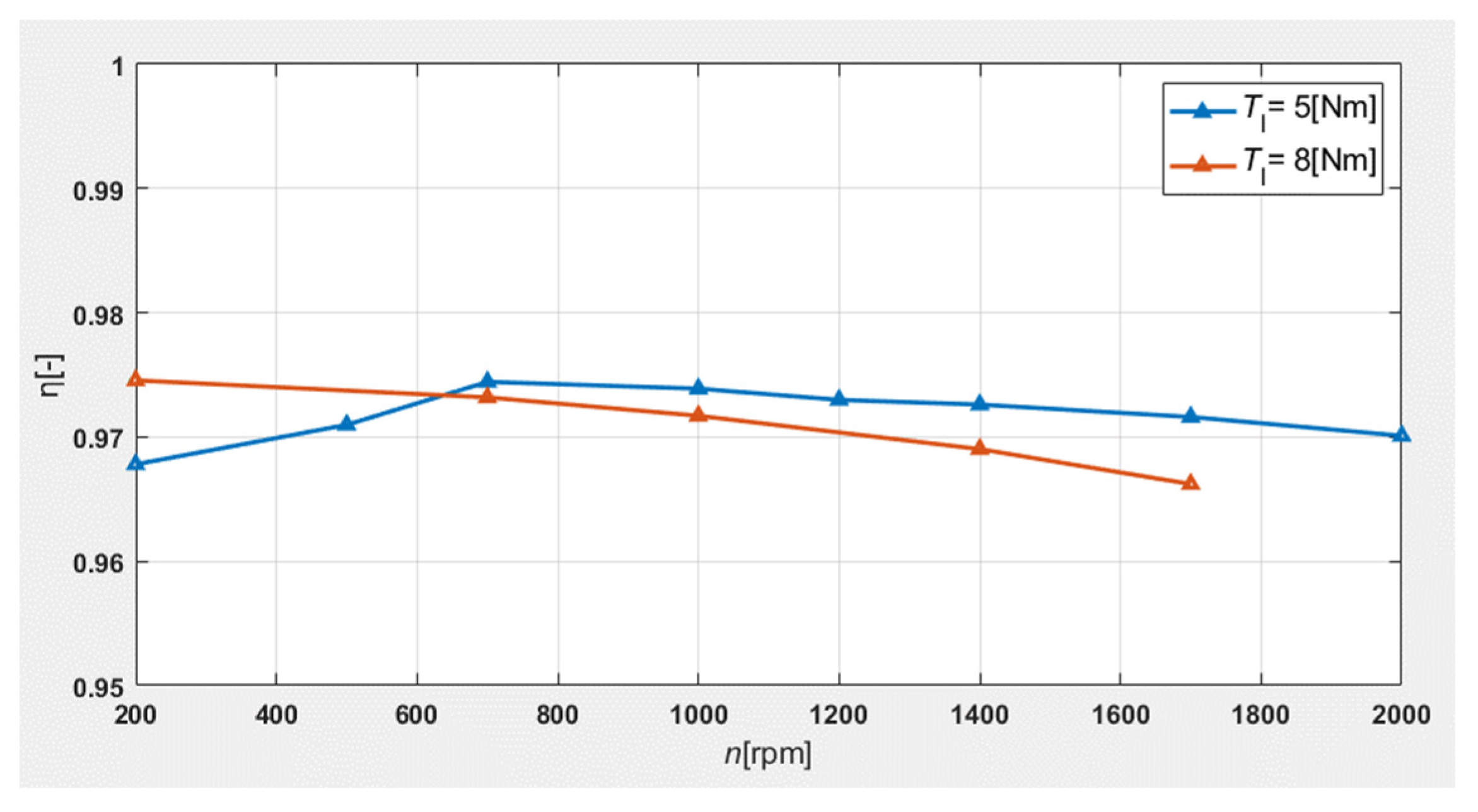
4.3. Laboratory Results
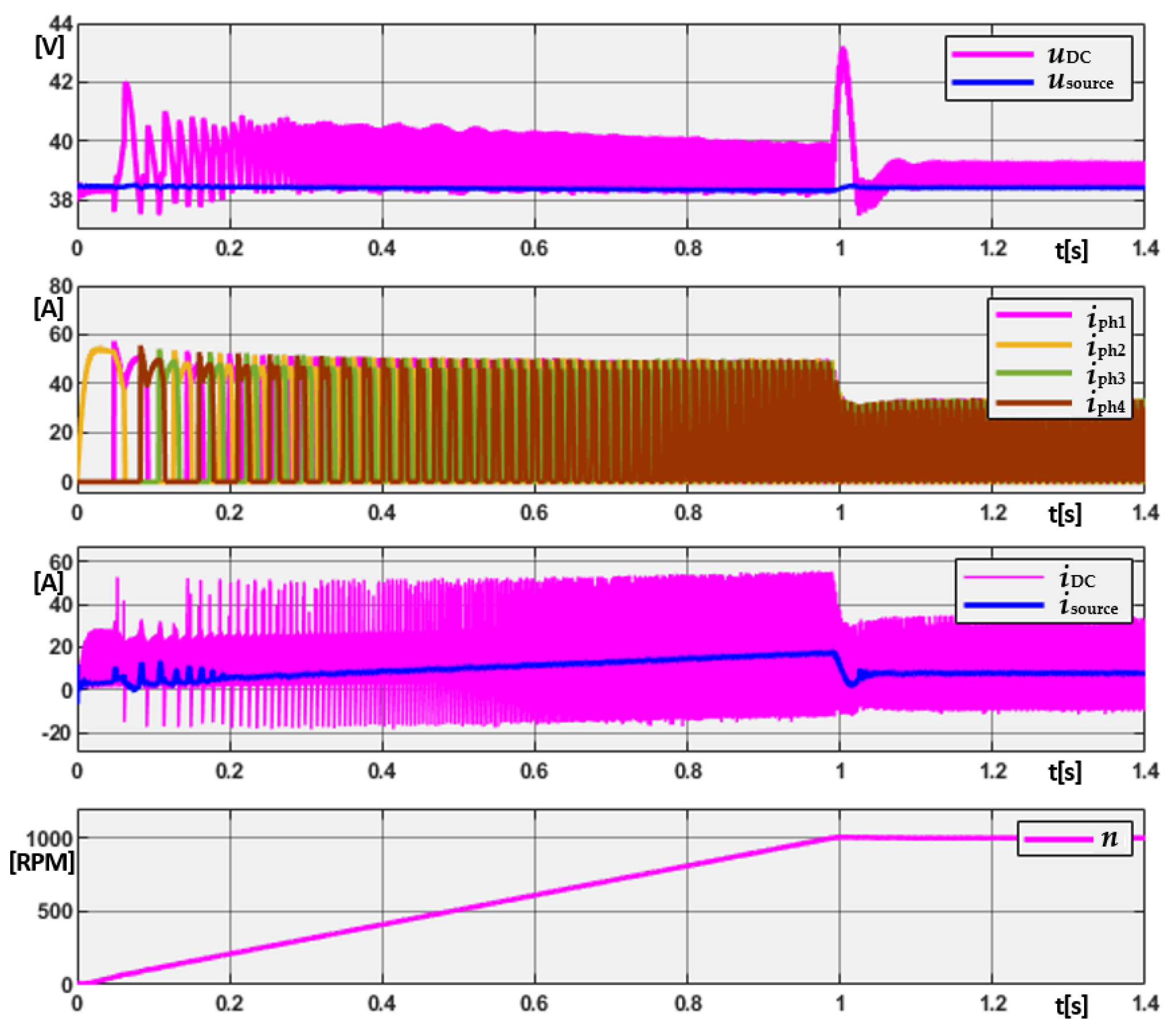
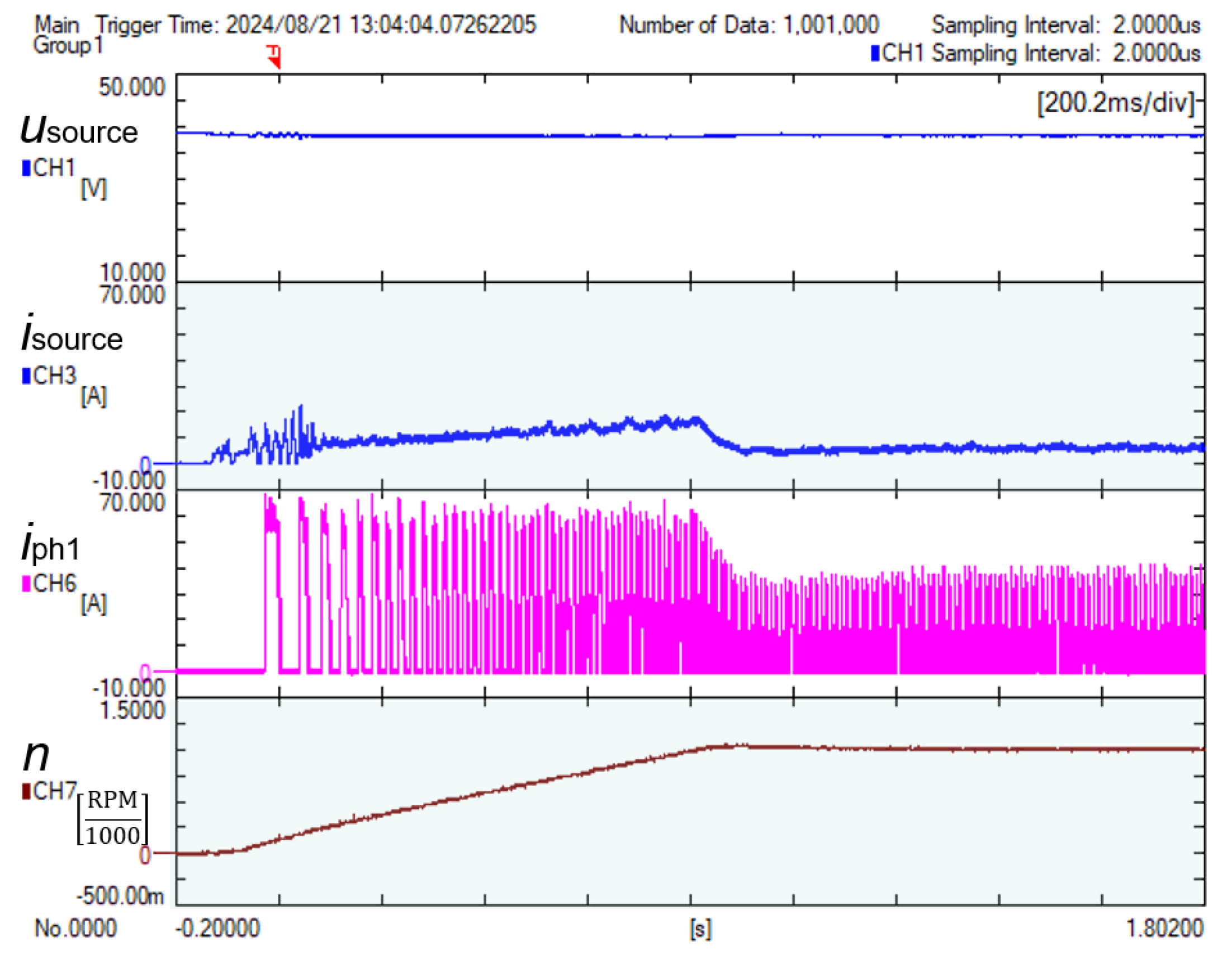
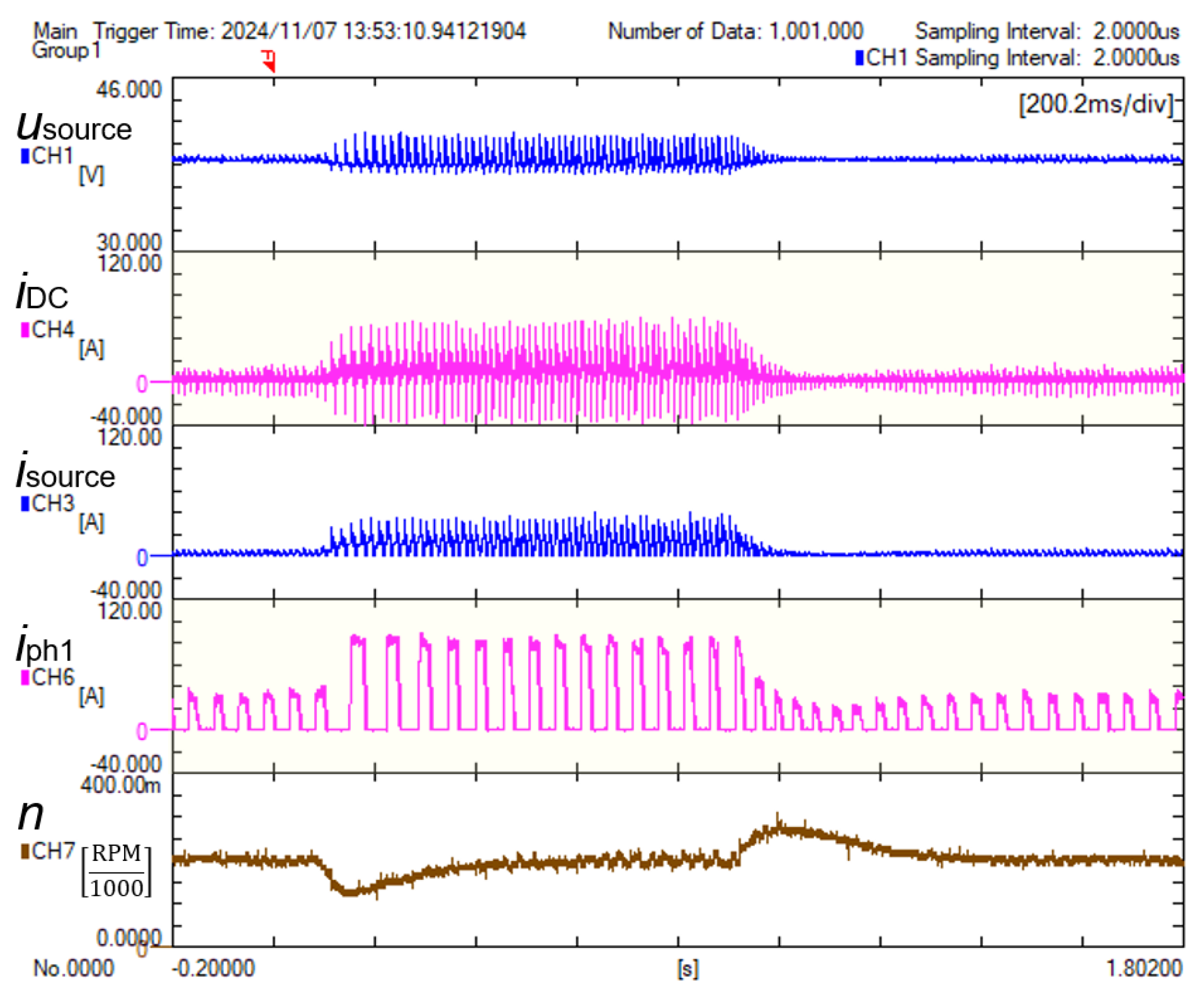
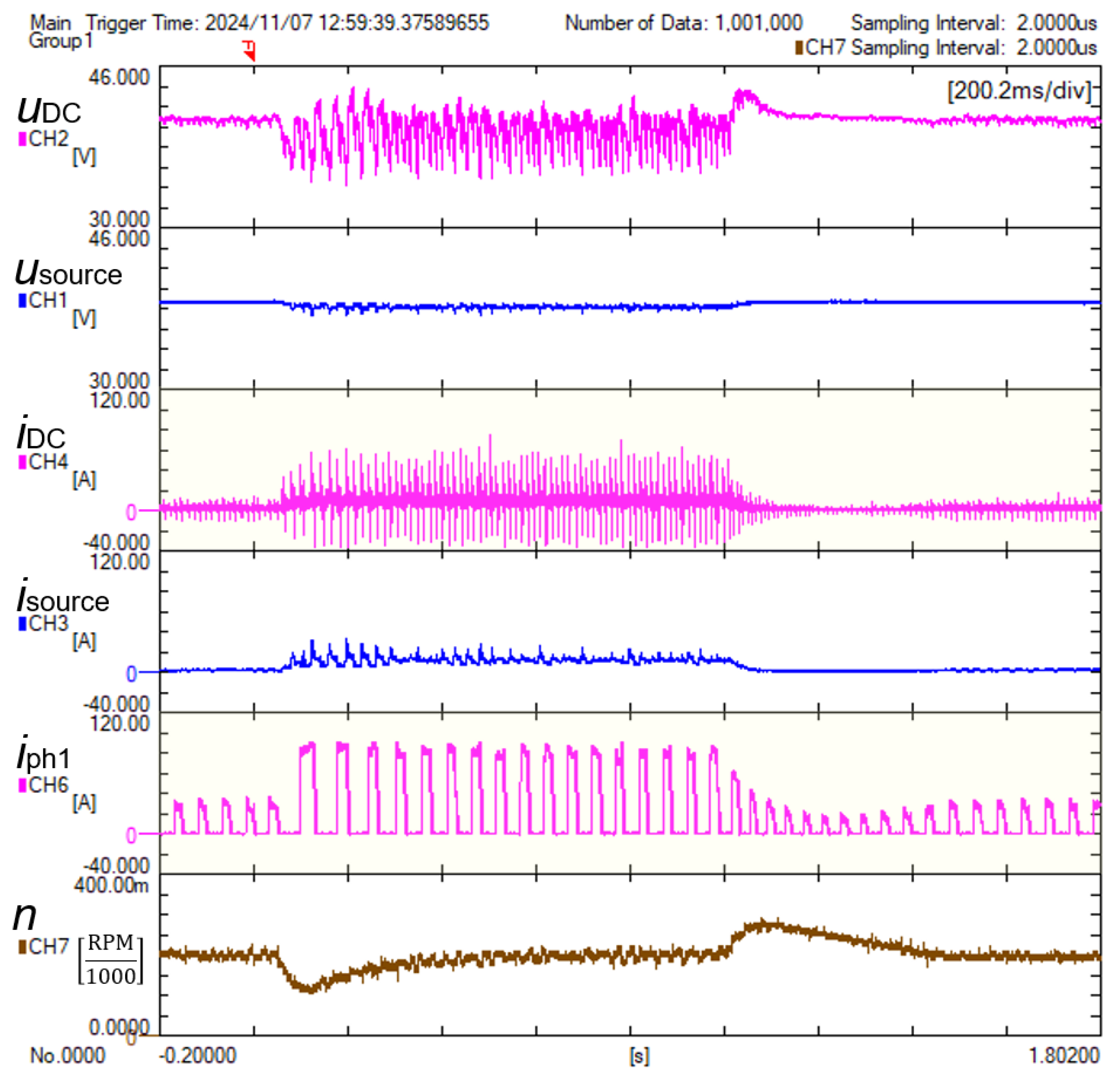
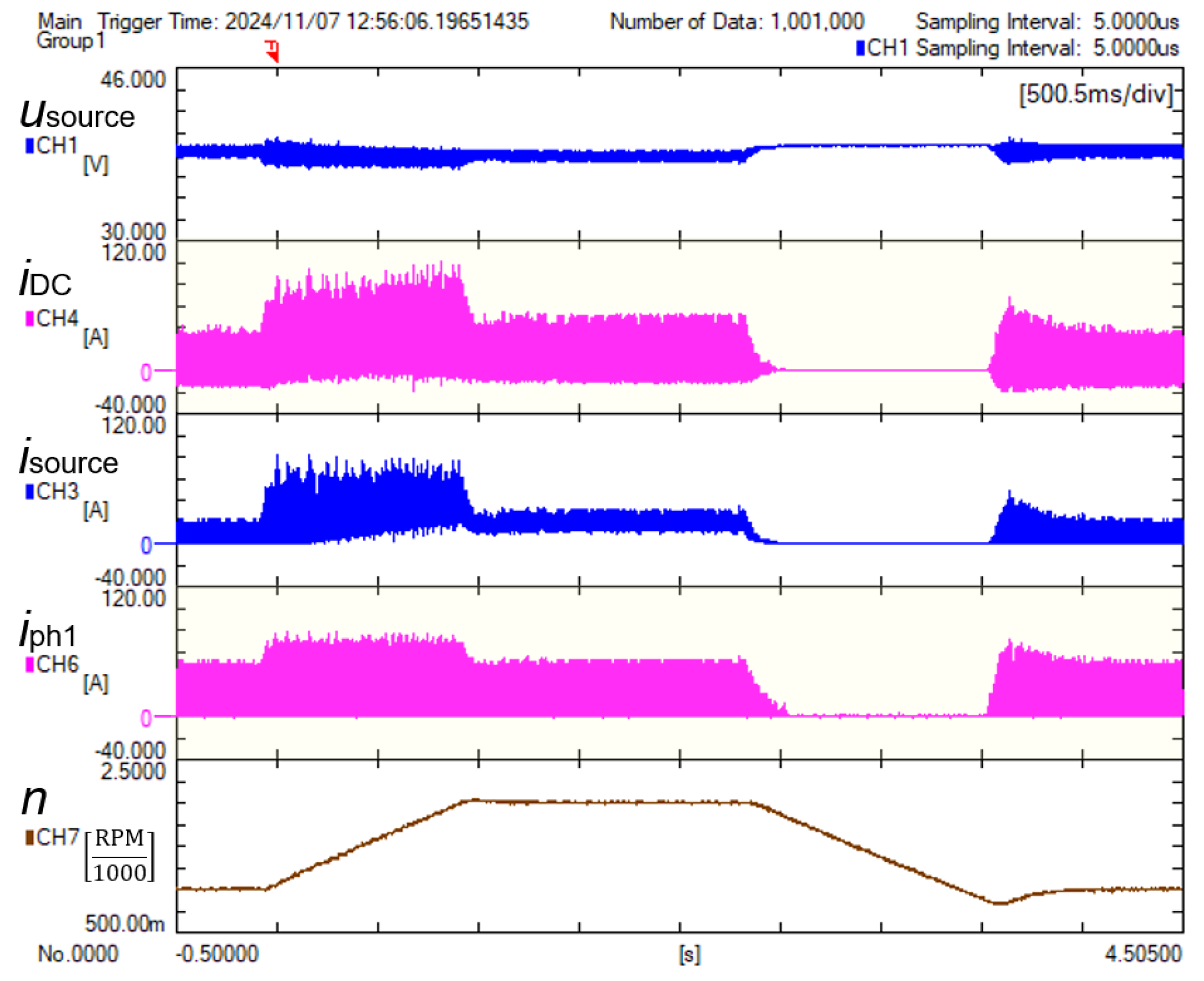
4.4. Dynamic Operating States of the SRM Drive
5. Conclusions
- -
- It is possible to effectively reduce the source current ripple over a wide speed range of the SRM by using a simple DC/DC boost converter integrated in series with the SRM power supply;
- -
- The proposed method of source current ripple reduction has a lower efficiency in the low rotor speed range, which means up to about 200 rpm for the SRM tested, it is possible to significantly reduce the rms source current and, thus, the losses in the power source.
- -
- Higher complexity and cost of the SRM control system,
- -
- Slight deterioration in the efficiency of the SRM power supply system due to the use of a DC/DC converter.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vermeer, W.; Mouli, G.R.C.; Bauer, P. Robust-Oriented Optimization of Switched Reluctance Motors Considering Manufacturing. IEEE Trans. Transp. Electr. 2022, 8, 2853–2861. [Google Scholar]
- Lan, Y.; Benomar, Y.; Deepak, K.; Aksoz, A.; El Baghdadi, M.; Bostanci, E.; Hegazy, O. Switched Reluctance Motors and Drive Systems for Electric Vehicle Powertrains: State of the Art Analysis and Future Trends. Energies 2021, 14, 2079. [Google Scholar] [CrossRef]
- Bogusz, P.; Korkosz, M.; Prokop, J. Current harmonics analysis as a method of electrical faults diagnostic in switched reluctance motors. In Proceedings of the 2007 IEEE International Symposium on Diagnostics for Electric Machines, Power Electronics and Drives, Cracow, Poland, 6–8 September 2007. [Google Scholar]
- Bogusz, P.; Korkosz, M.; Prokop, J.; Daraż, M. Analysis Performance of SRM Based on the Novel Dependent Torque Control Method. Energies 2021, 14, 8203. [Google Scholar] [CrossRef]
- Gundogmus, O.; Das, S.; Yasa, Y.; Elamin, M.; Sozer, Y.; Kutz, J.; Tylenda, J.; Wright, R.L. Acoustic Noise Mitigation in High Pole Count Switched Reluctance Machines Utilizing Skewing Method on Stator and Rotor Poles. IEEE Trans. Ind. Electron. 2022, 69, 5581–5593. [Google Scholar] [CrossRef]
- Wiguna, C.A.; Cai, Y.; Lilian, L.L.S.S.; Furqani, J.; Fujii, Y.; Kiyota, K.; Chiba, A. Vibration and Acoustic Noise Reduction in Switched Reluctance Motor by Selective Radial Force Harmonics Reduction. IEEE Open J. Ind. Appl. 2023, 4, 23–34. [Google Scholar] [CrossRef]
- Husain, M.E. Torque ripple minimization in switched reluctance motor drives by PWM current control. IEEE Trans. Power Electron. 1996, 11, 83–88. [Google Scholar] [CrossRef]
- Vujičić, V.P. Minimization of Torque Ripple and Copper Losses in Switched Reluctance Drive. IEEE Trans. Power Electron. 2012, 27, 388–399. [Google Scholar] [CrossRef]
- Jing, B.; Dang, X.; Liu, Z.; Ji, J. Torque Ripple Suppression of Switched Reluctance Motor with Reference Torque Online Correction. Machines 2023, 11, 179. [Google Scholar] [CrossRef]
- Kusumi, T.; Hara, T.; Umetani, K.; Hiraki, E. Phase-current waveform for switched reluctance motors to eliminate input-current ripple and torque ripple in low-power propulsion below magnetic saturation. IET Power Electron. 2020, 13, 3351–3359. [Google Scholar] [CrossRef]
- Bogusz, P. A switched reluctance motor control method limiting the maximum DC source current in the low-speed range. Bull. Pol. Acad. Sci. Tech. Sci. 2016, 64, 197–206. [Google Scholar] [CrossRef][Green Version]
- Han, X.; Lu, L.; Zheng, Y.; Feng, X.; Li, Z.; Li, J.; Ouyang, M. A review on the key issues of the lithium ion battery degradation among the whole life cycle. eTransportation 2019, 1, 100005. [Google Scholar] [CrossRef]
- Vermeer, W.; Mouli, G.R.C.; Bauer, P. A Comprehensive Review on the Characteristics and Modeling of Lithium-Ion Battery Aging. IEEE Trans. Trans. Electr. 2022, 8, 2205–2232. [Google Scholar] [CrossRef]
- Neuhaus, C.R.; Fuengwarodsakul, N.H.; De Doncker, R.W. Control Scheme for Switched Reluctance Drives with Minimized DC-Link Capacitance. IEEE Trans. Power Electron. 2008, 23, 2557–2564. [Google Scholar] [CrossRef]
- Yi, F.; Cai, W. Modeling, Control and Seamless Transition of Bi-directional Battery-Driven Switched Reluctance Motor/Generator Drive based on Integrated Multiport Power Converter for Electric Vehicle Applications. IEEE Trans. Power Electron. 2015, 31, 7099–7111. [Google Scholar] [CrossRef]
- Mohamadi, M.; Rashidi, A.; Nejad, S.M.S.; Ebrahimi, M. A Switched Reluctance Motor Drive Based on Quasi Z-Source Converter with Voltage Regulation and Power Factor Correction. IEEE Trans. Ind. Electron. 2017, 65, 8330–8339. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, Y. Recent Advances in DC-DC Converters for Electric Vehicles. In Proceedings of the 2023 International Conference on Materials Engineering, New Energy and Chemistry (MENEC 2023), Kuala Lumpur, Malaysia, 13–15 October 2023. [Google Scholar]
- Ismail, A.A.A.; Elnady, A. Advanced Drive System for DC Motor Using Multilevel DC/DC Buck Converter Circuit. IEEE Access 2019, 7, 54167–54178. [Google Scholar] [CrossRef]
- Hoseini, S.M.; Sadeghzadeh, S.M.; Beromi, Y.A. A new method for active power factor correction using a dual A new method for active power factor correction using a dual purpose inverter in a flyback converter. Turk. J. Electr. Eng. Comput. Sci. 2016, 24, 4736–4750. [Google Scholar] [CrossRef]
- Suppharangsan, W.; Wang, J. Switching technique for minimisation of DC-link capacitance in switched reluctance machine drives. IET Electr. Syst. Transp. 2015, 5, 185–193. [Google Scholar] [CrossRef][Green Version]
- Ghadbane, H.E.; Barkat, S.; Djerioui, A.; Houari, A.; Oproescu, M.; Bizon, N. Energy management of electric vehicle using a new strategy based on slap swarm optimization and differential flatness control. Sci. Rep. 2024, 14, 3629. [Google Scholar] [CrossRef]
- Cai, W.; Fan, Y.F. An Integrated Multiport Power Converter With Small Capacitance Requirement for Switched Reluctance Motor Drive. IEEE Trans. Power Electron. 2016, 31, 3016–3026. [Google Scholar] [CrossRef]
- Singh, B.; Anand, A. Power Factor Correction in Modified SEPIC Fed Switched Reluctance Motor Drives. IEEE Trans. Ind. Appl. 2018, 54, 4494–4505. [Google Scholar] [CrossRef]







| Switched Reluctance Motor | |
|---|---|
| Phase number | 4 |
| Poles stator/rotor number | 8/6 |
| Rated voltage | 48 V |
| Max. mechanical power | 3.3 kW |
| Rated speed | 4500 rpm |
| Stator pole width | 20° |
| Rotor pole width | 20.5° |
| Phase winding resistance | 14 mΩ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Daraż, M.; Bogusz, P. Influence of DC/DC Converter Control on Source Current Ripple of the Switched Reluctance Motor. Energies 2024, 17, 5711. https://doi.org/10.3390/en17225711
Daraż M, Bogusz P. Influence of DC/DC Converter Control on Source Current Ripple of the Switched Reluctance Motor. Energies. 2024; 17(22):5711. https://doi.org/10.3390/en17225711
Chicago/Turabian StyleDaraż, Mateusz, and Piotr Bogusz. 2024. "Influence of DC/DC Converter Control on Source Current Ripple of the Switched Reluctance Motor" Energies 17, no. 22: 5711. https://doi.org/10.3390/en17225711
APA StyleDaraż, M., & Bogusz, P. (2024). Influence of DC/DC Converter Control on Source Current Ripple of the Switched Reluctance Motor. Energies, 17(22), 5711. https://doi.org/10.3390/en17225711






