Breathing Planet Earth: Analysis of Keeling’s Data on CO2 and O2 with Respiratory Quotient (RQ), Part I: Global Respiratory Quotient (RQGlob) of Earth
Abstract
1. Introduction
2. Literature Review
2.1. Fosssil Fuel, Land Use and CO2
2.2. Keeling’s Data on CO2 and O2 and Saw Tooth Pattern
2.3. CO2 and Global Warming

2.4. CO2 Sources and Sinks, O2 Sources and Sinks, and Models
3. Materials and Methods
3.1. Respiratory Quotient (RQ) fr CO2 Sources
3.1.1. Respiratory Quotient (RQ)
3.1.2. Significance of Respiratory Quotient (RQ) and RQ Values for Nutrients and Fuels
3.2. Respiratory Quotient (RQ), Redfield Ratio (RR), Exchange Ratio (ExRExR), Photosynthetic Quotient (PQ) for CO2 Sinks
3.3. Relation between Respiratory Quotient (RQ) and CO2 Released in Tons for CO2 Sources
3.3.1. CO2 Sources
3.3.2. RQ for Incomplete Combustion
3.3.3. RQ for CO2 Sinks and GT of CO2 Sink per Unit Energy Input
- Generic PP: Cx(H2O)w (NH3)yHzH3PO4 or CxH3+2w+3y+z Ny Ow+4 P
- (i)
- The ocean plants growth via photosynthesis: (See Table 1) is given by,
- (ii)
- Planktonic marine algae: The Redfield–Ketchum–Richards Formula is given as (CH2O)106(NH3)16 (H3PO4), or C106 H263 O110 N16 P [53] and M = 3553.3 g/mol. Thus, x = 106, y = 16, and w = 106. The RQ is estimated at 0.77. For the RQ based on the traditional Redfield–Ketchum–Richards equation and algae production, see Refs. [43,68].
- (iii)
- Oceanic cyanobacteria and algae: photosynthesis and respiration, RQ = 0.77 [37].
- 2.
- Seaweed or Sargassum: A bright seaweed also absorbs CO2 and produces O2, but it reflects more of the sun’s radiation compared to PP. Using the ultimate analyses reported in [7,69], the chemical formula on the UCF basis is given as CH1.74N0.025O1.42S0.018 (See Table A1, Appendix A.1). Thus, for seaweed, RQBM = 1.57, which is unusually high compared to land-based biomasses. It serves as a good CO2 sink but decomposes and releases NH3 and H2S (odors like rotten eggs) along with CO2. For different seaweeds, Ref. [69] presents ultimate analyses; the author determined the chemical formula and estimated that the RQ of seaweeds could vary from 0.95 to 1.34 (Appendix A.1). The measured RQ values are from 0.99 to 2.38 (or PQ from 0.42 to 1.01), while the theoretical values are from 0.77 to 1 (PQ from 1.0 to 1.3) [70].
3.4. Respiratory Quotient (RQ) of Fossil Fuels and Biomass
3.4.1. RQ of Fossil Fuels (FF)
3.4.2. Respiratory Quotient for the Combined Fossil and Land Use (LU) CO2 (RQFFLU)
3.5. Global Respiratory Quotient (RQGlob) for Planet Earth
3.6. Data
- (i).
- (ii)
- (iii)
- Respiration Quotient for FF (RQFF) and LU (RQLU), RQLBM, and RQOWBM.
- (iv)
- Curve fit constants for linear and quadratic fits of Keeling’s data on CO2 and O2 vs. years.
- (a)
- = 460.21 in 2006 [73]. HHVO2 = 448 kJ/mole of O2 = 0.448 ∗ 10−3 Giga J/mole or 448 Exa J/Peta mole.
- (b)
- For the year 2006, the oxygen consumption rate = 1.027 Peta mols/year with energy per year of 460.21 Exa J/year.
- (c)
- RQFF = 0.75, RQFFLU = 0.775 with energy fraction from LU, EFLU = 0.11; RQLBM, = 1, RQOWBM = 0.87, See also Ref. [77], which quotes PQ = 1.1 or RQLBM = 0.91 for terrestrial plants.
4. Results and Discussion
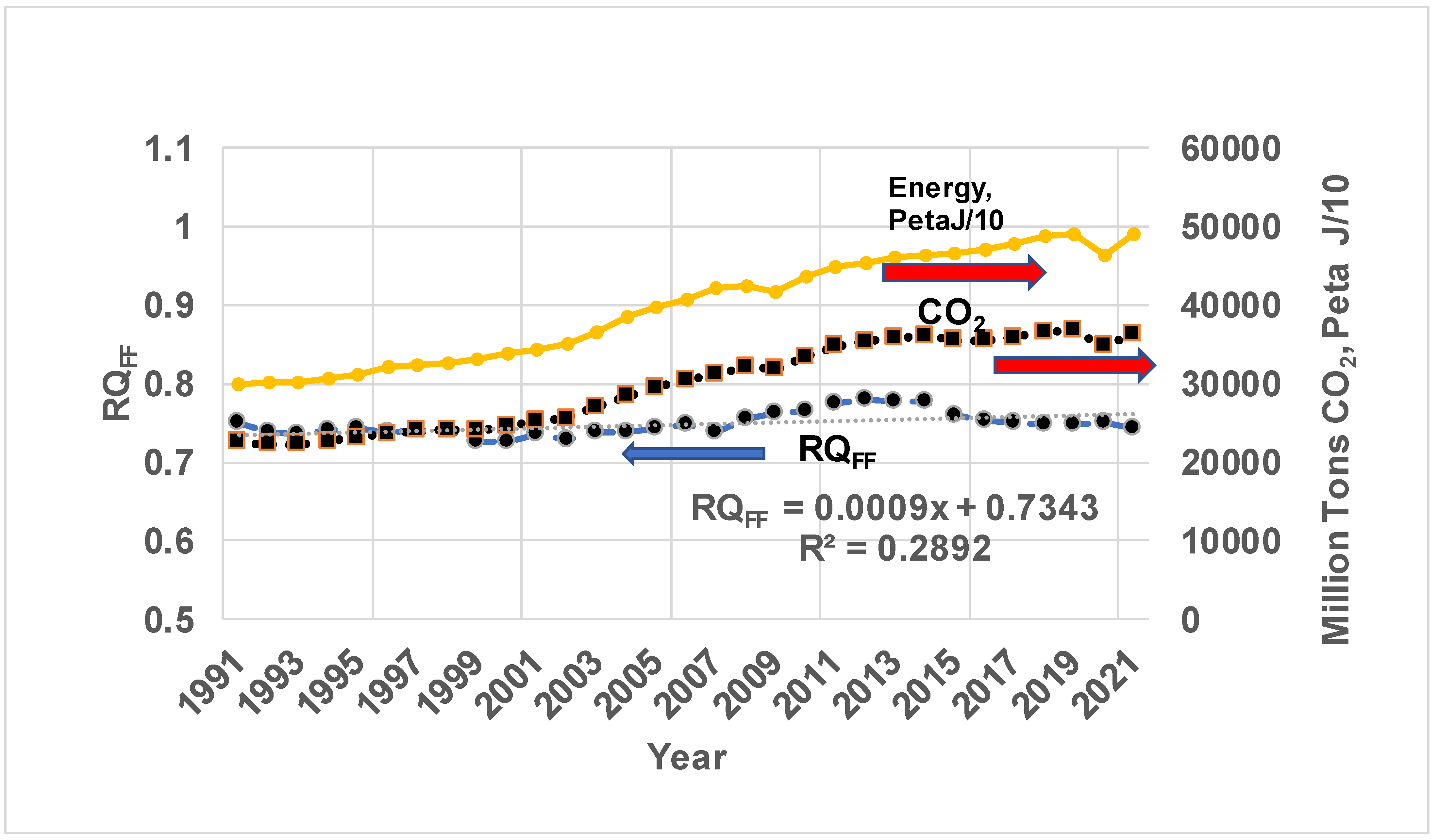
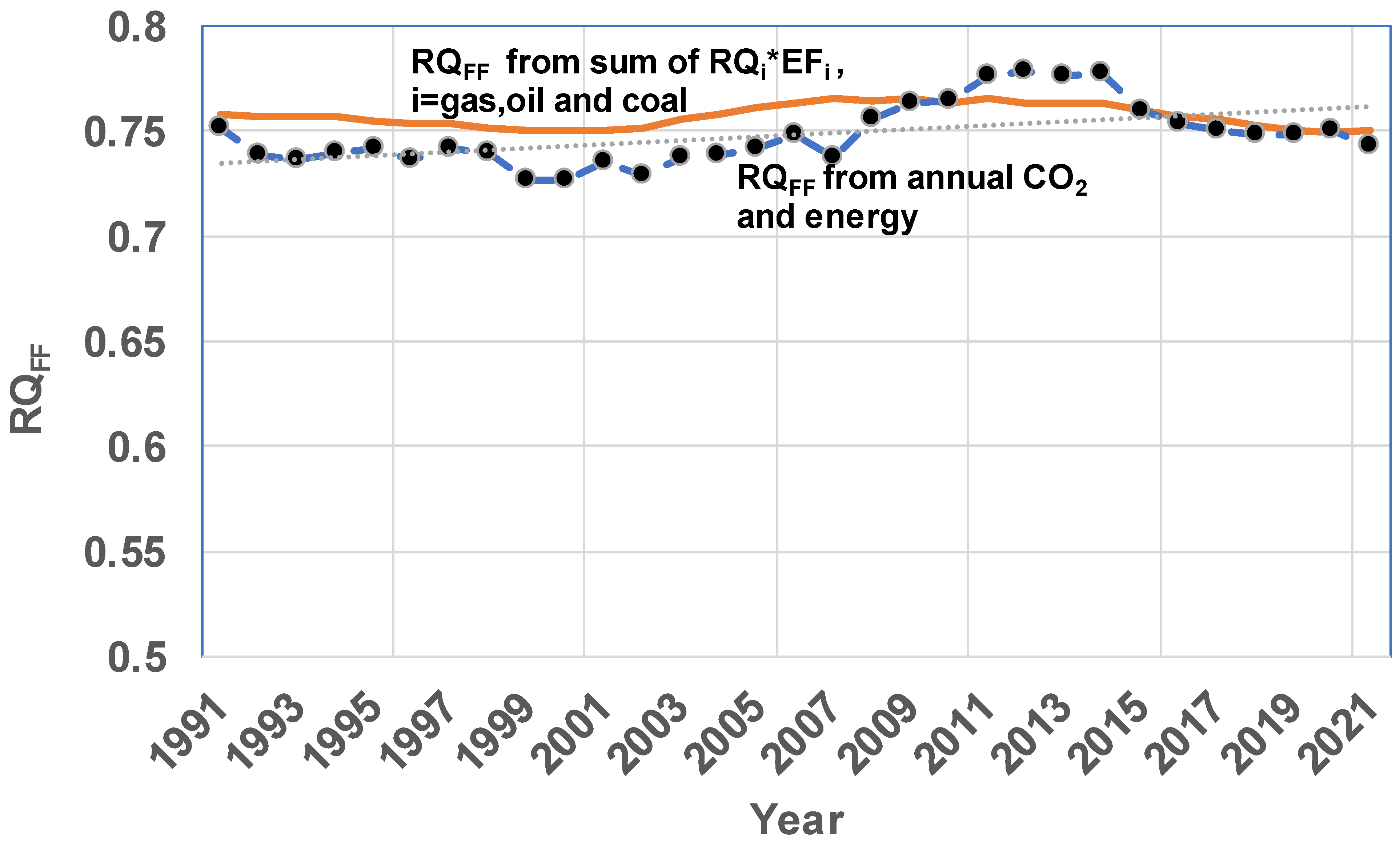
4.1. RQ of Fossil Fuels (FF)
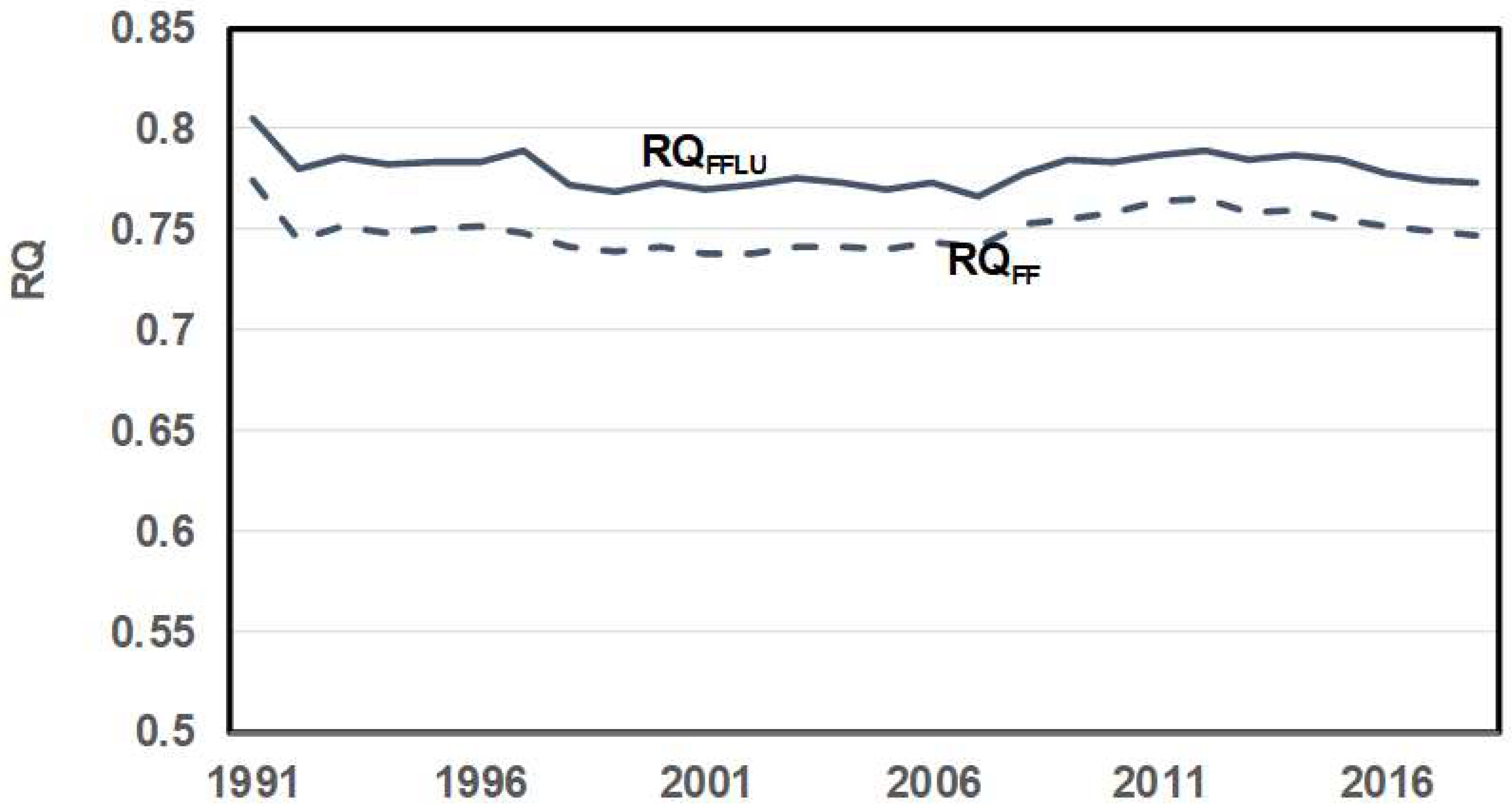
4.2. RQ of FFLU {Combined FF and LU}
4.3. RQ of Land Biomass (RQLBM) and Ocean Water Based Biomass (RQOWBM)
4.4. Keeling’s Data on CO2 and O2 in ppm and Curve Fits
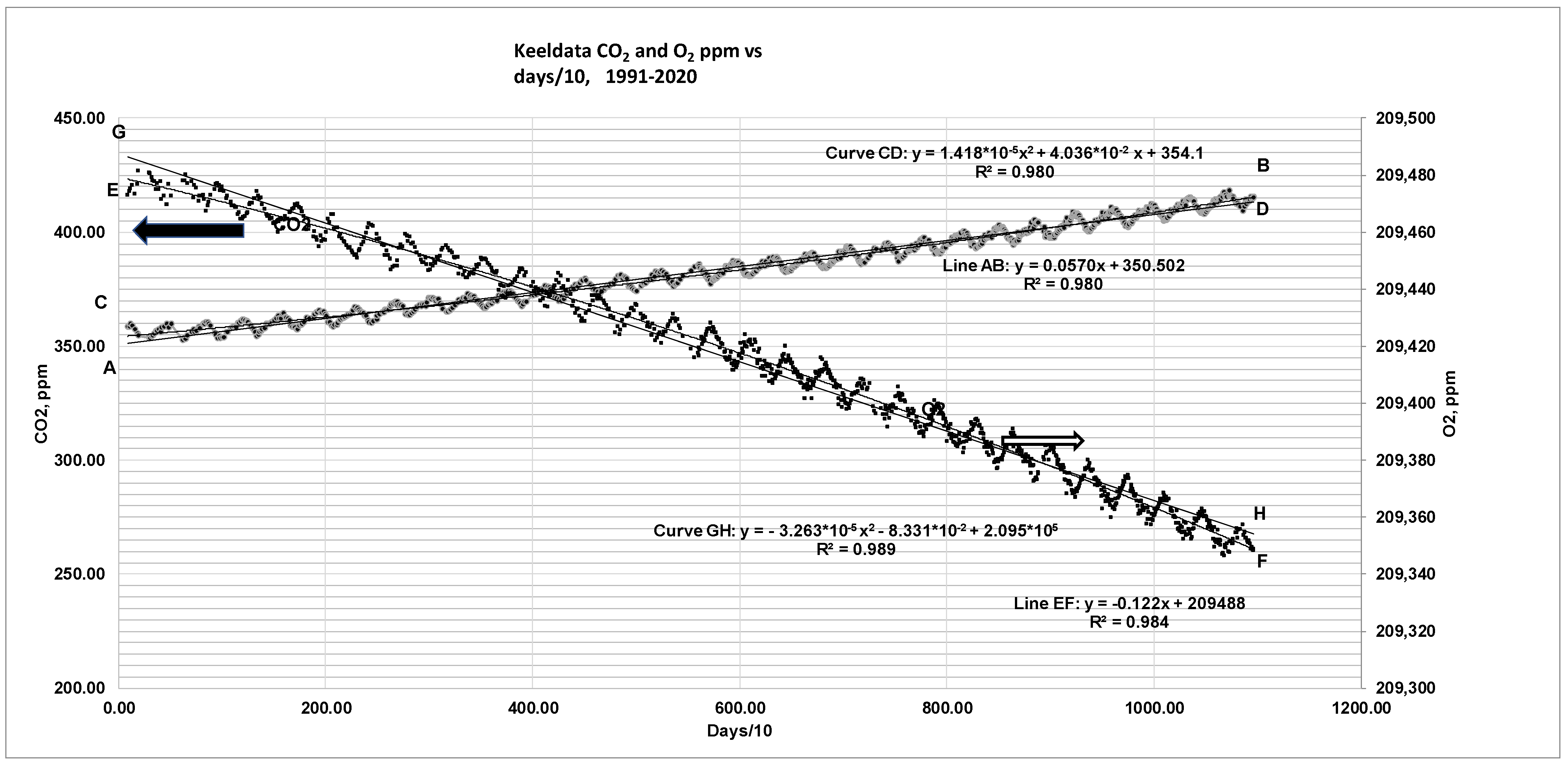
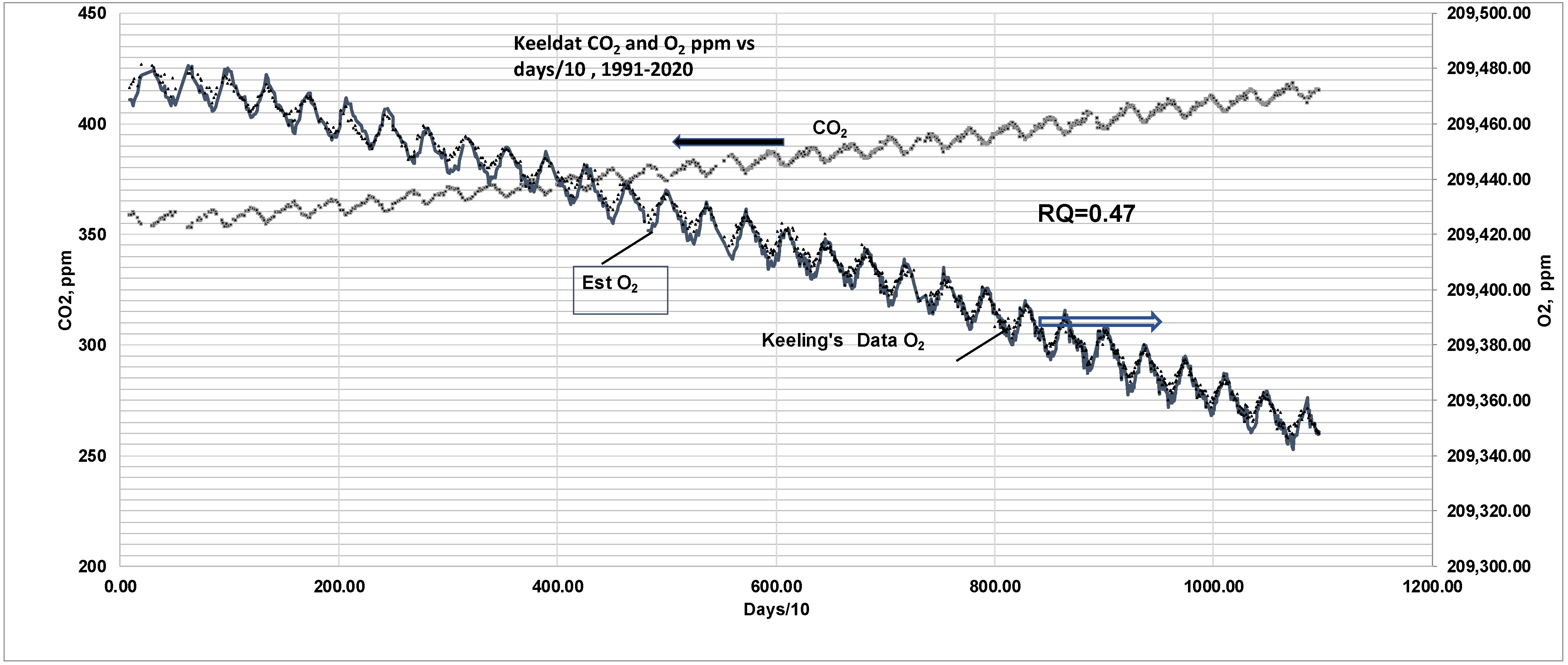
4.4.1. Keeling’s Data on CO2 and O2
4.4.2. The Curve Fits to Keeling’s Data and Estimation of d[CO2]/dt and d[O2]/dt
Linear Fit for CO2 and O2
4.4.3. Quadratic Fit for CO2 and O2 of Table 2
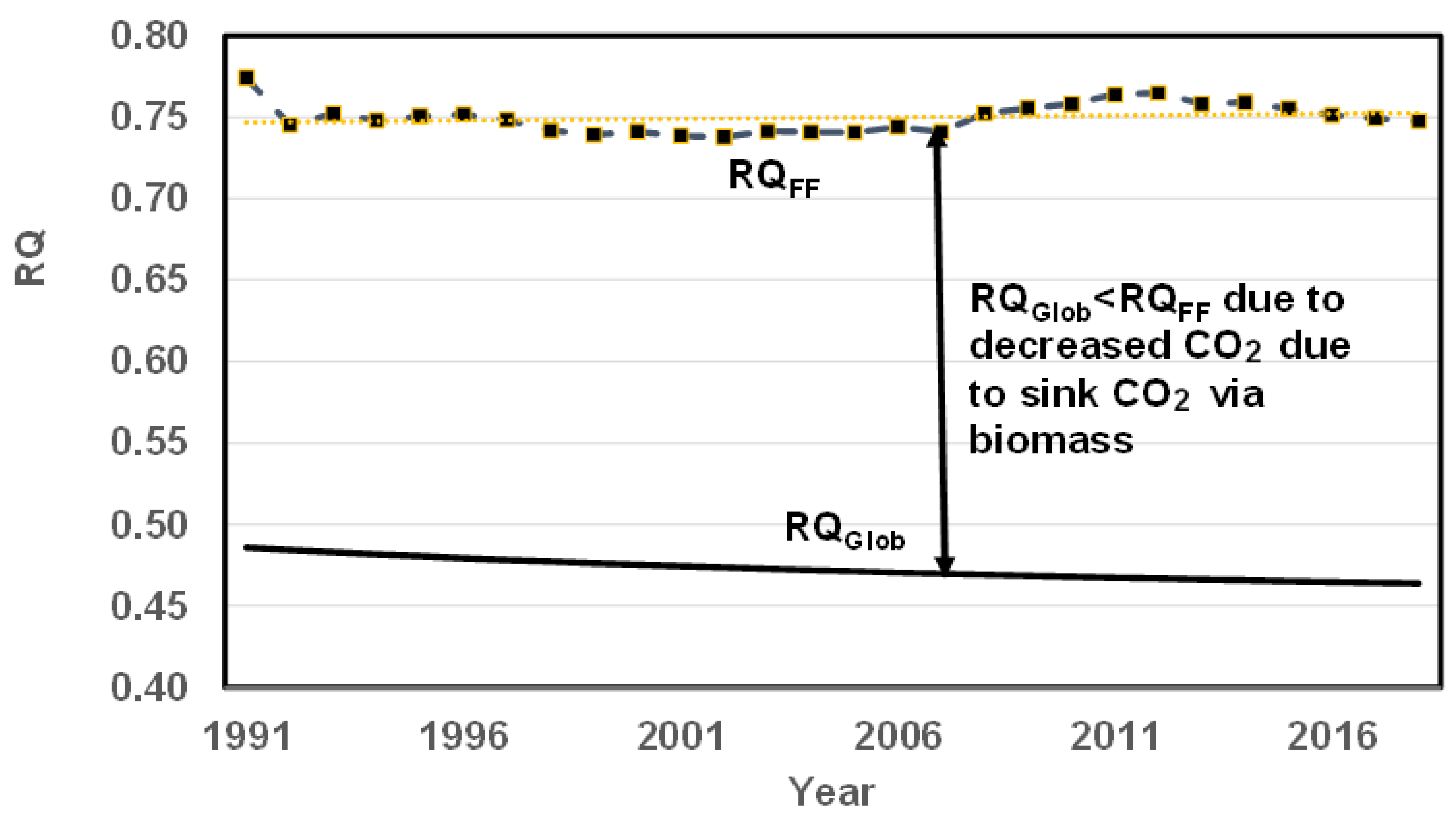
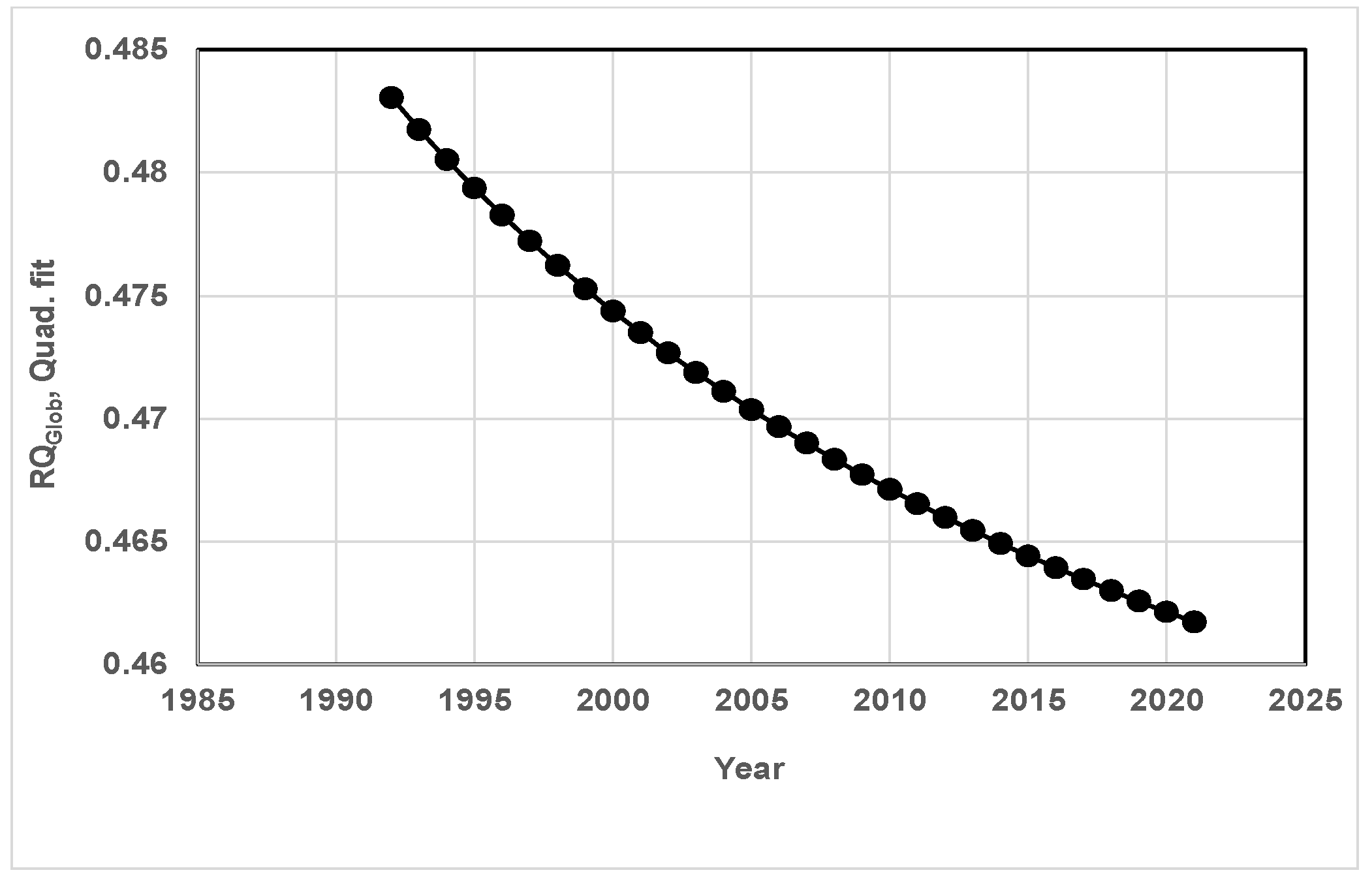
4.5. Estimation of Global Respiration Quotient (RQGlob)
4.5.1. Global RQ Based on CO2 Added to Atm and O2 depleted from Atm
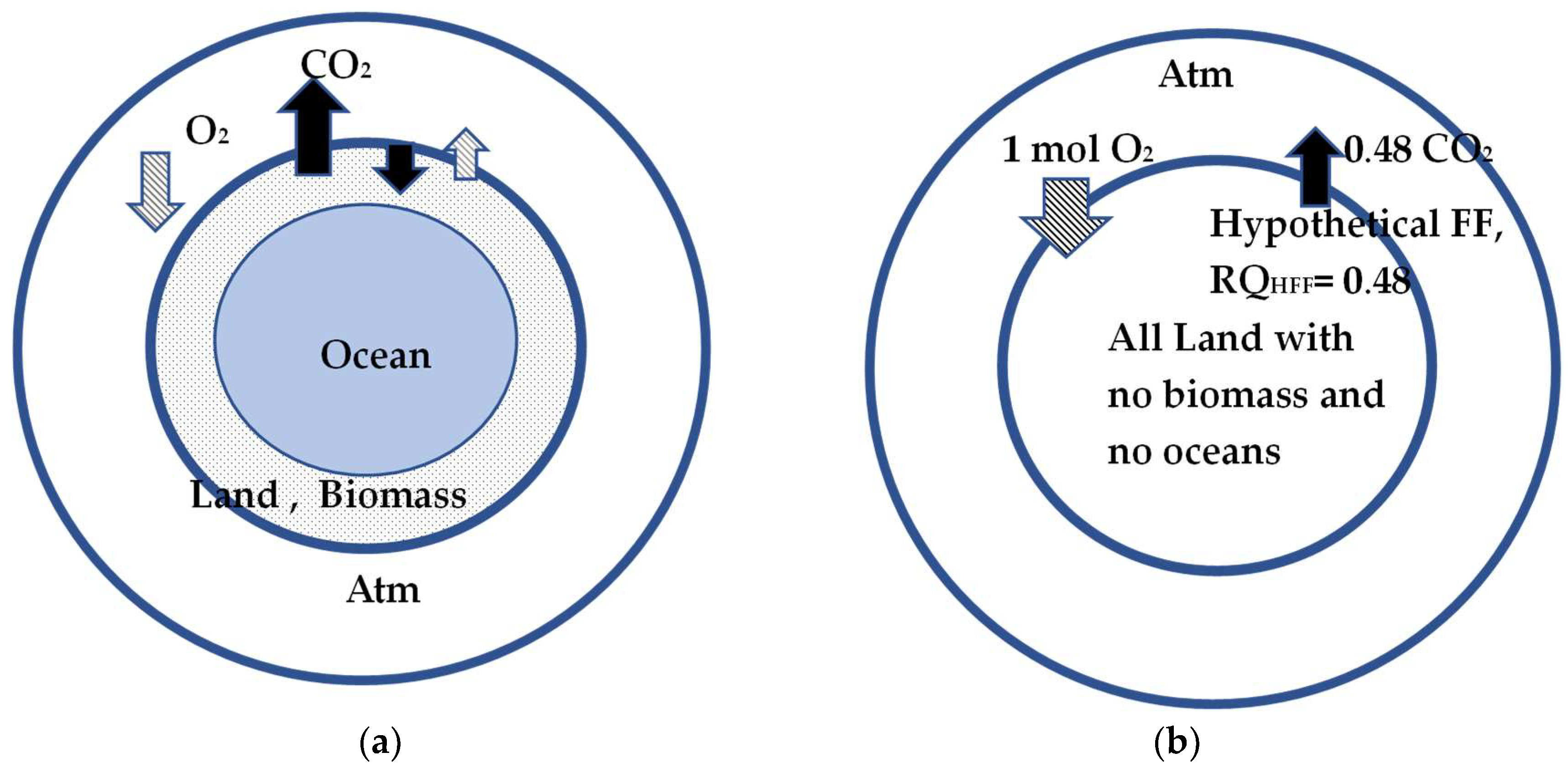
4.5.2. RQGlob < RQFFLU and Physical Meaning of RQGlob
4.5.3. RQGlob and Rationality for RQGlob < RQFF
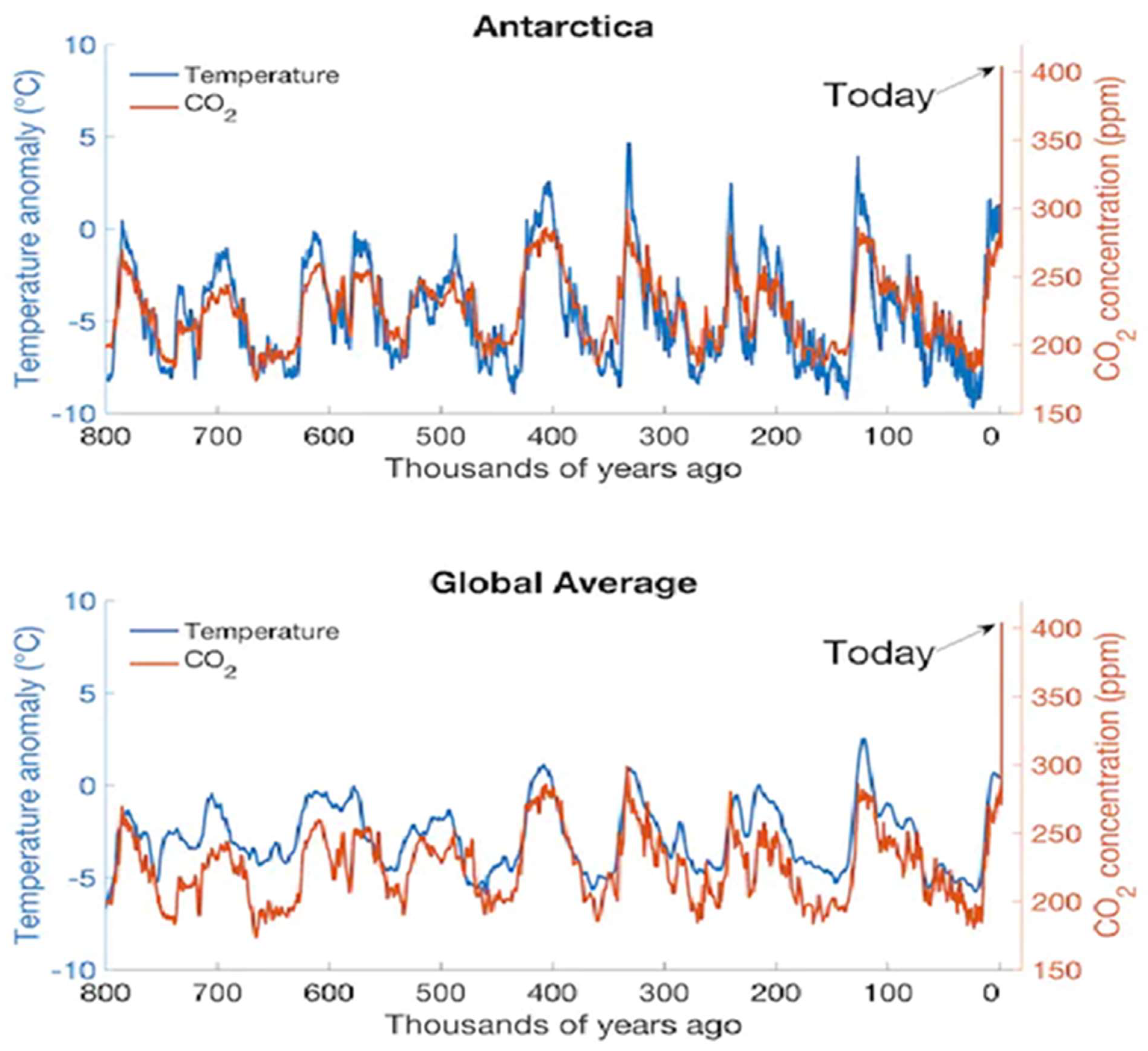
4.5.4. Predicted Saw-Tooth Pattern of O2 Given CO2 Data and RQGlob
4.5.5. Death of BS vs. Death of Green Planet Earth
4.5.6. Earth Tilt Angle and Mining and Drilling of Fossil Fuels
4.5.7. Effects of Presence of CH4 in the Atmosphere
4.5.8. RQGlob and CO2 in Giga Tons per Exa J
5. Conclusions
6. Future Work
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Atm | Atmosphere |
| BS | Biological system GPP: gross prod, total prod |
| CSI,CEng | See Section 3.3.2 on incomplete combustion/Flare |
| (s) | Carbon sink o source rate |
| CH | Carbohydrate |
| CO2 eq | CO2 equivalent |
| DAF | Dry ash free |
| Energy release rate from FFLU | |
| EPICA | European Project for Ice Coring in Antarctica |
| ExR | Exchange ratio = 1/RQ |
| F | Fat |
| FF | Fossil fuel |
| FFLU | Fossil fuel and land use |
| FW | Fall or Autumn (Sep, Oct, Nov) and Winter (Dec, Jan, Feb) |
| GCB | Global Carbon Budget |
| GPP | Gross Primary Production |
| GWP | Global warming potential-CO2 Equivalent |
| Giga J | 109 J = 106 kilo J = 103 Mega J = 10 −6 Peta J = 10−9 Exa J |
| GT | Giga tons, 109 Tons |
| HC | Hydrocarbon |
| HHVO₂ | Higher or gross heating value per Peta Oxygen moles |
| HSFF | Hypothetical Single Fossil Fuel |
| LBM | Land biomass |
| LOWBM | Combined land and ocean water biomasses |
| LU | Land use |
| M | Molecular weight |
| MOM | Marine organic matter |
| Mole rates of CO2, O2 per year | |
| CO2 release rate from FFLU and O2 consumption by FFLU, Peta moles/year | |
| NBS | Non-biological systems |
| NC,nut | Number of C atoms in nutrient |
| NCO₂,BM | number of moles of CO2 used by biomass for growth |
| NO₂,BM | number of moles of O2 from biomass |
| NEP | Net Ecosystem Production |
| NPP | Net Primary Production, = GPP- respiration cost |
| Ocn | Ocean |
| OWBM | Ocean water biomass |
| NOAA | National Oceanic and Atmospheric Administration |
| NPP | Net Primary Product in ecosystem |
| Per meg | 4.76 Per Meg = 1 ppm |
| PP | Phytoplankton |
| Ppb | Parts per billion |
| Ppm | Parts per million or molecules per million molecules 1 ppm in atm = 7.77 GT for CO2, 2.12 GT for C, 1 ppm O2 in atm = 5.65 GT [85] |
| PQ | Photosynthetic quotient {= 1/RQ} |
| PS | Photosynthesis |
| Q | Heat |
| ρ | Density |
| RgQ | Regeneration Quotient (=1/RQ) |
| RQ | Respiratory Quotient, Subscript (i) BM Biomass, (ii) FF: Fossil Fuel (iii) FFLU: FF and land use, (iv) Glob: Global (v) Nut: nutrient, (vi) Vent: Ventilated |
| RR | Redfield ratio = 1/RQ |
| SS | Spring (Mar, Apr, May) and Summer (June, July, Aug) |
| St | Storage |
| UCF | Unit Carbon Formula |
| Volume flow rate | |
| Yk | Mass fraction of element k, g of k/g of mixture |
| z | Ratio of Oxygen moles supplied by ocean biomass to atm to total oxygen moles supplied by both Land and Ocean biomasses |
Appendix A
Appendix A.1. Respiration Quotient (RQ) for Land Biomasses and Sea Weeds
| Name | H/C | N/C | O/C | S/C | O/H | HHV, kJ/g | YC | YH | YN | YO | YS | RQBM |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Field crops | ||||||||||||
| Alfalfa seed straw | 1.373 | 0.0183 | 0.654 | 1.602 × 10−4 | 0.476 | 18.45 | 0.468 | 0.054 | 0.01 | 0.407 | 0.0002 | 0.984 |
| Bean straw | 1.547 | 0.0166 | 0.785 | 8.718 × 10−5 | 0.507 | 17.46 | 0.430 | 0.0559 | 0.0083 | 0.449 | 0.0001 | 1.006 |
| Corn cobs | 1.499 | 0.0086 | 0.733 | 8.042 × 10−5 | 0.489 | 18.77 | 0.466 | 0.0587 | 0.0047 | 0.455 | 0.0001 | 0.992 |
| Corn stover | 1.515 | 0.0120 | 0.745 | 8.582 × 10−5 | 0.492 | 17.65 | 0.437 | 0.0556 | 0.0061 | 0.433 | 0.0001 | 0.994 |
| Cotton stalks | 1.527 | 0.0261 | 0.744 | 1.898 × 10−4 | 0.487 | 15.83 | 0.395 | 0.0507 | 0.012 | 0.391 | 0.0002 | 0.990 |
| Rice straw (fall) | 1.318 | 0.0144 | 0.657 | 7.173 × 10−4 | 0.499 | 16.28 | 0.418 | 0.0463 | 0.007 | 0.366 | 0.0008 | 0.998 |
| Rice straw (weathered) | 1.351 | 0.0230 | 0.768 | 1.732 × 10−3 | 0.568 | 14.56 | 0.346 | 0.0393 | 0.0093 | 0.354 | 0.0016 | 1.046 |
| Wheat straw | 1.376 | 0.0121 | 0.685 | 9.539 × 10−4 | 0.497 | 17.51 | 0.432 | 0.05 | 0.0061 | 0.394 | 0.0011 | 0.997 |
| Orchard prunings | ||||||||||||
| Almond prunings | 1.226 | 0.0110 | 0.598 | 7.302 × 10−5 | 0.488 | 20.01 | 0.513 | 0.0529 | 0.0066 | 0.409 | 0.0001 | 0.993 |
| Black Walnut | 1.390 | 0.0038 | 0.652 | 7.522 × 10−5 | 0.469 | 19.83 | 0.498 | 0.0582 | 0.0022 | 0.433 | 0.0001 | 0.979 |
| English Walnut | 1.346 | 0.0064 | 0.651 | 7.534 × 10−5 | 0.484 | 19.63 | 0.497 | 0.0563 | 0.0037 | 0.431 | 0.0001 | 0.989 |
| Vineyard prunings | ||||||||||||
| Cabernet Sauvignon | 1.493 | 0.0153 | 0.707 | 3.216 × 10−4 | 0.474 | 19.03 | 0.466 | 0.0585 | 0.0083 | 0.439 | 0.0004 | 0.980 |
| Chenin Blanc | 1.459 | 0.0154 | 0.655 | 5.461 × 10−4 | 0.449 | 19.13 | 0.480 | 0.0589 | 0.0086 | 0.419 | 0.0007 | 0.964 |
| Pinot Noir | 1.468 | 0.0156 | 0.685 | 7.947 × 10−5 | 0.467 | 19.05 | 0.471 | 0.0582 | 0.0086 | 0.430 | 0.0001 | 0.976 |
| Thompson seedless | 1.449 | 0.0139 | 0.687 | 7.912 × 10−5 | 0.474 | 19.35 | 0.474 | 0.0577 | 0.0077 | 0.433 | 0.0001 | 0.981 |
| Tokay | 1.449 | 0.0135 | 0.670 | 2.353 × 10−4 | 0.462 | 19.31 | 0.478 | 0.0582 | 0.0075 | 0.426 | 0.0003 | 0.973 |
| Energy crops | ||||||||||||
| Camaldulensis | 1.425 | 0.0052 | 0.674 | 7.645 × 10−5 | 0.473 | 19.42 | 0.490 | 0.0587 | 0.003 | 0.440 | 0.0001 | 0.981 |
| Globulus | 1.461 | 0.0069 | 0.688 | 7.775 × 10−5 | 0.471 | 19.23 | 0.482 | 0.0592 | 0.0039 | 0.442 | 0.0001 | 0.979 |
| Grandis | 1.449 | 0.0027 | 0.701 | 7.751 × 10−5 | 0.484 | 19.35 | 0.483 | 0.0589 | 0.0015 | 0.451 | 0.0001 | 0.988 |
| Casuarina | 1.426 | 0.0104 | 0.670 | 1.541 × 10−4 | 0.469 | 19.44 | 0.486 | 0.0583 | 0.0059 | 0.434 | 0.0002 | 0.979 |
| Cattails | 1.452 | 0.0148 | 0.742 | 3.486 × 10−4 | 0.511 | 17.81 | 0.430 | 0.0525 | 0.0074 | 0.425 | 0.0004 | 1.007 |
| Popular | 1.436 | 0.0083 | 0.677 | 7.732 × 10−5 | 0.471 | 19.38 | 0.485 | 0.0585 | 0.0047 | 0.437 | 0.0001 | 0.980 |
| Sudan grass | 1.427 | 0.0233 | 0.660 | 6.722 × 10−4 | 0.462 | 17.39 | 0.446 | 0.0535 | 0.0121 | 0.392 | 0.0008 | 0.973 |
| Forest residue | ||||||||||||
| Black Locust | 1.338 | 0.0096 | 0.620 | 7.384 × 10−5 | 0.464 | 19.71 | 0.507 | 0.0571 | 0.0057 | 0.419 | 0.0001 | 0.976 |
| Chaparral | 1.288 | 0.0099 | 0.643 | 2.396 × 10−4 | 0.499 | 18.61 | 0.469 | 0.0508 | 0.0054 | 0.402 | 0.0003 | 0.999 |
| Madrone | 1.476 | 0.0011 | 0.703 | 1.561 × 10−4 | 0.476 | 19.41 | 0.480 | 0.0596 | 0.0006 | 0.450 | 0.0002 | 0.983 |
| Manzanita | 1.466 | 0.0030 | 0.696 | 1.555 × 10−4 | 0.475 | 19.3 | 0.482 | 0.0594 | 0.0017 | 0.447 | 0.0002 | 0.982 |
| Ponderosa Pine | 1.446 | 0.0010 | 0.676 | 2.282 × 10−4 | 0.467 | 20.02 | 0.493 | 0.0599 | 0.0006 | 0.444 | 0.0003 | 0.977 |
| Ten Oak | 1.475 | 0.0022 | 0.693 | 7.835 × 10−5 | 0.470 | 18.93 | 0.478 | 0.0593 | 0.0012 | 0.441 | 0.0001 | 0.978 |
| Redwood | 1.404 | 0.0008 | 0.636 | 2.219 × 10−4 | 0.453 | 20.72 | 0.506 | 0.0598 | 0.0005 | 0.429 | 0.0003 | 0.968 |
| White Fur | 1.451 | 0.0009 | 0.686 | 7.645 × 10−5 | 0.472 | 19.95 | 0.490 | 0.0598 | 0.0005 | 0.448 | 0.0001 | 0.980 |
| Food and fiber processing wastes | ||||||||||||
| Almond hulls | 1.392 | 0.0180 | 0.666 | 8.181 × 10−5 | 0.478 | 18.22 | 0.458 | 0.0536 | 0.0096 | 0.406 | 0.0001 | 0.985 |
| Almond shells | 1.578 | 0.0221 | 0.705 | 1.666 × 10−4 | 0.447 | 19.38 | 0.450 | 0.0597 | 0.0116 | 0.423 | 0.0002 | 0.960 |
| Babassu husks | 1.269 | 0.0044 | 0.631 | 2.978 × 10−4 | 0.497 | 19.92 | 0.503 | 0.0537 | 0.0026 | 0.423 | 0.0004 | 0.998 |
| Sugarcane bagasse | 1.420 | 0.0073 | 0.663 | 8.362 × 10−5 | 0.467 | 17.33 | 0.448 | 0.0535 | 0.0038 | 0.396 | 0.0001 | 0.977 |
| Coconut fiber dust | 1.194 | 0.0077 | 0.592 | 1.192 × 10−3 | 0.495 | 20.05 | 0.503 | 0.0505 | 0.0045 | 0.396 | 0.0016 | 0.996 |
| Cocoa hulls | 1.289 | 0.0530 | 0.515 | 9.321 × 10−4 | 0.399 | 19.04 | 0.482 | 0.0523 | 0.0298 | 0.331 | 0.0012 | 0.938 |
| Cotton gin trash | 1.580 | 0.0453 | 0.689 | 0 | 0.436 | 16.42 | 0.396 | 0.0526 | 0.0209 | 0.363 | 0 | 0.952 |
| Macadamia shells | 1.091 | 0.0057 | 0.548 | 6.885 × 10−5 | 0.502 | 21.01 | 0.544 | 0.0499 | 0.0036 | 0.397 | 0.0001 | 1.001 |
| Olive pits | 1.518 | 0.0063 | 0.669 | 1.535 × 10−4 | 0.441 | 21.39 | 0.488 | 0.0623 | 0.0036 | 0.435 | 0.0002 | 0.957 |
| Peach pits | 1.324 | 0.0052 | 0.554 | 3.534 × 10−4 | 0.419 | 20.82 | 0.530 | 0.059 | 0.0032 | 0.391 | 0.0005 | 0.949 |
| Peanut hulls | 1.419 | 0.0305 | 0.649 | 9.822 × 10−4 | 0.457 | 18.64 | 0.458 | 0.0546 | 0.0163 | 0.396 | 0.0012 | 0.970 |
| Pistachio shells | 1.440 | 0.0098 | 0.668 | 7.678 × 10−5 | 0.464 | 19.26 | 0.488 | 0.0591 | 0.0056 | 0.434 | 0.0001 | 0.974 |
| Rice hulls | 1.248 | 0.0084 | 0.657 | 1.829 × 10−4 | 0.526 | 16.14 | 0.410 | 0.043 | 0.004 | 0.359 | 0.0002 | 1.017 |
| Walnut shells | 1.359 | 0.0036 | 0.651 | 7.495 × 10−5 | 0.479 | 20.18 | 0.500 | 0.0571 | 0.0021 | 0.434 | 0.0001 | 0.986 |
| Wheat dust | 1.466 | 0.0630 | 0.638 | 1.720 × 10−3 | 0.436 | 16.2 | 0.414 | 0.051 | 0.0304 | 0.352 | 0.0019 | 0.953 |
| Trees | ||||||||||||
| Maple wood (dry) | 1.414 | 0.0042 | 0.619 | 0.000 | 0.438 | 16.2 | 0.506 | 0.0602 | 0.0025 | 0.417 | 0 | 0.958 |
| Mesquite (as rec) | 1.358 | 0.0122 | 0.578 | 2.578 × 10−4 | 0.426 | 16.7 | 0.436 | 0.0498 | 0.0062 | 0.336 | 0.0003 | 0.952 |
| Junifer (as rec) | 1.370 | 0.0049 | 0.563 | 7.599 × 10−5 | 0.411 | 19 | 0.493 | 0.0568 | 0.0028 | 0.370 | 0.0001 | 0.943 |
| Sea Weeds [69] | ||||||||||||
| Sargassum tenerrimum | 1.741 | 0.0248 | 1.420 | 1.809 × 10−2 | 0.816 | - | 0.321 | 0.047 | 0.0093 | 0.607 | 0.0155 | 1.345 |
| Sargassum sp. 1 | 1.593 | 0.0532 | 0.965 | 0.000 | 0.606 | - | 0.403 | 0.054 | 0.025 | 0.518 | 0 | 1.092 |
| Sargassum sp. 2 | 1.777 | 0.0461 | 0.674 | 0.000 | 0.379 | 14.7 | 0.255 | 0.0381 | 0.0137 | - | 0 | 0.903 |
| Sargassum horneri | 1.512 | 0.0161 | 0.647 | 1.355 × 10−2 | 0.428 | 15.44 | 0.489 | 0.0622 | 0.0092 | 0.422 | 0.0177 | 0.936 |
| Sargassum fluitans Dirty | 1.629 | 0.0460 | 0.725 | 1.442 × 10−2 | 0.445 | 13.87 | 0.270 | 0.037 | 0.0145 | 0.261 | 0.0104 | 0.944 |
| Sargassum fluitans Wash | 1.523 | 0.0362 | 0.706 | 7.579 × 10−3 | 0.464 | 15.27 | 0.391 | 0.05 | 0.0165 | 0.367 | 0.0079 | 0.966 |
| OTHERS | 0.000 | 0.0000 | 0.000 | 0.000 | 0.000 | 0 | 0.000 | 0 | 0 | 0.000 | 0 | 0.000 |
| Pineapple | 1.898 | 0.0171 | 0.799 | 0.000 | 0.421 | 15.27 | 0.401 | 0.064 | 0.008 | 0.427 | 0 | 0.930 |
| Grass | 1.585 | 0.0190 | 0.717 | 8.325 × 10−4 | 0.452 | 15.27 | 0.450 | 0.06 | 0.01 | 0.430 | 0.001 | 0.963 |
Appendix A.2. Saw Tooth Pattern Using RQGlob

Similarly, O2 at C is given as
References
- Available online: https://www.biologyonline.com/dictionary/life (accessed on 8 September 2022).
- Available online: https://www.cropnutrition.com/nutrient-knowledge/oxygen (accessed on 22 December 2021).
- Available online: https://greencover.com/category/seed-production/ (accessed on 8 November 2022).
- Available online: https://archive.ipcc.ch/ipccreports/sres/land_use/index.php?idp=24 (accessed on 24 October 2023).
- Boden, T.A.; Marland, G.; Andres, R.J. Global, Regional, and National Fossil-Fuel CO2 Emissions. In Carbon Dioxide Information Analysis Center, Oak Ridge National Laboratory; U.S. Department of Energy: Oak Ridge, TN, USA, 2010. [Google Scholar]
- Annamalai, K. Respiratory quotient (RQ), exhaust gas analyses, CO2 Emission and applications in automobile engineering. Adv. Automob. Eng. 2013, 2, 2–4. [Google Scholar] [CrossRef]
- Annamalai, K.; Thanapal, S.; Ranjan, D. Ranking renewable and fossil fuels on global warming potential using respiratory quotient (RQ) Concept. J. Combust. 2018, 2018, 1270708. [Google Scholar] [CrossRef]
- Hansen, J.; Sato, M.; Ruedy, R.; Lacis, A.; Oinas, V. Global warming in the twenty-first century: An alternative scenario. Proc. Natl. Acad. Sci. USA 2000, 97, 9875–9880. [Google Scholar] [CrossRef] [PubMed]
- Crutzen, P.J.; Mosier, A.R.; Smith, K.A.; Winiwarter, W. N2O release from agro-biofuel production negates global warming reduction by replacing fossil fuels. Atmos. Chem. Phys. Discuss. 2007, 7, 11191–11205. [Google Scholar]
- Hotsmark, B. Quantifying the global warming potential of CO2 emissions from wood fuels. Glob. Energy 2015, 7, 195–206. [Google Scholar]
- Nejat, P.; Jomehzadeh, F.; Taheri, M.M.; Gohari, M.; Majid, M.Z.A. A global review of energy consumption, CO2 emissions and policy in the residential sector (with an overview of the top ten CO2 emitting countries). Renew. Sustain. Energy Rev. 2015, 43, 843–862. [Google Scholar] [CrossRef]
- Keeling, C.D.; Piper, S.C.; Bacastow, R.B.; Wahlen, M.; Whorf, T.P.; Heimann, M.; Meijer, H.A. Atmospheric CO2 and 13CO2 Exchange with the Terrestrial Biosphere and Oceans from 1978 to 2000: Observations and Carbon Cycle Implications. In A History of Atmospheric CO2 and Its Effects on Plants, Animals, and Ecosystems; Ecological Studies; Springer: New York, NY, USA, 2005; Volume 177, pp. 83–113. [Google Scholar]
- Saravanan, R. Climate Demon; Cambridge Univ Press: Cambridge, UK, 2021. [Google Scholar]
- Balkanski, Y.; Monfray, P.; Battle, M.; Heimann, M. Ocean primary production derived from satellite data: An evaluation with atmospheric oxygen measurements. Glob. Biogeochem. Cycles 1999, 13, 257–271. [Google Scholar] [CrossRef]
- Available online: https://askdruniverse.wsu.edu/2020/01/10/trees-create-oxygen/ (accessed on 16 October 2022).
- Keeling, R.F. Scripps O2 Program Data; UC San Diego Library Digital Collections: San Diego, CA, USA, 2019. [Google Scholar] [CrossRef]
- Huang, J.; Huang, J.; Liu, X.; Li, C.; Ding, L.; Yu, H. The global oxygen budget and its future projection. Sci. Bull. 2018, 63, 1180. [Google Scholar] [CrossRef]
- Available online: https://scripps.ucsd.edu/news/broken-record-atmospheric-carbon-dioxide-levels-jump-again#:~:text=Scripps%20recorded%20a%20May%20CO,an%20elevation%20of%2013%2C600%20feet (accessed on 14 October 2023).
- Duursma, E.K.; Boission, M.P.R.M. Global oceanic and atmospheric oxygen stability considered in relation to the carbon cycle and to different time scales. Oceanol. Acta 1994, 17, 117–141. [Google Scholar]
- Duffy, K.A.; Schwalm, C.R.; Arcus, V.L.; Koch, G.W.; Liang, L.L.; Schipper, L.A. How close are we to the temperature tipping point of the terrestrial biosphere? Sci. Adv. 2021, 7, 3. [Google Scholar] [CrossRef]
- Available online: https://www.chegg.com/homework-help/questions-and-answers/data-let-s-use-1951-1980-average-global-temperature-14-c-base-temperature-create-new-colum-q34424338 (accessed on 24 October 2023).
- Jouzel, J.; Masson-Delmotte, V. EPICA Dome C Ice Core 800KYr deuterium data and temperature estimates. Pangaea 2007. [Google Scholar] [CrossRef]
- Lüthi, D.; Le Floch, M.; Bereiter, B.; Blunier, T.; Barnola, J.-M.; Siegenthaler, U.; Raynaud, D.; Jouzel, J.; Fischer, H.; Kawamura, K. High-resolution carbon dioxide concentration record 650,000–800,000 years before present. Nature 2008, 453, 379–382. [Google Scholar] [CrossRef] [PubMed]
- Jouzel, J.; Masson-Delmotte, V.; Cattani, O.; Dreyfus, G.; Falourd, S.; Hoffmann, G.; Minster, B.; Nouet, J.; Barnola, J.M.; Chappellaz, J.; et al. Orbital and millennial Antarctic climate variability over the past 800,000 years. Science 2007, 317, 793–797. [Google Scholar] [CrossRef] [PubMed]
- Available online: https://eng.libretexts.org/Bookshelves/Environmental_Engineering_(Sustainability_and_Conservation)/Energy_Conservation_for_Environmental_Protection_(Pisupati) (accessed on 24 October 2023).
- Available online: https://www.saildrone.com/news/hurricane-sam-saildrone-sails-back-into-eye-storm (accessed on 24 October 2023).
- Available online: https://www.nytimes.com/interactive/2023/05/09/magazine/hurricane-saildrone.html?unlocked_article_code=VUzdTI4_2Wkbe3pfTGzCSB6C-YwQ-xsFx00o1N8VWsm9ruYqPH8NHVCHj7epbQZdWW5v_5AfzFD3A-GAeKfNoskNsNxBqdlL8EnPCOqk7lhC3wec5TfyBq0X_-hXbKz4fvsT-B (accessed on 9 May 2023).
- Available online: https://urldefense.com/v3/__https://www.weather.gov/media/slc/ClimateBook/Annual*20Average*20Temperature*20By*20Year.pdf__;JSUlJQ!!KwNVnqRv!EkkZzI7LzrTnM3yjfkgKjKtJtqVJ9HO57RQ3sIC32HeaVd51lXEd0bjoolC_4xrzE98kvNco2hXO6U33z4lgxFAy5A$ (accessed on 10 May 2023).
- Zhu, Q.; Jiang, H.; Liu, J.; Peng, C.; Fang, X.; Yu, S.; Zhou, G.; Wei, X.; Ju, W. Forecasting carbon budget under climate change and CO2 fertilization for subtropical region in China using integrated biosphere simulator (IBIS) model. Pol. J. Ecol. 2011, 59, 3–24. Available online: https://pubs.usgs.gov/publication/70036163 (accessed on 10 May 2023).
- Friedlingstein, P.; O’Sullivan, M.; Jones, M.W.; Andrew, R.M.; Gregor, L.; Hauck, J.; Le Quéré, C.; Luijkx, I.T.; Olsen, A.; Peters, G.P.; et al. Global Carbon Budget 2022. Earth Syst. Sci. Data 2022, 14, 4811–4900. [Google Scholar] [CrossRef]
- Gasser, T.; Crepin, L.; Quilcaille, Y.; Houghton, R.A.; Ciais, P.; Obersteiner, M. Historical CO2 emissions from land use and land cover change and their uncertainty. J. Biogeosci. 2020, 17, 4075–4101. [Google Scholar] [CrossRef]
- Watson, R.T.; Noble, J.R.; Bolin, B.; Ravindranath, N.H.; Verardo, D.J.; Dokken, D.J. (Eds.) Land Use, Land-Use Change and Forestry; IPCC: Geneva, Switzerland; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Gloege, L.; Yan, M.; Zheng, T.; McKinley, G.A. Improved quantification of ocean carbon uptake by using machine learning to merge global models and pCO2 data. J. Adv. Model. Earth Syst. 2022, 14, e2021MS002620. [Google Scholar] [CrossRef]
- Terhaar, J.; Frölicher, T.L.; Joos, F. Observation-constrained estimates of the global ocean carbon sink from Earth system models. J. Biogeosci. 2022, 19, 4431–4457. [Google Scholar] [CrossRef]
- Li, H.; Ilyina, T.; Loughran, T.; Spring, A.; Pongratz, J. Reconstructions and predictions of the global carbon budget with an emission-driven Earth system model. Earth Syst. Dyn. 2023, 14, 101–119. [Google Scholar] [CrossRef]
- Yakir, D. Large rise in carbon uptake by land plants. Nature 2017, 544, 39–40. [Google Scholar] [CrossRef][Green Version]
- David, K. Expected Limits on the Potential for Carbon Dioxide Removal from Artificial Upwelling. J. Front. Mar. Sci. 2022, 9, 841894. [Google Scholar] [CrossRef]
- Li, C.; Huang, J.; Ding, L.; Liu, X.; Han, D.; Huang, J. Estimation of Oceanic and Land Carbon Sinks Based on the Most Recent Oxygen Budget. Earth’s Fut. 2021, 9, e2021EF002124. [Google Scholar] [CrossRef]
- Matthews, H.D.; Zickfeld, K.; Knutti, R.; Allen, M.R. Focus on cumulative emissions, global carbon budgets and the implications for climate mitigation targets. Environ. Res. Lett. 2018, 13, 010201. [Google Scholar] [CrossRef]
- Available online: https://github.com/openclimatedata/global-carbon-budget/blob/main/data/global-carbon-budget.csv#L2 (accessed on 9 May 2023).
- Available online: https://spacemath.gsfc.nasa.gov/earth/6Page54.pdf (accessed on 9 November 2023).
- Moreno, A.R.; Garcia, C.A.; Larkin, A.A.; Lee, J.A.; Wang, W.L.; Moore, J.K.; Primeau, F.W.; Martiny, A.C. Latitudinal gradient in the respiration quotient and the implications for ocean oxygen availability. Proc. Natl. Acad. Sci. USA 2020, 117, 22866–22872. [Google Scholar] [CrossRef] [PubMed]
- Redfield, A.C.; Ketchum, B.H.; Richards, F.A. The influence of organisms on the composition of seawater. Sea 1963, 2, 26–77. [Google Scholar]
- Silva, C.; Annamalai, K. Entropy Generation and Human Aging: Lifespan Entropy and Effect of Physical Activity Level. J. Entropy 2008, 10, 100–123. [Google Scholar] [CrossRef]
- Annamalai, K.; Puri, I.K.; Jog, M.A. Advanced Thermo-Dynamics Engineering, 2nd ed.; CRC/Taylor and Francis: Boca Raton, FL, USA, 2011. [Google Scholar]
- Al-Saady, N.M.; Blackmore, C.M.; Bennett, E.D. High fat, low carbohydrate, enteral feeding lowers PaCO2 and reduces the period of ventilation in artificially ventilated patients. Intensive Care Med. 1989, 15, 290–295. [Google Scholar] [CrossRef]
- Available online: https://en.wikipedia.org/wiki/Respiratory_quotient (accessed on 11 October 2023).
- Available online: https://www.frontiersin.org/articles/10.3389/fendo.2018.00806/full (accessed on 11 October 2023).
- Available online: https://www.lung.org/lung-health-diseases/lung-disease-lookup/copd/living-with-copd/nutrition (accessed on 11 October 2023).
- Pujia, A.; Mazza, E.; Ferro, Y.; Gazzaruso, C.; Coppola, A.; Doldo, P.; Grembiale, R.D.; Pujia, R.; Romeo, S.; Montalcini, T. Lipid Oxidation Assessed by Indirect Calorimetry Predicts Metabolic Syndrome and Type 2 Diabetes. Front. Endocrinol. 2018, 9, 806. [Google Scholar] [CrossRef]
- Patel, H.; Kerndt, C.C.; Bhardwaj, A. Physiology, Respiratory Quotient; StatPearls Publishing: Treasure Island, FL, USA, 2023. Available online: https://www.ncbi.nlm.nih.gov/books/NBK531494 (accessed on 24 August 2023).
- Available online: https://en.wikipedia.org/wiki/Composition_of_the_human_body (accessed on 19 August 2023).
- Anderson, L.A. On the hydrogen and oxygen content of marine phytoplankton. Deep-Sea Res. 1995, 42, 1675–1680. [Google Scholar] [CrossRef]
- Available online: https://en.wikipedia.org/wiki/Phytoplankton (accessed on 23 August 2023).
- Keeling, R.F. The atmospheric oxygen cycle: The oxygen isotopes of atmospheric CO2 and O2 and the O2/N2 ratio. Rev. Geophys. 1995, 33, 1253–1262. [Google Scholar] [CrossRef]
- Pries, C.H.; Angert, A.; Castanha, C.; Hilman, B.; Torn, M.S. Using respiration quotients to track changing sources of soil respiration seasonally and with experimental warming. Biogeosciences 2020, 17, 3045–3055. [Google Scholar] [CrossRef]
- Robinson, C. Microbial Respiration, the Engine of Ocean Deoxygenation. Front. Mar. Sci. 2019, 5, 533. [Google Scholar] [CrossRef]
- Tanioka, T.; Matsumoto, K. Stability of marine organic matter respiration stoichiometry. Geophys. Res. Lett. 2020, 47, e2019GL085564. [Google Scholar] [CrossRef]
- Riser, S.C.; Johnson, K.S. Net production of oxygen in the subtropical ocean. Nature 2008, 451, 323–325. [Google Scholar] [CrossRef] [PubMed]
- Ríos, A.f.; Fraga Pérez, F.F.; Figueiras, F.G. Chemical composition of phytoplankton and Particulate Organic Matter in the Ría de Vigo (NW Spain). Sci. Mar. 1998, 62, 257–271. [Google Scholar] [CrossRef]
- Available online: https://oceanservice.noaa.gov/facts/exploration.html (accessed on 11 May 2023).
- Available online: https://www.noaa.gov/education/resource-collections/ocean-coasts/ocean-acidification (accessed on 10 October 2023).
- Mann, K.H.; Lazier, J.R.N. Chapter 10: The Oceans oand global Climate Change: Physcal and Bilofical Aspects. In Dynamics of Marine Ecosystems: Biological-Physical Interactions in the Oceans; Blackwell Scientific Publications: Boston, MA, USA, 1991; pp. 390–422. [Google Scholar]
- Martin, M.S.; Vernet, M.; Cape, M.R.; Mesa, E.; Huertas, A.D.; Reigstad, M.; Wassmann, P.; Duarte, C.M. Relationship Between Carbon- and Oxygen-Based Primary Productivity in the Arctic Ocean, Svalbard Archipelago. Front. Mar. Sci. 2019, 6, 468. [Google Scholar] [CrossRef]
- Annamalai, K. Oxygen Deficient (OD) Combustion and Metabolism: Allometric Laws of Organs and Kleiber’s Law from OD Metabolism? Systems 2021, 9, 54. [Google Scholar] [CrossRef]
- Popovic, M. Thermodynamic properties of microorganisms: Determination and analysis of enthalpy, entropy, and Gibbs free energy of biomass, cells and colonies of 32 microorganism species. Heliyon 2019, 5, 6. [Google Scholar] [CrossRef] [PubMed]
- Kort, E.A.; Plant, G.; Brandt, A.R.; Chen, Y.; Fordice, G.; Negron AM, G.; Schwietzke, S.; Smith, M.; And Zavala-Araiza, D. Inefficient and unlit natural gas flares both emit large quantities of methane. Science 2022, 377, 1566–1571. [Google Scholar] [CrossRef]
- Available online: https://chem.libretexts.org/Bookshelves/General_Chemistry/Book%3A_ChemPRIME_(Moore_et_al.)/03%3A_Using_Chemical_Equations_in_Calculations/3.03%3A_The_Limiting_Reagent/3.3.02%3A_Environment-_TSP_Ecological_Stoichiometry_and_Algal_Blooms (accessed on 28 October 2022).
- Sosa Olivier, J.A.; Laines Canepa, J.R.; Guerrero Zarate, D.; González Díaz, A.; Figueiras Jaramillo, D.A.; Osorio García, H.K.; Evia López, B. Bioenergetic valorization of Sargassum fluitans in the Mexican Caribbean: The determination of the calorific value and washing mechanism. Energy 2022, 10, 45–63. [Google Scholar] [CrossRef]
- Rosenberg, G.; Littler, D.S.; Littler, M.M.; Oliveira, E.C. Primary production and photosynthetic quotients of seaweeds from Sao Paulo State, Brazil. Bot. Mar. 1995, 38, 369–377. [Google Scholar] [CrossRef]
- Available online: https://energy.appstate.edu/research/work-areas/cdiac-appstate (accessed on 20 August 2022).
- Available online: https://data.ess-dive.lbl.gov/portals/CDIAC (accessed on 13 May 2023).
- Available online: https://github.com/openclimatedata/global-carbon-budget/blob/main/archive/Global_Carbon_Budget_2019v1.0.xlsx (accessed on 2 May 2023).
- Available online: https://www.ipcc.ch/report/land-use-land-use-change-and-forestry/ (accessed on 13 January 2022).
- Available online: https://cdiac.ess-dive.lbl.gov/ftp/ndp030/global (accessed on 14 January 2023).
- Fossil Fuels ‘Stubbornly’ Dominating Global Energy Despite Surge in Renewables: Energy Institute. Available online: https://www.spglobal.com/commodityinsights/en/market-insights/latest-news/oil/062623-fossil-fuels-stubbornly-dominating-global-energy-despite-surge-in-renewables-energy-institute (accessed on 16 November 2022).
- Severinghaus, J.P. Studies of the Terrestrial O2 and Carbon Cycles in Sand Dune Gases and in Biosphere 2. Ph.D. Thesis, Columbia University, New York, NY, USA, 1995. [Google Scholar]
- Available online: https://ourworldindata.org/grapher/annual-change-fossil-fuels?tab=tabl (accessed on 10 August 2023).
- Available online: https://ourworldindata.org/ (accessed on 18 October 2022).
- Friedlingstein, P.; O’Sullivan, M.; Jones, M.W.; Andrew, R.M.; Hauck, J.; Olsen, A.; Peters, G.P.; Peters, W.; Pongratz, J.; Sitch, S.; et al. Global Carbon Budget 2020. Earth Syst. Sci. Data 2020, 12, 3269–3340. [Google Scholar] [CrossRef]
- Keeling, R.F. Measuring correlations between atmospheric oxygen and carbondioxide mole fractions—A preliminary-study in urban air. J. Atmos. Chem. 1988, 7, 153–176. [Google Scholar] [CrossRef]
- Annamalai, K.; Sweeten, J.M.; Ramalingam, S.C. Estimation of gross heating values of biomass fuels. Trans. ASAE 1987, 30, 1205–1208. [Google Scholar] [CrossRef]
- Available online: https://ourworldindata.org/fossil-fuels#global-fossil-fuel-consumption (accessed on 24 August 2023).
- Available online: https://scrippsco2.ucsd.edu/data/atmospheric_co2/primary_mlo_co2_record.html (accessed on 8 August 2023).
- Ballantyne, A.P.; Alden, C.B.; Miller, J.B.; Tans, P.P.; White, J.W.C. Increase in observed net carbon dioxide uptake by land and oceans during the past 50 years. Nature 2012, 488, 70–72. [Google Scholar] [CrossRef] [PubMed]
- Annamalai, K. Breathing Planet Earth: Global Respiratory Quotient (RQglob) From Keeling’s Data and CO2-Budget among the Atmosphere, Land and Oceans. J. Energies 2023. under review. [Google Scholar]
- Available online: https://www.osha.gov/laws-regs/standardinterpretations/2007-04-02-0 (accessed on 6 November 2023).
- Keeling, R.F.; Powell, F.L.; Shaffer, G.; Robbins, P.A.; Simonson, T.S. Impacts of Changes in Atmospheric O2 on Human Physiology. Is There a Basis for Concern? Front. Physiol. 2021, 12, 571137. [Google Scholar] [CrossRef]
- Silva, M.; Lourenço, L.; Alves, R.D.F.B.; Sousa, L.F.; Almeida, S.E.D.S.; Farnese, F.S. Different ways to die in a changing world: Consequences of climate change for tree species performance and survival through an ecophysiological perspective. Ecol. Evol. 2019, 9, 11979–11999. [Google Scholar] [CrossRef]
- Available online: https://www.ncei.noaa.gov/products/ocean-carbon-acidification-data-system (accessed on 1 November 2023).
- Available online: https://www.epa.gov/climate-indicators/climate-change-indicators-ocean-acidity (accessed on 5 November 2023).
- Available online: https://keelingcurve.ucsd.edu/2013/06/04/why-does-atmospheric-co2-peak-in-may (accessed on 13 October 2023).
- Seo, K.-W.; Ryu, D.; Eom, J.; Jeon, T.; Kim, J.-S.; Youm, K.; Chen, J.; Wilson, C.R. Drift of Earth’s Pole Confirms Groundwater Depletion as a Significant Contributor to Global Sea Level Rise 1993–2010. Geophys. Res. Lett. 2023, 50, e2023GL103509. [Google Scholar] [CrossRef]
- Humans Are Pumping Out So Much Groundwater That It’s Changing Earth’s Tilt. Available online: https://www.space.com/earth-tilt-changed-by-groundwater-pumping (accessed on 8 November 2023).
- Kumar, B. Consequences of Fossil Fuel Extraction on the Climate Change of the Earth. In Proceedings of the AGU Chapman Conference on Complexity and Extreme Events in Geosciences in India, Chapman Conference on Complexity and Extreme Events in Geosciences, National Geographical Research Institute, Hyderabd, India, 15–19 February 2010. [Google Scholar]
- Ebeling, J.M.; Jenkins, B.M. Physical and chemical properties of biomass fuels. Trans. ASAE 1985, 28, 898–902. [Google Scholar] [CrossRef]
- Popp, J.; Lakner, Z.; Harangi-Rákos, M.; Fari, M. The effect of bioenergy expansion: Food, energy, and environment. Renew. Sustain. Energy Rev. 2014, 32, 559–578. [Google Scholar] [CrossRef]

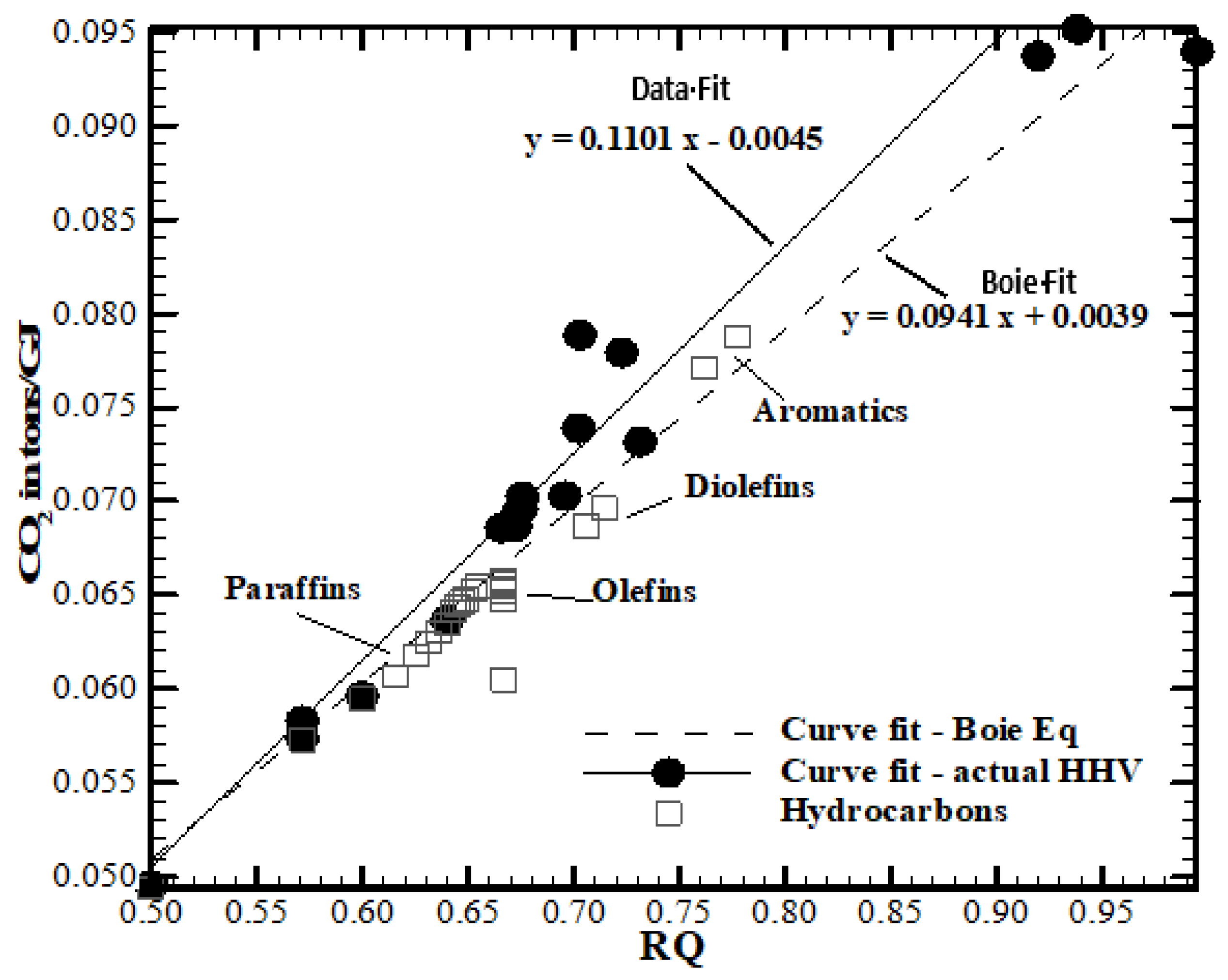

| Fuel | “h” or H/C | “o” or O/C, | RQ | HHV (kJ/kg) 1 |
|---|---|---|---|---|
| Wyoming coal | 0.70 | 0.18 | 0.92 | 18,347 |
| Gasoline (C8H18), YC = 0.85, ρ = 0.75 kg/L | 2.25 | 0.00 | 0.64 | 47,968 |
| Diesel (C12H23), ρ = 0.84 kg/L | 1.92 | 0.00 | 0.68 | 46,350 |
| #6 low S fuel oils | 0.12 | 0 | 0.73 | 42,900 |
| Methane CH4 | 4.00 | 0.00 | 0.50 | 55,426 |
| Organic acid (e.g., malic acid, C4H6O5) M = 134.1 | 1.5 | 1.25 | 1.33 | 9993 |
| Sargassum 2 (seaweed), CH1.74N0.025O1.42S0.018. | 1.74 | 1.42 | 1.57 | 20,120 (DAF) |
| Human Body wet (60% H2O, 40% dry) {C1.54H3.24N0.228O0.733, Ca0.037P0.032}.3.33 H2O(l), or {1.54 CH2.1N0.15O0.48Ca0.024P0.021}. 3.33 H2O(l) based on Ultimate analysis in Ref [52] | 2.1 | 0.48 | 0.76 | 23,500 (Boie, dry, no P oxid) |
| Generic PP: Cx(H2O)w (NH3)yHzH3PO4 (a) Without Nitrification (b) With Nitrification | {3 + 2w + 3y + z}/x {3 + 2w + 3y + z}/x | (w + 4)/x (w + 4)/x | 1 + (1/4)(z/x) 1 + 2(y/x) + (1/4)(z/x) | |
| Ocean Plankton CxH2w+3y+z+3NyO4P, [53] | x/{x + 2y + z/4) | |||
| Planktonic marine algae 3: Redfield–Ketchum–Richards Formula: C106 H263 O110 N16 P [53]; x = 106, y = 16, w = 106, C106 H263 O110 N16 P + 138 02 = 106 CO2 + 16 HN03 + H3P04 + 122 H2O RQ = 0.72 to 1.11 Note 1 | 2.481 | 1.038 | 0.77, 0.72 to 1.11 with an average of 0.82 [54] | |
| Keeling land vegetation via PS and respiration [55] | 0.95 (land) | |||
| Carbo-hydrate, CH (e.g., glucose) C6H12O6 or CH2O or sugars | 1.0 | 1.0 | 1.0 | |
| Fats C16H32O2 [7] | 2.0 | 0.1 | 0.7 | |
| Lipids: C40H74O5 | 1.85 | 0.125 | 0.73 or 0.68–0.8 [56] | |
| PProteins, C4.57H9.03N1.27O2.25S0.046 [45] | 1.98 | 0.49 | 0.8–0.9, see also [56] |
| Parameter of Interest | Linear Fit | Quadratic Fit |
|---|---|---|
| CO2 in atm., ppm | a1 ∗ years + a0, linear fit, years = current year-1991 a1 = 2.082 ppm /year, a0 = 350.5 ppm, R2 = 0.9709 when all data (multiple data within a year) was used {Fit: 381.7 ppm in 2006, Data in 2006 (average): 381.5 ppm} (average) | c2 ∗ years2 + c1 ∗ years + c0, years = current year-1991 c2 = 0.01889, c1 = 1.473, c0 = 354.1, R2 = 0.9804 when all data (multiple data within a year) was used Fit: 380.5 ppm in 2006, data in 2006: 381.5} |
| O2 in atm., ppm | b1 ∗ years + b0 b1 = −4.438 ppm/year, b0 = 209488, R2 = 0.9839 {Fit O2: 209,421, Data O2 = 209,423 ppm} | d2 ∗ years 2 + d1 ∗ years + d0, d2 = −0.04347265 ppm/year2, d1 = −3.0332 ppm/year., d0 = 209,480 ppm, R2 = 0.9896, {Fit O2: 209,425, with no of years since 1991 = 15, data O2 = 209,423 ppm} |
| d[CO2]/dt = CO2 change per year, ppm in atm./year | a1, positive, (Constant and independent of year) {2.082 ppm/year} | 2 ∗ c2 ∗ year + c1 = {0.0378 ∗ year + 1.473} {Fit 2.041 ppm/year with no. of years since 1991 = 15} |
| d[CO2]/dt, CO2 added to atm. GT in/year or required amount of CO2 to be sequestered if d[CO2]/dt needs to be zero. | a1 ∗ 7.77, (Constant and independent {16.2 GT of CO2 added per year or 4.41 GT of C/year} | {2 ∗ c2 ∗ year + c1} ∗ 7.77 = {0.0378 ∗ year + 1.473} ∗ 7.77 {15.9 GT/year or 4.33 GT of C/year} |
| d[O2]/dt = O2 change per year, ppm in atm./year | b1, negative, (Constant and independent of year {−4.438 ppm/year} | 2 ∗ d2 ∗ years + d1 = −0.0870 ∗ year − 3.0332 = −4.0438 {−4.305 ppm/year} |
| d[O2]/dt, Net O2 removed from atm. In GT/year or required amount of O2 to be added if d[|O2|]/dt in atm. Needs to be 0. | |b1| ∗ 5.65, (Constant and independent {25.07 GT of O2 depleted year} | {2 ∗ d2 ∗ year + d1} ∗ 5.65 = |{0.0870 ∗ year − 3.0332}| ∗ 5.65 {24.3 GT/year} |
| , | , (Constant and independent of year) {RQG, lob = 0.469} | , decreases slightly with years, {RQGlob = 0.470} |
| Earth’s annual mass loss rate due to Net C(s) mined, GT per year | RQGlob ∗ |b1| ∗ 0.177, 1 or a1 ∗ 2.12, 4.43 GT/year} | RQGlob ∗ 0.177 ∗ |2 ∗ d2 ∗ year + d1| Or |2 ∗ d2 ∗ year + d1| ∗ 2.12, {4.334 GT/year} |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Annamalai, K. Breathing Planet Earth: Analysis of Keeling’s Data on CO2 and O2 with Respiratory Quotient (RQ), Part I: Global Respiratory Quotient (RQGlob) of Earth. Energies 2024, 17, 299. https://doi.org/10.3390/en17020299
Annamalai K. Breathing Planet Earth: Analysis of Keeling’s Data on CO2 and O2 with Respiratory Quotient (RQ), Part I: Global Respiratory Quotient (RQGlob) of Earth. Energies. 2024; 17(2):299. https://doi.org/10.3390/en17020299
Chicago/Turabian StyleAnnamalai, Kalyan. 2024. "Breathing Planet Earth: Analysis of Keeling’s Data on CO2 and O2 with Respiratory Quotient (RQ), Part I: Global Respiratory Quotient (RQGlob) of Earth" Energies 17, no. 2: 299. https://doi.org/10.3390/en17020299
APA StyleAnnamalai, K. (2024). Breathing Planet Earth: Analysis of Keeling’s Data on CO2 and O2 with Respiratory Quotient (RQ), Part I: Global Respiratory Quotient (RQGlob) of Earth. Energies, 17(2), 299. https://doi.org/10.3390/en17020299








