A Thermodynamic-Based Black-Box Modeling Approach for the Comprehensive Analysis of Vortex Tube Applications
Abstract
1. Introduction
- Fluid mechanical approaches;
- Thermodynamic black-box approaches.
2. Methodology
2.1. Review of the Available Vortex Tube Modeling Approaches
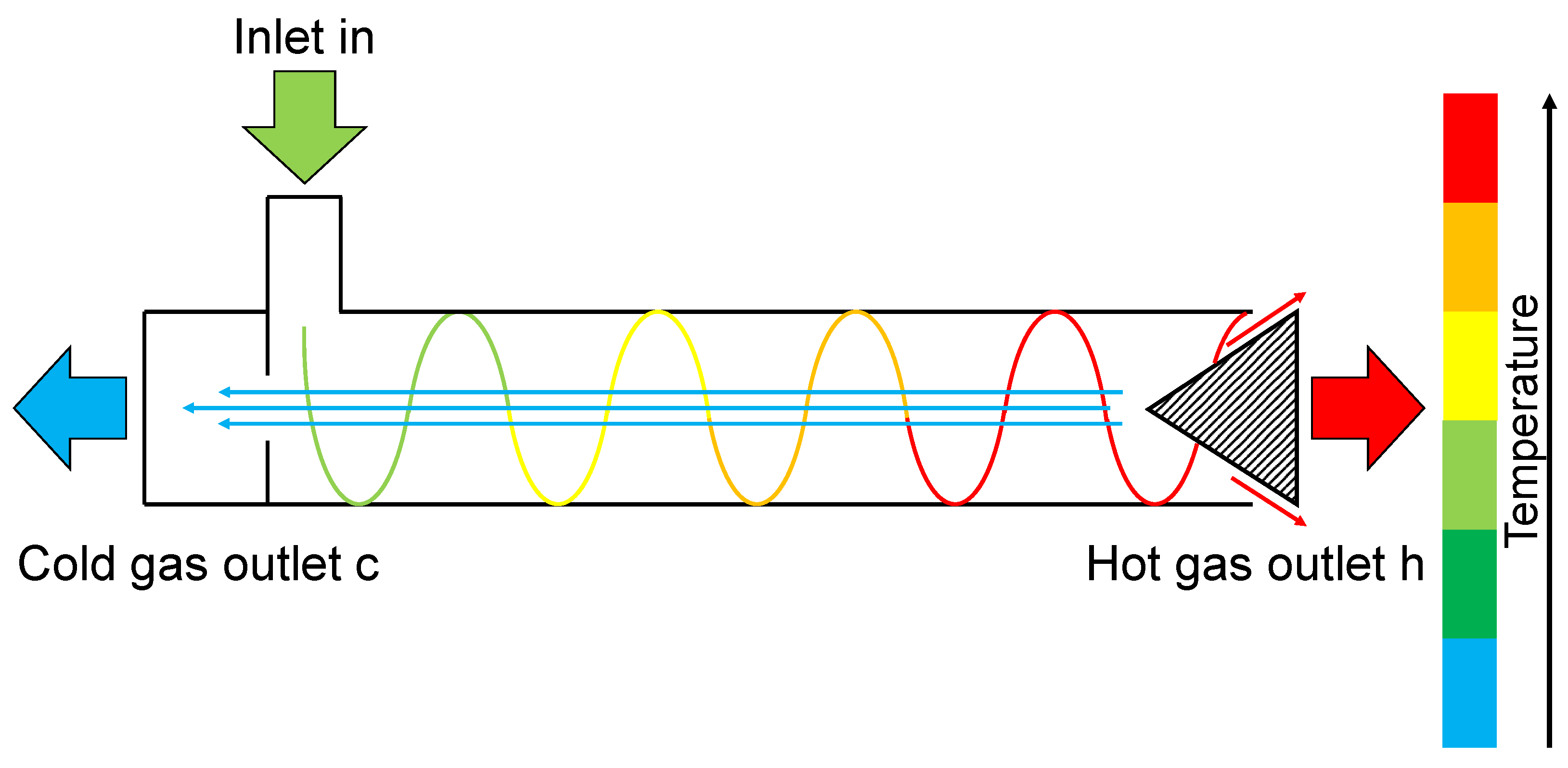
2.2. Derivation of New Black-Box Modeling Approach
2.3. Evaluation of Measured Data
- Neglect of the inequality of the energy balance;
- Correction of the entropy balance by means of heat flow;
- Correction of one output flow enthalpy and adiabatic entropy balance.
3. Results
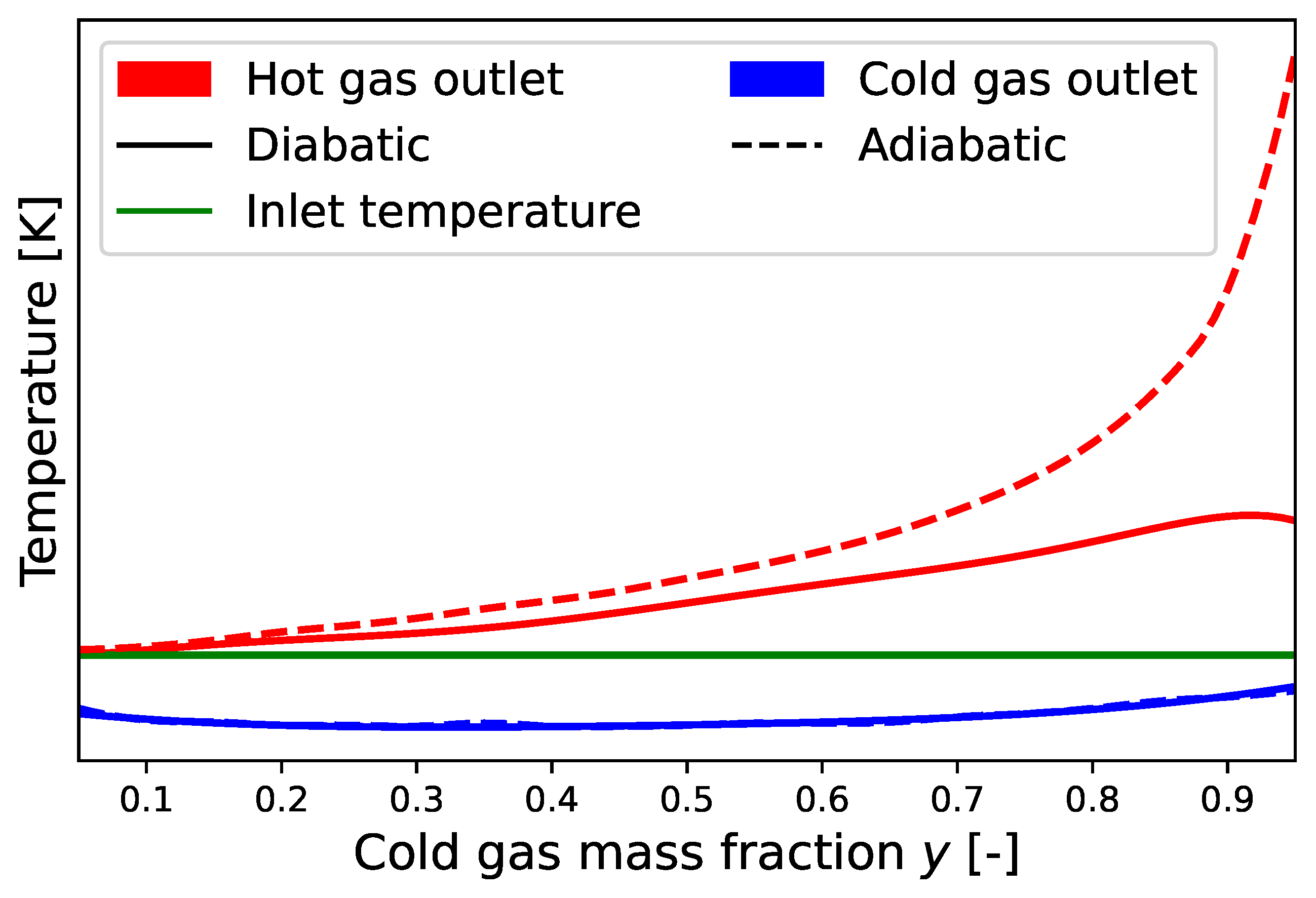
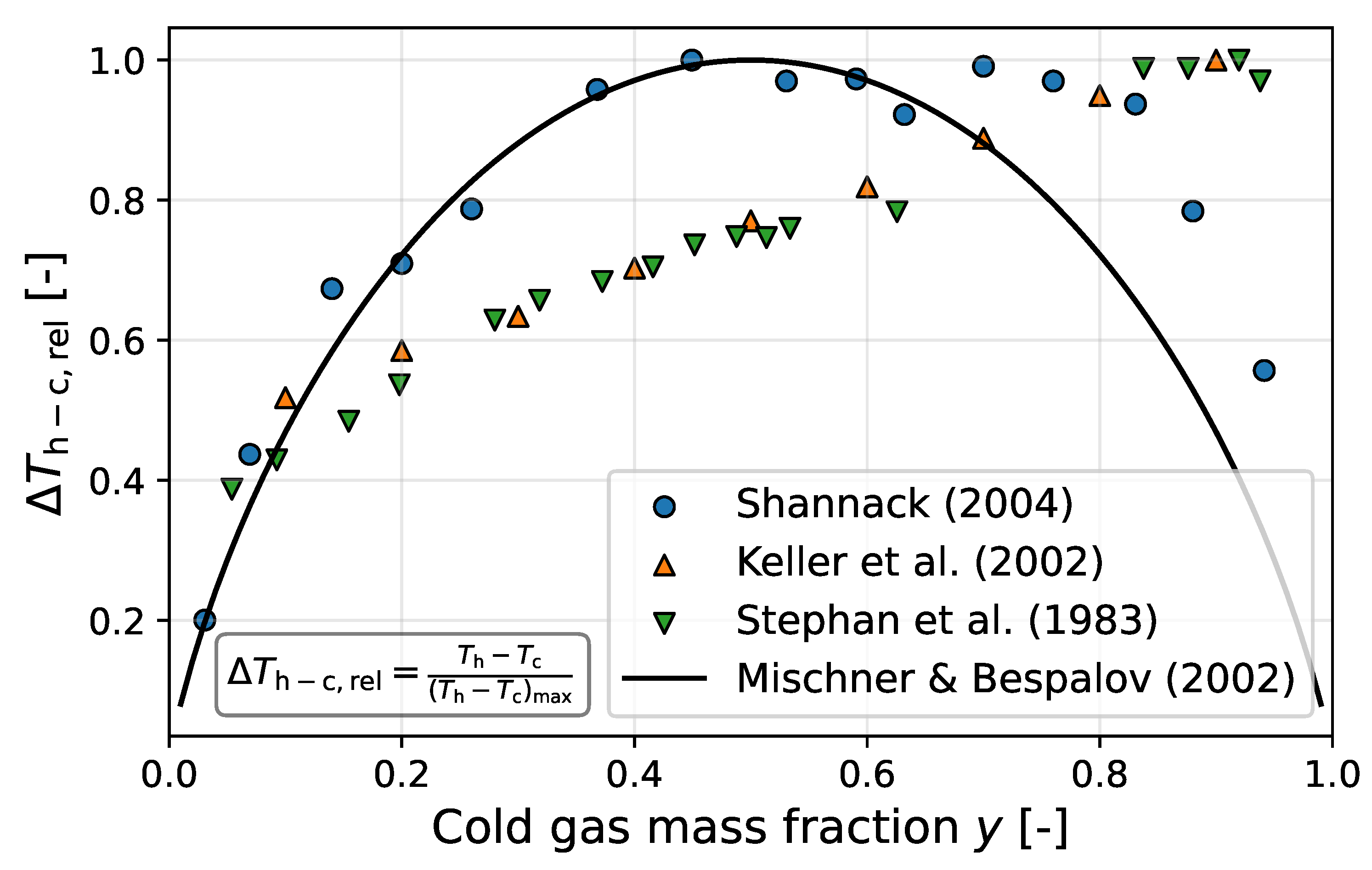
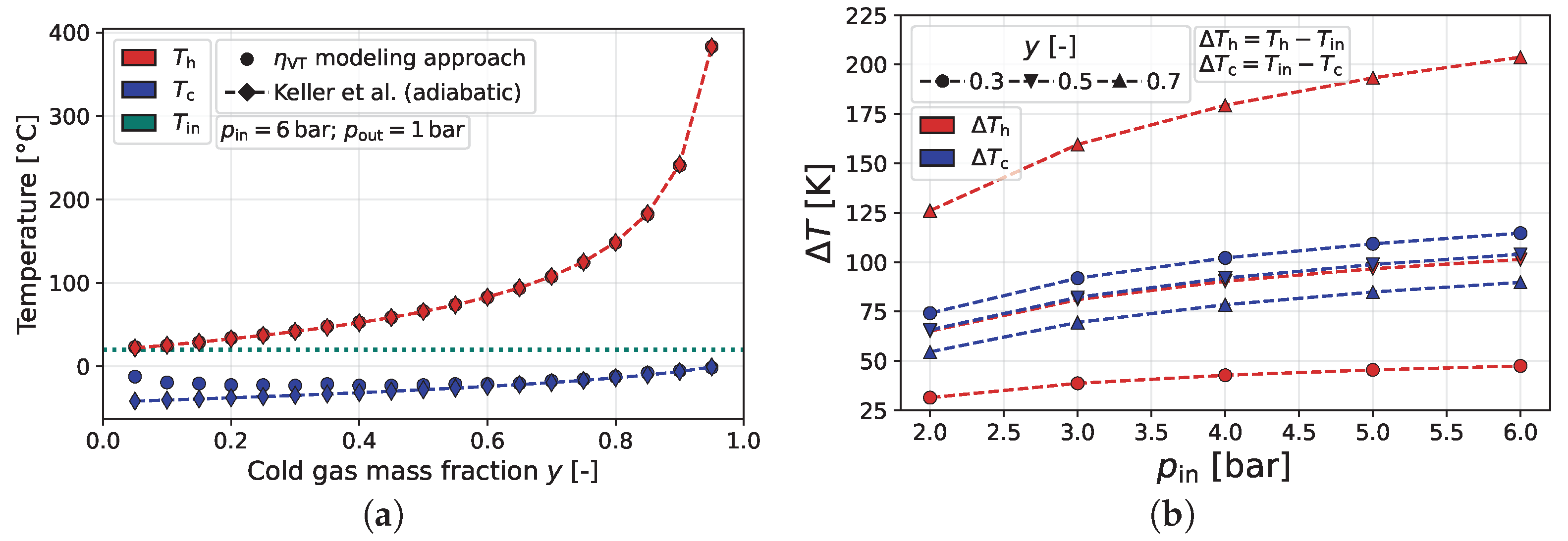
3.1. Cold Gas Mass Fraction Influence
- A maximum turning point;
- A steady increase.
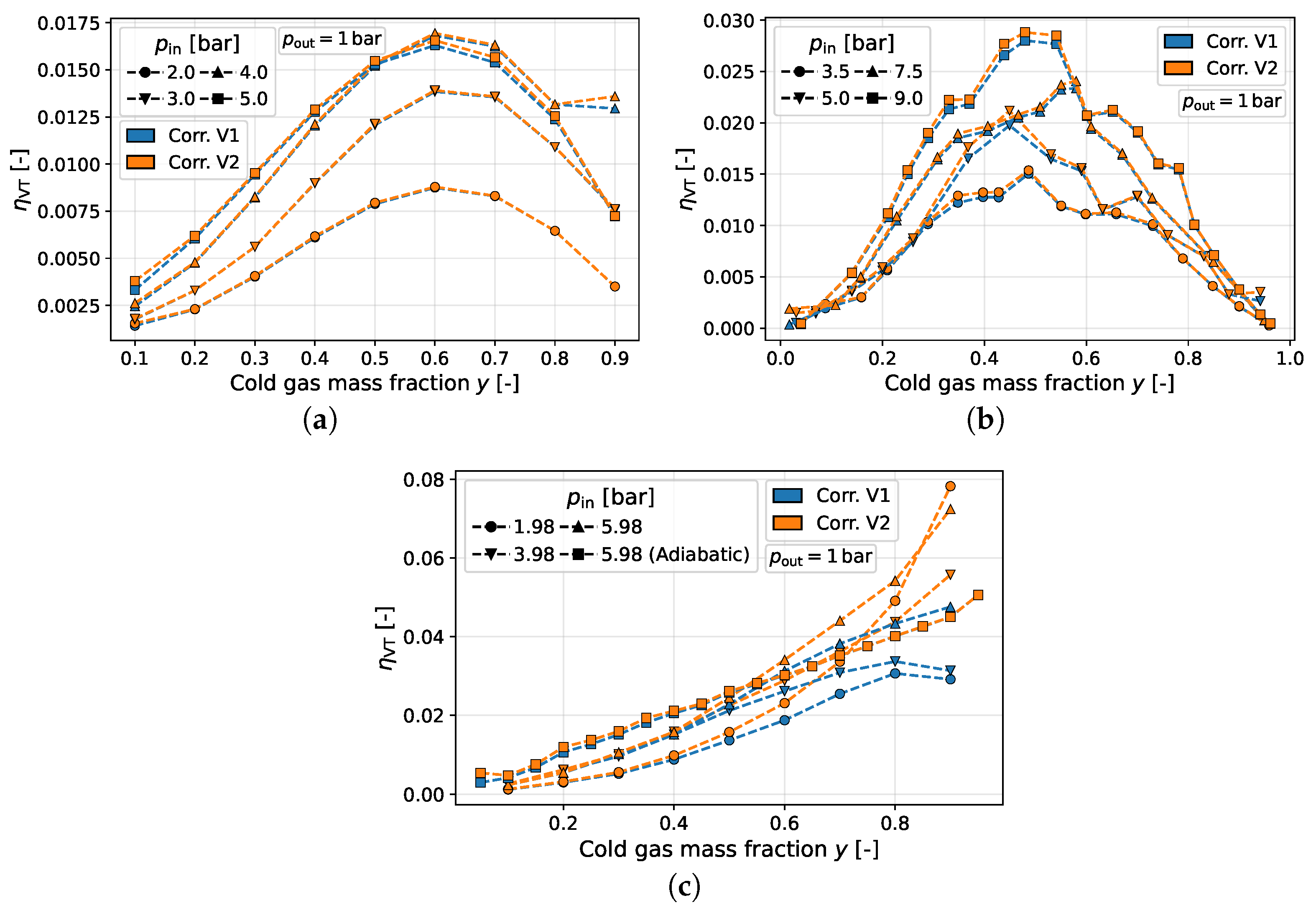
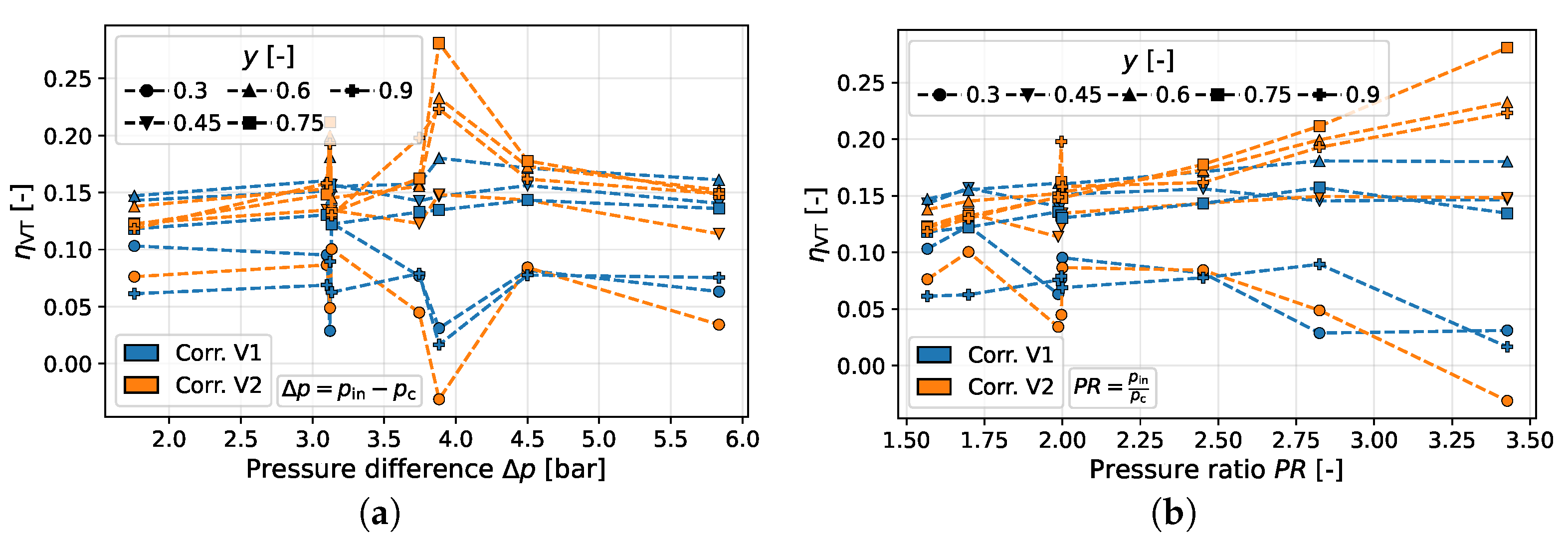
3.2. Pressure Influence
3.3. Further Influences of the Vortex Tube Efficiency
4. Discussion
5. Conclusions
- For the derivation of using experimental data, it is necessary to consider heat transfer to and from the ambient.
- The literature data do not provide a clear and comprehensive trend for the developed expression of , especially for the influence of the pressure ratio or the geometric influence.
- A simple conservative yet physical-based formulation of is capable of depicting the vortex tube behavior for air in alignment with measurement data.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Symbols | ||
| Symbol | Unit | Description |
| A | Area | |
| c | m/s | Velocity |
| J/(kg K) | Specific heat capacity at constant pressure | |
| d | m | Diameter |
| f | - | Function |
| - | Empirical constant | |
| - | Empirical constant | |
| h | J/kg | Specific enthalpy |
| J/kg | Corrected specific enthalpy | |
| H | - | Vortex tube coefficient |
| L | m | Length |
| kg/s | Mass flow | |
| M | kg/mol | Molar mass |
| p | Pa | Pressure |
| - | Pressure ratio | |
| J/kg | Specific heat flow | |
| R | J/(mol K) | Ideal gas constant |
| - | Rossby number | |
| s | J/(kg K) | Specific entropy |
| J/(kg K) | Corrected specific entropy | |
| T | K | Temperature |
| K | Corrected temperature | |
| X | - | De-dimensionalized pressure difference |
| y | - | Cold gas mass fraction |
| Greek symbols | ||
| Symbol | Unit | Description |
| - | Isentropic exponent | |
| - | Difference between two values | |
| - | Efficiency | |
| kg/ | Density | |
| - | Mass fraction | |
| Indices and abbreviations | ||
| Note: Indices which belong to one specific symbol (e.g., ) are not shown in this table. | ||
| Symbol | Description | |
| A | Component A of a binary mixture | |
| B | Component B of a binary mixture | |
| c | Cold outlet | |
| Corr | Correction | |
| h | Hot outlet | |
| h-c | Difference between hot and cold outlet | |
| i | Component i of a mixture | |
| in | Inlet | |
| irr | Irreversible | |
| mix | Mixutre | |
| out | Outlet | |
| p | Constant pressure | |
| ref | Reference | |
| rel | Relative | |
| sep | Separation | |
| th | Theoretically | |
| throt | Throttling valve | |
| T | Constant temperature | |
| V1 | Variant 1 | |
| V2 | Variant 2 | |
| VT | Vortex tube | |
References
- Ranque, G. Experiments on expansion in a vortex with simultaneous exhaust of hot air and cold air. J. Phys. Radium 1933, 4, 112–114. [Google Scholar]
- Hilsch, R. The use of expansion of gases in a centrifugal field as a cooling process. Rev. Sci. Instrum. 1947, 18, 108–113. [Google Scholar] [CrossRef] [PubMed]
- Keller, J.U.; Göbel, M.U.; Staudt, R. Das Wirbelrohr: Bemerkungen zu den Grundlagen und neuen energietechnischen Anwendungen. In Moderne Wege der Energieversorgung, Deutsche Physikalische Gesellschaft—Arbeitskreis Energie, Vorträge Leipzig Tagung (2002); The Physikzentrum Bad Honnef (PBH): Bad Honnef, Germany, 2003; pp. 125–161. [Google Scholar]
- Devade, K.D. Augmentation of Refrigeration Effect of Vortex Tube. Ph.D. Thesis, Shivaji University, Kolhapur, India, 2017. [Google Scholar]
- Collins, R.L.; Lovelace, R.B. Experimental Study of Two-Phase Propane Expanded through the Ranque-Hilsch Tube. J. Heat Transf. 1979, 101, 300–305. [Google Scholar] [CrossRef]
- Hamdan, M.O.; Al-Omari, S.A.; Oweimer, A.S. Experimental study of vortex tube energy separation under different tube design. Exp. Therm. Fluid Sci. 2018, 91, 306–311. [Google Scholar] [CrossRef]
- Stephan, K.; Lin, S.; Durst, M.; Huang, F.; Seher, D. An investigation of energy separation in a vortex tube. Int. J. Heat Mass Transf. 1983, 26, 341–348. [Google Scholar] [CrossRef]
- Parker, M.J.; Straatman, A.G. Experimental study on the impact of pressure ratio on temperature drop in a Ranque-Hilsch vortex tube. Appl. Therm. Eng. 2021, 189, 116653. [Google Scholar] [CrossRef]
- Thakare, H.; Monde, A.; Parekh, A. Experimental, computational and optimization studies of temperature separation and flow physics of vortex tube: A review. Renew. Sustain. Energy Rev. 2015, 52, 1043–1071. [Google Scholar] [CrossRef]
- Kirmaci, V.; Kaya, H. Effects of working fluid, nozzle number, nozzle material and connection type on thermal performance of a Ranque–Hilsch vortex tube: A review. Int. J. Refrig. 2018, 91, 254–266. [Google Scholar] [CrossRef]
- Subudhi, S.; Sen, M. Review of Ranque–Hilsch vortex tube experiments using air. Renew. Sustain. Energy Rev. 2015, 52, 172–178. [Google Scholar] [CrossRef]
- Thakare, H.R.; Parekh, A.D. Experimental investigation & CFD analysis of Ranque–Hilsch vortex tube. Energy 2017, 133, 284–298. [Google Scholar] [CrossRef]
- Promvonge, P.; Eiamsa-ard, S. Investigation on the Vortex Thermal Separation in a Vortex Tube Refrigerator. ScienceAsia 2005, 31, 215–223. [Google Scholar] [CrossRef]
- Aghagoli, A.; Sorin, M. Thermodynamic performance of a CO2 vortex tube based on 3D CFD flow analysis. Int. J. Refrig. 2019, 108, 124–137. [Google Scholar] [CrossRef]
- Ambedkar, P.; Dutta, T. CFD analysis of different working gases in Ranque-Hilsch vortex tube. Mater. Today Proc. 2023, 72, 761–767. [Google Scholar] [CrossRef]
- Zangana, L.M.K.; Barwari, R.R.I. The effect of convergent-divergent tube on the cooling capacity of vortex tube: An experimental and numerical study. Alex. Eng. J. 2020, 59, 239–246. [Google Scholar] [CrossRef]
- Bazgir, A.; Heydari, A.; Nabhani, N. Investigation of the thermal separation in a counter-flow Ranque-Hilsch vortex tube with regard to different fin geometries located inside the cold-tube length. Int. Commun. Heat Mass Transf. 2019, 108, 104273. [Google Scholar] [CrossRef]
- Sharma, T.; Rao, A.; K, M. Numerical Analysis of a Vortex Tube: A Review. Arch. Comput. Methods Eng. 2016, 24, 251–280. [Google Scholar] [CrossRef]
- Shannak, B.A. Temperature separation and friction losses in vortex tube. Heat Mass Transf. 2004, 40, 779–785. [Google Scholar] [CrossRef]
- Mansour, A.; Lagrandeur, J.; Poncet, S. Analysis of transcritical CO2 vortex tube performance using a real gas thermodynamic model. Int. J. Therm. Sci. 2022, 177, 107555. [Google Scholar] [CrossRef]
- Mischner, J.; Bespalov, V. Zur Thermo- und Gasdynamik des Ranque-Hilsch-Wirbelrohrs; VDI Verlag: Düsseldorf, Germany, 2002. [Google Scholar]
- Liu, Y.F.; Geng, C.J.; Jin, G.Y. Vortex Tube Expansion Transcritical CO2 Heat Pump Cycle. In Proceedings of the Digital Manufacturing & Automation III; Trans Tech Publications Ltd.: Bäch, Switzerland, 2012; Applied Mechanics and Materials; Volume 190, pp. 1340–1344. [Google Scholar] [CrossRef]
- Zhao, J.; Ning, J. Performance analysis on CO2 heat pump cycle with a vortex tube. In Proceedings of the 3rd International Conference on Smart Materials and Nanotechnology in Engineering (SMNE 2016), Sanya, China, 1–2 March 2016; pp. 183–188. [Google Scholar]
- Cetin, T.H.; Zhu, J. Thermodynamic assessment of a novel self-condensing sCO2 recompression system with vortex tube. Energy Convers. Manag. 2022, 269, 116110. [Google Scholar] [CrossRef]
- Exair—Vortex Tube for Spot Cooling. Available online: https://www.exair.com/products/vortex-tubes-and-spot-cooling-products/vortex-tubes.html (accessed on 8 March 2024).
- FILTAN-Wirbelrohrabscheider. Available online: http://www.filtan-gas.com/deutsch/wirbel_a_de.html (accessed on 29 November 2022).
- Liu, Y.; Sun, Y.; Tang, D. Analysis of a CO2 Transcritical Refrigeration Cycle with a Vortex Tube Expansion. Sustainability 2019, 11, 2021. [Google Scholar] [CrossRef]
- Fulton, C.D. Ranque’s tube. Refrig. Eng. 1950, 58, 473–479. [Google Scholar]
- Ahlborn, B.K.; Keller, J.U.; Rebhan, E. The Heat Pump in a Vortex Tube. J. Non-Equilib. Thermodyn. 1998, 23, 159–165. [Google Scholar] [CrossRef]
- Piralishvili, S.A.; Fuzeeva, A.A. Similarity of the energy-separation process in vortex Ranque tubes. J. Eng. Phys. Thermophys. 2006, 79, 27–32. [Google Scholar] [CrossRef]
- O’Connell, J.P. Detailed thermodynamics for analysis and design of Ranque-Hilsch vortex tubes. AIChE J. 2018, 64, 1067–1074. [Google Scholar] [CrossRef]
- Saidi, M.; Allaf Yazdi, M. Exergy model of a vortex tube system with experimental results. Energy 1999, 24, 625–632. [Google Scholar] [CrossRef]
- Williams, A. The Cooling of Methane with Vortex Tubes. J. Mech. Eng. Sci. 1971, 13, 369–375. [Google Scholar] [CrossRef]
- Kırmacı, V.; Uluer, O.; Dincer, K. An Experimental Investigation of Performance and Exergy Analysis of a Counterflow Vortex Tube Having Various Nozzle Numbers at Different Inlet Pressures of Air, Oxygen, Nitrogen, and Argon. J. Heat Transf. 2010, 132. [Google Scholar] [CrossRef]
- Han, X.; Li, N.; Wu, K.; Wang, Z.; Tang, L.; Chen, G.; Xu, X. The influence of working gas characteristics on energy separation of vortex tube. Appl. Therm. Eng. 2013, 61, 171–177. [Google Scholar] [CrossRef]
- Zhu, J. Experimental Investigation of Vortex Tube and Vortex Nozzle for Applications in Air-Conditioning, Refrigeration, and Heat Pump Systems. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign, IL, USA, 2015. [Google Scholar]
- Sarifudin, A.; Wijayanto, D.S.; Widiastuti, I.; Pambudi, N.A. Dataset of Comprehensive Thermal Performance on Cooling the Hot Tube Surfaces of Vortex Tube at Different Pressure and Fraction. Data Brief 2020, 30, 105611. [Google Scholar] [CrossRef] [PubMed]
- Majidi, D.; Alighardashi, H.; Farhadi, F. Best vortex tube cascade for highest thermal separation. Int. J. Refrig. 2018, 85, 282–291. [Google Scholar] [CrossRef]



| Author | Advantages | Disadvantages |
|---|---|---|
| Shannak [19] |
|
|
| Mansour et al. [20] |
|
|
| Mischner and Bespalov [21] |
|
|
| Keller et al. [3] |
|
|
| Liu et al. [22] |
|
|
| Assumptions |
|---|
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sager, R.; Petersen, N.H.; Wirsum, M. A Thermodynamic-Based Black-Box Modeling Approach for the Comprehensive Analysis of Vortex Tube Applications. Energies 2024, 17, 4180. https://doi.org/10.3390/en17164180
Sager R, Petersen NH, Wirsum M. A Thermodynamic-Based Black-Box Modeling Approach for the Comprehensive Analysis of Vortex Tube Applications. Energies. 2024; 17(16):4180. https://doi.org/10.3390/en17164180
Chicago/Turabian StyleSager, Robert, Nils Hendrik Petersen, and Manfred Wirsum. 2024. "A Thermodynamic-Based Black-Box Modeling Approach for the Comprehensive Analysis of Vortex Tube Applications" Energies 17, no. 16: 4180. https://doi.org/10.3390/en17164180
APA StyleSager, R., Petersen, N. H., & Wirsum, M. (2024). A Thermodynamic-Based Black-Box Modeling Approach for the Comprehensive Analysis of Vortex Tube Applications. Energies, 17(16), 4180. https://doi.org/10.3390/en17164180






