Deposition Distribution and Thermal Resistance Analysis of Fins in Heat Exchangers
Abstract
1. Introduction
2. Numerical Models and Methods
2.1. Continuous Phase
- (1)
- mass conservation equation
- (2)
- momentum conservation equation
- (3)
- turbulent model
2.2. Discrete Phase
2.2.1. Particle Transport Model
2.2.2. Random Function
2.2.3. Critical Velocity Deposition Model
2.2.4. Particle Removal Model
2.2.5. Dynamic Mesh
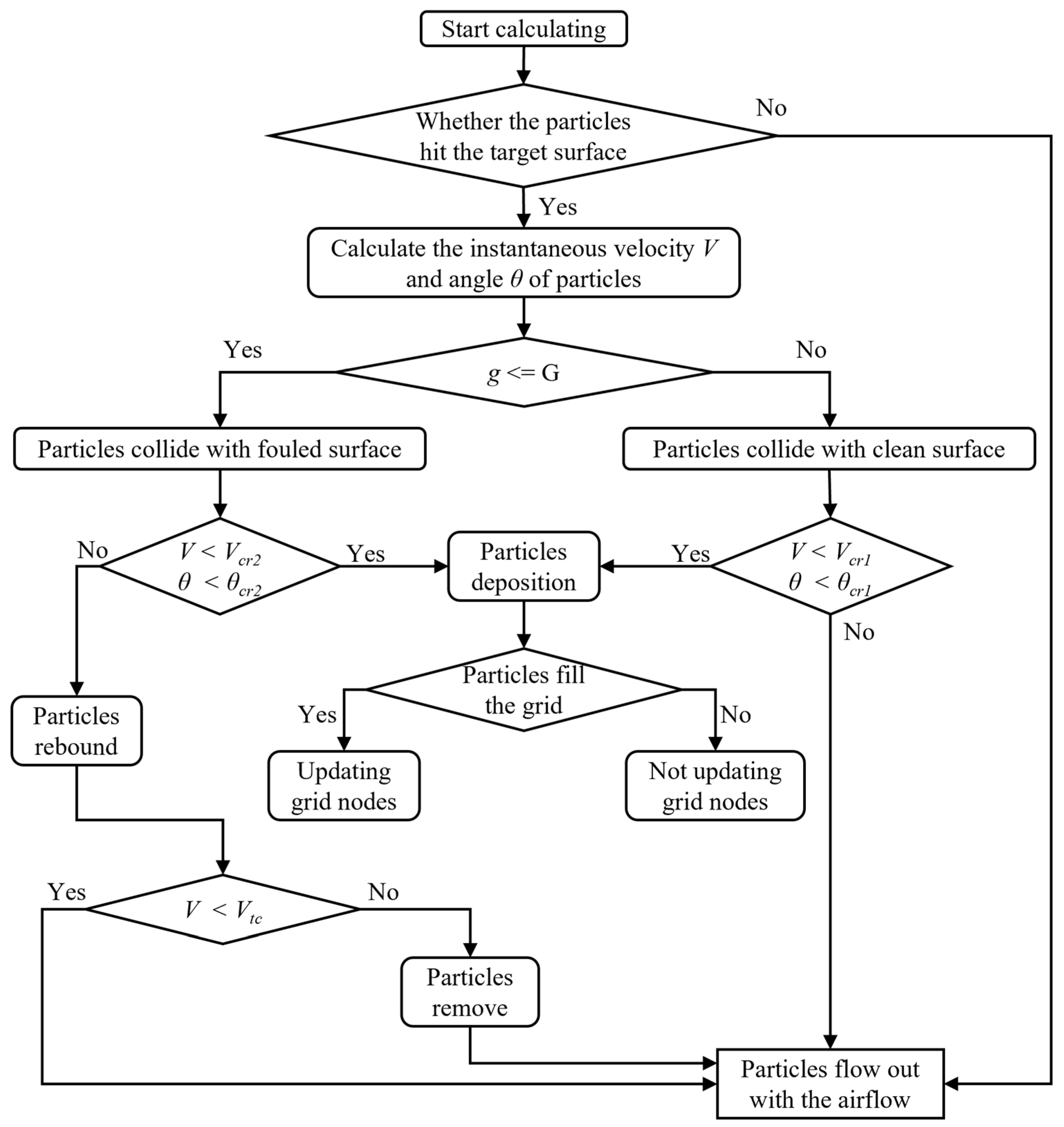
2.3. Calculation Process
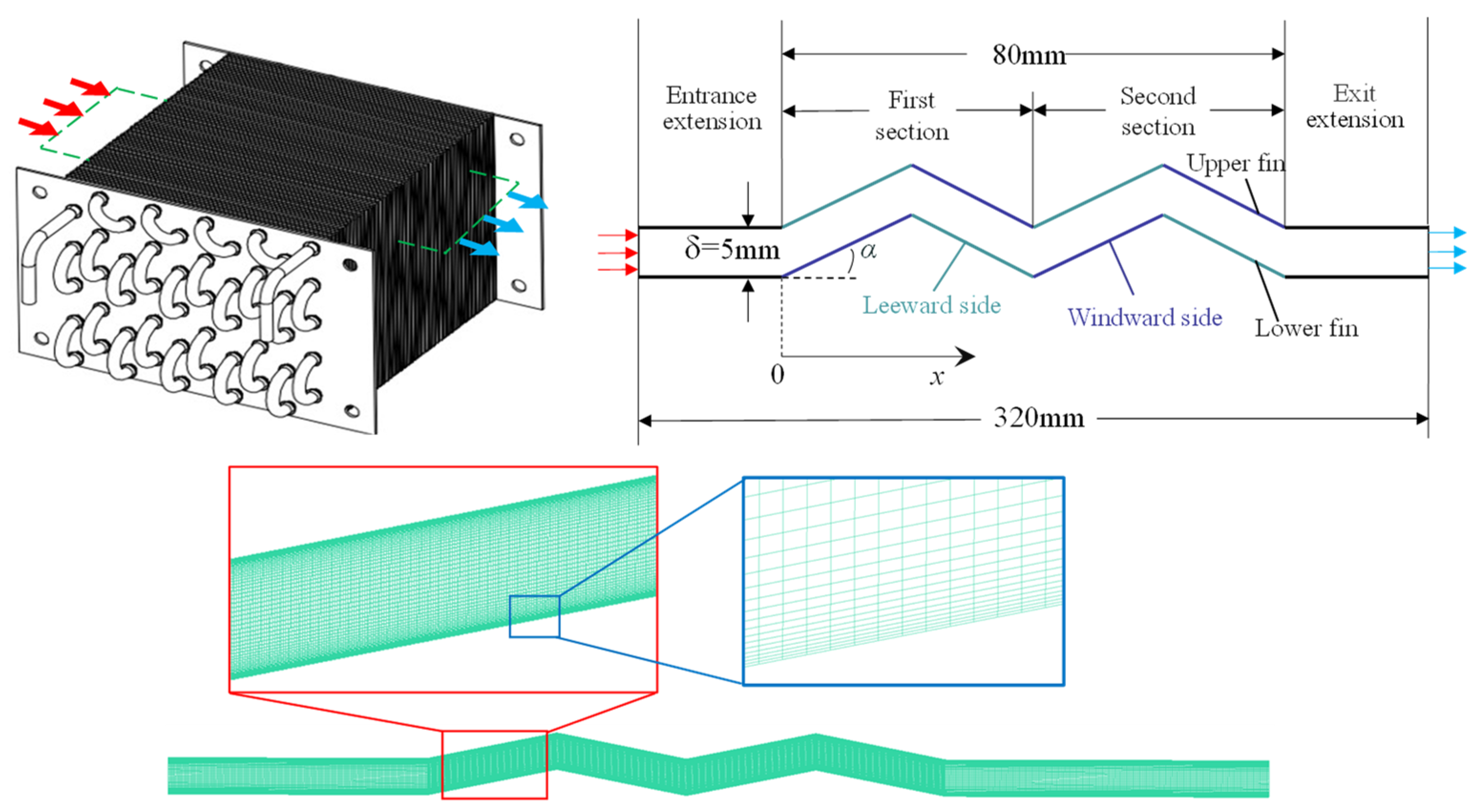
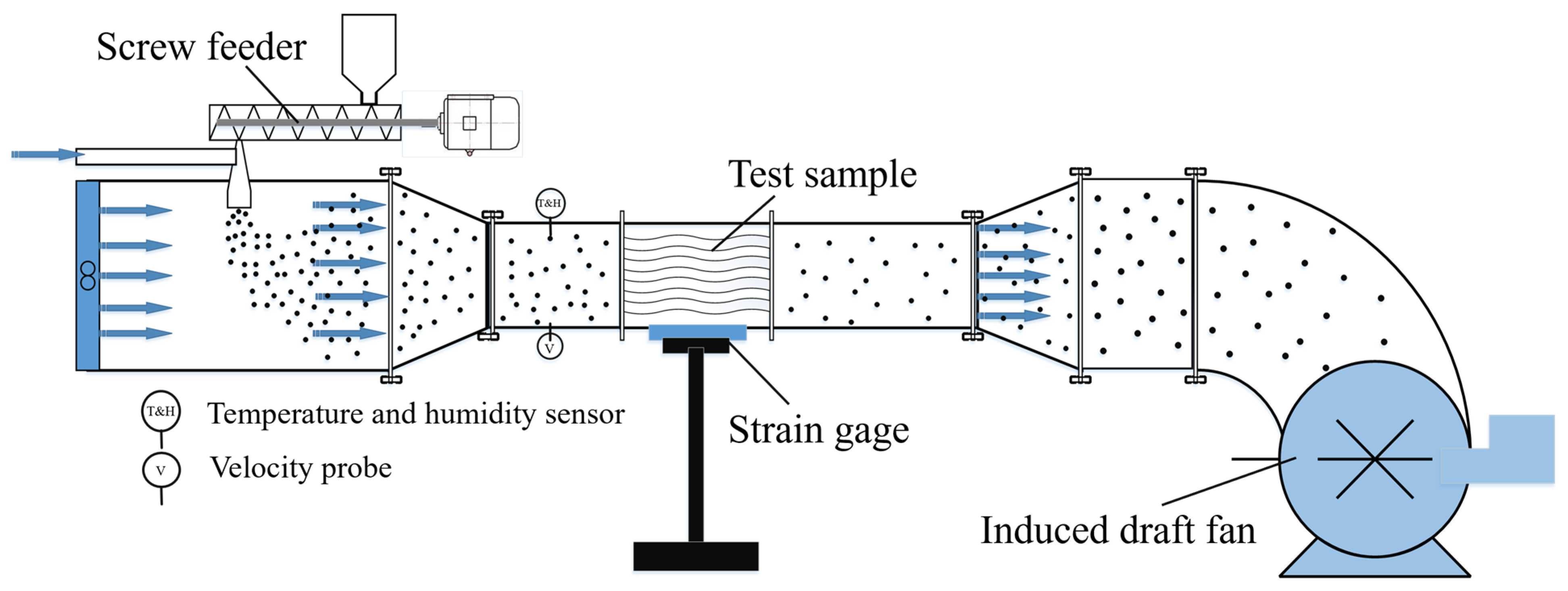
2.4. Physical Model and Boundary Conditions
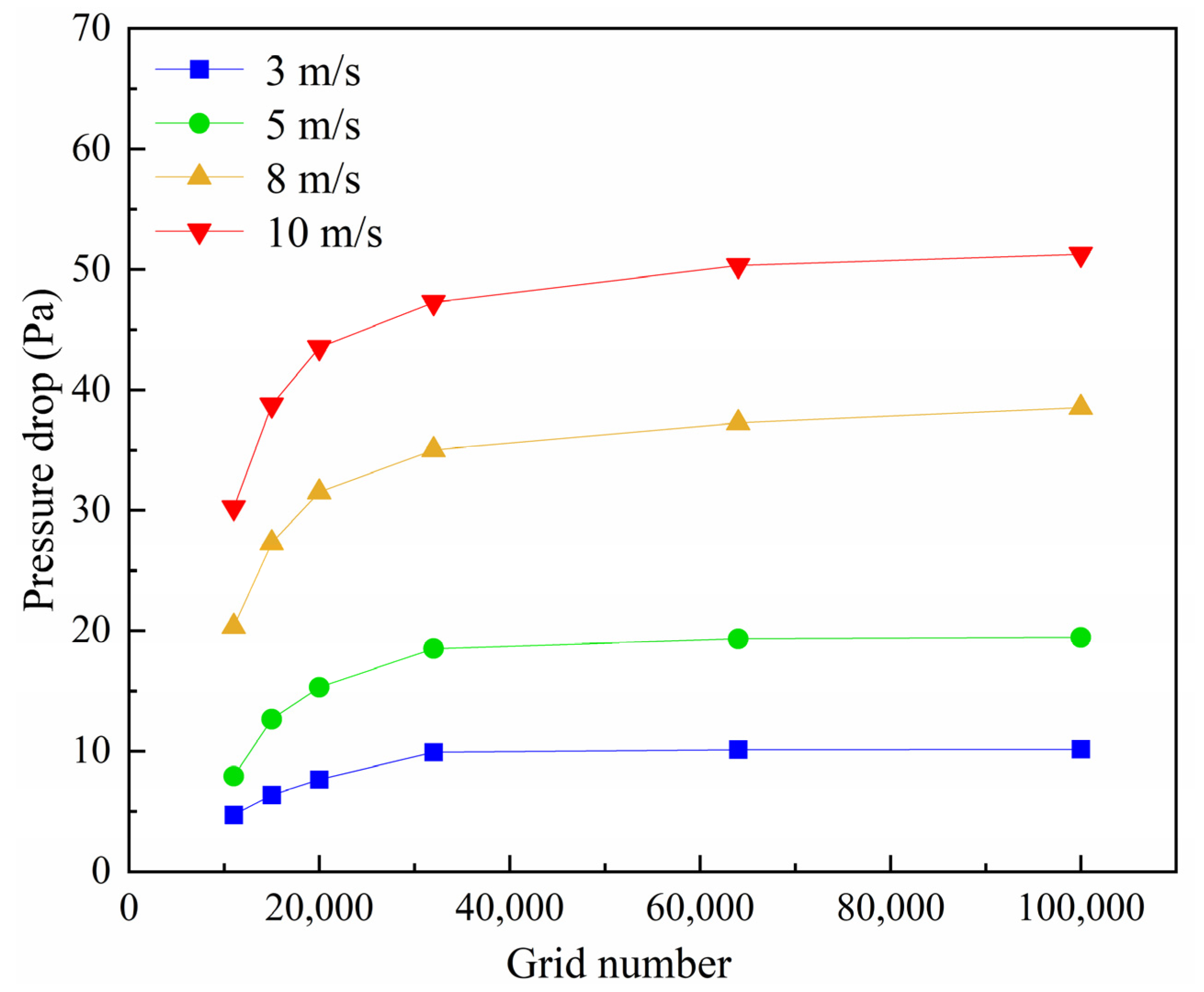
2.5. Grid Independence Verification
2.6. Characteristic Parameter
- (1)
- Collision rate
- (2)
- Deposition rate
- (3)
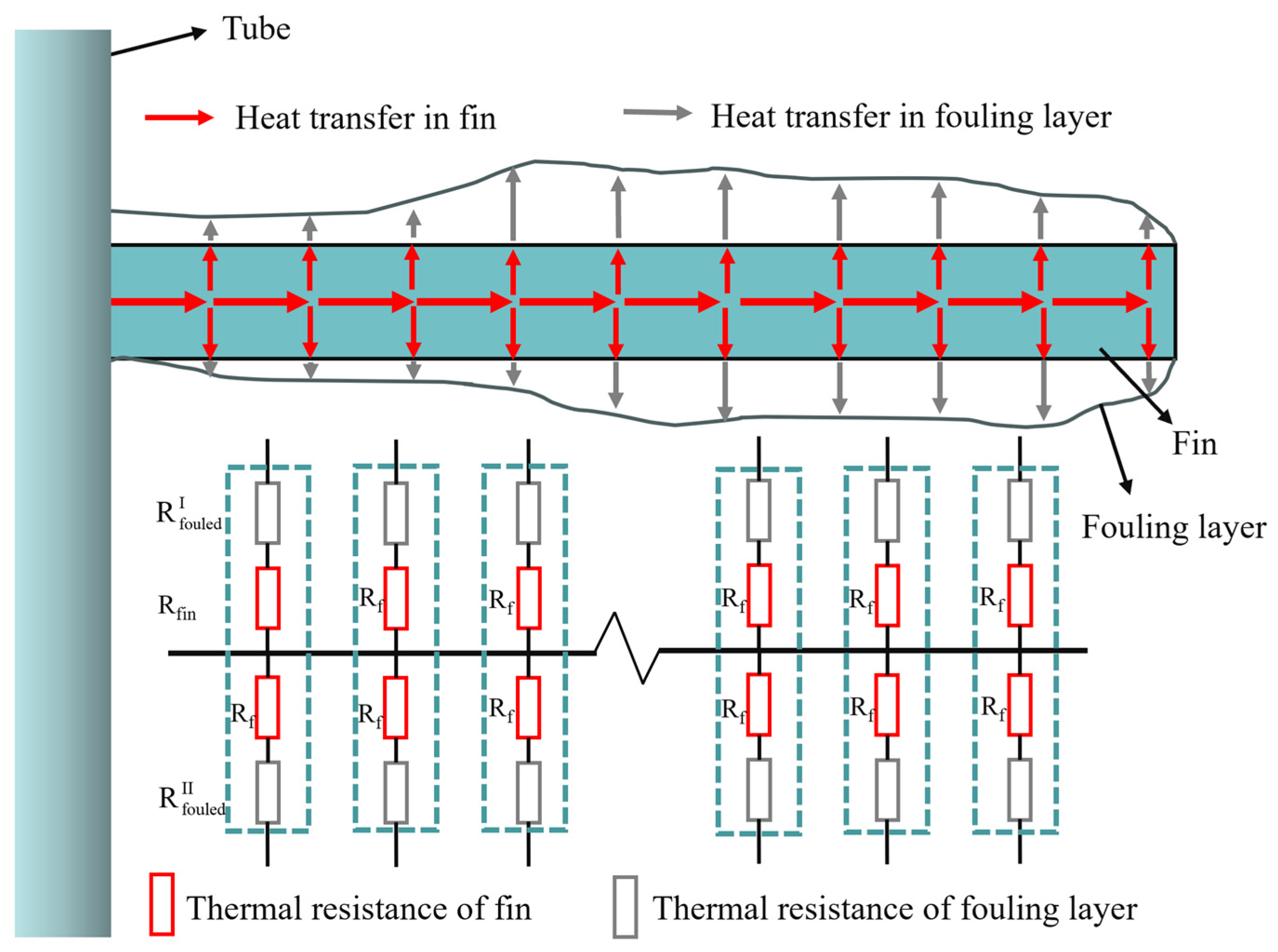
- Improved thermal resistance calculation
- (4)
- Efficiency of fin
3. Experimental Verification and Uncertainties
4. Results and Discussion
4.1. Experimental Verification Results
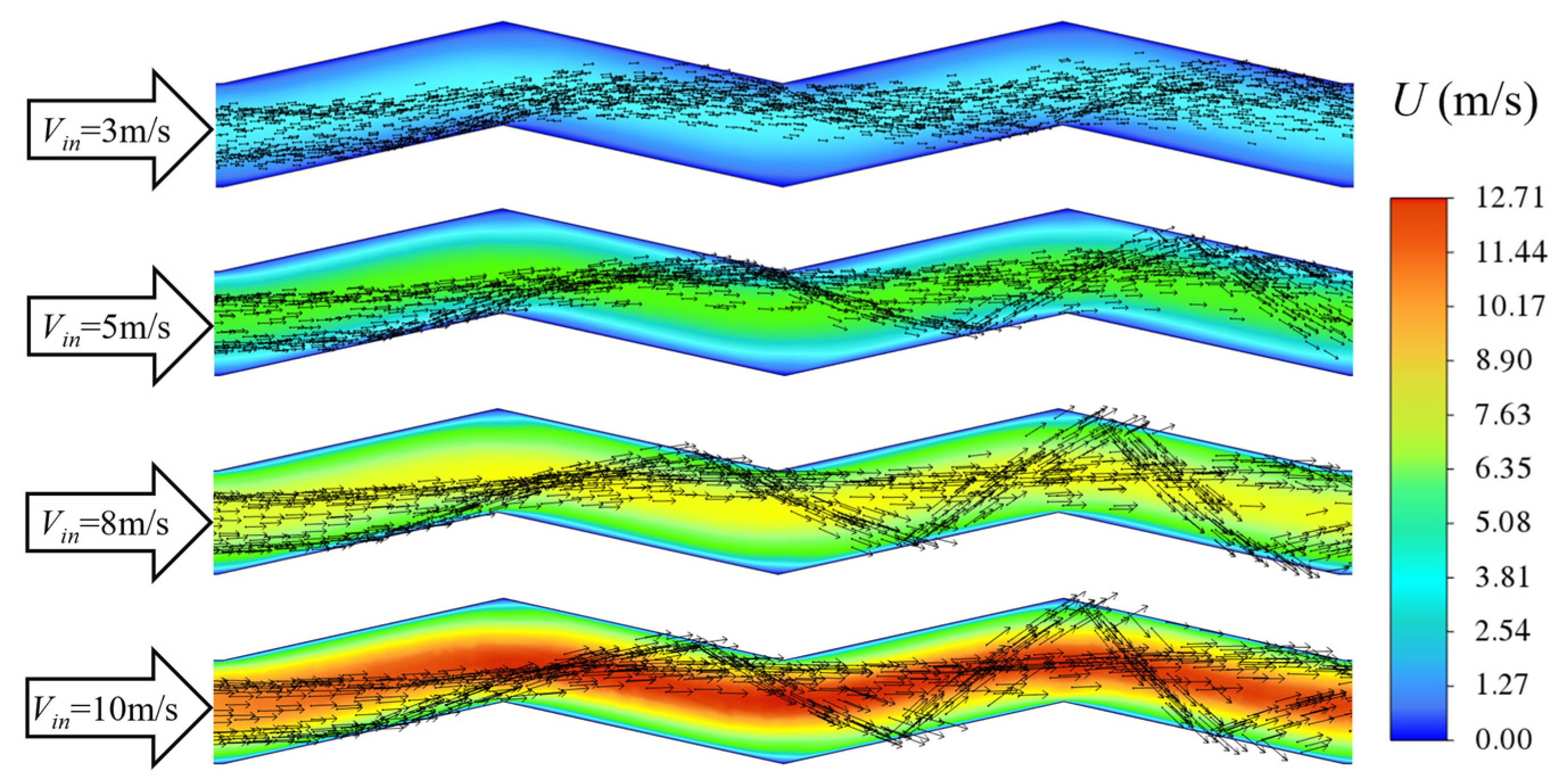
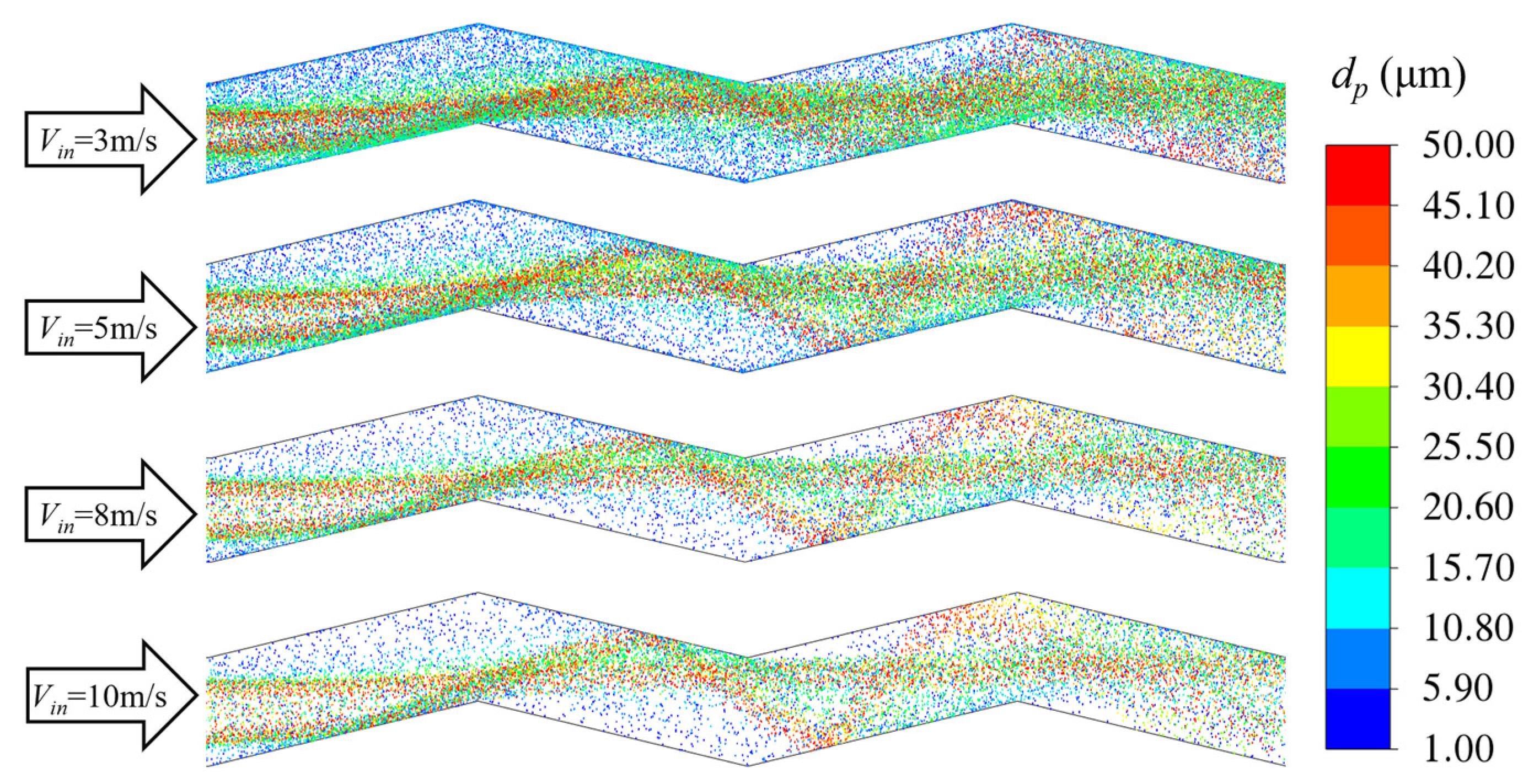
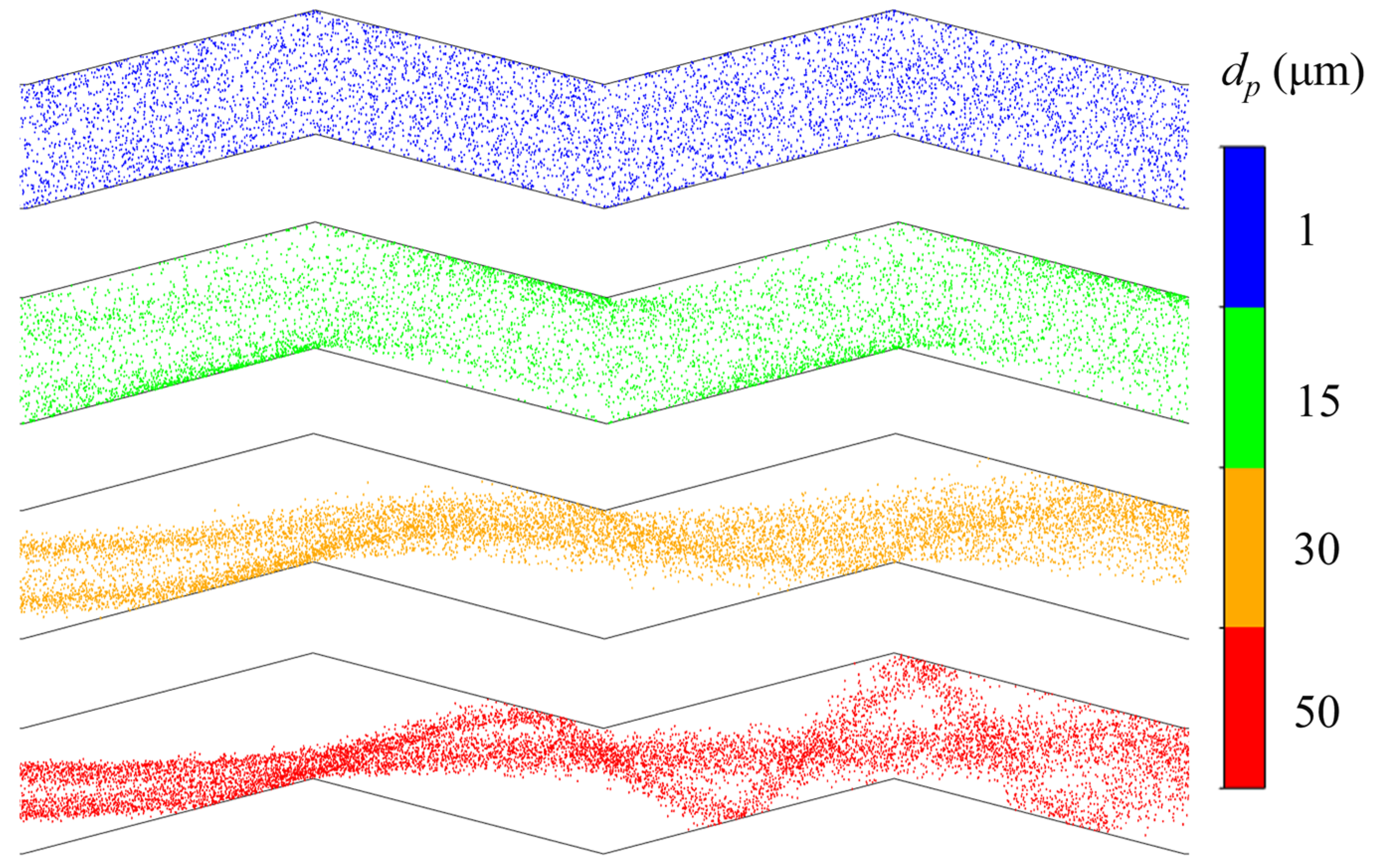
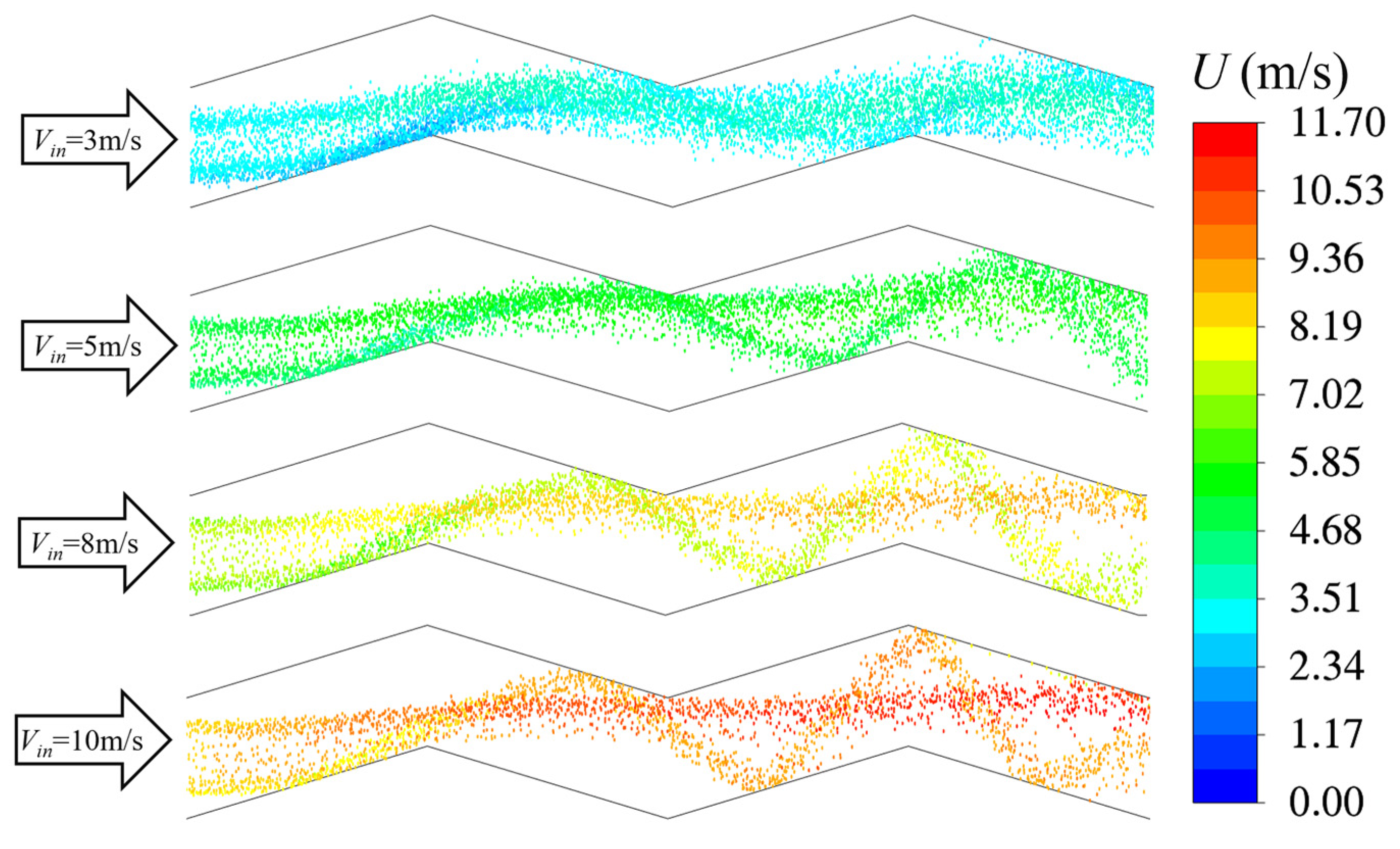
4.2. The Flow Characteristics and Particle Field Distribution
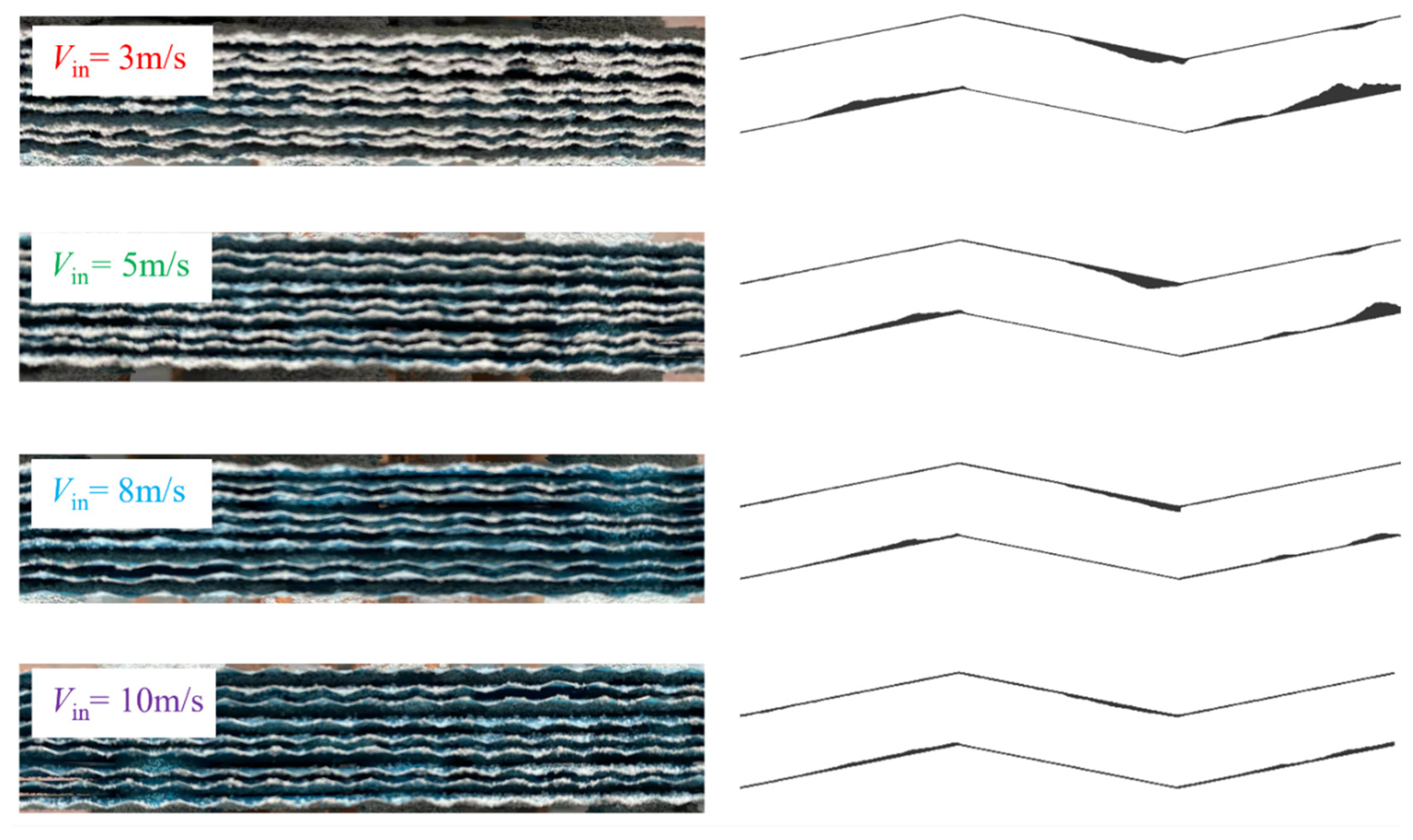
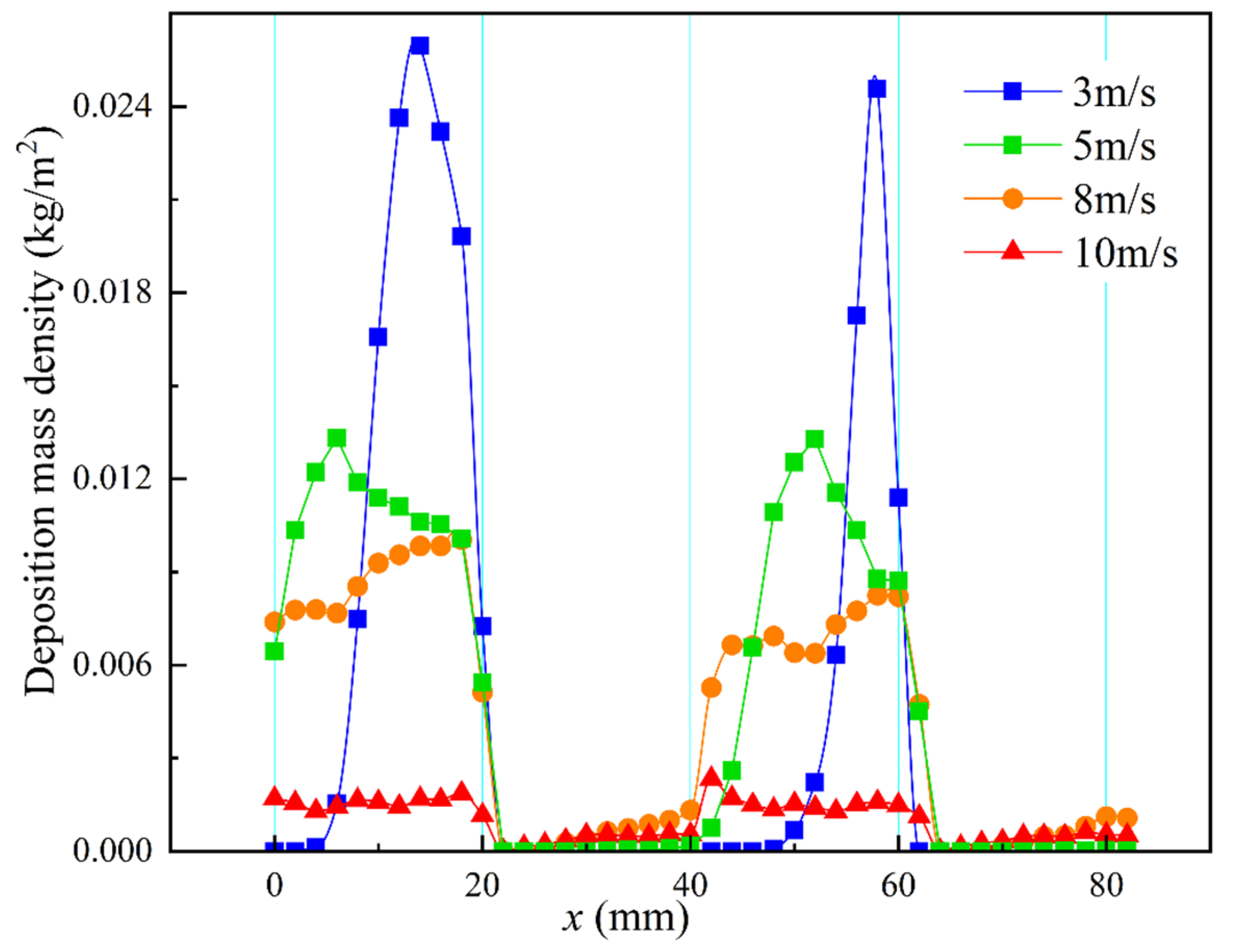
4.3. The Effect of Flow Velocity on Deposition
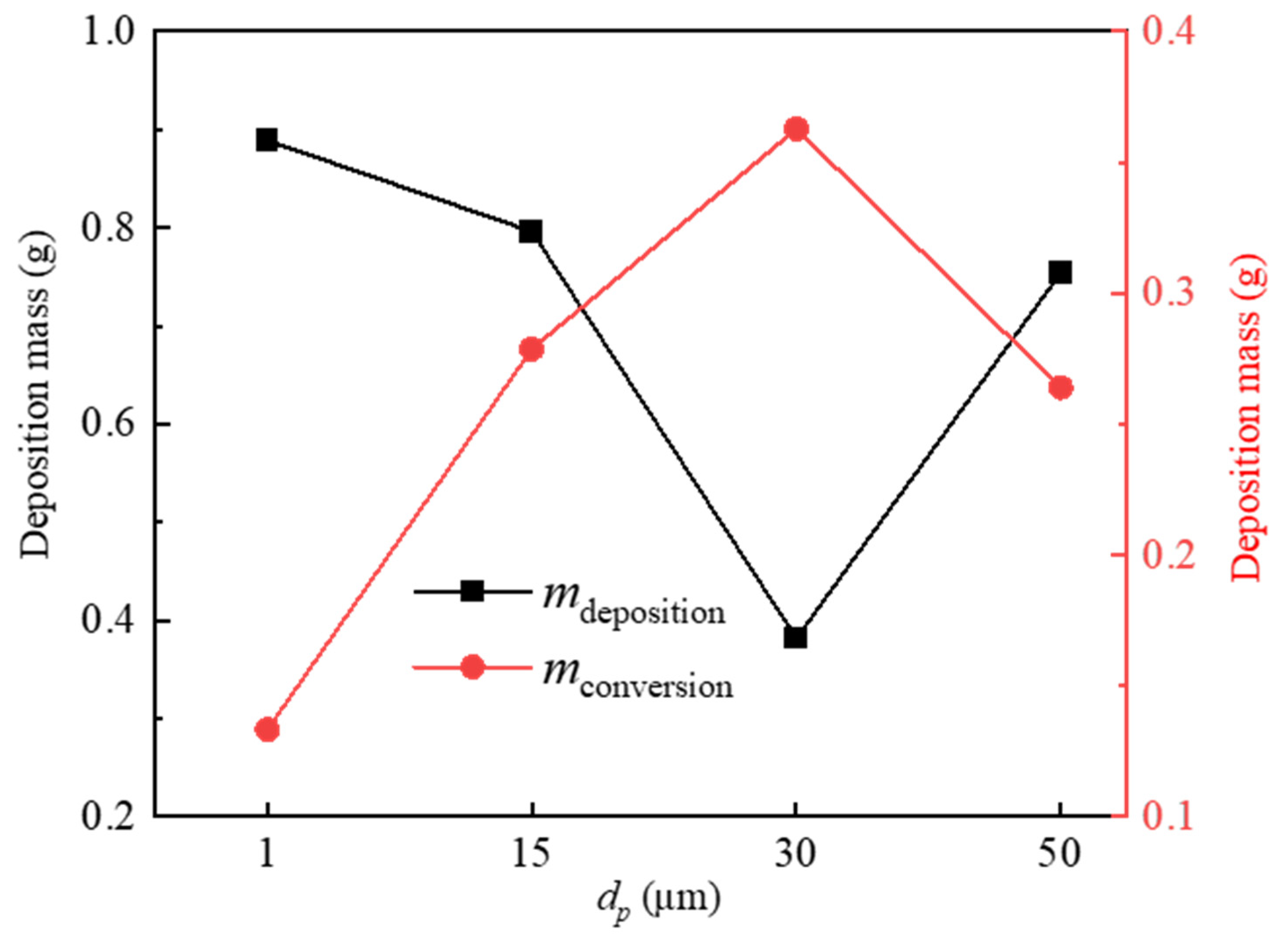
4.4. The Effect of Particle Size on Deposition
4.5. Deposition and Thermal Resistance Analysis of Multiple Particle Diameters on Fins
5. Conclusions
- (1)
- Random function is used to improve the existing fly ash particle deposition model. The improved model’s simulation results are compared to relevant experimental data, verifying the model’s accuracy.
- (2)
- The flow field between fins and the motion of particles is analyzed. The distribution of the flow field is similar, with zones of higher velocities distributed at the center of the flow channel; small particles fill the entire flow channel, while large particles are concentrated and distributed; and the collision rate and deposition rate increase with the increase of particle size.
- (3)
- For 30 μm particles, as the velocity increases, the deposition on the fins becomes less and more uniform; at 3 m/s, small particles deposit uniformly, while large particles deposit in a concentrated way to form peaks. When a certain particle size is introduced separately, there is a situation where small particles deposit more. When converted to all particle sizes, the situation flips.
- (4)
- For multi-particles, the deposition positions of the upper and lower fins are staggered. The deposition on the windward side is obviously more than that on the leeward side. With the increase in flow velocity, the thickness of the deposition layer decreases, and the maximum value of the deposition mass gradually decreases. Furthermore, the mass distribution curve changes from unimodal to bimodal.
- (5)
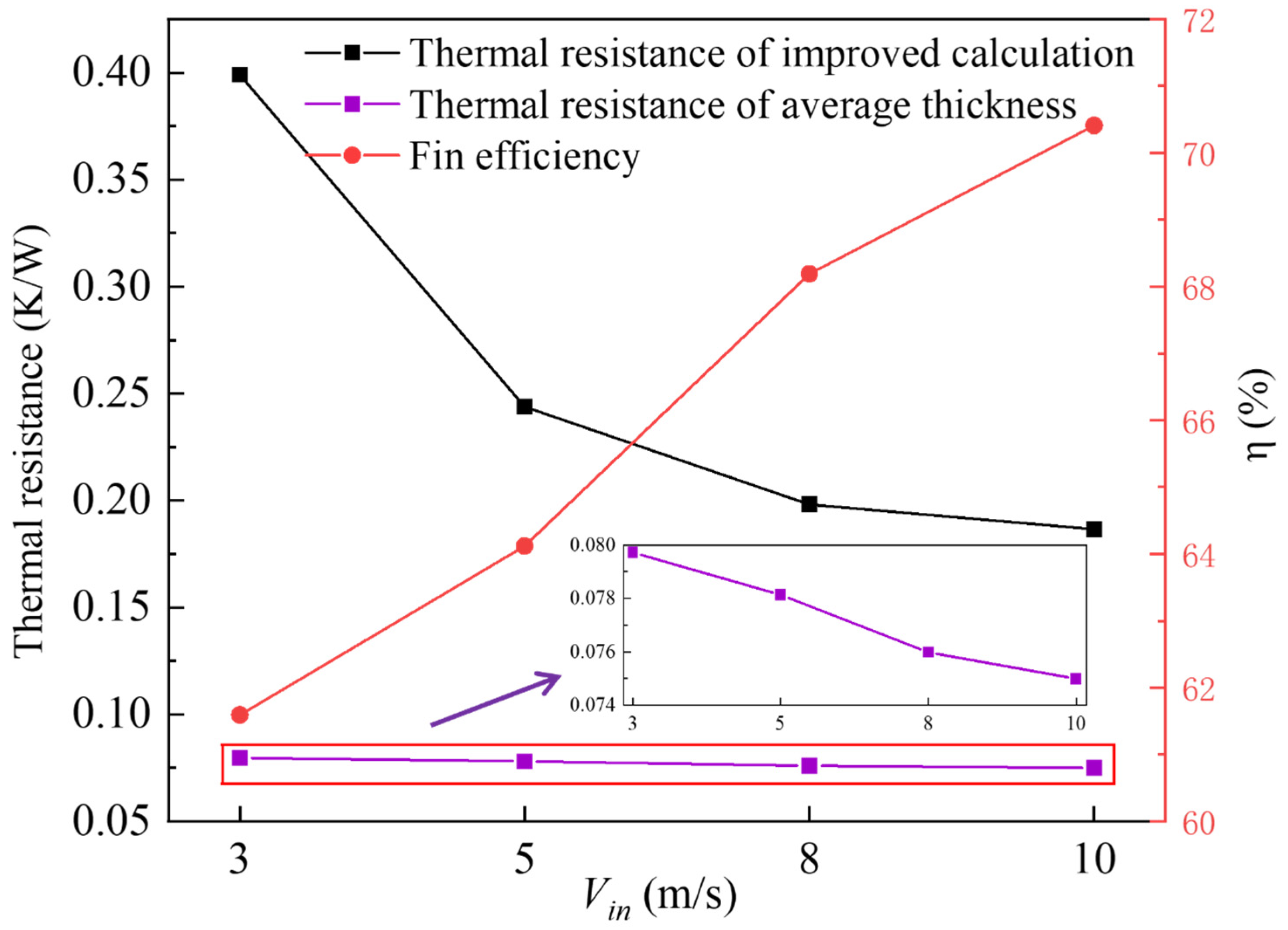
- The thermal resistance of the average and improved methods and efficiency were calculated. Due to the efficiency being calculated with average thickness, the trend is similar to that of average thickness thermal resistance. A comparison was made between the average thickness and the improved method of thermal resistance, and it was found that the improved method better reflects the overall impact of uneven thermal resistance.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vosough, A.; Assari, M.R.; Peyghambarzadeh, S.M.; Azizi, S. Influence of fluid flow rate on the fouling resistance of calcium sulfate aqueous solution in subcooled flow boiling condition. Int. J. Therm. Sci. 2020, 154, 106397. [Google Scholar] [CrossRef]
- Ahn, Y.; Cheong, S.; Jung, Y.; Lee, J. An Experimental Study of the Air-Side Particulate Fouling in Fin-and-Tube Heat Exchangers of Air Conditioners. Int. Refrig. Air Cond. Conf. 2003, 20, 873–877. [Google Scholar] [CrossRef][Green Version]
- Kaiser, S.; Antonijevic, D.; Tsotsas, E. Formation of fouling layers on a heat exchanger element exposed to warm, humid and solid loaded air streams. Exp. Therm. Fluid Sci. 2002, 26, 291–297. [Google Scholar] [CrossRef]
- Soltani, M.; Ahmadi, G. On particle adhesion and removal mechanisms in turbulent flows. J. Adhes. Sci. Technol. 1994, 8, 763–785. [Google Scholar] [CrossRef]
- Brach, R.M.; Dunn, P.F. A Mathematical Model of the Impact and Adhesion of Microsphers. Aerosol Sci. Technol. 1992, 16, 51–64. [Google Scholar] [CrossRef]
- Konstandopoulos, A.G. Particle sticking/rebound criteria at oblique impact. J. Aerosol Sci. 2006, 37, 292–305. [Google Scholar] [CrossRef]
- El-Batsh, H. Modeling Particle Deposition on Compressor and Turbine Blade Surfaces. Ph.D. Thesis, Vienna University of Technology, Vienna, Austria, September 2001. [Google Scholar]
- Herterich, J.G.; Griffiths, I.M. A mathematical model of the erosion process in a channel bend. Tribol. Int. 2021, 163, 107175. [Google Scholar] [CrossRef]
- Bohnet, M. Fouling of heat transfer surfaces. Chem. Eng. Technol.-CET 1987, 10, 113–125. [Google Scholar] [CrossRef]
- Paz, C.; Suárez, E.; Eirís, A.; Porteiro, J. Development of a Predictive CFD Fouling Model for Diesel Engine Exhaust Gas Systems. Heat Transf. Eng. 2013, 34, 674–682. [Google Scholar] [CrossRef]
- Li, J.; Xu, Z.; Han, Z. A practical Eulerian approach for predicting the deposition characteristics of fly ash particles on the outer surface of tubes. Int. J. Heat Mass Transf. 2022, 189, 122654. [Google Scholar] [CrossRef]
- Jiang, H.; Lu, L.; Sun, K. Simulation of particle deposition in ventilation duct with a particle–wall impact model. Build. Environ. 2010, 45, 1184–1191. [Google Scholar] [CrossRef]
- Pan, Y.; Si, F.; Xu, Z.; Romero, C.E. An integrated theoretical fouling model for convective heating surfaces in coal-fired boilers. Powder Technol. 2011, 210, 150–156. [Google Scholar] [CrossRef]
- Wang, Y.C.; Tang, G.H. Numerical investigation on the coupling of ash deposition and acid vapor condensation on the H-type fin tube bank. Appl. Therm. Eng. 2018, 139, 524–534. [Google Scholar] [CrossRef]
- Zhang, Y.; Du, X.; Yue, M.; Yan, M.; Shi, Y. Heat transfer and ash deposition performance of heat exchange surface in waste incineration flue gas. Int. J. Heat Mass Transf. 2020, 155, 119691. [Google Scholar] [CrossRef]
- Zhan, F.; Ding, G.; Zhuang, D. Numerical model of particle deposition on wet fin surfaces of heat exchanger under dehumidifying conditions. Int. J. Heat Mass Transf. 2020, 149, 119258. [Google Scholar] [CrossRef]
- Liu, P.; Liu, W.; Gong, K.; Han, C.; Zhang, H.; Sui, Z.; Hu, R. Numerical Study on Particulate Fouling Characteristics of Flue with a Particulate Fouling Model Considering Deposition and Removal Mechanisms. Energies 2022, 15, 8708. [Google Scholar] [CrossRef]
- Han, Z.; Yang, X.; Li, J.; Xu, Z. A waste heat exchanger fly ash particle deposition model with dynamic mesh and random function method. Case Stud. Therm. Eng. 2022, 40, 102502. [Google Scholar] [CrossRef]
- García Pérez, M.; Vakkilainen, E.; Hyppänen, T. 2D dynamic mesh model for deposit shape prediction in boiler banks of recovery boilers with different tube spacing arrangements. Fuel 2015, 158, 139–151. [Google Scholar] [CrossRef]
- Xu, Z.; Li, J.; Han, Z.; Yang, X. Investigation of deposition characteristics on tube-bundle heat exchanger with an improved ash particle model. Powder Technol. 2023, 413, 118027. [Google Scholar] [CrossRef]
- Guo, Z.; Li, N.; Klemeš, J.J.; Wang, Q.; Zeng, M. Mechanisms and strategies for ash deposition reduction in flue gas heat exchanger. Clean Technol. Environ. Policy 2021, 24, 77–93. [Google Scholar] [CrossRef]
- Tang, S.-Z.; He, Y.-L.; Wang, F.-L.; Tao, Y.-B. Parametric study on fouling mechanism and heat transfer characteristics of tube bundle heat exchangers for reducing fouling considering the deposition and removal mechanisms. Fuel 2018, 211, 301–311. [Google Scholar] [CrossRef]
- Zhang, F.; Liu, Z.; Liu, Z.; Liu, Y. Experimental Study of Particle Deposition on Surface at Different Mainstream Velocity and Temperature. Energies 2019, 12, 747. [Google Scholar] [CrossRef]
- Bell, I.H.; Groll, E.A. Air-side particulate fouling of microchannel heat exchangers: Experimental comparison of air-side pressure drop and heat transfer with plate-fin heat exchanger. Appl. Therm. Eng. 2011, 31, 742–749. [Google Scholar] [CrossRef]
- Chen, X.; Ji, X.; Feng, J.; Heng, L.; Zhao, L. Laboratory Study on Adhesive Ash Deposition Characteristics of Ammonium Bisulfate in Conditions Simulating an Air Preheater for Hard Coal Combustion. Energies 2023, 16, 6513. [Google Scholar] [CrossRef]
- Mason, D.; Douch, N.; Heikal, M. Air Side Fouling of Compact Heat Exchangers. In Proceedings of the 2nd IMechE Automobile Division Southern Centre Conference on Total Vehicle Technology, Brighton, UK, 11–12 November 2002; pp. 131–144. [Google Scholar]
- Nagendra, K.; Tafti, D.K.; Viswanathan, A.K. Modeling of soot deposition in wavy-fin exhaust gas recirculator coolers. Int. J. Heat Mass Transf. 2011, 54, 1671–1681. [Google Scholar] [CrossRef]
- Han, Z.; Xu, Z.; Yu, X.; Sun, A.; Li, Y. Numerical simulation of ash particles deposition in rectangular heat exchange channel. Int. J. Heat Mass Transf. 2019, 136, 767–776. [Google Scholar] [CrossRef]
- Välikangas, T.; Hærvig, J.; Kuuluvainen, H.; Dal Maso, M.; Peltonen, P.; Vuorinen, V. Deposition of dry particles on a fin-and-tube heat exchanger by a coupled soft-sphere DEM and CFD. Int. J. Heat Mass Transf. 2020, 149, 119046. [Google Scholar] [CrossRef]
- Lu, H.; Wang, Y.; Li, H.; Zhao, W. Numerical Simulation of Turbulent Structure and Particle Deposition in a Three-Dimensional Heat Transfer Pipe with Corrugation. Energies 2024, 17, 321. [Google Scholar] [CrossRef]
- Aziz, A.; Luardini, V. Analytical and numerical modeling of steady periodic heat transfer in extended surfaces. Comput. Mech. 1994, 14, 387–410. [Google Scholar] [CrossRef]
- Tseng, J.-K.; Shih, C.-L.; Wu, W.-J. Analysis of transient heat transfer in straight fins of various shapes with its base subjected to a decayed exponential function of time in heat flux. Comput. Struct. 1993, 47, 289–297. [Google Scholar]
- Atouei, S.A.; Hosseinzadeh, K.; Hatami, M.; Ghasemi, S.E.; Sahebi, S.A.R.; Ganji, D.D. Heat transfer study on convective–radiative semi-spherical fins with temperature-dependent properties and heat generation using efficient computational methods. Appl. Therm. Eng. 2015, 89, 299–305. [Google Scholar] [CrossRef]
- Zhan, F.; Zhuang, D.; Ding, G. An analytical model for predicting the particle-deposited fin efficiency. Int. J. Refrig. 2018, 92, 235–245. [Google Scholar] [CrossRef]
- Zheng, Z.; Yang, W.; Cai, Y.; Wang, Q.; Zeng, G. Dynamic simulation on ash deposition and heat transfer behavior on a staggered tube bundle under high-temperature conditions. Energy 2020, 190, 116390. [Google Scholar] [CrossRef]
- Ai, W.; Kuhlman, J.M. Simulation of Coal Ash Particle Deposition Experiments. Energy Fuels 2011, 25, 708–718. [Google Scholar] [CrossRef]
- Zhou, H.; Zhang, K.; Li, Y.; Zhang, J.; Zhou, M. Simulation of ash deposition in different furnace temperature with a 2D dynamic mesh model. J. Energy Inst. 2019, 92, 1743–1756. [Google Scholar] [CrossRef]
- Hong, W.; Wang, B.; Zheng, J. Numerical study on the influence of fine particle deposition characteristics on wall roughness. Powder Technol. 2020, 360, 120–128. [Google Scholar] [CrossRef]
- Tang, S.-Z.; Wang, F.-L.; Ren, Q.; He, Y.-L. Fouling characteristics analysis and morphology prediction of heat exchangers with a particulate fouling model considering deposition and removal mechanisms. Fuel 2017, 203, 725–738. [Google Scholar] [CrossRef]
- Lu, H.; Quan, Y. A CFD study of particle deposition in three-dimensional heat exchange channel based on an improved deposition model. Int. J. Heat Mass Transf. 2021, 178, 121633. [Google Scholar] [CrossRef]
- Yuan, S.; Fan, Y.; Chen, B.; Li, J.; Gao, L.; Zhang, S. Forming and stripping of the wall film and the influence on gas–liquid separation. Asia-Pac. J. Chem. Eng. 2020, 15, e2447. [Google Scholar] [CrossRef]
- Fu, L.; Liu, P.; Li, G. Numerical investigation on ash fouling characteristics of flue gas heat exchanger. Appl. Therm. Eng. 2017, 123, 891–900. [Google Scholar] [CrossRef]
- Wang, F.-L.; He, Y.-L.; Tong, Z.-X.; Tang, S.-Z. Real-time fouling characteristics of a typical heat exchanger used in the waste heat recovery systems. Int. J. Heat Mass Transf. 2017, 104, 774–786. [Google Scholar] [CrossRef]
- Wang, F.-L.; He, Y.-L.; Tang, S.-Z.; Tong, Z.-X. Parameter study on the fouling characteristics of the H-type finned tube heat exchangers. Int. J. Heat Mass Transf. 2017, 112, 367–378. [Google Scholar] [CrossRef]







| Property | Value |
|---|---|
| Density (kg/m3) | 2250 |
| Diameter (μm) | 1~75 |
| Equivalent Young’s modulus (Pa) | 3.8 × 1010 |
| Surface energy (J/m2) | 0.3 |
| Thermal conductivity (W/(m·K)) | 1.4 |
| Parameter | Instrument | Range | Uncertainty |
|---|---|---|---|
| Air velocity (Vin) | Handheld anemometer HC-001 | 0.1–30 m/s | ±0.001 m/s |
| Air-side pressure drop (ΔP) | Handheld pressure gauge AS510 | 0–100 hpa | ±0.003 hpa |
| Air relative humidity (RH) | Temperature and humidity sensor | 0–100% | ±1.4% |
| Air temperature (T) | Temperature and humidity sensor | −50–100 °C | ±0.1 °C |
| Rotational speed of screw spindle (r) | Screw feeder 5IK120RGN | 10–100 r/min | ±1 r/min |
| Weight of test sample (m) | Analytical balance ES-S | 0–220 g | ±0.l mg |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, W.; Zhang, S.; Yang, Q.; Zhang, L.; Ge, C.; Wang, A.; Bu, S.; Lv, W.; Zhang, L. Deposition Distribution and Thermal Resistance Analysis of Fins in Heat Exchangers. Energies 2024, 17, 3952. https://doi.org/10.3390/en17163952
Xu W, Zhang S, Yang Q, Zhang L, Ge C, Wang A, Bu S, Lv W, Zhang L. Deposition Distribution and Thermal Resistance Analysis of Fins in Heat Exchangers. Energies. 2024; 17(16):3952. https://doi.org/10.3390/en17163952
Chicago/Turabian StyleXu, Weigang, Shijian Zhang, Quan Yang, Lei Zhang, Chongsheng Ge, Ao Wang, Shi Bu, Weibing Lv, and Lin Zhang. 2024. "Deposition Distribution and Thermal Resistance Analysis of Fins in Heat Exchangers" Energies 17, no. 16: 3952. https://doi.org/10.3390/en17163952
APA StyleXu, W., Zhang, S., Yang, Q., Zhang, L., Ge, C., Wang, A., Bu, S., Lv, W., & Zhang, L. (2024). Deposition Distribution and Thermal Resistance Analysis of Fins in Heat Exchangers. Energies, 17(16), 3952. https://doi.org/10.3390/en17163952





