Review of Geomagnetically Induced Current Proxies in Mid-Latitude European Countries
Abstract
1. Introduction
2. Methodology
2.1. Proxies of the Geomagnetically Induced Current Intensity
2.2. E-Field Estimation
2.3. GIC Modeling
3. Issues Connected to the Geomagnetically Induced Currents in Mid-Latitude European Countries
3.1. Greece
3.2. Spain
3.3. Italy
3.4. Czech Republic
3.5. Slovakia
3.6. Austria
3.7. Poland
4. Discussion
5. Summary
- 1.
- Mid-latitude countries can be under geomagnetically induced currents’ impact during intense geomagnetic storms.
- 2.
- Modeling the evolution of coronal mass ejections, the geoelectric field, and geomagnetically induced currents needs to be effective and practically available in real-time.
- 3.
- In order to properly validate the models of geomagnetically induced currents and the geoelectric field, there is a requirement for close cooperation between transmission line operators and researchers involved in modeling and forecasting.
- 4.
- To precisely model geomagnetically induced currents, we need information on the power grid topology. Thus, close cooperation between the scientific community and national operators is needed.
- 5.
- There is a need for continuous monitoring of the Earth’s vicinity to build a well-funded system awareness, as the Space Weather Service Network does in the frame of the European Space Agency or the Space Weather Prediction Center of the National Oceanic and Atmospheric Administration.
- 6.
- The development of the research on the impact of space weather phenomena on the functioning of power grids in mid-latitude European countries can be highly accelerated due to the creation within the structures of the European Union of an authority responsible for creating a framework for collecting, unifying, and processing data on the instability of transmission lines.
- 7.
- The absence of regular and extensive geomagnetically induced current measurements in mid-latitude European countries must be resolved.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| APG | Austrian Power Grid AG |
| CME | Coronal mass ejection |
| EGF | Electrical grid failure |
| GIC | Geomagnetically induced current |
| HAC | Hierarchical agglomerative clustering |
| LCi | Local Current index |
| LDi | Local Disturbance index |
| LSTM | Long short-term memory network |
| PCA | Principal component analysis |
| P/S | Pipe-to-soil |
| SOM | Self-organizing maps |
| SSC | Sudden storm commencement |
| SSN | Sunspot number |
| TF | Transformer failure |
References
- Di Fino, L.; Romoli, G.; Amantini, S.; Boretti, V.; Lunati, L.; Berucci, C.; Messi, R.; Rizzo, A.; Albicocco, P.; Donato, C.D.; et al. Radiation measurements in the International Space Station, Columbus module, in 2020–2022 with the LIDAL detector. Life Sci. Space Res. 2023, in press. [Google Scholar] [CrossRef]
- Di Fino, L.; Zaconte, V.; Stangalini, M.; Sparvoli, R.; Picozza, P.; Piazzesi1, R.; Narici1, L.; Larosa1, M.; Moro, D.D.; Casolino, M.; et al. Solar particle event detected by ALTEA on board the International Space Station. The March 7th, 2012 X5.4 flare. J. Space Weather Space Clim. 2014, 4, A19. [Google Scholar] [CrossRef]
- Fang, T.W.; Kubaryk, A.; Goldstein, D.; Li, Z.; Fuller-Rowell, T.; Millward, G.; Singer, H.J.; Steenburgh, R.; Westerman, S.; Babcock, E. Space Weather Environment During the SpaceX Starlink Satellite Loss in February 2022. Space Weather 2022, 20, 11. [Google Scholar] [CrossRef]
- Pesnell, D.W. Solar Cycle Predictions (Invited Review). Sol. Phys. 2012, 281, 507–532. [Google Scholar] [CrossRef]
- Meier, M.M.; Berger, T.; Jahn, T.; Matthiä, D.; Plettenberg, M.C.; Scheibinger, M.; Schennetten, K.; Wirtz, M. Impact of the South Atlantic Anomaly on radiation exposure at flight altitudes during solar minimum. Sci. Rep. 2023, 13, 9348. [Google Scholar] [CrossRef]
- Mishev, A.; Usoskin, I. Numerical model for computation of effective and ambient dose equivalent at flight altitudes. Application for dose assessment during GLEs. J. Space Weather Space Clim. 2015, 5, A10. [Google Scholar] [CrossRef]
- Fagundes, P.R.; Cardoso, F.A.; Fejer, B.G.; Venkatesh, K.; Ribeiro, B.A.G.; Pillat, V.G. Positive and negative GPS-TEC ionospheric storm effects during the extreme space weather event of March 2015 over the Brazilian sector. J. Geophys. Res. Space Phys. 2016, 121, 5613–5625. [Google Scholar] [CrossRef]
- Schrijver, C.J.; Kauristie, K.; Aylward, A.D.; Denardini, C.M.; Gibson, S.E.; Glover, A.; Gopalswamy, N.; Grande, M.; Hapgood, M.; Heynderickx, D.; et al. Understanding space weather to shield society: A global road map for 2015–2025 commissioned by COSPAR and ILWS. Adv. Space Res. 2015, 55, 2745–2807. [Google Scholar] [CrossRef]
- Sloan, T.; Wolfendale, A.W. Cosmic rays and climate change over the past 1000 million years. New Astron. 2013, 25, 45–49. [Google Scholar] [CrossRef]
- Mavromichalaki, H.; Papailiou, M.; Dimitrova, S.; Babayev, E.S.; Loucas, P. Space weather hazards and their impact on human cardio-health state parameters on Earth. Nat. Hazards 2012, 64, 1447–1459. [Google Scholar] [CrossRef]
- Alania, M.V.; Gil, A.; Modzelewska, R. On statistical relationship of solar, geomagnetic and human activities. Adv. Space Res. 2004, 34, 1602–1606. [Google Scholar] [CrossRef] [PubMed]
- Wang, C.; Rosen, I.G.; Tsurutani, B.T.; Verkhoglyadova, O.P.; Meng, X.; Mannucci, A.J. Statistical characterization of ionosphere anomalies and their relationship to space weather events. J. Space Weather Space Clim. 2016, 6, A5. [Google Scholar] [CrossRef]
- Eastwood, J.P.; Biffis, E.; Hapgood, M.A.; Green, L.; Bisi, M.M.; Bentley, R.D.; Wicks, R.; McKinnell, L.A.; Gibbs, M.; Burnett, C. The Economic Impact of Space Weather: Where Do We Stand? Risk Anal. 2017, 37, 206–218. [Google Scholar] [CrossRef] [PubMed]
- Pulkkinen, A.; Viljanen, A.; Pajunpää, K.; Pirjola, R. Recordings and occurrence of geomagnetically induced currents in the Finnish natural gas pipeline network. J. Appl. Geophys. 2001, 48, 219–231. [Google Scholar] [CrossRef]
- Joo, B.S.; Woo, J.W.; Lee, J.H.; Jeong, I.; Ha, J.; Lee, S.H.; Kim, S. Assessment of the Impact of Geomagnetic Disturbances on Korean Electric Power Systems. Energies 2018, 11, 1920. [Google Scholar] [CrossRef]
- Gil, A.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Wawrzynczak, A.; Zakrzewska, S. Does time series analysis confirms the relationship between space weather effects and the failures of electrical grids in South Poland? J. Math. Ind. 2019, 9, 7. [Google Scholar] [CrossRef]
- Lakhina, G.S.; Hajra, R.; Tsurutani, B.T. Encyclopedia of Solid Earth Geophysics; Springer International Publishing: Berlin/Heidelberg, Germany, 2021; pp. 523–527. [Google Scholar] [CrossRef]
- Pirjola, R. Review on The Calculation of Surface Electric an Magnetic Fields and of Geomagnetically Induced Currents in Ground-Based Technological Systems. Surv. Geophys. 2002, 23, 71–90. [Google Scholar] [CrossRef]
- Kelbert, A. The Role of Global/Regional Earth Conductivity Models in Natural Geomagnetic Hazard Mitigation. Surv. Geophys. 2019, 41, 115–166. [Google Scholar] [CrossRef]
- Tsurutani, B.T.; Hajra, R. The Interplanetary and Magnetospheric causes of Geomagnetically Induced Currents (GICs)>10 A in the Mantsala Finland Pipeline: 1999 through 2019. J. Space Weather Space Clim. 2021, 11, 32. [Google Scholar] [CrossRef]
- Oliveira, D.M.; Arel, D.; Raeder, J.; Zesta, E.; Ngwira, C.M.; Carter, B.A.; Yizengaw, E.; Halford, A.J.; Tsurutani, B.T.; Gjerloev, J.W. Geomagnetically induced currents caused by interplanetary shocks with different impact angles and speeds. Space Weather 2018, 16, 636–647. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Tsurutani, B.T. Criteria of interplanetary parameters causing intense magnetic storms (Dst < −100 nT). Planet. Space Sci. 1987, 35, 1101. [Google Scholar] [CrossRef]
- Gosling, J.T. Coronal mass ejections: The link between solar and geomagnetic activity. Phys. Fluids B 1993, 5, 2638–2645. [Google Scholar] [CrossRef]
- Tsurutani, B.T.; Gonzalez, W.D.; Kamide, Y. Magnetic storms. Surv. Geophys. 1997, 18, 363–383. [Google Scholar] [CrossRef]
- Heyns, M.J.; Lotz, S.I.; Gaunt, C.T. Geomagnetic pulsations driving geomagnetically induced currents. Space Weather 2021, 19, e2020SW002557. [Google Scholar] [CrossRef]
- Marshall, R.A.; Dalzell, M.; Waters, C.L.; Goldthorpe, P.; Smith, E.A. Geomagnetically induced currents in the New Zealand power network. Space Weather 2012, 10. [Google Scholar] [CrossRef]
- Barlow, W.H. On the Spontaneous Electrical Currents Observed in the Wires of the Electric Telegraph. Philos. Trans. R. Soc. Lond. Ser. I 1849, 139, 61–72. [Google Scholar]
- Burbank, J.E. Earth-currents and a proposed method for their investigation. Terr. Magn. Atmos. Electr. (J. Geophys. Res.) 1905, 10, 23. [Google Scholar] [CrossRef]
- Albertson, V.; Thorson, J.; Clayton, R.; Tripathy, S. Solar-Induced-Currents in Power Systems: Cause and Effects. IEEE Trans. Power Appar. Syst. 1973, 92, 471–477. [Google Scholar] [CrossRef]
- Zakharenkova, I.; Cherniak, I. Effects of storm-induced equatorial plasma bubbles on GPS-based kinematic positioning at equatorial and middle latitudes during the September 7–8, 2017, geomagnetic storm. GPS Solut. 2021, 25, 132. [Google Scholar] [CrossRef]
- Gopalswamy, N. History and development of coronal mass ejections as a key player in solar terrestrial relationship. Geosci. Lett. 2016, 3, 8. [Google Scholar] [CrossRef]
- Kataoka, R.; Asaoka, Y.; Torii, S.; Toshio, T.; Shunsuke, O.; Tadahisa, T.; Yuki, S.; Yosui, A.; Masaki, M. Relativistic electron precipitation at International Space Station: Space weather monitoring by Calorimetric Electron Telescope. Geophys. Res. Lett. 2016, 43, 4119–4125. [Google Scholar] [CrossRef]
- Bhaskar, A.; Vichare, G. Forecasting of SYMH and ASYH indices for geomagnetic storms of solar cycle 24 including St. Patrick’s day, 2015 storm using NARX neural network. J. Space Weather. Space Clim. 2019, 9, A12. [Google Scholar] [CrossRef]
- Gil, A.; Berendt-Marchel, M.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Tomasik, L.; Wawrzaszek, A.; Wawrzynczak, A. Evaluating the relationship between strong geomagnetic storms and electric grid failures in Poland using the geoelectric field as a GIC proxy. J. Space Weather Space Clim. 2021, 11, 30. [Google Scholar] [CrossRef]
- Bolduc, L. GIC observations and studies in the Hydro-Québec power system. J. Atmos.-Solar Terr. Phys. 2002, 64, 1793–1802. [Google Scholar] [CrossRef]
- Wik, M.; Viljanen, A.; Pirjola, R.; Pulkkinen, A.; Wintoft, P.; Lundstedt, H. Calculation of geomagnetically induced currents in the 400 kV power grid in southern Sweden. Space Weather 2008, 6, 7. [Google Scholar] [CrossRef]
- Carter, B.A.; Yizengaw, E.; Pradipta, R.; Weygand, J.M.; Piersanti, M.; Pulkkinen, A.; Moldwin, M.B.; Norman, R.; Zhang, K. Geomagnetically induced currents around the world during the 17 March 2015 storm. J. Geophys. Res. Space Phys. 2016, 121, 496–507. [Google Scholar] [CrossRef]
- Anuar, N.M.; Kasran, F.A.M.; Zatul, I.A.L.; Ab Rahim, S.A.E.; Manut, A.; Jusoh, M.H.; Hadi, N.A.; Yoshikawa, A. Estimation of Time Derivative of Horizontal Geomagnetic Component for GIC Assesment in Malaysia during Quiet Period. In Proceedings of the 8th International Conference on System Engineering and Technology (ICSET), Bandung, Indonesia, 15–16 October 2018; Institute of Electrical and Electronics Engineers Inc.: Bandung, Indonesia, 2018; pp. 118–122. [Google Scholar]
- Pulkkinen, A.; Lindahl, S.; Viljanen, A.; Pirjola, R. Geomagnetic storm of 29–31 October 2003: Geomagnetically induced currents and their relation to problems in the Swedish high-voltage power transmission system. Space Weather 2005, 3. [Google Scholar] [CrossRef]
- Zois, J.P. Solar activity and transformer failures in the Greek national electric grid. J. Space Weather Space Clim. 2013, 3, A32. [Google Scholar] [CrossRef]
- Cid, C.; Guerrero, A.; Saiz, E.; Halford, A.J.; Kellerman, A.C. Developing the LDi and LCi geomagnetic indices, an example of application of the AULs framework. Space Weather 2019, 18, e2019SW002171. [Google Scholar] [CrossRef]
- Tozzi, R.; De Michelis, P.; Coco, I.; Giannattasio, F. A preliminary risk assessment of geomagnetically induced currents over the Italian territory. Space Weather 2019, 17, 46–58. [Google Scholar] [CrossRef]
- Berrilli, F.; Bifaretti, S.; Bonaiuto, V.; Consolini, G.; Del Moro, D.; Orrù, L.; Santo, L.; Silletti, F.; Spena, A.; Terlizzi, C. A Statistical Approach to Analyze Possible Correlations Between Space Weather Events and Recorded Failures on the Italian Transmission Grid. In Proceedings of the 2022 AEIT International Annual Conference (AEIT), Rome, Italy, 3–5 October 2022; pp. 1–6. [Google Scholar]
- Hejda, P.; Bochníček, J. Geomagnetically induced pipe-to-soil voltages in the Czech oil pipelines during October–November 2003. Ann. Geophys. 2005, 23, 3089–3093. [Google Scholar] [CrossRef]
- Vybostokova, T.; Svanda, M. Statistical Analysis of the Correlation between Anomalies in the Czech Electric Power Grid and Geomagnetic Activity. Space Weather 2019, 17, 1208–1218. [Google Scholar] [CrossRef]
- Svanda, M.; Mourenas, D.; Zertova, K.; Vybostokova, T. Immediate and delayed responses of power lines and transformers in the Czech electric power grid to geomagnetic storms. J. Space Weather Space Clim. 2020, 10, 26. [Google Scholar] [CrossRef]
- Vybostokova, T.; Svanda, M. Correlation of anomaly rates in the Slovak electric transmission grid with geomagnetic activity. Adv. Space Res. 2022, 70, 3769–3780. [Google Scholar] [CrossRef]
- Halbedl, T.; Renner, H.; Sakulin, M.; Achleitner, G. Measurement and analysis of neutral point currents in a 400-kV-network. In Proceedings of the 2014 Electric Power Quality and Supply Reliability Conference (PQ), Rakvere, Estonia, 11–13 June 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 65–68. [Google Scholar] [CrossRef]
- Bailey, R.L.; Halbedl, T.; Schattauer, I.; Römer, A.; Achleitner, G.; Beggan, C.D.; Wesztergom, V.; Egli, R.; Leonhardt, R. Modelling geomagnetically induced currents in midlatitude Central Europe using a thin-sheet approach. Ann. Geophys. 2017, 35, 751–761. [Google Scholar] [CrossRef]
- Gil, A.; Berendt-Marchel, M.; Modzelewska, R.; Siluszyk, A.; Siluszyk, M.; Wawrzaszek, A.; Wawrzynczak, A. Analysis of Geoeffective Impulsive Events on the Sun During the First Half of Solar Cycle 24. Sol. Phys. 2023, 298, 26. [Google Scholar] [CrossRef]
- Abda, Z.M.K.; Aziz, N.F.A.; Kadir, M.Z.A.A.; Rhazali, Z.A. A Review of Geomagnetically Induced Current Effects on Electrical Power System: Principles and Theory. IEEE Access 2020, 8, 200237–200258. [Google Scholar] [CrossRef]
- Rajput, V.N.; Boteler, D.H.; Rana, N.; Saiyed, M.; Anjana, S.; Shah, M. Insight into impact of geomagnetically induced currents on power systems: Overview, challenges and mitigation. Electr. Power Syst. Res. 2021, 192, 106927. [Google Scholar] [CrossRef]
- Boteler, D.H.; Pirjola, R.J. Modeling geomagnetically induced currents. Space Weather 2017, 15, 258–276. [Google Scholar] [CrossRef]
- Viljanen, A.; Kauristie, K.; Pajunpää, K. On induction effects at EISCAT and IMAGE magnetometer stations. Geophys. J. Int. 1995, 121, 893–906. [Google Scholar] [CrossRef][Green Version]
- Dimmock, A.P.; Rosenqvist, L.; Hall, J.O.; Viljanen, A.; Yordanova, E.; Honkonen, I.; André, M.; Sjöberg, E.C. The GIC and geomagnetic response over Fennoscandia to the 7–8 September 2017 geomagnetic storm. Space Weather 2019, 17, 989–1010. [Google Scholar] [CrossRef]
- Dimmock, A.P.; Welling, D.T.; Rosenqvist, L.; Forsyth, C.; Freeman, M.P.; Rae, I.J.; Viljanen, A.; Vandegriff, E.; Boynton, R.J.; Balikhin, M.A.; et al. Modeling the geomagnetic response to the September 2017 space weather event over Fennoscandia using the space weather modeling framework: Studying the impacts of spatial resolution. Space Weather 2021, 19, e02683. [Google Scholar] [CrossRef]
- Gjerloev, J.W. The SuperMAG data processing technique. J. Geophys. Res. 2012, 117, A09213. [Google Scholar] [CrossRef]
- Clilverd, M.A.; Rodger, C.J.; Freeman, M.P.; Brundell, J.B.; Mac Manus, D.H.; Dalzell, M.; Clarke, E.; Thomson, A.W.P.; Richardson, G.S.; MacLeod, F.; et al. Geomagnetically induced currents during the 07–08 September 2017 disturbed period: A global perspective. J. Space Weather. Space Clim. 2021, 11, 33. [Google Scholar] [CrossRef]
- Jankowski, J.; Sucksdorff, C. Iaga Guide for Magnetic Measurements and Observatory Practice; International Association of Geomagnetism and Aeronomy: Paris, France, 1996. [Google Scholar]
- Wawrzaszek, A.; Gil, A.; Modzelewska, R.; Tsurutani, B.T.; Wawrzaszek, R. Analysis of Large Geomagnetically Induced Currents During the 7–8 September 2017 Storm: Geoelectric Field Mapping. Space Weather 2023, 21, e2022SW003383. [Google Scholar] [CrossRef]
- Buonsanto, M. Ionospheric Storms—A Review. Space Sci. Rev. 1999, 88, 563–601. [Google Scholar] [CrossRef]
- Boteler, D.H.; Pirola, R.J.; Nevanlinna, H. The effects of geomagnetic disturbances on electrical systems at the Earth’s surface. Adv. Space Res. 1998, 22, 17–27. [Google Scholar] [CrossRef]
- Viljanen, A.; Amm, O.; Pirjola, R. Modeling geomagnetically induced currents during different ionospheric situations. J. Geophys. Res. 1999, 104, 28059–28071. [Google Scholar] [CrossRef]
- Trichtchenko, L.; Boteler, D.H. Modelling geomagnetically induced currents using geomagnetic indices and data. IEEE Trans. Plasma Sci. 2004, 2004 32, 1459–1467. [Google Scholar] [CrossRef]
- Viljanen, A. Relation of Geomagnetically Induced Currents and Local Geomagnetic 700 Variations. IEEE Trans. Power Deliv. 1998, 13, 1285–1290. [Google Scholar] [CrossRef]
- Mac Manus, D.H.; Rodger, C.J.; Dalzell, M.; Thomson, A.W.P.; Clilverd, M.A.; Petersen, T.; Wolf, M.M.; Thomson, N.R.; Divett, T. Long term Geomagnetically Induced Current Observations in New Zealand: Earth return Corrections and Geomagnetic Field Driver. Space Weather 2017, 15, 1020–1038. [Google Scholar] [CrossRef]
- Kappenman, J. Storm sudden commencement events and the associated geomagnetically induced current risks to ground-based systems at low latitude and mid latitude locations. Space Weather 2003, 1, 1016. [Google Scholar] [CrossRef]
- Viljanen, A.; Pirjola, R. Influence of spatial variations of the geoelectric field on geomagnetically induced currents. J. Space Weather. Space Clim. 2017, 7, A22. [Google Scholar] [CrossRef]
- Svanda, M.; Smickova, A.; Vybostokova, T. Modelling of geomagnetically induced currents in the Czech transmission grid. Earth Planets Space 2021, 73, 229. [Google Scholar] [CrossRef]
- Marshall, R.A.; Waters, C.L.; Sciffer, M.D. Spectral analysis of pipe-to-soil potentials with variations of the Earth’s magnetic field in the Australian region. Space Weather 2010, 8, S05002. [Google Scholar] [CrossRef]
- Tozzi, R.; Coco, I.; De Michelis, P.; Giannattasio, F. Latitudinal dependence of geomagnetically induced currents during geomagnetic storms. Ann. Geophys. 2019, 62, GM448. [Google Scholar] [CrossRef]
- Boteler, D.H.; Pirjola, R.J.; Marti, L. Analytic calculation of geoelectric fields due to geomagnetic disturbances: A test case. IEEE Access 2019, 7, 147029. [Google Scholar] [CrossRef]
- Boteler, D.H.; Pirjola, R.J. Numerical calculation of geoelectric fields that affect critical infrastructure. Int. J. Geosci. 2019, 10, 930–949. [Google Scholar] [CrossRef]
- Adam, A.; Pracser, E.; Wesztergom, V. Estimation of the electric resistivity distribution (EURHOM) in the European lithosphere in the frame of the EURISGIC WP2 project. Acta Geod. Geophys. Hung. 2012, 47, 377–387. [Google Scholar] [CrossRef]
- Viljanen, A.; Pirjola, R.; Prácser, E.; Katkalov, J.; Wik, M. Geomagnetically induced currents in Europe: Modelled occurrence in a continent-wide power grid. J. Space Weather. Space Clim. 2014, 4, A09. [Google Scholar] [CrossRef]
- Boteler, D. On choosing Fourier transforms for practical geoscience applications. Int. J. Geosci. 2012, 3, 952–959. [Google Scholar] [CrossRef][Green Version]
- Viljanen, A.; Pirjola, R.J. Geomagnetically induced currents in the Finnish high-voltage power system. Surv. Geophys. 1994, 15, 383–408. [Google Scholar] [CrossRef]
- Lehtinen, M.; Pirjola, R.J. Currents produced in earthed conductor networks by geomagnetically-induced electric fields. Ann. Geophys. 1985, 1985 3, 479–484. [Google Scholar]
- Pirjola, R.; Lehtinen, M. Currents produced in the Finnish 400 kV power transmission grid and in the Finnish natural gas pipeline by geomagnetically induced electric fields. Ann. Geophys. 1985, 3, 485–491. [Google Scholar]
- Ni, Y.F.; Wang, Z.D.; Jarman, P. GIC Simulation Study for Part of UK Transmission System by ATP/EMTP. In Proceedings of the IEEE Power & Energy Society General Meeting, Chicago, IL, USA, 16–20 July 2017. [Google Scholar]
- Viljanen, A.; Koistinen, A.; Pajunpää, K.; Pirjola, R.; Posio, P.; Pulkkinen, A. Recordings of Geomagnetically Induced Currents in the Finnish Natural Gas Pipeline-Summary of an 11-year Period. Geophysica 2010, 46, 59–67. [Google Scholar]
- Kozyreva, O.V.; Pilipenko, V.A.; Belakhovsky, V.B.; Sakharov, Y.A. Ground geomagnetic field and GIC response to March 17, 2015, storm. Earth Planets Space 2018, 70, 157. [Google Scholar] [CrossRef]
- Zois, J.P. Solar activity and transformer failures in the Greek national electric grid I: Linear phenomena, talk given during the 8th. In European Space Weather Week-Session 3A, GIC Advances and Developing Mitigation Procedures; University of Namur: Namur, Belgium, 2011. [Google Scholar]
- Torta, J.M.; Serrano, L.; Regué, J.R.; Sánchez, A.M.; Roldán, E. Geomagnetically induced currents in a power grid of northeastern Spain. Space Weather 2012, 10. [Google Scholar] [CrossRef]
- Marsal, S.; Torta, J.M.; Curto, J.J.; Canillas-Pérez, V.; Cid, O.; Ibańez, M.; Marcuello, A. Validating GIC modeling in the Spanish power grid by differential magnetometry. Space Weather 2021, 19, e2021SW002905. [Google Scholar] [CrossRef]
- Torta, J.M.; Marsal, S.; Ledo, J.; Queralt, P.; Canillas-Perez, V.; Pina-Varas, P.; Curto, J.J.; Marcuello, A.; Martí, A. New detailed modeling of GICs in the Spanish power transmission grid. Space Weather 2021, 19, e2021SW002805. [Google Scholar] [CrossRef]
- Marsal, S.; Torta, J.M.; Canillas-Perez, V.; Curto, J.J. A new standalone tool for DC-equivalent network generation and GIC calculation in power grids with multiple voltage levels. Space Weather 2022, 20, e2021SW002984. [Google Scholar] [CrossRef]
- Torta, J.M.; Marsal, S.; Pina-Varas, P.; Hafizi, R.; Marti, A.; Campanya, J.; Canillas-Pérez, V.; Curto, J.J.; Ledo, J.; Queralt, P.; et al. Expected geomagnetically induced currents in the Spanish islands power transmission grids. Space Weather 2023, 21, e2023SW003426. [Google Scholar] [CrossRef]
- Pirjola, R. Electromagnetic induction in the Earth by a plane wave or by fields of line current harmonic in time and space. Geophysica 1982, 18, 1–161. [Google Scholar]
- Piersanti, M.; De Michelis, P.; Del Moro, D.; Tozzi, R.; Pezzopane, M.; Consolini, G.; Marcucci, M.F.; Laurenza, M.; Di Matteo, S.; Pignalberi, A.; et al. From the Sun to Earth: Effects of the 25 August 2018 geomagnetic storm. Ann. Geophys. 2020, 38, 703–724. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Joselyn, J.A.; Kamide, Y.; Kroehl, H.W.; Rostoker, G.; Tsurutani, B.T.; Vasyliunas, V.M. What is a geomagnetic storm? J. Geophys. 1994, 99, 5771–5792. [Google Scholar] [CrossRef]
- Halbedl, T.; Renner, H.; Bailey, R.L.; Leonhardt, R.; Achleitner, G. Analysis of the impact of geomagnetic disturbances on the Austrian transmission grid. In Proceedings of the 19th Power Systems Computation Conference, Genoa, Italy, 20–24 June 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 1–5. [Google Scholar] [CrossRef]
- Bailey, R.L.; Halbedl, T.; Schattauer, I.; Achleitner, G.; Leonhardt, R. Validating GIC models with measurements in Austria: Evaluation of accuracy and sensitivity to input parameters. Space Weather 2018, 16, 887–902. [Google Scholar] [CrossRef]
- Albert, D.; Schachinger, P.; Bailey, R.L.; Renner, H.; Achleitner, G. Analysis of long-term GIC measurements in transformers in Austria. Space Weather 2022, 20, e2021SW002912. [Google Scholar] [CrossRef]
- Bailey, R.L.; Leonhardt, R. Automated detection of geomagnetic storms with heightened risk of GIC. Earth Planets Space 2016, 68, 716. [Google Scholar] [CrossRef]
- Bailey, R.L.; Leonhardt, R.; Möstl, C.; Beggan, C.; Reiss, M.A.; Bhaskar, A.; Weiss, A.J. Forecasting GICs and geoelectric fields from solar wind data using LSTMs: Application in Austria. Space Weather 2022, 20, e2021SW002907. [Google Scholar] [CrossRef]
- Raport Najwyzszej Izby Kontroli. Informacja o wynikach kontroli. In Zapewnienie Mocy Wytworczych w Elektroenergetyce Konwencjonalnej, KGP-4101-001-00/2014, Nr ewid. 17/2015/P/14/018/KGP, Warszawa; 2015. Available online: https://www.nik.gov.pl/kontrole/P/14/018/KGP/ (accessed on 15 July 2023).
- Gil, A.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Wawrzaszek, A.; Wawrzynczak, A. Signs of geoeffective space weather events in cosmic rays during the first half of the solar cycle 24. In NMDB@Home 2020; Kiel University Publishing: Kiel, Germany, 2021; pp. 119–124. [Google Scholar] [CrossRef]
- Gil, A.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Wawrzynczak, A.; Pozoga, M.; Tomasik, L. The Solar Event of 14–15 July 2012 and Its Geoeffectiveness. Sol. Phys. 2020, 295, 135. [Google Scholar] [CrossRef]
- Gil, A.; Modzelewska, R.; Wawrzaszek, A.; Piekart, B.; Milosz, T. Solar Rotation Multiples in Space-Weather Effects. Sol. Phys. 2021, 296, 128. [Google Scholar] [CrossRef]
- Viljanen, A.; Pirjola, R. Statistics on geomagnetically-induced currents in the Finnish 400 kV power system based on recordings of geomagnetic variations. J. Geomagnet. Geoelectr. 1989, 41, 411–420. [Google Scholar] [CrossRef]
- Gil, A.; Glavan, V.; Wawrzaszek, A.; Modzelewska, R.; Tomasik, L. Katz Fractal Dimension of Geoelectric Field during Severe Geomagnetic Storms. Entropy 2021, 23, 1531. [Google Scholar] [CrossRef] [PubMed]
- Gil, A.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Wawrzynczak, A.; Pozoga, M.; Domijanski, S. Transmission Lines in Poland and Space Weather Effects. Energies 2020, 13, 2359. [Google Scholar] [CrossRef]
- Gaunt, C.T.; Coetzee, G. Transformer failures in regions incorrectly considered to have low GIC-risk. In Power Tech; Institute of Electrical and Electronics Engineers: Lausanne, Switzerland, 2007; pp. 807–812. [Google Scholar] [CrossRef]
- Bejmert, D.; Boehme, K.; Kereit, M.; Rebizant, W. HV Transformer Protection and Stabilization under Geomagnetically Induced Currents. Energies 2020, 13, 4693. [Google Scholar] [CrossRef]
- Panicali, A.R.; Barbosa, C.F. Effect of the integration path on grounding measurements. Electr. Power Syst. Res. 2021, 194, 107062. [Google Scholar] [CrossRef]
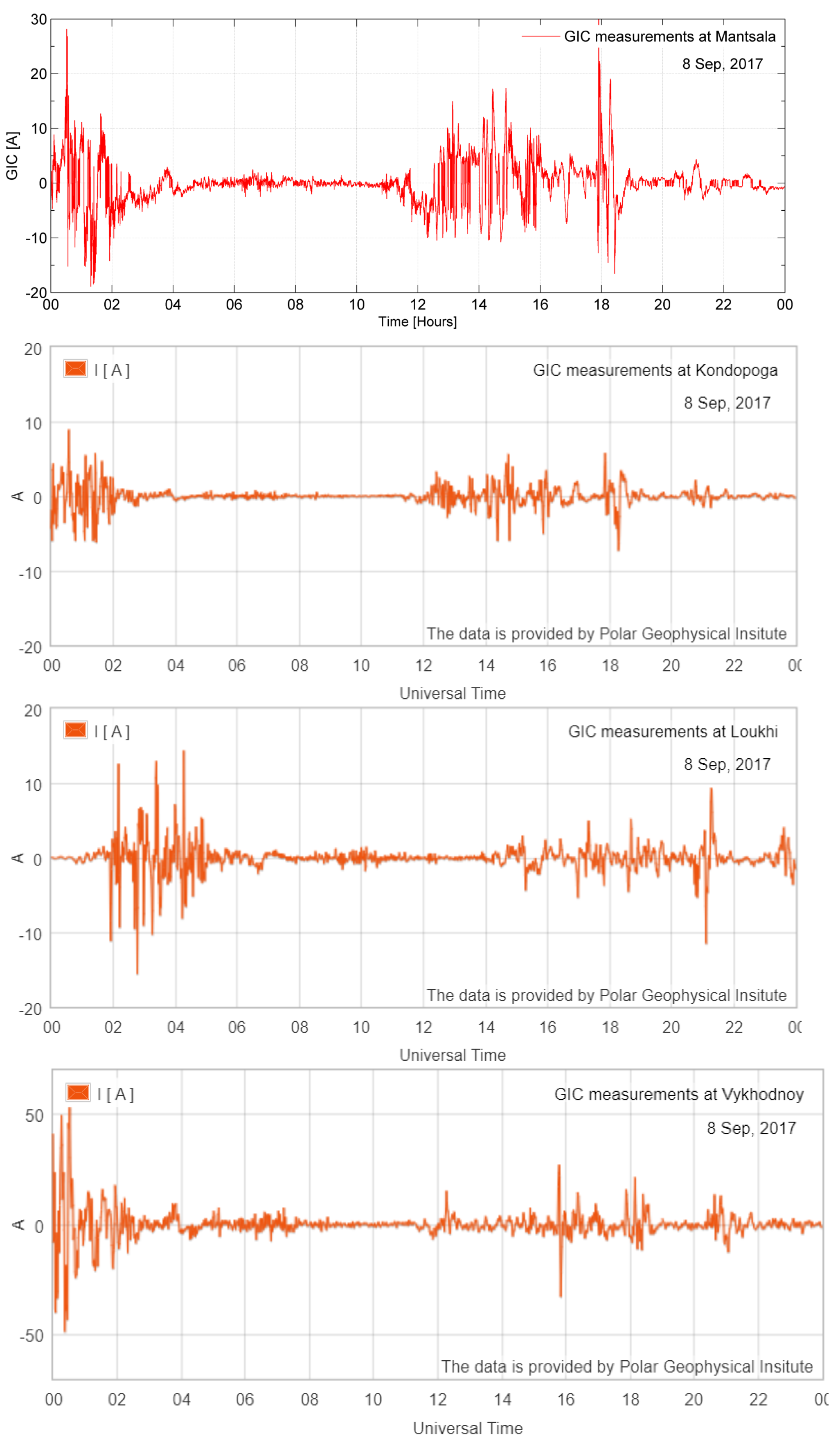
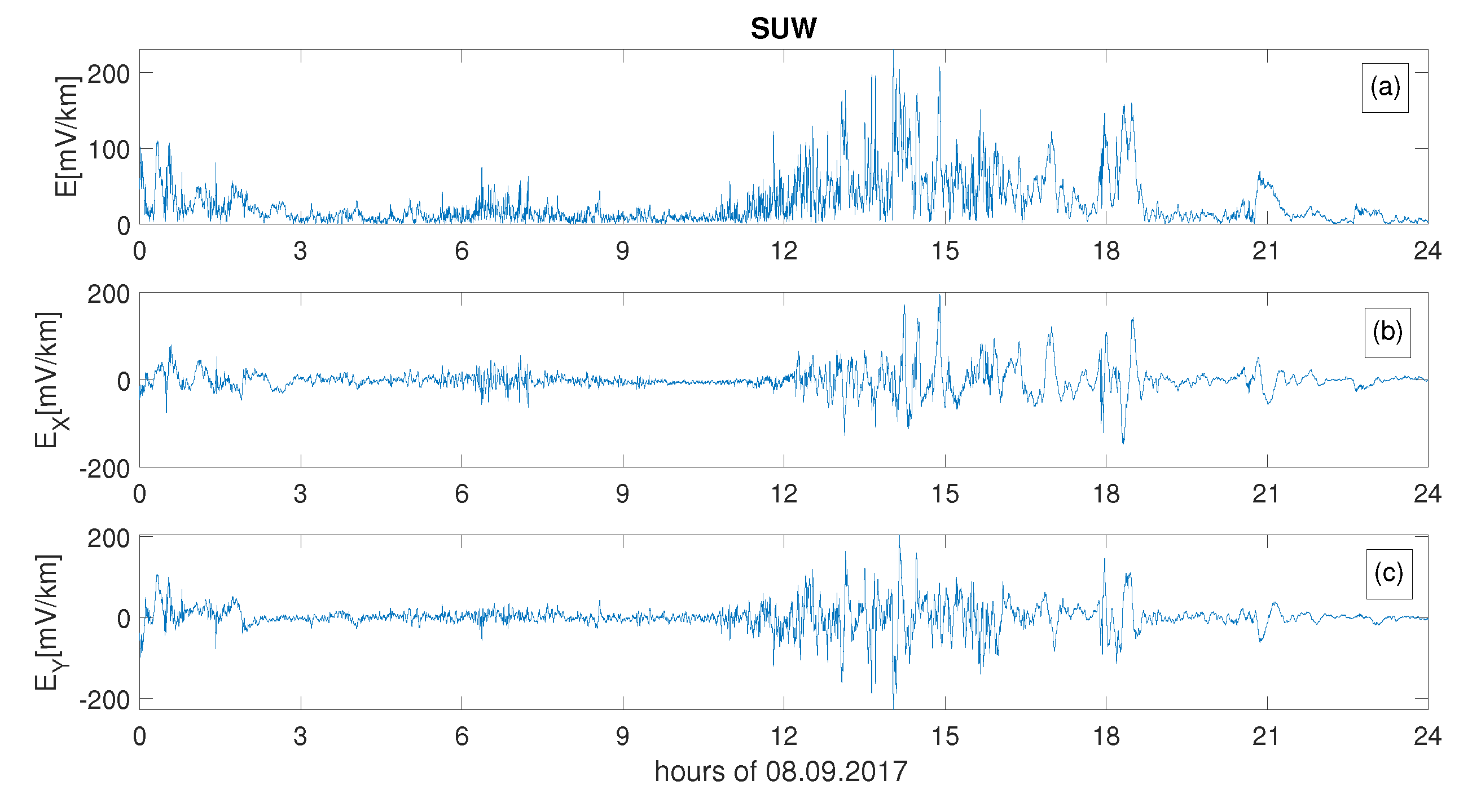
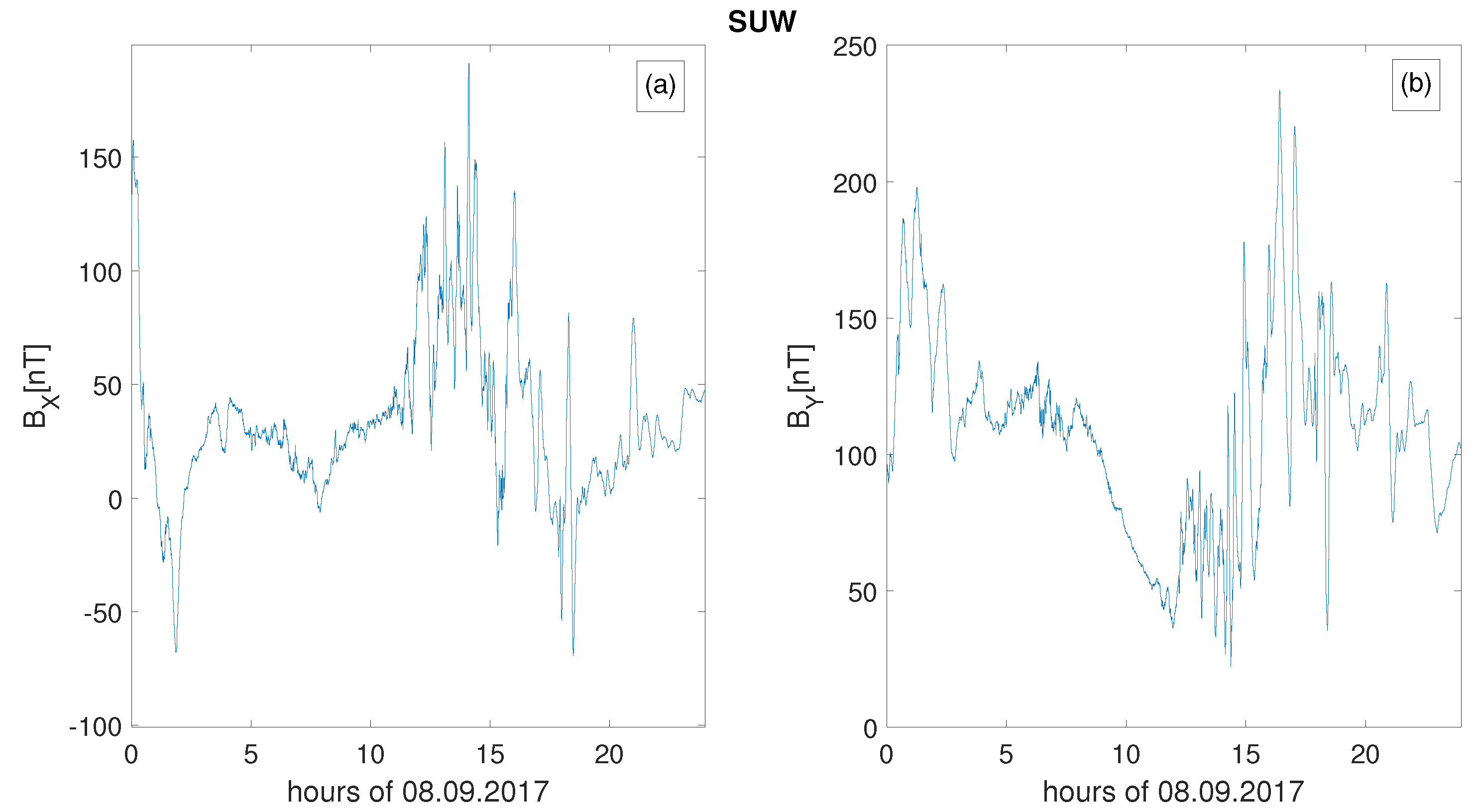
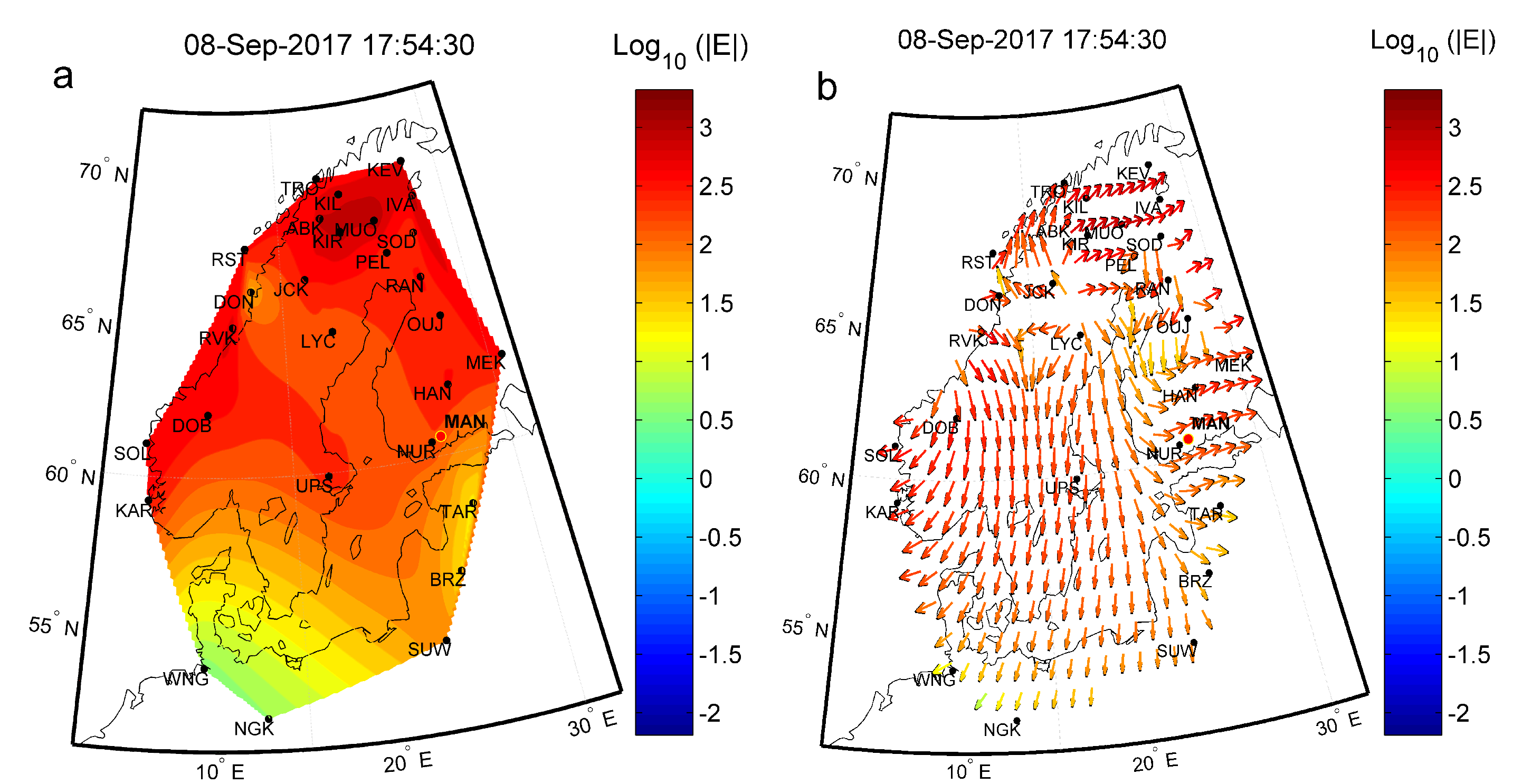
| Dataset ID | Voltage Level | Type | Span |
|---|---|---|---|
| D1 | Very high voltage | Equipment: transformers, ES | 9 years |
| D2 | High voltage | Equipment | 6 years |
| D3 | Very high voltage | Equipment | 6 years |
| D4 | High and low voltage | Power lines | 7 years |
| D5 | High and low voltage | Equipment and power lines | 7 years |
| D6 | High and low voltage | Equipment | 7 years |
| D7 | Very high voltage | Power lines | 10 years |
| D8 | High voltage | Transformers | 10 years |
| D9 | Very high voltage | Transformers | 10 years |
| D10 | Very high and high voltage | Electrical substations | 10 years |
| D11 | Very high voltage | Power lines | 10 years |
| D12 | High voltage | Power lines | 10 years |
| The Main Groups | Number in 2010 | % in 2010 | |
|---|---|---|---|
| A | meteorological effects | 3653 | 14.3 |
| B | operational shutdowns | 16,614 | 64.9 |
| C | vandalism | 824 | 3.2 |
| D | aging (Ag) | 1917 | 7.5 |
| E | electronics devices (Ed) | 32 | 0.1 |
| F | unidentified (Un) | 2576 | 10 |
| TOTAL | 25,616 | 100.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gil, A.; Berendt-Marchel, M.; Modzelewska, R.; Siluszyk, A.; Siluszyk, M.; Wawrzaszek, A.; Wawrzynczak, A. Review of Geomagnetically Induced Current Proxies in Mid-Latitude European Countries. Energies 2023, 16, 7406. https://doi.org/10.3390/en16217406
Gil A, Berendt-Marchel M, Modzelewska R, Siluszyk A, Siluszyk M, Wawrzaszek A, Wawrzynczak A. Review of Geomagnetically Induced Current Proxies in Mid-Latitude European Countries. Energies. 2023; 16(21):7406. https://doi.org/10.3390/en16217406
Chicago/Turabian StyleGil, Agnieszka, Monika Berendt-Marchel, Renata Modzelewska, Agnieszka Siluszyk, Marek Siluszyk, Anna Wawrzaszek, and Anna Wawrzynczak. 2023. "Review of Geomagnetically Induced Current Proxies in Mid-Latitude European Countries" Energies 16, no. 21: 7406. https://doi.org/10.3390/en16217406
APA StyleGil, A., Berendt-Marchel, M., Modzelewska, R., Siluszyk, A., Siluszyk, M., Wawrzaszek, A., & Wawrzynczak, A. (2023). Review of Geomagnetically Induced Current Proxies in Mid-Latitude European Countries. Energies, 16(21), 7406. https://doi.org/10.3390/en16217406






