A Literature Review of the Positive Displacement Compressor: Current Challenges and Future Opportunities
Abstract
:1. Introduction
2. Indicators of Compressor Performance
2.1. Basic Equations for Positive Displacement Compressors
2.2. Leakage
2.3. Heat Transfer
2.3.1. Convection Heat Transfer
2.3.2. Conduction Heat Transfer
2.4. Friction and Lubrication
2.5. Valve Dynamic and Port Characteristics
2.6. Capacity Control
3. Recent Innovative Modelling in Compressor Research
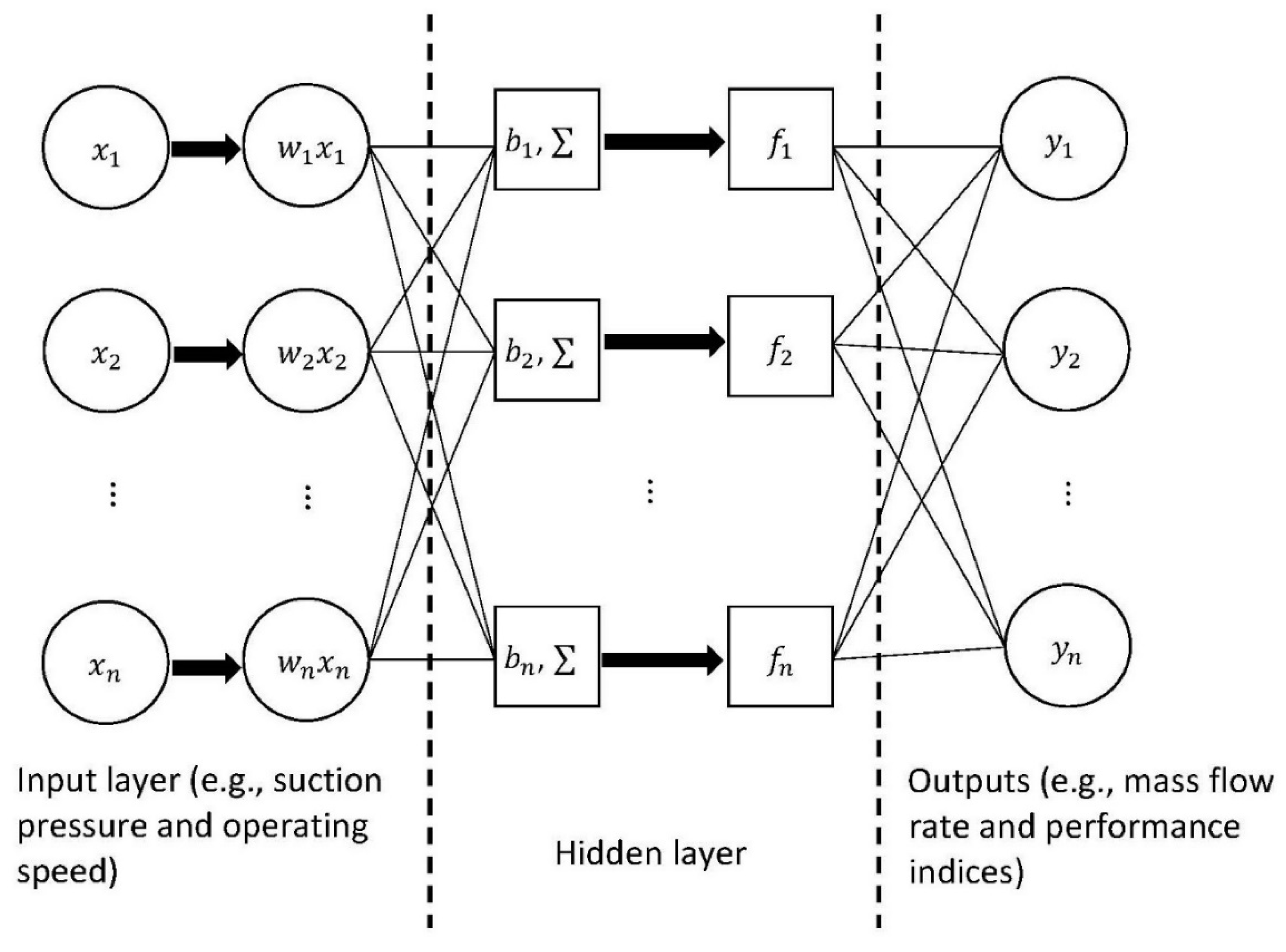
3.1. Artificial Neural Network
3.2. Genetic Algorithm Optimisation
3.3. Other Methods
4. Challenges and Opportunities for Future Studies
5. Conclusions
Funding
Conflicts of Interest
Nomenclature
| A | Heat transfer area |
| AL | Flow area of leakage |
| ANN | Artificial neural network |
| C | Discharge coefficient |
| CFD | Computational fluid dynamics |
| COP | Coefficient of performance |
| Dc | Diameter of curvature |
| Dh | Hydraulic diameter |
| FAD | Free air delivery |
| GA | Genetic algorithm |
| h | Heat transfer coefficient |
| k | Thermal conductivity |
| n | Polytropic index |
| ηv | Volumetric efficiency |
| ηisen | Isentropic efficiency |
| ηp | Polytropic efficiency |
| ηiso | Isothermal efficiency |
| ηm | Mechanical efficiency |
| Mass flow rate | |
| Wisen | Isentropic work |
| Wp | Polytropic work |
| Wiso | Isothermal work |
| Ws | Mechanical work |
| WR | Work required |
| γ | Ratio of specific heats |
| Q | Heating capacity |
| R | Ideal gas constant |
| St | Strouhal number |
| SPSA | Simultaneous perturbation stochastic approximation |
| SVM | Support vector machine |
| T | Temperature |
| ΔT | Temperature difference |
| P | Pressure |
| PCA | Principal component analysis |
| PLS | Partial least squares |
| Re | Reynolds number |
| Pr | Prandtl number |
| Nu | Nusselt number |
| V | Compression chamber volume |
| Vi | Theoretical volume |
| Vs | Swept volume |
References
- Stouffs, P.; Tazerout, M.; Wauters, P. Thermodynamic analysis of reciprocating compressors. Int. J. Therm. Sci. 2001, 40, 52–66. [Google Scholar] [CrossRef]
- Sultan, I.A.; Phung, T.H. (Eds.) Positive Displacement Machines: Modern Design Innovations and Tools; Academic Press: Cambridge, MA, USA, 2019. [Google Scholar]
- Spark, I.J.; Lu, K. The orbital displacer: Implications and applications. In Positive Displacement Machines; Sultan, I.A., Phung, T.H., Eds.; Academic Press: Cambridge, MA, USA, 2019; pp. 3–34. [Google Scholar]
- Li, J.; Jia, X.; Wu, Z.; Peng, X. The cavity profile of a diaphragm compressor for a hydrogen refueling station. Int. J. Hydrogen Energy 2014, 39, 3926–3935. [Google Scholar] [CrossRef]
- Liang, K. A review of linear compressors for refrigeration. Int. J. Refrig. 2017, 84, 253–273. [Google Scholar] [CrossRef]
- Yanagisawa, T.; Shimizu, T.; Chu, I.; Ishijima, K. Motion analysis of rolling piston in rotary compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 21–23 July 1982; Purdue e-Pubs: West Lafayette, IN, USA, 1982. [Google Scholar]
- Stosic, N.; Smith, I.K.; Kovacevic, A. Numerical and experimental research in heat transfer to screw compressor rotors. In Proceedings of the ASME/JSME Thermal Engineering Joint Conference, Honolulu, HI, USA, 13–17 March 2011; Volume 38921, p. T20050. [Google Scholar]
- Chen, Y.; Halm, N.P.; Groll, E.A.; Braun, J.E. Mathematical modeling of scroll compressors—Part I: Compression process modeling. Int. J. Refrig. 2002, 25, 731–750. [Google Scholar] [CrossRef]
- Prescient & Strategic Intelligence. Compressor Market Research Report (2020–2030). 2020. Available online: https://www.psmarketresearch.com/market-analysis/compressor-market (accessed on 10 July 2021).
- Silva, W.L.V.; Souza, L.C.O.; Bortolaia, L.A.; Paula, M.R.D.; Leal, E.M. Study of the electricity consumption reduction of a compressed air system: The case of a steelmaking company. REM-Int. Eng. J. 2017, 70, 421–428. [Google Scholar] [CrossRef]
- Vittorini, D.; Cipollone, R. Energy saving potential in existing industrial compressors. Energy 2016, 102, 502–515. [Google Scholar] [CrossRef]
- Saidur, R.; Rahim, N.A.; Hasanuzzaman, M. A review on compressed-air energy use and energy savings. Renew. Sustain. Energy Rev. 2010, 14, 1135–1153. [Google Scholar] [CrossRef]
- Sultan, I.A. The limaçon of Pascal: Mechanical generation and utilization for fluid processing. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2005, 219, 813–822. [Google Scholar] [CrossRef]
- Heidari, M.; Mortazavi, M.; Rufer, A. Design, modeling and experimental validation of a novel finned reciprocating compressor for Isothermal Compressed Air Energy Storage applications. Energy 2017, 140, 1252–1266. [Google Scholar] [CrossRef]
- Hu, Y.S.; Wei, H.J.; Xu, J.; Du, Z.C.; Yang, S.; Ren, L.P. The Theoretical and Experimental Research of a Novel Rotary Cylinder Compressor. In IOP Conference Series: Materials Science and Engineering, Proceedings of the International Conference on Compressors and Their Systems, City, University of London, 9–11 September 2019; IOP Publishing: Bristol, UK, 2019; Volume 604, Number 1; p. 012073. [Google Scholar]
- Shin, M.; Na, S.; Lee, J.; Min, B.; Choi, G. Model analysis of a novel compressor with a dual chamber for high-efficiency systems. Appl. Therm. Eng. 2019, 158, 113717. [Google Scholar] [CrossRef]
- Shakya, P.; Ooi, K.T. Introduction to Coupled Vane compressor: Mathematical modelling with validation. Int. J. Refrig. 2020, 117, 23–32. [Google Scholar] [CrossRef]
- Gao, X.; Liu, Y. Design, modeling and characteristics research of a novel self-air-cooling reciprocating compressor. Int. J. Refrig. 2021, 128, 62–70. [Google Scholar] [CrossRef]
- Lu, K.; Sultan, I.A.; Phung, T.H. Mathematical modeling and parametric study of the limaçon rotary compressor. Int. J. Refrig. 2021, 134, 219–231. [Google Scholar] [CrossRef]
- Prasad, B.S. Heat Transfer in Reciprocating Compressors: A Review. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Anaheim, CA, USA, 15–20 November 1998; American Society of Mechanical Engineers: New York, NY, USA, 1998; Volume 15991, pp. 361–365. [Google Scholar]
- Stosic, N.; Smith, I.K.; Kovacevic, A.; Mujic, E. Review of mathematical models in performance calculation of screw compressors. Int. J. Fluid Mach. Syst. 2011, 4, 271–288. [Google Scholar] [CrossRef]
- Ueno, K.; Bye, R.E.; Hunter, K.S. Compressor Efficiency Definitions. 2003. Available online: http://vairex.com/resources/VAIREX_Compressor_Efficiency_Def_12May03.pdf (accessed on 17 August 2023).
- Cho, N.K.; Youn, Y.; Lee, B.C.; Min, M.K. The characteristics of tangential leakage in scroll compressors for air-conditioners. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 25–28 July 2000; Purdue e-Pubs: West Lafayette, IN, USA, 2000. [Google Scholar]
- Yuan, X.; Chen, Z.; Fan, Z. Calculating model and experimental investigation of gas leakage. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1992; Purdue e-Pubs: West Lafayette, IN, USA, 1992. [Google Scholar]
- Kim, G.; Min, B.; Na, S.; Choi, G.; Kim, D. Estimation of leakage through radial clearance during compression process of a rolling piston rotary compressor. J. Mech. Sci. Technol. 2017, 31, 6033–6040. [Google Scholar] [CrossRef]
- Pereira, E.L.; Deschamps, C.J. Numerical analysis and correlations for radial and tangential leakage of gas in scroll compressors. Int. J. Refrig. 2020, 110, 239–247. [Google Scholar] [CrossRef]
- Lu, K.; Sultan, I.A.; Phung, T.H. A comparative study of two embodiments of the limaçon rotary compressor based on theoretical modelling of apex seal dynamics and leakage. Int. J. Refrig. 2023, 145, 467–480. [Google Scholar] [CrossRef]
- Yanagisawa, T.; Shimizu, T. Leakage losses with a rolling piston type rotary compressor. I. Radical clearance on the rolling piston. Int. J. Refrig. 1985, 8, 75–84. [Google Scholar] [CrossRef]
- Yanagisawa, T.; Shimizu, T. Leakage losses with a rolling piston type rotary compressor. II. Leakage losses through clearances on rolling piston faces. Int. J. Refrig. 1985, 8, 152–158. [Google Scholar] [CrossRef]
- Rodgers, R.J.; Nieter, J.J. Comprehensive analysis of leakage in rotary compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 23–26 July 1996; Purdue e-Pubs: West Lafayette, IN, USA, 1996. [Google Scholar]
- Kang, D.J.; Kim, J.W.; Sohn, C.B. Effects of leakage flow model on the thermodynamic performance of a scroll compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 16–19 July 2002; Purdue e-Pubs: West Lafayette, IN, USA, 2002. [Google Scholar]
- Teh, Y.L.; Ooi, K.T. Theoretical study of a novel refrigeration compressor-Part III: Leakage loss of the revolving vane (RV) compressor and a comparison with that of the rolling piston type. Int. J. Refrig. 2009, 32, 945–952. [Google Scholar] [CrossRef]
- Silva, L.R.; Deschamps, C.J. Modeling of gas leakage through compressor valves. Int. J. Refrig. 2015, 53, 195–205. [Google Scholar] [CrossRef]
- Aw, K.T.; Ooi, K.T. Leakage study of a Lubricant-free Revolving Vane Compressor. Int. J. Refrig. 2021, 124, 122–133. [Google Scholar] [CrossRef]
- Ferreira, R.T.S.; Lilie, D.E.B. Evaluation of the leakage through the clearance between piston and cylinder in hermetic compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 11–13 July 1984; Purdue e-Pubs: West Lafayette, IN, USA, 1984. [Google Scholar]
- Ishii, N.; Bird, K.; Sano, K.; Oono, M.; Iwamura, S.; Otokura, T. Refrigerant leakage flow evaluation for scroll compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 23–26 July 1996; Purdue e-Pubs: West Lafayette, IN, USA, 1996. [Google Scholar]
- Lohn, S.K.; Pereira, E.L.L. Numerical investigation of the gas leakage through the piston-cylinder clearance of reciprocating compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 2014; Purdue e-Pubs: West Lafayette, IN, USA, 2014. [Google Scholar]
- Annand, W.J.D.; Thermodynamics and Fluid Mechanics Group. Heat transfer in the cylinders of reciprocating internal combustion engines. Proc. Inst. Mech. Eng. 1963, 177, 973–996. [Google Scholar]
- Adair, R.P.; Qvale, E.B.; Pearson, J.T. Instantaneous heat transfer to the cylinder wall in reciprocating compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 17–20 July 1972; Purdue e-Pubs: West Lafayette, IN, USA, 1972. [Google Scholar]
- Liu, R.; Zhou, Z. Heat transfer between gas and cylinder wall of refrigerating reciprocating compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 12–15 July 1984; Purdue e-Pubs: West Lafayette, IN, USA, 1984. [Google Scholar]
- Tan, K.M.; Ooi, K.T. Heat transfer in compression chamber of a revolving vane (RV) compressor. Appl. Therm. Eng. 2011, 31, 1519–1526. [Google Scholar] [CrossRef]
- Hsieh, W.H.; Wu, T.T. Experimental investigation of heat transfer in a high-pressure reciprocating gas compressor. Exp. Therm. Fluid Sci. 1996, 13, 44–54. [Google Scholar] [CrossRef]
- Disconzi, F.P.; Deschamps, C.J.; Pereira, E.L. Development of an in-cylinder heat transfer correlation for reciprocating compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 16–19 July 2012; Purdue e-Pubs: West Lafayette, IN, USA, 2012. [Google Scholar]
- Ooi, K.T.; Zhu, J. Convective heat transfer in a scroll compressor chamber: A 2-D simulation. Int. J. Therm. Sci. 2004, 43, 677–688. [Google Scholar] [CrossRef]
- Jang, K.; Jeong, S. Experimental investigation on convective heat transfer mechanism in a scroll compressor. Int. J. Refrig. 2006, 29, 744–753. [Google Scholar] [CrossRef]
- Rak, J.; Pietrowicz, S. Internal flow field and heat transfer investigation inside the working chamber of a scroll compressor. Energy 2020, 202, 117700. [Google Scholar] [CrossRef]
- Padhy, S.K.; Dwivedi, S.N. Heat transfer analysis of a rolling-piston rotary compressor. Int. J. Refrig. 1994, 17, 400–410. [Google Scholar] [CrossRef]
- Ooi, K.T. Heat transfer study of a hermetic refrigeration compressor. Appl. Therm. Eng. 2003, 23, 1931–1945. [Google Scholar] [CrossRef]
- Sanvezzo, J., Jr.; Deschamps, C.J. A heat transfer model combining differential and integral formulations for thermal analysis of reciprocating compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 16–19 July 2012; Purdue e-Pubs: West Lafayette, IN, USA, 2012. [Google Scholar]
- Dutra, T.; Deschamps, C.J. Experimental characterization of heat transfer in the components of a small hermetic reciprocating compressor. Appl. Therm. Eng. 2013, 58, 499–510. [Google Scholar] [CrossRef]
- Patil, V.C.; Acharya, P.; Ro, P.I. Experimental investigation of heat transfer in liquid piston compressor. Appl. Therm. Eng. 2019, 146, 169–179. [Google Scholar] [CrossRef]
- Stosic, N. On heat transfer in screw compressors. Int. J. Heat Fluid Flow 2015, 51, 285–297. [Google Scholar] [CrossRef]
- Yanagisawa, T.; Shimizu, T. Friction losses in rolling piston type rotary compressors. III. Int. J. Refrig. 1985, 8, 159–165. [Google Scholar] [CrossRef]
- Ooi, K.T. Design optimization of a rolling piston compressor for refrigerators. Appl. Therm. Eng. 2005, 25, 813–829. [Google Scholar] [CrossRef]
- Teh, Y.L.; Ooi, K.T. Theoretical study of a novel refrigeration compressor–Part I: Design of the revolving vane (RV) compressor and its frictional losses. Int. J. Refrig. 2009, 32, 1092–1102. [Google Scholar] [CrossRef]
- Liu, Y.; Hung, C.; Chang, Y. Design optimization of scroll compressor applied for frictional losses evaluation. Int. J. Refrig. 2010, 33, 615–624. [Google Scholar] [CrossRef]
- Yang, B.; Bradshaw, C.R.; Groll, E.A. Modeling of a semi-hermetic CO2 reciprocating compressor including lubrication submodels for piston rings and bearings. Int. J. Refrig. 2013, 36, 1925–1937. [Google Scholar] [CrossRef]
- Bianchi, G.; Cipollone, R. Friction power modeling and measurements in sliding vane rotary compressors. Appl. Therm. Eng. 2015, 84, 276–285. [Google Scholar] [CrossRef]
- Gu, H.; Zhou, X.; Chen, Y.; Wu, J.; Wu, Z.; Jiang, Y.; Sundén, B. Analysis, modeling and simulations of an innovative sliding vane rotary compressor with a rotating cylinder. Energy Convers. Manag. 2021, 230, 113822. [Google Scholar] [CrossRef]
- Kim, H.J.; Lancey, T.W. Numerical study on the lubrication oil distribution in a refrigeration rotary compressor. Int. J. Refrig. 2003, 26, 800–808. [Google Scholar] [CrossRef]
- Afshari, F.; Comakli, O.; Lesani, A.; Karagoz, S. Characterization of lubricating oil effects on the performance of reciprocating compressors in air–water heat pumps. Int. J. Refrig. 2017, 74, 505–516. [Google Scholar] [CrossRef]
- Wu, X.; Xing, Z.; He, Z.; Wang, X.; Chen, W. Effects of lubricating oil on the performance of a semi-hermetic twin screw refrigeration compressor. Appl. Therm. Eng. 2017, 112, 340–351. [Google Scholar] [CrossRef]
- Ozsipahi, M.; Kose, H.A.; Cadirci, S.; Kerpicci, H.; Gunes, H. Experimental and numerical investigation of lubrication system for reciprocating compressor. Int. J. Refrig. 2019, 108, 224–233. [Google Scholar] [CrossRef]
- Valenti, G.; Colombo, L.; Murgia, S.; Lucchini, A.; Sampietro, A.; Capoferri, A.; Araneo, L. Thermal effect of lubricating oil in positive-displacement air compressors. Appl. Therm. Eng. 2013, 51, 1055–1066. [Google Scholar] [CrossRef]
- Pizarro-Recabarren, R.A.; Barbosa, J.R. The effect of the lubricating oil on heat transfer in a hermetic reciprocating compressor. J. Braz. Soc. Mech. Sci. Eng. 2016, 38, 189–208. [Google Scholar] [CrossRef]
- Nagata, S.; Nozaki, T.; Akizawa, T. Analysis of dynamic behavior of suction valve using strain gauge in reciprocating compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 12–15 July 2010; Purdue e-Pubs: West Lafayette, IN, USA, 2010. [Google Scholar]
- Ma, Y.; He, Z.; Peng, X.; Xing, Z. Experimental investigation of the discharge valve dynamics in a reciprocating compressor for trans-critical CO2 refrigeration cycle. Appl. Therm. Eng. 2012, 32, 13–21. [Google Scholar] [CrossRef]
- Wang, Y.; Xue, C.; Feng, J.; Peng, X. Experimental investigation on valve impact velocity and inclining motion of a reciprocating compressor. Appl. Therm. Eng. 2013, 61, 149–156. [Google Scholar] [CrossRef]
- Mu, G.; Wang, F.; Mi, X.; Gao, G. Dynamic modeling and analysis of compressor reed valve based on movement characteristics. Appl. Therm. Eng. 2019, 150, 522–531. [Google Scholar] [CrossRef]
- Egger, A.; Almbauer, R.; Dür, L.; Hopfgartner, J.; Lang, M. Multi-Response optimization applied to a mechanically assisted reed valve of a hermetic reciprocating compressor. Int. J. Refrig. 2020, 119, 119–130. [Google Scholar] [CrossRef]
- Huang, B.; Xie, F. Dynamic analysis of the discharge valve of the rotary compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 2008; Purdue e-Pubs: West Lafayette, IN, USA, 2008. [Google Scholar]
- Teh, Y.L.; Ooi, K.T.; Djamari, D.W. Theoretical study of a novel refrigeration compressor–Part II: Performance of a rotating discharge valve in the revolving vane (RV) compressor. Int. J. Refrig. 2009, 32, 1103–1111. [Google Scholar] [CrossRef]
- Yu, X.; Ren, Y.; Tan, Q.; Lu, Z.; Jia, X.; Wang, X. Study on the torsional movement of a reed valve in a rotary compressor. Adv. Mech. Eng. 2018, 10, 1687814018778402. [Google Scholar] [CrossRef]
- Min, B.; Na, S.; Yang, J.; Choi, G. Geometric correlation of discharge coefficients for discharge valve system in rolling piston rotary compressor. J. Mech. Sci. Technol. 2018, 32, 3943–3954. [Google Scholar] [CrossRef]
- Kim, H.J.; Ahn, J.M.; Hwang, S.W.; Kiem, M.K. Performance analysis of a twin rotary compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–19 July 2004; Purdue e-Pubs: West Lafayette, IN, USA, 2004. [Google Scholar]
- Mujic, E.; Kovacevic, A.; Stosic, N.; Smith, I.K. The influence of port shape on gas pulsations in a screw compressor discharge chamber. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2008, 222, 211–223. [Google Scholar] [CrossRef]
- Sultan, I.A.; Schaller, C.G. Optimum positioning of ports in the limaçon gas expanders. J. Eng. Gas Turbines Power 2011, 133, 103002. [Google Scholar] [CrossRef]
- Lim, Y.D.; Lin, M.; Ooi, K.T. Simulation study of multi-chamber rotary compressor. In IOP Conference Series: Materials Science and Engineering, Proceedings of the 10th International Conference on Compressors and Their Systems, City, University of London, 11–13 September 2017; IOP Publishing: Bristol, UK, 2017; Volume 232, Number 1; p. 012064. [Google Scholar]
- Zhao, R.; Li, W.; Zhuge, W. Unsteady characteristic and flow mechanism of a scroll compressor with novel discharge port for electric vehicle air conditioning. Int. J. Refrig. 2020, 118, 403–414. [Google Scholar] [CrossRef]
- Gu, H.; Chen, Y.; Wu, J.; Jiang, Y.; Sundén, B. Impact of discharge port configurations on the performance of sliding vane rotary compressors with a rotating cylinder. Appl. Therm. Eng. 2021, 186, 116526. [Google Scholar] [CrossRef]
- Hong, W.; Jin, J.; Wu, R.; Zhang, B. Theoretical analysis and realization of stepless capacity regulation for reciprocating compressors. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2009, 223, 205–213. [Google Scholar] [CrossRef]
- Tuymer, W.J. Stepless variable capacity control. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 10–12 July 1974; Purdue e-Pubs: West Lafayette, IN, USA, 1974. [Google Scholar]
- Bin, T.; Yuanyang, Z.; Liansheng, L.; Guangbin, L.; Le, W.; Qichao, Y.; Haiping, X.; Feng, Z.; Wenhui, M. Thermal performance analysis of reciprocating compressor with stepless capacity control system. Appl. Therm. Eng. 2013, 54, 380–386. [Google Scholar] [CrossRef]
- Wang, Y.; Jiang, Z.; Zhang, J.; Zhou, C.; Liu, W. Performance analysis and optimization of reciprocating compressor with stepless capacity control system under variable load conditions. Int. J. Refrig. 2018, 94, 174–185. [Google Scholar] [CrossRef]
- Jin, J.; Hong, W. Valve dynamic and thermal cycle model in stepless capacity regulation for reciprocating compressor. Chin. J. Mech. Eng. 2012, 25, 1151–1160. [Google Scholar] [CrossRef]
- Tang, B.; Zhao, Y.; Li, L.; Wang, L.; Liu, G.; Yang, Q.; Xu, H.; Zhu, F.; Meng, W. Dynamic characteristics of suction valves for reciprocating compressor with stepless capacity control system. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2014, 228, 104–114. [Google Scholar] [CrossRef]
- Liu, G.; Zhao, Y.; Tang, B.; Li, L. Dynamic performance of suction valve in stepless capacity regulation system for large-scale reciprocating compressor. Appl. Therm. Eng. 2016, 96, 167–177. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhou, C.; Wang, Y.; Zhang, J.; Liu, W.; Sun, X. Optimization Design of Actuator Parameters in Multistage Reciprocating Compressor Stepless Capacity Control System Based on NSGA-II. Math. Probl. Eng. 2020, 2020, 7581845. [Google Scholar] [CrossRef]
- Zhang, J.; Zhou, C.; Jiang, Z.; Wang, Y.; Sun, X. Optimization Design of Actuator Parameters with Stepless Capacity Control System Considering the Effect of Backflow Clearance. Appl. Sci. 2020, 10, 2703. [Google Scholar] [CrossRef]
- Sun, X.; Zhang, J.; Wang, Y.; Wang, J.; Qi, Z. Optimization of capacity control of reciprocating compressor using multi-system coupling model. Appl. Therm. Eng. 2021, 195, 117175. [Google Scholar] [CrossRef]
- Li, W. Simplified steady-state modeling for variable speed compressor. Appl. Therm. Eng. 2013, 50, 318–326. [Google Scholar] [CrossRef]
- Qureshi, T.Q.; Tassou, S.A. Variable-speed capacity control in refrigeration systems. Appl. Therm. Eng. 1996, 16, 103–113. [Google Scholar] [CrossRef]
- Aprea, C.; Mastrullo, R.; Renno, C. Experimental analysis of the scroll compressor performances varying its speed. Appl. Therm. Eng. 2006, 26, 983–992. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, Z.; Wang, J.; Jiang, W.; Feng, Q. Research of thermal dynamic characteristics for variable load single screw refrigeration compressor with different capacity control mechanism. Appl. Therm. Eng. 2017, 110, 1172–1182. [Google Scholar] [CrossRef]
- Yaqub, M.; Zubair, S.M. Performance evaluation of hot-gas by-pass capacity control schemes for refrigeration and air-conditioning systems. Energy 2000, 25, 543–561. [Google Scholar] [CrossRef]
- Jeong, S.K.; Lee, D.B.; Hong, K.H. Comparison of system performance on hot-gas bypass and variable speed compressor in an oil cooler for machine tools. J. Mech. Sci. Technol. 2014, 28, 721–727. [Google Scholar] [CrossRef]
- Wang, B.; Han, L.; Shi, W.; Li, X. Modulation method of scroll compressor based on suction gas bypass. Appl. Therm. Eng. 2012, 37, 183–189. [Google Scholar] [CrossRef]
- Hollingsworth, J.; Phillippi, G.; Hinchliff, M.; Kulhanek, C.; Rimpel, A.M.; Maywald, F. Reciprocating Compressors. In Compression Machinery for Oil and Gas; Gulf Professional Publishing: Houston, TX, USA, 2019; pp. 167–252. [Google Scholar]
- Wang, L.; Liu, G.B.; Zhao, Y.Y.; Li, L.L. Performance comparison of capacity control methods for reciprocating compressors. In IOP Conference Series: Materials Science and Engineering, Proceedings of the 9th International Conference on Compressors and Their Systems, London, UK, 7–9 September 2015; IOP Publishing: Bristol, UK, 2015; Volume 90, Number 1; p. 012029. [Google Scholar]
- Yaqub, M.; Zubair, S.M. Thermodynamic analysis of capacity-control schemes for refrigeration and air-conditioning systems. Energy 1996, 21, 463–472. [Google Scholar] [CrossRef]
- Holdack-Janssen, H.; Kruse, H. Continuous and discontinuous capacity control for high speed refrigeration compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 11–13 July 1984; Purdue e-Pubs: West Lafayette, IN, USA, 1984. [Google Scholar]
- Ooi, K.T.; Wong, T.N. A computer simulation of a rotary compressor for household refrigerators. Appl. Therm. Eng. 1997, 17, 65–78. [Google Scholar] [CrossRef]
- Sultan, I.A. A surrogate model for interference prevention in the limaçon-to-limaçon machines. Eng. Comput. 2007, 24, 437–449. [Google Scholar] [CrossRef]
- Jain, A.K.; Mao, J.; Mohiuddin, K.M. Artificial neural networks: A tutorial. Computer 1996, 29, 31–44. [Google Scholar] [CrossRef]
- Sanaye, S.; Dehghandokht, M.; Mohammadbeigi, H.; Bahrami, S. Modeling of rotary vane compressor applying artificial neural network. Int. J. Refrig. 2011, 34, 764–772. [Google Scholar] [CrossRef]
- Namdeo, R.; Manepatil, S.; Saraswat, S. Detection of valve leakage in reciprocating compressor using artificial neural network (ANN). In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 2008; Purdue e-Pubs: West Lafayette, IN, USA, 2008. [Google Scholar]
- Belman-Flores, J.M.; Ledesma, S.; Barroso-Maldonado, J.M.; Navarro-Esbrí, J. A comparison between the modeling of a reciprocating compressor using artificial neural network and physical model. Int. J. Refrig. 2015, 59, 144–156. [Google Scholar] [CrossRef]
- Tian, Z.; Gu, B.; Yang, L.; Lu, Y. Hybrid ANN–PLS approach to scroll compressor thermodynamic performance prediction. Appl. Therm. Eng. 2015, 77, 113–120. [Google Scholar] [CrossRef]
- Zendehboudi, A.; Li, X.; Wang, B. Utilization of ANN and ANFIS models to predict variable speed scroll compressor with vapor injection. Int. J. Refrig. 2017, 74, 475–487. [Google Scholar] [CrossRef]
- Whitley, D. A genetic algorithm tutorial. Stat. Comput. 1994, 4, 65–85. [Google Scholar] [CrossRef]
- Huang, Y.M.; Tsay, S.N. Mechanical efficiency optimization of a sliding vane rotary compressor. J. Press. Vessel Technol. 2009, 131, 061601. [Google Scholar] [CrossRef]
- Chen, Q.J.; Ooi, K.T. Geometric Optimisation of a Coupled Vane Compressor. In IOP Conference Series: Materials Science and Engineering, Proceedings of the 12th International Conference on Compressors and Their Systems (Compressors 2021), London, UK, 6–8 September 2021; IOP Publishing: Bristol, UK, 2021; Volume 1180, Number 1; p. 012028. [Google Scholar]
- Silva, E.; Dutra, T. Piston trajectory optimization of a reciprocating compressor. Int. J. Refrig. 2021, 121, 159–167. [Google Scholar] [CrossRef]
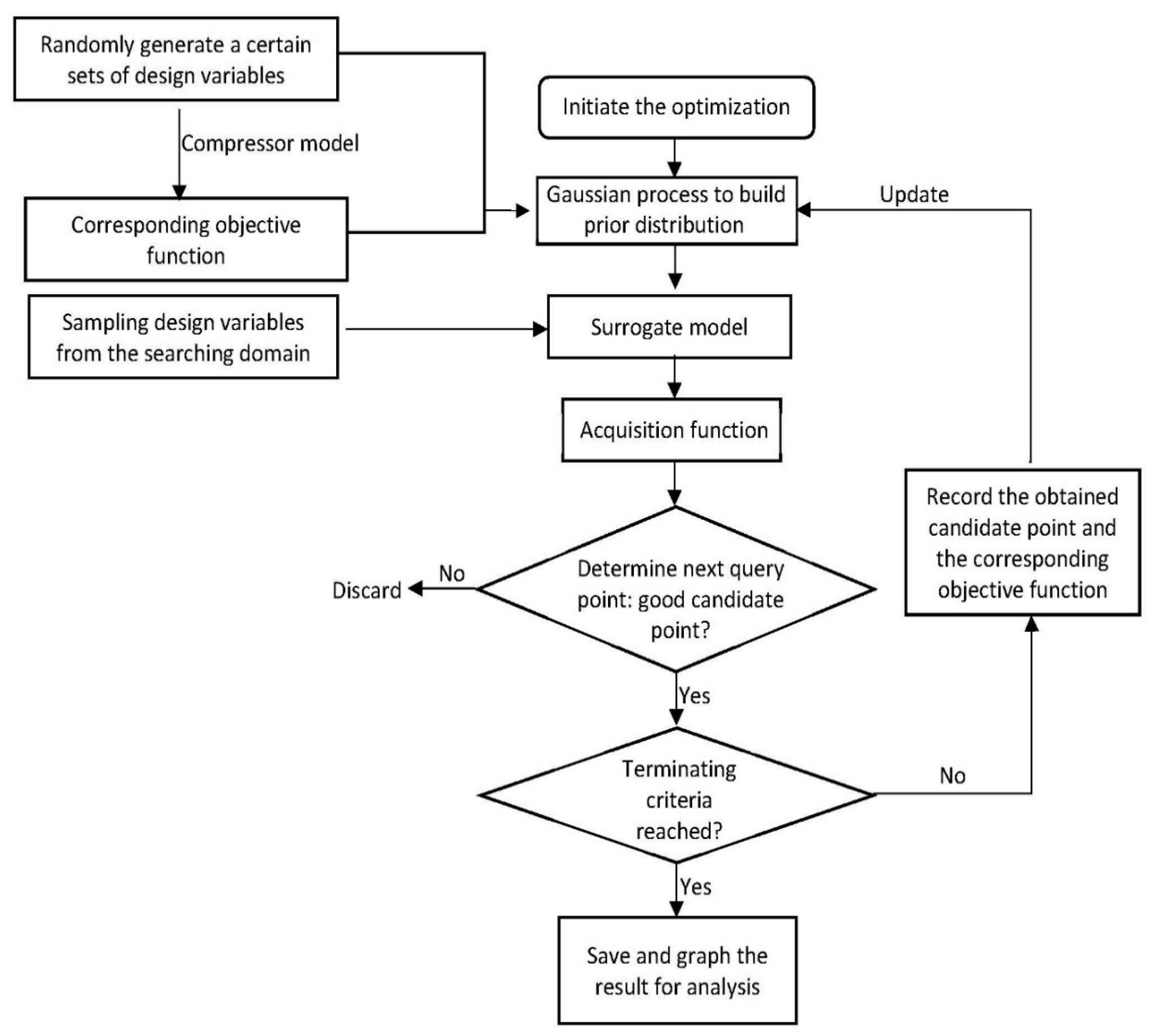
- Lu, K.; Phung, T.H.; Sultan, I.A. On the Design of a Class of Rotary Compressors Using Bayesian Optimization. Machines 2021, 9, 219. [Google Scholar] [CrossRef]
- Lu, K.; Sultan, I.; Phung, T. Geometric design of the limaçon rotary compressor using Bayesian optimization. In AIP Conference Proceedings; AIP Publishing: New York, NY, USA, 2022; Volume 2681, Number 1. [Google Scholar]
- Sultan, I.A.; Kalim, A. Improving reciprocating compressor performance using a hybrid two-level optimisation approach. Eng. Comput. 2011, 28, 616–636. [Google Scholar] [CrossRef]
- Phung, T.H.; Sultan, I.A. Geometric design of the limaçon-to-circular fluid processing machine. J. Mech. Des. 2021, 143, 103501. [Google Scholar] [CrossRef]
- Qi, G.; Zhu, Z.; Erqinhu, K.; Chen, Y.; Chai, Y.; Sun, J. Fault-diagnosis for reciprocating compressors using big data and machine learning. Simul. Model. Pract. Theory 2018, 80, 104–127. [Google Scholar] [CrossRef]
- Lu, K.; Sultan, I.A.; Phung, T.H. A study on the use of machine learning methods to improve reciprocating compressor reliability via torque tailoring. In Proceedings of the 2021 International Conference on Maintenance and Intelligent Asset Management (ICMIAM), Ballarat, Australia, 12–15 December 2021; IEEE: New York, NY, USA, 2021; pp. 1–6. [Google Scholar]
- Ma, J.; Ding, X.; Horton, W.T.; Ziviani, D. Development of an automated compressor performance mapping using artificial neural network and multiple compressor technologies. Int. J. Refrig. 2020, 120, 66–80. [Google Scholar] [CrossRef]
- AHRI. 2020 Standard for Performance Rating of Positive Displacement Refrigerant Compressors; AHRI: Washington, DC, USA, 2020. [Google Scholar]
- Afshari, F.; Comakli, O.; Adiguzel, N.; Ghasemi Zavaragh, H. Influence of refrigerant properties and charge amount on performance of reciprocating compressor in air source heat pump. J. Energy Eng. 2017, 143, 04016025. [Google Scholar] [CrossRef]
- Karnaz, J.; Seeton, C.; Dixon, L. Identifying lubricant options for compressor bearing designs. In IOP Conference Series: Materials Science and Engineering, Proceedings of the 10th International Conference on Compressors and Their Systems, City, University of London, 11–13 September 2017; IOP Publishing: Bristol, UK, 2017; Volume 232, Number 1; p. 012092. [Google Scholar]
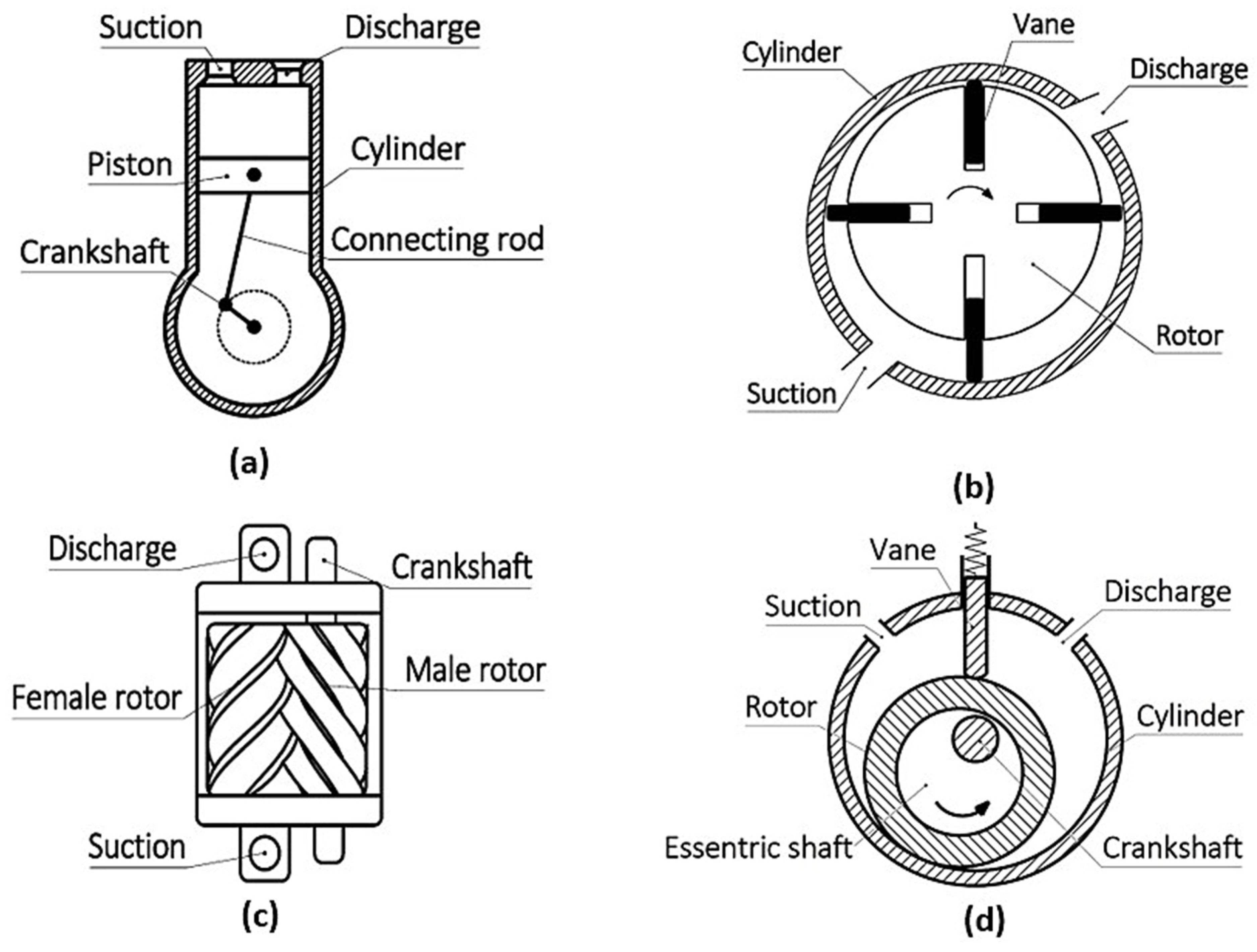
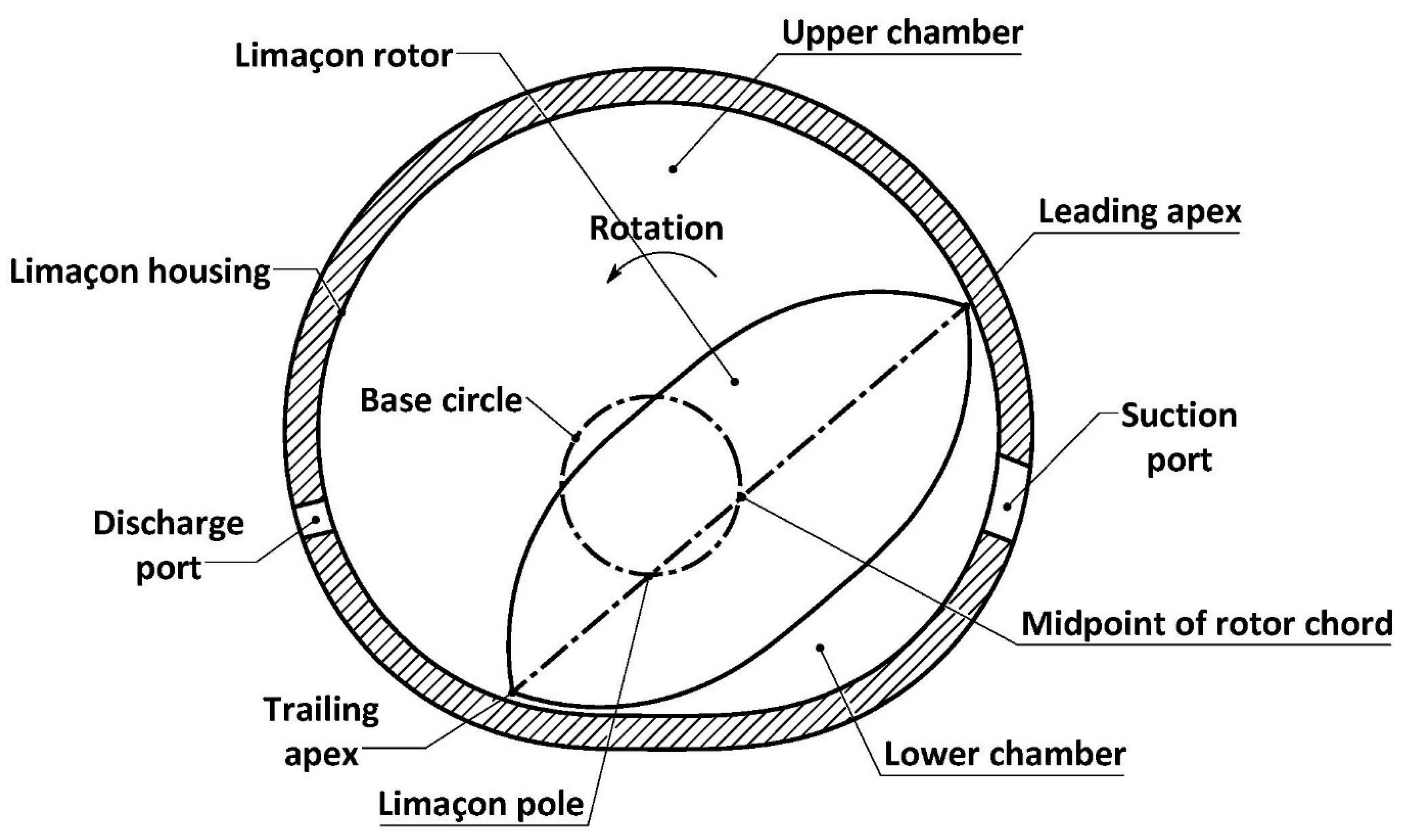
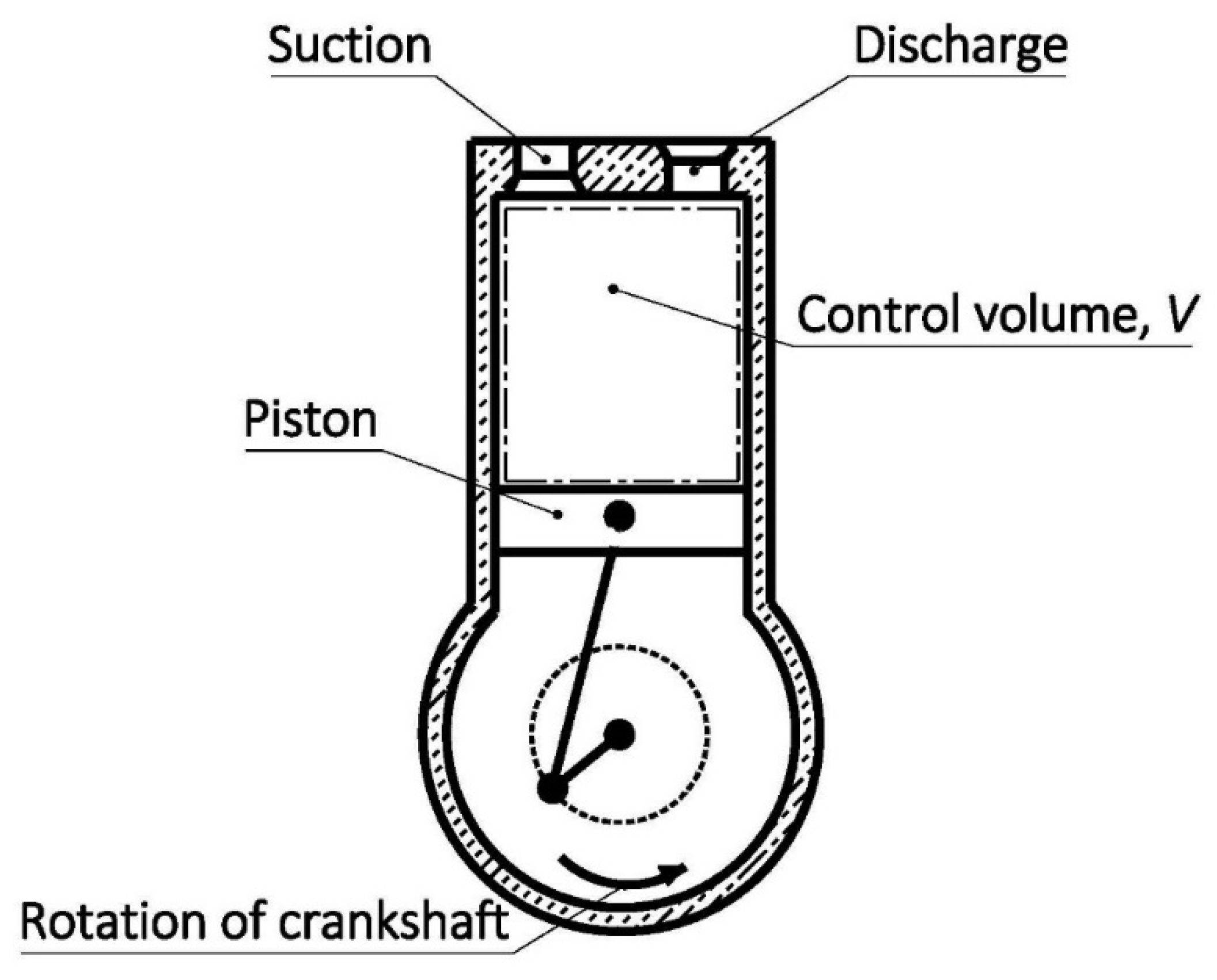

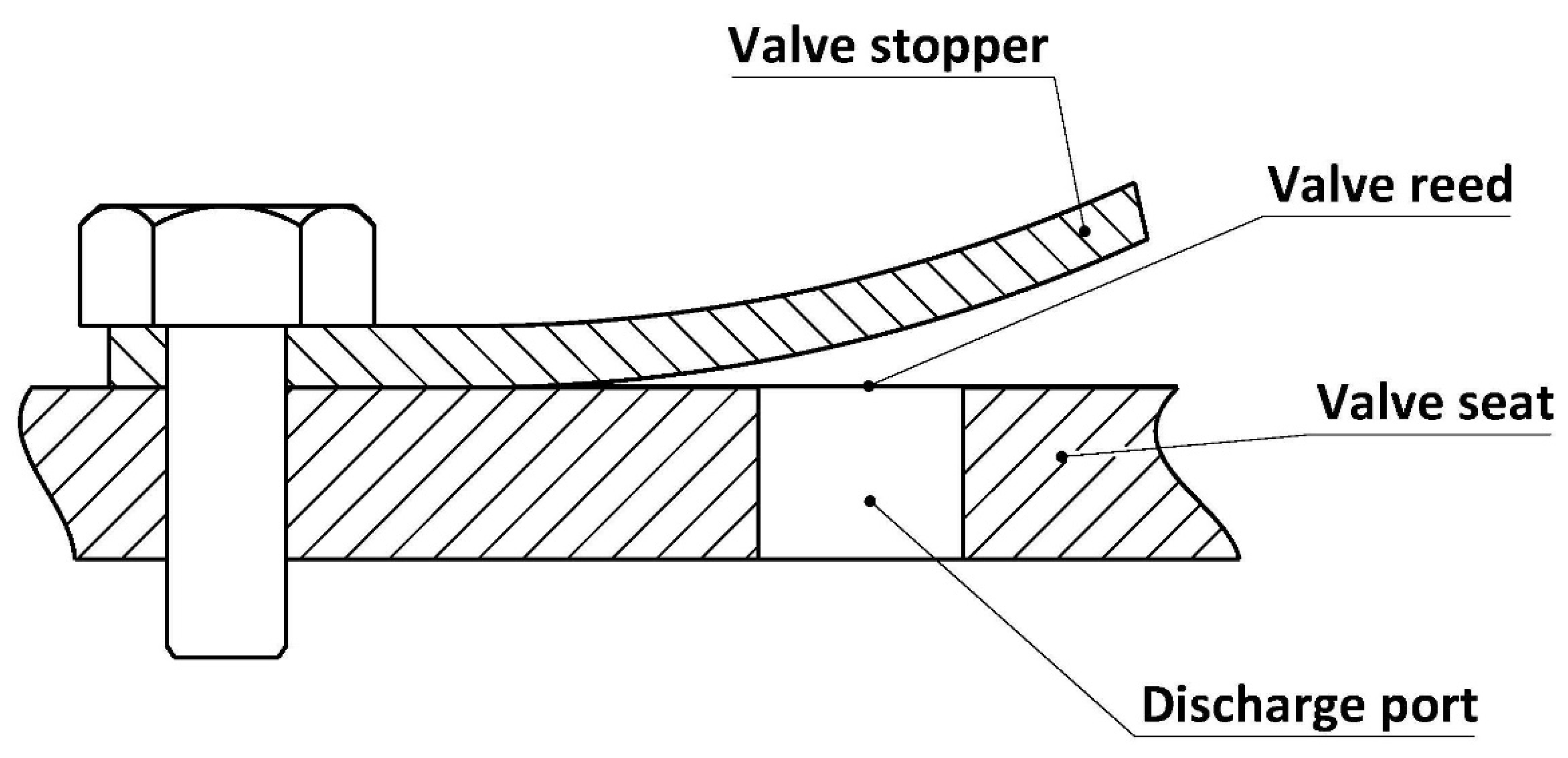

| Type | Operating Features | Mechanism | Pros and Cons |
|---|---|---|---|
| Reciprocating | Displacing element moves reciprocally and linearly | Piston | Pros:
|
| Diaphragm | Pros:
| ||
| Linear | Pros:
| ||
| Rotary | Displacing element features circular or near-circular motion | Rolling piston | Pros:
|
| Vane | Pros:
| ||
| Screw | Pros:
| ||
| Scroll | Pros:
|
| Reference | Type | The Main Features of the Design |
|---|---|---|
| Heidari et al. [14] | Reciprocating |
|
| Hu et al. [15] | Rotary |
|
| Shin et al. [16] | Rotary |
|
| Shakya and Ooi [17] | Rotary |
|
| Gao and Liu [18] | Reciprocating |
|
| Lu et al. [19] | Rotary |
|
| Leakage Model | Features | References |
|---|---|---|
| Isentropic flow model |
| [23,24,25,26] |
| Fanno flow model |
| [28,29,30,31,32,33,34] |
| Incompressible flow model |
| [35,36,37] |
| References | Nusselt Number Correlation | Application | |
|---|---|---|---|
| Adair et al. [39] | Nu = 0.053Re0.8Pr0.6 | Reciprocating compressor | |
| Liu and Zhou [40] | Nu = 0.75Re0.8Pr0.6 | Reciprocating compressor | |
| Hsieh and Wu [42] | Pure compression | Reciprocating compressor | |
| Compression with discharge | where is the Reynolds number based on the velocity at the beginning of the discharge process | ||
| Pure expansion | |||
| Expansion with suction | |||
| Disconzi et al. [43] | Suction | Nu = 0.08Re0.9Pr0.6 | Reciprocating compressor |
| Compression | Nu = 0.08Re0.8Pr0.6 | ||
| Discharge | Nu = 0.08Re0.8Pr0.6 | ||
| Expansion | Nu = 0.012Re0.8Pr0.6 | ||
| Jang and Jeong [45] | where Dc is the diameter of the curvature, and St is the Strouhal number | Scroll compressor | |
| Methods | Principle | Pros and Cons | References |
|---|---|---|---|
| Stepless control | The closing of the suction valve is delayed by an external force, allowing excess fluid to flow out of the working chamber | Pros:
| [81,82,83,84,85,86,87,88,89,90] |
| Speed control | Operating speed is regulated to match the working load | Pros:
| [91,92,93,94] |
| Bypass | Compressed fluid is bypassed to the suction line before delivering, thus reducing the capacity | Pros:
| [95,96,97] |
| Clearance pocket | Additional clearance volume is introduced to the working chamber | Pros:
| [98,99,100,101] |
| Suction throttling | Reduce the suction pressure by throttling the fresh charge via the control valve at the suction | Pros:
| [98,99,100,101] |
| Methods | Type of Compressor | Applications | References |
|---|---|---|---|
| ANN | Sliding vane | Detection of valve failure | [105] |
| Reciprocating | Mathematical modelling | [106,107] | |
| Scroll | Performance prediction | [108,109] | |
| GA | Sliding vane | Improvement of mechanical efficiency | [111] |
| Coupled vane | Optimisation of rotor-chamber configuration | [112] | |
| Reciprocating | Optimisation of valve actuator and piston trajectory | [88,89,113] | |
| Bayesian optimisation | Limaçon (rotary) | Optimisation of port geometry | [114,115] |
| SPSA | Reciprocating | Optimisation of piston trajectory | [116] |
| Limaçon (rotary) | Machine geometric design | [117] | |
| PCA | Reciprocating | Fault diagnosis | [118] |
| Reliability improvement | [119] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, K.; Sultan, I.A.; Phung, T.H. A Literature Review of the Positive Displacement Compressor: Current Challenges and Future Opportunities. Energies 2023, 16, 7035. https://doi.org/10.3390/en16207035
Lu K, Sultan IA, Phung TH. A Literature Review of the Positive Displacement Compressor: Current Challenges and Future Opportunities. Energies. 2023; 16(20):7035. https://doi.org/10.3390/en16207035
Chicago/Turabian StyleLu, Kui, Ibrahim A. Sultan, and Truong H. Phung. 2023. "A Literature Review of the Positive Displacement Compressor: Current Challenges and Future Opportunities" Energies 16, no. 20: 7035. https://doi.org/10.3390/en16207035
APA StyleLu, K., Sultan, I. A., & Phung, T. H. (2023). A Literature Review of the Positive Displacement Compressor: Current Challenges and Future Opportunities. Energies, 16(20), 7035. https://doi.org/10.3390/en16207035







