Direct Driven Hydraulic System for Skidders
Abstract
:1. Introduction
2. Analysis of the Static Cylinder Force of Skidder Rear Plate
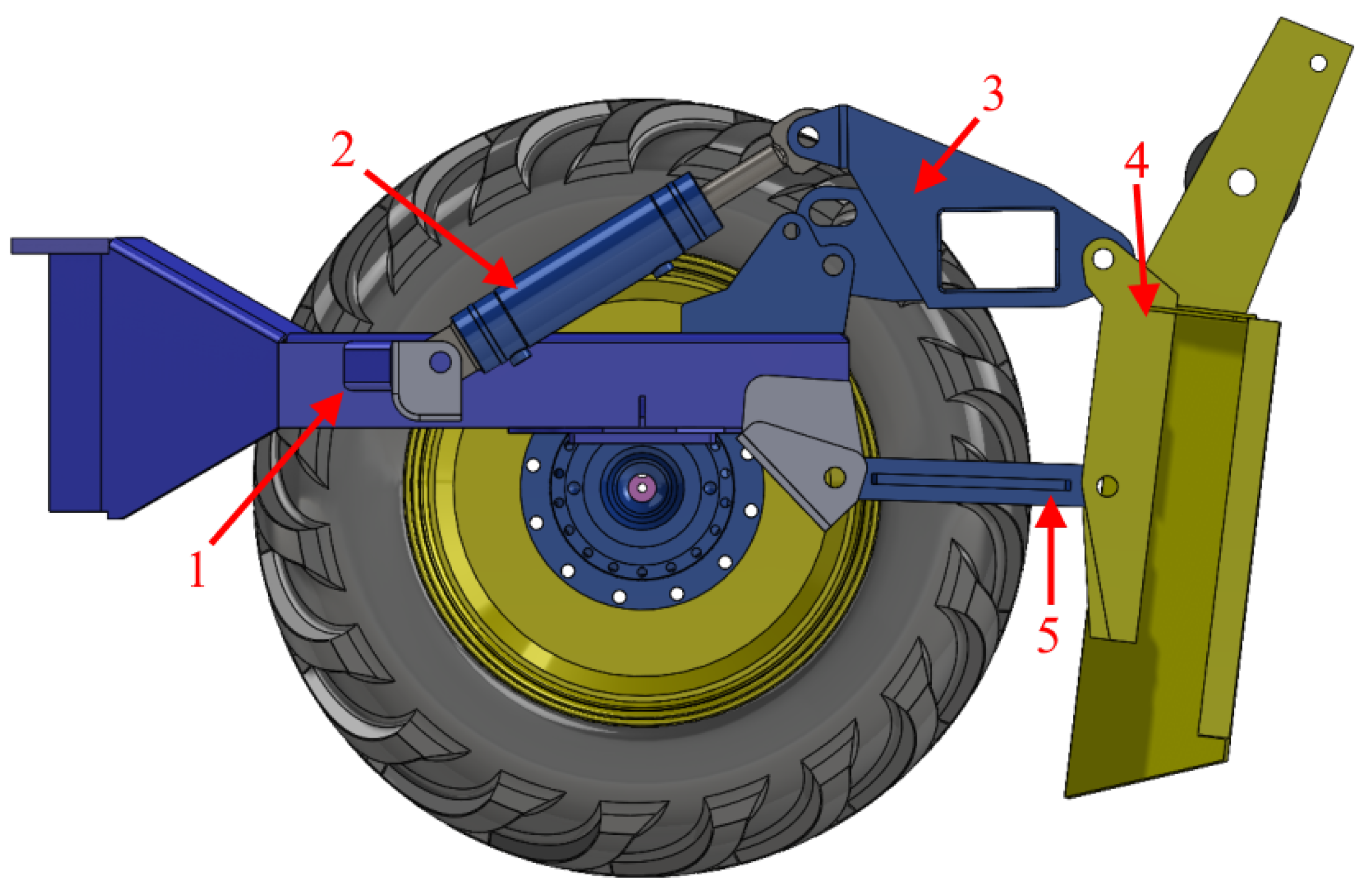
2.1. Outline of the Skidder Rear Plate Mechanical System
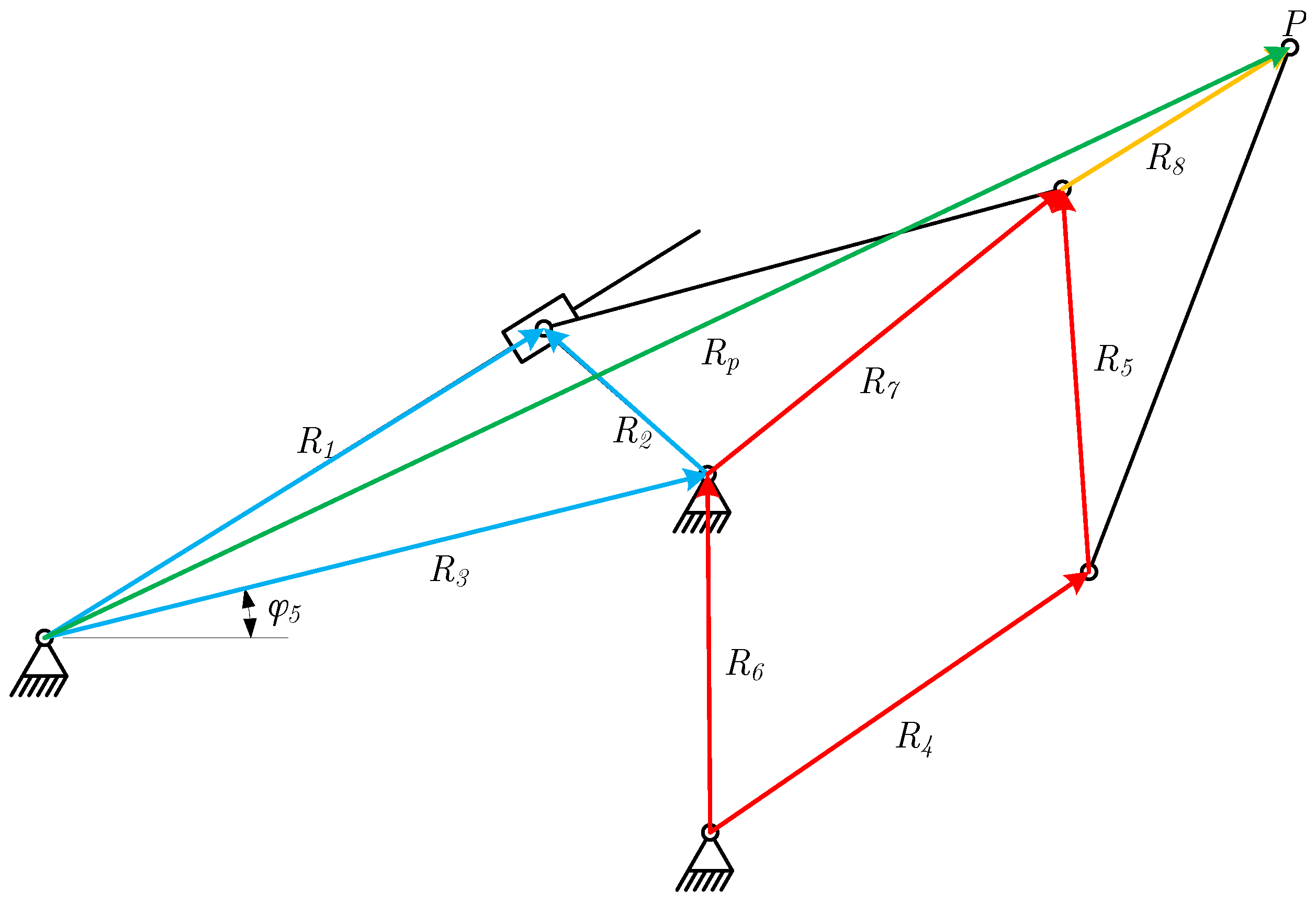
2.2. Position Analysis
2.3. Virtual Work
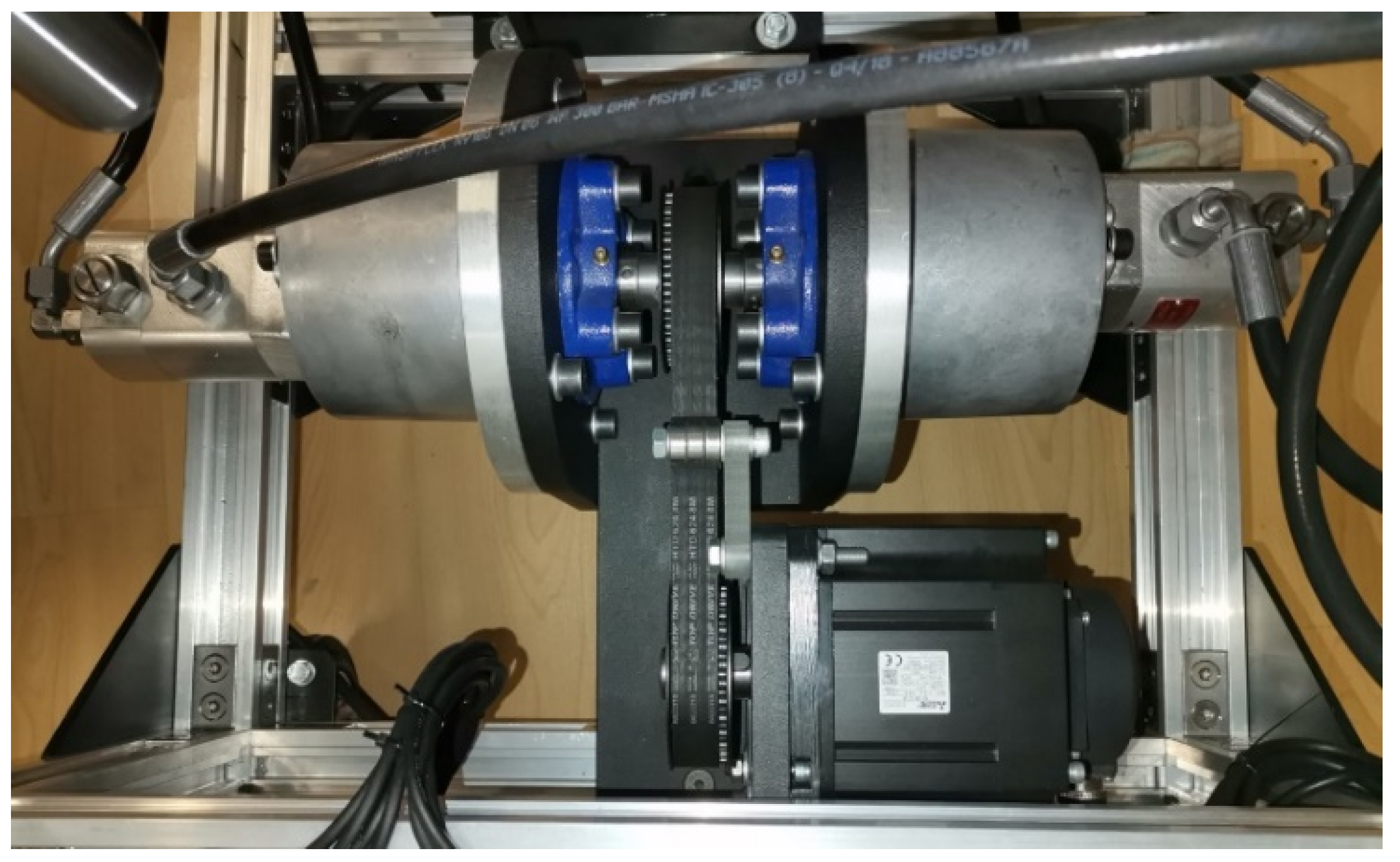
3. Experimental Setup
4. Experimental Investigation into Energy Efficiency
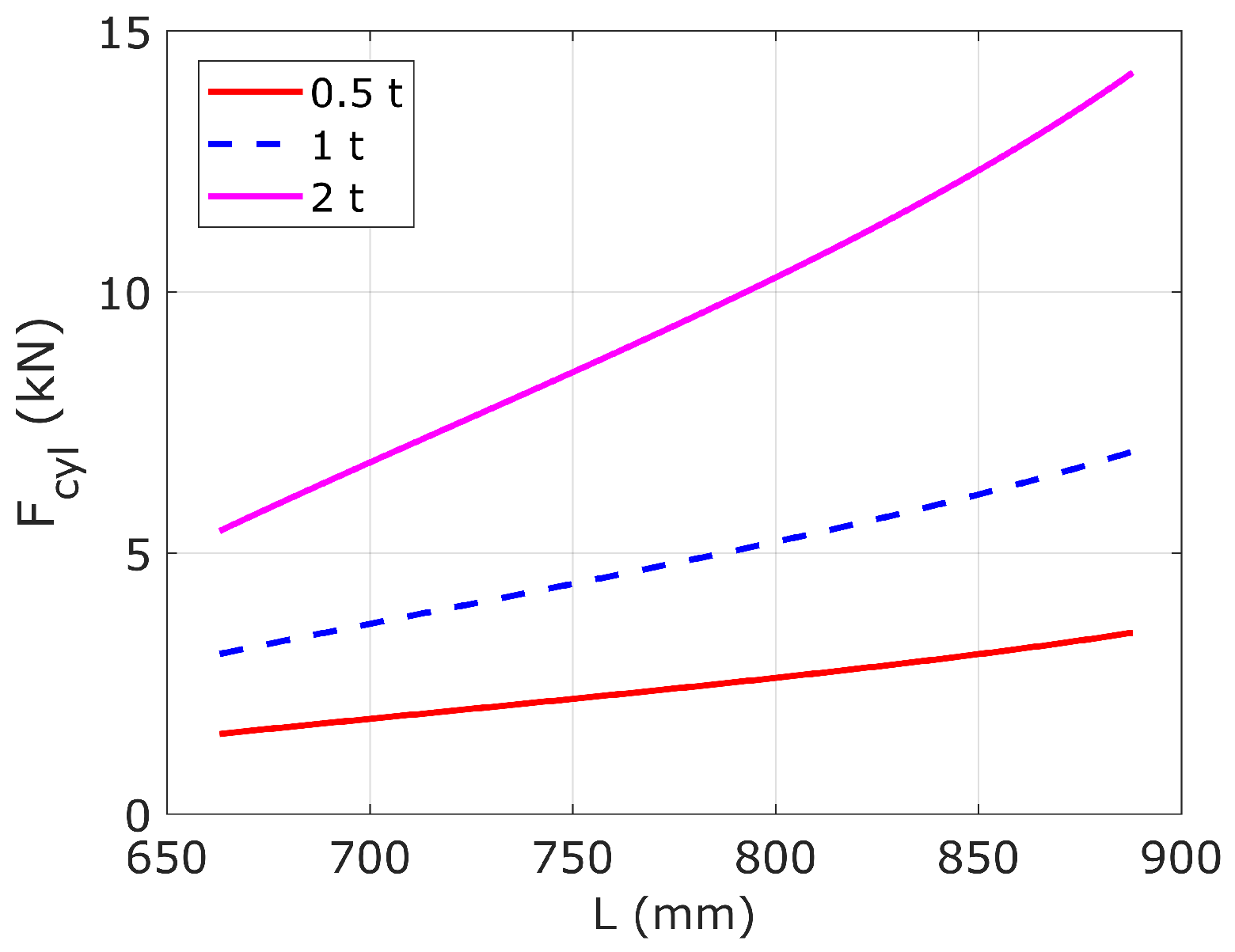
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Description |
| , | piston areas |
| current fuel price | |
| input energy | |
| output energy | |
| cylinder force | |
| horizontal force | |
| vertical force | |
| equivalent fuel consumption | |
| fuel price | |
| L | cylinder length |
| link length | |
| , | x and y position of point P |
| n | lifting cycles per day |
| , | pressure inside cylinder chambers |
| input power of the system | |
| cylinder power | |
| q | specific fuel consumption |
| position vectors | |
| v | piston velocity |
| W | virtual work |
| workdays per year | |
| , , | angels of triangle links |
| link angle | |
| fuel density |
References
- Fonseca, A.; Aghazadeh, F.; de Hoop, C.; Ikuma, L.; Al-Qaisi, S. Effect of noise emitted by forestry equipment on workers’ hearing capacity. Int. J. Ind. Ergon. 2015, 46, 105–112. [Google Scholar] [CrossRef]
- Potočnik, I.; Poje, A. Forestry ergonomics and occupational safety in high ranking scientific journals from 2005–2016. Croat. J. For. Eng. J. Theory Appl. For. Eng. 2017, 38, 291–310. [Google Scholar]
- Rong-Feng, S.; Xiaozhen, Z.; Chengjun, Z. Study on Drive System of Hybrid Tree Harvester. Sci. World J. 2017, 2017, 8636204. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Karlušić, J.; Cipek, M.; Pavković, D.; Benić, J.; Šitum, Ž.; Pandur, Z.; Šušnjar, M. Simulation Models of Skidder Conventional and Hybrid Drive. Forests 2020, 11, 921. [Google Scholar] [CrossRef]
- Karlušić, J.; Cipek, M.; Pavković, D.; Šitum, Ž.; Benić, J.; Šušnjar, M. Benefit Assessment of Skidder Powertrain Hybridization Utilizing a Novel Cascade Optimization Algorithm. Sustainability 2020, 12, 10396. [Google Scholar] [CrossRef]
- Knežević, I. Preoblikovanje Razvodnika Dnage šumskog Traktora za Pogon svih kotača i Vitla (Reshaping Power Distributor of Skidder to Drive all the Wheels and Winches). Master’s Thesis, University of Zagreb, Zagreb, Croatia, 2010. [Google Scholar]
- Gillich, N.; Sîrbu, N.; Vlase, S.; Marin, M. Study of Metallic Housing of the Adder Gearbox to Reduce the Noise and to Improve the Design Solution. Metals 2021, 11, 912. [Google Scholar] [CrossRef]
- Solazzi, L. Design and experimental tests on hydraulic actuator made of composite material. Compos. Struct. 2020, 232, 111544. [Google Scholar] [CrossRef]
- Torregrossa, D.; Capitanescu, F. Optimization models to save energy and enlarge the operational life of water pumping systems. J. Clean. Prod. 2019, 213, 89–98. [Google Scholar] [CrossRef]
- Bagirov, A.; Ahmed, S.; Barton, A.; Mala-Jetmarova, H.; Al Nuaimat, A.; Sultanova, N.; GWMWater, H. Comparison of metaheuristic algorithms for pump operation optimization. In Proceedings of the Water Distribution Systems Analysis Conference, Adelaide, Australia, 24–27 September 2012; pp. 886–896. [Google Scholar]
- Benić, J.; Šitum, Ž. Position Controller for Direct Driven Electro-Hydraulic System. In Proceedings of the International Conference Fluid Power 2019: Conference Proceedings, Tampere, Finland, 22–24 May 2019. [Google Scholar] [CrossRef]
- Agostini, T.; Negri, V.D.; Minav, T.; Pietola, M. Effect of Energy Recovery on Efficiency in Electro-Hydrostatic Closed System for Differential Actuator. Actuators 2020, 9, 12. [Google Scholar] [CrossRef] [Green Version]
- Turunen, A.; Minav, T.; Hanninen, H.; Pietola, M. Experimental Investigation of Direct Drive Hydraulic Units Implemented in a Mining Loader. In Proceedings of the 2018 Global Fluid Power Society PhD Symposium (GFPS), Samara, Russia, 18–20 July 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Cerman, O.; Hušek, P. Adaptive fuzzy sliding mode control for electro-hydraulic servo mechanism. Expert Syst. Appl. 2012, 39, 10269–10277. [Google Scholar] [CrossRef]
- Cukla, A.R.; Izquierdo, R.; Borges, F.A.; Perondi, E.A.; Lorini, F.J. Optimum Cascade Control Tuning of a Hydraulic Actuator Based on Firefly Metaheuristic Algorithm. IEEE Lat. Am. Trans. 2018, 16, 384–390. [Google Scholar] [CrossRef]
- dos Santos Coelho, L.; Cunha, M.A.B. Adaptive cascade control of a hydraulic actuator with an adaptive dead-zone compensation and optimization based on evolutionary algorithms. Expert Syst. Appl. 2011, 38, 12262–12269. [Google Scholar] [CrossRef]
- Wang, R.; Tan, C.; Xu, J.; Wang, Z.; Jin, J.; Man, Y. Pressure Control for a Hydraulic Cylinder Based on a Self-Tuning PID Controller Optimized by a Hybrid Optimization Algorithm. Algorithms 2017, 10, 19. [Google Scholar] [CrossRef] [Green Version]
- Niraula, A.; Zhang, S.; Minav, T.; Pietola, M. Effect of Zonal Hydraulics on Energy Consumption and Boom Structure of a Micro-Excavator. Energies 2018, 11, 2088. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.; Minav, T.; Pietola, M. Decentralized Hydraulics for Micro Excavator. In Proceedings of the 15th Scandinavian International Conference on Fluid Power (SICFP’17), Linköping, Sweden, 7–9 June 2017; pp. 187–195. [Google Scholar]
- Minav, T.; Bonato, C.; Sainio, P.; Pietola, M. Direct Driven Hydraulic Drive. In Proceedings of the 9th International Fluid Power Conference (9. IFK), Aachen, Germany, 24–26 March 2014; pp. 508–517. [Google Scholar]
- Minav, T.; Heikkinen, J.; Schimmel, T.; Pietola, M. Direct Driven Hydraulic Drive: Effect of Oil on Efficiency in Sub-Zero Conditions. Energies 2019, 12, 219. [Google Scholar] [CrossRef] [Green Version]
- Benić, J.; Šitum, Ž.; Cipek, M.; Pavković, D.; Kasać, J. Comparison of Sliding Mode Controller for Classical and Direct Driven Electrohydraulic System. In Proceedings of the Scandinavian International Conference on Fluid Power, Linköping, Sweden, 31 May–2 June 2021; pp. 26–35. [Google Scholar]
- Benić, J.; Karlušić, J.; Šitum, Ž.; Cipek, M.; Pavković, D. Potential Use of Novel Efficient Direct Driven Electro-Hydraulic System for Skidders. In Proceedings of the 16th Conference on Sustainable Development of Energy, Water and Environment Systems (SDEWES), Dubrovnik, Croatia, 10–15 October 2021. [Google Scholar]
- Prasanna, G.K. Geometric performance parameters of three-point hitch linkage system of a 2WD Indian tractor. Res. Agric. Eng. 2016, 61, 47–53. [Google Scholar] [CrossRef] [Green Version]
- Pan, Y.C.; Cai, G.W.; Zhang, Z.; Chen, Y.; Li, Y.Z. Kinematics and Kinetostatic Analysis of a New Type of Mechanical Excavator with Controllable Mechanism. Appl. Mech. Mater. 2011, 141, 334–338. [Google Scholar]
- Hitner d.o.o. Available online: http://hittner.hr/ (accessed on 23 November 2021).
- Development of Hybrid Skidder: HiSkid. Available online: https://www.hiskid.hr/ (accessed on 23 November 2021).
- Norton, R. Design of Machinery: An Introduction to the Synthesis and Analysis of Mechanisms and Machines; McGraw-Hill Higher Education: Boston, MA, USA, 2004. [Google Scholar]
- Đuka, A.; Pentek, T.; Horvat, D.; Poršinsky, T. Modelling of Downhill timber skidding: Bigger load–bigger slope. Croat. J. For. Eng. J. Theory Appl. For. Eng. 2016, 37, 139–150. [Google Scholar]
- Kasac, J.; Majetic, D.; Brezak, D. An algebraic approach to on-line signal denoising and derivatives estimation. J. Frankl. Inst. 2018, 355, 7799–7825. [Google Scholar] [CrossRef] [Green Version]
- MELSEC iQ-F Series. Available online: https://www.mitsubishielectric.com/fa/products/cnt/plcf/items/index.html (accessed on 23 November 2021).




| Component | Model |
|---|---|
| Cylinder | Hidromehanika |
| Valves (2/2 and 4/2) | ARGO-HYTOS SD2E-A2/L2I12M2-A |
| Proportional valve | ARGO-HYTOS PRM2-063Z11/8-24EKBK1N2V-A 4/3 |
| A—side pump | ARGO-HYTOS GP1-4.8B-SAVB-SGBGB-N002 |
| B—side pump | ARGO-HYTOS GP1-2.5B-SAVB-SGBGB-N002 |
| Power pack pump | Hydronit gear pump 3.7 cm3/rev |
| AC motor | Končar 5AZCD 90SB-4 B14 F115 1.1 kW |
| Servo motor | Mitsubishi electric servo motor HG-SN152JK |
| Motor controller | Mitsubishi electric servo drive MR-JE-200A |
| Control unit | Mitsubishi electric PLC FX5U-32MT/ESS |
| Pressure sensors | HYDAC HDA 7446-A-100-000 |
| Flow sensors | HYDAC EVS 3106-A-0020-000 |
| Linear position sensor | MICRO-EPSILON WDS-500-P60-SR-U |
| F (kN) | 1.6 | 2 | 2.6 | 3 | |
|---|---|---|---|---|---|
| Classical system | (kJ) | 77.8 | 78.7 | 80 | 80 |
| (kJ) | 1.4 | 1.7 | 2.3 | 2.5 | |
| DDH system | (kJ) | 11.4 | 12.3 | 15.1 | 16.2 |
| (kJ) | 1.4 | 1.7 | 2.3 | 2.5 |
| (kJ) | Fuel Consumption (L) | Lowering/Lifting Cycles per Day | Workdays per Year | Fuel Price (EUR) | |
|---|---|---|---|---|---|
| Classical system | 80 | 0.0056 | 54 | 250 | 105.84 |
| DDH system | 16.2 | 0.0011 | 54 | 250 | 20.79 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Benić, J.; Karlušić, J.; Šitum, Ž.; Cipek, M.; Pavković, D. Direct Driven Hydraulic System for Skidders. Energies 2022, 15, 2321. https://doi.org/10.3390/en15072321
Benić J, Karlušić J, Šitum Ž, Cipek M, Pavković D. Direct Driven Hydraulic System for Skidders. Energies. 2022; 15(7):2321. https://doi.org/10.3390/en15072321
Chicago/Turabian StyleBenić, Juraj, Juraj Karlušić, Željko Šitum, Mihael Cipek, and Danijel Pavković. 2022. "Direct Driven Hydraulic System for Skidders" Energies 15, no. 7: 2321. https://doi.org/10.3390/en15072321
APA StyleBenić, J., Karlušić, J., Šitum, Ž., Cipek, M., & Pavković, D. (2022). Direct Driven Hydraulic System for Skidders. Energies, 15(7), 2321. https://doi.org/10.3390/en15072321








