Heat Transfer and Flow of a Gel Fuel in Corrugated Channels
Abstract
1. Introduction
2. Methods
2.1. Mathematical Model
2.1.1. Governing Equations
2.1.2. Constitutive Equations
2.1.3. Expanded Form of the Governing Equations
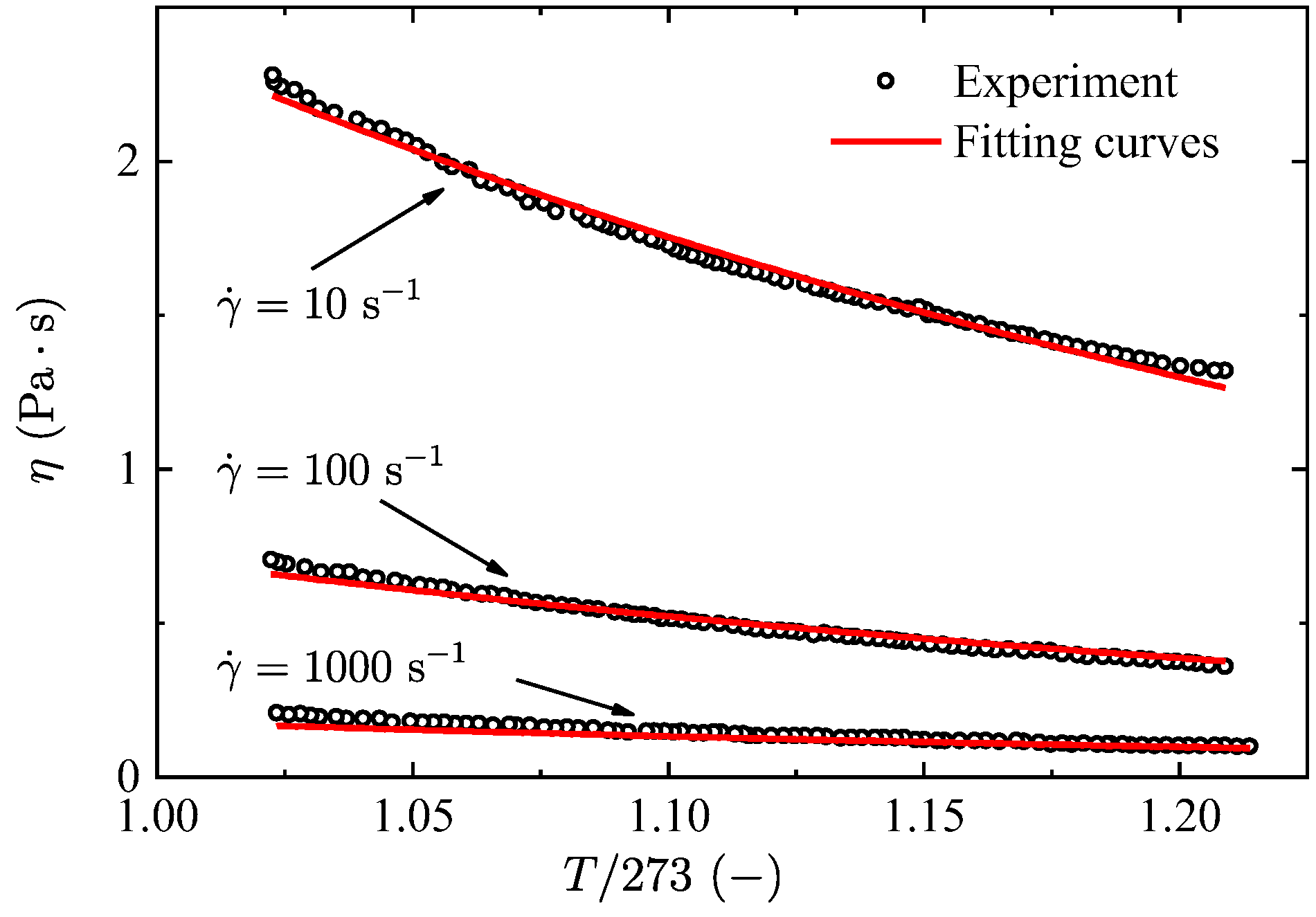
2.2. Parameters in the Constitutive Equation
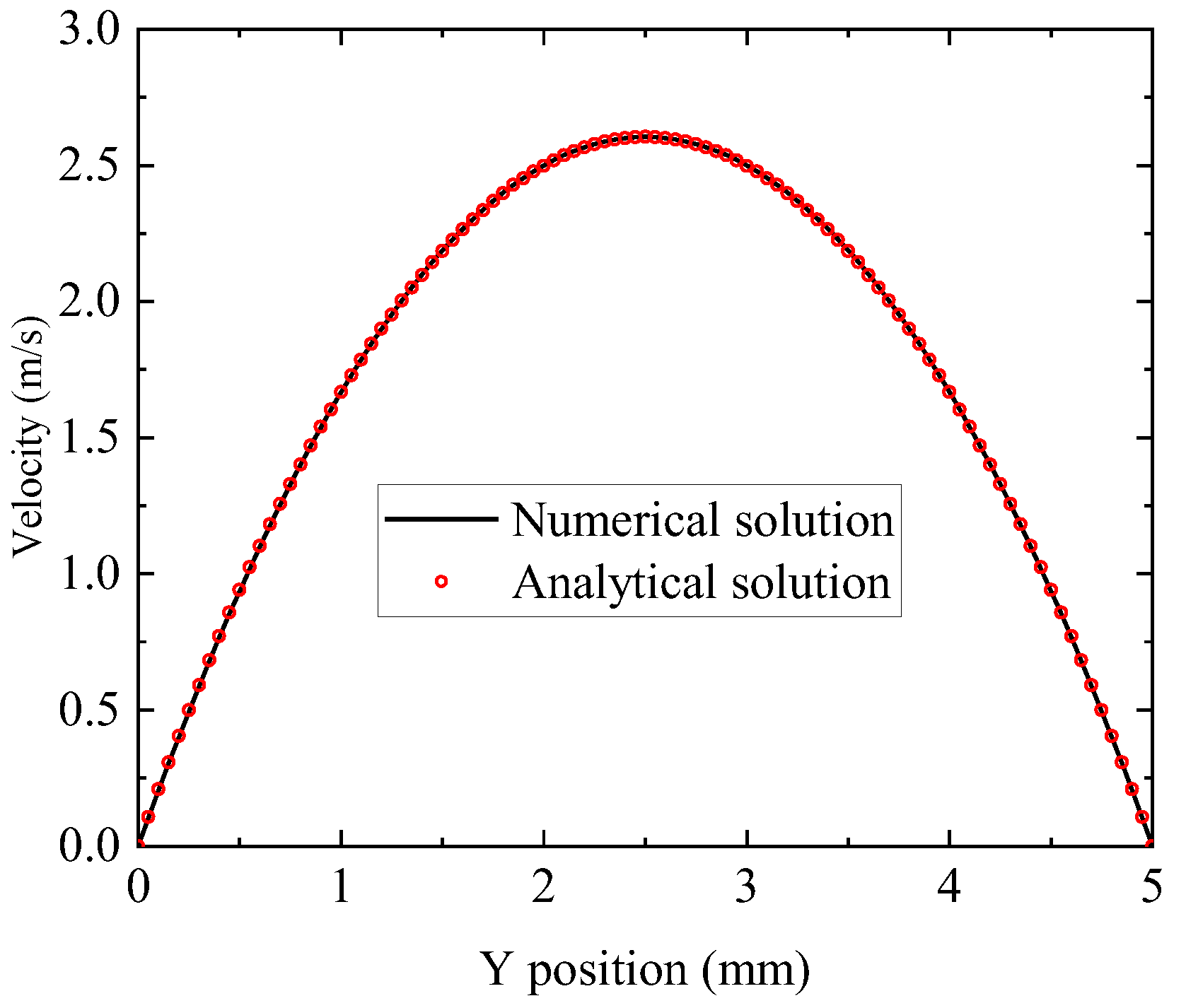
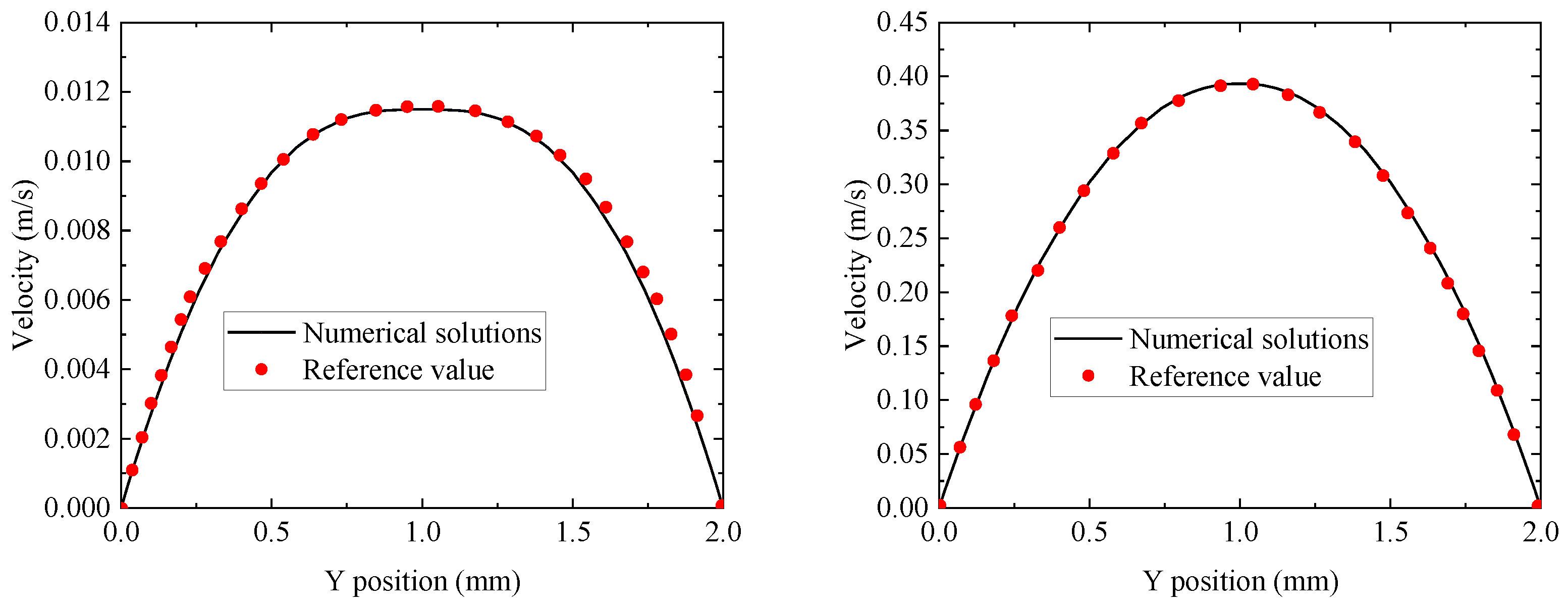
3. Problem Descriptions
4. Results and Discussion
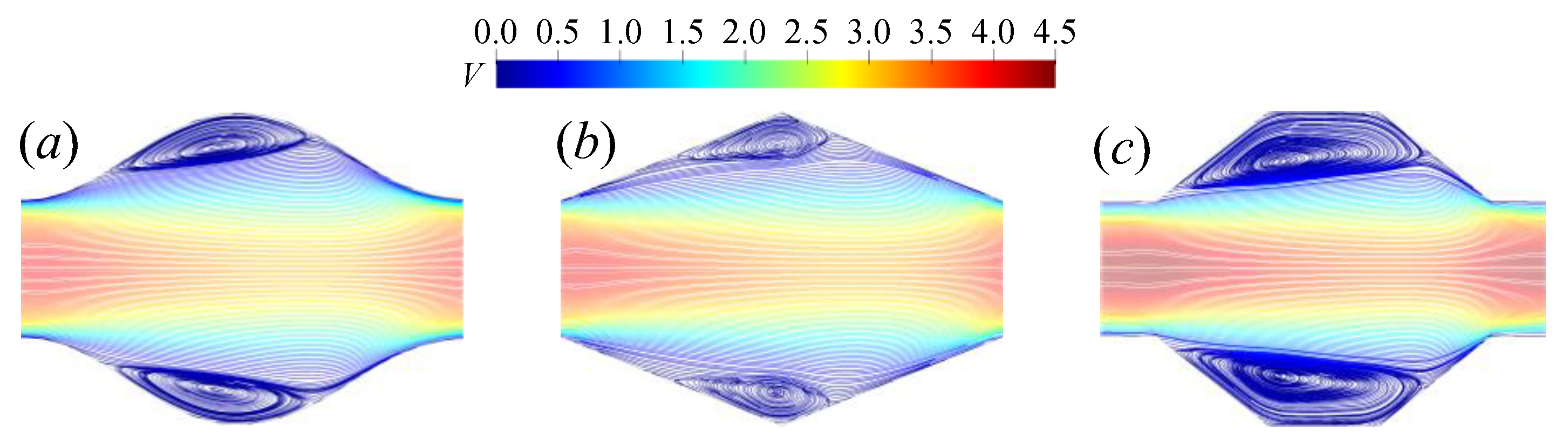
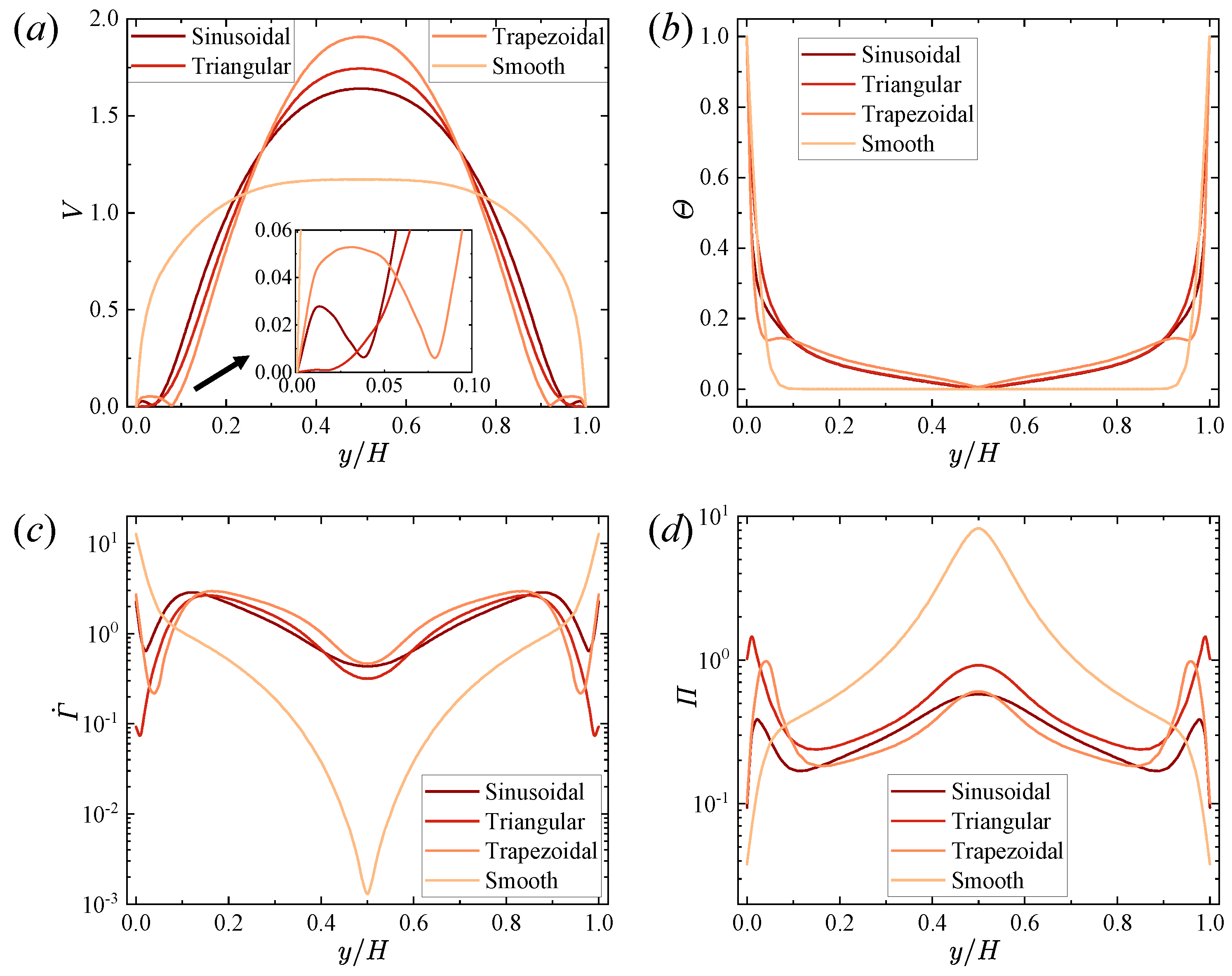
4.1. Effect of Corrugation Profiles
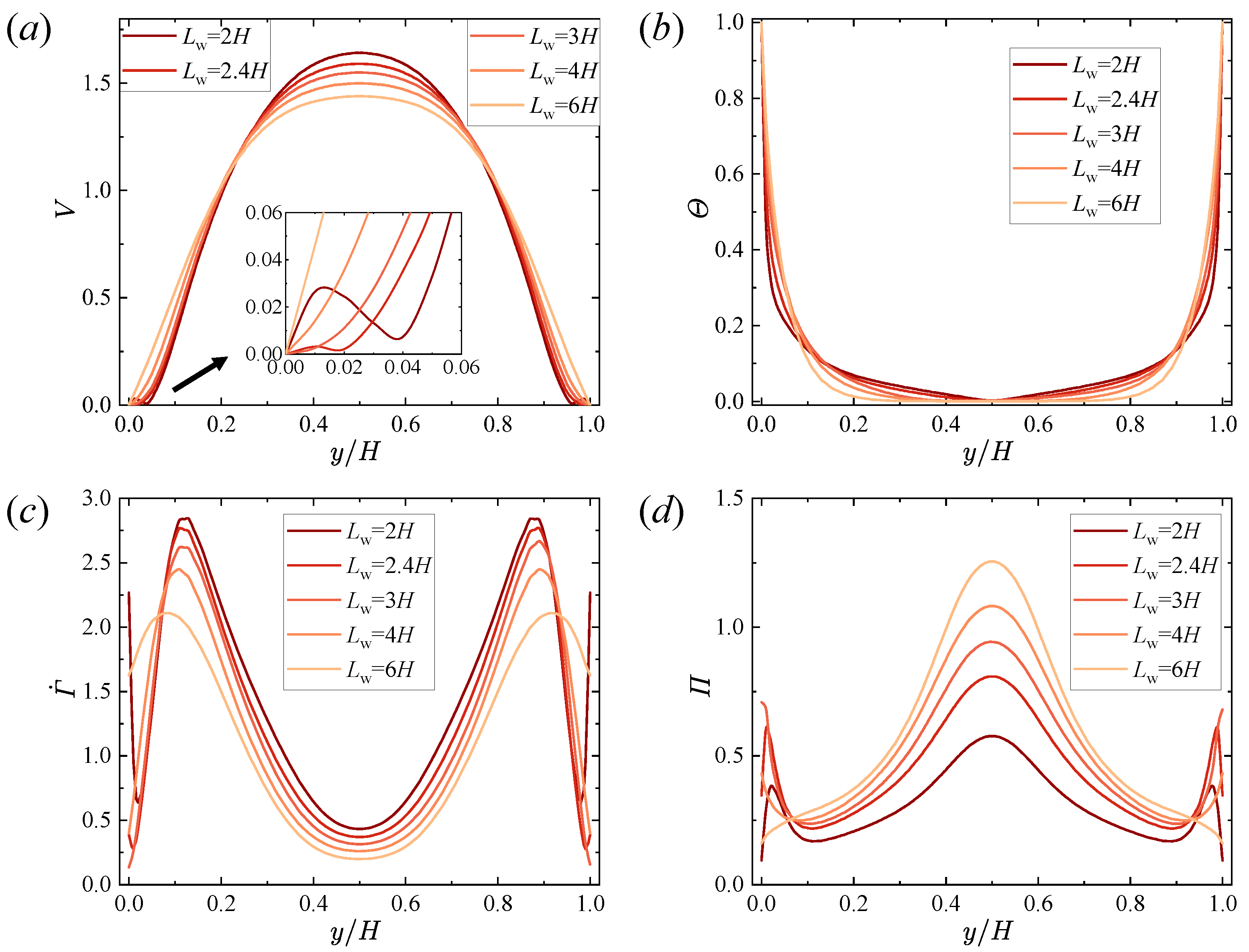
4.2. Effect of Wavelength
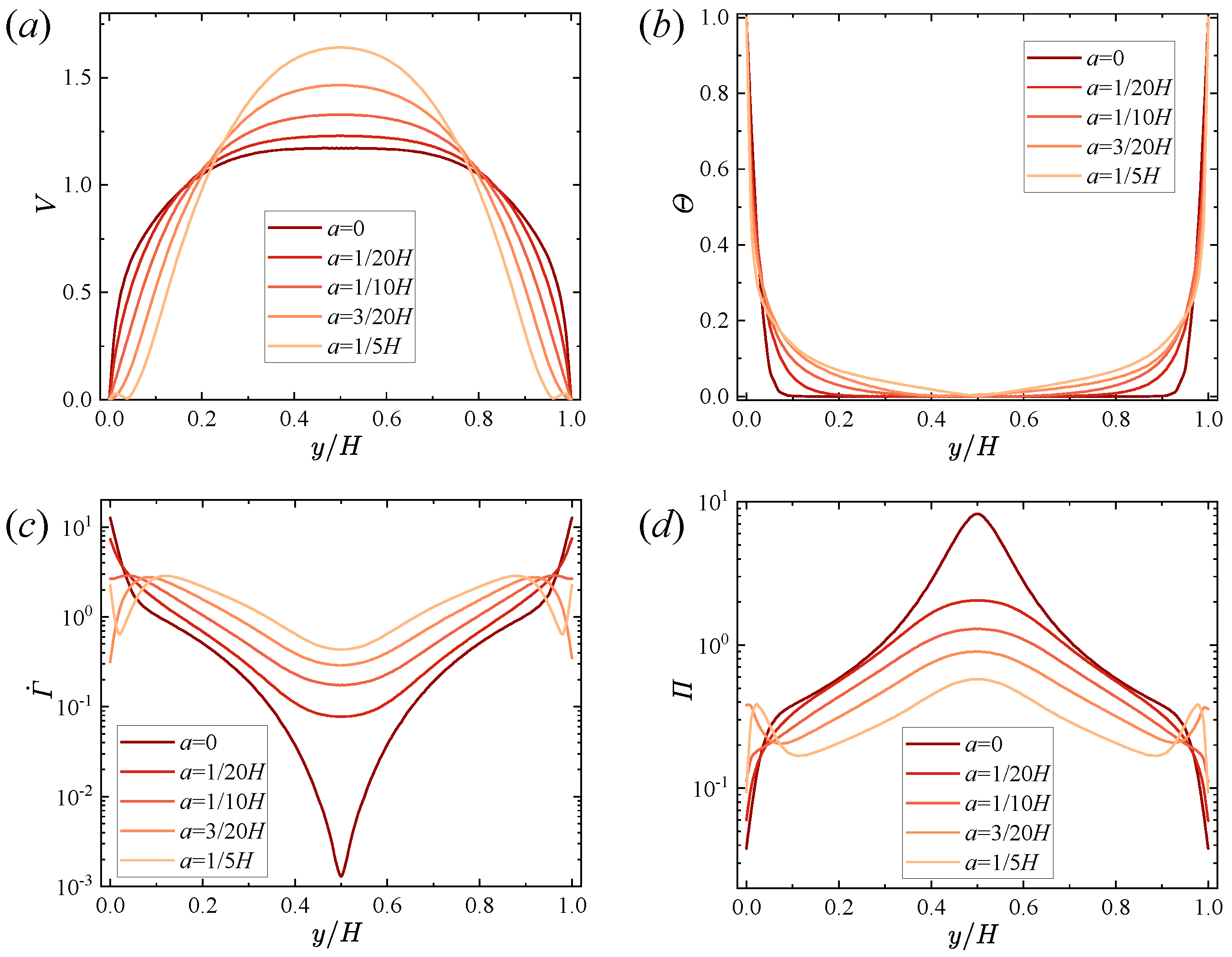
4.3. Effect of Wave Amplitude
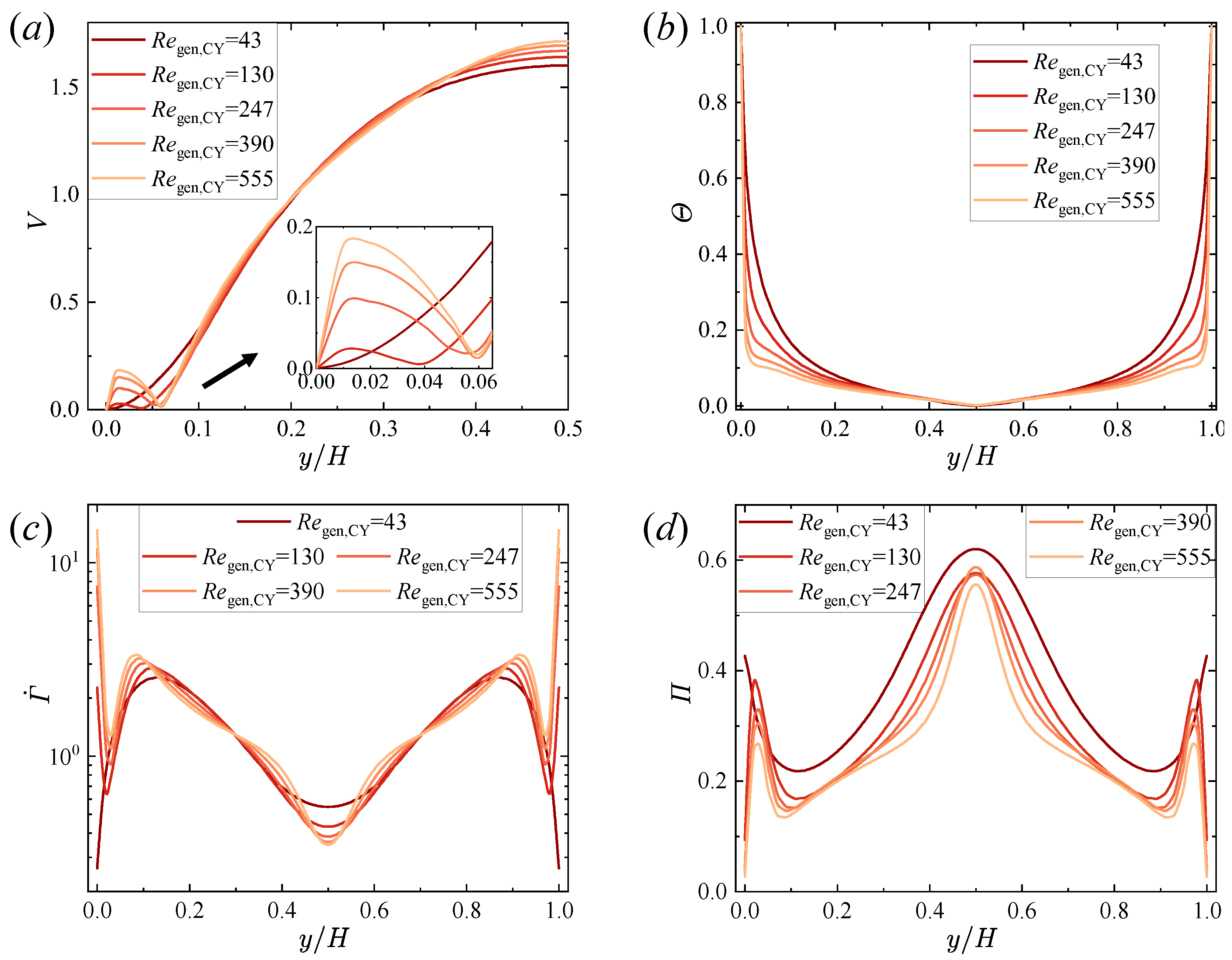
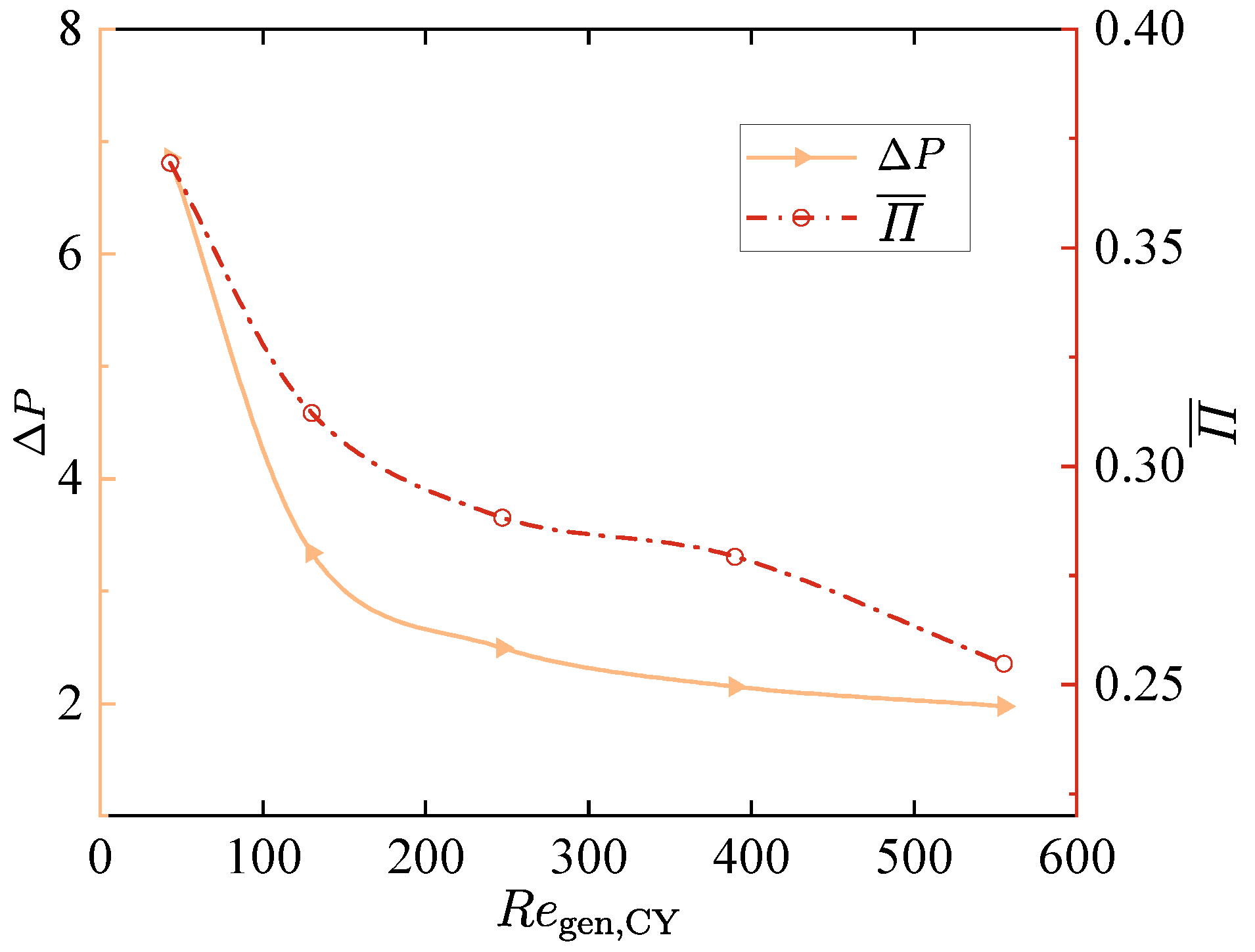
4.4. Effect of the Reynolds Number
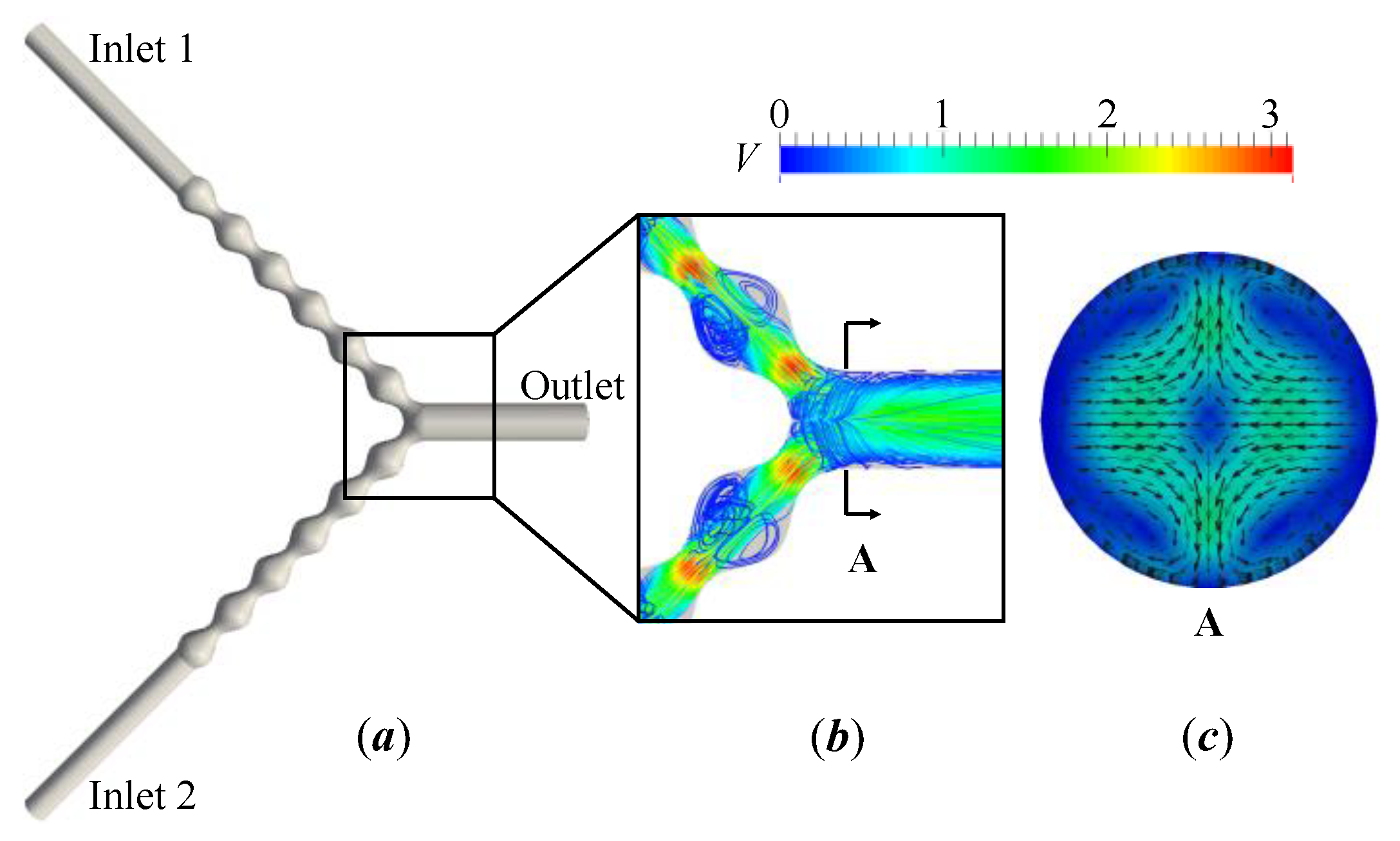
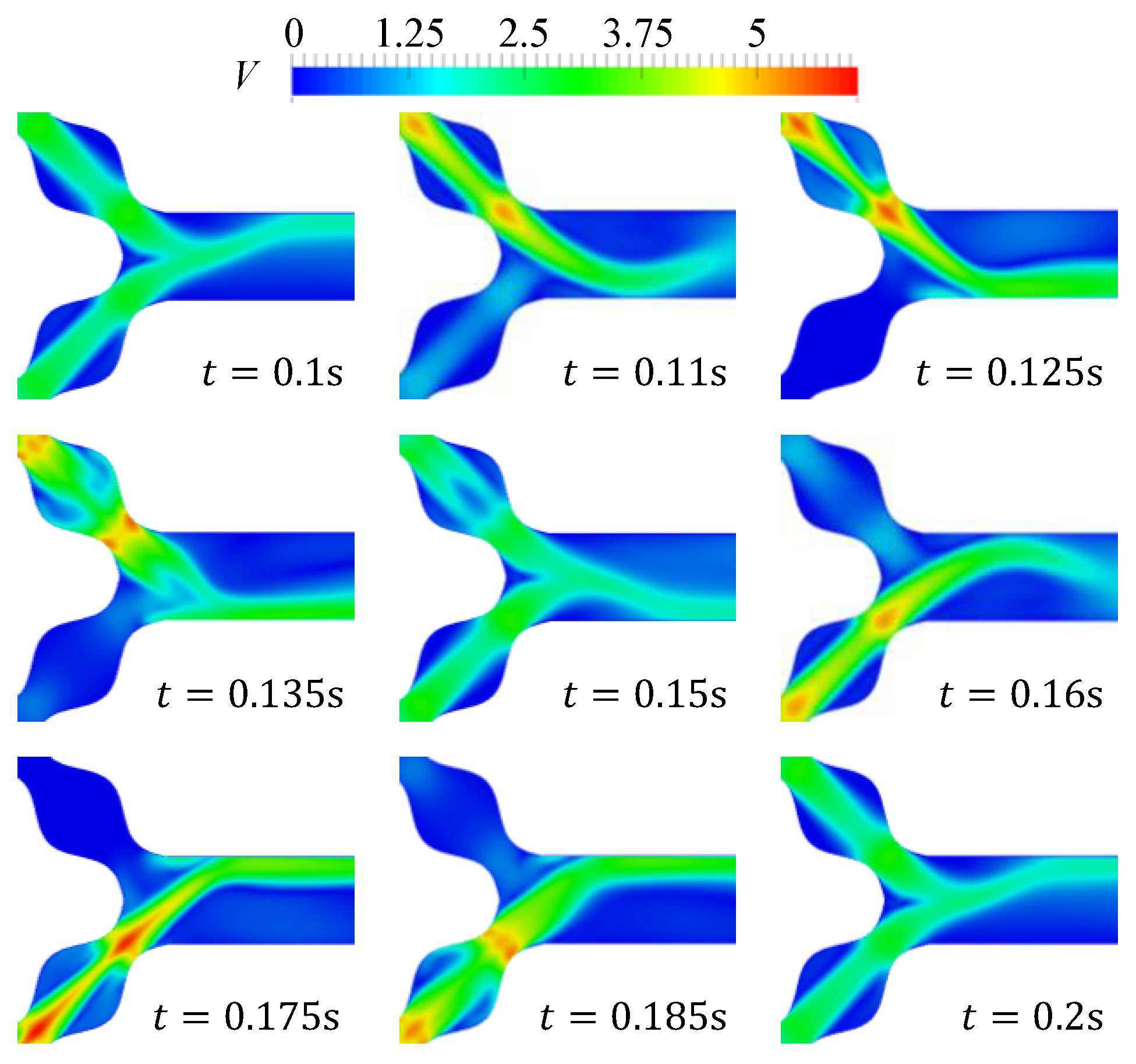
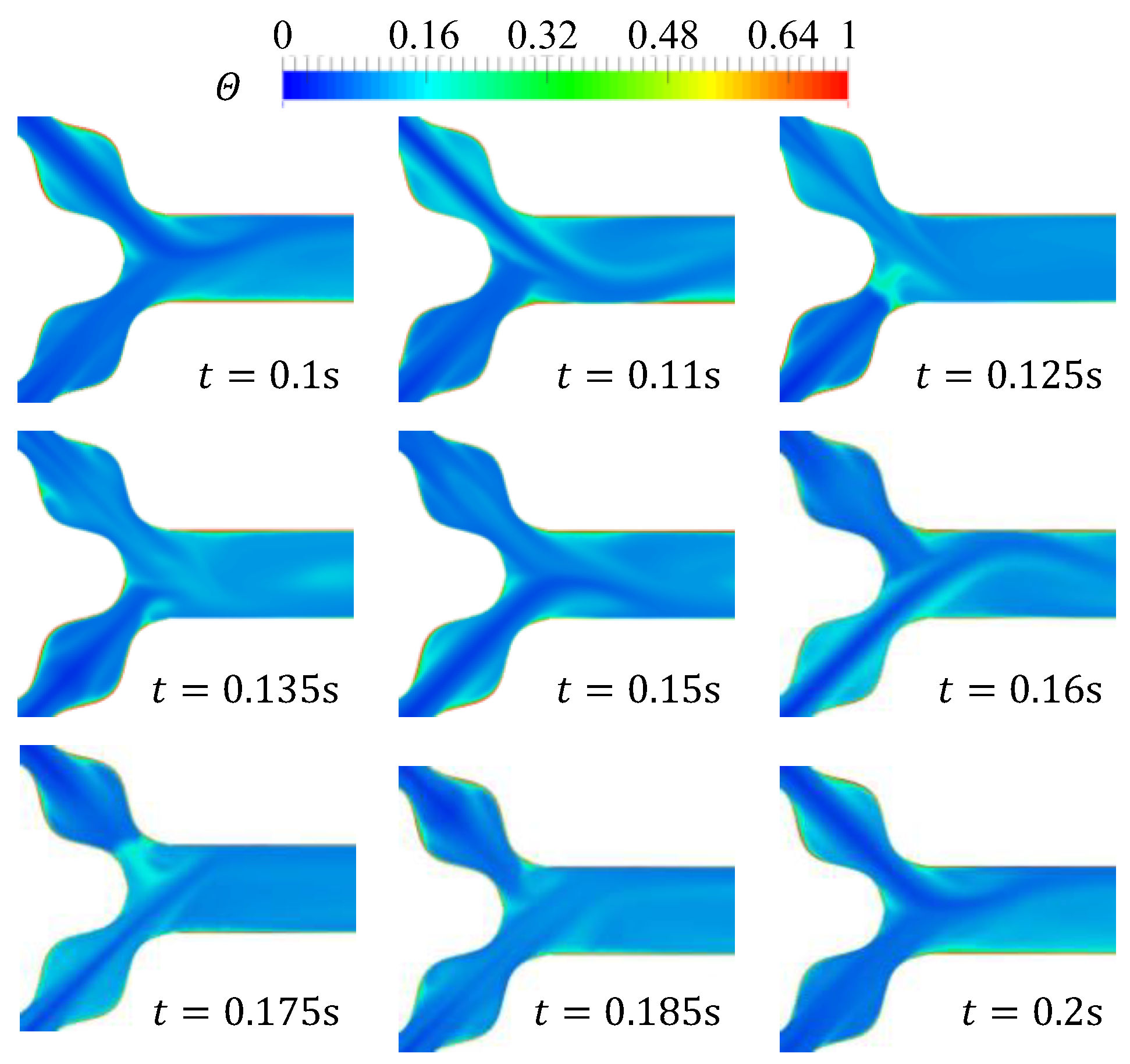
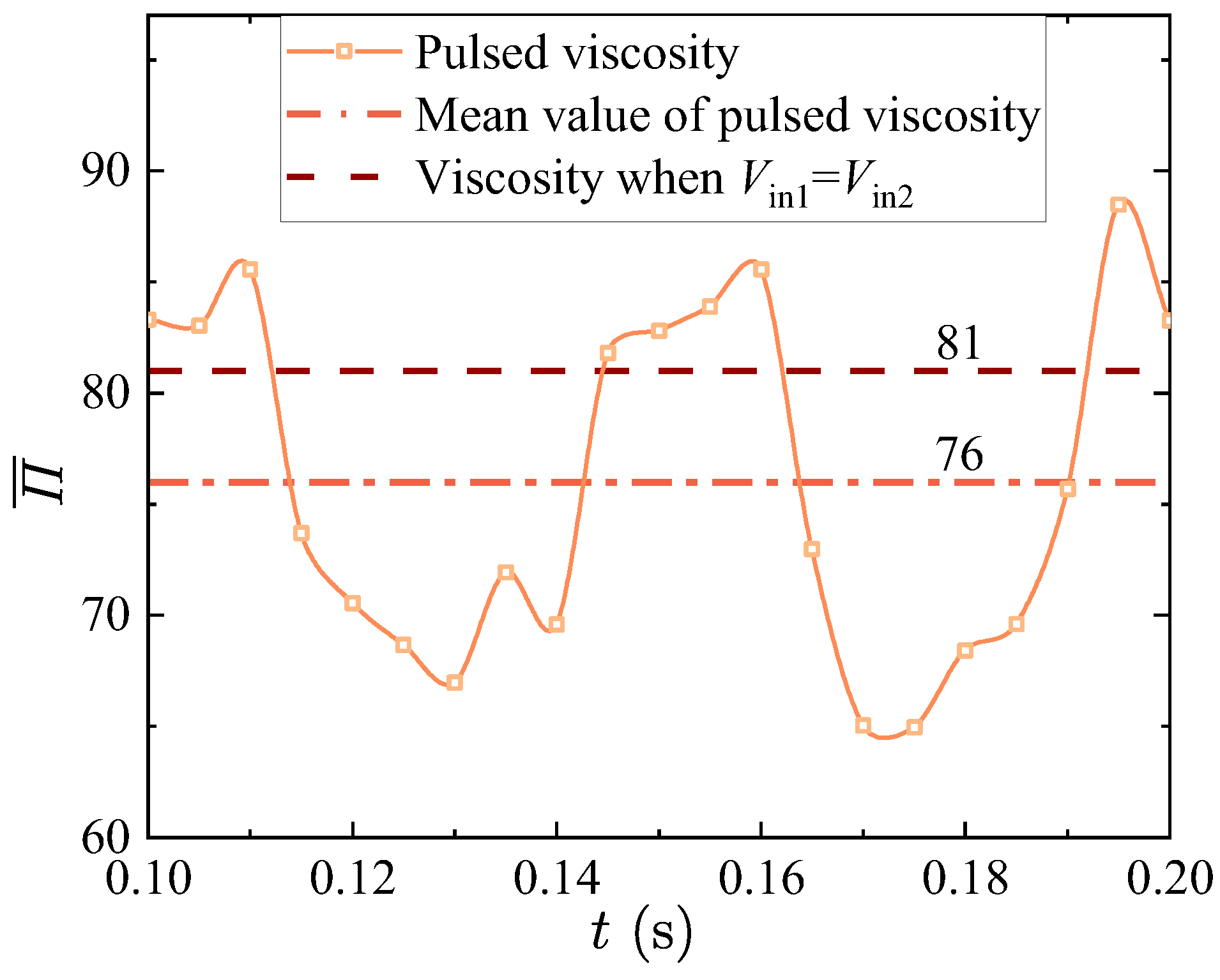
4.5. Flow and Heat Transfer in a Y-Shape Corrugated Pipe
5. Conclusions
- The recirculation regions generated by the corrugations enhance the heat convection and are beneficial for reducing the viscosity, while simultaneously the pressure drop seems to increase due to the viscous dissipation caused by the circulation. For different corrugation profiles, the sinusoidal corrugation can achieve lower viscosity with a lower pressure drop compared with the triangular and the trapezoidal corrugations.
- For the sinusoidal corrugation, a shorter wavelength and a deeper wave amplitude seem to be better for reducing the viscosity, but it also has the adverse consequence of the increased pressure drop. Furthermore, in the range of the parameters studied in this paper, a larger Reynolds number is more desirable for both lowering the pressure drop and reducing the viscosity.
- Compared with a smooth straight pipe, a Y-shape corrugated pipe with a constant inlet velocity can reduce the mean apparent viscosity by 70.8%, going up to 72.6% if a pulse inlet velocity is applied; this can significantly enhance the gel fuel atomization and thus improve the combustion efficiency.
- In terms of practical significance, compared with straight pipes, corrugated pipes with heated walls can effectively reduce the apparent viscosity of the gel at the outlet of the pipe, which will further facilitate the atomization of the gel. This has significant application prospects in propulsion processes using kerosene gel as fuel.
- For future work, we mention that the current paper mainly studies the viscosity reduction in gels due to temperature variations. From the conclusion, it can be seen that the effect is very significant. The working conditions and the overall composition of the pipeline are also different from the actual propulsion systems. The next step should be to carry out research on the actual engineering conditions and pipeline structure and explore the effects of viscosity reduction at the engineering application level.
Author Contributions
Funding
Conflicts of Interest
References
- Ciezki, H.K.; Hürttlen, J.; Naumann, K.W.; Negri, M.; Ramsel, J.; Weiser, V. Overview of the German Gel Propulsion Technology. In Proceedings of the 50th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, Cleveland, OH, USA, 28–30 July 2014; pp. 1–16. [Google Scholar]
- Arnold, R.; Anderson, W. Droplet burning of JP-8/Silica gels. In Proceedings of the 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2010; pp. 1–12. [Google Scholar]
- Ciezki, H.K.; Naumann, K.W.; Weiser, V. Status of Gel Propulsion in the Year 2010 with a Special View on the German Activities. In Proceedings of the Deutschland Luft-und Raumfahrtkongress, Hamburg, Germany, 31 August–29 September 2010. [Google Scholar]
- Natan, B.; Rahimi, S. The status of gel propellants in year 2000. Int. J. Energ. Mater. Chem. Propuls. 2002, 5, 172–194. [Google Scholar] [CrossRef]
- Gafni, G.; Kuznetsov, A.; Har-Lev, D.; Natan, B. Experimental Investigation of a Ramjet Combustor Using an Aluminized Gel Fuel. In Proceedings of the 49th AIAA/ASME/SAE/ASEE Joint PropulsionConference, San Jose, CA, USA, 14–17 July 2013; p. 3748. [Google Scholar]
- Matsibeker, E.; Natan, B. Numerical Solution of the Flowfield in an Aluminized Gel Fuel Ramjet. In Proceedings of the 49th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, San Jose, CA, USA, 14–17 July 2013; p. 3976. [Google Scholar]
- Natan, B.; Haddad, A.; Arieli, R. Performance assessments of a boron containing gel fuel ramjet. In Proceedings of the 47th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 5–8 January 2009; p. 1421. [Google Scholar]
- Doi, M. Explanation for the 3.4-power law for viscosity of polymeric liquids on the basis of the tube model. J. Polym. Sci. Polym. Phys. Ed. 1983, 21, 667–684. [Google Scholar] [CrossRef]
- Doi, M. Explanation for the 3.4-power law of viscosity of polymeric liquids on the basis of the tube model. J. Polym. Sci. Polym. Lett. Ed. 1981, 19, 265–273. [Google Scholar] [CrossRef]
- Krieger, I.M. Rheology of monodisperse latices. Adv. Colloid Interface Sci. 1972, 3, 111–136. [Google Scholar] [CrossRef]
- Rahimi, S.; Natan, B. Numerical solution of the flow of power-law gel propellants in converging injectors. Propellants Explos. Pyrotech. 2000, 25, 203–212. [Google Scholar] [CrossRef]
- Rahimi, S.; Natant, B. The injection process of gel fuels. In Proceedings of the 33rd Joint Propulsion Conference and Exhibit, Seattle, WA, USA, 6–9 July 1997. [Google Scholar]
- Rahimi, S.; Natan, B. Flow of Gel Fuels in Tapered Injectors. J. Propuls. Power 2000, 16, 458–464. [Google Scholar] [CrossRef]
- Yoon, C.; Heister, S.D.; Xia, G.; Merkle, C.L. Numerical modeling of injection of shear-thinning gel propellants through plain-orifice atomizer. J. Propuls. Power 2011, 27, 944–954. [Google Scholar] [CrossRef]
- Cao, Q.-L.; Massoudi, M.; Liao, W.-H.; Feng, F.; Wu, W.-T. Flow Characteristics of Water-HPC Gel in Converging Tubes and Tapered Injectors. Energies 2019, 12, 1643. [Google Scholar] [CrossRef]
- Middleman, S. Fundamentals of Polymer Processing; McGraw-Hill: New York, NY, USA, 1977. [Google Scholar]
- Carreau, P.J.; De Kee, D.; Chhabra, R.J. Rheology of Polymeric Systems; Hanser/Gardner Publications: Cincinnati, OH, USA, 1997. [Google Scholar]
- Shin, S.; Cho, Y.I. Laminar heat transfer in a rectangular duct with a non-Newtonian fluid with temperature-dependent viscosity. Int. J. Heat Mass Transf. 1994, 37, 19–30. [Google Scholar] [CrossRef]
- Rahimi, S.; Peretz, A.; Natan, B. Rheological matching of gel propellants. J. Propuls. Power 2010, 26, 376–379. [Google Scholar] [CrossRef]
- Rahimi, S.; Natan, B. Atomization characteristics of gel fuels. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998; p. 3830. [Google Scholar]
- Cao, Q.L.; Wu, W.T.; Liao, W.H.; Feng, F.; Massoudi, M. Effects of temperature on the flow and heat transfer in gel fuels: A numerical study. Energies 2020, 13, 821. [Google Scholar] [CrossRef]
- Kareem, Z.S.; Jaafar, M.N.M.; Lazim, T.M.; Abdullah, S.; Abdulwahid, A.F. Passive heat transfer enhancement review in corrugation. Exp. Therm. Fluid Sci. 2015, 68, 22–38. [Google Scholar] [CrossRef]
- Hong, Y.; Du, J.; Wang, S.; Huang, S.-M. Heat transfer and flow behaviors of a wavy corrugated tube. Appl. Therm. Eng. 2017, 126, 151–166. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Rashidi, S.; Esfahani, J.A. Influences of corrugation profiles on entropy generation, heat transfer, pressure drop, and performance in a wavy channel. Appl. Therm. Eng. 2017, 116, 278–291. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, Y.; Li, Y.; Han, H.; Li, B. Numerical study on fully-developed turbulent flow and heat transfer in inward corrugated tubes with double-objective optimization. Int. J. Heat Mass Transf. 2018, 120, 782–792. [Google Scholar] [CrossRef]
- Saikia, A.; Dalal, A.; Pati, S. Thermo-hydraulic transport characteristics of non-Newtonian fluid flows through corrugated channels. Int. J. Therm. Sci. 2018, 129, 201–208. [Google Scholar]
- Afrouzi, H.H.; Ahmadian, M.; Moshfegh, A.; Toghraie, D.; Javadzadegan, A. Statistical analysis of pulsating non-Newtonian flow in a corrugated channel using Lattice-Boltzmann method. Phys. A Stat. Mech. Appl. 2019, 535, 122486. [Google Scholar] [CrossRef]
- Hilo, A.K.; Talib, A.R.A.; Iborra, A.A.; Sultan, M.T.H.; Hamid, M.F.A. Effect of corrugated wall combined with backward-facing step channel on fluid flow and heat transfer. Energy 2020, 190, 116294. [Google Scholar] [CrossRef]
- Wu, W.T.; Aubry, N.; Massoudi, M. Flow of granular materials modeled as a non-linear fluid. Mech. Res. Commun. 2013, 52, 62–68. [Google Scholar] [CrossRef]
- Slattery, J.C. Advanced Transport Phenomena; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Winterton, R.H.S. Early study of heat transfer: Newton and Fourier. Heat Transf. Eng. 2001, 22, 3–11. [Google Scholar] [CrossRef]
- Zhang, M.; Che, Z.; Chen, J.; Zhao, H.; Yang, L.; Zhong, Z.; Lu, J. Experimental Determination of Thermal Conductivity of Water–Agar Gel at Different Concentrations and Temperature. J. Chem. Eng. Data 2010, 56, 859–864. [Google Scholar] [CrossRef]
- Macosko, C.W.; Larson, R.G. Rheology: Principles, Measurements, and Applications; Wiley-VCH: Weinheim, Germany, 1994. [Google Scholar]
- Bird, R.B.; Curtiss, C.F.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids: Volume 2: Kinetic Theory; John Wiley & Sons: Hoboken, NJ, USA, 1987. [Google Scholar]
- Arnold, R.; Anderson, W.; Santos, P.H.S.; de Ridder, M.; Campanella, O.H. Comparison of Monomethylhydrazine/hydroxypropylcellulose and Hydrocarbon/silica Gels. In Proceedings of the 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2010; p. 422. [Google Scholar]
- Miao, L.; Massoudi, M. Heat transfer analysis and flow of a slag-type fluid: Effects of variable thermal conductivity and viscosity. Int. J. Non-Linear Mech. 2015, 76, 8–19. [Google Scholar] [CrossRef]
- Miao, L.; Massoudi, M. Effects of shear dependent viscosity and variable thermal conductivity on the flow and heat transfer in a slurry. Energies 2015, 8, 11546–11574. [Google Scholar] [CrossRef]
- Szeri, A.Z. Fluid Film Lubrication: Theory and Design; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Massoudi, M.; Christie, I. Effects of variable viscosity and viscous dissipation on the flow of a third grade fluid in a pipe. Int. J. Non-Linear Mech. 1995, 30, 687–699. [Google Scholar] [CrossRef]
- OpenFOAM Programmer’s Guide; Version 2.1.0; OpenCFD: Bracknell, UK, 2011.
- Rahimi, S.; Peretz, A.; Natan, B. On Shear Rheology of Gel Propellants. Propellants Explos. Pyrotech. 2010, 32, 165–174. [Google Scholar] [CrossRef]
- Siebert, M.W.; Fodor, P.S. Newtonian and non-newtonian blood flow over a backward-facing step—A case study. In Proceedings of the COMSOL Conference, Boston, MA, USA, 8–10 October 2009. [Google Scholar]
- Wu, W.-T.; Massoudi, M.; Yan, H. Heat transfer and flow of nanofluids in a Y-type intersection channel with multiple pulsations: A numerical study. Energies 2017, 10, 492. [Google Scholar] [CrossRef]


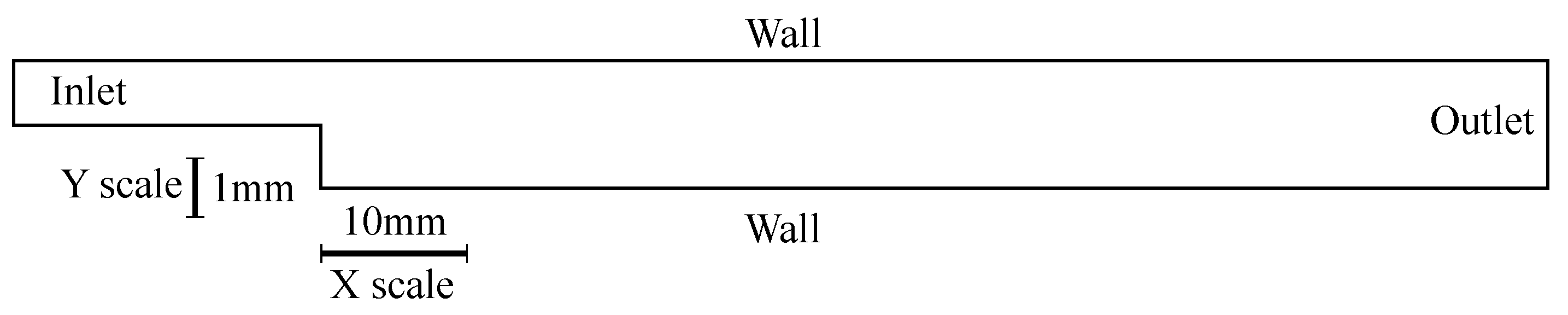


| Conservation Equation | Discretization |
|---|---|
| Gradient | Gauss linear |
| convection | bounded Gauss upwind |
| Diffusion | Gauss linear corrected |
| Time | Euler |
| Boundary Type | Pressure | Velocity | Temperature |
|---|---|---|---|
| Cold Wall | Zero gradient | Fixed value (0) | Fixed value (298 K) |
| Heat wall | Zero gradient | Fixed value (0) | Fixed value (372 K) |
| Inlet | Zero gradient | Fixed value | Fixed value (298 K) |
| Outlet | Fixed value (0) | Zero gradient | Zero gradient |
| Research Points | |||
|---|---|---|---|
| Corrugation profile | 1/5H | 2H | 130 |
| Wavelength | 1/5H | 2H, 2.4H, 3H, 4H, 6H | 130 |
| Wave amplitude | 1/20H, 1/10H, 3/20H, 1/5H | 2H | 130 |
| Reynolds number | 1/5H | 2H | 43, 130, 247, 390, 555 |
| Mesh Numbers | ||
|---|---|---|
| Case1 | 12,000 | 0.18208 |
| Case2 | 24,000 | 0.18559 |
| Case3 | 48,000 | 0.18474 |
| Case4 | 96,000 | 0.18768 |
| Sinusoidal | Triangular | Trapezoidal | Smooth | |
|---|---|---|---|---|
| 3.34 | 4.24 | 5.65 | 1.03 | |
| 0.31 | 0.49 | 0.34 | 1.72 | |
| PEC | 1.19 | 1.04 | 1.13 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, H.; Feng, F.; Cao, Q.-L.; Zhou, C.; Wu, W.-T.; Massoudi, M. Heat Transfer and Flow of a Gel Fuel in Corrugated Channels. Energies 2022, 15, 7287. https://doi.org/10.3390/en15197287
Zhou H, Feng F, Cao Q-L, Zhou C, Wu W-T, Massoudi M. Heat Transfer and Flow of a Gel Fuel in Corrugated Channels. Energies. 2022; 15(19):7287. https://doi.org/10.3390/en15197287
Chicago/Turabian StyleZhou, Hao, Feng Feng, Qin-Liu Cao, Changsheng Zhou, Wei-Tao Wu, and Mehrdad Massoudi. 2022. "Heat Transfer and Flow of a Gel Fuel in Corrugated Channels" Energies 15, no. 19: 7287. https://doi.org/10.3390/en15197287
APA StyleZhou, H., Feng, F., Cao, Q.-L., Zhou, C., Wu, W.-T., & Massoudi, M. (2022). Heat Transfer and Flow of a Gel Fuel in Corrugated Channels. Energies, 15(19), 7287. https://doi.org/10.3390/en15197287








