Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence
Abstract
:1. Introduction
2. DNS Dataset and Spectral Budget Analysis
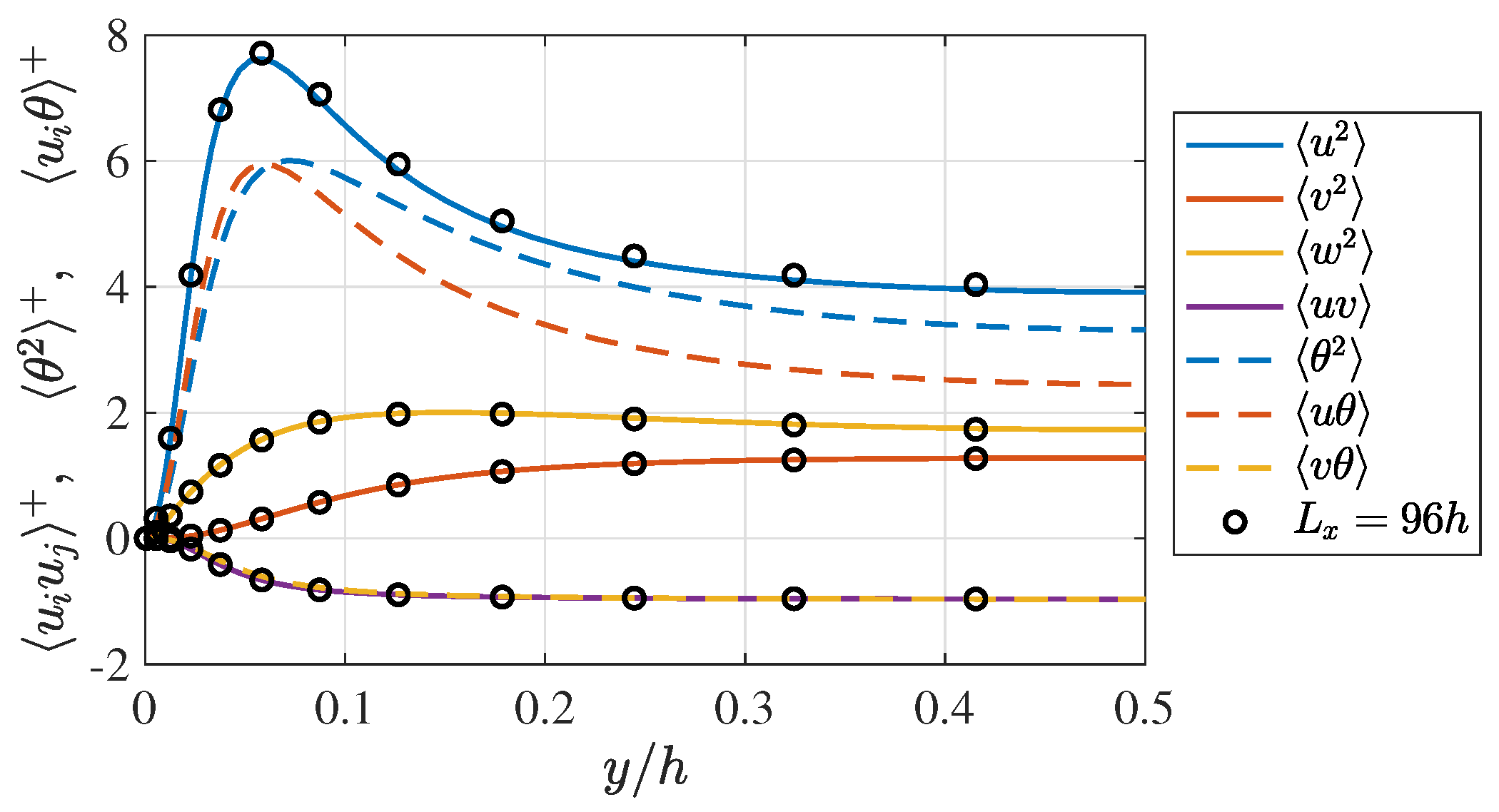
2.1. DNS Dataset of a Turbulent Plane Couette Flow with Temperature Transport
2.2. Spectral Analysis of the Reynolds-Stress Transport and Turbulent Heat Transfers
2.2.1. Transport of Reynolds Stresses
2.2.2. Transport of Temperature Fluctuation and Velocity-Temperature Correlations
3. Results
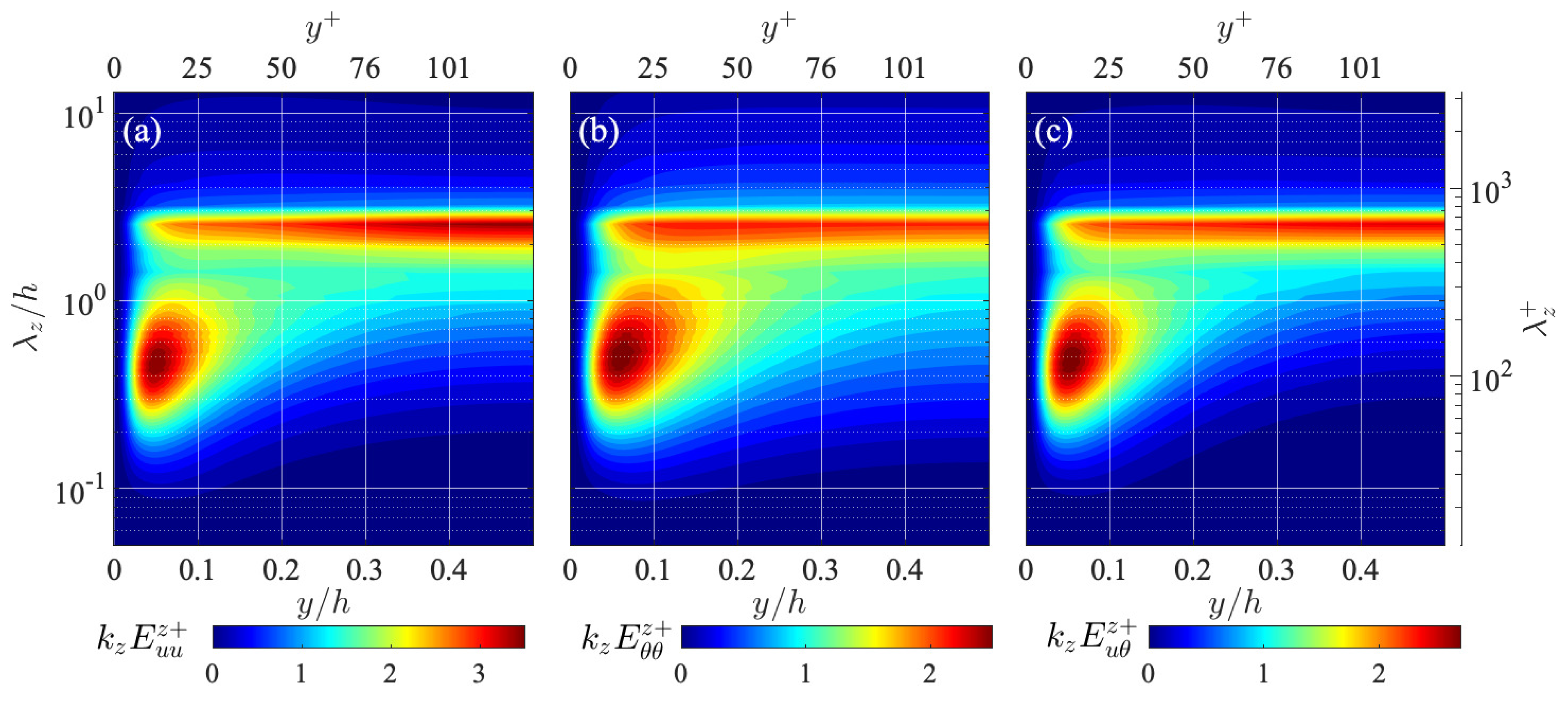
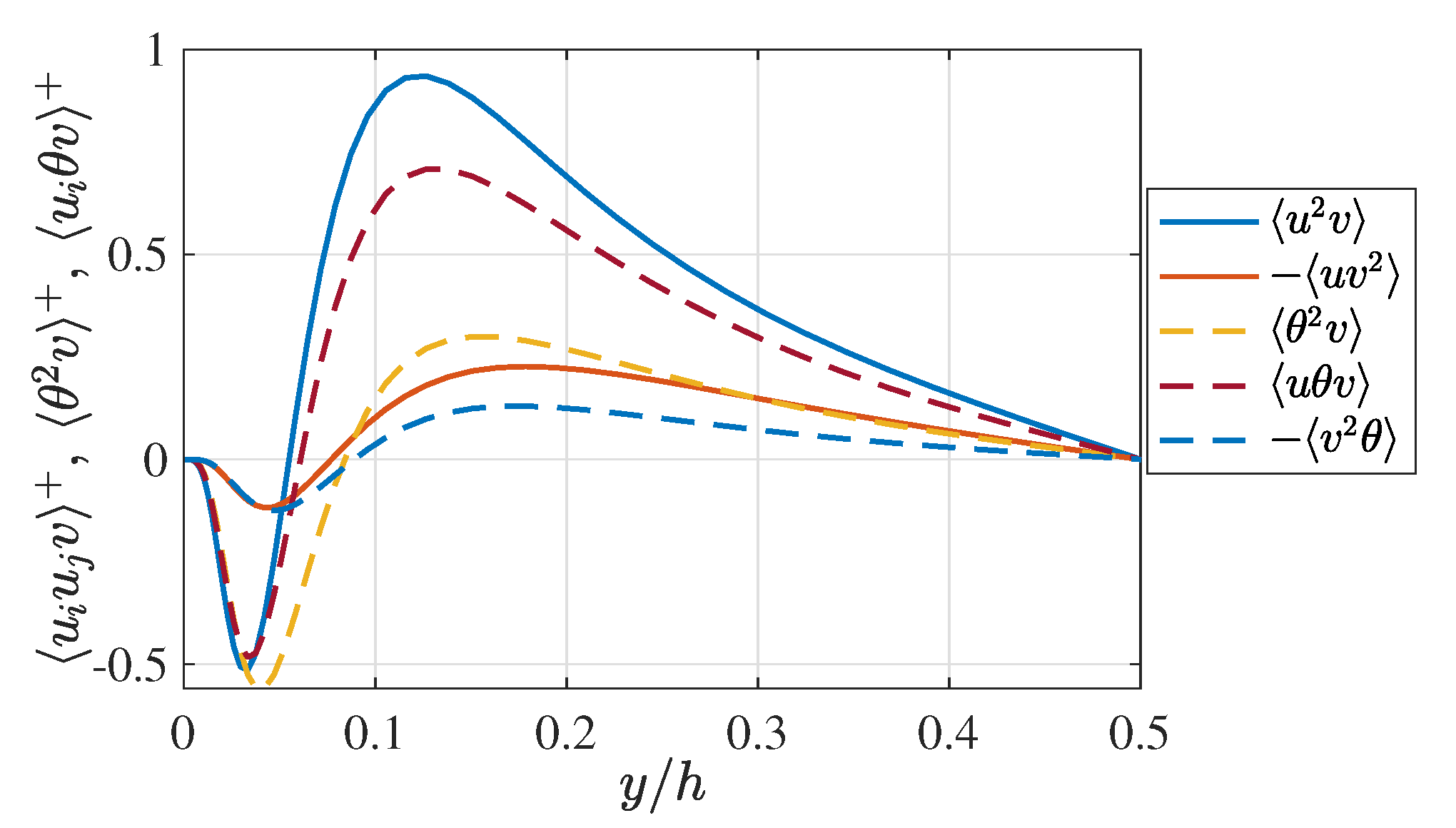
3.1. Spectra of Temperature-Related Statistics and Their Interscale and Spatial Transports
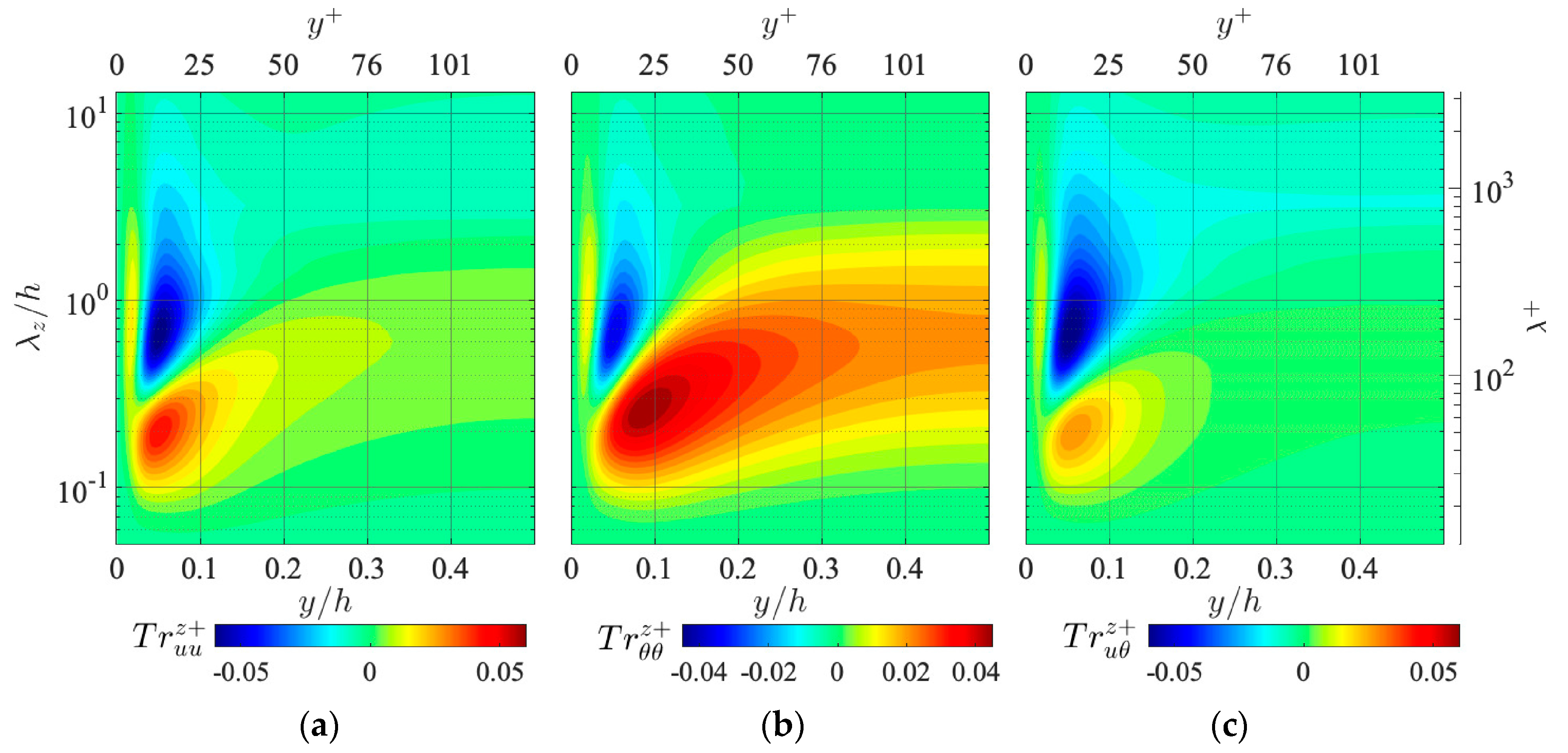
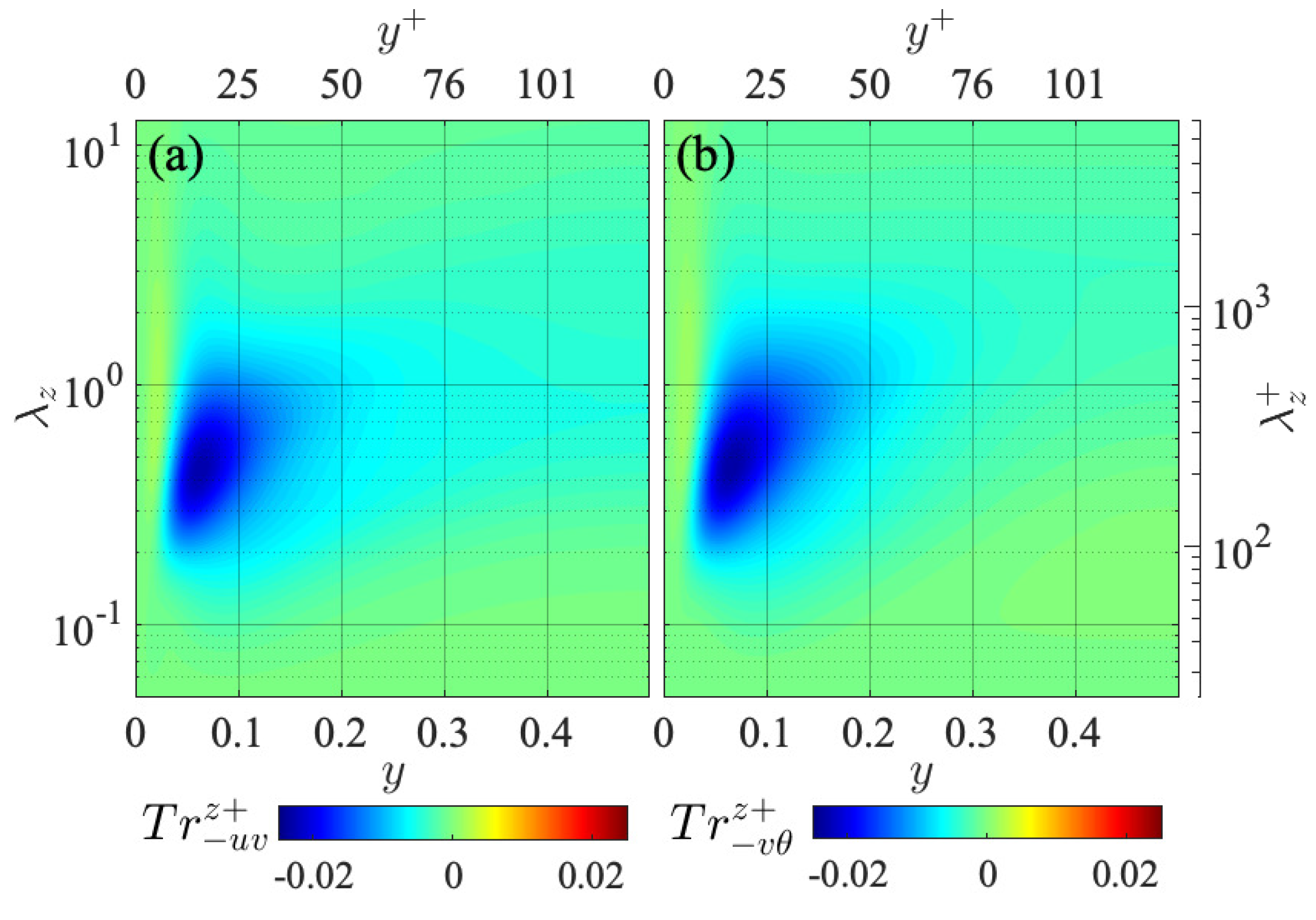
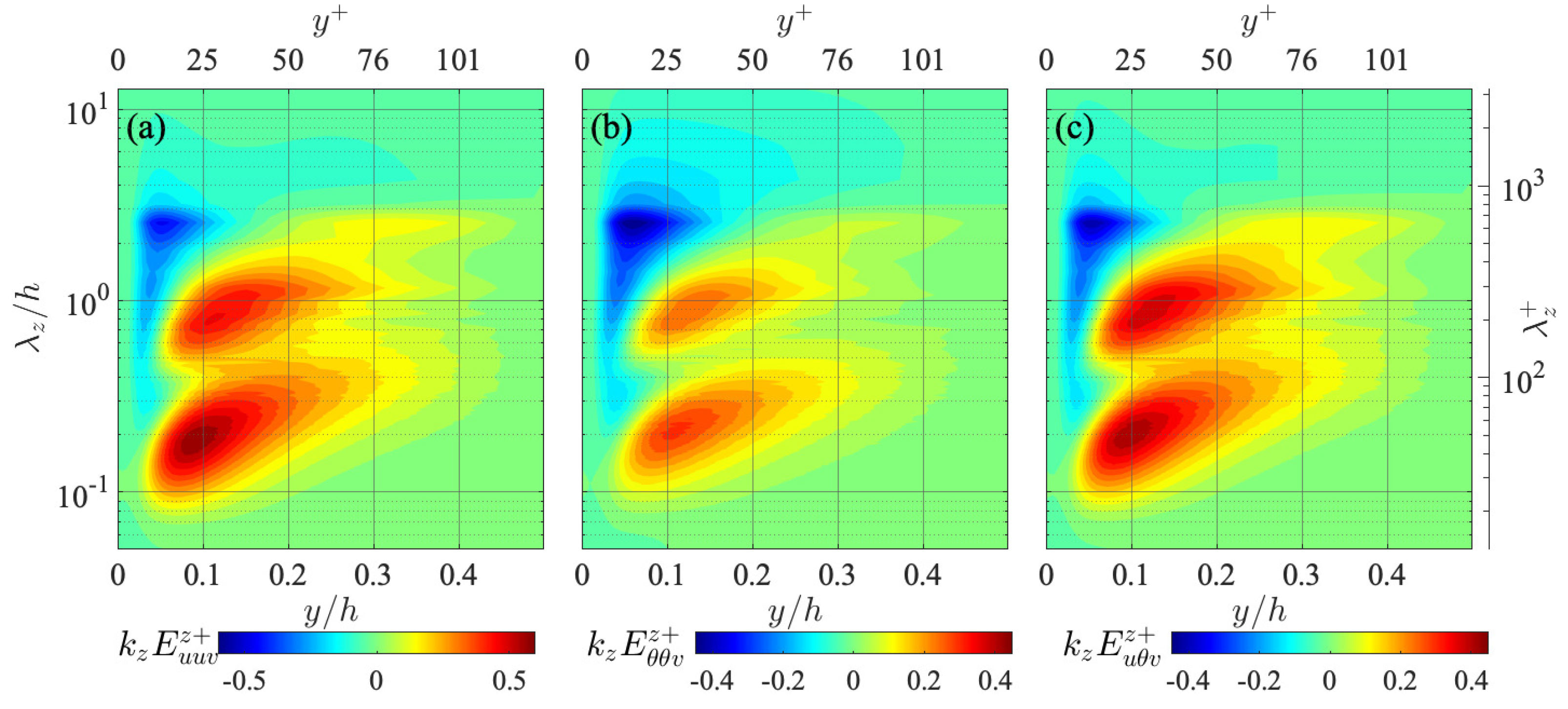
3.2. Interscale Fluxes of the Reynolds Stresses and Temperature-Related Statistics
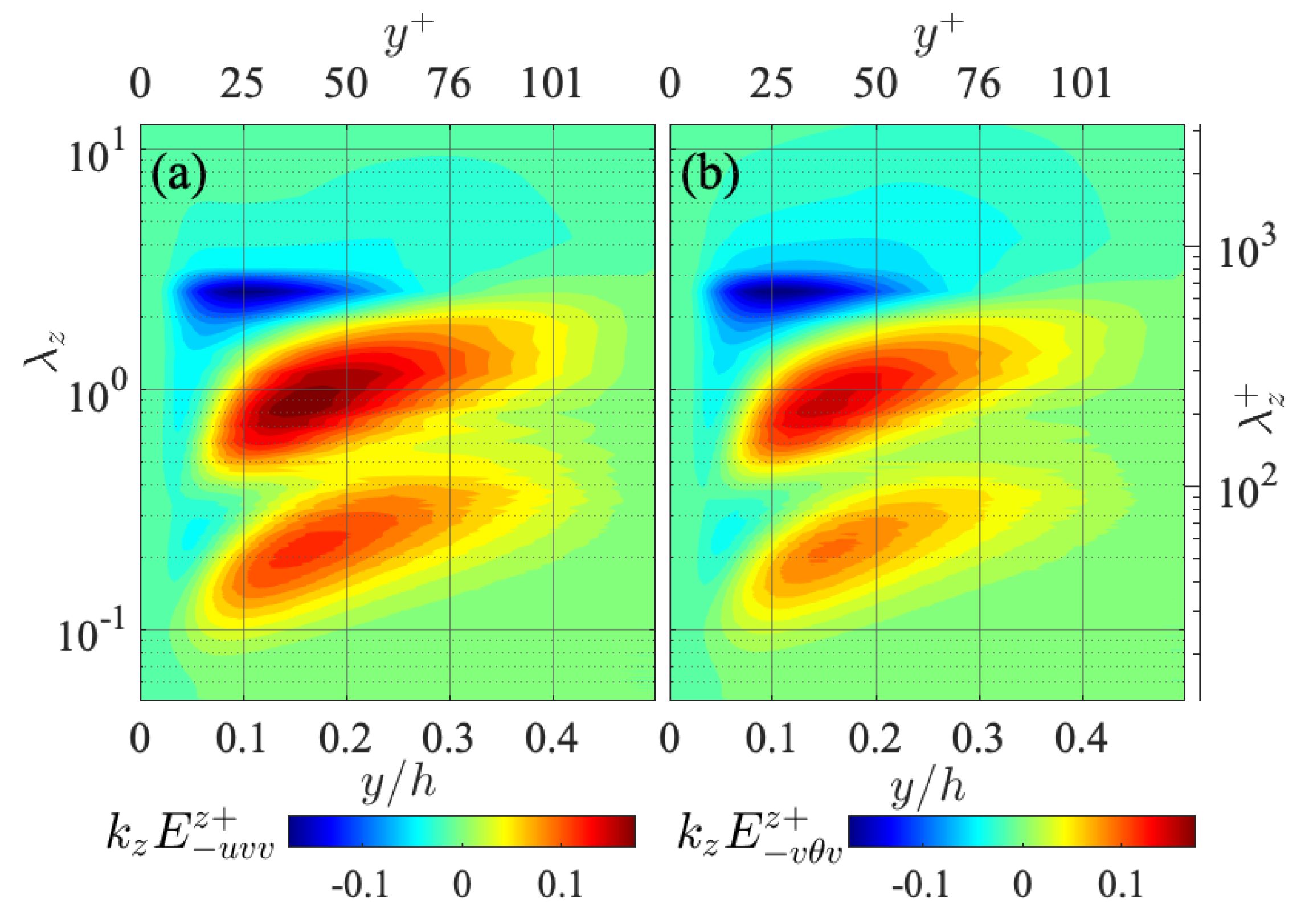
3.3. Spectra of Spatial Turbulent Fluxes of the Reynolds Stresses and Temperature-Related Statistics
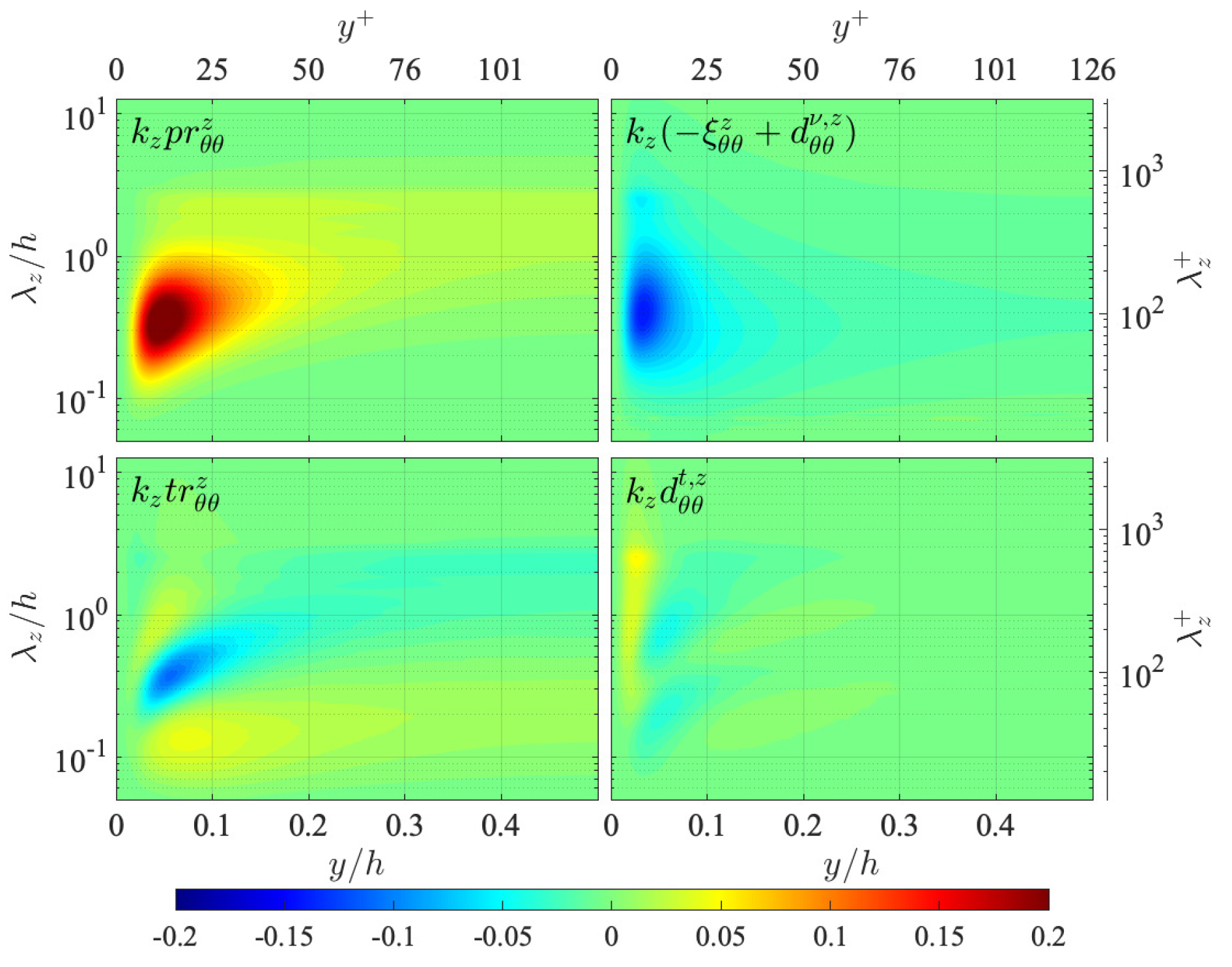
3.4. Budget Analysis of Spectral Transport Equations
4. Discussion and Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Spanwise one-dimensional spectra of | |
| Spanwise one-dimensional spectra of | |
| , , , , , , | Spanwise one-dimensional spectra of , , |
| , , , , and | |
| Spanwise one-dimensional spectra of | |
| Spanwise one-dimensional spectra of | |
| , , , , | Spanwise one-dimensional spectra of turbulent spatial |
| fluxes , , , , and | |
| h | Full channel height |
| Spanwise wavenumber | |
| Cutoff spanwise wavenumber for large- and | |
| small-scale decomposition | |
| , | Streamwise and spanwise lengths of computational |
| domain | |
| p | Pressure fluctuation |
| Prandtl number defined as | |
| Reynolds number defined as | |
| Friction Reynolds number defined as | |
| , | Temperature of top and bottom wall |
| Temperature difference between top and bottom wall, | |
| Friction temperature defined as , where | |
| and are mean wall heat flux and fluid specific | |
| heat at constant pressure, respectively | |
| Interscale flux of in spanwise length scales | |
| , , , , | Interscale flux of , , , , in |
| spanwise length scales | |
| U | Mean streamwise velocity |
| Translating speed of top wall | |
| u, v, w | Fluctuation of streamwise, wall-normal, and spanwise |
| velocity components | |
| Fluctuation of velocity component in direction | |
| Friction velocity defined as , where is | |
| mean wall shear stress | |
| x, y, z | Axes of the coordinates in the streamwise, wall-normal, |
| and spanwise directions | |
| Thermal diffusivity of fluid | |
| Half channel height, i.e., | |
| Kinematic viscosity of fluid | |
| Density of fluid |
| , | Mean temperature and temperature fluctuation |
| spanwise wavelength, i.e., | |
| 〈〉 | Averaged quantities |
| Terms in transport equations | |
| , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, and turbulent spatial transport | |
| terms in transport equation | |
| , | Pressure-strain correlation and pressure diffusion |
| terms in transport equation | |
| , , , | Production, viscous dissipation, viscous diffusion, and |
| turbulent spatial transport terms in transport | |
| equation | |
| , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, and turbulent spatial transport terms | |
| in transport equation | |
| , , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| , | Pressure-strain and pressure transport terms in |
| transport equation | |
| , , , , | Production, viscous dissipation, viscous diffusion, |
| turbulent spatial transport, and interscale transport | |
| terms in transport equation | |
| , , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| , , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| , , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| , , , , , | Production, viscous dissipation, pressure-strain, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| , , , , , | Pressure-strain, viscous dissipation, pressure transport, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| , , , , | Pressure-strain, viscous dissipation, viscous diffusion, |
| turbulent spatial transport, and interscale transport | |
| terms in transport equation | |
| , , , , , | Production, viscous dissipation, pressure-gradient, |
| viscous diffusion, turbulent spatial transport, and | |
| interscale transport terms in transport equation | |
| Superscripts | |
| L, S | Large- and small-scale part |
| + | Scaled viscous units, based on , , |
Appendix A. Spectral Budgets of the Reynolds Stress Transports

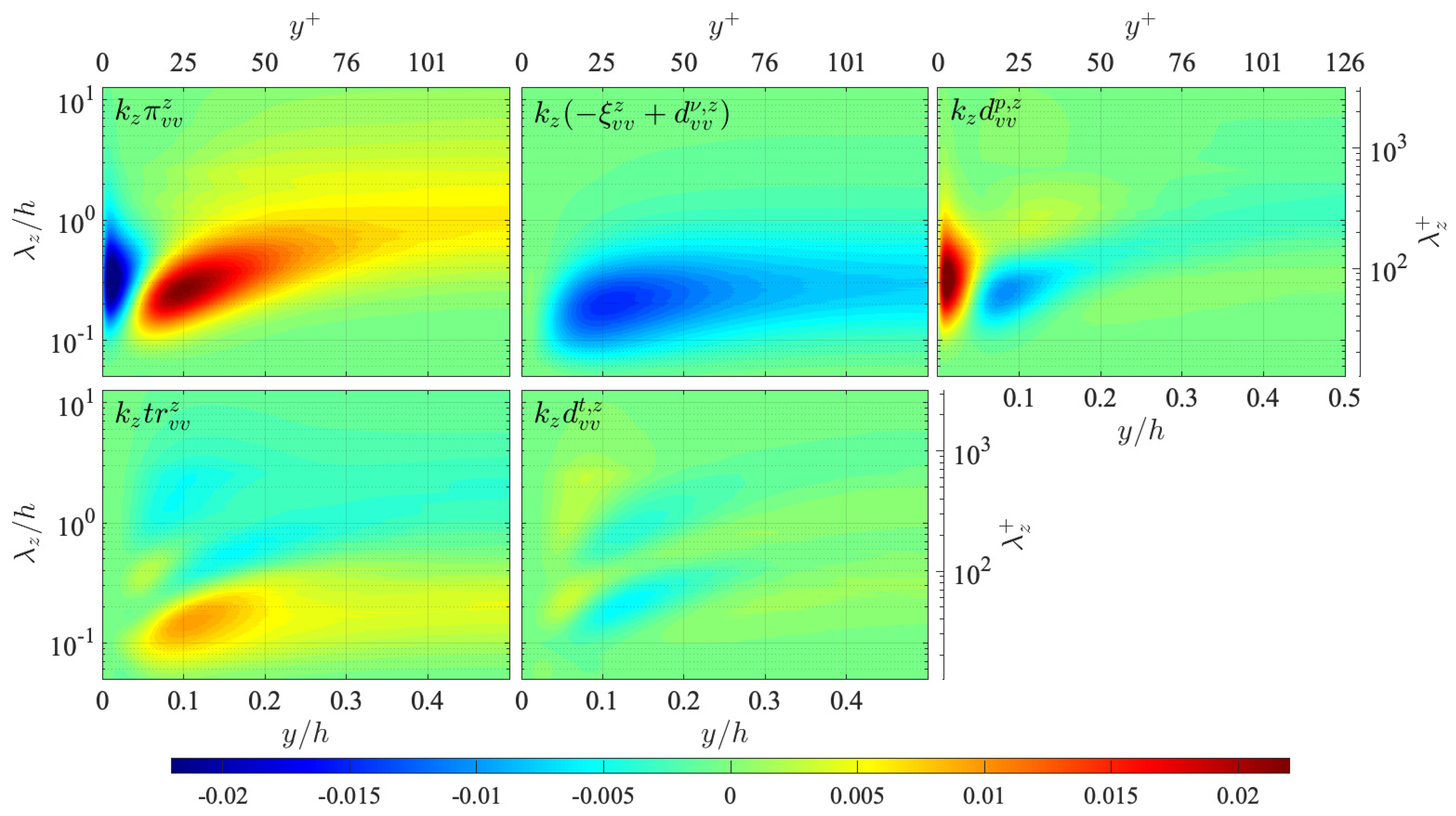
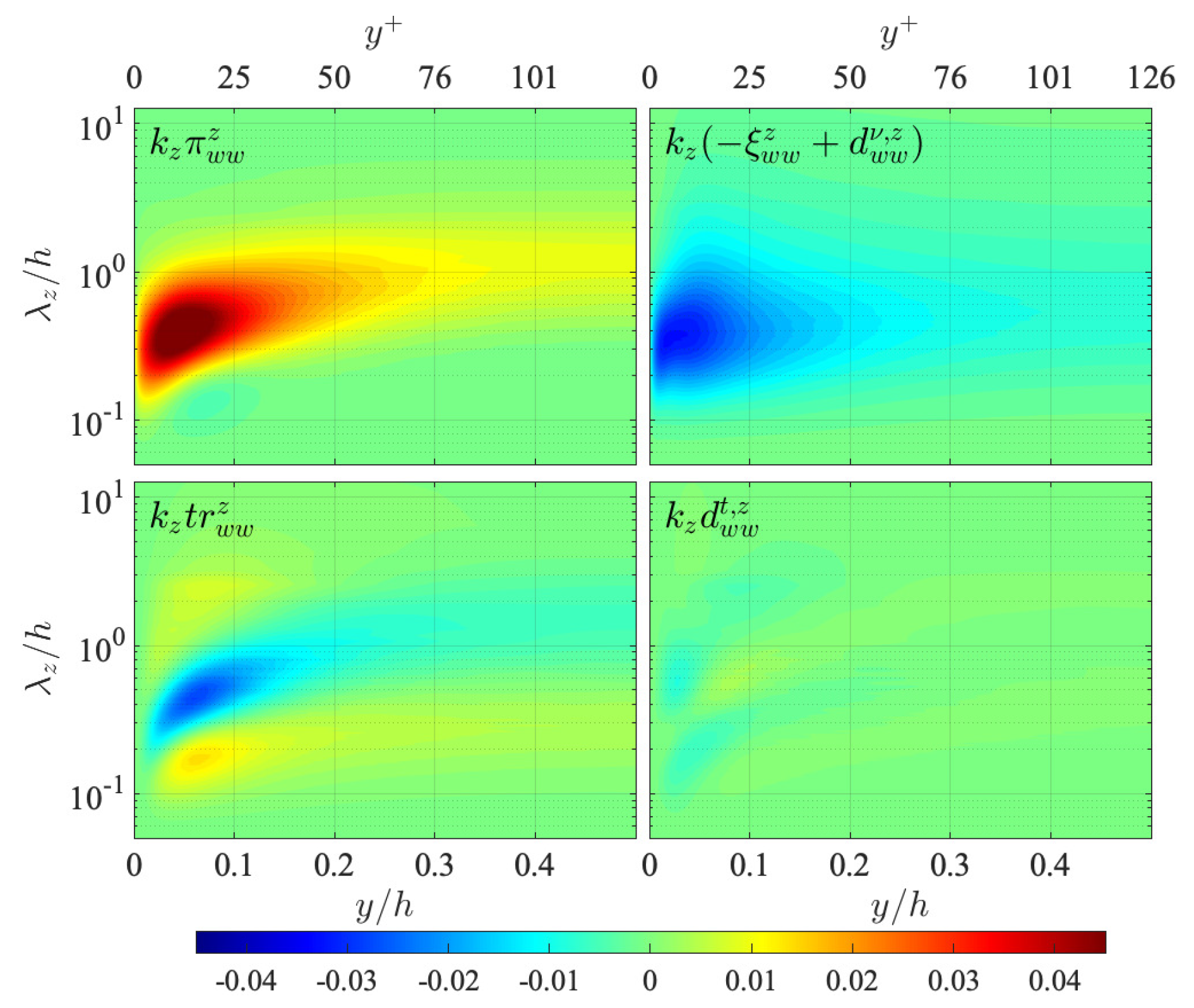
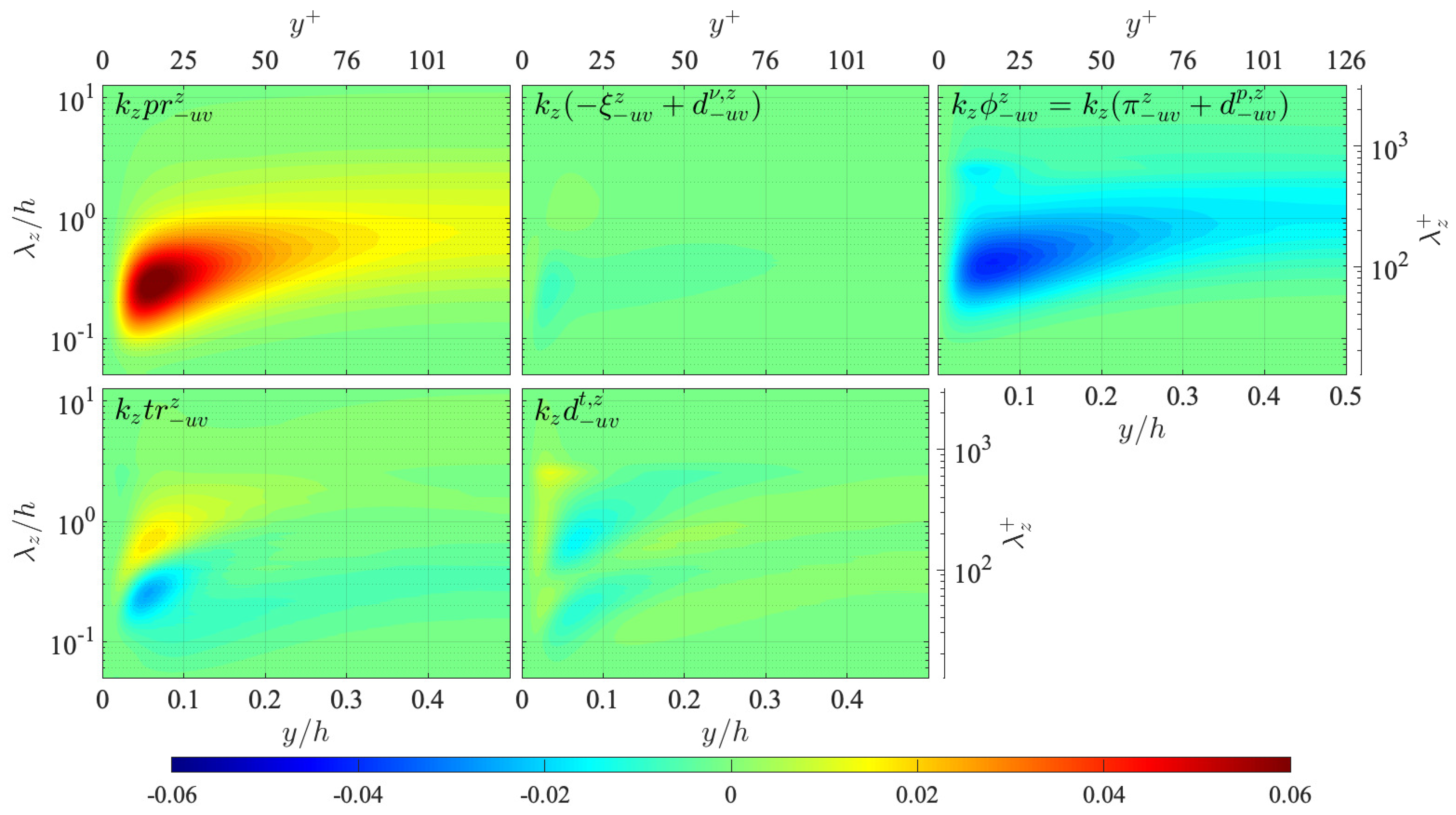
References
- Kawata, T.; Alfredsson, P.H. Inverse Interscale Transport of the Reynolds shear stress in plane Couette turbulence. Phys. Rev. Lett. 2018, 120, 244501. [Google Scholar] [CrossRef]
- Wang, W.; Pan, C.; Wang, J. Energy transfer structures associated with large-scale motions in a turbulent boundary layer. J. Fluid Mech. 2020, 906, A14. [Google Scholar] [CrossRef]
- Doohan, P.; Wills, A.P.; Hwang, Y. Minimal multi-scale dynamics of near-wall turbulence. J. Fluid Mech. 2021, 913, A8. [Google Scholar] [CrossRef]
- Kawata, T.; Tsukahara, T. Scale interactions in turbulent plane Couette flows in minimal domains. J. Fluid Mech. 2021, 911. [Google Scholar] [CrossRef]
- Chan, C.; Schlatter, P.; Chin, R. Interscale transport mechanisms in turbulent boundary layers. J. Fluid Mech. 2021, 921, A13. [Google Scholar] [CrossRef]
- Mizuno, Y. Spectra of energy transport in turbulent channel flows for moderate Reynolds numbers. J. Fluid Mech. 2016, 805, 171–187. [Google Scholar]
- Lee, M.; Moser, R.D. Spectral analysis of the budget equation in turbulent channel flows at high Reynolds number. J. Fluid Mech. 2019, 860, 886–938. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Yang, Z.; Wu, T.; Wang, S. Coherent structures associated with interscale energy transfer in turbulent channel flows. Phys. Rev. Fluids 2021, 6, 104601. [Google Scholar] [CrossRef]
- Cimarelli, A.; De Angelis, E.; Casciola, C.M. Paths of energy in turbulent channel flows. J. Fluid Mech. 2013, 715, 436–451. [Google Scholar] [CrossRef]
- Cimarelli, A.; De Angelis, E.; Jiménez, J.; Casciola, C.M. Cascades and wall-normal fluxes in turbulent channel flows. J. Fluid Mech. 2016, 796, 417–436. [Google Scholar]
- Chiarini, A.; Mauriello, M.; Gatti, D.; Quadrio, M. Ascending-descending and direct-inverse cascades of Reynolds stresses in turbulent Couette flow. J. Fluid Mech. 2021, 930, A9. [Google Scholar] [CrossRef]
- Smits, A.; McKeon, B.; Marusic, I. High–Reynolds number wall turbulence. Annu. Rev. Fluid Mech. 2011, 43, 353–375. [Google Scholar] [CrossRef] [Green Version]
- Cho, M.; Hwang, Y.; Choi, H. Scale interactions and spectral energy transfer in turbulent channel flow. J. Fluid Mech. 2018, 854, 474–504. [Google Scholar] [CrossRef]
- Hamba, F. Turbulent energy density in scale space for inhomogeneous turbulence. J. Fluid Mech. 2018, 842, 532–553. [Google Scholar] [CrossRef]
- Kawamura, H.; Ohsaka, K.; Abe, H.; Yamamoto, K. DNS of turbulent heat transfer in channel flow with low to medium-high Prandtl number fluid. Int. J. Heat Fluid Flow 1998, 19, 482–491. [Google Scholar] [CrossRef]
- Kawamura, H.; Abe, H.; Matsuo, Y. DNS of turbulent heat transfer in channel flow with respect to Reynolds and Prandtl number effects. Int. J. Heat Fluid Flow 1999, 20, 196–207. [Google Scholar] [CrossRef]
- Hane, S.; Tsukahara, T.; Iwamoto, K.; Kawamura, H. DNS of turbulent heat transfer in plane Couette flow. In Proceedings of the International Heat Transfer Conference, Sydney, Australia, 13–18 August 2006. [Google Scholar] [CrossRef]
- Tsukahara, T.; Iwamoto, K.; Kawamura, H. On the large-scale structure of turbulent heat transfer in a plane Couette flow. Therm. Sci. Eng. 2007, 15, 151–161. (In Japanese) [Google Scholar]
- Antonia, R.A.; Abe, H. Analogy between velocity and scalar fields in a turbulent channel flow. J. Fluid Mech. 2009, 628, 241–268. [Google Scholar] [CrossRef]
- Abe, H.; Kawamura, H.; Toh, S.; Itano, T. Effect of the streamwise computational domain size on DNS of a turbulent channel flow at high Reynolds number. In Advances in Turbulence XI; Palma, J.M.L.M., Lopes, A.S., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 233–235. [Google Scholar]
- Alcántara-Ávila, F.; Hoyas, S.; Pérez-Quiles, M.J. DNS of thermal channel flow up to Reτ=2000 for medium to low Prandtl numbers. Int. J. Heat Mss Transf. 2018, 127, 349–361. [Google Scholar] [CrossRef]
- Alcántara-Ávila, F.; Gandía-Barberá, S.; Hoyas, S. Evidences of persisting thermal structures in Couette flows. Int. J. Heat Fluid Flow 2019, 76, 287–295. [Google Scholar] [CrossRef]
- Alcántara-Ávila, F.; Hoyas, S.; Jezabel Pérez-Quiles, M. Direct numerical simulation of thermal channel flow for Reτ=5000 and Pr=0.71. J. Fluid Mech. 2021, 916, A29. [Google Scholar] [CrossRef]
- Alcántara-Ávila, F.; Hoyas, S. Direct numerical simulation of thermal channel flow for medium–high Prandtl numbers up to Reτ=2000. Int. J. Heat Mss Transf. 2021, 176, 1214112. [Google Scholar] [CrossRef]
- Komminaho, J.; Lundbladh, A.; Johansson, A.V. Very large structures in plane turbulent Couette flow. J. Fluid Mech. 1996, 320, 259–285. [Google Scholar] [CrossRef]
- Kitoh, O.; Nakabayashi, K.; Nishimura, F. Experimental study on mean velocity and turbulence characteristics of plane Couette flow: Low-Reynolds-number effects and large longitudinal vortical structure. J. Fluid Mech. 2005, 539, 199–227. [Google Scholar] [CrossRef]
- Tsukahara, T.; Kawamura, H.; Shingai, K. DNS of turbulent Couette flow with emphasis on the large-scale structure in the core region. J. Turbul. 2006, 7, N19. [Google Scholar] [CrossRef]
- Kitoh, O.; Umeki, M. Experimental study on large-scale streak structure in the core region of turbulent plane Couette flow. Phys. Fluids 2008, 20, 025107. [Google Scholar] [CrossRef]
- Avsarkisov, V.; Hoyas, S.; Oberlack, M.; García-Galache, J.P. Turbulent plane Couette flow at moderately high Reynolds number. J. Fluid Mech. 2015, 751, R1. [Google Scholar] [CrossRef]
- Orlandi, P.; Bernardini, M.; Pirozzoli, S. Poiseuille and Couette flows in the transitional and fully turbulent regime. J. Fluid Mech. 2015, 770, 424–441. [Google Scholar] [CrossRef]
- Lee, M.; Moser, R.D. Extreme-scale motions in turbulent plane Couette flows. J. Fluid Mech. 2018, 842, 128–145. [Google Scholar] [CrossRef] [Green Version]
- Kawata, T.; Tsukahara, T. Spectral analysis on dissimilarity between the turbulent heat and momentum transport in plane Couette turbulence. Phys. Fluids 2022. [Google Scholar] [CrossRef]
- Kawata, T.; Alfredsson, P.H. Scale interactions in turbulent rotating planar Couette flow: Insight through the Reynolds stress transport. J. Fluid Mech. 2019, 879, 255–295. [Google Scholar] [CrossRef]
- Toh, S.; Itano, T. Interaction between a large-scale structure and near-wall structures in channel flow. J. Fluid Mech. 2005, 524, 249–262. [Google Scholar] [CrossRef]
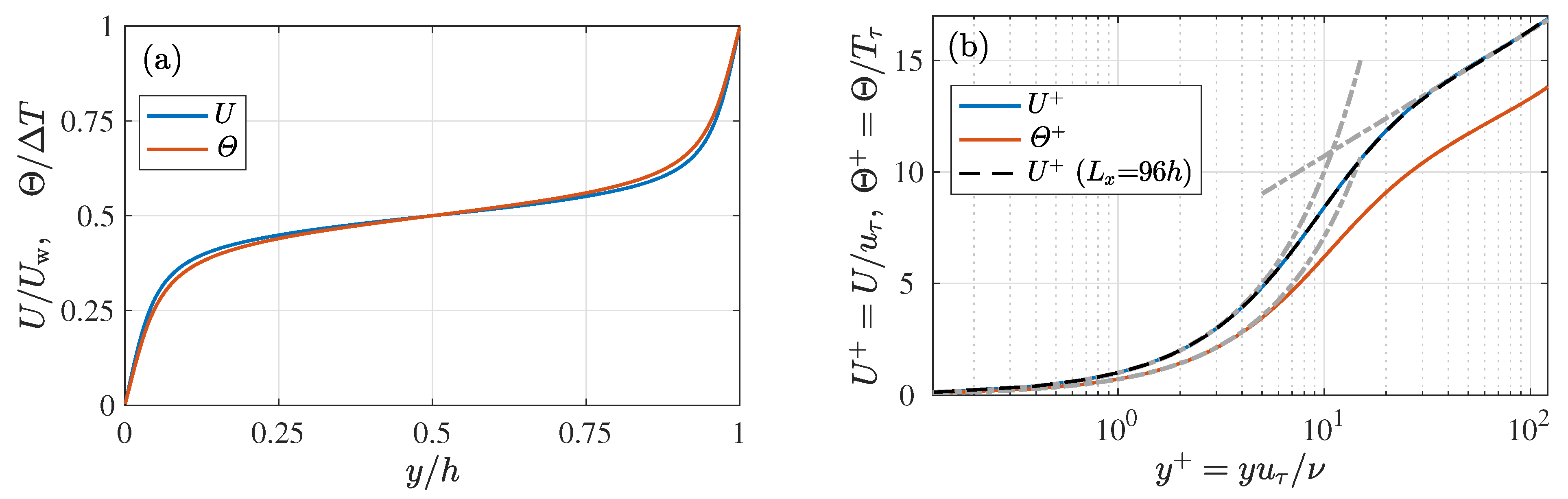

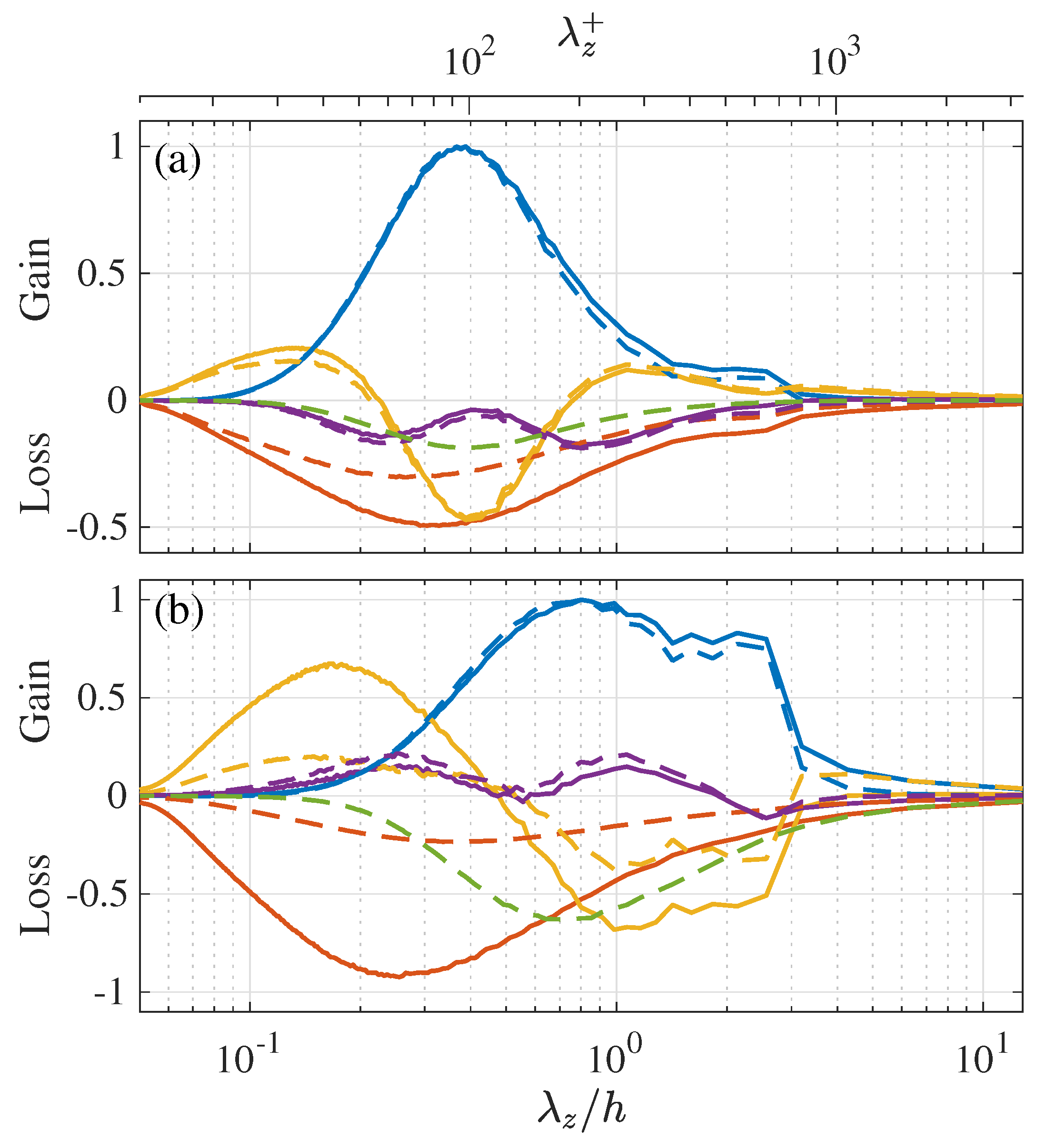

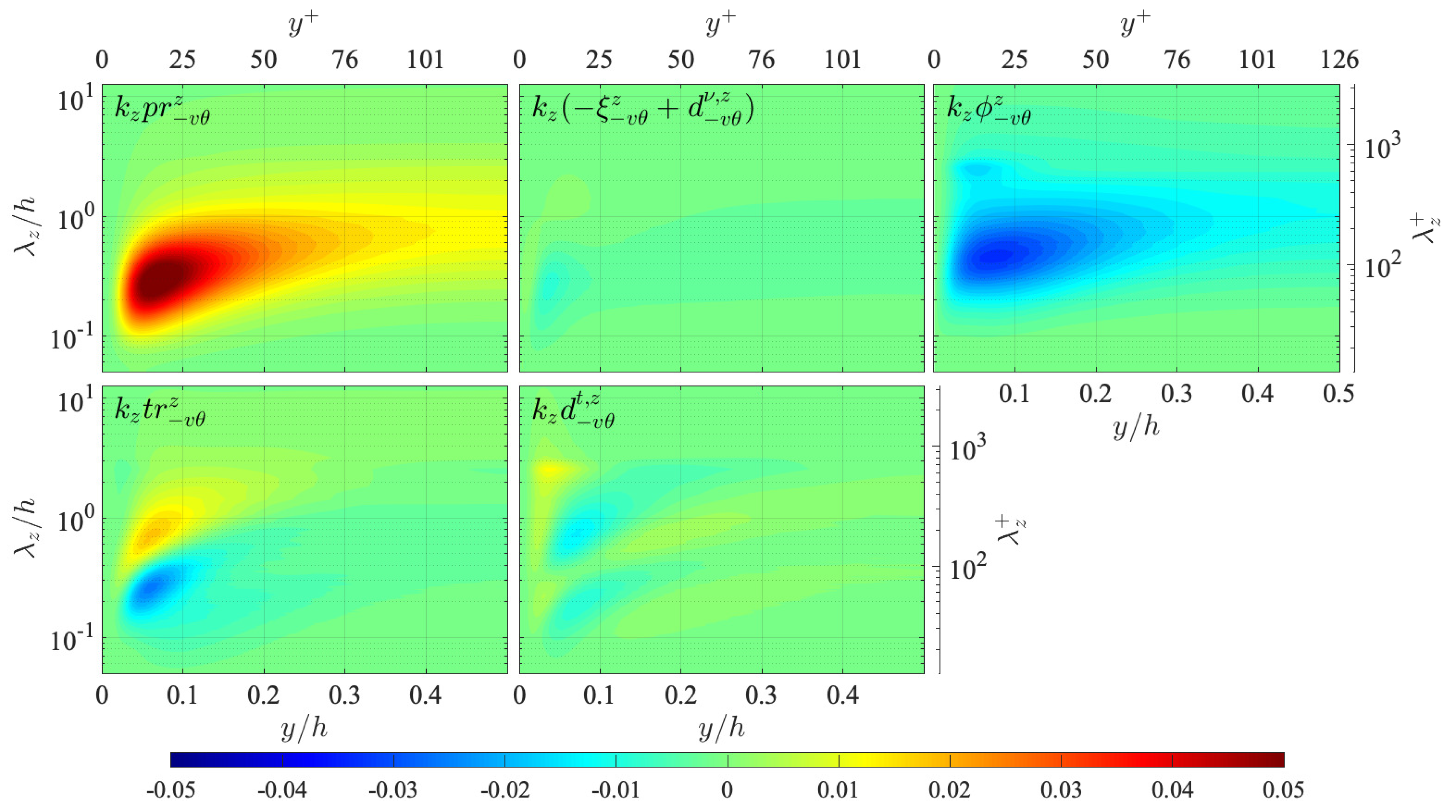
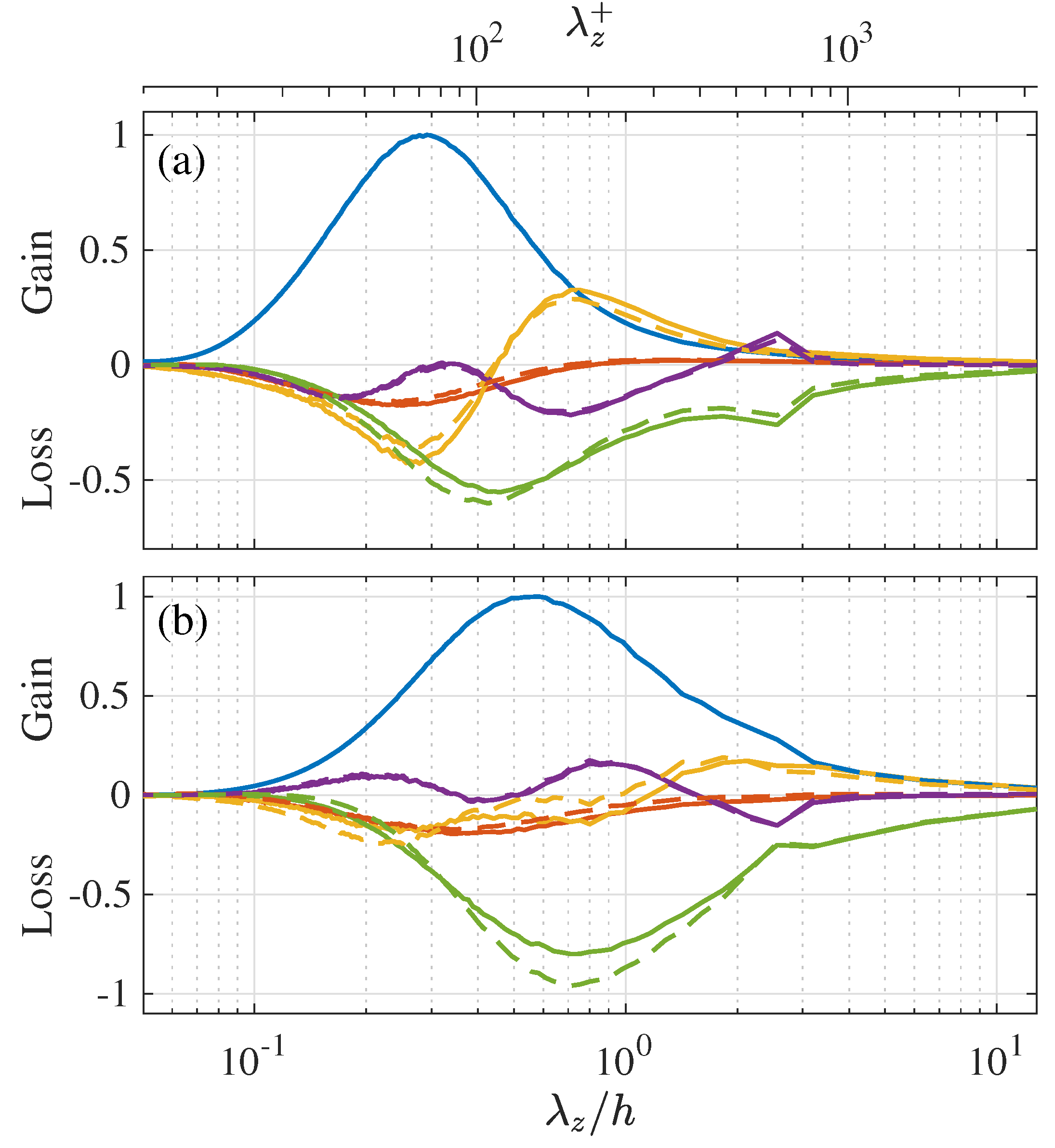
| (24 h, 12.8 h) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kawata, T.; Tsukahara, T. Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence. Energies 2022, 15, 5258. https://doi.org/10.3390/en15145258
Kawata T, Tsukahara T. Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence. Energies. 2022; 15(14):5258. https://doi.org/10.3390/en15145258
Chicago/Turabian StyleKawata, Takuya, and Takahiro Tsukahara. 2022. "Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence" Energies 15, no. 14: 5258. https://doi.org/10.3390/en15145258
APA StyleKawata, T., & Tsukahara, T. (2022). Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence. Energies, 15(14), 5258. https://doi.org/10.3390/en15145258







