Self-Excited Acoustical System Frequency Monitoring for Refractory Concrete under Uniaxial Compression
Abstract
1. Introduction
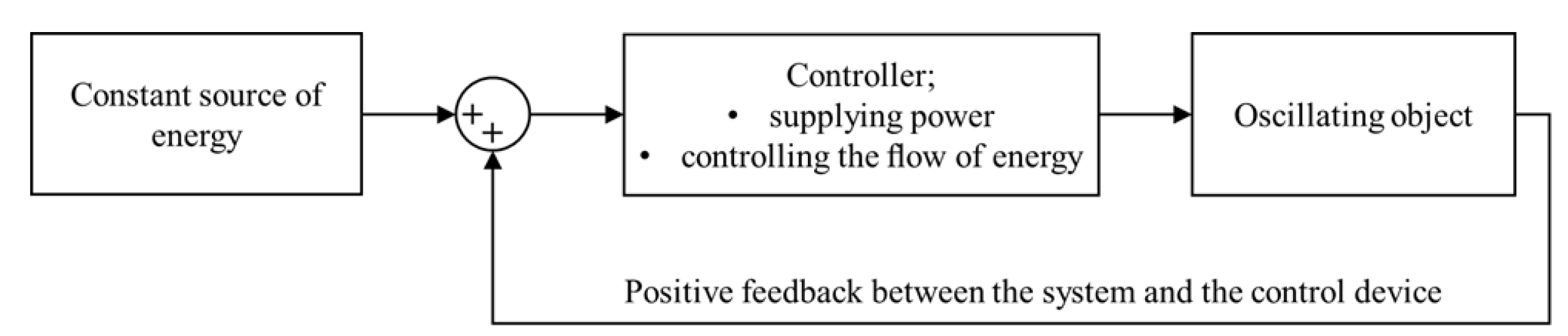
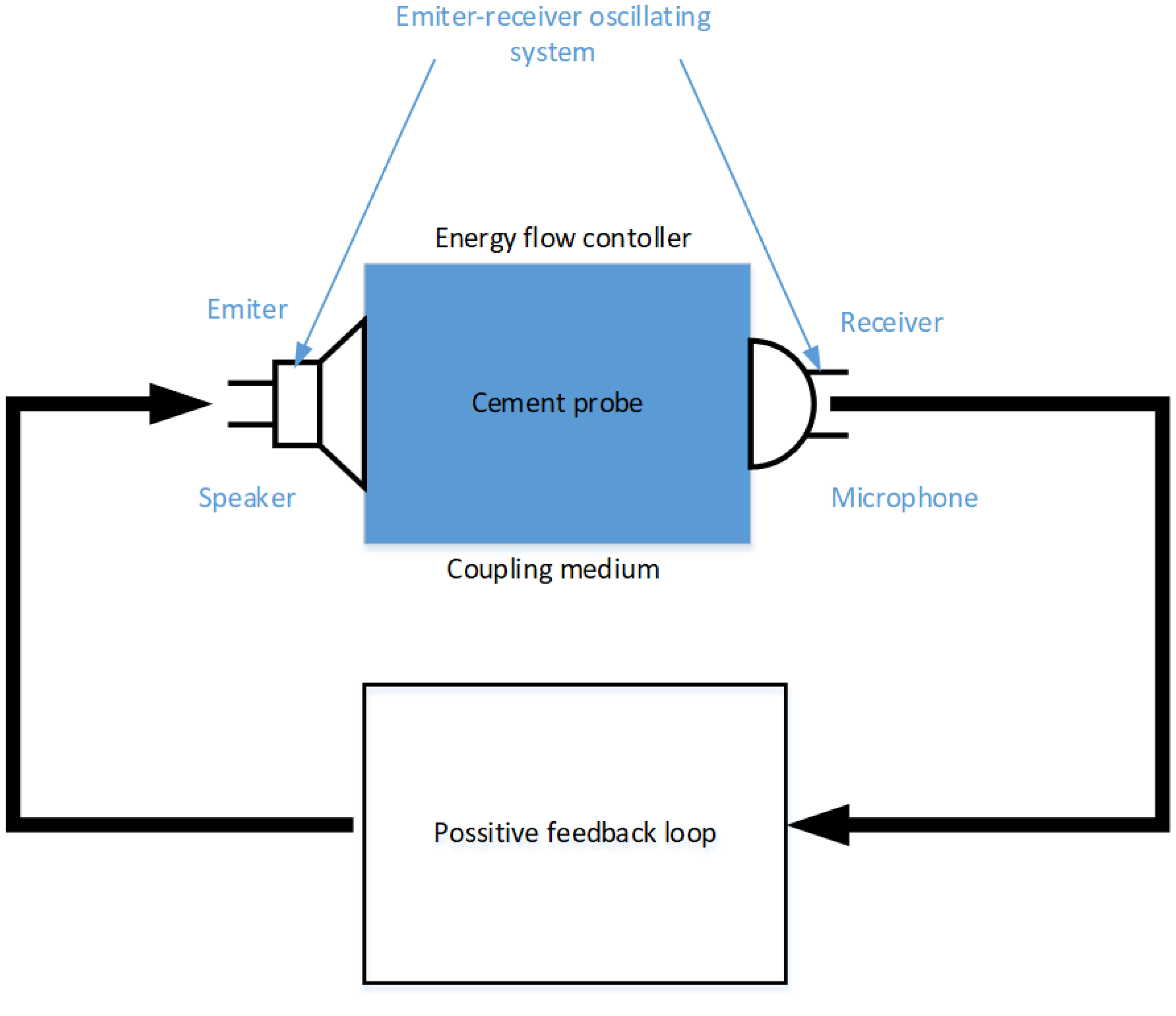
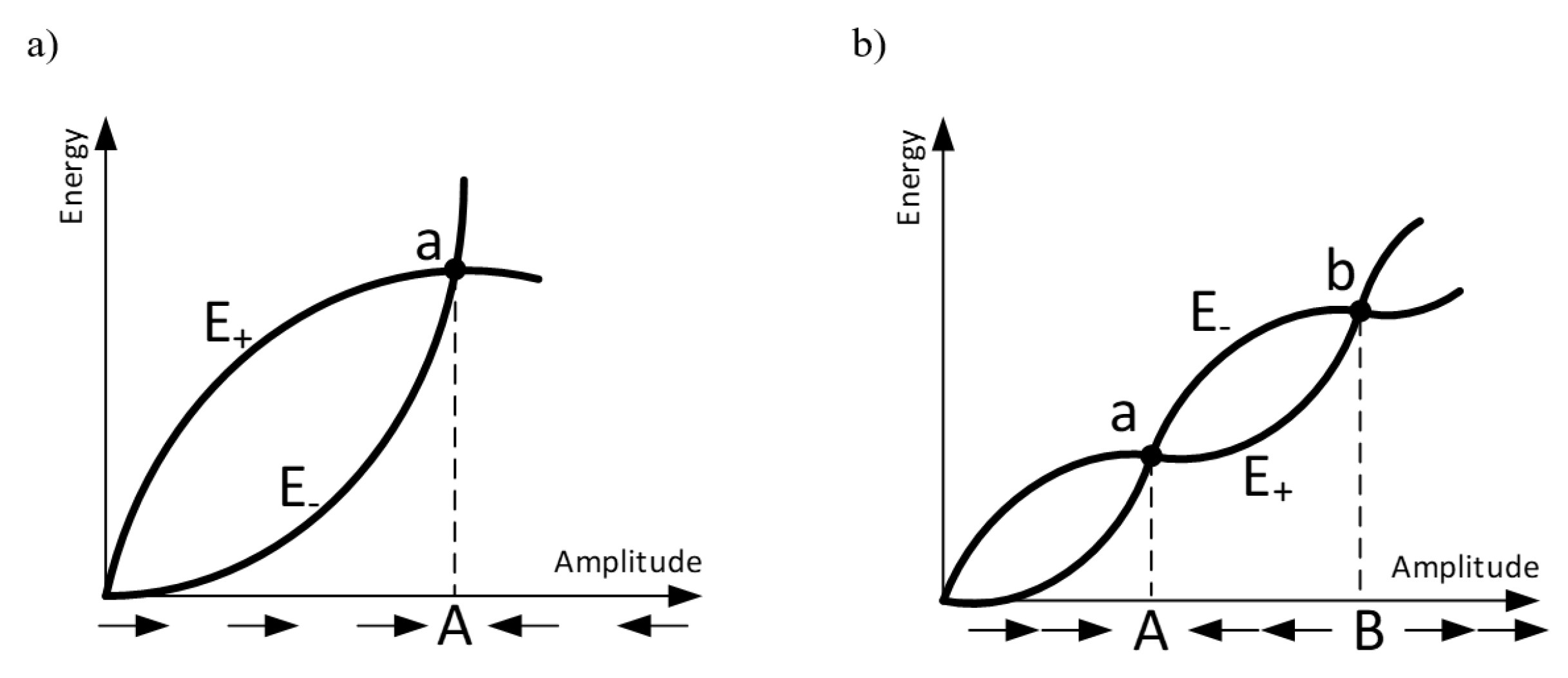
2. The Self-Excited Acoustical System (SAS) for Indirect Stress Changes Monitoring
- —wave velocity of the stresses sample [],
- —wave velocity of unstressed sample [],
- —acoustoelastic coefficient [],
- —stress [].
- the energy source (constant),
- oscillating object,
- energy supply regulator for an oscillating object,
- positive feedback loop.
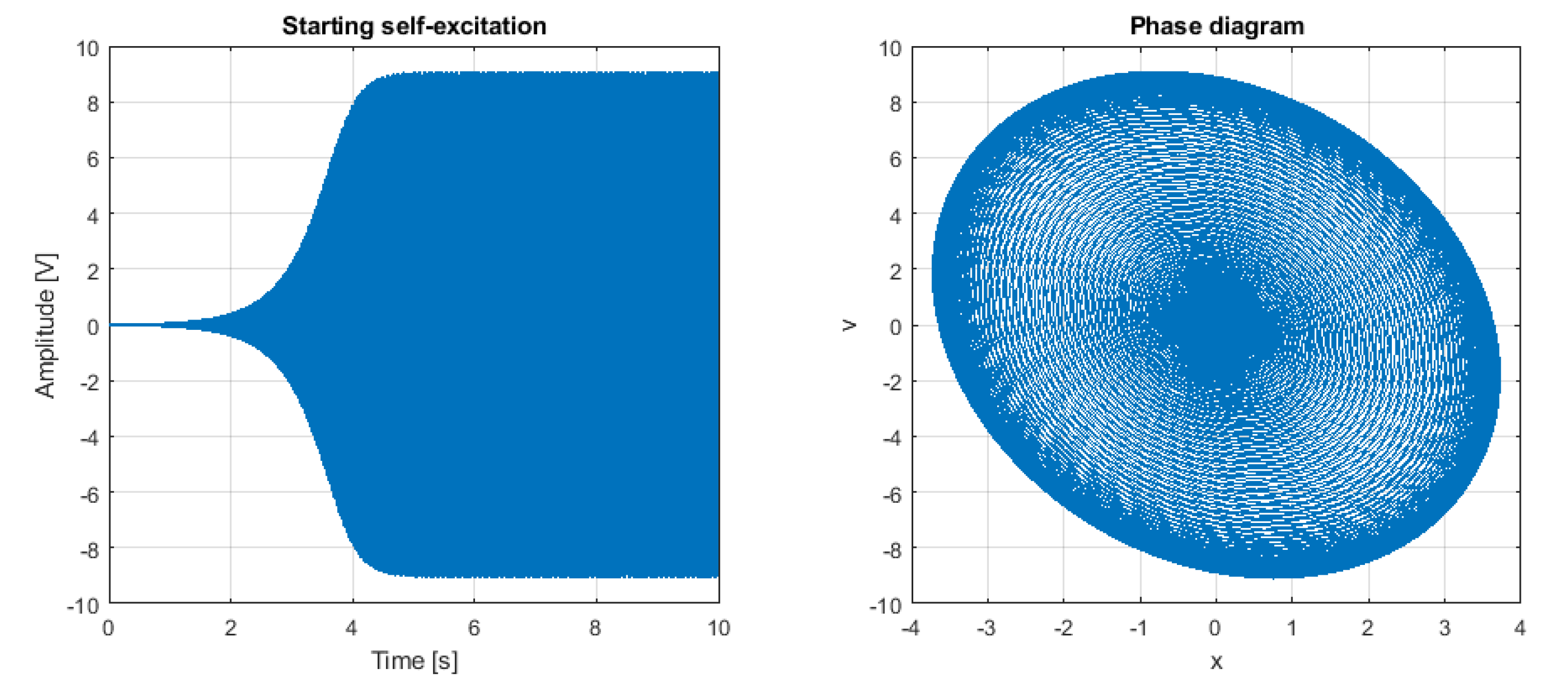
3. Research Methodology
4. Experimental
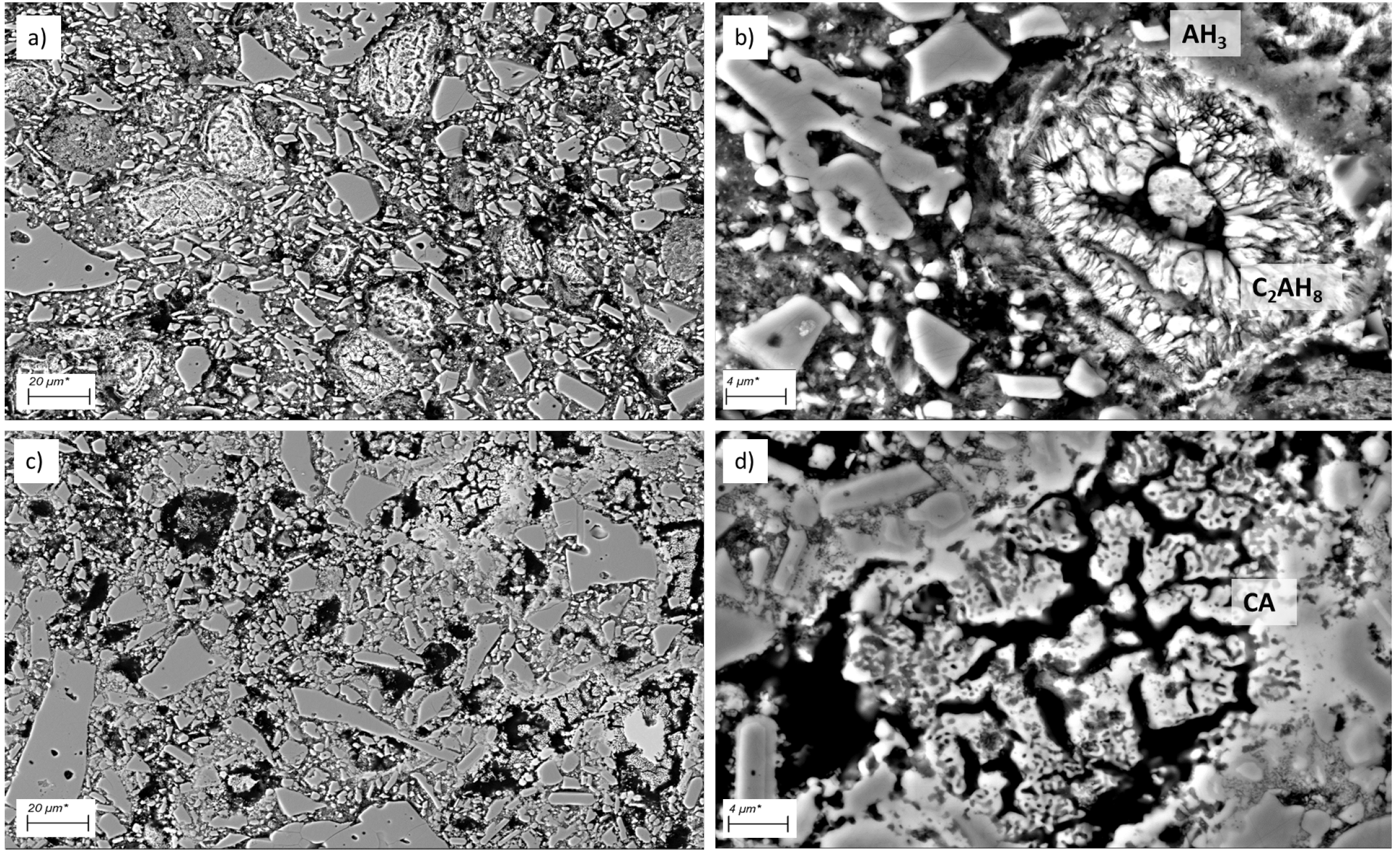
4.1. Materials Preparation and Characterization Techniques
4.2. SAS Resonance Frequency Monitoring for Concrete Samples with Different Thermal History
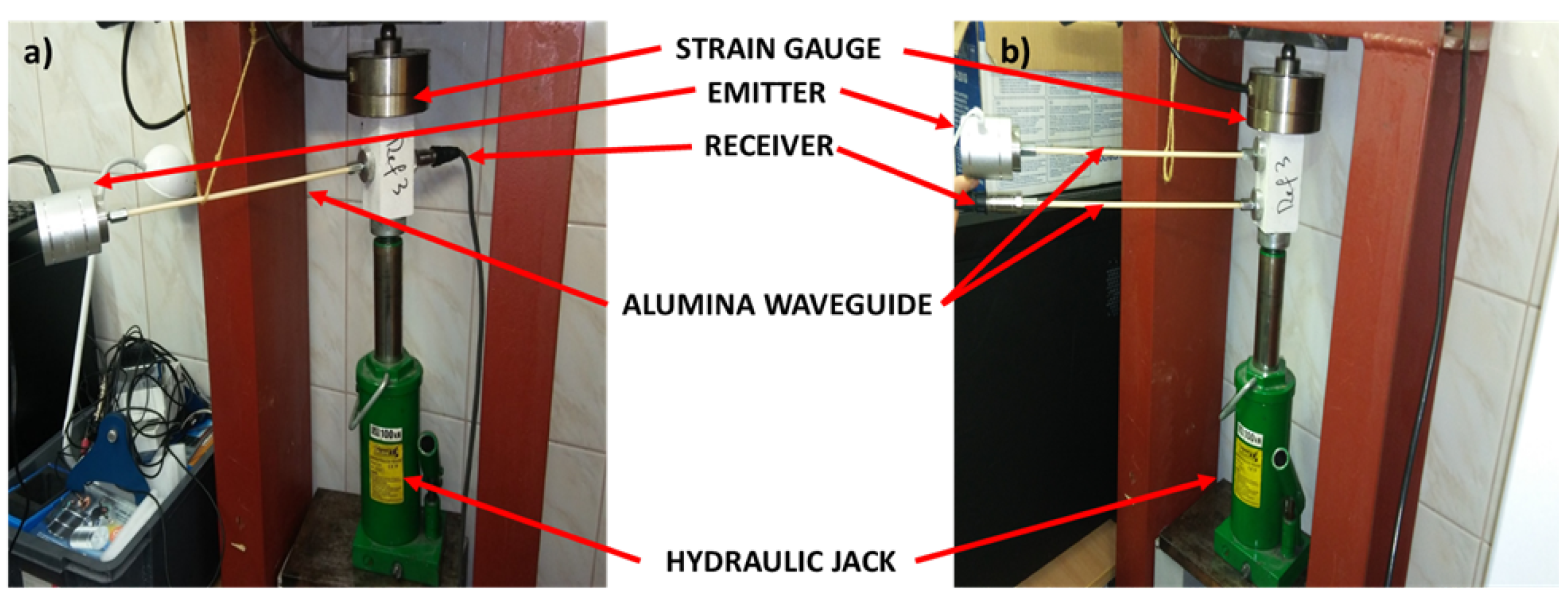
4.3. Introduction of Alumina Waveguide to SAS
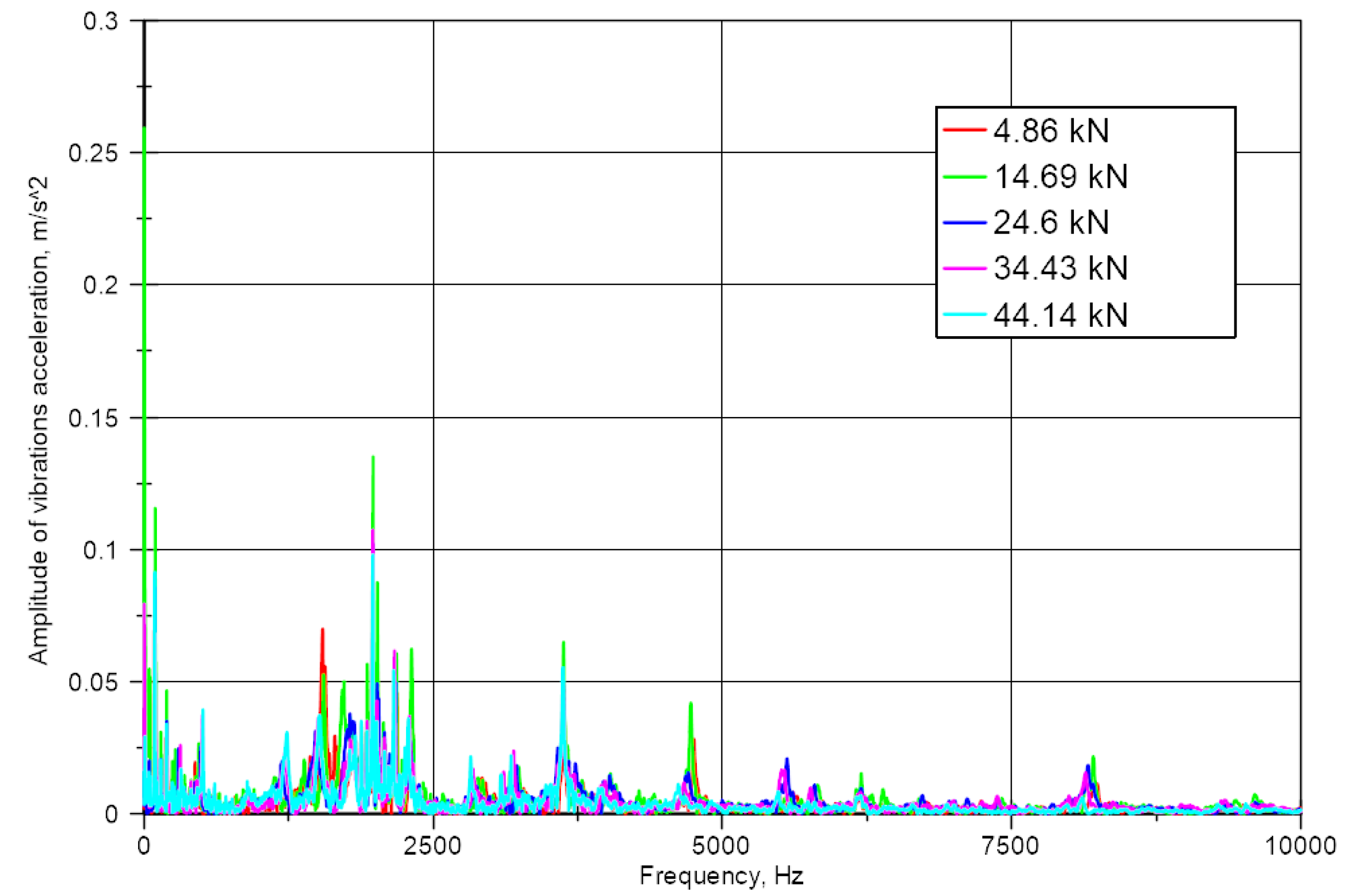
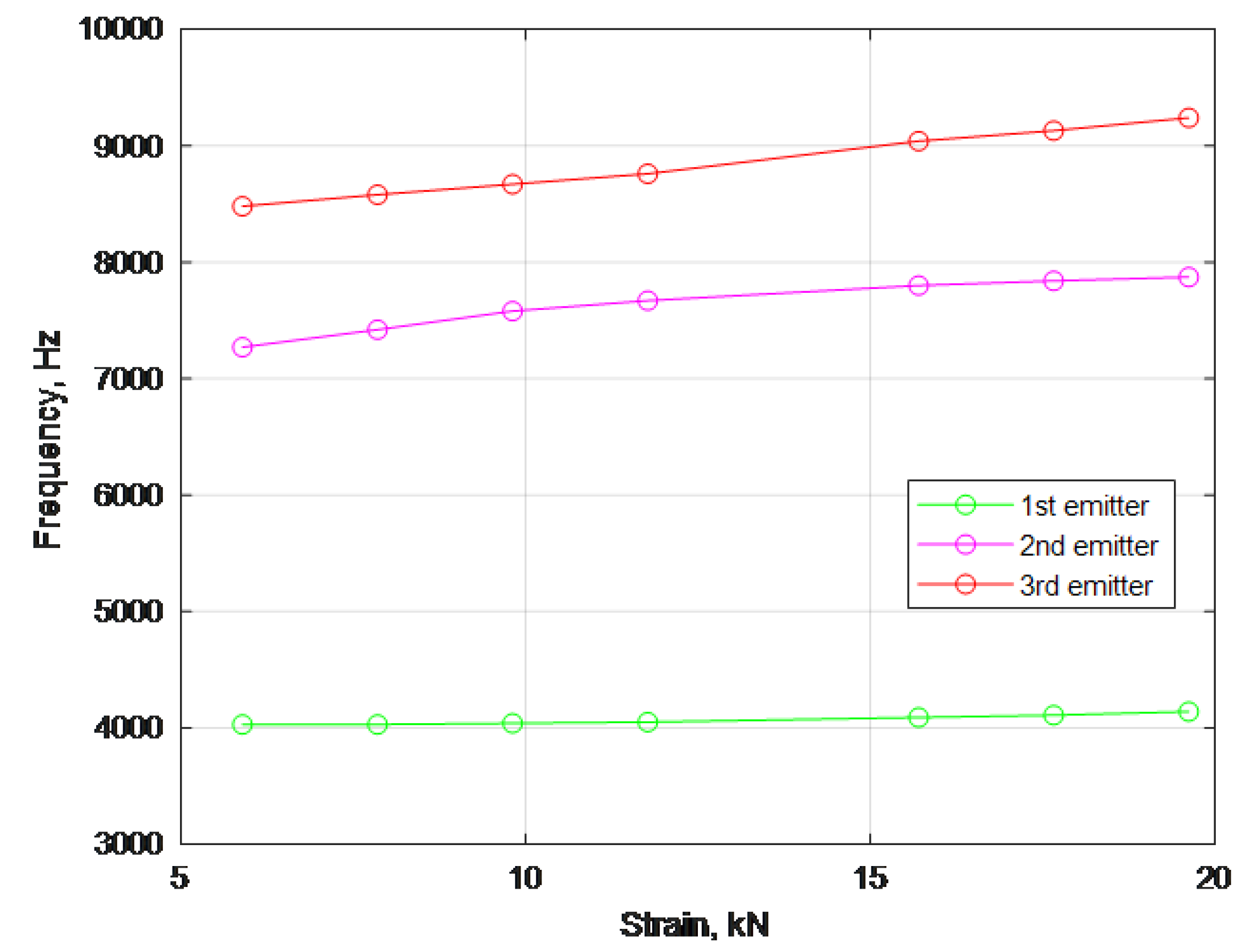
5. Results
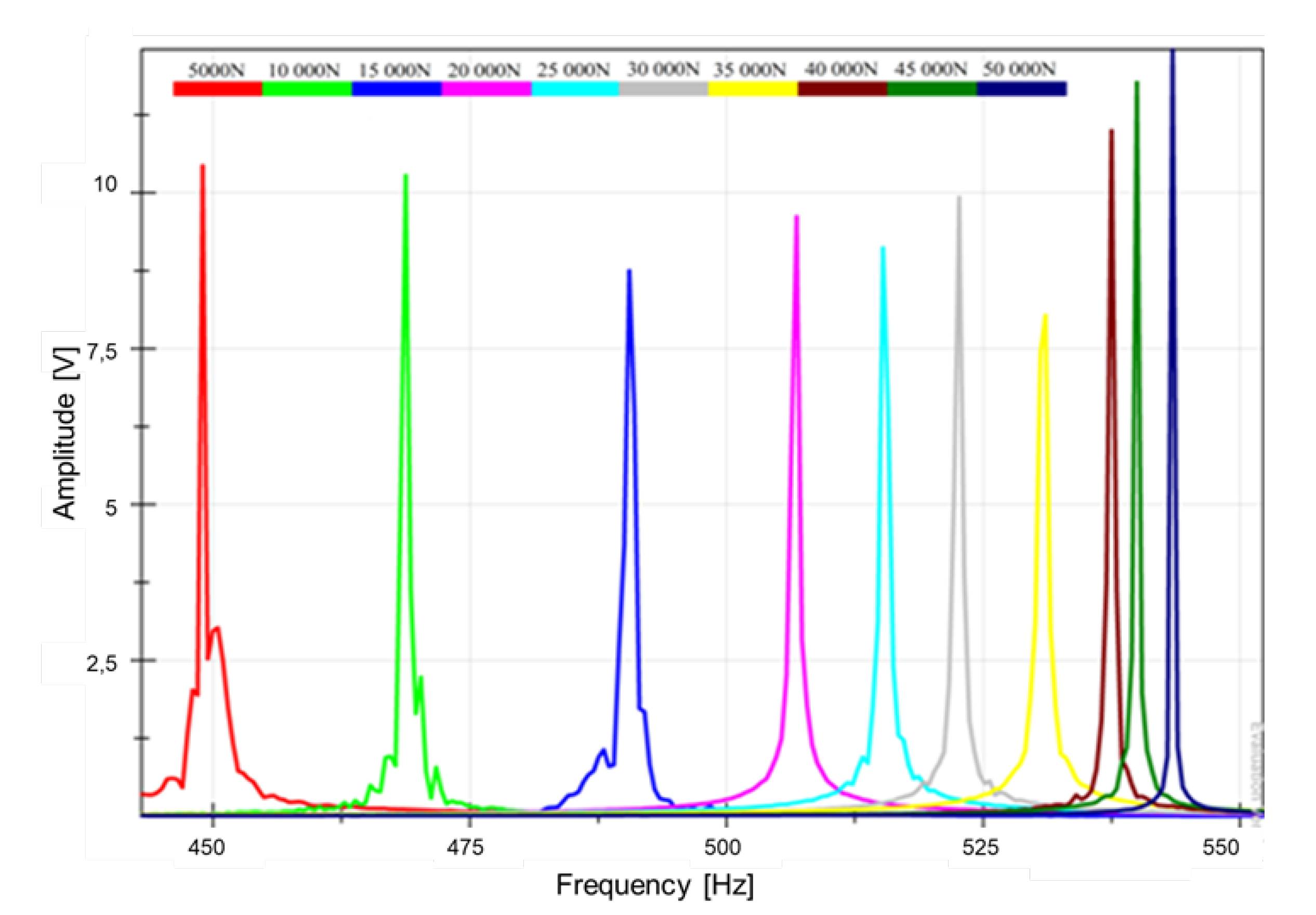
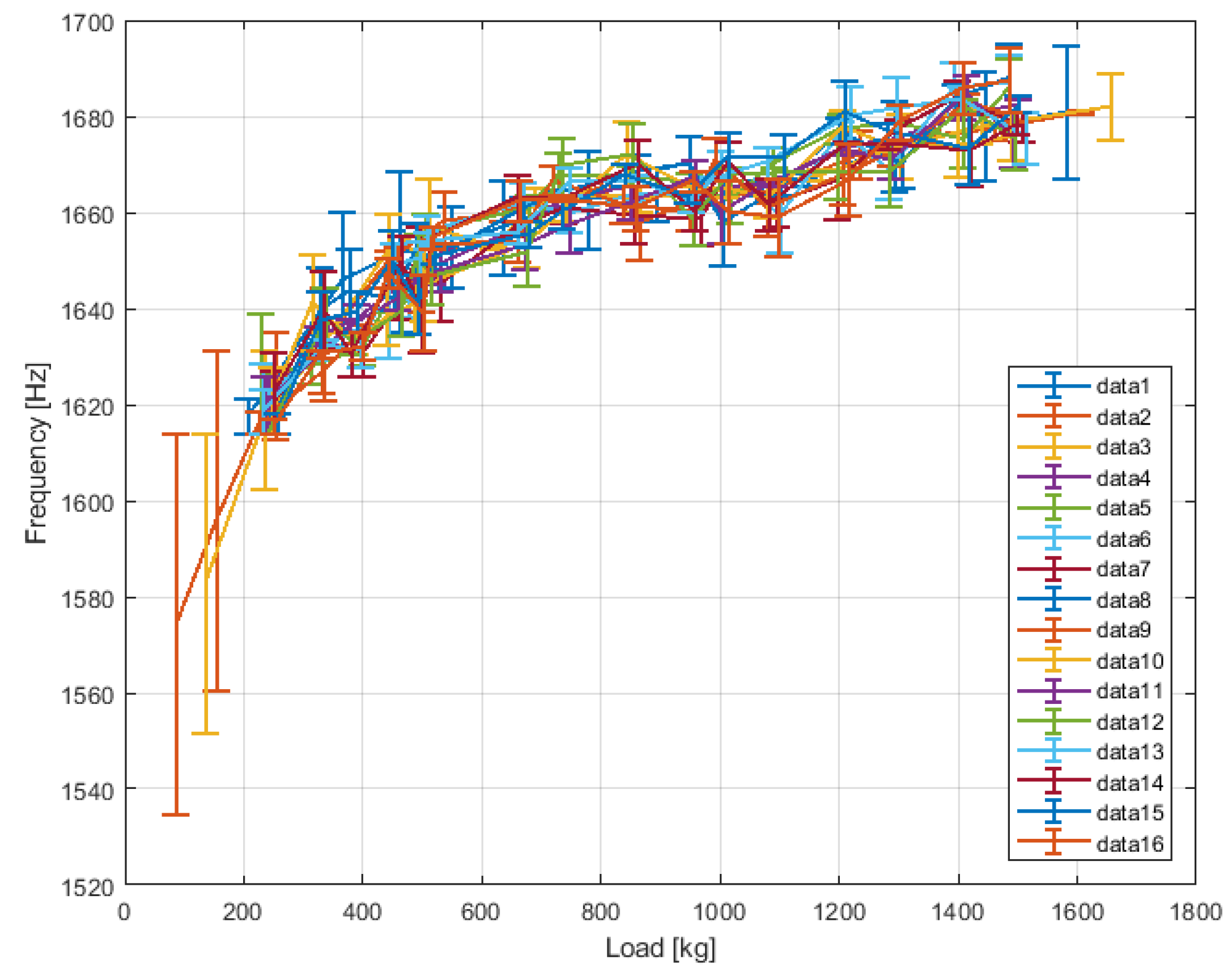
5.1. Load and Resonance Frequency Characteristics of Materials with Different Thermal History
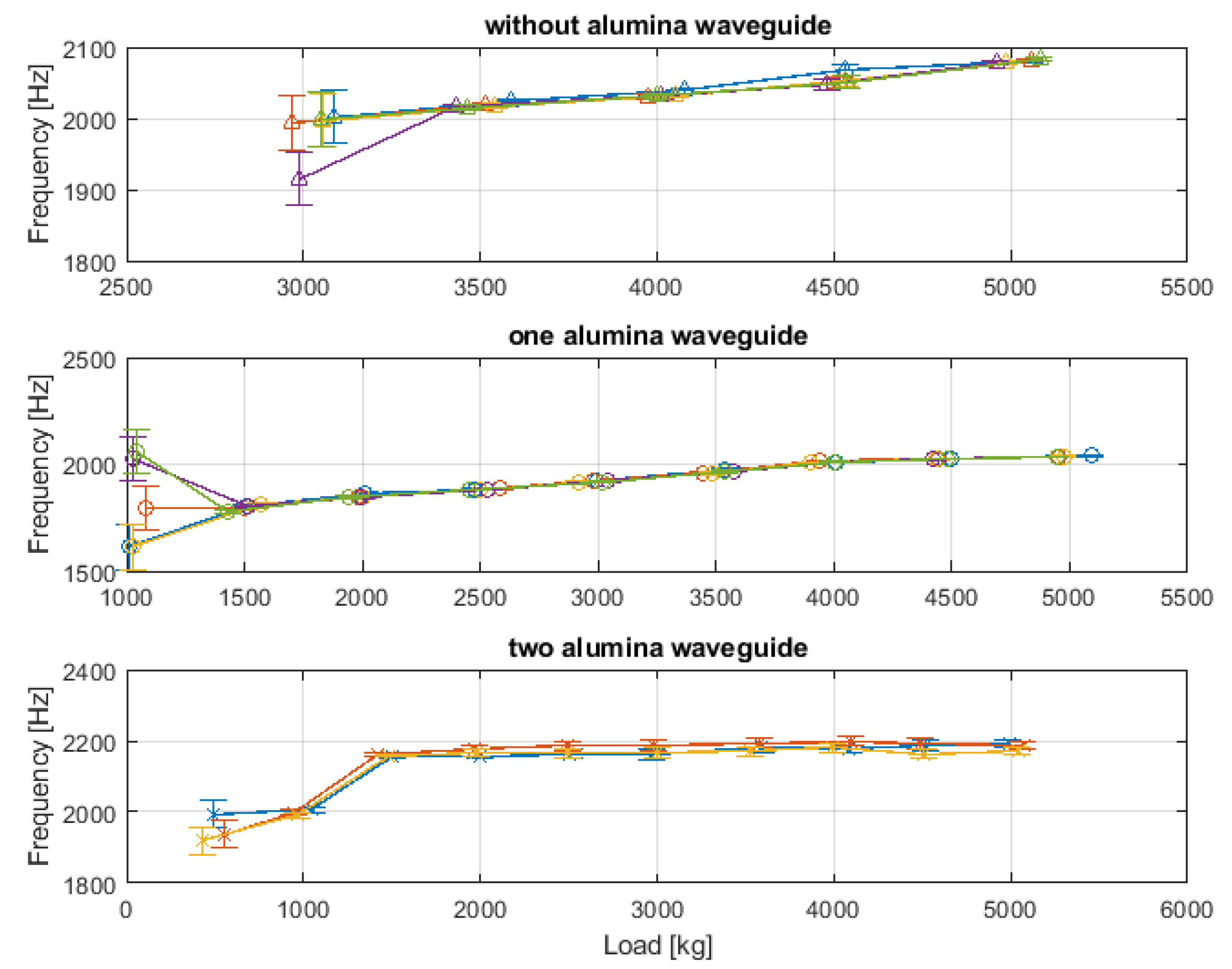
5.2. Resonance Frequency Monitoring with SAS Working in Conjunction with Alumina Waveguide
6. Discussion
6.1. Influence of the Thermal History on the Load and Resonance Frequency Characteristics
6.2. Introduction of Alumina Waveguide to SAS
- —frequency of the SAS system [],
- —time constant for the measurement stand [s],
- —delay time for wave transmission between the emitter and receiver [s],
- —dumping coefficient [−].
6.3. Limitations of the Study
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Steiner, R.; Lammer, G.; Spiel, C.; Jandl, C. Refractories 4.0. BHM Berg-Hüttenmännische Monatshefte 2017, 162, 514–520. [Google Scholar] [CrossRef]
- Tomsu, F.; Palco, S. Refractory monolithics versus shaped refractory products. Interceram Int. Ceram. Rev. 2017, 66, 20–23. [Google Scholar] [CrossRef]
- Lee, W.; Zhang, S.; Karakus, M. Refractories: Controlled microstructure composites for extreme environments. J. Mater. Sci. 2004, 39, 6675–6685. [Google Scholar] [CrossRef]
- Jin, J.; Rivière, J.; Ohara, Y.; Shokouhi, P. Dynamic acousto-elastic response of single fatigue cracks with different microstructural features: An experimental investigation. J. Appl. Phys. 2018, 124, 075303. [Google Scholar] [CrossRef]
- Ankay, B.; Zhang, C. Acoustoelastic evaluation of ultra-high performance concretes. In AIP Conference Proceedings; AIP Publishing LLC.: Melville, NY, USA, 2019; Volume 2102, p. 110002. [Google Scholar] [CrossRef]
- Gutiérrez-Vargas, G.; Ruiz, A.; Kim, J.Y.; Jacobs, L.J. Characterization of thermal embrittlement in 2507 super duplex stainless steel using nonlinear acoustic effects. NDT E Int. 2018, 94, 101–108. [Google Scholar] [CrossRef]
- Kwaśniewki, J.; Dominik, I.; Lalik, K.; Holewa, K. Influence of acoustoelastic coefficient on wave time of flight in stress measurement in piezoelectric self-excited system. Mech. Syst. Signal Process. 2016, 78, 143–155. [Google Scholar] [CrossRef]
- Masurkar, F.; Tse, P.W.; Yelve, N.P. Theoretical and experimental measurement of intrinsic and fatigue induced material nonlinearities using Lamb wave based nonlinearity parameters. Meas. J. Int. Meas. Confed. 2020, 151, 107148. [Google Scholar] [CrossRef]
- Yang, Y.; Ng, C.T.; Mohabuth, M.; Kotousov, A. Finite element prediction of acoustoelastic effect associated with Lamb wave propagation in pre-stressed plates. Smart Mater. Struct. 2019, 28, 095007. [Google Scholar] [CrossRef]
- Pei, N.; Bond, L.J. Comparison of acoustoelastic Lamb wave propagation in stressed plates for different measurement orientations. J. Acoust. Soc. Am. 2017, 142, EL327–EL331. [Google Scholar] [CrossRef]
- Chen, P.; He, X.; Wang, X. Ultrasonic Measurement of Axial Stress Using High-frequency Cylindrical Guided Wave. IEEE Sens. J. 2020, 21, 6691–6697. [Google Scholar] [CrossRef]
- Bompan, K.F.; Haach, V.G. Ultrasonic tests in the evaluation of the stress level in concrete prisms based on the acoustoelasticity. Constr. Build. Mater. 2018, 162, 740–750. [Google Scholar] [CrossRef]
- Shokouhi, P.; Zoëga, A.; Wiggenhauser, H.; Fischer, G. Surface wave velocity-stress relationship in uniaxially loaded concrete. ACI Mater. J. 2012, 109, 131–139. [Google Scholar] [CrossRef][Green Version]
- Lott, M.; Remillieux, M.C.; Garnier, V.; Le Bas, P.Y.; Ulrich, T.J.; Payan, C. Nonlinear elasticity in rocks: A comprehensive three-dimensional description. Phys. Rev. Mater. 2017, 1, 023603. [Google Scholar] [CrossRef]
- Pau, A.; Vestroni, F. The role of material and geometric nonlinearities in acoustoelasticity. Wave Motion 2019, 86, 79–90. [Google Scholar] [CrossRef]
- Muñoz, C.Q.G.; Márquez, F.P.G. A new fault location approach for acoustic emission techniques in wind turbines. Energies 2016, 9, 40. [Google Scholar] [CrossRef]
- Perugia, I.; Schöberl, J.; Stocker, P.; Wintersteiger, C. Tent pitching and Trefftz-DG method for the acoustic wave equation. Comput. Math. Appl. 2020, 79, 2987–3000. [Google Scholar] [CrossRef]
- Carcione, A.; Blanloeuil, P.; Rose, L.R.; Wang, C.H.; Veidt, M. Modulated high frequency excitation approach to nonlinear ultrasonic NDT. J. Sound Vib. 2019, 446, 238–248. [Google Scholar] [CrossRef]
- Abbasi, Z.; Ozevin, D. The influence of ultrasonic frequency on shear stress measurement using acoustoelasticity. In AIP Conference Proceedings; AIP Publishing LLC.: Melville, NY, USA, 2016. [Google Scholar] [CrossRef]
- Dubuc, B.; Ebrahimkhanlou, A.; Salamone, S. Higher order longitudinal guided wave modes in axially stressed seven-wire strands. Ultrasonics 2018, 84, 382–391. [Google Scholar] [CrossRef]
- Dominik, I.; Lalik, K.; Flaga, S. Modeling of self-excited stress measurement system. J. Low Freq. Noise Vib. Act. Control 2020, 146134842092945. [Google Scholar] [CrossRef]
- Lalik, K.; Dominik, I.; Ćwia̧kała, P.; Kwaśniewski, J. Integrated stress measurement system in tower crane mast. Meas. J. Int. Meas. Confed. 2017, 102, 47–56. [Google Scholar] [CrossRef]
- Skrzypkowski, K.; Korzeniowski, W.; Zagórski, K.; Dominik, I.; Lalik, K. Fast, non-destructive measurement of roof-bolt loads. Studia Geotech. Mech. 2019, 41. [Google Scholar] [CrossRef]
- Kwaśniewski, J.; Dominik, I.; Lalik, K. A Self-Excited Acoustical System for Stress Measurement in a Cement Plant. Mech. Control 2012, 31, 29. [Google Scholar] [CrossRef]
- Kwaśniewki, J.; Dominik, I.; Lalik, K. Application of self-oscillating system for stress measurement in metal. J. Vibroeng. 2012, 14, 1429–2955. [Google Scholar]
- Roebben, G.; Bollen, B.; Brebels, A.; Van Humbeeck, J.; der Biest, O. Impulse excitation apparatus to measure resonant frequencies, elastic moduli, and internal friction at room and high temperature. Rev. Sci. Instrum. 1997, 68, 4511–4515. [Google Scholar] [CrossRef]
- Bradt, R.C.; Alton, N. Elastic properties of refractories- their role and characterization. Refract. Appl. News 2007, 12. [Google Scholar]
- Etzold, S.; Tarabeux, J.; Porada, R.; Tonnesen, T.; Telle, R. Thermomechanical behavior of high-alumina refractory castables containing partially stabilized zirconia with different grain shapes. In Proceedings of the 15th Biennial Worldwide Congress UNITECR, Santiago, Chile, 26–29 September 2017; pp. 54–57. [Google Scholar]
- Huger, M.; Fargeot, D.; Gault, C. High-temperature measurement of ultrasonic wave velocity in refractory materials. High Temp.-High Press. 2002, 34, 193–201. [Google Scholar] [CrossRef]
- Lundqvist, P.; Rydén, N. Acoustoelastic effects on the resonance frequencies of prestressed concrete beams—Short-term measurements. NDT E Int. 2012, 50, 36–41. [Google Scholar] [CrossRef]
- Shokouhi, P.; Zoëga, A.; Wiggenhauser, H. Nondestructive investigation of stress-induced damage in concrete. Adv. Civ. Eng. 2010, 2010, 740189. [Google Scholar] [CrossRef]
- Popovics, S.; Popovics, J.S. Effect of stresses on the ultrasonic pulse velocity in concrete. Mater. Struct. 1991, 24, 15–23. [Google Scholar] [CrossRef]
- Berthaud, Y. Damage measurements in concrete via an ultrasonic technique. Part I experiment. Cem. Concr. Res. 1991, 21, 73–82. [Google Scholar] [CrossRef]
- Chaix, J.F.; Lillamand, I.; Ploix, M.A.; Garnier, V.; Corneloup, G. Study of acoustoelasticity behavior of concrete material under uniaxial compression. J. Acoust. Soc. Am. 2008, 123, 3847. [Google Scholar] [CrossRef]
- Larose, E.; Hall, S. Monitoring stress related velocity variation in concrete with a 2 × 10-5 relative resolution using diffuse ultrasound. J. Acoust. Soc. Am. 2009, 125, 1853–1856. [Google Scholar] [CrossRef]
- Ghassemi Kakroudi, M.; Yeugo-Fogaing, E.; Huger, M.; Gault, C.; Chotard, T. Influence of the thermal history on the mechanical properties of two alumina based castables. J. Eur. Ceram. Soc. 2009, 29, 3197–3204. [Google Scholar] [CrossRef]
- Tonnesen, T.; Telle, R. Thermal shock damage in castables: Microstructural changes and evaluation by a damping method. CFI Ceram. Forum Int. 2007, 84, E131–E136. [Google Scholar]
- Skrzypkowski, K.; Korzeniowski, W.; Zagórski, K.; Dudek, P. Application of long expansion rock bolt support in the underground mines of legnica-głogów copper district. Studia Geotech. Mech. 2017, 39, 47–57. [Google Scholar] [CrossRef]



| Parameter | Piezo: PS-X-03-6/500 | Piezo: PS-X-03-6/1000 | Electromechanical | |
|---|---|---|---|---|
| 1 | Weight | 40 [g] | 35 [g] | 10 [g] |
| 2 | Flat frequency range | 50 [kHz] | 100 [kHz] | 115 [kHz] |
| 3 | Capacity | <250 [nF] | <30 [nF] | - |
| 4 | Stroke | 2.4 [µm] | 1,2 [µm] | 2.5 [mm] |
| 5 | Preload on piezo | 400 [N] | 500 [N] | - |
| 6 | Blocking force | 5 [kN] | 5 [kN] | 1 [kN] |
| 7 | Piezoelectric modulus (d33) | 1.22 × 10 [m/V] | 1.22 × 10 [m/V] | - |
| Sample | Volumetric Density [g/cm] | RFDA | Resonance | SAS | ||||
|---|---|---|---|---|---|---|---|---|
| Frequency | Acoustic | Piezo- | ||||||
| E | E | Emitter | Emitter | |||||
| [GPa] | [Hz] | () | [GPa] | [Hz] | [Hz] | [Hz] | ||
| Non-sintered | 2.47 | 34.8 | 3151 | 4.23 | 35.9 | 7150 | 1543 | 3404 |
| Sintered | 2.49 | 45.5 | 3505 | 5.08 | 46.5 | 8050 | 6945 | 14,350 |
| Acoustic Emitter | Piezo-Emitter | ||
|---|---|---|---|
| 100–1500 [kg] | 100–800 [kg] | 100–800 [kg] | |
| Non-sintered | |||
| Sintered | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kieliba, I.; Dominik, I.; Lalik, K.; Tonnesen, T.; Szczerba, J.; Telle, R. Self-Excited Acoustical System Frequency Monitoring for Refractory Concrete under Uniaxial Compression. Energies 2021, 14, 2222. https://doi.org/10.3390/en14082222
Kieliba I, Dominik I, Lalik K, Tonnesen T, Szczerba J, Telle R. Self-Excited Acoustical System Frequency Monitoring for Refractory Concrete under Uniaxial Compression. Energies. 2021; 14(8):2222. https://doi.org/10.3390/en14082222
Chicago/Turabian StyleKieliba, Ilona, Ireneusz Dominik, Krzysztof Lalik, Thorsten Tonnesen, Jacek Szczerba, and Reiner Telle. 2021. "Self-Excited Acoustical System Frequency Monitoring for Refractory Concrete under Uniaxial Compression" Energies 14, no. 8: 2222. https://doi.org/10.3390/en14082222
APA StyleKieliba, I., Dominik, I., Lalik, K., Tonnesen, T., Szczerba, J., & Telle, R. (2021). Self-Excited Acoustical System Frequency Monitoring for Refractory Concrete under Uniaxial Compression. Energies, 14(8), 2222. https://doi.org/10.3390/en14082222








