Development of an Elevation–Fresnel Linked Mini-Heliostat Array
Abstract
1. Introduction
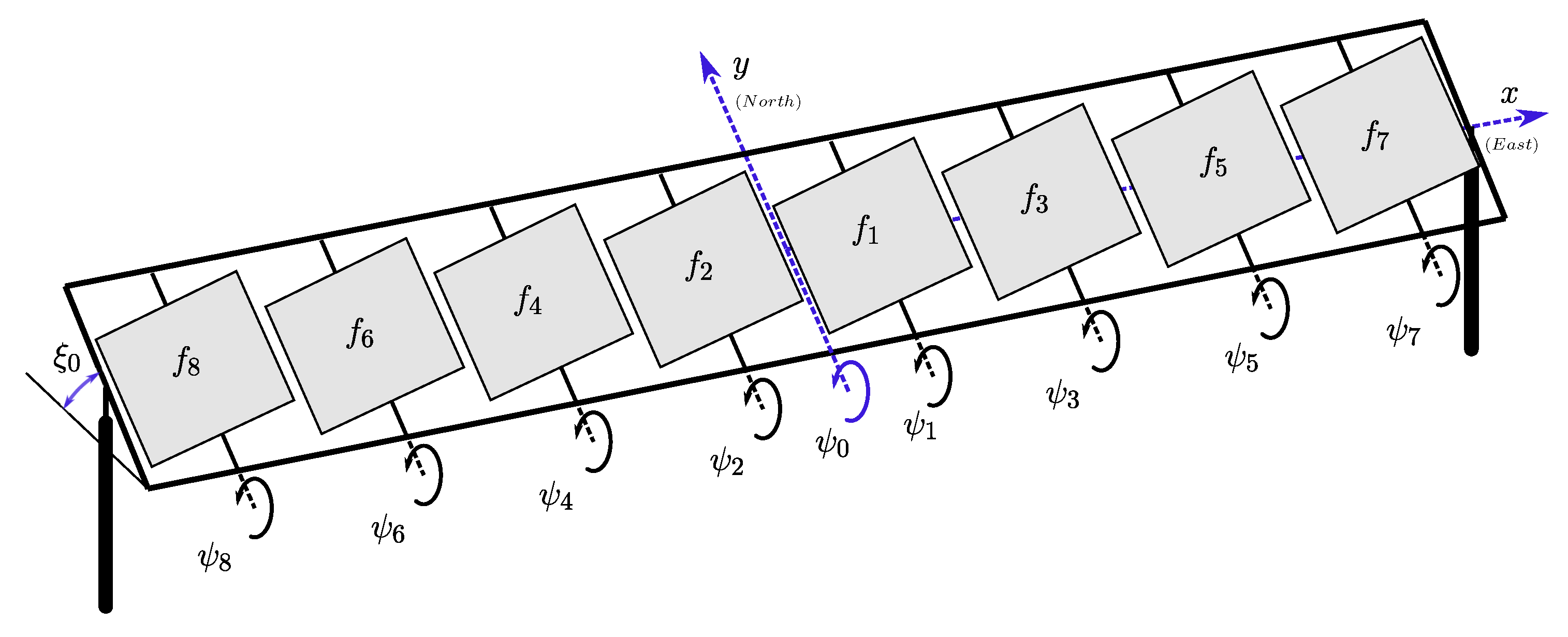
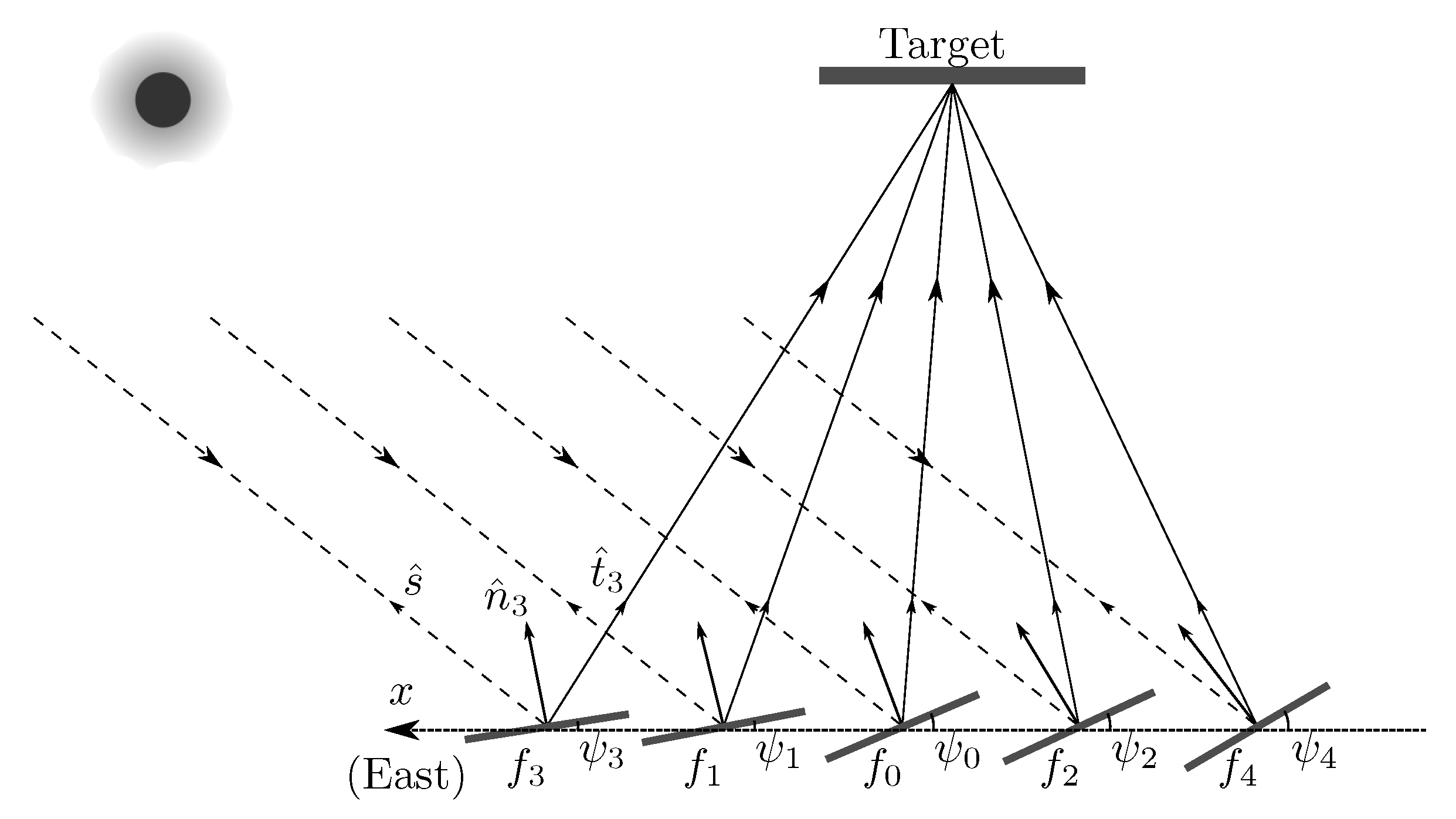
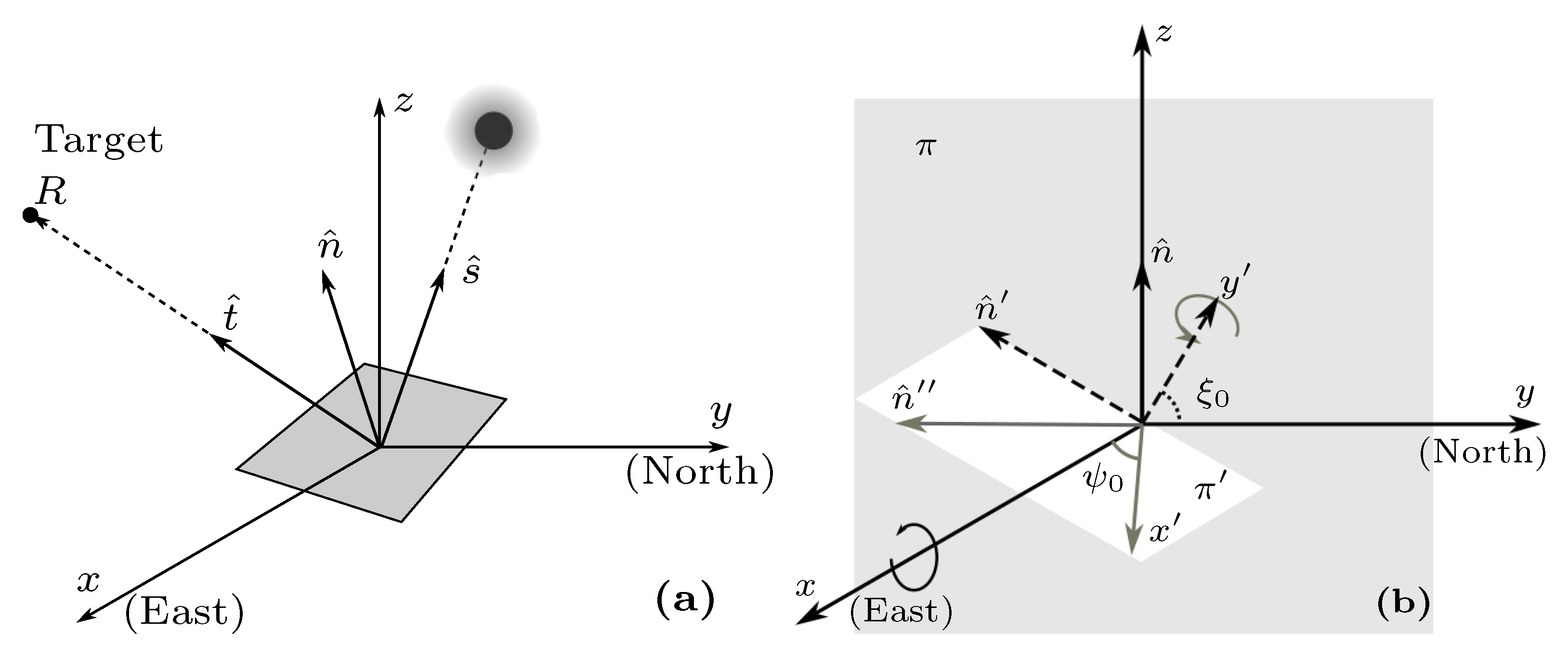
2. Methodology
2.1. Theoretical Model
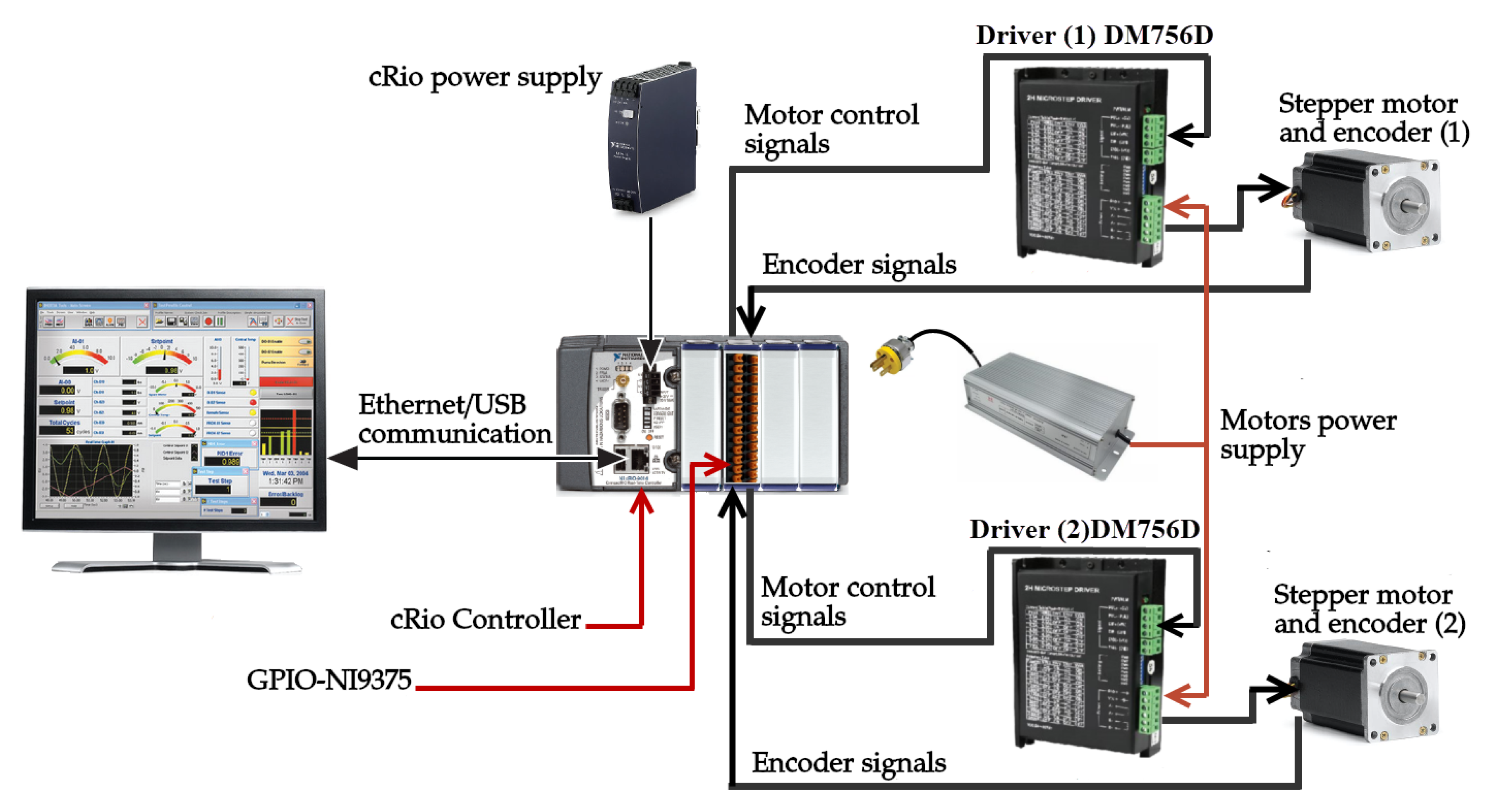
2.2. Experimental Methods
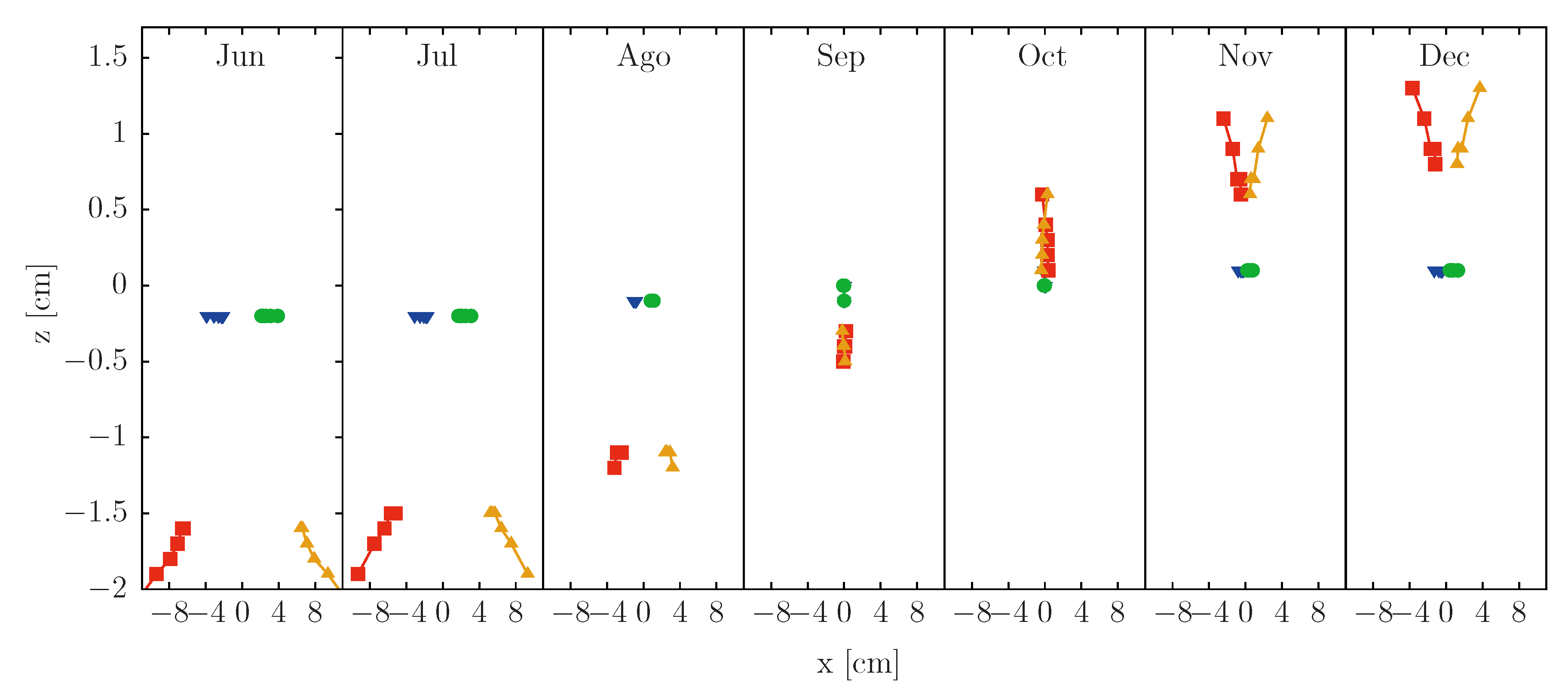
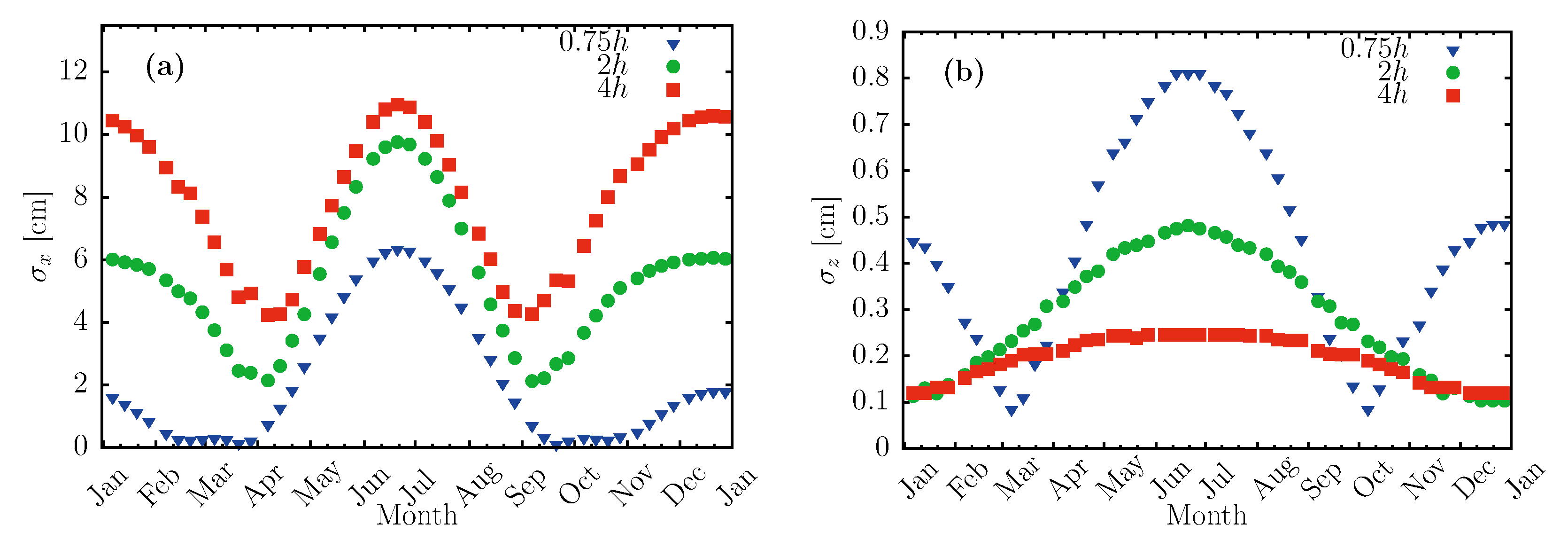
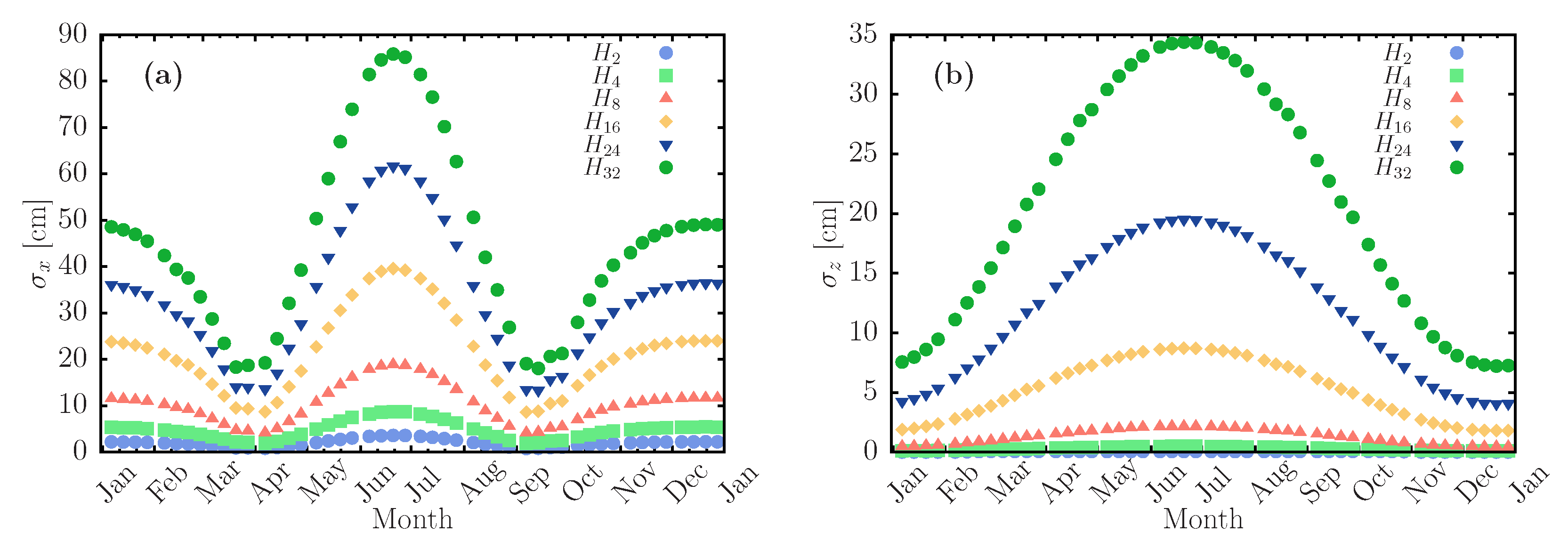
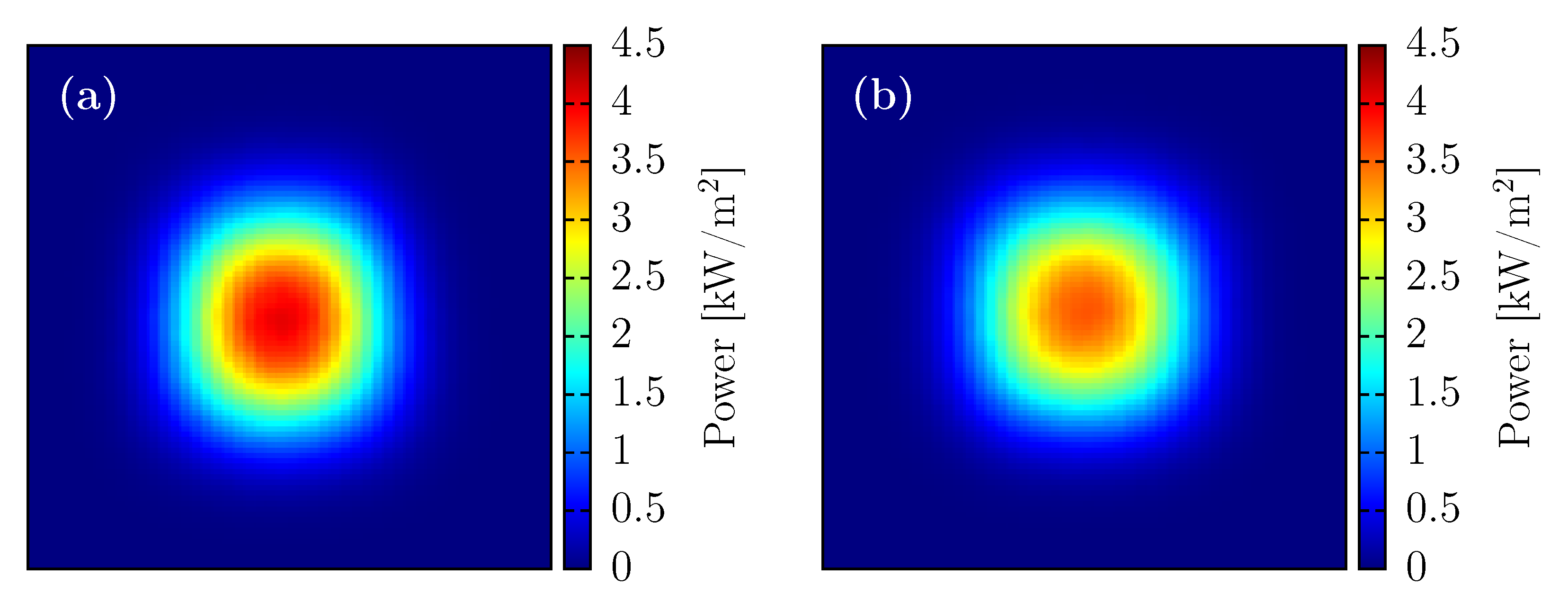
3. Results
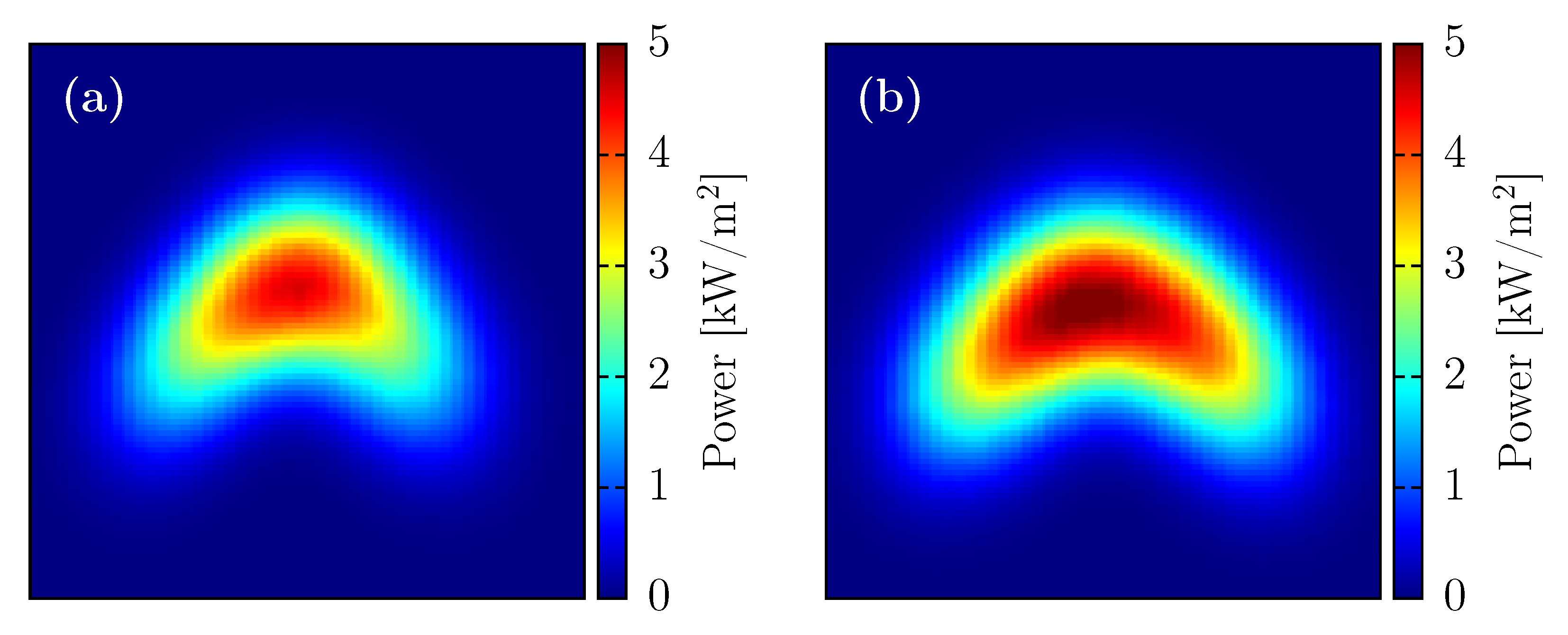
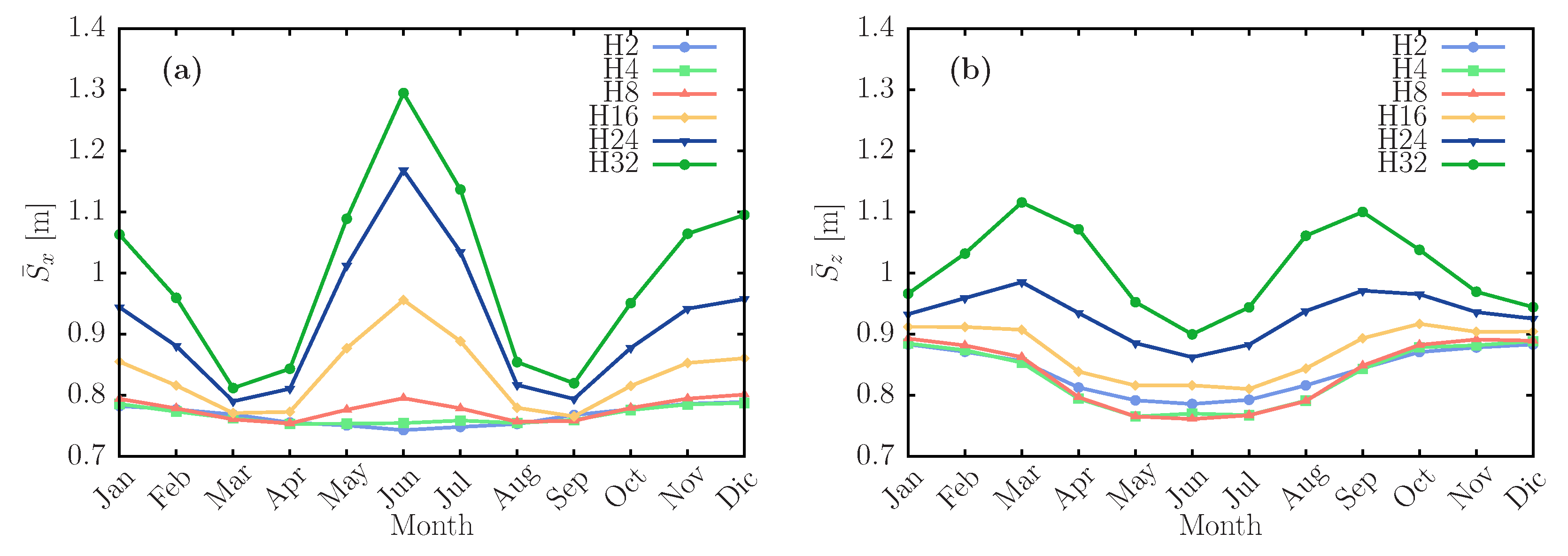
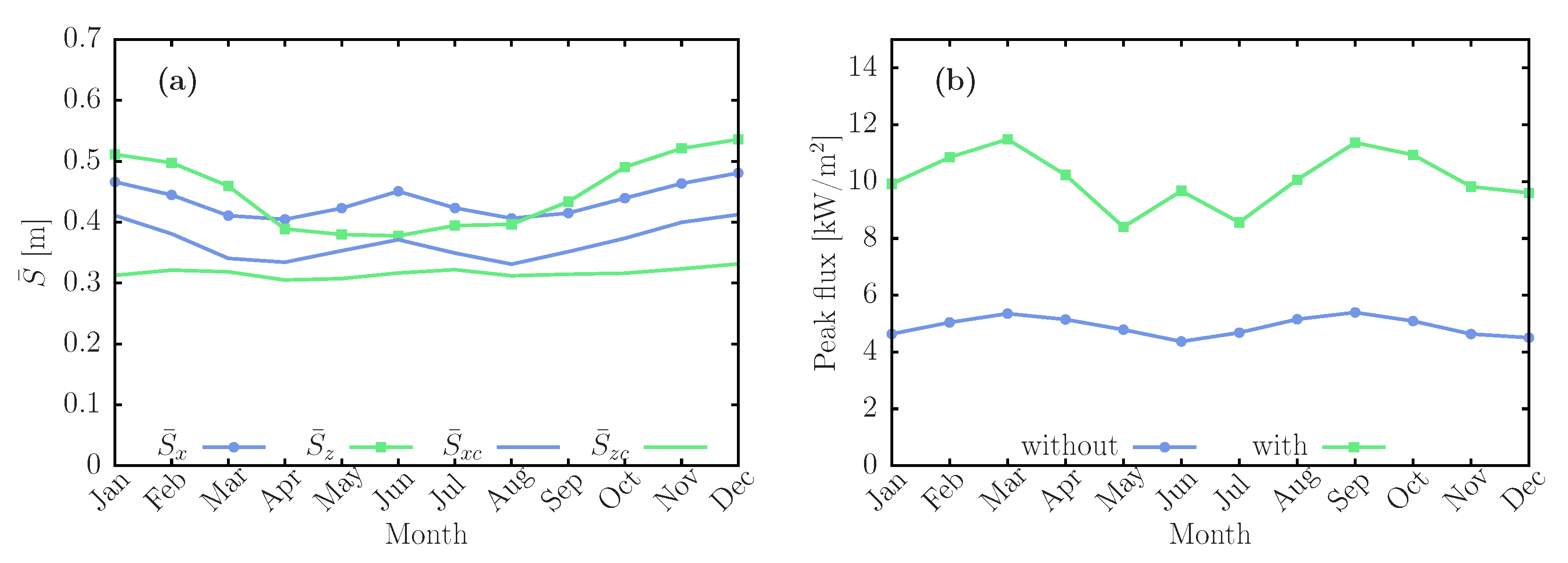
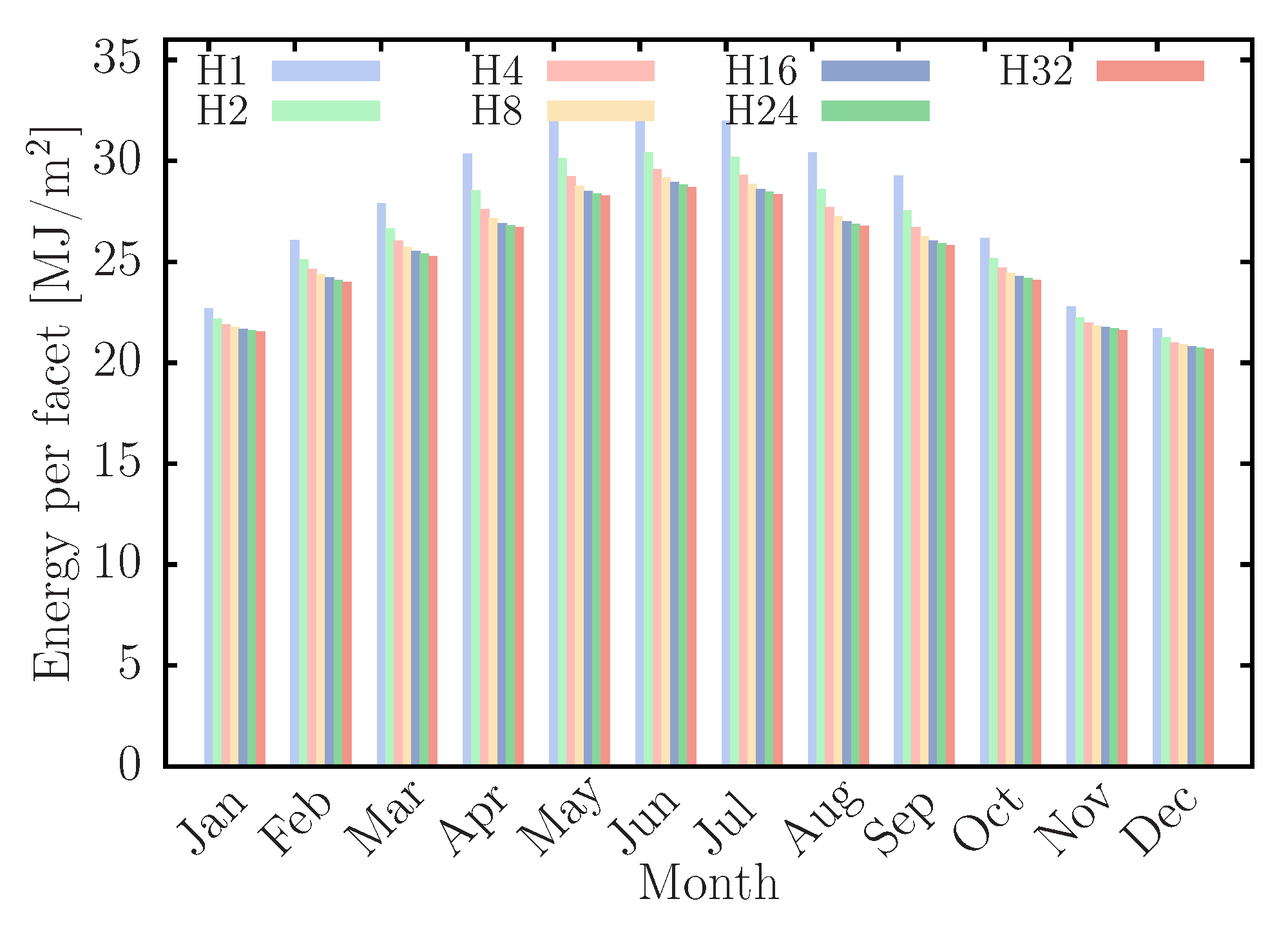
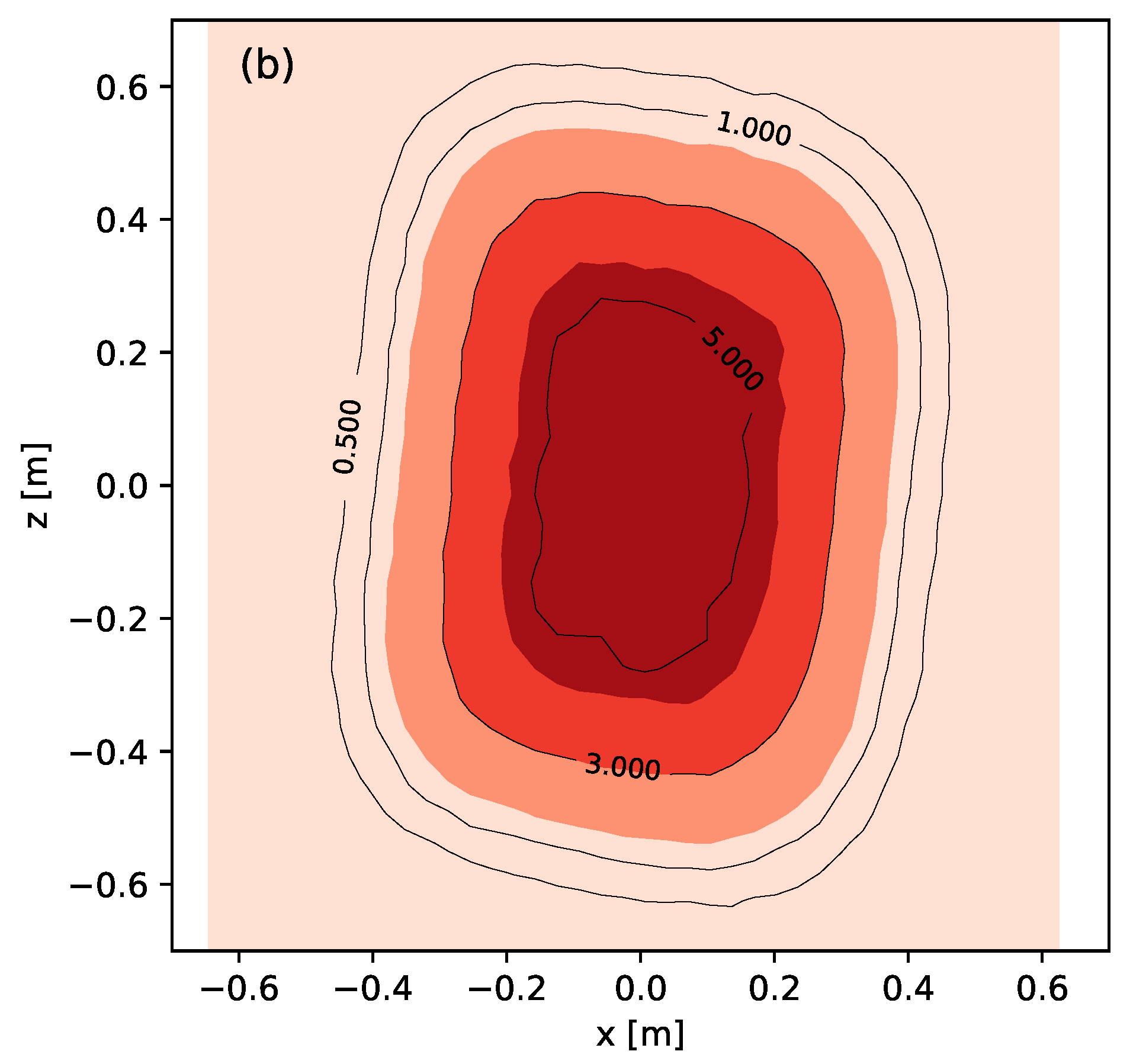
3.1. Theoretical Analysis
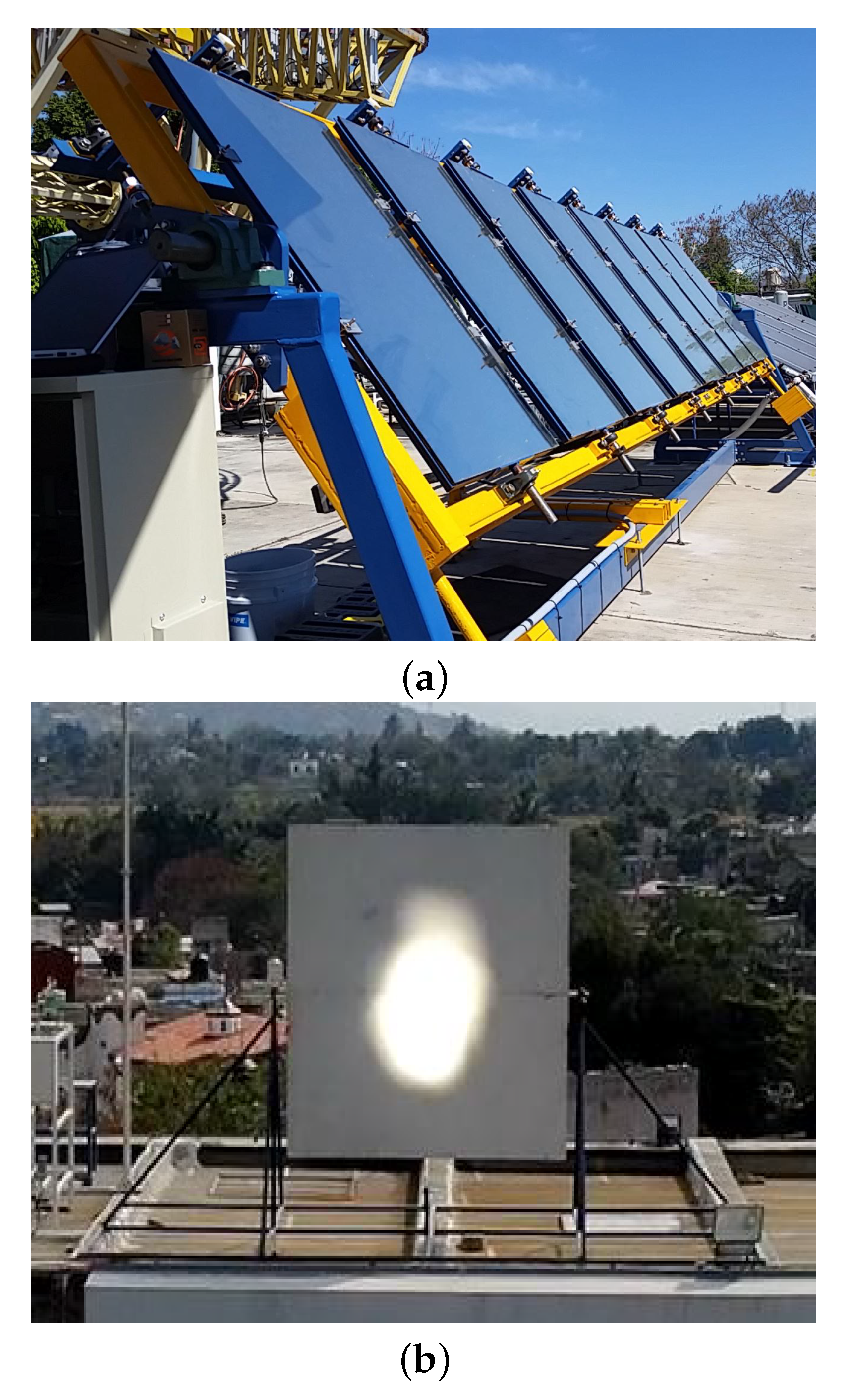
3.2. Prototype
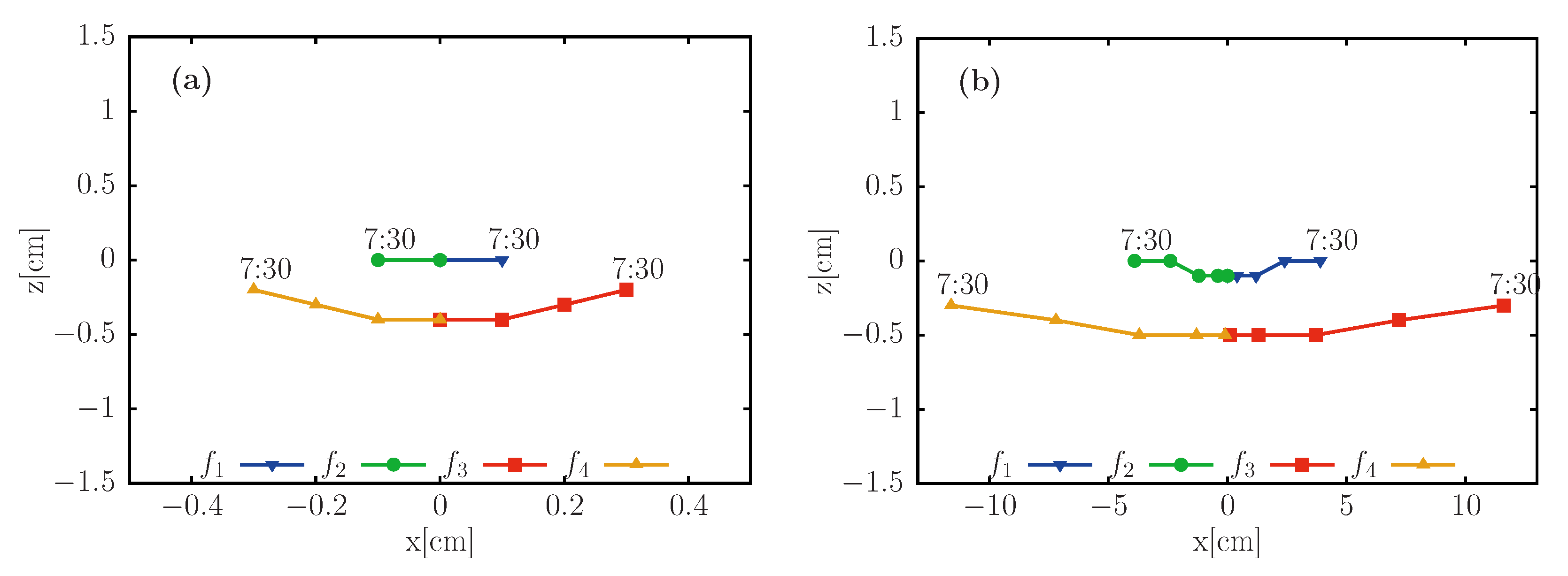
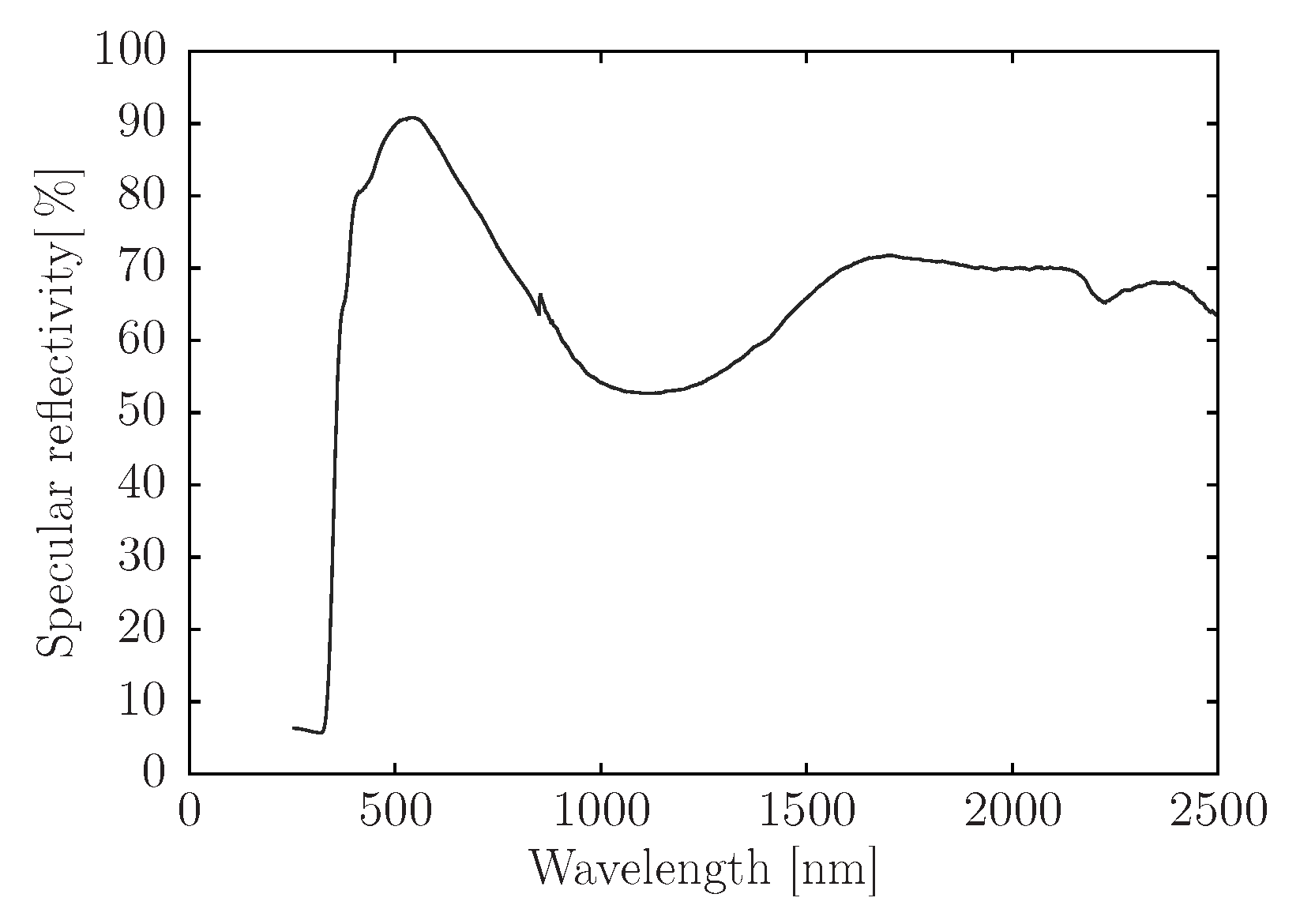
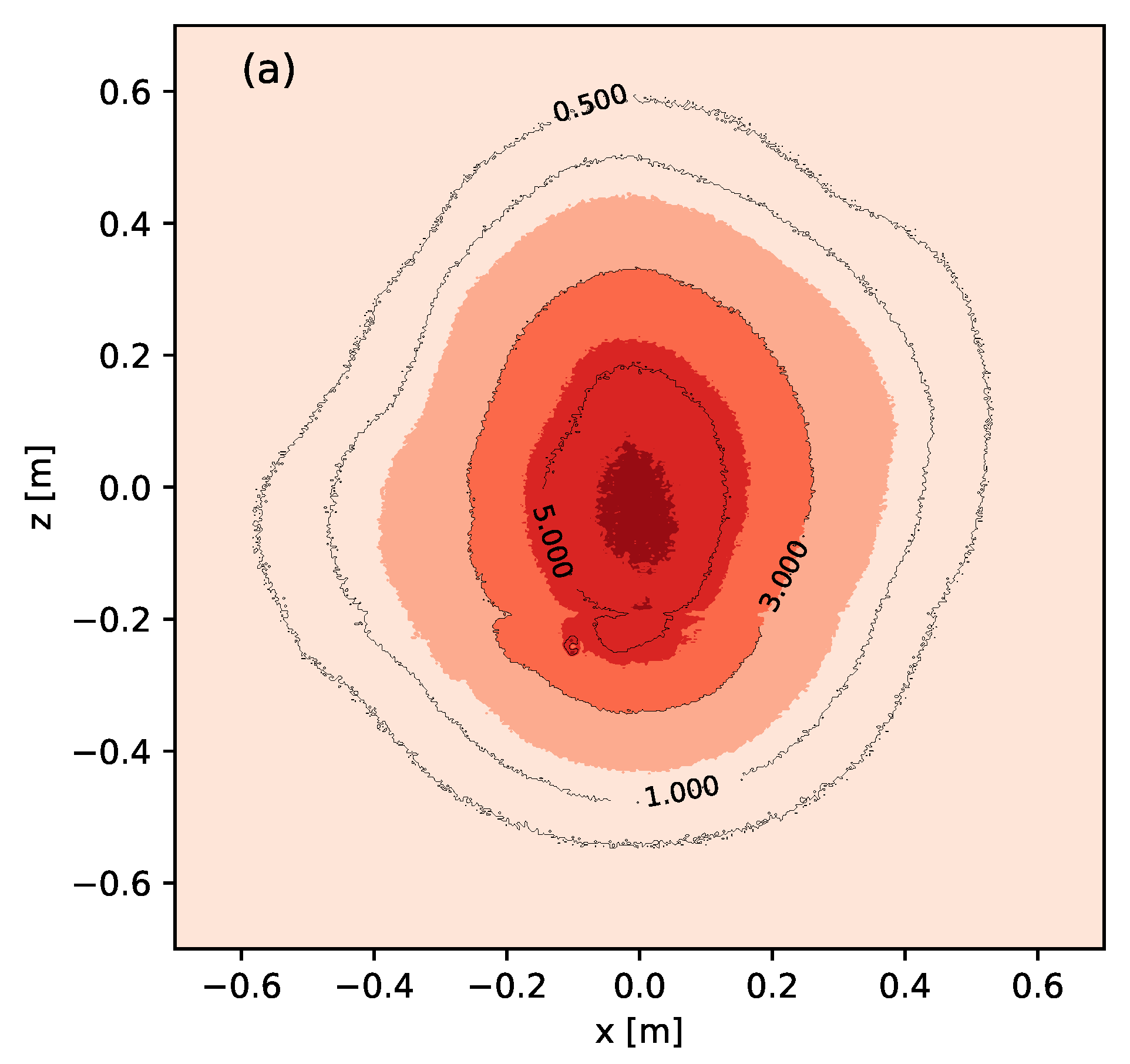
3.3. Experimental Results and Comparison
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Vant-Hull, L. Central tower concentrating solar power (CSP) systems. In Concentrating Solar Power Technology; Elsevier: Amsterdam, The Netherlands, 2012; pp. 240–283. [Google Scholar] [CrossRef]
- Ho, C.K. Advances in central receivers for concentrating solar applications. Sol. Energy 2017, 152, 38–56. [Google Scholar] [CrossRef]
- Behar, O.; Khellaf, A.; Mohammedi, K. A review of studies on central receiver solar thermal power plants. Renew. Sustain. Energy Rev. 2013, 23, 12–39. [Google Scholar] [CrossRef]
- Li, L.; Coventry, J.; Bader, R.; Pye, J.; Lipiński, W. Optics of solar central receiver systems: A review. Opt. Express 2016, 24, A985–A1007. [Google Scholar] [CrossRef] [PubMed]
- Ortega, J.I.; Burgaleta, J.I.; Téllez, F.M. Central receiver system solar power plant using molten salt as heat transfer fluid. J. Sol. Energy Eng. 2008, 130, 024501. [Google Scholar] [CrossRef]
- Göttsche, J.; Hoffschmidt, B.; Schmitz, S.; Sauerborn, M.; Buck, R.; Teufel, E.; Badstübner, K.; Ifland, D.; Rebholz, C. Solar concentrating systems using small mirror arrays. J. Sol. Energy Eng. 2010, 132, 011003. [Google Scholar] [CrossRef]
- Pfahl, A. Survey of Heliostat Concepts for Cost Reduction. J. Sol. Energy Eng. 2013, 136, 014501. [Google Scholar] [CrossRef]
- Coventry, J.; Pye, J. Heliostat Cost Reduction—Where to Now? Energy Procedia 2014, 49, 60–70. [Google Scholar] [CrossRef][Green Version]
- Pfahl, A.; Coventry, J.; Röger, M.; Wolfertstetter, F.; Vásquez-Arango, J.F.; Gross, F.; Arjomandi, M.; Schwarzbözl, P.; Geiger, M.; Liedke, P. Progress in heliostat development. Sol. Energy 2017, 152, 3–37. [Google Scholar] [CrossRef]
- Schell, S. Design and evaluation of esolar’s heliostat fields. Sol. Energy 2011, 85, 614–619. [Google Scholar] [CrossRef]
- Kolb, G.J.; Davenport, R.; Gorman, D.; Lumia, R.; Thomas, R.; Donnelly, M. Heliostat cost reduction. In Proceedings of the ASME 2007 Energy Sustainability Conference, Long Beach, CA, USA, 27–30 July 2007; pp. 1077–1084. [Google Scholar] [CrossRef][Green Version]
- Schramek, P.; Mills, D.R.; Stein, W.; Le Lièvre, P. Design of the Heliostat Field of the CSIRO Solar Tower. J. Sol. Energy Eng. 2009, 131, 024505. [Google Scholar] [CrossRef]
- Domínguez-Bravo, C.A.; Bode, S.J.; Heiming, G.; Richter, P.; Carrizosa, E.; Fernández-Cara, E.; Frank, M.; Gauché, P. Field-design optimization with triangular heliostat pods. AIP Conf. Proc. 2016, 1734, 070006. [Google Scholar] [CrossRef]
- Dunham, M.; Kasetty, R.; Mathur, A.; Lipiński, W. Optical analysis of a heliostat array with linked tracking. J. Sol. Energy Eng. 2013, 135, 034501. [Google Scholar] [CrossRef]
- Amsbeck, L.; Buck, R.; Pfahl, A.; Uhlig, R. Optical performance and weight estimation of a heliostat with ganged facets. J. Sol. Energy Eng. 2007, 130, 011010. [Google Scholar] [CrossRef]
- Yellowhair, J.; Andraka, C.; Armijo, K.; Ortega, J.; Clair, J. Optical performance modeling and analysis of a tensile ganged heliostat concept. In Proceedings of the ASME 2019 13th International Conference on Energy Sustainability, ES 2019, Collocated with the ASME 2019 Heat Transfer Summer Conference, Washington, DC, USA, 14–17 July 2019. [Google Scholar] [CrossRef]
- Mills, D.R. Linear Fresnel reflector (LFR) technology. In Concentrating Solar Power Technology; Lovegrove, K., Stein, W., Eds.; Woodhead Publishing Series in Energy; Woodhead Publishing: Cambridge, UK, 2012; pp. 153–196. [Google Scholar] [CrossRef]
- Buck, R.; Teufel, E. Comparison and Optimization of Heliostat Canting Methods. J. Sol. Energy Eng. 2009, 131, 011001. [Google Scholar] [CrossRef]
- Grena, R. An algorithm for the computation of the solar position. Sol. Energy 2008, 82, 462–470. [Google Scholar] [CrossRef]
- Dopos, A. LK Scripting Language Reference; NREL: Golden, CO, USA, 2017. [Google Scholar]
- Wendelin, T.; Dobos, A.; Lewandowski, A. SolTrace: A Ray-Tracing Code for Complex Solar Optical Systems. Contract 2013, 303, 275–3000. [Google Scholar]
- Martínez-Manuel, L.; Peña-Cruz, M.; Villa-Medina, M.; Ojeda-Bernal, C.; Prado-Zermeño, M.; Prado-Zermeño, I.; Pineda-Arellano, C.; Carrillo, J.; Salgado-Tránsito, I.; Martell-Chavez, F. A 17.5 kWel high flux solar simulator with controllable flux-spot capabilities: Design and validation study. Sol. Energy 2018, 170, 807–819. [Google Scholar] [CrossRef]
- Moreno-Cruz, I. Análisis de un Sistema de Seguimiento Solar Para Arreglos de Helióstatos Acoplados. Ph.D. Thesis, Instituto de Energías Renovables, UNAM, Temixco, Mexico, 2019. [Google Scholar]
- Chong, K. Optical analysis for simplified astigmatic correction of non-imaging focusing heliostat. Sol. Energy 2010, 84, 1356–1365. [Google Scholar] [CrossRef]
- Renzi, M.; Bartolini, C.M.; Santolini, M.; Arteconi, A. Efficiency assessment for a small heliostat solar concentration plant. Int. J. Energy Res. 2015, 39, 265–278. [Google Scholar] [CrossRef]
- Díaz-Félix, L.; Escobar-Toledo, M.; Waissman, J.; Pitalúa-Díaz, N.; Arancibia-Bulnes, C. Evaluation of Heliostat Field Global Tracking Error Distributions by Monte Carlo Simulations. Energy Procedia 2014, 49, 1308–1317. [Google Scholar] [CrossRef]
- Bonanos, A.; Faka, M.; Abate, D.; Hermon, S.; Blanco, M. Heliostat surface shape characterization for accurate flux prediction. Renew. Energy 2019, 142, 30–40. [Google Scholar] [CrossRef]
- Iriarte-Cornejo, C.; Arancibia-Bulnes, C.; Hinojosa, J.; Peña-Cruz, M.I. Effect of spatial resolution of heliostat surface characterization on its concentrated heat flux distribution. Sol. Energy 2018, 174, 312–320. [Google Scholar] [CrossRef]
- Sánchez-González, A.; Caliot, C.; Ferrière, A.; Santana, D. Determination of heliostat canting errors via deterministic optimization. Sol. Energy 2017, 150, 136–146. [Google Scholar] [CrossRef]


| Parameter | Value | Units |
|---|---|---|
| Number of facets | 8 | – |
| Facet length | 1.00 | m |
| Facet width | 0.60 | m |
| Mirror material | Back silvered glass | – |
| Mirror thickness | 6 | mm |
| Structure material | ASTM A36 Steel | – |
| Spacing between facets | 0.02 | m |
| Length of the elevation frame | 5.062 | m |
| Width of the elevation frame | 1.13 | m |
| Height of the array | 1.6 | m |
| Type of actuator | Linear jack /stepper motor | – |
| Elevation range | [5, 80] | deg |
| Facet rotation range | [−53.71, 53.71] | deg |
| Facet | ||||||||
|---|---|---|---|---|---|---|---|---|
| Canting angle (deg) | 1.884 | 1.346 | 0.808 | 0.269 | −0.269 | −0.808 | −1.346 | −1.884 |
| Slope error (mrad) | 1.36 | 0.75 | 1.41 | 1.68 | 0.87 | 1.26 | 1.32 | 0.77 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moreno-Cruz, I.; Castro, J.C.; Álvarez-Brito, O.; Mota-Nava, H.B.; Ramírez-Zúñiga, G.; Quiñones-Aguilar, J.J.; Arancibia-Bulnes, C.A. Development of an Elevation–Fresnel Linked Mini-Heliostat Array. Energies 2020, 13, 4012. https://doi.org/10.3390/en13154012
Moreno-Cruz I, Castro JC, Álvarez-Brito O, Mota-Nava HB, Ramírez-Zúñiga G, Quiñones-Aguilar JJ, Arancibia-Bulnes CA. Development of an Elevation–Fresnel Linked Mini-Heliostat Array. Energies. 2020; 13(15):4012. https://doi.org/10.3390/en13154012
Chicago/Turabian StyleMoreno-Cruz, Isaías, Juan Carlos Castro, Omar Álvarez-Brito, Hilda B. Mota-Nava, Guillermo Ramírez-Zúñiga, José J. Quiñones-Aguilar, and Camilo A. Arancibia-Bulnes. 2020. "Development of an Elevation–Fresnel Linked Mini-Heliostat Array" Energies 13, no. 15: 4012. https://doi.org/10.3390/en13154012
APA StyleMoreno-Cruz, I., Castro, J. C., Álvarez-Brito, O., Mota-Nava, H. B., Ramírez-Zúñiga, G., Quiñones-Aguilar, J. J., & Arancibia-Bulnes, C. A. (2020). Development of an Elevation–Fresnel Linked Mini-Heliostat Array. Energies, 13(15), 4012. https://doi.org/10.3390/en13154012





