Fault Ride-Through Enhancement of Grid Supporting Inverter-Based Microgrid Using Delayed Signal Cancellation Algorithm Secondary Control
Abstract
1. Introduction
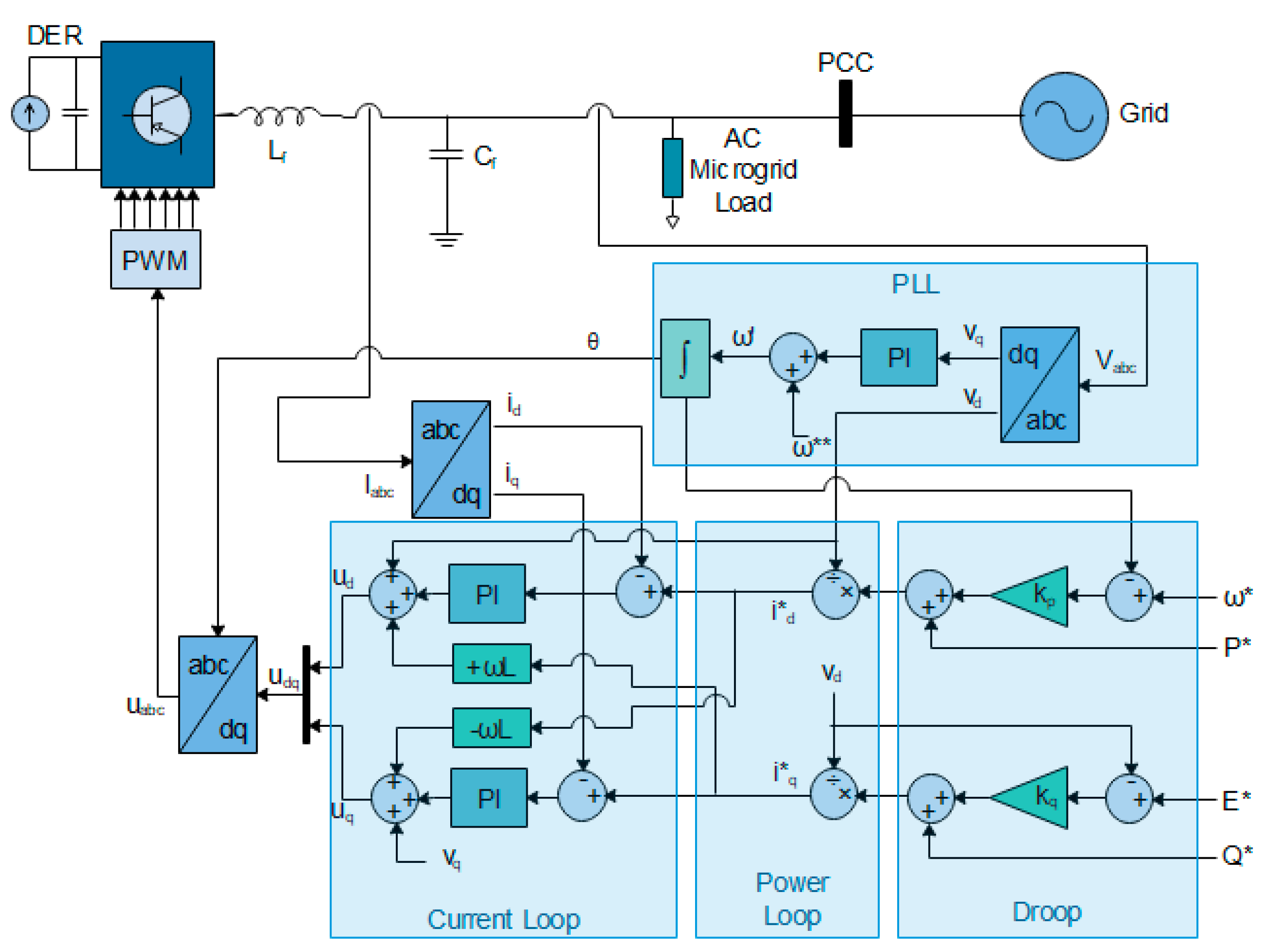
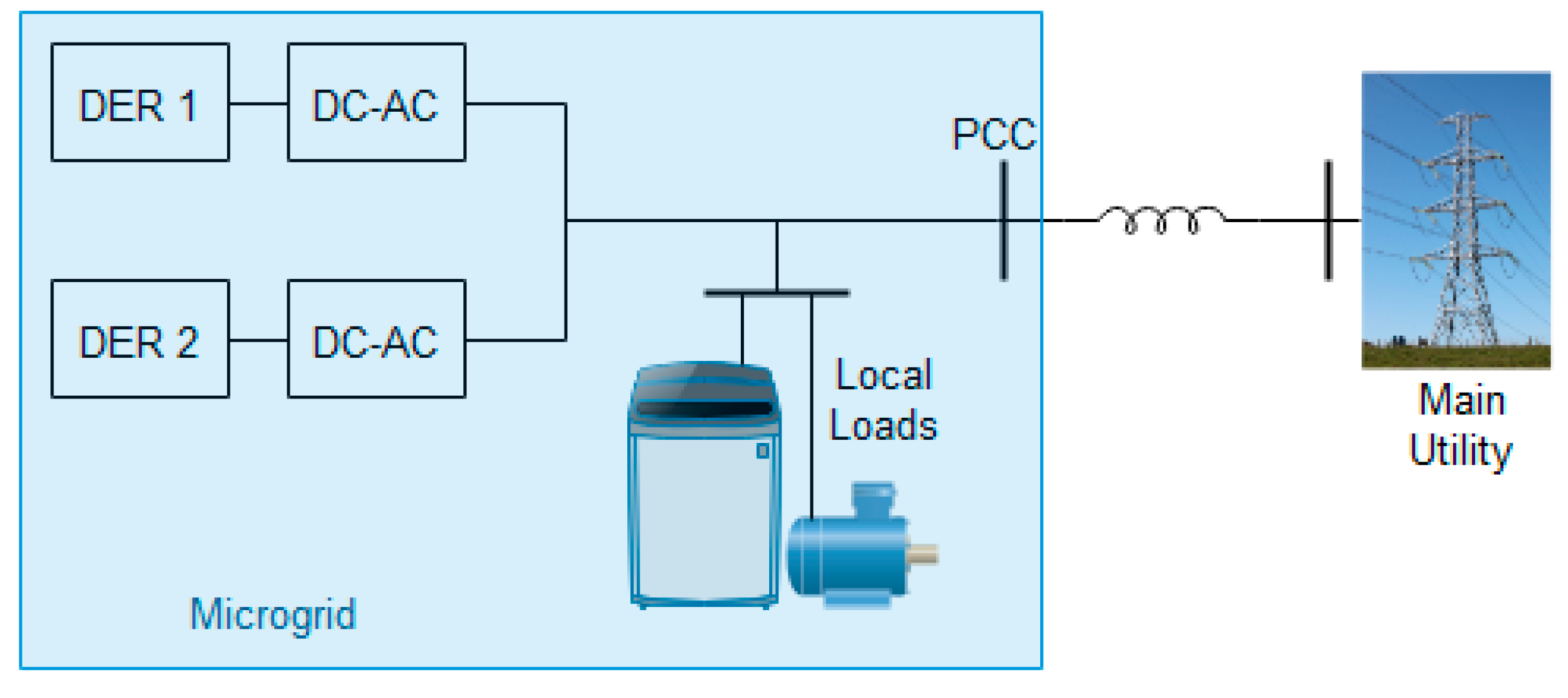
2. Grid Supporting Microgrid Modeling
2.1. Output LC Filter
2.2. Grid Synchronization
2.3. Primary Control
2.3.1. Droop-Based Power Control
2.3.2. Power/Voltage Loop Control
2.3.3. Current Loop Control
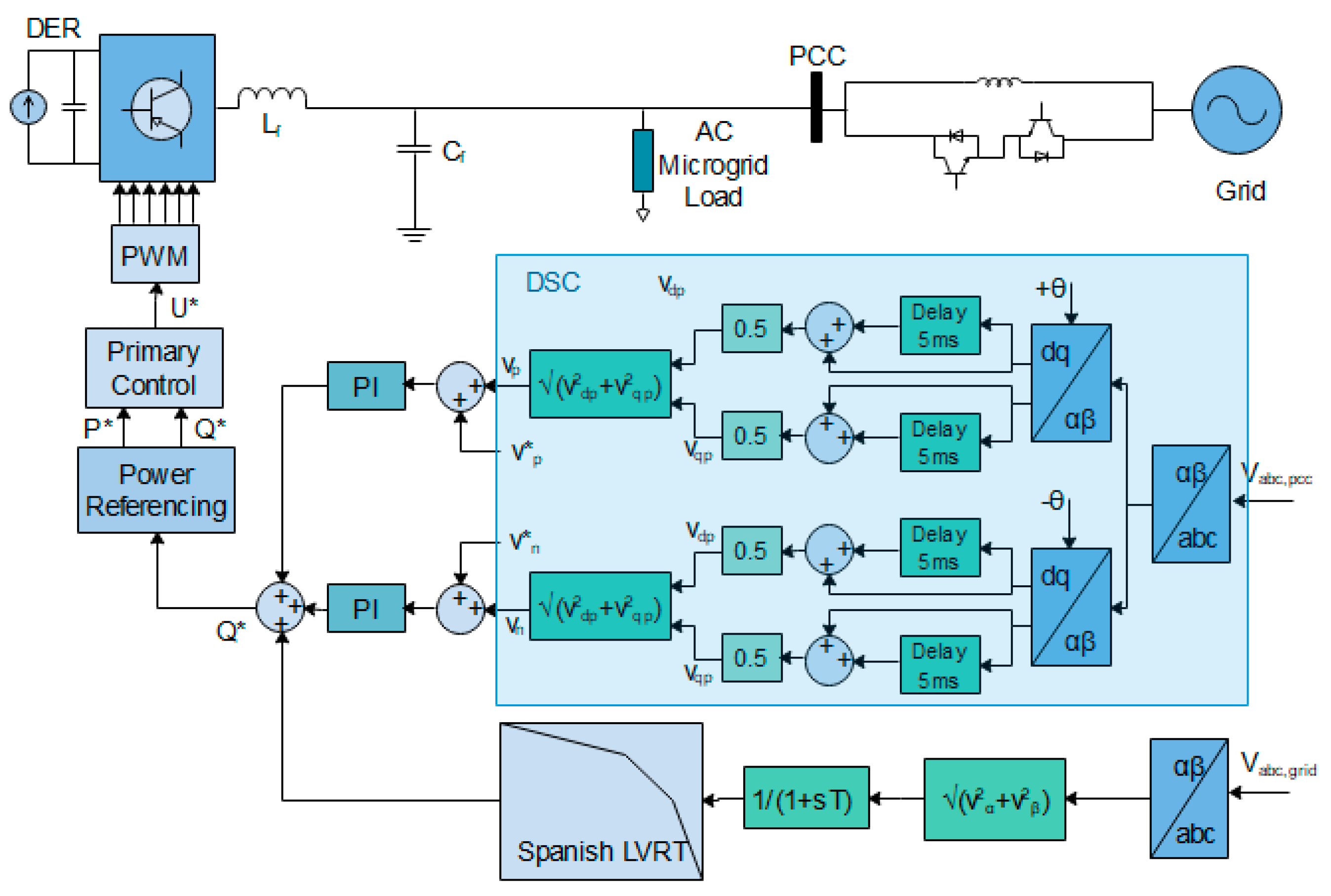
3. Proposed LVRT/FRT Scheme
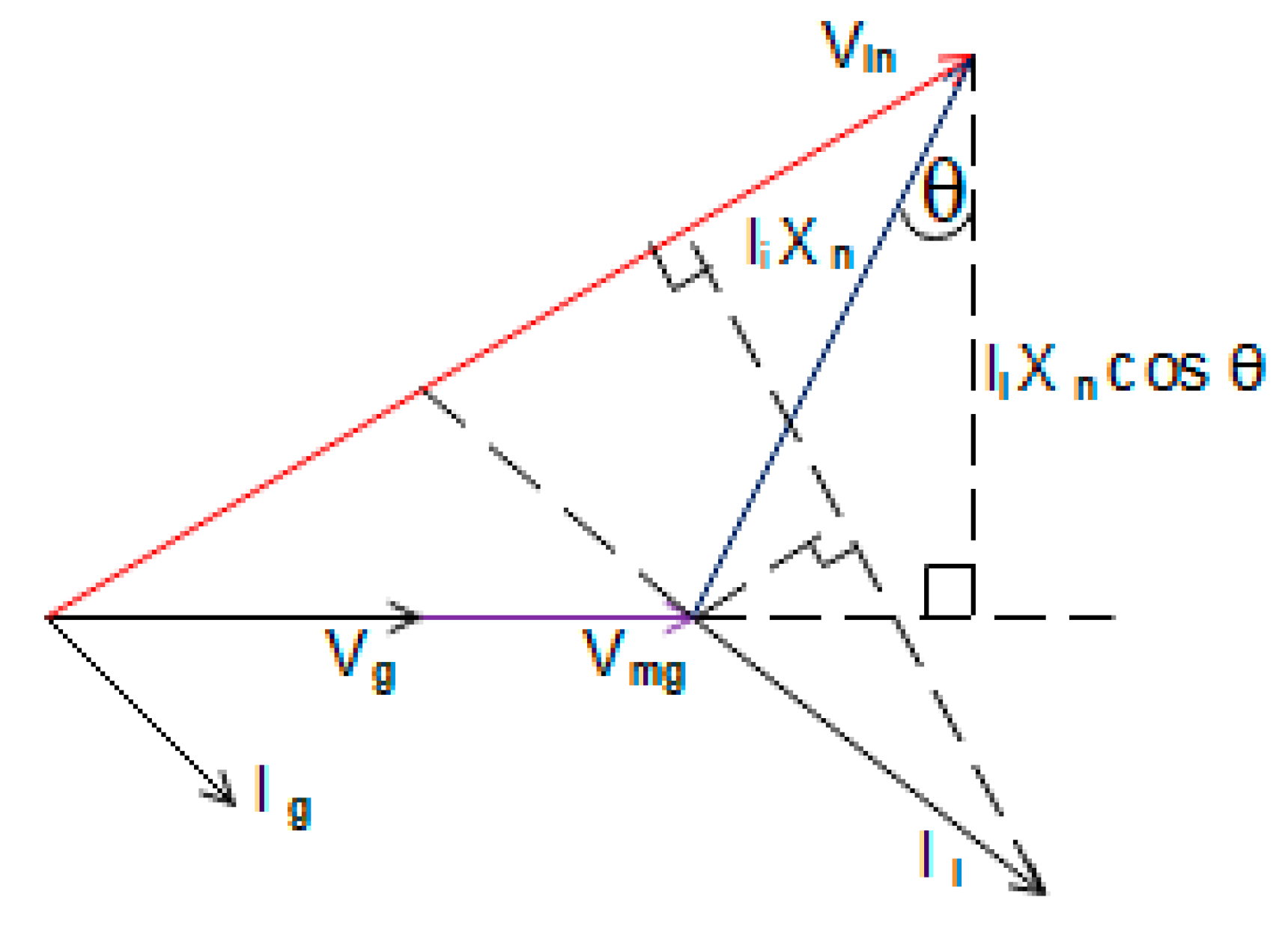
3.1. Secondary Voltage Control
3.2. DSC Algorithm for Unbalance Detection and PCC Voltage
4. Power Flow and Switched Reactor
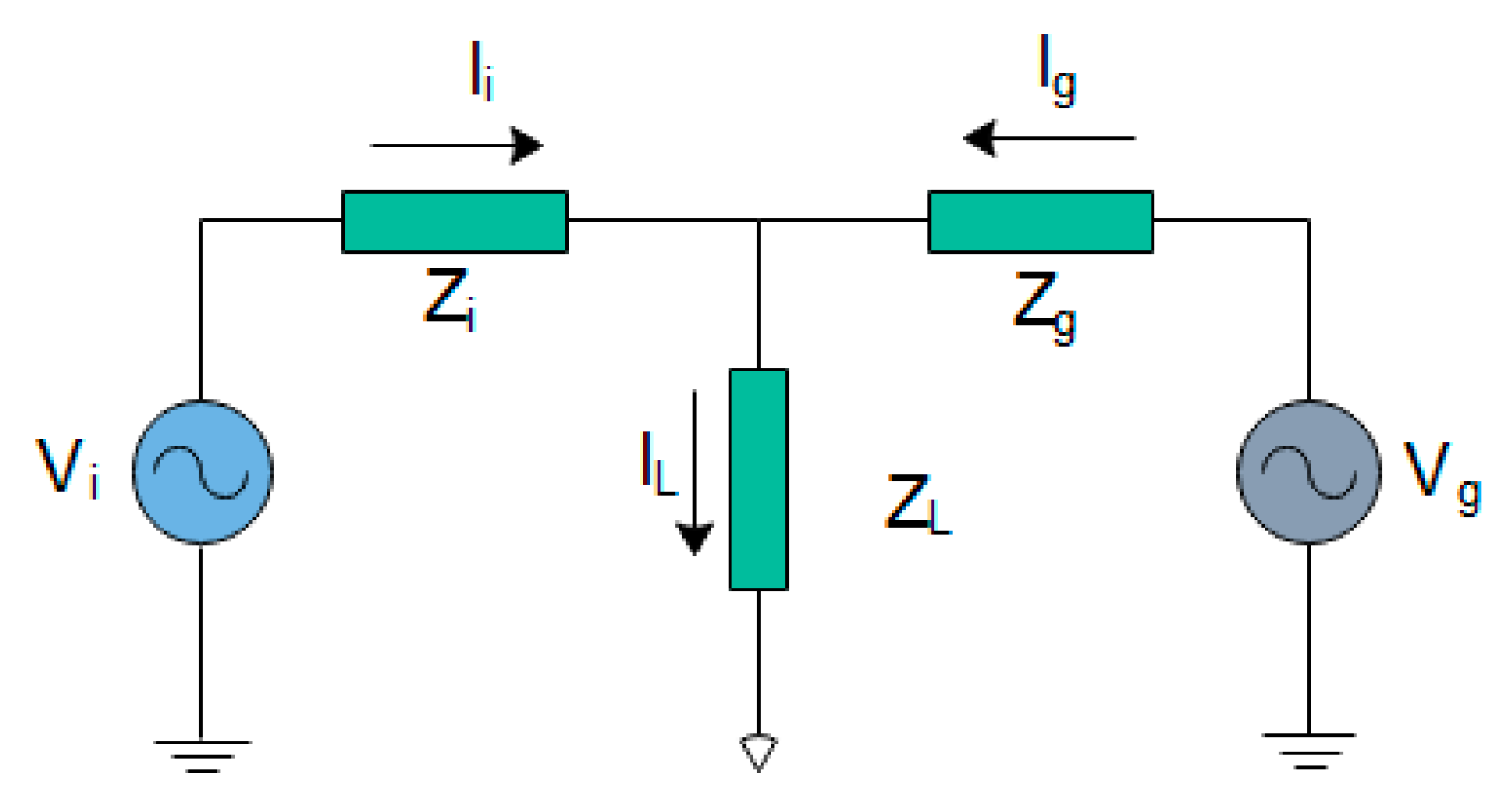
4.1. Voltage Source Inverter and Grid Interactive Power Flow
4.2. Switched Inductance: Sizing and Switching
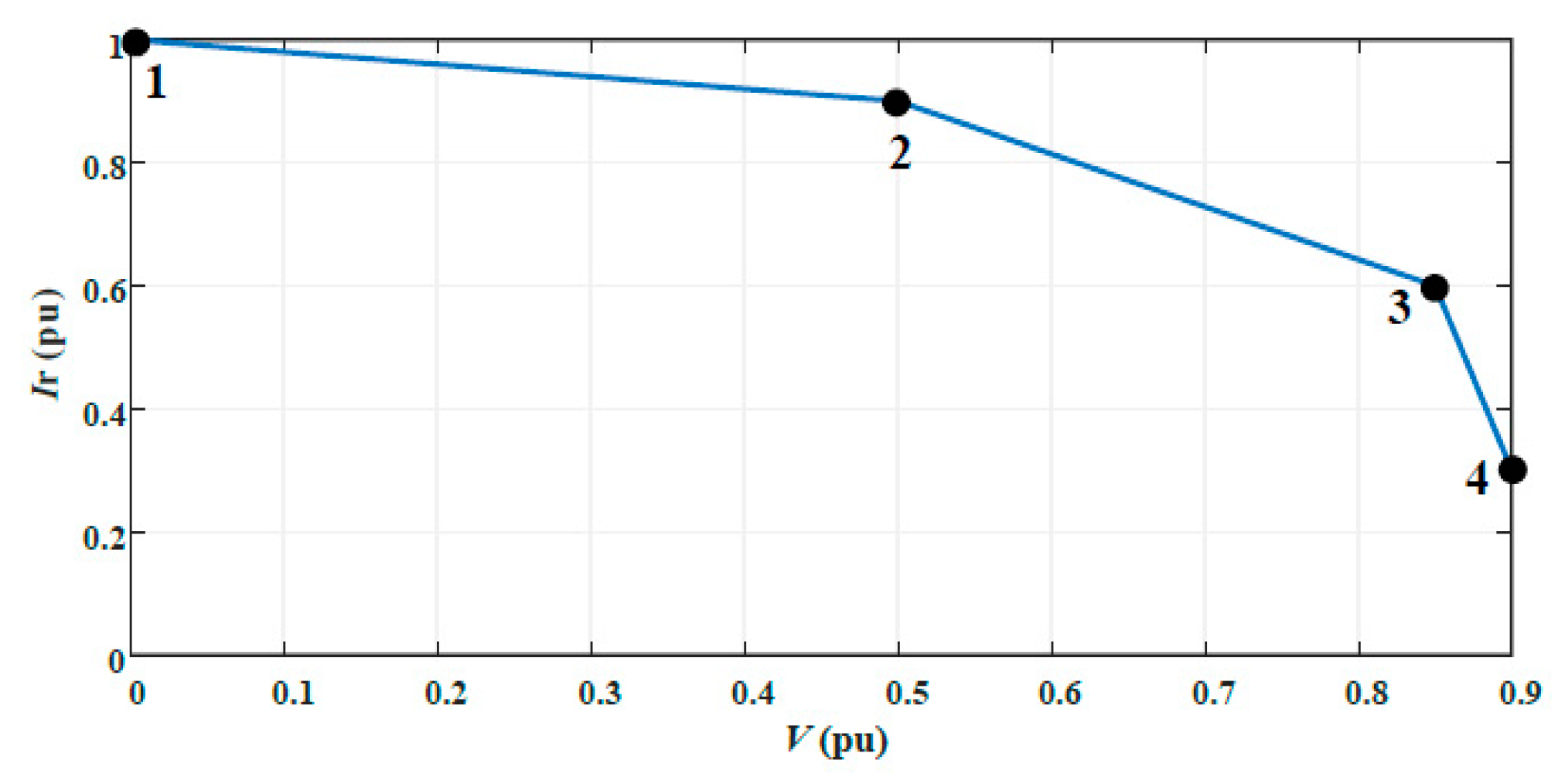
4.3. Active Power Referencing and Fault Current Limiting
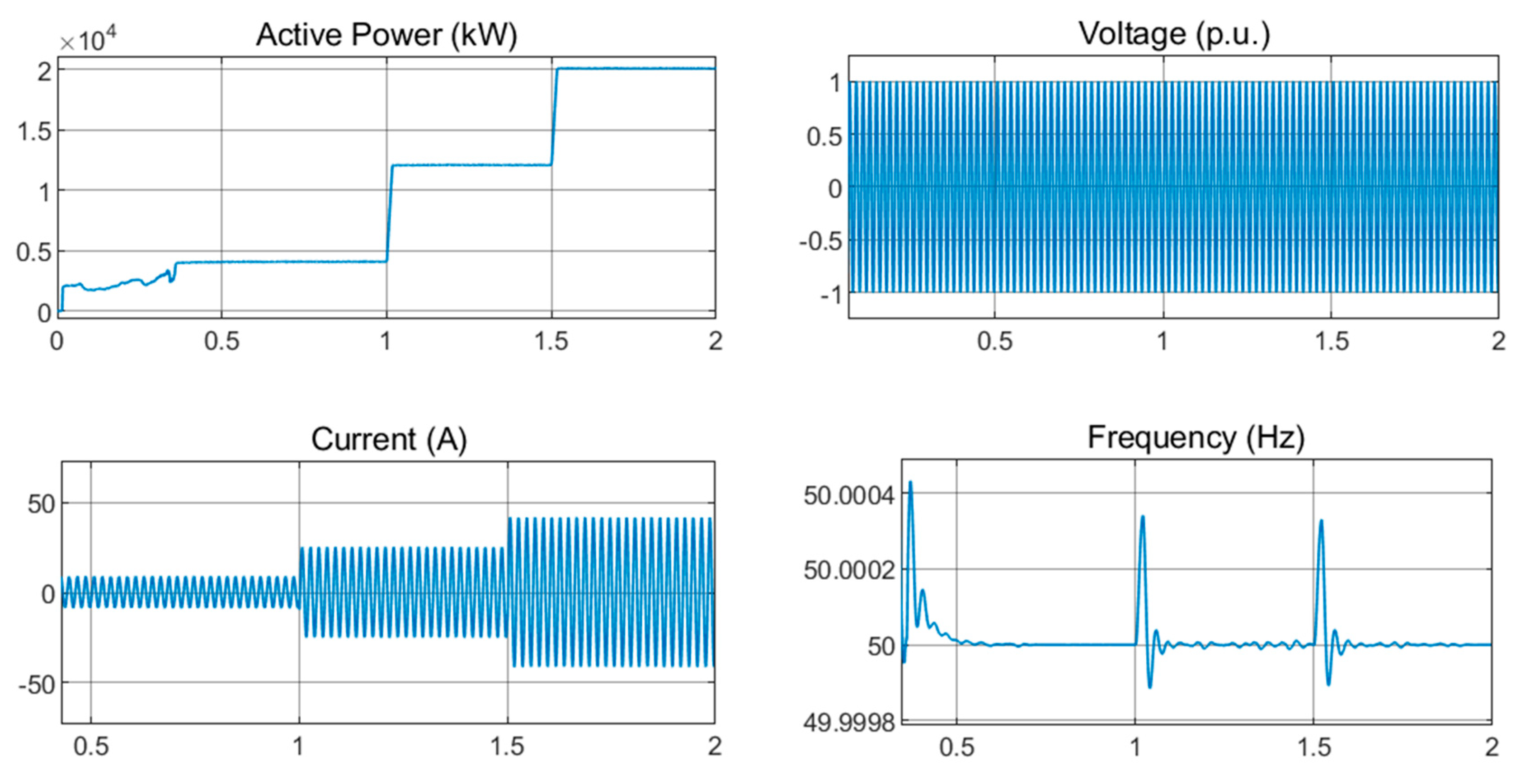
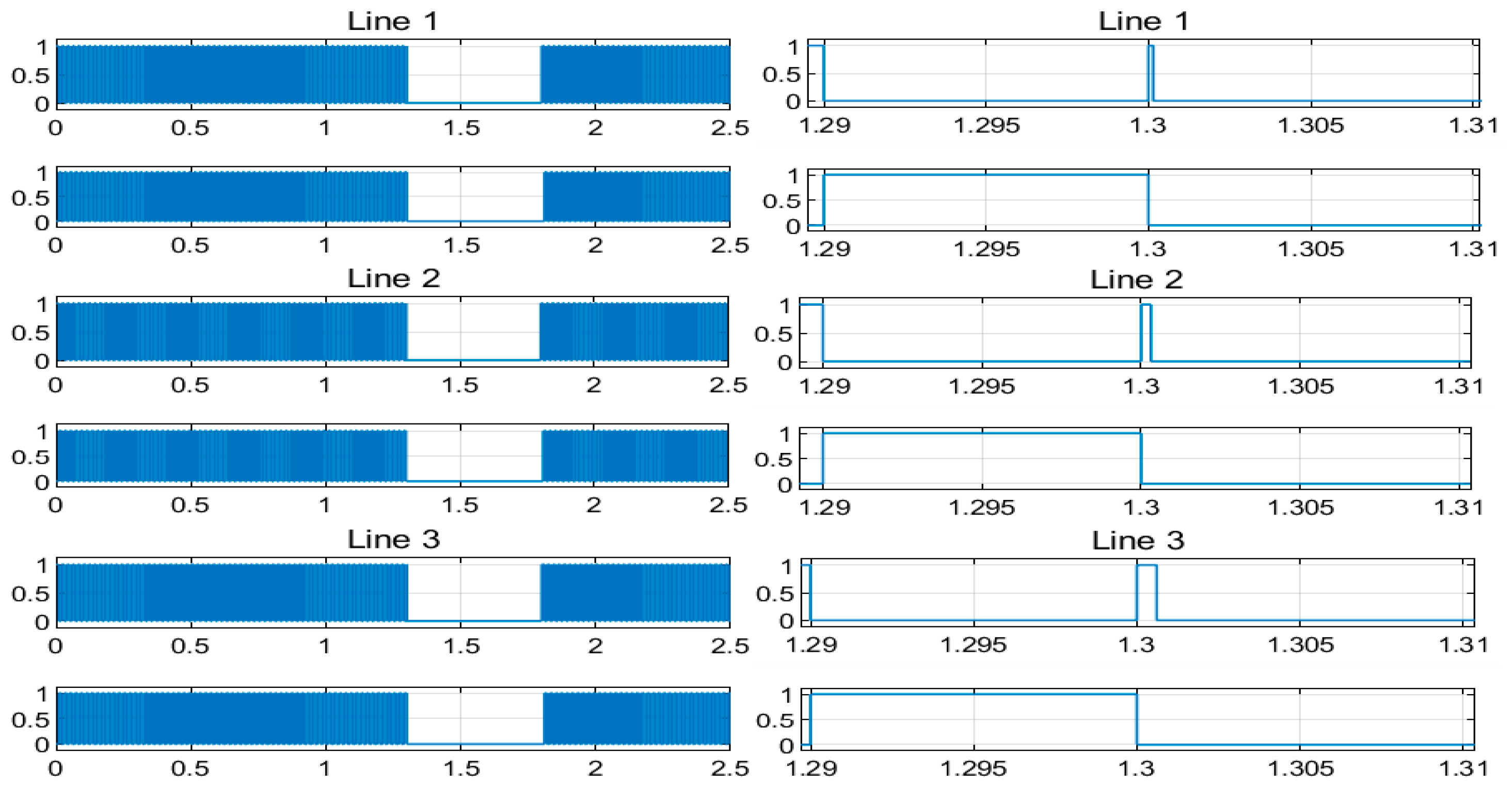
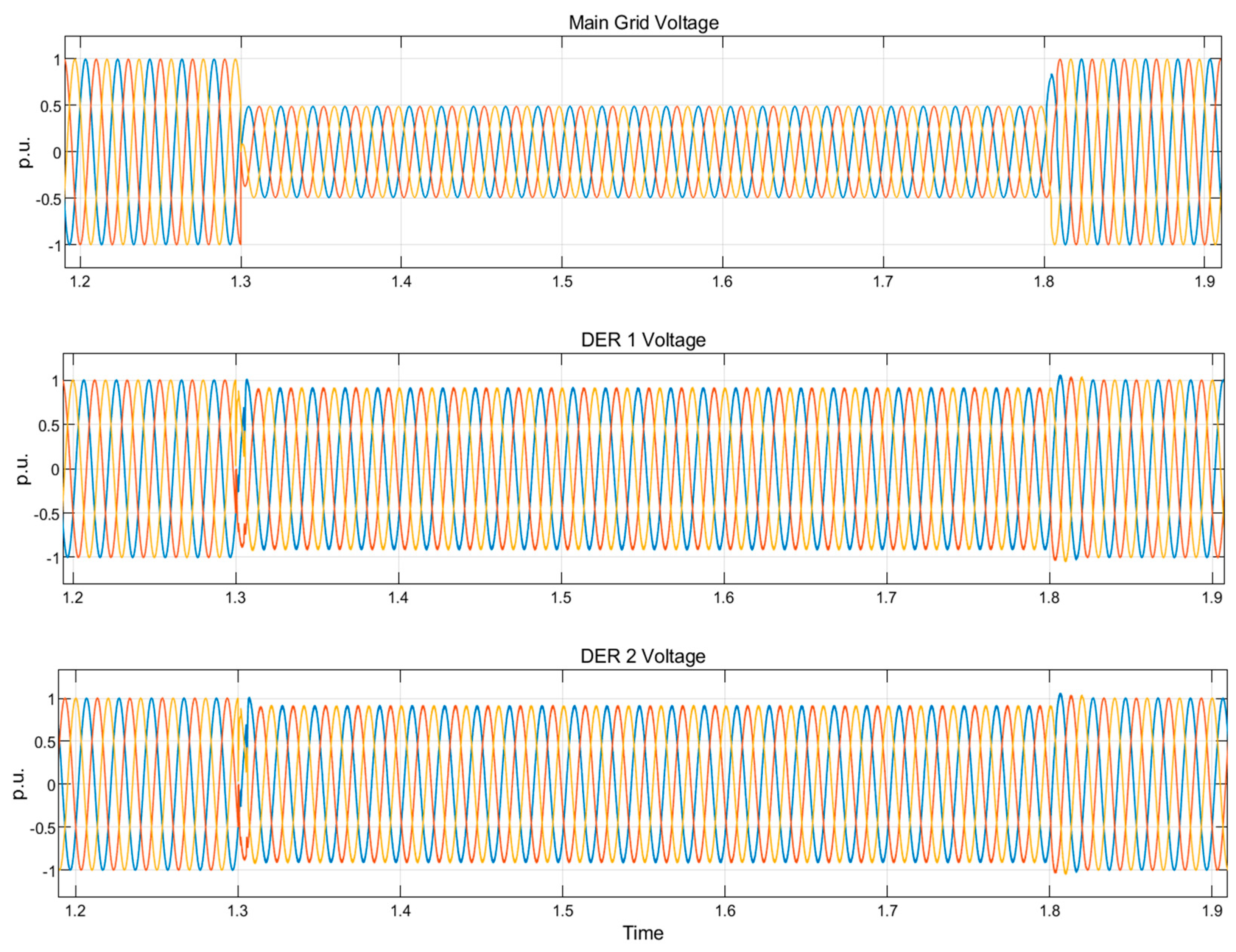
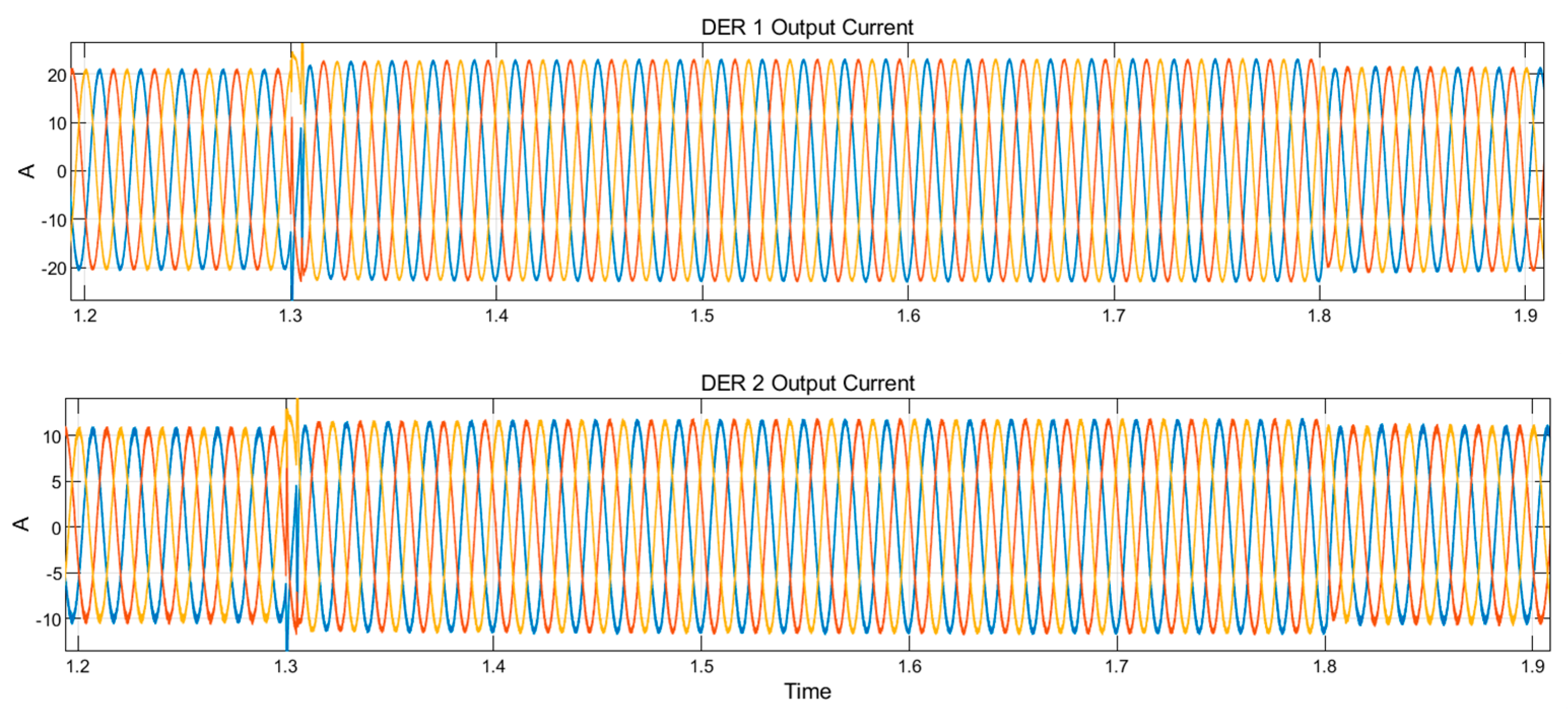
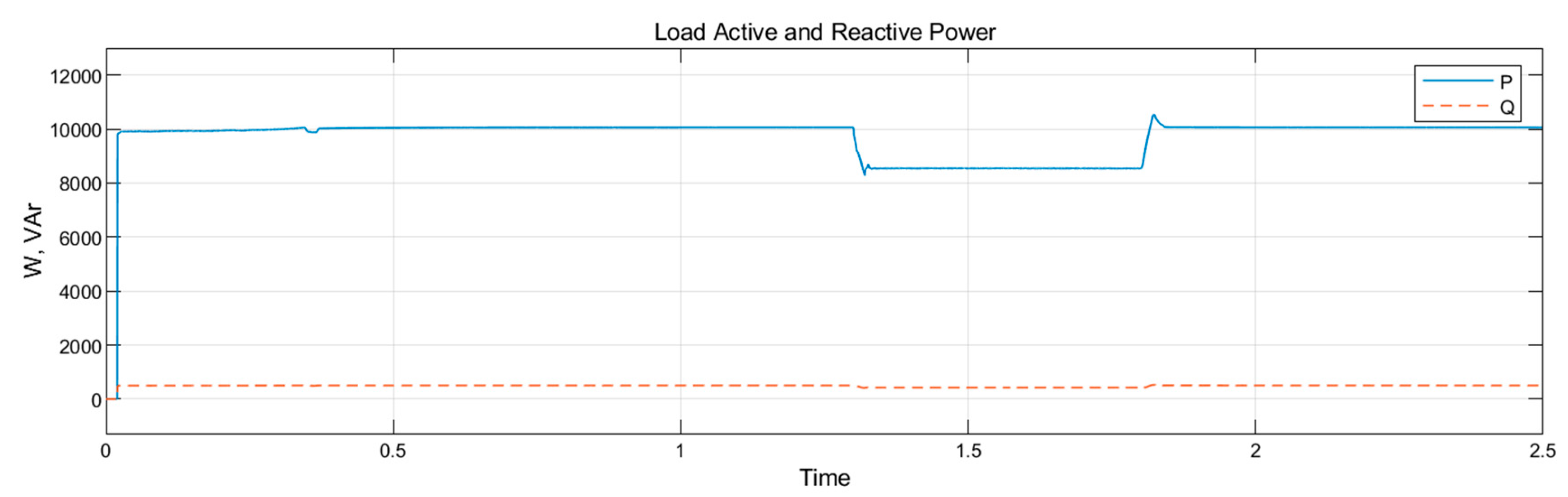
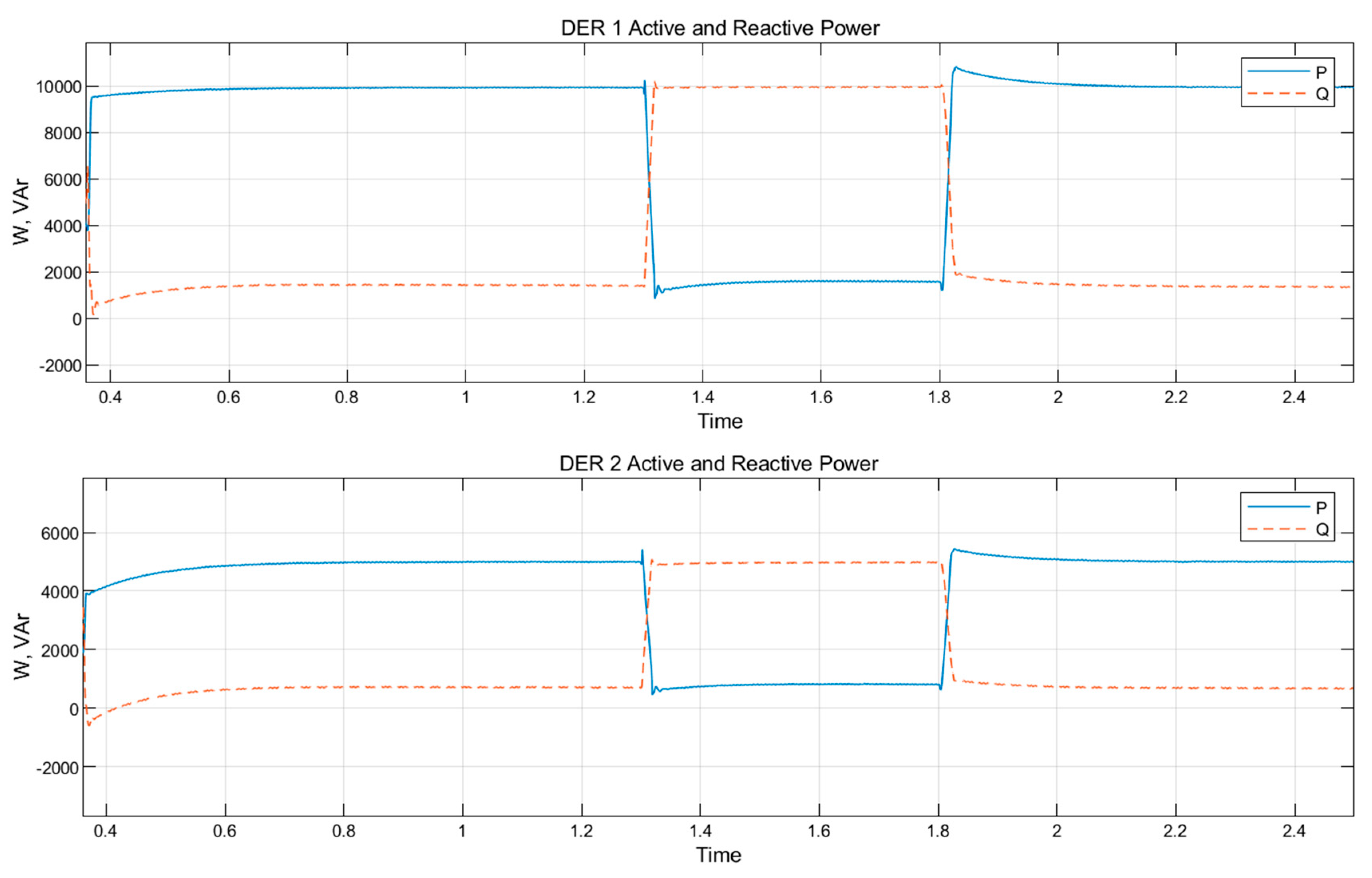
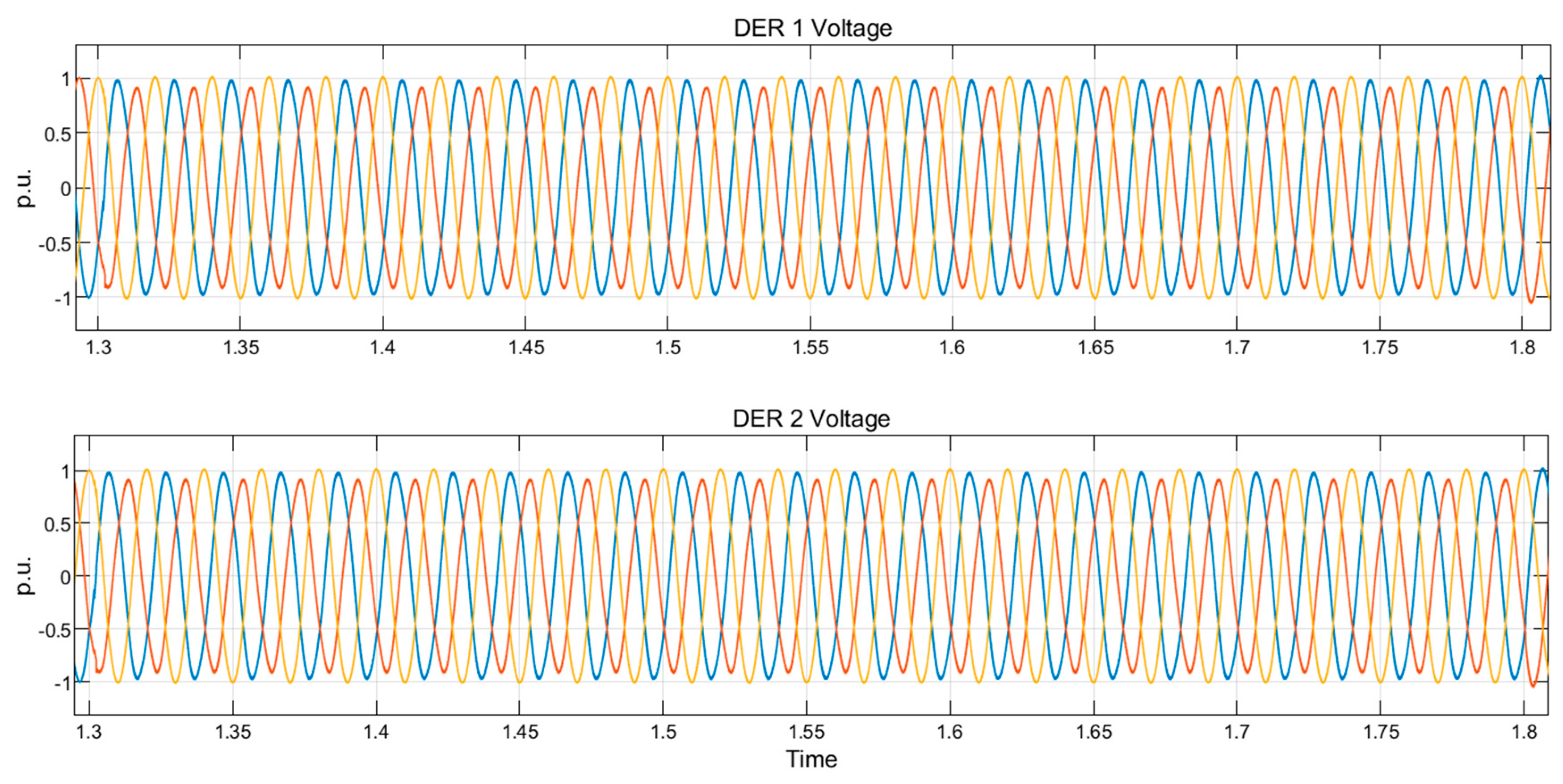
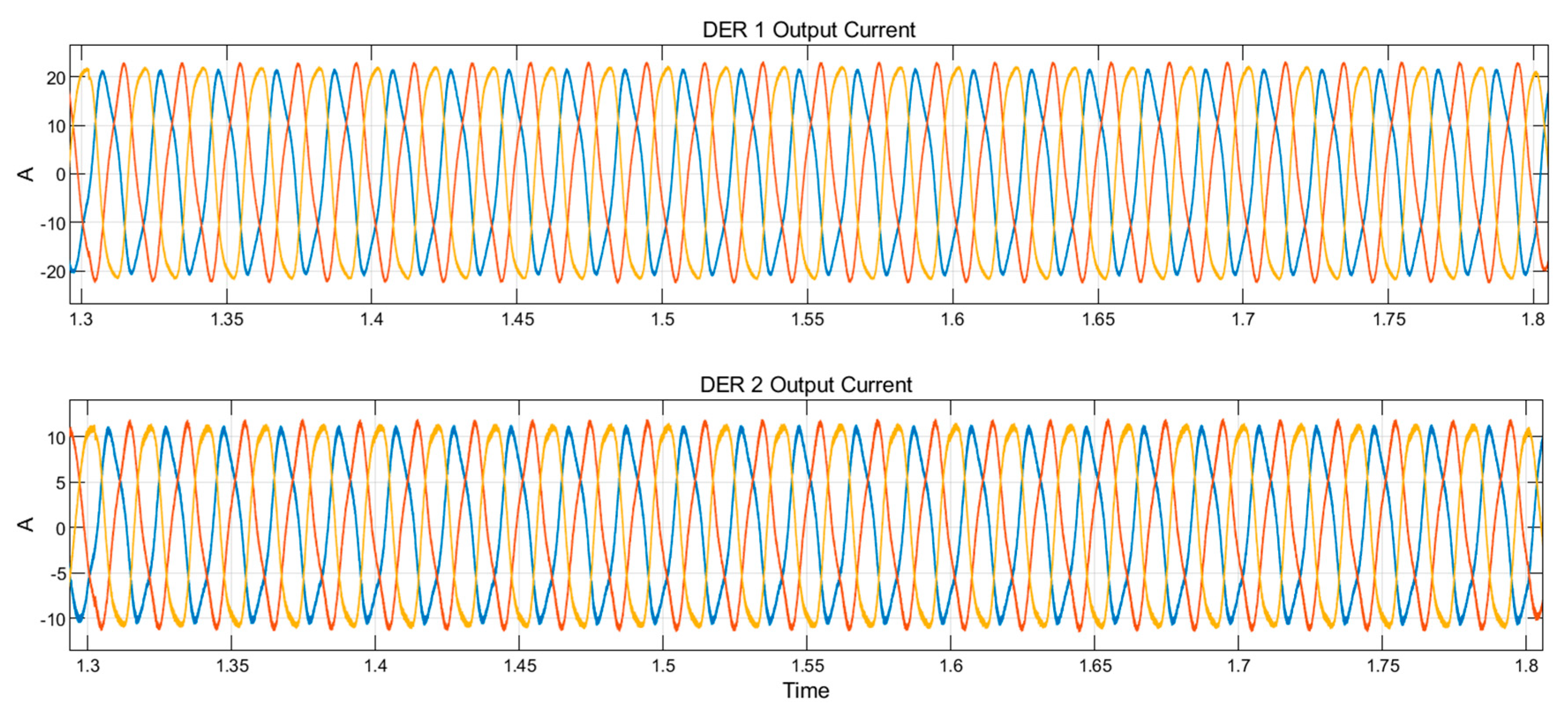
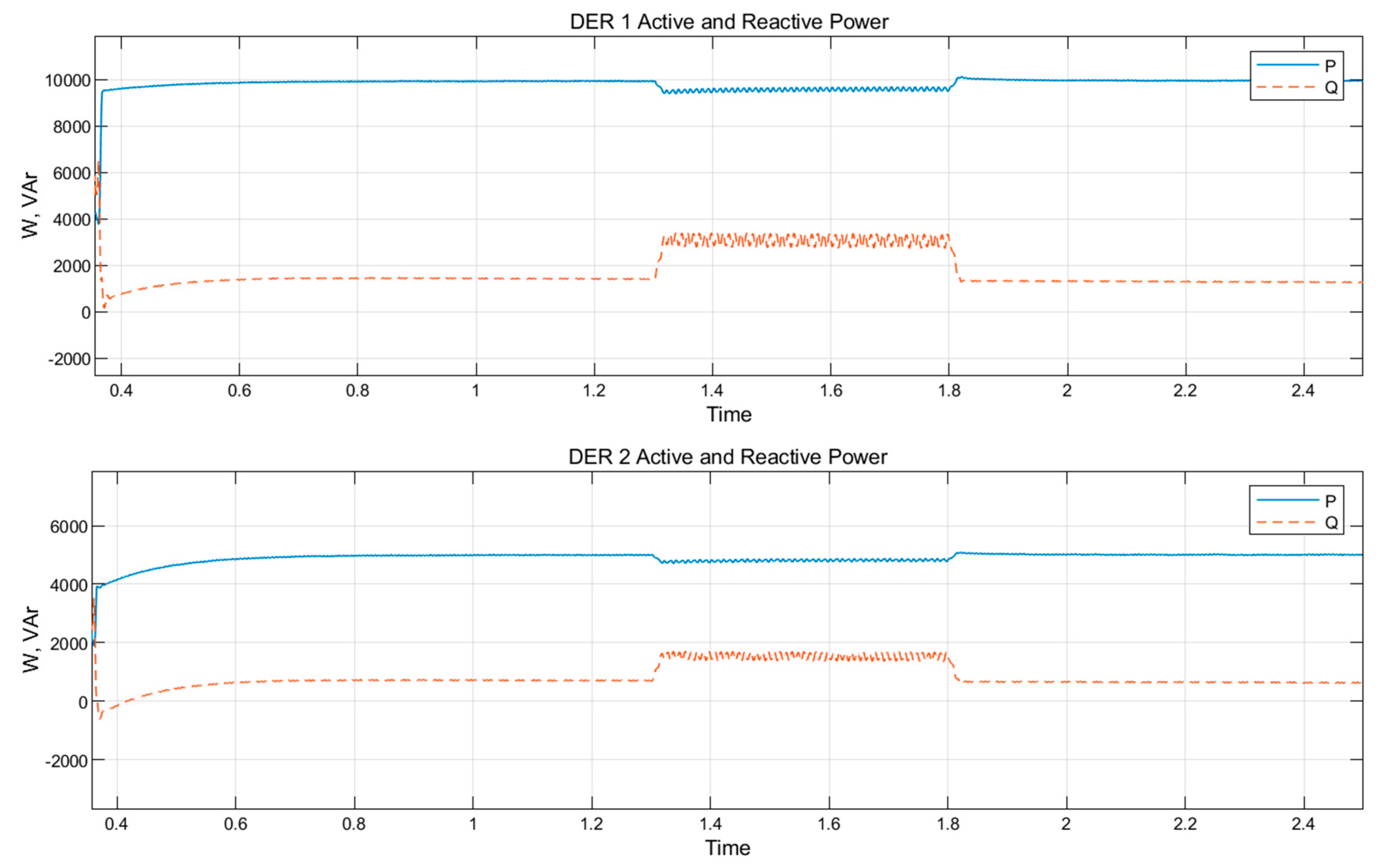
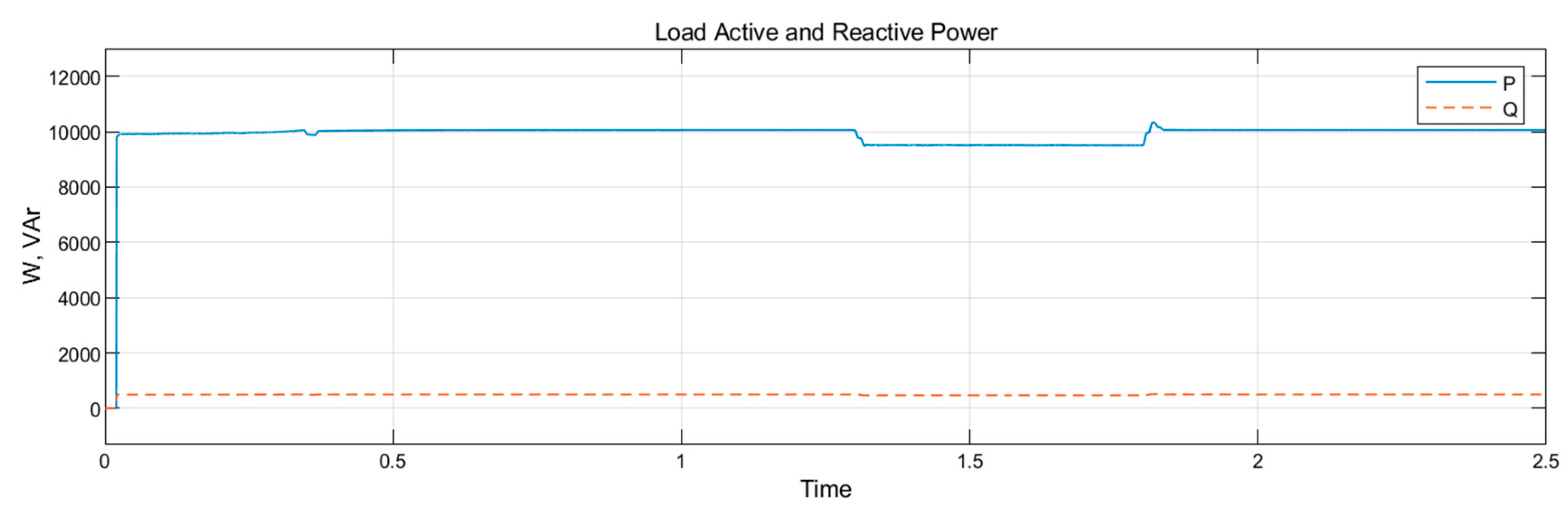
5. Results and Discussions
5.1. Symmetrical Fault
5.2. Asymmetrical Fault
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Viral, R.; Khatod, D.K. Optimal planning of distributed generation systems in distribution system: A review. Renew. Sustain. Energy Rev. 2012, 16, 5146–5165. [Google Scholar] [CrossRef]
- Tan, X.; Li, Q.; Wang, H. Advances and trends of energy storage technology in Microgrid. Int. J. Electr. Power Energy Syst. 2013, 44, 179–191. [Google Scholar] [CrossRef]
- Lai, J.; Lu, X.; Li, X.; Tang, R.-L. Distributed Multiagent-Oriented Average Control for Voltage Restoration and Reactive Power Sharing of Autonomous Microgrids. IEEE Access 2018, 6, 25551–25561. [Google Scholar] [CrossRef]
- Strasser, T.; Andren, F.; Kathan, J.; Cecati, C.; Buccella, C.; Siano, P.; Leitao, P.; Zhabelova, G.; Vyatkin, V.; Vrba, P.; et al. A Review of Architectures and Concepts for Intelligence in Future Electric Energy Systems. IEEE Trans. Ind. Electron. 2015, 62, 2424–2438. [Google Scholar] [CrossRef]
- Saleem, H.A. Microgrid Modeling and Grid Interconnection Studies. Master’s Thesis, University of Tennessee, Knoxville, TN, USA, 2014. [Google Scholar]
- Lu, X.; Wang, J.; Guerrero, J.M. Virtual Impedance Based Fault Current Limiters for Inverter Dominated AC Microgrids. IEEE Trans. Smart Grid 2018, 3053, 1599–1612. [Google Scholar] [CrossRef]
- Khadkikar, V. Enhancing Electric Power Quality Using UPQC: A Comprehensive Overview. IEEE Trans. Power Electron. 2012, 27, 2284–2297. [Google Scholar] [CrossRef]
- Sadeghkhani, I.; Esmail, M.; Golshan, H. A Current Limiting Strategy to Improve Fault Ride-Through of Inverter Interfaced Autonomous Microgrids. IEEE Trans. Smart Grid 2017, 8, 2138–2148. [Google Scholar] [CrossRef]
- Pogaku, N.; Prodanović, M.; Green, T.C. Modeling, analysis and testing of autonomous operation of an inverter-based microgrid. IEEE Trans. Power Electron. 2007, 22, 613–625. [Google Scholar] [CrossRef]
- Rocabert, J.; Luna, A.; Blaabjerg, F.; Rodriguez, P. Control of Power Converters in AC Microgrids. IEEE Trans. Power Electron. 2012, 27, 4734–4749. [Google Scholar] [CrossRef]
- Baghaee, H.R.; Mirsalim, M.; Gharehpetian, G.B.; Talebi, H.A. A new current limiting strategy and fault model to improve fault ride-through capability of inverter interfaced DERs in autonomous microgrids. Sustain. Energy Technol. Assess. 2017, 24, 71–81. [Google Scholar] [CrossRef]
- Guerrero, J.M.; GarciadeVicuna, L.; Matas, J.; Castilla, M.; Miret, J. A Wireless Controller to Enhance Dynamic Performance of Parallel Inverters in Distributed Generation Systems. IEEE Trans. Power Electron. 2004, 19, 1205–1213. [Google Scholar] [CrossRef]
- Roslan, A.M.; Ahmed, K.H.; Finney, S.J.; Williams, B.W. Improved Instantaneous Average Current-Sharing Control Scheme for Parallel-Connected Inverter Considering Line Impedance Impact in Microgrid Networks. IEEE Trans. Power Electron. 2011, 26, 702–716. [Google Scholar] [CrossRef]
- Caldognetto, T.; Tenti, P. Microgrids Operation Based on Master–Slave Cooperative Control. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 1081–1088. [Google Scholar] [CrossRef]
- Wang, C.; Li, X.; Guo, L.; Li, Y. A seamless operation mode transition control strategy for a microgrid based on master-slave control. Sci. China Technol. Sci. 2012, 55, 1644–1654. [Google Scholar] [CrossRef]
- Papadimitriou, C.N.; Zountouridou, E.I.; Hatziargyriou, N.D. Review of hierarchical control in DC microgrids. Electr. Power Syst. Res. 2015, 122, 159–167. [Google Scholar] [CrossRef]
- Tayab, U.B.; Roslan, M.A.B.; Hwai, L.J.; Kashif, M. A review of droop control techniques for microgrid. Renew. Sustain. Energy Rev. 2017, 76, 717–727. [Google Scholar] [CrossRef]
- Zhao, X.; Guerrero, J.M.; Savaghebi, M.; Vasquez, J.C.; Wu, X.; Sun, K. Low-Voltage Ride-Through Operation of Power Converters in Grid-Interactive Microgrids by Using Negative-Sequence Droop Control. IEEE Trans. Power Electron. 2017, 32, 3128–3142. [Google Scholar] [CrossRef]
- Kroposki, B.; Pink, C.; DeBlasio, R.; Thomas, H.; Simões, M.; Sen, P.K. Benefits of Power Electronic Interfaces for Distributed Energy Systems. IEEE Trans. Energy Convers. 2010, 25, 901–908. [Google Scholar] [CrossRef]
- Gkavanoudis, S.I.; Demoulias, C.S. A Control Strategy for Enhancing the Fault Ride-Through Capability of a Microgrid During Balanced and Unbalanced Grid Voltage Sags. Sustain. Energy Grids Netw. 2015, 3, 1–11. [Google Scholar] [CrossRef]
- Glover, J.D.; Sarma, M.S.; Overbye, T.J. Power System Analysis and Design, 5th ed.; Global Engineering Publisher: Stamford, CT, USA, 2012; ISBN 9781111425777. [Google Scholar]
- Kothari, D.P.; Nagrath, I.J. Modern Power System Analysis, 3rd ed.; Tata McGraw Hill Education Private Limited: New Delhi, India, 2003; ISBN 9780070494893. [Google Scholar]
- Buraimoh, E.; Davidson, I.E. Comparative Analysis of the Fault Ride-Through Capabilities of the VSG Methods of Microgrid Inverter Control under Faults. In Proceedings of the SAUPEC/RobMech/PRASA 2019, Bloemfontein, South Africa, 28–30 January 2019; pp. 400–405. [Google Scholar]
- Ramana Reddy, K.V.; Ramesh Babu, N.; Sanjeevikumar, P. A Review on Grid Codes and Reactive Power Management in Power Grids with WECS; Springer: Singapore, 2018; pp. 525–539. [Google Scholar]
- Meegahapola, L.; Datta, M.; Nutkani, I.; Conroy, J. Role of fault ride-through strategies for power grids with 100% power electronic-interfaced distributed renewable energy resources. Wiley Interdiscip. Rev. Energy Environ. 2018, 7, e292. [Google Scholar] [CrossRef]
- Wang, X.; Yang, Z.; Fan, B.; Xu, W. Control Strategy of Three-Phase Photovoltaic Inverter under Low-Voltage Ride-Through Condition. Math. Probl. Eng. 2015, 2015, 1–23. [Google Scholar] [CrossRef]
- Zhao, X. Power System Support Functions Provided by Smart Inverters—A Review. CPSS Trans. Power Electron. Appl. 2018, 3, 25–35. [Google Scholar] [CrossRef]
- Luna, A.; Rocabert, J.; Candela, J.I.; Hermoso, J.R.; Teodorescu, R.; Blaabjerg, F.; Rodriguez, P. Grid Voltage Synchronization for Distributed Generation Systems Under Grid Fault Conditions. IEEE Trans. Ind. Appl. 2015, 51, 3414–3425. [Google Scholar] [CrossRef]
- Chen, L.; Chen, H.; Yang, J.; Zhu, L.; Tang, Y.; Koh, L.H.; Xu, Y.; Zhang, C.; Liao, Y.; Ren, L. Comparison of Superconducting Fault Current Limiter and Dynamic Voltage Restorer for LVRT Improvement of High Penetration Microgrid. IEEE Trans. Appl. Supercond. 2017, 27, 1–7. [Google Scholar] [CrossRef]
- Rezkallah, M.; Chandra, A.; Hamadi, A.; Ibrahim, H.; Ghandour, M. Power Quality in Smart Grids. In Pathways to a Smarter Power System; Elsevier Ltd.: Amsterdam, The Netherlands, 2019; pp. 225–245. ISBN 9780081025925. [Google Scholar]
- Hagh, M.T.; Khalili, T. A Review of Fault Ride-Through of PV and Wind Renewable Energies in Grid Codes. Int. J. Energy Res. 2018, 43, 1342–1356. [Google Scholar] [CrossRef]
- Kou, W.; Wei, D. Fault ride through strategy of inverter-interfaced microgrids embedded in distributed network considering fault current management. Sustain. Energy Grids Netw. 2018, 15, 43–52. [Google Scholar] [CrossRef]
- Zamani, M.A.; Yazdani, A.; Sidhu, T.S. A Control Strategy for Enhanced Operation of Inverter-Based Microgrids Under Transient Disturbances and Network Faults. IEEE Trans. Power Deliv. 2012, 27, 1737–1747. [Google Scholar] [CrossRef]
- Buraimoh, E.; Davidson, I.E. Development of an IGBT-Diode based Fault Current Limiter for Fault Ride-Through Enhancement in Microgrid Application. In Proceedings of the IEEE PES/IAS PowerAfrica, Abuja, Nigeria, 20–23 August 2019; pp. 190–195. [Google Scholar]
- He, H.; Chen, L.; Yin, T.; Cao, Z.; Yang, J.; Tu, X.; Ren, L. Application of a SFCL for Fault Ride-Through Capability Enhancement of DG in a Microgrid System and Relay Protection Coordination. IEEE Trans. Appl. Supercond. 2016, 26, 1–8. [Google Scholar] [CrossRef]
- Choi, D.H.; Yoo, J.I.; Kim, D.; Lee, S.H.; Park, J.W. Analysis on Effect of SFCL Applied to an Isolated Microgrid with a Dynamic Load Model. IEEE Trans. Appl. Supercond. 2017, 27, 1–4. [Google Scholar] [CrossRef]
- Naderi, S.B.; Negnevitsky, M.; Jalilian, A.; Tarafdar Hagh, M.; Muttaqi, K.M. Optimum Resistive Type Fault Current Limiter: An Efficient Solution to Achieve Maximum Fault Ride-Through Capability of Fixed-Speed Wind Turbines During Symmetrical and Asymmetrical Grid Faults. IEEE Trans. Ind. Appl. 2017, 53, 538–548. [Google Scholar] [CrossRef]
- Rashid, G.; Ali, M.H. Fault ride through capability improvement of DFIG based wind farm by fuzzy logic controlled parallel resonance fault current limiter. Electr. Power Syst. Res. 2017, 146, 1–8. [Google Scholar] [CrossRef]
- Ghanbari, T.; Farjah, E. Unidirectional Fault Current Limiter: An Efficient Interface Between the Microgrid and Main Network. IEEE Trans. Power Syst. 2013, 28, 1591–1598. [Google Scholar] [CrossRef]
- Piya, P.; Ebrahimi, M.; Karimi-Ghartemani, M.; Khajehoddin, S.A. Fault Ride-Through Capability of Voltage-Controlled Inverters. IEEE Trans. Ind. Electron. 2018, 65, 7933–7943. [Google Scholar] [CrossRef]
- Palizban, O.; Kauhaniemi, K.; Guerrero, J.M. Microgrids in active network management—Part I: Hierarchical control, energy storage, virtual power plants, and market participation. Renew. Sustain. Energy Rev. 2014, 36, 428–439. [Google Scholar] [CrossRef]
- Palizban, O.; Kauhaniemi, K.; Guerrero, J.M. Microgrids in active network management—Part II: System operation, power quality and protection. Renew. Sustain. Energy Rev. 2014, 36, 440–451. [Google Scholar] [CrossRef]
- Li, X.; Zhang, H.; Shadmand, M.B.; Balog, R.S. Model Predictive Control of a Voltage-Source Inverter with Seamless Transition between Islanded and Grid-Connected Operations. IEEE Trans. Ind. Electron. 2017, 64, 7906–7918. [Google Scholar] [CrossRef]
- Kleftakis, V.; Lagos, D.; Papadimitriou, C.; Hatziargyriou, N.D. Seamless Transition between Interconnected and Islanded Operation of DC Microgrids. IEEE Trans. Smart Grid 2019, 10, 248–256. [Google Scholar] [CrossRef]
- Ochs, D.S.; Mirafzal, B.; Sotoodeh, P. A method of seamless transitions between grid-tied and stand-alone modes of operation for utility-interactive three-phase inverters. IEEE Trans. Ind. Appl. 2014, 50, 1934–1941. [Google Scholar] [CrossRef]
- Micallef, A.; Apap, M.; Spiteri-Staines, C.; Guerrero, J.M. Single-Phase Microgrid with Seamless Transition Capabilities between Modes of Operation. IEEE Trans. Smart Grid 2015, 6, 2736–2745. [Google Scholar] [CrossRef]
- Rasheduzzaman, M.; Kimball, J.W. Modeling and Tuning of an Improved Delayed-Signal-Cancellation PLL for Microgrid Application. IEEE Trans. Energy Convers. 2019, 34, 712–721. [Google Scholar] [CrossRef]
- Gude, S.; Chu, C.C. Three-Phase PLLs by Using Frequency Adaptive Multiple Delayed Signal Cancellation Prefilters under Adverse Grid Conditions. IEEE Trans. Ind. Appl. 2018, 54, 3832–3844. [Google Scholar] [CrossRef]
- Huang, Q.; Rajashekara, K. An Improved Delayed Signal Cancellation PLL for Fast Grid Synchronization under Distorted and Unbalanced Grid Condition. IEEE Trans. Ind. Appl. 2017, 53, 4985–4997. [Google Scholar] [CrossRef]
- Karimi, M.; Mokhtari, H.; Iravani, M.R. Wavelet based on-line disturbance detection for power quality applications. IEEE Trans. Power Deliv. 2000, 15, 1212–1220. [Google Scholar] [CrossRef]
- Mokhtari, H.; Karimi-Ghartemani, M.; Iravani, M.R. Experimental performance evaluation of a wavelet-based on-line voltage detection method for power quality applications. IEEE Trans. Power Deliv. 2002, 17, 161–172. [Google Scholar] [CrossRef]
- Cardenas, R.; Diaz, M.; Rojas, F.; Clare, J. Fast Convergence Delayed Signal Cancellation Method for Sequence Component Separation. IEEE Trans. Power Deliv. 2015, 30, 2055–2057. [Google Scholar] [CrossRef]
- Golestan, S.; Freijedo, F.D.; Vidal, A.; Yepes, A.G.; Guerrero, J.M.; Doval-Gandoy, J. An Efficient Implementation of Generalized Delayed Signal Cancellation PLL. IEEE Trans. Power Electron. 2016, 31, 1085–1094. [Google Scholar] [CrossRef]
- Contreras, C.; Guajardo, D.; Diaz, M.; Rojas, F.; Espinoza, M.; Cardenas, R. Fast Delayed Signal Cancellation based PLL for unbalanced grid conditions. In Proceedings of the 2018 IEEE International Conference on Automation/Congress of the Chilean Association of Automatic Control, Concepción, Chile, 17–19 October 2018; pp. 1–6. [Google Scholar]
- Wang, Y.F.; Li, Y.W. Analysis and digital implementation of cascaded delayed-signal-cancellation PLL. IEEE Trans. Power Electron. 2011, 26, 1067–1080. [Google Scholar] [CrossRef]
- Mahlooji, M.H.; Mohammadi, H.R.; Rahimi, M. A review on modeling and control of grid-connected photovoltaic inverters with LCL filter. Renew. Sustain. Energy Rev. 2018, 81, 563–578. [Google Scholar] [CrossRef]
- Ahmad, A.A.; Abrishamifar, A.; Farzi, M. A New Design Procedure for Output LC Filter of Single Phase Inverters. In Proceedings of the 3rd International Conference on Power Electronics and Intelligent Transportation System, Shenzhen, China, 13–14 November 2010; pp. 86–91. [Google Scholar]
- Gomes, C.C.; Cupertino, A.F.; Pereira, H.A. Damping techniques for grid-connected voltage source converters based on LCL filter: An overview. Renew. Sustain. Energy Rev. 2018, 81, 116–135. [Google Scholar] [CrossRef]
- Che, L.; Shahidehpour, M.; Alabdulwahab, A.; Al-Turki, Y. Hierarchical Coordination of a Community Microgrid With AC and DC Microgrids. IEEE Trans. Smart Grid 2015, 6, 3042–3051. [Google Scholar] [CrossRef]
- Dissanayake, A.M.; Ekneligoda, N.C. Transient Optimization of Parallel Connected Inverters in Islanded AC Microgrids. IEEE Trans. Smart Grid 2018, 10, 1–12. [Google Scholar] [CrossRef]
- Guo, Y.; Lu, X.; Chen, L.; Zheng, T.; Wang, J.; Mei, S. Functional-Rotation—Based Active Dampers in AC Microgrids with Multiple Parallel Interface. IEEE Trans. Ind. Appl. 2018, 9994, 1–9. [Google Scholar] [CrossRef]
- Wei, B.; Guerrero, J.M.; Vásquez, J.C.; Guo, X. A Circulating-Current Suppression Method for Parallel Connected Voltage Source Inverters (VSI) with Common DC and AC Buses Xiaoqiang Guo. IEEE Trans. Ind. Appl. 2017, 9994, 1–11. [Google Scholar]
- Sun, Y.; Hou, X.; Yang, J.; Han, H.; Su, M.; Guerrero, J.M. New Perspectives on Droop Control in AC Microgrid. IEEE Trans. Ind. Electron. 2017, 64, 5741–5745. [Google Scholar] [CrossRef]
- Mehrasa, M.; Pouresmaeil, E.; Sepehr, A.; Pournazarian, B.; Marzband, M.; Catalão, J.P.S. Control technique for the operation of grid-tied converters with high penetration of renewable energy resources. Electr. Power Syst. Res. 2019, 166, 18–28. [Google Scholar] [CrossRef]
- Liu, J.; Miura, Y.; Bevrani, H.; Ise, T. Enhanced Virtual Synchronous Generator Control for Parallel Inverters in Microgrids. IEEE Trans. Smart Grid 2017, 8, 2268–2277. [Google Scholar] [CrossRef]
- Ramirez, D.; Martinez-Rodrigo, F.; de Pablo, S.; Carlos Herrero-de Lucas, L. Assessment of a non linear current control technique applied to MMC-HVDC during grid disturbances. Renew. Energy 2017, 101, 945–963. [Google Scholar] [CrossRef]
- Morales, A.; Robe, X.; Sala, M.; Prats, P.; Aguerri, C.; Torres, E. Advanced grid requirements for the integration of wind farms into the Spanish transmission system. IET Renew. Power Gener. 2008, 2, 47–59. [Google Scholar] [CrossRef]
- Jiménez, F.; Gómez-Lázaro, E.; Fuentes, J.A.; Molina-García, A.; Vigueras-Rodríguez, A. Validation of a double fed induction generator wind turbine model and wind farm verification following the Spanish grid code. Wind Energy 2012, 15, 645–659. [Google Scholar] [CrossRef]
- Bakkar, M.; Bogarra, S.; Córcoles, F.; Saura, J.; Moreno, M. Power Control Strategies During Voltage Sags According to Spanish Grid Code. Renew. Energy Power Qual. J. 2018, 1, 493–498. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, Y.; Wang, S.; Chen, J.; Gong, C. Impedance-Phased Dynamic Control Method for Grid-Connected Inverters in a Weak Grid. IEEE Trans. Power Electron. 2017, 32, 274–283. [Google Scholar] [CrossRef]
- Davari, M.; Mohamed, Y.A.-R.I. Robust Vector Control of a Very Weak-Grid-Connected Voltage-Source Converter Considering the Phase-Locked Loop Dynamics. IEEE Trans. Power Electron. 2017, 32, 977–994. [Google Scholar] [CrossRef]
- Sadeghkhani, I.; Esmail, M.; Golshan, H.; Mehrizi-sani, A.; Guerrero, J.M. Low-Voltage Ride-Through of A Droop-Based Three-Phase Four-Wire Grid-Connected Microgrid. IET Gener. Transm. Distrib. 2018, 12, 1906–1914. [Google Scholar] [CrossRef]
- Camacho, A.; Castilla, M.; Miret, J.; Vasquez, J.C.; Alarcon-Gallo, E. Flexible Voltage Support Control for Three-Phase Distributed Generation Inverters Under Grid Fault. IEEE Trans. Ind. Electron. 2013, 60, 1429–1441. [Google Scholar] [CrossRef]
- Camacho, A.; Castilla, M.; Miret, J.; Borrell, A.; de Vicuna, L.G. Active and Reactive Power Strategies With Peak Current Limitation for Distributed Generation Inverters During Unbalanced Grid Faults. IIEEE Trans. Ind. Electron. 2015, 62, 1515–1525. [Google Scholar] [CrossRef]
- Haque, M.M.; Wolfs, P. A review of high PV penetrations in LV distribution networks: Present status, impacts and mitigation measures. Renew. Sustain. Energy Rev. 2016, 62, 1195–1208. [Google Scholar] [CrossRef]
- Standard EN 50160. Voltage Characteristics of Electricity Supploed by Public Distribution Systems; European Committee on Electro-Technical Standardization: Brussels, Belgium, 2001. [Google Scholar]
- International Electrotechnical Commission. Limits for Harmonic Current Emissions; International Electrotechnical Commission: Geneva, Switzerland, 2000. [Google Scholar]
- Sadeghkhani, I.; Golshan, M.E.H.; Mehrizi-Sani, A.; Guerrero, J.M.; Ketabi, A. Transient Monitoring Function-Based Fault Detection for Inverter-Interfaced Microgrids. IEEE Trans. Smart Grid 2018, 9, 2097–2107. [Google Scholar] [CrossRef]
- Dhar, S.; Patnaik, R.K.; Dash, P.K. Fault Detection and Location of Photovoltaic Based DC Microgrid Using Differential Protection Strategy. IEEE Trans. Smart Grid 2018, 9, 4303–4312. [Google Scholar] [CrossRef]



| Parameters | Descriptions | Values |
|---|---|---|
| kVA1 | DER 1 rated power | 12 kVA |
| kVA2 | DER 2 rated power | 6 kVA |
| V abc | Voltage (phase-phase) | 400 V |
| V dc | DC bus voltage | 1100 V |
| f | Frequency | 50 Hz |
| C | LC filter capacitance | 2.31 μF |
| L | LC filter inductance | 11 mH |
| Parameters | Descriptions | Values |
|---|---|---|
| ωcut | Cut-off angular frequency | 100π |
| E | Single-phase voltage reference | 330 V |
| Kp I | Direct-quadrature current loop P gain | 100 |
| Ki I | Direct-quadrature current loop I gain | 1000 |
| Kp PCC+- | Positive sequence and negative sequence P gain | 0.0125 |
| Ki PCC+- | Positive sequence and negative sequence I gain | 2 |
| Parameters | Descriptions | Values |
|---|---|---|
| f min | PLL minimum frequency | 45 Hz |
| Kp PLL | Regulator P gain | 180 |
| Ki PLL | Regulator I gain | 3200 |
| Kd PLL | Regulator D gain | 1 |
| Parameters | Descriptions | Values |
|---|---|---|
| Lr | Reactor inductance | 0.005 |
| Ron | Switch internal resistance | 0.001 |
| Rs | Switch snubber resistance | 0.00001 |
| Voltage Sag | DER | Signal | Total Harmonic Distortion (%) | ||
|---|---|---|---|---|---|
| Pre-Fault | Fault | Post-Fault | |||
| 70% | 1 | Voltage | 0.33 | 1.17 | 0.33 |
| Current | 2.01 | 2.15 | 1.99 | ||
| 2 | Voltage | 0.33 | 1.17 | 0.33 | |
| Current | 3.94 | 1.50 | 3.67 | ||
| 60% | 1 | Voltage | 0.32 | 1.20 | 0.33 |
| Current | 2.06 | 2.06 | 2.09 | ||
| 2 | Voltage | 0.32 | 1.20 | 0.33 | |
| Current | 3.78 | 3.64 | 3.74 | ||
| 50% | 1 | Voltage | 0.32 | 1..25 | 0.33 |
| Current | 2.06 | 2.05 | 2.01 | ||
| 2 | Voltage | 0.32 | 1.25 | 0.33 | |
| Current | 3.78 | 3.37 | 3.84 | ||
| Fault Type | Voltage Unbalance | |
|---|---|---|
| Grid | Microgrid | |
| L-G | 24.14% | 4.44% |
| L-L-G | 24.31% | 7.13% |
| L-L | 35.36% | 12.20% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buraimoh, E.; Davidson, I.E.; Martinez-Rodrigo, F. Fault Ride-Through Enhancement of Grid Supporting Inverter-Based Microgrid Using Delayed Signal Cancellation Algorithm Secondary Control. Energies 2019, 12, 3994. https://doi.org/10.3390/en12203994
Buraimoh E, Davidson IE, Martinez-Rodrigo F. Fault Ride-Through Enhancement of Grid Supporting Inverter-Based Microgrid Using Delayed Signal Cancellation Algorithm Secondary Control. Energies. 2019; 12(20):3994. https://doi.org/10.3390/en12203994
Chicago/Turabian StyleBuraimoh, Elutunji, Innocent E. Davidson, and Fernando Martinez-Rodrigo. 2019. "Fault Ride-Through Enhancement of Grid Supporting Inverter-Based Microgrid Using Delayed Signal Cancellation Algorithm Secondary Control" Energies 12, no. 20: 3994. https://doi.org/10.3390/en12203994
APA StyleBuraimoh, E., Davidson, I. E., & Martinez-Rodrigo, F. (2019). Fault Ride-Through Enhancement of Grid Supporting Inverter-Based Microgrid Using Delayed Signal Cancellation Algorithm Secondary Control. Energies, 12(20), 3994. https://doi.org/10.3390/en12203994







