Numerical Simulation on Deflecting Hydraulic Fracture with Refracturing Using Extended Finite Element Method
Abstract
1. Introduction
2. Numerical Model
2.1. Governing Equations of Seepage-Stress
2.2. Approximation of Extended Finite Element Method (XFEM)
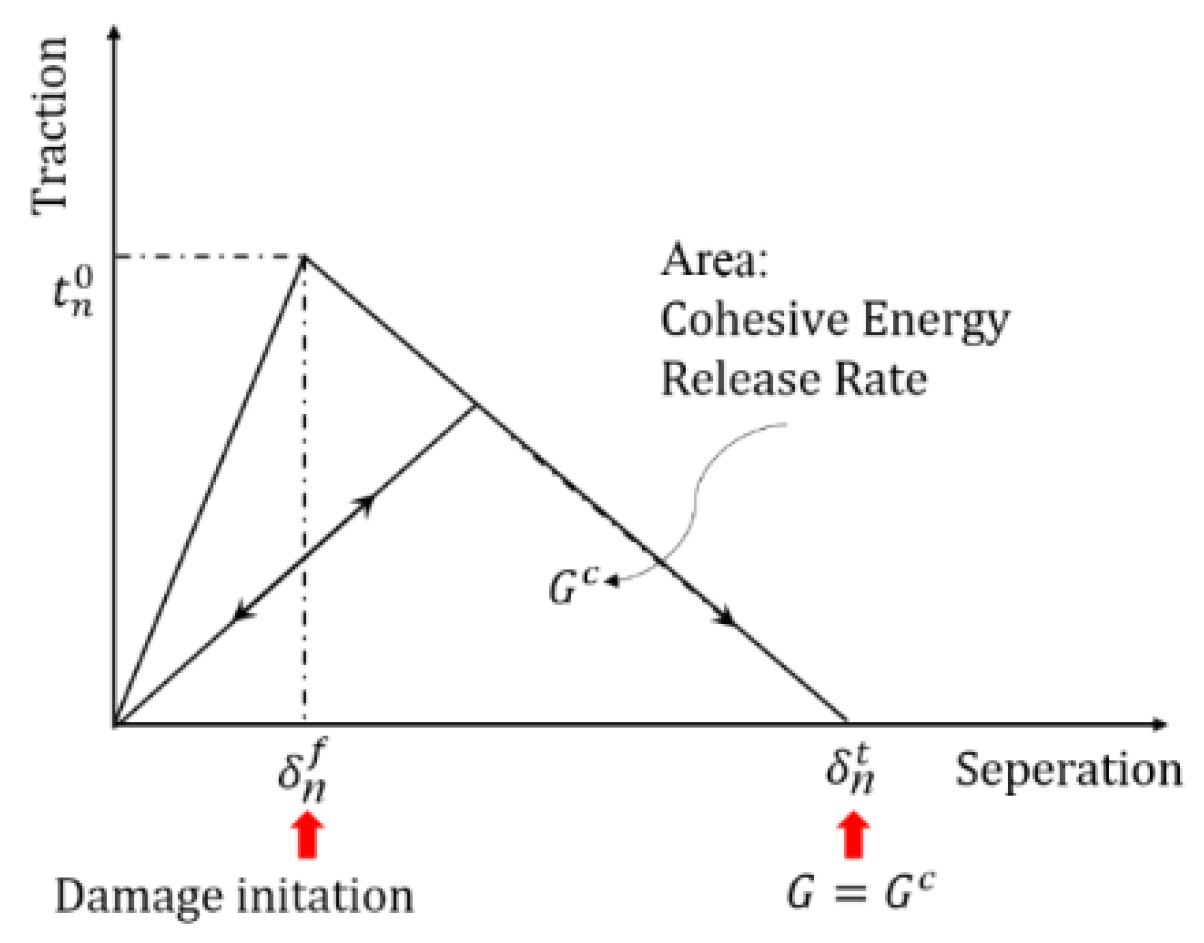
2.3. Crack Initiation and Damage Evolution
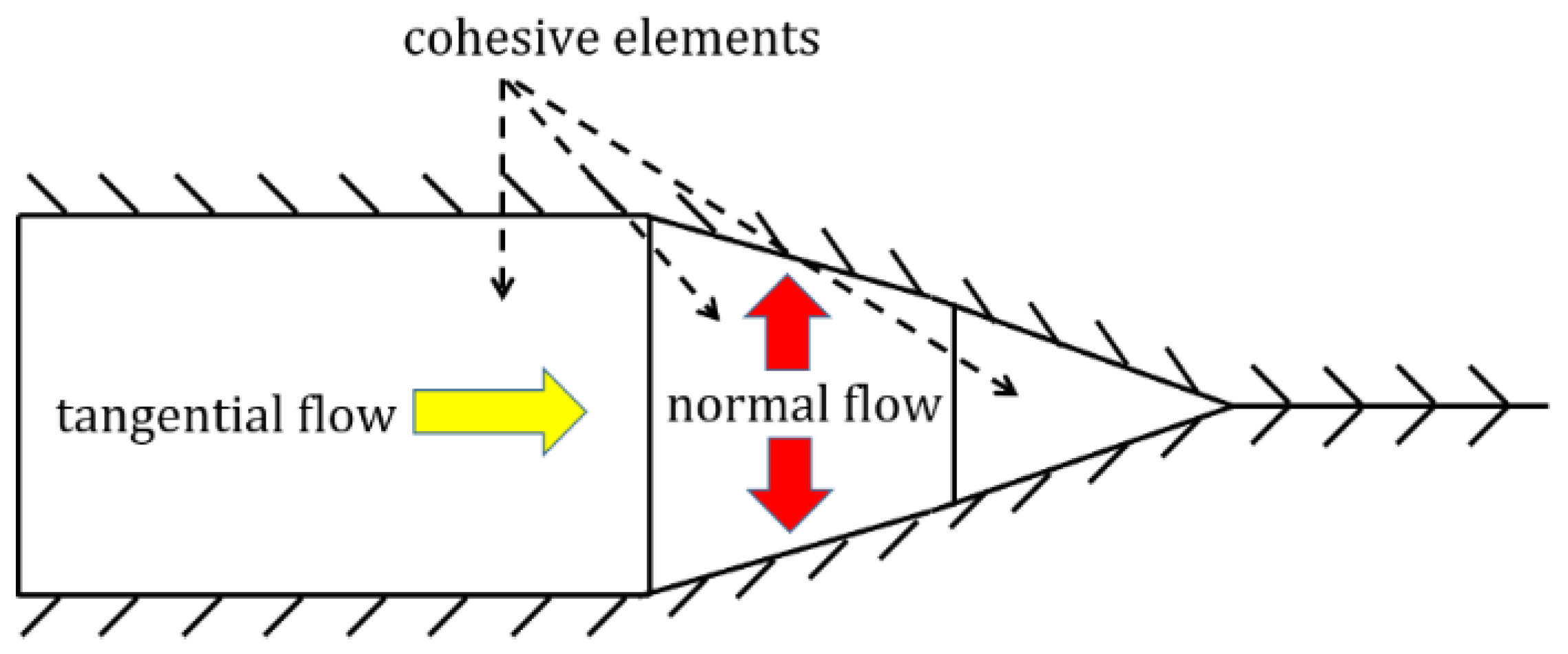
2.4. Fluid Flow within the Fracture
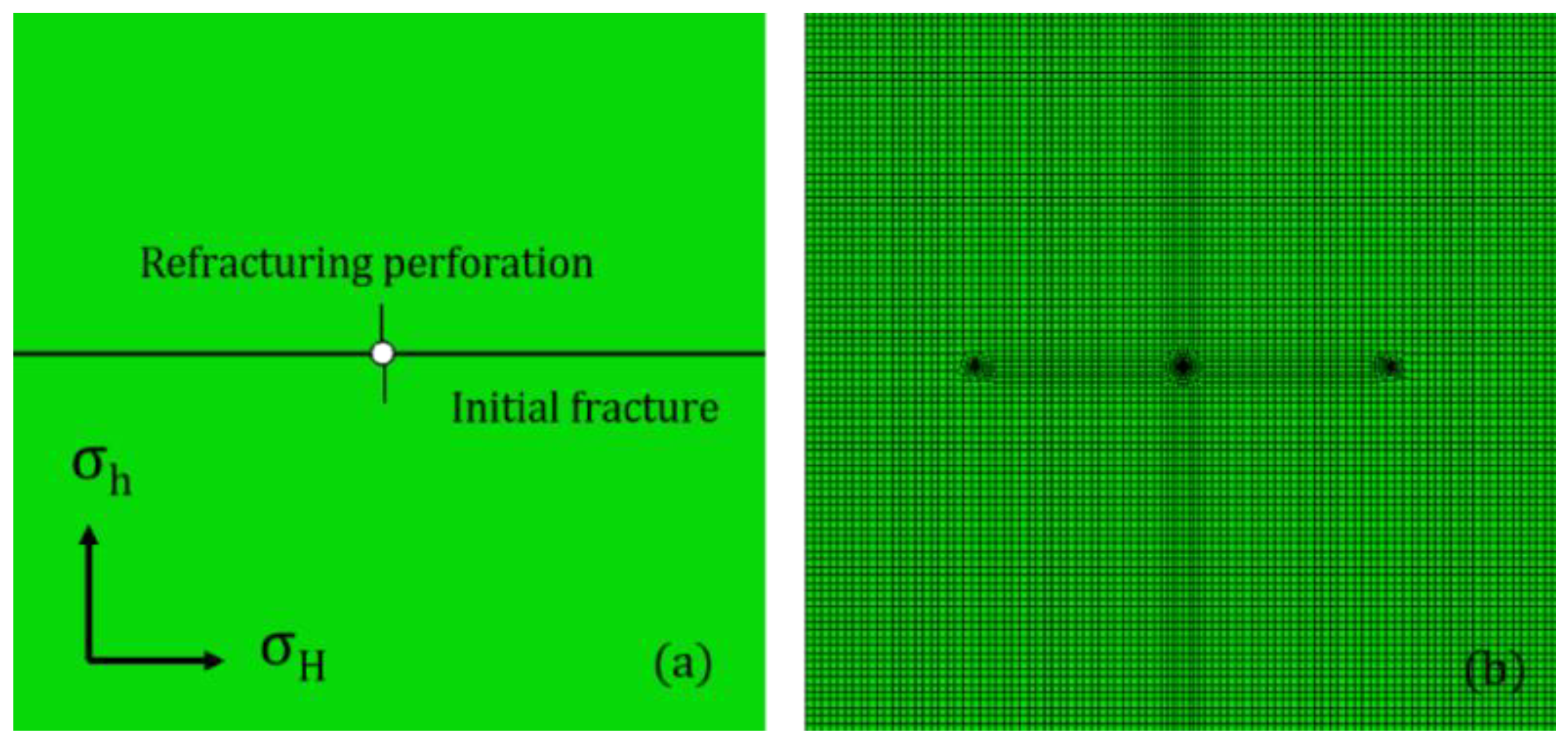
3. Model Construction and Verification
4. Results and Discussion
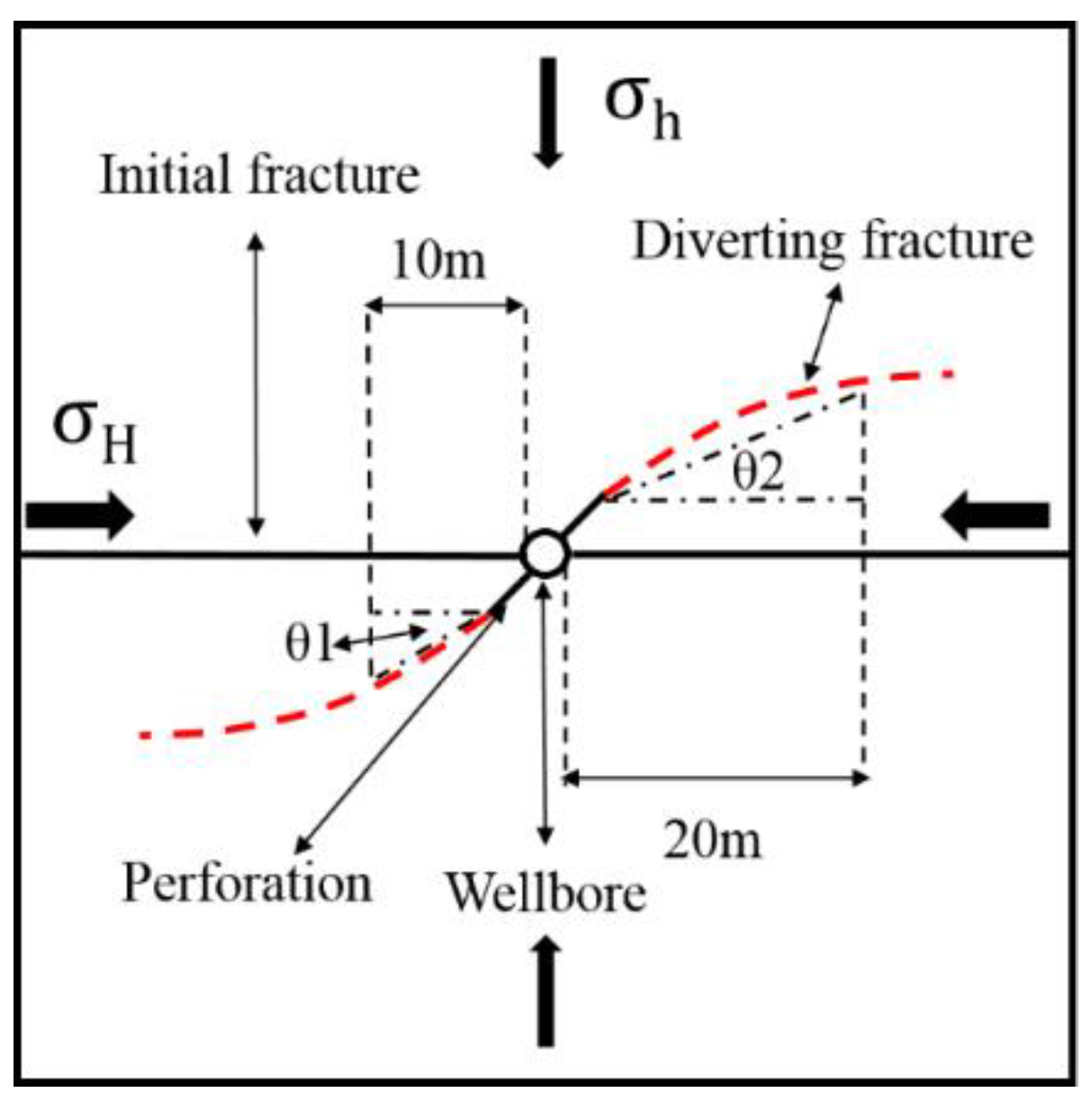
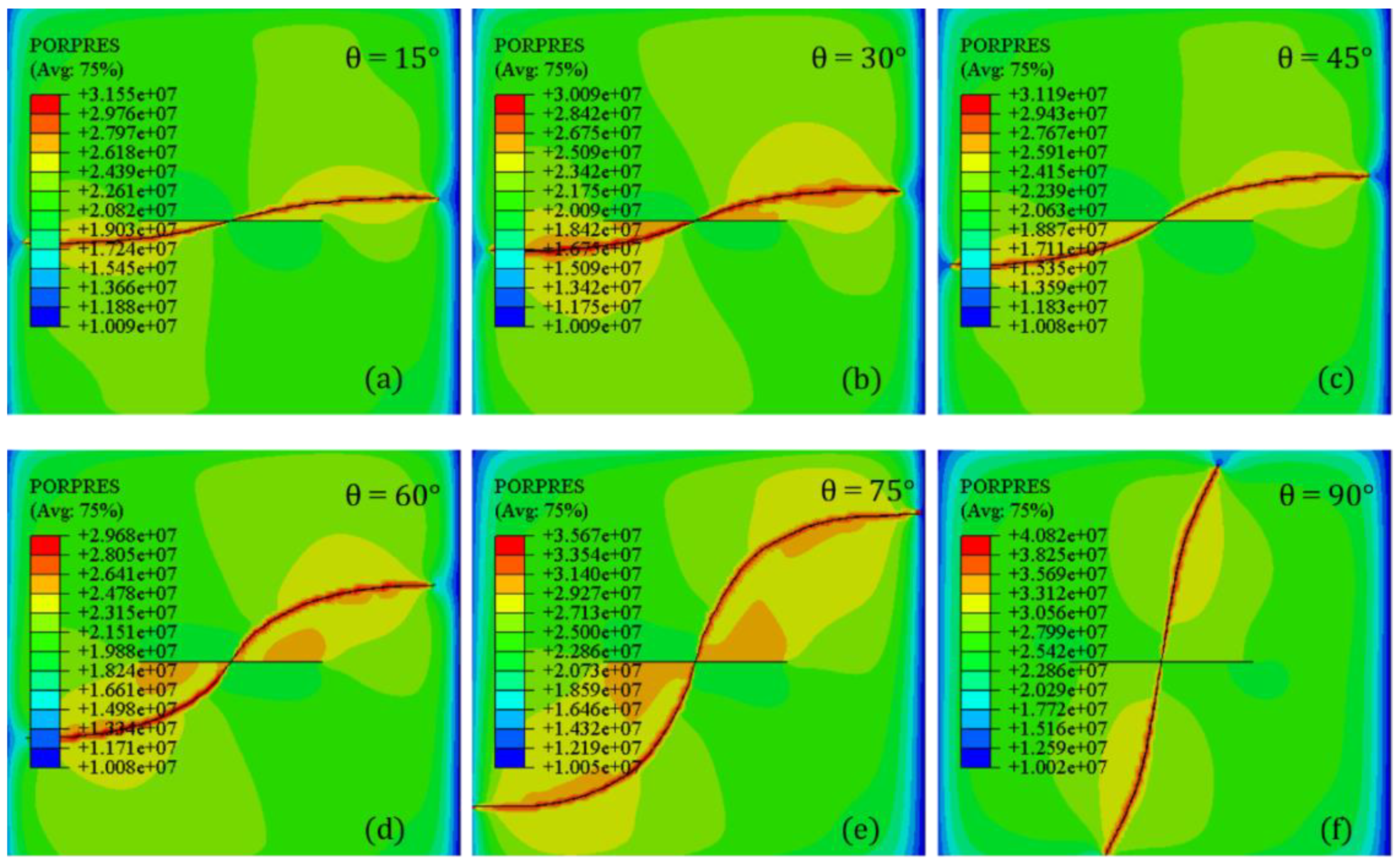
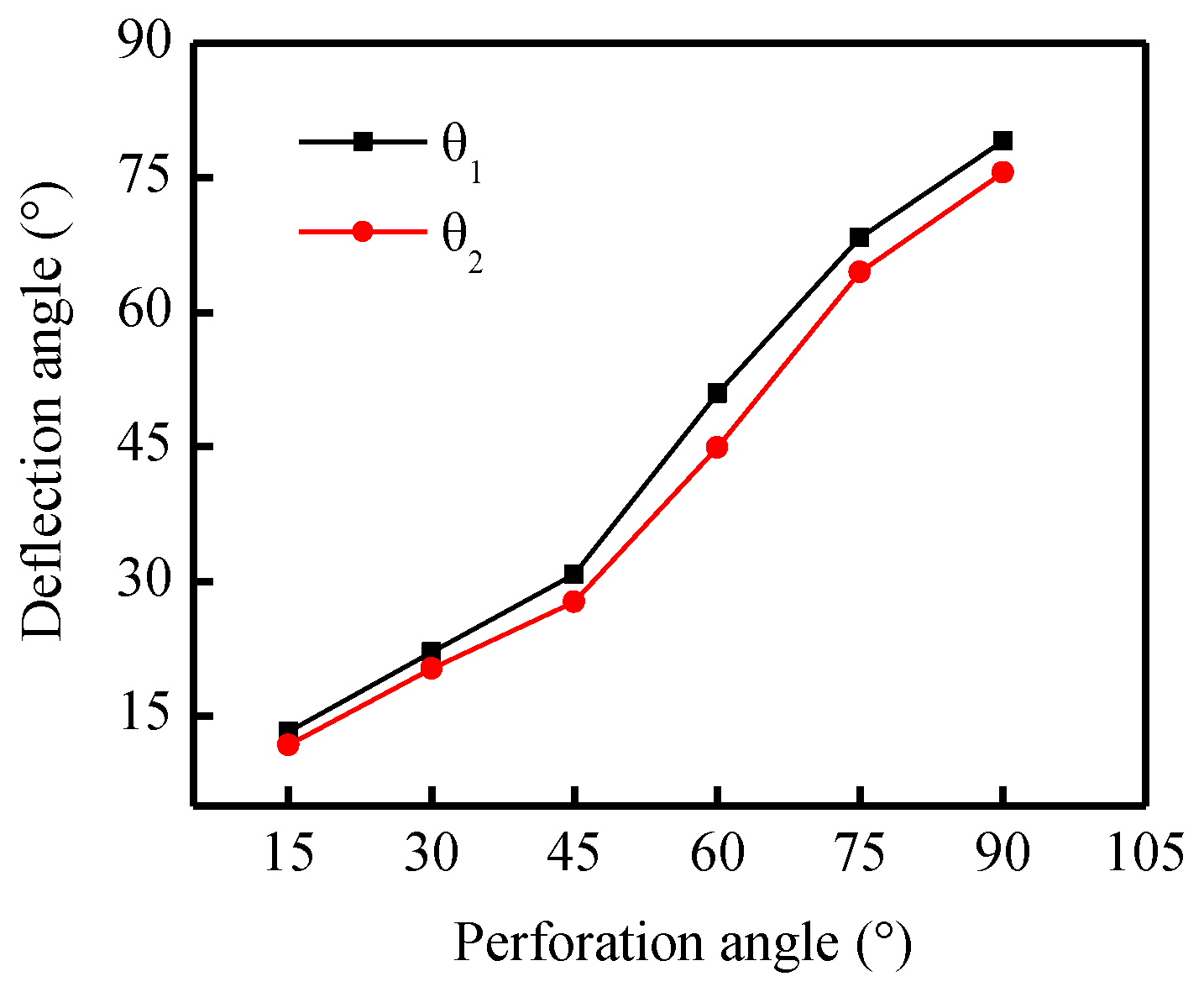
4.1. Perforation Azimuth Angle
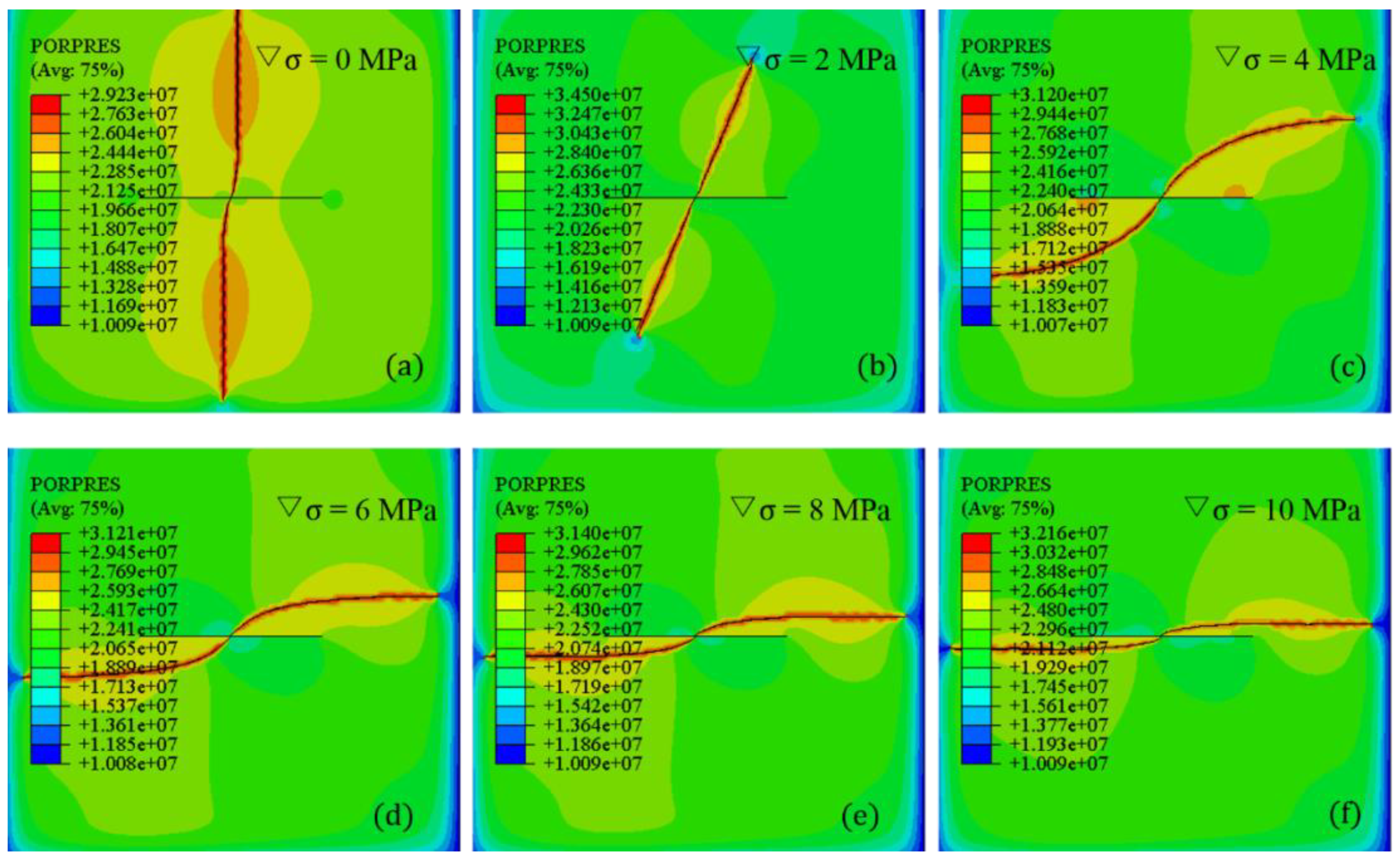
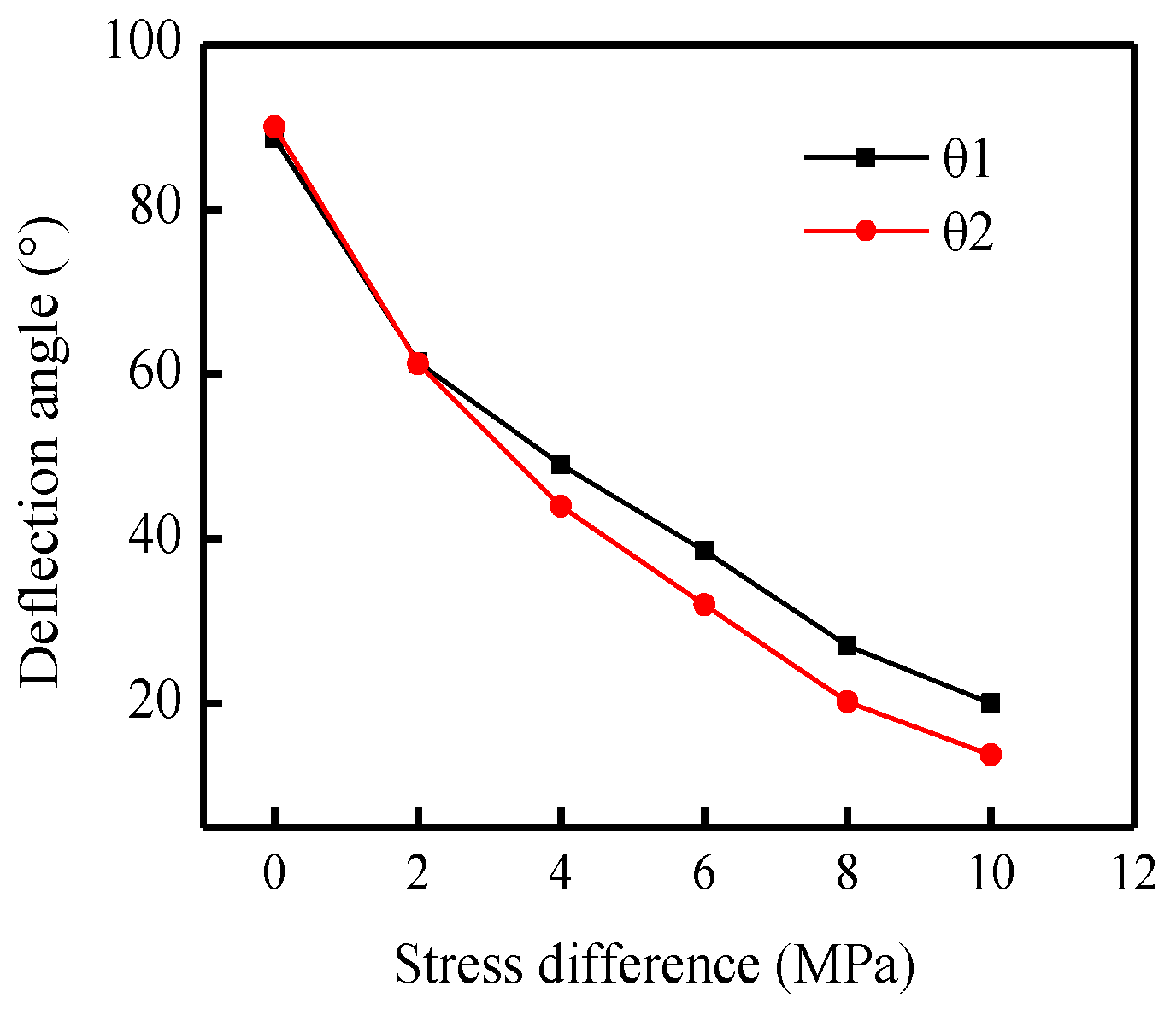
4.2. Horizontal Stress Difference
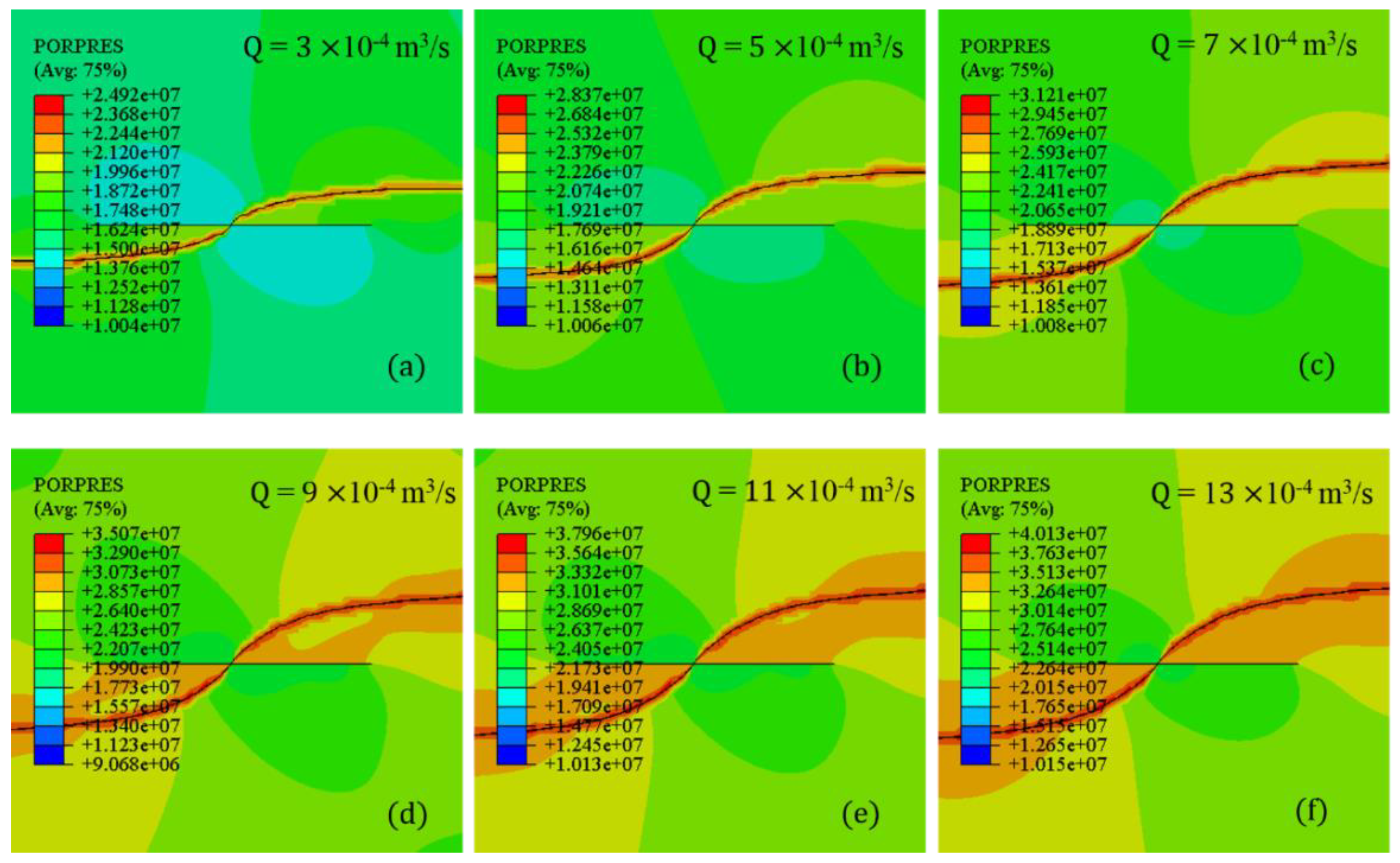
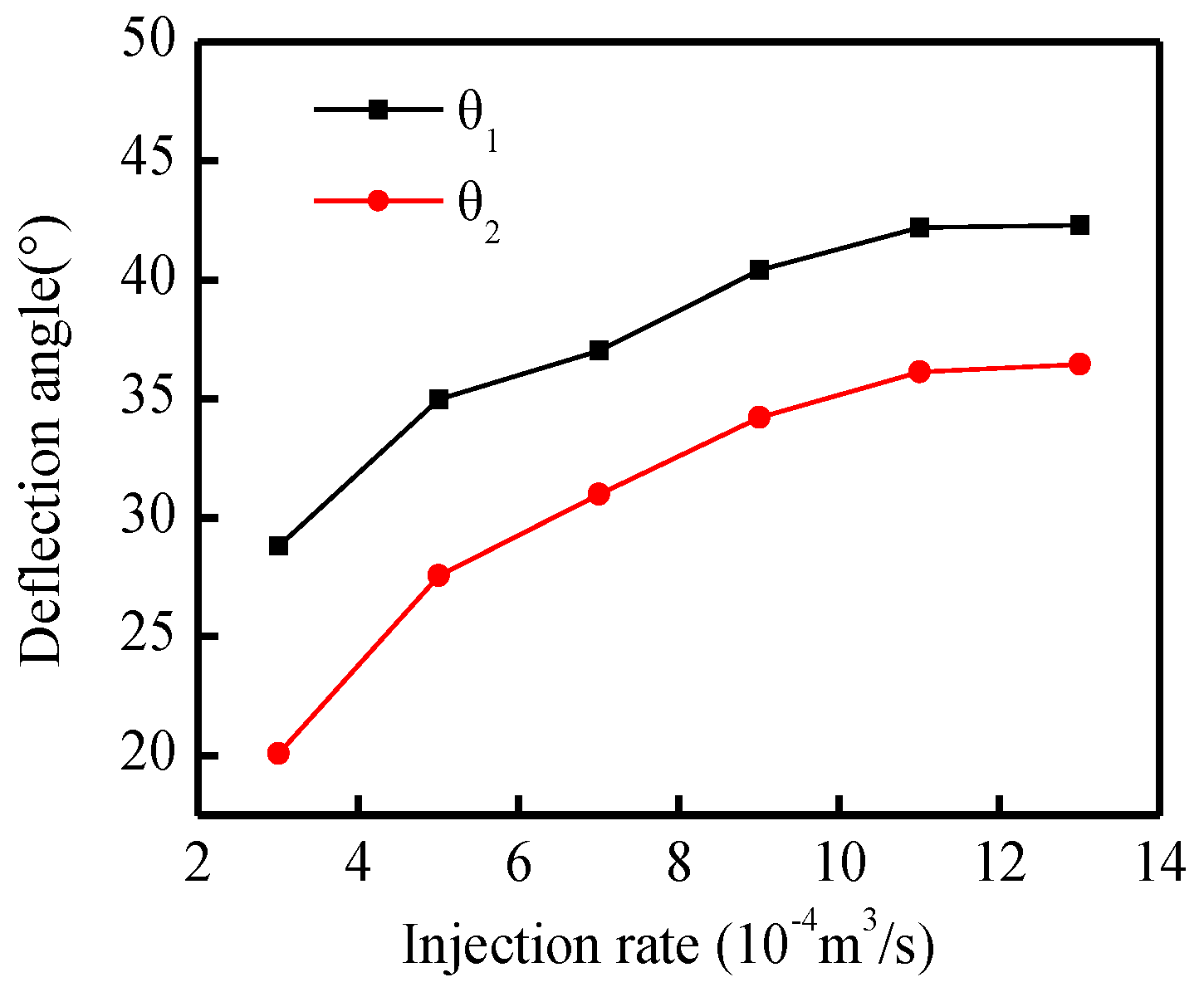
4.3. Injection Rate of the Fracturing Fluid
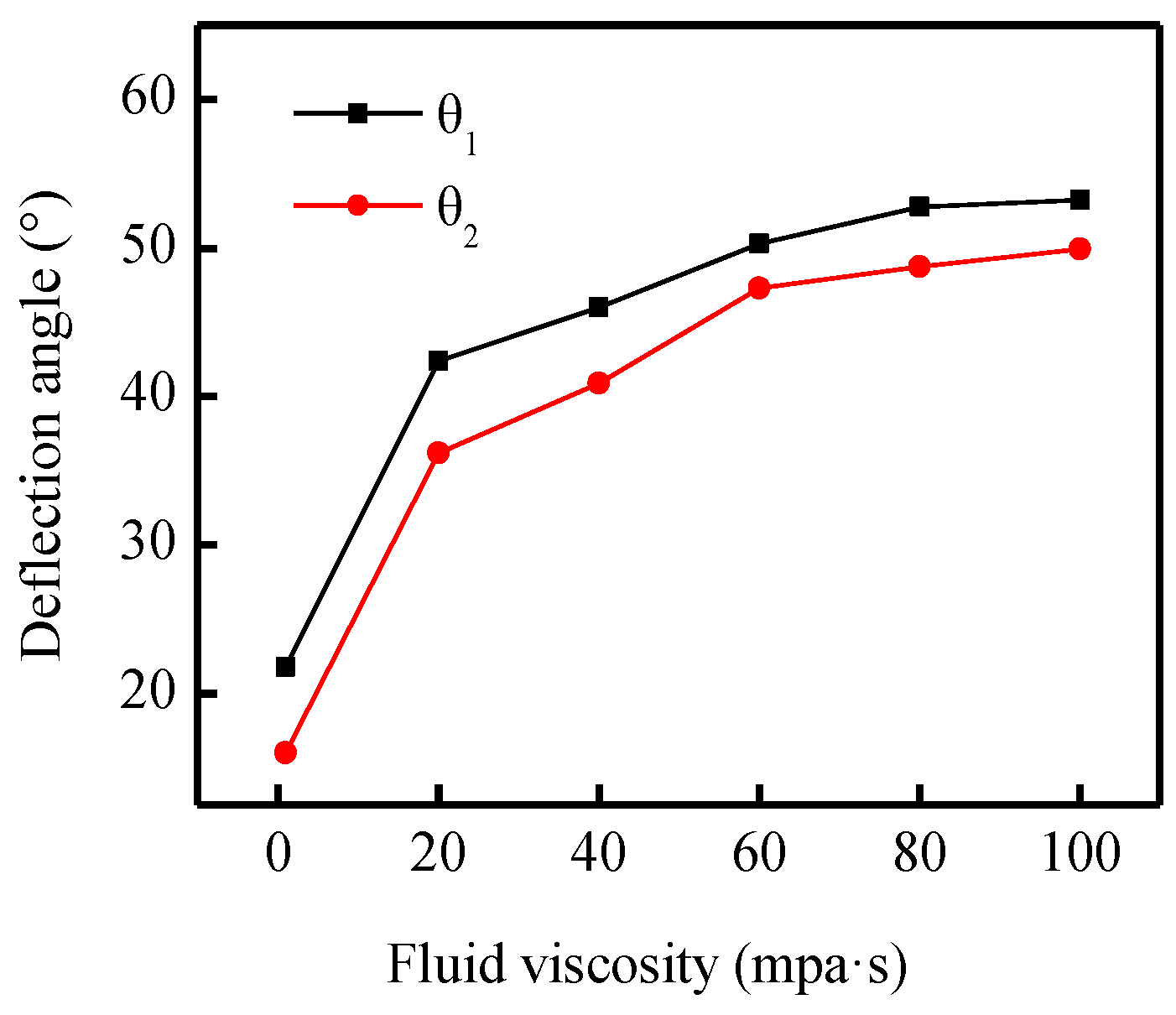
4.4. Fluid Viscosity
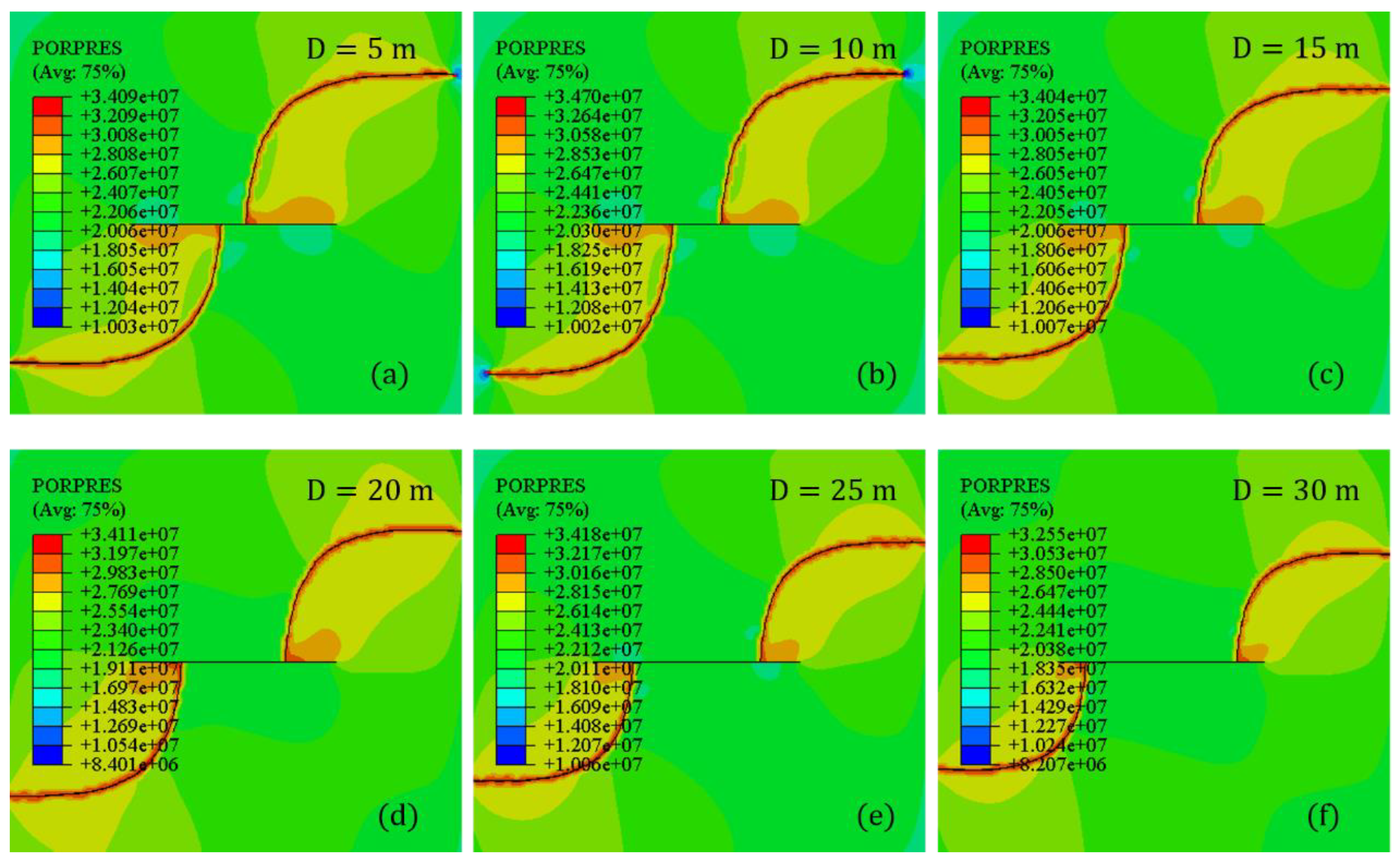
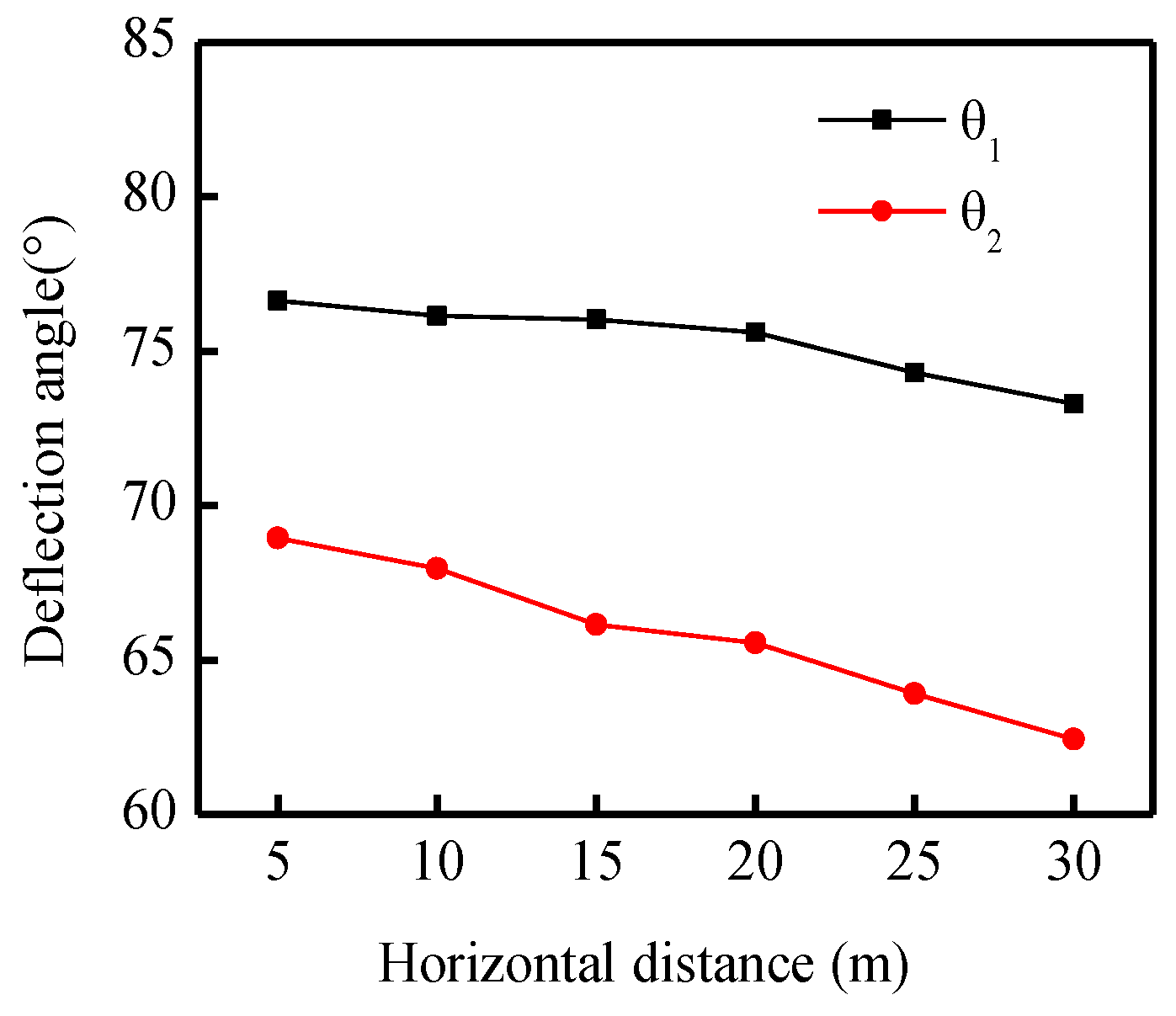
4.5. Initial Location of the Diverting Fracture
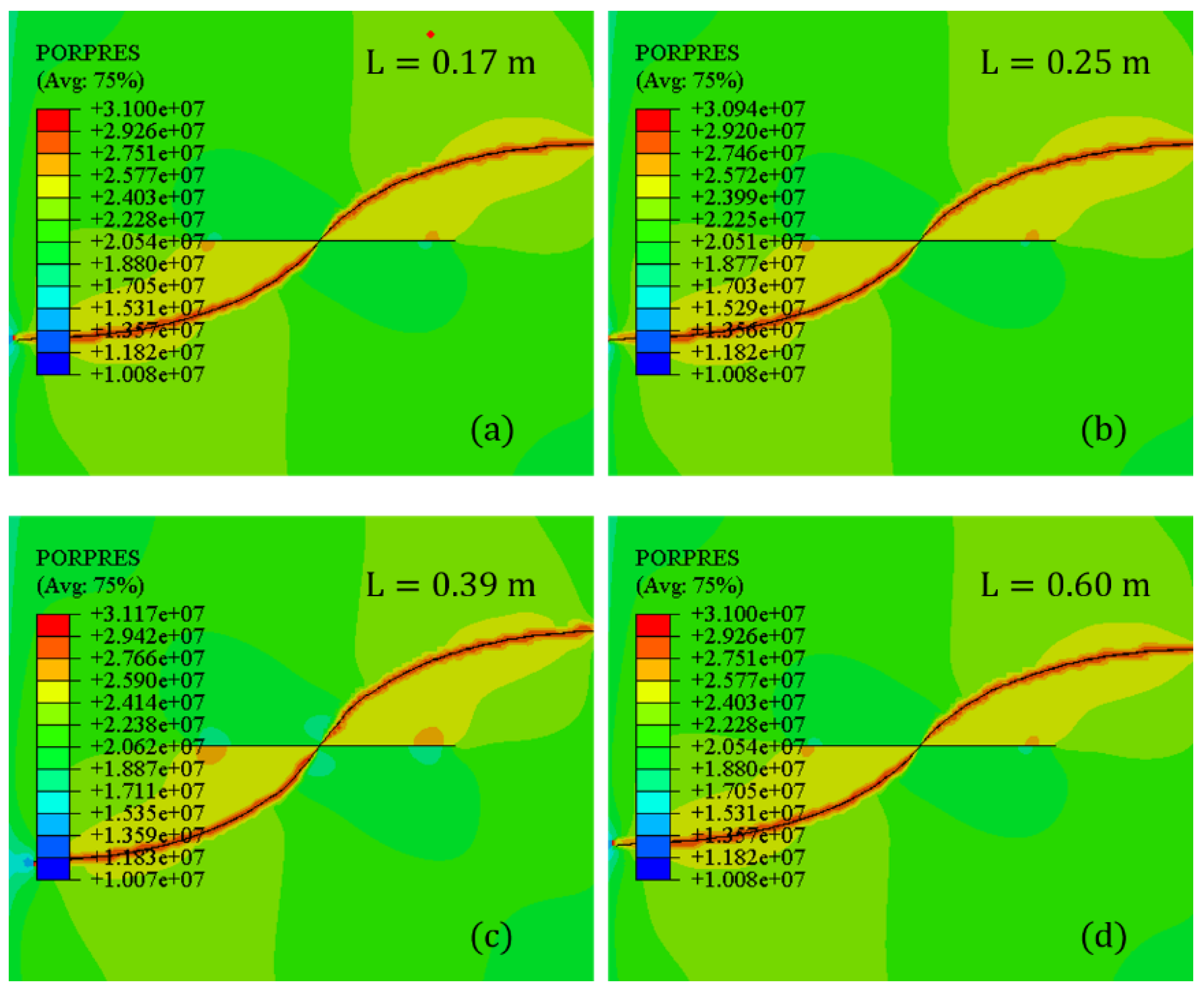
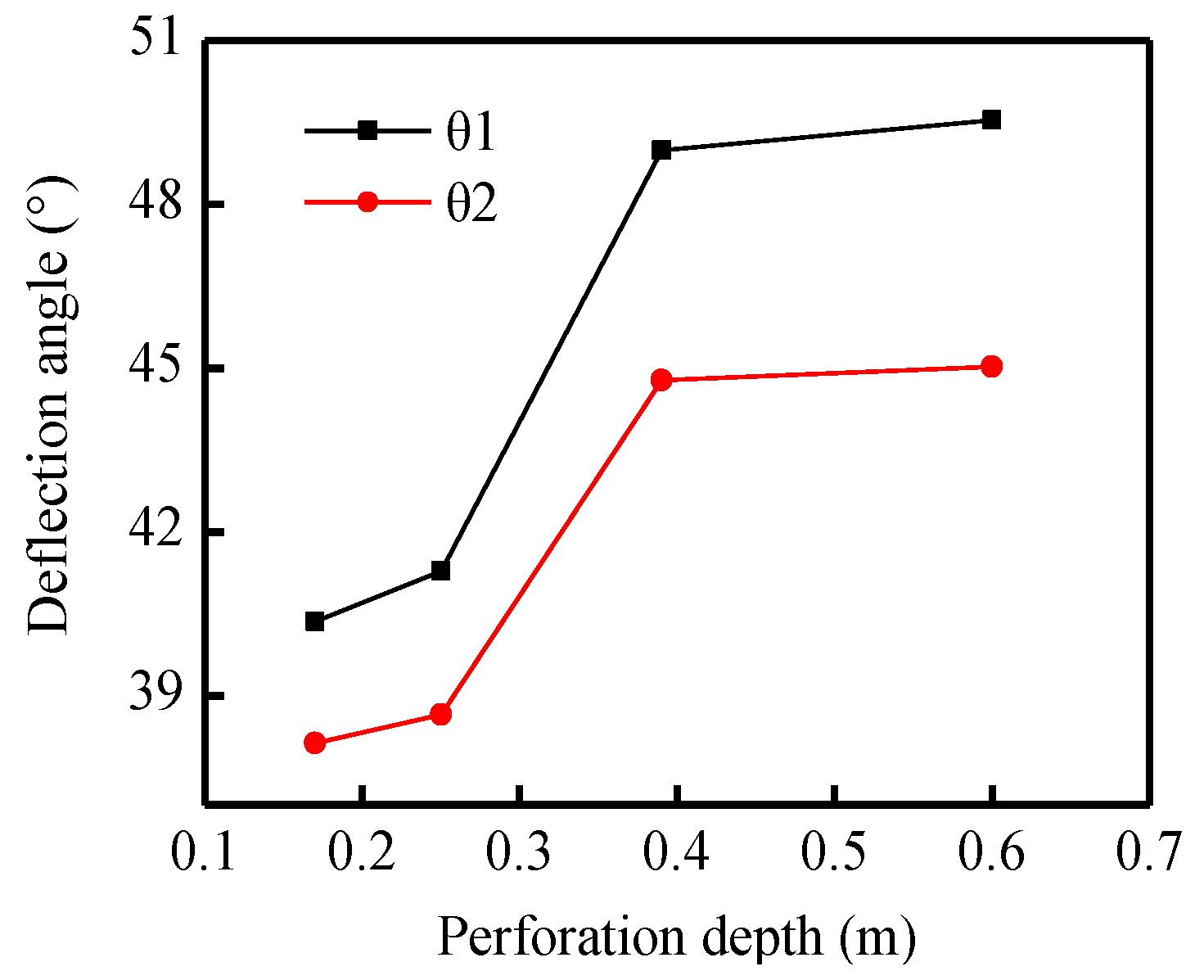
4.6. Perforation Depth
5. Conclusions
- (1)
- The existence of the first-stage fracture can significantly change the in situ stress field or redirect the direction of the stress field relative to lower horizontal stress difference. With an increase of the stress difference, the diverting fracture deflected easily to the direction of the maximum horizontal stress after initiating from the perforation. For particularly low stress difference such as ∇σ = 2 MPa, the deflection of the diverting fracture did not occur, instead it propagated along the perforation azimuth angle.
- (2)
- Perforation parameters including azimuth angle and depth can influence the deflecting geometries of the diverting fracture. Higher perforation depth and azimuth angle can result in a higher deflection angle and consequently a more curving fracture.
- (3)
- Fluid properties such as the injection rate and fluid viscosity are vital factors that affect the propagation behaviors of the diverting fracture. The deflection angle is sensitive to the change of the injection rate and fluid viscosity, thereby resulting in a more curving diverting fracture with higher values. To generate the preferred diverting fracture, higher injection rate and fluid viscosity are recommended. Furthermore, there are no additional changes of deflection angles when the injection rate and viscosity exceed the value of 11 × 10−4 m3/s and 80 mpa·s, respectively.
- (4)
- For far-field plugging and diverting, the deflecting behavior were influenced by the initial location of the diverting fracture. The location near the root of the initial fracture can enhance the stress reorientation resulting in a more curved fracture. Thus a plugging location near the root of the initial fracture is preferred.
Author Contributions
Funding
Conflicts of Interest
References
- Xu, Y.; Ezulike, O.D.; Zolfaghari, A.; Dehghanpour, H.; Virues, C. Complementary Surveillance Microseismic and Flowback Data Analysis: An Approach to Evaluate Complex Fracture Networks. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dubai, UAE, 26–28 September 2016; p. 30. [Google Scholar]
- Haggerty, R.; Fleming, S.W.; Meigs, L.C.; McKenna, S.A. Tracer tests in a fractured dolomite: 2. Analysis of mass transfer in single-well injection-withdrawal tests. Water Resour. Res. 2001, 37, 1129–1142. [Google Scholar] [CrossRef]
- Zolfaghari, A.; Dehghanpour, H.; Ghanbari, E.; Bearinger, D. Fracture Characterization Using Flowback Salt-Concentration Transient. SPE J. 2016, 21, 233–244. [Google Scholar] [CrossRef]
- Sepehri, J.; Soliman, M.Y.; Morse, S.M. Application of Extended Finite Element Method to Simulate Hydraulic Fracture Propagation from Oriented Perforations. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, The Woodlands, TX, USA, 3–5 February 2015; p. 15. [Google Scholar]
- Cafaro, D.C.; Drouven, M.G.; Grossmann, I.E. Optimization models for planning shale gas well refracture treatments. AiChE J. 2016, 62, 4297–4307. [Google Scholar] [CrossRef]
- Rezaei, A.; Bornia, G.; Rafiee, M.; Soliman, M.; Morse, S. Analysis of refracturing in horizontal wells: Insights from the poroelastic displacement discontinuity method. Int. J. Numer. Anal. Methods Geomech. 2018, 42, 1306–1327. [Google Scholar] [CrossRef]
- Deng, J.Q.; Lin, C.; Yang, Q.; Liu, Y.R.; Tao, Z.F.; Duan, H.F. Investigation of directional hydraulic fracturing based on true tri-axial experiment and finite element modeling. Comput. Geotech. 2016, 75, 28–47. [Google Scholar] [CrossRef]
- Fan, T.-G.; Zhang, G.-Q. Laboratory investigation of hydraulic fracture networks in formations with continuous orthogonal fractures. Energy 2014, 74, 164–173. [Google Scholar] [CrossRef]
- Zhou, J.; Jin, Y.; Chen, M. Experimental investigation of hydraulic fracturing in random naturally fractured blocks. Int. J. Rock Mech. Min. Sci. 2010, 47, 1193–1199. [Google Scholar] [CrossRef]
- Zou, Y.; Zhang, S.; Zhou, T.; Zhou, X.; Guo, T. Experimental Investigation into Hydraulic Fracture Network Propagation in Gas Shales Using CT Scanning Technology. Rock Mech. Rock Eng. 2015, 49, 33–45. [Google Scholar] [CrossRef]
- Zhou, J.; Chen, M.; Jin, Y.; Zhang, G.-Q. Analysis of fracture propagation behavior and fracture geometry using a tri-axial fracturing system in naturally fractured reservoirs. Int. J. Rock Mech. Min. Sci. 2008, 45, 1143–1152. [Google Scholar] [CrossRef]
- Weng, X. Modeling of complex hydraulic fractures in naturally fractured formation. J. Unconvent. Oil Gas Resour. 2015, 9, 114–135. [Google Scholar] [CrossRef]
- Zeng, Q.; Yao, J. Numerical simulation of fracture network generation in naturally fractured reservoirs. J. Nat. Gas Sci. Eng. 2016, 30, 430–443. [Google Scholar] [CrossRef]
- Zhang, X.; Jeffrey, R.G.; Wu, B. Mechanics of edge crack growth under transient pressure and temperature conditions. Int. J. Solids Struct. 2015, 69–70, 11–22. [Google Scholar] [CrossRef]
- Meyer, B.R.; Bazan, L.W. A Discrete Fracture Network Model for Hydraulically Induced Fractures—Theory, Parametric and Case Studies. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, The Woodlands, TX, USA, 24–26 January 2011; p. 36. [Google Scholar]
- Yan, C.; Zheng, H. FDEM-flow3D: A 3D hydro-mechanical coupled model considering the pore seepage of rock matrix for simulating three-dimensional hydraulic fracturing. Comput. Geotech. 2017, 81, 212–228. [Google Scholar] [CrossRef]
- Carrier, B.; Granet, S. Numerical modeling of hydraulic fracture problem in permeable medium using cohesive zone model. Eng. Fract. Mech. 2012, 79, 312–328. [Google Scholar] [CrossRef]
- Sarris, E.; Papanastasiou, P. The influence of the cohesive process zone in hydraulic fracturing modelling. Int. J. Fract. 2011, 167, 33–45. [Google Scholar] [CrossRef]
- Moës, N.; Dolbow, J.; Belytschko, T. A finite element method for crack growth without remeshing. Int. J. Numer. Methods Eng. 1999, 46, 131–150. [Google Scholar] [CrossRef]
- Belytschko, T.; Black, T. Elastic crack growth in finite elements with minimal remeshing. Int. J. Numer. Methods Eng. 1999, 45, 601–620. [Google Scholar] [CrossRef]
- Dahi Taleghani, A.; Olson, J.E. How Natural Fractures Could Affect Hydraulic-Fracture Geometry. SPE J. 2013, 19, 161–171. [Google Scholar] [CrossRef]
- Qi, M.; Li, M.; Guo, T.; Liu, C.; Gao, S.; Tang, S. Influence of Oriented Perforation Design on Refracture Reorientation: Simulation and Experiment. J. Energy Resour. Technol. 2018, 140, 082903. [Google Scholar] [CrossRef]
- Wang, B.; Zhou, F.; Wang, D.; Liang, T.; Yuan, L.; Hu, J. Numerical simulation on near-wellbore temporary plugging and diverting during refracturing using XFEM-Based CZM. J. Nat. Gas Sci. Eng. 2018, 55, 368–381. [Google Scholar] [CrossRef]
- Gordeliy, E.; Abbas, S.; Prioul, R. Modeling of Near-Wellbore Fracture Reorientation Using a Fluid-Coupled 2D XFEM Algorithm. In Proceedings of the 50th U.S. Rock Mechanics/Geomechanics Symposium, Houston, TX, USA, 26–29 June 2016; p. 9. [Google Scholar]
- Taleghani, A.D.; Gonzalez-Chavez, M.; Hao, H.; Asala, H. Numerical simulation of hydraulic fracture propagation in naturally fractured formations using the cohesive zone model. J. Petrol. Sci. Eng. 2018, 165, 42–57. [Google Scholar] [CrossRef]
- Li, J.; Xiao, W.; Hao, G.; Dong, S.; Hua, W.; Li, X. Comparison of Different Hydraulic Fracturing Scenarios in Horizontal Wells Using XFEM Based on the Cohesive Zone Method. Energies 2019, 12, 1232. [Google Scholar] [CrossRef]
- Vahab, M.; Akhondzadeh, S.; Khoei, A.R.; Khalili, N. An X-FEM investigation of hydro-fracture evolution in naturally-layered domains. Eng. Fract. Mech. 2018, 191, 187–204. [Google Scholar] [CrossRef]
- Gong, D.G.; Qu, Z.Q.; Li, J.X.; Qu, G.Z.; Cao, Y.C.; Guo, T.K. Extended finite element simulation of hydraulic fracture based on ABAQUS platform. J. Petrol. Sci. Eng. 2016, 27, 1512–1520. [Google Scholar]
- Wang, X.; Liu, C.; Wang, H.; Liu, H.; Wu, H. Comparison of consecutive and alternate hydraulic fracturing in horizontal wells using XFEM-based cohesive zone method. J. Petrol. Sci. Eng. 2016, 143, 14–25. [Google Scholar] [CrossRef]
- Wang, X.L.; Shi, F.; Liu, C.; Lu, D.T.; Liu, H.; Wu, H.A. Extended finite element simulation of fracture network propagation in formation containing frictional and cemented natural fractures. J. Nat. Gas Sci. Eng. 2017, 50, 309–324. [Google Scholar] [CrossRef]
- Shi, F.; Wang, X.; Liu, C.; Liu, H.; Wu, H. An XFEM-based method with reduction technique for modeling hydraulic fracture propagation in formations containing frictional natural fractures. Eng. Fract. Mech. 2017, 173, 64–90. [Google Scholar] [CrossRef]
- Li, J.; Dong, S.; Hua, W.; Li, X.; Pan, X. Numerical Investigation of Hydraulic Fracture Propagation Based on Cohesive Zone Model in Naturally Fractured Formations. Processes 2019, 7, 28. [Google Scholar] [CrossRef]
- Nguyen, V.P.; Lian, H.; Rabczuk, T.; Bordas, S. Modelling hydraulic fractures in porous media using flow cohesive interface elements. Eng. Geol. 2017, 225, 68–82. [Google Scholar] [CrossRef]
- Yao, Y. Linear Elastic and Cohesive Fracture Analysis to Model Hydraulic Fracture in Brittle and Ductile Rocks. Rock Mech. Rock Eng. 2012, 45, 375–387. [Google Scholar] [CrossRef]
- Guo, J.; Zhao, X.; Zhu, H.; Zhang, X.; Pan, R. Numerical simulation of interaction of hydraulic fracture and natural fracture based on the cohesive zone finite element method. J. Nat. Gas Sci. Eng. 2015, 25, 180–188. [Google Scholar] [CrossRef]
- Haddad, M.; Sepehrnoori, K. Simulation of Multiple-Stage Fracturing in Quasibrittle Shale Formations Using Pore Pressure Cohesive Zone Model. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Denver, CO, USA, 25–27 August 2014; p. 16. [Google Scholar]
- Liu, C.; Wang, X.; Deng, D.; Zhang, Y.; Zhang, Y.; Wu, H.; Liu, H. Optimal spacing of sequential and simultaneous fracturing in horizontal well. J. Nat. Gas Sci. Eng. 2016, 29, 329–336. [Google Scholar] [CrossRef]
- Haddad, M.; Sepehrnoori, K. Simulation of hydraulic fracturing in quasi-brittle shale formations using characterized cohesive layer: Stimulation controlling factors. J. Unconvent. Oil Gas Resour. 2015, 9, 65–83. [Google Scholar] [CrossRef]
- Qu, Z.; Tian, Y.; Li, J.; Guo, T.; Li, X.; Liu, X. Numerical simulation study on fracture extension and morphology of multi-cluster staged fracturing for horizontal wells. J. China Univ. Petrol. 2017, 41, 102–109. [Google Scholar]
- Wang, D.; Zhou, F.; Ge, H.; Shi, Y.; Yi, X.; Xiong, C.; Liu, X.; Wu, Y.; Li, Y. An experimental study on the mechanism of degradable fiber-assisted diverting fracturing and its influencing factors. J. Nat. Gas Sci. Eng. 2015, 27, 260–273. [Google Scholar] [CrossRef]
- Feng, Y.; Gray, K.E. Modeling of curving hydraulic fracture propagation from a wellbore in a poroelastic medium. J. Nat. Gas Sci. Eng. 2018, 53, 83–93. [Google Scholar] [CrossRef]
- Zhang, R.; Hou, B.; Shan, Q.; Tan, P.; Wu, Y.; Gao, J.; Guo, X. Hydraulic fracturing initiation and near-wellbore nonplanar propagation from horizontal perforated boreholes in tight formation. J. Nat. Gas Sci. Eng. 2018, 55, 337–349. [Google Scholar] [CrossRef]
- Yin, J.; Xie, J.; Datta-Gupta, A.; Hill, A.D. Improved characterization and performance prediction of shale gas wells by integrating stimulated reservoir volume and dynamic production data. J. Petrol. Sci. Eng. 2015, 127, 124–136. [Google Scholar] [CrossRef]
- Zhao, J.; Chen, X.; Li, Y.; Fu, B. Simulation of simultaneous propagation of multiple hydraulic fractures in horizontal wells. J. Petrol. Sci. Eng. 2016, 147, 788–800. [Google Scholar] [CrossRef]
- Wang, D.; Zhou, F.; Ding, W.; Ge, H.; Jia, X.; Shi, Y.; Wang, X.; Yan, X. A numerical simulation study of fracture reorientation with a degradable fiber-diverting agent. J. Nat. Gas Sci. Eng. 2015, 25, 215–225. [Google Scholar] [CrossRef]
- Feng, Y.; Gray, K.E. Parameters controlling pressure and fracture behaviors in field injectivity tests: A numerical investigation using coupled flow and geomechanics model. Comput. Geotech. 2017, 87, 49–61. [Google Scholar] [CrossRef]
- Guo, T.; Zhang, S.; Zou, Y.; Xiao, B. Numerical simulation of hydraulic fracture propagation in shale gas reservoir. J. Nat. Gas Sci. Eng. 2015, 26, 847–856. [Google Scholar] [CrossRef]
- Guo, T.; Zhang, S.; Qu, Z.; Zhou, T.; Xiao, Y.; Gao, J. Experimental study of hydraulic fracturing for shale by stimulated reservoir volume. Fuel 2014, 128, 373–380. [Google Scholar] [CrossRef]
- Beugelsdijk, L.J.L.; de Pater, C.J.; Sato, K. Experimental Hydraulic Fracture Propagation in a Multi-Fractured Medium. In Proceedings of the SPE Asia Pacific Conference on Integrated Modelling for Asset Management, Yokohama, Japan, 25–26 April 2000; p. 8. [Google Scholar]
- Olson, J.E. Multi-fracture propagation modeling: Applications to hydraulic fracturing in shales and tight gas sands. In Proceedings of the 42nd U.S. Rock Mechanics Symposium (USRMS), San Francisco, CA, USA, 29 June–2 July 2008; p. 8. [Google Scholar]
- Zhang, X.; Jeffrey, R.G.; Bunger, A.P.; Thiercelin, M. Initiation and growth of a hydraulic fracture from a circular wellbore. Int. J. Rock Mech. Min. Sci. 2011, 48, 984–995. [Google Scholar] [CrossRef]
- Yu, J.; Shen, F.; Gu, Q. Influence of perforation parameters on hydraulic fracturing of fracture pressure in horizontal well. Petrol. Geol. Recovery Effic. 2011, 18, 105–106. [Google Scholar] [CrossRef]



| Parameters | Values |
|---|---|
| Model dimension | 200 × 200 m |
| Wellbore radius | 10 cm |
| Perforation length | 0.1–0.6 m |
| Perforation angle, θ | 0°–90° |
| Maximum horizontal stress, σH | 15–25 MPa |
| Minimum horizontal stress, σh | 15 MPa |
| Vertical stress, σv | 25 MPa |
| Young’s modulus, E | 12.79 GPa |
| Poisson’s ratio, υ | 0.25 |
| Tensile strength, σt | 1.82 MPa |
| Fracture energy, Gc | 28 N/mm |
| Permeability, k | 10 mD |
| Leak-off coefficient, c | 1 × 10−14 m/(pa·s) |
| Porosity, φ | 0.25 |
| Fluid viscosity, μ | 1–100 mpa·s |
| Injection rate, Q | 3 × 10−4–13 × 10−4 m3/s |
| Initial pore pressure, P | 10 MPa |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Dong, S.; Hua, W.; Yang, Y.; Li, X. Numerical Simulation on Deflecting Hydraulic Fracture with Refracturing Using Extended Finite Element Method. Energies 2019, 12, 2044. https://doi.org/10.3390/en12112044
Li J, Dong S, Hua W, Yang Y, Li X. Numerical Simulation on Deflecting Hydraulic Fracture with Refracturing Using Extended Finite Element Method. Energies. 2019; 12(11):2044. https://doi.org/10.3390/en12112044
Chicago/Turabian StyleLi, Jianxiong, Shiming Dong, Wen Hua, Yang Yang, and Xiaolong Li. 2019. "Numerical Simulation on Deflecting Hydraulic Fracture with Refracturing Using Extended Finite Element Method" Energies 12, no. 11: 2044. https://doi.org/10.3390/en12112044
APA StyleLi, J., Dong, S., Hua, W., Yang, Y., & Li, X. (2019). Numerical Simulation on Deflecting Hydraulic Fracture with Refracturing Using Extended Finite Element Method. Energies, 12(11), 2044. https://doi.org/10.3390/en12112044





