Time-Resolved Temperature Map Prediction of Concentration Photovoltaics Systems by Means of Coupled Ray Tracing Flux Analysis and Thermal Quadrupoles Modelling
Abstract
1. Introduction
2. Materials and Methods
2.1. Locations, Instruments, Software and Methodology
- (1)
- First, a Fresnel Lens model has been implemented by script, based on the software Tonatiuh (Section 2.2 and Section 2.2.1).
- (2)
- Design parameters from a commercial lens (aperture, transmissivity and prism pitch) have been introduced to the Tonatiuh model.
- (3)
- The commercial Fresnel lens has been experimentally characterized (focal length and spot spatial profile at a position below focal point, see Section 2.2.2).
- (4)
- Inspired by the commercial lens aperture, focal length and measured spot profile, the Tonatiuh Fresnel lens prisms angles were adjusted till they deliver the same focal length as the commercial lens.
- (5)
- Subsequently, both experimental and Tonatiuh spots were compared at a position below the focal point (see Section 3.1).
- (6)
- The so-obtained synthetic spots, inspired by the commercial Fresnel lens, were combined with DNI time series for the highest irradiance day of 2015, thus generating a synthetic irradiance data set, resolved in space and time, and inspired by a real Fresnel lens specifications and real irradiance data (see Section 2.2.3).
- (7)
- The irradiance data set is then introduced to the thermal quadrupole model described in Section 2.3. Temperature maps for three solar cells with different sizes are computed and a parametric study is executed (Section 3.2.1), it allows scaling the concentration ratio and preserving the operation temperature within safe ranges.
- (8)
- Finally, the possibilities of this work approach concerning the assessment of thermal gradients induced by fast tracking point perturbations are discussed in Section 3.2.3.
2.2. Irradiance Map Definition
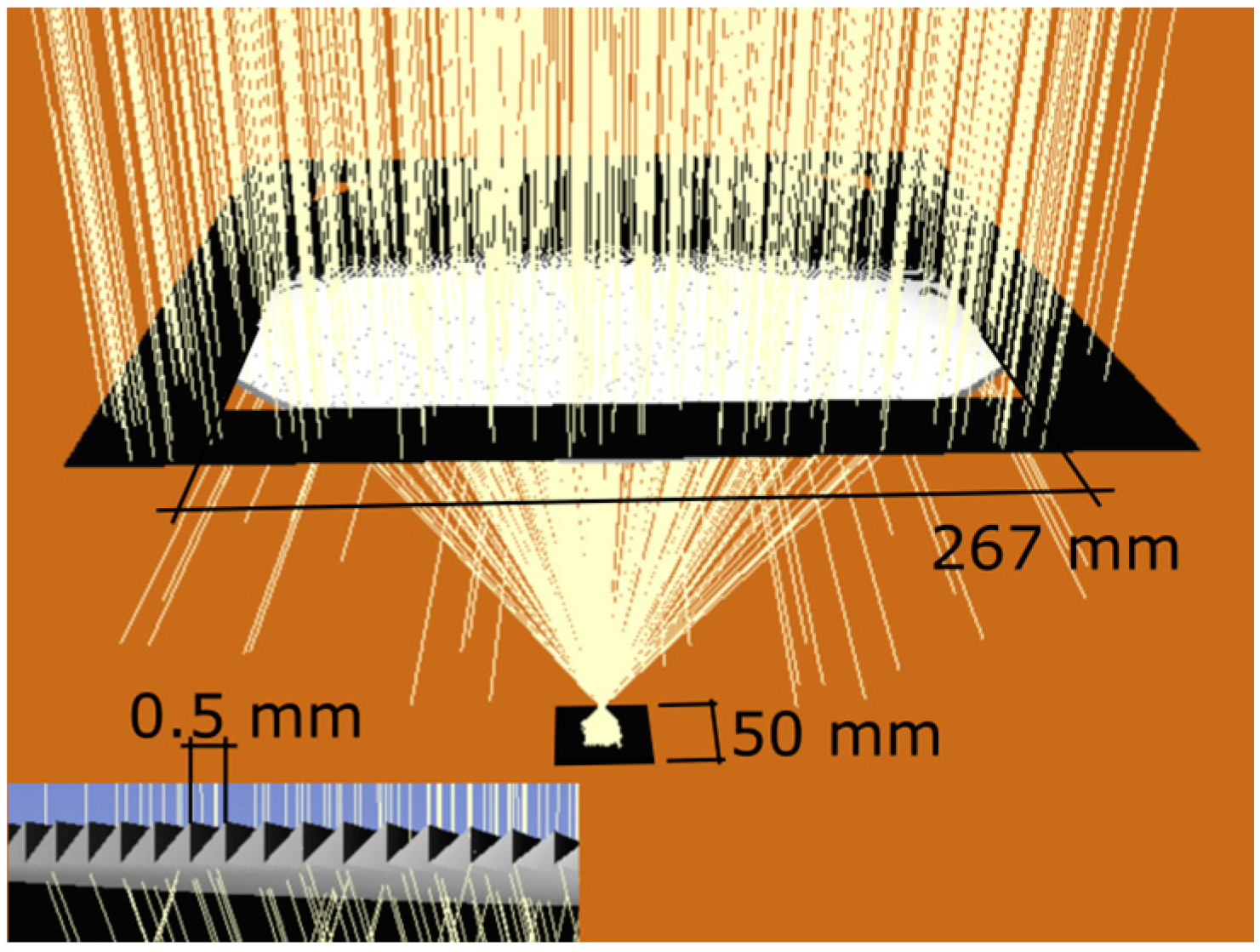
2.2.1. Implementing Fresnel Lenses with Tonatiuh
2.2.2. Characterising the Fresnel Lens That Inspires Tonatiuh Script: Experimental Design
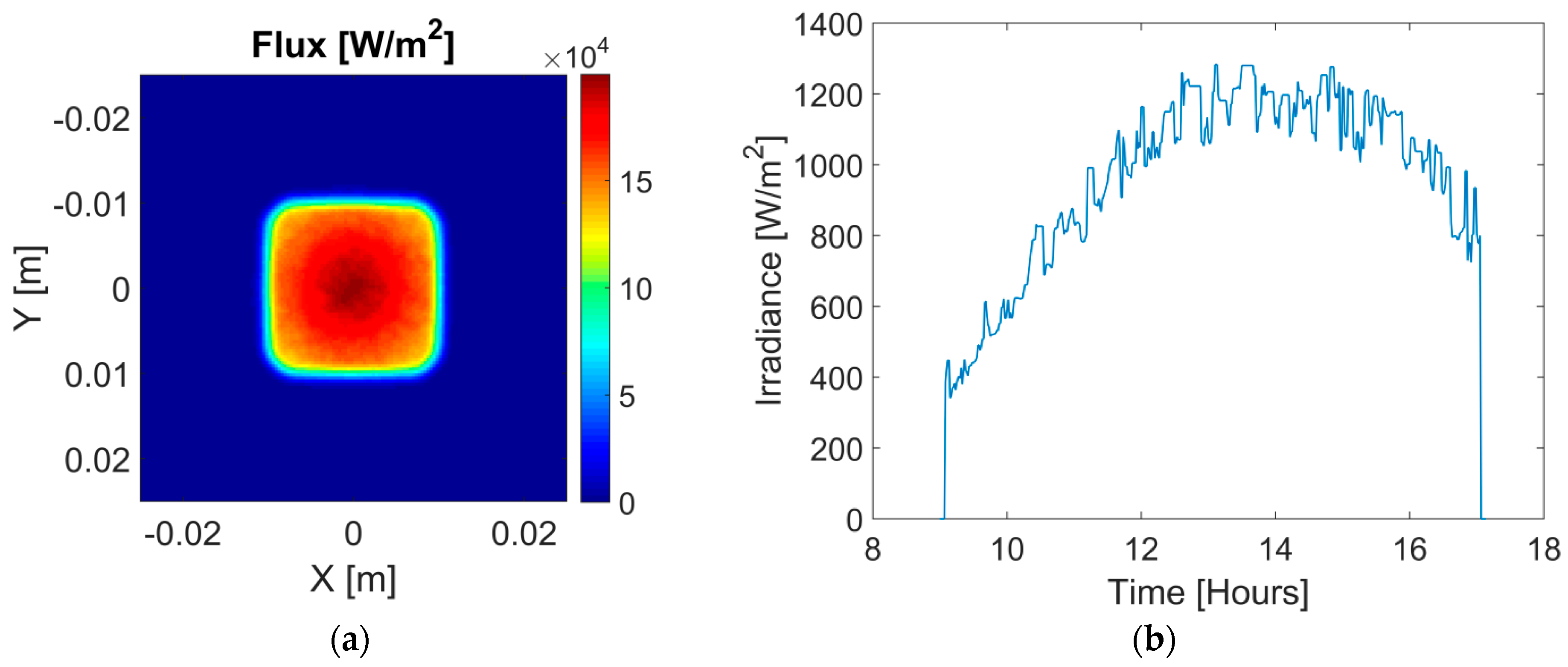
2.2.3. Combining Flux Maps Modeled through Tonatiuh with Synthetic Ambient Irradiance Series
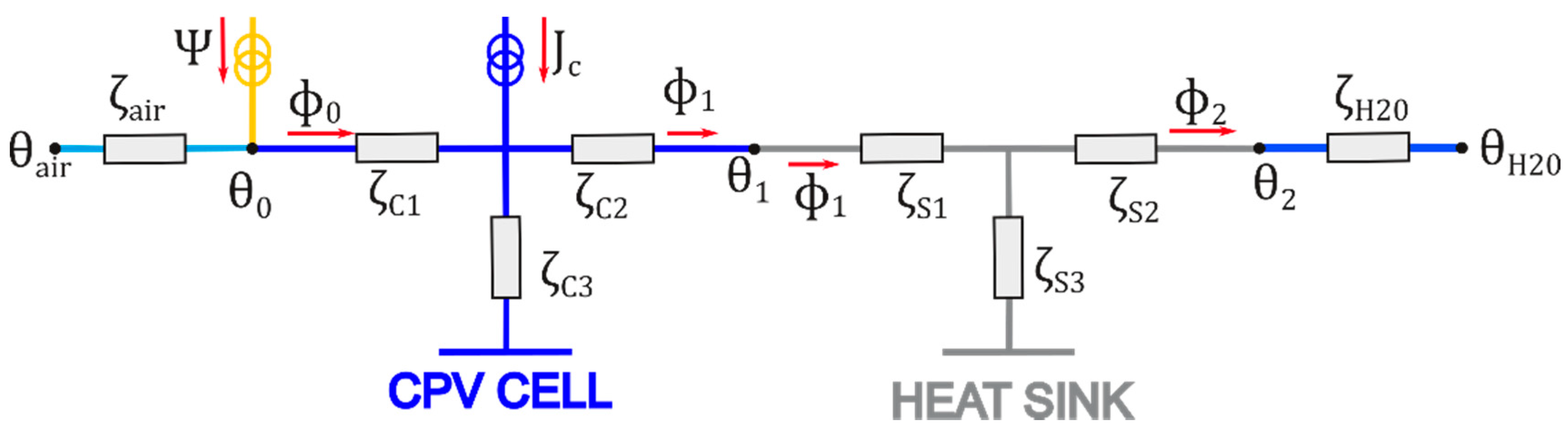
2.3. Thermal Quadrupoles Model
3. Coupling Ray Tracing Analysis + Thermal Quadrupoles Model: Results
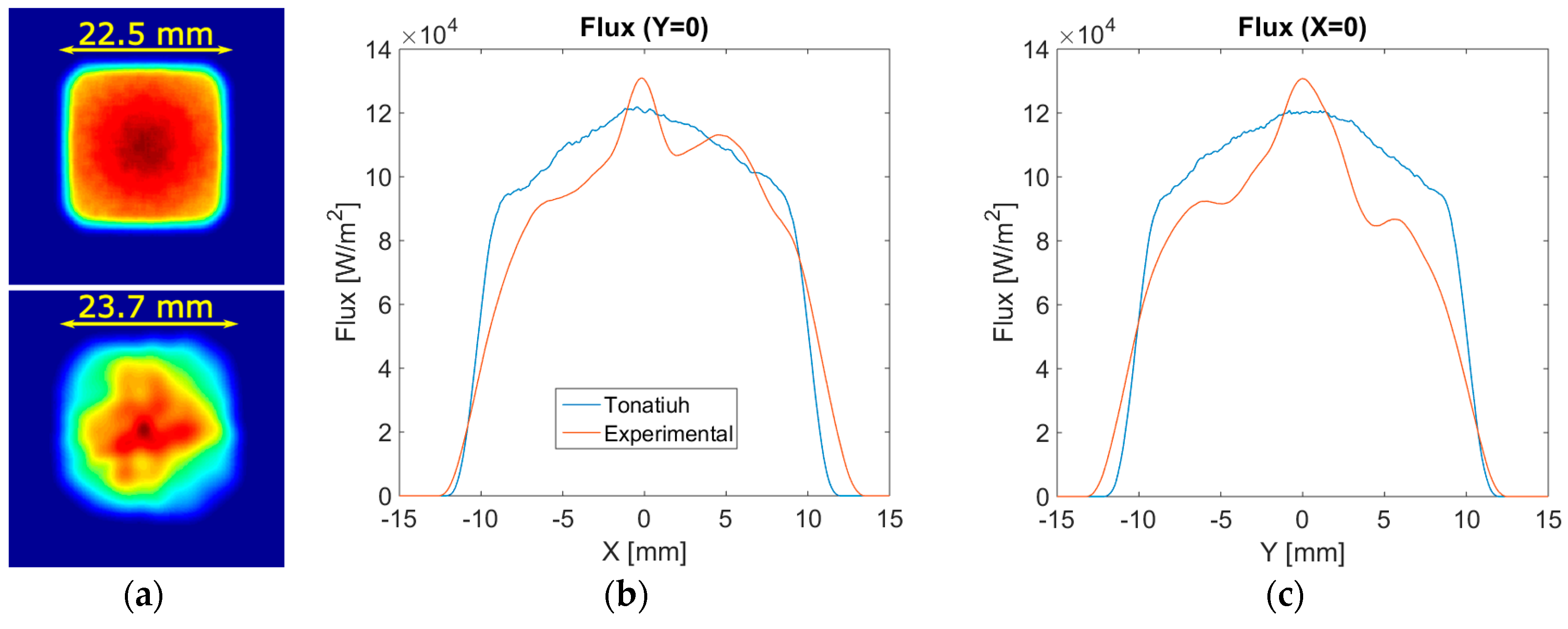
3.1. Tonatiuh Fresnel Lens Model: Comparison with Commercial Lens Spot
3.2. Time-Resolved Temperature Mapping Simulation Results
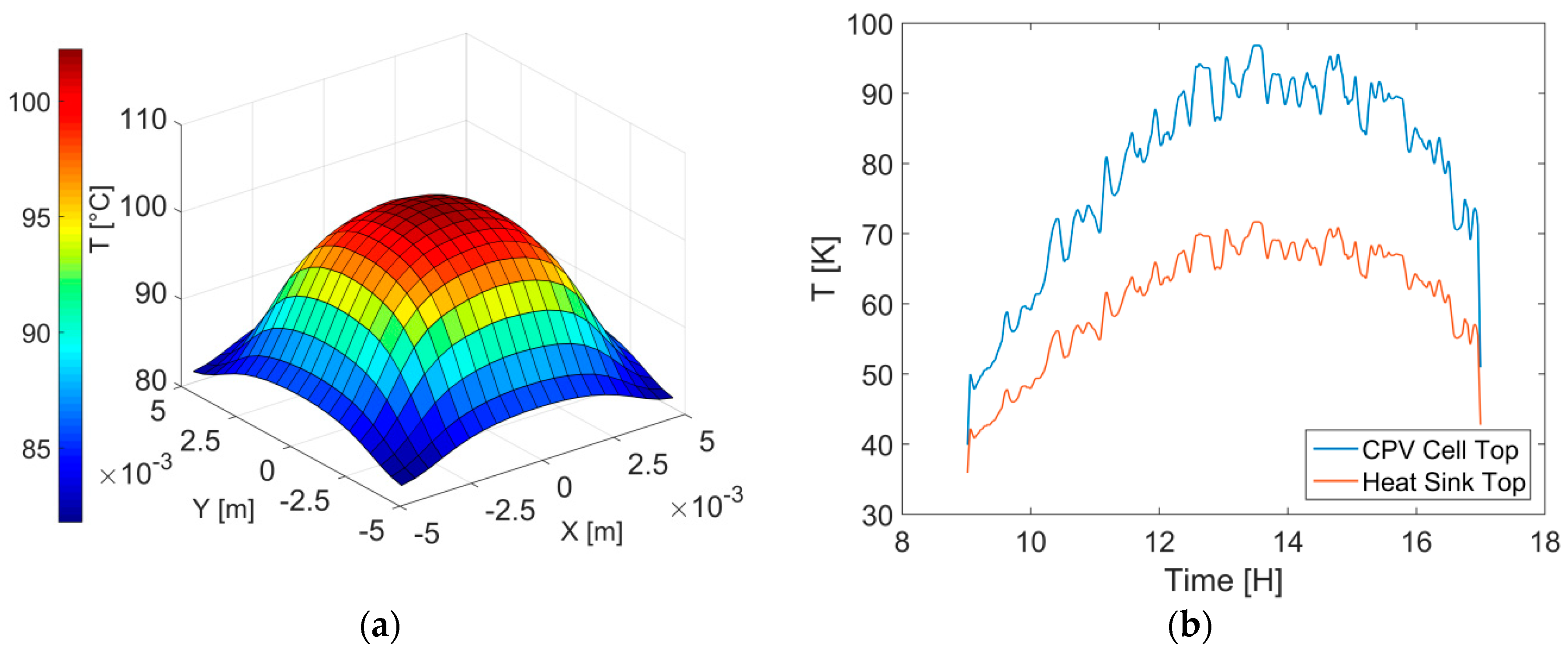
3.2.1. Uneven Irradiance Profiles and High Temperature Gradients
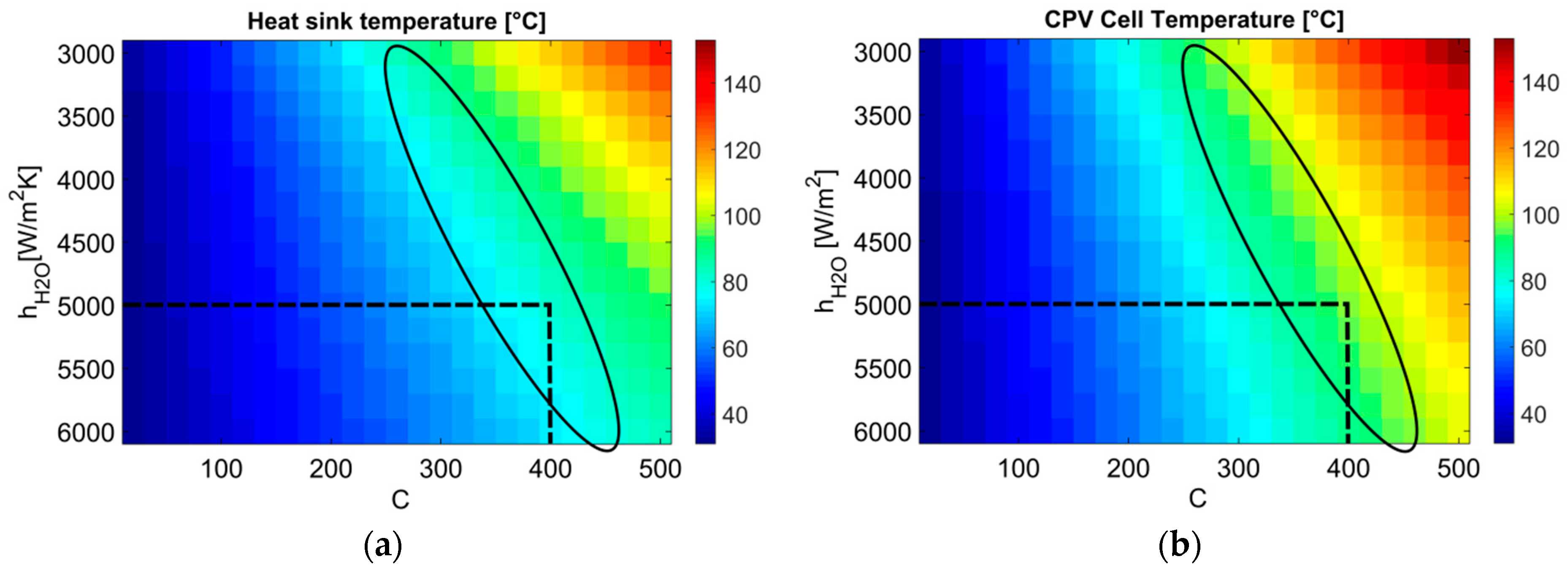
3.2.2. Concentrator Scaling and Operation Temperature Optimization
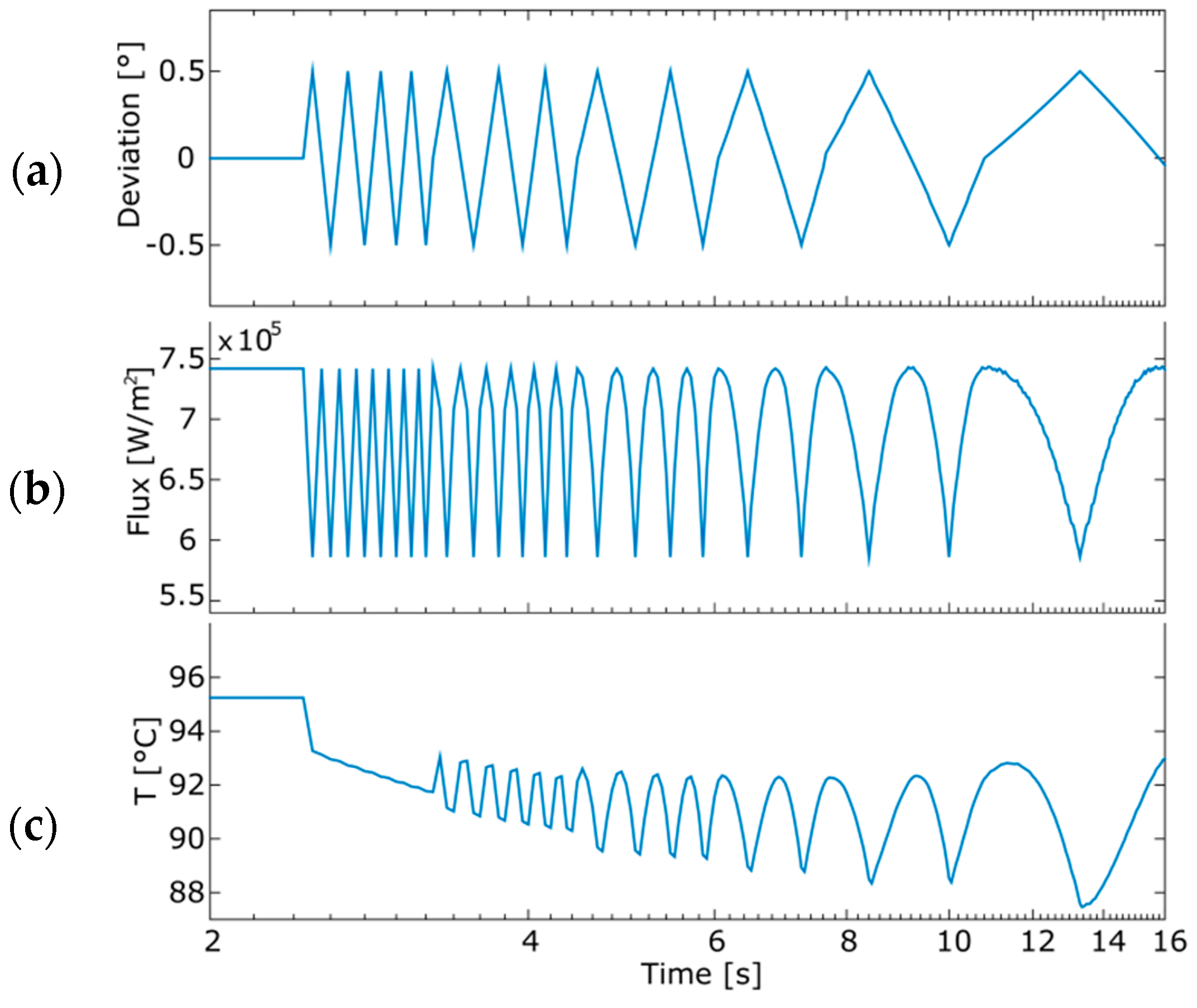
3.2.3. Predicting Temperature Variations Due to Tracking Point Perturbations
3.3. Computing Time
4. Discussion
5. Conclusions and Perspectives
Potential for Reliability Studies
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Appendix A. Full Mathematical Development of Thermal Quadrupole Model
A.1. Flux Excitation
A.2. Thermal—Electric Coupling

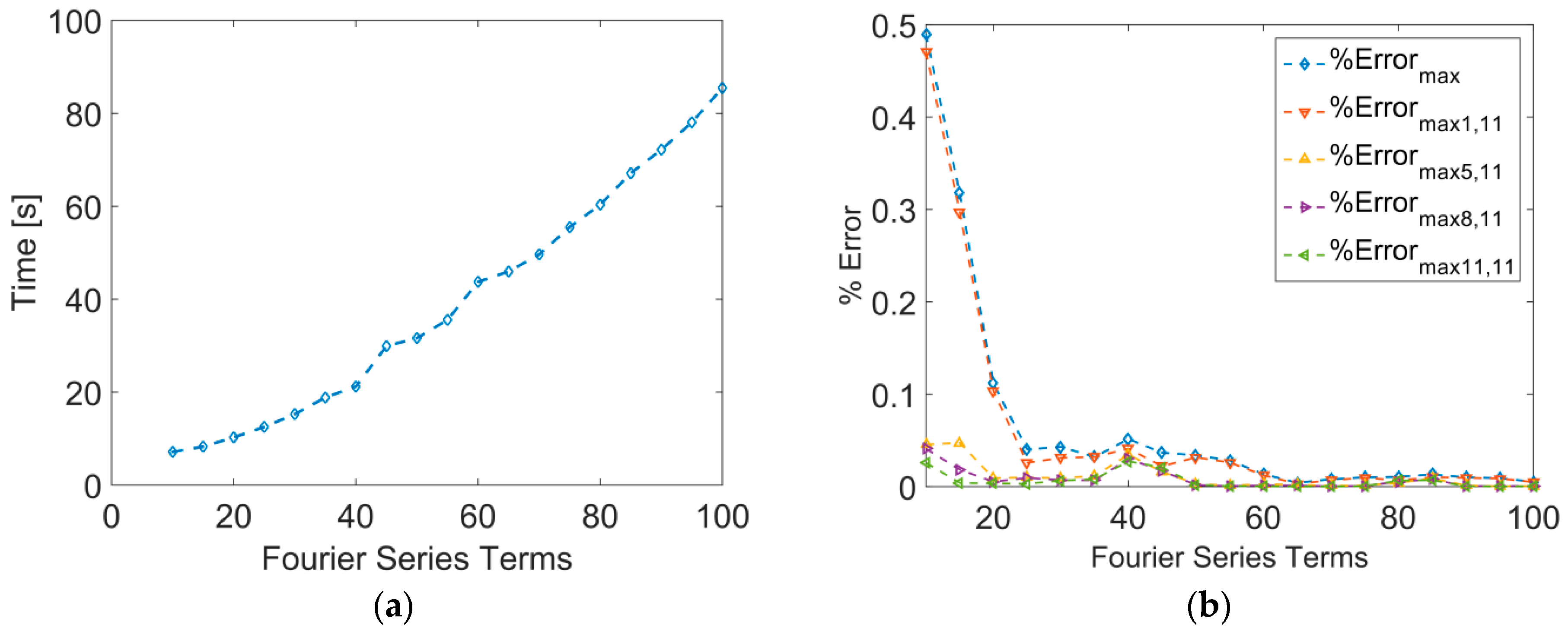
Appendix B. Truncate Inverse Fourier Transform Infinite Series and Computing Time Optimization
References
- Philipps, S.P.; Bett, A.W.; Horowitz, K.; Kurtz, S. Current Status of Concentrator Photovoltaic (CPV) Technology; National Renewable Energy Lab.: Golden, CO, USA, 2015. [Google Scholar]
- Baig, H.; Heasman, K.C.; Mallick, T.K. Non-uniform illumination in concentrating solar cells. Renew. Sustain. Energy Rev. 2012, 16, 5890–5909. [Google Scholar] [CrossRef]
- Franklin, E.T.; Coventry, J.S. Effects of Highly Non-uniform Illumination Distribution on Electrical Performance of Solar Cells. 2002. Available online: http://hdl.handle.net/1885/40832 (accessed on 16 July 2018).
- Lin, C.-K.; Lin, C.-Y.; Wu, J.-C.; Bett, A.W.; McConnell, R.D.; Sala, G.; Dimroth, F. Simulation Of Deformation Induced Sun Tracking Error In A High Concentrated Photovoltaic System. AIP Conf. Proc. 2010, 1277, 149–152. [Google Scholar]
- Chumakov, Y.S.; Rumyantsev, V.D.; Ascheulov, Y.V.; Chekalin, A.V. A comparative analysis of wind pressure on flat and stair-step constructions of solar plant trackers. St. Petersbg. Polytech. Univ. J. Phys. Math. 2015, 1, 299–304. [Google Scholar] [CrossRef]
- Stafford, B.; Davis, M.; Chambers, J.; Martinez, M.; Sanchez, D. Tracker accuracy: Field experience, analysis, and correlation with meteorological conditions. In Proceedings of the 2009 34th IEEE Photovoltaic Specialists Conference (PVSC), Philadelphia, PA, USA, 7–12 June 2009. [Google Scholar]
- Sanchez, D.; Trujillo, P.; Martinez, M.; Ferrer, J.P.; Rubio, F. CPV performance versus soiling effects: Cleaning policies. AIP Conf. Proc. 2012, 1477, 348–351. [Google Scholar]
- Calvo-Parra, G.; Martínez, M.; Sánchez, D.; de la Rubia, Ó.; Chatenay, P. Soiling effects comparison between CPV plants in continental and desert climates. AIP Conf. Proc. 2017, 1881, 020005. [Google Scholar]
- Luque-Heredia, I.; Moreno, J.; Magalhaes, P.; Cervantes, R.; Quemere, G.; Laurent, O. 11 Inspira’s CPV Sun Tracking. In Concentrator Photovoltaics; Springer: Berlin/Heidelberg, Germany, 2007; pp. 221–251. [Google Scholar]
- Victoria, M.; Domínguez, C.; Antón, I.; Sala, G. Comparative analysis of different secondary optical elements for aspheric primary lenses. Opt. Express 2009, 17, 6487. [Google Scholar] [CrossRef] [PubMed]
- Whitfield, G.; Bentley, R.; Weatherby, C.; Hunt, A.; Mohring, H.-D.; Klotz, F.; Keuber, P.; Miñano, J.; Alarte-Garvi, E. The development and testing of small concentrating PV systems. Sol. Energy 1999, 67, 23–34. [Google Scholar] [CrossRef]
- Leutz, R.; Suzuki, A.; Akisawa, A.; Kashiwagi, T. Nonideal concentration of nonimaging linear Fresnel lenses. Int. Soc. Opt. Photonics 2001, 4446, 100. [Google Scholar] [CrossRef]
- Lin, M.; Sumathy, K.; Dai, Y.J.; Zhao, X.K. Performance investigation on a linear Fresnel lens solar collector using cavity receiver. Sol. Energy 2014, 107, 50–62. [Google Scholar] [CrossRef]
- Renno, C.; De Giacomo, M. Dynamic Simulation of a CPV/T System Using the Finite Element Method. Energies 2014, 7, 7395–7414. [Google Scholar] [CrossRef]
- Li, Y.; Jing, D. Investigation of the performance of photovoltaic/thermal system by a coupled TRNSYS and CFD simulation. Sol. Energy 2017, 143, 100–112. [Google Scholar] [CrossRef]
- Sweet, T.K.N.; Rolley, M.H.; Li, W.; Paul, M.C.; Gao, M.; Knox, A.R. Experimental characterization and multi-physics simulation of a triple-junction cell in a novel hybrid III:V concentrator photovoltaic–thermoelectric receiver design with secondary optical element. Energy Procedia 2017, 142, 809–814. [Google Scholar] [CrossRef]
- Theristis, M.; O‘donovan, T.S. Electrical-thermal analysis of III–V triple-junction solar cells under variable spectra and ambient temperatures. Sol. Energy 2015, 118, 533–546. [Google Scholar] [CrossRef]
- Ahmad, N.; Ota, Y.; Araki, K.; Lee, K.-H.; Yamaguchi, M.; Nishioka, K. Optical and thermal simulation for wide acceptance angle CPV module. AIP Conf. Proc. 2017, 1881, 20001. [Google Scholar] [CrossRef]
- Baig, H.; Sellami, N.; Chemisana, D.; Rosell, J.; Mallick, T.K. Performance analysis of a dielectric based 3D building integrated concentrating photovoltaic system. Sol. Energy 2014, 103, 525–540. [Google Scholar] [CrossRef]
- Cotal, H.; Frost, J. Heat Transfer Modeling of Concentrator Multijunction Solar Cell Assemblies Using Finite Difference Techniques. In Proceedings of the 2010 35th IEEE Photovoltaic Specialists Conference, Honolulu, HI, USA, 20–25 June 2010. [Google Scholar]
- Min, C.; Nuo-Fu, C.; Jin-Xiang, D.; Li-Ying, L. Thermal modeling optimization and experimental validation for a single concentrator solar cell system with a heat sink. Chin. Phys. B 2013. [Google Scholar] [CrossRef]
- Theristis, M.; Fernández, E.F.; Sumner, M.; O‘donovan, T.S. Multiphysics modelling and experimental validation of high concentration photovoltaic modules. Energy Convers. Manag. 2017, 139, 122–134. [Google Scholar] [CrossRef]
- A’Oliverio, L.; Timò, G.; Minuto, A.; Groppelli, P. Thermal Simulations of A Cpv Point Focus Module Using Comsol Multiphysics. Sol. Energy Technol. 2010, 1, 55–64. [Google Scholar]
- Maillet, D.; André, S.; Batsale, J.C.; Degiovann, A.; Moyne, C. Thermal Quadrupoles: Solving the Heat Equation Through Integral Transforms; Wiley: Hoboken, NJ, USA, 2000; ISBN 0471983209. [Google Scholar]
- Bendada, A.; Erchiqui, F.; Lamontagne, M. Pulsed thermography in the evaluation of an aircraft composite using 3D thermal quadrupoles and mathematical perturbations. Inverse Probl. 2005, 21, 857–877. [Google Scholar] [CrossRef]
- Gardarein, J.-L.; Corre, Y.; Rigollet, F.; Le Niliot, C.; Reichle, R.; Andrew, P. Thermal quadrupoles approach for two-dimensional heat flux estimation using infrared and thermocouple measurements on the JET tokamak. Int. J. Therm. Sci. 2009, 48, 1–13. [Google Scholar] [CrossRef]
- Fudym, O.; Pradère, C.; Batsale, J.-C. An analytical two-temperature model for convection–diffusion in multilayered systems: Application to the thermal characterization of microchannel reactors. Chem. Eng. Sci. 2007, 62, 4054–4064. [Google Scholar] [CrossRef]
- Gerardin, J.; Aumeunier, M.-H.; Firdaouss, M.; Gardarein, J.-L.; Rigollet, F. Investigation on reduced thermal models for simulating infrared images in fusion devices. J. Phys. Conf. Ser. 2016, 745, 032019. [Google Scholar] [CrossRef]
- Pailhes, J.; Pradere, C.; Battaglia, J.-L.; Toutain, J.; Kusiak, A.; Aregba, A.W.; Batsale, J.-C. Thermal quadrupole method with internal heat sources. Int. J. Therm. Sci. 2012, 53, 49–55. [Google Scholar] [CrossRef]
- Blanco, M.J. Tonatiuh: An Object Oriented, Distributed Computing, Monte-Carlo Ray Tracer for the Design and Simulation of Solar Concentrating Systems. Available online: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.131.5087&rep=rep1&type=pdf (accessed on 16 July 2018).
- Den Iseger, P. Numerical Transform Inversion Using Gaussian Quadrature. Probab. Eng. Inform. Sc. 2006, 20, 1–44. [Google Scholar] [CrossRef]
- Blanco, M.J.; Amieva, J.M.; Mancillas, A. The Tonatiuh Software Development Project: An Open Source Approach to the Simulation of Solar Concentrating Systems. In Computers and Information in Engineering; ASME: New York, NY, USA, 2005; Volume 2005, pp. 157–164. [Google Scholar]
- Garcia, P.; Ferriere, A.; Bezian, J.-J. Codes for solar flux calculation dedicated to central receiver system applications: A comparative review. Sol. Energy 2008, 82, 189–197. [Google Scholar] [CrossRef]
- Osório, T.; Horta, P.; Larcher, M.; Pujol-Nadal, R.; Hertel, J.; Wet Van Rooyen, D.; Heimsath, A.; Schneider, S.; Benitez, D.; Frein, A.; et al. Ray-tracing software comparison for linear focusing solar collectors. AIP Conf. Proc. 2016, 1734, 020017. [Google Scholar] [CrossRef]
- Hecht, E. Optics; Addison-Wesley: Boston, MA, USA, 2002; ISBN 0805385665. [Google Scholar]
- Hornung, T.; Nitz, P. Light diffraction by concentrator Fresnel lenses. Prog. Photovolt. Res. Appl. 2013, 21, 827–837. [Google Scholar] [CrossRef] [PubMed]
- FresnelFactory.com—Fresnel Lens. Available online: http://www.fresnelfactory.com/fresnel-lens-fl220-285-f220-fresnel-magnifying-lens.html (accessed on 7 June 2018).
- Toutain, J.; Battaglia, J.-L.; Pradere, C.; Pailhes, J.; Kusiak, A.; Aregba, W.; Batsale, J.-C. Numerical Inversion of Laplace Transform for Time Resolved Thermal Characterization Experiment. J. Heat Trans. 2011, 133, 044504. [Google Scholar] [CrossRef]
- Davis, A. Fresnel lens solar concentrator derivations and simulations. In Novel Optical Systems Design and Optimization XIV; Koshel, R.J., Gregory, G.G., Eds.; International Society for Optics and Photonics: Washington, DC, USA, 2011; Volume 8129, p. 81290J. [Google Scholar]
- Parretta, A. Optics of solar concentrators Part I: Theoretical models of light collection. Int. J. Opt. Appl. 2013, 3, 27–39. [Google Scholar] [CrossRef]
- Parretta, A.; Antonini, A. Optics of solar concentrators. Part II: Models of light collection of 3D-CPCs under direct and collimated beams. Int. J. Opt. Appl. 2013, 3, 72–102. [Google Scholar] [CrossRef]
- Goodfellow Germanio. Available online: http://www.goodfellow.com/S/Germanio.html (accessed on 8 June 2018).
- Goodfellow Aluminio. Available online: http://www.goodfellow.com/S/Aluminio.html (accessed on 8 June 2018).
- Wakefield-vette Thermal Joint Compund. Available online: http://www.wakefield-vette.com/products/productlist/productdetails/tabid/341/CategoryID/153/ProductID/308/Default.aspx (accessed on 8 June 2018).
- Culham, J.R.; Yovanovich, M.M.; Lee, S. Thermal Modeling of Isothermal Cuboids and Rectangular Heat Sinks Cooled by Natural Convection. IEEE Trans. Compon. Packag. Manuf. Technol. Part A 1995, 18, 559–566. [Google Scholar] [CrossRef]
- Silverman, T.J.; Bosco, N.; Kurtz, S. Relative lifetime prediction for CPV die-attach layers. In Proceedings of the 2012 IEEE International Reliability Physics Symposium (IRPS), Anaheim, CA, USA, 15–19 April 2012. [Google Scholar]
- Bosco, N.; Silverman, T.J.; Kurtz, S. Modeling Thermal Fatigue in CPV Cell Assemblies Preprint. In Modeling Thermal Fatigue in Cpv Cell Assemblies; National Renewable Energy Lab.: Golden, CO, USA, 2011; p. 16560. [Google Scholar]
- Xu, X.; Meyers, M.M.; Sammakia, B.G.; Murray, B.T.; Chen, C. Performance and Reliability Analysis of Hybrid Concentrating Photovoltaic/Thermal Collectors with Tree-Shaped Channel Nets’ Cooling System. IEEE Trans. Compon. Packag. Manuf. Technol. 2013, 3, 967–977. [Google Scholar] [CrossRef]
- Xu, X.; Meyers, M.M.; Sammakia, B.G.; Murray, B.T. Thermal Modeling and Life Prediction of Water-Cooled Hybrid Concentrating Photovoltaic/Thermal Collectors. J. Sol. Energy Eng. 2012, 135, 011010. [Google Scholar] [CrossRef]
- Cao, M.; Butler, S.; Benoit, J.T.; Jiang, Y.; Radhakrishnan, R.; Chen, Y.; Bendapudi, S.; Horne, S. Thermal Stress Analysis/Life Prediction of Concentrating Photovoltaic Module. J. Sol. Energy Eng. 2008, 130, 021011. [Google Scholar] [CrossRef]
- Ota, Y.; Nishioka, K. Estimation of thermal stress in concentrator cells using structural mechanics simulation. AIP Conf. Proc. 2014, 1616, 25–28. [Google Scholar] [CrossRef]
- Renno, C.; Landi, G.; Petito, F.; Neitzert, H.C. Influence of a degraded triple-junction solar cell on the CPV system performances. Energy Convers. Manag. 2018, 160, 326–340. [Google Scholar] [CrossRef]
- Bosco, N.; Kurtz, S.R. Quantifying the thermal fatigue of CPV modules. AIP Conf. Proc. 2011, 1277, 225–228. [Google Scholar]
- Peharz, G.; Ferrer Rodríguez, J.P.; Siefer, G.; Bett, A.W. Investigations on the temperature dependence of CPV modules equipped with triple-junction solar cells. Prog. Photovolt. Res. Appl. 2011, 19, 54–60. [Google Scholar] [CrossRef]
- Saloux, E.; Teyssedou, A.; Sorin, M. Explicit model of photovoltaic panels to determine voltages and currents at the maximum power point. Sol. Energy 2011, 85, 713–722. [Google Scholar] [CrossRef]



| Author | Thermal Model | Time Regime | Spatial Resolution | Electrical Model |
|---|---|---|---|---|
| Renno et al. [14] | FEM | Transient | 3D | Single diode |
| Li et al. [15] | FEM | Transient | 3D | Energy efficiency |
| Sweet et al. [16] | FEM | Transient | 3D | Single diode |
| Theristis et al. [17] | FEM | Steady state | 3D | Single diode |
| Ahmad et al. [18] | FEM | Steady state | 3D | Single unit equivalent circuit |
| Baig et al. [19] | FEM | Steady state | 3D | Single diode |
| Cotal et al. [20] | Finite differences | Steady state | 3D | - |
| Min et al. [21] | FEM | Steady state | 3D | - |
| Theristis et al. [22] | FEM | Steady state and transient | 3D | Single diode |
| Oliverio et al. [23] | FEM | Steady state and transient | 3D, 2D | - |
| Type | Description | Details |
|---|---|---|
| Location | CIO, Aguascalientes | Research Institute, Fresnel lens experiments, DNI measurements (21°50′42.7″ N 102°20′37.5″ W) |
| Location | CICY, Mérida | Research Institute, Tonatiuh and Thermal quadrupoles simulations |
| Software | Tonatiuh [30] | Open source, for ray tracing analysis, simulate sun ray vectors throughout year, and deliver irradiance maps upon custom receiver surfaces |
| Software | Matlab 2014b | Thermal quadrupoles simulations |
| Script | Den Iseger Algorithm [31] | Inverse Laplace transformation |
| Laptop | Hp Envy | Processor Intel core i5-4210U, 8 GB RAM; Tonatiuh and Matlab scripts execution |
| CCD camera | MAKO, Allied vision | 8 bits, monochrome, GigE; Irradiance spot spatial profile measurements |
| Irradiance Sensor | Gardon-Schmidt-Boelter | Water cooled sensor (SBG01) by Hukseflux, calibrated in accordance to the ISO 14934-3 standard; Flux measurements |
| Solar station | Solys2 | Scientific Grade (Kipp&Zonen Solys2 + SHP1 pyrheliometer), Direct Normal Irradiance (DNI) and ambient temperature measurements |
| Fresnel Lens | FL220-285 | PMMA, Square aperture concentrator 267 × 267 mm2, 220 mm focal length and transmissivity of 0.92. Fresnel lens model by Tonatiuh was inspired by this commercial lens |
| Specification | Value |
|---|---|
| Model | FL220-285 |
| Type | Point focus |
| Material | PMMA |
| Size | 285 × 285 mm |
| Active Area | 267 × 267 mm |
| Transmissivity | 0.92 |
| Focal length | 220 mm |
| Pitch | 0.5 mm |
| Spot Property | Tonatiuh | Experimental |
|---|---|---|
| Total Power | 39.2 W | 31.2 W |
| Max Flux | 1.2 × 105 W/m2 | 1.3 × 105 W/m2 |
| Average Flux | 7.7 × 104 W/m2 | 5.5 × 104 W/m2 |
| Property | CPV Cell (Ge) | Heat Sink (Al) | Thermal Glue |
|---|---|---|---|
| Thermal conductivity [W/m∙K] | 60.2 | 237 | 0.735 |
| Density [K/m3] | 5320 | 2700 | - |
| Specific heat [J/Kg K] | 322 | 900 | - |
| Concentration Factor | 0–500 | ||
| Heat sink-liquid convection coefficient (W/m2·K) | 3000–6000 | ||
| Cooling water temperature | 29 °C | ||
| Flux (x, y) | 1 Day | 1 Week | 1 Month (30 Days) | |||
|---|---|---|---|---|---|---|
| Impulse | Convolution | Impulse | Convolution | Impulse | Convolution | |
| Know spatial function | 8.2 | 0.02 | 8.2 | 0.23 | 8.2 | 0.68 |
| Unknown spatial function (M by N impulses) | 994 | 23 | 994 | 130 | 994 | 742 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mateos-Canseco, A.; Peña-Cruz, M.I.; Díaz-Ponce, A.; Battaglia, J.-L.; Pradère, C.; Patino-Lopez, L.D. Time-Resolved Temperature Map Prediction of Concentration Photovoltaics Systems by Means of Coupled Ray Tracing Flux Analysis and Thermal Quadrupoles Modelling. Energies 2018, 11, 2042. https://doi.org/10.3390/en11082042
Mateos-Canseco A, Peña-Cruz MI, Díaz-Ponce A, Battaglia J-L, Pradère C, Patino-Lopez LD. Time-Resolved Temperature Map Prediction of Concentration Photovoltaics Systems by Means of Coupled Ray Tracing Flux Analysis and Thermal Quadrupoles Modelling. Energies. 2018; 11(8):2042. https://doi.org/10.3390/en11082042
Chicago/Turabian StyleMateos-Canseco, Alejandro, Manuel I. Peña-Cruz, Arturo Díaz-Ponce, Jean-Luc Battaglia, Christophe Pradère, and Luis David Patino-Lopez. 2018. "Time-Resolved Temperature Map Prediction of Concentration Photovoltaics Systems by Means of Coupled Ray Tracing Flux Analysis and Thermal Quadrupoles Modelling" Energies 11, no. 8: 2042. https://doi.org/10.3390/en11082042
APA StyleMateos-Canseco, A., Peña-Cruz, M. I., Díaz-Ponce, A., Battaglia, J.-L., Pradère, C., & Patino-Lopez, L. D. (2018). Time-Resolved Temperature Map Prediction of Concentration Photovoltaics Systems by Means of Coupled Ray Tracing Flux Analysis and Thermal Quadrupoles Modelling. Energies, 11(8), 2042. https://doi.org/10.3390/en11082042





