Total Harmonic Distortion Oriented Finite Control Set Model Predictive Control for Single-Phase Inverters
Abstract
:1. Introduction
- (1)
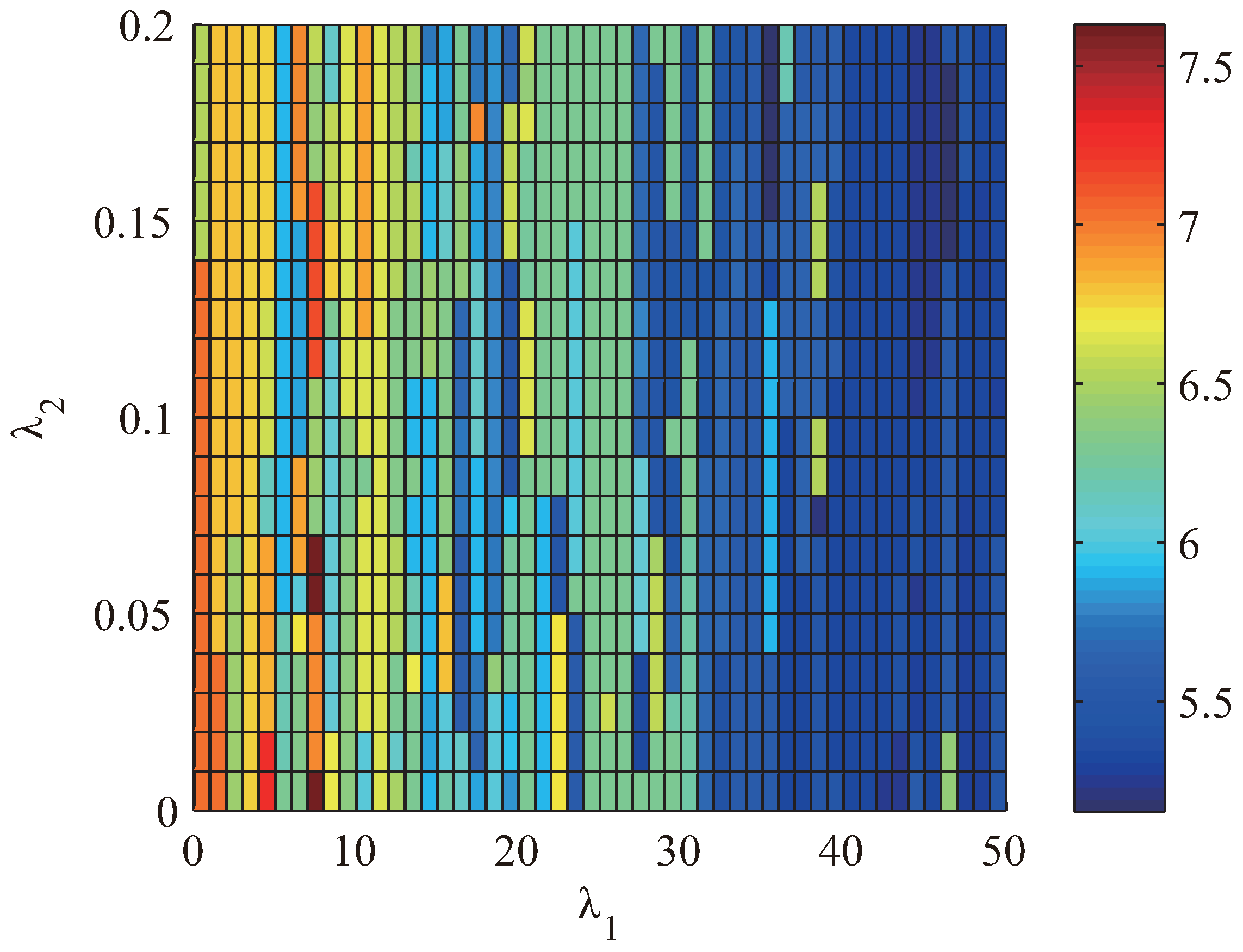
- Based on FCS MPC frame, the proposed THD oriented FCS MPC controller is realized by ameliorating the cost function. Different from traditional one, a linear combination with weight factors of the fundamental tracking error, instantaneous THD value and DC component in current constitutes the cost function. Offline optimization is used for the selection of weight factors.
- (2)
- To avoid calculation complexity in real-time THD control, the items of the cost function are obtained by iterative algorithm. Then, switching state can be chosen after minimizing cost function and achieve the goal of THD reduction.
2. System Description and Problem Statement
2.1. System Description
2.2. Problem Statement
3. Controller Design
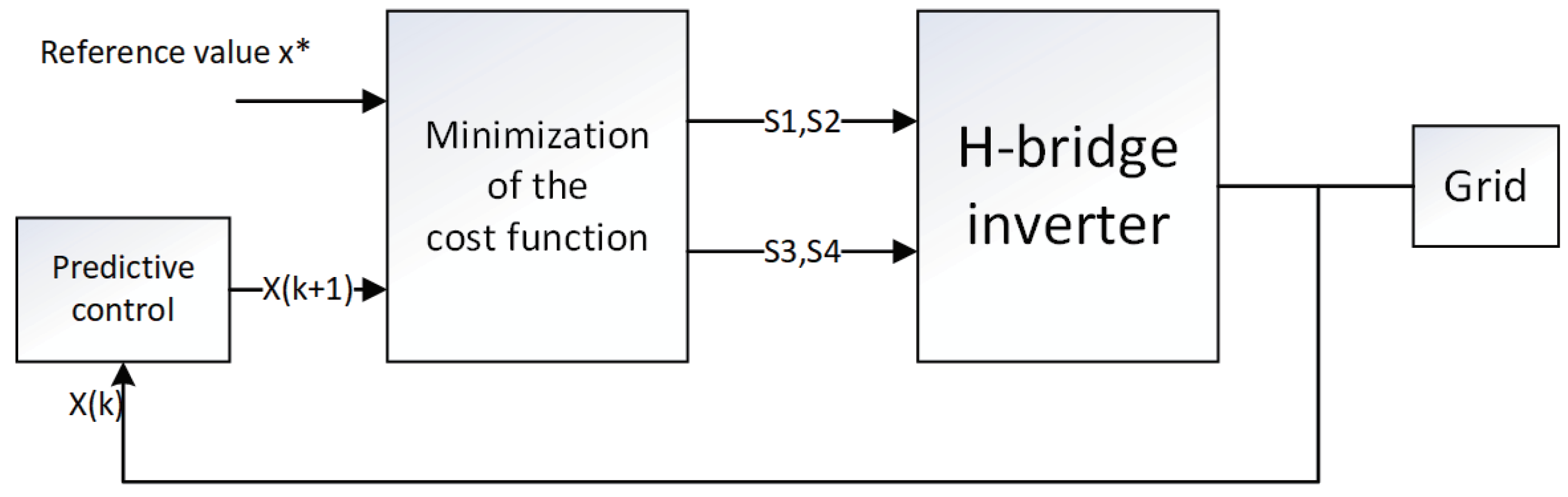
3.1. Control Scheme
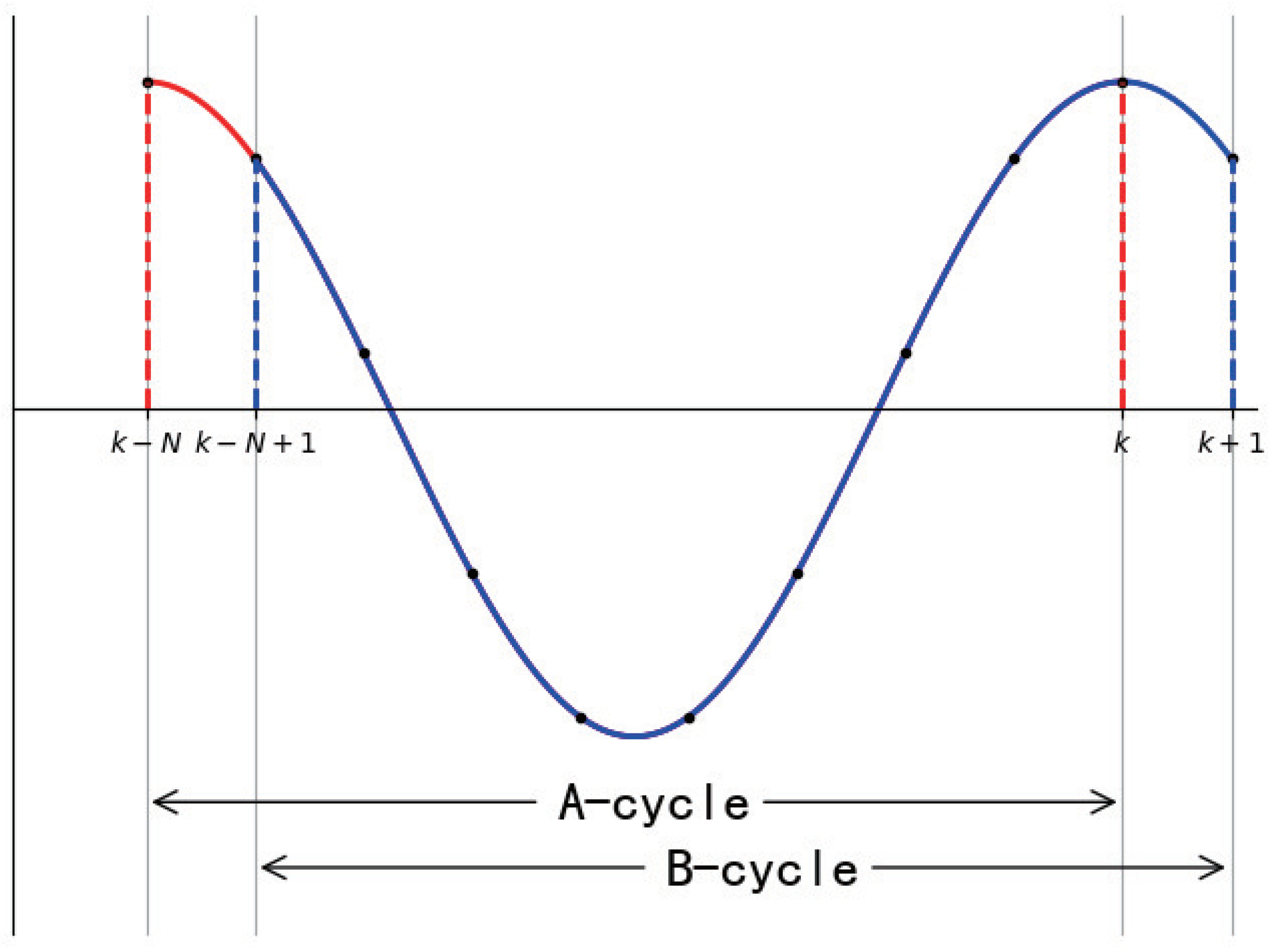
3.2. THD Prediction
3.3. Fundamental Wave Extraction
3.4. FCS MPC Scheme
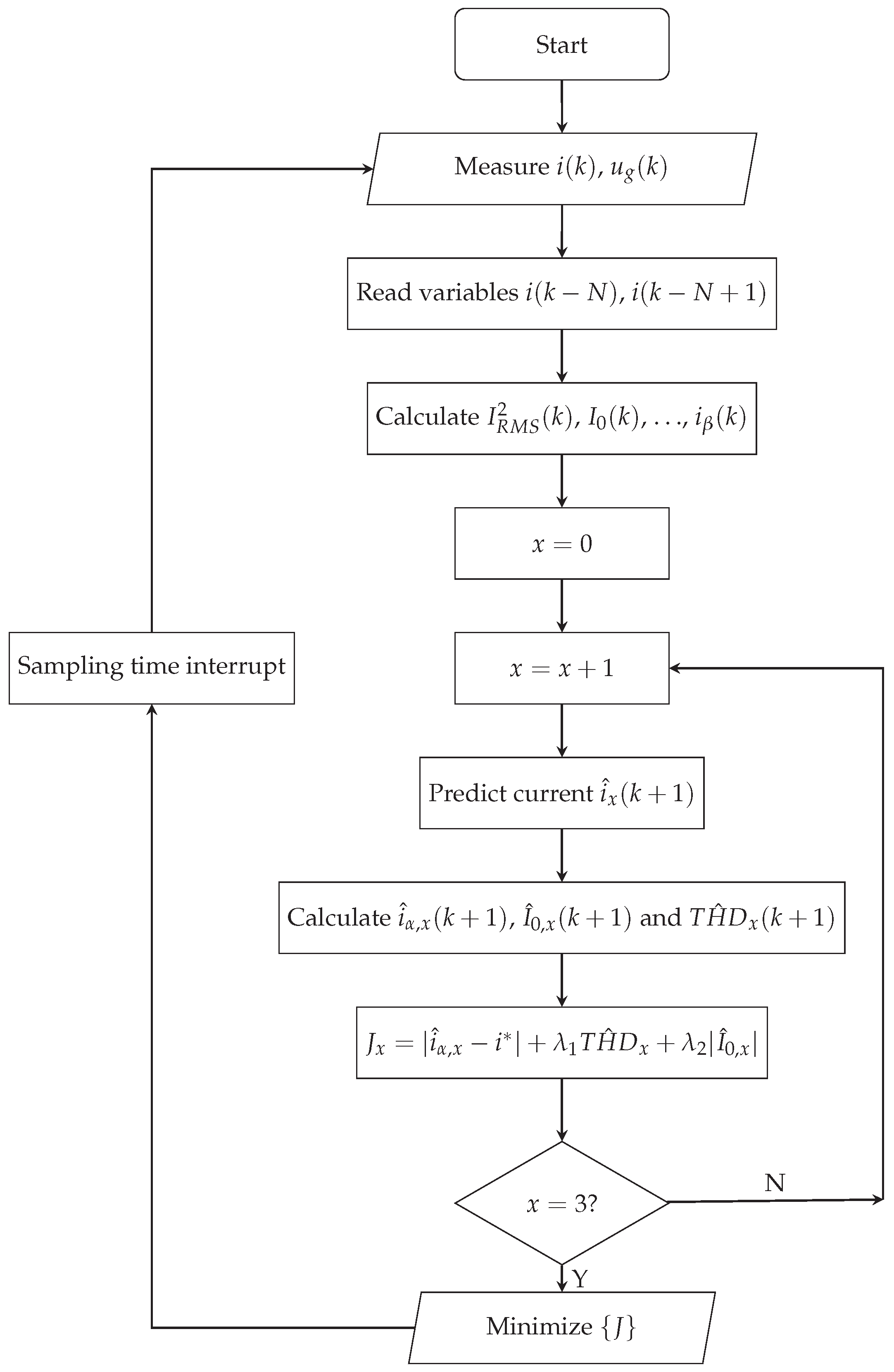
3.5. Close Loop Realization
- Step 1
- Initialize digital controller.
- Step 2
- Measure and by sensors, and read from memory.
- Step 3
- Step 4
- Initialize the inner loop with .
- Step 5
- Add 1 to cycle count value x, and select the xth switch state to calculate the corresponding predicted current .
- Step 6
- Step 7
- Calculate the cost function by Equation (26).
- Step 8
- Judge ? If yes, then next step, else jump to Step 5.
- Step 9
- Select the optimal corresponding to the minimum cost J, then send the switching signals to inverters and return to Step 2.
4. Simulation Verification
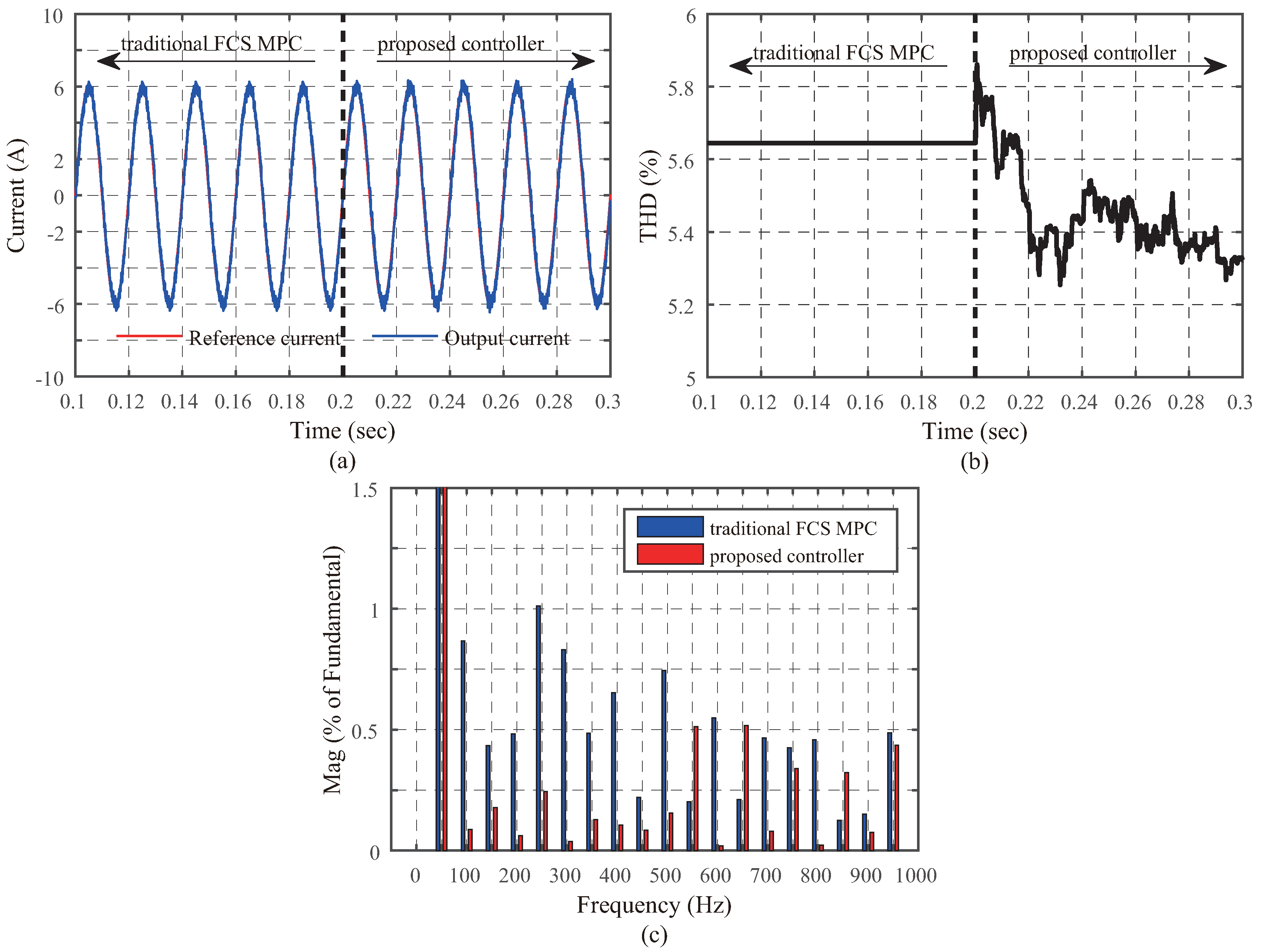
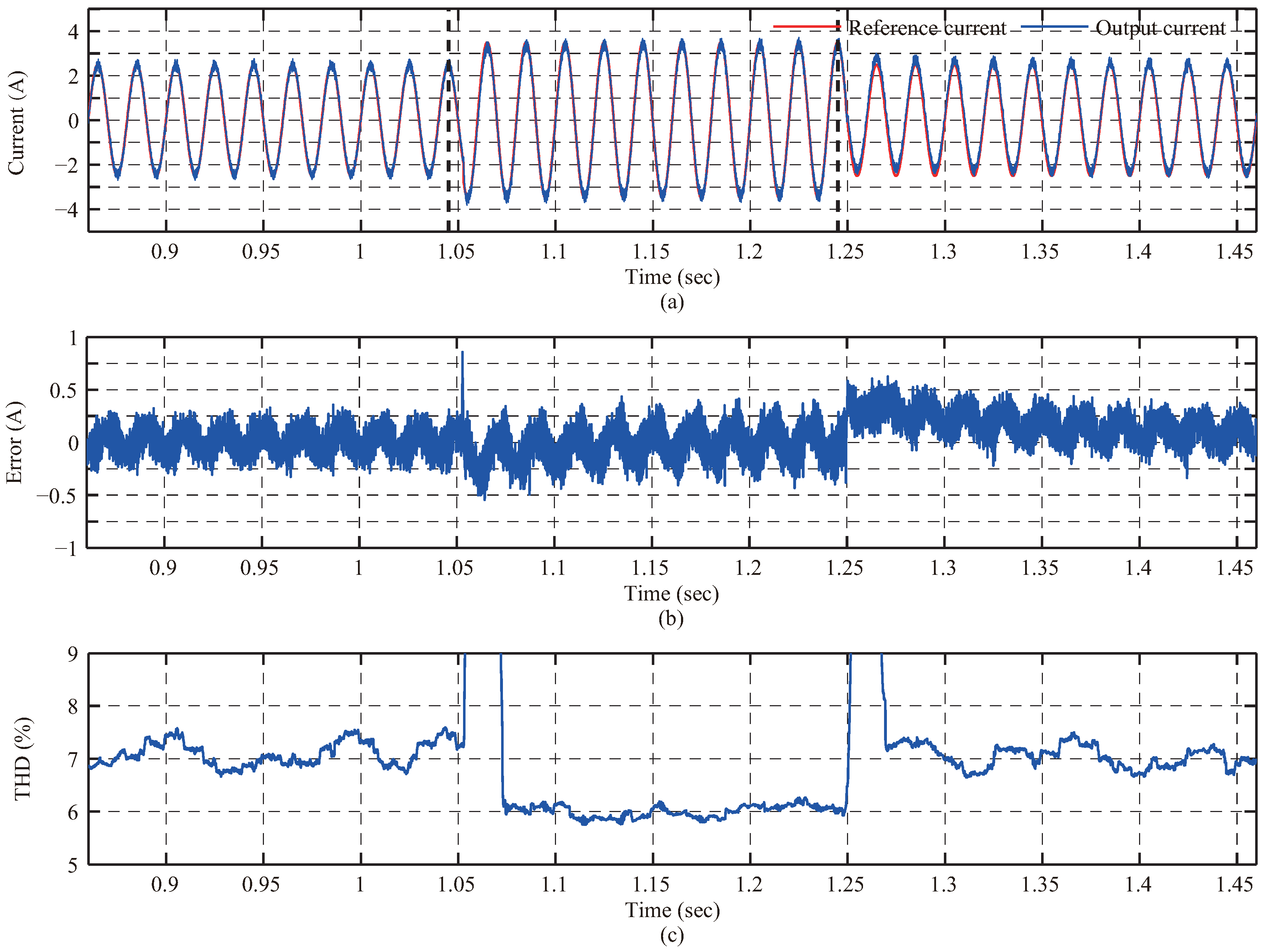
5. Experimental Verification
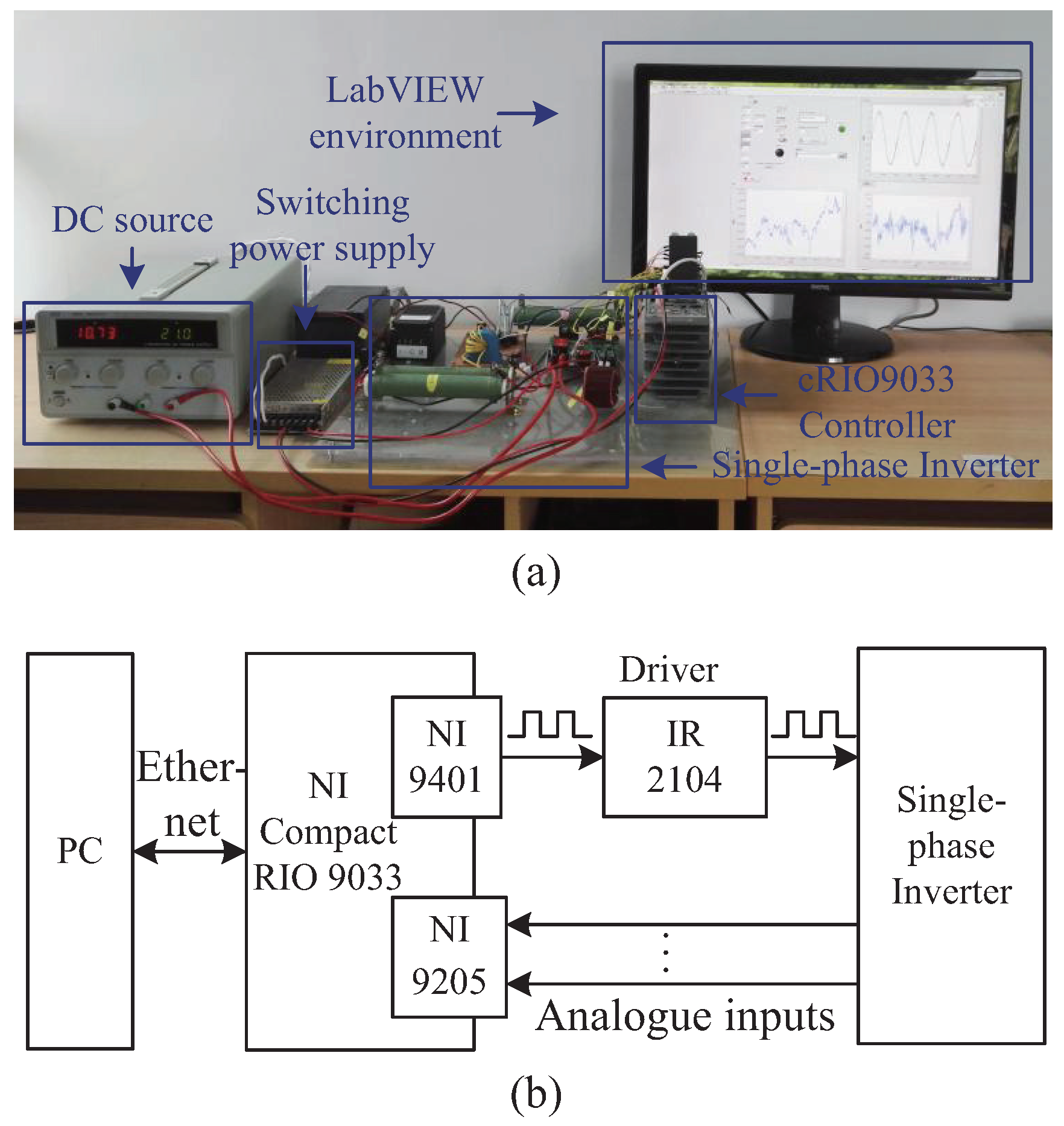
5.1. Test Bench
5.2. Experimental Results
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Dordevic, O.; Jones, M.; Levi, E. Analytical Formulas for Phase Voltage RMS Squared and THD in PWM Multiphase Systems. IEEE Trans. Power Electron. 2015, 30, 1645–1656. [Google Scholar] [CrossRef] [Green Version]
- Zhong, Q.C.; Hornik, T. Cascaded Current-Voltage Control to Improve the Power Quality for a Grid-Connected Inverter with a Local Load. IEEE Trans. Ind. Electron. 2013, 60, 1344–1355. [Google Scholar] [CrossRef]
- Chen, D.; Zhang, J.; Qian, Z. An Improved Repetitive Control Scheme for Grid-Connected Inverter with Frequency-Adaptive Capability. IEEE Trans. Ind. Electron. 2013, 60, 814–823. [Google Scholar] [CrossRef]
- Noman, A.M.; Al-Shamma’a, A.A.; Addoweesh, K.E.; Alabduljabbar, A.A.; Alolah, A.I. Cascaded Multilevel Inverter Topology Based on Cascaded H-Bridge Multilevel Inverter. Energies 2018, 11, 895. [Google Scholar] [CrossRef]
- Franquelo, L.G.; Rodriguez, J.; Leon, J.I.; Kouro, S. The age of multilevel converters arrives. IEEE Ind. Electron. Mag. 2008, 2, 28–39. [Google Scholar] [CrossRef]
- Alishah, R.S.; Nazarpour, D.; Hosseini, S.H.; Sabahi, M. Reduction of Power Electronic Elements in Multilevel Converters Using a New Cascade Structure. IEEE Trans. Ind. Electron. 2015, 62, 256–269. [Google Scholar] [CrossRef]
- Jahani, F.; Monfared, M. A Multilevel AC/AC Converter with Reduced Number of Switches. IEEE Trans. Ind. Electron. 2018, 65, 1244–1253. [Google Scholar] [CrossRef]
- Metri, J.I.; Vahedi, H.; Kanaan, H.Y.; Al-Haddad, K. Real-Time Implementation of Model-Predictive Control on Seven-Level Packed U-Cell Inverter. IEEE Trans. Ind. Electron. 2016, 63, 4180–4186. [Google Scholar] [CrossRef] [Green Version]
- Jayalath, S.; Hanif, M. Generalized LCL-Filter Design Algorithm for Grid-Connected Voltage-Source Inverter. IEEE Trans. Ind. Electron. 2017, 64, 1905–1915. [Google Scholar] [CrossRef]
- Pandey, R.; Tripathi, R.N.; Hanamoto, T. Comprehensive Analysis of LCL Filter Interfaced Cascaded H-Bridge Multilevel Inverter-Based DSTATCOM. Energies 2017, 10, 346. [Google Scholar] [CrossRef]
- Liu, Y.; Lai, C.M. LCL Filter Design with EMI Noise Consideration for Grid-Connected Inverter. Energies 2018, 11, 1646. [Google Scholar] [CrossRef]
- Fang, J.; Li, X.; Yang, X.; Tang, Y. An Integrated Trap-LCL Filter with Reduced Current Harmonics for Grid-Connected Converters under Weak Grid Conditions. IEEE Trans. Power Electron. 2017, 32, 8446–8457. [Google Scholar] [CrossRef]
- Wu, W.; He, Y.; Blaabjerg, F. An LLCL Power Filter for Single-Phase Grid-Tied Inverter. IEEE Trans. Power Electron. 2012, 27, 782–789. [Google Scholar] [CrossRef]
- Napoles, J.; Leon, J.I.; Portillo, R.; Franquelo, L.G.; Aguirre, M.A. Selective harmonic mitigation technique for high-power converters. IEEE Trans. Ind. Electron. 2010, 57, 2315–2323. [Google Scholar] [CrossRef]
- Srndovic, M.; Zhetessov, A.; Alizadeh, T.; Familiant, Y.; Grandi, G.; Ruderman, A. Simultaneous Selective Harmonic Elimination and THD Minimization for a Single-Phase Multilevel Inverter with Staircase Modulation. IEEE Trans. Ind. Appl. 2018, 54, 1532–1541. [Google Scholar] [CrossRef]
- Ahmadi, D.; Zou, K.; Li, C.; Huang, Y.; Wang, J. A universal selective harmonic elimination method for high-power inverters. IEEE Trans. Power Electron. 2011, 26, 2734–2752. [Google Scholar] [CrossRef]
- Massrur, H.R.; Niknam, T.; Mardaneh, M.; Rajaei, A.H. Harmonic Elimination in Multilevel Inverters Under Unbalanced Voltages and Switching Deviation Using a New Stochastic Strategy. IEEE Trans. Ind. Inform. 2016, 12, 716–725. [Google Scholar] [CrossRef]
- Kavousi, A.; Vahidi, B.; Salehi, R.; Bakhshizadeh, M.K.; Farokhnia, N.; Fathi, S.H. Application of the Bee Algorithm for Selective Harmonic Elimination Strategy in Multilevel Inverters. IEEE Trans. Power Electron. 2012, 27, 1689–1696. [Google Scholar] [CrossRef]
- Shen, K.; Zhao, D.; Mei, J.; Tolbert, L.M.; Wang, J.; Ban, M.; Ji, Y.; Cai, X. Elimination of Harmonics in a Modular Multilevel Converter Using Particle Swarm Optimization-Based Staircase Modulation Strategy. IEEE Trans. Ind. Electron. 2014, 61, 5311–5322. [Google Scholar] [CrossRef]
- Yuan, J.; Pan, J.; Fei, W.; Cai, C.; Chen, Y.; Chen, B. An Immune-Algorithm-Based Space-Vector PWM Control Strategy in a Three-Phase Inverter. IEEE Trans. Ind. Electron. 2013, 60, 2084–2093. [Google Scholar] [CrossRef]
- Agarwal, P.; Agarwal, V. A novel approach of space vector modulation for cycloinverter using genetic algorithm. IET Power Electron. 2013, 6, 1723–1731. [Google Scholar] [CrossRef]
- Yang, S.H.; Yang, Y.H.; Chen, K.H.; Lin, Y.H.; Tsai, T.Y.; Lin, S.R.; Lee, C. A Low-THD Class-D Audio Amplifier with Dual-Level Dual-Phase Carrier Pulsewidth Modulation. IEEE Trans. Ind. Electron. 2015, 62, 7181–7190. [Google Scholar] [CrossRef]
- Coppola, M.; Napoli, F.D.; Guerriero, P.; Iannuzzi, D.; Daliento, S.; Pizzo, A.D. An FPGA-Based Advanced Control Strategy of a Grid-Tied PV CHB Inverter. IEEE Trans. Power Electron. 2016, 31, 806–816. [Google Scholar] [CrossRef]
- Attia, H.A.; Tan, K.S.F.; Hang, S.C.; Hew, W.P.; Khateb, A.E. Confined Band Variable Switching Frequency Pulse Width Modulation (CB-VSF PWM) for Single-Phase Inverter with LCL Filter. IEEE Trans. Power Electron. 2017, 32, 8593–8605. [Google Scholar] [CrossRef]
- Rajapakse, G.; Jayasinghe, S.; Fleming, A.; Negnevitsky, M. A Model Predictive Control-Based Power Converter System for Oscillating Water Column Wave Energy Converters. Energies 2017, 10, 1631. [Google Scholar] [CrossRef]
- Kouro, S.; Cortes, P.; Vargas, R.; Ammann, U.; Rodriguez, J. Model Predictive Control-A Simple and Powerful Method to Control Power Converters. IEEE Trans. Ind. Electron. 2009, 56, 1826–1838. [Google Scholar] [CrossRef]
- Kouro, S.; Perez, M.A.; Rodriguez, J.; Llor, A.M. Model Predictive Control: MPC’s Role in the Evolution of Power Electronics. IEEE Ind. Electron. Mag. 2015, 9, 8–21. [Google Scholar] [CrossRef]
- Aguilera, R.P.; Acuna, P.; Yu, Y.; Konstantinou, G.; Townsend, C.D.; Wu, B.; Agelidis, V.G. Predictive Control of Cascaded H-Bridge Converters Under Unbalanced Power Generation. IEEE Trans. Ind. Electron. 2017, 64, 4–13. [Google Scholar] [CrossRef] [Green Version]
- Rodríguez, J.; Pontt, J.; Silva, C.A.; Correa, P.; Lezana, P.; Cortés, P.; Ammann, U. Predictive current control of a voltage source inverter. IEEE Trans. Ind. Electron. 2007, 54, 495–503. [Google Scholar] [CrossRef]
- Bayhan, S.; Trabelsi, M.; Abu-Rub, H.; Malinowski, M. Finite-Control-Set Model-Predictive Control for a Quasi-Z-Source Four-Leg Inverter Under Unbalanced Load Condition. IEEE Trans. Ind. Electron. 2017, 64, 2560–2569. [Google Scholar] [CrossRef]
- Viinamäki, J.; Kuperman, A.; Suntio, T. Grid-Forming-Mode Operation of Boost-Power-Stage Converter in PV-Generator-Interfacing Applications. Energies 2017, 10, 1033. [Google Scholar] [CrossRef]
- Monfared, M.; Sanatkar, M.; Golestan, S. Direct active and reactive power control of single-phase grid-tie converters. IET Power Electron. 2012, 5, 1544–1550. [Google Scholar] [CrossRef]



| Parameters | Descriptions | Value |
|---|---|---|
| L | The filter inductance | 5 mH |
| R | The ESR of L | 1 Ω |
| The input DC voltage | 48 V | |
| The back electromotive force | 20 (V) | |
| The output AC frequency | 50 Hz | |
| The control sampling frequency | 10 kHz |
| Parameter | Model |
|---|---|
| Controller | NI compactRIO 9033 |
| Acquisition card | NI 9205 |
| Execution unit | NI 9401 |
| Current sensor | LAH50-P |
| Isolation IC | 74HC244 |
| Gate Driver IC | IR2104 |
| Switch tube | IPB044N15N5 |
| DC source | MPS-6010LP-1 |
| Switching power supply | D-220DC 24 V/12 V |
| Parameters | Descriptions | Value |
|---|---|---|
| L | The load inductance | 6.085 mH |
| R | The load resistance | 5.46 Ω |
| The input DC voltage | 21 V | |
| The back electromotive force | 0 V | |
| The output AC frequency | 50 Hz | |
| The control sampling frequency | 10 kHz |
| i* (A) | THD1 (%) a | THD2 (%) b | Decline (%) | ||
|---|---|---|---|---|---|
| 1 | 2.6 | 0.03 | 14.60 | 13.71 | 6.08 |
| 2 | 7.0 | 0.05 | 8.21 | 8.00 | 2.56 |
| 3 | 15.0 | 0.07 | 6.27 | 6.20 | 1.12 |
| 4 | 32.0 | 0.10 | 4.93 | 4.88 | 1.01 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Li, R.; Feng, H. Total Harmonic Distortion Oriented Finite Control Set Model Predictive Control for Single-Phase Inverters. Energies 2018, 11, 3467. https://doi.org/10.3390/en11123467
Li P, Li R, Feng H. Total Harmonic Distortion Oriented Finite Control Set Model Predictive Control for Single-Phase Inverters. Energies. 2018; 11(12):3467. https://doi.org/10.3390/en11123467
Chicago/Turabian StyleLi, Po, Ruiyu Li, and Haifeng Feng. 2018. "Total Harmonic Distortion Oriented Finite Control Set Model Predictive Control for Single-Phase Inverters" Energies 11, no. 12: 3467. https://doi.org/10.3390/en11123467
APA StyleLi, P., Li, R., & Feng, H. (2018). Total Harmonic Distortion Oriented Finite Control Set Model Predictive Control for Single-Phase Inverters. Energies, 11(12), 3467. https://doi.org/10.3390/en11123467





