Engineering Simulation Tests on Multiphase Flow in Middle- and High-Yield Slanted Well Bores

Abstract

Previous multiphase pipe flow tests have mainly been conducted in horizontal and vertical pipes, with few tests conducted on multiphase pipe flow under different inclined angles. In this study, in light of mid–high yield and highly deviated wells in the Middle East and on the basis of existent multiphase flow pressure research on well bores, multiphase pipe flow tests were conducted under different inclined angles, liquid rates, and gas rates. A pressure prediction model based on Mukherjee model, but with new coefficients and higher accuracy for well bores in the study block, was obtained. It was verified that the newly built pressure drawdown prediction model tallies better with experimental data, with an error of only 11.3%. The effect of inclination, output, and gas rate on the flow pattern, liquid holdup, and friction in the course of multiphase flow were analyzed comprehensively, and six kinds of classical flow regime maps were verified with this model. The results showed that for annular and slug flow, the Mukherjee flow pattern map had a higher accuracy of 100% and 80–100%, respectively. For transition flow, Duns and Ros flow pattern map had a higher accuracy of 46–66%.

1. Introduction

Multiphase flow of oil, gas, and water universally exist in the course of oil and gas exploitation as the multiphase flow in well bores have a great impact on the selection of rational oil production method, the adjustment of production parameters, improvement in lift efficiency, and achievement of optimal profits [1,2,3]. Therefore, it is very important to study the multiphase flow features of well bores, with the flow pattern and pressure loss especially crucial for oil recovery engineering. In petroleum engineering, the multiphase flow is mostly studied as per the following procedure: Firstly, lab simulation test is conducted. Then, based on the test phenomena and data analysis, the flow parameters in the course of multiphase flow are figured out to build a pressure drawdown prediction model. Finally, the field production data is used to verify the model. Although research on multiphase pipe flow in well bores has been going on for more than half a century, a general method to discriminate the flow pattern and predict the pressure variation has still not been developed.

The main task of multiphase flow research is to analyze the flow behaviors of each phase and the mixed phase and build their correlating relations. As the flow pattern variation is the most intuitive and fundamental phenomenon, analysis on forming mechanisms and features of flow pattern is the foundation for studying the features of multiphase flow. However, there is no uniform standard for the classification of flow patterns yet, and therefore most are classified according to the variation of flow shapes. This method often results in big errors as it is restricted by objective conditions, such as observation instrument, and affected by subjective factors, such as the observer. At present, the flow patterns mostly used for classification include bubble flow, slug flow, churn flow, annular flow, laminar flow, and wave flow, with the latter two mainly occurring in horizontal or slightly inclined strings.

The pressure variation of oil and gas well system is not only a foundation for judging the natural flow of oil and gas wells and studying the production effects of various lift modes, but it is also an important parameter considered in the optimum design of oil and gas wells to guarantee high and stable yield. Currently, research on wellbore pressure drawdown mostly regard oil, gas, and water as gas and liquid phases, taking the flow features of each flow pattern as the basis to figure out the pressure distribution along the well bore based on momentum conservation and energy conservation. There are very few research projects that regard oil, gas, and water as three phases due to the complicated oil–water mixture property and an unclear understanding of the flow pattern and formation mechanism.

After the multiphase flow technology was first applied in petroleum engineering in the 1950s, researchers like Govier, Griffith, Fancher, and Brown presented some related expressions to calculate pressure loss based on experiments [4,5,6,7,8]. However, these related expressions were mostly derived based on small pipe diameter and vertical pipe and were not introduced in practical applications. Starting from the 1960s, researchers like Duns and Ros, Hagedorn and Brown, Orkiszawski, Govier, and Aziz [8,9,10,11] were devoted to study more applicable equations and obtained good results. The related expressions they presented had higher accuracy when the flow conditions matched; however, they did not take the effect of inclined angle into account [3,11]. Beggs and Brill (1973) [12] derived multiphase-flow-related expression suitable for any inclined angle in the lab, but the experimental flow media only included air and water, and the maximal experimental pipe diameter was only 1.5 inch, meaning its scope of application was restricted. Barnea et al (1986) [13] believed that laminar flow would not occur when the inclined angle was larger than 10°, but Shoham (1990) [14] believed that 20° inclined angle was the limit for the occurrence of laminar flow.

In the following decades, based on the previous research results, many researchers have committed to improving the accuracy of parameters related to multiphase flow and studying the temperature field of multiphase flow. On the basis of two-phase flow pattern classification method of Taitel and Barnea, Chen (1992) [15] proposed a comprehensive mechanical model for the flow characteristic of gas–liquid two-phase flow in vertical tubes. Barnea (1993) [16] proposed a model that can be applied to calculate the slug length of the slug flow at any desired position along the pipe. Liu (1995) [17] put forward a calculation method for multiphase flow in wellbore that is suitable for different reservoir types, including black oil, volatile oil, condensate gas, moisture, dry gas, etc. Liao (1998) [18] divided the multiphase flow into bubble flow, slug flow, turbulence flow, and annular flow and established the calculation formula of the split pressure gradient with higher accuracy compared to other correlations under the condition of high gas to oil ratio. Hibiki (1999) [19] held that the basic structure of the two-phase flow can be characterized by two fundamental geometrical parameters—known as void fraction and interfacial area concentration—and that the mechanism of the interfacial area transport depended on the bubble mixing length, turbulence intensity, and so on. Zhang (2001) [20] developed a new correlation for two-phase friction pressure drop in small diameter tubes by modifying the previous correlation. Hou (2004) [21] believed that the relevant parameters in the multiphase flow analysis of the wellbore are closely related to the well depth. On account of this, assuming that the calculation parameters in the microsegment are unchanged, a correlation model of the pressure gradient was established. Chen (2006) [22] investigated the flow boiling flow patterns in small diameters and sketched a new flow map that showed the transition boundaries of slug to churn and churn to annular flow depended strongly on the diameter. Yu (2008) [23] established a temperature field model for multiphase flow in a vertical wellbore based on multiphase flow dynamics and heat transfer theory. Cheng (2008) [24] researched the two-phase flow patterns in adiabatic and diabatic conditions and recommended that objective methods should be developed to gain more accurate flow pattern date and that the impact of the physical properties on flow pattern needed to be studied further. Liu (2009) [11] analyzed the flow pattern characteristics of inclined wellbore, considered that the mist flow model is more suitable for wells with high gas–liquid ratio, and established a new mist flow model. Yuichi Murai (2010) [25] designed three types of ultrasound interface detection techniques to capture the interface for two-phase flows, and the echo intensity technique and local Doppler technique were found to be appropriate for turbulent interfaces and bubbles, respectively. Gao (2014) [26] plotted the flow pattern of the vertical wellbore two-phase flow with the mixture Reynolds number and the ratio of the apparent velocity of the gas and liquid phase as the horizontal and vertical coordinates. Swanand (2014) [27] presented new equations for a flow-pattern-independent drift flux model that, in his opinion, is applicable to gas–liquid two-phase flow covering a wide range of pipe diameters. Zhou (2016) [28] studied the characteristic of high gas–liquid two-phase flow and found a new type of flow pattern called oscillatory impulse flow. Montoya (2016) [29] researched the characteristics of churn-turbulent flow and suggested computational fluid dynamics (CFD) models as an appropriate method for predicting highly turbulent flow. Liu (2017) [30] performed comprehensive work to model the flow regime transition criteria for upward two-phase flows in vertical rod bundles and proposed a new flow regime transition criteria model based on the analysis of the underlying physics of the upward two-phase flow behavior. Lu (2018) [31] studied the effects of flow regime, pipe size, and flow orientation on two-phase frictional pressure drop analysis and recommended that Lockhart–Martinelli approach be used to predict pressure drop.

In summary, there are still some defects in the research of multiphase flow. This includes: (1) The previous researches on pressure calculation methods of multiphase pipe flow mainly concentrated on horizontal and vertical pipes, and research on multiphase pipe flow under the conditions of different inclined angles is insufficient. (2) The available pressure calculation methods of multiphase pipe flow were mostly empirical and semiempirical methods and were all obtained within certain experimental scope; the calculation results were not always correct when exceeding the scope, and the application of these methods is therefore largely restricted. In particular, there are few experimental researches on the multiphase pipe flow under the conditions of large output from slanted well bores.

Therefore, in line with multiple well types, complicated casing program, various inclined angles, high production proration of single well, high gas–oil ratio (GOR > 100 m³/m³), low viscosity (1.2–5.7 cp), and heavy crude (19–29 API°) in the Middle East, it is necessary to conduct experimental research on multiphase pipe flow at high yield and different inclined angles. This will help find the flow patterns of gas and liquid in oil wells with high yield and different inclined angles accurately and optimize the multiphase flow pressure gradient computation method suitable for high-yield inclined pipes.

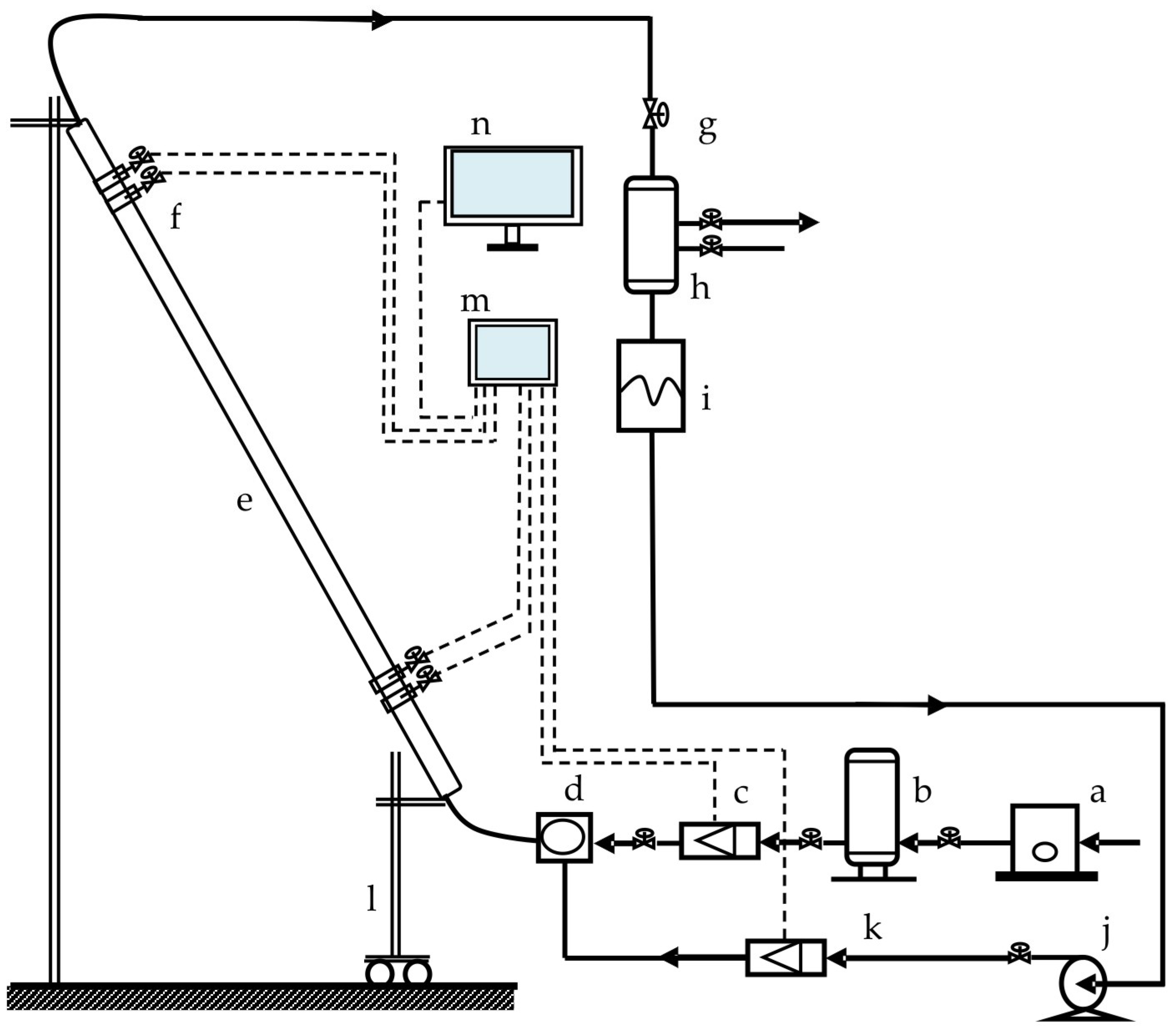
2. Experimental System of Multiphase Pipe Flow

2.1. Experimental Apparatus

The experimental apparatus was composed of the following parts: well bore simulation experiment interval, experimental fluid feeding system, experimental fluid measurement and control system, and experimental fluid flow rate and pressure acquisition system. The well bore simulation interval adopted a DN60 straight pipe, which was about 14 m long in total, 9.5 m long effectively, transparent, and visible. With the experimental apparatus, the oil, gas, and water can realize a multiphase flow in a pipe with 0–90° inclined angles, various flow patterns like bubble flow and mist flow can be observed, and parameters like flow rate, pressure differential pressure, liquid holdup, and temperature of multiphase flow fluid in the pipe can be measured. The fluid flow rate and pressure can be controlled manually or automatically. The experimental apparatus and its parameters are listed as in Figure 1 and

Table 1. Parameters of experimental apparatus.

Experimental Pipe	Atmospheric Pressure (0–0.8 × 104 KPa)	DN60 Straight Pipe
Experimental inclined angle	0–90°
Experimental media	Air, water
Maximum flow rate of media	water	20 m3/h
	Displacement of air compressor	35 m3/min
Measuring range of flow meter	water	0–20 m3/h
	Air	0–35 m3/min
Measuring accuracy of flow meter	water	±0.5%
	Air	±1%
Working pressure scope	Atmospheric pressure (×104 KPa)	0–0.8
Measuring accuracy of pressure gauge	Ordinary pressure signal: ±0.1%; pressure loss calculation interval: ±0.025–0.04%
Medium temperature	Atmospheric temperature −90 °C; measuring accuracy of temperature probe: ±0.5%
High speed camera	500 frame/s, 1920 × 1080 resolution, length of exposure: 1 μs, recording time ≥5 s

respectively.

With air and water as media and under conditions of the same pipe diameter, different inclined angles, and different fluid and gas rates, the experiments simulated the flow rules and pressure drawdown variation rules of gas–liquid two-phase flow in the well bore; tested the flow behavior, liquid holdup, and differential pressure at any inclined angle, well fluid, and gas flow rate; and then optimized and obtained the multiphase flow pressure prediction method suitable for middle and high-yield slanted well bores.

2.2. Experimental Contents

2.2.1. Preparation before Experiment

• Before the commencement of the experiment, the pipeline airtightness and clearance of process pipeline pathway were firstly checked. Then, it was checked whether the compressor, water pump, each instrument, and recording software could normally work.
• Experimental contents were determined, and the inclined angles of test pipes were selected based on the testing program.
• The test stand was raised with a hoist; when a required angle was attained, the lifting hoist was stopped.
• The air inlet and outlet valve switches of the selected test pipe were opened, and the valves of other test pipes were closed.
• Inspection was repeated to ensure the process was correct, the experimental pipeline ports were open, and there was no pressure buildup phenomenon.

2.2.2. Experimental Procedure

• The software system of the console was opened, and the testing string inclination was adjusted.
• The air compressor was started, and the water pump was opened on the console.
• The fluid and gas volumes entering the string were adjusted. The gas volume was adjusted by slowly adjusting the opening of the air inlet valve, whereas the fluid volume was adjusted by slowly adjusting the pump frequency and the opening of the reflux valve; simultaneously, the instrument readings on the console were observed until the target values were reached.
• After the target values were reached, the experimental phenomena were observed, and the pressure, differential pressure, temperature, fluid volume, and gas volume displayed on the instrument were recorded.
• The experimental data recording time range was set, the test data was saved, a high speed camera was used to take photographs, and the fluid flow patterns in the pipe were recorded and saved.
• After the gas and liquid in test string was stopped with quick closing valve and the liquid was still, the height of liquid in the plexi-glass tubular was read, and the liquid holdup was figured out.
• The gas volume and the liquid volume in the testing string as well as the inclined angle of the string were readjusted. Then, the above procedures were repeated, and the gas flow rate, liquid flow rate, pressure, differential pressure, temperature, flow behavior, and liquid holdup tested at different inclined angles were recorded.
• After the experiment was finished, the water pump and air compressor were shut off, the test stand was placed horizontally, and the power switches of the computer and console were turned off.

2.2.3. Experimental Parameters

The influence of inclined angle, liquid and gas volume on two-phase flow was analyzed. And the specific experimental parameters are shown in

Table 2. Parameters of experiment.

Variable	Parameter
Pipe diameter (mm)	60
Inclined angle (°)	0, 15, 30, 45, 60, 75, 90
Liquid volume (m3/day)	50, 100, 150, 200, 250, 300, 350, 400
Gas volume (m3/day)	5000, 10,000, 15,000, 20,000, 25,000, 30,000, 48,000

.

3. Experimental Analysis

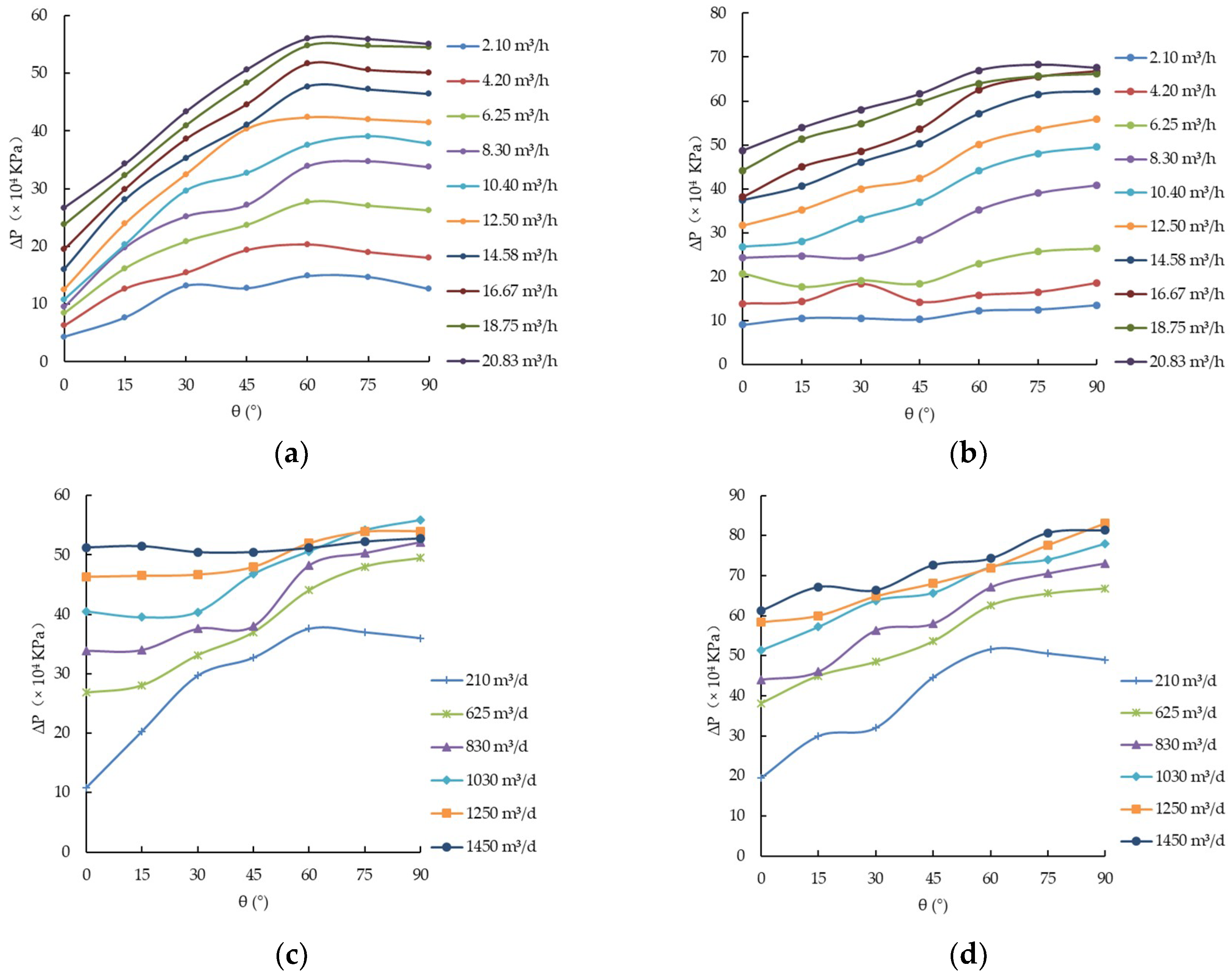
3.1. Analysis of Factors Affecting Pressure Drawdown

Figure 2a shows that when the liquid volume ranged 2.10–20.83 m³/h and the gas volume was higher than or equaled 210 m³/h, the pressure drawdown firstly rose and then reduced with the increase of the inclined angle; when the gas volume was 625 m³/h, the pressure drawdown rose with the increase of the inclined angle. The leading cause for this was that when the inclined angle ranged 0–60°, the pressure drawdown of gravity term gradually increased, and the total pressure drawdown increased with the increase of the inclined angle. When the inclined angle was higher than 60°, although the pressure drawdown of gravity term also gradually increased, the liquid carrying capability of gas increased, the liquid holdup reduced (also shown in the liquid holdup map below), and the total pressure drawdown reduced with the increase of the inclined angle.

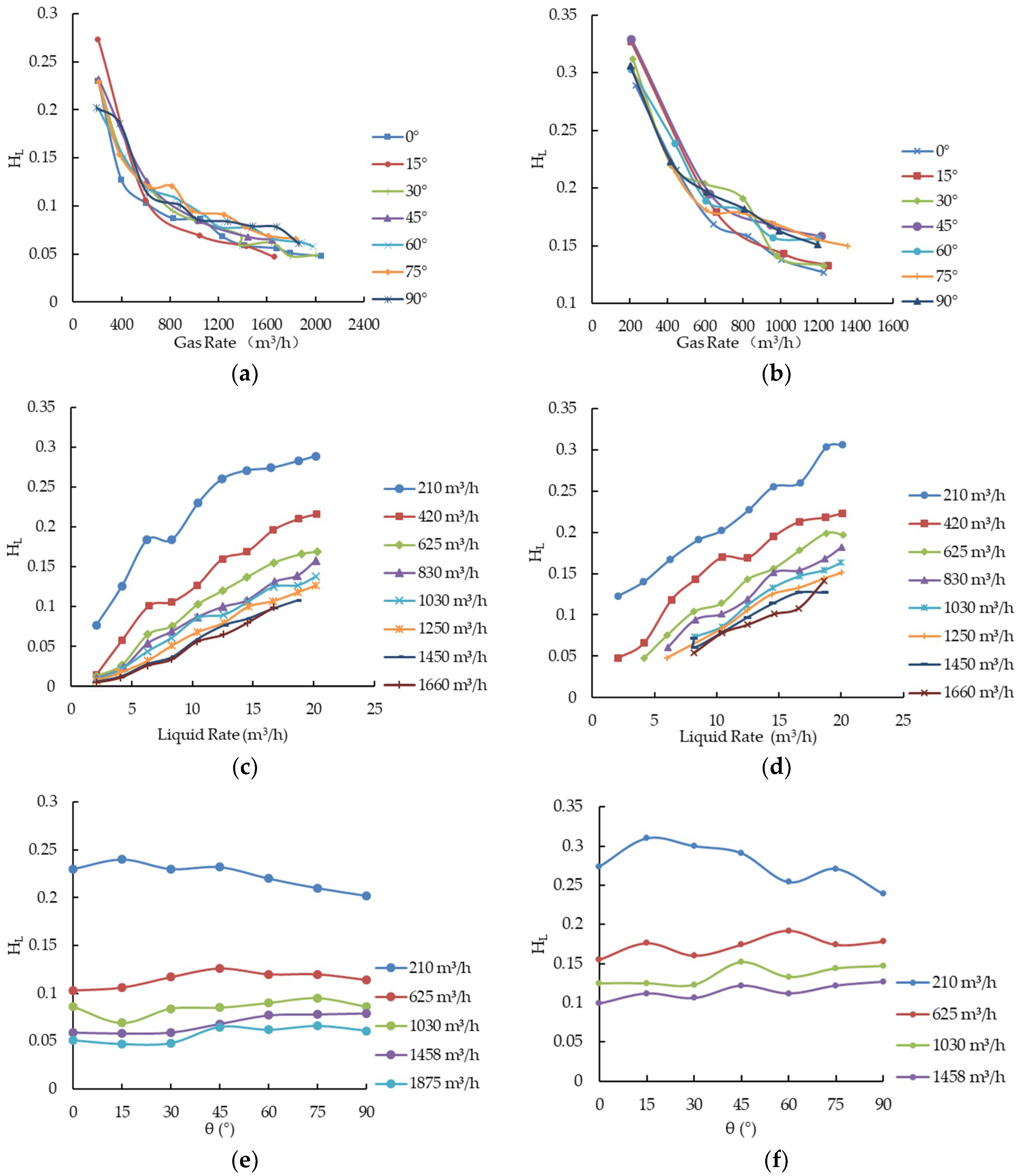
3.2. Analysis on Influential Factors of Liquid Holdup

Under the conditions of the same liquid volume and gas volume, the variation rule of liquid holdup with different inclined angles is shown in Figure 3. At the same liquid volume, as the gas volume increased, the liquid holdup fell. In particular, the liquid holdup fell faster when the gas injection rate was 200–700 m³/h, but the liquid holdup fell more slowly when the gas injection rate was higher than 700 m³/h. At the same gas injection rate, as the liquid volume increased, the liquid holdup rose. At the same liquid volume and gas volume, the liquid holdup exhibited a trend of firstly rising and then falling with the increase of the inclined angle; however, observed from the total liquid volume (at gas injection rate of more than 210 m³/h), the liquid holdup changed little at 0–90°.

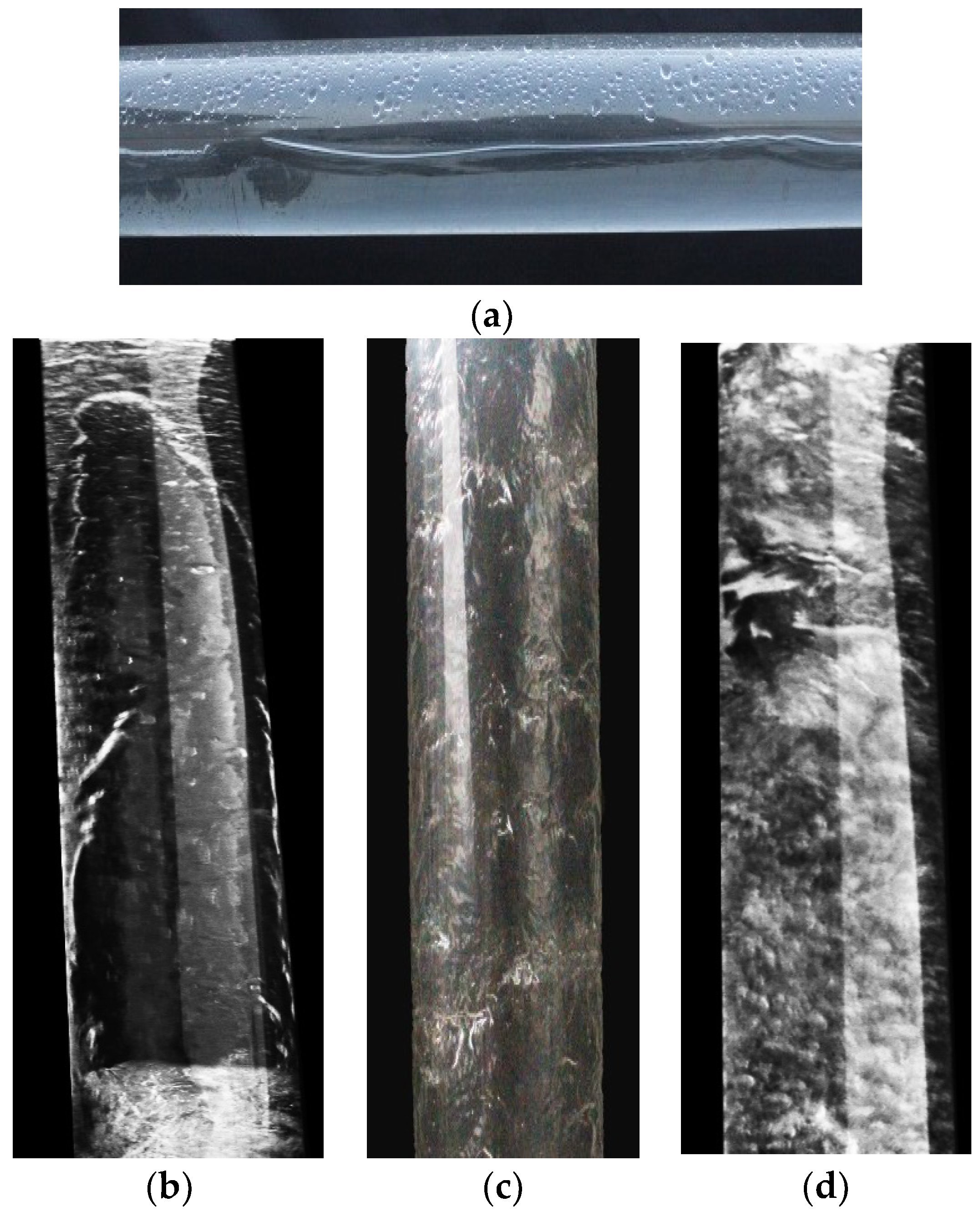
3.3. Flow Pattern Variation at Different Inclined Angles

Under the experimental conditions, when the gas volume was small, laminar flow occurred, but no laminar flow occurred in the inclined state. In the horizontal and inclined states, the most common flow patterns were slug flow and transition flow; annular flow was rare, and no bubble flow or mist flow occurred.

In the horizontal state, only laminar flow, slug flow and transition flow occurred; furthermore, laminar flow and transition flow occurred only under the conditions of very small or very large gas volume, and slug flow appeared in most cases. At the same liquid volume, as the gas volume increased, in the experiment of horizontal state, the flow pattern converted from laminar flow to slug flow and then to transition flow.

With the increase of inclination angle of the tube, due to the action of gravity, laminar flow no longer occurred at 15°, 30°, 45°, 60°, 75°, and 90°. At the same liquid volume, as the gas volume increased, in the experiment of inclined angles of 15°, 30°, 45°, 60°, 75°, and 90°, the flow pattern tended to convert from slug flow to transition flow and then to annular flow.

The typical flow patterns observed in the experiment are shown as in Figure 4.

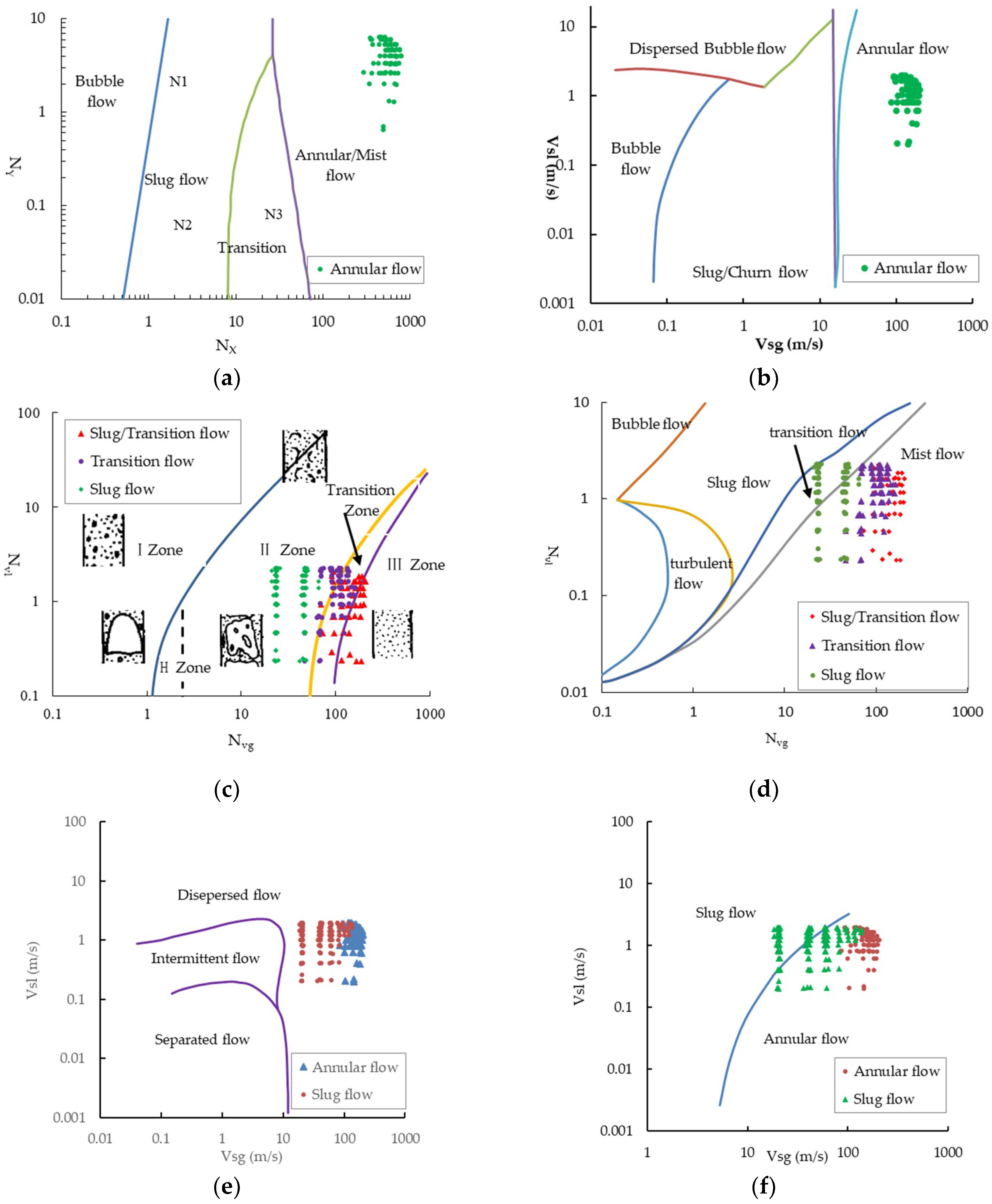
3.4. Verification of Flow Pattern Maps

At present, in the identification of multiphase flow patterns, the commonly used empirical flow pattern maps include Duns and Ros flow pattern map [7], Hewitt and Roberts flow pattern map [32], Aziz flow pattern map [10], Gould flow pattern map [33], Ansari flow pattern map [34], Beggs–Brill flow pattern map [12], and Mukherjee flow pattern map [1]. In this study, based on the experimental data, the identification effects of the above flow pattern maps were verified, and Figure 5 shows the verification results as follows:

• For the annular flow, Beggs–Brill flow pattern map, Mukherjee flow pattern map, and Aziz flow pattern map were the most accurate, i.e., out of the 469 groups of experiments, all the annular flows were consistent with the flow pattern maps; however, in the Ansari flow pattern map and Hewitt and Roberts flow pattern map, most of the experimental data points exceeded their estimation range, indicating that the experiments in this study exceeded the application scope of these two flow pattern maps.
• For the slug flow, the Mukherjee flow pattern map and Duns and Ros flow pattern map were the most accurate, i.e., at inclined angles of 0–90°, the judgment accuracy reached 80–100%; for the transition flow, the Duns and Ros flow pattern map was the most accurate, with an accuracy of 46–66%.

3.5. Verification of Liquid Holdup and Pressure Drawdown

As shown in Figure 6a, for the calculation of liquid holdup, when the inclined angle was between 0° and 15°, the calculation results of Beggs and Brill were more accurate, and when the inclined angle ranged between 30 and 90°, the calculation results of Mukherjee and Beggs were more accurate. As shown in Figure 6b, for the calculation of pressure drawdown, when the inclined angle ranged from 0° to 60°, the calculation results of Aziz were more accurate, when the inclined angle was between 75° and 90°, the calculation results of Hasan [35] were more accurate, and when the inclined angle ranges from 0 to 90°, the JPI model [18] was the most stable.

3.6. New Model for Calculating Liquid Holdup and Pressure Drawdown

3.6.1. Simulation of the New Model

Beggs–Brill (Mukherjee adopted the same calculation method) [1] presented the calculation equation of total pressure drawdown:

$$-\frac{\mathrm{dp}}{\mathrm{dz}}=\frac{\left[{\rho }_{\mathrm{l}}{H}_{\mathrm{l}}+{\rho }_{\mathrm{g}}\left(1-H_{\mathrm{l}}\right)\right]\mathrm{g}\sin \theta +\frac{\lambda Gv}{2DA}}{1-\left\{\left[{\rho }_{\mathrm{l}}{H}_{\mathrm{l}}+{\rho }_{\mathrm{g}}\left(1-H_{\mathrm{l}}\right)\right]v{v}_{\mathrm{sg}}\right\}/p}$$

(1)

The calculation equation of total pressure gradient shows that at the time of calculating the total pressure gradient of gas–liquid two-phase flow, the calculation of liquid holdup H_l and resistance coefficient λ along the tube must be mastered. In the study, the experimental data was used to match the calculation methods of liquid holdup and resistance coefficient along the tube, respectively.

1. Simulation of liquid holdup

Based on experimental data and regression analysis, Mukherjee presented the law of liquid holdup for gas and liquid inclined pipe flow, i.e., [1],

$$H_{\mathrm{l}}=\exp \left[\left(c_1+c_2\sin \theta +c_3{\sin }^2\theta +c_4{N}_{\mathrm{l}}{}^2\right)\frac{N_{\mathrm{vg}}{}^{c_5}}{N_{\mathrm{vl}}{}^{c_6}}\right]$$

(2)

$$N_{\mathrm{vl}}=v_{\mathrm{sl}}{(\frac{{\rho }_{\mathrm{l}}}{g\sigma })}^{0.25}$$

(3)

$$N_{\mathrm{vg}}=v_{\mathrm{sg}}{(\frac{{\rho }_{\mathrm{l}}}{g\sigma })}^{0.25}$$

(4)

$$N_{\mathrm{l}}={\mu }_{\mathrm{l}}{(\frac{\mathrm{g}}{{\rho }_{\mathrm{l}}{\sigma }^3})}^{0.25}$$

(5)

where ν_sl is the apparent velocity of liquid phase (m/s); ν_sg is the apparent velocity of gas phase (m/s); σ is the surface tension of liquid phase (N/m); μ_l is the viscosity of liquid phase (mPa·s); c₁–c₆ are all empirical constants.

The relationship between the experimental liquid holdup H_l and the correlated numbers N_vl, and N_l presented by Mukherjee were matched, and the obtained new empirical constants are listed in

Table 3. Empirical constants.

c1	c2	c3	c4	c5	c6
−0.592	0.0236	−0.011	0.063	0.470	0.177

.

2. Simulation of resistance coefficient along the tube

Referring to the Mukherjee’s calculation method for resistance coefficient of gas–liquid two-phase flow along the tube [1], the resistance coefficient λ along the tube is the function of no slip resistance coefficient along the tube ƒ_m, i.e., the resistance coefficient along the tube is calculated as:

$$\mathsf{\lambda }=f_{\mathrm{r}}{f}_{\mathrm{m}}$$

(6)

In this study, the relation of friction coefficient ƒ_r worked out with experimental data is as follows:

$$f_{\mathrm{r}}=\left(4.326\sin \theta +10.284\right)H_{\mathrm{r}}$$

(7)

in which the relative liquid holdup H_r is as follows:

$$H_{\mathrm{r}}={H_{\mathrm{l}}}^{\prime }/H_{\mathrm{l}}$$

(8)

$$H_{\mathrm{l}}^{\prime }=\frac{v_{\mathrm{sl}}}{v_{\mathrm{sl}}+v_{\mathrm{sg}}}$$

(9)

where ν_sg is the apparent velocity of gas (m/s); ν_sl is the apparent velocity of liquid (m/s); and H_l is the liquid holdup.

The no slip resistance coefficient along the tube ƒ_m is calculated directly using the equation presented by Mukherjee:

$$\mathrm{R}{e}_m\le 2300,f_{\mathrm{m}}=\frac{64}{R_{e_m}}$$

(10)

$$\mathrm{R}{e}_m＞2300,f_{\mathrm{m}}={[1.14-21\mathrm{g}\left(\frac{k}{D}+\frac{21.25}{\mathrm{R}{e}_m{}^{0.9}}\right)]}^{-2}$$

(11)

in which the no slip Reynolds number is as follows:

$$\mathrm{R}{e}_m=\frac{v_{\mathrm{m}}{\rho }_{\mathrm{m}}D}{{\mu }_{\mathrm{ns}}}$$

(12)

The no slip mixture density is as follows:

$${\rho }_{\mathrm{m}}=\left(1-H_{\mathrm{l}}^{\prime }\right){\rho }_{\mathrm{g}}+{H_{\mathrm{l}}}^{\prime }{\rho }_{\mathrm{l}}$$

(13)

where ρ_l is the density of liquid phase (kg/m³); ρ_g is the density of gas phase (kg/m³).

Viscosity of no slip mixture is as follows:

$${\mu }_{\mathrm{ns}}=\left(1-H_{\mathrm{l}}^{\prime }\right){\mu }_{\mathrm{g}}+{H_{\mathrm{l}}}^{\prime }{\mu }_{\mathrm{l}}$$

(14)

3.6.2. Comparison of Calculation Errors

The new model and six commonly used pressure calculation models were adopted to predict the pressure gradient under experimental conditions, and the experimental results were then compared.

Table 4. Comparison of prediction errors of models.

Models	Beggs	Mukherjee	Aziz	Hasan	JPI	Orkiszewski	New Models
Errors (%)	30.3	68.3	71.7	66.8	34.1	38.1	11.3

shows that the error of the new model was 11.3%, suggesting it had a better prediction effect than the six commonly used pressure calculation methods.

4. Conclusions

By analyzing and summing up the variation law of flow pattern, pressure drawdown, and liquid holdup with the output and gas injection rate at the tube inclined angles of 0°, 15°, 30°, 45°, 60°, 75°, and 90° as well as conducting verification analysis on several multiphase flow prediction methods, the following conclusions have been drawn:

• At the same liquid volume, as the gas volume increases, the flow pattern in the horizontal state tends to convert from laminar flow to slug flow and then to transition flow, whereas in the inclined state, the flow pattern tends to convert from slug flow to transition flow and then to annular flow.
• Under the experimental conditions, the Beggs–Brill flow pattern map, Mukerherjee flow pattern map, and Aziz flow pattern map are the most accurate for the judgment of annular flow, with an accuracy of 100%; the Mukherjee flow pattern map and Duns and Ros flow pattern map have a 80–100% accuracy in judging slug flow; and the Duns and Ros flow pattern map has a 46–66% accuracy in identifying transition flow.
• The liquid holdup and pressure drawdown are both affected by the gas injection rate, liquid volume, and inclined angle. When the inclined angle ranges 0–60°, the pressure drawdown increases with the increase of inclined angle; when the inclined angle exceeds 60°, the pressure drawdown reduces with the increase of inclined angle.
• Under the experimental conditions, the errors of six pressure drawdown prediction models are all bigger; therefore, a pressure drawdown model with new coefficients has been matched, with an error of only 11.3%.