Impact of Casing Eccentricity on Cement Sheath
Abstract
1. Introduction
2. Field Observations
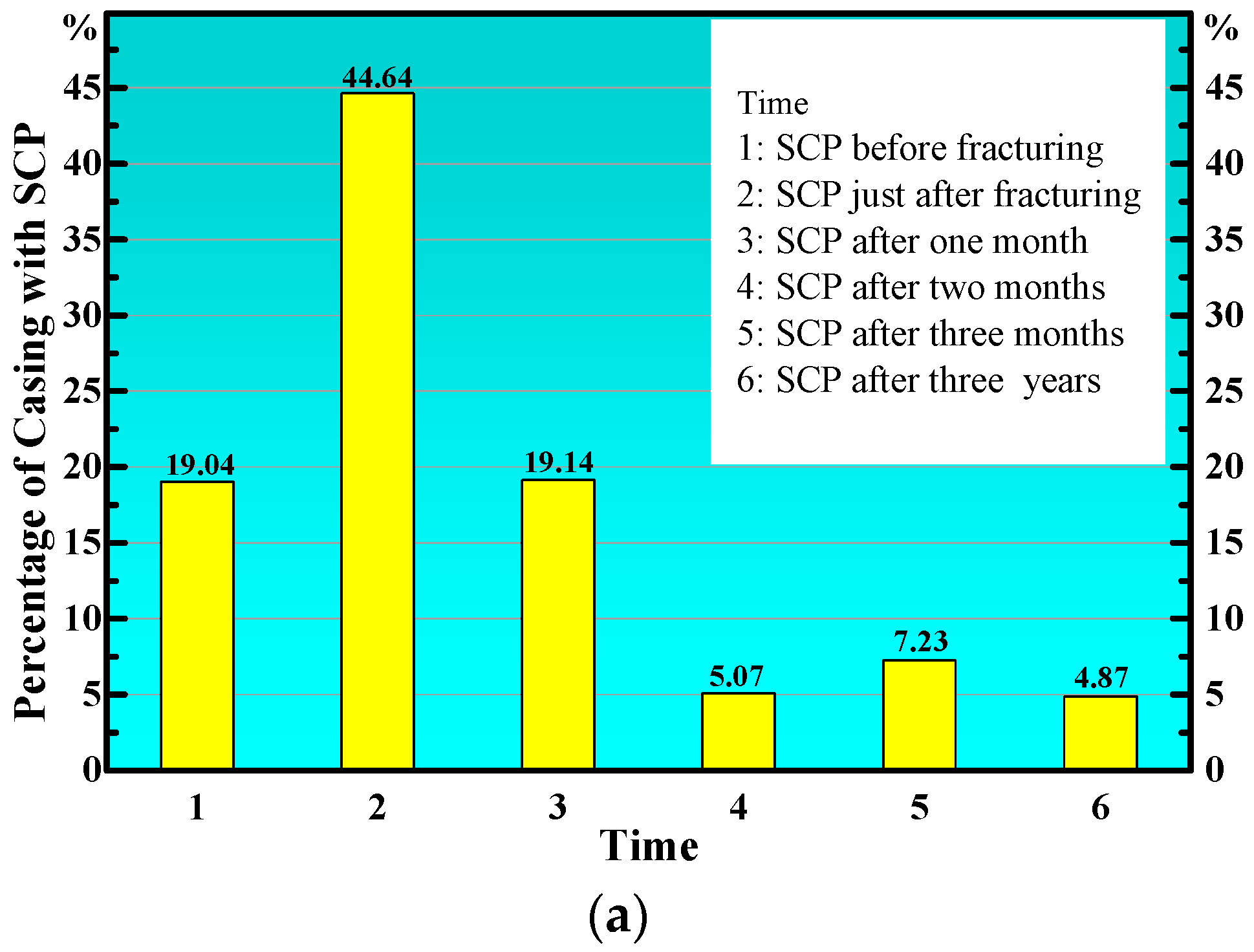
2.1. Sustained Casing Pressure in Shale Gas Wells
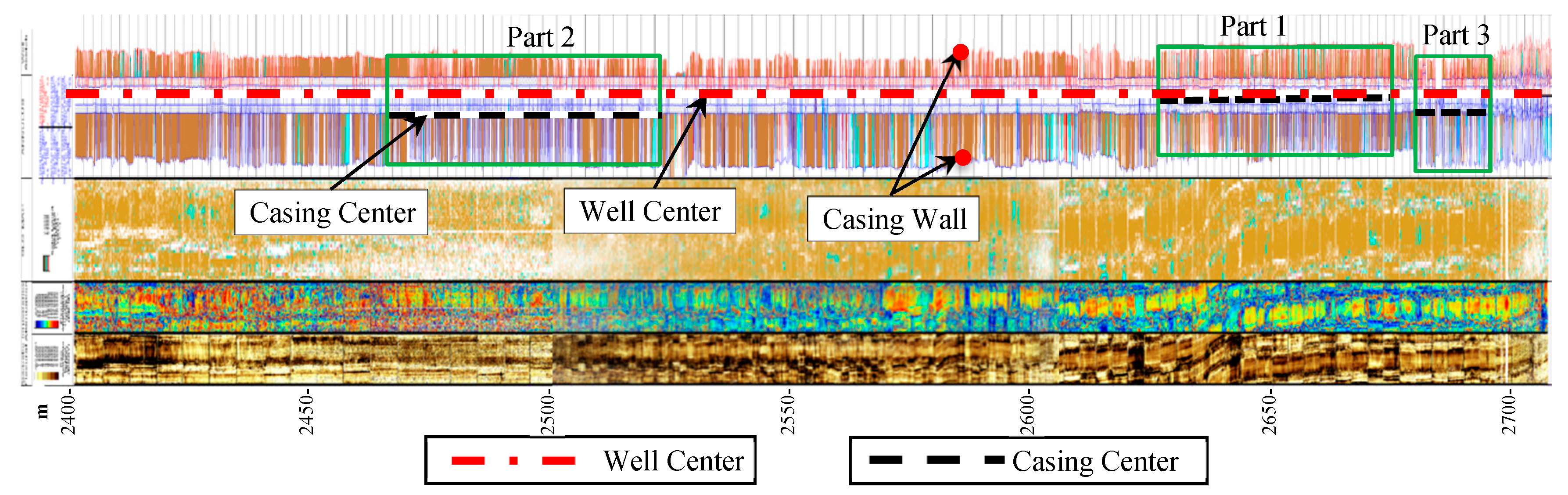
2.2. Casing Eccentricity in Shale Gas Wells
3. Mechanic Model
3.1. Stress on Interfaces
3.2. Stress in Cement Sheath
- Tectonic stresses are assumed to be isotropic;
- As we focus on analyzing the effect of eccentricity on the stress of the cement sheath, the external loads on the surfaces of the cement sheath are treated to be equal to the stress calculated according to Equation (1);
- The cementing job is well done and no discontinuity exists in the cement sheath;
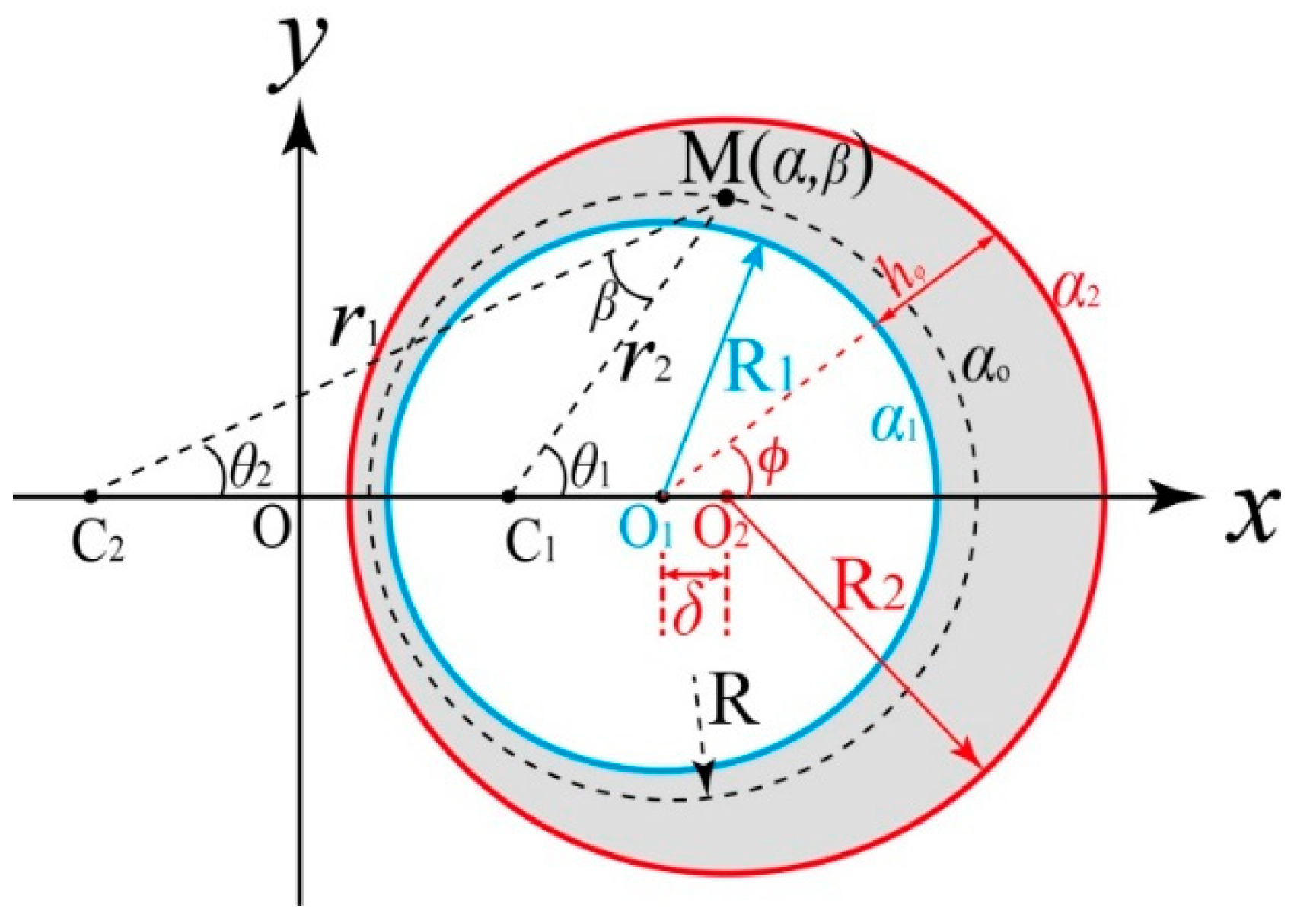
3.2.1. Mechanistic Model of an Eccentric Cement Sheath
3.2.2. Model Accuracy Verification
4. Stress in an Eccentric Cement Sheath
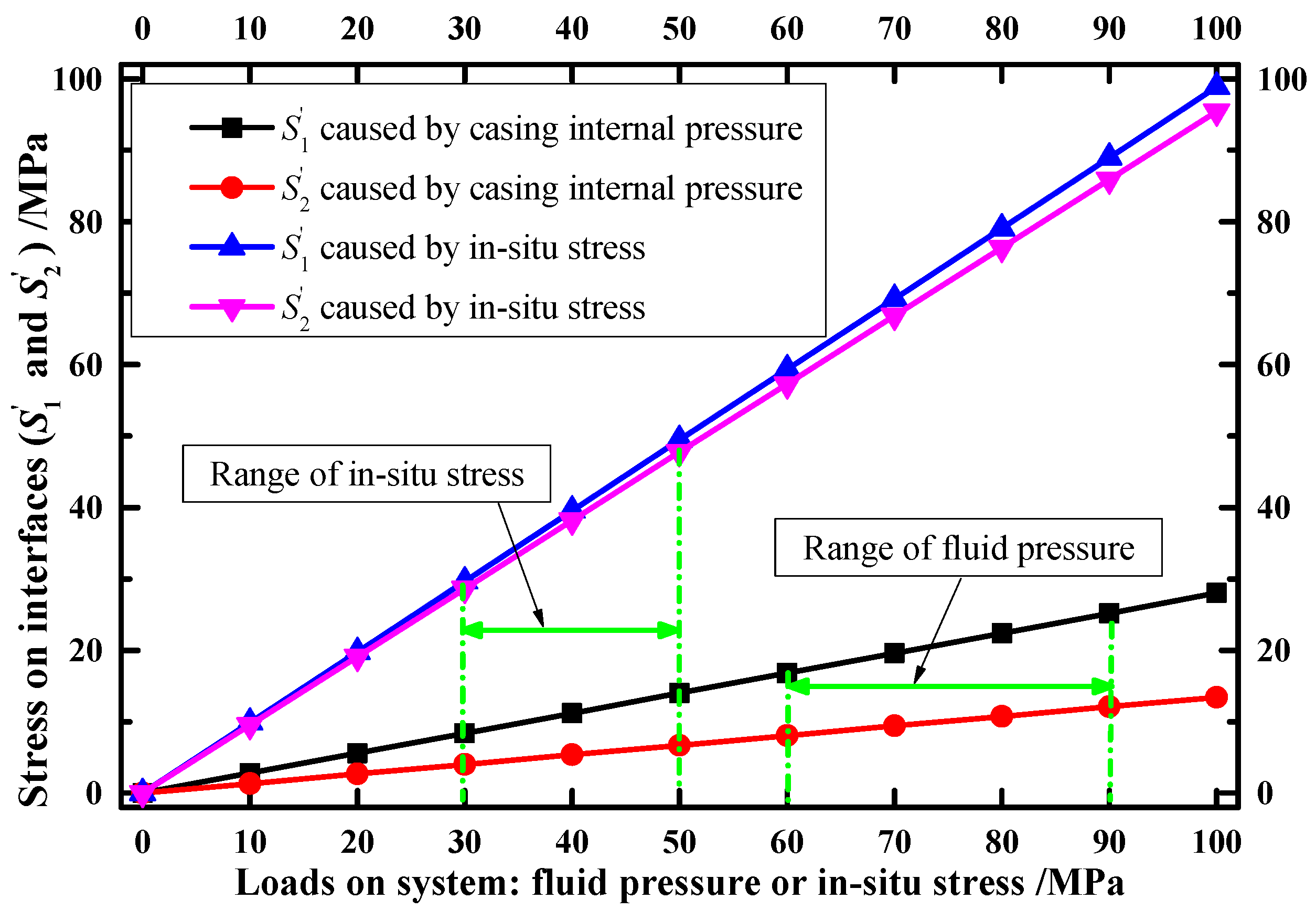
4.1. Contribution of Crustal Loads
4.1.1. Variation of Circumferential Stress with Radius
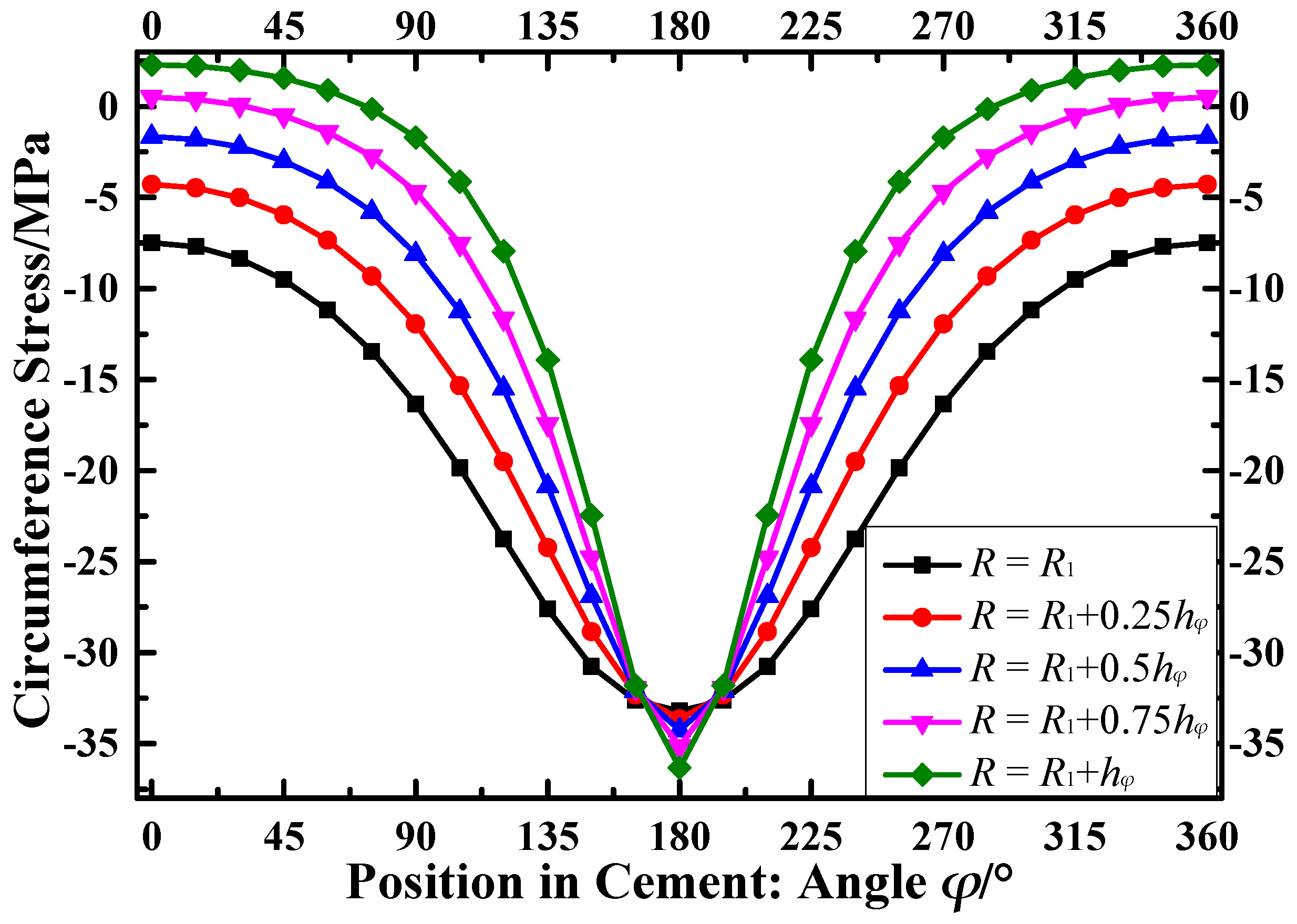
4.1.2. Variation of Circumferential Stress with Eccentricity
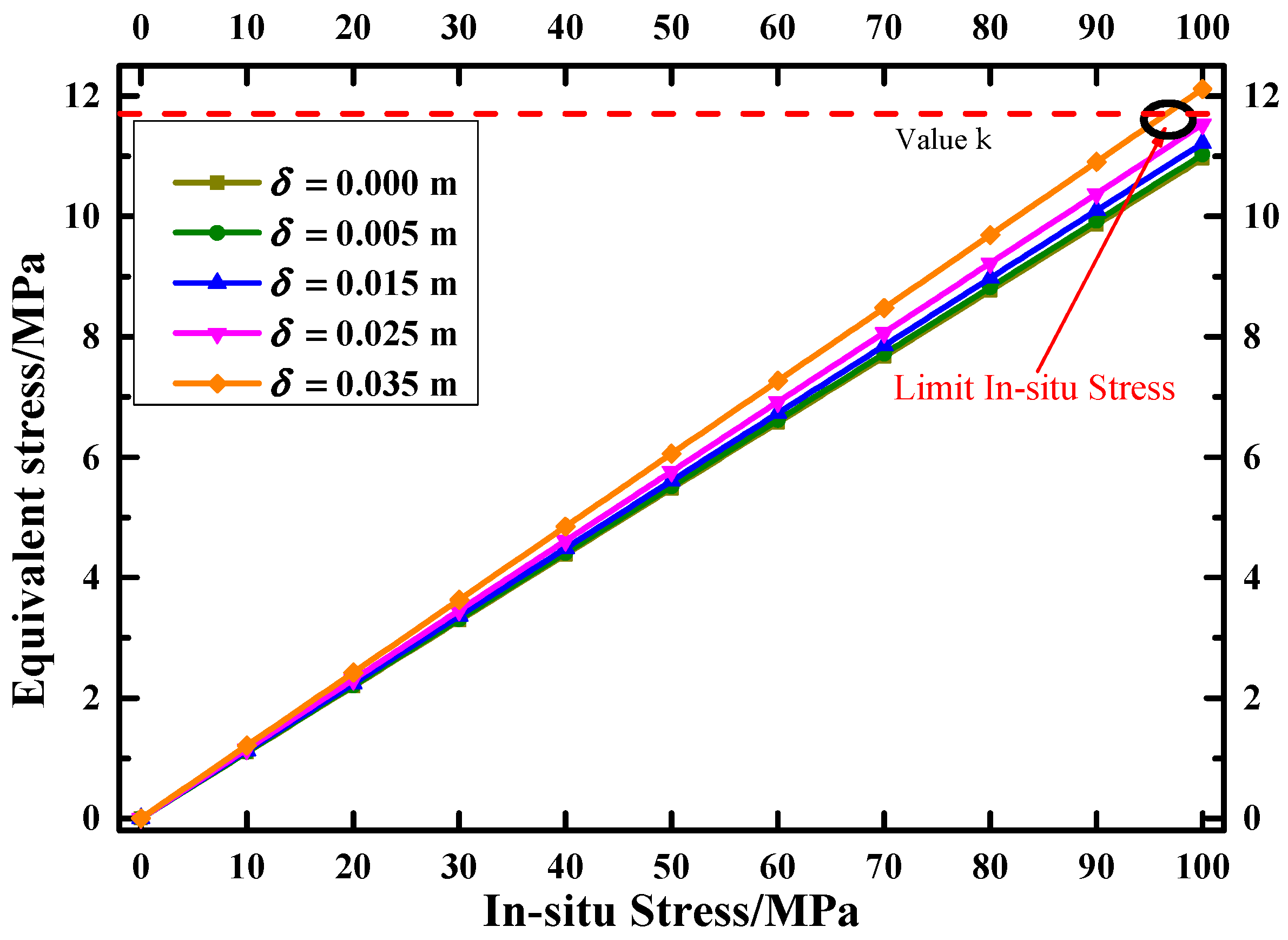
4.1.3. Equivalent Stress
4.2. Contribution of Fluid Pressure
4.2.1. Variation of Circumferential Stress with Radius
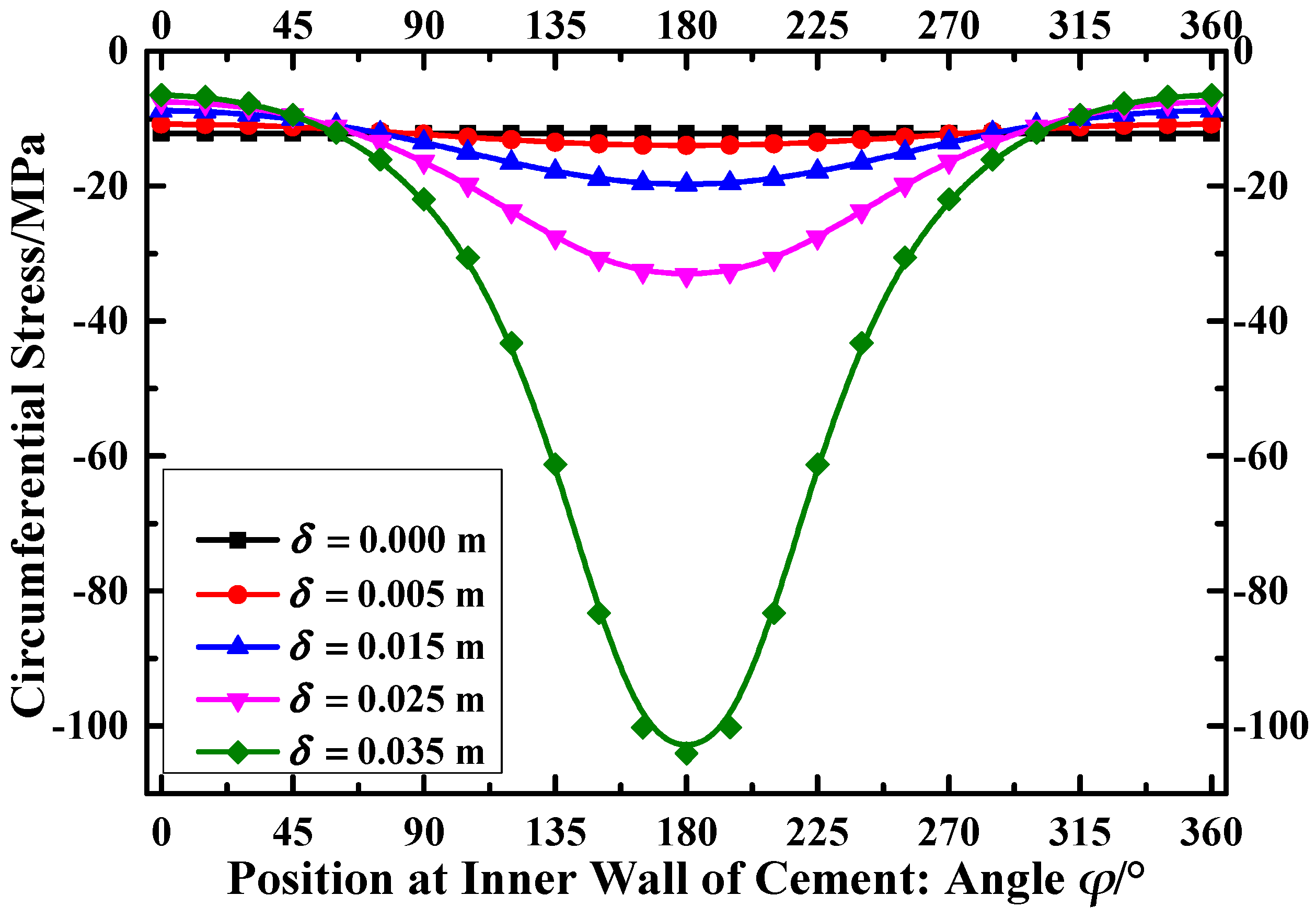
4.2.2. Variation of Circumferential Stress with Eccentricity
4.2.3. Equivalent Stress
5. Sensitivity Analysis and Case Study
5.1. Casing Thickness
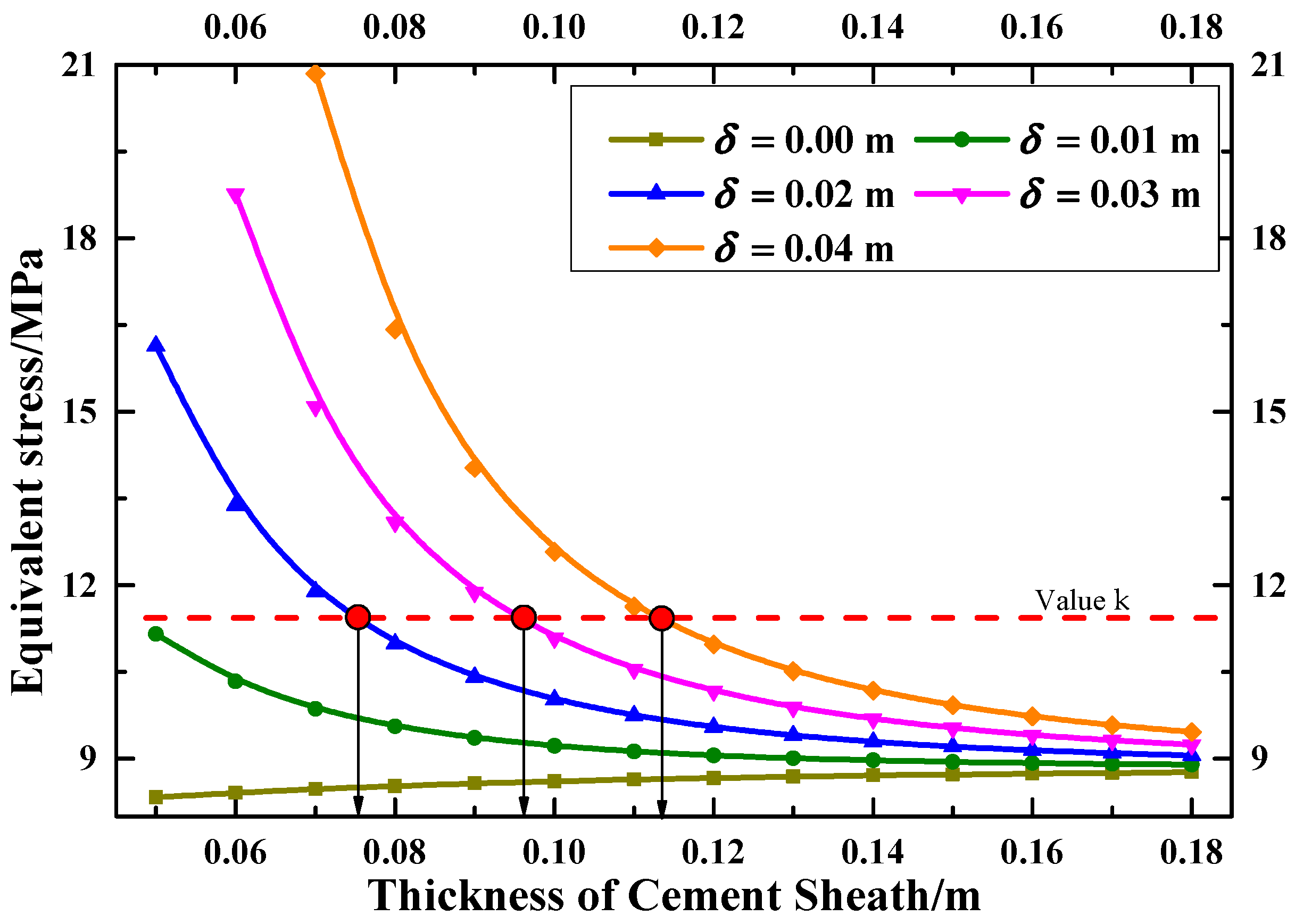
5.2. Thickness of the cement sheath
5.3. Elastic Modulus of Cement Sheath
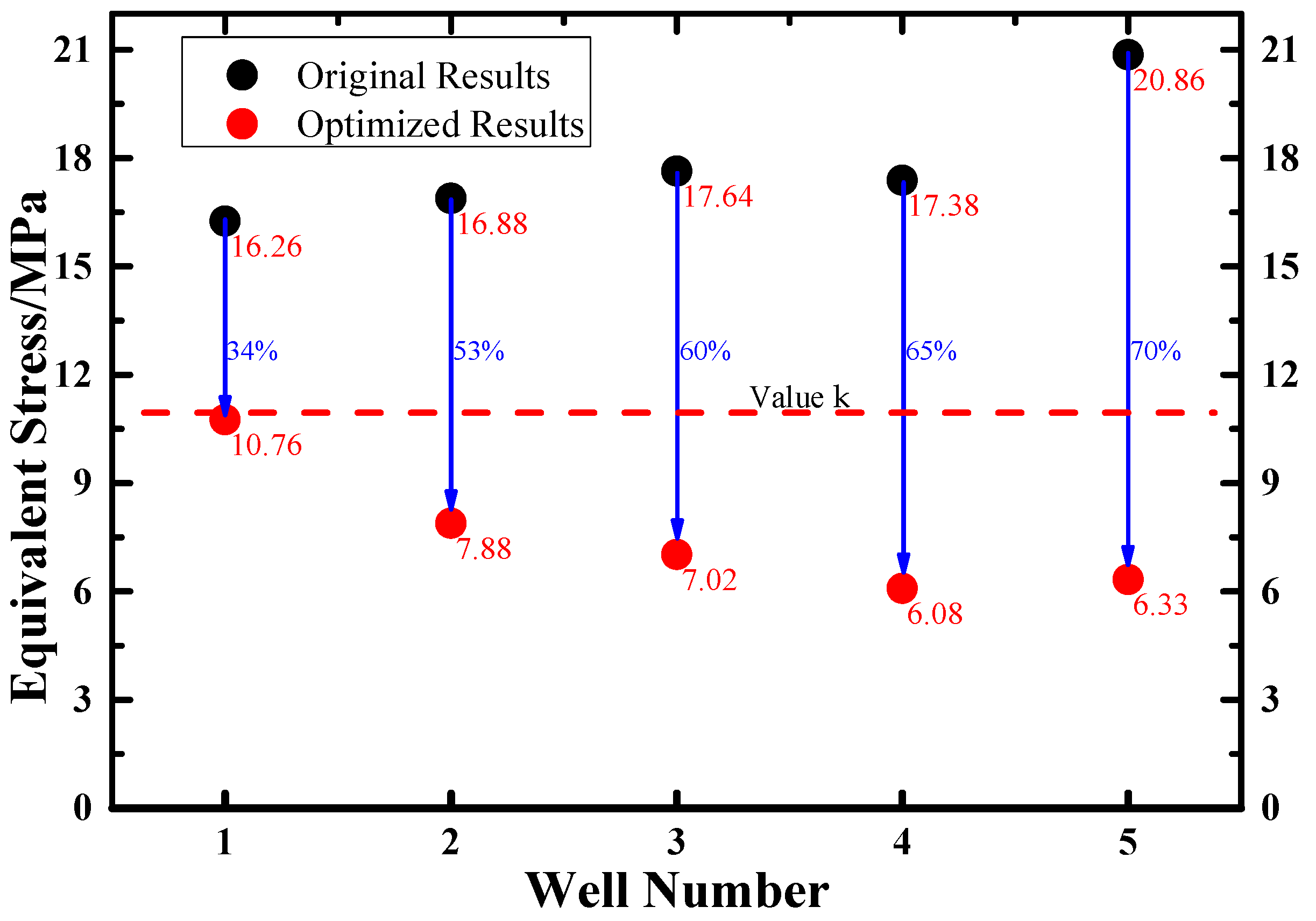
5.4. Case Study
6. Conclusions
- (1)
- By employing the bipolar coordinate system, a method for calculating stress in the cement sheath I proposed that considers casing eccentricity. The analytical model is verified by FEA.
- (2)
- The casing eccentricity will dramatically increase stress in the cement sheath and expedite cement sheath failure. It is important to decrease the risk of cement failure by improving casing centralization, and increasing casing thickness.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Fluid pressure in casing | |
| Crustal stress | |
| Radial stress on first interface and second interface with no casing eccentricity | |
| , | Radial stress on first interface and second interface with casing eccentricity |
| λ | positive real number |
| α, β | Coordinate axes of the bipolar coordinate system |
| R | Radius of this circle in cement sheath |
| hφ | Thickness of the cement sheath at any section |
| ζ | Bipolar coordinate variable |
| ξ, η | Bipolar coordinate component |
| r1 | Radius of circle α1 |
| r2 | Radius of circle α2 |
| Radial stress in cement sheath | |
| Circumferential stress in cement sheath | |
| Shear stress in cement sheath | |
| k | Shear yield strength of cement sheath |
Appendix A
References
- Thiercelin, M.J.; Dargaud, B.; Baret, J.F.; Rodriguez, W.J. Cement design based on cement mechanical response. Soc. Pet. Eng. 1998, 13, 266–273. [Google Scholar]
- Dusseault, M.B.; Gray, M.N.; Nawrocki, P.A. Why oilwells leak: Cement behavior and long-term consequences. In Proceedings of the International Oil and Gas Conference and Exhibition in China, Beijing, China, 7–10 November 2000. [Google Scholar]
- Zhu, H.Y.; Deng, J.G.; Zhao, J.; Zhao, H.; Liu, H.L.; Wang, T. Cementing failure of the casing-cement-rock interfaces during hydraulic fracturing. Comput. Concr. 2014, 14, 91–107. [Google Scholar] [CrossRef]
- Taleghani, A.D.; Klimenko, D. An analytical solution for microannulus cracks developed around a wellbore. J. Energy Resour. Technol. 2015, 137. [Google Scholar] [CrossRef]
- Wang, W.; Taleghani, A.D. Impact of hydraulic fracturing on cement sheath integrity: A modeling approach. J. Nat. Gas Sci. Eng. 2017, 44, 265–277. [Google Scholar] [CrossRef]
- Chu, W.; Shen, J.; Yang, Y.; Li, Y.; Gao, D.L. Calculation of micro-annulus size in casing-cement sheath-formation system under continuous internal casing pressure change. Pet. Explor. Dev. 2015, 42, 414–421. [Google Scholar] [CrossRef]
- Goodwin, K.J.; Crook, R.J. Cement sheath stress failure. SPE Drill. Eng. 1992, 7, 291–296. [Google Scholar] [CrossRef]
- Fang, J.; Gu, Y.H.; Mi, F.Z. A numerical analysis of casing collapse under non-uniform load. China Pet. Mach. 1999, 27, 34–37. [Google Scholar]
- Wang, W.; Taleghani, A.D. Three-dimensional analysis of cement sheath integrity around Wellbores. J. Pet. Sci. Eng. 2014, 121, 38–51. [Google Scholar] [CrossRef]
- Wang, Y.B.; Gao, D.L.; Fang, J. Mechanical characteristics analysis of casing-cement ring-formation multilayer composite system. Chin. J. Appl. Mech. 2014, 31, 387–392. [Google Scholar]
- Fan, M.; Li, J.; Liu, G. New method to analyse the cement sheath integrity during the volume fracturing of shale gas. Energies 2018, 11, 750. [Google Scholar] [CrossRef]
- Kim, J.; Moridis, G.J.; Martinez, E.R. Investigation of possible wellbore cement failures during hydraulic fracturing operations. J. Pet. Sci. Eng. 2016, 139, 254–263. [Google Scholar] [CrossRef]
- De Andrade, J.; Sangesland, S. Cement sheath failure mechanisms: Numerical estimates to design for long-term well integrity. J. Pet. Sci. Eng. 2016, 147, 682–698. [Google Scholar] [CrossRef]
- De Andrade, J.; Torsæter, M.; Todorovic, J.; Opedal, N.; Stroisz, A.; Vralstad, T. Influence of casing centralization on cement sheath integrity during thermal cycling. In Proceedings of the IADC/SPE Drilling Conference and Exhibition, Fort Worth, TX, USA, 4–6 March 2014. [Google Scholar]
- Tao, Q.; Chen, X. Causal analysis and countermeasures on B sustained casing pressure of shale-gas horizontal wells in the Sichuan Basin. Oil Drill. Prod. Technol. 2017, 39, 588–593. [Google Scholar]
- Wu, J.L. Elastic Mechanics, 2nd ed.; Higher Education Press: Beijing, China, 2011. [Google Scholar]
- Liu, K.; Wang, Y.B.; Gao, D.L.; Li, X.Y.; Zhang, Y. Effects of hydraulic fracturing on horizontal wellbore for shale gas. Acta Petrolei Sinica 2016, 37, 406–414. [Google Scholar]
- Liu, K.; Gao, D.L.; Taleghani, A.D. Analysis on integrity of cement sheath in the vertical section of wells during hydraulic fracturing. J. Pet. Sci. Eng. 2018, 168, 370–379. [Google Scholar] [CrossRef]
- Timoshenko, S.; Goodier, J.N. Theory of Elasticity, 2nd ed.; Mcgraw Hill: New York, NY, USA, 1951. [Google Scholar]
- Jeffery, G.B. IX. Plane stress and plane strain in bipolar co-ordinates. Philos. Trans. R. Soc. Lond. A 1921, 221, 265–293. [Google Scholar] [CrossRef]
- Liu, K.; Gao, D.L.; Zeng, J.; Fang, J.; Wang, Y.B. Annulus pressure analysis of a shale gas well under varied temperatures and pressures. Pet. Drill. Technol. 2017, 45, 8–14. [Google Scholar]
- Liu, K.; Gao, D.L.; Wang, Y.B.; Yang, Y.C. Effect of local loads on shale gas well integrity during hydraulic fracturing process. J. Nat. Gas Sci. Eng. 2017, 37, 291–302. [Google Scholar] [CrossRef]
- Jun, L.; Mian, C.; Gonghui, L.; Hui, Z. Elastic-plastic analysis of casing-concrete sheath-rock combination. Acta Petrolei Sinica 2005, 26, 99–103. [Google Scholar]








| Outer Diameter/m | Thickness/mm | Elastic Modulus/GPa | Poisson Ratio | |
|---|---|---|---|---|
| Casing | 0.1397 | 12.14 | 210 | 0.25 |
| Cement | 0.2223 | 41.28 | 20 | 0.3 |
| Formation | ≥10 | ≥10 | 25 | 0.3 |
| ID (m) | Thickness (m) | Elastic Modulus (GPa) | Poisson Ratio | Compressive Strength (MPa) | k (MPa) | |
|---|---|---|---|---|---|---|
| Casing | 0.1–0.4 | 0.012 | 210 | 0.25 | N/A | N/A |
| Cement | 0.124–0.424 | 0.065 | 15 | 0.3 | 67 MPa | 9.5 |
| Formation | 0.254–0.554 | >10 | 25 | 0.3 | N/A | N/A |
| Well Number | Casing IR/mm | Casing OR/mm | Thickness of Cement Sheath/mm | Eccentricity/mm | Cement EM/GPa | Tectonic Stress/MPa | Pump Pressure/MPa | |
|---|---|---|---|---|---|---|---|---|
| 1 | In. Para. | 60.68 | 69.85 | 38.1 | 26 | 15 | 62 | 68 |
| Op. Para. | 58.68 | N/A | 50.8 | N/A | N/A | N/A | N/A | |
| 2 | In. Para. | 57.80 | 69.85 | 38.1 | 17 | 15 | 69 | 74 |
| Op. Para. | 56.80 | N/A | 50.8 | N/A | N/A | N/A | N/A | |
| 3 | In. Para. | 51.36 | 63.50 | 20.65 | 8 | 13 | 70 | 80 |
| Op. Para. | N/A | N/A | 36.50 | N/A | N/A | N/A | N/A | |
| 4 | In. Para. | 54.30 | 63.50 | 20.65 | 18 | 13 | 66 | 78 |
| Op. Para. | 50.30 | N/A | 36.50 | N/A | N/A | N/A | N/A | |
| 5 | In. Para. | 48.59 | 57.15 | 19.05 | 12 | 13 | 40 | 76 |
| Op. Para. | 45.15 | N/A | 42.85 | N/A | N/A | N/A | N/A | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, K.; Gao, D.; Taleghani, A.D. Impact of Casing Eccentricity on Cement Sheath. Energies 2018, 11, 2557. https://doi.org/10.3390/en11102557
Liu K, Gao D, Taleghani AD. Impact of Casing Eccentricity on Cement Sheath. Energies. 2018; 11(10):2557. https://doi.org/10.3390/en11102557
Chicago/Turabian StyleLiu, Kui, Deli Gao, and Arash Dahi Taleghani. 2018. "Impact of Casing Eccentricity on Cement Sheath" Energies 11, no. 10: 2557. https://doi.org/10.3390/en11102557
APA StyleLiu, K., Gao, D., & Taleghani, A. D. (2018). Impact of Casing Eccentricity on Cement Sheath. Energies, 11(10), 2557. https://doi.org/10.3390/en11102557




