Solid-Liquid Interfacial Effects on Residual Oil Distribution Utilizing Three-Dimensional Micro Network Models
Abstract
:1. Introduction
2. Existence Condition and Range of the Solid–Liquid Interfacial Effects
2.1. Van der Waals Force
2.2. The Form and Scope of Action
3. Mathematical Model
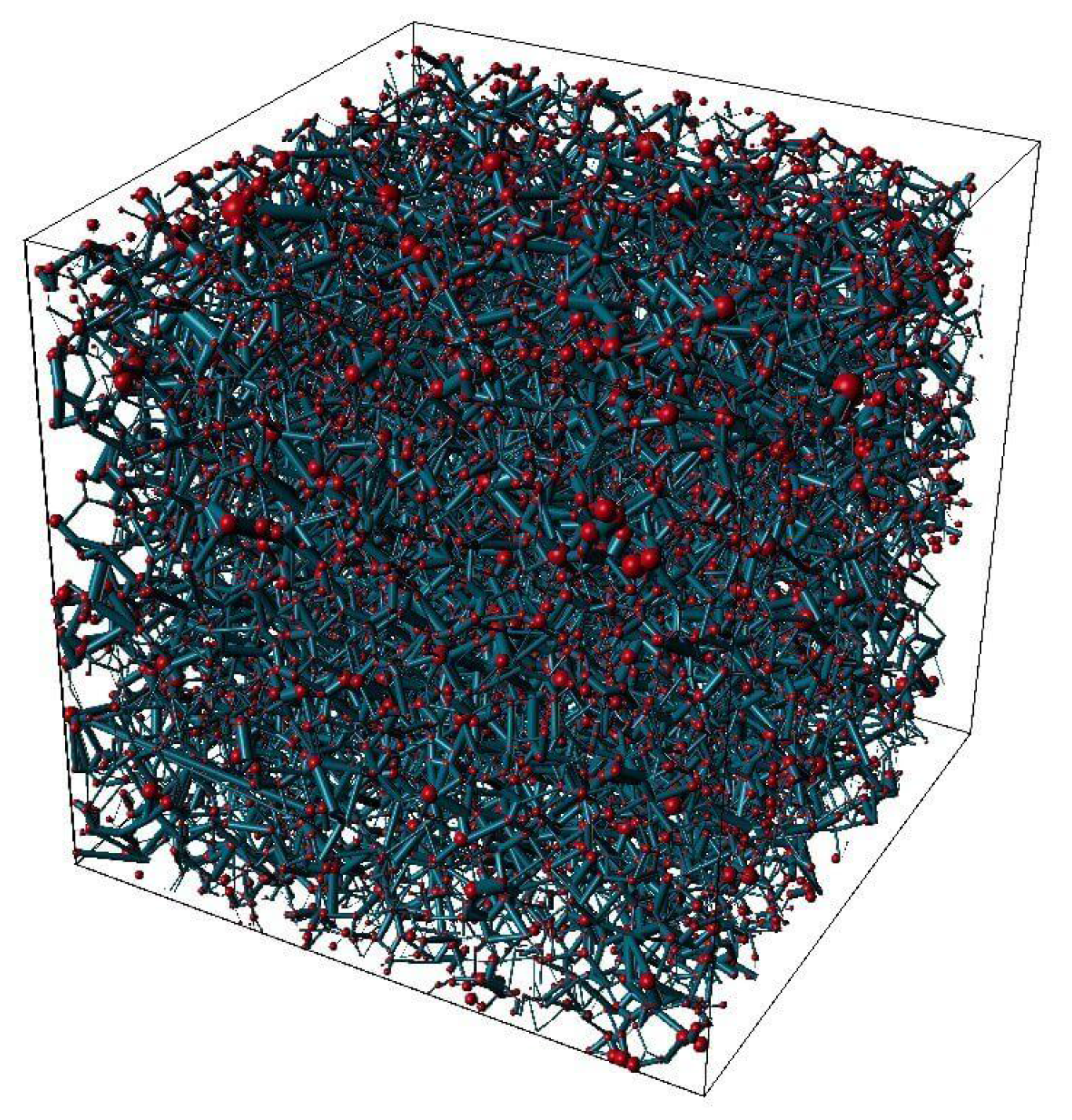
3.1. Establishment of the 3D Network Model
3.2. A Mathematical Model with Van der Waals Force
3.3. Microscopic Simulation Model of Oil–Water Two-Phase System
3.3.1. Primary Oil Flooding
3.3.2. Secondary Water Flooding
3.4. Calculation of Macroscopic Parameters
3.4.1. Water Saturation
3.4.2. Relative Permeability
4. Simulation Results and Discussion
4.1. Water-Wet Reservoir
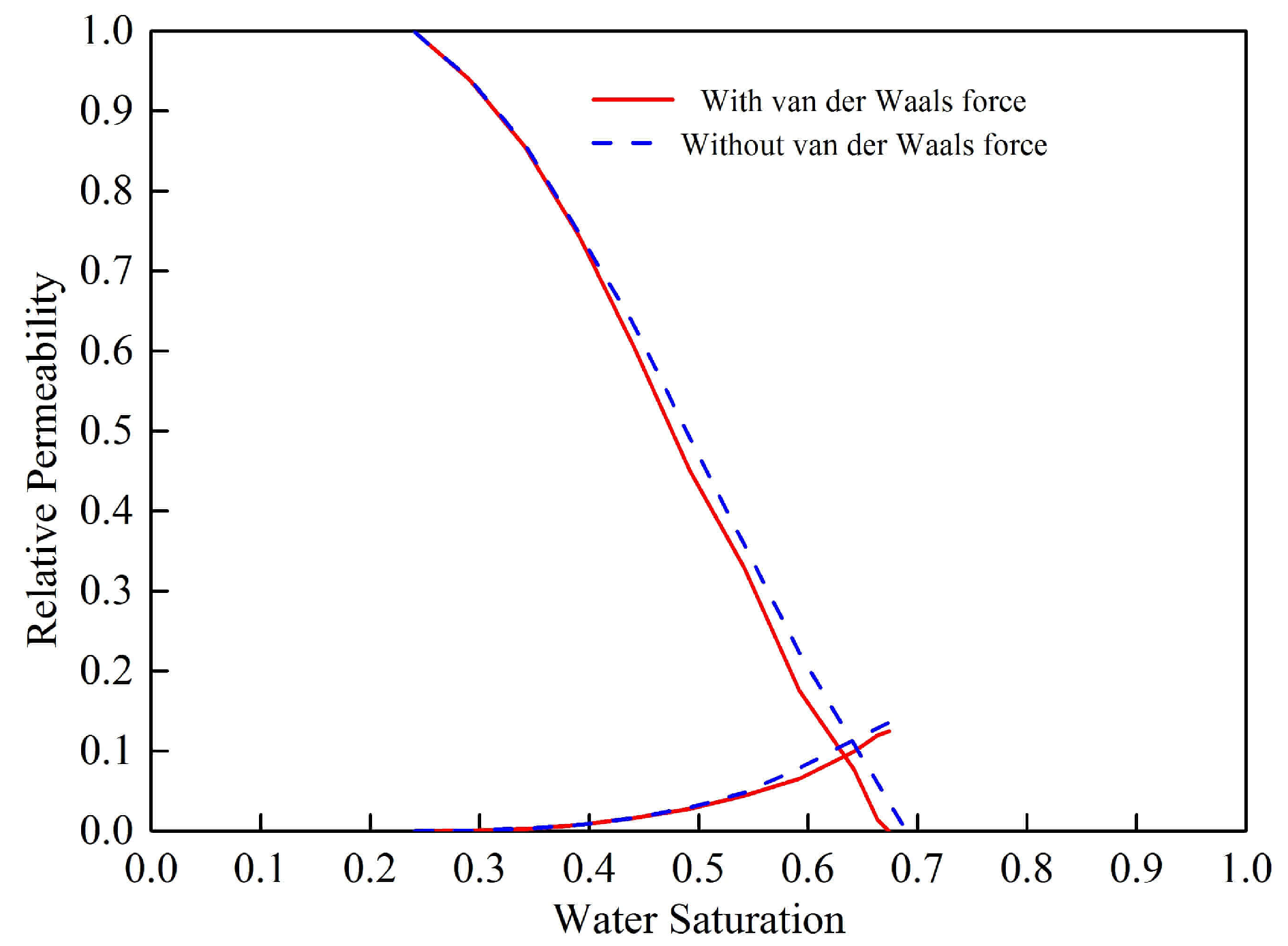
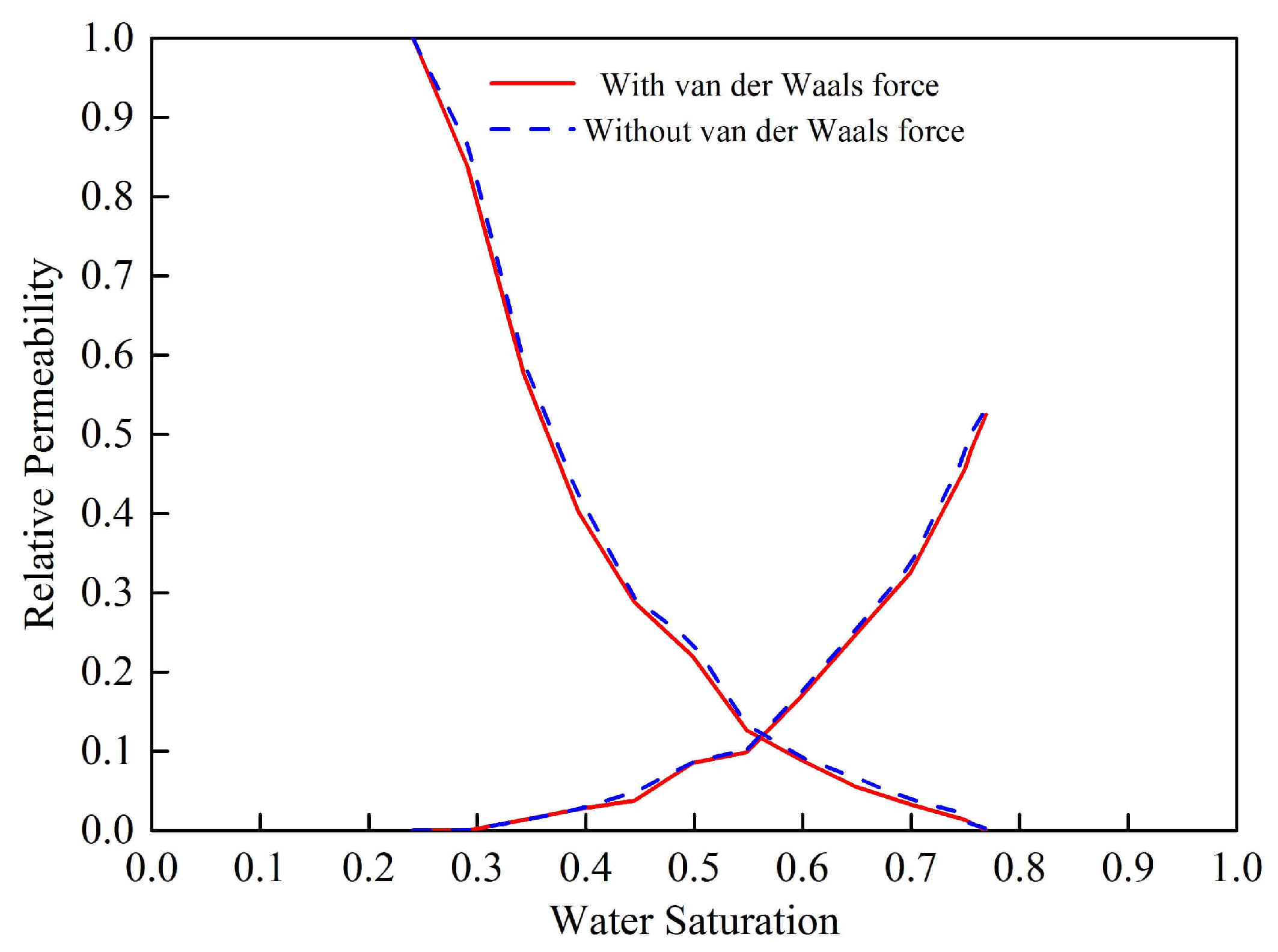
4.1.1. Relative Permeability
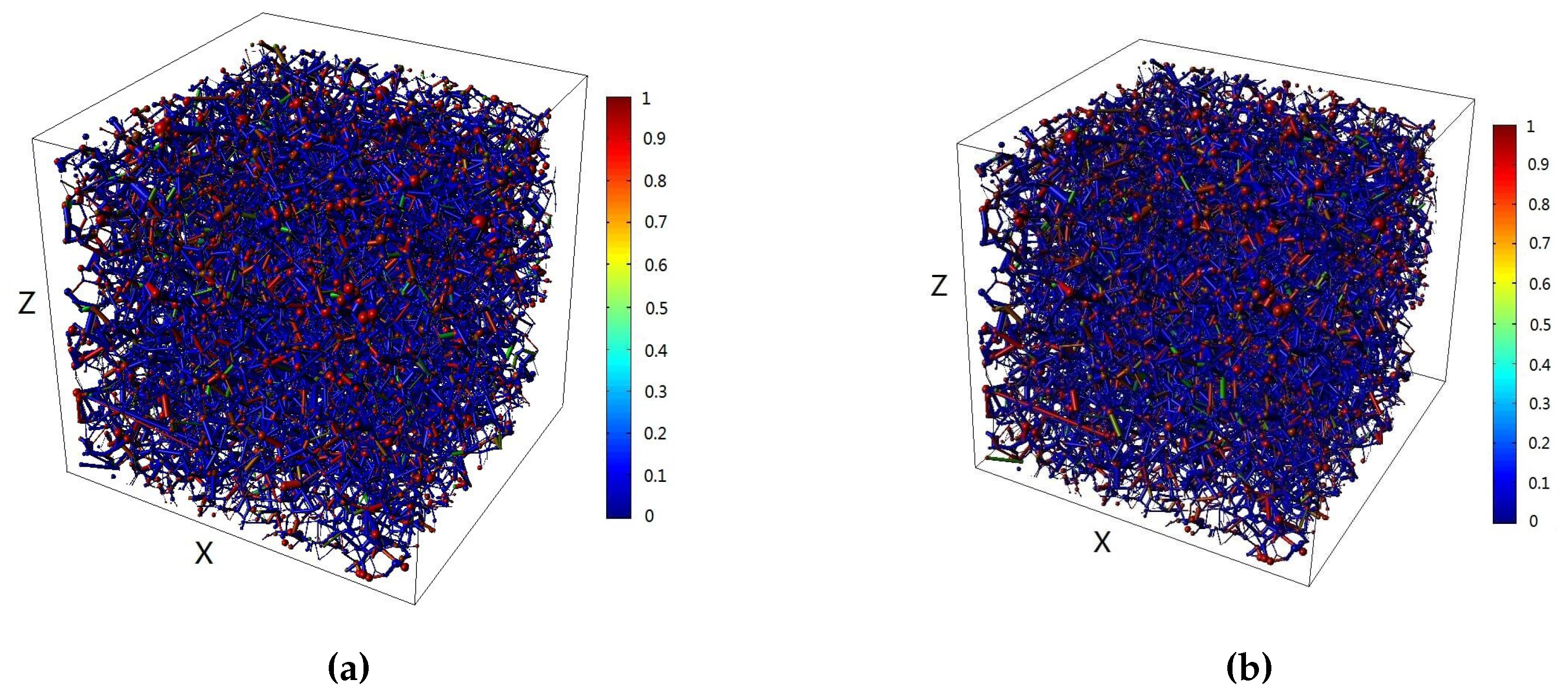
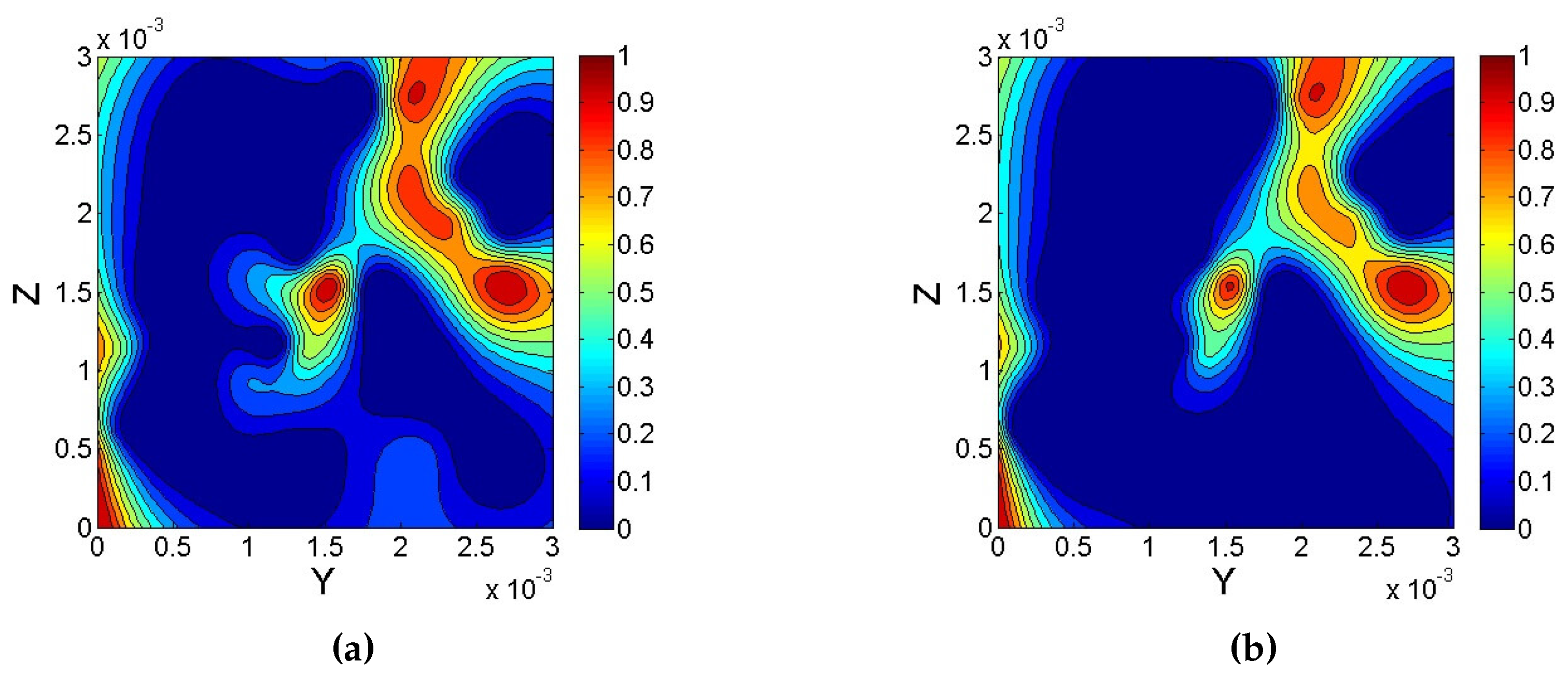
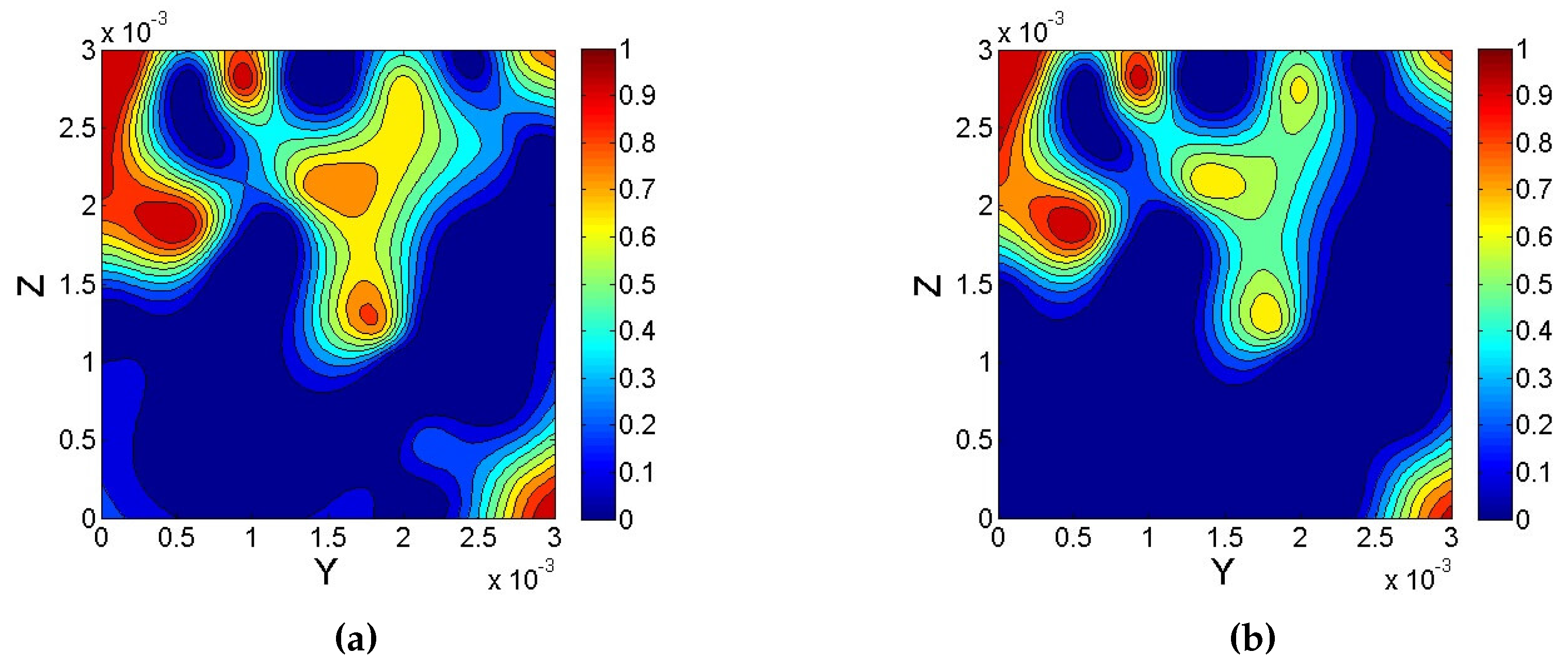
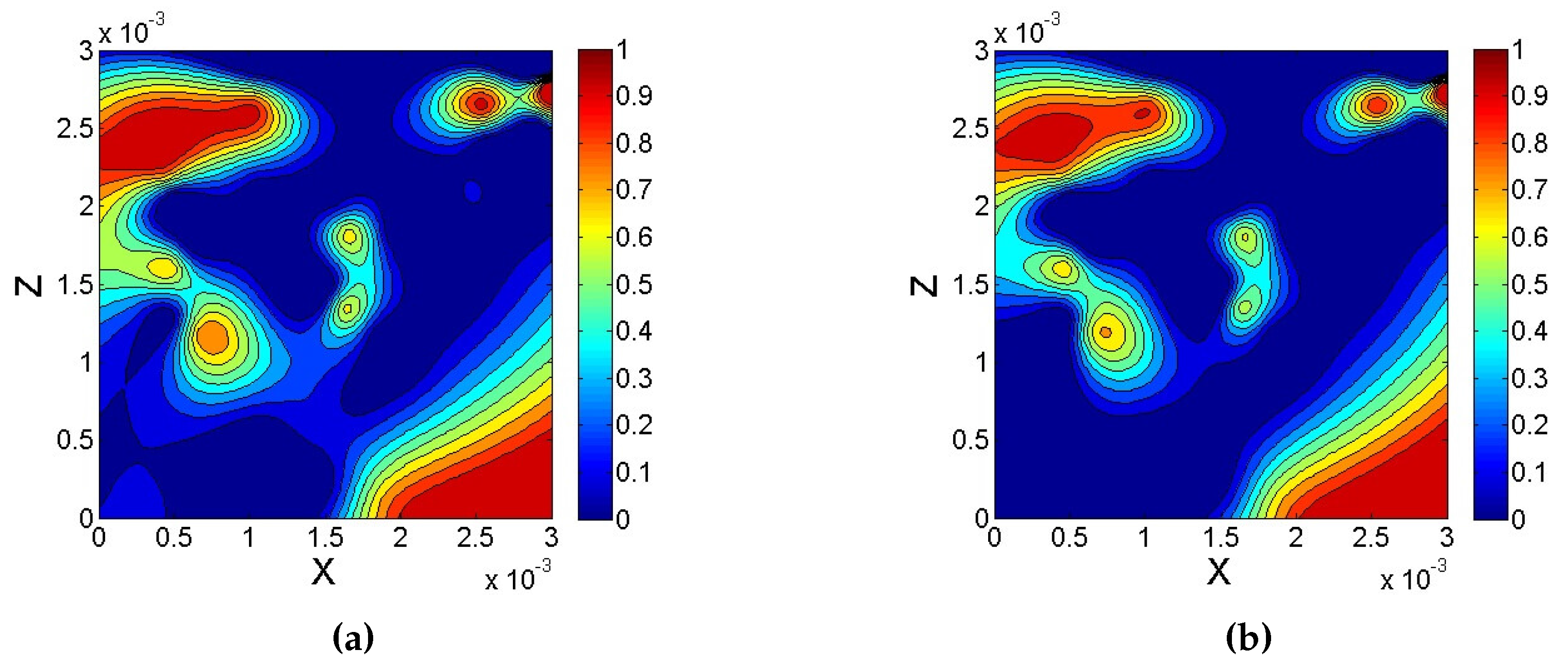
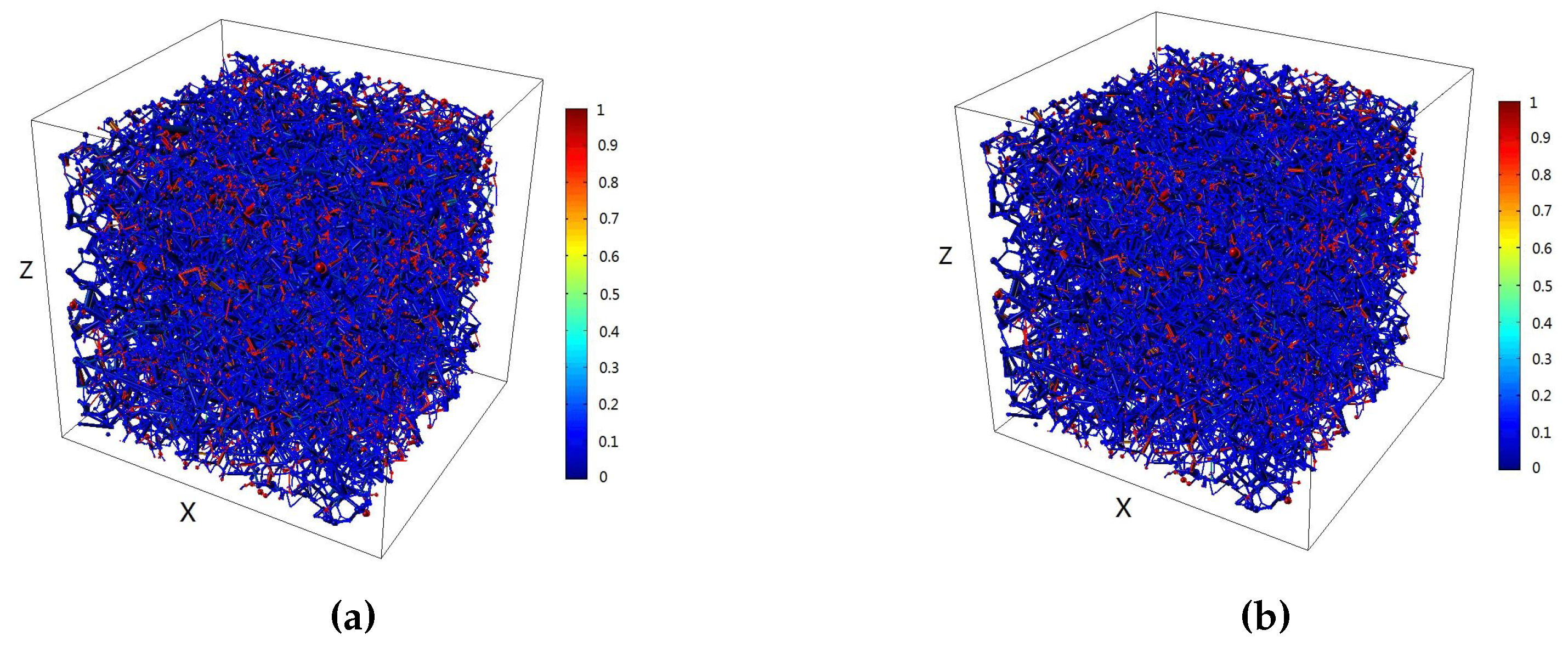
4.1.2. Residual Oil Distribution
4.2. Oil-Wet Reservoir
4.2.1. Relative Permeability
4.2.2. Residual Oil Distribution
4.3. Effects of Micro-Structure on Residual Oil
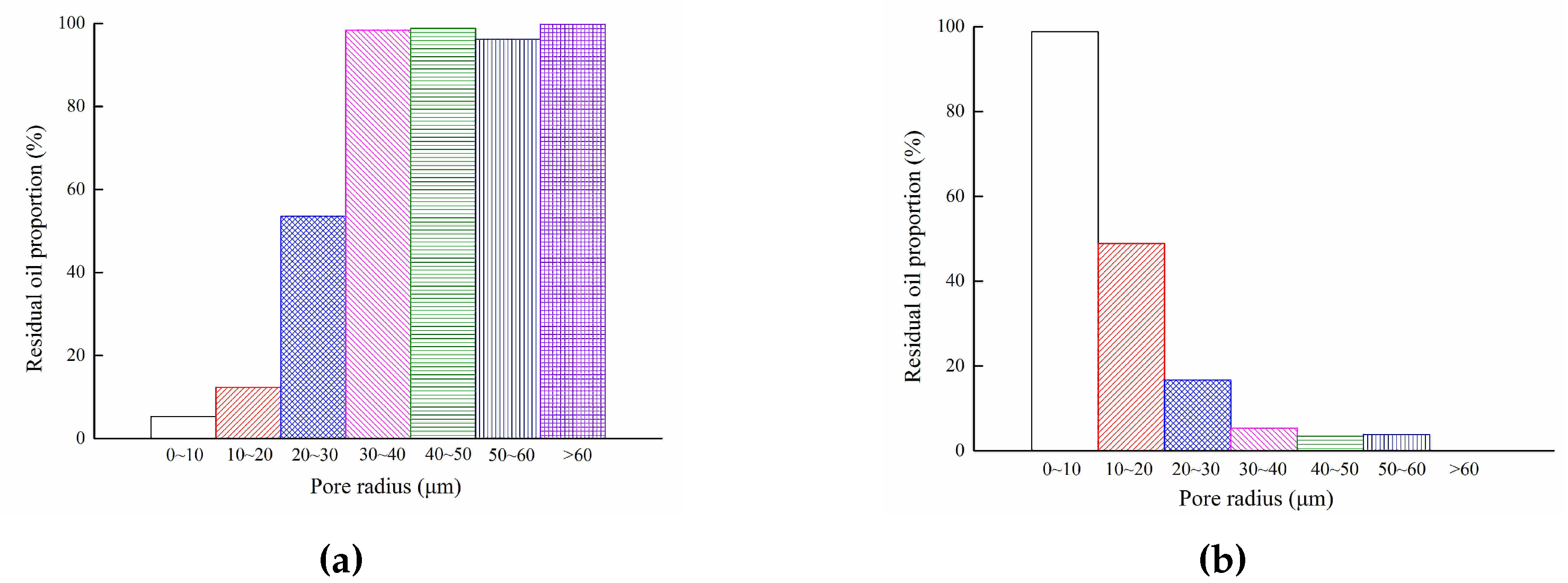
4.3.1. Pore Radius
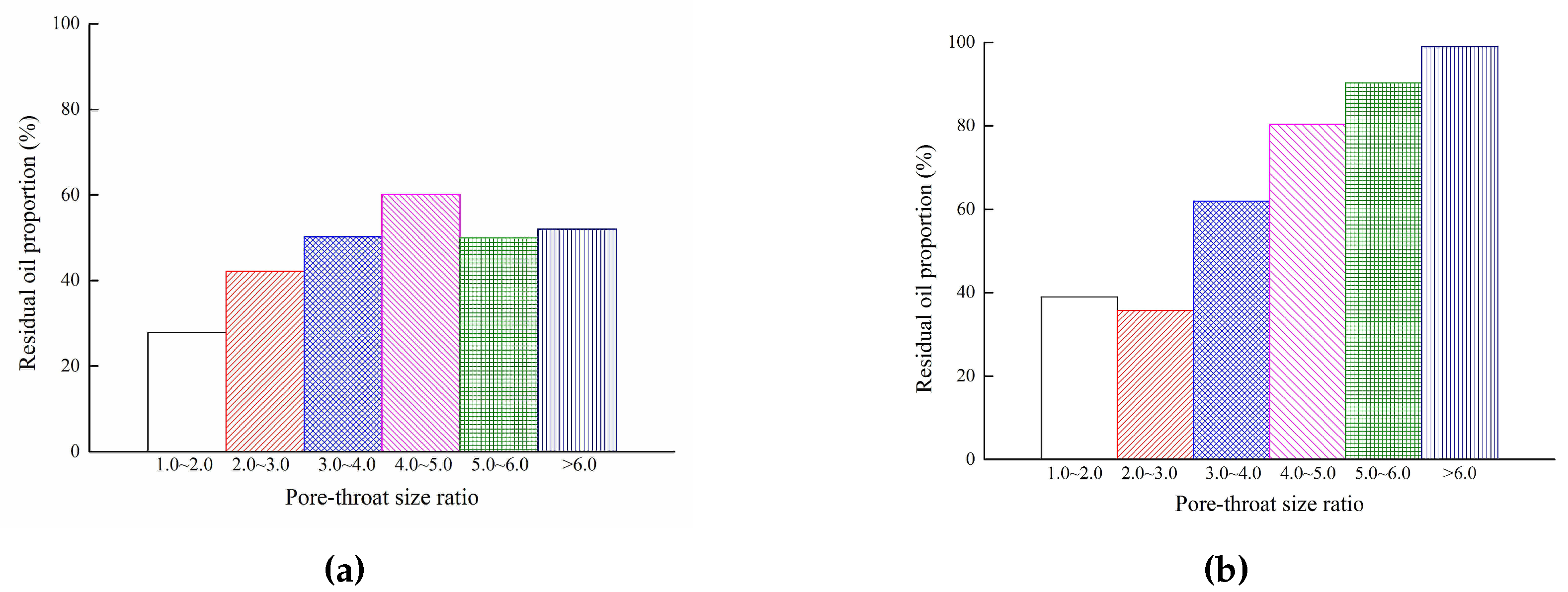
4.3.2. Pore–Throat Size Ratio
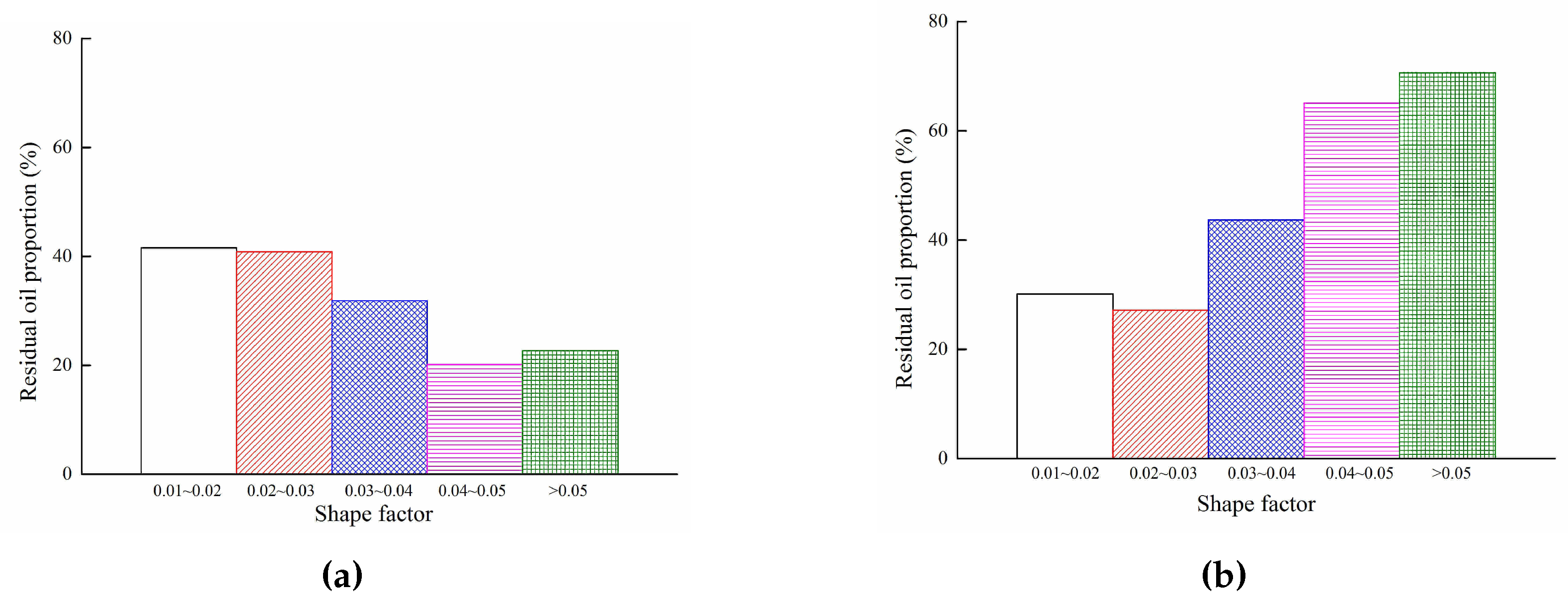
4.3.3. Shaping Factor
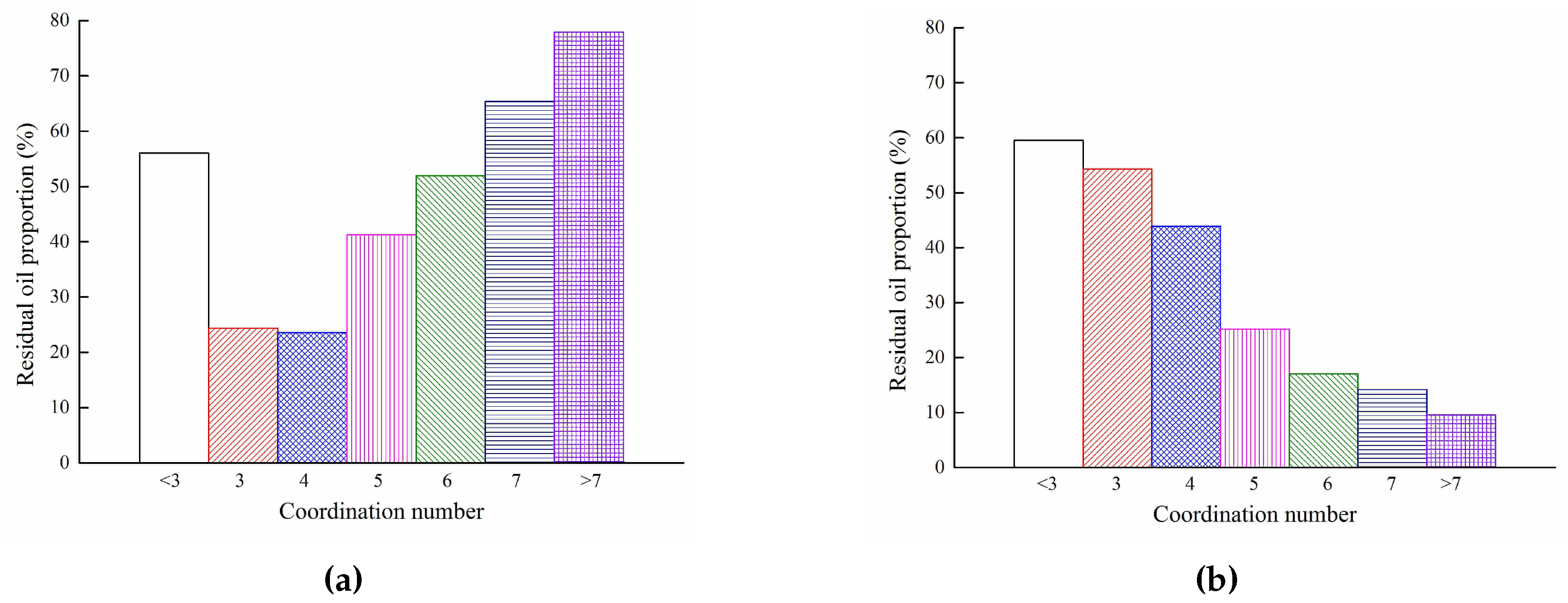
4.3.4. Coordination Number
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Blunt, M.J. Flow in porous media-pore-network models and multiphase flow. Curr. Opin. Colloid Interface Sci. 2001, 6, 197–207. [Google Scholar] [CrossRef]
- Fatt, I. The network model of porous media. Pet. Trans. AIME 1956, 207, 144–159. [Google Scholar]
- Dullien, F.A.L.; Lai, F.S.Y.; Macdonald, I.F. Hydraulic continuity of residual wetting phase in porous media. J. Colloid Interface Sci. 1986, 109, 201–218. [Google Scholar] [CrossRef]
- Dullien, F.A.L.; Zarcone, C.; Macdonald, I.F.; Collins, A.; Bochard, R.D.E. The effects of surface roughness on the capillary pressure curves and the heights of capillary rise in glass bead packs. J. Colloid Interface Sci. 1989, 127, 362–372. [Google Scholar] [CrossRef]
- Aker, E.; MålØy, K.J.; Hansen, A.; Batrouni, G.G. A two-dimensional network simulator for two-phase flow in porous media. Transp. Porous Media 1989, 32, 163–186. [Google Scholar] [CrossRef]
- Ochi, J.; Vernoux, J.F. A two-dimensional network model to simulate permeability decrease under hydrodynamic effect of particle release and capture. Transp. Porous Media 1999, 37, 303–325. [Google Scholar] [CrossRef]
- Markicevic, B.; Bazylak, A.; Djilali, N. Determination of transport parameters for multiphase flow in porous gas diffusion electrodes using a capillary network model. J. Power Sources 2007, 171, 706–717. [Google Scholar] [CrossRef]
- Hu, X.T.; Yun, L. Study of microcosmic distribution of residual oil with stochastic simulation in networks. Acta Pet. Sin. 2000, 21, 46–51. [Google Scholar]
- Wang, J.X.; Wu, X.D.; PAN, X.W. The influence of aqueous phase trapping on gas flow by pore scale network model. Pet. Explor. Dev. 2003, 30, 113–115. [Google Scholar]
- Blunt, M.; King, P. Relative permeabilities from two-and three-dimensional pore-scale network modelling. Transp. Porous Media 1991, 6, 407–433. [Google Scholar] [CrossRef]
- Øren, P.E.; Bakke, S. Reconstruction of Berea sandstone and pore-scale modelling of wettability effects. J. Pet. Sci. Eng. 2003, 39, 177–199. [Google Scholar]
- Valvatne, P.H.; Blunt, M.J. Predictive pore-scale modeling of two-phase flow in mixed wet media. Water Resour. Res. 2004, 40, W07406. [Google Scholar] [CrossRef]
- Hou, J.; Li, Z.Q.; Guan, J.T.; Wang, K.W.; Chen, Y.M. Water flooding microscopic seepage mechanism research based on three-dimension network model. Chin. J. Theor. Appl. Mech. 2005, 37, 783–787. [Google Scholar]
- Yao, J.; Tao, J.; Aifen, L. Research on oil-water two-phase flow using 3D random network model. Acta Pet. Sin. 2007, 28, 94–101. [Google Scholar]
- Zhao, X.; Blunt, M.J.; Yao, J. Pore-scale modeling: Effects of wettability on waterflood oil recovery. J. Pet. Sci. Eng. 2010, 71, 169–178. [Google Scholar] [CrossRef]
- Ryazanov, A.V.; van Dijke, M.I.J.; Sorbie, K.S. Two-phase pore-network modelling: Existence of oil layers during water invasion. Transp. Porous Media 2009, 80, 79–99. [Google Scholar] [CrossRef]
- Bashtani, F.; Maini, B.; Kantzas, A. Single-phase and two-phase flow properties of mesaverde tight sandstone formation; random-network modeling approach. Adv. Water Resour. 2016, 94, 174–184. [Google Scholar] [CrossRef]
- Al-Dhahli, A.R.S.; Geiger, S.; van Dijke, M.I.J. Three-phase pore-network modeling for reservoirs with arbitrary wettability. SPE J. 2012, 18, 285–295. [Google Scholar] [CrossRef]
- Al-Dhahli, A.; van Dijke, M.I.J.; Geiger, S. Accurate modelling of pore-scale films and layers for three-phase flow processes in clastic and carbonate rocks with arbitrary wettability. Transp. Porous Media 2013, 98, 259–286. [Google Scholar] [CrossRef]
- Blunt, M.J.; Jackson, M.D.; Piri, M.; Valvatne, P.H. Detailed physics, predictive capabilities and macroscopic consequences for pore-network models of multiphase flow. Adv. Water Resour. 2002, 25, 1069–1089. [Google Scholar] [CrossRef]
- Piri, M.; Blunt, M.J. Three-dimensional mixed-wet random pore-scale network modeling of two-and three-phase flow in porous media. I. Model description. Phys. Rev. E 2005, 71, 026–301. [Google Scholar] [CrossRef] [PubMed]
- Sinha, P.K.; Wang, C.Y. Pore-network modeling of liquid water transport in gas diffusion layer of a polymer electrolyte fuel cell. Electrochim. Acta 2007, 52, 7936–7945. [Google Scholar] [CrossRef]
- Al-Kharusi, A.S.; Blunt, M.J. Multiphase flow predictions from carbonate pore space images using extracted network models. Water Resour. Res. 2008, 44, W06S01. [Google Scholar] [CrossRef]
- Raman, A.; van Dijke, M.I.J. Study of the Effects of Wettability Changes on Three-Phase Flow in Porous Media through Pore Network Modelling. In Proceedings of the Offshore Technology Conference Asia, Kuala Lumpur, Malaysia, 22–25 March 2016. [Google Scholar]
- Ren, L.; Qu, W.; Li, D. Interfacial electrokinetic effects on liquid flow in microchannels. Int. J. Heat Mass Transf. 2001, 44, 3125–3134. [Google Scholar] [CrossRef]
- Ren, C.L.; Li, D. Improved understanding of the effect of electrical double layer on pressure-driven flow in microchannels. Anal. Chim. Acta 2005, 531, 15–23. [Google Scholar] [CrossRef]
- Hsieh, S.S.; Lin, C.Y. Convective heat transfer in liquid microchannels with hydrophobic and hydrophilic surfaces. Int. J. Heat Mass Transf. 2009, 52, 260–270. [Google Scholar] [CrossRef]
- Jain, A.; Jensen, M.K. Analytical modeling of electrokinetic effects on flow and heat transfer in microchannels. Int. J. Heat Mass Transf. 2007, 50, 5161–5167. [Google Scholar] [CrossRef]
- Hwang, P.W.; Soong, C.Y. Investigation of variable-property microchannel flows with electro-thermo-hydrodynamic interactions at constant pressure gradient or constant flow rate. Int. J. Heat Mass Transf. 2008, 51, 210–223. [Google Scholar] [CrossRef]
- Li, Y.; Lei, Q.; Liu, X.G.; Xiao, H.M. Characteristics of micro scale nonlinear filtration. Pet. Explor. Dev. 2011, 38, 336–340. [Google Scholar]
- Pfahler, J.; Harley, J.; Bau, H.; Zemel, J. Liquid transport in micron and submicron channels. Sens. Actuators A Phys. 1990, 22, 431–434. [Google Scholar] [CrossRef]
- Gad-el-Hak, M. The fluid mechanics of microdevices-the Freeman scholar lecture. J. Fluids Eng. 1999, 121, 5–33. [Google Scholar] [CrossRef]
- Oliveira, R.S.; Andrade, J.S., Jr.; Andrade, R.F. Fluid flow through Apollonian packings. Phys. Rev. E 2010, 81, 047302. [Google Scholar] [CrossRef] [PubMed]
- Zeng, B.; Cheng, L.; Li, C. Low velocity non-linear flow in ultra-low permeability reservoir. J. Pet. Sci. Eng. 2011, 80, 1–6. [Google Scholar] [CrossRef]
- Mattia, D.; Calabrò, F. Explaining high flow rate of water in carbon nanotubes via solid-liquid molecular interactions. Microfluid. Nanofluid. 2012, 13, 125–130. [Google Scholar] [CrossRef] [Green Version]
- Frolov, A.I.; Kirchner, K.; Kirchner, T.; Fedorov, M.V. Molecular-scale insights into the mechanisms of ionic liquids interactions with carbon nanotubes. Faraday Discuss. 2012, 154, 235–247. [Google Scholar] [CrossRef] [PubMed]
- Li, T.; Song, H.; Wang, J.; Wang, Y.; Killough, J. An analytical method for modeling and analysis gas-water relative permeability in nanoscale pores with interfacial effects. Int. J. Coal Geol. 2016, 159, 71–81. [Google Scholar] [CrossRef]
- Zhang, X.L.; Zhu, W.Y.; Cai, Q.; Liu, Q.P.; Wang, X.F.; Lou, Y. Analysis of weakly compressible fluid flow in nano/micro-size circular tubes considering solid wall force. J. Univ. Sci. Technol. B 2014, 36, 569–575. [Google Scholar]
- Wang, X.F.; Zhu, W.Y.; Deng, Q.J.; Zhang, X.L.; Lou, Y.; Gao, Y. Micro Circular Tube Flow Mathematical Model with the Effect of Van der Waals Force; Trans Tech Publications: Zürich, Switzerland, 2014; Volume 448, pp. 3975–3981. [Google Scholar]
- Wang, X.F.; Zhu, W.Y.; Deng, Q.J.; Liu, Q.P.; Sui, X.G.; Lou, Y.; Gao, Y.; Zhang, X.L. Dynamic network model considering solid-liquid molecule interaction in porous media. J. Univ. Sci . Technol. B 2014, 36, 145–152. [Google Scholar]
- Ma, J.; Sanchez, J.P.; Wu, K.; Couples, G.D.; Jiang, Z. A pore network model for simulating non-ideal gas flow in micro-and nano-porous materials. Fuel 2014, 116, 498–508. [Google Scholar] [CrossRef]
- Deng, Q.J.; Zhu, W.Y.; Wang, X.F.; Sui, X.G.; Wang, X.J. Seepage model considering micro forces in porous media. J. Univ. Sci. Technol. B 2014, 36, 415–423. [Google Scholar]
- Kovscek, A.R.; Wong, H.; Radke, C.J. A pore-level scenario for the development of mixed wettability in oil reservoirs. AlChE J. 1993, 39, 1072–1085. [Google Scholar] [CrossRef]
- Fenwick, D.H.; Blunt, M.J. Network modeling of three-phase flow in porous media. SPE J. 1998, 3, 86–96. [Google Scholar] [CrossRef]
- Mason, G.; Morrow, N.R. Capillary behavior of a perfectly wetting liquid in irregular triangular tubes. J. Colloid Interface Sci. 1991, 141, 262–274. [Google Scholar] [CrossRef]
- Valvatne, P.H.; Piri, M.; Lopez, X.; Blunt, M.J. Predictive pore-scale modeling of single and multiphase flow. Transp. Porous Media 2005, 58, 23–41. [Google Scholar] [CrossRef]
- Øren, P.E.; Bakke, S.; Arntzen, O.J. Extending predictive capabilities to network models. SPE J. 1998, 3, 324–336. [Google Scholar]
- Lenormand, R.; Zarcone, C.; Sarr, A. Mechanisms of the displacement of one fluid by another in a network of capillary ducts. J. Fluid Mech. 1983, 135, 337–353. [Google Scholar] [CrossRef]
- Lerdahl, T.R.; Øren, P.E.; Bakke, S. A predictive network model for three-phase flow in porous media. In Proceedings of the 2000 SPE/DOE Improved Oil Recovery Symposium, Tulsa, Oklahoma, 3–5 April 2000. Paper SPE 59311. [Google Scholar]
- Geim, A.K.; Dubonos, S.V.; Grigorieva, I.V.; Novoselov, K.S.; Zhukov, A.A.; Shapoval, S.Y. Microfabricated adhesive mimicking gecko foot-hair. Nat. Mater. 2003, 2, 461–463. [Google Scholar] [CrossRef] [PubMed]
- Hou, J. Network modeling of residual oil displacement after polymer flooding. J. Petrol. Sci. Eng. 2007, 59, 321–332. [Google Scholar] [CrossRef]
- Gao, H.; Li, T.; Yang, L. Quantitative determination of pore and throat parameters in tight oil reservoir using constant rate mercury intrusion technique. J. Pet. Explor. Prod. Technol. 2016, 6, 309–318. [Google Scholar] [CrossRef]
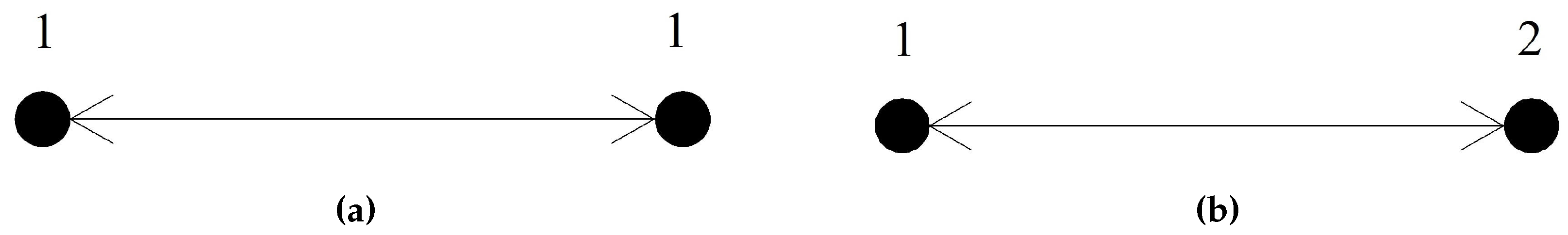

| Parameter | Unit | Value | Parameter | Unit | Value |
|---|---|---|---|---|---|
| Network size | mm | 3 × 3 × 3 | Initial contact angle | 0 | |
| Pore radius | m | 3.6–73.5 | Water-wet contact angle | 40–50 | |
| Throat radius | m | 0.9–56.9 | Oil-wet contact angle | 130–140 | |
| Throat length | m | 0–78.94 | Oil–water interfacial tension | mN/m | 30 |
| Average pore–throat ratio | 2.06 | Oil density | g/cm3 | 0.88 | |
| Average coordination number | 4.19 | Water density | g/cm3 | 1 | |
| Porosity | % | 24 | Oil viscosity | mPa·s | 10 |
| Absolute permeability | D | 2.5 | Water viscosity | mPa·s | 1 |
| Solid wall Hamaker constant | J | 3–50 × | Water Hamaker constant | J | 2 × |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, W.; Li, B.; Liu, Y.; Song, H.; Wang, X. Solid-Liquid Interfacial Effects on Residual Oil Distribution Utilizing Three-Dimensional Micro Network Models. Energies 2017, 10, 2059. https://doi.org/10.3390/en10122059
Zhu W, Li B, Liu Y, Song H, Wang X. Solid-Liquid Interfacial Effects on Residual Oil Distribution Utilizing Three-Dimensional Micro Network Models. Energies. 2017; 10(12):2059. https://doi.org/10.3390/en10122059
Chicago/Turabian StyleZhu, Weiyao, Bingbing Li, Yajing Liu, Hongqing Song, and Xiaofeng Wang. 2017. "Solid-Liquid Interfacial Effects on Residual Oil Distribution Utilizing Three-Dimensional Micro Network Models" Energies 10, no. 12: 2059. https://doi.org/10.3390/en10122059
APA StyleZhu, W., Li, B., Liu, Y., Song, H., & Wang, X. (2017). Solid-Liquid Interfacial Effects on Residual Oil Distribution Utilizing Three-Dimensional Micro Network Models. Energies, 10(12), 2059. https://doi.org/10.3390/en10122059




