1. Introduction
Attempts to time the stock market to make an above-average return is one of the most common practices among individual investors. Empirical evidence from behavioral finance suggests that investors who try to time the market often meet with disastrous outcomes (
Odean 1999;
Barber and Odean 1999,
2000;
Antoons 2018a;
Stevens 2021). Overconfidence and availability heuristic play major roles in forming such behavior among investors. On the institutional side, tactical asset allocation (TAA) and strategic sector rotations are sold as strategies that will produce a positive alpha. However, these strategies often produce a more negative alpha before fees when compared to that of the static buy and hold strategies (
Kanuri et al. 2021). Be it TAA or market timing, they all use some measures to gauge if certain equities (or the equity market) are too expensive, or whether the market is too optimistic/pessimistic. The CBOE Volatility Index (the VIX) and market sentiment index (MSI) are often used as contrarian indicators for market sentiments. On the valuation side, dividend yields and the current market P/E ratio relative to the historic average have often been mentioned as valuable tools. See
Campbell and Thompson (
2008),
Welch and Goyal (
2008),
Hollstein et al. (
2000),
Zhou et al. (
2023), and
Goyal et al. (
2021) for examples. In recent years, another measure has gotten a lot of attention: the CAPE Ratio.
The CAPE Ratio (the cyclically adjusted price-to-earnings ratio, commonly known as the Shiller P/E), outlined in
Campbell and Shiller (
1998), is a valuation measure that measures the relative value of the US equity market. If the current CAPE Ratio value is higher (lower) than the historical average, then it would imply potentially lower (higher) than average returns going forward. If the CAPE Ratio is much higher than the historical average, it signals a possible imminent decline in the equity market. Thus, as an anticipatory strategy, when the CAPE Ratio is too high, would be to rotate out of equity and into bonds or other safer assets and wait until the market has corrected before going back into equities. On the other hand, if the CAPE Ratio is lower than historic average, more asset weights on equity could produce above-average returns in the future.
For the tactical strategies stated above to succeed, the average CAPE Ratio must be stable over time, and there should be a negative correlation between the CAPE Ratio and the forward excess returns. In this paper, I show that there is an upward trend in the CAPE Ratio. The trend has been more pronounced in recent decades. The upward trend coincided with a low-interest-rate environment in recent decades and a major shift in the major components of the S&P 500 Index. Furthermore, I show that the correlation coefficient between the CAPE Ratio and forward excess returns is positive rather than negative, as suggested in Campbell and Shiller’s original paper. To account for these results that are contradictory to the original paper, I propose a simple tracking error approach that can account for the structural change in the S&P500 Index components that contributed to a higher earnings growth rate and the low-interest-rate environment in recent decades, as well as the effects they might have on the CAPE Ratio. It shows that when these factors are taken into consideration, the value of CAPE Ratio can be much higher than the historical average, and the equity market will still not be considered as overvalued. Therefore, attempting to time the stock market to generate excess returns with the CAPE Ratio is fruitless.
This paper contributes to the existing literatures by proposing a tracking error approach with the simpler Gordon (Constant) Growth Model that can account for structural change in the S&P500 Index and the effect a low interest rate might have on the CAPE Ratio. It provides a rationale for the persistently high CAPE Ratio in recent decades and offer advice on what other factors investors should consider before they decide if they should adjust their portfolio composition between equity and other asset classes should they decide to beat the market average return.
2. Background and Literature Review
The CAPE Ratio has gotten so much more attentions in recent years is because it is said to have predicted the DotCom Bubble in 1999 to 2000. Because of the availability heuristic (
Kahneman and Tversky 1982), the CAPE Ratio has been used as a popular reference point in financial press to show the potential for market bubbles when the CAPE Ratio is much higher than the historic average. With an historic average of around 16.5 (up to 1999), the CAPE Ratio value was 2.5 times larger than its historic level prior to the crash of the Dot Com Bubble, 2 times as much prior to Black Tuesday in 1929, and 1.8 times larger before the 2008 Financial Crisis. Thus, journalists argue that it shows that the CAPE Ratio is great at predicting major market corrections. Outside of these three major historic events in the US stock market history, however, the CAPE Ratio has not been proven to be particularly useful in predicting future stock returns.
If the CAPE Ratio is a useful contrarian indicator, it should have negative correlation coefficient. However, the correlation coefficient between the CAPE Ratio and the 10-year annualized excess stock return (which the CAPE Ratio aims to predict) has been positive (0.67) since 1952. The contributing factors to the mediocre performance of the CAPE Ratio in recent years might be due to the structural change that occurred in S&P500 Index’s components and a persistently low risk-free rate in recent decades that caused a shift in the distribution of the CAPE Ratio. The structural changes in the S&P500 component led to a much higher earnings growth rate when compared to the historical average. Since the CAPE Ratio uses the average of the earnings from the last 10 years, it will bias downward when the forward growth rate is higher than the historic average. On the other hand, a persistently low risk-free rate led to lower required returns for risky assets. These two factors combined would justify a higher P/E ratio (and thus, a higher CAPE Ratio) for equities.
Because the CAPE Ratio paper was written mainly for practitioners, there are relatively few academic papers that discuss the merits and shortfalls of the ratio.
Antoons (
2018b),
Asness et al. (
2017),
Keimling (
2016), and
Siegel (
2013) analyze the predictive power of the ratio, and
Siegel (
2016) analyzes some of the structural changes in the S&P500 index component and accounting standard that are not captured by the CAPE Ratio.
It is important to note that while the CAPE Ratio was developed by
Campbell and Shiller (
1998), the authors cautioned that issues with the dividend yield
1 (dividend/price ratio) and long-term interest rate could have altered the conclusion they make in the paper. Furthermore, the predictive power of the CAPE Ratio on future returns is exceptionally poor ex post. They predicted that based on the result up to January of 1997, with an exceptionally high level of CAPE Ratio value of 28, the S&P500 Index would have a negative 40% return over the next 10 years. The S&P500 Index was at 800 in January of 1997, and it increased to 1500 in January of 2007 (87.5% return over that 10-year period, including the DotCom Bubble). The long-term performance of the CAPE Ratio is exceptionally poor.
The short-term performance of the CAPE Ratio’s predictive power is not much better.
Antoons (
2018b) provides a comprehensive analysis of the predictive power of CAPE Ratio in predicting the equity market returns over 1-year, 3-year, 5-year, and 10-year periods. The study shows that the predictive power over the 1-year period is zero. While there is some predictive power over the 5-year and 10-year periods, the predictive power of extremely high (market bubbles) and low (market crashes) CAPE Ratios is also close to zero. In other words, marking timing based on the CAPE Ratio is next to impossible in the short term (
Asness et al. 2017;
Keimling 2016;
Siegel 2013). Over the long term, the higher predictive power for extreme cases might simply be a result of regression to the mean (which can be observed by the market P/E ratio). However, to achieve a higher return by timing the market with the CAPE Ratio, an investor must be willing to sell stocks when the CAPE Ratio is too high and hold the funds in cash. Then, the investor must reinvest the cash into stocks when the CAPE Ratio falls below the historic average. Only a few investors would have the patience to hold cash for more than a few months, let alone a few years. For an investor who does use the CAPE Ratio time the stock market, they would have had to hold cash for 28 out of the last 30 years (since 1992) since the CAPE Ratio only fell below the historic average in 2008 and 2009 during the 2008 Financial Crisis. They would have had to get out of the stock market again in mid-2009 and missed out all on all the major rallies in the stock market over the last 3 decades. Clear, using the historic average as a market-timing tool will not work.
Siegel (
2016) suggests that CAPE Ratio’s poor performance in recent years might have something to do with the fact that it does not account for the change in accounting rules for how earnings are calculated in recent decades. In recent years, when companies report earnings, they often report the GAAP number for net income (used in the CAPE Ratio calculation) and the operating income, which excludes non-recurring items and are often much higher than the GAAP net income figure. Most analysts focus on a company’s operating income rather than the GAAP net income. Once the downward bias on reported earnings is accounted for, the CAPE Ratio is shown to underestimate the likely equity returns in the future.
Overall, the empirical evidence points to a poor out-of-sample performance of the CAPE Ratio as a tactical allocation tool. One possible hypothesis is that using the historic average of the CAPE Ratio as the benchmark might be too simplistic. The denominator for the CAPE Ratio already utilized the average EPS of the S&P 500 Index. Using the historic average of a measure based on average would impose no growth on the average for extended period. In the next section, I will show that a simple change in the P/E ratio equation can pave the way for incorporating growth and changes in risk-free rate. I will use the new equation to calculate the P/E ratio and compare it with the true P/E ratio. The difference between the true P/E ratio and the theoretical value given by the new equation is the tracking error. I will then show how to incorporate the tracking error and adjust the growth rate of dividends and changes in the risk-free rate to come up with a more appropriate value for the P/E ratio.
3. The Derivation of the Tracking Error Approach
To understand how the tracking error approach works, we start with the connection between the P/E ratio given by the Constant (Gordon) Growth Model (
Gordon 1959) and the CAPE Ratio formula.
The Constant (Gordon) Growth Model is
where
b is the retention rate,
ROE is the return on equity,
D1 =
EPS1 ×(1 −
b), and
g =
ROE ×
b.
If we divide the equation above by
EPS1, we obtain
ROE ≠ r and
ROE ×
b < r.
The left side of the equation above is known as the forward P/E ratio. Since the required return is in the denominator, declines in required return will push the P/E ratio upward.
Since
EPS1 = EPS0 × (1 +
g), we can rewrite Equation (2) as
Equation (3) expresses trailing P/E ratio as a function of plow-back ratio (1—payout ratio), the discount rate, and the growth rate. Increases in the plow-back ratio (which lead to a higher growth rate) will lead to an increase in the P/E ratio as well. Similarly, reductions in required return will have similar effects.
To see the connection between CAPE and Equation (3), we can replace the trailing 1-year EPS with trailing 10-year average EPS adjusted for inflation, and then we can rewrite the P/E ratio in CAPE Ratio form:
where
EPS10 is the trailing 10-year average EPS adjusted for inflation,
g10 is the average growth rate over the last 10 years, and
b10 is the average plow-back ratio over the last 10 years. The substitution of the 1-year growth rate and EPS for the 10-year figures might seem unreasonable. But the above exercise is just to show how the two formulas are related. The number we obtain using Equation (3) will not be the same as the true P/E ratio for the S&P500 Index. Likewise, the number we obtain with Equation (4) will not be exactly like the real CAPE Ratio. But the downward (or upward) adjustment we need to make for the value we can obtain with Equation (3) can be applied to Equation (4) as well.
Another useful feature of using this tracking error approach is that the time frame under consideration in Equation (4) can be easily adjusted. With rapid changes in the components of the S&P 500 Index that have the most weights, 10 years might seem like an eternity, whereas a shorter time frame from 1 year to 3 years might be more appropriate. Since the structural changes in the S&P500 Index in recent years favor high-growth and a high plow-back ratio, the 10-year time frame imposed by the CAPE Ratio might be too restrictive (this could explain the relatively poor predictive power in recent decades). Likewise, the Federal Reserve’s policies related to the interest rate also adjust much more frequently than the 10-year time frame imposed by the CAPE Ratio. Therefore, both the discount rate and growth rate should be adjusted more frequently, which can be accomplished in our model.
4. Data and Analysis
We obtained data from Shiller’s website that keep track of the CAPE Ratio.
Table 1 shows the summary statistics.
The argument that we should compare the current CAPE Ratio value to the historic value is also highly questionable according to the summary statistics. None of the sub-sample appears to be from the same distribution as the full sample. If we break the data into two periods, before and after the Fed’s active management of inflation rate, we can see drastically different values for the two periods. For the historic period from 1881 (when the first CAPE Ratio value is available) to July of 2023, the average is 17.3. However, the average value for CAPE Ratio pre-1983 was 14.69, while the value for post-1983 was 24.15, which is 58% higher than the average in the pre-1983 period. We choose 1983 as the structural break point because that is when the Fed started to focus on controlling inflation more aggressively and T-bill returns started to decline and have stayed at a historic low level ever since. Moreover, there has been a steady increase in the average and minimum values, while maintaining a relatively stable standard deviation since 2001. The year 2010 is a major milestone for the S&P500 in terms of structural change as it was the beginning of the tech sector’s domination in the market cap weight.
A simple regression analysis (see
Table 2) shows that the slope for the time trend was positive 0.42 for the CAPE Ratio since 1983, which was compared to 0.083 for the entire data sample. The intercept term also went up from 11.43 to 14.73 during that period.
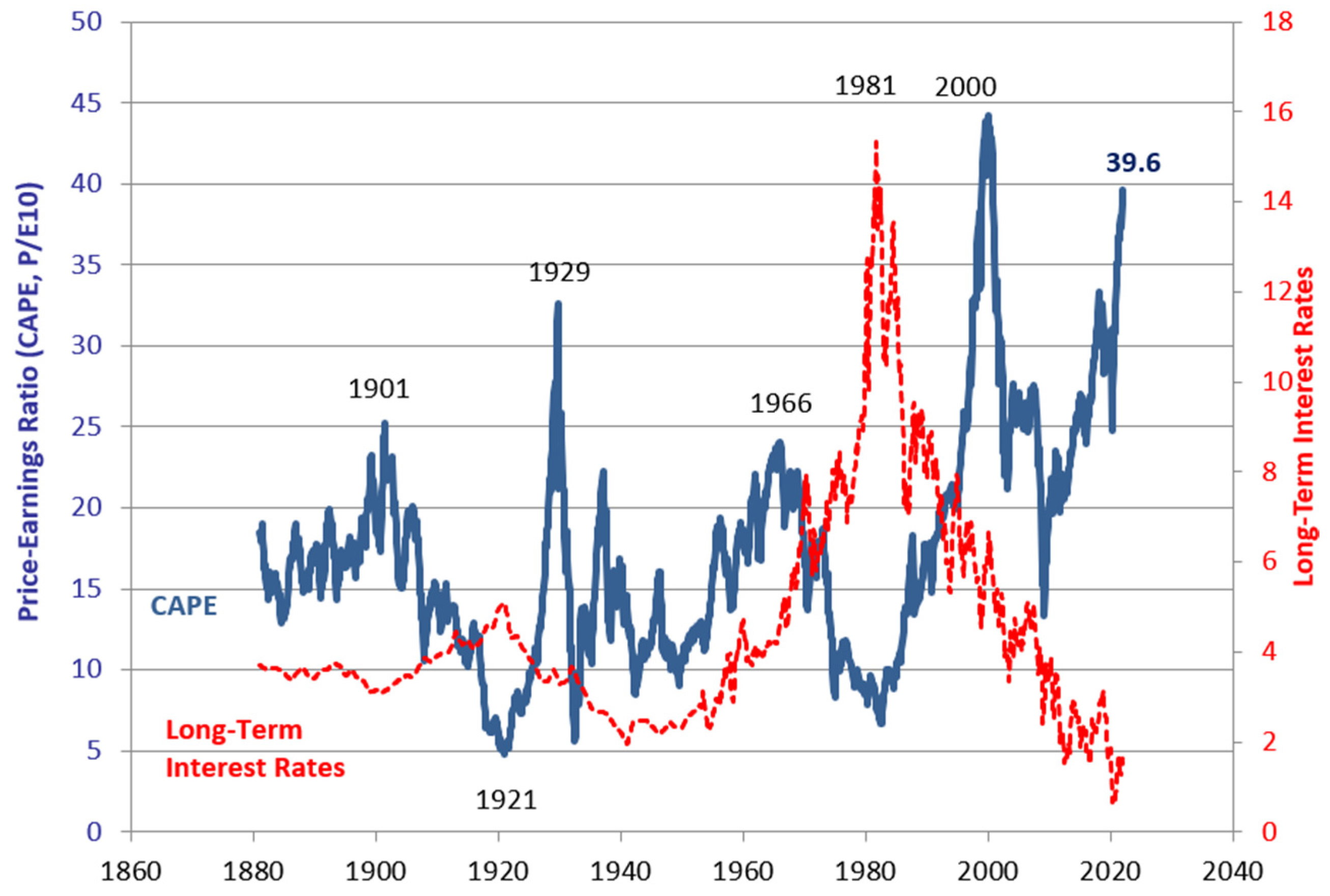
Evidence of structural change in the CAPE Ratio is also visible in the data provided by Shiller. In the CAPE–long-term interest rates plot (
Figure 1), there is a more stable positive correlation between the CAPE Ratio and long-term interest rates prior to the Oil Crisis in 1975. Since 1975, the correlation has been negative, as the Fed started to play a more active role in maintaining the level of inflation. As the interest rates started to decline, the value of the CAPE Ratio kept on rising except during the 3 years following the burst of the DotCom Bubble and the 2008 Financial Crisis.
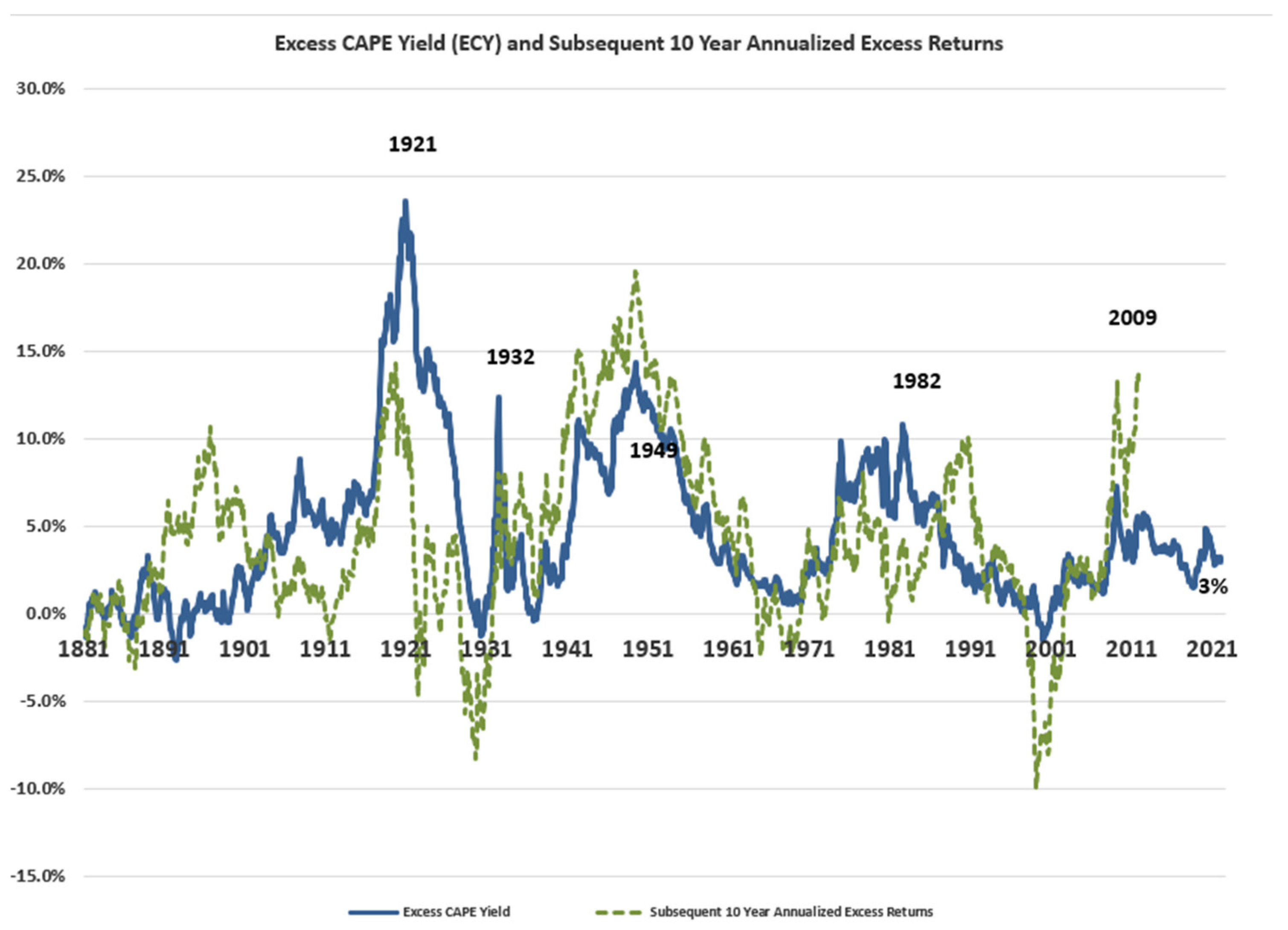
Figure 2 shows the relationship between the Excess CAPE Yield (ECY) and the subsequent 10-year annualized excess returns. The ECY is defined as the inverse of the CAPE Ratio minus the 10-year real interest rate. If the value for the ECY is high, the CAPE Ratio would be low. If the CAPE Ratio is a useful tool for predicting future 10-year excess returns, it should have a positive lead effect. However, the data show no clear sign of the lead–lag effect throughout the entire period. As mentioned, in previous sections, I found that the correlation coefficient between CAPE Ratio and 10-year excess returns is close to zero for the entire data sample. From 1952 onward, the correlation coefficient is positive 0.67. It suggests that higher values of CAPE Ratio will lead to higher excess returns for the next 10 years, contrary to what the CAPE Ratio aims to predict.
After the dust of the 2008 Financial Crisis settled, the components of the S&P500 Index also began to change substantially. Historically, companies in the top 10 of the S&P 500 Index in terms of market capitalization accounted for less than 20% of the index value prior to 2010 (although they briefly surpassed 20% in 2000). By the end of 2020, the top 10 companies in the S&P 500 Index accounted for more than 30% of the market capitalization due to the inclusion of companies in the tech industry that typically do not pay dividends. Moreover, these large companies typically had much lower earnings in the past and very high projected earnings growth rates going forward than their non-tech counterparts do. As such, they command much higher P/E multiples than most stocks do. Six of the top ten companies by market capitalization in 2010 no longer made the list in 2020. XOM, GE, CVX, IBM, WMT, and PG were replaced by AMZN, TSLA, FB, GOOGL, JJ, and JPM. Seven of the top ten companies in the S&P500 Index paid dividends in 2010. Only four of the top ten paid dividends in 2020. Not coincidental, companies that do not pay dividends typically have a higher EPS growth rate.
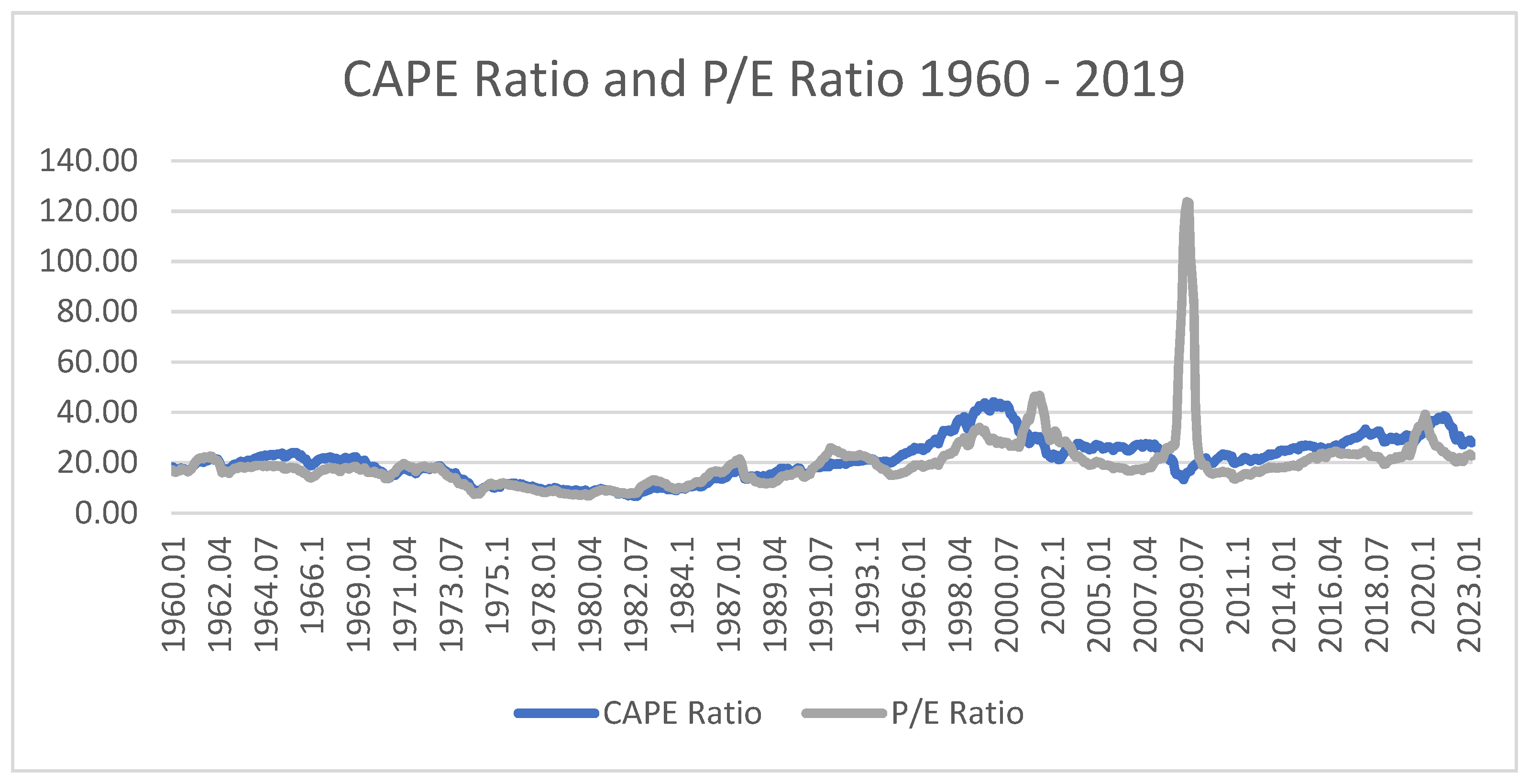
One noticeable company (as of August 2023) is Nvidia (NVDA). In 2010, Nvidia had total net income of USD 0.254 billion. By the 2023 fiscal year, the net income was USD 10.32 billion. The company had compounded annual earnings growth rate of 61.7% over 12.5 years and pays 0.02% net income as its dividend. Nvidia did not make it to the S&P500 Index until 2016, and it replaced JJ by 2021. Four of the top ten companies in the S&P500 made no meaningful profit before 2010 (AMZN, FB, NVDA, and TSLA). The earnings for AMZN, FB, and GOOGL can swing wildly, and their revenues affect NVDA greatly since NVDA is their main supplier for computer chips. These structural changes in the S&P500 Index means that the P/E part of the CAPE Ratio must have changed as well. However, the correlation between CAPE Ratio and P/E ratio broke down long before 2010.
Figure 3 shows that the deviation from long-term correlation between the two measures might have started as early as 1990.
With the observed structural changes in mind, we can start to analyze the effects of changes in the payout ratio and growth rate on the P/E ratio. Since Equation (4) is flexible in terms of time, we can use the right-hand side of Equation (4) with different values from 1960 to 2019. The average payout ratio for the S&P 500 in 1960–2019 was 44.52. We used 1960 as the cut-off point because it would give us 20 years of data when the CAPE Ratio and interest rate are positively correlated. The majority of the rapid changes in the S&P500 components took place after the 1990s. The average payout ratio from 1960 to 1990 was 50.92, and the average payout ratio from 1991 to 2019 was 37.68. Beginning in 2010, the average payout ratio dropped to 35.2%. The annual growth rate of dividends from 1960 to 2019 was 5.92%. Since 2003 (after the DotCom Bubble), it has been 7.82% (the earnings growth rate since 2010 has been a staggering 11.18%, which is about twice as much as the historic average). The S&P 500 has a return of 10.03% (capital gain + dividend). These values give us a baseline historic P/E of 11.47 for the S&P 500 Index based on the Constant Growth Model. Of course, the S&P 500 Index had an average P/E of 16.61 during this period, not 11.47. Therefore, the Constant Growth Model only captures 69.05% of the real P/E value for the S&P500 Index over the last 60 years.
Next, we looked at the values for payout ratio and earnings growth rate in the most recent decade. From 2010 to 2019, the S&P500 Index has a payout ratio of 35.2%, with an earnings growth rate of 11.18% and an average annual return of 13.04% (note that we had to use the 13.04% rate of return rather than the average return since the growth rate is higher than the average return). Plugging these values into Equation (4), the P/E ratio for the last 10 years should be 21.01. When comparing this value to the historic average of 11.47, the last 10 years P/E ratio value should be 83.17% above the historic value. Therefore, if the P/E ratio is 16.6 for the S&P 500 over the last 60 years, the CAPE Ratio for the last 10 years should be 30.4 () for the market to be considered fairly valued.
The calculations I performed for the 2010–2019 period are made with the assumption that the required return is 13.04%, which is more than 3% above the historic average. The earnings growth rate of 11.18% is also much higher than the historic average. A total of 3% above historic average return is also not a reasonable assumption going forward. If I reduce both values by 3% and assume a 35% payout ratio, I still obtain a fair value P/E of 20.47 relative to the 11.47, which is a 77% increase compared to that of the historic average. This gives us a 29.38 as the fair value for the CAPE Ratio. The CAPE Ratio at the end of 2019 was 28.34, which is below the fair value found with equation (4) under a set of more conservative assumptions.
An alternative approach to track the errors between the P/E ratio and CAPE Ratio is by comparing the historic average and recent trends.
Table 3 summarizes the results from 1960–2019. The historic average P/E ratio has a value of 16. The historic average for the CAPE Ratio until 2019 was 16.61, resulting in an error of 10%. The errors show a steady increase from 1960 to the most recent 10-year average at 43.7%. This trend is also a good indication that there are structural changes in these ratios.
5. Adjusting for the Impacts of Interest Rate
Another major structural change in the financial market over the last few decades was enacted by the Federal Reserves, which set the policies that affect the risk-free rate. The risk-free rate has been about 3% on average over the last 100 years. However, the risk-free rate has been kept historically low for extended periods since the 2008 Financial Crisis to stimulate economic growth (and inflation). Under a low risk-free rate environment, if we keep the equity risk premium the same as the historic average of 7.02%, the required return for risky assets should not be the 10.02% used in my previous calculations. If we assume that the risk-free is at 1%, thus giving us a required return for risky assets of 8.02%, we obtain a baseline P/E ratio of 22.33, which is almost twice as high as the historic average based on Equation (4). Therefore, a CAPE Ratio of 32 would be considered a bargain compared to the value I obtained with the tracking error ().
Of course, when the risk-free rate does go up to around 2% (the average over the last two decades), the P/E ratio will be substantially lower than 22.33 (down to 13.49 to be exact) if we assume that the 7.02% risk premium holds. However, if we consider the downward trend in the risk premium we observed in recent years and assume that risk premium does settle at 5.5%, then we can have close to a 4.5% risk-free rate and still be able to obtain the 20 or higher value for the P/E ratio (for the S&P500 Index), or 30 or higher for the CAPE Ratio, and the market is still considered fairly valued. Therefore, unless the CAPE Ratio is substantially higher than 30, there is no reason (if there is ever) for investors to get out of the equity market in hope of potentially be able to get back in at a much lower price level after the burst of the “market bubble”. However, if the market risk premium and/or the risk-free rate were to increase only by small value, the implied CAPE can be substantially lower. If the risk-free rate goes up to 4.5%, the implied CAPE will be 20.46. In a volatile market, both the risk premium and risk-free values can increase at the same time, causing the CAPE’s fair value to decline even more drastically. In a hypothetical situation where the risk-free rate is at 4.5% and the risk premium is at 8%, the fair value for the CAPE Ratio will decline to 16.
Table 4 provides a summary of the impacts of rising interest rate and changes in the growth rate have on the CAPE Ratio. In
Table 5, we assume a baseline assumption of 7.6% for the dividend growth rate, 7% for the risk premium, and a payout ratio of 35.6%. At a historic low average interest rate of 2%, the implied fair value for the CAPE Ratio is 27.36. However, at 3% (the historic average), the fair value for the CAPE Ratio is 16 (which is the historic level for the average CAPE Ratio). In
Table 5, we use a higher growth rate (the average from the most recent 10 years and the last 20 years) assumption. If we use a higher growth rate for dividends, the base level for the risk-free rate had to be at least 3% for the CAPE Ratio to have a positive value. At a 3% risk-free rate, the CAPE Ratio would be 77.96! It would take the risk-free rate going up to 5% for the CAPE Ratio to be brought down to the historic average. At a 6% risk free rate, however, the implied CAPE Ratio will be 11.14.
In summary, using the tracking error approach I proposed in this paper, when the structural change in the S&P500 Index and persistently low-interest environment from 2008 are considered, I found that the value of CAPE Ratio can be substantially higher than the historical average. The result shows that P/E ratio for the S&P500 should be 34.7% higher that the historic average since 1983. For the period between 2010 and 2019, the P/E ratio for the S&P500 should be 83.17% higher than the historic average. Therefore, the CAPE Ratio value for the S&P500 to be considered “average” could be as high as 30.42. Through induction, my findings in this paper suggest that the relatively high CAPE Ratio in recent decades (particularly over the last decade) can be justified because of the higher earnings growth rates among the stocks in S&P500 Index and lower interest rate environment we have witnessed in recent decades. However, the CAPE Ratio is very sensitive to changes in the risk premium, the risk-free interest rate, and the growth rate assumptions. Because of the CAPE Ratio’s restriction on having the 10-year moving average earnings as the denominator, its predictive performance is not as good as the simple P/E ratio since the simple P/E ratio allows for much shorter periods. In short, investors should focus on the future growth rate of earnings and the direction of future interest rates rather than the relative value of the CAPE when making asset allocation decisions.
6. Conclusions
Relatively high values for the CAPE Ratio are often cited as causes for concern about a pending market bubble. Fearing an imminent crash due to a high value of CAPE Ratio, investors might be tempted to rotate out of equities into bonds and cash, or they might wait for the market to crash before getting back into equities. These types of market-timing practices can have significant negative impacts on investment returns in the long run. As I have shown in this paper, outside of the three major catastrophic market events in the US equity market over the last 100 years, the performance of the CAPE Ratio as a market value predictor has been relatively muted. Other critics have pointed out that the changes in the structure of the S&P500 Index and the US economy have made the CAPE Ratio out of date. In this paper, I show that using a simple tracking error approach with the P/E ratio implied by the Gordon Growth Model and making changes to the growth rate and dividend payout ratio assumptions can account for most of the upward trend in the CAPE Ratio in recent decades.
As such, when forecasting future returns on equities, investors should pay more attention to the effect of risk-free rate on the total risky asset returns and the earnings growth rate rather than the implied future yield made by the CAPE Ratio. In other words, market timing using the CAPE Ratio is not as useful as popular financial journalists might have suggested. Of course, the best practice for achieving a good, long-term financial performance is using dollar cost averaging and stay in the market through ups and downs rather than trying to time the market. Rotating out of equities into alternative assets to capture the alpha might lead to underperformance. If the investors still want to use the CAPE Ratio as a market-timing tool, they should consider the new average value from 2010 with a time trend of 0.42, not the historic average of 17.3.
Suggestions for future research: The results in this paper show that the P/E ratio can capture structural changes more rapidly than CAPE Ratio can. One possible idea is to investigate if the predictive performance of using shorter time-frame CAPE ratios (e.g., using 5-year or 3-year, rather than 10-year ones) is better. Alternatively, researchers can investigate what other factors are driving the structural changes. These could be trading frequencies, holding periods, or investor reactions to good or bad news.