Research on Elastic–Plastic Contact Behavior of Hemisphere Flattened by a Rigid Flat
Abstract
:1. Introduction
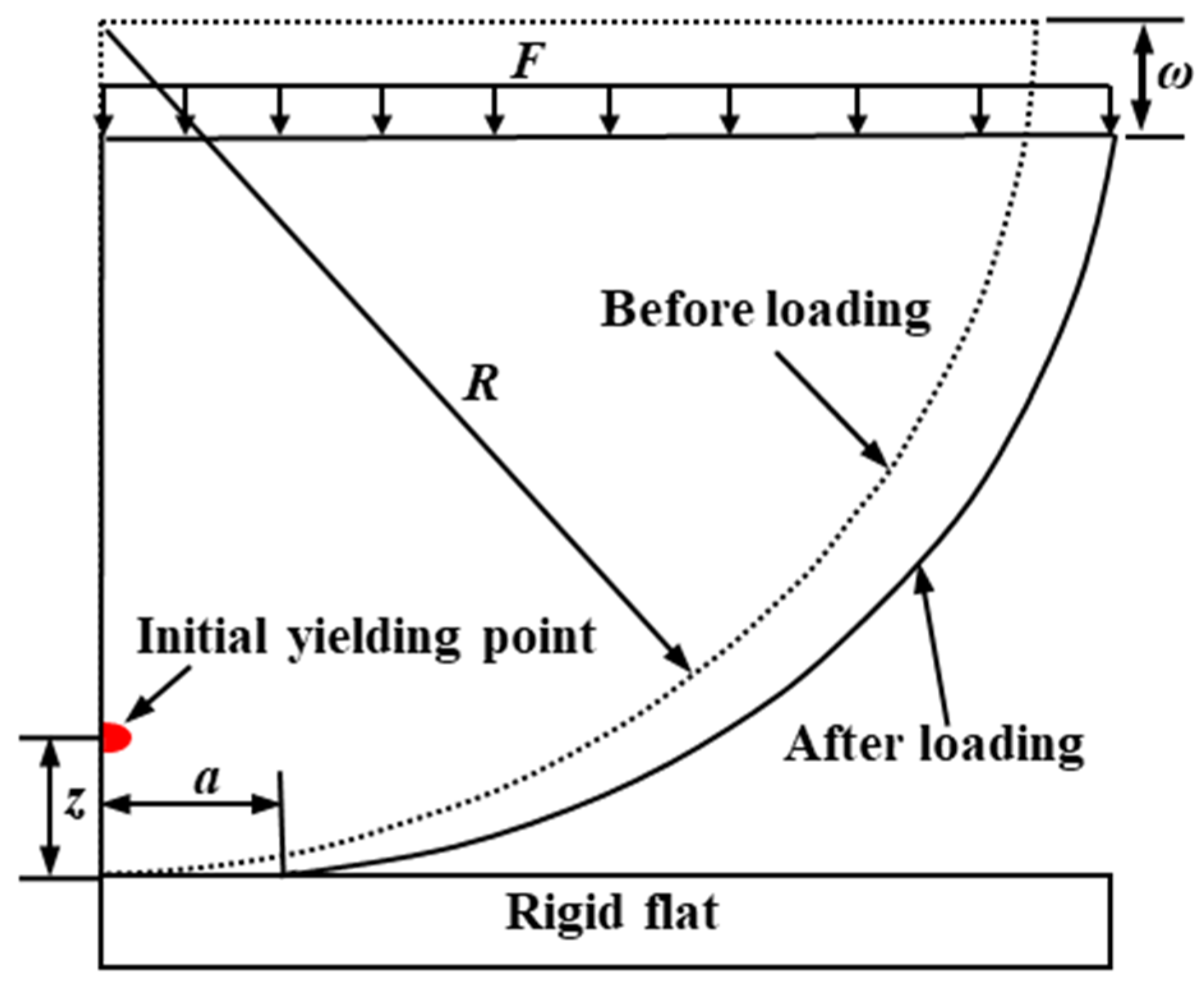
2. Critical Formula
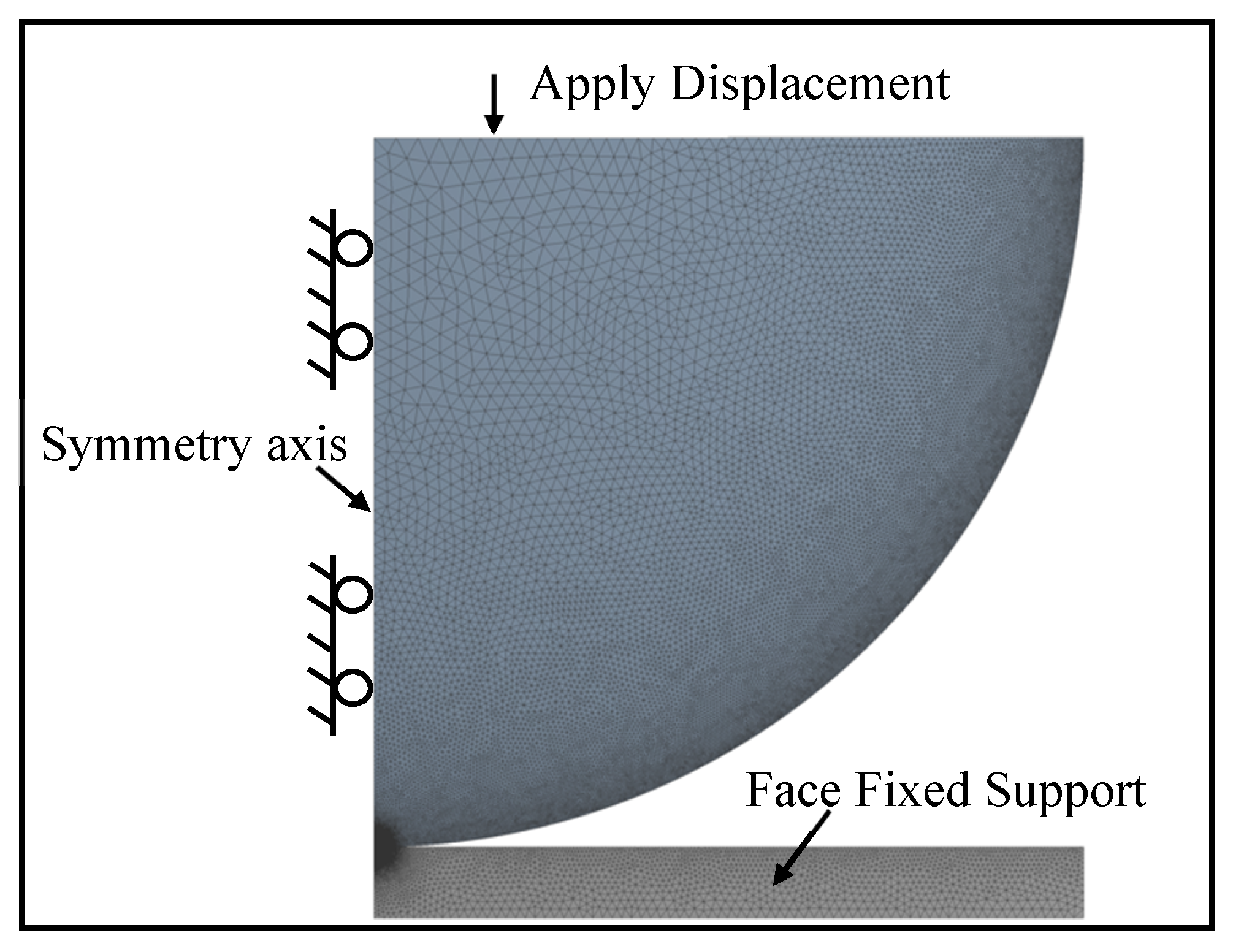
3. Finite Element Model
4. Results and Discussion
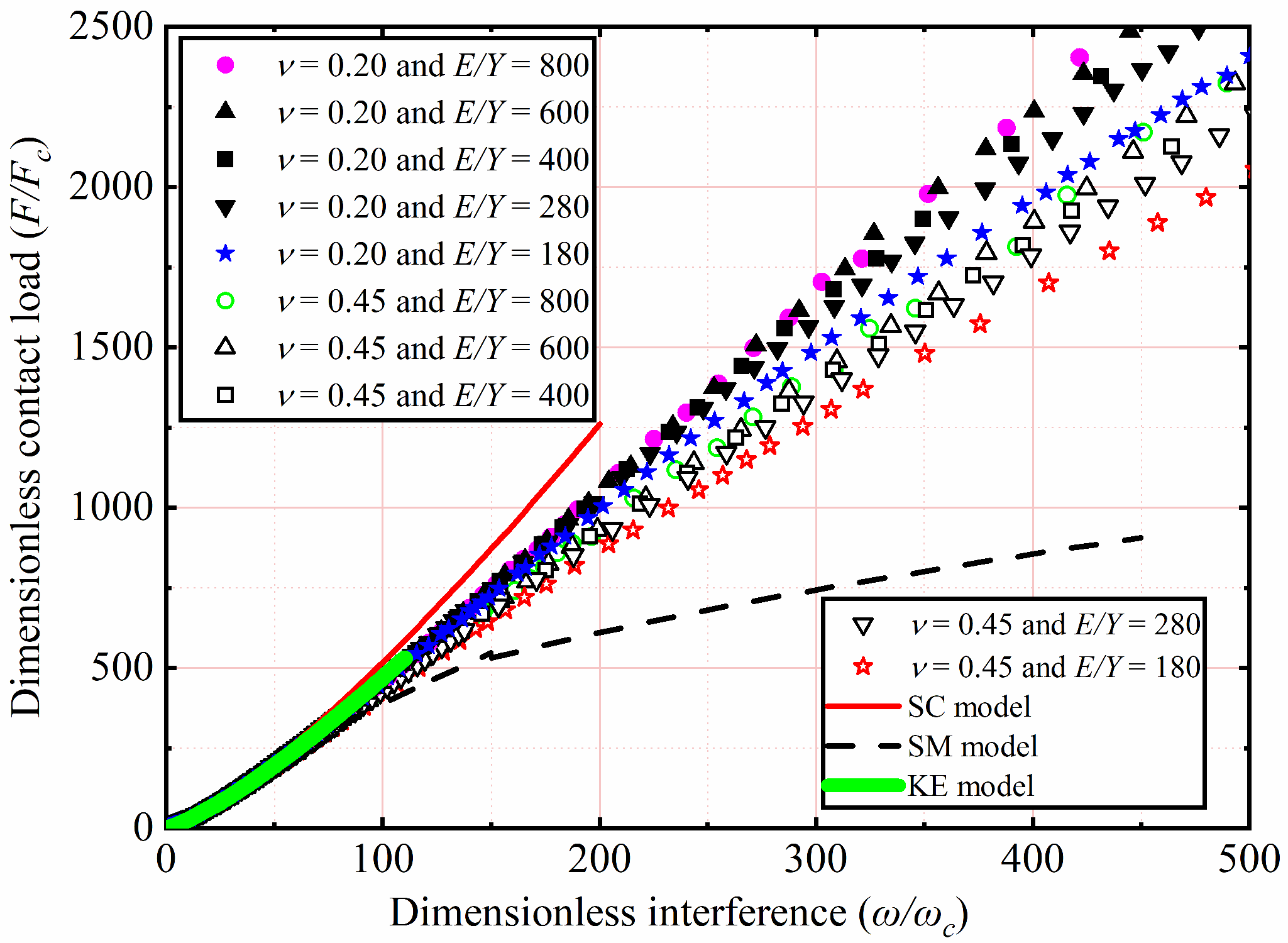
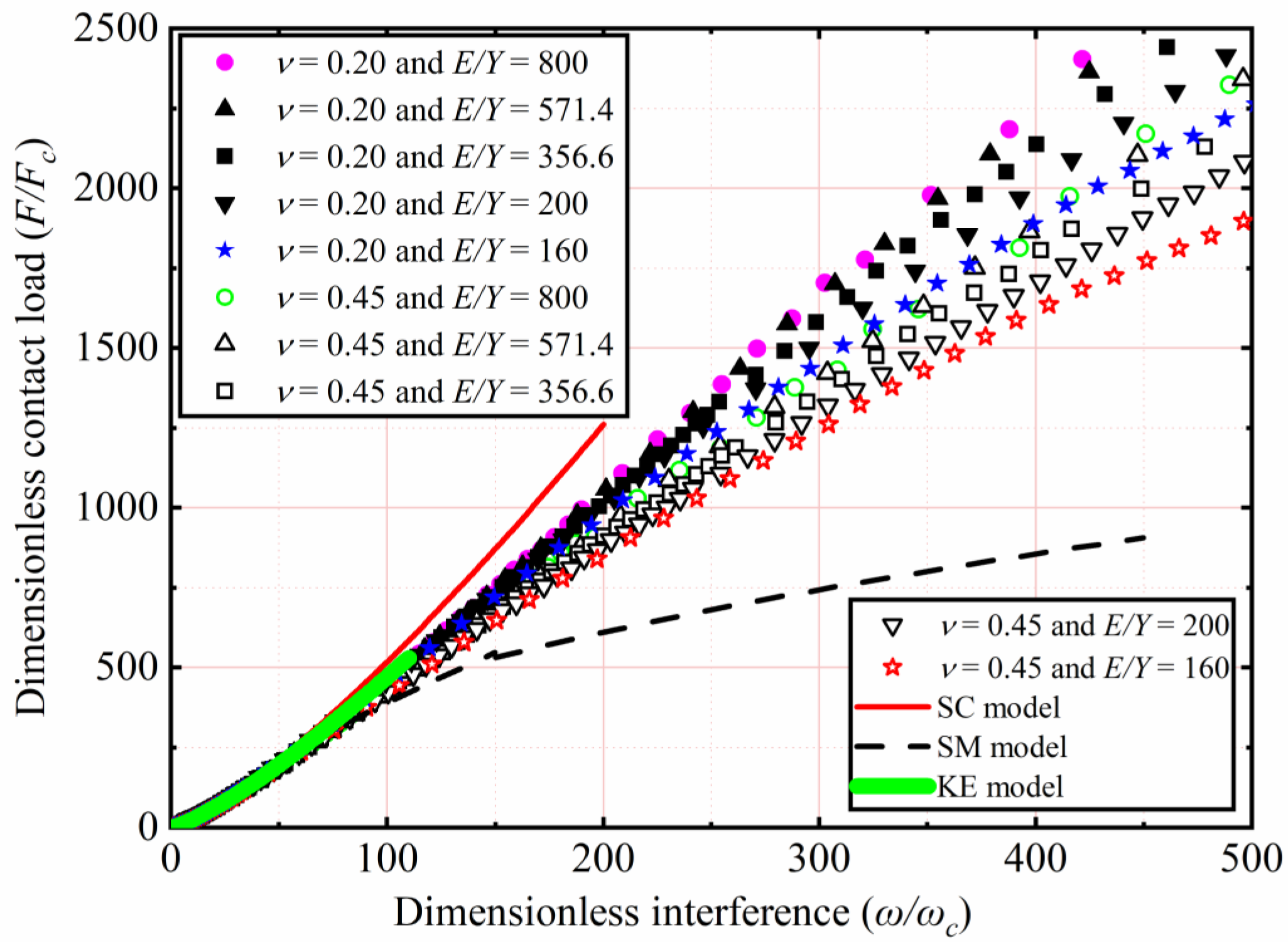
4.1. Contact Load
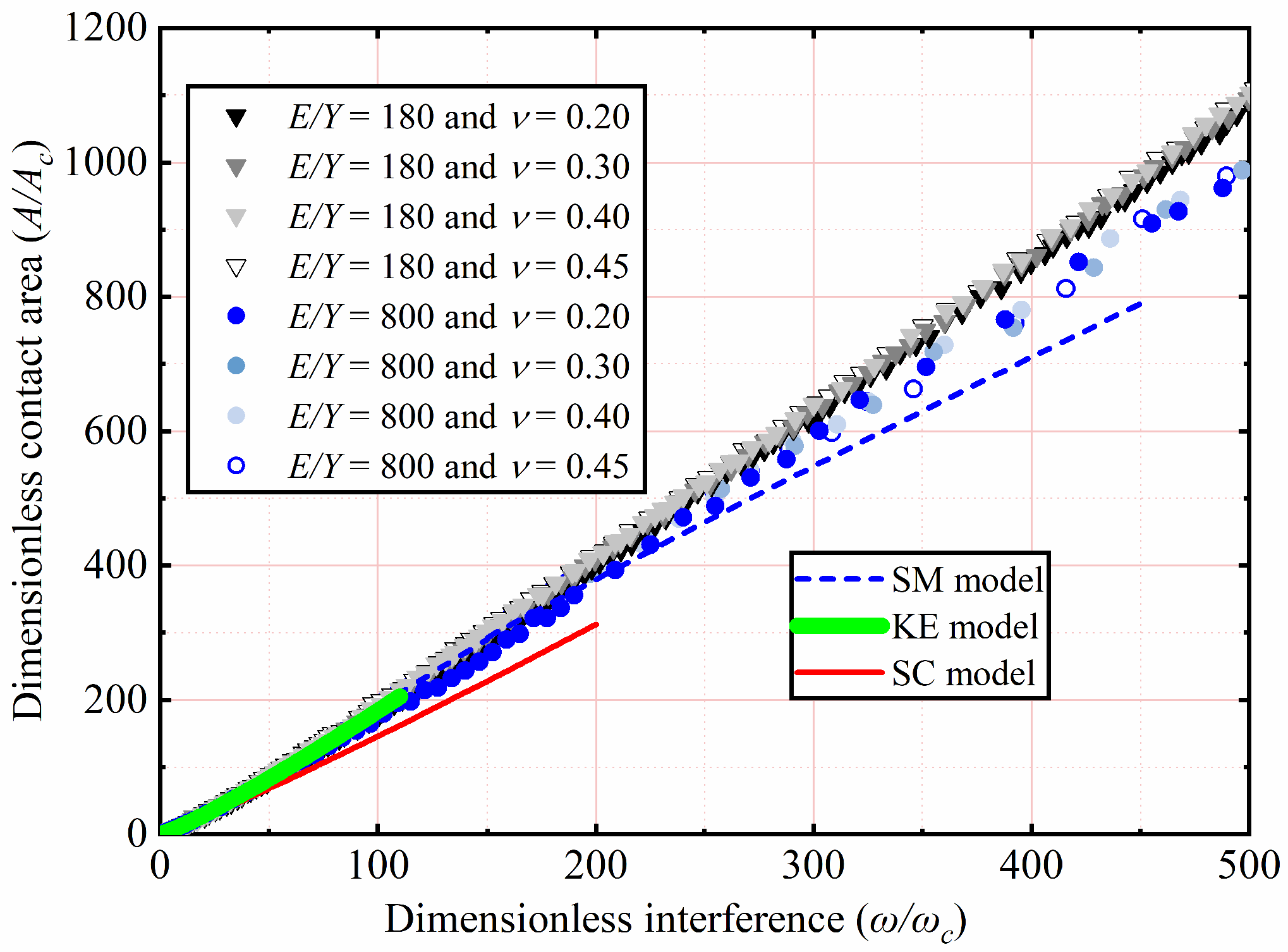
4.2. Contact Area
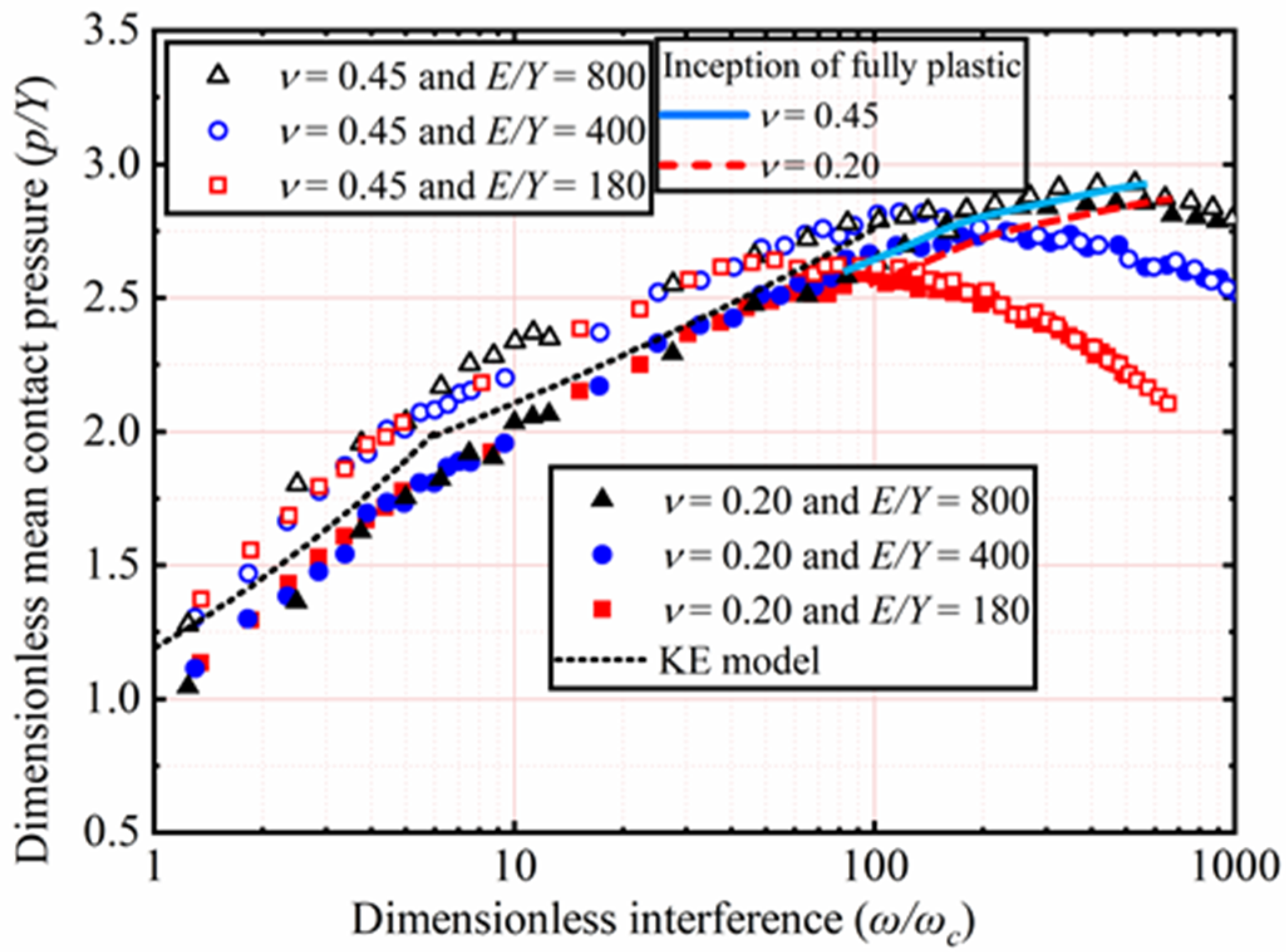
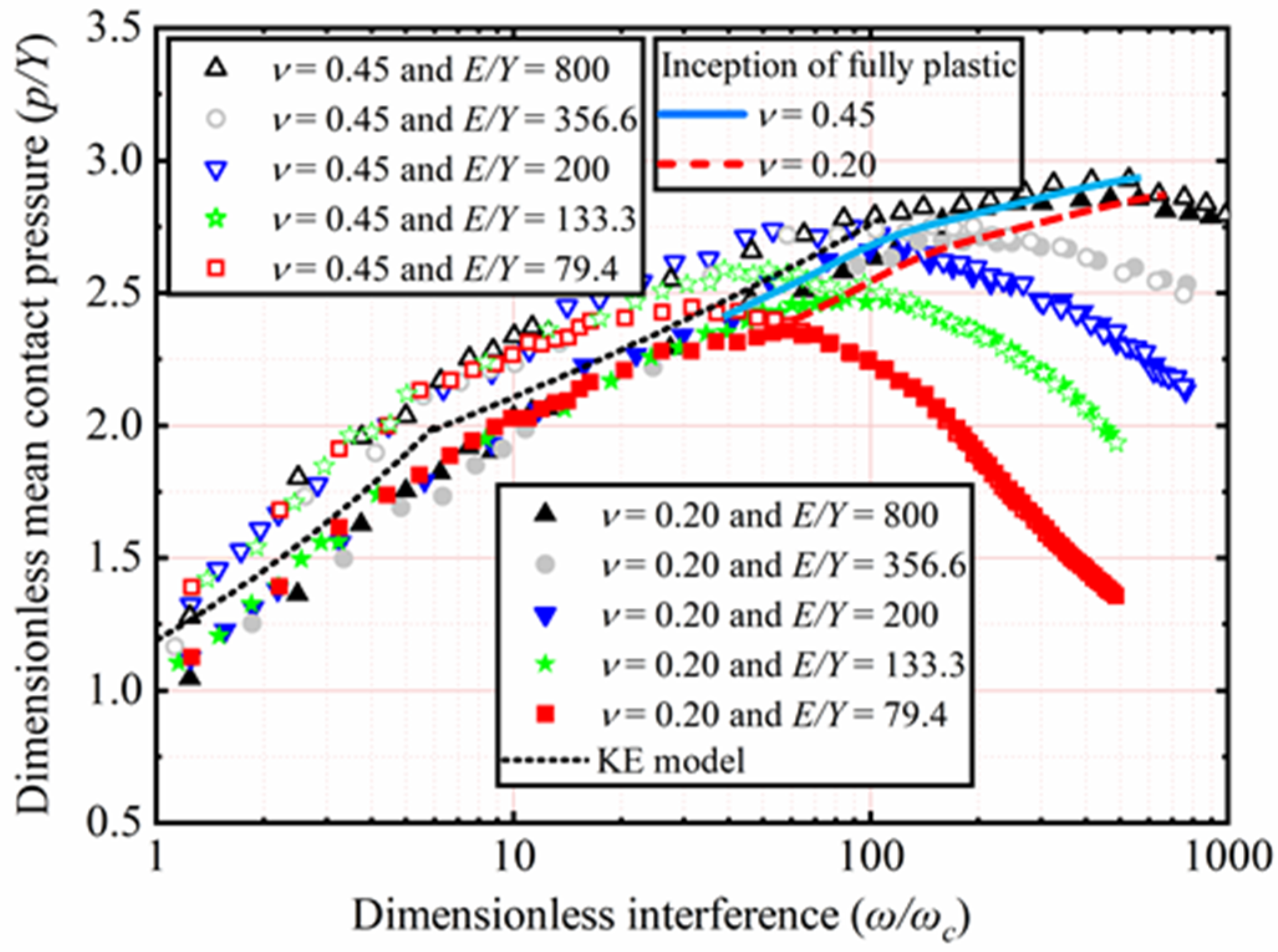
4.3. Contact Pressure
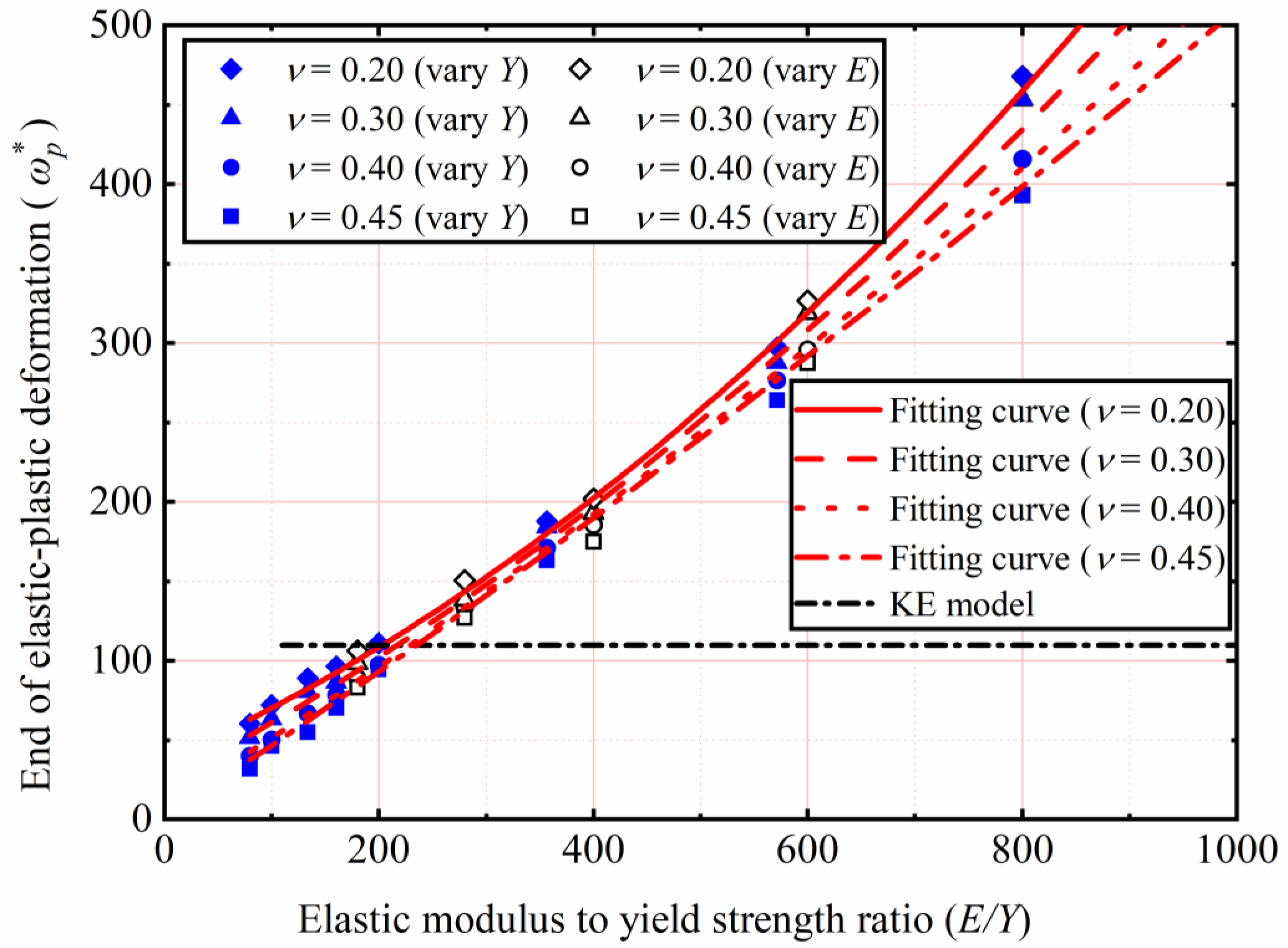
4.4. Elastic–Plastic Range
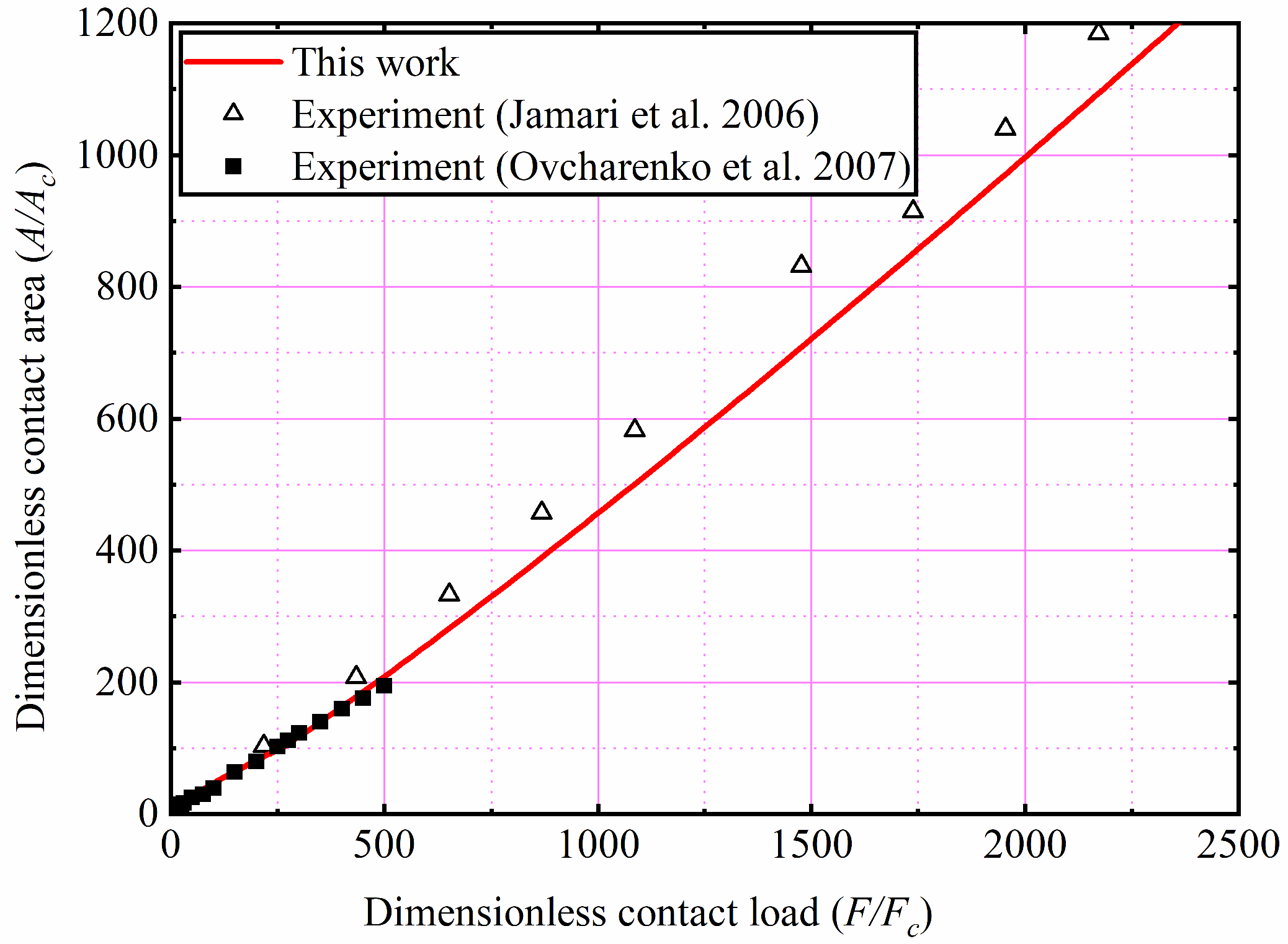
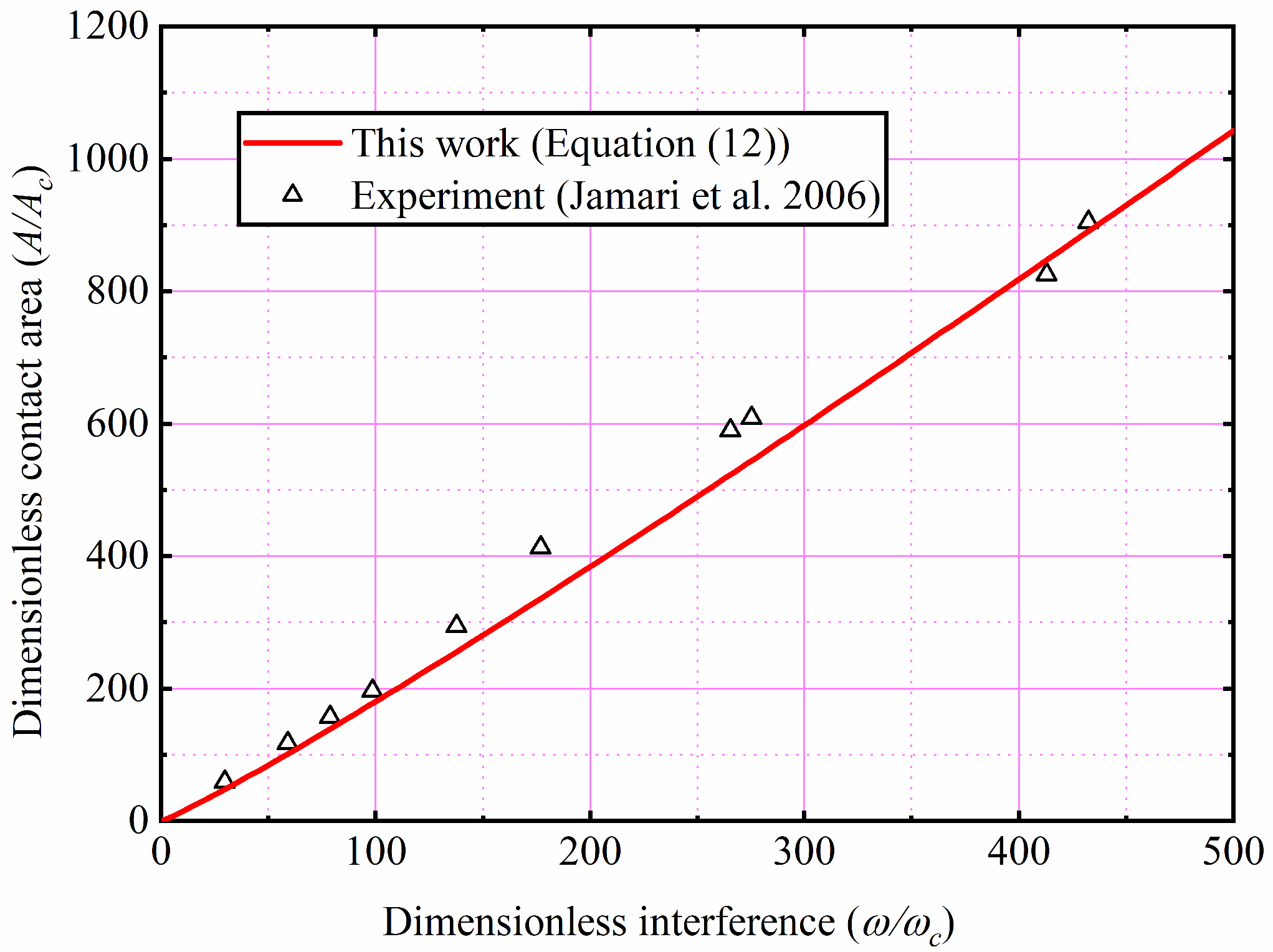
4.5. Comparison with Experimental Results
5. Conclusions
- (1)
- The present study considers a large range of dimensionless interference from 1 to 500. A new elastic–plastic constitutive model is proposed to predict the contact area and load based on the curve fitting the finite analyses results. Compared with previous models and experiments, the rationality of the present model is verified.
- (2)
- p/Y is mainly affected by the ν in the elastic–plastic range, and the higher the ν, the higher p/Y. However, this influence disappears in the fully plastic range. The maximum of p/Y is not a constant value for the different E, Y, and ν. The higher E/Y, the higher the maximum of p/Y is. Moreover, the higher ν, the higher the maximum value of p/Y is when E/Y is constant. However, the effects of ν are less than that of E and Y for the maximum value of p/Y. In the plastic range, p/Y decreases with increasing interference. Additionally, the lower E/Y, the more noticeable this trend is.
- (3)
- The boundaries between the elastic, elastic–plastic, and fully plastic deformation regimes are determined according to the interference, maximum mean contact pressure, Poisson’s ratio, and the elastic modulus to yield strength ratio. When the interference is small, the contact state is purely elastic, and the contact parameters can be calculated according to the Hertz formula. When the interference increases, the contact state changes from the purely elastic to the elastic–plastic. The present work shows that the JG model can more reasonably determine the inception of elastic–plastic deformation regime. The end of the elastic–plastic deformation regime is defined according to the interference corresponding to the maximum contact pressure. New dimensionless constitutive relationships are proposed to predict the contact parameters in the elastic–plastic range.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a | Contact radius |
| p | Mean contact pressure |
| p0 | Maximum Hertzian pressure |
| A | Contact area |
| C | Critical yield stress coefficient |
| E | Equivalent elastic modulus |
| F | Contact load |
| Critical interference value at the inception fully plastic | |
| R | The radius of asperity |
| Y | Yield strength |
| ν | Poisson’s ratio |
| ω | Normal interference of asperity |
| Scripts | |
| 1 | The value of asperity |
| 2 | The value of rigid flat |
| c | Critical values at yielding inception |
| p | Critical values at the end of the elastic–plastic range |
| * | Dimensionless |
| e | Elastic range |
| ep | Elastic–plastic range |
References
- Wang, H.; Yin, X.; Hao, H.; Chen, W.; Yu, B. The correlation of theoretical contact models for normal elastic-plastic impacts. Int. J. Solids Struct. 2020, 182, 15–33. [Google Scholar] [CrossRef]
- Edmans, B.D.; Sinka, I.C. Unloading of elastoplastic spheres from large deformations. Powder Technol. 2020, 374, 618–631. [Google Scholar] [CrossRef]
- Johnson, K.L. Contact Mechanics; Cambridge University: Cambridge, UK, 1985. [Google Scholar]
- Ghaednia, H.; Wang, X.; Saha, S.; Xu, Y.; Sharma, A.; Jackson, R.L. A review of elastic-plastic contact mechanics. Appl. Mech. Rev. 2017, 69, 30. [Google Scholar] [CrossRef] [Green Version]
- Weng, P.; Yin, X.; Hu, W.; Yuan, H.; Chen, C.; Ding, H.; Yu, B.; Xie, W.; Jiang, L.; Wang, H. Piecewise linear deformation characteristics and a contact model for elastic-plastic indentation considering indenter elasticity. Tribol. Int. 2021, 162, 107–114. [Google Scholar] [CrossRef]
- Li, B.; Li, P.; Zhou, R.; Feng, X.; Zhou, K. Contact mechanics in tribological and contact damage-related problems: A review. Tribol. Int. 2022, 171, 107534. [Google Scholar] [CrossRef]
- Heath, C.E.; Feng, S.; Day, J.P.; Graham, A.L.; Ingber, M.S. Near-contact interactions between a sphere and a plane. Phys. Rev. E 2008, 77, 1110–1114. [Google Scholar] [CrossRef]
- Li, L.; Etsion, I.; Talke, F.E. Elastic–plastic spherical contact modeling including roughness effects. Tribol. Lett. 2010, 40, 357–363. [Google Scholar] [CrossRef] [Green Version]
- Updike, D.P.; Kalnins, A. Contact pressure between an elastic spherical shell and a rigid plate. J. Appl. Mech. 1972, 39, 1110–1114. [Google Scholar] [CrossRef]
- Kutzner, I.; Trepczynski, A.; Heller, M.O.; Bergmann, G. Knee adduction moment and medial contact force—facts about the correlation during gait. J. Biomech. 2012, 45, S381. [Google Scholar] [CrossRef]
- Chu, N.R.; Jackson, R.L.; Wang, X.; Gangopadhyay, A.; Ghaednia, H. Evaluating elastic-plastic wavy and spherical asperity based statistical and multi-scale rough surface contact models with deterministic results. Materials 2021, 14, 3864. [Google Scholar] [CrossRef]
- Gao, Z.; Fu, W.; Wang, W.; Kang, W.; Liu, Y. The study of anisotropic rough surfaces contact considering lateral contact and interaction between asperities. Tribol. Int. 2018, 126, 270–282. [Google Scholar] [CrossRef]
- Yuan, Y.; Cheng, Y.; Liu, K.; Gan, L. A revised Majumdar and Bushan model of elastoplastic contact between rough surfaces. Appl. Surf. Sci. 2017, 425, 1138–1157. [Google Scholar] [CrossRef]
- Ren, W.; Zhang, C.; Sun, X. Electrical contact resistance of contact bodies with cambered surface. IEEE Access 2020, 8, 93857–93867. [Google Scholar] [CrossRef]
- Angadi, S.V.; Jackson, R.L.; Pujar, V.; Tushar, M.R. A comprehensive review of the finite element modeling of electrical connectors including their contacts. IEEE T Comp. Pack. Man. 2020, 10, 836–844. [Google Scholar] [CrossRef]
- Jackson, R.L.; Ghaednia, H.; Elkady, Y.A.; Bhavnani, S.H.; Knight, R.W. A closed-form multiscale thermal contact resistance model. ieee transactions on components. IEEE T Comp. Pack. Man. 2012, 2, 1158–1171. [Google Scholar]
- Ghaednia, H.; Jackson, R.L.; Gao, J. A Third Body Contact Model for Particle Contaminated Electrical Contacts; IEEE: Piscataway, NJ, USA, 2014. [Google Scholar]
- Lontin, K.; Khan, M. Interdependence of friction, wear, and noise: A review. Friction 2021, 9, 1319–1345. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, C.; Hu, B.; Li, Y. An adhesive wear model of rough gear surface considering modified load distribution factor. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2022, 1, 135065012210748. [Google Scholar] [CrossRef]
- Ghaednia, H.; Jackson, R.L. The Effect of nanoparticles on the real area of contact, friction, and wear. J. Tribol. 2013, 135, 041603. [Google Scholar] [CrossRef]
- Gholami, R.; Ghaemi, K.H.; Silani, M.; Akbarzadeh, S. Experimental and numerical investigation of friction coefficient and wear volume in the mixed-film lubrication regime with ZNO nano-particle. J. Appl. Fluid Mech. 2020, 13, 993–1001. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Williamson, J.B.P. Contact of nominally fat surfaces. Proc. R. Soc. Lond. A 1966, 295, 300–319. [Google Scholar]
- Hertz, H. Ueber die Berührung Fester Elastischer Körper. J. Reine. Angew. Math. 1881, 92, 156–171. [Google Scholar]
- Lin, L.P.; Lin, J.F. An elastoplastic microasperity contact model for metallic materials. J. Tribol. 2005, 127, 666–672. [Google Scholar]
- Chang, W.R.; Etsion, I.; Bogy, D.B. An elastic-plastic model for the contact of rough surfaces. J. Tribol. 1987, 109, 257–263. [Google Scholar] [CrossRef]
- Chang, W.R.; Etsion, I.; Bogy, D.B. Adhesion model for metallic rough surfaces. J. Tribol. 1988, 110, 50–56. [Google Scholar] [CrossRef]
- Jamari, J.; Schipper, D.J. Experimental investigation of fully plastic contact of a sphere against a hard flat. J. Tribol. 2006, 128, 230–235. [Google Scholar] [CrossRef] [Green Version]
- Ovcharenko, A.; Halperin, G.; Verberne, G.; Etsion, I. In situ investigation of the contact area in elastic–plastic spherical contact during loading-unloading. Tribol. Lett. 2007, 25, 153–1607. [Google Scholar] [CrossRef]
- Sahoo, P.; Chatterjee, B. Effect of strain hardening on elastic-plastic contact of a deformable sphere against a rigid flat under full stick contact condition. Adv. Tribol. 2012, 2012, 1–8. [Google Scholar]
- Sahoo, P.; Chatterjee, B. Finite element based unloading of an elastic plastic spherical stick contact for varying tangent modulus and hardening rule. Int. J. Surf. Eng. Interdiscip. Mater. Sci. 2013, 1, 13–32. [Google Scholar]
- Kogut, L.; Etsion, I. Elastic-plastic contact analysis of a sphere and a rigid flat. J. Appl. Mech 2002, 69, 657–662. [Google Scholar] [CrossRef] [Green Version]
- Green, I. Poisson ratio effects and critical values in spherical and cylindrical hertzian contacts. Int. J. Appl. Mech. Eng. 2004, 10, 451–462. [Google Scholar]
- Jackson, R.L.; Green, I. A finite element study of elasto-plastic hemispherical contact against a rigid flat. J. Tribol. 2005, 127, 343–354. [Google Scholar] [CrossRef] [Green Version]
- Tabor, D. The Hardness of Metals; Clarendon Press: Oxford, UK, 1951. [Google Scholar]
- Quicksall, J.J.; Jackson, R.L.; Green, I. Elasto-plastic hemispherical contact models for various mechanical properties. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2004, 218, 313–322. [Google Scholar] [CrossRef]
- Kogut, L.; Komvopoulos, K. Analysis of the spherical indentation cycle for elastic-perfectly plastic solids. J. Mater. Res. 2004, 19, 3641–3653. [Google Scholar] [CrossRef]
- Brizmer, V.; Kligerman, Y.; Etsion, I. The effect of contact conditions and material properties on the elasticity terminus of a spherical contact. Int. J. Solids Struct. 2006, 43, 5736–5749. [Google Scholar] [CrossRef] [Green Version]
- Shankar, S.; Mayuram, M.M. A finite element based study on the elastic-plastic transition behavior in a hemisphere in contact with a rigid flat. J. Tribol. 2008, 130, 6. [Google Scholar] [CrossRef]
- Shankar, S.; Mayuram, M.M. Effect of strain hardening in elastic-plastic transition behavior in a hemisphere in contact with a rigid flat. Int. J. Solids Struct. 2008, 45, 3009–3020. [Google Scholar] [CrossRef] [Green Version]
- Malayalamurthi, R.; Marappan, R. Elastic-plastic contact behavior of a sphere loaded against a rigid flat, mech. Adv. Mater. Struc. 2008, 15, 364–370. [Google Scholar] [CrossRef]
- Sahoo, P.; Chatterjee, B. A finite element study of elastic-plastic hemispherical contact behavior against a rigid flat under varying modulus of elasticity and sphere radius. Engineering 2010, 2, 205–211. [Google Scholar] [CrossRef] [Green Version]
- Sahoo, P.; Chatterjee, B.; Adhikary, D. Finite element based elastic-plastic contact behavior of a sphere against a rigid flat—Effect of strain hardening. Int. J. Eng. Technol. 2010, 2, 1–6. [Google Scholar]
- Megalingam, A.; Mayuram, M.M. A comprehensive elastic-plastic single-asperity contact model. Tribol. T. 2014, 57, 324–335. [Google Scholar] [CrossRef]
- Megalingam, A.; Hanumanth Ramji, K.S. A complete elastic-plastic spherical asperity contact model with the effect of isotropic strain hardening. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2021, 235, 820–829. [Google Scholar] [CrossRef]
- Ghaednia, H.; Mifflin, G.; Lunia, P.; O’Neill, E.O.; Brake, M.R.W. Strain hardening from elastic-perfectly plastic to perfectly elastic indentation single asperity contact. Front. Mech. Eng. 2020, 6, 60. [Google Scholar] [CrossRef]
- Ghaednia, H.; Brake, M.R.W.; Berryhill, M.; Jackson, R.L. Strain hardening from elastic-perfectly plastic to perfectly elastic flattening single asperity contact. J. Tribol. 2019, 141, 11. [Google Scholar] [CrossRef] [Green Version]
- Ghaednia, H.; Pope, S.A.; Jackson, R.L.; Marghitu, D.B. A comprehensive study of the elasto-plastic contact of a sphere and a flat. Tribol. Int. 2016, 93, 78–90. [Google Scholar] [CrossRef]
- Ashby, M.F.; Jones, D.R.H. Engineering Materials 1: An Introduction to Properties, Applications, and Design, 4th ed.; Butter-worth-Heinemann: Amsterdam, The Netherlands, 2012; p. 472. [Google Scholar]
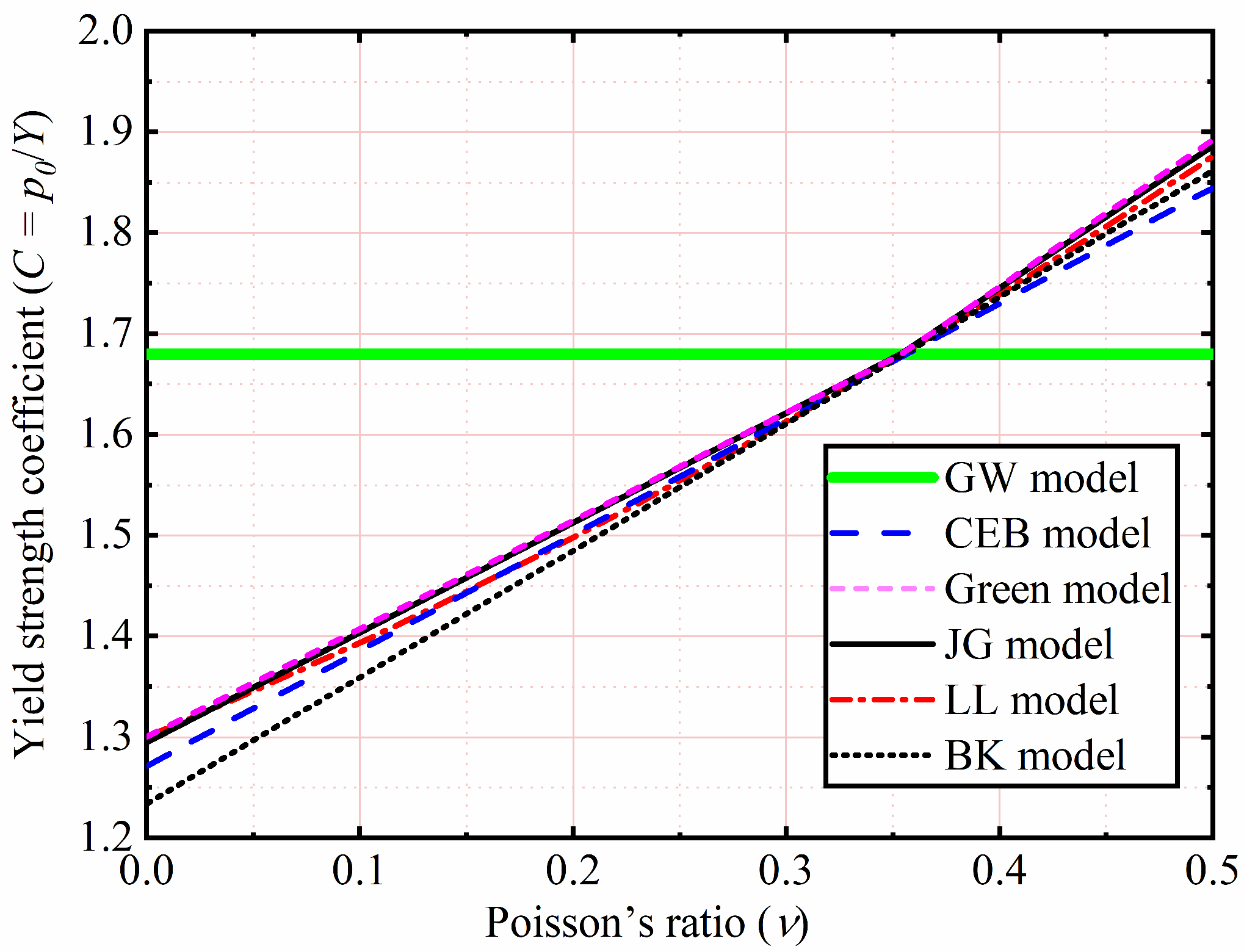




| Models | p0 | K or H | Yield Strength Coefficient (C) |
|---|---|---|---|
| GW model | p0 = 0.6H or p0 = CY | H = 2.8Y | 1.68 |
| CEB model | p0 = KH or p0 = CY | K = 0.41ν + 0.454 H = 2.8Y | 1.148ν + 1.2712 |
| Green model | p0 = CY | - | 0.54373ν2 + 0.8782ν + 1.30075 |
| JG model | p0 = CY | - | 1.295 exp(0.736ν) |
| LL model | p0 = KH or p0 = CY | K = 0.1943ν2 + 0.3141ν + 0.4645 H = 2.8Y | 0.54404ν2 + 0.87948ν + 1.3006 |
| BK model | p0 = CY | - | 1.256ν + 1.234 |
| No.1 | E (GPa) | Y (GPa) | E/Y | ω/ωc | R (mm) | ν |
|---|---|---|---|---|---|---|
| 1 | 45 | 0.25 | 180 | 500 | 1 | 0.2, 0.3, 0.4, 0.45 |
| 2 | 70 | 0.25 | 280 | |||
| 3 | 100 | 0.25 | 400 | |||
| 4 | 150 | 0.25 | 600 | |||
| 5 | 200 | 0.25 | 800 |
| No.2 | E (GPa) | Y (GPa) | E/Y | ω/ωc | R (mm) | ν |
|---|---|---|---|---|---|---|
| 1 | 200 | 2.52 | 79.4 | 500 | 1 | 0.2, 0.3, 0.4, 0.45 |
| 2 | 200 | 2 | 100 | |||
| 3 | 200 | 1.5 | 133.3 | |||
| 4 | 200 | 1.25 | 160 | |||
| 5 | 200 | 1 | 200 | |||
| 6 | 200 | 0.56 | 356.6 | |||
| 7 | 200 | 0.35 | 571.4 | |||
| 8 | 200 | 0.25 | 800 |
| E/Y | m | n | q |
|---|---|---|---|
| 133.3 < E/Y < 200 | 1.756 − (1.5 × 10−4) (E/Y) − 0.1ν | 5.51 | 0.982 − (1 × 10−4) (E/Y) − 0.1ν |
| 200 ≤ E/Y ≤ 800 | 1.610 + (2.0 × 10−5) (E/Y) − 0.1ν | 3.52 | 1.094 − (2 × 10−5) (E/Y) − 0.1ν |
| 1 < ω/ωc ≤ 5 | 5 < ω/ωc ≤ 90 | 90 < ω/ωc ≤ 5.4 (E/Y) | 5.4 (E/Y) < ω/ωc ≤ 500 | |
|---|---|---|---|---|
| m | 1.00 | 1.70 | 0.024 (E/Y) − 3ν + 7.3 | 0.65 (E/Y) − 30.5ν + 7.74 |
| n | 1.55 | 1.20 | 0.84 | 0.48 |
| 1 < ω/ωc ≤ 10 | 10 < ω/ωc ≤ 500 | |
|---|---|---|
| m | 1.02 | 1.3 |
| n | 1.171 − (2 × 10−5) (E/Y) | 1.096 − (2 × 10−5) (E/Y) |
| 1 < ω/ωc ≤ 5 | 5 < ω/ωc ≤ 90 | 90 < ω/ωc ≤ 5.4 (E/Y) | 5.4 (E/Y) < ω/ωc ≤ 500 | |
|---|---|---|---|---|
| m | 0.998 | 0.997 | 0.0073 (E/Y) + 2.13 | 0.0093 (E/Y) + 2.26 |
| n | 1.14 | 1.15 | 0.923 | 0.903 |
| m | n | q | t | |
|---|---|---|---|---|
| 1 < ω/ωc ≤ 5 | 1 | 1.55 | 0.997 | 1.14 |
| 1.89 | 1.206 − 0.1ν | 1.1 | 1.10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.; Chen, J.; Wang, C.; Liu, D.; Zhu, L. Research on Elastic–Plastic Contact Behavior of Hemisphere Flattened by a Rigid Flat. Materials 2022, 15, 4527. https://doi.org/10.3390/ma15134527
Zhang W, Chen J, Wang C, Liu D, Zhu L. Research on Elastic–Plastic Contact Behavior of Hemisphere Flattened by a Rigid Flat. Materials. 2022; 15(13):4527. https://doi.org/10.3390/ma15134527
Chicago/Turabian StyleZhang, Wangyang, Jian Chen, Chenglong Wang, Di Liu, and Linbo Zhu. 2022. "Research on Elastic–Plastic Contact Behavior of Hemisphere Flattened by a Rigid Flat" Materials 15, no. 13: 4527. https://doi.org/10.3390/ma15134527