The Effects of Imitation Dynamics on Vaccination Behaviours in SIR-Network Model
Abstract
1. Introduction
2. Materials and Methods
2.1. Technical Background
2.1.1. Basic Reproduction Number
2.1.2. Vaccination Model with Imitation Dynamics
2.1.3. Multi-City Epidemic Model
2.2. Methods
2.2.1. Integrated Model
- Individual’s responsiveness to changes in disease prevalence,
- Adjusted imitation rate,
- Vaccination failure rate,
2.2.2. Vaccination Available to Newborns Only
2.2.3. Vaccination Available to the Entire Susceptible Class
2.2.4. Vaccination Available to the Entire Susceptible Class with Committed Vaccine Recipients
2.2.5. Model Parameterisation
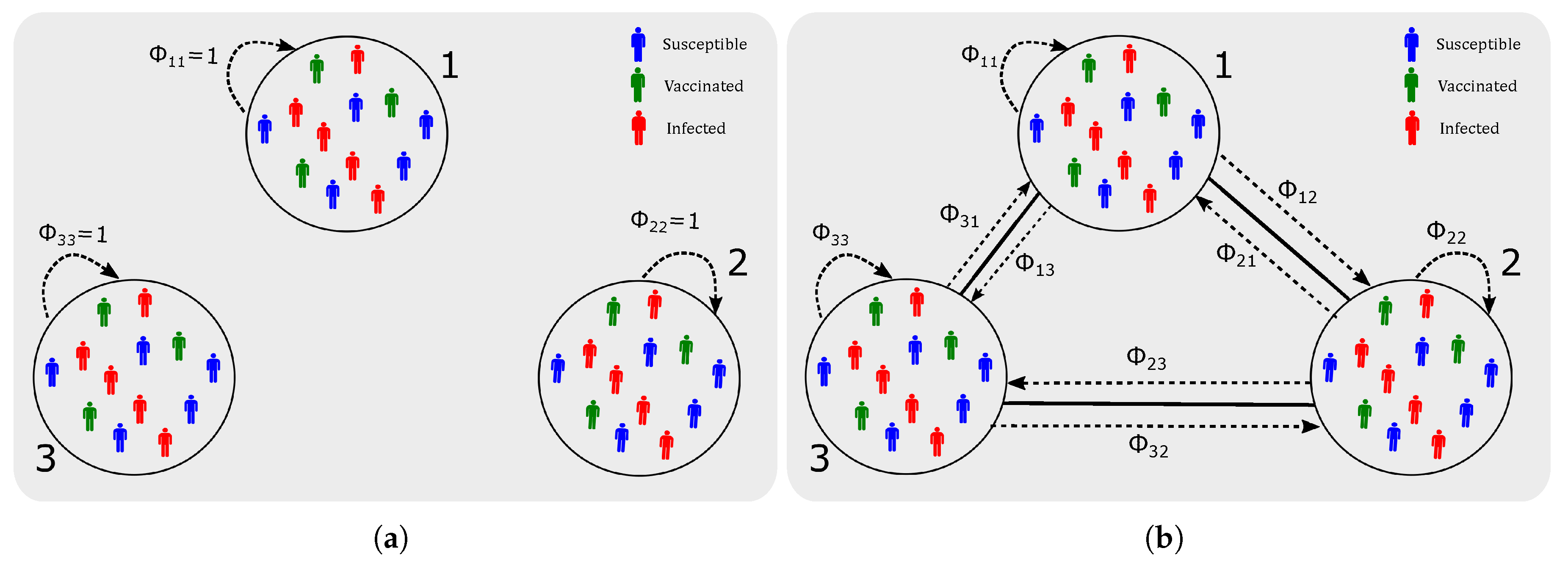
- a pilot case of a network with 3 nodes (suburbs),
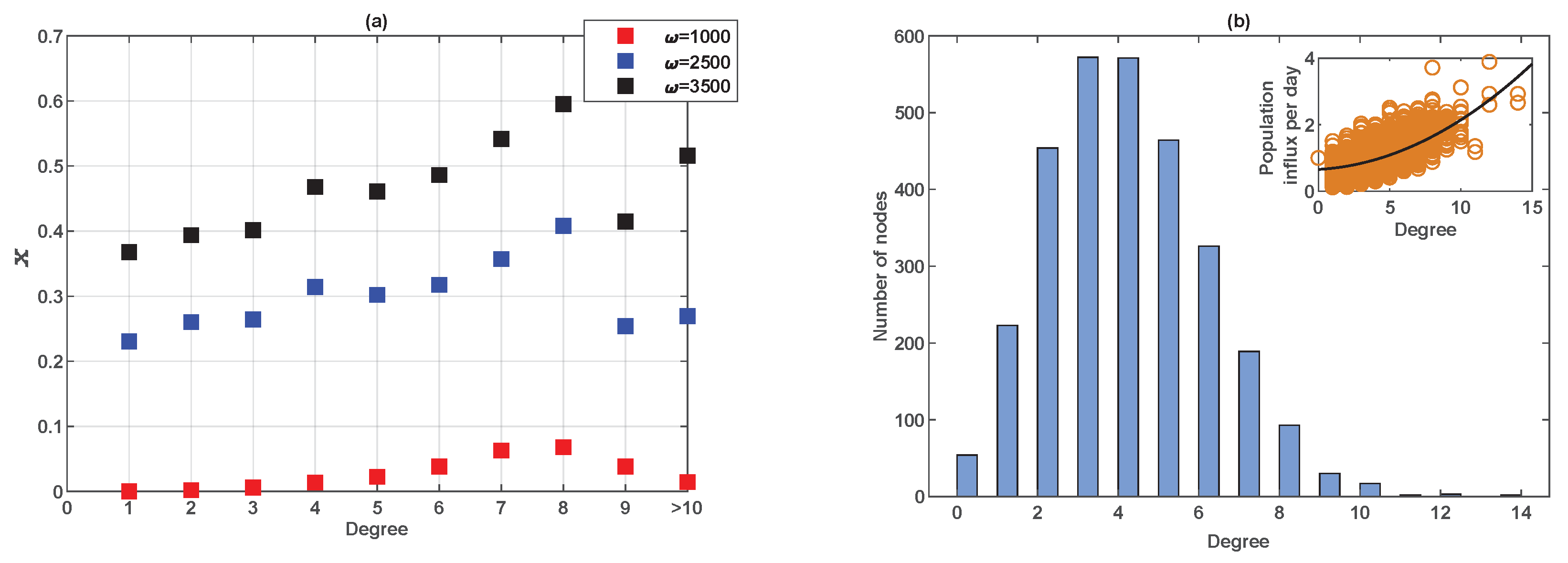
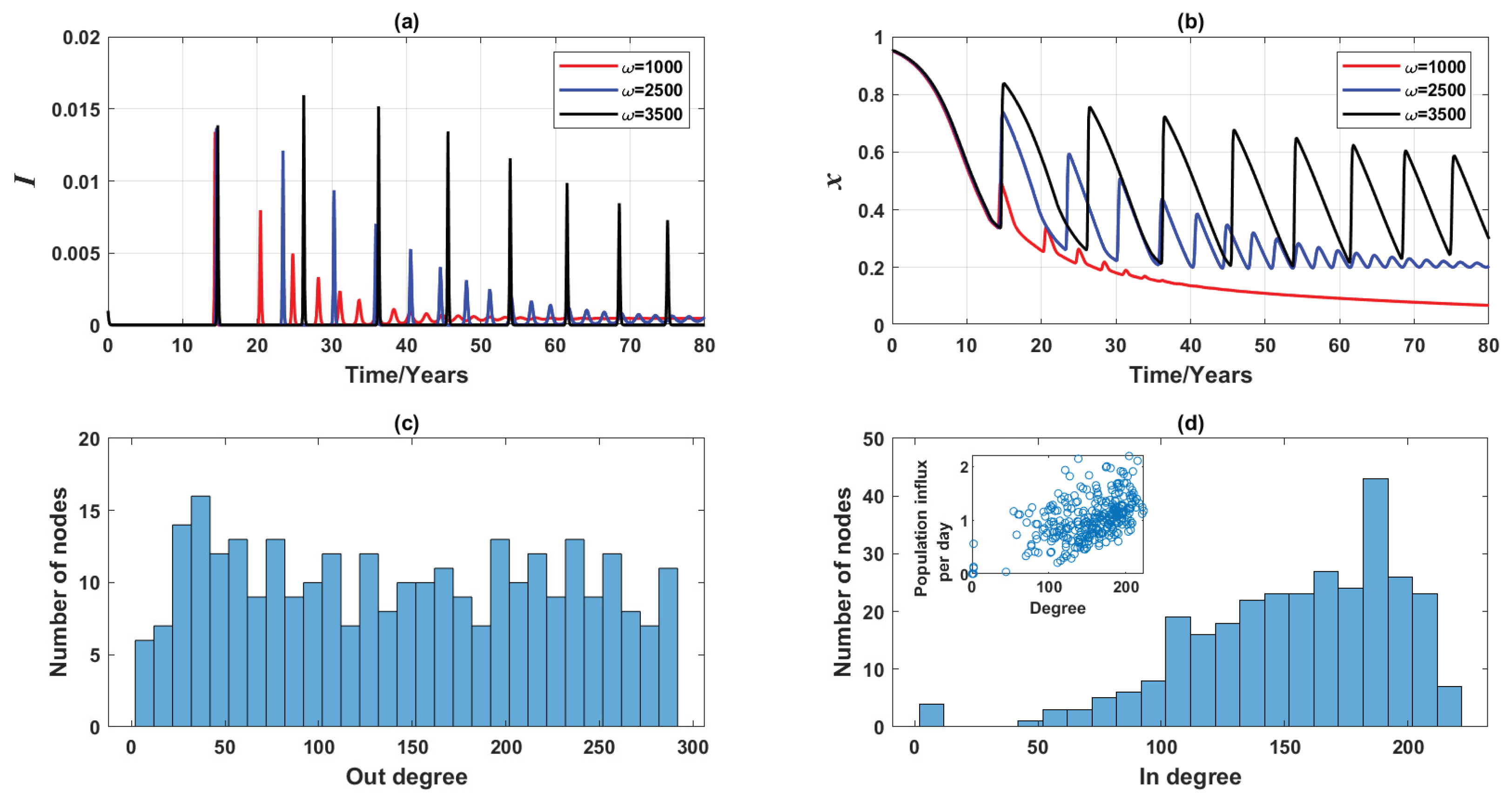
- an Erdös-Rényi random network [38] with 3000 nodes (suburbs), and
3. Results
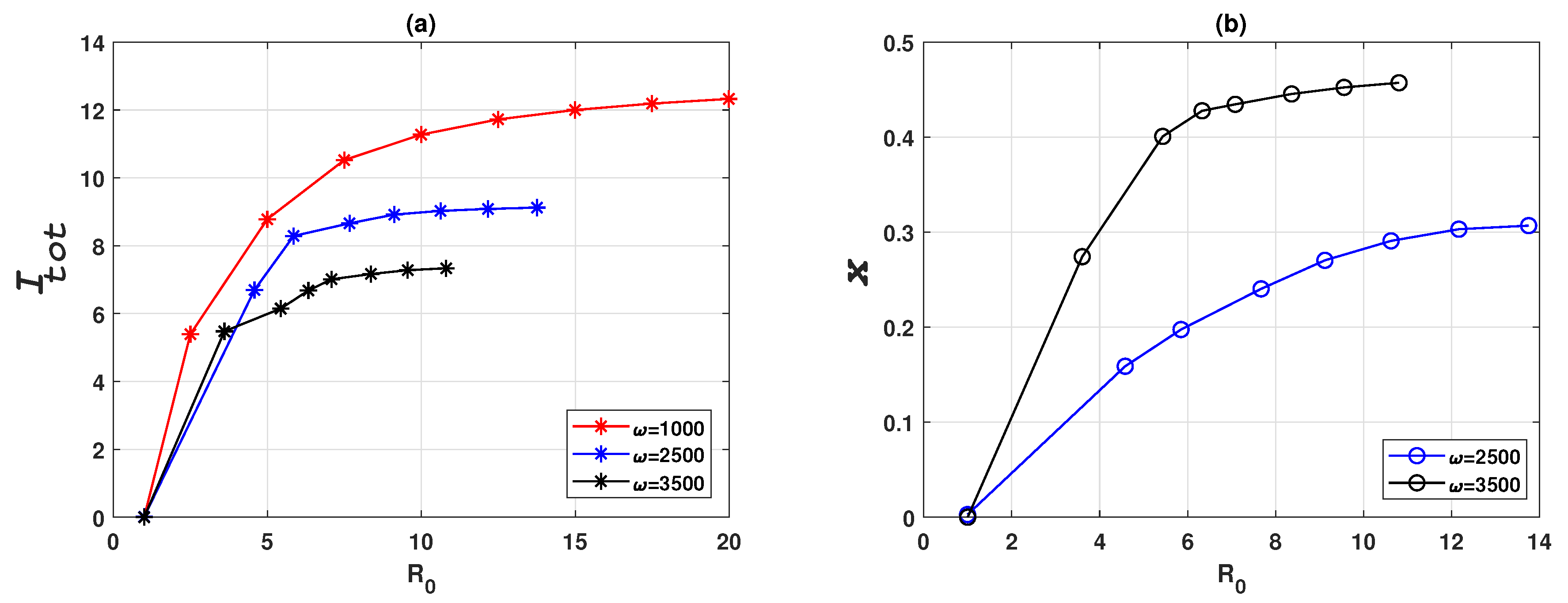
3.1. 3-Node Network
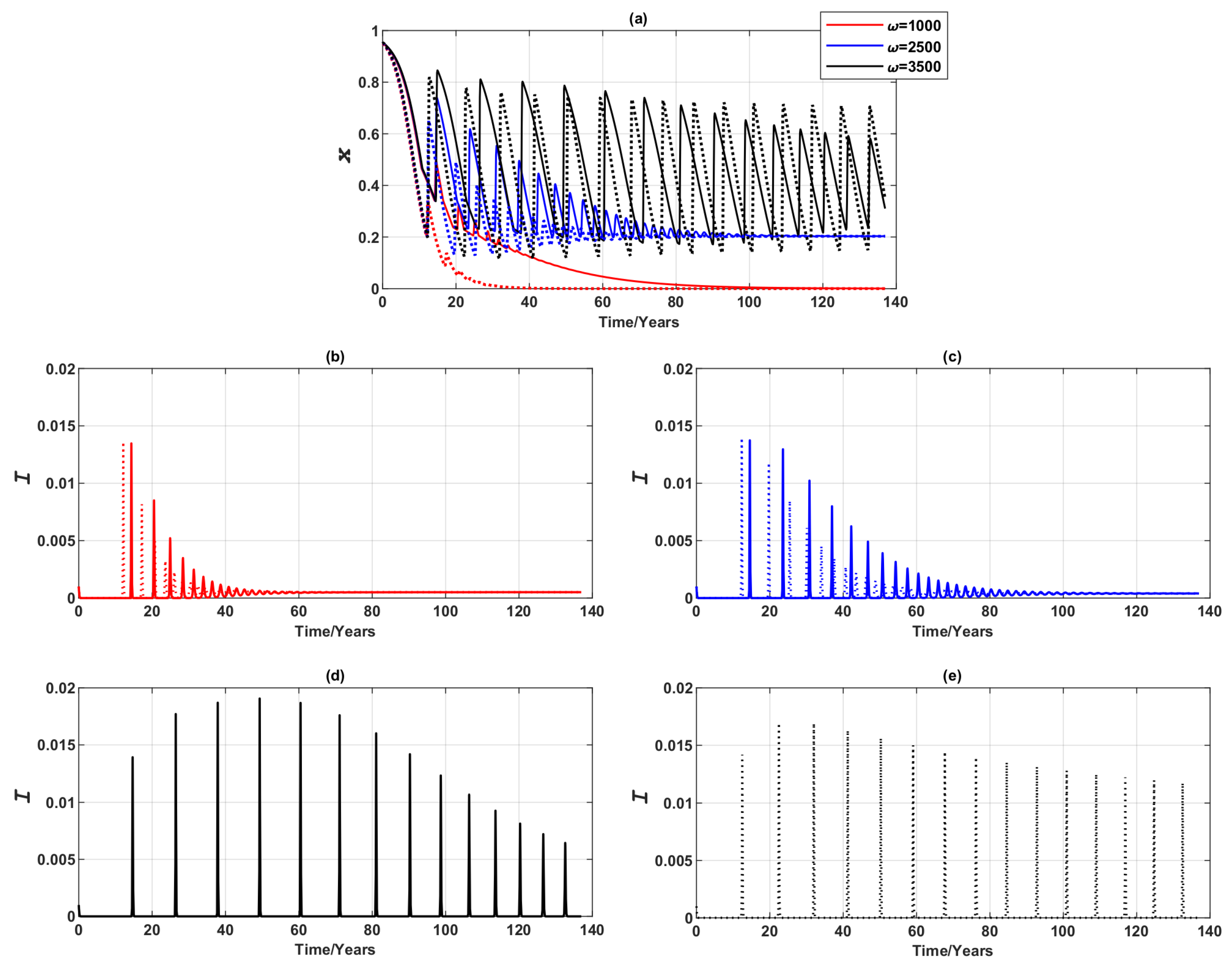
3.1.1. Vaccinating Newborns Only
3.1.2. Vaccinating the Entire Susceptible Class
3.2. Erdös-Rényi Random Network of 3000 Nodes
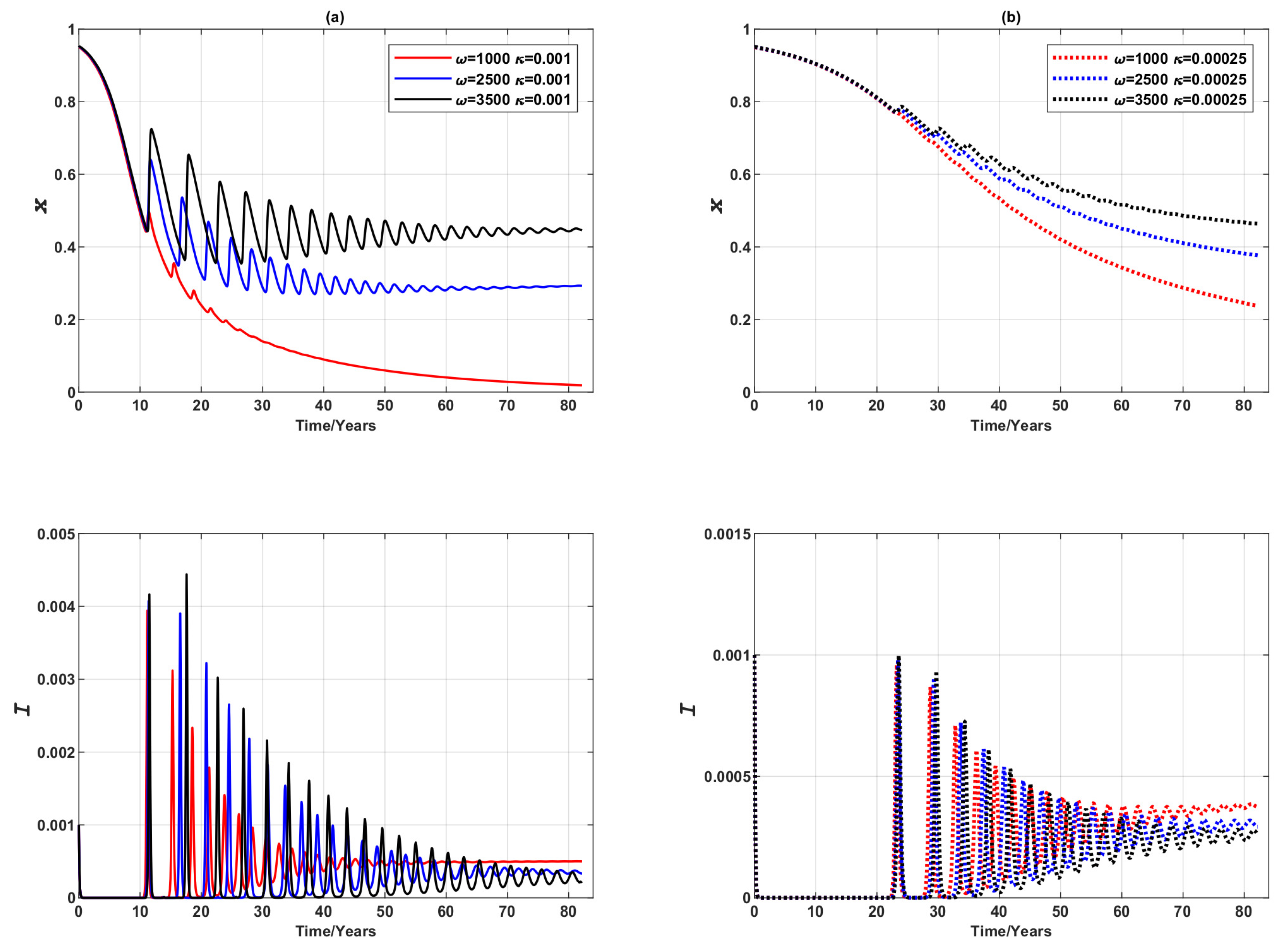
3.2.1. Vaccinating Newborns Only
3.2.2. Vaccinating Entire Susceptible Class
4. Discussion and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Next Generation Operator Approach
Appendix B. Propositions and Proofs
Appendix C. Preliminary Analysis of the Oscillatory Behaviour of Epidemic and Vaccination Dynamics When Vaccinating Newborns
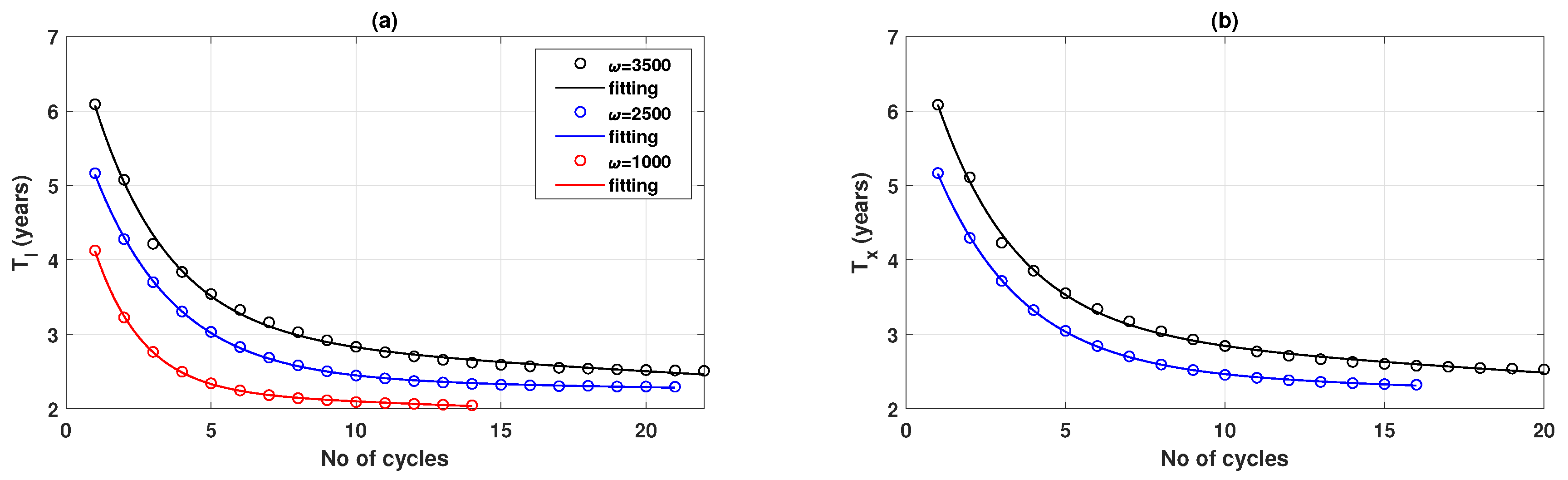
| SSE | R-Square | Adjusted R-Square | RMSE | |
| 0.03564 | 0.9980 | 0.9977 | 0.04450 | |
| 0.002712 | 0.9998 | 0.9997 | 0.01260 | |
| 0.0008870 | 0.9980 | 0.9997 | 0.009418 | |
| SSE | R-Square | Adjusted R-Square | RMSE | |
| 0.03076 | 0.9982 | 0.9979 | 0.04385 | |
| 0.001248 | 0.9999 | 0.9998 | 0.01020 |
| a | b | c | d | |
| 4.593 (4.406,4.78) | −0.3882 (−0.4247,−0.3516) | 2.983 (2.832,3.134) | −0.008774 (−0.01176,−0.005788) | |
| 3.951 (3.9,4.003) | −0.3616 (−0.3732,−0.35) | 2.399 (2.353,2.446) | −0.00239 (−0.003547,−0.001234) | |
| 3.468 (3.398,3.538) | −0.6031 (−0.628,−0.5782) | 2.237 (2.195,2.28) | −0.006735 (−0.008434,−0.005035) | |
| a | b | c | d | |
| 4.535 (4.341,4.73) | −0.3971 (−0.4385,−0.3557) | 3.066 (2.889,3.244) | −0.01052 (−0.0142,−0.006853) | |
| 3.882 (3.827,3.937) | −0.3717 (−0.3857,−0.3577) | 2.492 (2.422,2.561) | −0.004952 (−0.006928,−0.002976) |

References
- World Heath Organization. Basic Epidemiology; World Heath Organization: Geneva, Switzerland, 2006; pp. 125–127. [Google Scholar]
- Jansen, V.A.A.; Stollenwerk, N.; Jensen, H.; Ramsay, M.; Edmunds, W.; Rhodes, C. Measles outbreaks in a population with declining vaccine uptake. Science 2003, 301, 804. [Google Scholar] [CrossRef]
- Bauch, C.T. Imitation dynamics predict vaccinating behaviour. Proc. R. Soc. B 2005, 272, 1669–1675. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Bauch, C.; Bhattacharyya, S.; d’Onofrio, A.; Manfredi, P.; Perc, M.P.N.; Salathè, M.; Zhao, D. Statistical physics of vaccination. Phys. Rep. 2016, 664, 1–113. [Google Scholar] [CrossRef]
- Bauch, C.T.; Bhattacharyya, S. Evolutionary game theory and social learning can determine how vaccine scares unfold. PLoS Comput. Biol. 2012, 8, e1002452. [Google Scholar] [CrossRef] [PubMed]
- Fu, F.; Rosenbloom, D.; Wang, L.; Nowak, M. Imitation dynamics of vaccination behaviour on social networks. Proc. R. Soc. B 2010, 278, 42–49. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Wu, Z.; Zhang, L. Impact of committed individuals on vaccination behaviour. Phys. Rev. E 2012, 86, 051132. [Google Scholar] [CrossRef]
- Zhang, H.; Fu, F.; Zhang, W.; Wang, B. Rational behaviour is a ‘double-edged sword’ when considering voluntary vaccination. Physica A 2012, 391, 4807–4815. [Google Scholar] [CrossRef]
- Zhang, Y. The impact of other-regarding tendencies on the spatial vaccination network. Chaos Solitons Fractals 2013, 2013, 209–215. [Google Scholar] [CrossRef]
- Li, Q.; Li, M.; Lv, L.; Guo, C.; Lu, K. A new prediction model of infectious diseases with vaccination strategies based on evolutionary game theory. Chaos Solitons Fractals 2017, 104, 51–60. [Google Scholar] [CrossRef]
- Feng, X.; Bin, W.; Long, W. Voluntary vaccination dilemma with evolving psychological perceptions. J. Theor. Biol. 2018, 439, 65–75. [Google Scholar] [CrossRef]
- Bauch, C.; Earn, D.J.D. Vaccination and the theory of games. Proc. Natl. Acad. Sci. USA 2004, 101, 13391–13394. [Google Scholar] [CrossRef] [PubMed]
- Bauch, C.; Earn, D.J.D. Transients and attractors in epidemics. Proc. R. Soc. B 2003, 270, 1573–1578. [Google Scholar] [CrossRef] [PubMed]
- Bauch, C.; Galvani, A.P.; Earn, D. Group interest versus self-interest in smallpox vaccination policy. Proc. Natl. Acad. Sci. USA 2003, 100, 10564–10567. [Google Scholar] [CrossRef] [PubMed]
- Perisic, A.; Bauch, C. A simulation analysis to characterize the dynamics of vaccinating behaviour on contact networks. BioMed Cent. 2009, 9, 77. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Andrews, M.; Wu, Z.; Wang, L.; Bauch, C. Coupled disease–behavior dynamics on complex networks: A review. Phys. Life Rev. 2015, 15, 1–29. [Google Scholar] [CrossRef] [PubMed]
- Arino, J.; van den Driessche, P. The Basic Reproduction Number in a Multi-city Compartmental Epidemic Model; Benvenuti, L., de Santis, A., Farina, L., Eds.; Springer: Berlin/Heidelberg, Germany, 2003; Volume 294, pp. 135–142. [Google Scholar]
- Arino, J.; van den Driessche, P. A multi-city epidemic model. Math. Popul. Stud. 2003, 10, 175–193. [Google Scholar] [CrossRef]
- Stolerman, L.; Coombs, D.; Boatto, S. SIR-network model and its application to dengue fever. J. Appl. Math. 2015, 75, 2581–2609. [Google Scholar] [CrossRef]
- Pei, S.; Kandula, S.; Yang, W.; Shaman, J. Forecasting the spatial transmission of influenza in the United States. Proc. Natl. Acad. Sci. USA 2018, 115, 2752–2757. [Google Scholar] [CrossRef]
- Belik, V.; Geisel, T.; Brockmann, D. Natural Human Mobility Patterns and Spatial Spread of Infectious Diseases. Phys. Rev. X 2011, 111, 011001. [Google Scholar] [CrossRef]
- Diekmann, O.; Heesterbeek, J.A.P.; Metz, J.A.J. On the definition and the computation of the basic reproduction ratio R0 in models for infectious diseases in heterogeneous populations. J. Math. Biol. 1990, 28, 365–382. [Google Scholar] [CrossRef]
- Anderson, R.M.; May, R.M. Infectious Diseases of Humans; Oxford University Press: Oxford, UK, 1991. [Google Scholar]
- Harding, N.; Nigmatullin, R.; Prokopenko, M. Thermodynamic efficiency of contagions: A statistical mechanical analysis of the SIS epidemic model. Interface Focus 2018, 8, 20180036. [Google Scholar] [CrossRef] [PubMed]
- Hartfield, M.; Alizon, S. Introducing the Outbreak Threshold in Epidemiology. PLoS Pathog. 2013, 96, e1003277. [Google Scholar] [CrossRef] [PubMed]
- Pastor-Satorras, R.; Castellano, C.; Mieghem, P.; Vespignani, A. Epidemic processes in complex networks. Rev. Mod. Phys. 2015, 87, 925. [Google Scholar] [CrossRef]
- Van den Driessche, P.; Watmough, J. Further notes on the basic reproduction number. In Mathematical Epidemiology; Wu, J., Ed.; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Erten, E.; Lizier, J.; Piraveenan, M.; Prokopenko, M. Criticality and information dynamics in epidemiological models. Entropy 2017, 19, 194. [Google Scholar] [CrossRef]
- Brauer, F. Compartmental Models in Epidemiology. In Mathematical Epidemiology; Wu, J., Ed.; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Australian Government, Department of Health. National Immunisation Program Schedule. 2018. Available online: https://beta.health.gov.au/health-topics/immunisation/immunisation-throughout-life/national-immunisation-program-schedule (accessed on 9 July 2019).
- Van den Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef]
- Diekmann, O.; Heesterbeek, J.; Roberts, M.G. The construction of next-generation matrices for compartmental epidemic models. J. R. Soc. Interface 2010, 7, 873–885. [Google Scholar] [CrossRef] [PubMed]
- Seneta, E. Non-Negative Matrices and Markov Chains; Springer: New York, NY, USA, 1981; pp. 61–67. [Google Scholar]
- Bai, Z. Global dynamics of a SEIR model with information dependent vaccination and periodically varying transmission rate. Math. Methods Appl. Sci. 2015, 38, 2403–2410. [Google Scholar] [CrossRef]
- Buonomo, B.; d’Onofrio, A.; Lacitignola, D. Modelling of pseudo-rational exemption to vaccination for SEIR diseases. J. Math. Anal. Appl. 2013, 404, 385–398. [Google Scholar] [CrossRef]
- Sheppeard, V.; Forssman, B.; Ferson, M.; Moreira, C.; Campbell-Lloyd, S.; Dwyer, D.; McAnulty, J. Vaccine failures and vaccine effectiveness in children during measles outbreaks in New South Wales, March–May 2006. Commun. Dis. Intell. (CDI) 2009, 33, 21. [Google Scholar]
- Guerra, F.; Bolotin, S.; Lim, G.; Heffernan, J.; Deeks, S.; Li, Y.; Crowcroft, N. The basic reproduction number (R0) of measles: A systematic review. Lancet Infect. Dis. 2017, 17, e420–e428. [Google Scholar] [CrossRef]
- Erdös, P.; Rényi, A. On random graphs. Publ. Math. 1959, 6, 290–297. [Google Scholar]
- Australian Bureau of Statistics. Census of Population and Housing: Understanding the Census and Census Data, Australia, 2016; Australian Bureau of Statistics: Canberra, Australia, 2017. Available online: https://www.abs.gov.au/ausstats/abs@.nsf/Lookup/2900.0main+features100722016 (accessed on 11 July 2019).
- Fair, K.; Zachreson, C.; Prokopenko, M. Creating a surrogate commuter network from Australian Bureau of Statistics census data. Sci. Data 2019. accepted. [Google Scholar]
- Cardillo, A.; Reyes-Suárez, C.; Naranjo, F.; Gómez-Gardeñes, J. Evolutionary vaccination dilemma in complex networks. Phys. Rev. E 2013, 88, 032803. [Google Scholar] [CrossRef] [PubMed]
- Barabási, A. Scale-free Properties. In Network Science; Cambridge University Press: Cambridge, UK, 2016; Chapter 4; pp. 3–9. [Google Scholar]
- Brockmann, D.; Helbing, D. The Hidden Geometry of Complex, Network-Driven Contagion Phenomena. Science 2013, 342, 1337–1342. [Google Scholar] [CrossRef] [PubMed]
- Steinegger, B.; Cardillo, A.; Rios, P.; Gómez-Gardeñes, J.; Arenas, A. Interplay between cost and benefits triggers nontrivial vaccination uptake. Phys. Rev. E 2018, 97, 032308. [Google Scholar] [CrossRef] [PubMed]
- Pastor-Satorras, R.; Vespignani, A. Immunization of complex networks. Phys. Rev. E 2002, 65, 036104. [Google Scholar] [CrossRef] [PubMed]
- Cliff, O.M.; Harding, N.; Piraveenan, M.; Erten, Y.; Gambhir, M.; Prokopenko, M. Investigating spatiotemporal dynamics and synchrony of influenza epidemics in Australia: An agent-based modelling approach. Simul. Model. Pract. Theory 2018, 87, 412–431. [Google Scholar] [CrossRef]
- Zachreson, C.; Fair, K.M.; Cliff, O.M.; Harding, N.; Piraveenan, M.; Prokopenko, M. Urbanization affects peak timing, prevalence, and bimodality of influenza pandemics in Australia: Results of a census-calibrated model. Sci. Adv. 2018, 4, eaau5294. [Google Scholar] [CrossRef]
- Kasthurirathna, D.; Piraveenan, M. Emergence of scale-free characteristics in socio-ecological systems with bounded rationality. Sci. Rep. 2015, 5, 10448. [Google Scholar] [CrossRef]
- Kasthurirathna, D.; Piraveenan, M.; Uddin, S. Modeling networked systems using the topologically distributed bounded rationality framework. Complexity 2016, 21, 123–137. [Google Scholar] [CrossRef]
- Balcan, D.; Vespignani, D. Phase transitions in contagion processes mediated by recurrent mobility patterns. Nat. Phys. 2011, 7, 581–586. [Google Scholar] [CrossRef] [PubMed]
- Piraveenan, M.; Prokopenko, M.; Hossain, L. Percolation Centrality: Quantifying Graph-Theoretic Impact of Nodes during Percolation in Networks. PLoS ONE 2013, 8, e53095. [Google Scholar] [CrossRef] [PubMed]
- Thedchanamoorthy, G.; Piraveenan, M.; Uddin, S.; Senanayake, U. Influence of vaccination strategies and topology on the herd immunity of complex networks. Soc. Netw. Anal. Min. 2014, 4, 213. [Google Scholar] [CrossRef]
- Piraveenan, M.; Prokopenko, M.; Zomaya, A. Assortativeness and information in scale-free networks. Eur. Phys. J. B 2009, 67, 291–300. [Google Scholar] [CrossRef]
- Badham, J.; Stocker, R. The impact of network clustering and assortativity on epidemic behaviour. Theor. Popul. Biol. 2010, 77, 71–75. [Google Scholar] [CrossRef]
- Granell, C.; Gómez, S.; Arenas, A. Dynamical Interplay between Awareness and Epidemic Spreading in Multiplex Networks. Phys. Rev. Lett. 2013, 111, 128701. [Google Scholar] [CrossRef]
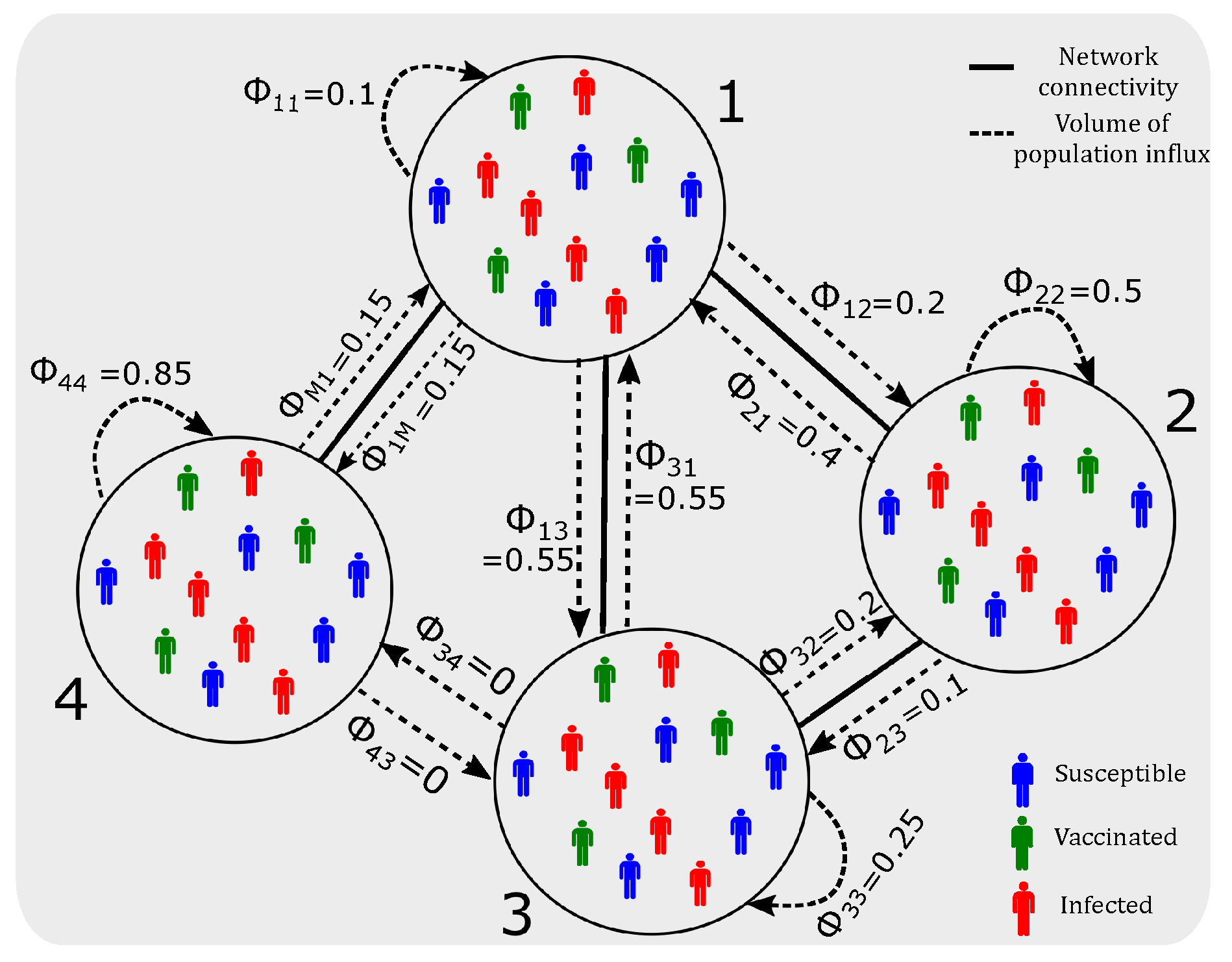
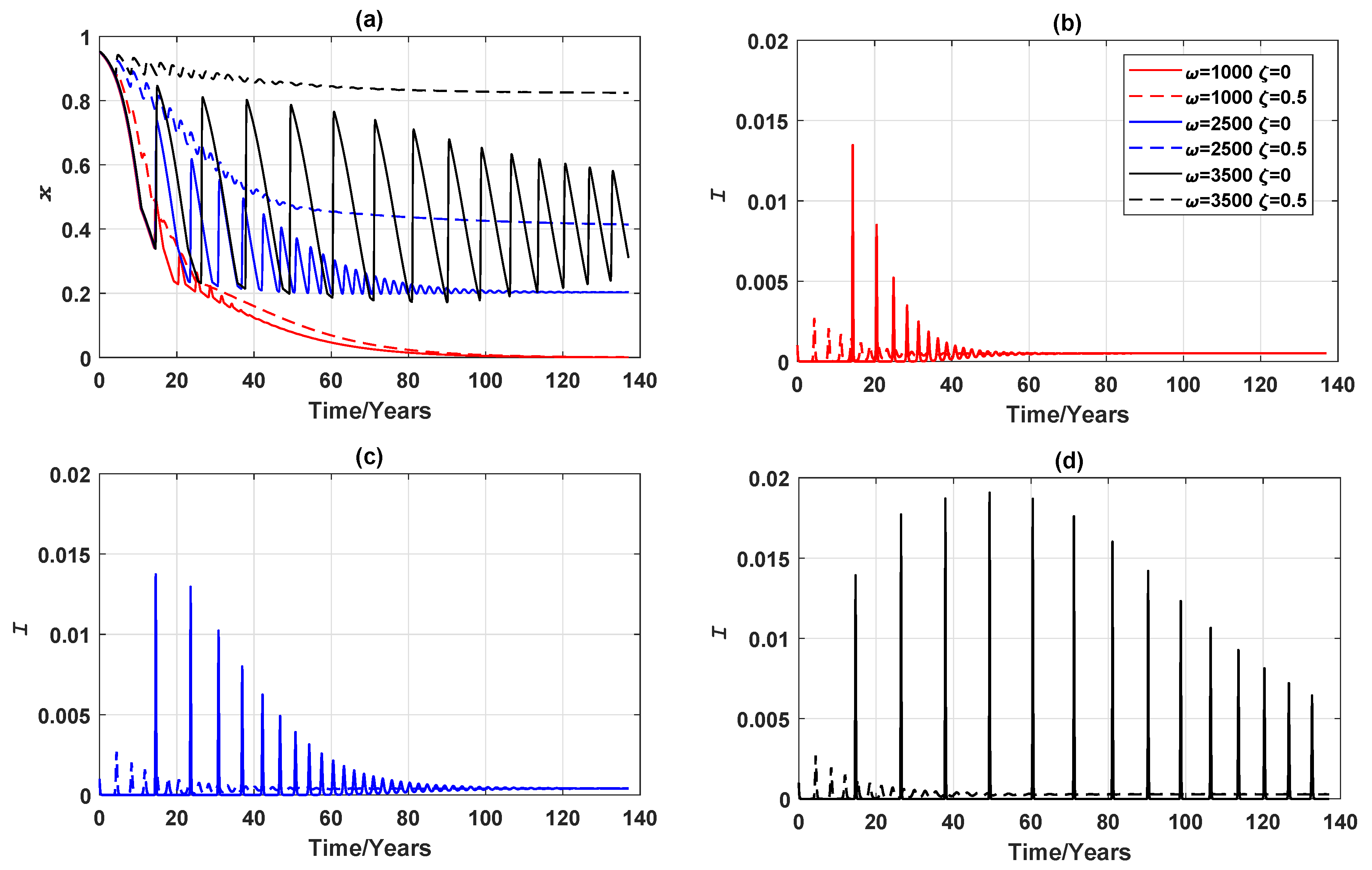
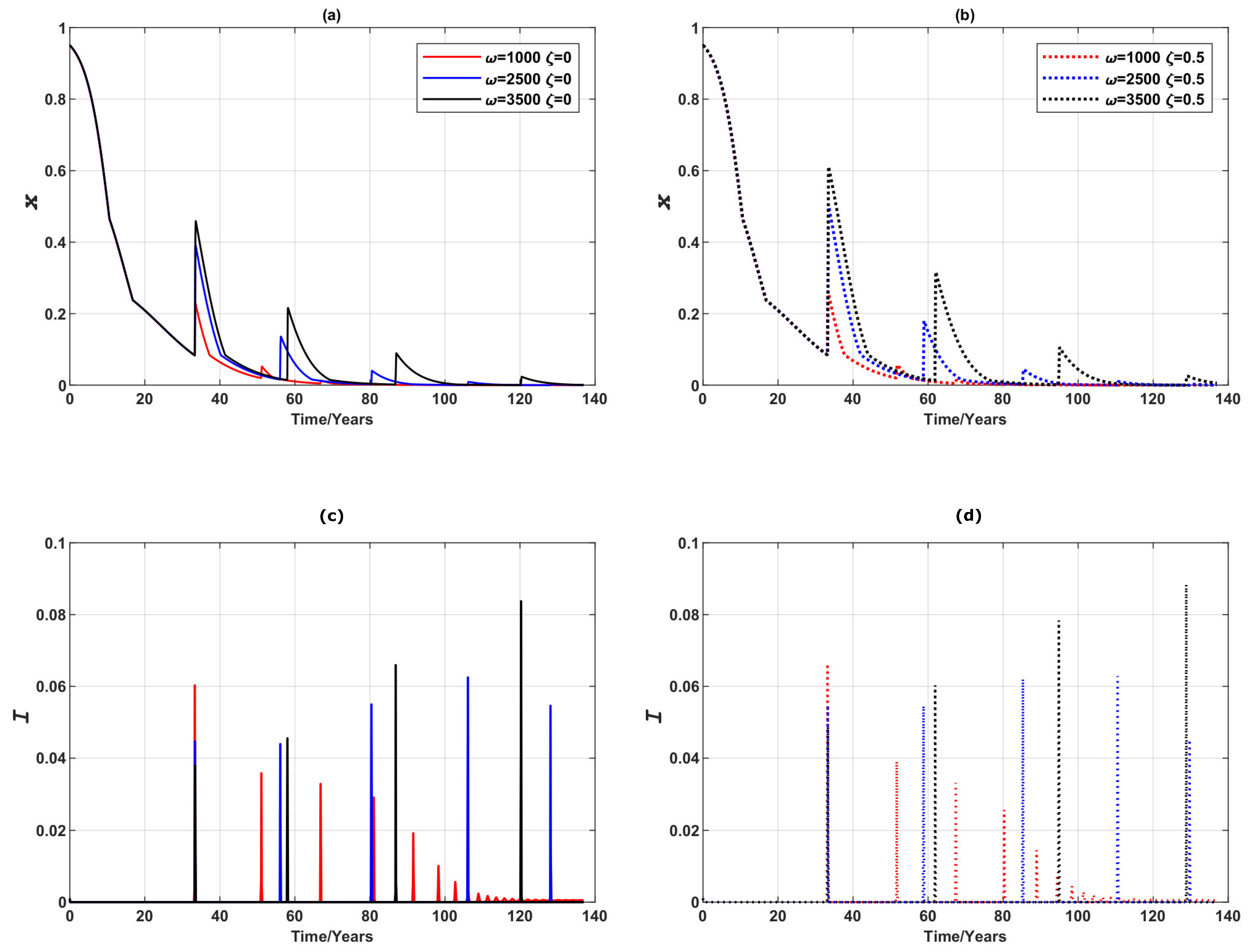
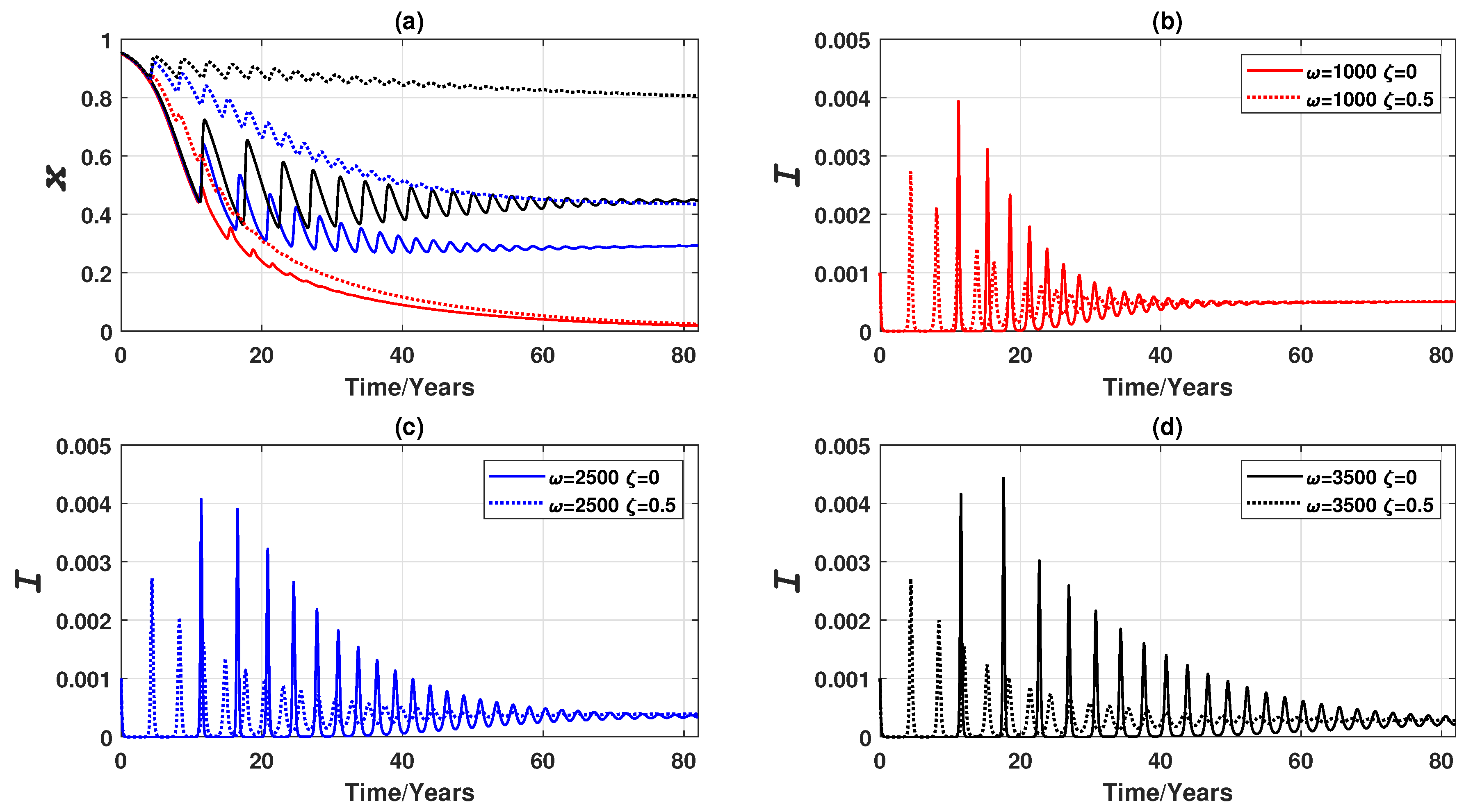
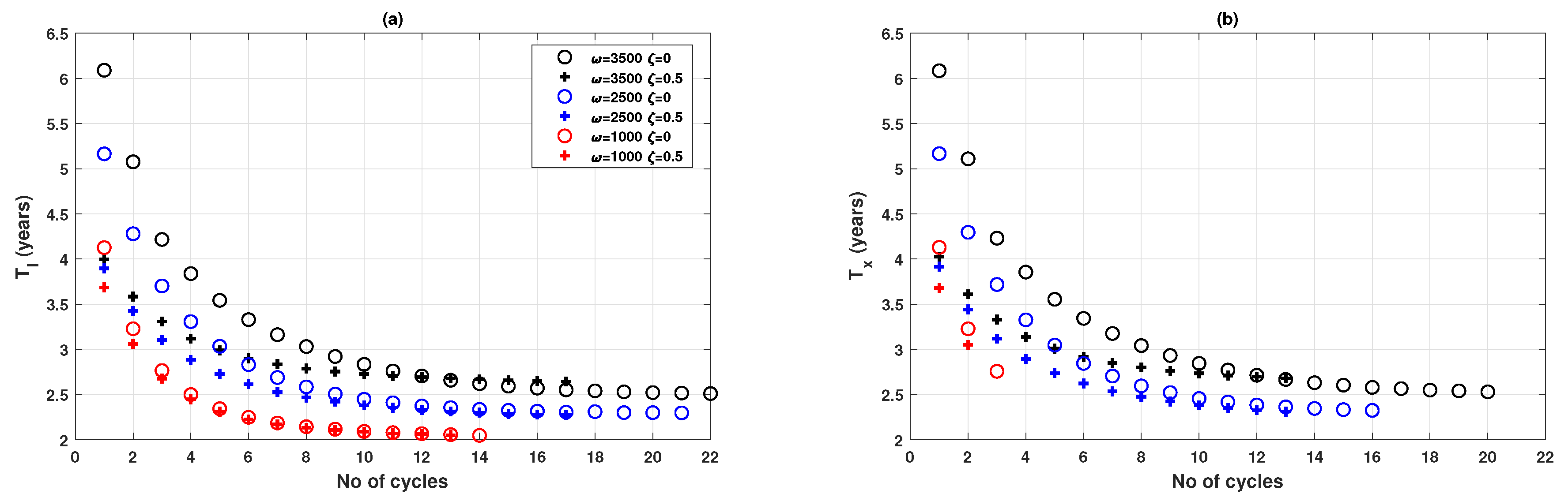
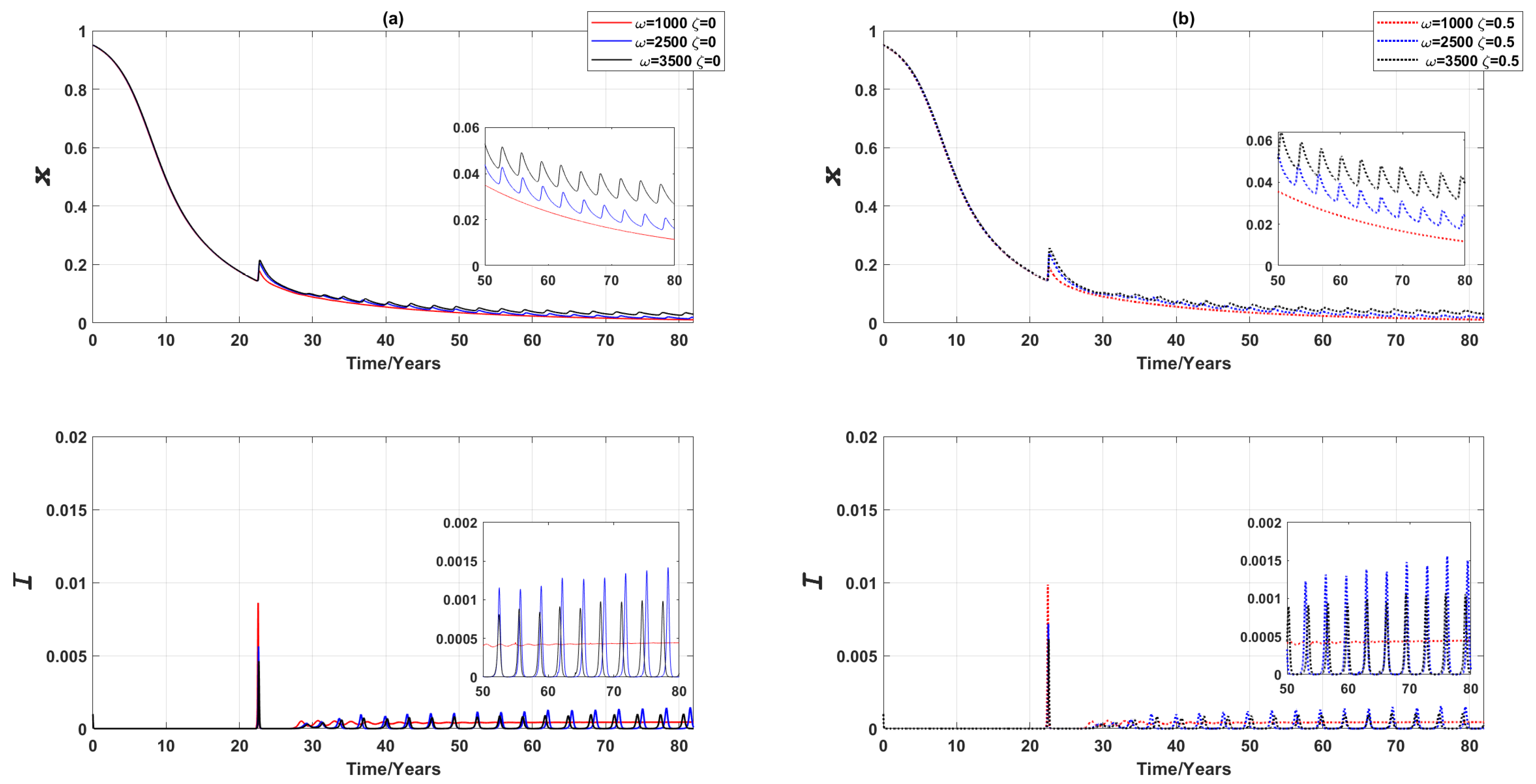
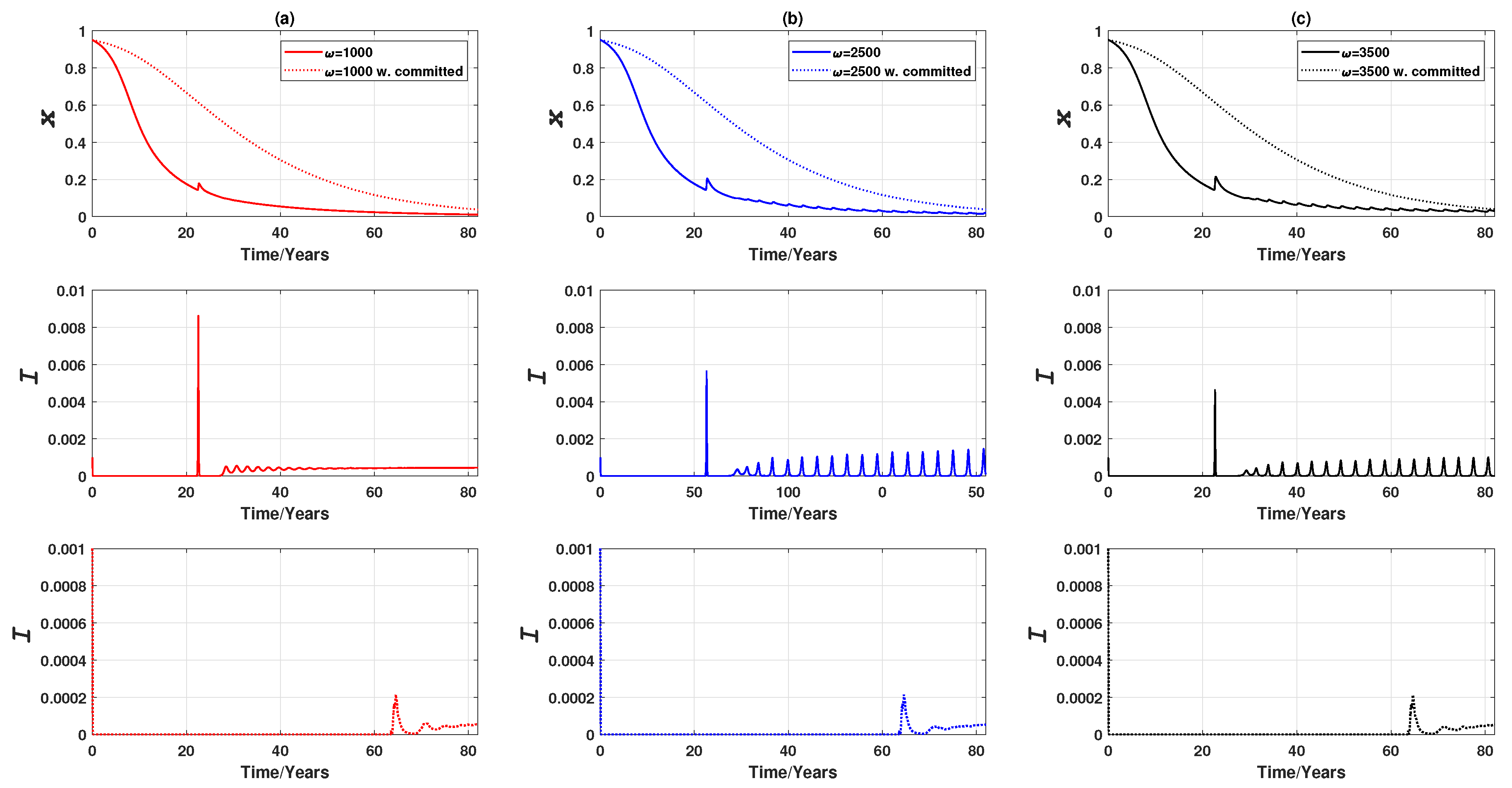
| Parameter | Interpretation | Baseline Value | References |
|---|---|---|---|
| Average length of recovery period (days) | 10 | [37] | |
| Basic reproduction number | 15 | [37] | |
| Mean birth and death rate (days) | 0.000055 | [3] | |
| Vaccination failure rate | [0,1] | Assumed | |
| Imitation rate | 0.001 | [3] | |
| Responsiveness to changes in disease prevalence | [1000,3500] | [3] | |
| Fraction of residents from node i travelling to j | [0,1] | Network connectivity | |
| (See Figures 10b and 11d | |||
| for degree distribution.) | |||
| I | Initial condition | 0.001 | [3] |
| S | Initial condition | 0.05 | [3] |
| x | Initial condition | 0.95 | [3] |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, S.L.; Piraveenan, M.; Prokopenko, M. The Effects of Imitation Dynamics on Vaccination Behaviours in SIR-Network Model. Int. J. Environ. Res. Public Health 2019, 16, 2477. https://doi.org/10.3390/ijerph16142477
Chang SL, Piraveenan M, Prokopenko M. The Effects of Imitation Dynamics on Vaccination Behaviours in SIR-Network Model. International Journal of Environmental Research and Public Health. 2019; 16(14):2477. https://doi.org/10.3390/ijerph16142477
Chicago/Turabian StyleChang, Sheryl Le, Mahendra Piraveenan, and Mikhail Prokopenko. 2019. "The Effects of Imitation Dynamics on Vaccination Behaviours in SIR-Network Model" International Journal of Environmental Research and Public Health 16, no. 14: 2477. https://doi.org/10.3390/ijerph16142477
APA StyleChang, S. L., Piraveenan, M., & Prokopenko, M. (2019). The Effects of Imitation Dynamics on Vaccination Behaviours in SIR-Network Model. International Journal of Environmental Research and Public Health, 16(14), 2477. https://doi.org/10.3390/ijerph16142477





