Distributed Non-Fragile State Estimation for Uncertain Nonlinear Systems of Sensor Networks Subject to Sensor Nonlinearities
Abstract
1. Introduction
- (1)
- Firstly, the three essential issues, i.e., the target plant model uncertainties, the state estimator gain variation, and the sensor nonlinearities, are all considered in a unified framework, which approximates the sensor network implementation much more practically. Especially, our work makes one of the first attempts to deal with sensor nonlinearities in the scope of distributed sensor networks.
- (2)
- Secondly, in order to capture the distributed sensor network information exchanges, the model transformation for distributed state estimation errors is performed and new sufficient conditions are established, which leads to the resulting error system being able to achieve a desired passivity performance index from the energy point of view.
- (3)
- Finally, the theoretical derivations and findings are presented in the form of linear matrix inequalities, which can be conveniently calculated with feasible solutions, and the corresponding simulation example is given to verify the effectiveness of our proposed methods.
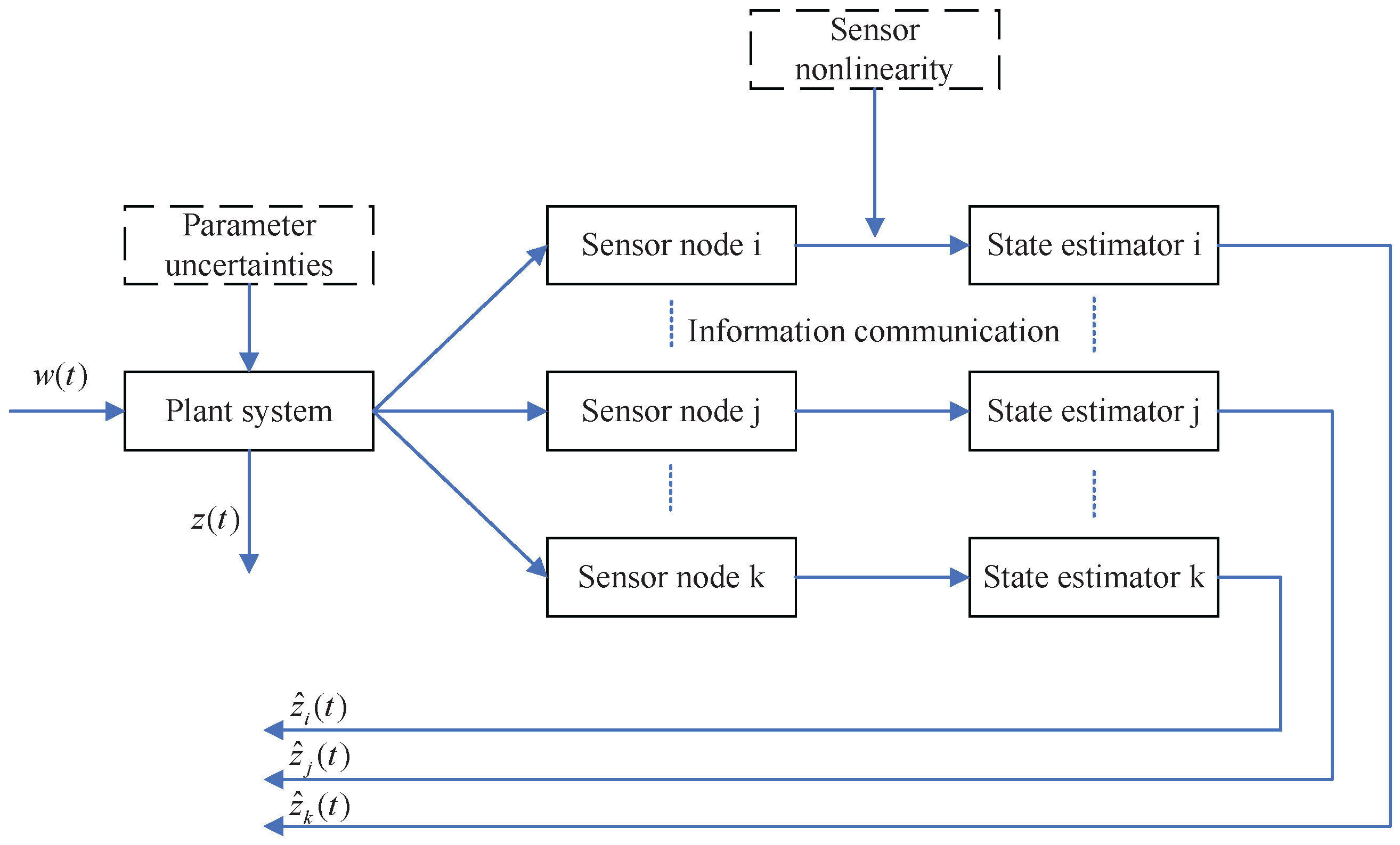
2. Problem Formulation
2.1. Nonlinear Target Plant Model
2.2. Distributed Sensor Network
3. Main Results
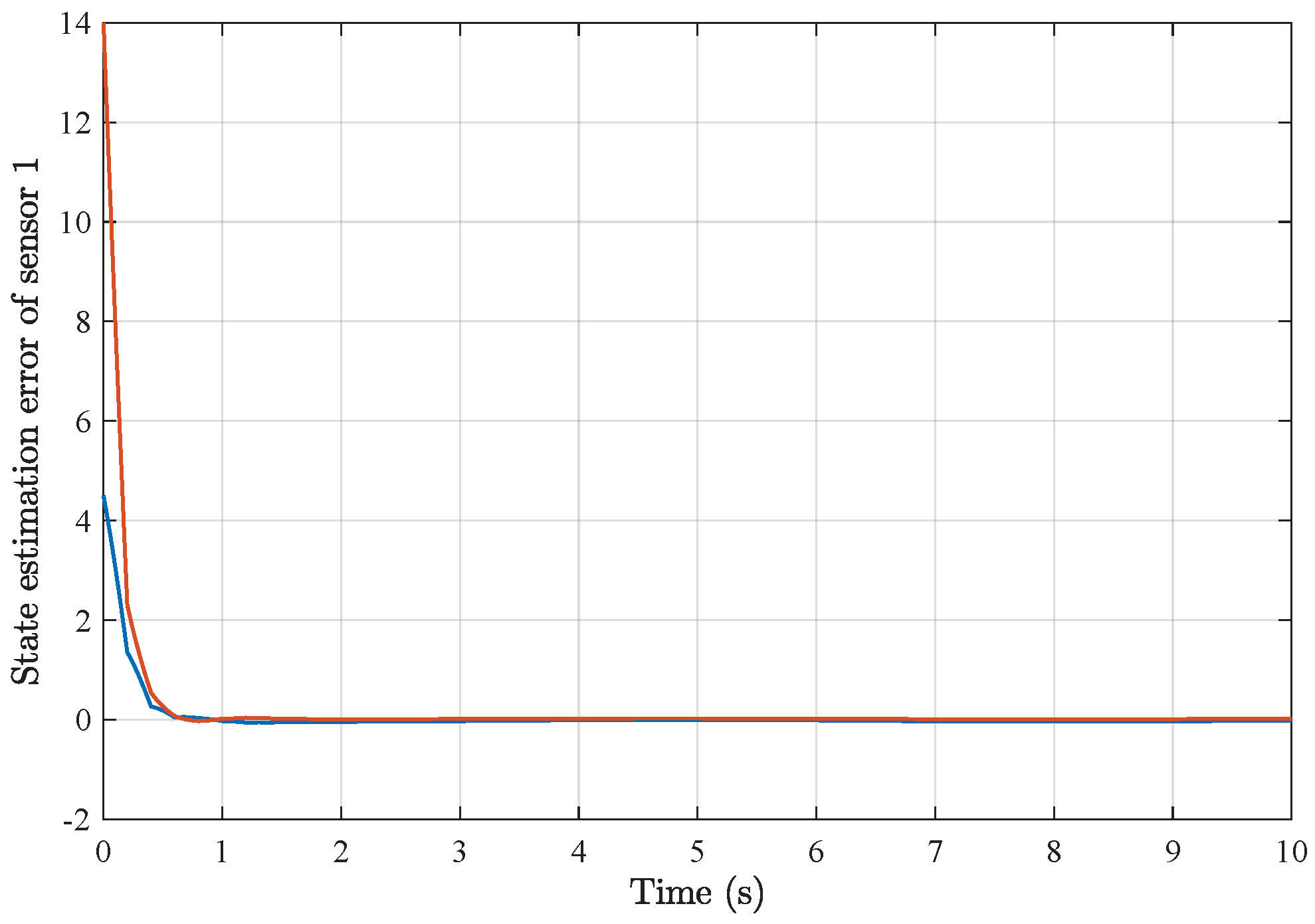
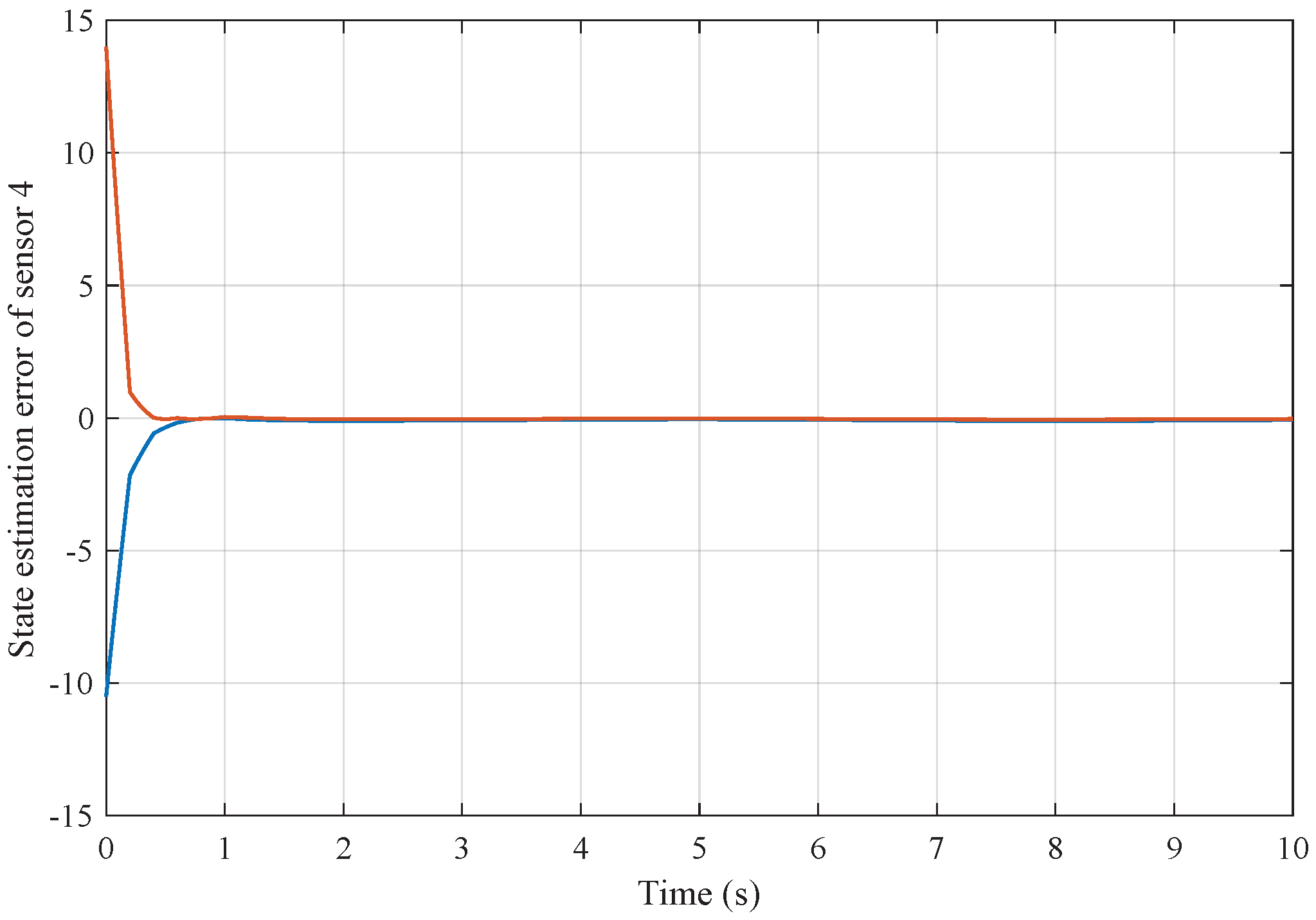
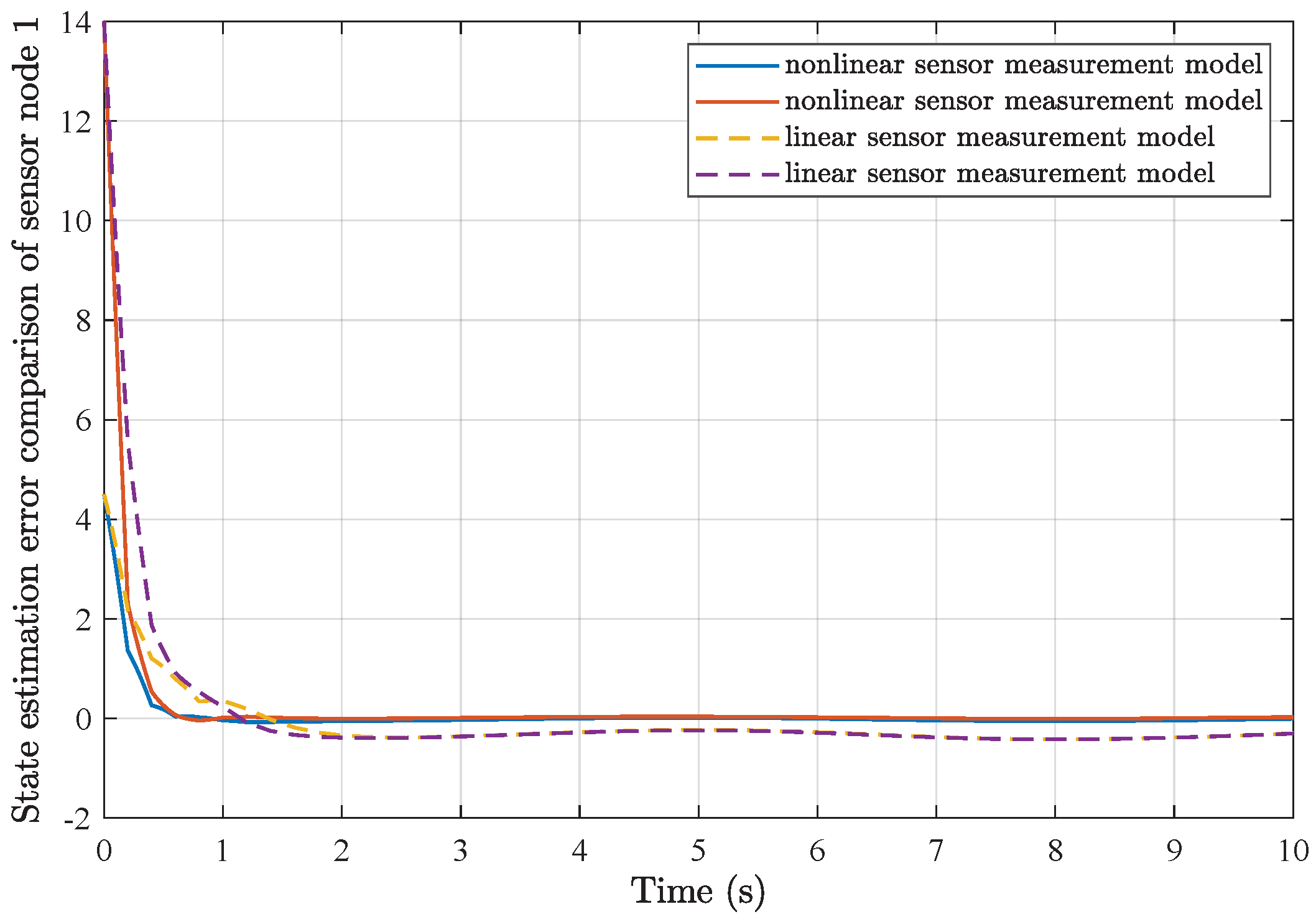
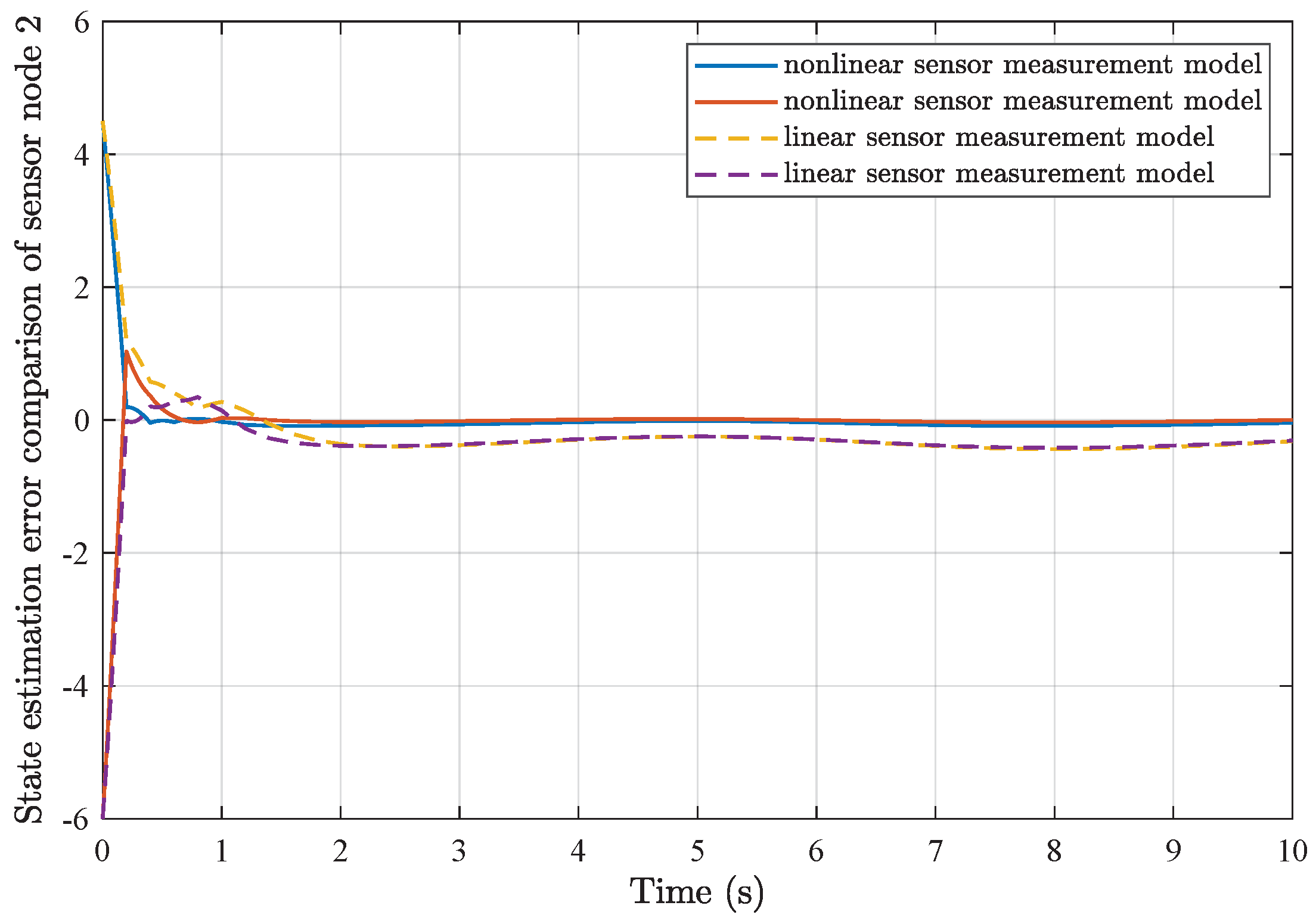
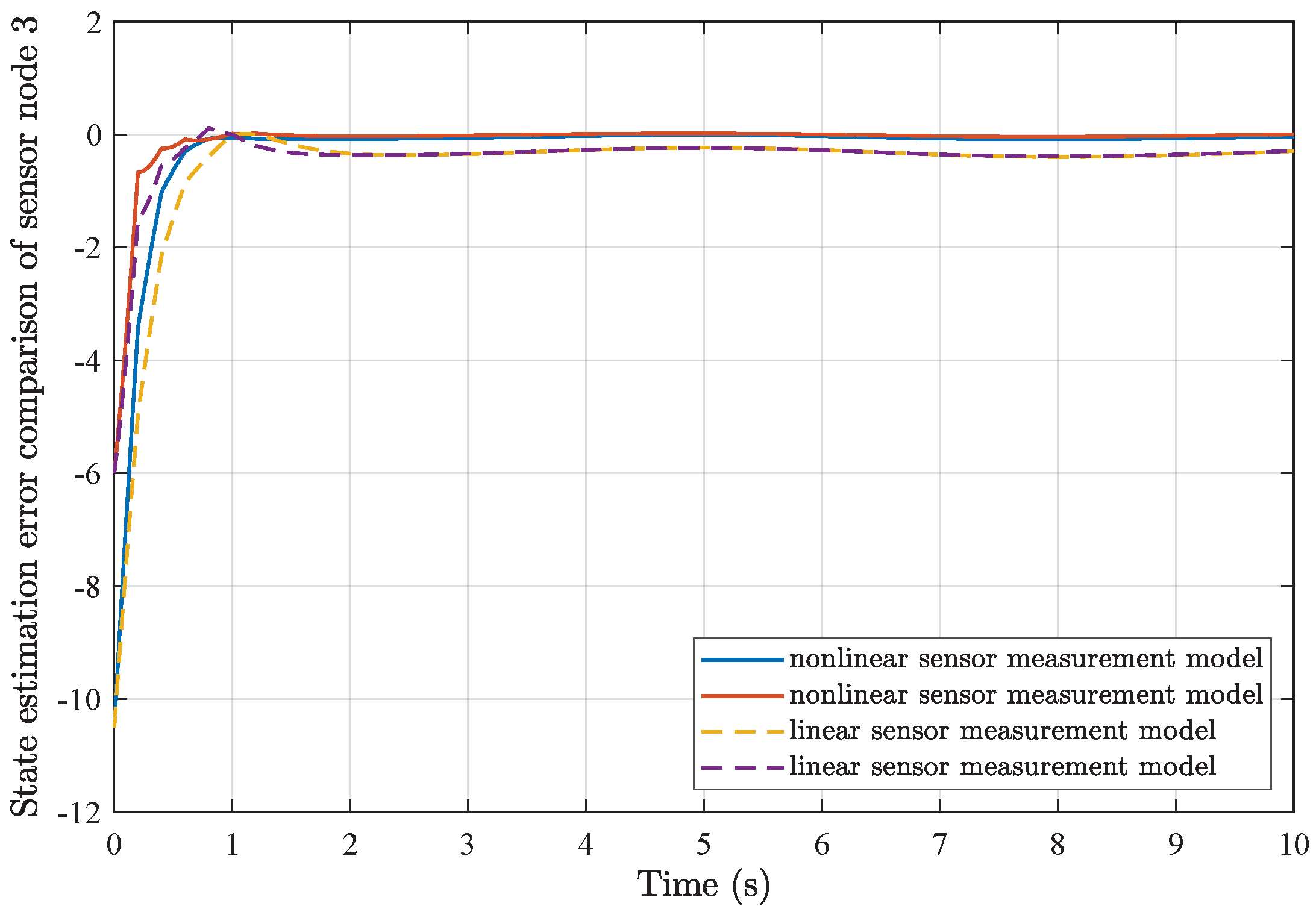
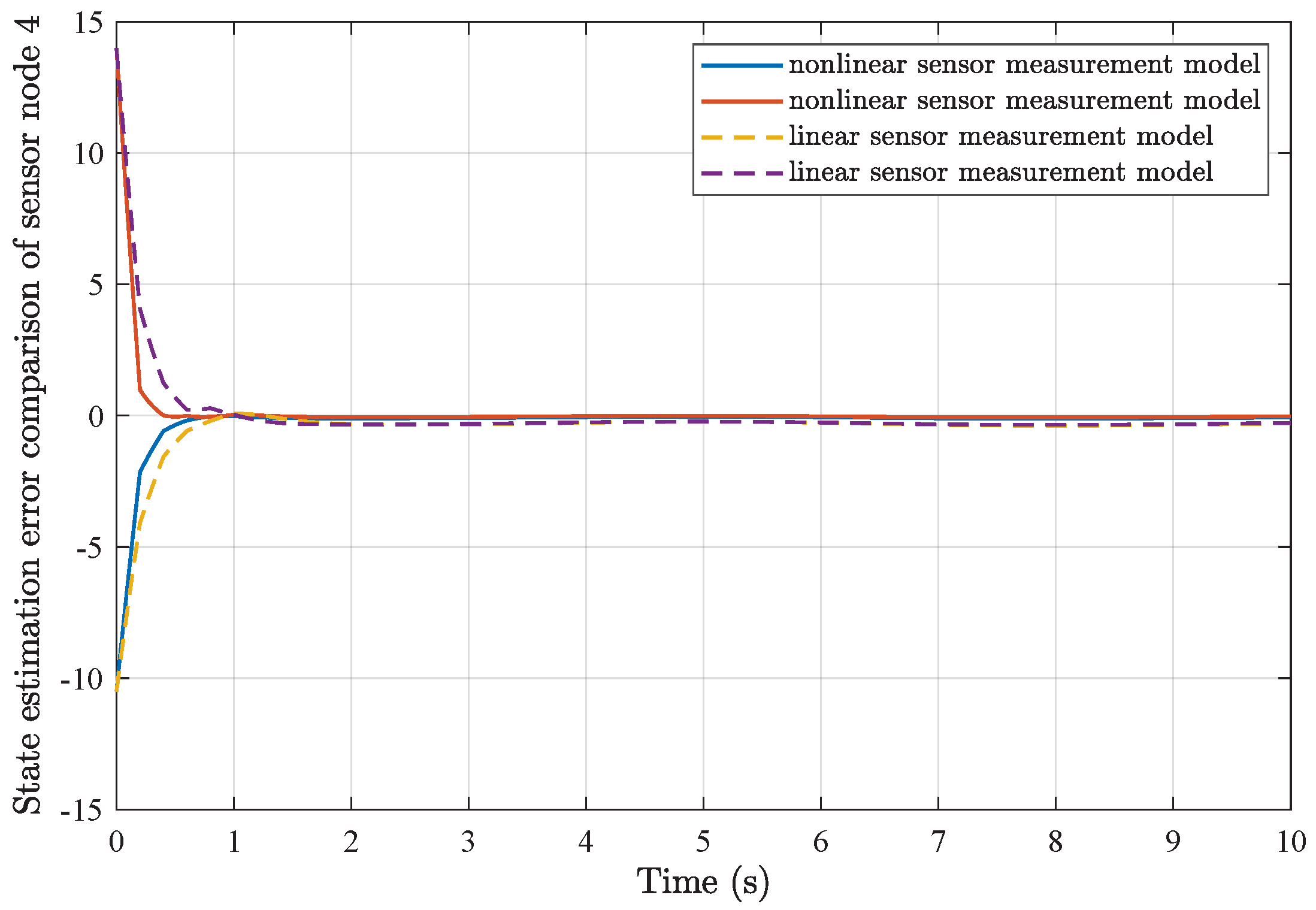
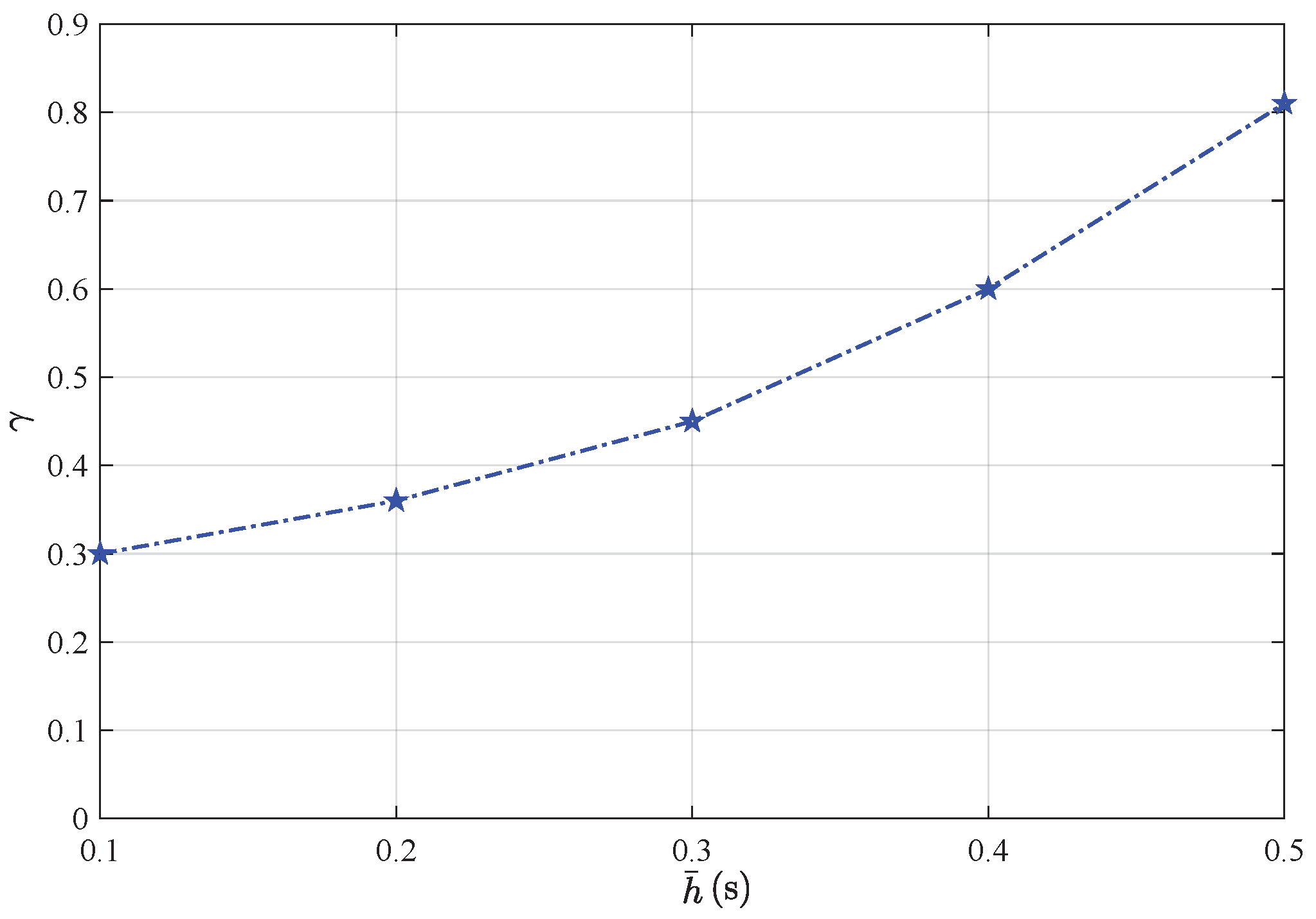
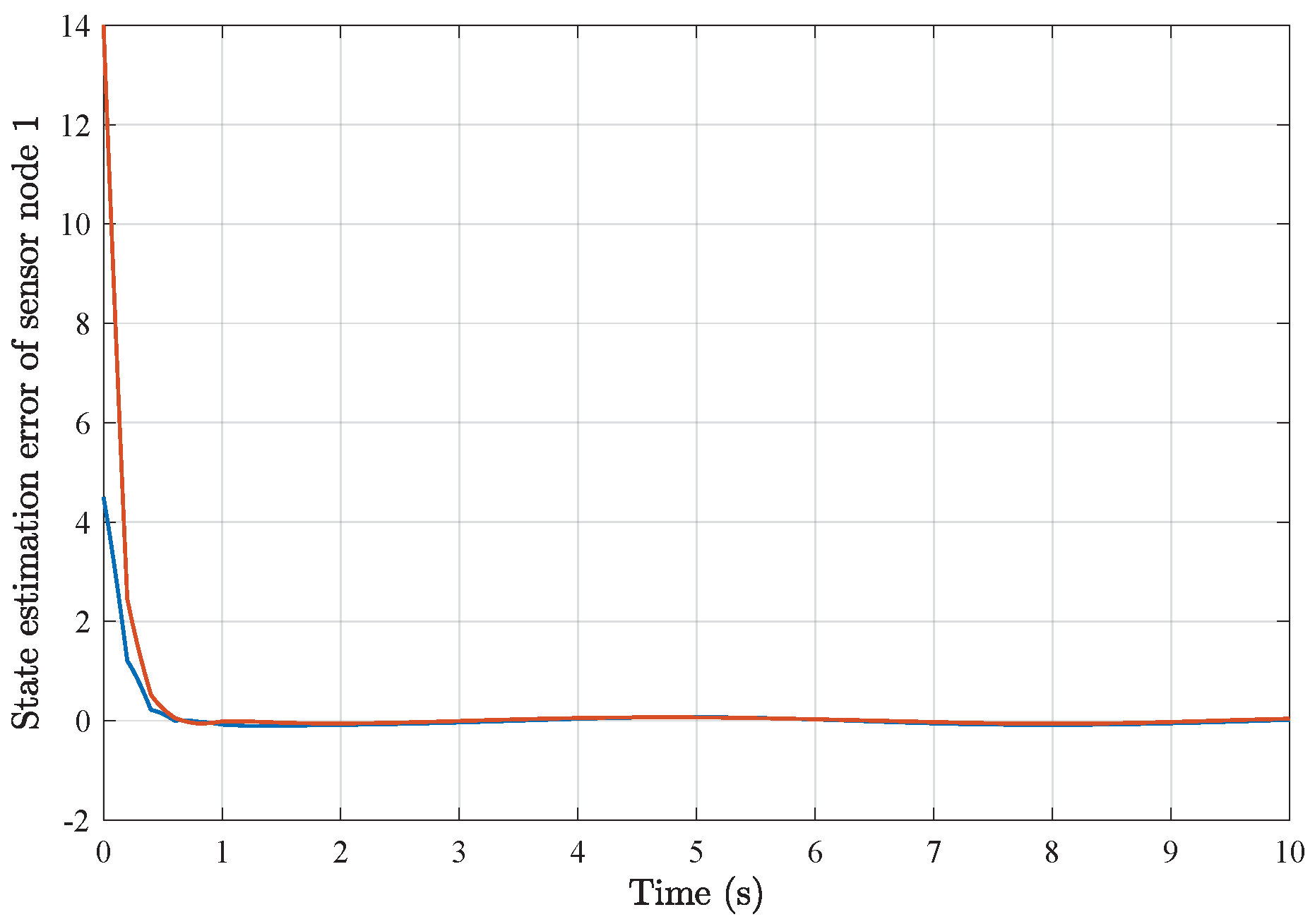
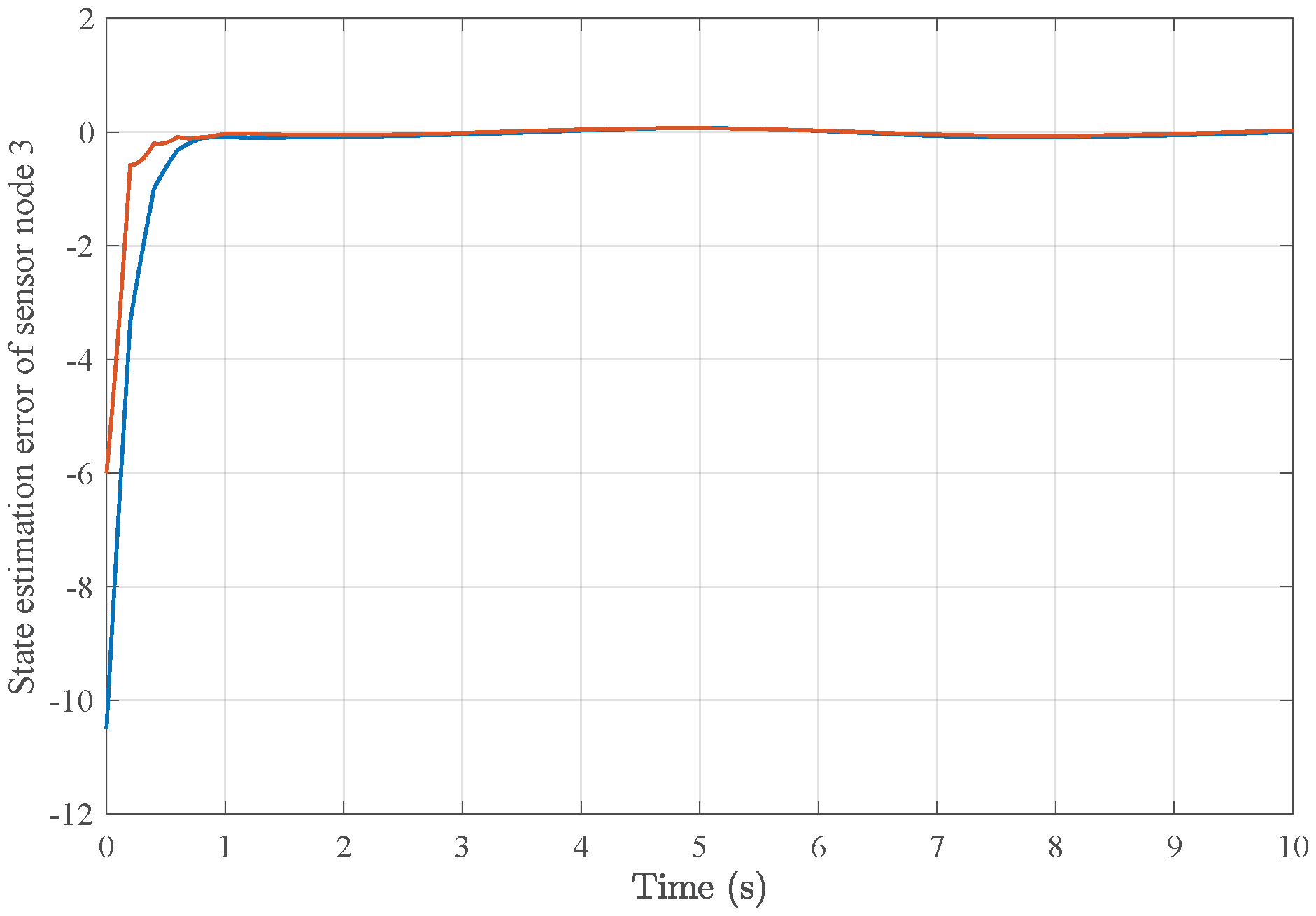
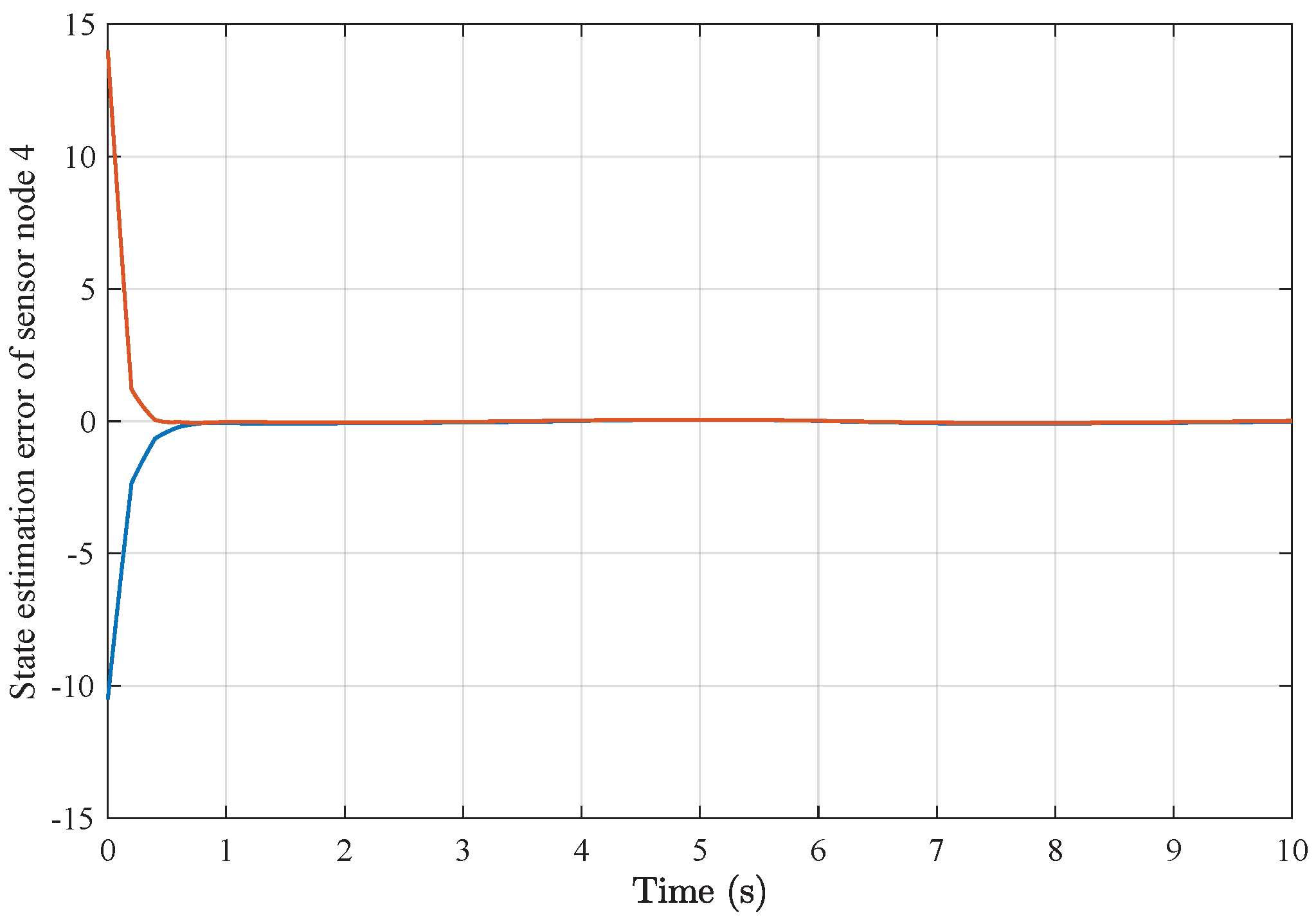
4. Illustrative Examples
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Oliveira, L.M.; Rodrigues, J.J. Wireless Sensor Networks: A Survey on Environmental Monitoring. J. Commun. 2011, 6, 143–151. [Google Scholar] [CrossRef]
- Gungor, V.C.; Lu, B.; Hancke, G.P. Opportunities and challenges of wireless sensor networks in smart grid. IEEE Trans. Ind. Electron. 2010, 57, 3557–3564. [Google Scholar] [CrossRef]
- Ota, N.; Wright, P. Trends in wireless sensor networks for manufacturing. Int. J. Manuf. Res. 2006, 1, 3–17. [Google Scholar]
- BenSaleh, M.S.; Saida, R.; Kacem, Y.H.; Abid, M. Wireless sensor network design methodologies: A survey. J. Sens. 2020, 2020, 9592836. [Google Scholar]
- Cheng, L.; Wu, C.; Zhang, Y.; Wu, H.; Li, M.; Maple, C. A survey of localization in wireless sensor network. Int. J. Distrib. Sens. Netw. 2012, 8, 962523. [Google Scholar]
- Wang, Y.A.; Shen, B.; Zou, L.; Han, Q.L. A survey on recent advances in distributed filtering over sensor networks subject to communication constraints. Int. J. Netw. Dyn. Intell. 2023, 2, 100007. [Google Scholar]
- Zhang, D.; Cai, W.; Xie, L.; Wang, Q.G. Nonfragile distributed filtering for T–S fuzzy systems in sensor networks. IEEE Trans. Fuzzy Syst. 2014, 23, 1883–1890. [Google Scholar]
- Modalavalasa, S.; Sahoo, U.K.; Sahoo, A.K.; Baraha, S. A review of robust distributed estimation strategies over wireless sensor networks. Signal Process. 2021, 188, 108150. [Google Scholar]
- Cho, J.J.; Ding, Y.; Chen, Y.; Tang, J. Robust calibration for localization in clustered wireless sensor networks. IEEE Trans. Autom. Sci. Eng. 2009, 7, 81–95. [Google Scholar]
- Williams, J.L.; Fisher, J.W.; Willsky, A.S. Approximate dynamic programming for communication-constrained sensor network management. IEEE Trans. Signal Process. 2007, 55, 4300–4311. [Google Scholar]
- Sinopoli, B.; Sharp, C.; Schenato, L.; Schaffert, S.; Sastry, S.S. Distributed control applications within sensor networks. Proc. IEEE 2003, 91, 1235–1246. [Google Scholar] [CrossRef]
- Niu, R.; Varshney, P.K.; Cheng, Q. Distributed detection in a large wireless sensor network. Inf. Fusion 2006, 7, 380–394. [Google Scholar] [CrossRef]
- Wang, Z.; Niu, Y. Distributed estimation and filtering for sensor networks. Taylor Fr. J. 2011, 42, 1421–1425. [Google Scholar] [CrossRef]
- Doraiswami, R.; Cheded, L. Fault diagnosis of a sensor network: A distributed filtering approach. J. Dyn. Syst. Meas. Control 2013, 135, 051002. [Google Scholar] [CrossRef]
- Hu, J.; Liang, J.; Chen, D.; Ji, D.; Du, J. A recursive approach to non-fragile filtering for networked systems with stochastic uncertainties and incomplete measurements. J. Frankl. Inst. 2015, 352, 1946–1962. [Google Scholar] [CrossRef]
- Liu, L.; Ma, L.; Zhang, J.; Bo, Y. Distributed non-fragile set-membership filtering for nonlinear systems under fading channels and bias injection attacks. Int. J. Syst. Sci. 2021, 52, 1192–1205. [Google Scholar] [CrossRef]
- Ali, M.S.; Vadivel, R.; Saravanakumar, R. Event-triggered state estimation for Markovian jumping impulsive neural networks with interval time-varying delays. Int. J. Control 2019, 92, 270–290. [Google Scholar] [CrossRef]
- Ding, D.; Wang, Z.; Shen, B. Recent advances on distributed filtering for stochastic systems over sensor networks. Int. J. Gen. Syst. 2014, 43, 372–386. [Google Scholar] [CrossRef]
- Oliveira, L.L.d.; Eisenkraemer, G.H.; Carara, E.A.; Martins, J.B.; Monteiro, J. Mobile localization techniques for wireless sensor networks: Survey and recommendations. ACM Trans. Sens. Netw. 2023, 19, 1–39. [Google Scholar] [CrossRef]
- Wan, J.; Hu, Z.; Cai, J.; Luo, Y.; Mei, C.; Han, A. Non-fragile dissipative filtering of cyber–physical systems with random sensor delays. ISA Trans. 2020, 104, 115–121. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, H.; Ding, X. Non-fragile filtering for large-scale power systems with sensor networks. IET Gener. Transm. Distrib. 2017, 11, 968–977. [Google Scholar] [CrossRef]
- Niu, Y.; Ho, D.W.; Li, C.W. Filtering for discrete fuzzy stochastic systems with sensor nonlinearities. IEEE Trans. Fuzzy Syst. 2010, 18, 971–978. [Google Scholar] [CrossRef]
- Wang, Z.; Xu, Y.; Lu, R.; Peng, H. Finite-time state estimation for coupled Markovian neural networks with sensor nonlinearities. IEEE Trans. Neural Netw. Learn. Syst. 2015, 28, 630–638. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, S.; Li, Y.; Chu, Y.; Zhang, Z. Asynchronous finite-time state estimation for semi-Markovian jump neural networks with randomly occurred sensor nonlinearities. Neurocomputing 2021, 432, 240–249. [Google Scholar] [CrossRef]
- Yan, H.; Zhang, H.; Yang, F.; Huang, C.; Chen, S. Distributed H∞ Filtering for Switched Repeated Scalar Nonlinear Systems with Randomly Occurred Sensor Nonlinearities and Asynchronous Switching. IEEE Trans. Syst. Man Cybern. Syst. 2017, 48, 2263–2270. [Google Scholar] [CrossRef]
- Dong, H.; Ding, S.X.; Ren, W. Distributed filtering with randomly occurring uncertainties over sensor networks: The channel fading case. Int. J. Gen. Syst. 2014, 43, 254–266. [Google Scholar] [CrossRef]
- Song, H.; Ding, D.; Dong, H.; Yi, X. Distributed filtering based on Cauchy-kernel-based maximum correntropy subject to randomly occurring cyber-attacks. Automatica 2022, 135, 110004. [Google Scholar] [CrossRef]
- Wu, Z.G.; Shi, P.; Shu, Z.; Su, H.; Lu, R. Passivity-based asynchronous control for Markov jump systems. IEEE Trans. Autom. Control. 2016, 62, 2020–2025. [Google Scholar] [CrossRef]
- Geromel, J.; de Oliveira, M.C.; Hsu, L. LMI characterization of structural and robust stability. Linear Algebra Its Appl. 1998, 285, 69–80. [Google Scholar] [CrossRef]
- Park, P.; Ko, J.W.; Jeong, C. Reciprocally convex approach to stability of systems with time-varying delays. Automatica 2011, 47, 235–238. [Google Scholar] [CrossRef]
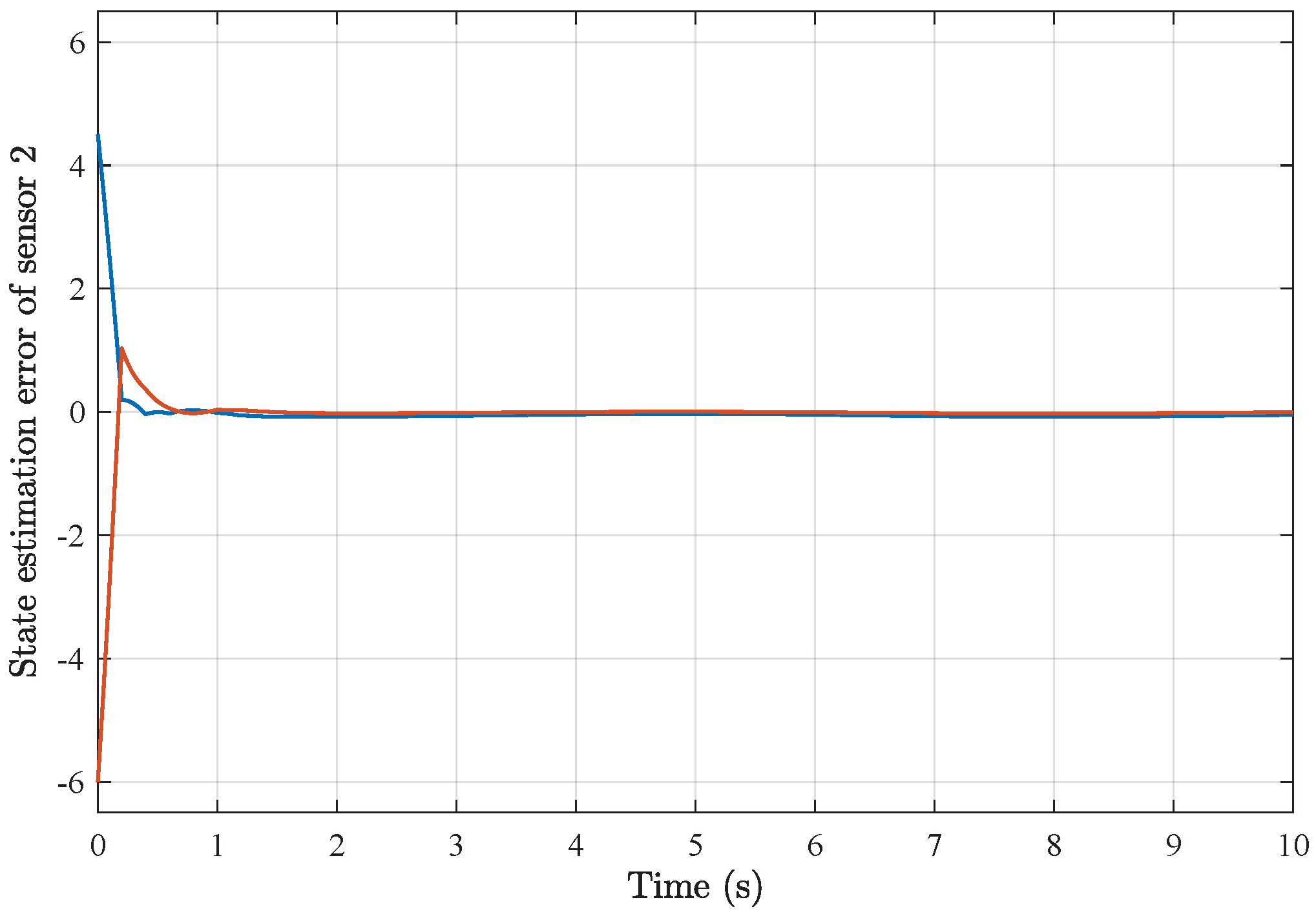





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, S.; Xu, K.; Huang, F. Distributed Non-Fragile State Estimation for Uncertain Nonlinear Systems of Sensor Networks Subject to Sensor Nonlinearities. Sensors 2025, 25, 1962. https://doi.org/10.3390/s25071962
Tian S, Xu K, Huang F. Distributed Non-Fragile State Estimation for Uncertain Nonlinear Systems of Sensor Networks Subject to Sensor Nonlinearities. Sensors. 2025; 25(7):1962. https://doi.org/10.3390/s25071962
Chicago/Turabian StyleTian, Shihui, Ke Xu, and Fengshan Huang. 2025. "Distributed Non-Fragile State Estimation for Uncertain Nonlinear Systems of Sensor Networks Subject to Sensor Nonlinearities" Sensors 25, no. 7: 1962. https://doi.org/10.3390/s25071962
APA StyleTian, S., Xu, K., & Huang, F. (2025). Distributed Non-Fragile State Estimation for Uncertain Nonlinear Systems of Sensor Networks Subject to Sensor Nonlinearities. Sensors, 25(7), 1962. https://doi.org/10.3390/s25071962






