A Current Sensing Cross-Component Induction Magnetometer for Use in Time-Domain Borehole Geophysical Electromagnetic Surveys
Abstract
1. Introduction
2. Inductive and Other Magnetic EM Sensors
2.1. Sensor Considerations
2.2. Sensitivity of a Wideband Magnetometer Using Current-to-Voltage Conversion
2.3. Sensitivity Corner Frequency
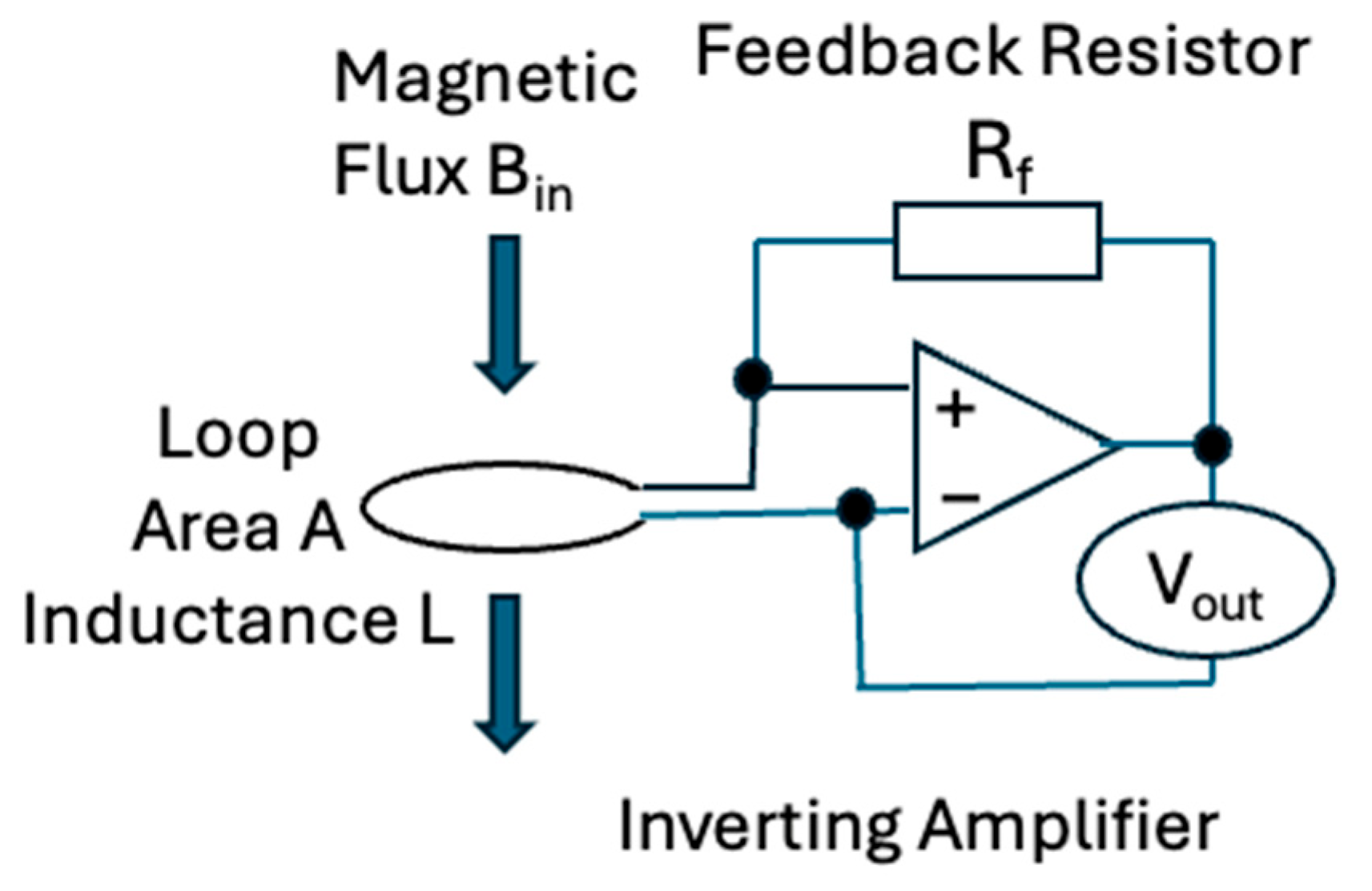
3. Proposed Design
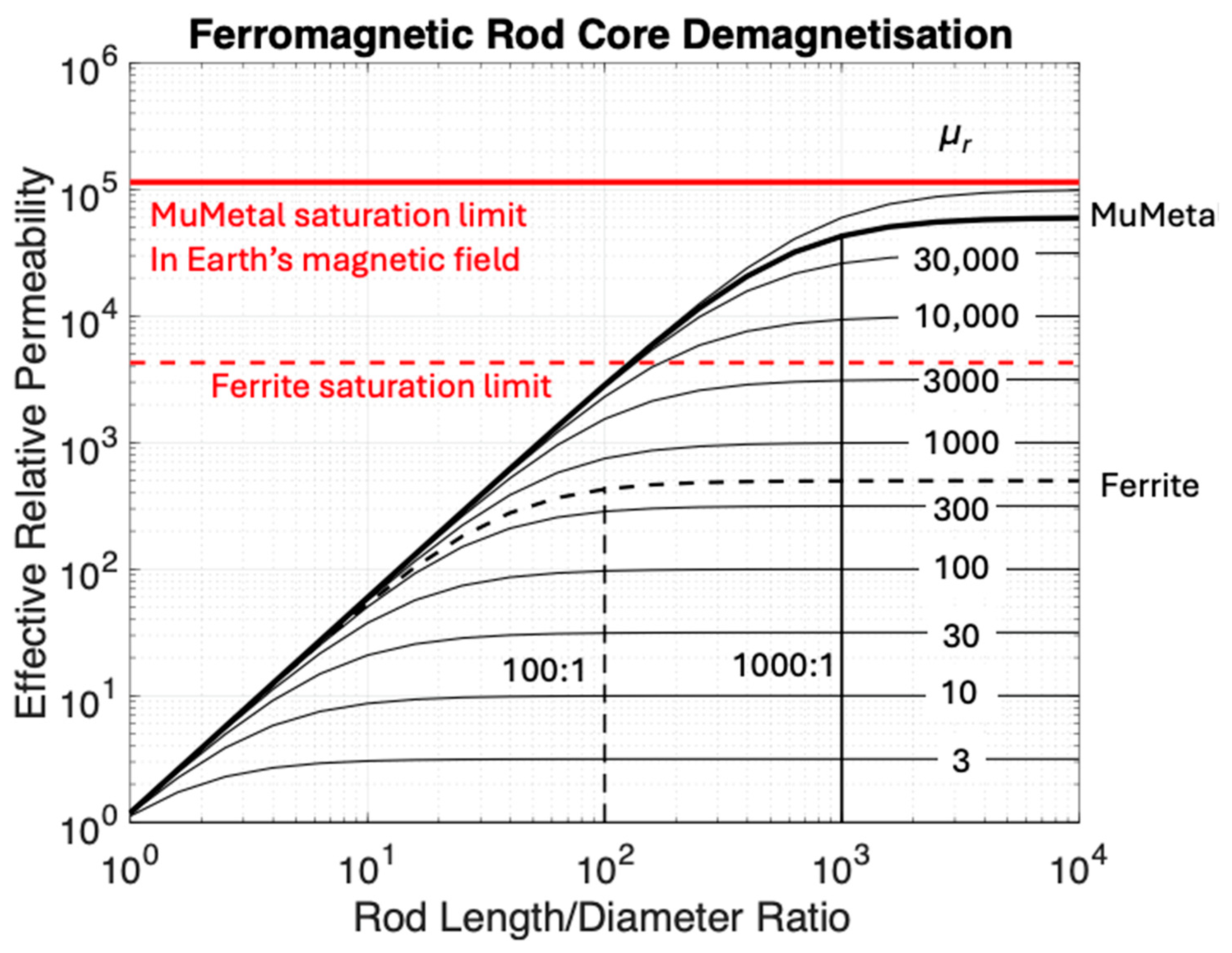
3.1. Ferromagnetic Cores
3.2. Cross-Component Considerations
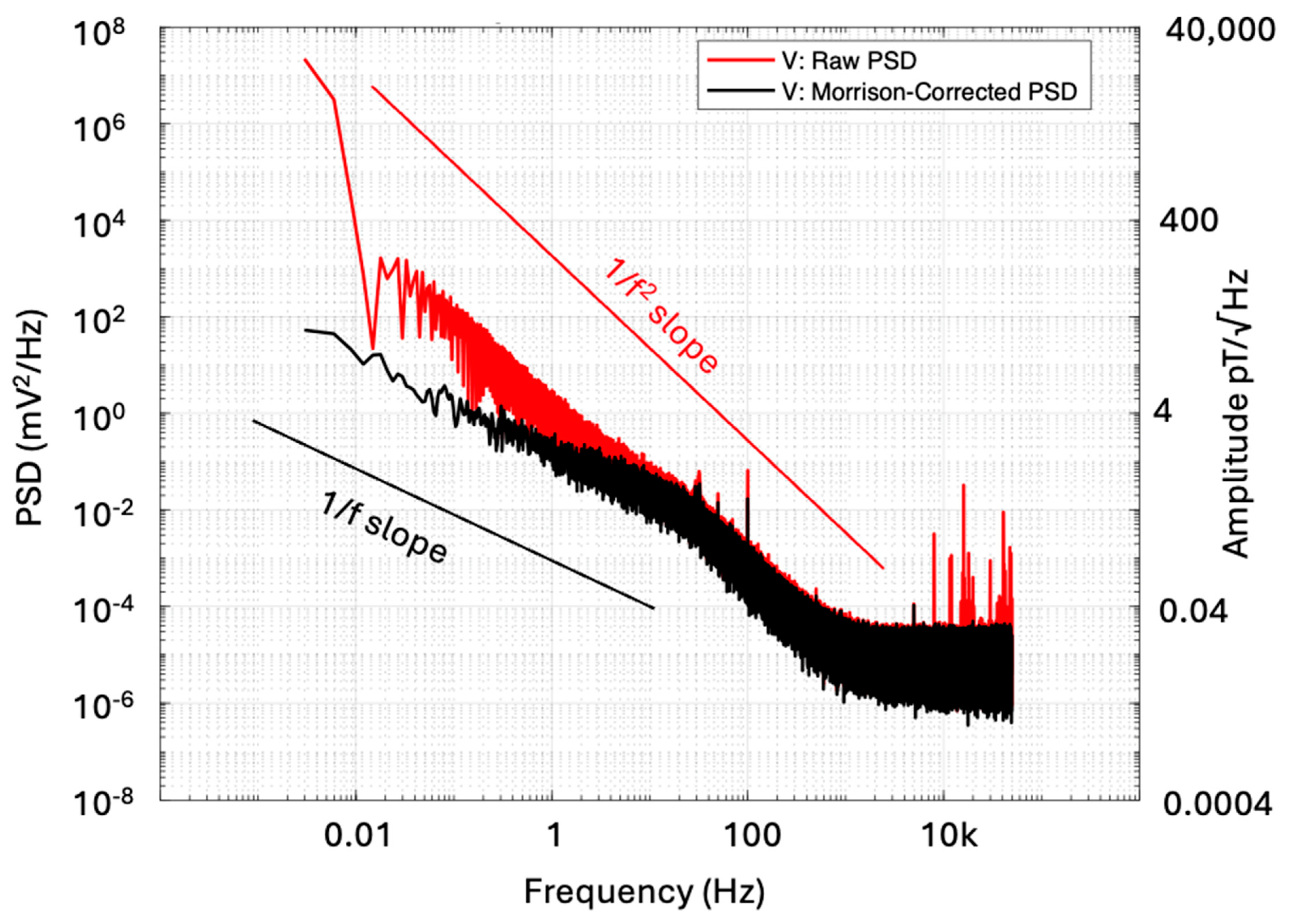
4. Results
4.1. Sensor Construction
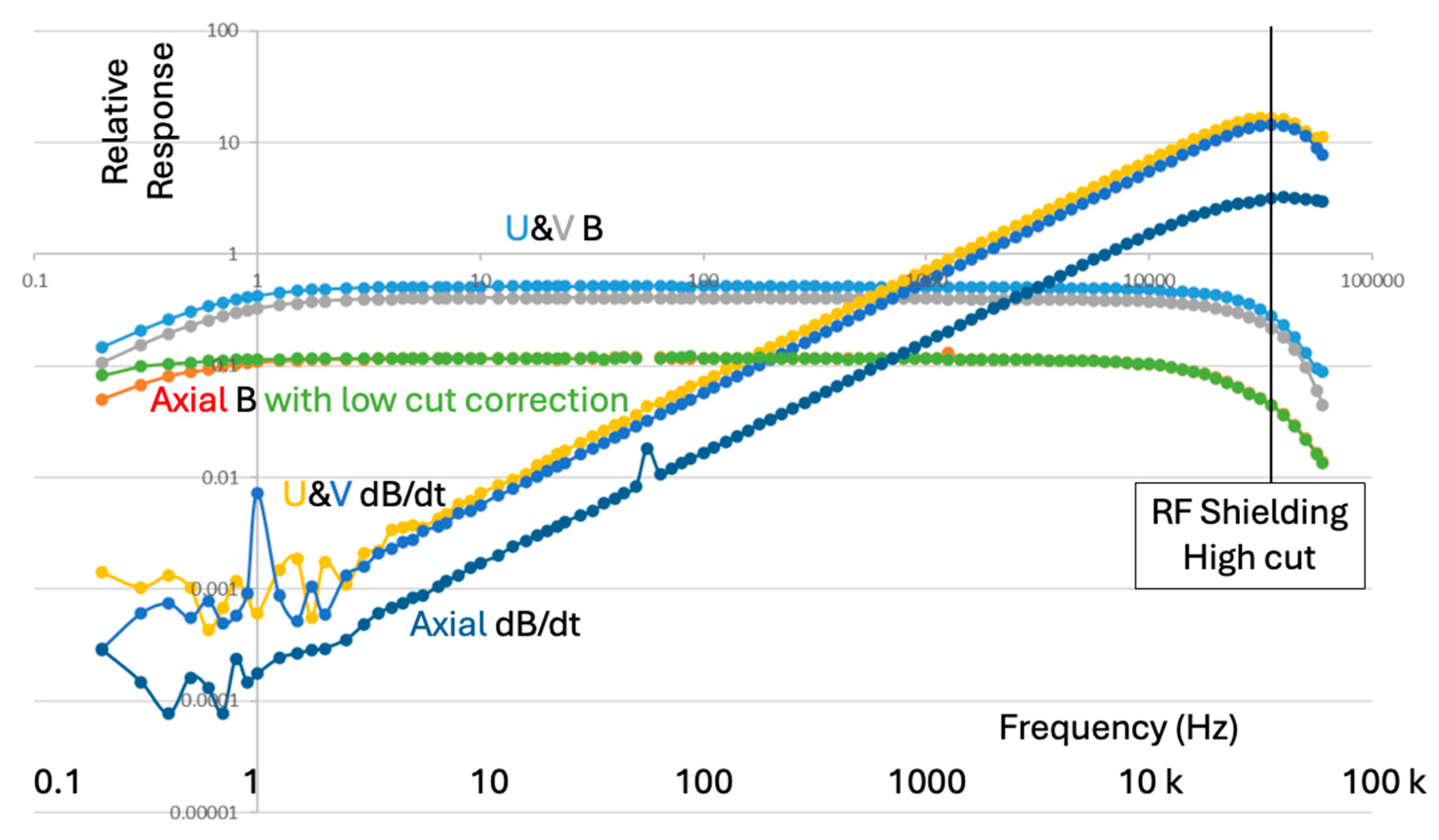
4.2. Corner Frequency Measurements
4.3. Sensor Comparison
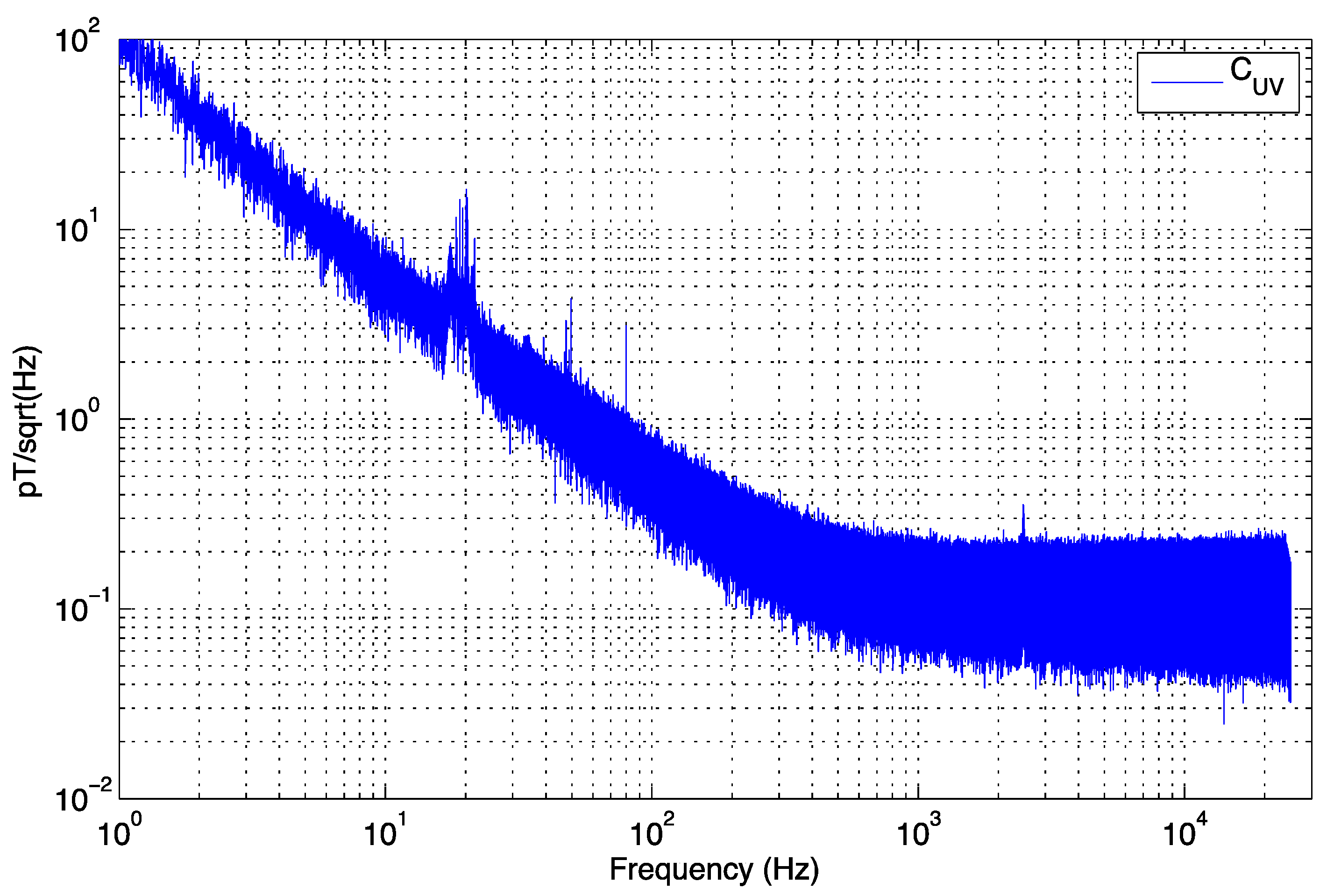
4.4. Magnetic Sensor Sensitivity
4.5. Sensor Noise
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
= |1 + εA|2 [E(CC*} + E[nA nA*] − (1 + εB) (1 + εA) [E(CC*]
= (εA − εB − εBεA* + εAεA*) E(CC*) + (1 − 2Re(εA) + εAεA*) E[nA nA*]
References
- Dentith, M.; Mudge, S.T. Geophysics for the Mineral Exploration Geoscientist; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Everett, M.E. Near-Surface Applied Geophysics; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Lamontagne, Y. (Ed.) Deep exploration with EM in boreholes. In Proceedings of the Exploration 07: Fifth Decennial International Conference on Mineral Exploration, Toronto, ON, Canada, 9–12 September 2007. [Google Scholar]
- Schmelz, M.; Stolz, R. Superconducting quantum interference device (SQUID) magnetometers. In High Sensitivity Magnetometers; Springer: Cham, Switzerland, 2017; pp. 279–311. [Google Scholar]
- Staacke, R.; John, R.; Wunderlich, R.; Horsthemke, L.; Knolle, W.; Laube, C.; Glösekötter, P.; Burchard, B.; Abel, B.; Meijer, J. Isotropic scalar quantum sensing of magnetic fields for industrial application. Adv. Quantum Technol. 2020, 3, 2000037. [Google Scholar] [CrossRef]
- Spies, B. Depth of investigation in electromagnetic sounding methods. Geophysics 1989, 54, 872–888. [Google Scholar] [CrossRef]
- Tumański, S. Induction coil sensors—A review. Meas. Sci. Technol. 2007, 18, R31. [Google Scholar] [CrossRef]
- Hamad, J.; Macnae, J. Using induction coil sensor optimization techniques for designing compact geophysical transmitters. ASEG Ext. Abstr. 2015, 2015, 1–4. [Google Scholar] [CrossRef]
- Chen, C.; Liu, F.; Lin, J.; Wang, Y. Investigation and optimization of the performance of an air-coil sensor with a differential structure suited to helicopter TEM exploration. Sensors 2015, 15, 23325–23340. [Google Scholar] [CrossRef]
- Grosz, A. Analytical Optimization of Low-Frequency Search Coil Magnetometers. IEEE Sens. J. 2012, 8, 2719–2723. [Google Scholar] [CrossRef]
- Liu, K.; Zhu, W.; Yan, B.; Liu, L.; Fang, G. Ultralow noise preamplifier and optimization method for induction magnetometers. IEEE Sens. J. 2015, 15, 3293–3300. [Google Scholar] [CrossRef]
- Coillot, C.; Moutoussamy, J.; Boda, M.; Leroy, P. New ferromagnetic core shapes for induction sensors. J. Sens. Sens. Syst. 2014, 3, 1–8. [Google Scholar] [CrossRef][Green Version]
- Shi, H.; Wang, Y.; Lin, J.; Li, J. Numerical optimization of the tube-cored induction magnetometer weight under specific noise constraints. IEEE Sens. J. 2017, 17, 3302–3308. [Google Scholar] [CrossRef]
- Stolz, R.; Schiffler, M.; Becken, M.; Thiede, A.; Schneider, M.; Chubak, G.; Marsden, P.; Bergshjorth, A.B.; Schaefer, M.; Terblanche, O. SQUIDs for magnetic and electromagnetic methods in mineral exploration. Miner. Econ. 2022, 35, 467–494. [Google Scholar] [CrossRef]
- Asten, M.W.; Duncan, A.C. The quantitative advantages of using B-field sensors in time-domain EM measurement for mineral exploration and unexploded ordnance search. Geophysics 2012, 77, WB137–WB148. [Google Scholar] [CrossRef]
- Purss, M.B.; Cull, J.P. B-field probes for downhole magnetometric resistivity surveys. Explor. Geophys. 2003, 34, 233–240. [Google Scholar] [CrossRef]
- De la Vergne, J. Hard Rock Miner’s Handbook. In Tempe/North Bay; McIntosh Engineering: Princes Risborough, UK, 2003; ISBN 0-968006-1-6. [Google Scholar]
- Bishop, J.; Lewis, R. Introduction to the 1996 Special Volume on DHEM. Explor. Geophys. 1996, 27, 37–39. [Google Scholar] [CrossRef]
- Hodges, D.G.; Crone, J.D.; Pemberton, R. A New Multiple Component Downhole Pulse EM Probe for Directional Interpretation. In Proceedings of the 4th International MLGS/KEGS Symposium on Borehole Geophysics for Minerals, Geotechnical and Groundwater Applications, Toronto, ON, Canada, 18–22 August 1991. [Google Scholar]
- Soininen, H.; Hongisto, H.; Jokinen, J.; Mononen, R. SlimBORIS Drill-Hole EM System; European Association of Geoscientists & Engineers: Odijk, The Netherlands, 2001. [Google Scholar]
- Dehmel, G. Magnetic field sensors: Induction coil (search coil) sensors. In Sensors Set: A Comprehensive Survey; Wiley: Hoboken, NJ, USA, 1995; pp. 205–253. [Google Scholar]
- Song, S.; Deng, M.; Chen, K.; Jin, S. A new borehole electromagnetic receiver developed for controlled-source electromagnetic methods. Geosci. Instrum. Methods Data Syst. 2021, 10, 55–64. [Google Scholar] [CrossRef]
- Han, F.; Harada, S.; Sasada, I. Fluxgate and search coil hybrid: A low-noise wide-band magnetometer. IEEE Trans. Magn. 2012, 48, 3700–3703. [Google Scholar] [CrossRef]
- Shi, H.; Wang, Y.; Chen, S.; Lin, J. A dumbbell-shaped hybrid magnetometer operating in DC-10 kHz. Rev. Sci. Instrum. 2017, 88, 125001. [Google Scholar] [CrossRef]
- Tashiro, K.; Hattori, G.-Y.; Wakiwaka, H. (Eds.) Magnetic flux concentration methods for magnetic energy harvesting module. EPJ Web Conf. 2013, 40, 06011. [Google Scholar] [CrossRef]
- Ziegler, S.; Woodward, R.C.; Iu, H.H.-C.; Borle, L.J. Current sensing techniques: A review. IEEE Sens. J. 2009, 9, 354–376. [Google Scholar] [CrossRef]
- Prance, R.; Clark, T.; Prance, H. Compact broadband gradiometric induction magnetometer system. Sens. Actuators A Phys. 1999, 76, 117–121. [Google Scholar] [CrossRef]
- Prance, R.; Clark, T.; Prance, H. Ultra low noise induction magnetometer for variable temperature operation. Sens. Actuators A Phys. 2000, 85, 361–364. [Google Scholar] [CrossRef]
- Prance, R.; Clark, T.; Prance, H. Compact Room-Temperature Induction Magnetometer with Superconducting Quantum Interference Device Level Field Sensitivity. Rev. Sci. Instrum. 2003, 74, 3735–3739. [Google Scholar] [CrossRef]
- Macnae, J.; Kratzer, T. Joint sensing of B and dB/dt responses. ASEG Ext. Abstr. 2013, 2013, 1–4. [Google Scholar] [CrossRef]
- Duncan, A. (Ed.) Advances in ground and borehole EM survey technology to 2017. In Proceedings of the Exploration 17: Sixth Decennial International Conference on Mineral Exploration, Toronto, ON, Canada, 22–25 October 2017. [Google Scholar]
- Bichurin, M.; Petrov, R.; Sokolov, O.; Leontiev, V.; Kuts, V.; Kiselev, D.; Wang, Y. Magnetoelectric Magnetic Field Sensors: A Review. Sensors 2021, 21, 6232. [Google Scholar] [CrossRef] [PubMed]
- Luong, V.S.; Jeng, J.-T.; Lu, C.-C.; Hsu, H.-Y. Low-noise tunneling-magnetoresistance vector magnetometers with flux chopping technique. Measurement 2017, 109, 297–303. [Google Scholar] [CrossRef]
- Eadie, T.; Staltari, G. Introduction to downhole electromagnetic methods. Explor. Geophys. 1987, 18, 247–254. [Google Scholar] [CrossRef]
- Accomando, F.; Florio, G. Applicability of Small and Low-Cost Magnetic Sensors to Geophysical Exploration. Sensors 2024, 24, 7047. [Google Scholar] [CrossRef]
- Wei, S.; Liao, X.; Zhang, H.; Pang, J.; Zhou, Y. Recent progress of fluxgate magnetic sensors: Basic research and application. Sensors 2021, 21, 1500. [Google Scholar] [CrossRef]
- Ripka, P. Magnetic Sensors and Magnetometers; Artech House: London, UK, 2021. [Google Scholar]
- Alan Payne Associates. The Inductance of Ferrite Rod Antennas. 2021. Available online: https://www.researchgate.net/publication/351552681_The_Inductance_of_Ferrite_Rod_Antennas (accessed on 3 March 2025).
- Kaverine, E.; Palud, S.; Colombel, F.; Himdi, M. Investigation on an effective magnetic permeability of the rod-shaped ferrites. Prog. Electromagn. Res. Lett. 2017, 65, 43–48. [Google Scholar] [CrossRef]
- Kiran, M.R.; Islam, M.R.; Muttaqi, K.M.; Sutanto, D.; Raad, R. A Comprehensive Review of Advanced Core Materials-Based High-Frequency Magnetic Links Used in Emerging Power Converter Applications. IEEE Access 2024, 12, 107769–107799. [Google Scholar] [CrossRef]
- Schwarze, G. (Ed.) Advanced Electrical Materials and Components Development—An Update. In Proceedings of the 3rd International Energy Conversion Engineering Conference, San Francisco, CA, USA, 15–18 August 2005. [Google Scholar]
- Barr, R.; Jones, D.L.; Rodger, C. ELF and VLF radio waves. J. Atmos. Sol.-Terr. Phys. 2000, 62, 1689–1718. [Google Scholar] [CrossRef]
- Morrison, H.F.; Conti, U.; Labson, V.F.; Nichols, E.A.; Goldstein, N.E. Field Tests of Noise in SQUID and Induction Coil Magnetometers; Lawrence Berkeley Laboratories: Berkeley CA, USA, 1984. [Google Scholar]
- Nichols, E.A.; Morrison, H.F.; Clarke, J. Signals and Noise in Measurements of Low-Frequency Geomagnetic Fields. J. Geophys. Res. Solid Earth 1988, 93, 13743–13754. [Google Scholar] [CrossRef]








| Sensor | Pros | Cons | Reference |
|---|---|---|---|
| Induction coil current sensor (CCIM) | Ideal for axial component; sensitive to long and short decays | Fair for cross-components; reduced long-time constant sensitivity | [21,30] |
| Feedback coil | Very good for axial component | Limited bandwidth with both low- and high-cut character. Poor for cross-component | [3,21] |
| Fluxgate | Good for slow decays and very compact so all 3 component sensors are identical | Limited bandwidth insensitive above a few kHz. Higher noise than other sensors above 10 Hz | [31] |
| Induction coil voltage sensor | Good for fast decays up to a resonant limit. Cross-component a problem | Poor cross-component signal/noise. Poor for slow decays | [7,21] |
| Analog differentiated current sensor | Best for fast decays. A simple addition to a current sensor | Sensitivity loss at low frequency and poor for slow decays | [30] |
| MagnetoElectric | Compact sensor with wide bandwidth | Noise levels no better than fluxgates at low frequency | [32] |
| Tunneling MagneoResistive | Compact | Noise levels orders of magnitude worse than other sensors | [33] |
| SQUID | Lowest noise | Cryostat will not fit in borehole; venting issue with fluid boiloff | [14] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamad, J.; Macnae, J. A Current Sensing Cross-Component Induction Magnetometer for Use in Time-Domain Borehole Geophysical Electromagnetic Surveys. Sensors 2025, 25, 1646. https://doi.org/10.3390/s25061646
Hamad J, Macnae J. A Current Sensing Cross-Component Induction Magnetometer for Use in Time-Domain Borehole Geophysical Electromagnetic Surveys. Sensors. 2025; 25(6):1646. https://doi.org/10.3390/s25061646
Chicago/Turabian StyleHamad, Joseph, and James Macnae. 2025. "A Current Sensing Cross-Component Induction Magnetometer for Use in Time-Domain Borehole Geophysical Electromagnetic Surveys" Sensors 25, no. 6: 1646. https://doi.org/10.3390/s25061646
APA StyleHamad, J., & Macnae, J. (2025). A Current Sensing Cross-Component Induction Magnetometer for Use in Time-Domain Borehole Geophysical Electromagnetic Surveys. Sensors, 25(6), 1646. https://doi.org/10.3390/s25061646






