Mathematical Modeling and Finite Element Simulation of the M8514-P2 Composite Piezoelectric Transducer for Energy Harvesting
Abstract
1. Introduction
2. Materials and Methods
2.1. Piezoelectric Material, Selection, and Orientation
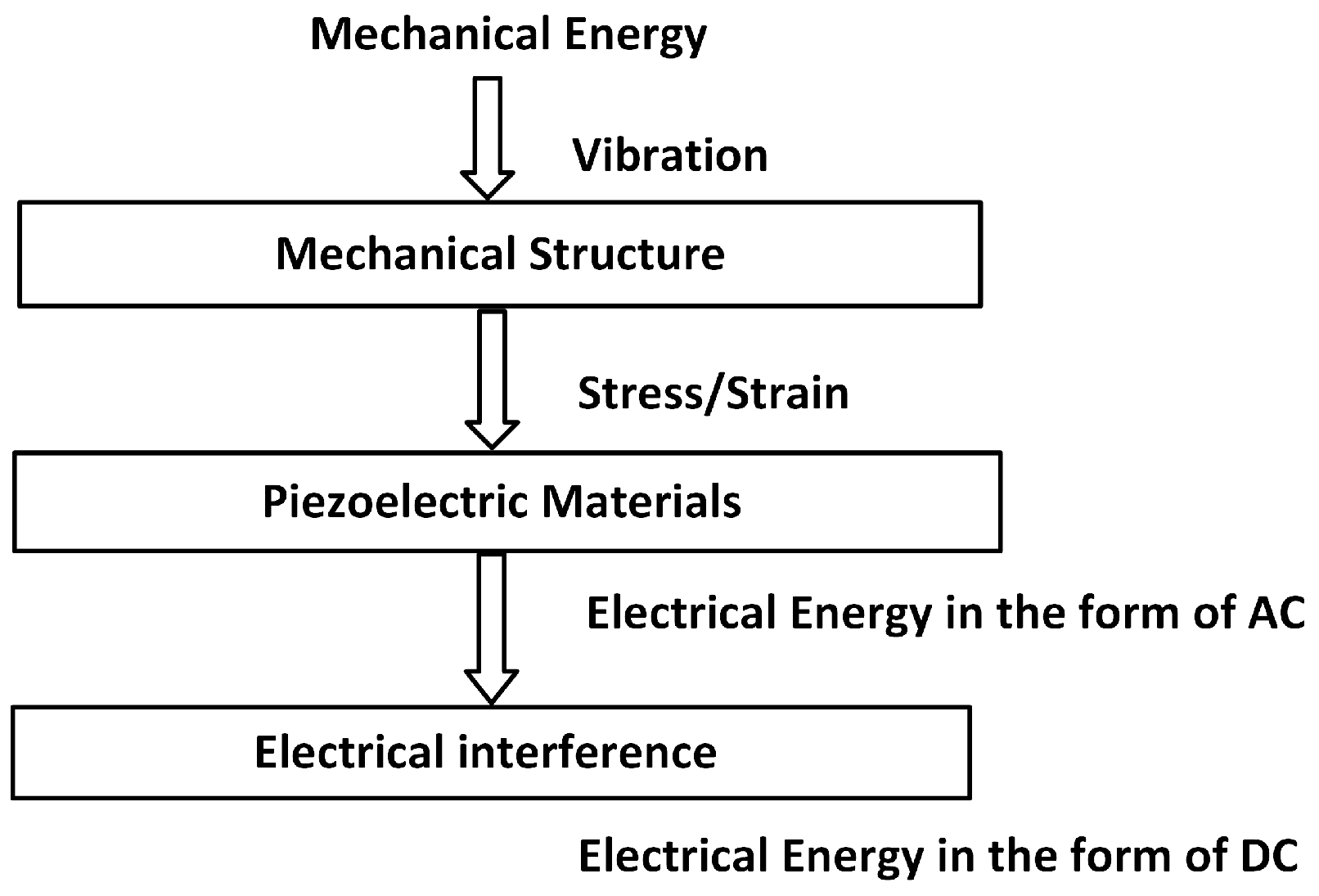
2.2. Modeling of Direct Piezoelectric Transducer for Energy Harvesting
2.3. Mathematical Modeling of MFC Piezo-Transducer Integrated with Cantilever Beam
- Stress–charge form:
- -
- The piezoelectric layer electrodes and the adhesive layer have a negligible thickness.
- -
- There is perfect bonding between the piezoelectric layer and the substrate layers.
- -
- A constant, uniform electric field exists throughout the thickness of the piezoelectric layer.
- -
- Proportional damping is present across all harvester configurations.
- -
- Effective flexural rigidity:
- -
- b: width of the piezoelectric layer;
- -
- : thickness of the piezoelectric layer;
- -
- : elastic modulus of the piezoelectric layer;
- -
- : piezoelectric stress constant;
- -
- : voltage across the piezoelectric layer;
- -
- and : positions along the beam where the macro fiber composite (MFC) is attached.
Natural Frequency and Mode Shape
- (fixed end):
- (free end):
- , , or and or .
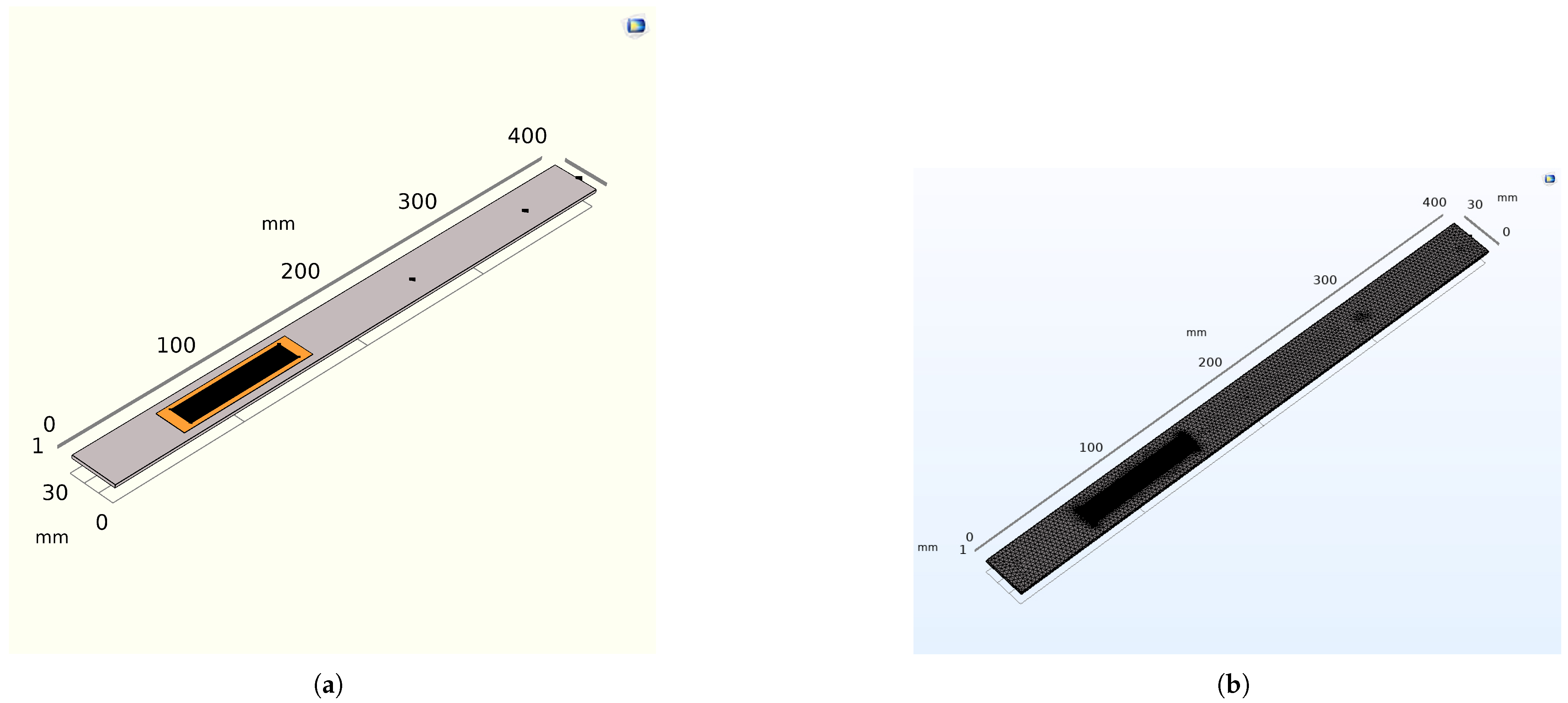
2.4. Numerical Modeling Using COMSOL Multiphysics
3. Results and Discussion
3.1. Component Parameters, Material Properties, and Dimensions for Analytical and Numerical Energy Harvester Model
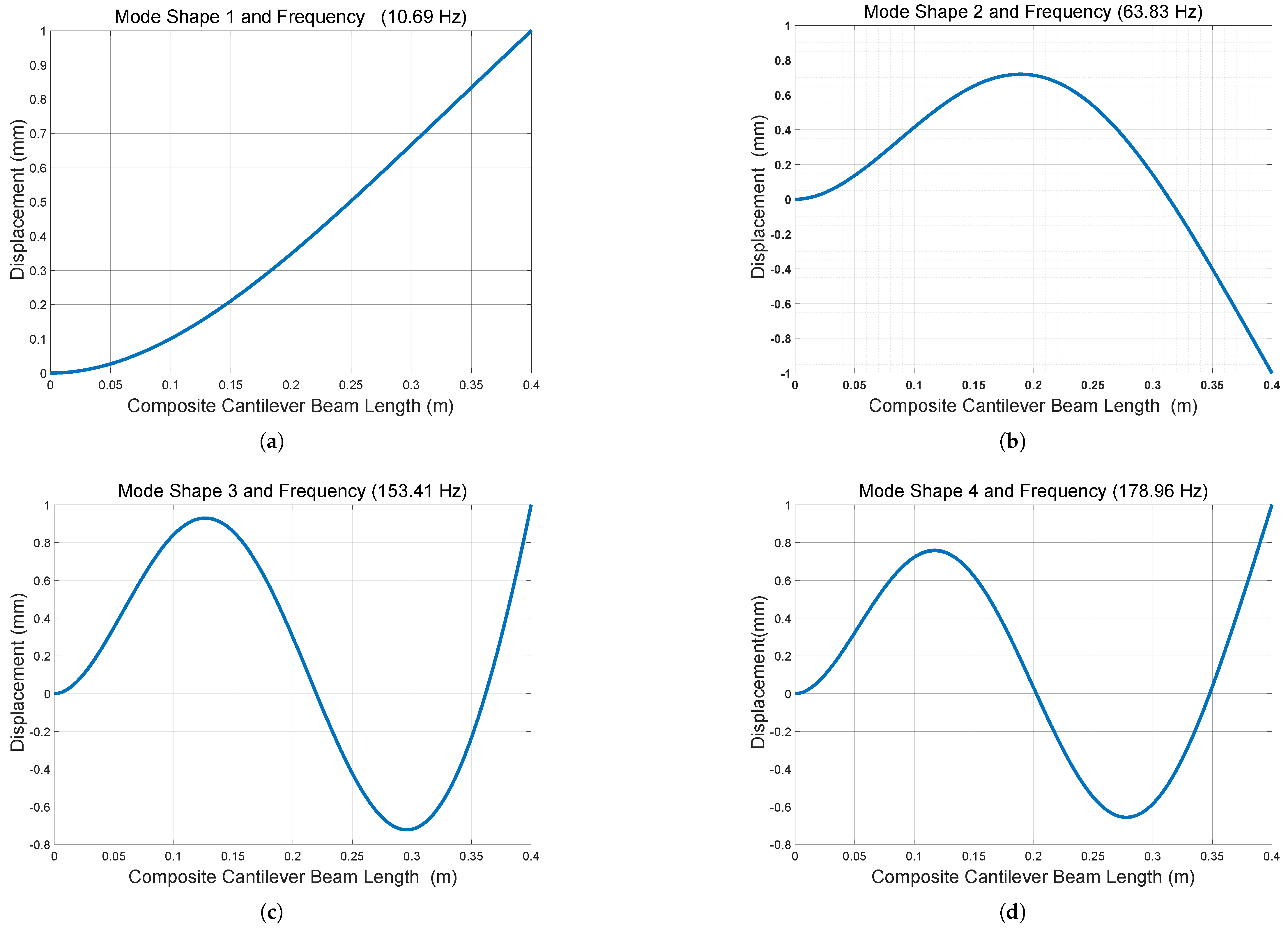
3.2. Analytical Design: Natural Frequency and Mode Shape
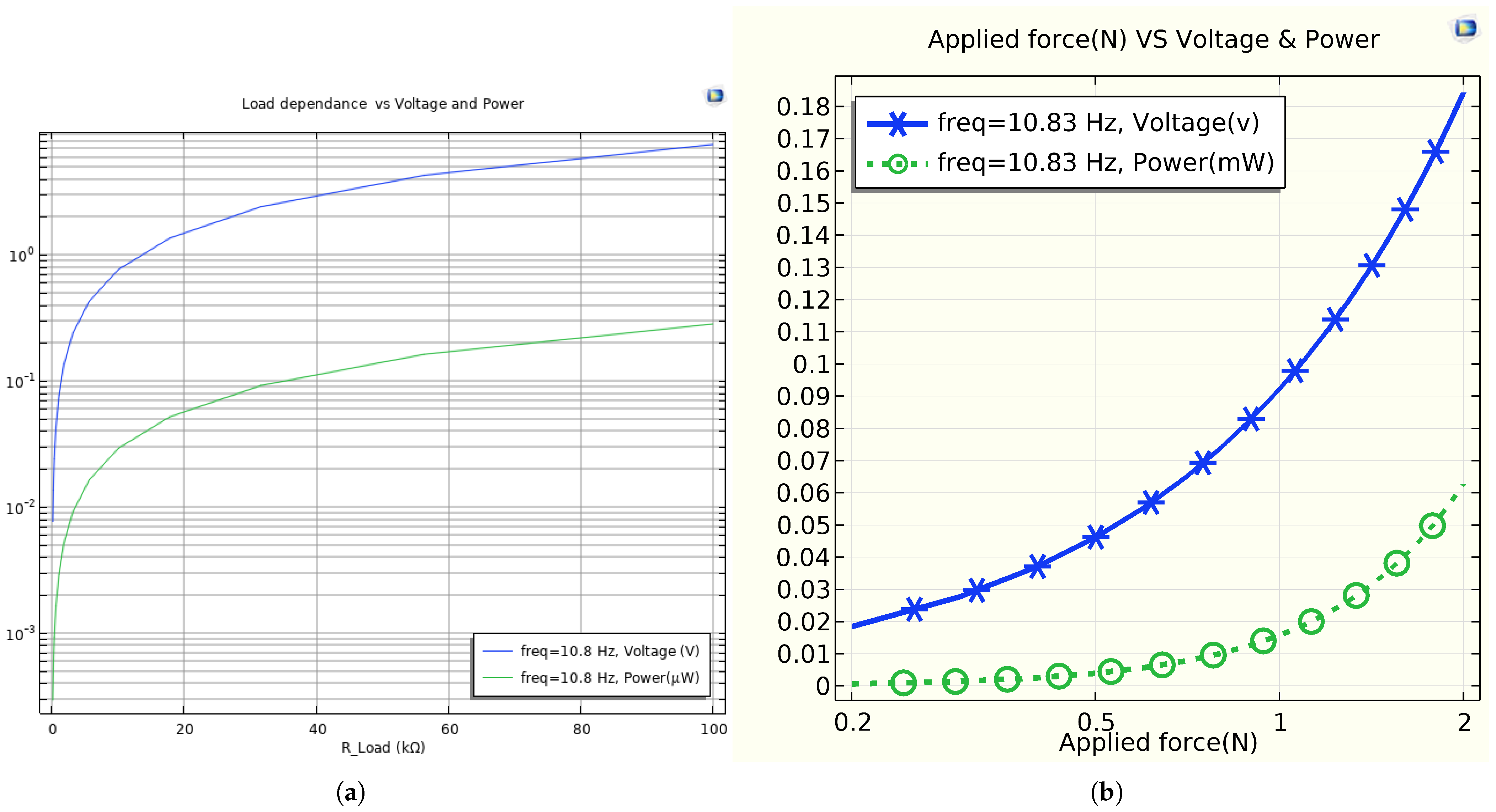
3.3. Numerical Modeling
3.4. Comparison of Analytical and Numerical Power Output

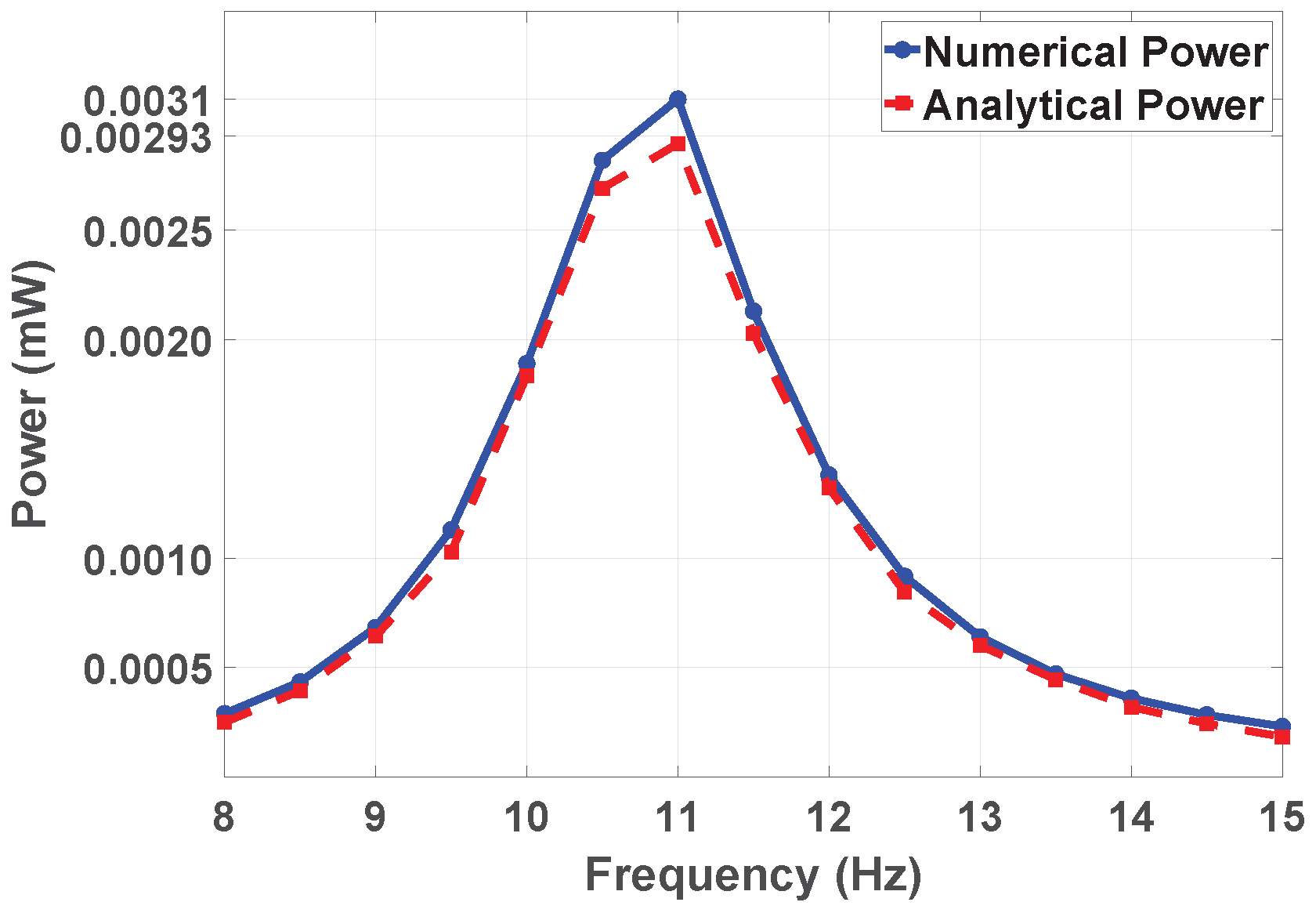
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| MFC | Macro fiber composite |
| COMSOL | Computational Solutions |
| PZT | Lead zirconate titanate |
| PEH | Piezoelectric energy harvester |
| S | Strain |
| Electric field | |
| EM | Electromagnetic |
| PZ | Piezoelectric |
| ES | Electrostatic |
| V | Voltage |
| IEEE | Institute of Electrical and Electronics Engineers |
| Stress | |
| D | Electric displacement |
| Compliance matrix at constant electric field | |
| d | Piezoelectric strain coefficient |
| Thickness of the piezoelectric layer | |
| Elastic modulus of the piezoelectric layer | |
| Piezoelectric stress constant | |
| x | Position along beam length |
| Equivalent density | |
| A | Area |
| E | Elastic modulus |
| Q | Electric charge |
| Damping effect | |
| P | Power |
| Polyvinylidene fluoride | |
| R | Resistance load |
| Angular frequency | |
| Natural frequency |
References
- Wakshume, D.G.; Płaczek, M.Ł. Optimizing Piezoelectric Energy Harvesting from Mechanical Vibration for Electrical Efficiency: A Comprehensive Review. Electronics 2024, 13, 987. [Google Scholar] [CrossRef]
- Cao, Y.; Cao, D.; He, G.; Ge, X.; Hao, Y. Vibration analysis and distributed piezoelectric energy harvester design for the L-shaped beam. Eur. J. Mech.-A/Solids 2021, 87, 104214. [Google Scholar] [CrossRef]
- TGómez Muñoz, C.Q.; Zamacola Alcalde, G.; García Márquez, F.P. Analysis and comparison of macro fiber composites and lead zirconate titanate (PZT) discs for an energy harvesting floor. Appl. Sci. 2020, 10, 5951. [Google Scholar] [CrossRef]
- Płaczek, M.Ł. Modeling and Production Process of the Energy Harvesting System Based on Mfc Piezoelectric Transducers: A Comprehensive Review. Int. J. Mod. Manuf. Technol. 2020, 13, 106–114. [Google Scholar]
- Płaczek, M.; Kokot, G. Modelling and laboratory tests of the temperature influence on the efficiency of the energy harvesting system based on MFC piezoelectric transducer. Sensors 2019, 19, 1558. [Google Scholar] [CrossRef]
- Kandukuri, T.R.; Liao, C.; Occhipinti, L.G. Modeling and Optimization of Energy Harvesters for Specific Applications Using COMSOL and Equivalent Spring Models. Sensors 2024, 24, 7509. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, Q.; He, J.; Huo, Z.; Zhou, R.; Han, X.; Jia, M.; Pan, C.; Wang, Z.L.; Zhai, J. Localizing strain via micro-cage structure for stretchable pressure sensor arrays with ultralow spatial crosstalk. Nat. Commun. 2023, 14, 1252. [Google Scholar] [CrossRef]
- Litak, G.; Kondratiuk, M.; Wolszczak, P.; Ambrożkiewicz, B.; Giri, A.M. Energy harvester based on a rotational pendulum supported with fem. Appl. Sci. 2024, 14, 3265. [Google Scholar] [CrossRef]
- Nechibvute, A.; Chawanda, A.; Luhanga, P. Piezoelectric energy harvesting devices: An alternative energy source for wireless sensors. Smart Mater. Res. 2012, 2012, 853481. [Google Scholar] [CrossRef]
- Izadgoshasb, I.; Lim, Y.Y.; Vasquez Padilla, R.; Sedighi, M.; Novak, J.P. Performance enhancement of a multiresonant piezoelectric energy harvester for low frequency vibrations. Energies 2019, 12, 2770. [Google Scholar] [CrossRef]
- Steiger, K.; Mokrỳ, P. Finite element analysis of the macro fiber composite actuator: Macroscopic elastic and piezoelectric properties and active control thereof by means of negative capacitance shunt circuit. IOP Publ. Mater. Struct. 2015, 14, 025026. [Google Scholar] [CrossRef]
- Elfrink, R.; Kamel, T.M.; Goedbloed, M.; Matova, S.; Hohlfeld, D.; van Andel, Y.; van Schaijk, R. Ultralow-power system for wireless sensing using vibration energy harvesting. IEEE Sens. J. 2011, 3, 702–771. [Google Scholar]
- Finkenzeller, K. RFID Handbook: Fundamentals and Applications in Contactless Smart Cards, RFID and Near-Field Communication; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Fang, L.H.; Rahim, R.A.; Fahmi, M.I.; Kupusamy, V. Modeling and Characterization of Piezoelectric Transducer for Sound Wave Energy Harvesting. J. Adv. Res. Fluid Mech. Therm. Sci. 2023, 103, 81–98. [Google Scholar] [CrossRef]
- Garg, O.; Sharma, S.; Preeti; Kaur, P. Piezoelectric energy harvesting: A developing scope for low-power applications. In Proceedings of the Second International Conference on Microelectronics, Ranchi, India, 13–14 May 2017; pp. 763–776. [Google Scholar]
- Erturk, A.; Inman, D.J. An experimentally validated bimorph cantilever model for piezoelectric energy harvesting from base excitations. Smart Mater. Struct. 2009, 10, 025009. [Google Scholar] [CrossRef]
- Erturk, A.; Inman, D. Piezoelectric Energy Harvesting; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Uchino, K. Piezoelectric energy harvesting systems—Essentials to successful developments. Energy Technol. 2018, 6, 829–848. [Google Scholar] [CrossRef]
- Kim, S.-B.; Park, H.; Kim, S.-H.; Wikle, H.C.; Park, J.-H.; Kim, D.-J. Comparison of MEMS PZT cantilevers based on d_{31} and d_{33} modes for vibration energy harvesting. J. Micro Electromech. Syst. 2012, 22, 81–98. [Google Scholar] [CrossRef]
- Xu, X.; Cao, D.; Yang, H.; He, M. Application of piezoelectric transducer in energy harvesting in pavement. Int. J. Pavement Res. Technol. 2018, 11, 388–395. [Google Scholar] [CrossRef]
- Wei, C.; Jing, X. A comprehensive review on vibration energy harvesting: Modelling and realization. Renew. Sustain. Energy Rev. 2017, 74, 1–18. [Google Scholar] [CrossRef]
- Lin, Y.C.; Tseng, K.S.; Ma, C.C. Investigation of resonant and energy harvesting characteristics of piezoelectric fiber composite bimorphs. Mater. Des. 2021, 197, 109267. [Google Scholar] [CrossRef]
- Kumar, A.; Sharma, A.; Kumar, R.; Vaish, R.; Chauhan, V.S. Finite element analysis of vibration energy harvesting using lead-free piezoelectric materials: A comparative study. J. Asian Ceram. Soc. 2014, 2, 138–143. [Google Scholar] [CrossRef][Green Version]
- Placzek, M.; Brzezny, M. Estimation of the power of a MFC type piezoelectric used for electric energy recovery from vibrations. IOP Conf. Ser. Mater. Sci. Eng. 2018, 400, 032007. [Google Scholar] [CrossRef]
- Hajheidari, P. Performance Enhancement of Cantilever Beam Piezoelectric Energy Harvesters. Ph.D. Thesis, Concordia University, Montreal, QC, Canada, 2019. [Google Scholar]
- Paknejad, A.; Rahimi, G.; Farrokhabadi, A.; Khatibi, M.M. Analytical solution of piezoelectric energy harvester patch for various thin multilayer composite beams. Compos. Struct. 2016, 154, 694–706. [Google Scholar] [CrossRef]
- Nbokassem, M.W. Modelling and Analysis of Piezoelectric Energy Harvester. Ph.D. Thesis, Instituto Superior de Engenharia de Lisboa, Lisboa, Portugal, 2019. [Google Scholar]
- Joshi, S.P. Non-linear constitutive relations for piezoceramic materials. Smart Mater. Struct. 1992, 1, 80. [Google Scholar] [CrossRef]
- Deraemaeker, A.; Nasser, H. Numerical evaluation of the equivalent properties of Macro Fiber Composite (MFC) transducers using periodic homogenization. Int. J. Solids Struct. 2010, 47, 3272–3285. [Google Scholar] [CrossRef]
- Degefa, T.G.; Płaczek, M.Ł.; Kokot, G. The study of the influence of temperature and low frequency on the performance of the laminated MFC piezoelectric energy harvester. Appl. Sci. 2022, 12, 12135. [Google Scholar] [CrossRef]
- Raja, V.; Umapathy, M.; Uma, G.; Praveen Kumar, B.; Premkumar, S. Design, analysis and experimental investigation of a rotational piezoelectric energy harvester with storage system. J. Mech. Sci. Technol. 2020, 14, 4475–4487. [Google Scholar] [CrossRef]
- Homayouni-Amlashi, A.; Mohand-Ousaid, A.; Rakotondrabe, M. Analytical modelling and optimization of a piezoelectric cantilever energy harvester with in-span attachment. Micromachines 2020, 10, 591. [Google Scholar] [CrossRef]
- Jemai, A.; Najar, F.; Chafra, M.; Ounaies, Z. Mathematical modeling of an active-fiber composite energy harvester with interdigitated electrodes. Shock Vib. 2014, 2014, 971597. [Google Scholar] [CrossRef]
- Raju, S.S.; Umapathy, M.; Uma, G. Design and analysis of high output piezoelectric energy harvester using non-uniform beam. Energy 2020, 27, 218–227. [Google Scholar] [CrossRef]
- Guo, L.; Wang, H. Multi-physics modeling of piezoelectric energy harvesters from vibrations for improved cantilever designs. Energy 2023, 263, 125870. [Google Scholar] [CrossRef]
- Rajora, A.; Dwivedi, A.; Vyas, A.; Gupta, S.; Tyagi, A. Energy harvesting estimation from the vibration of a simply supported beam. Int. J. Acoust. Vib. 2017, 22, 186–193. [Google Scholar] [CrossRef]
- Plagianakos, T.S.; Margelis, N.; Leventakis, N.; Bolanakis, G.; Vartholomeos, P.; Papadopoulos, E.G. Finite element-based assessment of energy harvesting in composite beams with piezoelectric transducers. J. Mater. Des. Appl. 2022, 236, 473–488. [Google Scholar] [CrossRef]
- Tommasino, D.; Moro, F.; Zumalde, E.; Kunzmann, J.; Doria, A. An Analytical–Numerical Method for Simulating the Performance of Piezoelectric Harvesters Mounted on Wing Slats. Actuators 2023, 12, 29. [Google Scholar] [CrossRef]
- Jia, Y.; Wei, X.; Xu, L.; Wang, C.; Lian, P.; Xue, S.; Al-Saadi, A.; Shi, Y. Multiphysics vibration FE model of piezoelectric macro fibre composite on carbon fibre composite structure. Compos. Part B Eng. 2019, 16, 376–385. [Google Scholar] [CrossRef]
- Kuang, Y.; Zhu, M. Evaluation and validation of equivalent properties of macro fibre composites for piezoelectric transducer modelling. Compos. Part B Eng. 2019, 158, 189–197. [Google Scholar] [CrossRef]
- Kurt, P.; Narayan, B.; Roscow, J.I.; Orhan, S. Modeling and simulation of a gauge shaped beam coupled with macro fiber composite for energy harvesting application. IOP Conf. Ser. Mater. Sci. Eng. 2020, 856, 012010. [Google Scholar] [CrossRef]
- Upadrashta, D.; Yang, Y. Finite element modeling of nonlinear piezoelectric energy harvesters with magnetic interaction. Smart Mater. Struct. 2015, 24, 045042. [Google Scholar] [CrossRef]
- Ibrahim, D.S.; Beibei, S.; Fatai, S.; Oluseyi, O.A.; Sharif, U. Numerical and experimental study of a gauge-shaped beam for improved performance of a piezoelectric energy harvester. Microsyst. Technol. 2021, 27, 4253–4268. [Google Scholar] [CrossRef]
- Kurt, P.; Narayan, B.; Roscow, J.I.; Orhan, S. Improving piezoelectric energy harvesting performance through mechanical stiffness matching. Mech. Adv. Mater. Struct. 2024, 31, 10721–10734. [Google Scholar] [CrossRef]

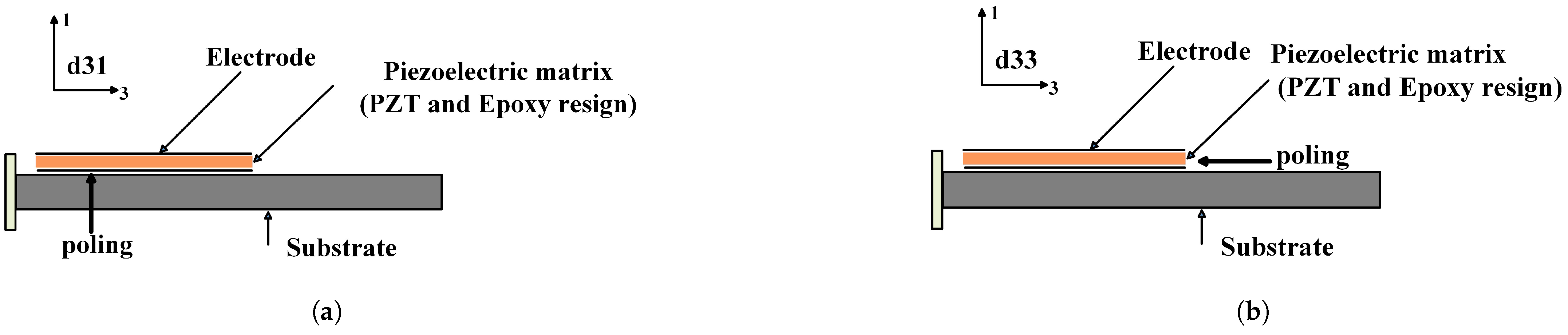
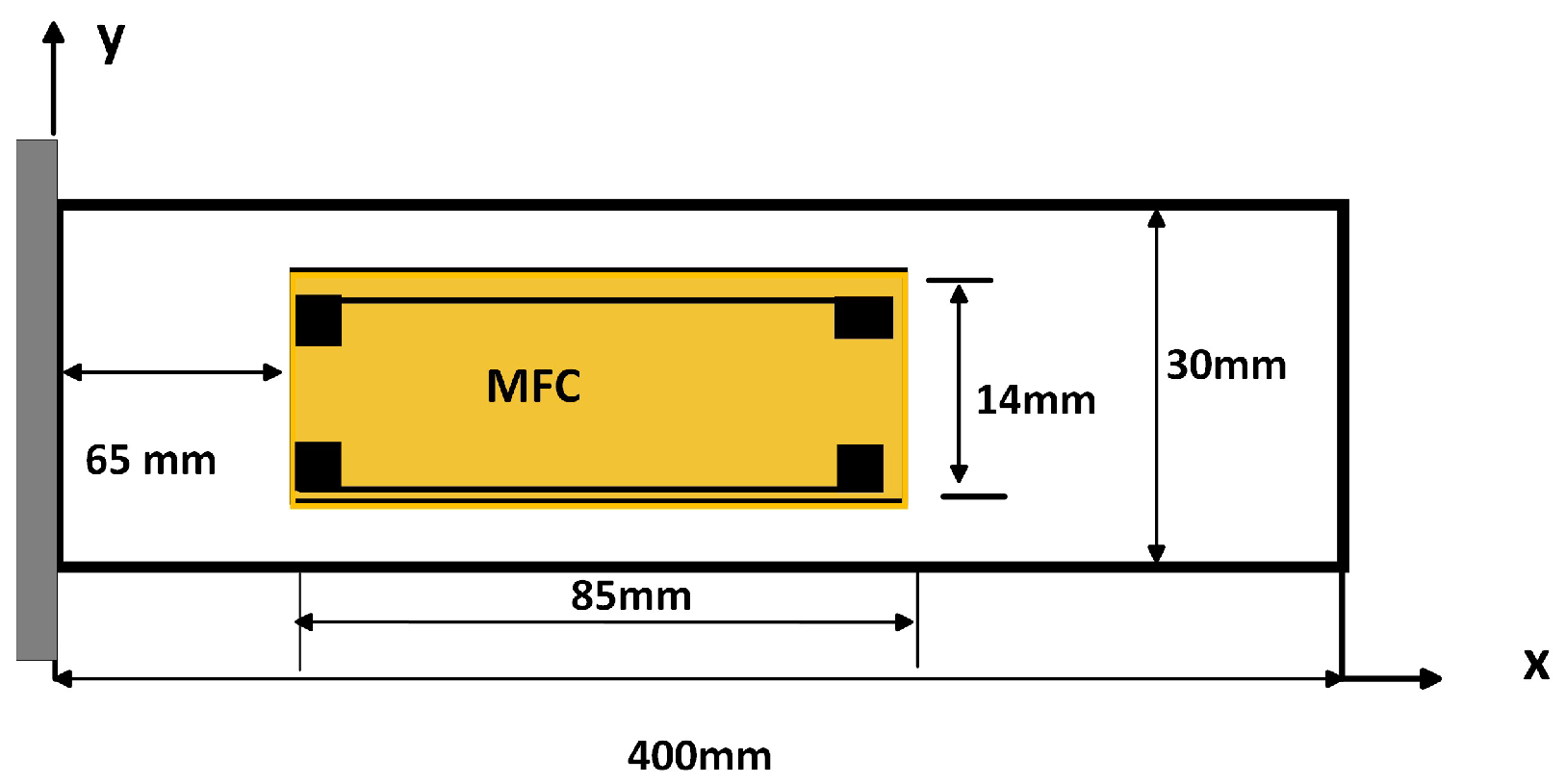
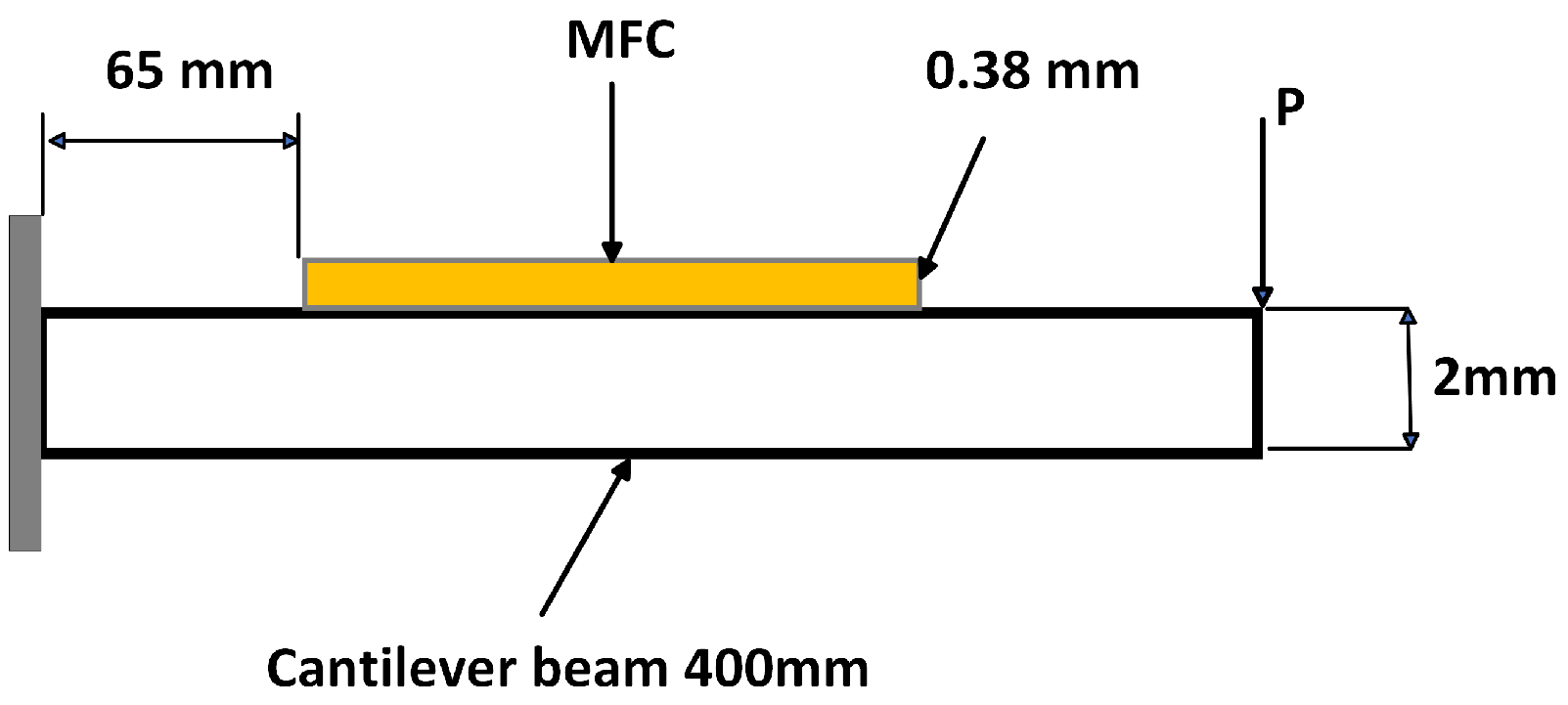



| Type of Material | Item | Value | Dimension (Unit) |
|---|---|---|---|
| Aluminum beam (substrate) | Length | 400 | mm |
| Width (W) | 30 | mm | |
| Thickness | 2 | mm | |
| Centroid of the aluminum | 1 | mm | |
| Area | 607 | mm2 | |
| Centroid () | 1 | mm | |
| Poisson ratio | 0.345 | – | |
| Elasticity | 70 | GPa | |
| m4 | |||
| M8514-P2 | Length | 85 | mm |
| Width | 14 | mm | |
| Thickness | 0.38 | mm | |
| mm2 | |||
| Centroid of the MFC | 2.19 | mm | |
| Total volume | 452.2 | mm3 | |
| mm2 | |||
| Centroid MFC () | 2.19 | mm | |
| 5160 | kg/m3 | ||
| Young’s modulus of the M8514-P2 | 30 × | Pa | |
| Elastic compliance constant | |||
| Piezoelectric constant coefficient | |||
| Capacitance of MFC () | F | ||
| m4 | |||
| Relative permittivity | 1700 | — | |
| Copper electrode | Length | 101 | mm |
| Width | 14 | mm | |
| Thickness | 0.01 | mm | |
| PZT-5A | Length | 0.3 | mm |
| Width | 14 | mm | |
| Thickness | 0.38 | mm | |
| Number of cells | 170 | – | |
| Volume of one cell | 1.596 | ||
| 7800 | |||
| Volume fraction of PZT | 0.6 | – | |
| Total volume of PZT-5A | 271.32 | mm3 | |
| Piezoelectric constant coefficient | m/v | ||
| Epoxy resin | Length | 0.2 | mm |
| Width | 14 | mm | |
| Thickness | 0.38 | mm | |
| Number of cells | 170 | – | |
| Volume of one cell | 1.064 | mm3 | |
| Volume fraction | 0.4 | – | |
| Total volume of epoxy resin | 180.88 | mm3 |
| Mode Shape | Roots of the Frequency Equation | Natural Frequency (Hz) | Angular Frequency (rad/s) |
|---|---|---|---|
| 1 | 1.875 | 10.693 | 67.578 |
| 2 | 4.694 | 63.82 | 397.96 |
| 3 | 7.855 | 153.41 | 961.75 |
| 4 | 10.996 | 178.96 | 1124.14 |
| 5 | 14.137 | 269.55 | 1693.63 |
| 6 | 17.278 | 355.07 | 12,218.72 |
| Parameter | Formula | Result | Unit |
|---|---|---|---|
| Centroid of Aluminum Beam () | 1 | mm | |
| Centroid of Al and MFC | mm | ||
| Composite Moment of Inertia | Nm2 | ||
| Total Mass per Unit Length of Composite Beam | 0.1655 | kg/m | |
| Total Second Moment | mm | ||
| Resonance Frequency | 10.693 | Hz | |
| Maximum Amplitude (X) | 3.3 | m | |
| Strain | — | — | – |
| Maximum Stress () | 1.33 | M Pa | |
| Electric Displacement | |||
| Voltage Output (V) | 0.175 | V | |
| Power Output (P) | 0.0029 | mW |
| Mode Shape | Natural Frequency (Hz) | Angular Frequency (rad/s) |
|---|---|---|
| 1 | 10.781 | 67.738 |
| 2 | 63.949 | 401.81 |
| 3 | 153.94 | 967.26 |
| 4 | 179.7 | 1129.1 |
| 5 | 271.15 | 1703.7 |
| 6 | 355.08 | 2231.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wakshume, D.G.; Płaczek, M.Ł. Mathematical Modeling and Finite Element Simulation of the M8514-P2 Composite Piezoelectric Transducer for Energy Harvesting. Sensors 2025, 25, 3071. https://doi.org/10.3390/s25103071
Wakshume DG, Płaczek MŁ. Mathematical Modeling and Finite Element Simulation of the M8514-P2 Composite Piezoelectric Transducer for Energy Harvesting. Sensors. 2025; 25(10):3071. https://doi.org/10.3390/s25103071
Chicago/Turabian StyleWakshume, Demeke Girma, and Marek Łukasz Płaczek. 2025. "Mathematical Modeling and Finite Element Simulation of the M8514-P2 Composite Piezoelectric Transducer for Energy Harvesting" Sensors 25, no. 10: 3071. https://doi.org/10.3390/s25103071
APA StyleWakshume, D. G., & Płaczek, M. Ł. (2025). Mathematical Modeling and Finite Element Simulation of the M8514-P2 Composite Piezoelectric Transducer for Energy Harvesting. Sensors, 25(10), 3071. https://doi.org/10.3390/s25103071









