Finite-Time Disturbance Observer-Based Adaptive Course Control for Surface Ships
Abstract
1. Introduction
- (1)
- Through the construction of auxiliary dynamics, a finite-time disturbance observer is developed to appraise time-varying external disturbances, ensuring that the disturbance estimation error converges in finite time. Moreover, by devising a self-tuning update law to estimate the maximum rate of the external disturbances’ derivative, the conservatism in the design of the disturbance observer is reduced.
- (2)
- By the combination of the finite-time disturbance observer and auxiliary dynamics, an adaptive heading control law based on the hyperbolic tangent function is developed to achieve accurate tracking of the ship’s heading. Furthermore, leveraging LaSalle’s Invariance Principle, a simple and effective method for system stability analysis is designed. Unlike traditional Lyapunov function-based proof methods, this stability condition is very lenient and simple to meet. Even if the scalar function used to demonstrate system stability is non-positive definite with a semi-negative definite derivative, it still ensures the long-term stability of the system.
2. Prior Knowledge and Problem Statement
2.1. Prior Knowledge
2.2. Problem Statement
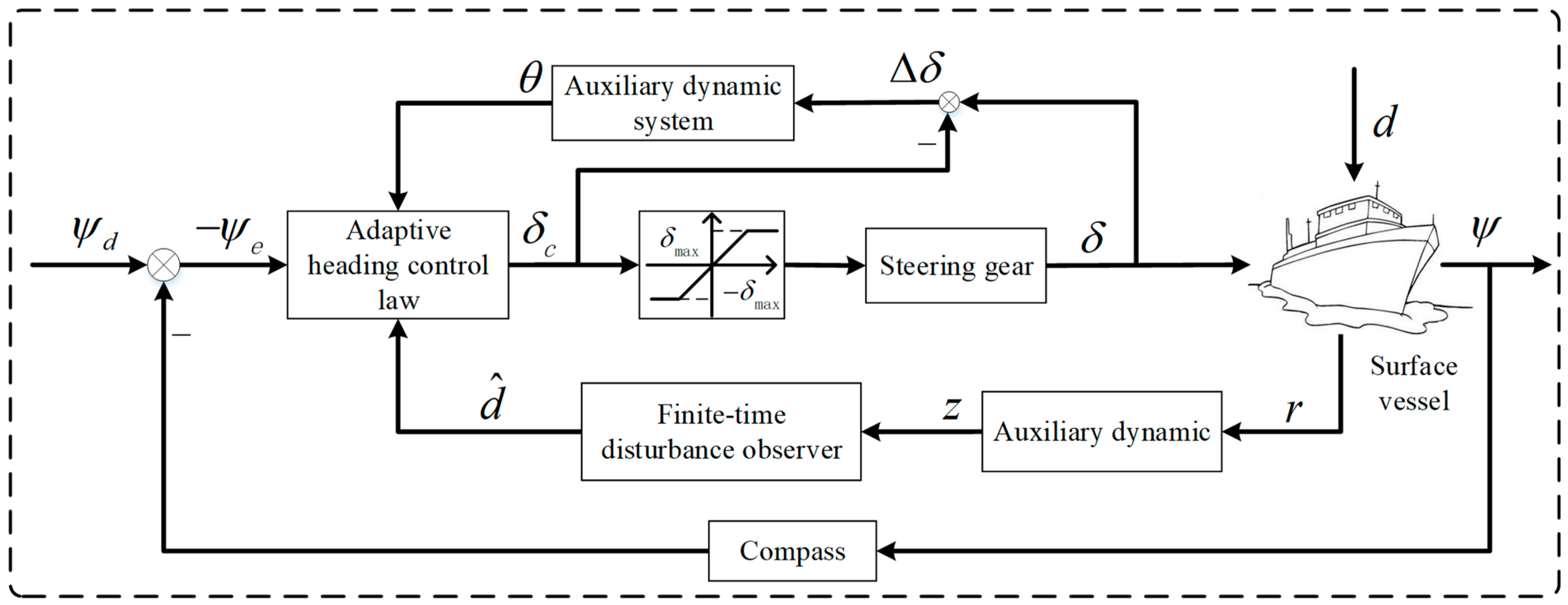
3. Adaptive Course Control Law Design
3.1. Finite-Time Disturbance Observer
3.2. Adaptive Course Control System
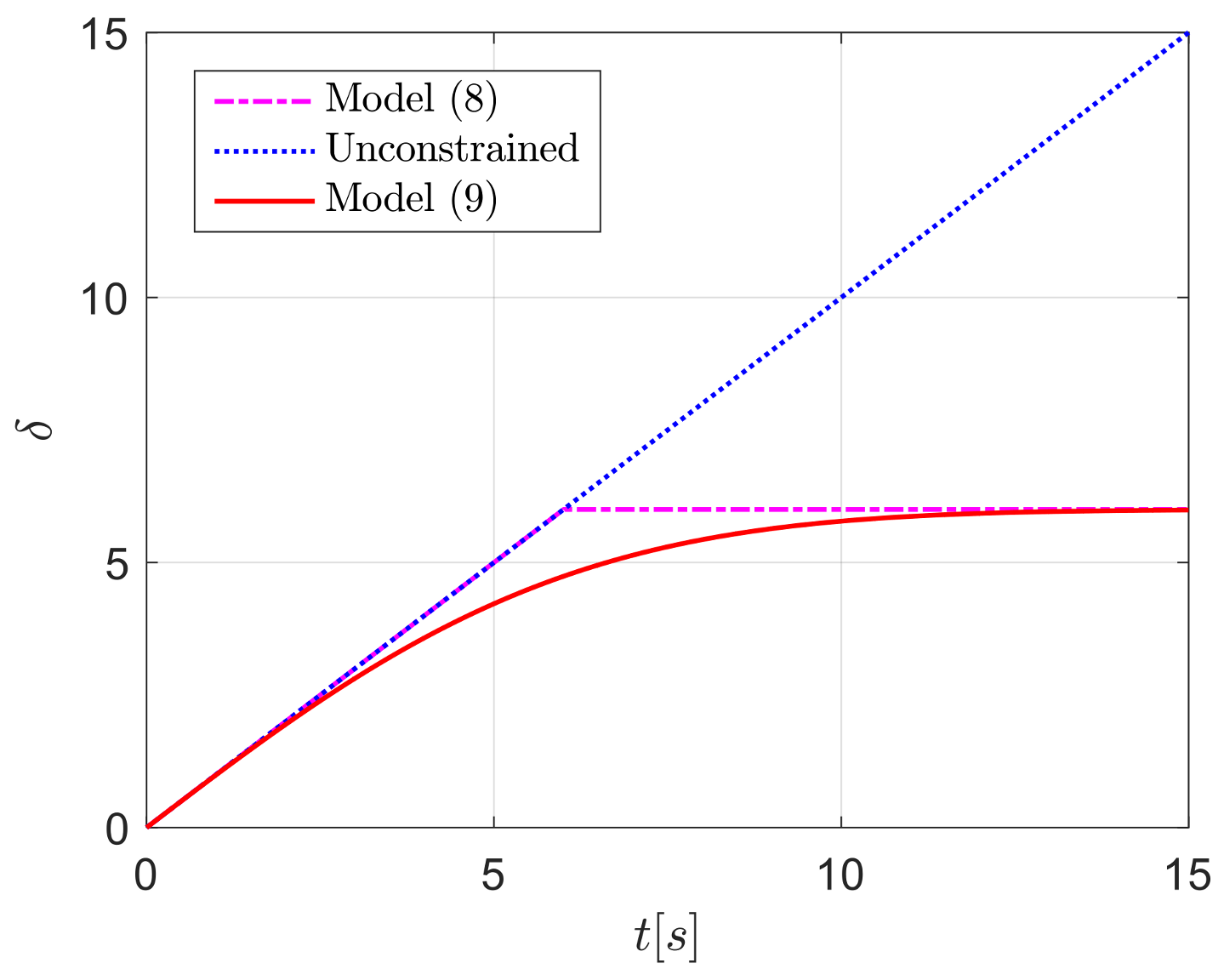
3.2.1. Auxiliary Dynamic System
3.2.2. Adaptive Course Control Law Based on Hyperbolic Tangent
- (1)
- The closed-loop system maintains stability;
- (2)
- Every signal within the closed-loop system remains ultimately consistently bounded;
- (3)
- The heading tracking error can reach a bounded closed set within a finite time.
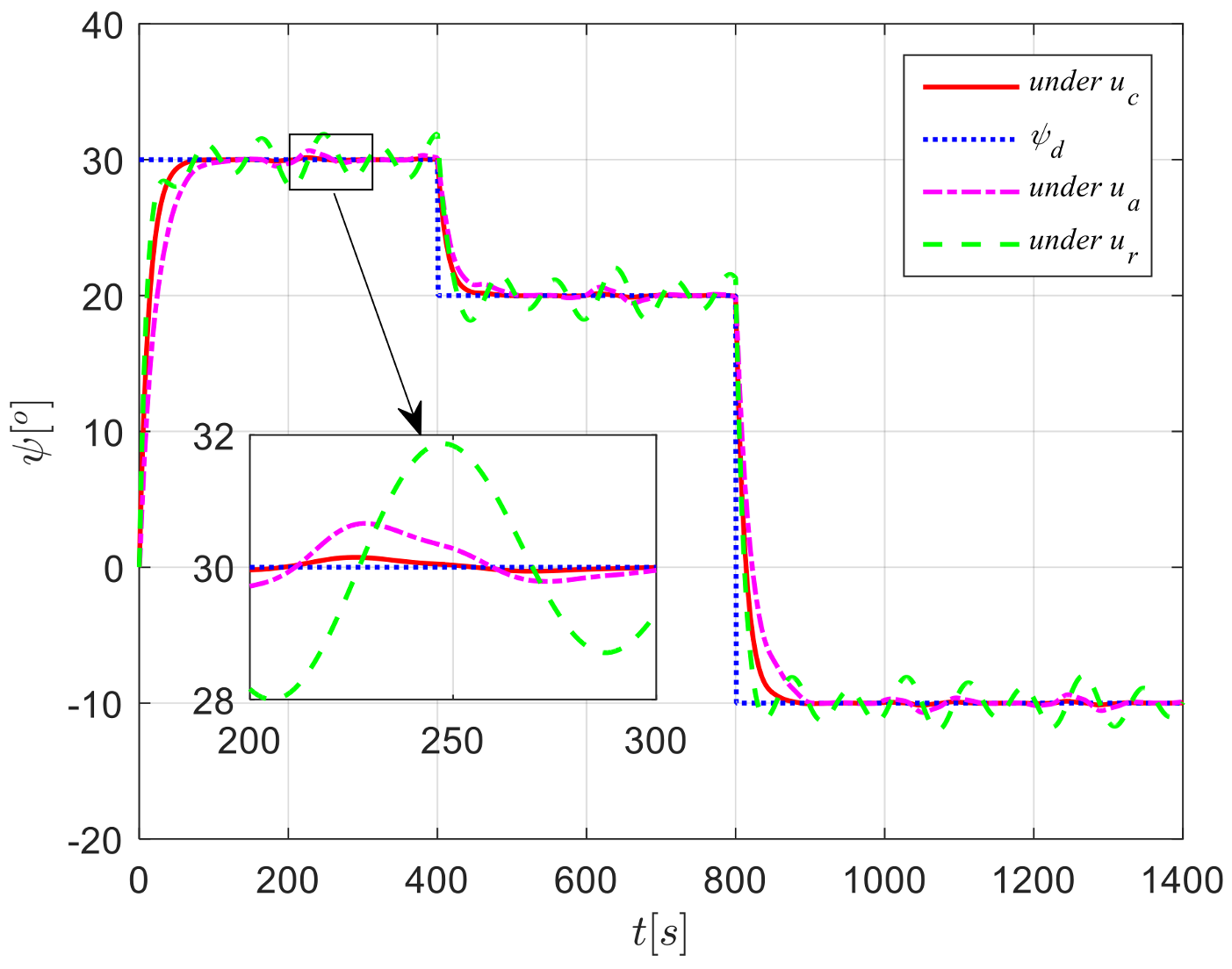
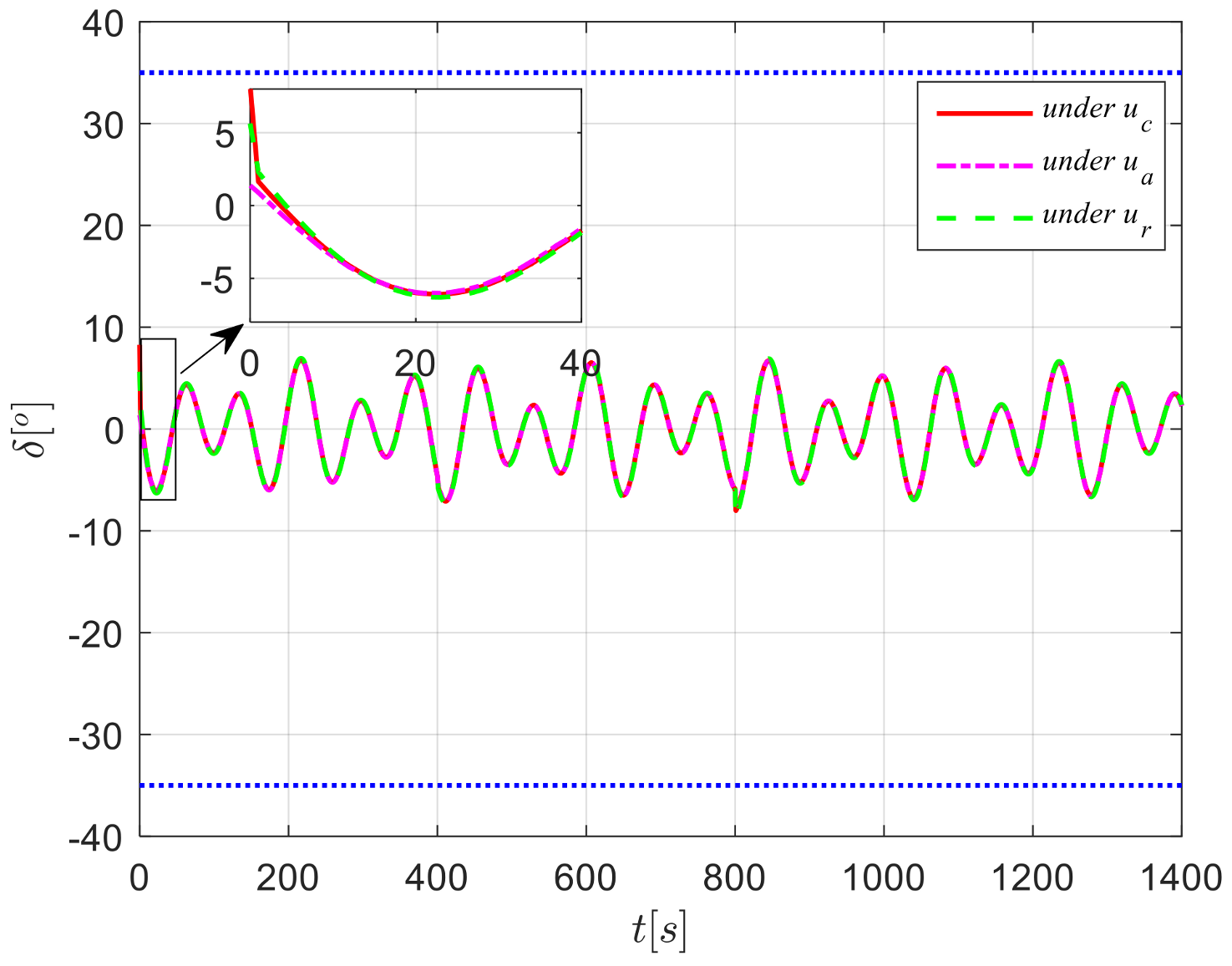
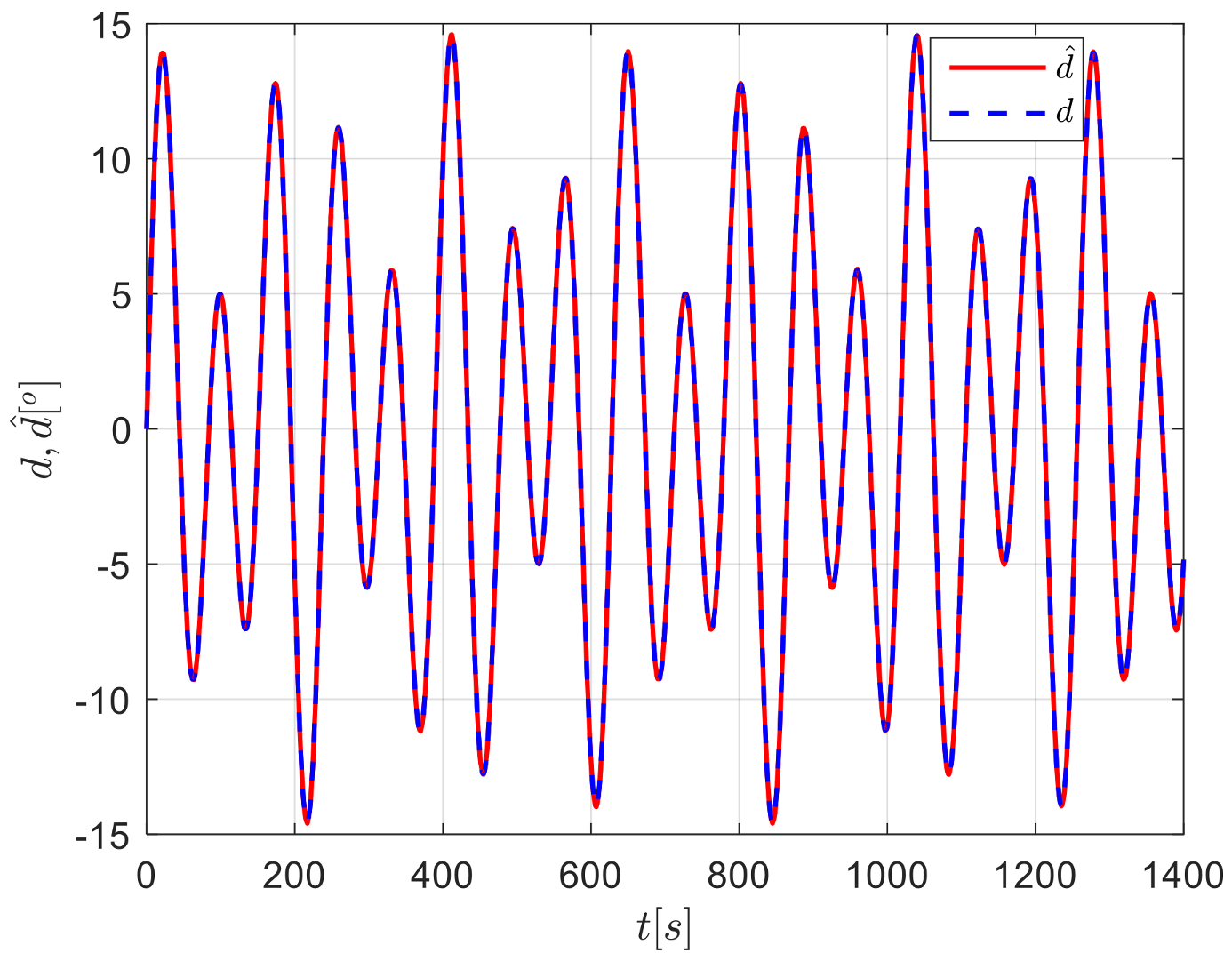
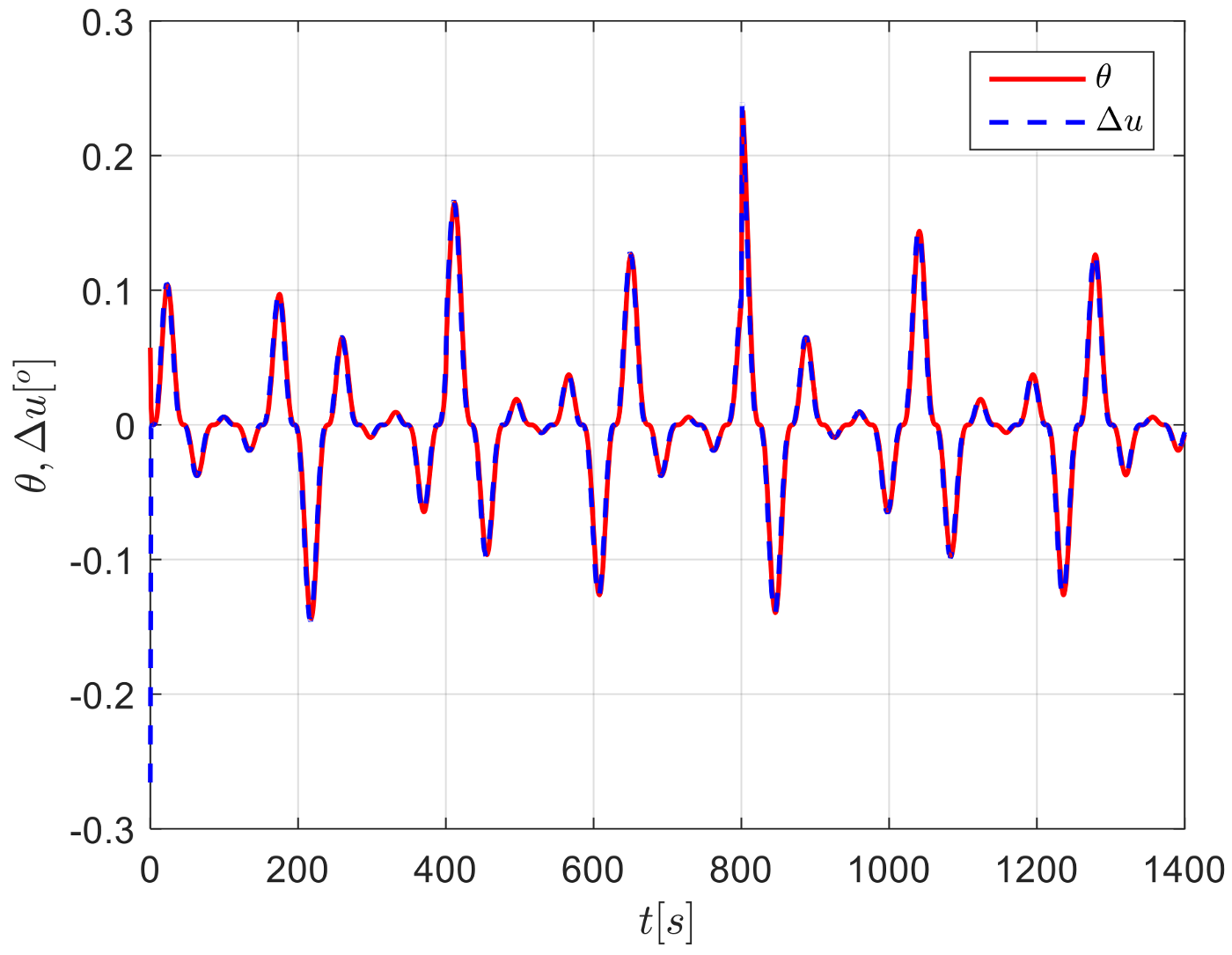
4. Simulation Analysis
4.1. Case 1
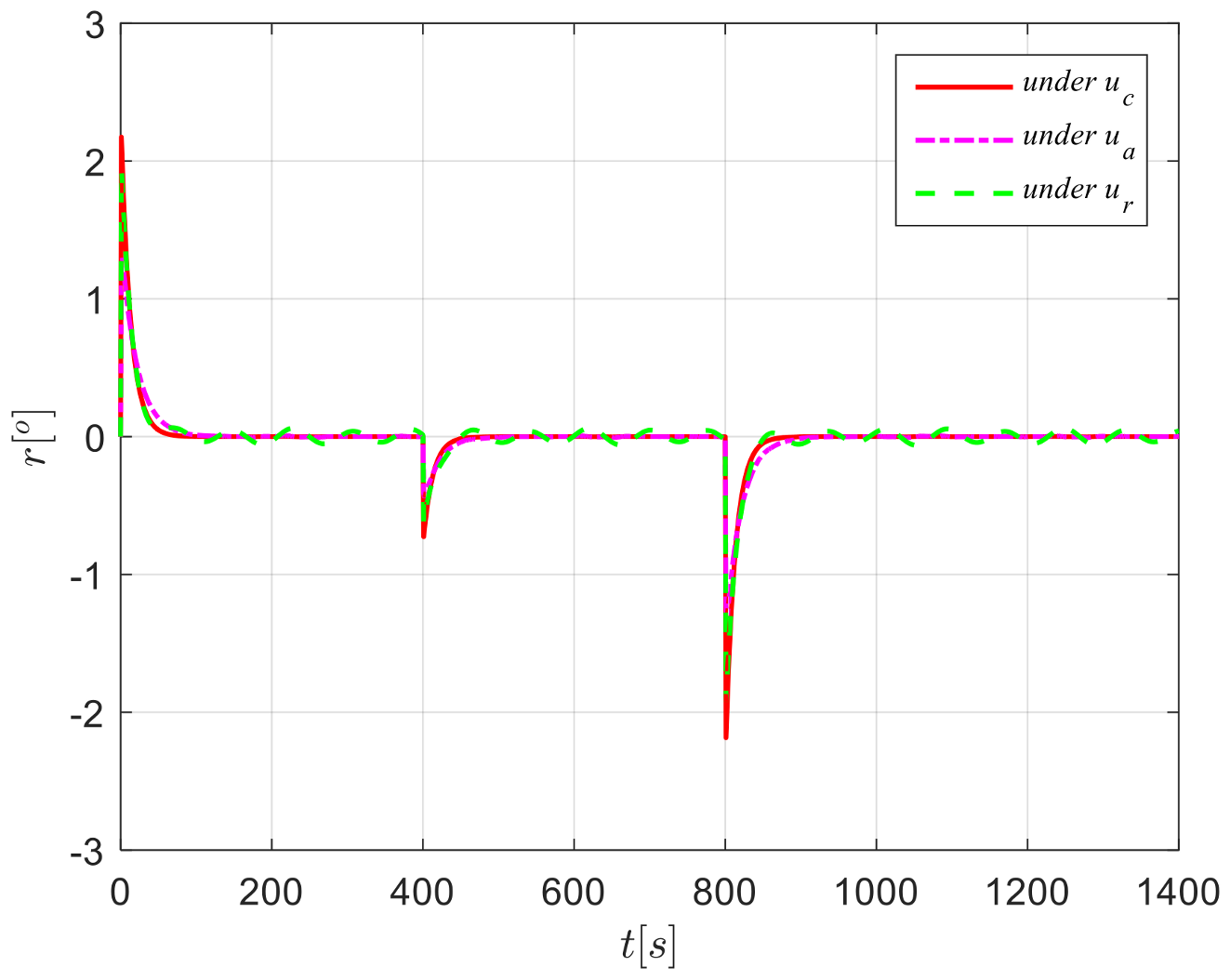
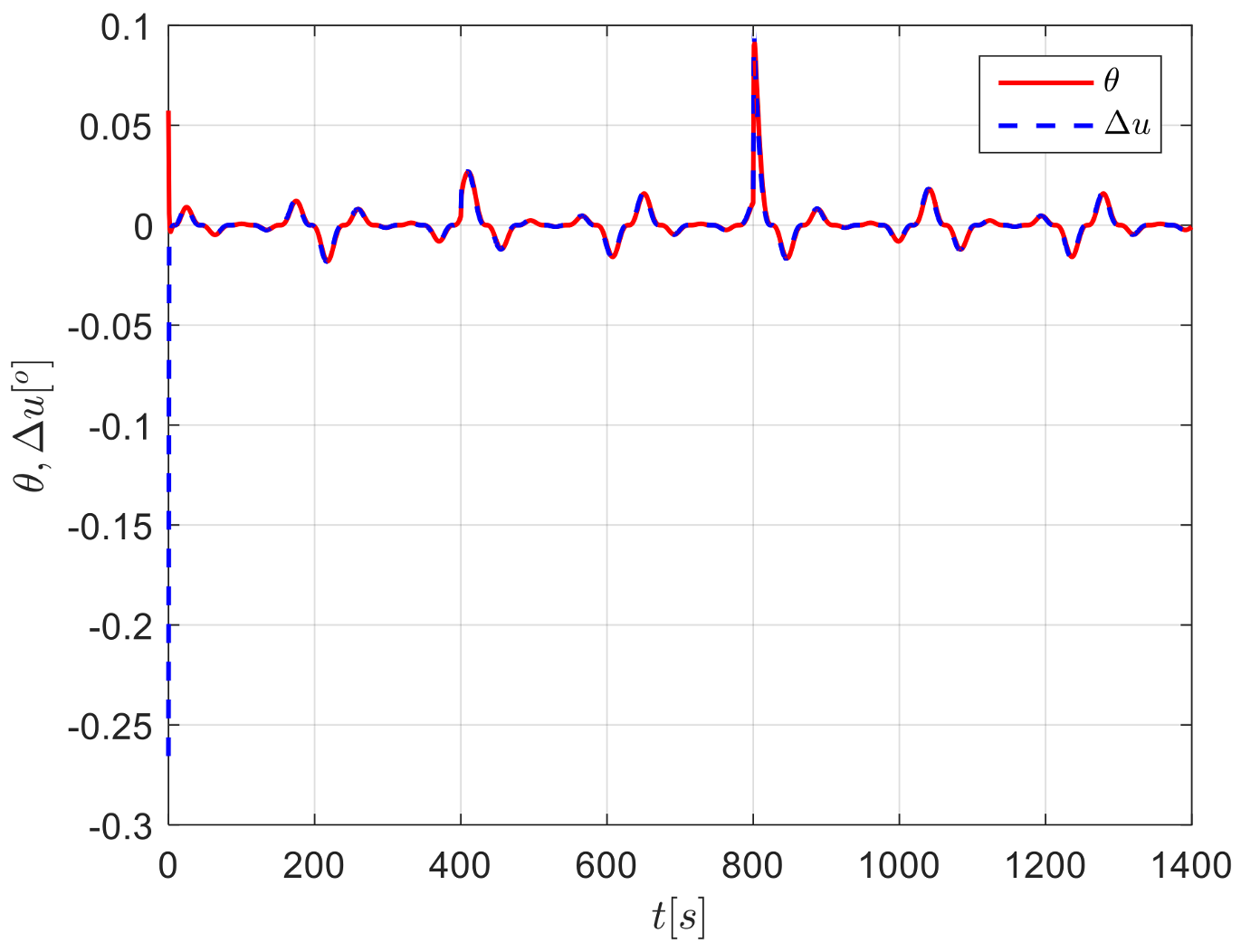
4.2. Case 2
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Symbol | Description | Symbol | Description |
|---|---|---|---|
| real number | sine function | ||
| n-dimensional real vector | minimum eigenvalues of a matrix | ||
| sign function | maximum eigenvalues of a matrix | ||
| absolute value of a constant or variable | |||
| second norm of a matrix | logarithmic function | ||
| hyperbolic tangent function | Gaussian error function | ||
| hyperbolic cosine function |
References
- Yan, Z.; Zhang, X.; Zhu, H.; Li, Z. Course-keeping control for ships with nonlinear feedback and zero-order holder component. Ocean Eng. 2020, 209, 107461. [Google Scholar] [CrossRef]
- Gong, C.L.; Su, Y.X.; Zhu, Q.X.; Zhang, D.H. Finite-time dynamic positioning control design for surface vessels with external disturbances, input saturation and error constraints. Ocean Eng. 2023, 276, 114259. [Google Scholar] [CrossRef]
- Lei, Z.; Guo, C. Disturbance rejection control solution for ship steering system with uncertain time delay. Ocean Eng. 2015, 95, 78–83. [Google Scholar] [CrossRef]
- Chen, C.Y.; Lataire, E.; Delefortrie, G. Experimental study of adaptive course controllers with nonlinear modulators for surface ships in shallow water. ISA Trans. 2023, 134, 417–430. [Google Scholar] [CrossRef] [PubMed]
- Yuan, J.; Liu, H.; Wan, J.; Li, H.; Zhang, W. Combined depth and heading control and experiment of ROV under the influence of residual buoyancy, current disturbance, and control dead zone. J. Field Robot. 2023, 40, 330–345. [Google Scholar] [CrossRef]
- Su, Y.X.; Gong, C.L.; Zhang, D.H.; Hu, X. Simple Dynamic Positioning Control Design for Surface Vessels with Input Saturation and External Disturbances. IEEE Trans. Circuits Syst. II Express Briefs 2023, 70, 1530–1534. [Google Scholar] [CrossRef]
- Nguyen, Q.D.; Pham, Q.D.; Thanh, N.T. An Optimal Homogenous Stability-Based Disturbance Observer and Sliding Mode Control for Secure Communication System. IEEE Access 2023, 11, 27317–27329. [Google Scholar] [CrossRef]
- Fu, H.; Yao, W.; Cajo, R.; Zhao, S. Trajectory Tracking Predictive Control for Unmanned Surface Vehicles with Improved Nonlinear Disturbance Observer. J. Mar. Sci. Eng. 2023, 11, 1874. [Google Scholar] [CrossRef]
- Dong, Z.; Li, J.; Liu, W.; Zhang, H.; Qi, S.; Zhang, Z. Adaptive Heading Control of Underactuated Unmanned Surface Vehicle Based on Improved Backpropagation Neural Network. Pol. Marit. Res. 2023, 30, 54–64. [Google Scholar] [CrossRef]
- Wang, Q.L.; Sun, C.Y.; Chen, Y.Y. Adaptive neural network control for course-keeping of ships with input constraints. Trans. Inst. Meas. Control 2019, 41, 1010–1018. [Google Scholar] [CrossRef]
- Shi, X.; Chen, P.; Chen, L. An Integrated Method for Ship Heading Control Using Motion Model Prediction and Fractional Order Proportion Integration Differentiation Controller. J. Mar. Sci. Eng. 2023, 11, 2294. [Google Scholar] [CrossRef]
- Nie, J.; Lin, X.G. FAILOS guidance law based adaptive fuzzy infinite-time path following control for underactuated MSV. Ocean Eng. 2020, 195, 106726. [Google Scholar] [CrossRef]
- Tang, F.; Wang, H.; Zhang, L.; Xu, N.; Ahmad, A.M. Adaptive optimized consensus control for a class of nonlinear multi-agent systems with asymmetric input saturation constraints and hybrid faults. Commun. Nonlinear Sci. Numer. Simul. 2023, 126, 107446. [Google Scholar] [CrossRef]
- Bai, Y.M.; Cao, Y.C.; Li, T.S. Optimized backstepping design for ship course following control based on actor-critic architecture with input saturation. IEEE Access 2019, 7, 73516–73528. [Google Scholar] [CrossRef]
- Hu, X.; Wei, X.; Han, J. Adaptive disturbance rejection for course tracking of marine vessels under actuator constraint. ISA Trans. 2020, 100, 82–91. [Google Scholar] [CrossRef] [PubMed]
- Zhang, D.F.; Lee, T.C.; Sun, X.M.; Wu, Y.H. Practical regulation of nonholonomic systems using virtual trajectories and LaSalle Invariance Principle. IEEE Trans. Syst. Man Cybern. Syst. 2020, 50, 1833–1839. [Google Scholar] [CrossRef]
- Gong, C.L.; Su, Y.X.; Zhang, D.H. Variable gain prescribed performance control for dynamic positioning of ships with positioning error constraints. J. Mar. Sci. Eng. 2022, 10, 74. [Google Scholar] [CrossRef]
- Moulay, E.; Perruquetti, W. Finite time stability and stabilization of a class of continuous systems. J. Math. Anal. Appl. 2006, 323, 1430–1443. [Google Scholar] [CrossRef]
- Huo, X.; Hu, Q.; Xiao, B. Finite-time fault tolerant attitude stabilization control for rigid spacecraft. ISA Trans. 2014, 53, 241–250. [Google Scholar] [CrossRef] [PubMed]
- Hu, Q.L.; Li, B.; Qi, J.T. Disturbance observer based finite-time attitude control for rigid spacecraft under input saturation. Aerosp. Sci. Technol. 2014, 39, 13–21. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, M.; Gong, C. Finite-Time Disturbance Observer-Based Adaptive Course Control for Surface Ships. Sensors 2024, 24, 4843. https://doi.org/10.3390/s24154843
Xu M, Gong C. Finite-Time Disturbance Observer-Based Adaptive Course Control for Surface Ships. Sensors. 2024; 24(15):4843. https://doi.org/10.3390/s24154843
Chicago/Turabian StyleXu, Ming, and Chenglong Gong. 2024. "Finite-Time Disturbance Observer-Based Adaptive Course Control for Surface Ships" Sensors 24, no. 15: 4843. https://doi.org/10.3390/s24154843
APA StyleXu, M., & Gong, C. (2024). Finite-Time Disturbance Observer-Based Adaptive Course Control for Surface Ships. Sensors, 24(15), 4843. https://doi.org/10.3390/s24154843





