Safe Robust Adaptive Motion Control for Underactuated Marine Robots
Abstract
1. Introduction
- Trajectory tracking of an underactuated marine vehicle is addressed amidst significant sources of uncertainties, encompassing time-varying external disturbances at both the Kinematics and Dynamics levels, unmodelled dynamics, time-varying water current speeds, lateral velocities, and estimator errors;
- Robust funnel control is proposed for trajectory tracking with formal stability guarantees, ensuring finite-time stability and safety;
- Control design eliminates the necessity for exact knowledge of the robot dynamics, enhancing design simplicity and feasibility through the utilization of straightforward feedback sensors. For instance, there is no requirement for lateral sensors or other complex sensors such as acceleration feedback;
- Leveraging developed mathematics and formulations, along with a TSMC and novel funnel boundary shape, designers can tailor to diverse requirements and uphold various safety measures across different initial conditions;
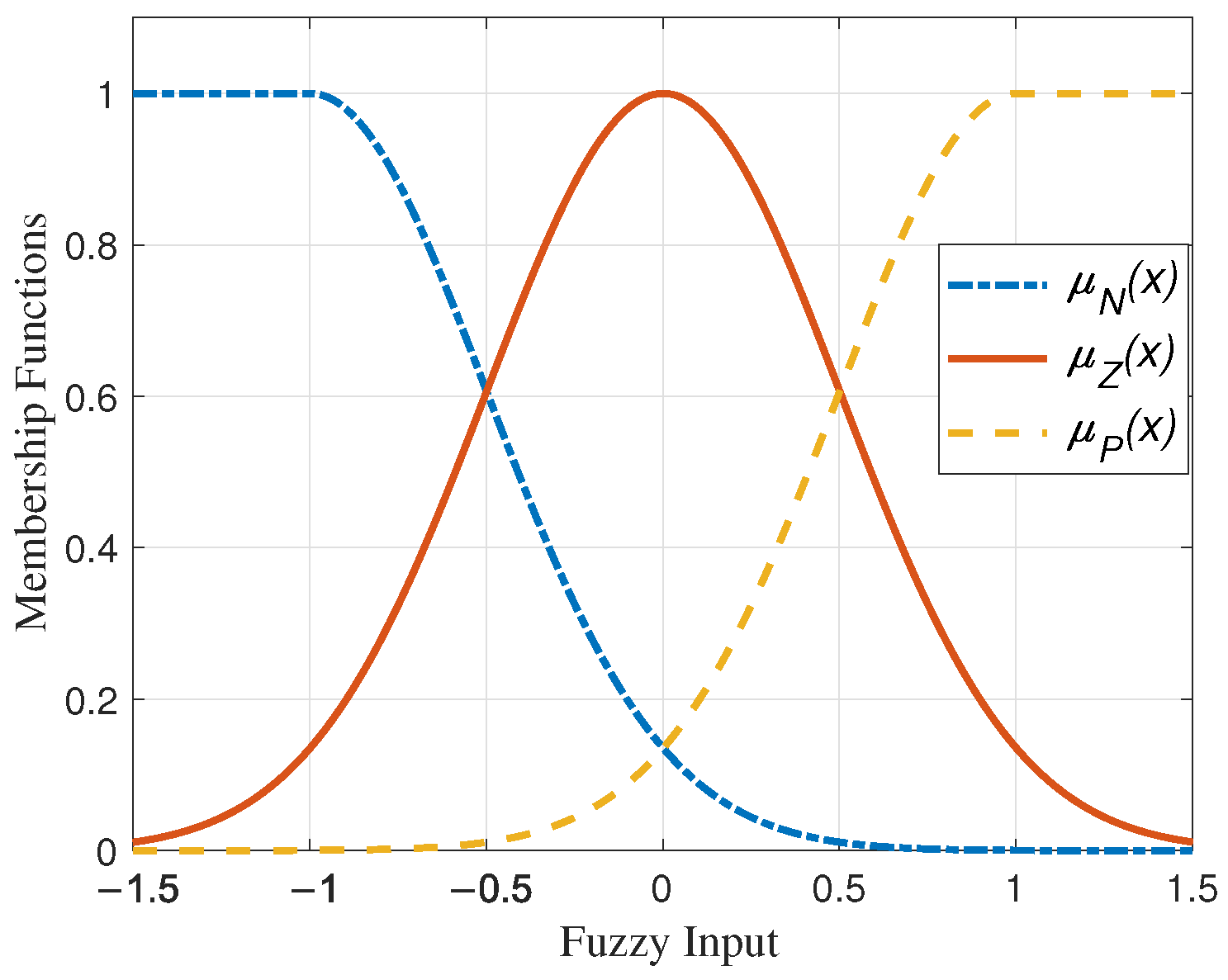
- Implementation of a fuzzy estimator facilitates the estimation of unmodelled dynamics in the robot, employing a simple design and straightforward inputs;
- Compensation for the imprecision of fuzzy systems is achieved through the integration of a robust controller.
2. Robot Kinematics and Dynamics
3. Proposed Controller
4. Analyzing Control System Stability
4.1. Overall Stability of the System
4.2. Internal Behavior of the System
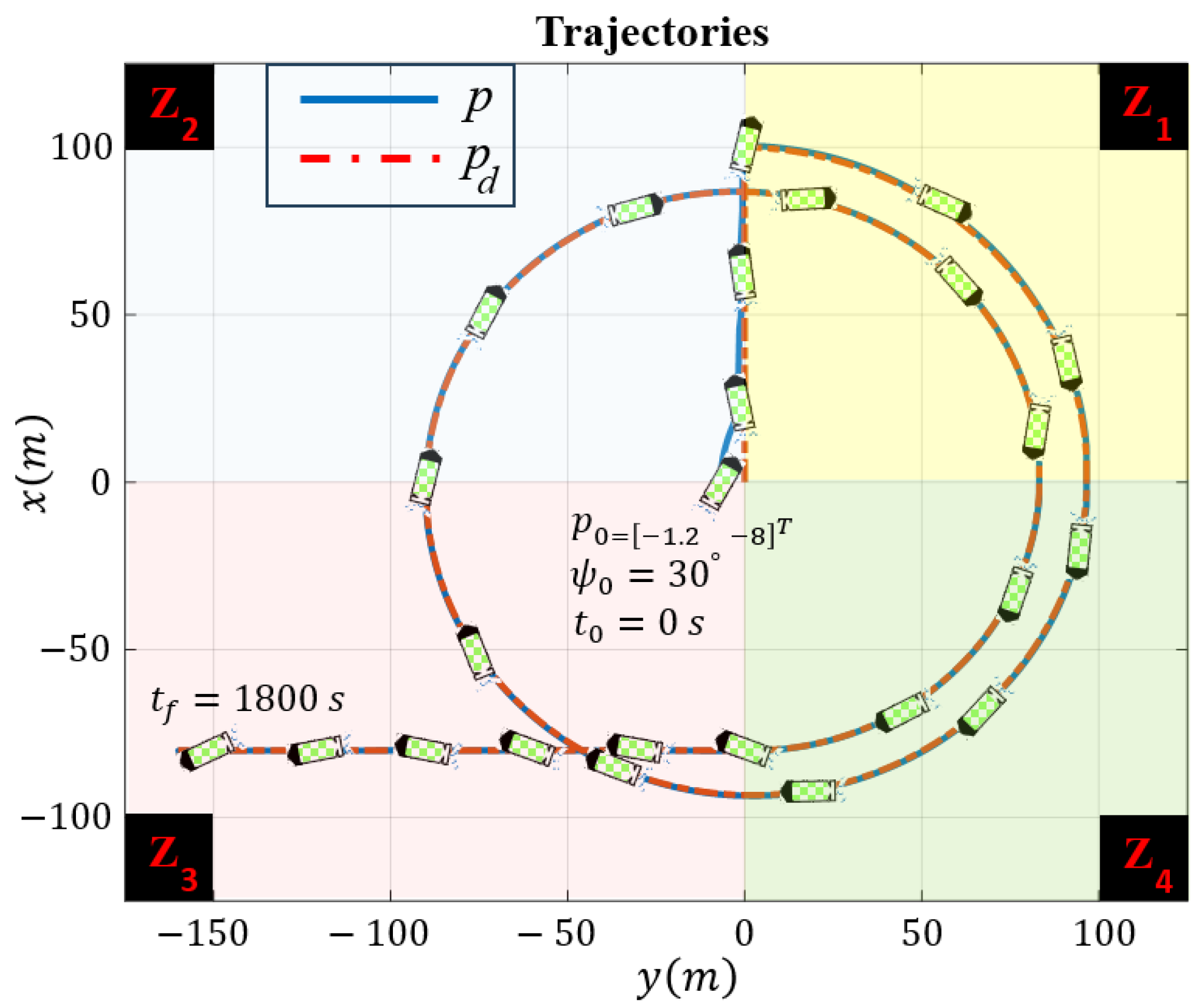
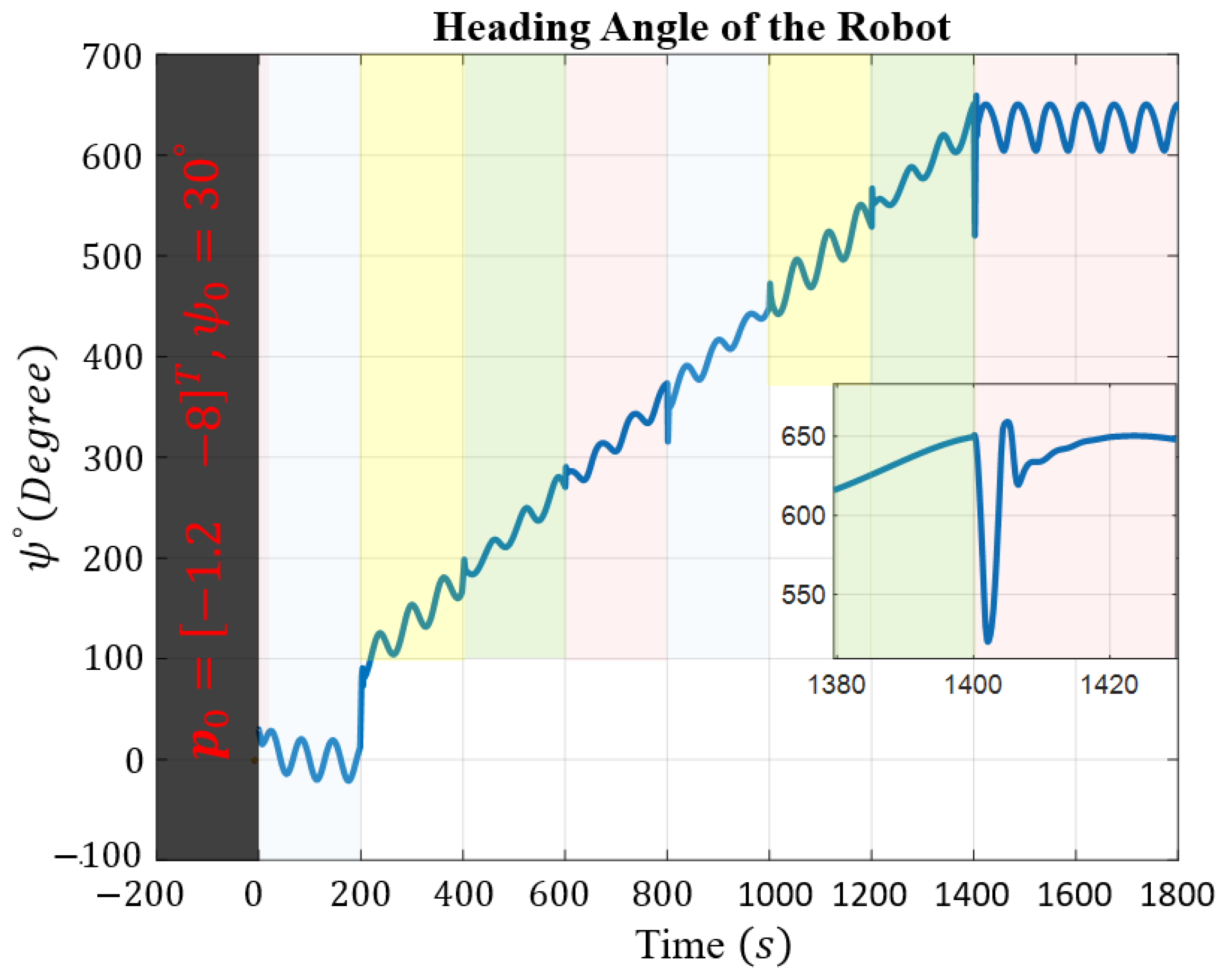
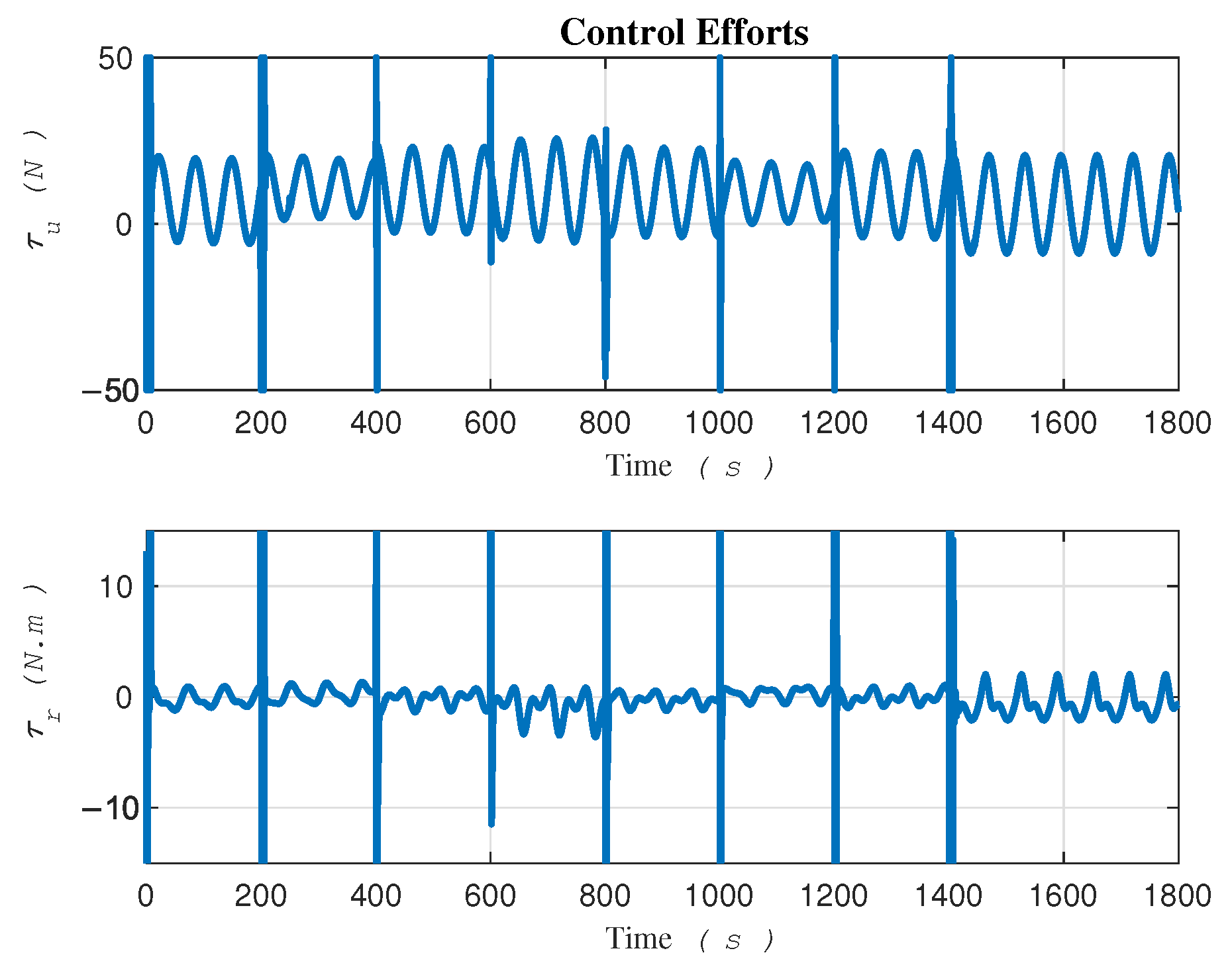
5. Simulation Results and Discussion
5.1. Simulation A: Proposed Method
- ;
- ;
- ;
- .
5.2. Simulation B: Gradient-Descent Based Control
5.3. Comparative Analysis and Interpretation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SMC | Sliding Mode Control |
| TSMC | Terminal Sliding Mode Control |
| FC | Funnel Control |
| CBF | Control Barrier Functions |
| SGPFS | Semi-Globally Practically Finite-Time Stability |
References
- Li, B.; Gao, X.; Huang, H.; Yang, H. Improved adaptive twisting sliding mode control for trajectory tracking of an AUV subject to uncertainties. J. Ocean Eng. 2024, 297, 116204. [Google Scholar] [CrossRef]
- Li, X.; Qin, H.; Li, L.; Xue, Y. Adaptive fixed-time fuzzy formation control for multiple AUV systems considering time-varying tracking error constraints and asymmetric actuator saturation. J. Ocean Eng. 2024, 297, 116936. [Google Scholar] [CrossRef]
- Elmokadem, T.; Zribi, M.; Youcef-Toumi, K. Terminal sliding mode control for the trajectory tracking of underactuated Autonomous Underwater Vehicles. J. Ocean Eng. 2017, 129, 613–625. [Google Scholar] [CrossRef]
- Aguiar, A.P.; Hespanha, J.P. Trajectory-tracking and path-following of underactuated autonomous vehicles with parametric modeling uncertainty. IEEE Trans. Autom. Control 2007, 52, 1362–1379. [Google Scholar] [CrossRef]
- Li, B.; Wen, S.; Yan, Z.; Wen, G.; Huang, T. A survey on the control lyapunov function and control barrier function for nonlinear-affine control systems. IEEE/CAA J. Autom. Sin. 2023, 10, 584–602. [Google Scholar] [CrossRef]
- Yan, S.; Shi, L.; Zhang, H.; Yao, S.; Zhou, Y. Safety-Critical Model-Free Adaptive Iterative Learning Control for Multi-agent Consensus using Control Barrier Functions. IEEE Trans. Circuits Syst. II 2024, 71, 221–225. [Google Scholar] [CrossRef]
- Bao, J.; Wang, H.; Liu, P.X. Adaptive finite-time tracking control for robotic manipulators with funnel boundary. Int. J. Adapt. Control Signal Process. 2020, 34, 575–589. [Google Scholar] [CrossRef]
- Li, Y.; Dong, S.; Li, K. Fuzzy adaptive finite-time event-triggered control of time-varying formation for nonholonomic multirobot systems. IEEE Trans. Intell. Veh. 2024, 9, 725–737. [Google Scholar] [CrossRef]
- Nazmara, G.R.; Fateh, M.M.; Ahmadi, S.M. A model-reference impedance control of robot manipulators using an adaptive fuzzy uncertainty estimator. Int. J. Comput. Intell. Syst. 2018, 11, 979–990. [Google Scholar] [CrossRef]
- Nazmara, G.R.; Fateh, M.M.; Ahmadi, S.M. Exponentially convergence for the regressor-free adaptive fuzzy impedance control of robots by gradient descent algorithm. Int. J. Syst. Sci. 2020, 51, 1883–1904. [Google Scholar] [CrossRef]
- Zhang, L.; Huang, B.; Liao, Y.; Wang, B. Finite-Time Trajectory Tracking Control for Uncertain Underactuated Marine Surface Vessels. IEEE Access 2019, 7, 102321–102330. [Google Scholar] [CrossRef]
- Ahmadi, S.M.; Taghadosi, M.B.; Nazmara, G. Adaptive finite-time impedance backstepping control for uncertain robotic systems interacting with unknown environments. Int. J. Control 2023, 96, 2671–2682. [Google Scholar] [CrossRef]
- Jiang, Z.P. Global tracking control of underactuated ships by Lyapunov’s direct method. J. Autom. 2002, 38, 301–309. [Google Scholar] [CrossRef]
- Lefeber, E.; Pettersen, K.Y.; Nijmeijer, H. Tracking control of an underactuated ship. IEEE Trans. Control Syst. Technol. 2003, 11, 52–61. [Google Scholar] [CrossRef]
- Nazmara, G.; Aguiar, A.P. Robust Path-Following Control of Underactuated Marine Vehicles Using Gradient-Descent Fuzzy Estimation. In Proceedings of the CONTROLO 2022, Caparica, Portugal, 6–8 July 2022. [Google Scholar]
- Vanni, F.; Aguiar, A.P.; Pascoal, A.M. Cooperative path-following of underactuated autonomous marine vehicles with logic-based communication. IFAC Proc. Vol. 2008, 41, 107–112. [Google Scholar] [CrossRef]
- Aguiar, A.P.; Pascoal, A.M. Dynamic positioning and way-point tracking of underactuated AUVs in the presence of ocean currents. Int. J. Control 2007, 10, 1092–1108. [Google Scholar] [CrossRef]
- Wang, L.X. A Course in Fuzzy Systems; Prentice-Hall Press: Hoboken, NJ, USA, 1999. [Google Scholar]
- Qian, C.; Lin, W. Non-Lipschitz continuous stabilizers for nonlinear systems with uncontrollable unstable linearization. J. SCL 2001, 42, 185–200. [Google Scholar] [CrossRef]
- Yu, S.; Yu, X.; Shirinzadeh, B.; Man, Z. Continuous finite-time control for robotic manipulators with terminal sliding mode. J. Autom. 2005, 41, 1957–1964. [Google Scholar] [CrossRef]
- Wang, N.; Pan, X. Path following of autonomous underactuated ships: A translation–rotation cascade control approach. IEEE/ASME Trans. Mechatronics 2019, 24, 2583–2593. [Google Scholar] [CrossRef]



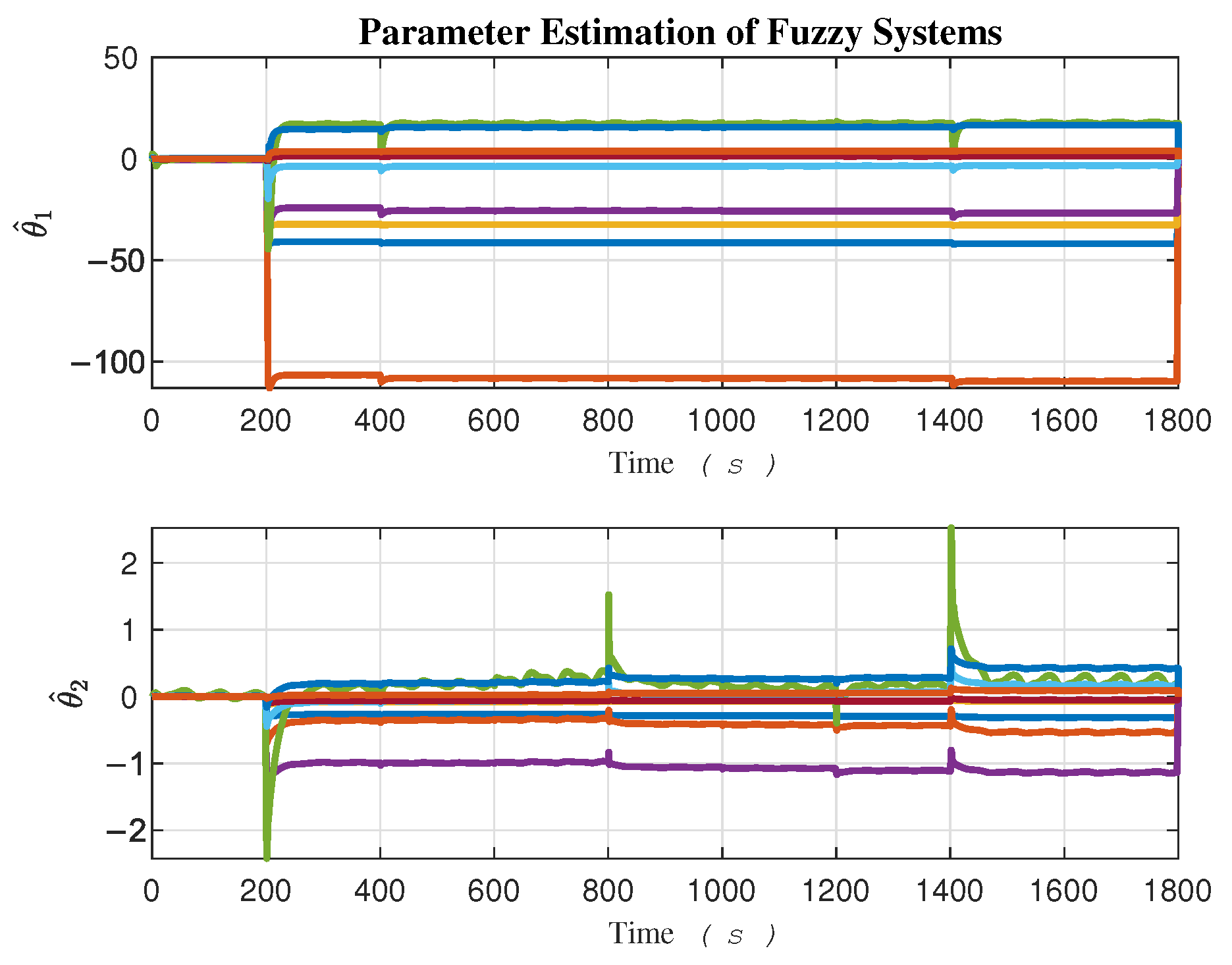

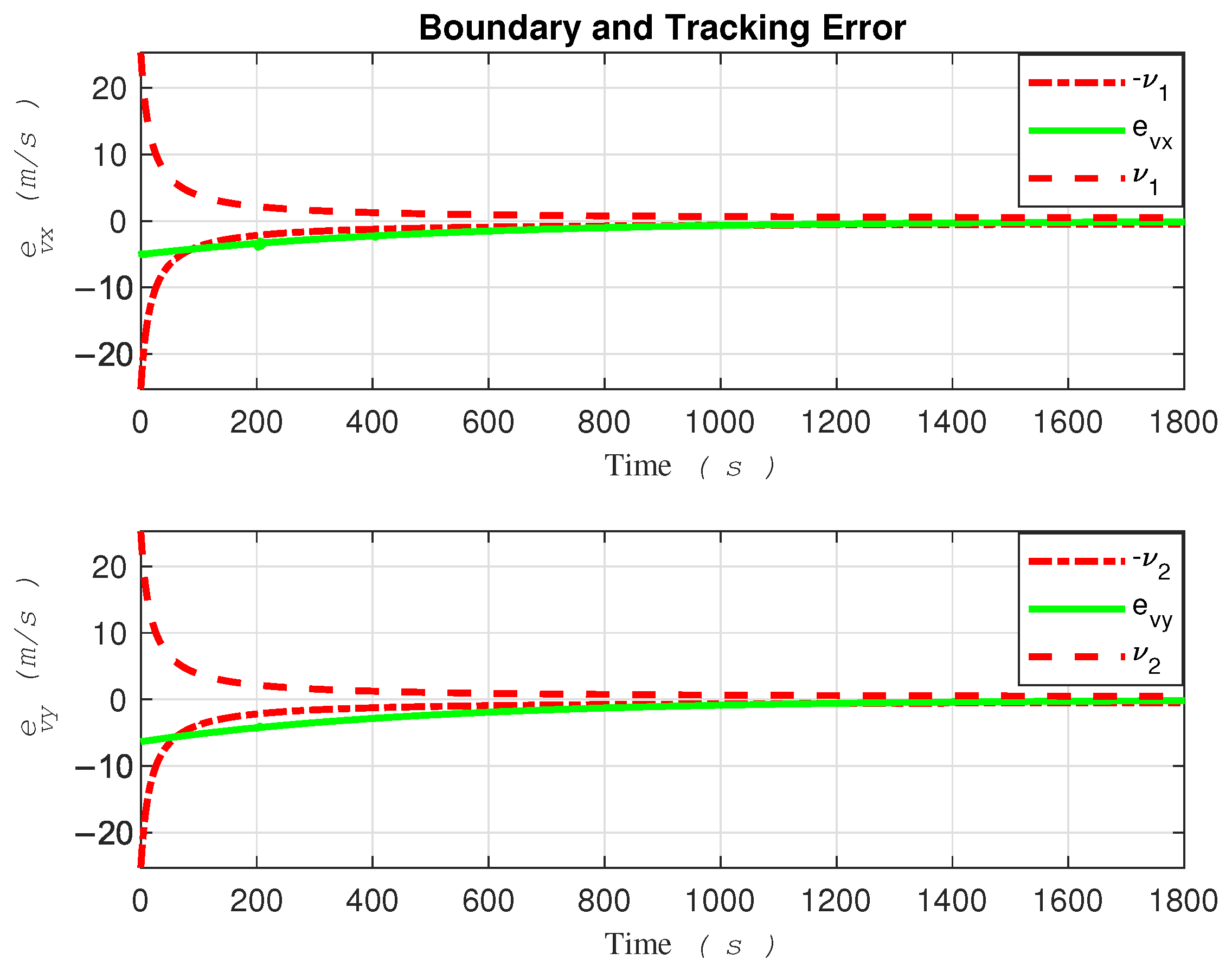
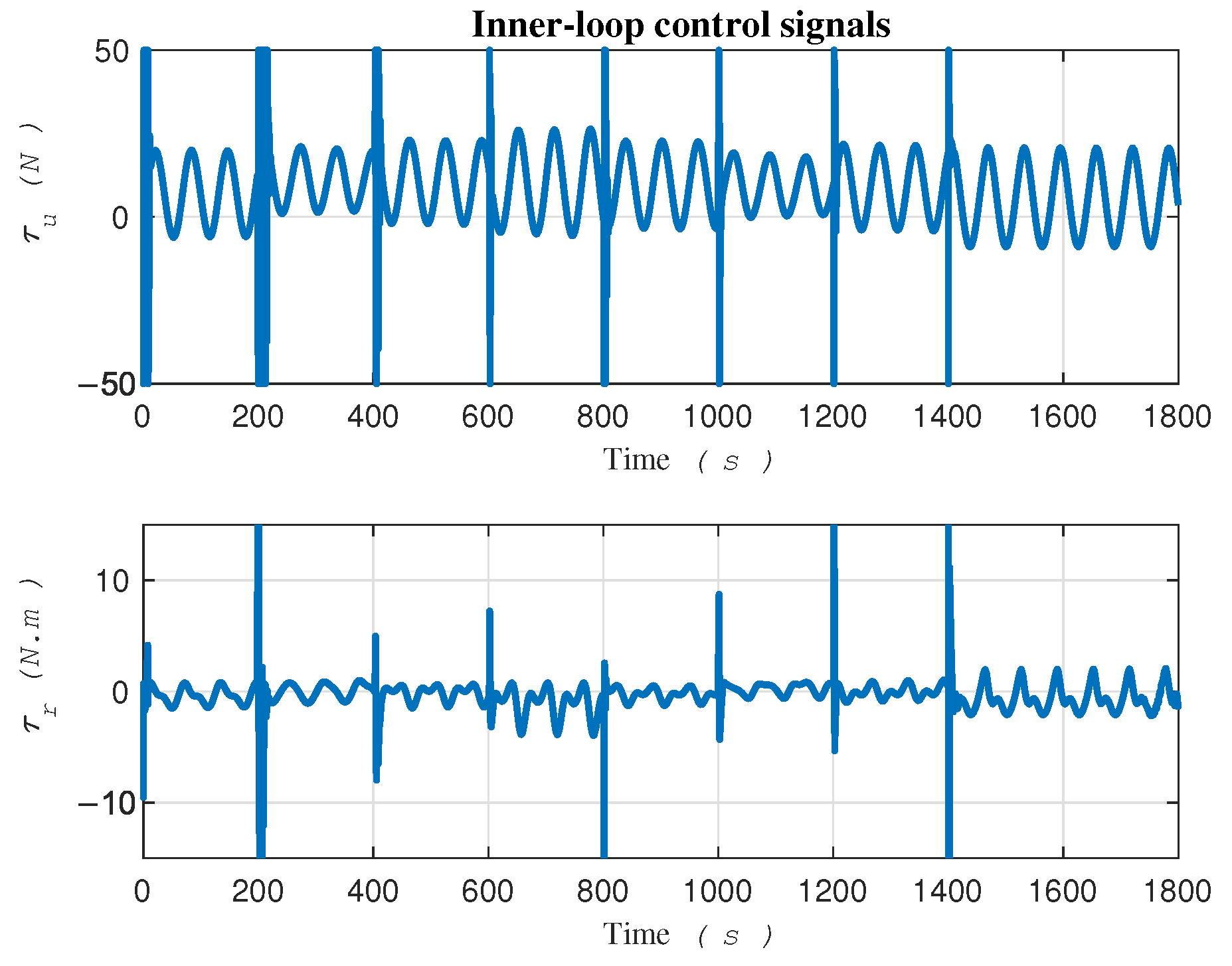


| 47.52 | 104.05 | 13.38 | 13.5 | 44.96 | 27.2 |
| 25 | 0.25 | 0.8 | 0.06 | 8 | 39.5 | 0.1 | 0.2 | 20 | 8 | 0.0005 | 0.1 | 0.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nazmara, G.R.; Aguiar, A.P. Safe Robust Adaptive Motion Control for Underactuated Marine Robots. Sensors 2024, 24, 3974. https://doi.org/10.3390/s24123974
Nazmara GR, Aguiar AP. Safe Robust Adaptive Motion Control for Underactuated Marine Robots. Sensors. 2024; 24(12):3974. https://doi.org/10.3390/s24123974
Chicago/Turabian StyleNazmara, G. Reza, and A. Pedro Aguiar. 2024. "Safe Robust Adaptive Motion Control for Underactuated Marine Robots" Sensors 24, no. 12: 3974. https://doi.org/10.3390/s24123974
APA StyleNazmara, G. R., & Aguiar, A. P. (2024). Safe Robust Adaptive Motion Control for Underactuated Marine Robots. Sensors, 24(12), 3974. https://doi.org/10.3390/s24123974










