Computation of the Characteristic Parameters of Coaxial Waveguides Used in Precision Sensors
Abstract
1. Introduction
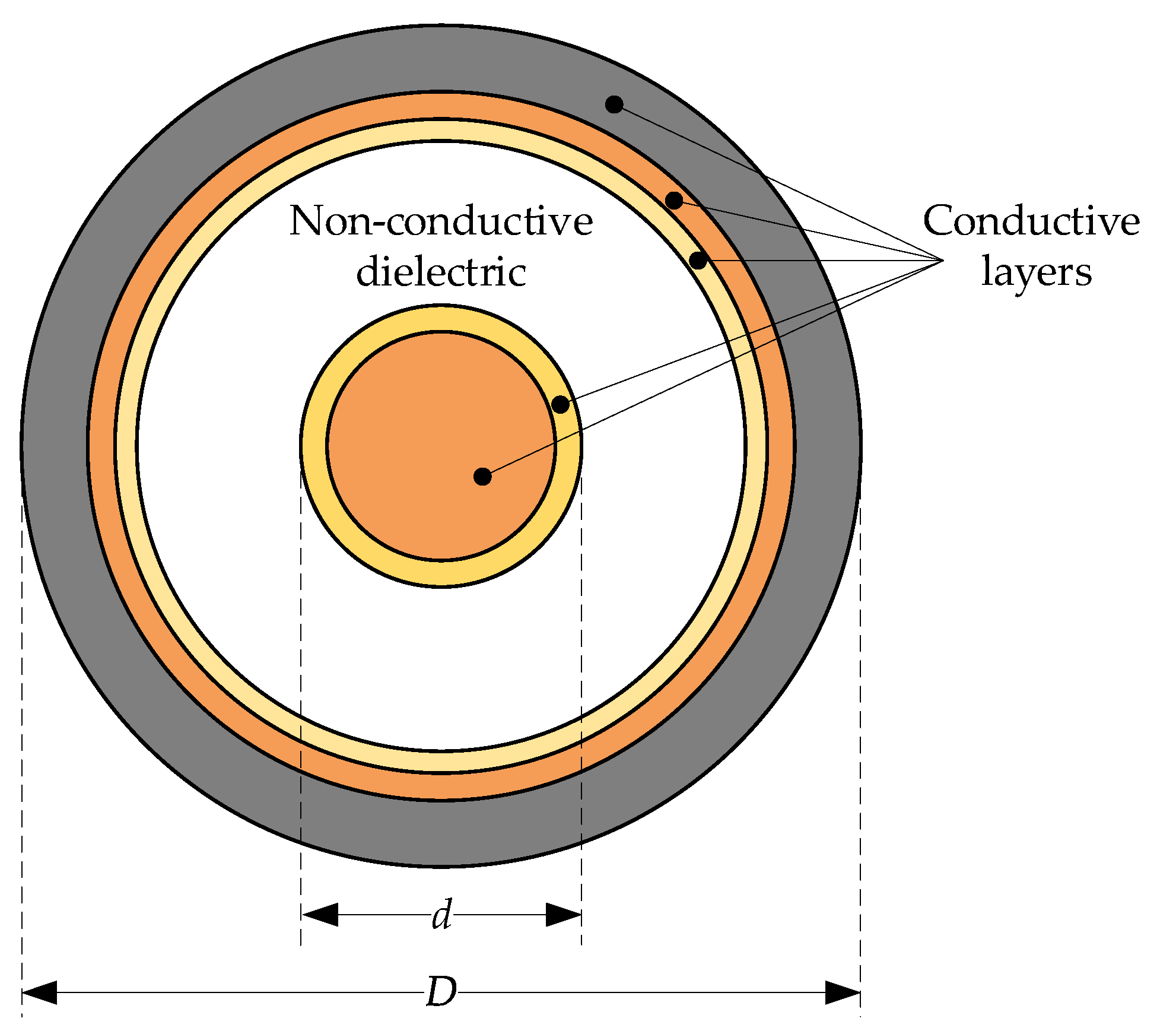
2. Multilayer Waveguide
3. Internal Impedance of the Outer Conductor
4. Results
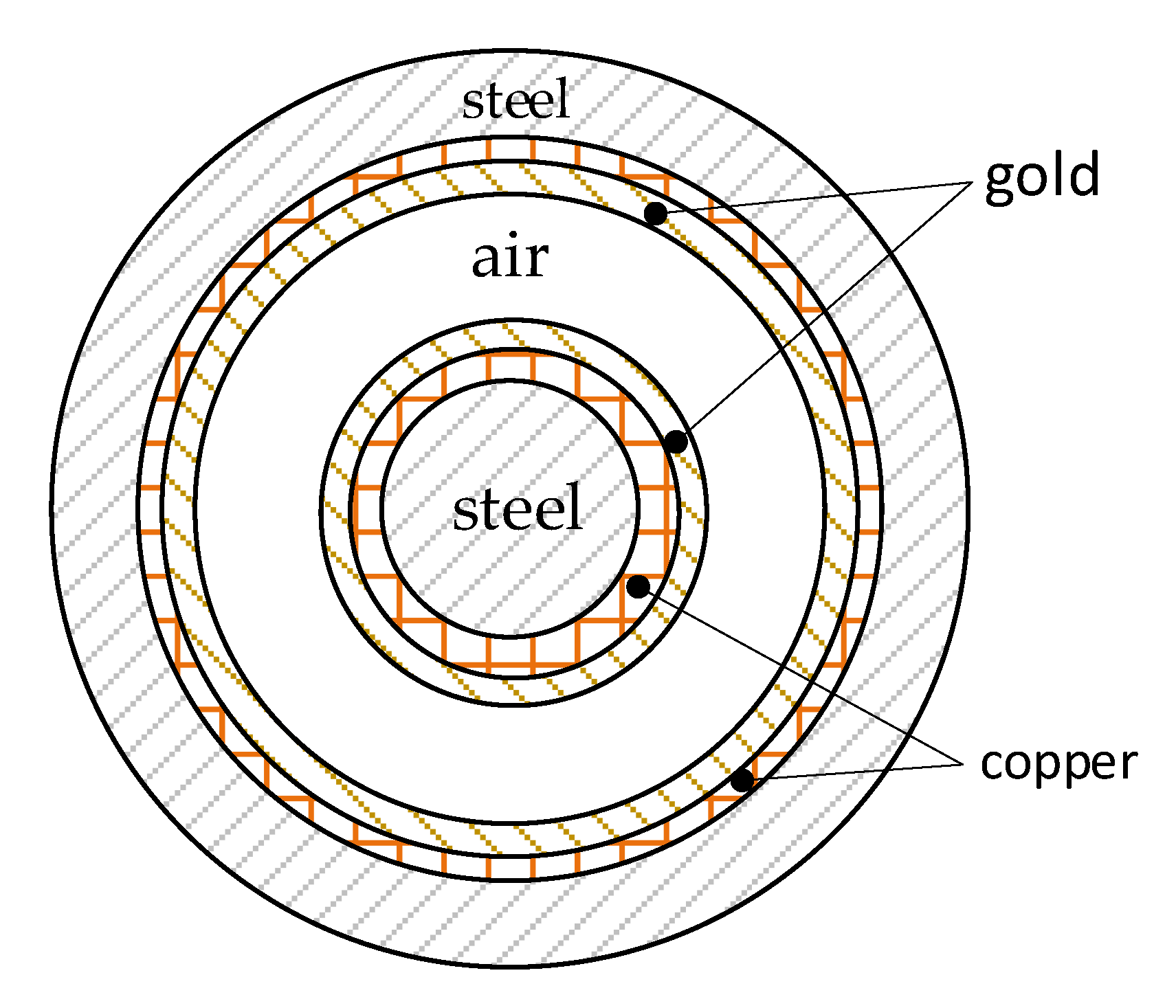
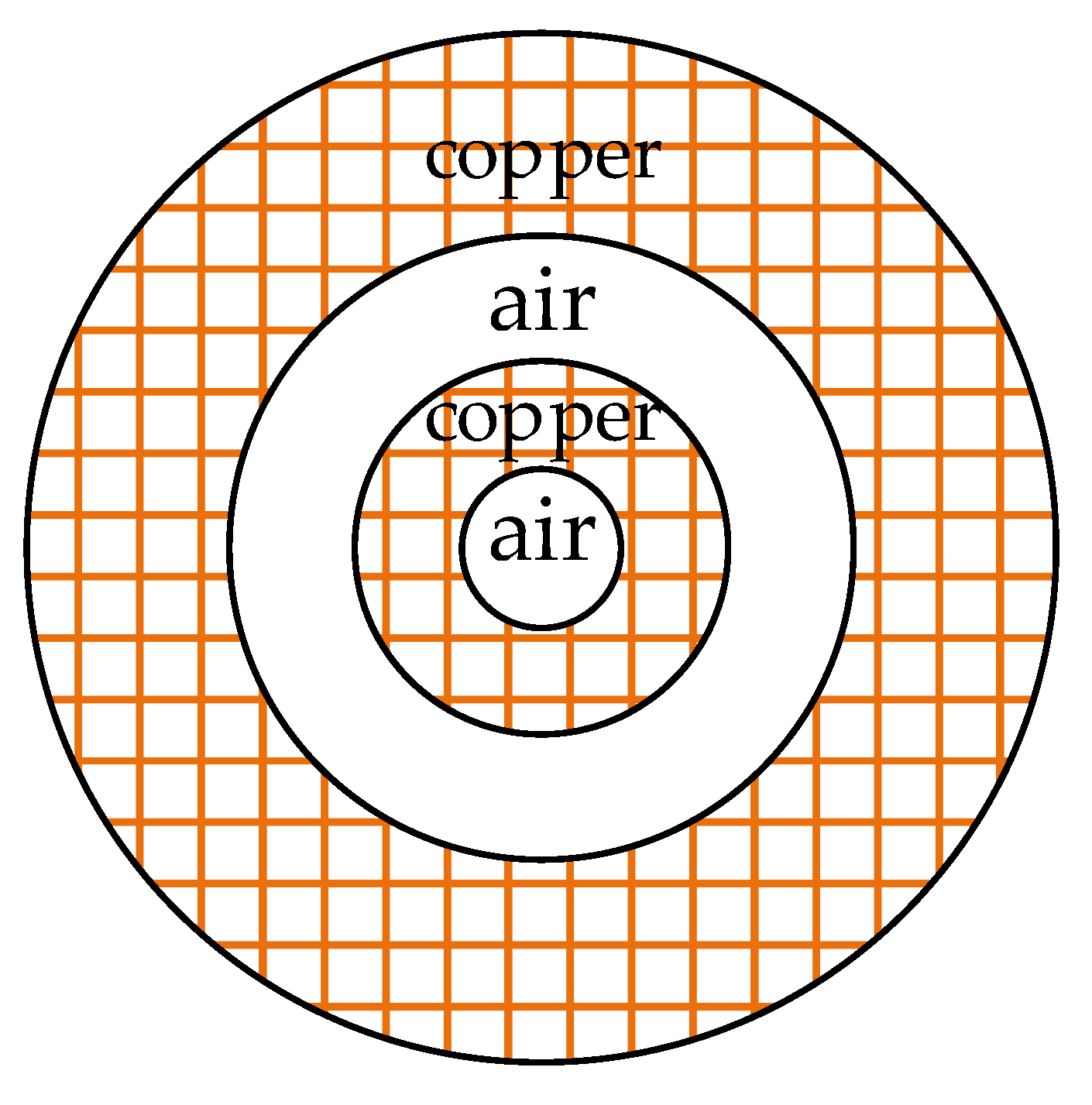
4.1. The Validation of the Algorithm
4.2. Comparison of the Computation Time for the Analytical Algorithms
5. Discussion
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kapranov, S.V.; Kouzaev, G.A. Study of Microwave Heating of Reference Liquids in a Coaxial Waveguide Reactor Using the Experimental, Semi-Analytical and Numerical Means. Int. J. Therm. Sci. 2019, 140, 505–520. [Google Scholar] [CrossRef]
- Tan, W.; Xiao, Y.; Li, C.; Zhu, K.; Luo, H.; Sun, H. A Wide-Band High-Efficiency Hybrid-Feed Antenna Array for Mm-Wave Wireless Systems. Electronics 2021, 10, 2383. [Google Scholar] [CrossRef]
- Bittner, T.; Bajodek, M.; Bore, T.; Vourc’h, E.; Scheuermann, A. Determination of the Porosity Distribution during an Erosion Test Using a Coaxial Line Cell. Sensors 2019, 19, 611. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Lin, C.-P.; Jheng, W.-H. A New TDR-Based Sensing Cable for Improving Performance of Bridge Scour Monitoring. Sensors 2020, 20, 6665. [Google Scholar] [CrossRef]
- Zhang, C.; Shi, Z.; Yang, H.; Zhou, X.; Wu, Z.; Jayas, D.S. A Novel, Portable and Fast Moisture Content Measuring Method for Grains Based on an Ultra-Wideband (UWB) Radar Module and the Mode Matching Method. Sensors 2019, 19, 4224. [Google Scholar] [CrossRef]
- Shan, Y.; Meng, Y.S.; Filipski, P.S. Evaluation of a Calorimetric Thermal Voltage Converter for RF–DC Difference up to 1 GHz. IEEE Trans. Instrum. Meas. 2014, 63, 467–472. [Google Scholar] [CrossRef]
- Filipski, P.S.; van Mullem, C.J.; Janik, D.; Klonz, M.; Kinard, J.R.; Lipe, T.E.; Waltrip, B.C. Comparison of High-Frequency AC-DC Voltage Transfer Standards at NRC, VSL, PTB, and NIST. IEEE Trans. Instrum. Meas. 2001, 50, 349–352. [Google Scholar] [CrossRef]
- Kubiczek, K.; Kampik, M.; Grzenik, M. A Novel Temperature Sensor for a Calorimetric Thermal Converter. Measurement 2022, 201, 111686. [Google Scholar] [CrossRef]
- Kyriazis, G.A.; de Souza, R.M.; Yasuda, E.S.; di Lillo, L. Modeling the AC–DC Transfer Difference of Wideband Cage-Type Current Shunts. IEEE Trans. Instrum. Meas. 2020, 69, 4436–4444. [Google Scholar] [CrossRef]
- Malinowski, M.; Kubiczek, K.; Kampik, M. A Precision Coaxial Current Shunt for Current AC-DC Transfer. Measurement 2021, 176, 109126. [Google Scholar] [CrossRef]
- Durandetto, P.; Sosso, A.; Monticone, E.; Trinchera, B.; Fretto, M.; Lacquaniti, V. Cryocooled Josephson Standards for AC Voltage Metrology. J. Phys. Conf. Ser. 2017, 841, 012031. [Google Scholar] [CrossRef]
- Kampik, M.; Musioł, K. Investigations of the High-Performance Source of Digitally Synthesized Sinusoidal Voltage for Primary Impedance Metrology. Measurement 2021, 168, 108308. [Google Scholar] [CrossRef]
- Musioł, K.; Kampik, M.; Koszarny, M. A New Sampling-Based Four-Terminal-Pair Digital Impedance Bridge. Meas. Sens. 2021, 18, 100307. [Google Scholar] [CrossRef]
- Musioł, K. Experimental Study of Digitizers Used in High-Precision Impedance Measurements. Energies 2022, 15, 4051. [Google Scholar] [CrossRef]
- van der Veen, A.M.H.; Cox, M.G. Getting Started with Uncertainty Evaluation Using the Monte Carlo Method in R. Accredit. Qual. Assur. 2021, 26, 129–141. [Google Scholar] [CrossRef]
- Khalili, S.M.R.; Botshekanan Dehkordi, M.; Carrera, E. A Nonlinear Finite Element Model Using a Unified Formulation for Dynamic Analysis of Multilayer Composite Plate Embedded with SMA Wires. Compos. Struct. 2013, 106, 635–645. [Google Scholar] [CrossRef]
- Song, Z.; Yahyaoui, W.; Duval, F.; Su, D.; Louis, A. Capturing Skin Effect with an Effective Nonuniform Mesh and Coupled R-L Circuits. Electron. Lett. 2011, 47, 94. [Google Scholar] [CrossRef]
- Hassanat, A.; Prasath, V.; Abbadi, M.; Abu-Qdari, S.; Faris, H. An Improved Genetic Algorithm with a New Initialization Mechanism Based on Regression Techniques. Information 2018, 9, 167. [Google Scholar] [CrossRef]
- Clayton, R. Paul Introduction to Electromagnetic Compatibility, 2nd ed.; Wiley-Interscience: New York, NY, USA, 2006. [Google Scholar]
- Jackson John David Classical Electrodynamics; Wiley: Hoboken, NJ, USA, 2011.
- Dommel, H.W. Overhead Line Parameters from Handbook Formulas and Computer Programs. IEEE Power Eng. Rev. 1985, PER-5, 38. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Dommel, H.W. EMTP Theory Book; Microtran Power System Analysis Corporation: Vancouver, BC, Canada, 1992. [Google Scholar]
- Vujević, S.; Boras, V.; Sarajčev, P. A Novel Algorithm for Internal Impedance Computation of Solid and Tubular Cylindrical Conductors. Int. Rev. Electr. Eng. 2009, 4, 1418–1425. [Google Scholar]
- Nahman, N.; Holt, D. Transient Analysis of Coaxial Cables Using the Skin Effect ApproximationA+Bsqrt{s}. IEEE Trans. Circuit Theory 1972, 19, 443–451. [Google Scholar] [CrossRef]
- Semlyen, A.; Deri, A. Time Domain Modelling of Frequency Dependent Three-Phase Transmission Line Impedance. IEEE Trans. Power Appar. Syst. 1985, PAS-104, 1549–1555. [Google Scholar] [CrossRef]
- Carson, J.R.; Gilbert, J.J. Transmission Characteristics of the Submarine Cable. Bell Syst. Tech. J. 1922, 1, 88–115. [Google Scholar] [CrossRef]
- Brandao Faria, J.A.M. A matrix approach for the evaluation of the internal impedance of multilayered cylindrical structures. Prog. Electromagn. Res. B 2011, 28, 351–367. [Google Scholar] [CrossRef]
- Kubiczek, K.; Kampik, M. Highly Accurate and Numerically Stable Matrix Computations of the Internal Impedance of Multilayer Cylindrical Conductors. IEEE Trans. Electromagn. Compat. 2020, 62, 204–211. [Google Scholar] [CrossRef]
- Vujević, S.; Lovrić, D.; Krolo, I.; Duvnjak, I. Computation of electric and magnetic field distribution inside a multilayer cylindrical conductor. Prog. Electromagn. Res. M 2020, 88, 53–63. [Google Scholar] [CrossRef]
- Kubiczek, K.; Kampik, M. Precision Calculations of the Characteristic Impedance of Complex Coaxial Waveguides Used in Wideband Thermal Converters of AC Voltage and Current. Int. J. Electron. Telecommun. 2022, 68, 527–533. [Google Scholar]
- COMSOL Multiphysics® v. 6.1. COMSOL AB, Stockholm, Sweden. Available online: www.comsol.com (accessed on 12 November 2022).
- Peres, P.L.D.; de Souza, C.R.; Bonatti, I.S. ABCD Matrix: A Unique Tool for Linear Two-Wire Transmission Line Modelling. Int. J. Electr. Eng. Educ. 2003, 40, 220–229. [Google Scholar] [CrossRef]
- Schelkunoff, S.A. The Electromagnetic Theory of Coaxial Transmission Lines and Cylindrical Shields. Bell Syst. Tech. J. 1934, 13, 532–579. [Google Scholar] [CrossRef]
- Vujevic, S.; Lovric, D.; Boras, V. High-Accurate Numerical Computation of Internal Impedance of Cylindrical Conductors for Complex Arguments of Arbitrary Magnitude. IEEE Trans. Electromagn. Compat. 2014, 56, 1431–1438. [Google Scholar] [CrossRef]
- Eaton, J.W.; Bateman, D.; Hauberg, S.; Wehbring, R. GNU Octave Version 6.1.0 Manual: A High-Level Interactive Language for Numerical Computations. 2020. Available online: https://www.gnu.org/software/octave/doc/v6.3.0/ (accessed on 17 October 2022).
- MATLAB; The MathWorks Inc.: Natick, MA, USA, 2022.
- Kubiczek, K.; Kampik, M. Highly Accurate and Numerically Stable Computations of Double-Layer Coaxial Waveguides. Eng. Comput. 2019, 36, 1384–1399. [Google Scholar] [CrossRef]


| f, Hz | |Zc|, Ω | ||
|---|---|---|---|
| New Algorithm | Algorithm from [30] | COMSOL FEM | |
| 100 | 25,424 | 25,424 | 25,428 |
| 101 | 8039.9 | 8039.9 | 8041.1 |
| 102 | 2542.4 | 2542.4 | 2543.2 |
| 103 | 804.00 | 804.00 | 804.32 |
| 104 | 254.34 | 254.34 | 254.31 |
| 105 | 83.227 | 83.227 | 83.259 |
| 106 | 50.382 | 50.382 | 50.382 |
| 107 | 48.737 | 48.737 | 48.742 |
| 108 | 48.534 | 48.534 | 48.542 |
| 109 | 48.368 | 48.368 | 48.379 |
| 4·109 | 48.318 | 48.318 | 48.328 |
| f, Hz | R, Ω/m | ||
|---|---|---|---|
| New Algorithm | Algorithm from [30] | COMSOL FEM | |
| 100 | 0.2806685 | 0.2806685 | 0.2806685 |
| 101 | 0.2806685 | 0.2806685 | 0.2806685 |
| 102 | 0.2806685 | 0.2806685 | 0.2806685 |
| 103 | 0.2806685 | 0.2806685 | 0.2806685 |
| 104 | 0.2806714 | 0.2806714 | 0.2806714 |
| 105 | 0.2809538 | 0.2809538 | 0.2809538 |
| 106 | 0.3003694 | 0.3003694 | 0.3003694 |
| 107 | 0.3733909 | 0.3733909 | 0.3733909 |
| 108 | 0.8968540 | 0.8968540 | 0.8968594 |
| 109 | 3.0633201 | 3.0633201 | 3.0635652 |
| 4·109 | 9.6498060 | 9.6498060 | 9.6366165 |
| f, Hz | L, nH/m | ||
|---|---|---|---|
| New Algorithm | Algorithm from [30] | COMSOL FEM | |
| 100 | 170.8578 | 170.8578 | 170.8578 |
| 101 | 170.8578 | 170.8578 | 170.8578 |
| 102 | 170.8578 | 170.8578 | 170.8578 |
| 103 | 170.8578 | 170.8578 | 170.8578 |
| 104 | 170.8575 | 170.8575 | 170.8575 |
| 105 | 170.8273 | 170.8273 | 170.8273 |
| 106 | 168.7718 | 168.7718 | 168.7718 |
| 107 | 164.0388 | 164.0388 | 164.0388 |
| 108 | 162.7767 | 162.7767 | 162.7769 |
| 109 | 161.6674 | 161.6674 | 161.6794 |
| 4·109 | 161.3358 | 161.3358 | 161.3464 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kubiczek, K. Computation of the Characteristic Parameters of Coaxial Waveguides Used in Precision Sensors. Sensors 2023, 23, 2324. https://doi.org/10.3390/s23042324
Kubiczek K. Computation of the Characteristic Parameters of Coaxial Waveguides Used in Precision Sensors. Sensors. 2023; 23(4):2324. https://doi.org/10.3390/s23042324
Chicago/Turabian StyleKubiczek, Krzysztof. 2023. "Computation of the Characteristic Parameters of Coaxial Waveguides Used in Precision Sensors" Sensors 23, no. 4: 2324. https://doi.org/10.3390/s23042324
APA StyleKubiczek, K. (2023). Computation of the Characteristic Parameters of Coaxial Waveguides Used in Precision Sensors. Sensors, 23(4), 2324. https://doi.org/10.3390/s23042324







