A Study on the Control Method of 6-DOF Magnetic Levitation System Using Non-Contact Position Sensors
Abstract
:1. Introduction
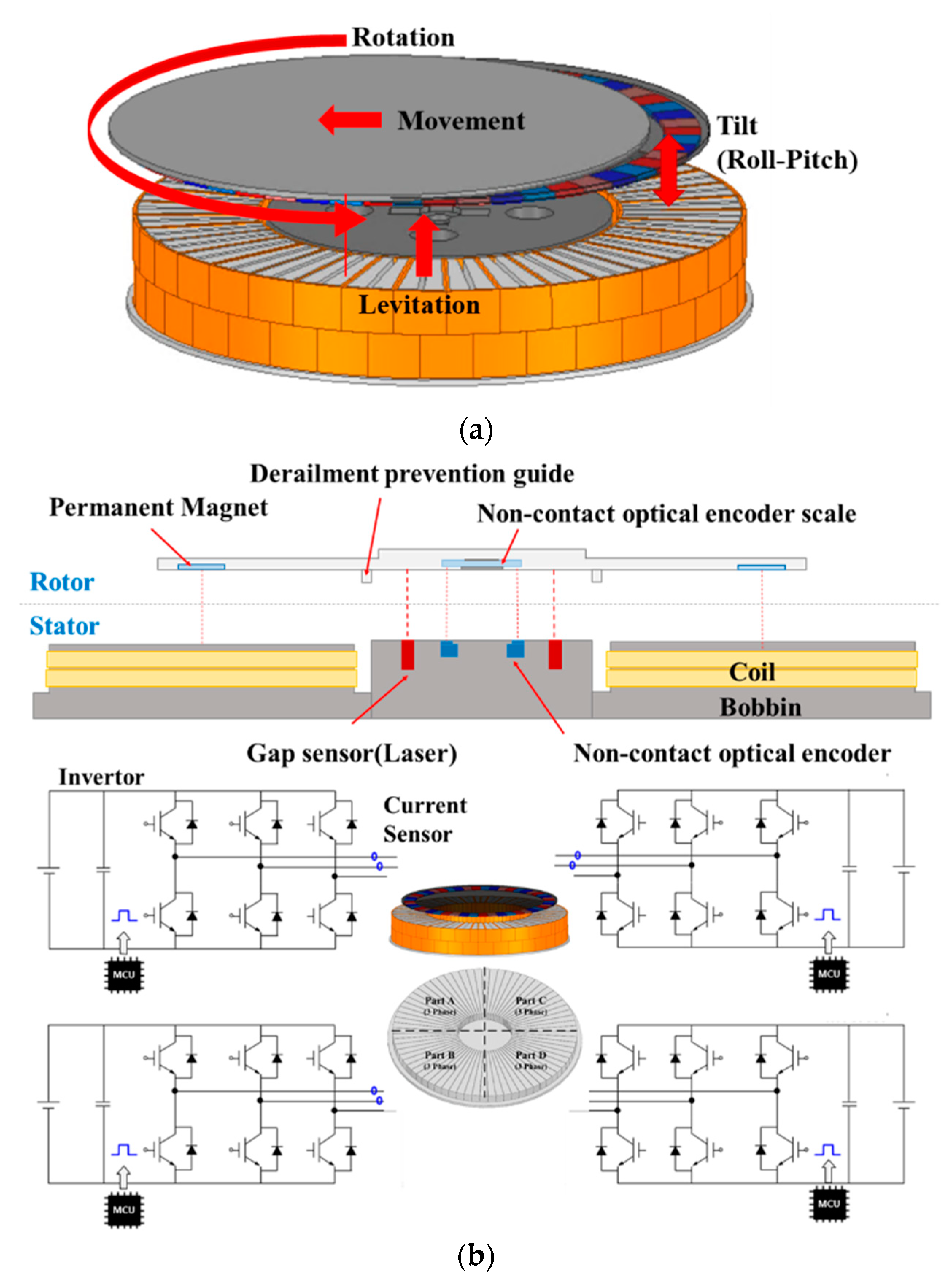
2. Design of 6-DOF Magnetic Levitation System
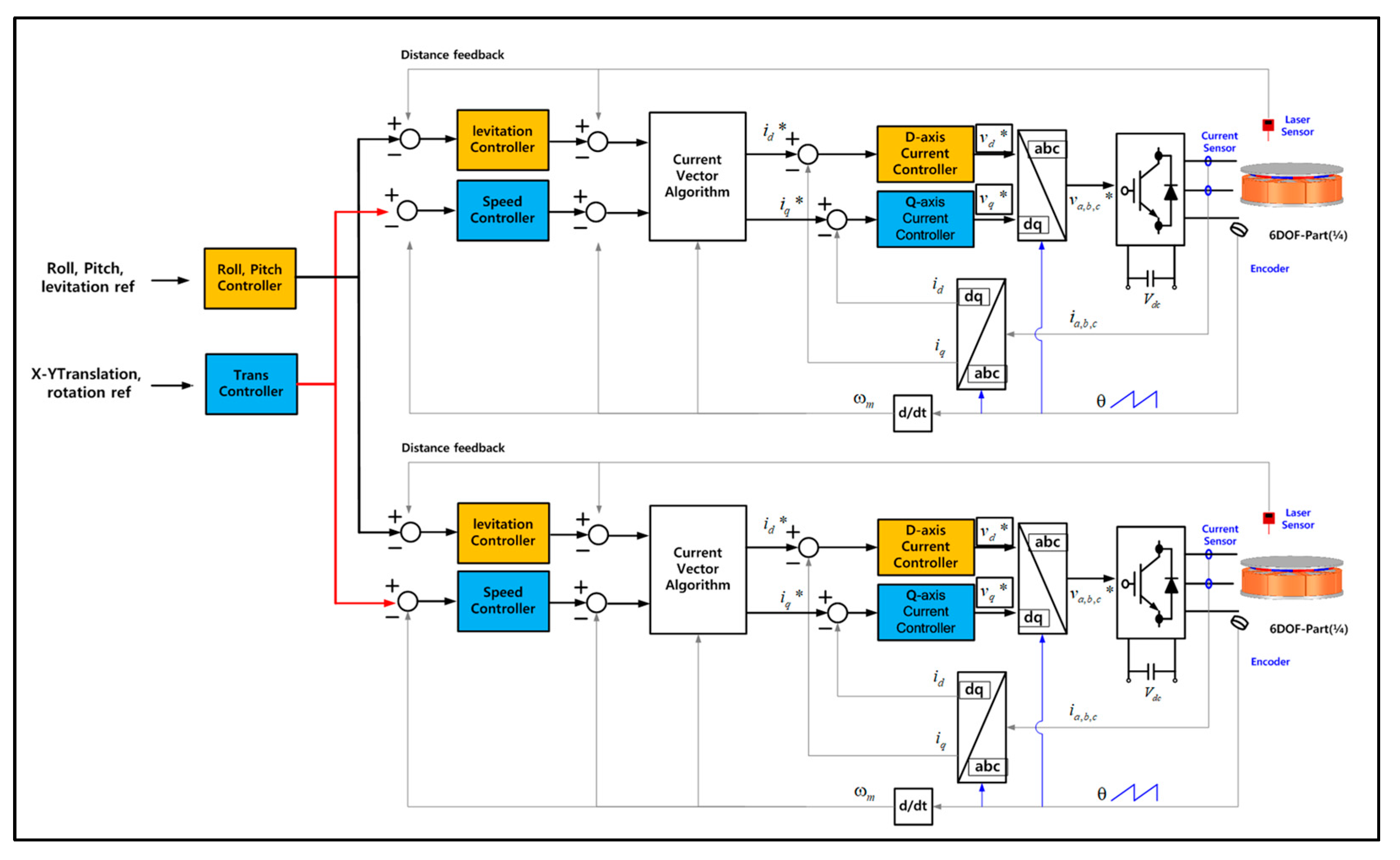
3. Control Method of 6-DOF Magnetic Levitation System Using Current Vector Control
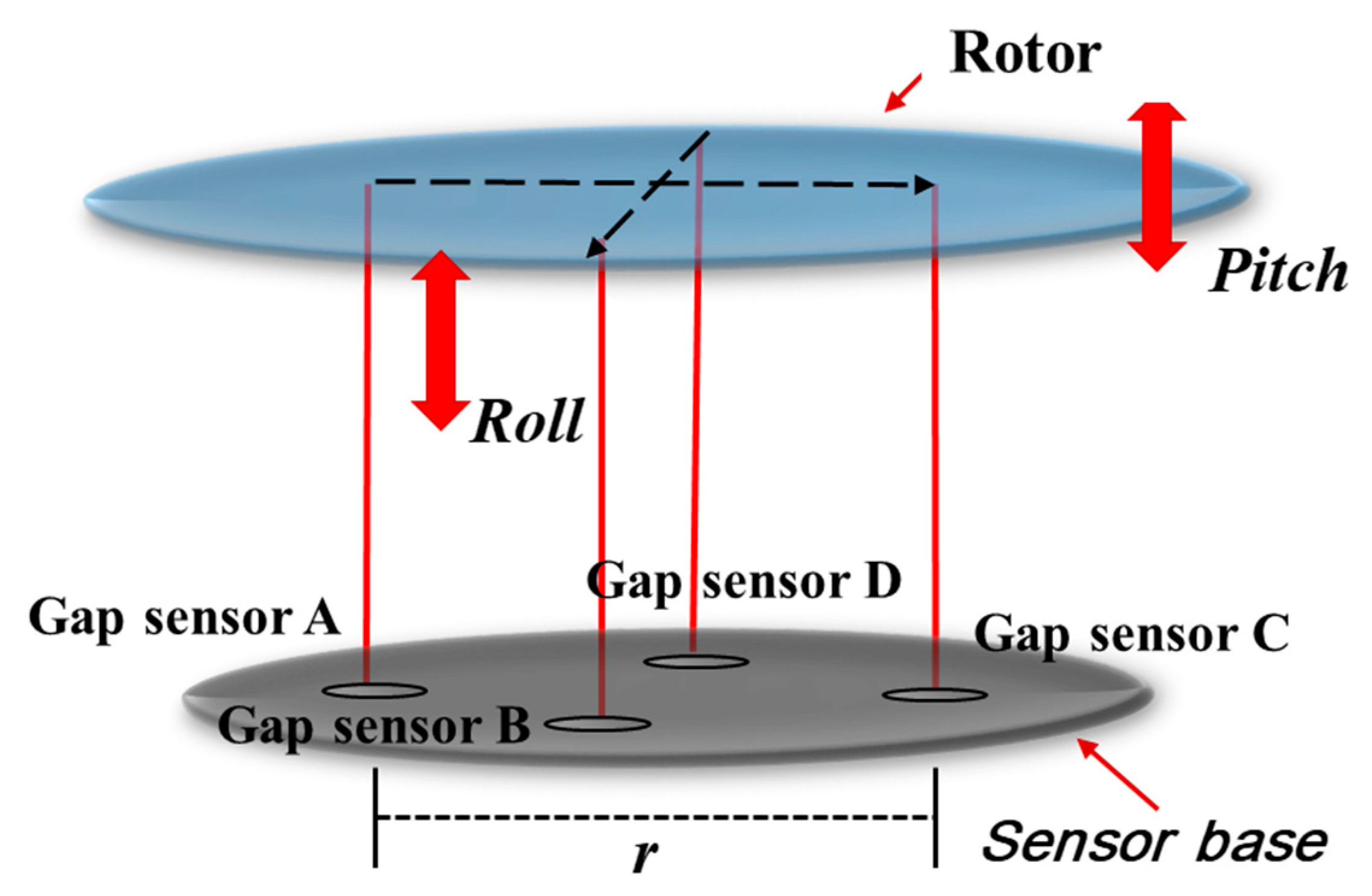
3.1. Control of the Magnetic Levitation
3.2. Control of the Tilt (Roll–Pitch)
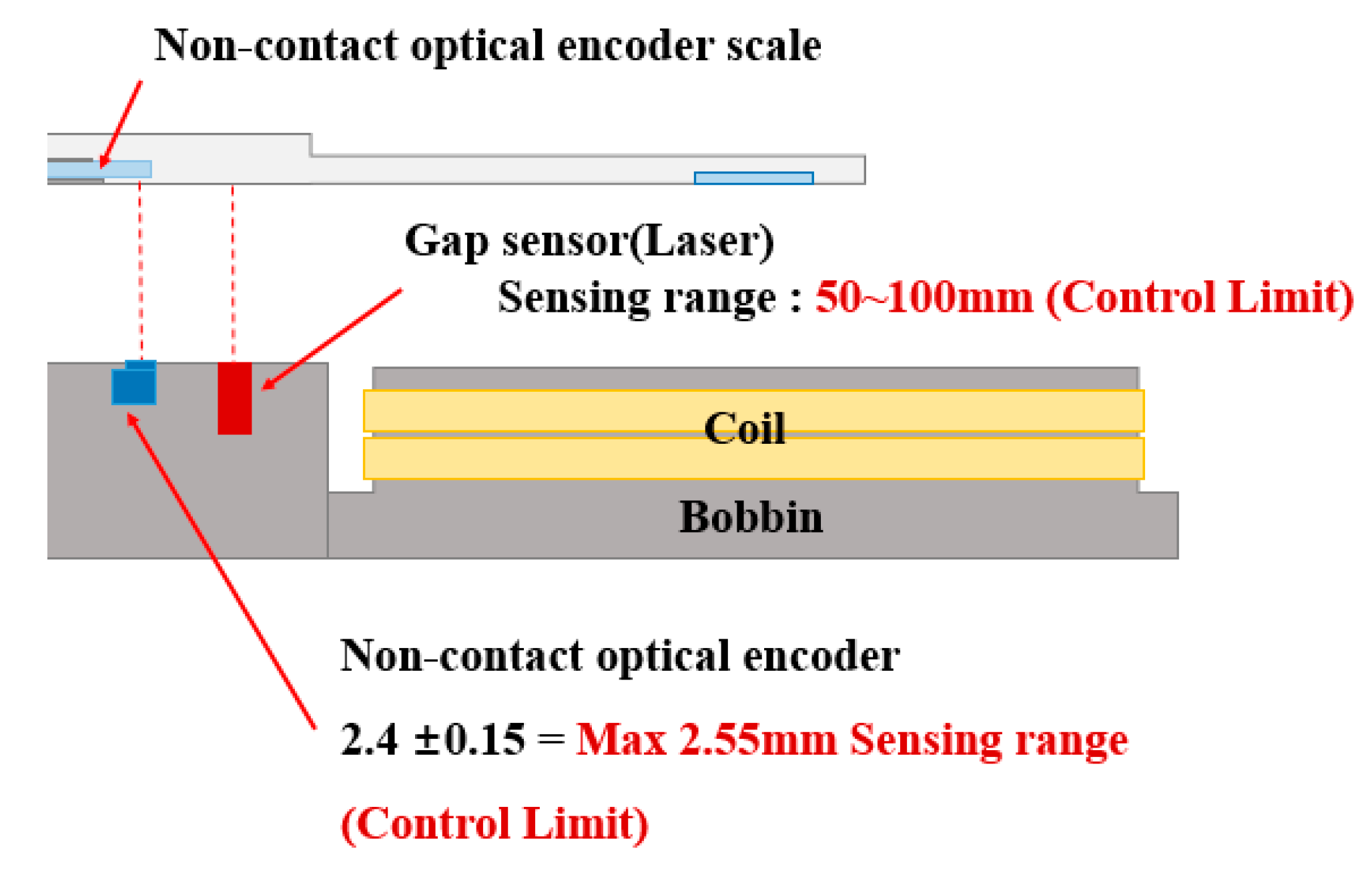
3.3. Method of Levitation and Tilt Sensing Using Gap Sensors
3.4. Control of the Rotation
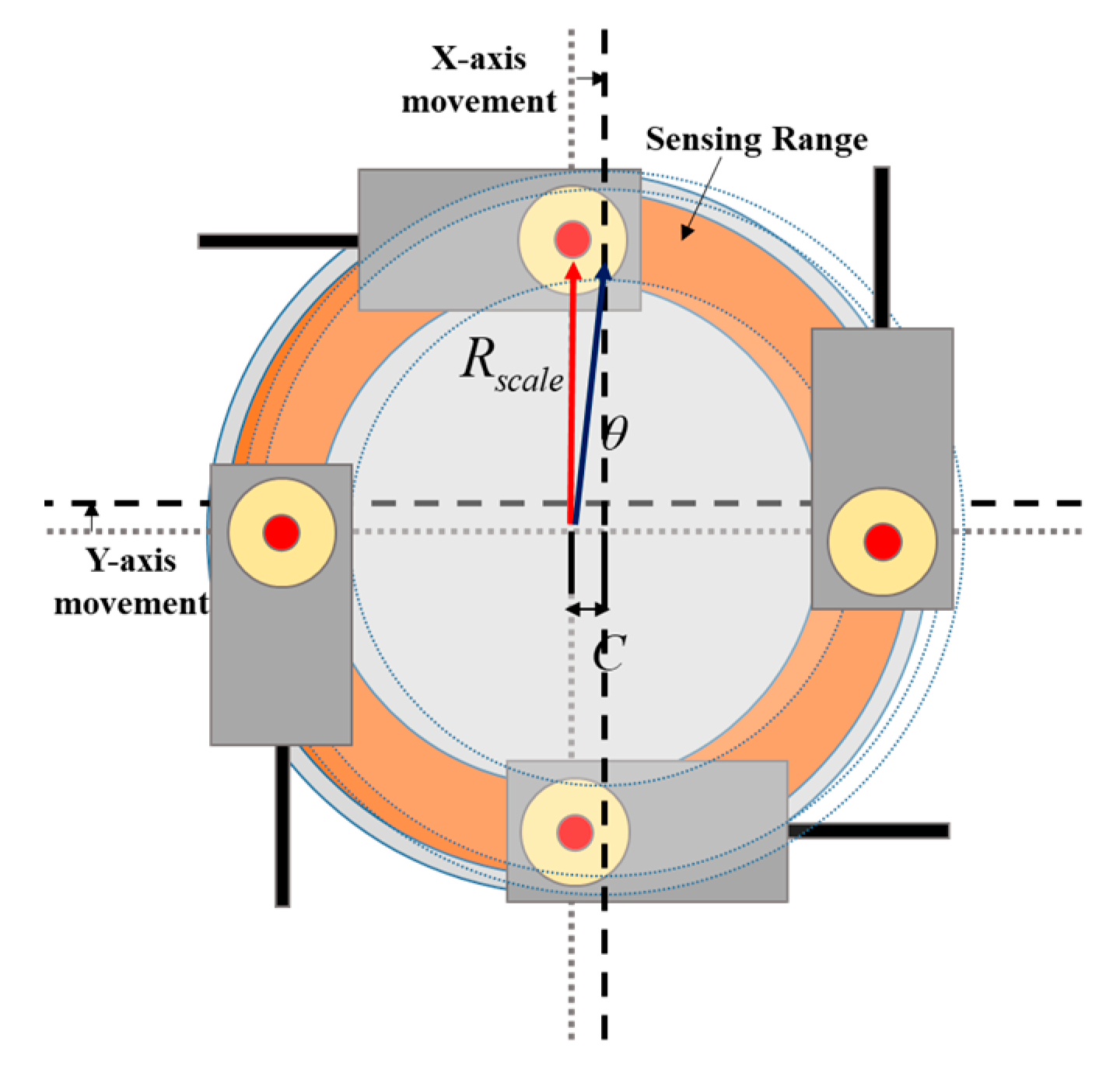
3.5. Control of the X–Y Axis Movement
3.6. Method of Rotation and X–Y Axis Movement Sensing Using Non-Contact Encoders
3.7. Integrated Driving Control of 6-DEF Magnetic Levitation System
4. Control Simulation of 6-DOF Magnetic Levitation System
4.1. Six Degrees of Freedom Magnetic Levitation System Modeling
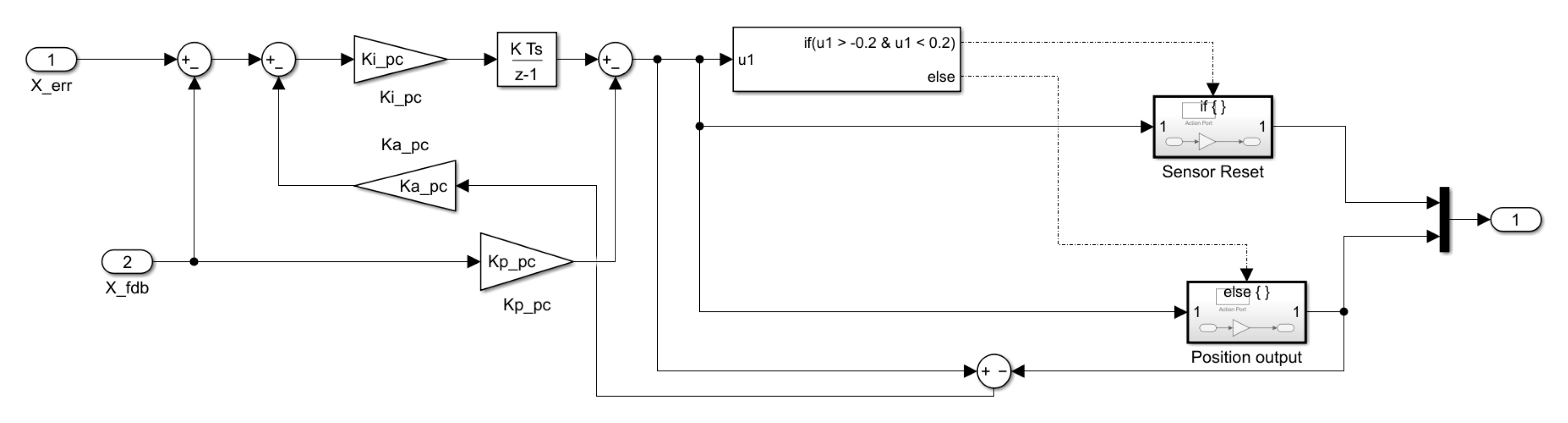
4.2. Control Algorithm Modeling for Out-of-Control and Derailment Prevention
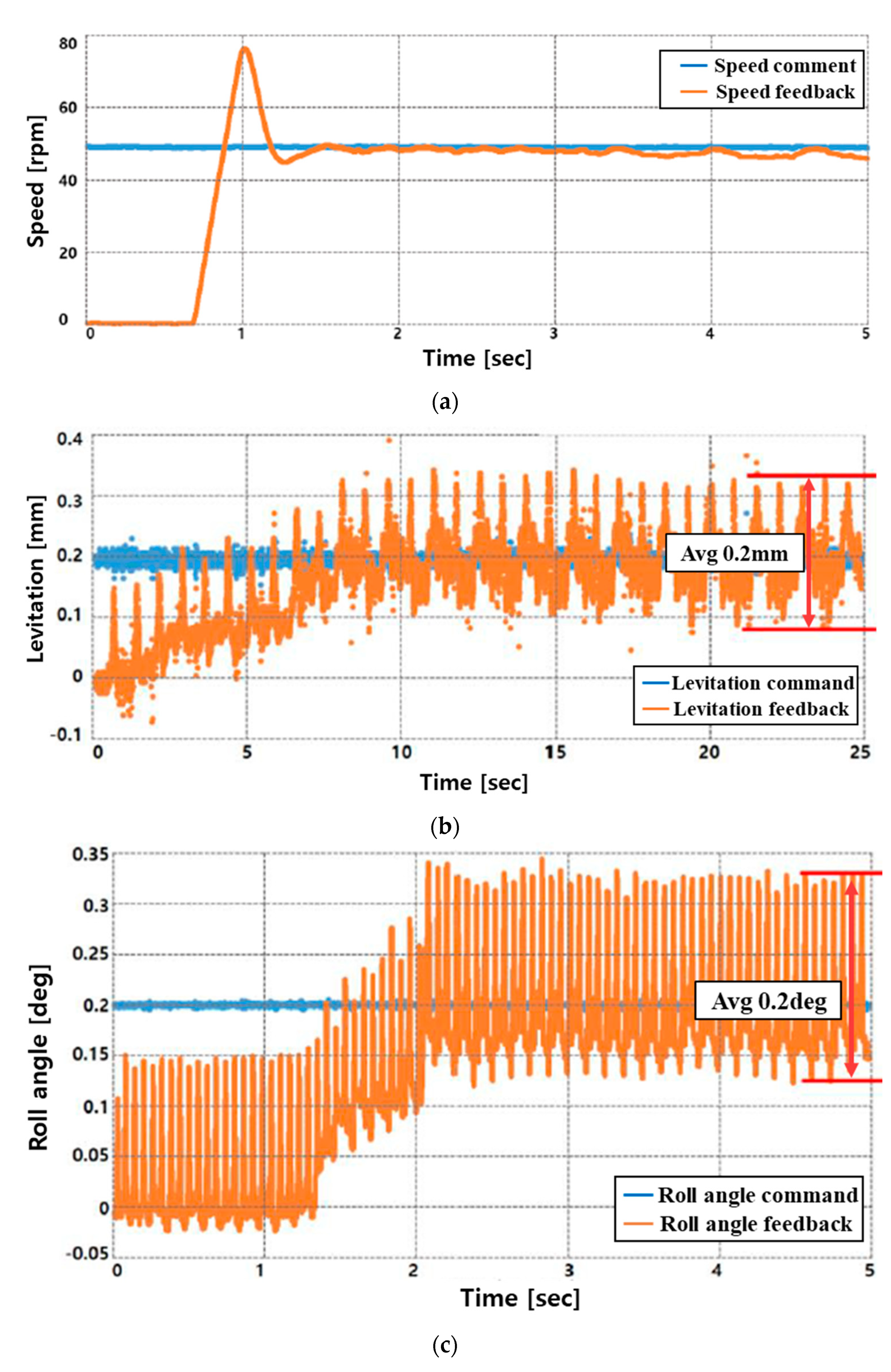
4.3. 6-DOF Magnetic Levitation System Simulation Result
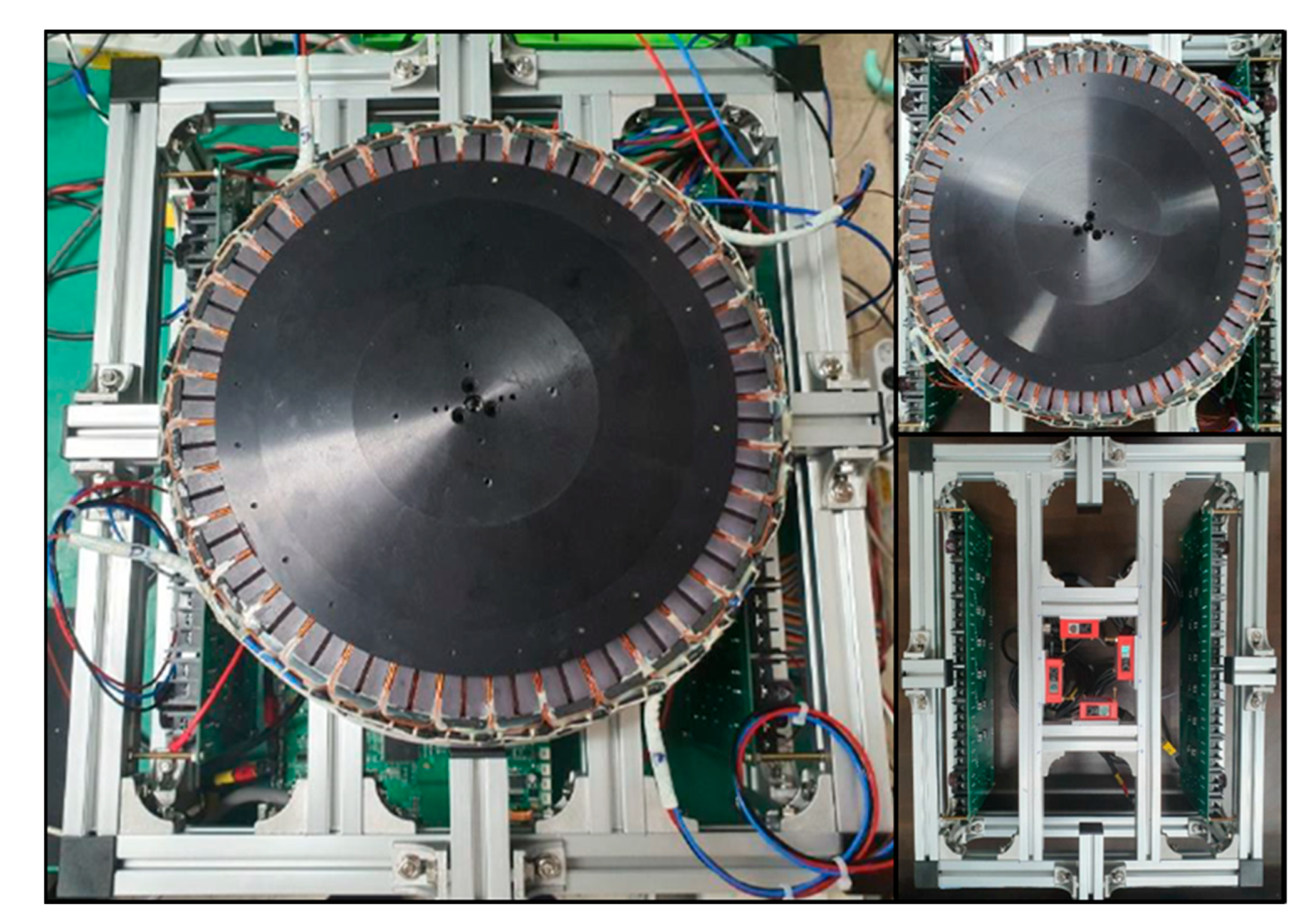
5. Performance Test of 6-DOF Magnetic Levitation System Using a Prototype
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- De Almeida, A.T.; Ferreira, F.J.T.E.; Andre, Q. Technical and Economical Considerations on Super High-Efficiency Three-Phase Motors. IEEE Trans. Ind. Appl. 2014, 50, 1274–1285. [Google Scholar] [CrossRef]
- Ko, W.; Ham, C. A Novel Approach to Analyze the Transient Dynamics of an Electrodynamics Suspension Maglev. IEEE Trans. Magn. 2007, 43, 2603–2605. [Google Scholar] [CrossRef]
- Sun, L.; Zhao, K.; Kou, B. An Electromagnetic Launcher with Magnetic Levitation Realized Based on Vector Control. IEEE Trans. Magn. 2009, 45, 467–470. [Google Scholar]
- Hwang, S.-H.; Kim, J.-M.; Bang, D.-J.; Kim, J.-W.; Koo, D.-H.; Kang, D.-H. Control of independent multi-phase transverse flux linear synchronous motor based on magnetic levitation. In Proceedings of the 2014 IEEE Applied Power Electronics Conference and Exposition—APEC 2014, Fort Worth, TX, USA, 16–20 March 2014. [Google Scholar]
- Kobayashi, S.; Ooshima, M.; Uddin, M.N. A Radial Position, Control Method of Bearingless Motor Based on d–q-Axis Current Control. IEEE Trans. Ind. Appl. 2013, 49, 1827–1835. [Google Scholar] [CrossRef]
- Lee, H.-I.; Yoo, S.-Y.; Noh, M.D. Toroidally-Wound Self-Bearing BLDC Motor with Lorentz Force. IEEE Trans. Magn. 2010, 46, 2148–2151. [Google Scholar] [CrossRef]
- Cao, J.; Zhu, Y.; Yin, W.; Xu, W. Electromagnetic Forces Acting on the Planar Armature of a Core-Type Synchronous Permanent-Magnet Planar Motor. IEEE Trans. Magn. 2009, 45, 3145–3150. [Google Scholar]
- Park, Y. Design and implementation of an electromagnetic levitation system for active magnetic bearing wheels. IET Control Theory Appl. 2014, 8, 139–148. [Google Scholar] [CrossRef]
- Ribani, P.; Urbano, N. Study on figure-eight-shaped coil electrodynamic suspension magnetic levitation systems without cross-connection. IEEE Trans. Magn. 2000, 36, 358–365. [Google Scholar] [CrossRef]
- Kim, Y.-K.; Jang, I.G.; Kim, Y.; Kim, K.-S.; Kim, S. Structural Optimization of a Novel 6-DOF Pose Sensor System for Enhancing Noise Robustness at a Long Distance. IEEE Trans. Ind. Electron. 2014, 61, 5622–5631. [Google Scholar]
- Kim, Y.-K.; Kim, K.-S.; Kim, S. A Portable and Remote 6-DOF Pose Sensor System with a Long Measurement Range Based on 1-D Laser Sensors. IEEE Trans. Ind. Electron. 2015, 62, 5722–5729. [Google Scholar] [CrossRef]
- Pan, Q.; Huang, F.; Chen, J.; He, L.G.; Li, W.; Feng, Z. High-Speed Low-Friction Piezoelectric Motors Based On Centrifugal Force. IEEE Trans. Ind. Electron. 2017, 64, 2158–2167. [Google Scholar] [CrossRef]
- Chen, L.; Hu, J.; Zhu, Y.; Du, S.; Chai, Y. A 6-DOF Measurement Solution for Permanent Magnet Synchronous Planar Motors Based on Motion Continuity Principle. IEEE Trans. Instrum. Meas. 2016, 65, 643–655. [Google Scholar] [CrossRef]













| System Classification | Variable Name | Value |
|---|---|---|
| Maglev Motor System Parameters | Number of System Magnet Poles | 24 |
| Rotor Weight [kg] | 1.33 | |
| Number of Stator Coils [Turn] | 15 | |
| Phase Resistance [Ω] Outer Diameter of Rotor [mm] | 11.5 261 | |
| Stator Outer Diameter [mm] | 316 | |
| System Controller Parameters | DC Link Voltage [V] | 60 |
| Phase Voltage Maximum [V] | ||
| Input Current Maximum [A] | 10 | |
| No Load Linkage Flux [Wb] | 0.02685 | |
| d-axis Inductance ( [mH] | 0.015 (1.7 (mH)) | |
| Current Controller Period [Hz] | 2 kHz | |
| -axis P gain | ||
| -axis I gain | ||
| Carrier Frequency | 0.00025 |
| Sensor Classification | Variable Name | Limit Value |
|---|---|---|
| Non−contact encoder sensor | Encoder X–Y axis sensing range [mm] | |
| Encoder Z-axis sensing range [mm] | 2.25~2.55 | |
| Gap Sensor | Gap Sensing range [mm] | 50~100 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jung, D.-H.; Lim, J.S. A Study on the Control Method of 6-DOF Magnetic Levitation System Using Non-Contact Position Sensors. Sensors 2023, 23, 905. https://doi.org/10.3390/s23020905
Jung D-H, Lim JS. A Study on the Control Method of 6-DOF Magnetic Levitation System Using Non-Contact Position Sensors. Sensors. 2023; 23(2):905. https://doi.org/10.3390/s23020905
Chicago/Turabian StyleJung, Dong-Hoon, and Jong Suk Lim. 2023. "A Study on the Control Method of 6-DOF Magnetic Levitation System Using Non-Contact Position Sensors" Sensors 23, no. 2: 905. https://doi.org/10.3390/s23020905
APA StyleJung, D.-H., & Lim, J. S. (2023). A Study on the Control Method of 6-DOF Magnetic Levitation System Using Non-Contact Position Sensors. Sensors, 23(2), 905. https://doi.org/10.3390/s23020905






