A Method for the Precise Coordinate Determination of an Inaccessible Location
Abstract
:1. Introduction
- The problem: to obtain precise geocentric coordinates of inaccessible locations for photogrammetry or LiDAR survey.
- A method is proposed based on the directional intersection of the inaccessible locations using a total station from two stations with measured GNSS coordinates.
- Solution: the non-linear directional intersection observation equations are formed.
- The equally weighted least-squares solution of the non-linear observational equations (LMA) is calculated.
- Linearisation of observation equations and formulation of the Gauss–Helmert model (GHM) is carried out.
- A solution of the GHM (the weighted least-squares method) is obtained.
- Field experiments are carried out. The results of the LMA and GHM are compared.
2. Materials and Methods
2.1. Method
2.1.1. Basic Relationships
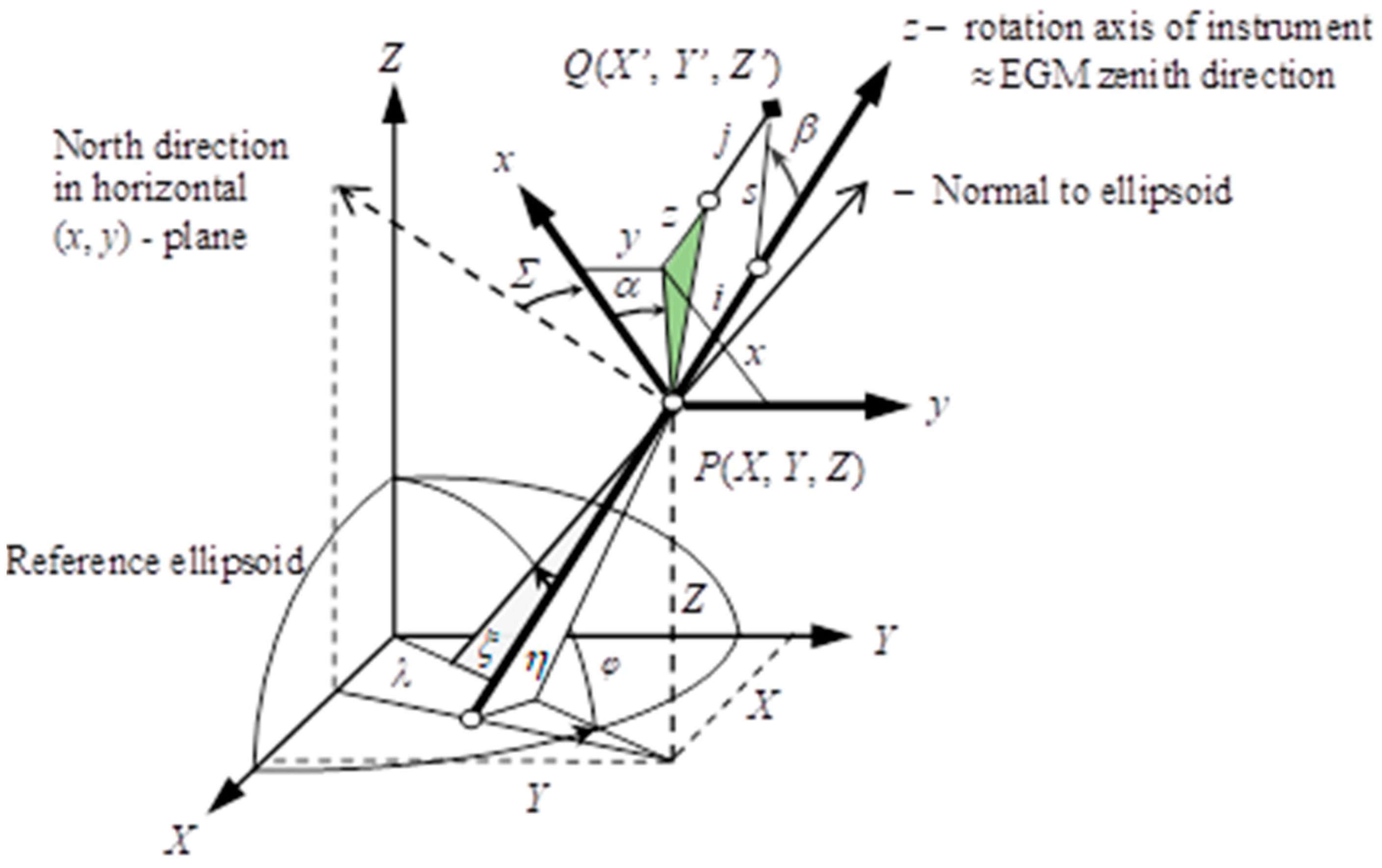
2.1.2. Least-Squares Solution of the Intersection Equations
2.1.3. Differential Relationships
2.1.4. LSQ Adjustment of the Intersection Based on the Gauss–Helmert Model
2.2. Experimental Design
2.3. Data
- Both experiments used a Leica Nova MS50 total station to measure the angles and distances (s, α, β). The heights of the instrument (i) and reflector (j) were also measured. The measurements obtained during the first experiment are listed in Table 1. The symbol ”A” denotes an inaccessible point, while 1 and 2 denote sites of the total stations from which measurements were carried out.
- The geocentric coordinates (X, Y, Z) of points 1 and 2 were measured using the Leica Viva GS08plus SmartAntenna GNSS receiver. The ASG-EUPOS service (https://www.asgeupos.pl/index.php, accessed on 25 September 2023) provided the RTK correction. The geocentric GNSS coordinates are listed in Table 2.
- The north-south ξ and east-west η components of the deflection of the vertical axis of the instrument, relative to the normal to ellipsoid, can be computed from an Earth Gravity Model, for example, EGM 2008 (https://www.usna.edu/Users/oceano/pguth/md_help/html/egm96.htm, accessed on 25 September 2023). The computations are based on the fundamental relations of the physical geodesy:ξ = Φ − φ,η = (Λ − λ) cos φwhereφ and λ are the latitude and longitude obtained from GNSS, andΦ and Λ are the astronomical latitude and longitude, respectively.
3. Results
3.1. Experiment 1
3.1.1. The Levenberg–Marquardt Algorithm (LMA) Adjustment
3.1.2. The Gauss–Helmert Model (GHM)
3.2. Experiment 2
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hofmann-Wallenhoff, B.; Lichtenegger, H.; Wasle, E. GNSS—Global Navigation Satellite Systems, GPS, GLONASS, GALILEO and More; Spinger: Wien, NY, USA, 1993; pp. 280–283. ISBN 978-3-211-73012-6. [Google Scholar]
- Skorupa, B. Wyznaczenie Współrzędnych Punktów Niedostępnych na Podstawie Opracowania Pomiarów Klasycznych i Sygnałów GPS, z Uwzględnieniem Wpływu Odchylenia Pionu, Geodezja T. 3; UWND AGH: Kraków, Poland, 1993; pp. 47–51. [Google Scholar]
- Osada, E.; Owczarek-Wesołowska, M.; Ficner, M.; Kurpiński, G. TotalStation/GNSS/EGM integrated geocentric positioning method. Surv. Rev. 2017, 49, 206–211. [Google Scholar] [CrossRef]
- Karsznia, K. Methods of adjustment and integration of spatial tacheometric traverses in connection to the GPS points and the geoid. In Zeszyty Naukowe Akademii Rolniczej we Wrocławiu; Geodezja i Urządzenia Rolne: Wrocław, Poland, 2004; pp. 69–87. [Google Scholar]
- Karsznia, K. A concept of surveying and adjustment of spatial tacheomoetric traverses in the applications of integrated geodesy. Acta Sci. Pol. Geod. Descr. Terr. 2008, 7, 35–46. [Google Scholar]
- Brown, N.; Kaloustian, S.; Roeckle, M. Monitoring of Open Pit Mines Using Combined GNSS Satellite Receivers and Robotic Total Stations, Slope Stability; Potvin, Y., Ed.; Australian Centre for Geomechanics: Perth, Australia, 2007; ISBN 978-0-9756756-8-7. Available online: https://papers.acg.uwa.edu.au/p/708_27_Brown (accessed on 14 September 2023).
- Yang, D.; Zou, J. Precise levelling in crossing river over 5 km using total station and GNSS. Sci. Rep. 2021, 11, 7492. [Google Scholar] [CrossRef] [PubMed]
- Borowski, L.; Pienko, M.; Wielgosz, P. Evaluation of Inventory Surveying of Façade Scaffolding Conducted During ORKWIZ Project. In Proceedings of the 2017 Baltic Geodetic Congress (BGC Geomatics), Gdansk, Poland, 22–25 June 2017; pp. 189–192. [Google Scholar] [CrossRef]
- Karsznia, K.; Osada, E. Photogrammetric Precise Surveying Based on the Adjusted 3D Control Linear Network Deployed on a Measured Object. Appl. Sci. 2022, 12, 4571. [Google Scholar] [CrossRef]
- Osada, E.; Owczarek-Wesołowska, M.; Sośnica, K. Gauss–Helmert Model for Total Station Positioning Directly in Geocentric Reference Frame Including GNSS Reference Points and Vertical Direction from Earth Gravity Model. J. Surv. Eng. 2019, 145, 04019013. [Google Scholar] [CrossRef]
- Kahmen, H.; Faig, W. Surveying; De Gruyter: Berlin, Germany; New York, NY, USA, 1988; pp. 439–460. ISBN 3-11-008303-5. [Google Scholar]
- Kahmen, H. Angewandte Geodäsie: Vermessungskunde; De Gruyter: Berlin, Germany; New York, NY, USA, 2020. [Google Scholar] [CrossRef]
- Ghilani, C.D.; Wolf, P.R. Elementary Surveying. In An Introduction to Geomatics; Pearson Education, Inc.: Upper Saddle River, NJ, USA, 2012; pp. 231–275. ISBN 978-0-13-255434-3. [Google Scholar]
- Cederholm, P.; Jensen, K. GPS Measurement of Inaccessible Detail Points. Surv. Rev. 2013, 41, 352–363. [Google Scholar] [CrossRef]
- Ai, S.; Wang, S.; Li, Y.; Liu, L. Multi-parameter adjustment for high-precision azimuthal intersection positioning. MethodsX 2020, 7, 100968. [Google Scholar] [CrossRef]
- Koch, K.-R. Robust estimations for the nonlinear Gauss Helmert model by the expectation maximization algorithm. J. Geodesy 2014, 88, 263–271. [Google Scholar] [CrossRef]
- Awange, J.L.; Grafarend, E.W.; Paláncz, B.; Zaletnyik, P. Positioning by intersection methods. In Algebraic Geodesy and Geoinformatics; Springer: Berlin/Heidelberg, Germany, 2010; pp. 249–263. [Google Scholar] [CrossRef]
- Wolf, H. Das geodätische Gauß-Helmert-Modell und seine Eigenschaften. Z. Für Vermess. 1978, 103, 41–43. [Google Scholar]
- Schaffrin, B.; Wieser, A. On weighted total least-squares adjustment for linear regression. J. Geodesy 2008, 82, 415–421. [Google Scholar] [CrossRef]
- Schaffrin, B.; Wieser, A. Total least-squares adjustment of condition equations. Stud. Geophys. Geod. 2011, 55, 529–536. [Google Scholar] [CrossRef]
- Snow, K. Topics in Total Least-Squares Adjustment within the Errors-In-Variables Model: Singular Cofactor Matrices and Prior Information. In The Ohio State University Columbus, Ohio, Report No. 502; Ohio State University: Columbus, OH, USA, 2012; pp. 23–39. Available online: https://kb.osu.edu/bitstream/handle/1811/78619/1/SES_GeodeticScience_Report_502.pdf (accessed on 27 September 2023).
- Chang, G. On least-squares solution to 3D similarity transformation problem under Gauss–Helmert model. J. Geod. 2015, 89, 573–576. [Google Scholar] [CrossRef]
- Zeng, W.; Fang, X.; Lin, Y.; Huang, X.; Yao, Y. On the errors-in-variables model with inequality constraints of dependent variables for geodetic transformation. Surv. Rev. 2017, 51, 166–171. [Google Scholar] [CrossRef]
- Marx, C. A weighted adjustment of a similarity transformation between two point sets containing errors. J. Geéod. Sci. 2017, 7, 105–112. [Google Scholar] [CrossRef]
- Kanatani, K.; Niitsuma, H. Optimal computation of 3D similarity: Gauss–Newton vs. Gauss–Helmert. Comput. Stat. Data Anal. 2012, 56, 4470–4483. [Google Scholar] [CrossRef]
- Neitzel, F.; Schaffrin, B. On the Gauss–Helmert model with a singular dispersion matrix where BQ is of smaller rank than B. J. Comput. Appl. Math. 2016, 291, 458–467. [Google Scholar] [CrossRef]
- Paláncz, B.; Awange, J.L.; Völgyesi, L. Pareto optimality solution of the Gauss-Helmert model. Acta Geod. Geophys. 2013, 48, 293–304. [Google Scholar] [CrossRef]
- Koch, K.R. Parameter Estimation and Hypothesis Testing in Linear Models; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2003; pp. 210–231. ISBN 3-540-65257-4. [Google Scholar]
- Karsznia, K.; Osada, E.; Muszyński, Z. Real-Time Adjustment and Spatial Data Inte-gration Algorithms Combining Total Station and GNSS Surveys with an Earth Gravity Model. Appl. Sci. 2023, 13, 9380. [Google Scholar] [CrossRef]
- Osada, E.; Karsznia, K.; Karsznia, I. Georeferenced measurements of building objects with their simultaneous shape detection. Surv. Rev. 2020, 52, 24–30. [Google Scholar] [CrossRef]
- Osada, E.; Sośnica, K.; Borkowski, A.; Owczarek-Wesołowska, M.; Gromczak, A. A Direct Georeferencing Method for Terrestrial Laser Scanning Using GNSS Data and the Vertical Deflection from Global Earth Gravity Models. Sensors 2017, 17, 1489. [Google Scholar] [CrossRef]
- Ranganathan, A. The Levenberg-Marquardt algorithm. Tutor. LM Algorithm 2004, 11, 101–110. [Google Scholar]
- Osada, E. Geodezyjne Pomiary Szczegółowe, 2nd ed.; UxLan: Wrocław, Poland, 2014; p. 398. [Google Scholar]
- Awange, J.L.; Grafarend, E.W. Solving Algebraic Computational Problems in Geodesy and Geoinformatics—The Answer to Modern Challenges; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2005; pp. 77–86. [Google Scholar]
- Awange, J.L. Partial Procrustes Solution of the Threedimensional Orientation Problem from GPS/LPS Observations. In Geodesy—The Challenge of the 3rd Millennium; Grafarend, E.W., Krumm, F.W., Schwarze, V.S., Eds.; Springer: Berlin/Heidelberg, Germany, 2003; pp. 277–286. Available online: https://link.springer.com/content/pdf/10.1007/978-3-662-05296-9.pdf (accessed on 15 August 2023).
- Nocedal, J.; Wright, S.J. Numerical Optimisation; Springer Science+Business Media, LLC: New York, NY, USA, 2006; pp. 101–133. ISBN 0-387-30303-0. [Google Scholar]
- Heiskanen, W.A.; Moritz, H. Physical Geodesy; W.H. Freeman and Company: San Francisco, CA, USA; London, UK, 1967; pp. 46–123. [Google Scholar]
- Hofmann-Wellenhof, B.; Moritz, H. Physical Geodesy; Springer: Wien, Germany; New York, NY, USA, 2006; pp. 173–233. ISBN 3-211-33544-7. [Google Scholar]
- Petit, G.; Luzum, B. IERS Conventions 2010, Frankfurt am Main: Verlag des Bundesamtes für Kartographie und Geodäsie. IERS Technical Note 36. 2010. Available online: https://www.iers.org/SharedDocs/Publikationen/EN/IERS/Publications/tn/TechnNote36/tn36.pdf (accessed on 27 September 2023).
- Hirt, C.; Marti, U.; Bürki, B.; Featherstone, W.E. Assessment of EGM2008 in Europe using accurate astrogeodetic vertical deflections and omission error estimates from SRTM/DTM2006.0 residual terrain model data. J. Geophys. Res. Atmos. 2010, 115, B10404. [Google Scholar] [CrossRef]
- Pavlis, N.K.; Holmes, S.A.; Kenyon, S.C.; Factor, J.K. The development and evaluation of the Earth Gravitational Model 2008 (EGM2008). J. Geophys. Res. Solid Earth 2012, 117, B04406. [Google Scholar] [CrossRef]
- Ogundare, J.O. Precision Surveying: The Principles and Geomatics Practice; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2015; pp. 237–267. ISBN 978-1-119-10251-9. [Google Scholar]

| Observation | s (m) | α (gon) | β (gon) | i (m) | j (m) |
|---|---|---|---|---|---|
| 1 to 2 | 37.121 | 0.0489 | 100.1286 | 1.611 | 1.500 |
| 1 to A | 43.571 | 339.2618 | 65.1532 | 1.611 | 2.150 |
| 2 to 1 | 37.124 | 71.5203 | 100.2894 | 1.635 | 1.500 |
| 2 to A | 40.953 | 141.2695 | 62.7610 | 1.635 | 2.150 |
| Std Dev. | 0.006 | 0.001 | 0.001 | 0.001 | 0.001 |
| Coordinate | 1 | 2 | A | Std Dev. |
|---|---|---|---|---|
| X (m) | 3,835,779.346 | 3,835,758.231 | 3,835,763.321 | 0.008 |
| Y (m) | 1,177,321.994 | 1,177,351.033 | 1,177,324.809 | 0.008 |
| Z (m) | 4,941,536.189 | 4,941,545.624 | 4,941,576.310 | 0.008 |
| Deflection of Vertical | 1 | 2 | Std Dev. |
|---|---|---|---|
| ξ (″) | 5.9926 | 5.9852 | 1 |
| η (″) | 6.2033 | 6.1967 | 1 |
| Observation | s (m) | α (gon) | β (gon) | i (m) | j (m) |
|---|---|---|---|---|---|
| 3 to 4 | 33.007 | 31.8735 | 100.4359 | 1.685 | 1.500 |
| 3 to B | 40.753 | 107.2129 | 63.2411 | 1.685 | 1.900 |
| 3 to C | 43.474 | 94.5445 | 65.8200 | 1.685 | 1.900 |
| 3 to D | 47.413 | 85.4091 | 68.9707 | 1.685 | 1.900 |
| 4 to 3 | 33.009 | 225.3364 | 100.2523 | 1.657 | 1.500 |
| 4 to B | 43.612 | 161.3899 | 65.8203 | 1.657 | 1.900 |
| 4 to C | 40.228 | 149.3560 | 62.6006 | 1.657 | 1.900 |
| 4 to D | 38.690 | 135.5829 | 60.9652 | 1.657 | 1.900 |
| Std Dev. | 0.006 | 0.001 | 0.001 | 0.001 | 0.001 |
| Point | 3 | 4 | B | C | D | Std Dev. |
|---|---|---|---|---|---|---|
| X (m) | 3,835,762.327 | 3,835,780.698 | 3,835,764.596 | 3,835,769.196 | 3,835,773.170 | 0.008 |
| Y (m) | 1,177,338.201 | 1,177,311.976 | 1,177,313.716 | 1,177,307.830 | 1,177,302.003 | 0.008 |
| Z (m) | 4,941,545.590 | 4,941,537.594 | 4,941,577.938 | 4,941,575.760 | 4,941,574.056 | 0.008 |
| Unknown | s(1–A) | s(2–A) | Σ1 | Σ2 |
|---|---|---|---|---|
| 43.572 m | 40.974 m | 73.4638 gon | 201.9942 gon | |
| Coordinates of A | ||||
| X (m) | 3,835,763.325 | |||
| Y (m) | 1,177,324.803 | |||
| Z (m) | 4,941,576.312 | |||
| Unknown | s(1–A) | s(2–A) | Σ1 | Σ2 |
|---|---|---|---|---|
| 43.576 m | 40.966 m | 73.4693 gon | 201.9980 gon | |
| Coordinates of A | ||||
| X (m) | 3,835,763.322 | |||
| Y (m) | 1,177,324.807 | |||
| Z (m) | 4,941,576.311 | |||
| Model | dX (m) | dy (m) | dz (m) |
|---|---|---|---|
| LMA | −0.004 | 0.007 | −0.002 |
| GHM | 0.002 | −0.002 | 0.001 |
| Target | δX (m) | δY (m) | δZ (m) | (m) |
|---|---|---|---|---|
| B | −0.006 | −0.001 | 0.006 | 0.008 |
| C | 0.003 | −0.003 | 0.009 | 0.010 |
| D | −0.003 | −0.013 | −0.003 | 0.014 |
| RMSE | 0.004 | 0.008 | 0.006 | - |
| Coordinates of A | |
|---|---|
| X (m) | 3,835,763.324 |
| Y (m) | 1,177,324.807 |
| Z (m) | 4,941,576.311 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Osada, E.; Owczarek-Wesołowska, M.; Karsznia, K.; Becek, K.; Muszyński, Z. A Method for the Precise Coordinate Determination of an Inaccessible Location. Sensors 2023, 23, 8199. https://doi.org/10.3390/s23198199
Osada E, Owczarek-Wesołowska M, Karsznia K, Becek K, Muszyński Z. A Method for the Precise Coordinate Determination of an Inaccessible Location. Sensors. 2023; 23(19):8199. https://doi.org/10.3390/s23198199
Chicago/Turabian StyleOsada, Edward, Magdalena Owczarek-Wesołowska, Krzysztof Karsznia, Kazimierz Becek, and Zbigniew Muszyński. 2023. "A Method for the Precise Coordinate Determination of an Inaccessible Location" Sensors 23, no. 19: 8199. https://doi.org/10.3390/s23198199
APA StyleOsada, E., Owczarek-Wesołowska, M., Karsznia, K., Becek, K., & Muszyński, Z. (2023). A Method for the Precise Coordinate Determination of an Inaccessible Location. Sensors, 23(19), 8199. https://doi.org/10.3390/s23198199







