Spatially Modulated Fiber Speckle for High-Sensitivity Refractive Index Sensing
Abstract
1. Introduction
2. Fiber Speckle Sensing Principle
2.1. Fiber Speckle Theory
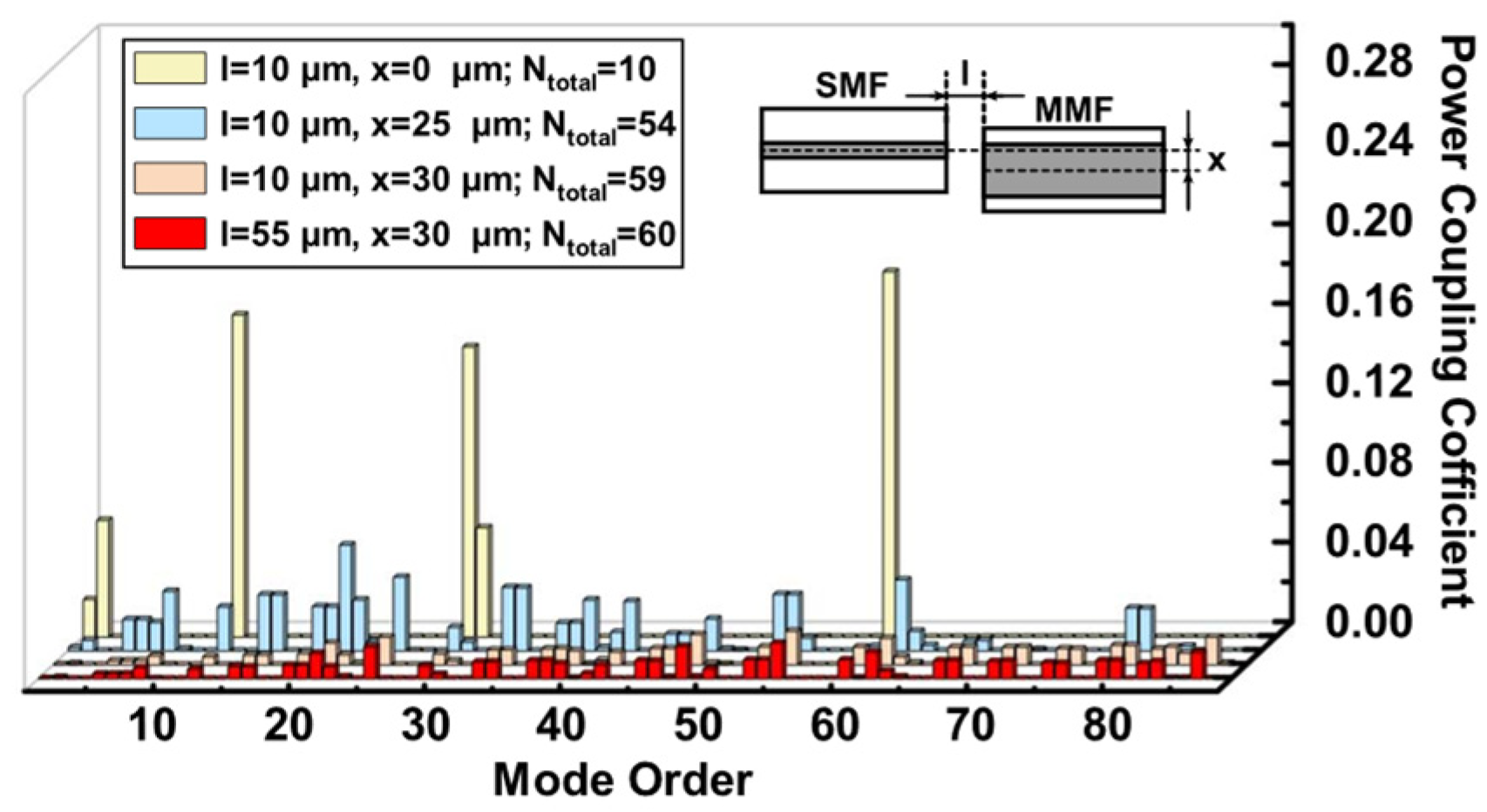
2.2. Mode Excitation in MMF
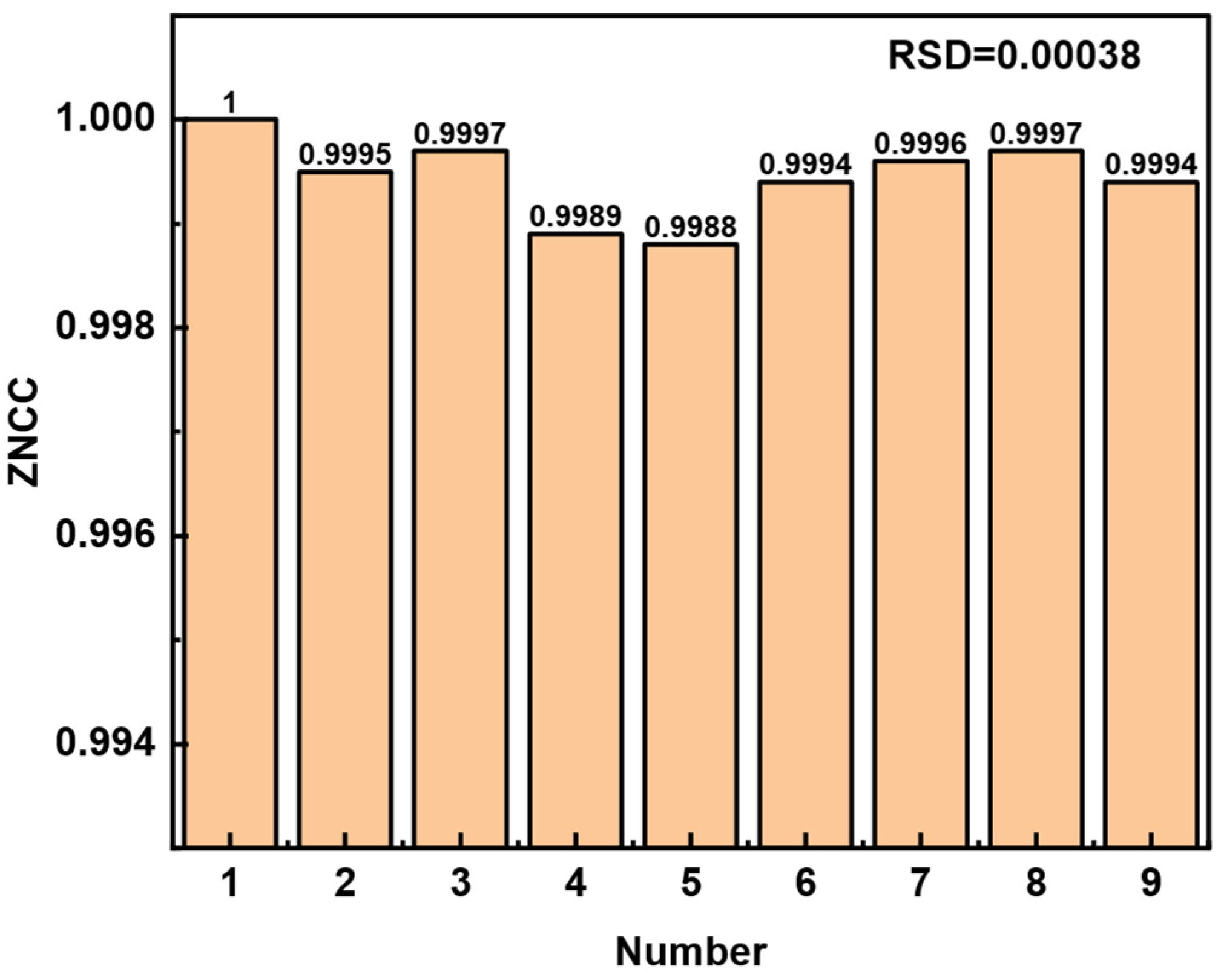
2.3. Digital Image Demodulation
3. Experimental Investigation and Results
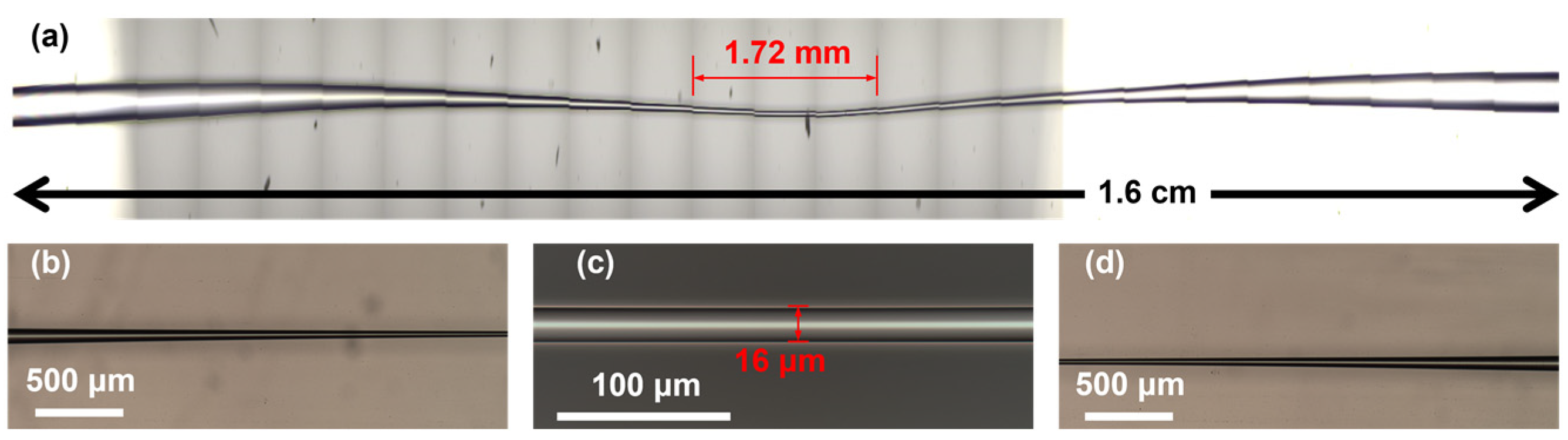
3.1. Experimental Setup
3.2. Mode Excitation and RI Measurement
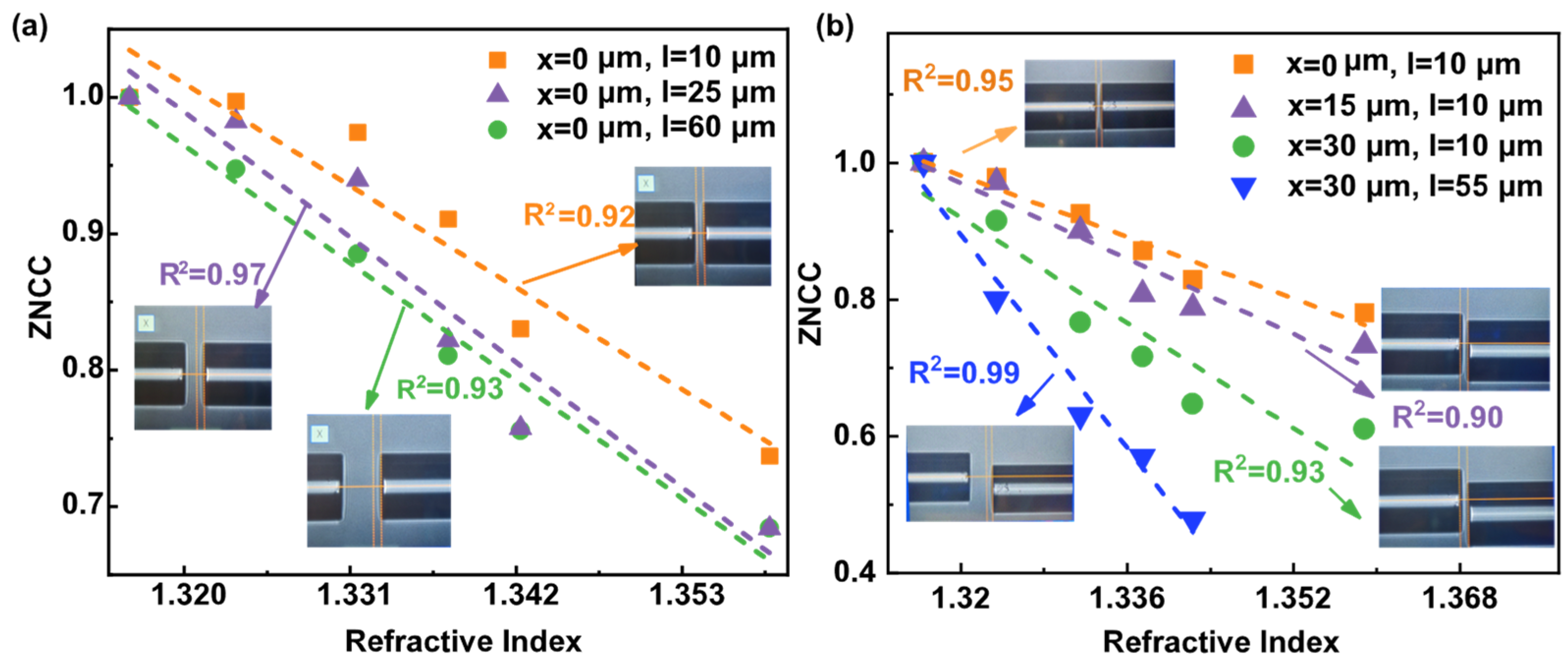
3.3. Investigation of FSS Sensitivity
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, H.; Hu, D.J.J.; Sun, Q.; Wei, L.; Li, K.; Liao, C.; Li, B.; Zhao, C.; Dong, X.; Tang, Y.; et al. Specialty optical fibers for advanced sensing applications. Opto-Electron. Sci. 2023, 2, 220025. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Z.; Tan, S.; Fang, F.; Yang, L.; Yuan, B.; Sun, Q. Recent advances in microfiber sensors for highly sensitive biochemical detection. J. Phys. D-Appl. Phys. 2019, 52, 493002. [Google Scholar] [CrossRef]
- Caucheteur, C.; Villatoro, J.; Liu, F.; Loyez, M.; Guo, T.; Albert, J. Mode-division and spatial-division optical fiber sensors. Adv. Opt. Photon. 2022, 14, 1–86. [Google Scholar] [CrossRef]
- Rahimi, A.; Honarvar, B.; Safari, M. The role of salinity and aging time on carbonate reservoir in low salinity seawater and smart seawater flooding. J. Pet. Sci. Eng. 2020, 187, 106739. [Google Scholar] [CrossRef]
- Majozi, N.P.; Salama, M.S.; Bernard, S.; Harper, D.M.; Habte, M.G. Remote sensing of euphotic depth in shallow tropical inland waters of Lake Naivasha using MERIS data. Remote Sens. Environ. 2014, 148, 178–189. [Google Scholar] [CrossRef]
- Gu, L.J.; He, X.G.; Zhang, M.; Lu, H.L. Advances in the Technologies for Marine Salinity Measurement. J. Mar. Sci. Eng. 2022, 10, 2024. [Google Scholar] [CrossRef]
- Li, X.; Nguyen, L.V.; Becker, M.; Ebendorff-Heidepriem, H.; Pham, D.; Warren-Smith, S.C. Simultaneous Measurement of Temperature and Refractive Index Using an Exposed Core Microstructured Optical Fiber. IEEE J. Sel. Top. Quantum Electron. 2020, 26, 5600107. [Google Scholar] [CrossRef]
- Xue, P.; Yu, F.D.; Wu, B.C.; Bao, H.Y.; Zheng, J. Investigation of a D-Shaped Plastic Optical Fiber Assisted by a Long Period Grating for Refractive Index Sensing. IEEE Sens. J. 2020, 20, 842–847. [Google Scholar] [CrossRef]
- Sui, P.X.; Zhang, A.L.; Pan, F.; Chang, P.X.; Pan, H.G.; Liu, F.; Wang, J.F.; Cao, C.B. High sensitivity refractive index sensor with wide detection range and high linearity based on LSPR in hollow-core anti-resonance fiber. Opt. Laser Technol. 2022, 155, 108427. [Google Scholar] [CrossRef]
- Pang, F.F.; Liu, H.H.; Chen, N.; Liu, Y.Q.; Zeng, X.L.; Chen, Z.Y.; Wang, T.Y. Cladding-mode resonance of a double-cladding fiber at a near modal cut-off wavelength for RI sensing. Meas. Sci. Technol. 2010, 21, 094028. [Google Scholar] [CrossRef]
- Yao, T.J.; Pu, S.L.; Zhao, Y.L.; Li, Y.Q. Ultrasensitive refractive index sensor based on parallel-connected dual Fabry-Perot interferometers with Vernier effect. Sens. Actuator A-Phys. 2019, 290, 14–19. [Google Scholar] [CrossRef]
- Yu, F.D.; Liu, B.S.; Zou, Q.Y.; Xue, P.; Zheng, J. Influence of temperature on the refractive index sensor based on a core-offset in-line fiber Mach-Zehnder interferometer. Opt. Fiber Technol. 2020, 58, 102293. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Zhou, A.; Qin, B.Y.; Deng, H.C.; Liu, Z.H.; Yang, J.; Yuan, L.B. Refractive Index Sensing Characteristics of Single-Mode Fiber-Based Modal Interferometers. J. Light. Technol. 2014, 32, 1734–1740. [Google Scholar] [CrossRef]
- Qian, Y.; Zhao, Y.; Wu, Q.L.; Yang, Y. Review of salinity measurement technology based on optical fiber sensor. Sens. Actuator B-Chem. 2018, 260, 86–105. [Google Scholar] [CrossRef]
- Zhang, A.Y.; Liu, Z.; Tu, Q.Q.; Ma, Q.C.; Zeng, H.R.; Deng, Z.P.; Jiang, R.J.; Mo, Z.F.; Liu, J.T.; Xia, C.M.; et al. Trace detection of cadmium (II) ions based on an air-hole-assisted multicore microstructured optical fiber. Sens. Actuator B-Chem. 2022, 365, 131941. [Google Scholar] [CrossRef]
- Liu, L.H.; Zhang, X.J.; Zhu, Q.; Li, K.W.; Lu, Y.; Zhou, X.H.; Guo, T. Ultrasensitive detection of endocrine disruptors via superfine plasmonic spectral combs. Light-Sci. Appl. 2021, 10, 181. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Wang, M.Y.; Zhu, N.S.; Han, B.; Liu, Y.X. Optical fiber hydrogen sensor based on self-assembled PDMS/Pd-WO3 microbottle resonator. Sens. Actuator B-Chem. 2023, 375, 132866. [Google Scholar] [CrossRef]
- Wang, H.R.; Luo, J.; Dai, W.Y.; Chen, S.J.; Fu, H.Y. A Differential Intensity-Modulated Refractive Index Sensor Using a Droplet-Like Fiber Cascaded With FBGs. J. Light. Technol. 2022, 40, 3098–3103. [Google Scholar] [CrossRef]
- Leal, A.G.; Frizera, A.; Marques, C.; Pontes, M.J. Optical Fiber Specklegram Sensors for Mechanical Measurements: A Review. IEEE Sens. J. 2020, 20, 569–576. [Google Scholar] [CrossRef]
- Liu, Y.; Qin, Q.; Liu, H.-h.; Tan, Z.-w.; Wang, M.-g. Investigation of an image processing method of step-index multimode fiber specklegram and its application on lateral displacement sensing. Opt. Fiber Technol. 2018, 46, 48–53. [Google Scholar] [CrossRef]
- Cai, Y.Z.; Liu, Y.; Li, G.D.; Qin, Q.; Pang, L.Z.; Ren, W.H.; Wei, J.; Wang, M.G. Reflective tactile sensor assisted by multimode fiber-based optical coupler and fiber specklegram. Opt. Laser Technol. 2023, 160, 109062. [Google Scholar] [CrossRef]
- Zhu, R.-z.; Wan, S.-j.; Xiong, Y.-f.; Feng, H.-g.; Chen, Y.; Lu, Y.-q.; Xu, F. Magnetic field sensing based on multimode fiber specklegrams. J. Light. Technol. 2021, 39, 3614–3619. [Google Scholar] [CrossRef]
- Cen, Q.Q.; Pian, S.J.; Liu, X.J.; Tang, Y.W.; He, X.Y.; Ma, Y.G. Microtaper leaky-mode spectrometer with picometer resolution. eLight 2023, 3, 9. [Google Scholar] [CrossRef]
- Feng, F.; Chen, W.; Chen, D.; Lin, W.; Chen, S.-C. In-situ ultrasensitive label-free DNA hybridization detection using optical fiber specklegram. Sens. Actuator B-Chem. 2018, 272, 160–165. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Lai, X.T.; Huang, H.L.; Wang, X.Y.; Li, H.R.; Chen, Z.Y.; Han, J.; Pu, J.X. Recognizing the orbital angular momentum (OAM) of vortex beams from speckle patterns. Sci. China-Phys. Mech. Astron. 2022, 65, 244211. [Google Scholar] [CrossRef]
- Arı, F.; Şerbetçi, H.; Navruz, İ. Tapered fiber optic refractive index sensor using speckle pattern imaging. Opt. Fiber Technol. 2023, 79, 103366. [Google Scholar] [CrossRef]
- Fujiwara, E.; da Silva, L.E.; Cabral, T.D.; de Freitas, H.E.; Wu, Y.T.; de Barros Cordeiro, C.M. Optical fiber specklegram chemical sensor based on a concatenated multimode fiber structure. J. Light. Technol. 2019, 37, 5041–5047. [Google Scholar] [CrossRef]
- Cabral, T.D.; Fujiwara, E.; Warren-Smith, S.C.; Ebendorff-Heidepriem, H.; Cordeiro, C.M.B. Multimode exposed core fiber specklegram sensor. Opt. Lett. 2020, 45, 3212–3215. [Google Scholar] [CrossRef]
- Mu, G.; Liu, Y.; Qin, Q.; Tan, Z.; Li, G.; Wang, M.; Yan, F. Refractive index sensing based on the analysis of D-shaped multimode fiber specklegrams. IEEE Photon. Technol. Lett. 2020, 32, 485–488. [Google Scholar] [CrossRef]
- Fujiwara, E.; Ri, Y.; Wu, Y.T.; Fujimoto, H.; Suzuki, C.K. Evaluation of image matching techniques for optical fiber specklegram sensor analysis. Appl. Opt. 2018, 57, 9845–9854. [Google Scholar] [CrossRef]
- Di Stefano, L.; Mattoccia, S.; Tombari, F. ZNCC-based template matching using bounded partial correlation. Pattern Recognit. Lett. 2005, 26, 2129–2134. [Google Scholar] [CrossRef]
- Karki, D.; Khanikar, T.; Naeem, K.; Lalam, N.; Ohodnicki, P. Optimization of the fourth self-imaging spectral response in magnetic fluid cladded-MMI fiber optic sensor for magnetometry. In Optical Waveguide and Laser Sensors II; SPIE: Bellingham, WA, USA, 2023; Volume 12532. [Google Scholar]
- Pan, B. Recent progress in digital image correlation. Exp. Mech. 2011, 51, 1223–1235. [Google Scholar] [CrossRef]






| Sensor Head | Sensitivity (RIU−1) | Resolution (RIU) | Response Range | Ref. |
|---|---|---|---|---|
| MMF-NCF-MMF | 18.70 | 5.35 × 10−4 | 1.33–1.36 | [27] |
| Exposed MMF | 10.97 | 4.60 × 10−4 | 1.33–1.375 Nonlinear | [28] |
| D-Shaped MMF | 10.57 | 2.12 × 10−5 | 1.40–1.44 | [29] |
| TMMF | 19.52 | 5.84 × 10−5 | 1.3164–1.3588 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, P.; Liu, H.; Zhou, Z.; Hu, J.; Wang, Y.; Peng, X.; Yuan, X.; Shu, Y.; Zhang, Y.; Dang, H.; et al. Spatially Modulated Fiber Speckle for High-Sensitivity Refractive Index Sensing. Sensors 2023, 23, 6814. https://doi.org/10.3390/s23156814
Guo P, Liu H, Zhou Z, Hu J, Wang Y, Peng X, Yuan X, Shu Y, Zhang Y, Dang H, et al. Spatially Modulated Fiber Speckle for High-Sensitivity Refractive Index Sensing. Sensors. 2023; 23(15):6814. https://doi.org/10.3390/s23156814
Chicago/Turabian StyleGuo, Penglai, Huanhuan Liu, Zhitai Zhou, Jie Hu, Yuntian Wang, Xiaoling Peng, Xun Yuan, Yiqing Shu, Yingfang Zhang, Hong Dang, and et al. 2023. "Spatially Modulated Fiber Speckle for High-Sensitivity Refractive Index Sensing" Sensors 23, no. 15: 6814. https://doi.org/10.3390/s23156814
APA StyleGuo, P., Liu, H., Zhou, Z., Hu, J., Wang, Y., Peng, X., Yuan, X., Shu, Y., Zhang, Y., Dang, H., Xu, G., Zhang, A., Xue, C., Hu, J., Shao, L., Chen, J., Li, J., & Shum, P. P. (2023). Spatially Modulated Fiber Speckle for High-Sensitivity Refractive Index Sensing. Sensors, 23(15), 6814. https://doi.org/10.3390/s23156814







