Semi-Coprime Array with Staggered Beam-Steering of Sub-Arrays
Abstract
1. Introduction
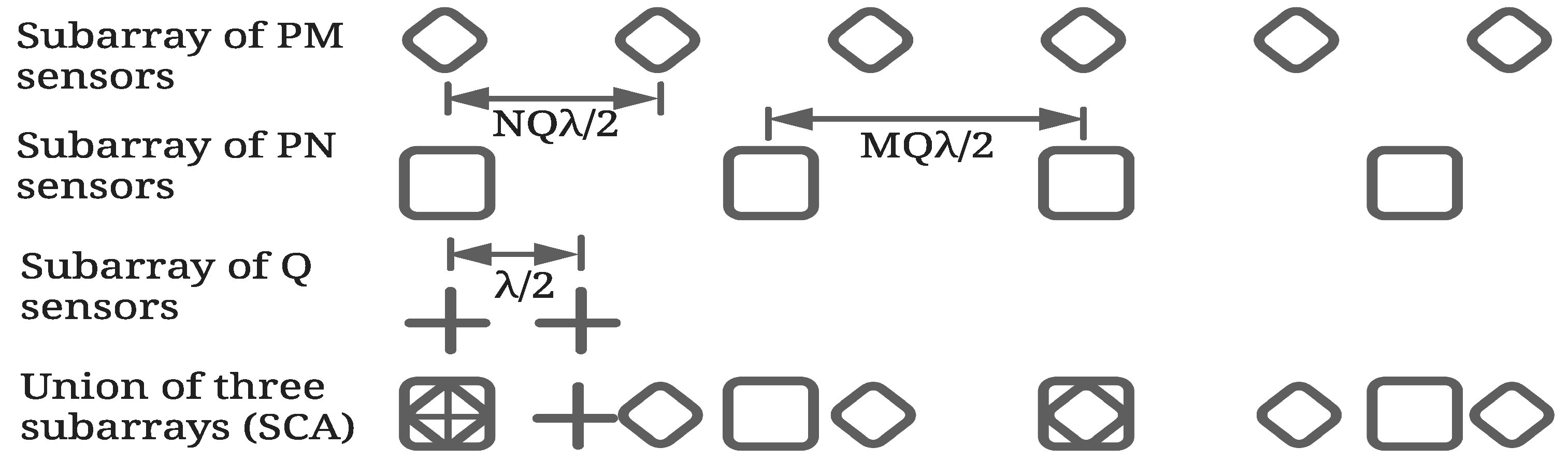
2. SCA: Basic and Proposed Forms
2.1. SCA
2.2. SCA with Staggered Steering (SCASS)
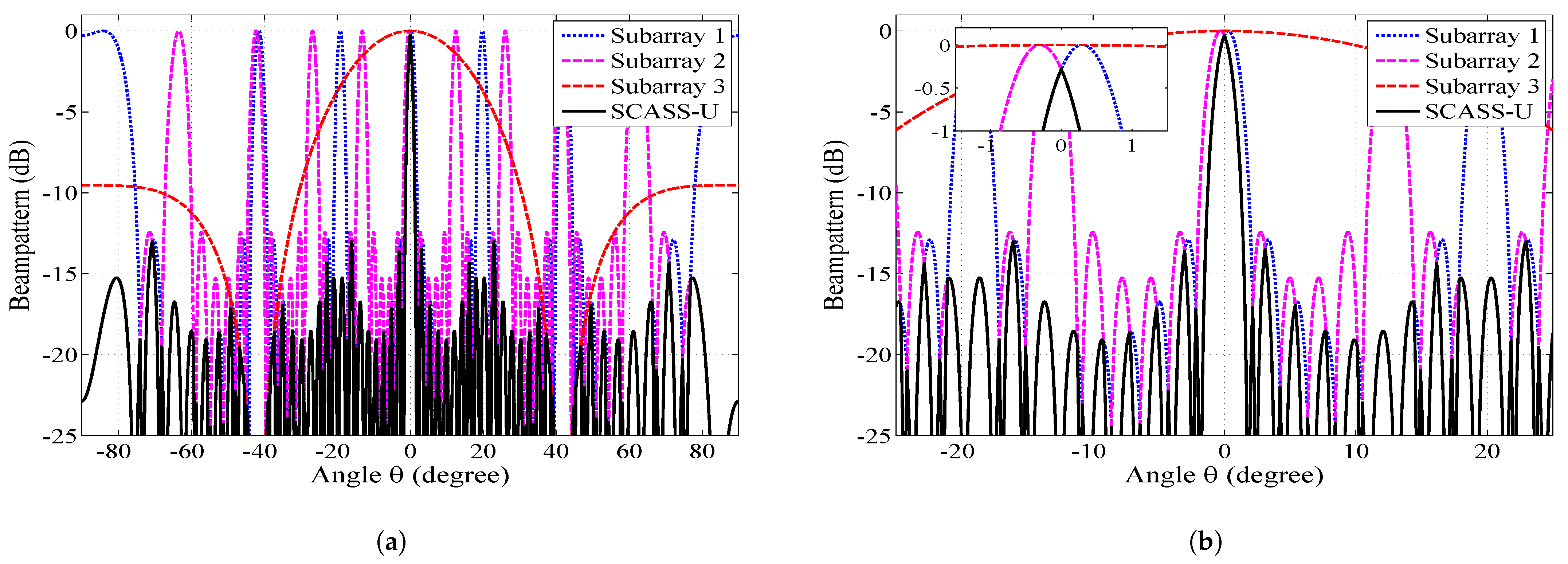
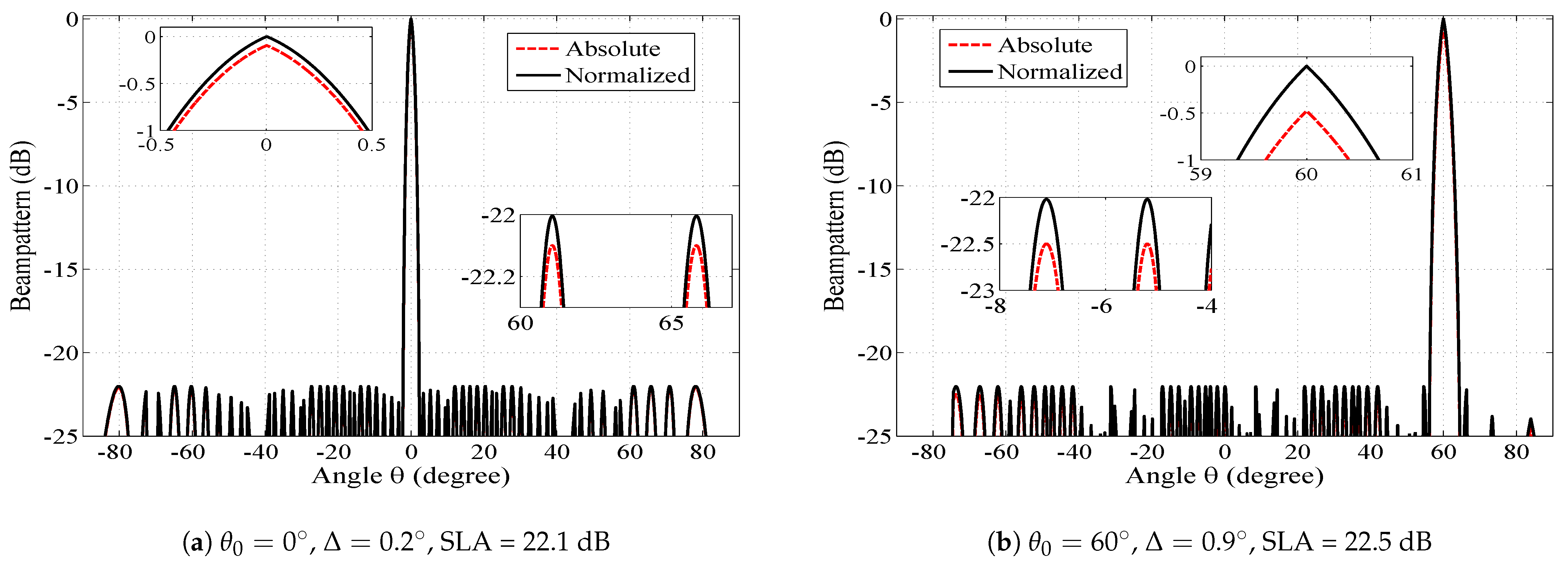
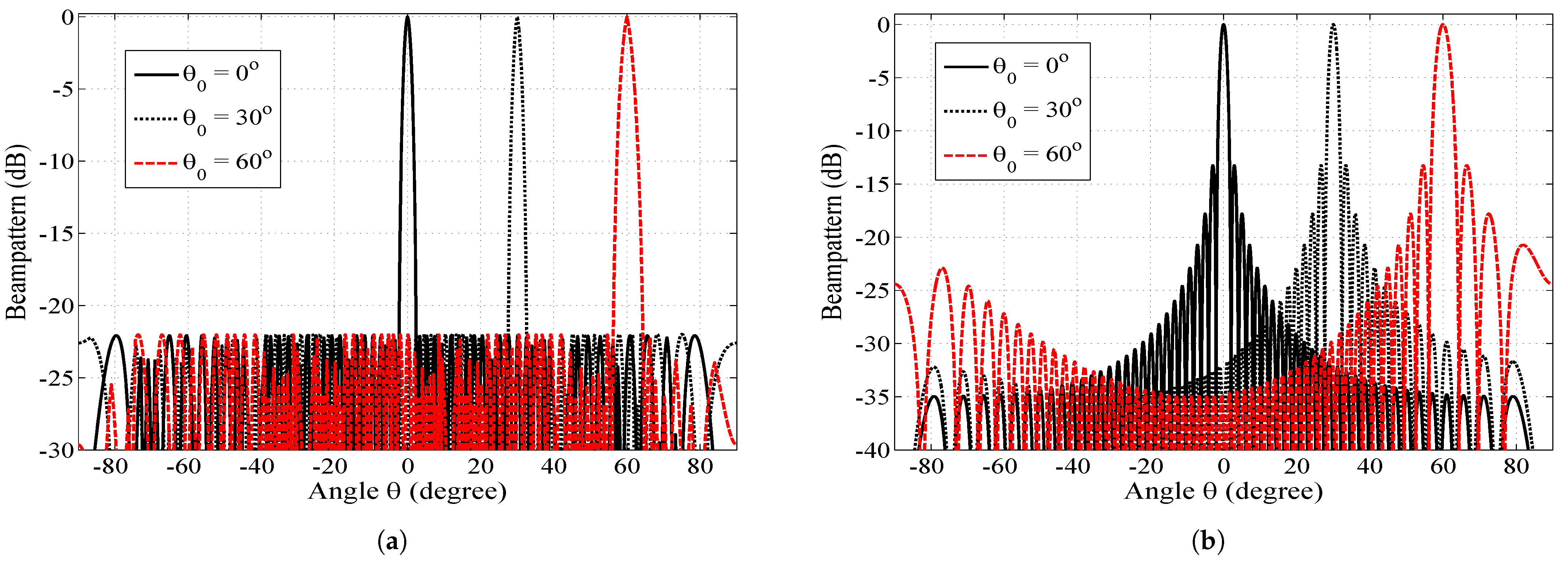
3. Simulations and Results
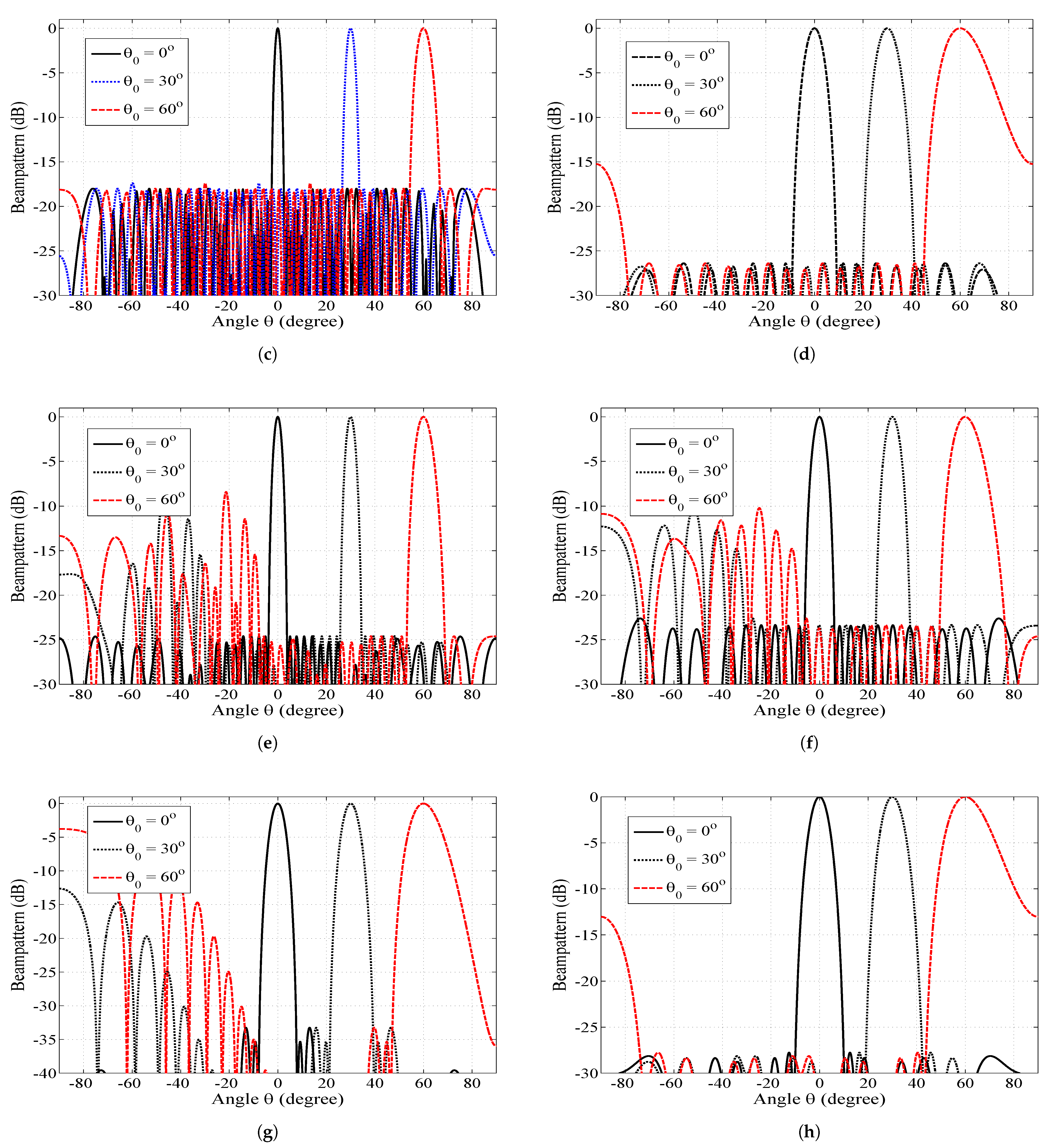
3.1. SCASS-U
3.2. SCASS-C
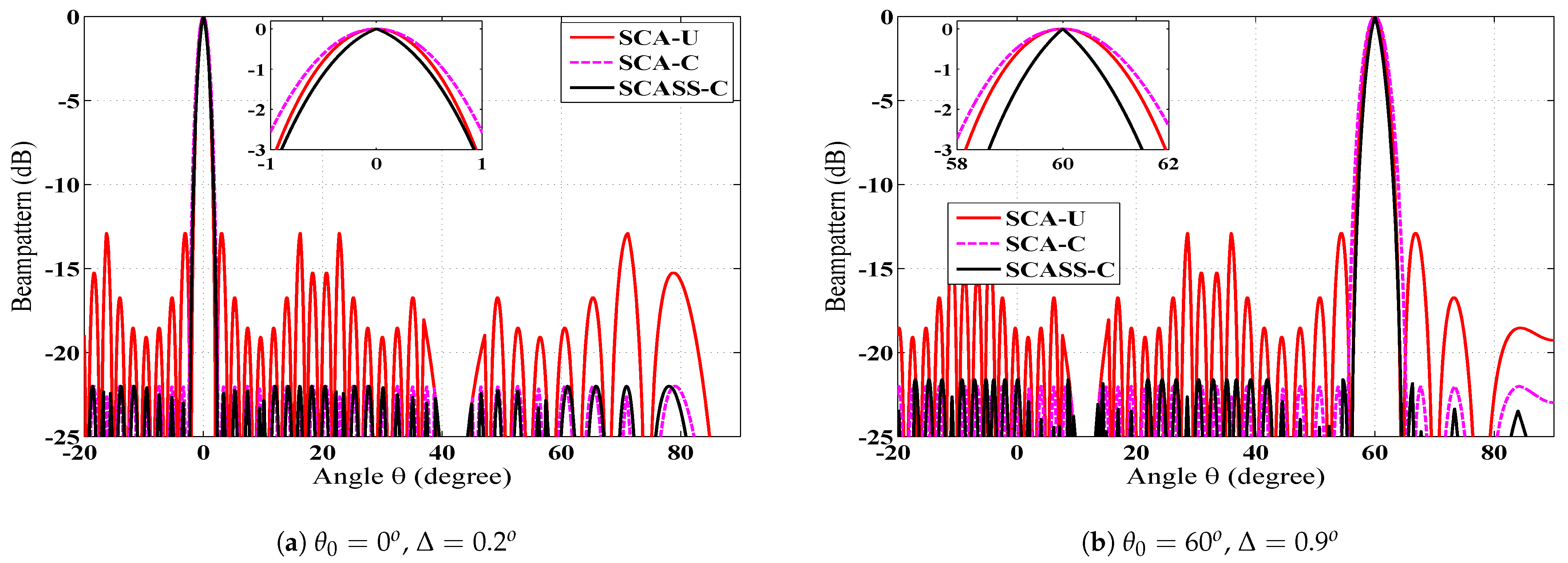
Comparison of the SCASS-C with Existing Arrays
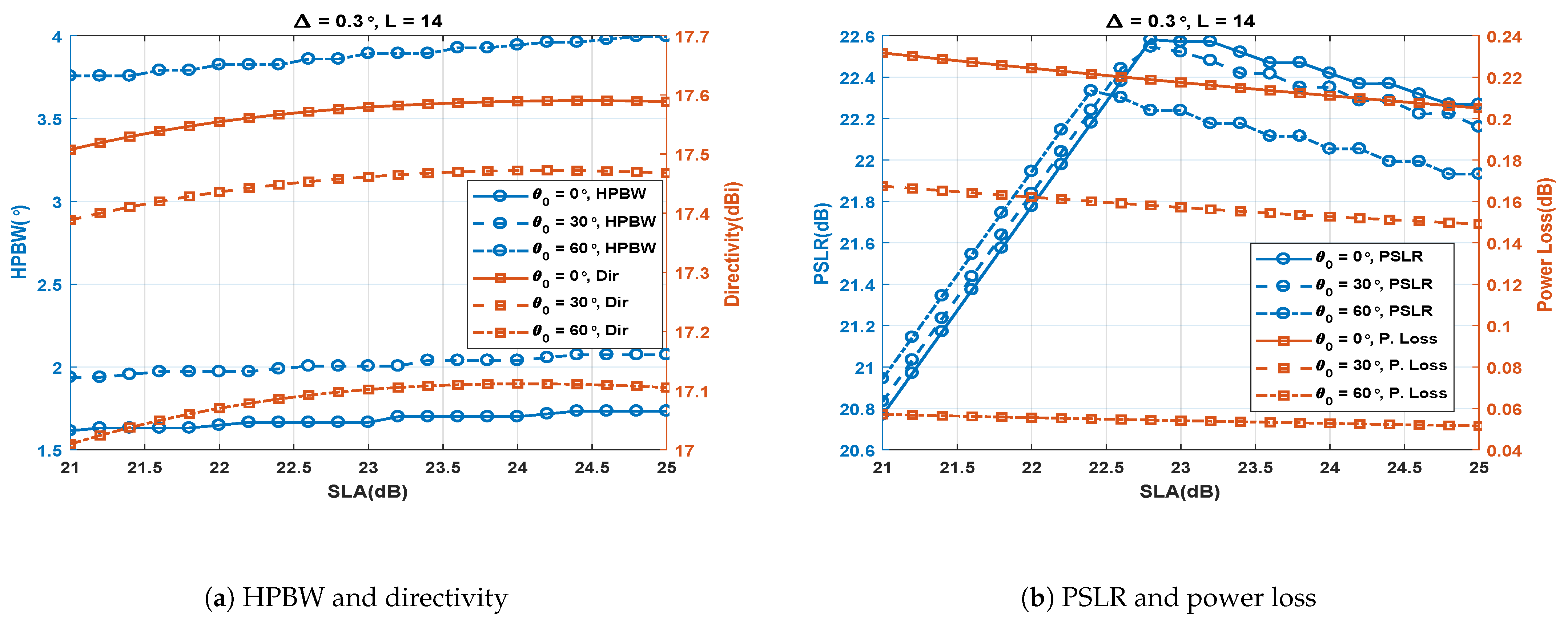
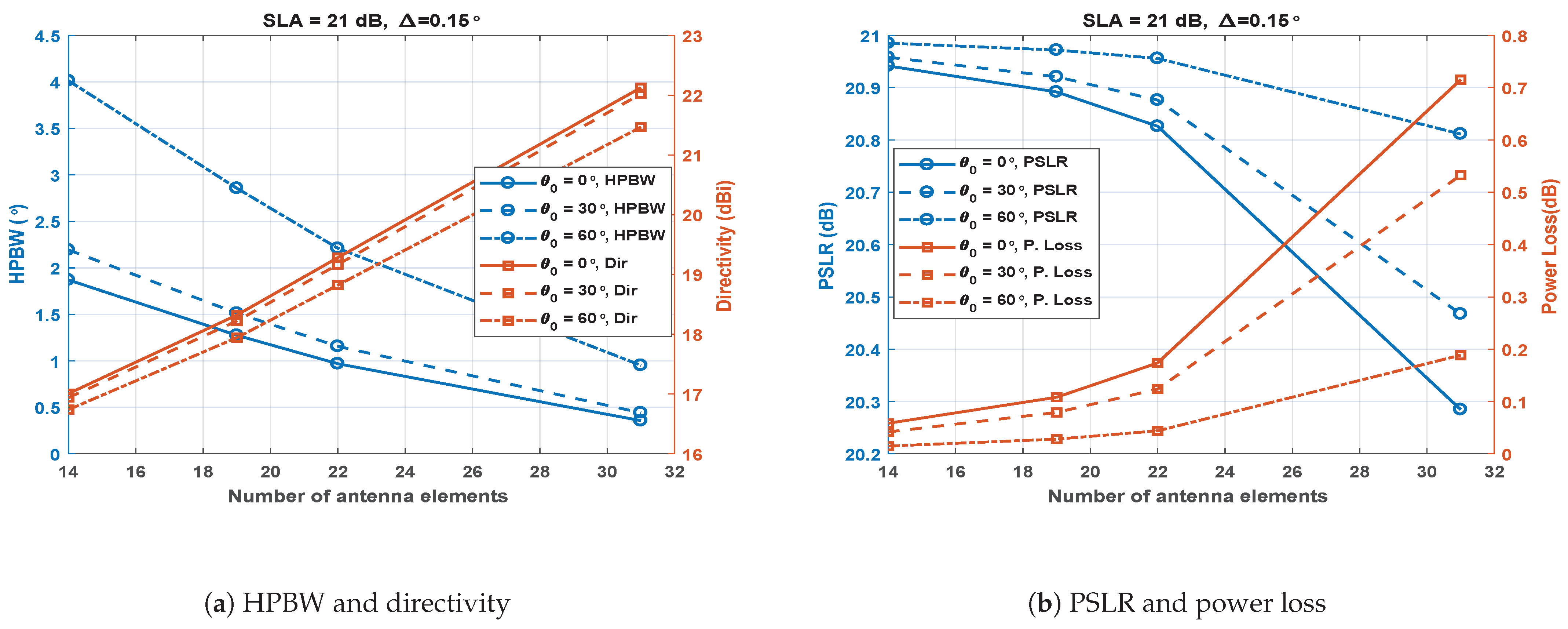
4. Parametric Analysis
4.1. Effect of for Fixed L and SLA
4.2. Effect of SLA for Fixed and L
4.3. Effect of L for Fixed and SLA
5. Conclusions and Future Work
Author Contributions
Funding
Conflicts of Interest
References
- Balanis, C.A. Antenna Theory; Wiley John & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Langston, L. Scanned directivity of linear arrays. IEEE Trans. Antennas Propag. 1971, 19, 282–284. [Google Scholar] [CrossRef]
- Sun, G.; Liu, Y.; Li, H.; Liang, S.; Wang, A.; Li, B. An antenna array sidelobe level reduction approach through invasive weed optimization. Int. J. Antennas Propag. 2018, 2018, 4867851. [Google Scholar] [CrossRef]
- Khalilpour, J.; Ranjbar, J.; Karami, P. A novel algorithm in a linear phased array system for side lobe and grating lobe level reduction with large element spacing. Analog. Integr. Circuits Signal Process. 2020, 104, 265–275. [Google Scholar] [CrossRef]
- Khan, W.; Saeed, S.; ur Rehman, A. Linear Antenna-Array with Log-increasing Inter-element Spacing and Non Uniform Weights. In Proceedings of the 2019 1st Global Power, Energy and Communication Conference (GPECOM), Nevsehir, Turkey, 12–15 June 2019; pp. 5–9. [Google Scholar] [CrossRef]
- Zaman, M.A.; Matin, M.A. Nonuniformly Spaced Linear Antenna Array Design Using Firefly Algorithm. Int. J. Microw. Sci. Technol. 2012, 2012, 256759. [Google Scholar] [CrossRef]
- Khalaj-Amirhosseini, M. To control the beamwidth of antenna arrays by virtually changing inter-distances. Int. J. RF Microw. Comput.-Aided Eng. 2019, 29, e21754. [Google Scholar] [CrossRef]
- Ridwan, M.; Abdo, M.; Jorswieck, E. Design of non-uniform antenna arrays using genetic algorithm. In Proceedings of the 13th International Conference on Advanced Communication Technology (ICACT2011), Gangwon, Republic of Korea, 13–16 February 2011; pp. 422–427. [Google Scholar]
- Kenane, E.; Djahli, F. Optimum design of non-uniform symmetrical linear antenna arrays using a novel modified invasive weeds optimization. Arch. Electr. Eng. 2016, 65, 5–18. [Google Scholar] [CrossRef]
- Rahman, S.U.; CAO, Q.; Ahmed, M.M.; Khalil, H. Analysis of linear antenna array for minimum side lobe level, half power beamwidth, and nulls control using PSO. J. Microw. Optoelectron. Electromagn. Appl. 2017, 16, 577–591. [Google Scholar] [CrossRef]
- Banerjee, S.; Mandal, D. Array pattern optimization for steerable linear isotropic antenna array using novel particle swarm optimization. J. Electromagn. Waves Appl. 2017, 31, 182–208. [Google Scholar] [CrossRef]
- Subhashini, K.R. Antenna array synthesis using a newly evolved optimization approach: Strawberry algorithm. J. Electr. Eng. 2019, 70, 317–322. [Google Scholar] [CrossRef]
- Enache, F.; Deparateanu, D.; Popescu, F. Optimization of non-uniform linear antenna array with linear and parabolic parameters variations. In Proceedings of the 2017 International Conference on Optimization of Electrical and Electronic Equipment (OPTIM) & 2017 Intl Aegean Conference on Electrical Machines and Power Electronics (ACEMP), Brasov, Romania, 25–27 May 2017; pp. 165–170. [Google Scholar] [CrossRef]
- Oraizi, H.; Fallahpour, M. Nonuniformly spaced linear array design for the specified beamwidth/sidelobe level or specified directivity/sidelobe level with coupling consideration. Prog. Electromagn. Res. M 2008, 4, 185–209. [Google Scholar] [CrossRef]
- Zaman, M.A.; Mamun, S.A.; Gaffar, M.; Choudhury, S.M.; Alam, M.M.; Matin, M.A. Phased Array Synthesis Using Modified Particle Swarm Optimization. J. Eng. Sci. Technol. Rev. 2011, 4, 68–73. [Google Scholar] [CrossRef]
- Liang, S.; Fang, Z.; Sun, G.; Liu, Y.; Qu, G.; Zhang, Y. Sidelobe Reductions of Antenna Arrays via an Improved Chicken Swarm Optimization Approach. IEEE Access 2020, 8, 37664–37683. [Google Scholar] [CrossRef]
- Wang, H.; Liu, C.; Wu, H.; Li, B.; Xie, X. Optimal Pattern Synthesis of Linear Array and Broadband Design of Whip Antenna Using Grasshopper Optimization Algorithm. Int. J. Antennas Propag. 2020, 2020, 5904018. [Google Scholar] [CrossRef]
- Adhikari, K.; Drozdenko, B. Design and Statistical Analysis of Tapered Coprime and Nested Arrays for the Min Processor. IEEE Access 2019, 7, 139601–139615. [Google Scholar] [CrossRef]
- Dolph, C. A Current Distribution for Broadside Arrays Which Optimizes the Relationship between Beam Width and Side-Lobe Level. Proc. IRE 1946, 34, 335–348. [Google Scholar] [CrossRef]
- Senapati, A.; Roy, J.S. Adaptive Beam Formation in Smart Antenna Using Tschebyscheff Distribution and Variants of Least MeanSquare Algorithm. Mikrotalasna Rev. 2016, 22, 11–16. [Google Scholar]
- Zhang, Y.; Wang, W.; Wang, R.; Deng, Y.; Jin, G.; Long, Y. A Novel NLFM Waveform with Low Sidelobes Based on Modified Chebyshev Window. IEEE Geosci. Remote Sens. Lett. 2019, 17, 814–818. [Google Scholar] [CrossRef]
- Li, M.; Zhang, Z.; Tang, M.C.; Yi, D.; Ziolkowski, R.W. Compact Series-Fed Microstrip Patch Arrays Excited with Dolph–Chebyshev Distributions Realized with Slow Wave Transmission Line Feed Networks. IEEE Trans. Antennas Propag. 2020, 68, 7905–7915. [Google Scholar] [CrossRef]
- Agha, M.H.; AL-Adwany, M.A.S.; Bayat, O.; Hamdoon, H.T. IFT and Chebyshev-based planar array thinning for adaptive interference suppression. J. Comput. Electron. 2023, 22, 333–349. [Google Scholar] [CrossRef]
- Xu, S.; Xu, H.X.; Wang, Y.; Xu, J.; Wang, C.; Pang, Z.; Luo, H. Circularly Polarized Antenna Array with Decoupled Quad Vortex Beams. Nanomaterials 2022, 12, 3083. [Google Scholar] [CrossRef]
- Xu, H.X.; Hu, G.; Han, L.; Jiang, M.; Huang, Y.; Li, Y.; Yang, X.; Ling, X.; Chen, L.; Zhao, J.; et al. Chirality-Assisted High-Efficiency Metasurfaces with Independent Control of Phase, Amplitude, and Polarization. Adv. Opt. Mater. 2019, 7, 1801479. [Google Scholar] [CrossRef]
- Xu, H.X.; Cai, T.; Zhuang, Y.Q.; Peng, Q.; Wang, G.M.; Liang, J.G. Dual-Mode Transmissive Metasurface and Its Applications in Multibeam Transmitarray. IEEE Trans. Antennas Propag. 2017, 65, 1797–1806. [Google Scholar] [CrossRef]
- Vaidyanathan, P.P.; Pal, P. Sparse sensing with co-prime samplers and arrays. IEEE Trans. Signal Process. 2010, 59, 573–586. [Google Scholar] [CrossRef]
- Liu, Y.; Buck, J.R. Detecting Gaussian signals in the presence of interferers using the coprime sensor arrays with the min processor. In Proceedings of the 2015 49th Asilomar conference on signals, systems and computers, Pacific Grove, CA, USA, 8–11 November 2015; pp. 370–374. [Google Scholar]
- Di Martino, G.; Iodice, A. Passive beamforming with coprime arrays. IET Radar Sonar Navig. 2016, 11, 964–971. [Google Scholar] [CrossRef]
- Moghadam, G.S.; Shirazi, A.B. Novel method for digital beamforming in co-prime sensor arrays using product and min processors. IET Signal Process. 2019, 13, 614–623. [Google Scholar] [CrossRef]
- Adhikari, K.; Buck, J.R.; Wage, K.E. Extending coprime sensor arrays to achieve the peak side lobe height of a full uniform linear array. EURASIP J. Adv. Signal Process. 2014, 148, 148. [Google Scholar] [CrossRef]
- Adhikari, K.; Buck, J.R.; Wage, K.E. Beamforming with extended co-prime sensor arrays. In Proceedings of the 2013 IEEE international conference on acoustics, speech and signal processing, Vancouver, BC, Canada, 26–31 May 2013; pp. 4183–4186. [Google Scholar]
- Adhikari, K. Beamforming with semi-coprime arrays. J. Acoust. Soc. Am. 2019, 145, 2841–2850. [Google Scholar] [CrossRef] [PubMed]
- Tokan, F.; Gunes, F. The multi-objective optimization of non-uniform linear phased arrays using the genetic algorithm. Prog. Electromagn. Res. B 2009, 17, 135–151. [Google Scholar] [CrossRef]
- Ward, H. Properties of Dolph-Chebyshev Weighting Functions. IEEE Trans. Aerosp. Electron. Syst. 1973, AES-9, 785–786. [Google Scholar] [CrossRef]

| Name of Array | L | Configurable Parameters | HPBW | PSLR (dB) | Dir (dBi) | Power Loss (dB) | |
|---|---|---|---|---|---|---|---|
| 14 | 0 | SLA = 22.10 dB, | 1.82 | 22.0 | 17.24 | 0.1 | |
| SCASS-C | 14 | 30 | SLA = 22.15 dB, | 1.99 | 22.0 | 17.44 | 0.15 |
| 14 | 60 | SLA = 22.5 dB, | 2.93 | 22.0 | 17.41 | 0.5 | |
| 14 | 0 | 1.89 | 12.9 | 15.65 | |||
| SCA-U [33] | 14 | 30 | 2.18 | 12.9 | 15.65 | ||
| 14 | 60 | 3.88 | 12.9 | 15.65 | |||
| 14 | 0 | SLA = 22 dB | 2.15 | 22 | 16.59 | ||
| SCA-C | 14 | 30 | SLA = 22 dB | 2.50 | 22 | 16.59 | |
| 14 | 60 | SLA = 22 dB | 4.38 | 22 | 16.59 | ||
| 14 | 0 | 1.80 | 13.25 | 17.48 | |||
| Novel CSA [30] | 14 | 30 | 2.10 | 13.25 | 17.48 | ||
| 14 | 60 | 3.60 | 13.25 | 17.48 | |||
| 18 | 0 | SLA = 18 dB | 2.94 | 18 | 14.84 | ||
| ECSA-C [18] | 18 | 30 | SLA = 18 dB | 3.40 | 18 | 14.84 | |
| 18 | 60 | SLA = 18 dB | 5.92 | 18 | 14.84 | ||
| 16 | 0 | 7.58 | 26.4 | 11.6 | |||
| SULA-IWO [3] | 16 | 30 | 8.70 | 26.4 | 11.6 | ||
| 16 | 60 | 15.6 | 15 | 11.6 | |||
| 32 | 0 | 3.27 | 24.6 | 15.12 | |||
| NULA-GO [17] | 32 | 30 | 3.77 | 8.4 | 14.38 | ||
| 32 | 60 | 6.57 | 8.4 | 13.9 | |||
| 20 | 0 | 5.30 | 22.6 | 13 | |||
| NULA-GA [14] | 20 | 30 | 6.17 | 10.2 | 12.44 | ||
| 20 | 60 | 10.84 | 10.2 | 12.1 | |||
| 21 | 0 | 5.71 | 33.3 | 12.84 | |||
| NULA-P [13] | 21 | 30 | 6.60 | 12.62 | 12.7 | ||
| 21 | 60 | 11.6 | 3.8 | 11.25 | |||
| SULA-PSO [10] | 16 | 0 | 7.98 | 27.8 | 11.38 | ||
| 16 | 30 | 9.23 | 27.8 | 11.38 | |||
| 16 | 60 | 16.23 | 13 | 11.38 | |||
| NULA-PSO [10] | 16 | 0 | 5 | 21.08 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, W.; Shahid, S.; Iqbal, W.; Rana, A.S.; Zahra, H.; Alathbah, M.; Abbas, S.M. Semi-Coprime Array with Staggered Beam-Steering of Sub-Arrays. Sensors 2023, 23, 5484. https://doi.org/10.3390/s23125484
Khan W, Shahid S, Iqbal W, Rana AS, Zahra H, Alathbah M, Abbas SM. Semi-Coprime Array with Staggered Beam-Steering of Sub-Arrays. Sensors. 2023; 23(12):5484. https://doi.org/10.3390/s23125484
Chicago/Turabian StyleKhan, Waseem, Saleem Shahid, Waleed Iqbal, Ahsan Sarwar Rana, Hijab Zahra, Moath Alathbah, and Syed Muzahir Abbas. 2023. "Semi-Coprime Array with Staggered Beam-Steering of Sub-Arrays" Sensors 23, no. 12: 5484. https://doi.org/10.3390/s23125484
APA StyleKhan, W., Shahid, S., Iqbal, W., Rana, A. S., Zahra, H., Alathbah, M., & Abbas, S. M. (2023). Semi-Coprime Array with Staggered Beam-Steering of Sub-Arrays. Sensors, 23(12), 5484. https://doi.org/10.3390/s23125484






