Simplified Mutual Inductance Calculation of Planar Spiral Coil for Wireless Power Applications
Abstract
:1. Introduction
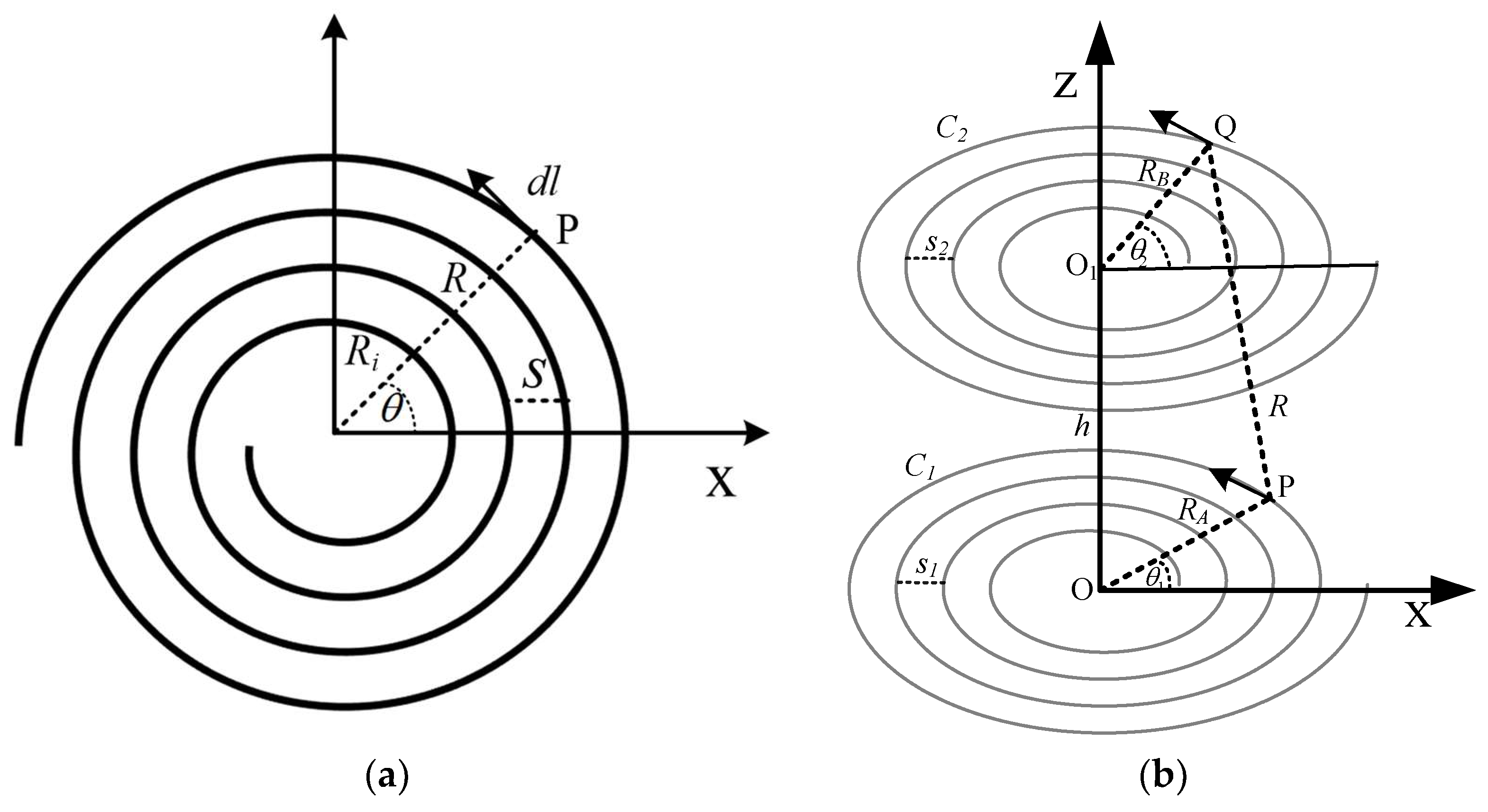
2. Mutual Inductance Equation Derivation
2.1. Two Perfect Aligned the Planar Spiral Coil
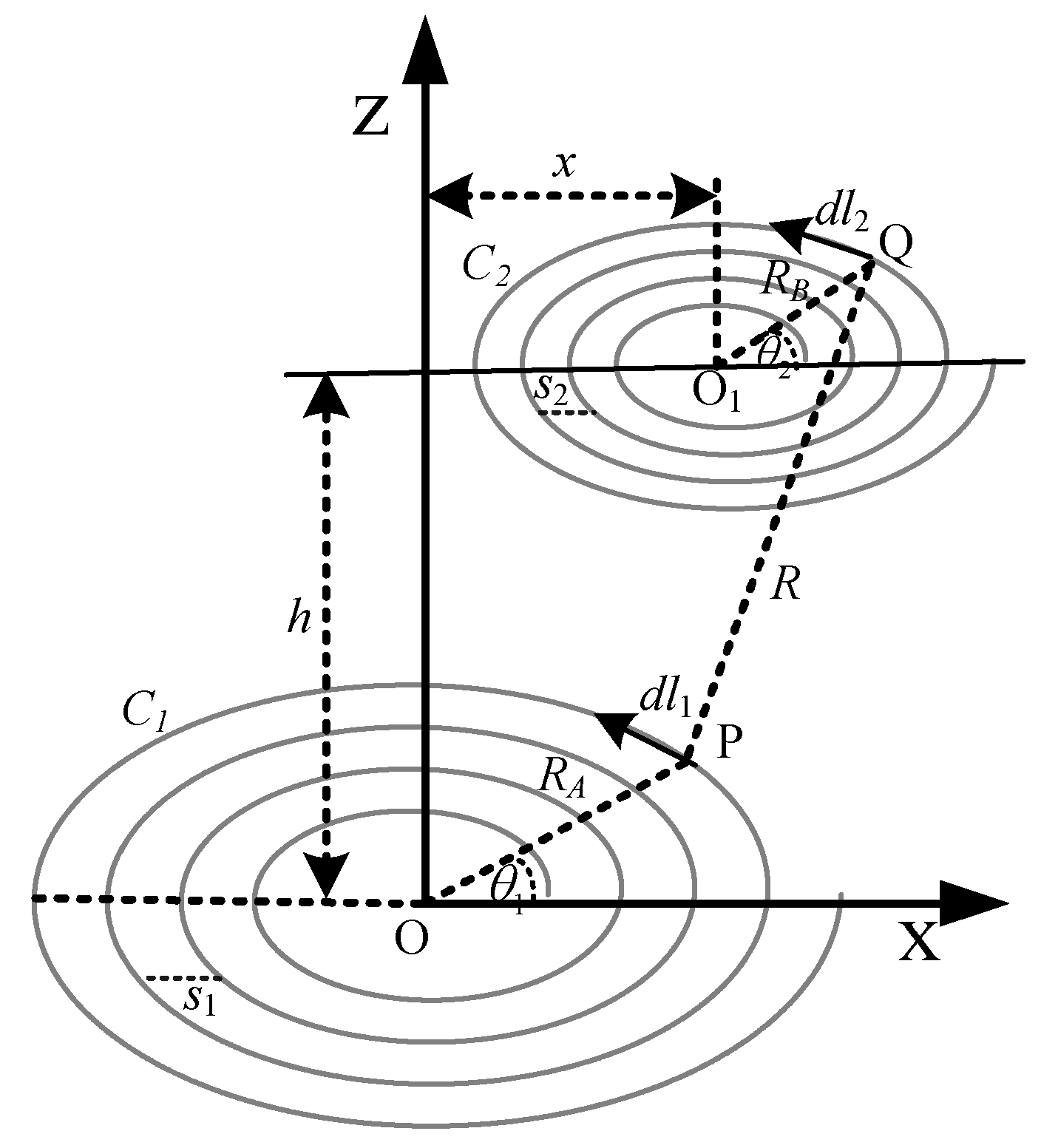
2.2. Lateral Misalignment of the Planar Spiral Coil
3. Simulation Verification
4. Experimental Verification
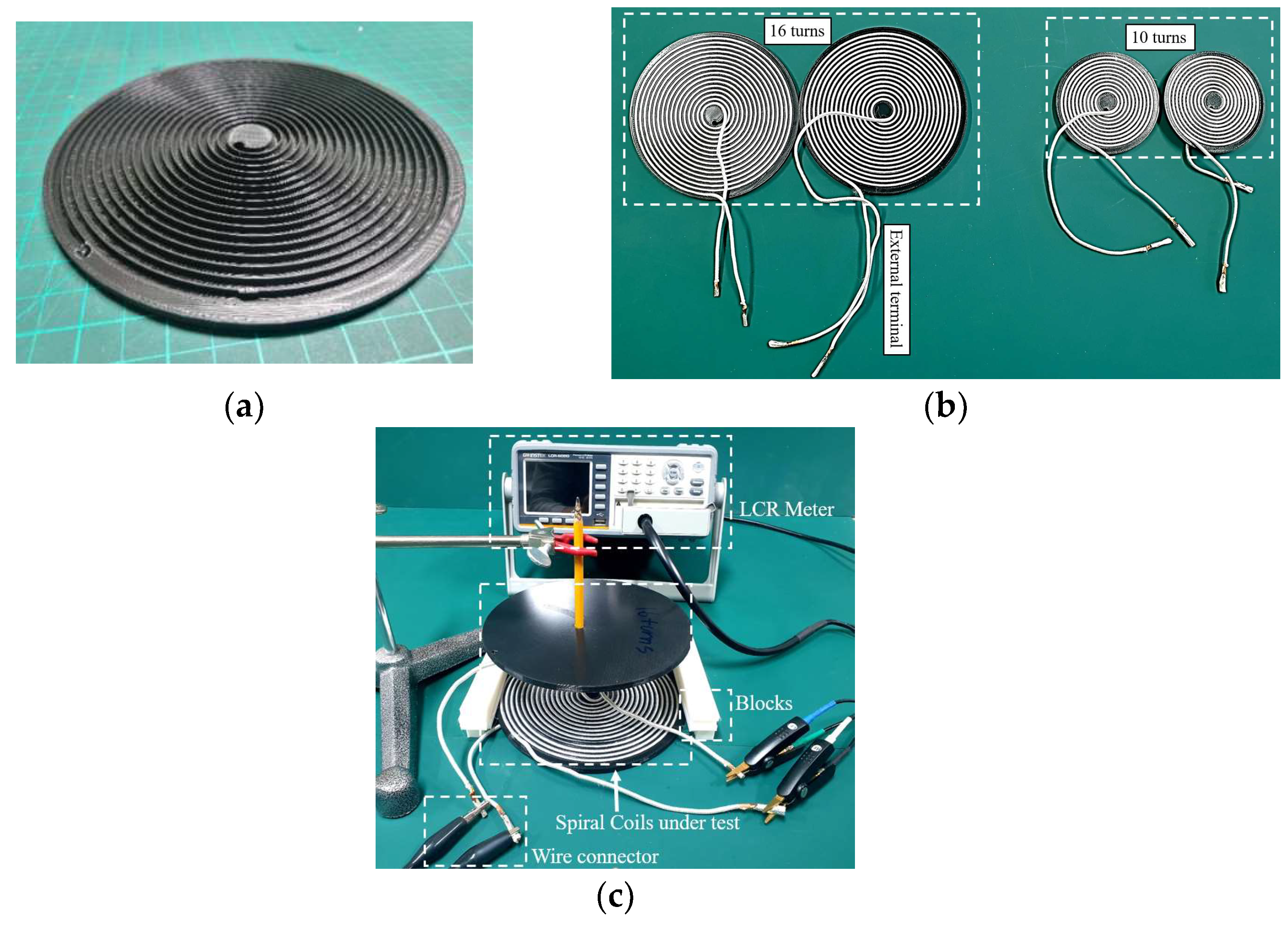
4.1. Selection of Wire and Its Measurement
4.2. Mutual Inductance Measurement Method
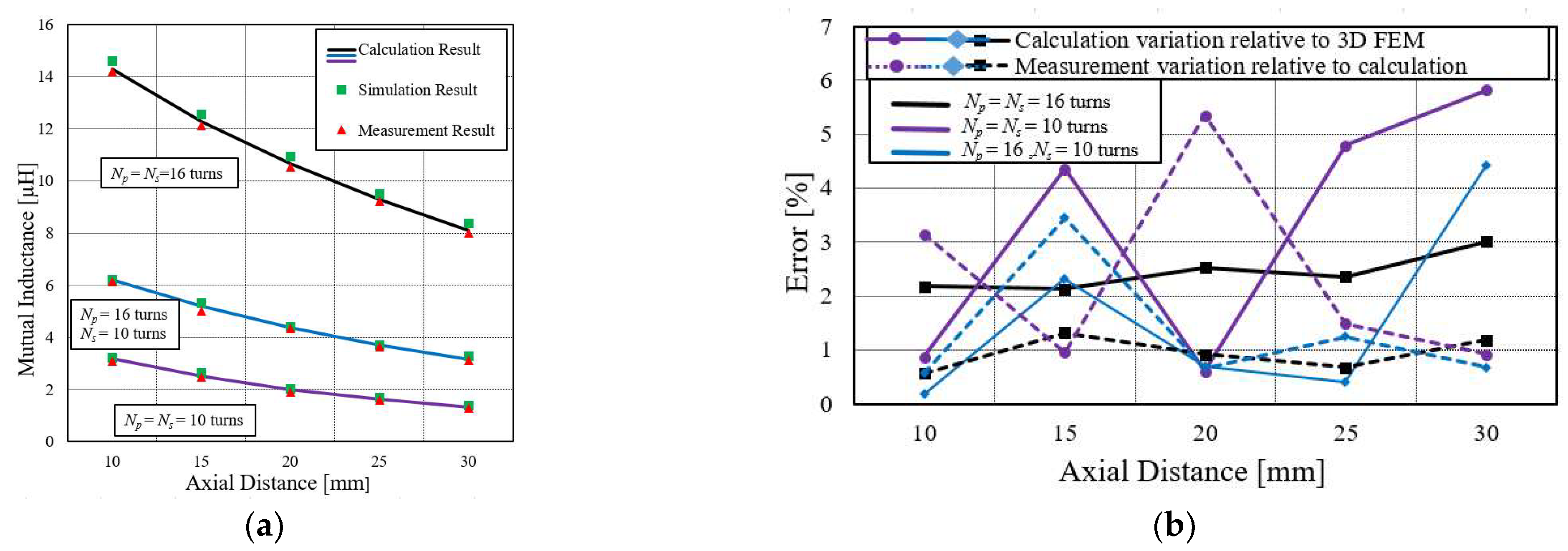
4.3. Mutual Inductances of Different Spiral Coil Configurations for Aligned Distances and Their Error Comparison
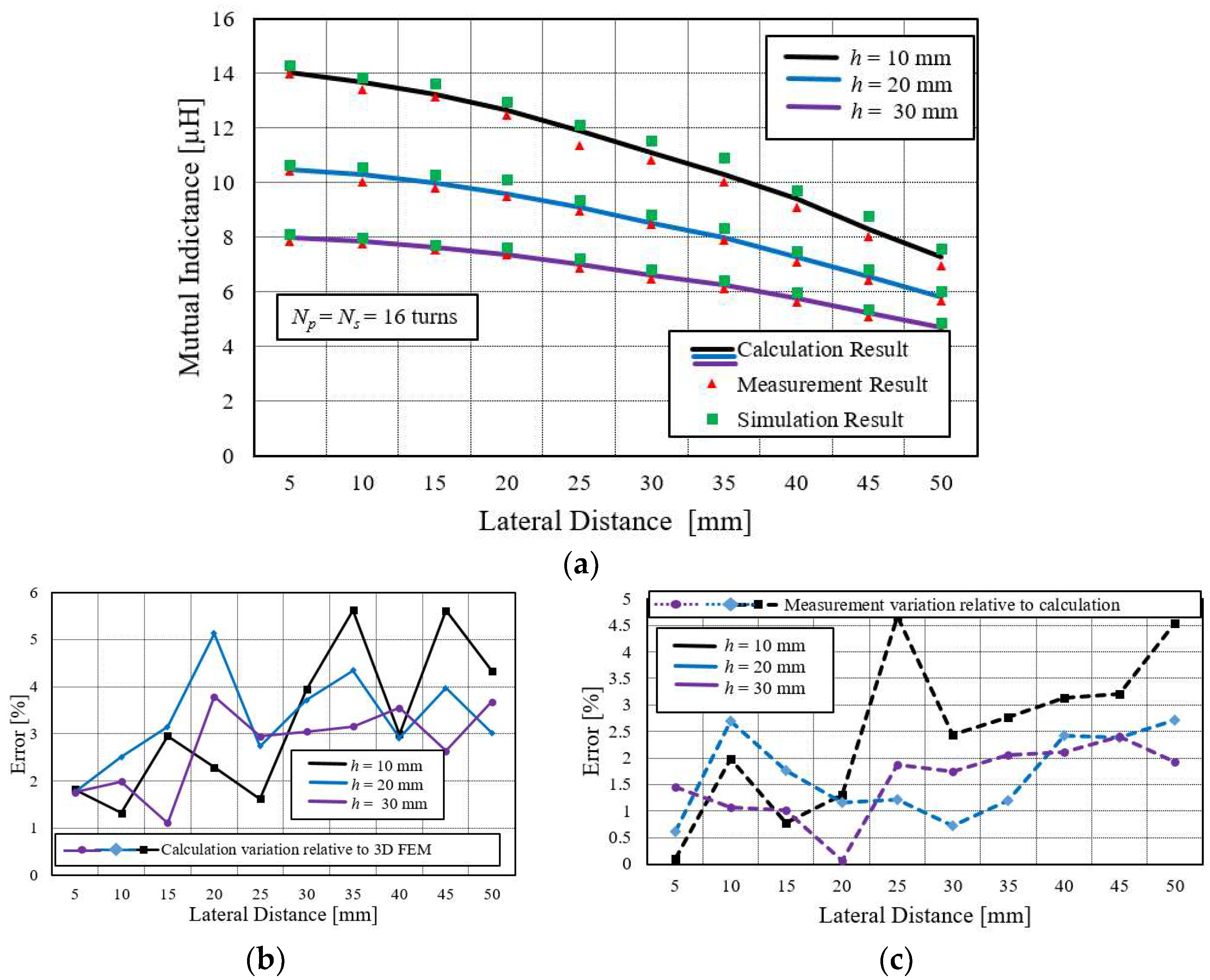
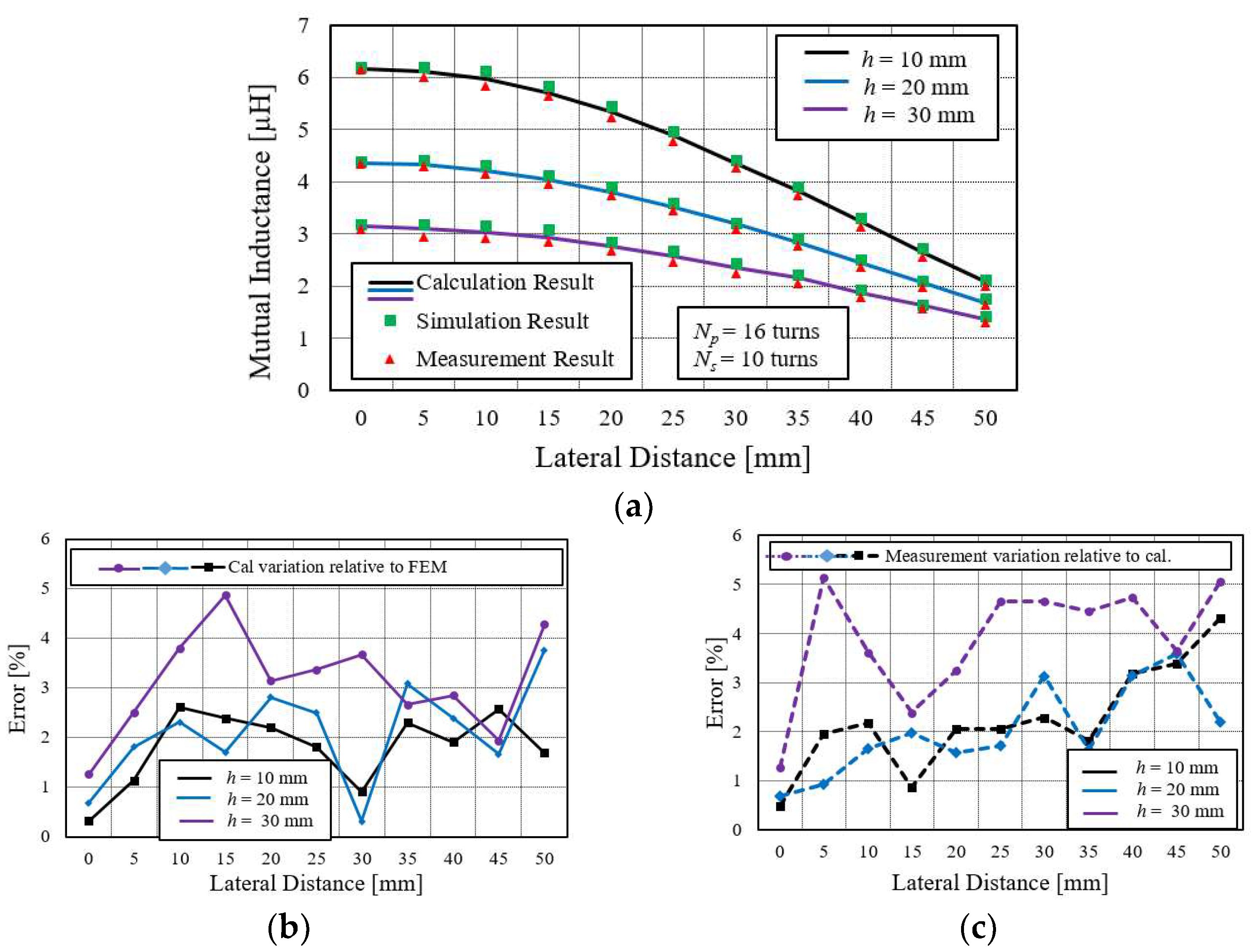
4.4. Behavior of Mutual Inductance between Two Large Primary and Large Secondary Spiral Coils under Lateral Misalignment and Their Error Comparison
4.4.1. Behavior of Mutual Inductance between Two Small Primary and Small Secondary Spiral Coils under Lateral Misalignment and Their Error Comparison
4.4.2. Behavior of Mutual Inductance between Large Primary and Small Secondary Spiral Coils under Lateral Misalignment and Their Error Comparison
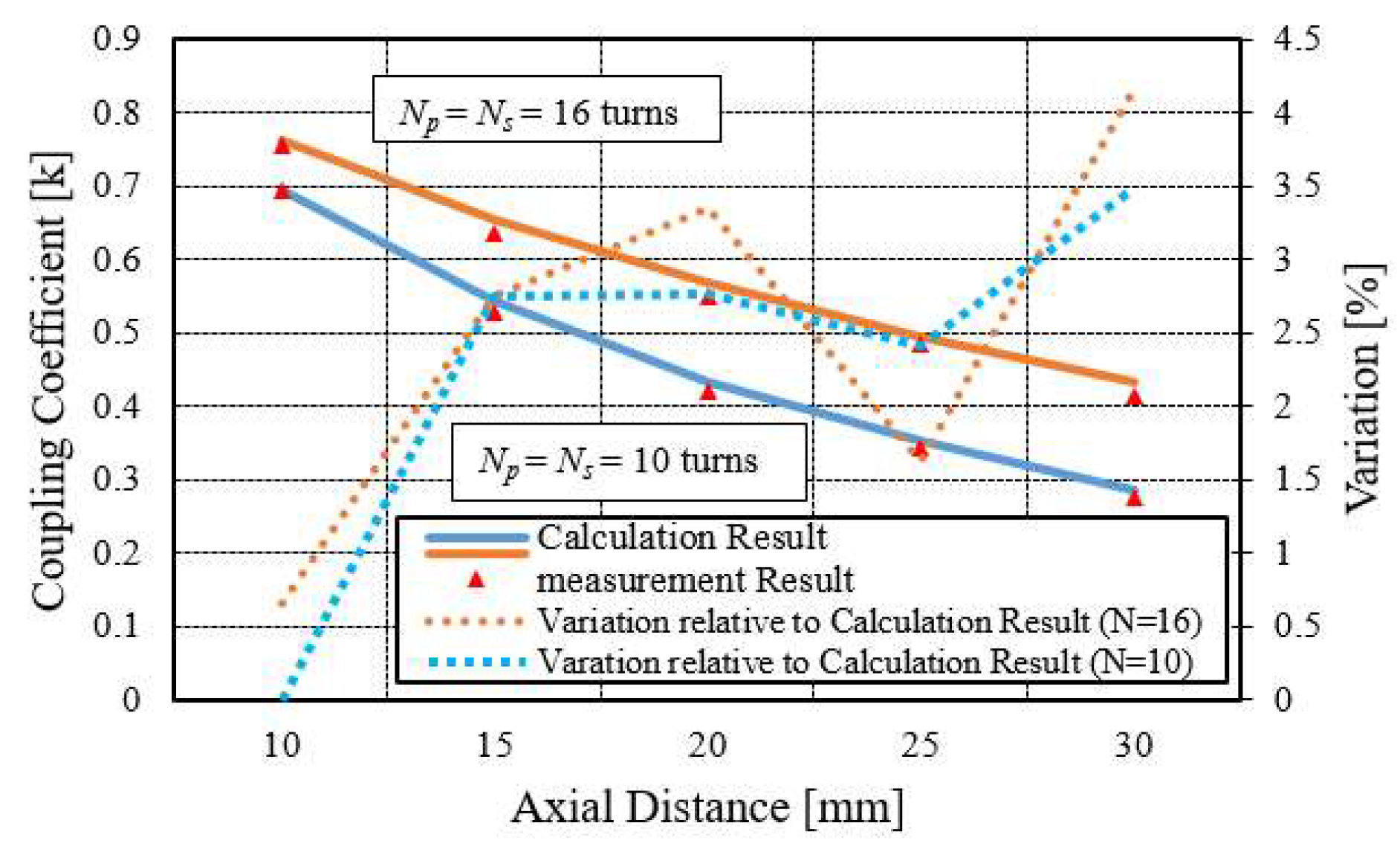
4.5. Coupling Coefficient of Spiral Coil
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khan, S.R.; Choi, G. Analysis and Optimization of Four-Coil Planar Magnetically Coupled Printed Spiral Resonators. Sensors 2016, 16, 1219. [Google Scholar] [CrossRef] [Green Version]
- Palagani, Y.; Mohanarangam, K.; Shim, J.H.; Choi, J.R. Wireless power transfer analysis of circular and spherical coils under misalignment conditions for biomedical implants. Biosens. Bioelectron. 2019, 141, 111283. [Google Scholar] [CrossRef]
- Khan, S.R.; Sumanth, K.P.; Gerard, C.; Marc, P.Y.D. Wireless power transfer techniques for implantable medical devices: A review. Sensors 2020, 20, 3487. [Google Scholar] [CrossRef] [PubMed]
- Krishnapriya, S.; Chandrakar, H.; Komaragiri, R.S.; Suja, K.J. Performance analysis of planar microcoils for biomedical wireless power transfer links. Sādhanā 2019, 44, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Haerinia, M.; Shadid, R. Wireless Power Transfer Approaches for Medical Implants: A Review. Signals 2020, 1, 209–229. [Google Scholar] [CrossRef]
- Nguyen, M.Q.; Hughes, Z.; Woods, P.; Seo, Y.-S.; Rao, S.; Chiao, J.-C. Field Distribution Models of Spiral Coil for Misalignment Analysis in Wireless Power Transfer Systems. IEEE Trans. Microw. Theory Tech. 2014, 62, 920–930. [Google Scholar] [CrossRef]
- Artan, N.S.; Amineh, R.K. Wireless Power Transfer to Implantable Medical Devices With Multi-Layer Planar Spiral Coils. In Research Anthology on Emerging Technologies and Ethical Implications in Human Enhancement; IGI Global: Hershey, PA, USA, 2021; pp. 457–481. [Google Scholar]
- Waffenschmidt, E. Wireless power for mobile devices. In Proceedings of the 2011 IEEE 33rd International Telecommunications Energy Conference (INTELEC), Amsterdam, The Netherlands, 9–13 October 2011; IEEE: Piscataway, NJ, USA, 2011; pp. 1–9. [Google Scholar]
- Lopez-Alcolea, F.J.; Del Real, J.V.; Roncero-Sanchez, P.; Torres, A.P. Modeling of a Magnetic Coupler Based on Single- and Double-Layered Rectangular Planar Coils with In-Plane Misalignment for Wireless Power Transfer. IEEE Trans. Power Electron. 2020, 35, 5102–5121. [Google Scholar] [CrossRef]
- Pon, L.L.; Leow, C.Y.; Rahim, S.K.A.; Eteng, A.; Kamarudin, M.R. Printed Spiral Resonator for Displacement-Tolerant Near-Field Wireless Energy Transfer. IEEE Access 2019, 7, 172055–172064. [Google Scholar] [CrossRef]
- Villa, J.L.; Jesús, S.; Andrés, L.; José, F.S. Design of a high frequency inductively coupled power transfer system for electric vehicle battery charge. Appl. Energy 2009, 86, 355–363. [Google Scholar] [CrossRef]
- Sallán, J.; Juan, L.; Villa, A.L.; José, F.S. Optimal design of ICPT systems applied to electric vehicle battery charge. IEEE Trans. Ind. Electron. 2009, 56, 2140–2149. [Google Scholar] [CrossRef]
- Miller, J.M.; Onar, O.C.; Chinthavali, M. Primary-Side Power Flow Control of Wireless Power Transfer for Electric Vehicle Charging. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 147–162. [Google Scholar] [CrossRef]
- Nguyen, D.H. Electric Vehicle—Wireless Charging-Discharging Lane Decentralized Peer-to-Peer Energy Trading. IEEE Access 2020, 8, 179616–179625. [Google Scholar] [CrossRef]
- Bouanou, T.; El Fadil, H.; Lassioui, A. Analysis and Design of Circular Coil Transformer in a Wireless Power Transfer System for Electric Vehicle Charging Application. In Proceedings of the 2020 International Conference on Electrical and Information Technologies (ICEIT), Rabat, Morocco, 4–7 March 2020; IEEE: Rabat, Morocco, 2020; pp. 1–6. [Google Scholar]
- Good, R.H. Elliptic integrals, the forgotten functions. Eur. J. Phys. 2001, 22, 119–126. [Google Scholar] [CrossRef]
- Maxwell, J.C. A Treatise on Electricity and Magnetism; Clarendon Press: Oxford, UK, 1873; Volume 1. [Google Scholar]
- Ramo, S.; John, R.W.; Theodore, V.D. Fields and Waves in Communication Electronics; John Wiley & Sons: Hoboken, NJ, USA, 1994. [Google Scholar]
- Ramrakhyani, A.K.; Mirabbasi, S.; Chiao, M. Design and Optimization of Resonance-Based Efficient Wireless Power Delivery Systems for Biomedical Implants. IEEE Trans. Biomed. Circuits Syst. 2011, 5, 48–63. [Google Scholar] [CrossRef]
- Raju, S.; Wu, R.; Chan, M.; Yue, C.P. Modeling of Mutual Coupling between Planar Inductors in Wireless Power Applications. IEEE Trans. Power Electron. 2014, 29, 481–490. [Google Scholar] [CrossRef]
- Liu, S.; Su, J.; Lai, J. Accurate Expressions of Mutual Inductance and Their Calculation of Archimedean Spiral Coils. Energies 2019, 12, 2017. [Google Scholar] [CrossRef] [Green Version]
- Maxwell-ANSYS; ANSYS, Inc.: Canonsburg, PA, USA, 2014.
- Kalantarov, P.L. Inductance Calculations; National Power Press: Moscow, Russia, 1955. [Google Scholar]
- Vaisanen, V.; Hiltunen, J.; Nerg, J.; Silventoinen, P. AC resistance calculation methods and practical design considerations when using litz wire. In Proceedings of the IECON 2013—39th Annual Conference of the IEEE Industrial Electronics Society, Vienna, Austria, 10–13 November 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 368–375. [Google Scholar]
- Rossmanith, H.; Marc, D.; Manfred, A.; Dietmar, E. Measurement and characterization of high frequency losses in nonideal litz wires. IEEE Trans. Power Electron. 2011, 26, 3386–3394. [Google Scholar] [CrossRef]
- Hussain, I.; Woo, D.-K. Self-Inductance Calculation of the Archimedean Spiral Coil. Energies 2022, 15, 253. [Google Scholar] [CrossRef]
- Matsumoto, H.; Neba, Y.; Ishizaka, K.; Itoh, R. Model for a Three-Phase Contactless Power Transfer System. IEEE Trans. Power Electron. 2011, 26, 2676–2687. [Google Scholar] [CrossRef]
- Liu, X.; Hui, S.Y.R. Optimal Design of a Hybrid Winding Structure for Planar Contactless Battery Charging Platform. IEEE Trans. Power Electron. 2008, 23, 455–463. [Google Scholar] [CrossRef]
- Kim, M.; Park, H.; Jung, J.-H. Design Methodology of 500 W Wireless Power Transfer Converter for High Power Transfer Efficiency. Trans. Korean Inst. Power Electron. 2016, 21, 356–363. [Google Scholar] [CrossRef]


| Parameters | Results | |||||
|---|---|---|---|---|---|---|
| h (mm) | s (mm) | Ri (mm) | Ro (mm) | FEM (µH) | (11) (µH) | Error (%) |
| 10 | 7.5 | 10 | 85 | 5.85 | 5.67 | 3.07 |
| 10 | 6.25 | 10 | 85 | 8.25 | 8.03 | 2.66 |
| 10 | 5.35 | 10 | 85 | 11.24 | 11.00 | 2.13 |
| 10 | 4.68 | 10 | 85 | 14.56 | 14.29 | 1.85 |
| Parameters | Results | |||||
|---|---|---|---|---|---|---|
| h (mm) | N (mm) | Ri (mm) | Ro (mm) | FEM (µH) | (11) (µH) | Error (%) |
| 10 | 10 | 10 | 85 | 5.85 | 5.67 | 3.07 |
| 10 | 10 | 10 | 95 | 6.61 | 6.40 | 3.17 |
| 10 | 10 | 10 | 105 | 7.39 | 7.15 | 3.24 |
| 10 | 10 | 10 | 115 | 8.19 | 7.91 | 3.41 |
| Parameters | Results | |||||
|---|---|---|---|---|---|---|
| h (mm) | N (mm) | Ri (mm) | s (mm) | FEM (µH) | (11) (µH) | Error (%) |
| 10 | 10 | 10 | 7.5 | 5.85 | 5.67 | 3.07 |
| 10 | 12 | 10 | 7.5 | 9.87 | 9.56 | 3.14 |
| 10 | 14 | 10 | 7.5 | 15.86 | 15.29 | 3.59 |
| 10 | 16 | 10 | 7.5 | 23.48 | 22.67 | 3.44 |
| Coil Size | N (mm) | Ri (mm) | s (mm) | Ro (mm) |
|---|---|---|---|---|
| Small Coil | 10 | 10 | 4 | 50 |
| Large Coil | 16 | 10 | 4.68 | 85 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hussain, I.; Woo, D.-K. Simplified Mutual Inductance Calculation of Planar Spiral Coil for Wireless Power Applications. Sensors 2022, 22, 1537. https://doi.org/10.3390/s22041537
Hussain I, Woo D-K. Simplified Mutual Inductance Calculation of Planar Spiral Coil for Wireless Power Applications. Sensors. 2022; 22(4):1537. https://doi.org/10.3390/s22041537
Chicago/Turabian StyleHussain, Iftikhar, and Dong-Kyun Woo. 2022. "Simplified Mutual Inductance Calculation of Planar Spiral Coil for Wireless Power Applications" Sensors 22, no. 4: 1537. https://doi.org/10.3390/s22041537
APA StyleHussain, I., & Woo, D.-K. (2022). Simplified Mutual Inductance Calculation of Planar Spiral Coil for Wireless Power Applications. Sensors, 22(4), 1537. https://doi.org/10.3390/s22041537






