On the Performance of Underlay Device-to-Device Communications
Abstract
:1. Introduction
2. State-of-the-Art
- To the best of authors knowledge, this is the first work that computes the outage probability, average rate, and amount of fading of the D2D networks under the impact of interference from D2D and cellular networks, as well as the background noise in closed-form expressions with three distinct power allocation schemes, namely, the equal, random, and path-loss based schemes.
- We derive the coverage probability of the cellular users under the aggregate interference of D2D transmission.
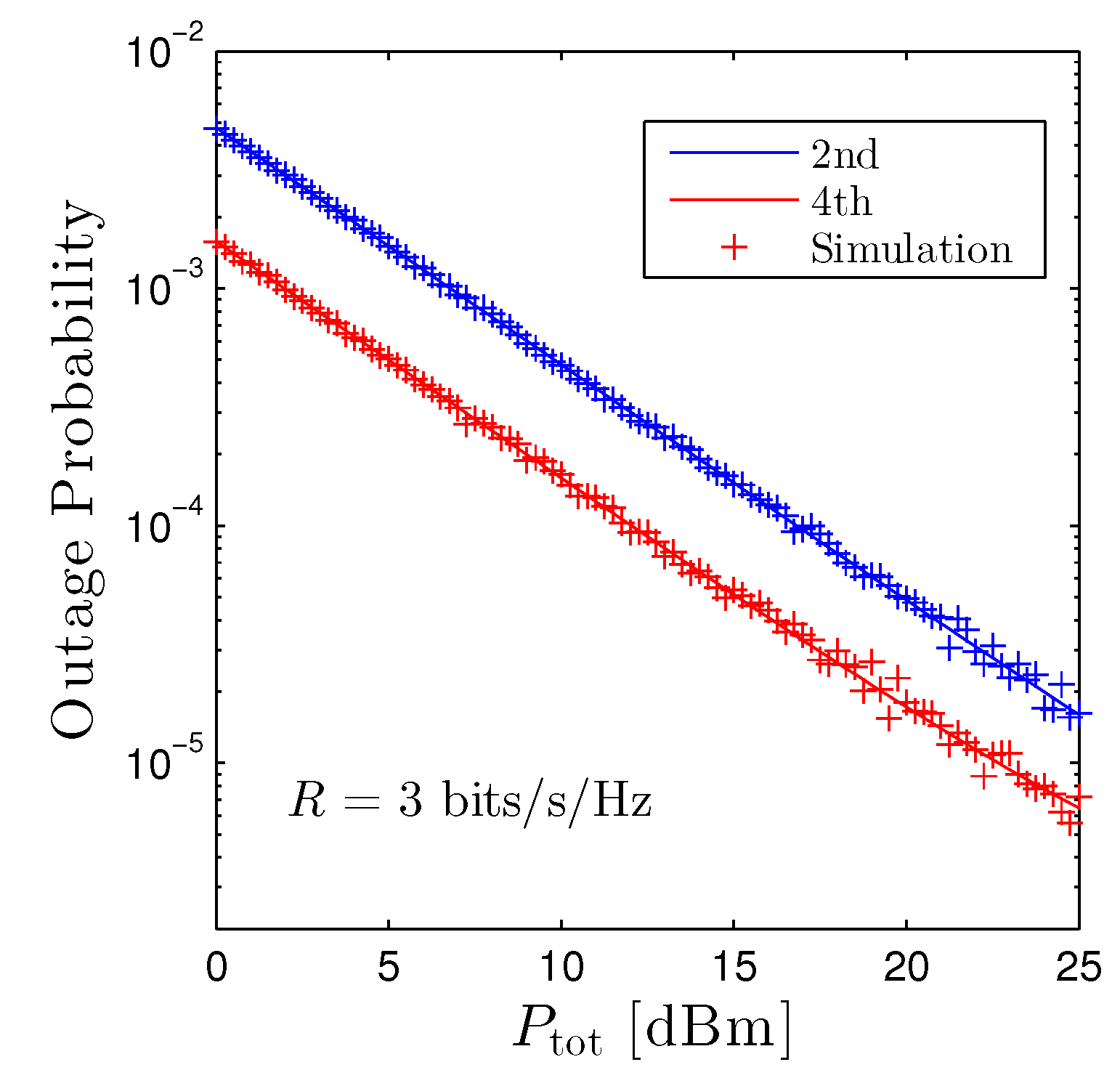
- The impact of the total transmit power of D2D networks on the performance of the OP is explicitly studied.
- Monte Carlo simulation results are presented to verify the accuracy of the proposed mathematical framework as well as to highlight the behavior of these metrics with respect to system parameters.
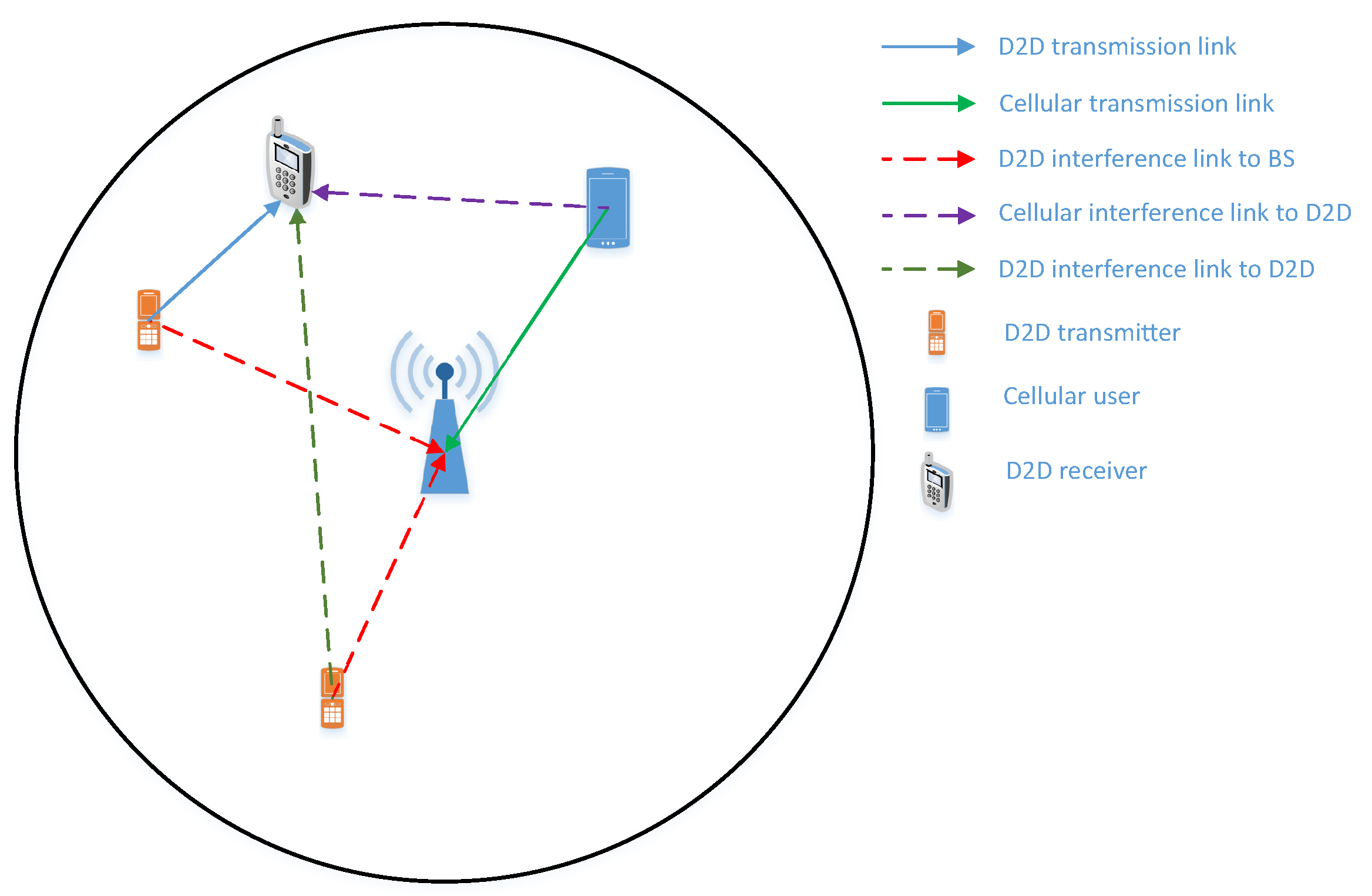
3. System Model
3.1. Channel Modeling
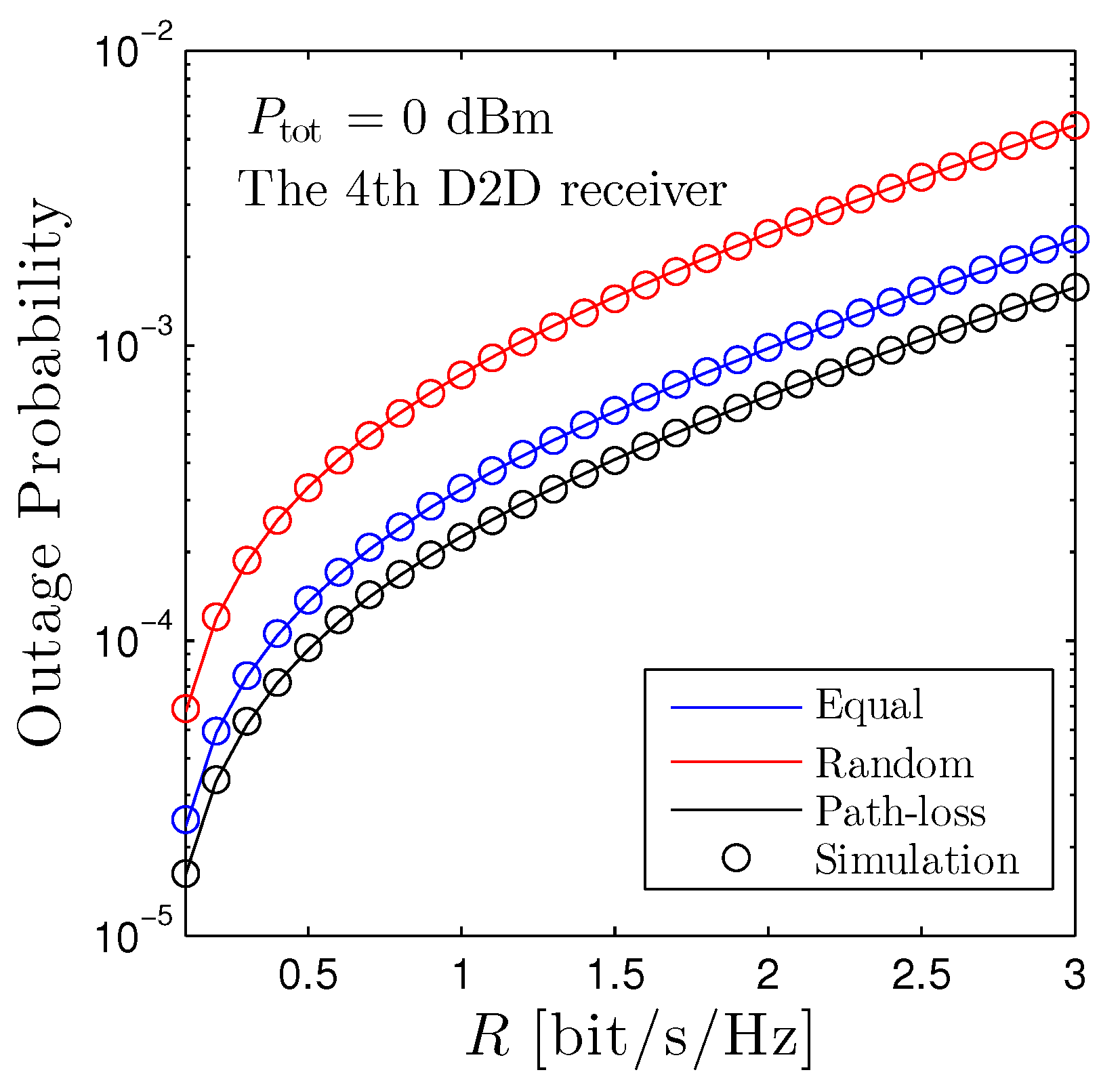
3.2. Power Allocation in D2D Networks
3.2.1. Equal Power Allocation
3.2.2. Random Power Allocation
3.2.3. Path-Loss-Based Power Allocation
3.3. Signal-to-Interference-Plus-Noise Ratio (SINR) at D2D Receivers and Base Station
4. Performance Analysis
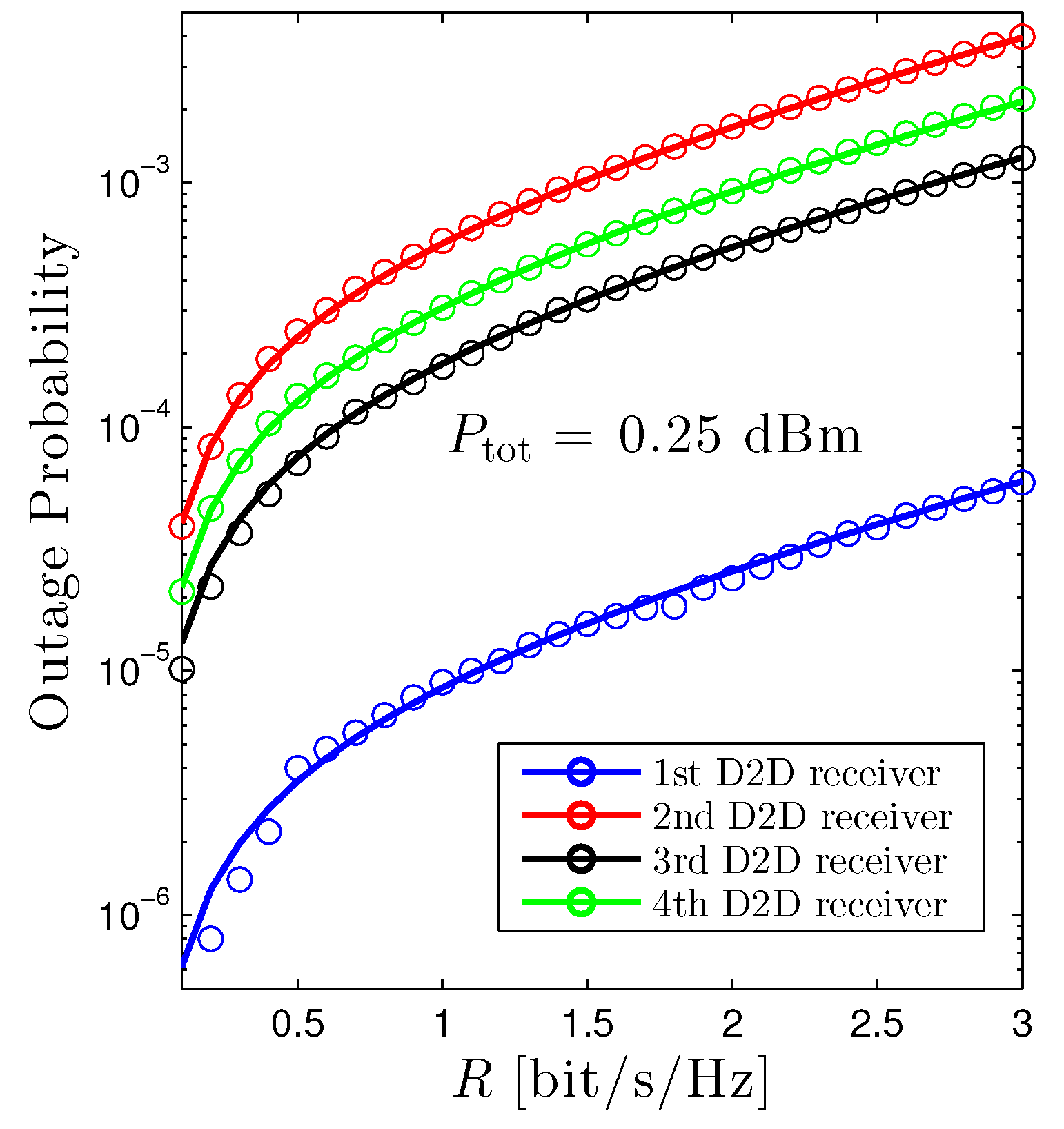
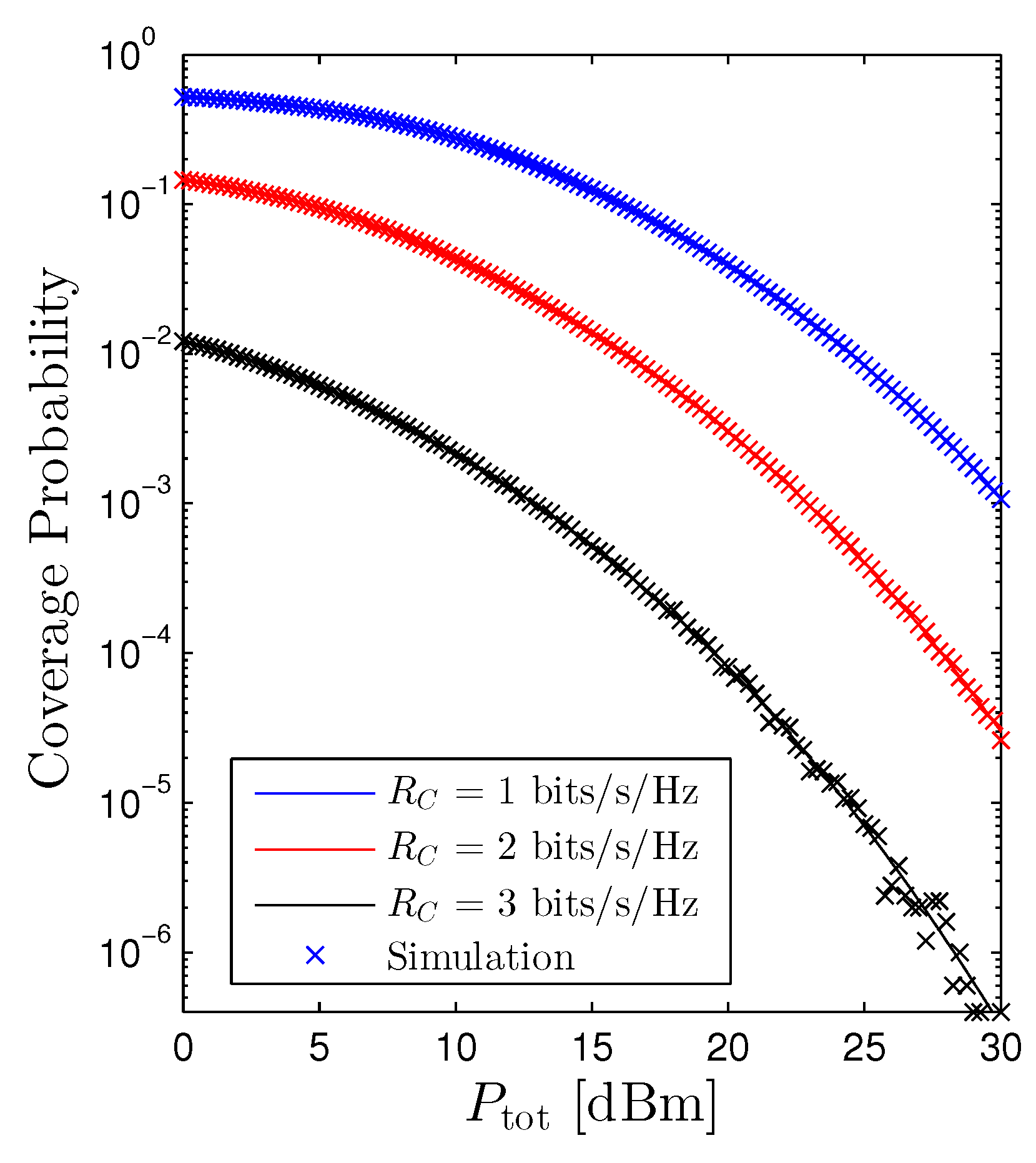
4.1. OP of the D2D User
4.2. Average Rate of D2D User
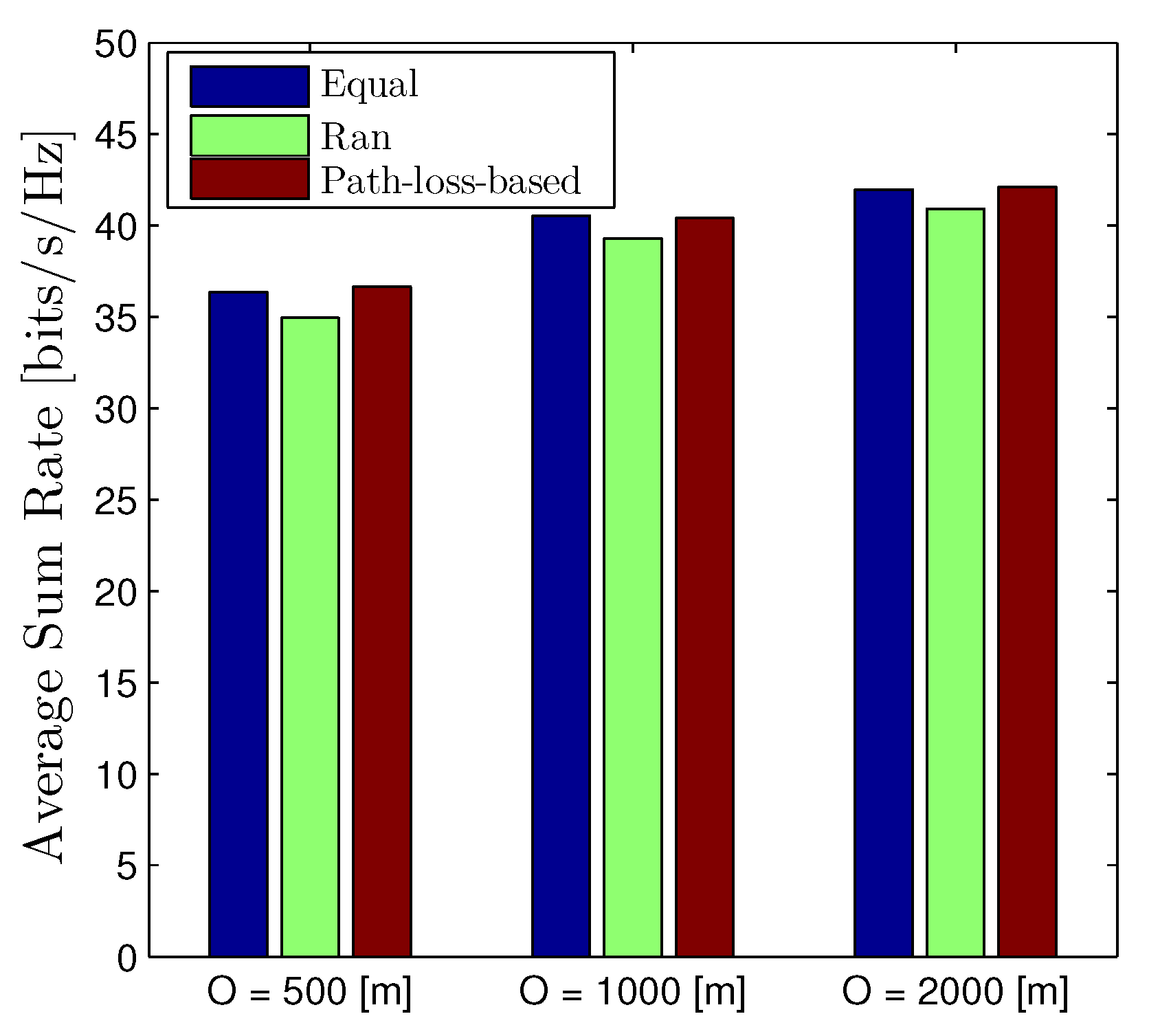
4.3. Average Sum Rate of D2D Networks
4.4. The Amount of Fading (AoF) of D2D Link
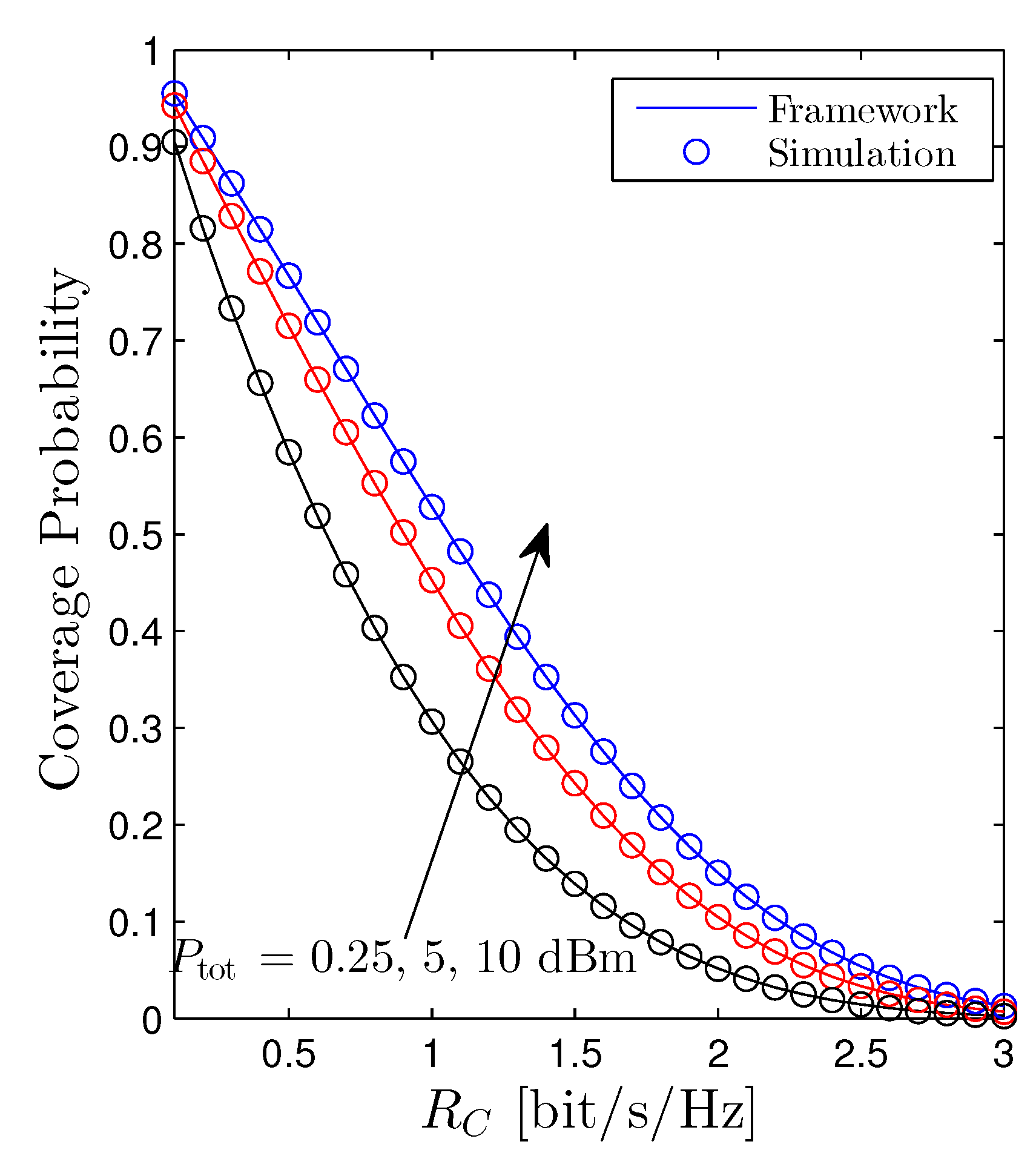
Coverage Probability of Cellular User
4.5. Performance Trends
5. Simulation Results
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Yoon, T.; Nguyen, T.H.; Nguyen, X.T.; Yoo, D.; Jang, B.; Nguyen, V.D. Resource Allocation for NOMA-Based D2D Systems Coexisting with Cellular Networks. IEEE Access 2018, 6, 66293–66304. [Google Scholar] [CrossRef]
- Hmila, M.; Fernández-Veiga, M.; Rodríguez-Pérez, M.; Herrería-Alonso, S. Non-Orthogonal Multiple Access for Unicast and Multicast D2D: Channel Assignment, Power Allocation and Energy Efficiency. Sensors 2021, 21, 3436. [Google Scholar] [CrossRef] [PubMed]
- Feng, D.; Lu, L.; Yuan-Wu, Y.; Li, G.Y.; Li, S.; Feng, G. Device-to-device communications in cellular networks. IEEE Commun. Mag. 2014, 52, 49–55. [Google Scholar] [CrossRef]
- Lee, J.; Lee, J.H. Performance Analysis and Resource Allocation for Cooperative D2D Communication in Cellular Networks With Multiple D2D Pairs. IEEE Commun. Lett. 2019, 23, 909–912. [Google Scholar] [CrossRef]
- Doppler, K.; Rinne, M.; Wijting, C.; Ribeiro, C.B.; Hugl, K. Device-to-device communication as an underlay to LTE-advanced networks. IEEE Commun. Mag. 2009, 47, 42–49. [Google Scholar] [CrossRef]
- Ghazanfari, A.; Björnson, E.; Larsson, E.G. Optimized Power Control for Massive MIMO With Underlaid D2D Communications. IEEE Trans. Commun. 2019, 67, 2763–2778. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, T.H.; Chien, T.V.; Ngo, H.Q.; Tran, X.N.; Björnson, E. Pilot Assignment for Joint Uplink-Downlink Spectral Efficiency Enhancement in Massive MIMO Systems With Spatial Correlation. IEEE Trans. Veh. Technol. 2021, 70, 8292–8297. [Google Scholar] [CrossRef]
- He, A.; Wang, L.; Chen, Y.; Wong, K.; Elkashlan, M. Spectral and Energy Efficiency of Uplink D2D Underlaid Massive MIMO Cellular Networks. IEEE Trans. Commun. 2017, 65, 3780–3793. [Google Scholar] [CrossRef] [Green Version]
- Xu, C.; Feng, J.; Huang, B.; Zhou, Z.; Mumtaz, S.; Rodriguez, J. Joint Relay Selection and Resource Allocation for Energy-Efficient D2D Cooperative Communications Using Matching Theory. Appl. Sci. 2017, 7, 491. [Google Scholar] [CrossRef] [Green Version]
- Kamal, M.S.; Kader, M.F.; Islam, S.M.R.; Yu, H. Device-to-Device Aided Cooperative Relaying Scheme Exploiting Spatial Modulation: An Interference Free Strategy. Sensors 2020, 20, 7048. [Google Scholar] [CrossRef]
- Abro, A.; Deng, Z.; Memon, K.A. A Lightweight Elliptic-Elgamal-Based Authentication Scheme for Secure Device-to-Device Communication. Future Internet 2019, 11, 108. [Google Scholar] [CrossRef] [Green Version]
- Xin, J.; Zhu, Q.; Liang, G.; Zhang, T. Performance Analysis of D2D Communication with Retransmission Mechanism in Cellular Networks. Appl. Sci. 2020, 10, 1097. [Google Scholar] [CrossRef] [Green Version]
- Mustafa, H.A.; Shakir, M.Z.; Imran, M.A.; Imran, A.; Tafazolli, R. Coverage Gain and Device-to-Device User Density: Stochastic Geometry Modeling and Analysis. IEEE Commun. Lett. 2015, 19, 1742–1745. [Google Scholar] [CrossRef] [Green Version]
- Tu, L.T.; Di Renzo, M. On the Energy Efficiency of Heterogeneous Cellular Networks With Renewable Energy Sources—A Stochastic Geometry Framework. IEEE Trans. Wirel. Commun. 2020, 19, 6752–6770. [Google Scholar] [CrossRef]
- Qiao, J.; Shen, X.S.; Mark, J.W.; Shen, Q.; He, Y.; Lei, L. Enabling device-to-device communications in millimeter-wave 5G cellular networks. IEEE Commun. Mag. 2015, 53, 209–215. [Google Scholar] [CrossRef]
- Al Hajj, M.; Wang, S.; Thanh Tu, L.; Azzi, S.; Wiart, J. A Statistical Estimation of 5G Massive MIMO Networks’ Exposure Using Stochastic Geometry in mmWave Bands. Appl. Sci. 2020, 10, 8753. [Google Scholar] [CrossRef]
- Tu, L.; Di Renzo, M. Analysis of millimeter wave cellular networks with simultaneous wireless information and power transfer. In Proceedings of the 2017 International Conference on Recent Advances in Signal Processing, Telecommunications & Computing (SigTelCom), Danang, Vietnam, 9–11 January 2017; pp. 39–43. [Google Scholar] [CrossRef] [Green Version]
- Giatsoglou, N.; Ntontin, K.; Kartsakli, E.; Antonopoulos, A.; Verikoukis, C. D2D-Aware Device Caching in mmWave-Cellular Networks. IEEE J. Sel. Areas Commun. 2017, 35, 2025–2037. [Google Scholar] [CrossRef] [Green Version]
- Alves, L.H.d.O.; Rebelatto, J.L.; Souza, R.D.; Brante, G. Network-Coded Cooperative LoRa Network with D2D Communication. IEEE Internet Things J. 2021. [Google Scholar] [CrossRef]
- Tu, L.T.; Bradai, A.; Pousset, Y. Coverage Probability and Spectral Efficiency Analysis of Multi-Gateway Downlink LoRa Networks. 10 Feb 2022. arXiv 2022, arXiv:2202.05014. [Google Scholar]
- Ming-Chun, L.; Molisch, A.F.; Ji, M. Throughput-Outage Scaling Behaviors for Wireless Single-Hop D2D Caching Networks with Physical Model–Analysis and Derivations. arXiv 2021, arXiv:2106.00300. [Google Scholar]
- Amer, A.; Ahmad, A.-M.; Hoteit, S. Resource Allocation for Downlink Full-Duplex Cooperative NOMA-Based Cellular System with Imperfect SI Cancellation and Underlaying D2D Communications. Sensors 2021, 21, 2768. [Google Scholar] [CrossRef] [PubMed]
- Do, D.-T.; Nguyen, M.-S.V.; Lee, B.M. Outage Performance Improvement by Selected User in D2D Transmission and Implementation of Cognitive Radio-Assisted NOMA. Sensors 2019, 19, 4840. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, X.; Cheng, W.; Xu, X. On the Exact and Asymptotic Analysis of Wireless Transmission over α − μ/Inverse Gamma Composite Fading Channels. In Proceedings of the 2020 International Conference on Wireless Communications and Signal Processing (WCSP), Nanjing, China, 21–23 October 2020; pp. 789–794. [Google Scholar] [CrossRef]
- Cheng, W.; Wang, X.; Xu, X. On the Performance Analysis of Wireless Transmission over α − μ/Inverse Gamma Composite Fading Channels. In Proceedings of the 2020 IEEE/CIC International Conference on Communications in China (ICCC Workshops), Xiamen, China, 28–30 July 2020; pp. 64–69. [Google Scholar] [CrossRef]
- Mankar, P.D.; Chen, Z.; Abd-Elmagid, M.A.; Pappas, N.; Dhillon, H.S. Throughput and Age of Information in a Cellular-Based IoT Network. IEEE Trans. Wirel. Commun. 2021, 20, 8248–8263. [Google Scholar] [CrossRef]
- Gorantla, B.V.R.; Mehta, N.B. Subchannel Allocation with Low Computational and Signaling Complexity in 5G D2D Networks. In Proceedings of the ICC 2021-IEEE International Conference on Communications, Xiamen, China, 28–30 July 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Cai, Y.; Ke, C.; Ni, Y.; Zhang, J.; Zhu, H. Power allocation for NOMA in D2D relay communications. China Commun. 2021, 18, 61–69. [Google Scholar] [CrossRef]
- Nguyen, P.C.; Rao, B.D. Fair Scheduling Policies Exploiting Multiuser Diversity in Cellular Systems With Device-to-Device Communications. IEEE Trans. Wirel. Commun. 2015, 14, 4757–4771. [Google Scholar] [CrossRef]
- Gu, J.; Bae, S.J.; Hasan, S.F.; Chung, M.Y. Heuristic Algorithm for Proportional Fair Scheduling in D2D-Cellular Systems. IEEE Trans. Wirel. Commun. 2016, 15, 769–780. [Google Scholar] [CrossRef]
- Fan, B.; Tian, H.; Jiang, L.; Vasilakos, A.V. A Social-Aware Virtual MAC Protocol for Energy-Efficient D2D Communications Underlying Heterogeneous Cellular Networks. IEEE Trans. Veh. Technol. 2018, 67, 8372–8385. [Google Scholar] [CrossRef]
- Saraereh, O.A.; Mohammed, S.L.; Khan, I.; Rabie, K.; Affess, S. An Efficient Resource Allocation Algorithm for Device-To-Device Communications. Appl. Sci. 2019, 9, 3816. [Google Scholar] [CrossRef] [Green Version]
- Zhang, L.; Xiao, M.; Wu, G.; Li, S. Efficient Scheduling and Power Allocation for D2D-Assisted Wireless Caching Networks. IEEE Trans. Commun. 2016, 64, 2438–2452. [Google Scholar] [CrossRef] [Green Version]
- Lin, Z.; Song, H.; Pan, D. A Joint Power and Channel Scheduling Scheme for Underlay D2D Communications in the Cellular Network. Sensors 2019, 19, 4799. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Song, X.; Ma, Y. A Novel Resource Allocation Scheme in NOMA-Based Cellular Network with D2D Communications. Future Internet 2020, 12, 8. [Google Scholar] [CrossRef] [Green Version]
- Yuan, H.; Guo, W.; Wang, S. Emergency route selection for D2D cellular communications during an urban terrorist attack. In Proceedings of the 2014 IEEE International Conference on Communications Workshops (ICC), Sydney, Australia, 10–14 June 2014; pp. 237–242. [Google Scholar] [CrossRef]
- Yuan, H.; Guo, W.; Jin, Y.L.; Wang, S.; Ni, M. Interference-Aware Multi-Hop Path Selection for Device-to-Device Communications in a Cellular Interference Environment. IET Commun. 2017, 11, 1741–1750. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.; Tang, J.; Coon, J.P. Optimal Routing for Multihop Social-Based D2D Communications in the Internet of Things. IEEE Internet Things J. 2018, 5, 1880–1889. [Google Scholar] [CrossRef] [Green Version]
- Singh, I.; Jaiswal, R.K.; Kumar, V.; Verma, R.; Singh, N.P.; Singh, G. Outage Probability of Device-to-Device Communication Underlaying Cellular Network over Nakagami/Rayleigh Fading Channels. In Proceedings of the 2019 9th International Conference on Emerging Trends in Engineering and Technology-Signal and Information Processing (ICETET-SIP-19), Nagpur, India, 1–2 November 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Jose, J.; Agarwal, A.; Gangopadhyay, R.; Debnath, S. Outage Analysis based Channel Allocation for Underlay D2D Communication in Fading Scenarios. In Proceedings of the 2019 International Conference on Wireless Communications Signal Processing and Networking (WiSPNET), Chennai, India, 21–23 March 2019; pp. 485–490. [Google Scholar] [CrossRef]
- Rodziewicz, M. Outage Probability of Device-to-Device Communications in Frequency Reuse-1 Networks. Mob. Netw. Appl. 2017, 22, 1058–1064. [Google Scholar] [CrossRef] [Green Version]
- Kusaladharma, S.; Zhang, Z.; Tellambura, C. Interference and Outage Analysis of Random D2D Networks Underlaying Millimeter-Wave Cellular Networks. IEEE Trans. Commun. 2019, 67, 778–790. [Google Scholar] [CrossRef]
- Hussain, Z.; Khan, A.u.R.; Mehdi, H.; Saleem, S.M.A. Analysis of D2D Communications over Gamma/Nakagami Fading Channels. Eng. Technol. Appl. Sci. Res. 2018, 8, 2693–2698. [Google Scholar] [CrossRef]
- Liu, Y.; Ding, Z.; Elkashlan, M.; Yuan, J. Nonorthogonal Multiple Access in Large-Scale Underlay Cognitive Radio Networks. IEEE Trans. Veh. Technol. 2016, 65, 10152–10157. [Google Scholar] [CrossRef] [Green Version]
- Renzo, M.D.; Zappone, A.; Lam, T.T.; Debbah, M. System-Level Modeling and Optimization of the Energy Efficiency in Cellular Networks—A Stochastic Geometry Framework. IEEE Trans. Wirel. Commun. 2018, 17, 2539–2556. [Google Scholar] [CrossRef]
- Thanh, T.L.; Renzo, M.D.; Coon, J.P. MIMO cellular networks with Simultaneous Wireless Information and Power Transfer. In Proceedings of the 2016 IEEE 17th International Workshop on Signal Processing Advances in Wireless Communications (SPAWC), Edinburgh, UK, 3–6 July 2016; pp. 1–5. [Google Scholar] [CrossRef] [Green Version]
- Tu, L.-T.; Bradai, A.; Pousset, Y.; Aravanis, A.I. Energy Efficiency Analysis of LoRa Networks. IEEE Wirel. Commun. Lett. 2021, 10, 1881–1885. [Google Scholar] [CrossRef]
- Xia, H.; Li, Y.; Zhang, H.; Natarajan, B. Availability of Ambient RF Energy in d-Dimensional Wireless Networks. Energies 2018, 11, 668. [Google Scholar] [CrossRef] [Green Version]
- Chien, T.V.; Björnson, E.; Larsson, E.G. Joint Pilot Design and Uplink Power Allocation in Multi-Cell Massive MIMO Systems. IEEE Trans. Wirel. Commun. 2018, 17, 2000–2015. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, T.N.; Tran, M.; Nguyen, T.-L.; Ha, D.-H.; Voznak, M. Multisource Power Splitting Energy Harvesting Relaying Network in Half-Duplex System over Block Rayleigh Fading Channel: System Performance Analysis. Electronics 2019, 8, 67. [Google Scholar] [CrossRef] [Green Version]
- Tin, P.T.; Phan, V.-D.; Nguyen, T.N.; Tu, L.-T.; Minh, B.V.; Voznak, M.; Fazio, P. Outage Analysis of the Power Splitting Based Underlay Cooperative Cognitive Radio Networks. Sensors 2021, 21, 7653. [Google Scholar] [CrossRef]
- Aravanis, A.I.; Tu, L.-T.; Muñoz, O.; Pascual-Iserte, A.; Di Renzo, M. A tractable closed form approximation of the ergodic rate in Poisson cellular networks. EURASIP J. Wirel. Commun. Netw. 2019, 2019, 187. [Google Scholar] [CrossRef]
- Nguyen, T.H.; Jung, W.; Tu, L.T.; Chien, T.V.; Yoo, D.; Ro, S. Performance Analysis and Optimization of the Coverage Probability in Dual Hop LoRa Networks With Different Fading Channels. IEEE Access 2020, 8, 107087–107102. [Google Scholar] [CrossRef]
- Chen, Z.; Ye, C.; Yuan, J.; Han, D. MGF-Based Mutual Approximation of Hybrid Fading: Performance of Wireless/Power Line Relaying Communication for IoT. Sensors 2019, 19, 2460. [Google Scholar] [CrossRef] [Green Version]
- Tin, P.T.; Nguyen, T.N.; Tran, D.-H.; Voznak, M.; Phan, V.-D.; Chatzinotas, S. Performance Enhancement for Full-Duplex Relaying with Time-Switching-Based SWIPT in Wireless Sensors Networks. Sensors 2021, 21, 3847. [Google Scholar] [CrossRef]
- Tu, L.-T.; Tung, P.L.; Chien, T.V.; Duy, T.T.; Hoa, N.T. Performance Evaluation of Incremental Relaying in Underlay Cognitive Radio Networks with Imperfect CSI. In Proceedings of the ICCE 2020, Phu Quoc Island, Vietnam, 13–15 January 2021; pp. 472–477. [Google Scholar] [CrossRef]
- Nguyen, T.N.; Quang Minh, T.H.; Tran, P.T.; Vozňák, M. Energy Harvesting over Rician Fading Channel: A Performance Analysis for Half-Duplex Bidirectional Sensor Networks under Hardware Impairments. Sensors 2018, 18, 1781. [Google Scholar] [CrossRef] [Green Version]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products, 7th ed.; Elsevier; Academic Press: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Lopez-Fernandez, J.; Lopez-Martinez, F.J. New Results on the Second Order Scattering Fading Model: Amount of Fading and Energy Detection. IEEE Trans. Veh. Technol. 2020, 69, 1037–1040. [Google Scholar] [CrossRef]
- Renzo, M.D.; Lam, T.T.; Zappone, A.; Debbah, M. A Tractable Closed-Form Expression of the Coverage Probability in Poisson Cellular Networks. IEEE Wirel. Commun. Lett. 2019, 8, 249–252. [Google Scholar] [CrossRef]
- Lam, T.T.; Renzo, M.D.; Coon, J.P. System-level analysis of receiver diversity in SWIPT-enabled cellular networks. J. Commun. Netw. 2016, 18, 926–937. [Google Scholar] [CrossRef] [Green Version]
- Thanh, T.L.; Renzo, M.D.; Coon, J.P. Stochastic Geometry Analysis of Receiver Diversity in Cellular Networks with SWIPT. In Proceedings of the 2018 IEEE 19th International Workshop on Signal Processing Advances in Wireless Communications (SPAWC), Kalamata, Greece, 25–28 June 2018; pp. 1–5. [Google Scholar] [CrossRef] [Green Version]
- Minallah, N.; Ullah, K.; Frnda, J.; Cengiz, K.; Awais Javed, M. Transmitter Diversity Gain Technique Aided Irregular Channel Coding for Mobile Video Transmission. Entropy 2021, 23, 235. [Google Scholar] [CrossRef] [PubMed]
- Do, D.-T.; Van Nguyen, M.-S.; Hoang, T.-A.; Lee, B.M. Exploiting Joint Base Station Equipped Multiple Antenna and Full-Duplex D2D Users in Power Domain Division Based Multiple Access Networks. Sensors 2019, 19, 2475. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, J.; Song, X.; Ma, Y.; Xie, Z. Power Efficient Secure Full-Duplex SWIPT Using NOMA and D2D with Imperfect CSI. Sensors 2020, 20, 5395. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, G.; Xu, H.; Ren, Y.; Chen, X.; Li, R. Outage Constrained Design in NOMA-Based D2D Offloading Systems. Electronics 2022, 11, 256. [Google Scholar] [CrossRef]
- Huang, W.; Han, Z.; Zhao, L.; Xu, H.; Li, Z.; Wang, Z. Resource Allocation for Intelligent Reflecting Surfaces Assisted Federated Learning System with Imperfect CSI. Algorithms 2021, 14, 363. [Google Scholar] [CrossRef]
- Thanh, T.L.; Bao, V.N.Q.; An, B. On the performance of outage probability in underlay cognitive radio with imperfect CSI. In Proceedings of the 2013 International Conference on Advanced Technologies for Communications (ATC 2013), Ho Chi Minh City, Vietnam, 16–18 October 2013; pp. 125–130. [Google Scholar] [CrossRef]
- Chien, T.V.; Tu, L.T.; Chatzinotas, S.; Ottersten, B. Coverage Probability and Ergodic Capacity of Intelligent Reflecting Surface-Enhanced Communication Systems. IEEE Commun. Lett. 2021, 25, 69–73. [Google Scholar] [CrossRef]
- Chien, T.V.; Papazafeiropoulos, A.K.; Tu, L.T.; Chopra, R.; Chatzinotas, S.; Ottersten, B. Outage Probability Analysis of IRS-Assisted Systems Under Spatially Correlated Channels. IEEE Wirel. Commun. Lett. 2021, 10, 1815–1819. [Google Scholar] [CrossRef]
- Chien, T.V.; Tu, L.T.; Tran, D.H.; Nguyen, H.V.; Chatzinotas, S.; Renzo, M.D.; Ottersten, B. Controlling smart propagation environments: Long-term versus short-term phase shift optimization. In Proceedings of the IEEE ICASSP 2022, Singapore, 22–27 May 2022. [Google Scholar]
- Cadambe, V.R.; Jafar, S.A. Interference Alignment and Degrees of Freedom of the K-User Interference Channel. IEEE Trans. Inf. Theory 2008, 54, 3425–3441. [Google Scholar] [CrossRef] [Green Version]
- Song, J.; Tu, L.; Renzo, M.D. On the feasibility of interference alignment in ultra-dense millimeter-wave cellular networks. In Proceedings of the 2016 50th Asilomar Conference on Signals, Systems and Computers, Pacific Grove, CA, USA, 6–9 November 2016; pp. 1176–1180. [Google Scholar] [CrossRef]




| Symbol | Definition |
|---|---|
| , | Expectation and probability operators |
| Channel coefficient between transmitter i and receiver j | |
| Large-scale path-loss between transmitter i and receiver j | |
| , | Path-loss constant, speed of light |
| v, , | Wavelength, carrier frequency, path-loss exponent |
| Transmission distance from node i to node j | |
| Total transmit power of D2D networks | |
| Transmit power of the k-th D2D transmitter under s scheme | |
| Transmit power of the cellular user | |
| Aggregate interference at the k-th D2D receiver from D2D networks | |
| Aggregate interference at the BS from D2D networks | |
| Weighted coefficient of the k-th D2D transmitter under random scheme | |
| N, O | Number of pair of D2D users, network area |
| , | Transmitted signals of the k-th D2D transmitter and cellular user |
| , | Received signals at the k-th D2D receiver and base station |
| , | AWGN noise at the k-th D2D receiver and base station |
| , | Noise variance at the k-th D2D receiver and base station |
| NF, Bw | Noise figure, transmission bandwidth |
| R, | Targeted rate of D2D networks and cellular networks |
| , | Exponential and logarithm functions |
| Exponential Integral function | |
| Cumulative distribution function (CDF) of RV X | |
| Complementary Cumulative distribution function (CCDF) of RV X | |
| Moment generating function (MGF) of RV X | |
| Probability density function (PDF) of RV X | |
| OP | Outage probability of the k-th D2D user under s allocation scheme |
| Average rate of the k-th D2D user under s allocation scheme | |
| Average sum rate of the D2D networks under s allocation scheme | |
| Amount of fading of the k-th D2D user under s allocation scheme | |
| Variance operator | |
| Coverage probability of cellular user under s allocation scheme | |
| BER | Bit error rate |
| BS | Base station |
| D2D | Device-to-device |
| FDMA | Frequency-division multiple access |
| LoRa | Long-range |
| MIMO | Multiple-input multiple-output |
| NOMA | Non-orthogonal multiple access |
| QoS | Quality-of-service |
| RVs | Random variables |
| SINR | Signal-to-interference-plus-noise ratio |
| TDMA | Time-division multiple access |
| e2e | end-to-end |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, T.N.; Nguyen, V.S.; Nguyen, H.G.; Tu, L.T.; Van Chien, T.; Nguyen, T.H. On the Performance of Underlay Device-to-Device Communications. Sensors 2022, 22, 1456. https://doi.org/10.3390/s22041456
Nguyen TN, Nguyen VS, Nguyen HG, Tu LT, Van Chien T, Nguyen TH. On the Performance of Underlay Device-to-Device Communications. Sensors. 2022; 22(4):1456. https://doi.org/10.3390/s22041456
Chicago/Turabian StyleNguyen, Tan Nhat, Van Son Nguyen, Hoai Giang Nguyen, Lam Thanh Tu, Trinh Van Chien, and Tien Hoa Nguyen. 2022. "On the Performance of Underlay Device-to-Device Communications" Sensors 22, no. 4: 1456. https://doi.org/10.3390/s22041456
APA StyleNguyen, T. N., Nguyen, V. S., Nguyen, H. G., Tu, L. T., Van Chien, T., & Nguyen, T. H. (2022). On the Performance of Underlay Device-to-Device Communications. Sensors, 22(4), 1456. https://doi.org/10.3390/s22041456







