Locating and Imaging Fiber Breaks in CFRP Using Guided Wave Tomography and Eddy Current Testing
Abstract
:1. Introduction
2. Characteristics of the Specimen
3. Locating Fiber Breaks Using Guided Wave Tomography
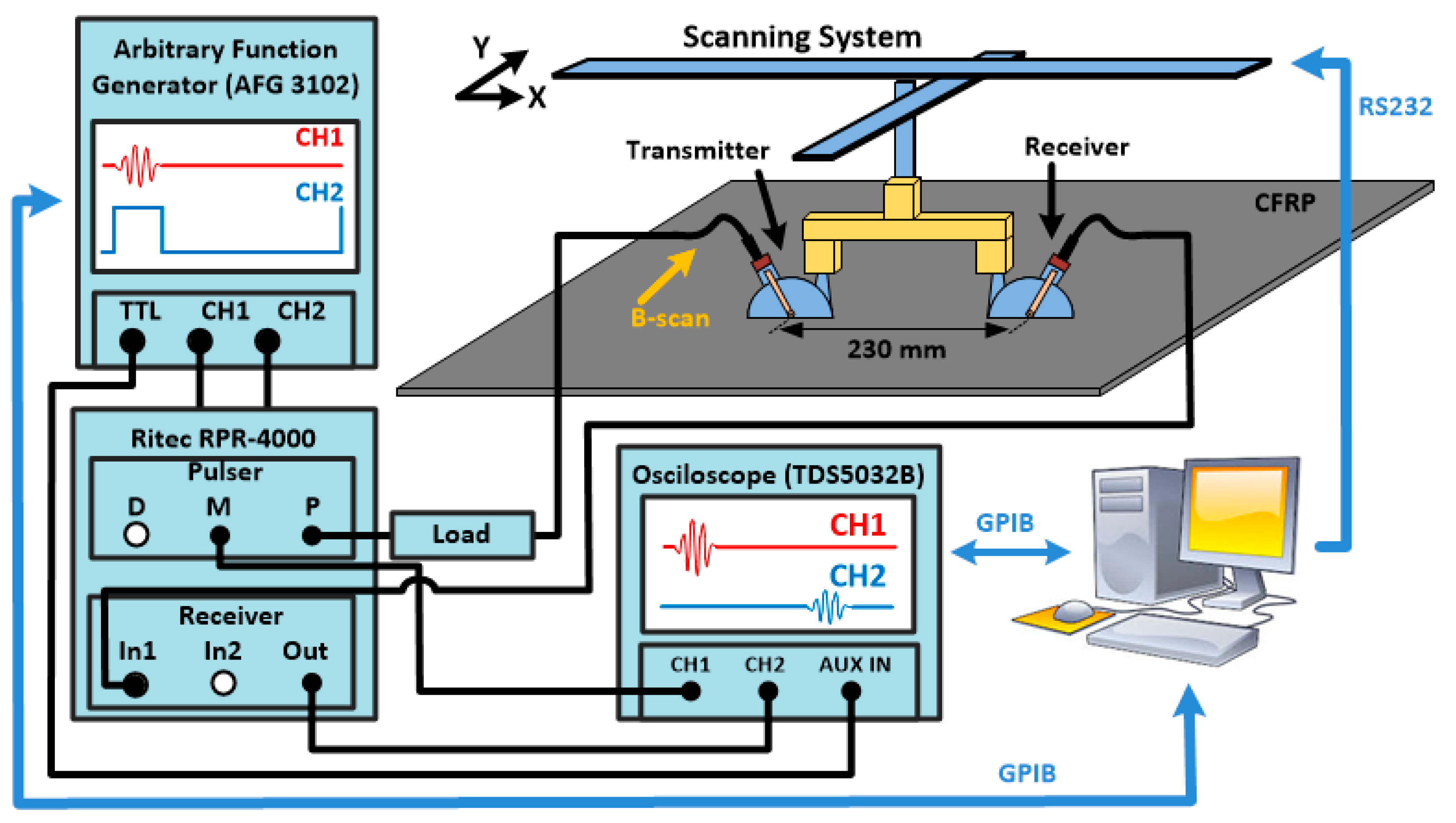
3.1. Experimental Setup for Guided Wave Testing
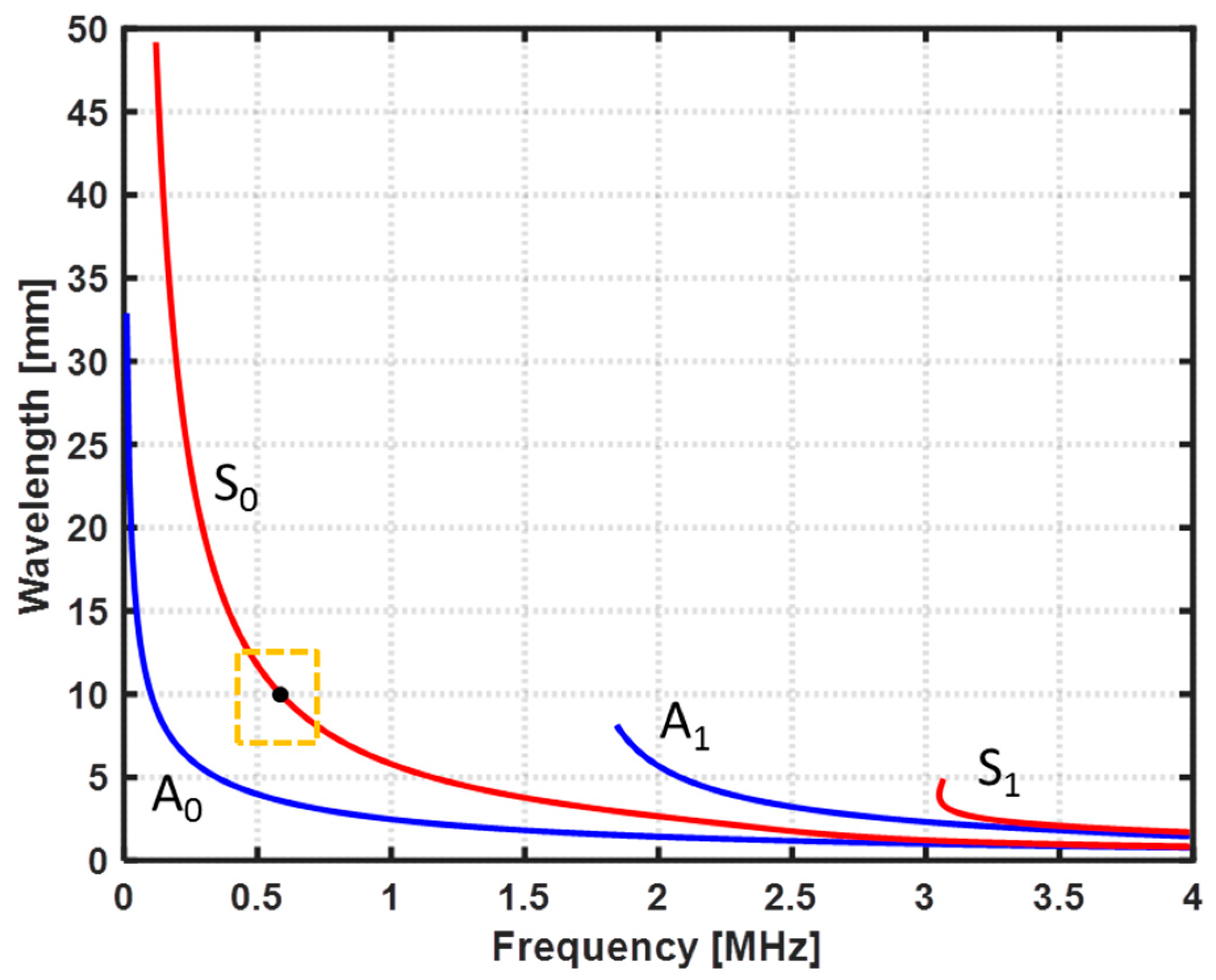
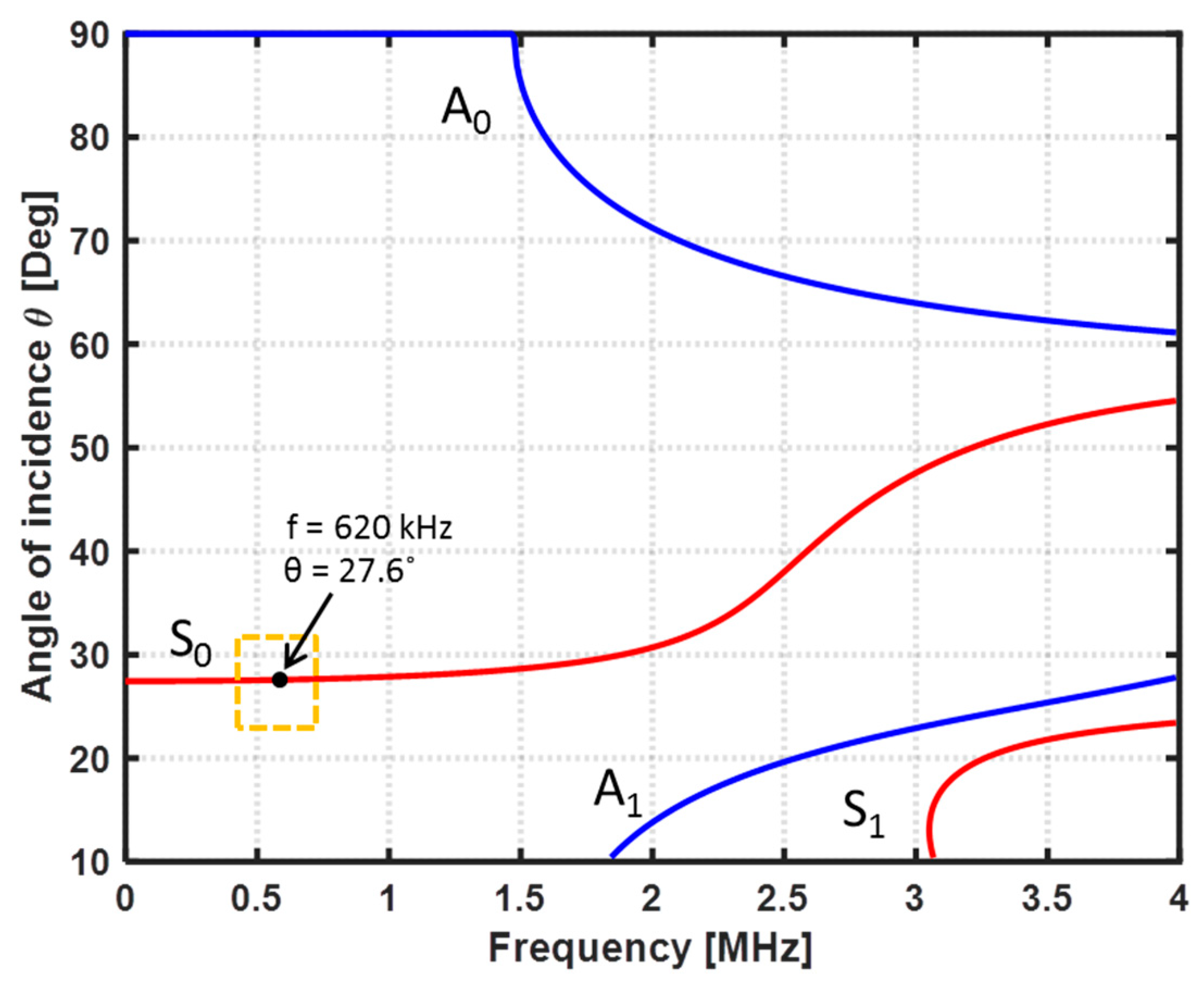
3.2. Selection of the Frequency and the Incident Angle of the Excitation Source
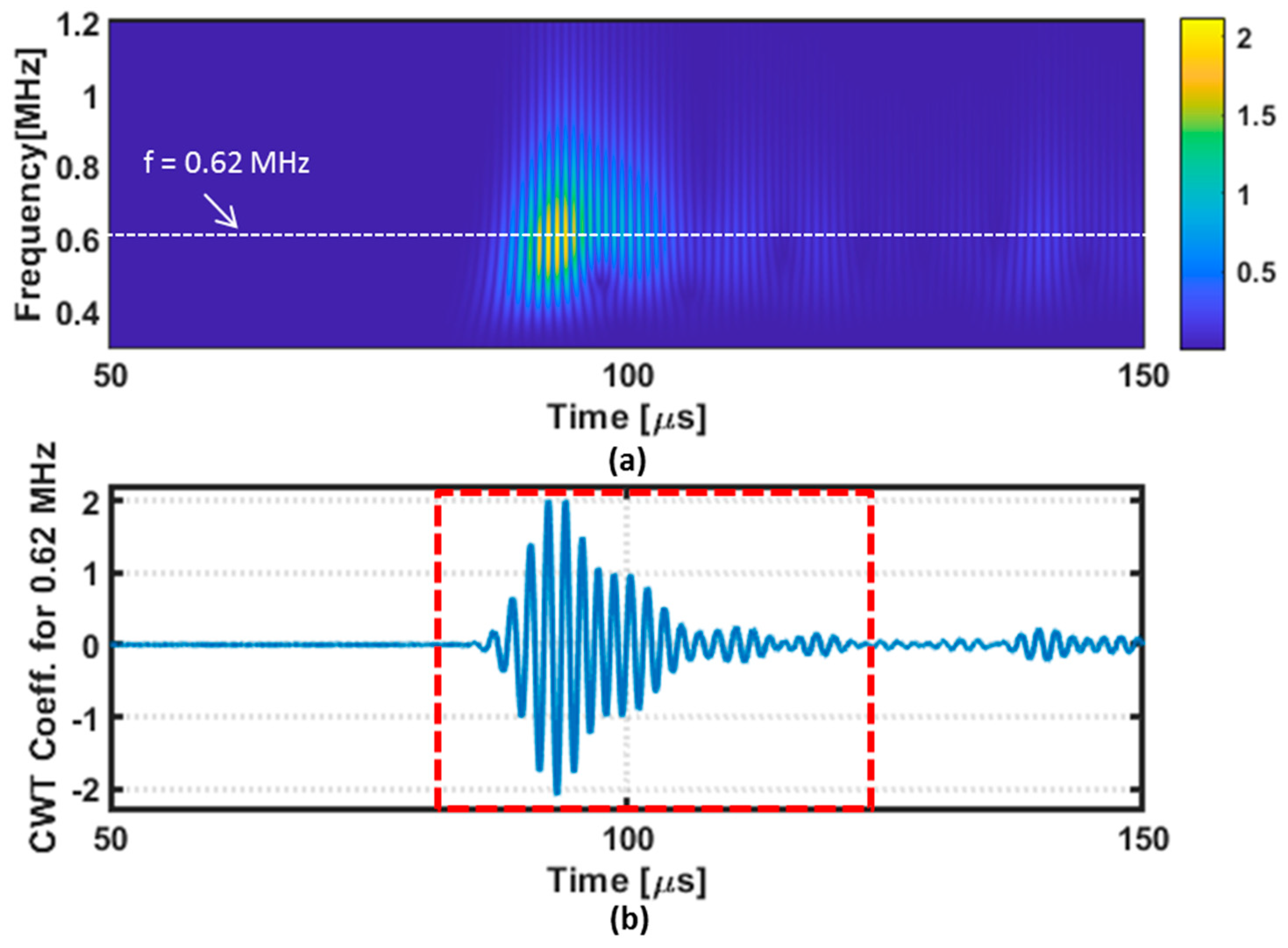
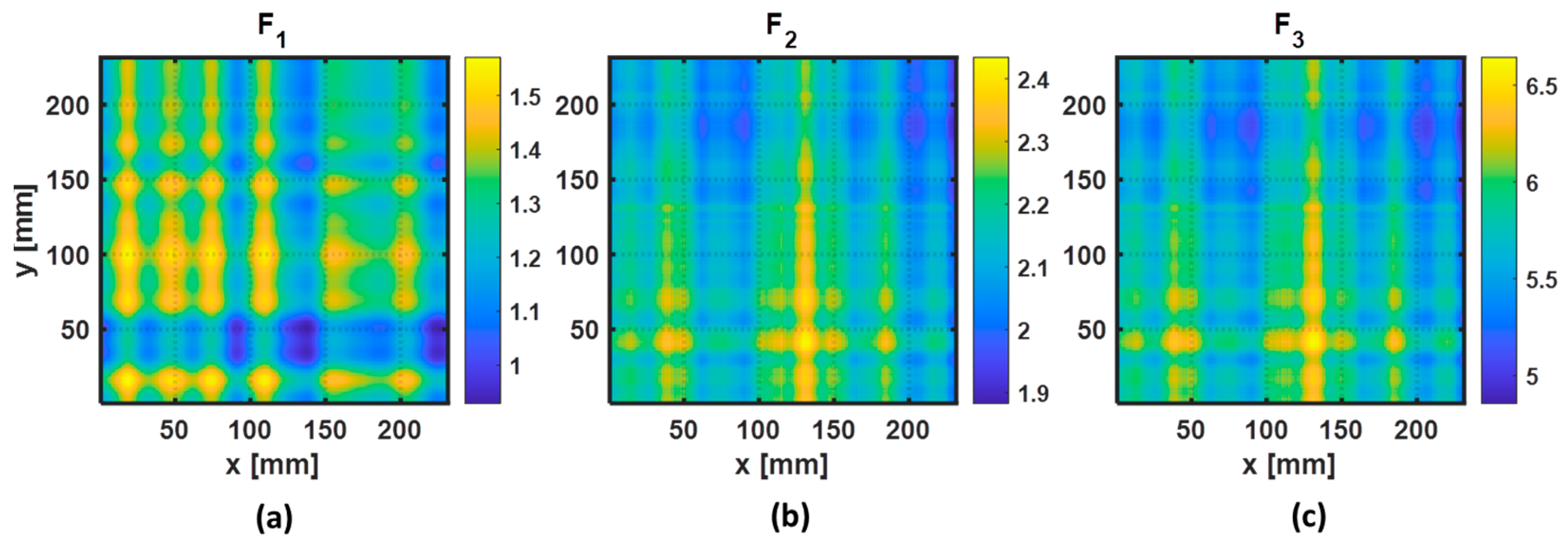
3.3. Experimental Measurements and Feature Extraction
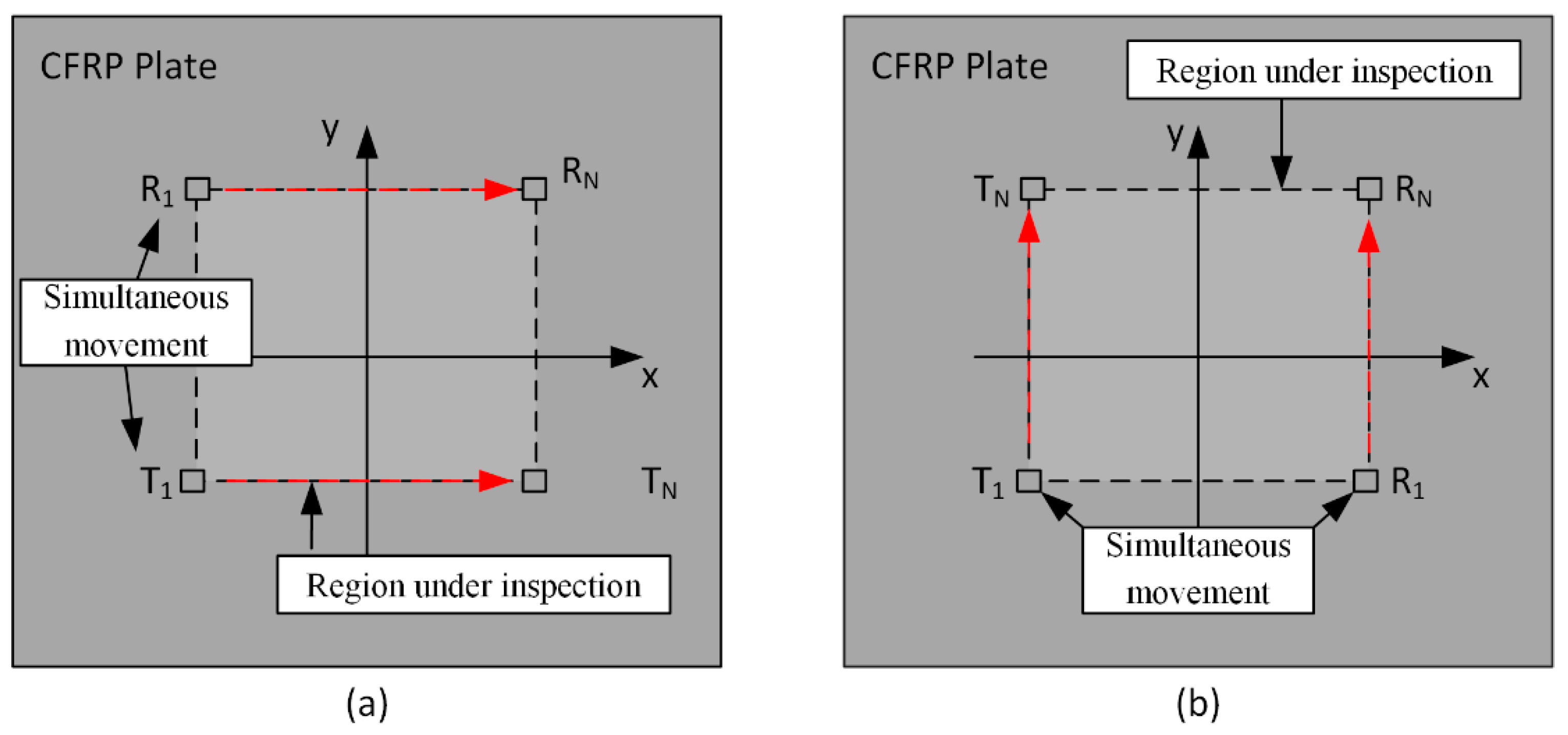
3.4. GWT Scan Procedure
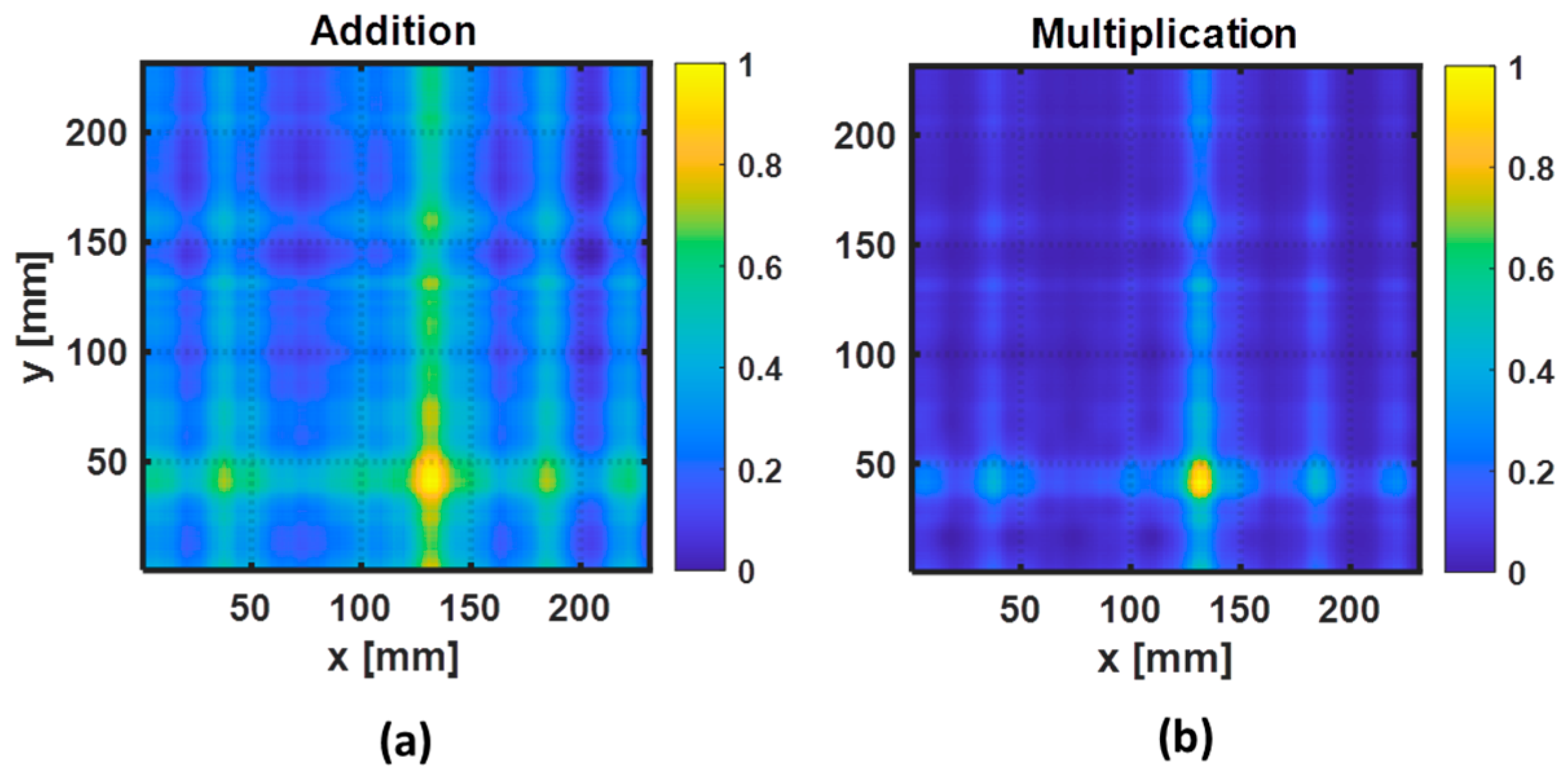
3.5. Method Used to Locate Fiber Breaks
4. Evaluating the Fiber Break Using an ECT Probe
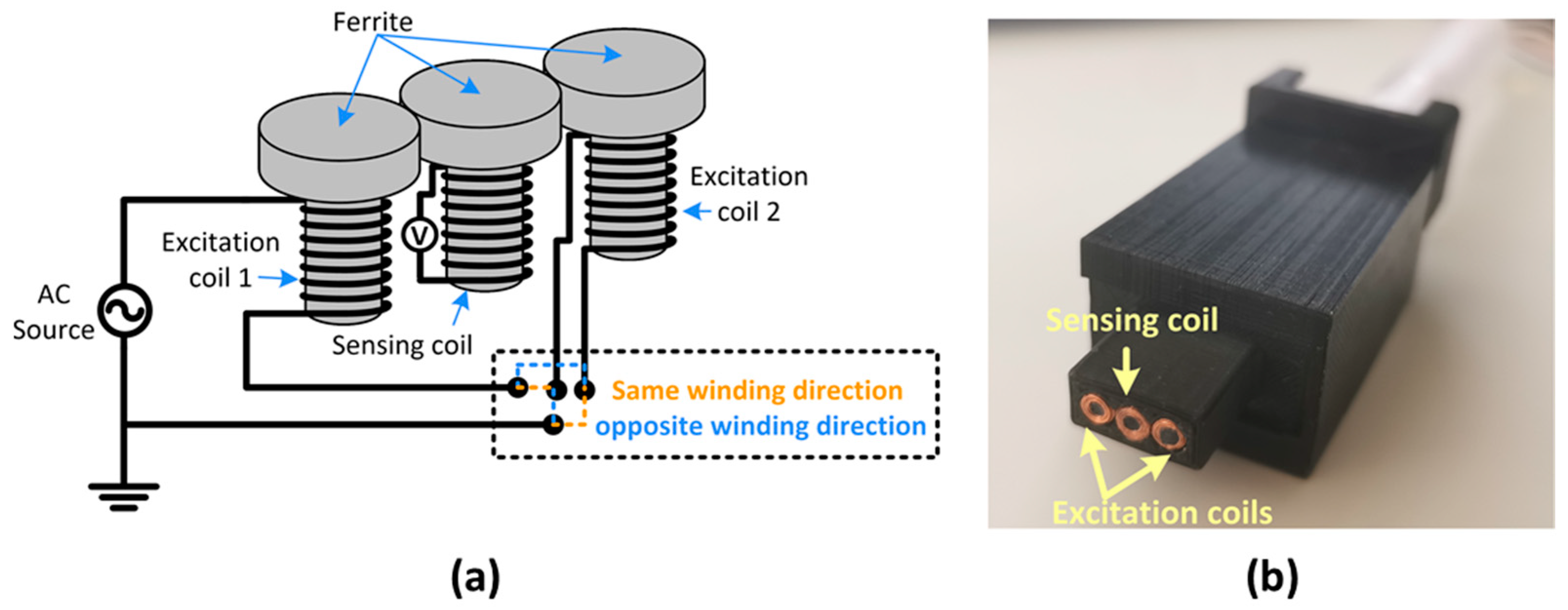
4.1. ECT Probe
4.2. ECT Scan Procedure
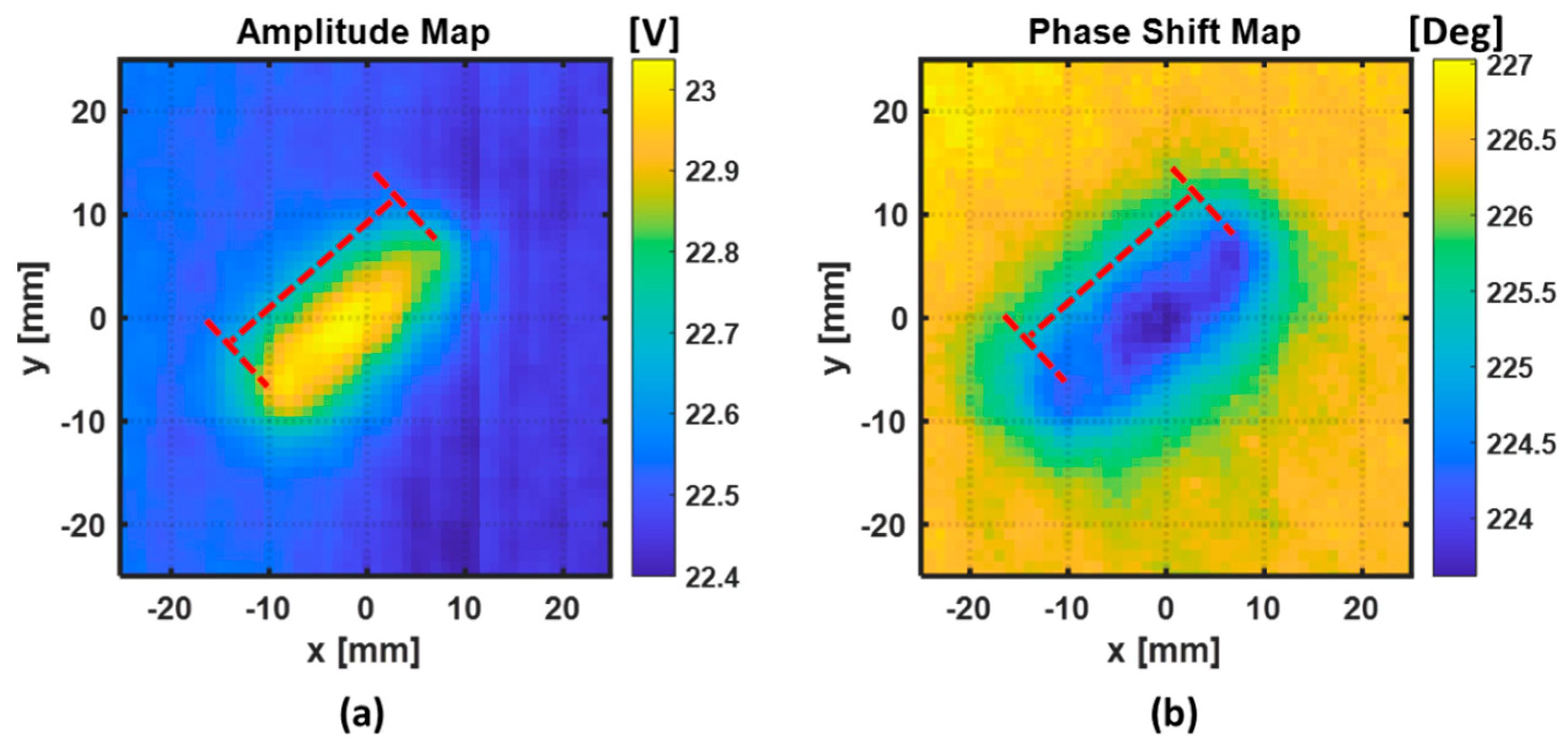
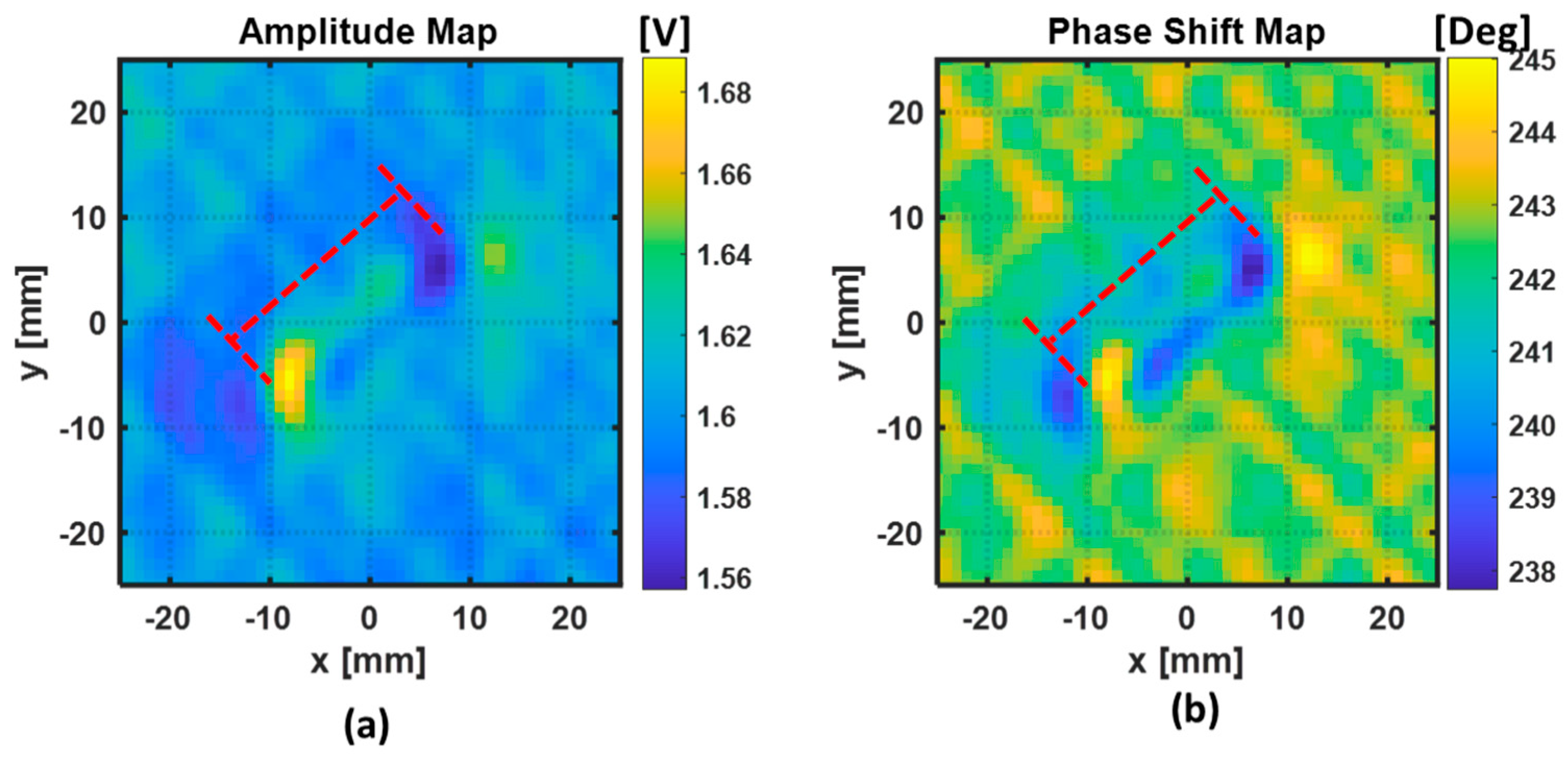
4.3. Characterization of the Fiber Break
5. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wallentine, S.M.; Uchic, M.D. A study on ground truth data for impact damaged polymer matrix composites. AIP Conf. Proc. 2018, 1949, 120002. [Google Scholar] [CrossRef]
- Yilmaz, B.; Ba, A.; Jasiuniene, E.; Bui, H.-K.; Berthiau, G. Evaluation of Bonding Quality with Advanced Nondestructive Testing (NDT) and Data Fusion. Sensors 2020, 20, 5127. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Xu, C.; Qi, B.; Hernandez, F. Detection of impact damage on PVA-ECC beam using infrared thermography. Appl. Sci. 2018, 8, 839. [Google Scholar] [CrossRef]
- Jun, C.; Qu, J.; Xu, X.; Ji, H. Research Advances in Eddy Current Testing for Maintenance of Carbon Fiber Reinforced Plastic Composites. Int. J. Appl. Electromagn. Mech. 2016, 51, 261–284. [Google Scholar] [CrossRef]
- James, R.; Faisal Haider, M.; Giurgiutiu, V.; Lilienthal, D. A Simulative and Experimental Approach toward Eddy Current Nondestructive Evaluation of Manufacturing Flaws and Operational Damage in CFRP Composites. J. Nondestruct. Eval. Diagn. Progn. Eng. Syst. 2020, 3, 011002. [Google Scholar] [CrossRef]
- Rao, B.P.C. Practical Eddy Current Testing; Alpha Science International Ltd.: Oxford, UK, 2007. [Google Scholar]
- Sardellitti, A.; Capua, G.D.; Laracca, M.; Tamburrino, A.; Ventr, S.; Ferrigno, L. A Fast ECT Measurement Method for the Thickness of Metallic Plates. IEEE Trans. Instrum. Meas. 2022, 71, 1–12. [Google Scholar] [CrossRef]
- Pasadas, D.J.; Ribeiro, A.L.; Ramos, H.G.; Rocha, T.J. Inspection of Cracks in Aluminum Multilayer Structures Using Planar ECT Probe and Inversion Problem. IEEE Trans. Instrum. Meas. 2017, 66, 920–927. [Google Scholar] [CrossRef]
- Yin, W.; Withers, P.J.; Sharma, U.; Peyton, A.J. Noncontact Characterization of Carbon-Fiber-Reinforced Plastics Using Multifrequency Eddy Current Sensors. IEEE Trans. Instrum. Meas. 2009, 58, 738–743. [Google Scholar] [CrossRef]
- Wu, D.; Cheng, F.; Yang, F.; Huang, C. Non-destructive testing for carbon-fiber-reinforced plastic (CFRP) using a novel eddy current probe. Compos. Part B Eng. 2019, 177, 107460. [Google Scholar] [CrossRef]
- Pasadas, D.J.; Ramos, H.G.; Baskaran, P.; Ribeirio, A.L. ECT in composite materials using double excitation coils and resonant excitation/sensing circuits. Measurement 2020, 161, 107859. [Google Scholar] [CrossRef]
- Rose, J.L. Ultrasonic Guided Waves in Solid Media; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Olisa, S.C.; Khan, M.A.; Starr, A. Review of Current Guided Wave Ultrasonic Testing (GWUT) Limitations and Future Directions. Sensors 2021, 21, 811. [Google Scholar] [CrossRef]
- Giridhara, G.; Rathod, V.T.; Naik, S.; Mahapatra, D.R.; Gopalakrishnan, S. Rapid localization of damage using a circular sensor array and Lamb wave based triangulation. Mech. Syst. Signal Process. 2010, 24, 2929–2946. [Google Scholar] [CrossRef]
- Feng, B.; Pasadas, D.J.; Ribeiro, A.L.; Ramos, G.R. Locating Defects in Anisotropic CFRP Plates Using ToF-Based Probability Matrix and Neural Networks. IEEE Trans. Instrum. Meas. 2019, 68, 1252–1560. [Google Scholar] [CrossRef]
- Druet, T.; Recoquillay, A.; Chapuis, B.; Moulin, E. Passive guided wave tomography for structural health monitoring. J. Acoust. Soc. Am. Acoust. Soc. Am. 2019, 146, 2395–2403. [Google Scholar] [CrossRef] [PubMed]
- Hettler, J.; Tabatabateipour, M.; Delrue, S.; Abeele, K. Application of a Probabilistic Algorithm for Ultrasonic Guided Wave Imaging of Carbon Composites. Phys. Procedia 2015, 70, 664–667. [Google Scholar] [CrossRef]
- Mei, H.; James, R.; Haider, M.F.; Giurgiutiu, V. Multimode Guided Wave Detection for Various Composite Damage Types. Appl. Sci. 2020, 10, 484. [Google Scholar] [CrossRef]
- Li, J.; Lu, Y.; Guan, R.; Qu, W. Guided waves for debonding identification in CFRP reinforced concrete beams. Constr. Build. Mater. 2017, 131, 388–399. [Google Scholar] [CrossRef]
- Sikdar, S.; Fiborek, P.; Kudela, P.; Banerjee, S.; Ostachowicz, W. Effects of debonding on Lamb wave propagation in a bonded composite structure under variable temperature conditions. Smart Mater. Struct. 2018, 28, 015021. [Google Scholar] [CrossRef]
- Shelke, A.; Kundu, T.; Amjad, U.; Hahn, K.; Grill, W. Mode-selective excitation and detection of ultrasonic guided waves for delamination detection in laminated aluminum plates. IEEE Trans Ultrason Ferroelectr Freq Control. 2011, 58, 567–577. [Google Scholar] [CrossRef]
- Gómez-Ullate, Y.; Espinosa, F.; Reynolds, P.; Mould, J. Selective excitation of Lamb Wave Modes in Thin Aluminium Plates using Bonded Piezoceramics: FEM Modelling and Measurements. In Proceedings of the 9th European Conference on NDT, Berlin, Germany, 7–9 September 2006; pp. 25–29. [Google Scholar]
- Sharma, S.; Mukherjee, A. A Non-Contact Technique for Damage Monitoring in Submerged Plates Using Guided Waves. J. Test. Eval. 2015, 43, 4. [Google Scholar] [CrossRef]
- Li, Y.; He, C.Y.; Song, G.; Wu, B. Crack detection in monocrystalline silicon solar cells using air-coupled ultrasonic lamb waves. NDT E Int. 2019, 102, 129–136. [Google Scholar] [CrossRef]
- Zhu, Y.; Zeng, X.; Deng, M.; Han, K.; Gao, D. Mode selection of nonlinear Lamb wave based on approximate phase velocity matching. NDT E Int. 2019, 102, 295–303. [Google Scholar] [CrossRef]
- Huber, A. Open Source Dispersion Calculator V2 Software from German Aerospace Center (DLR) Institute of Structures and Design and Center for Lightweight Production Technology. Available online: https://www.dlr.de/zlp/en/desktopdefault.aspx/tabid-14332/24874_read-61142/ (accessed on 14 August 2022).
- Pasadas, D.J.; Baskaran, P.; Ramos, H.G.; Ribeiro, A.L. Detection and Classification of Defects Using ECT and Multi-Level SVM Model. IEEE Sens. J. 2020, 20, 2329–2338. [Google Scholar] [CrossRef]




| Parameter | Quantity | Units |
|---|---|---|
| Density | 1152 | Kg/m3 |
| Tensile Modulus at 45° | 32.3 | GPa |
| Tensile Modulus at 0/90° | 37.2 | GPa |
| Poisson ratio | 0.33 | - |
| Features | Description | Definition |
|---|---|---|
| F1 | Peak | |
| F2 | Skewness | |
| F3 | Kurtosis |
| Features | Feature Description | IRTvariation (defect region) | IRTavg (defect free region) |
|---|---|---|---|
| F1 | Peak | 0.42 | 1.35 |
| F2 | Skewness | 0.28 | 2.15 |
| F3 | Kurtosis | 0.94 | 5.70 |
| Probe Configuration | Ap2p (V) | Aavg (V) | θp2p (Deg.) | Θavg (Deg.) |
|---|---|---|---|---|
| 1 | 0.64 | 22.4 | 7.27 | 226.41 |
| 2 | 0.13 | 1.60 | 3.41 | 242.71 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pasadas, D.J.; Barzegar, M.; Ribeiro, A.L.; Ramos, H.G. Locating and Imaging Fiber Breaks in CFRP Using Guided Wave Tomography and Eddy Current Testing. Sensors 2022, 22, 7377. https://doi.org/10.3390/s22197377
Pasadas DJ, Barzegar M, Ribeiro AL, Ramos HG. Locating and Imaging Fiber Breaks in CFRP Using Guided Wave Tomography and Eddy Current Testing. Sensors. 2022; 22(19):7377. https://doi.org/10.3390/s22197377
Chicago/Turabian StylePasadas, Dario J., Mohsen Barzegar, Artur L. Ribeiro, and Helena G. Ramos. 2022. "Locating and Imaging Fiber Breaks in CFRP Using Guided Wave Tomography and Eddy Current Testing" Sensors 22, no. 19: 7377. https://doi.org/10.3390/s22197377
APA StylePasadas, D. J., Barzegar, M., Ribeiro, A. L., & Ramos, H. G. (2022). Locating and Imaging Fiber Breaks in CFRP Using Guided Wave Tomography and Eddy Current Testing. Sensors, 22(19), 7377. https://doi.org/10.3390/s22197377







