Reflection Characteristics Measurements of Indoor Wireless Link in D-Band
Abstract
:1. Introduction
2. Principle of Reflection Measurements and Theoretical Model
2.1. Reflection Measurement
2.2. Reflection Theoretical Model
2.3. Minimum Mean Square Error Criterion
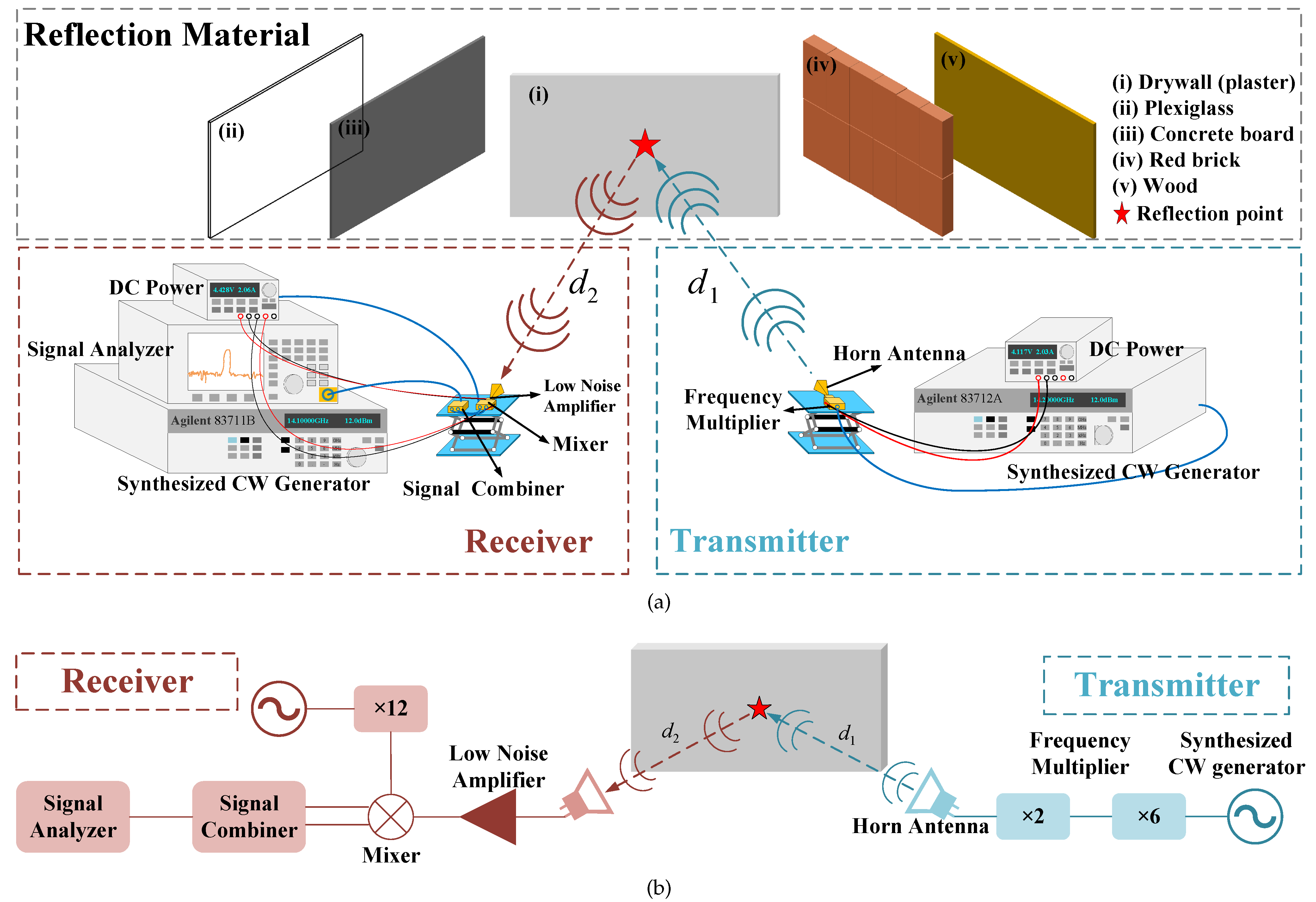
3. Methodology
- The reflection coefficients, expressed as , are measured based on Section 2.1;
- The ranges of two parameters, and , are set to calculate the theoretical reflection coefficients ;
- The dataset of theoretical is compared with that of measured to calculate the MSE;.
- With MMSE criterion, the set of theoretical closest to the measured is selected, and therefore, the optimal relative permittivity and optimal surface roughness are obtained in two ranges;
- These two optimal estimated parameters are compared with the data in previous research to prove the rationality and robustness of our method and experiment;
- Based on the steps above, the reflection characteristics can be analyzed.
4. Experimental Setup
5. Results and Discussions
5.1. Reflection Coefficients
5.2. Relative Permittivity
5.3. Surface Roughness
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Barnett, T.; Jain, S.; Andra, U.; Khurana, T. Cisco visual networking index (vni) complete forecast update, 2017–2022. In Americas/EMEAR Cisco Knowledge Network (CKN) Presentation; Cisco: San Francisco, CA, USA, 2018. [Google Scholar]
- Rappaport, T.S.; Xing, Y.; Kanhere, O.; Ju, S.; Madanayake, A.; Mandal, S.; Alkhateeb, A.; Trichopoulos, G.C. Wireless communications and applications above 100 GHz: Opportunities and challenges for 6G and beyond. IEEE Access 2019, 7, 78729–78757. [Google Scholar] [CrossRef]
- Kanhere, O.; Rappaport, T.S. Position location for futuristic cellular communications: 5G and beyond. IEEE Commun. Mag. 2021, 59, 70–75. [Google Scholar] [CrossRef]
- Boulogeorgos, A.A.A.; Alexiou, A.; Merkle, T.; Schubert, C.; Elschner, R.; Katsiotis, A.; Stavrianos, P.; Kritharidis, D.; Chartsias, P.K.; Kokkoniemi, J.; et al. Terahertz technologies to deliver optical network quality of experience in wireless systems beyond 5G. IEEE Commun. Mag. 2018, 56, 144–151. [Google Scholar] [CrossRef]
- Rappaport, T.S.; Xing, Y.; MacCartney, G.R.; Molisch, A.F.; Mellios, E.; Zhang, J. Overview of millimeter wave communications for fifth-generation (5G) wireless networks—With a focus on propagation models. IEEE Trans. Antennas Propag. 2017, 65, 6213–6230. [Google Scholar] [CrossRef]
- Yu, J. Photonics-assisted millimeter-wave wireless communication. IEEE J. Quantum Electron. 2017, 53, 1–17. [Google Scholar] [CrossRef]
- Kleine-Ostmann, T.; Nagatsuma, T. A review on terahertz communications research. J. Infrared Millim. Terahertz Waves 2011, 32, 143–171. [Google Scholar] [CrossRef]
- Li, X.; Yu, J.; Chang, G.K. Photonics-aided millimeter-wave technologies for extreme mobile broadband communications in 5G. J. Light. Technol. 2020, 38, 366–378. [Google Scholar] [CrossRef]
- Piesiewicz, R.; Kleine-Ostmann, T.; Krumbholz, N.; Mittleman, D.; Koch, M.; Schoebel, J.; Kurner, T. Short-range ultra-broadband terahertz communications: Concepts and perspectives. IEEE Antennas Propag. Mag. 2007, 49, 24–39. [Google Scholar] [CrossRef]
- Li, X.; Yu, J.; Zhao, L.; Wang, K.; Wang, C.; Zhao, M.; Zhou, W.; Xiao, J. 1-Tb/s millimeter-wave signal wireless delivery at D-band. J. Light. Technol. 2019, 37, 196–204. [Google Scholar] [CrossRef]
- Kurner, T.; Jacob, M. Application of ray tracing to derive channel models for future multi-gigabit systems. In Proceedings of the 2009 International Conference on Electromagnetics in Advanced Applications, Torino, Italy, 14–18 September 2009; pp. 517–520. [Google Scholar]
- Peter, M.; Keusgen, W.; Felbecker, R. Measurement and ray-tracing simulation of the 60 GHz indoor broadband channel: Model accuracy and parameterization. In Proceedings of the Second European Conference on Antennas and Propagation, EuCAP 2007, Edinburgh, UK, 11–16 November 2007; pp. 1–8. [Google Scholar]
- Yang, H.; Herben, M.H.; Smulders, P.F. Indoor radio channel fading analysis via deterministic simulations at 60 GHz. In Proceedings of the 2006 3rd International Symposium on Wireless Communication Systems, Valencia, Spain, 6–8 September 2006; pp. 144–148. [Google Scholar]
- Haneda, K.; Zhang, J.; Tan, L.; Liu, G.; Zheng, Y.; Asplund, H.; Li, J.; Wang, Y.; Steer, D.; Li, C.; et al. 5G 3GPP-like channel models for outdoor urban microcellular and macrocellular environments. In Proceedings of the 2016 IEEE 83rd Vehicular Technology Conference (VTC Spring), Nanjing, China, 15–18 May 2016; pp. 1–7. [Google Scholar]
- Petrov, V.; Komarov, M.; Moltchanov, D.; Jornet, J.M.; Koucheryavy, Y. Interference and SINR in millimeter wave and terahertz communication systems with blocking and directional antennas. IEEE Trans. Wirel. Commun. 2017, 16, 1791–1808. [Google Scholar] [CrossRef]
- Piesiewicz, R.; Jemai, J.; Koch, M.; Kurner, T. THz channel characterization for future wireless gigabit indoor communication systems. In Proceedings of the Terahertz and Gigahertz Electronics and Photonics IV, Bellingham, WA, USA, 23–25 January 2005; Volume 5727, pp. 166–176. [Google Scholar]
- Piesiewicz, R.; Kleine-Ostmann, T.; Krumbholz, N.; Mittleman, D.; Koch, M.; Kürner, T. Terahertz characterisation of building materials. Electron. Lett. 2005, 41, 1002–1004. [Google Scholar] [CrossRef]
- Sato, K.; Kozima, H.; Masuzawa, H.; Manabe, T.; Ihara, T.; Kasashima, Y.; Yamaki, K. Measurements of reflection characteristics and refractive indices of interior construction materials in millimeter-wave bands. In Proceedings of the 1995 IEEE 45th Vehicular Technology Conference. Countdown to the Wireless Twenty-First Century, Chicago, IL, USA, 25–28 July 1995; Volume 1, pp. 449–453. [Google Scholar]
- Sato, K.; Manabe, T.; Ihara, T.; Saito, H.; Ito, S.; Tanaka, T.; Sugai, K.; Ohmi, N.; Murakami, Y.; Shibayama, M.; et al. Measurements of reflection and transmission characteristics of interior structures of office building in the 60-GHz band. IEEE Trans. Antennas Propag. 1997, 45, 1783–1792. [Google Scholar] [CrossRef]
- Piesiewicz, R.; Jansen, C.; Mittleman, D.; Kleine-Ostmann, T.; Koch, M.; Kurner, T. Scattering analysis for the modeling of THz communication systems. IEEE Trans. Antennas Propag. 2007, 55, 3002–3009. [Google Scholar] [CrossRef]
- Piesiewicz, R.; Jansen, C.; Koch, M.; Kürner, T. Measurements and modeling of multiple reflections effect in building materials for indoor communication systems at THz frequencies. In Proceedings of the German Microwave Conference, Hamburg, Germany, 10–12 March 2008; pp. 1–4. [Google Scholar]
- Jacob, M.; Kürner, T.; Geise, R.; Piesiewicz, R. Reflection and transmission properties of building materials in D-band for modeling future mm-wave communication systems. In Proceedings of the Fourth European Conference on Antennas and Propagation, IEEE, New York, NY, USA, 12–16 April 2010; pp. 1–5. [Google Scholar]
- Ma, J.; Shrestha, R.; Moeller, L.; Mittleman, D.M. Invited article: Channel performance for indoor and outdoor terahertz wireless links. APL Photonics 2018, 3, 051601. [Google Scholar] [CrossRef]
- WP3K, I.R. Propagation Data and Prediction Models for the planning of Indoor Radio Communication Systems and Radio Local Area Networks in the Frequency Range 900 MHz to 100 GHz; Draft Revision of Recommendation ITU-R P. 1238. 1999. Available online: https://www.semanticscholar.org/paper/PROPAGATION-DATA-AND-PREDICTION-METHODS-FOR-THE-OF/dcda66da9e0b8642314ceb38f7e6f487a896b219 (accessed on 13 August 2022).
- Degenford, J.; Coleman, P. A quasi-optics perturbation technique for measuring dielectric constants. Proc. IEEE 1966, 54, 520–522. [Google Scholar] [CrossRef]
- Suslyaev, V.I.; Kochetkova, T.D.; Dunaevskii, G.E.; Dorozhkin, K.V. Research of dielectric properties of wood at frequencies 0.1 ÷ 0.5 THz. In Proceedings of the 2014 39th International Conference on Infrared, Millimeter, and Terahertz Waves (IRMMW-THz), Tucson, AZ, USA, 14–19 September 2014; pp. 1–2. [Google Scholar]
- Alawneh, I.; Barowski, J.; Rolfes, I. Measuring the permittivity of dielectric materials by using 140 ghz fmcw radar sensor. In Proceedings of the 2019 13th European Conference on Antennas and Propagation (EuCAP), Krakow, Poland, 31 March–5 April 2019; pp. 1–4. [Google Scholar]
- Rudd, R.; Craig, K.; Ganley, M.; Hartless, R. Building Materials and Propagation; Final Report; Office of Communications: London, UK, 2014; Volume 2604. [Google Scholar]
- Afsar, M.N. Precision millimeter-wave measurements of complex refractive index, complex dielectric permittivity, and loss tangent of common polymers. IEEE Trans. Instrum. Meas. 1987, 2, 530–536. [Google Scholar] [CrossRef]
- Alawneh, I.; Barowski, J.; Rolfes, I. Dielectric material characterization in the frequency range 68–92 GHz. In Proceedings of the 12th European Conference on Antennas and Propagation (EuCAP 2018), London, UK, 9–13 April 2018; pp. 1–4. [Google Scholar]
- Linda, A.C.; Christantus, N.O.; Cosmas, A.I. Investigating indoor scattering at mmWave frequencies. Int. J. Sci. Technol. Res. 2020, 9, 554–560. [Google Scholar]
- Landron, O.; Feuerstein, M.J.; Rappaport, T.S. In situ microwave reflection coefficient measurements for smooth and rough exterior wall surfaces. In Proceedings of the IEEE 43rd Vehicular Technology Conference, Secaucus, NJ, USA, 18–20 May 1993; pp. 77–80. [Google Scholar]
- Bose, A.; Foh, C.H. A practical path loss model for indoor WiFi positioning enhancement. In Proceedings of the 2007 6th International Conference on Information, Communications & Signal Processing, Singapore, 10–13 December 2007; pp. 1–5. [Google Scholar]
- Landron, O.; Feuerstein, M.J.; Rappaport, T.S. A comparison of theoretical and empirical reflection coefficients for typical exterior wall surfaces in a mobile radio environment. IEEE Trans. Antennas Propag. 1996, 44, 341–351. [Google Scholar] [CrossRef]
- Rappaport, T.S. Wireless Communications: Principles and Practice; Prentice Hall PTR: Hoboken, NJ, USA, 1996; Volume 2. [Google Scholar]
- Tsang, L.; Kong, J.A.; Ding, K.H.; Ao, C.O. Scattering of Electromagnetic Waves: Numerical Simulations; Wiley Online Library: Hoboken, NJ, USA, 2001; Volume 705. [Google Scholar]
- Mittal, G.; Singh, D. Critical analysis of microwave specular scattering response on roughness parameter and moisture content for bare periodic rough surfaces and its retrieval. Prog. Electromagn. Res. 2010, 100, 129–152. [Google Scholar] [CrossRef]
- Beckmann, P.; Spizzichino, A. The Scattering of Electromagnetic Waves from Rough Surfaces; Artech House, Inc.: Norwood, MA, USA, 1987. [Google Scholar]
- Boithias, L. Radio Wave Propagation; North Oxford Academic: Oxford, UK, 1987. [Google Scholar]
- Khatun, M.; Guo, C.; Mehrpouyan, H. Penetration and Reflection Characteristics in Millimeter-Wave Indoor Channels. In Proceedings of the 2021 IEEE-APS Topical Conference on Antennas and Propagation in Wireless Communications (APWC), Honolulu, HI, USA, 9–13 August 2021; pp. 1–5. [Google Scholar]
- Thajudeen, C.; Hoorfar, A.; Ahmad, F.; Dogaru, T. Measured complex permittivity of walls with different hydration levels and the effect on power estimation of TWRI target returns. Prog. Electromagn. Res. B 2011, 30, 177–199. [Google Scholar] [CrossRef] [Green Version]
- Cuinas, I.; Pugliese, J.P.; Hammoudeh, A.; Sanchez, M.G. Comparison of the electromagnetic properties of building materials at 5.8 GHz and 62.4 GHz. In Proceedings of the Vehicular Technology Conference Fall 2000, IEEE VTS Fall VTC2000, 52nd Vehicular Technology Conference (Cat. No. 00CH37152), Boston, MA, USA, 24–28 September 2000; Volume 2, pp. 780–785. [Google Scholar]
- Stevels, J. The electrical properties of glass. In Electrical Conductivity II/Elektrische Leitungsphänomene II; Springer: Berlin/Heidelberg, Germany, 1957; pp. 350–391. [Google Scholar]
- Stavrou, S.; Saunders, S. Review of constitutive parameters of building materials. In Proceedings of the Twelfth International Conference on Antennas and Propagation, 2003 (ICAP 2003) (Conf. Publ. No. 491), Exeter, UK, 31 March–3 April 2003; Volume 1, pp. 211–215. [Google Scholar]
- Ju, S.; Shah, S.H.A.; Javed, M.A.; Li, J.; Palteru, G.; Robin, J.; Xing, Y.; Kanhere, O.; Rappaport, T.S. Scattering mechanisms and modeling for terahertz wireless communications. In Proceedings of the ICC 2019-2019 IEEE International Conference on Communications (ICC), Shanghai, China, 20–24 May 2019; pp. 1–7. [Google Scholar]
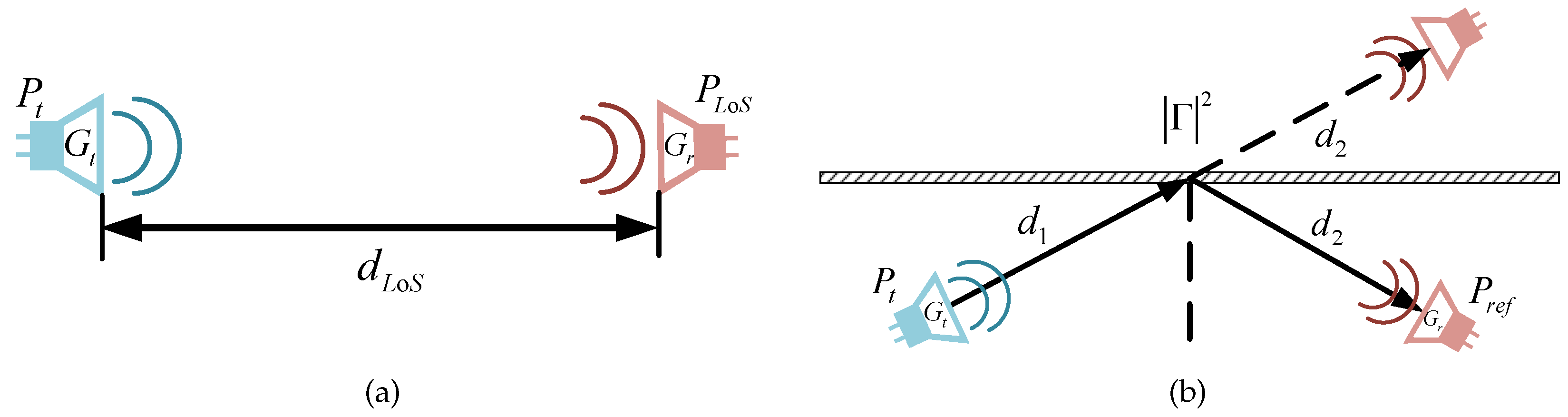







| Year | Frequency | Contributions |
|---|---|---|
| 1995 | 57.5, 78.5 and 95.9 GHz |
|
| 1997 | 60 GHz band |
|
| 2007 | 100–1000 GHz band |
|
| 2008 | 100–500 GHz |
|
| 2010 | 110–135 GHz |
|
| 2018 | 100, 200, 300 and 400 GHz |
|
| Year | Materials | Dielectric Properties | Equipment | Frequency | Results |
|---|---|---|---|---|---|
| 1966 | Plexiglass | Permittivity | Fabry-Perot resonator | 143 GHz | 2.60 [25] |
| 2005 | Plaster, glass and wood | Absorption coefficient and refractive index | THz time-domain spectroscopy | 70–350 GHz | Figures to show the measured parameters VS frequency [17]. |
| 2007 | Ingrain wallpaper and two plaster samples | Surface roughness | Commercially available equipment for optical 3D micro- and nanometrology | 100–1000 GHz | of the surface height is 0.13 mm, 0.05 mm and 0.15 mm, respectively [20]. |
| 2014 | Four wood species | Complex permittivity | Quasioptical Mach-Zahnder Interferometer with backward-wave oscillator | 100–500 GHz | There is slight deviation among species, but the overall range is 1.60–1.89 [26]. |
| 2019 | Gypsum | Permittivity | Frequency-modulated continuous-wave radar sensors | 122–169 GHz | 2.595 [27] |
| Material | Frequency | Permittivity |
|---|---|---|
| Wood | 1–100 GHz | 1.99 [28] |
| 110–170 GHz | 1.60–1.89 [26] | |
| Plexiglass | 143 GHz | 2.60 [25] |
| 60–300 GHz | 2.581–2.602 [29] | |
| Drywall (plaster) | 68–92 GHz | 2.80 [30] |
| 122–167 GHz | 2.595–2.602 [27] | |
| Concrete board | 1–95.9 GHz | 6.2–7.0 [24] |
| Red brick | 1–100 GHz | 3.75 [31] |
| Specifications | Values | ||||||
|---|---|---|---|---|---|---|---|
| Center frequency (GHz) | 110 | 120 | 130 | 140 | 150 | 160 | 170 |
| LO frequency (GHz) | 9.06 | 9.90 | 10.73 | 11.56 | 12.40 | 13.23 | 14.06 |
| IF frequency (GHz) | 1.2 | ||||||
| Tx/Rx antenna gain (dBi) | 25 | ||||||
| Tx/Rx azimuth HPBW | E plane: 9°/H plane: 10° | ||||||
| Tx/Rx polarization | Horizontal | ||||||
| Tx/Rx caliber (mm × mm) | 17.5 × 13.6 | ||||||
| Tx/Rx projection diameter (mm) | 19.1 | ||||||
| LNA gain (dB) | 35 | ||||||
| Material | Size (cm × cm) | Radius of First Fresnel Zone in D-Band (cm) |
|---|---|---|
| Wood | 49.2 × 35.8 | 2.10–2.61 |
| Plexiglass | 88.2 × 42.9 | |
| Drywall (plaster) | 56.1 × 37.5 | |
| Concrete board | 59.7 × 39.3 | |
| Red brick | 45.6 × 31.2 |
| Material | Reflection Loss (dB) | |||||
|---|---|---|---|---|---|---|
| 20° | 30° | 40° | 50° | 60° | 70° | |
| Wood | 17.3 | 19.1 | 20.8 | 26.3 | 27.7 | 14.0 |
| Plexiglass | 12.9 | 14.0 | 15.7 | 19.1 | 25.8 | 14.8 |
| Drywall | 13.2 | 14.4 | 16.1 | 18.7 | 26.6 | 15.8 |
| Concrete | 6.7 | 8.1 | 8.9 | 10.8 | 16.2 | 19.3 |
| Red brick | 10.0 | 10.8 | 13.2 | 18.4 | 21.4 | 15.9 |
| Material | (µm) | MMSE | ||
|---|---|---|---|---|
| Wood | 1.60–1.89 | 1.75–1.92 | 76.4–80.0 | 3.79 × |
| Plexiglass | 2.581–2.602 | 2.56–2.69 | 8.2–14.5 | 3.04 × |
| Drywall | 2.595–2.602 | 2.51–2.69 | 96.4–99.2 | 5.47 × |
| Concrete | 6.2–7.0 | 5.94–6.26 | 264.3–269.0 | 7.25 × |
| Red brick | 3.75 | 3.49–3.76 | 321.3–325.0 | 6.43 × |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; Wang, Y.; Li, W.; Ding, J.; Bian, C.; Wang, X.; Wang, C.; Li, C.; Zhong, Z.; Yu, J. Reflection Characteristics Measurements of Indoor Wireless Link in D-Band. Sensors 2022, 22, 6908. https://doi.org/10.3390/s22186908
Wang M, Wang Y, Li W, Ding J, Bian C, Wang X, Wang C, Li C, Zhong Z, Yu J. Reflection Characteristics Measurements of Indoor Wireless Link in D-Band. Sensors. 2022; 22(18):6908. https://doi.org/10.3390/s22186908
Chicago/Turabian StyleWang, Mingxu, Yanyi Wang, Weiping Li, Junjie Ding, Chengzhen Bian, Xinyi Wang, Chao Wang, Chao Li, Zhimeng Zhong, and Jianjun Yu. 2022. "Reflection Characteristics Measurements of Indoor Wireless Link in D-Band" Sensors 22, no. 18: 6908. https://doi.org/10.3390/s22186908
APA StyleWang, M., Wang, Y., Li, W., Ding, J., Bian, C., Wang, X., Wang, C., Li, C., Zhong, Z., & Yu, J. (2022). Reflection Characteristics Measurements of Indoor Wireless Link in D-Band. Sensors, 22(18), 6908. https://doi.org/10.3390/s22186908







