Quantization-Mitigation-Based Trajectory Control for Euler-Lagrange Systems with Unknown Actuator Dynamics
Abstract
1. Introduction
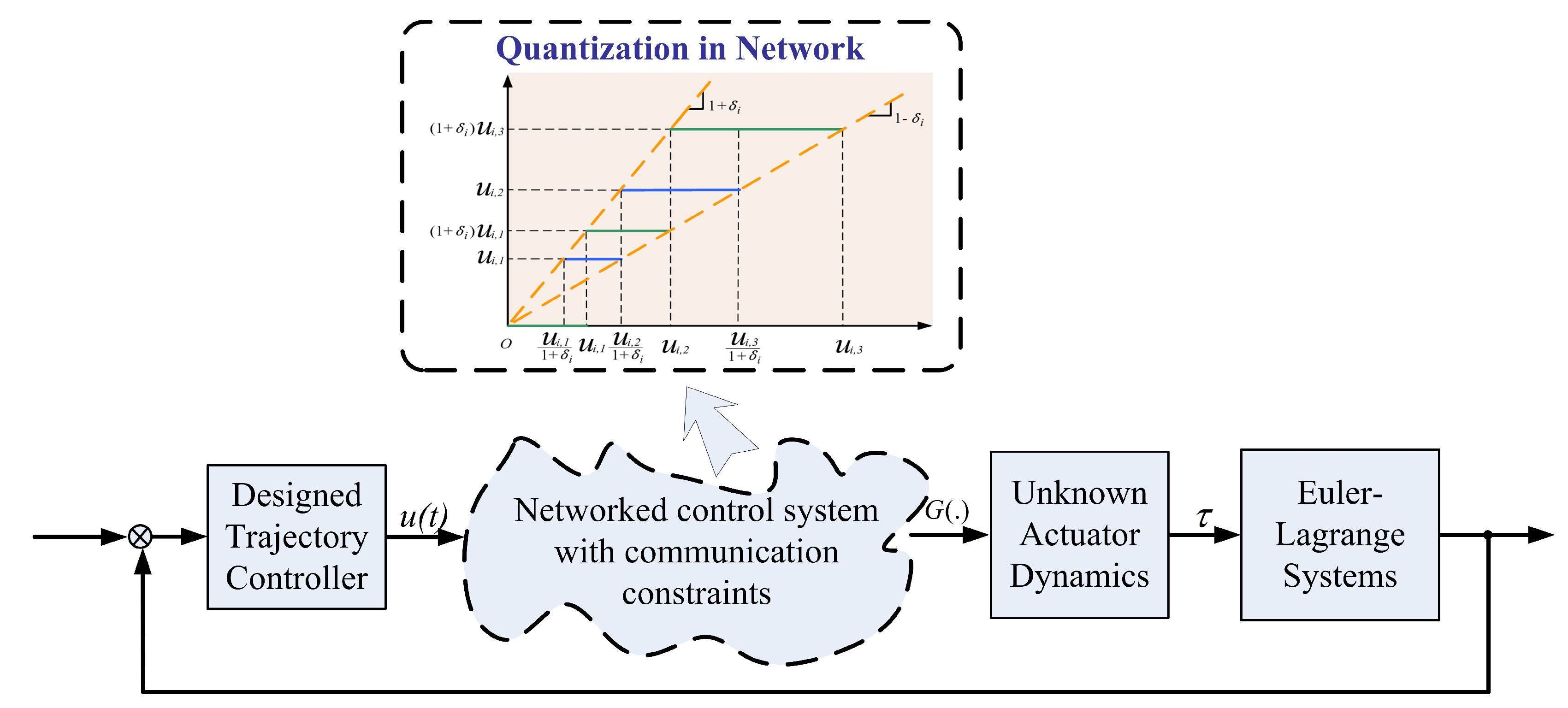
2. Problem Formulation
3. Control Design for Networked Euler-Lagrange Systems with Unknown Actuator Dynamics
3.1. Structural Properties of Euler-Lagrange Systems
3.2. Adaptive Method for Adjusting the Control Gain
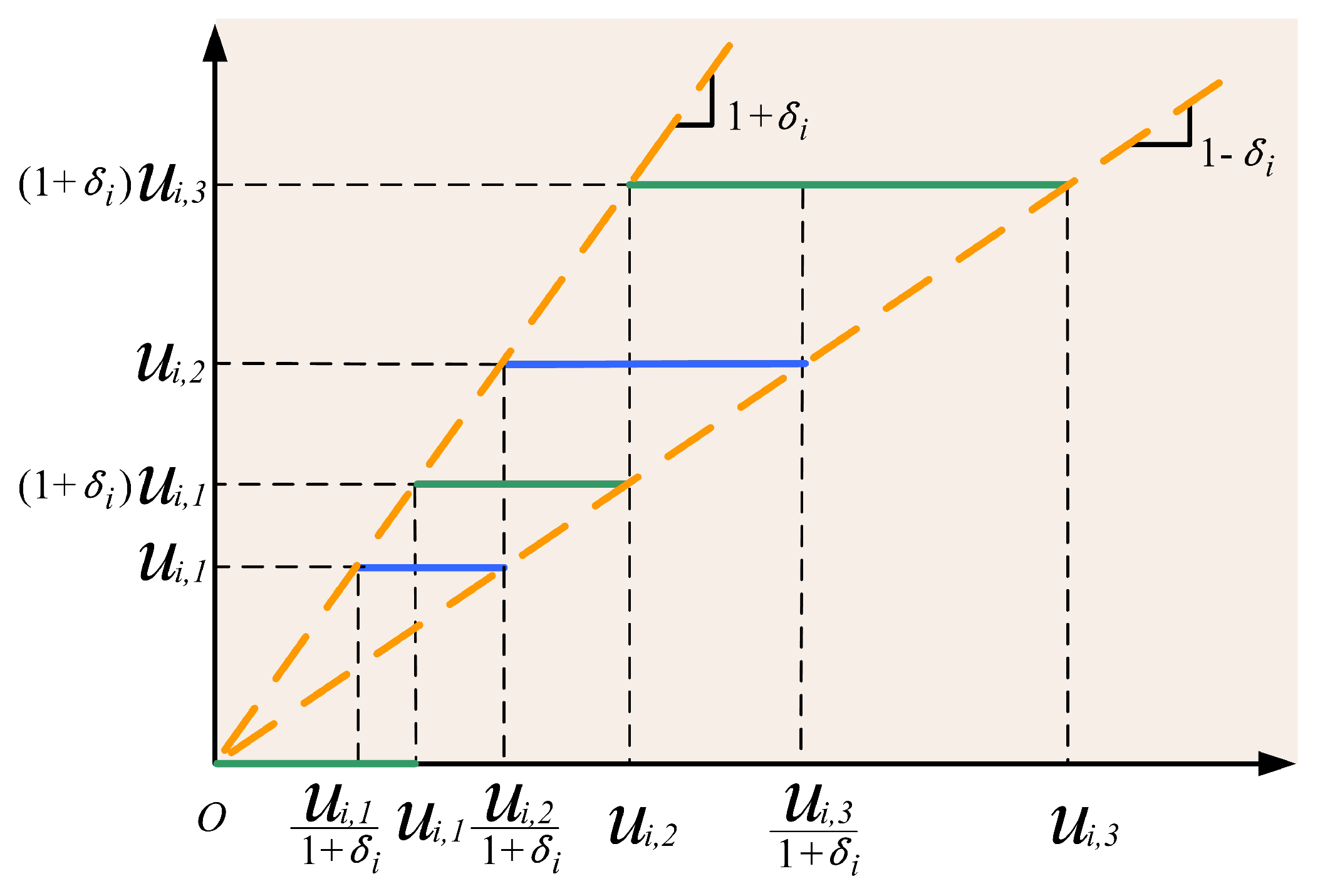
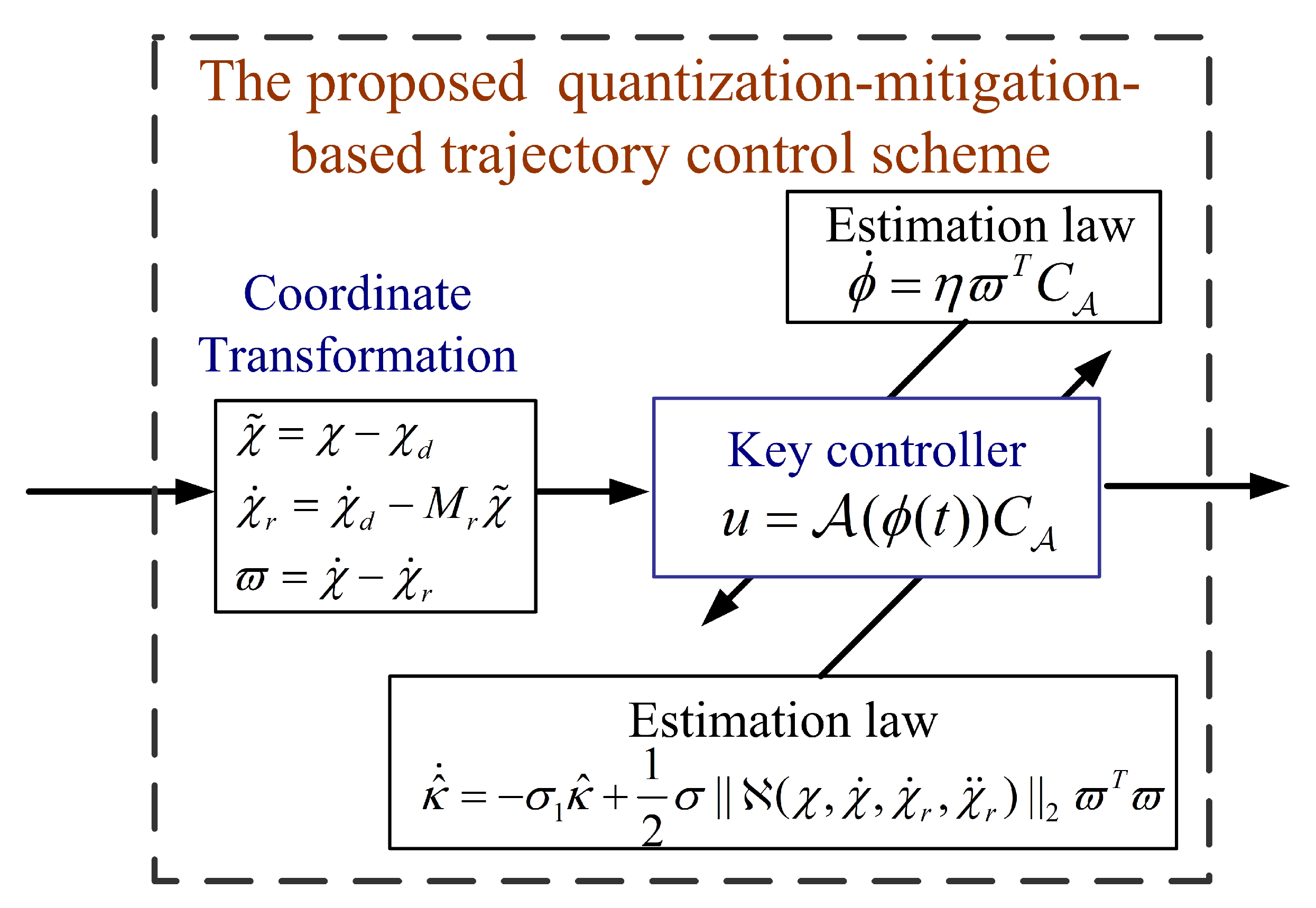
3.3. Quantization-Mitigation-Based Trajectory Control Design
3.4. Stability Proof via Lyapunov’s Direct Method
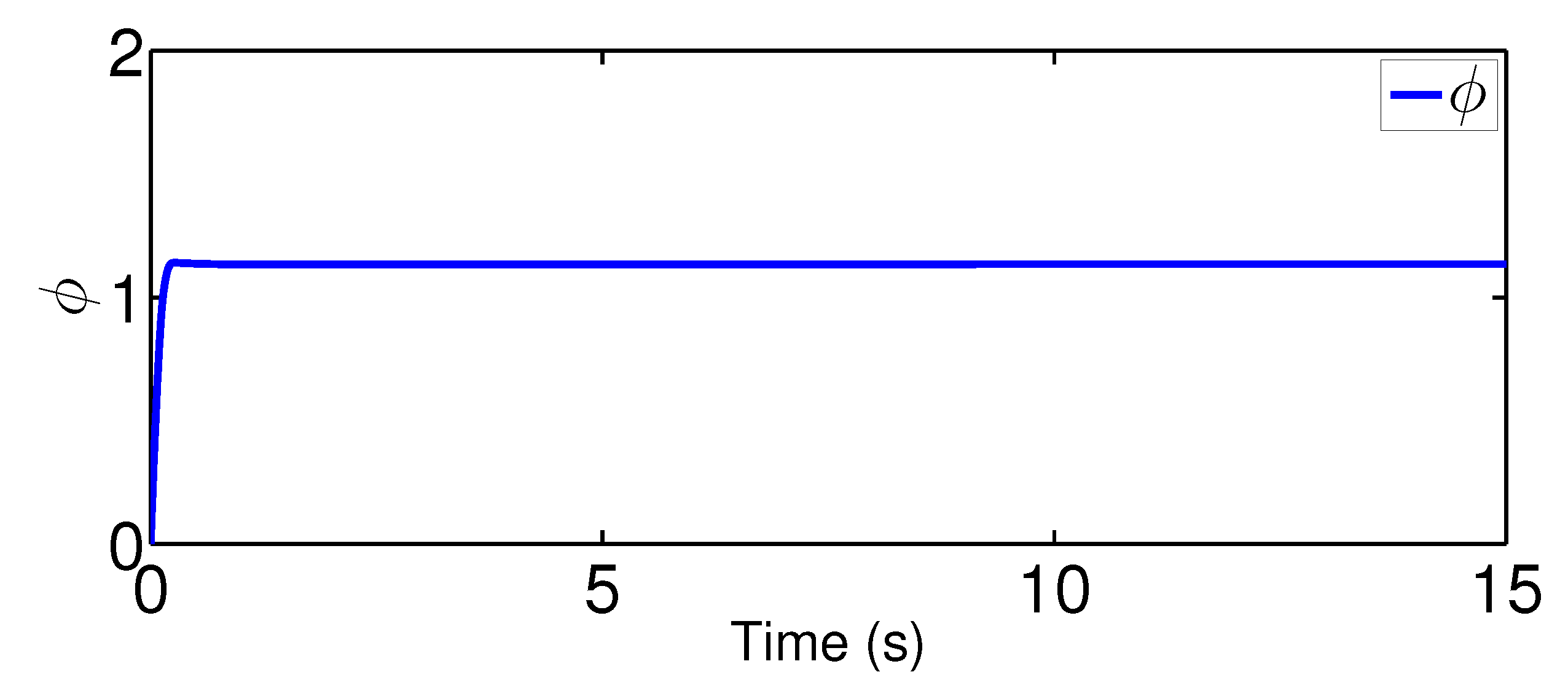
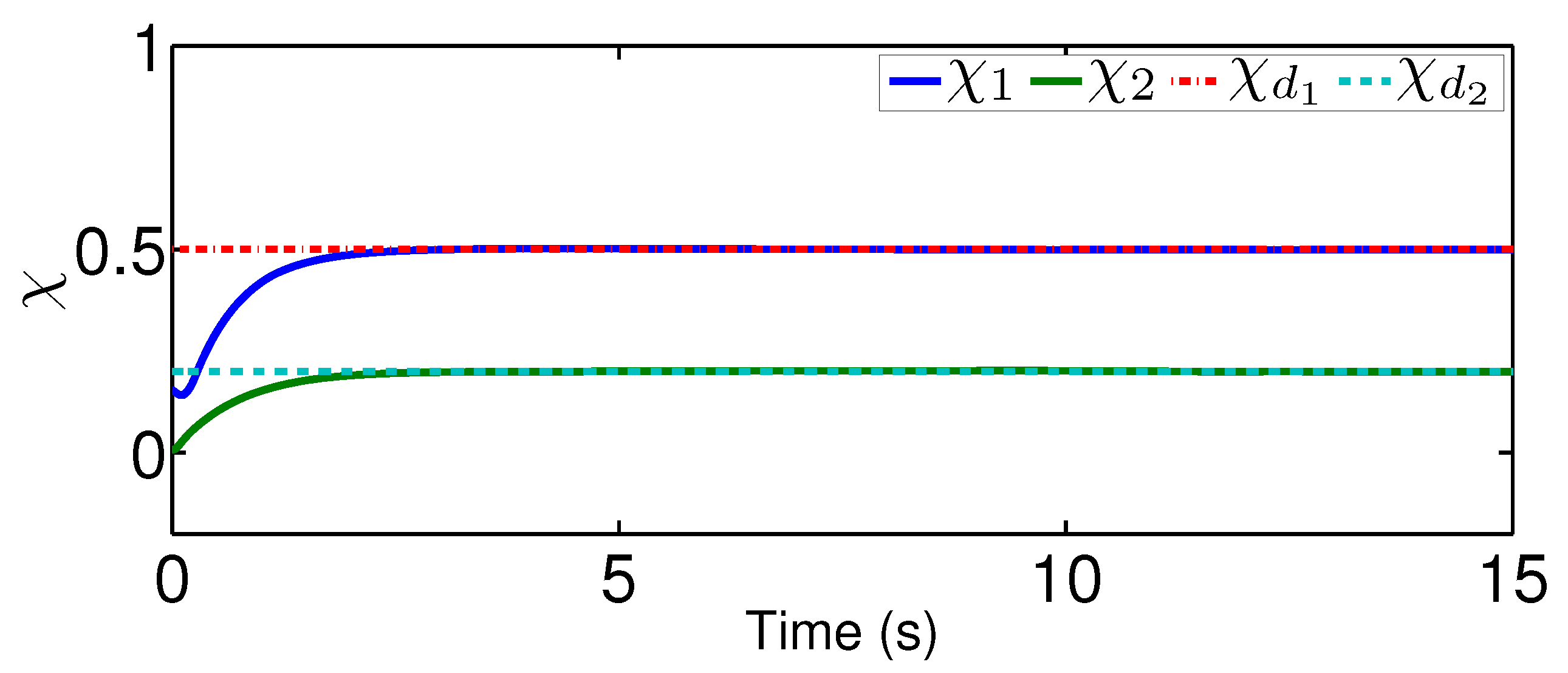
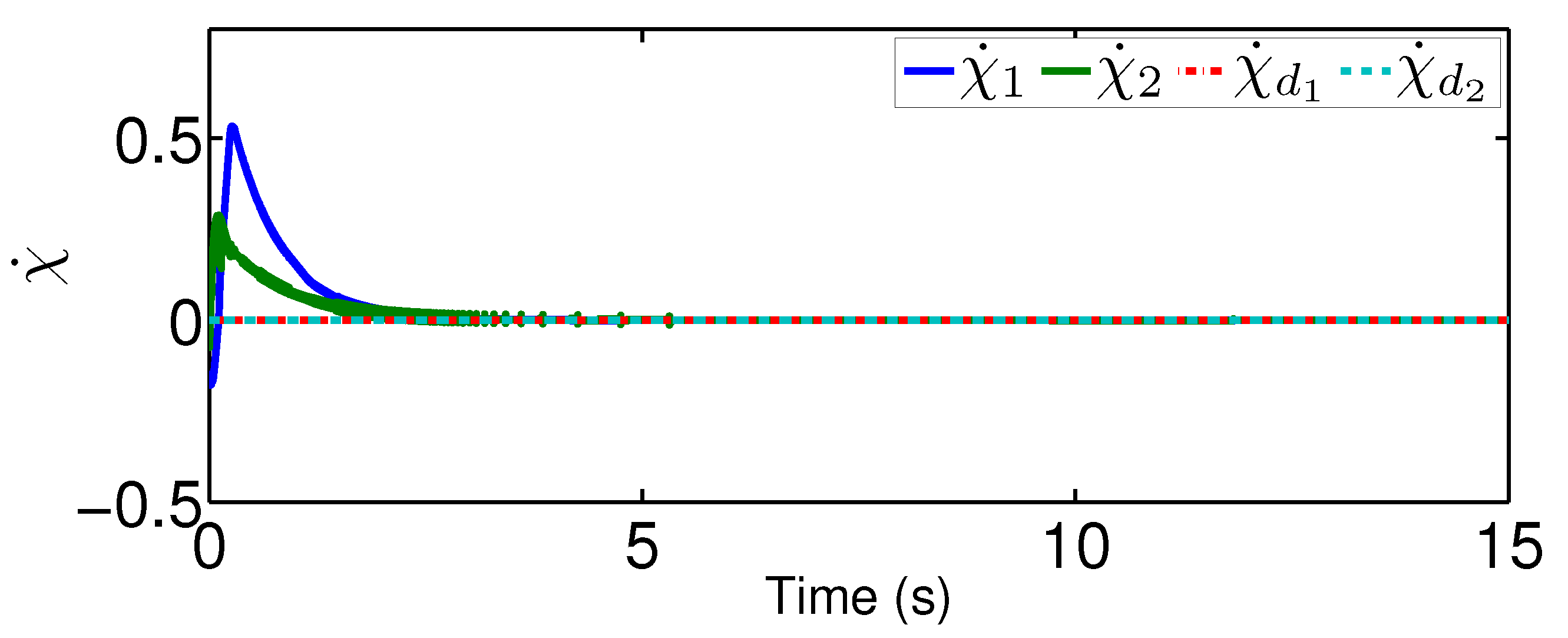
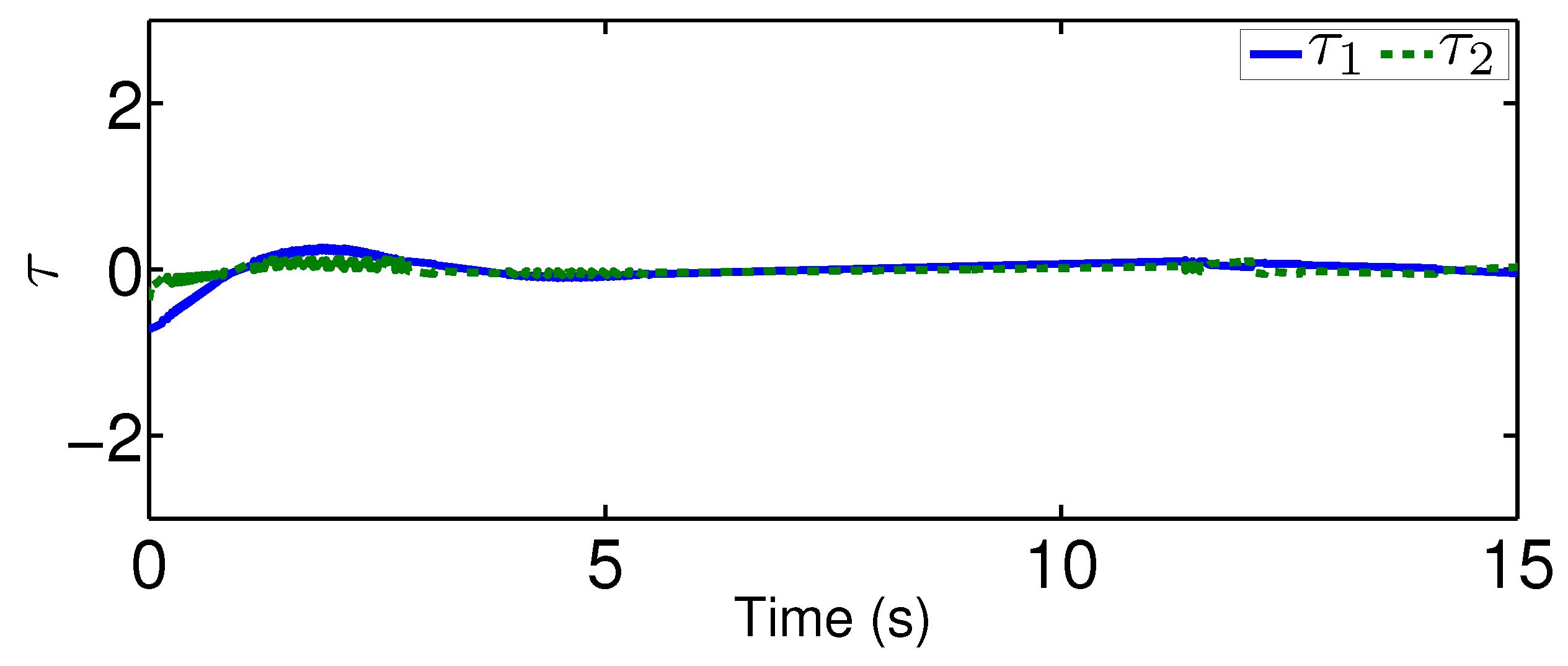
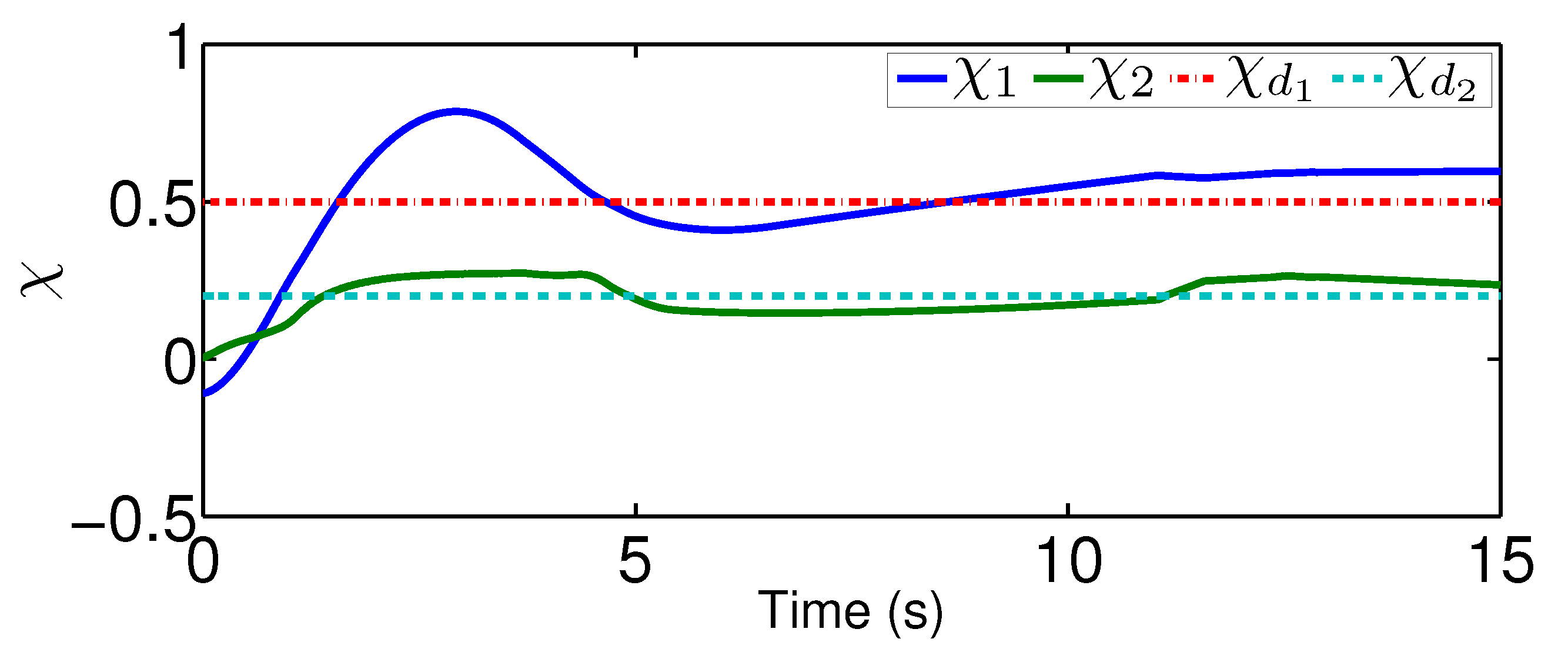
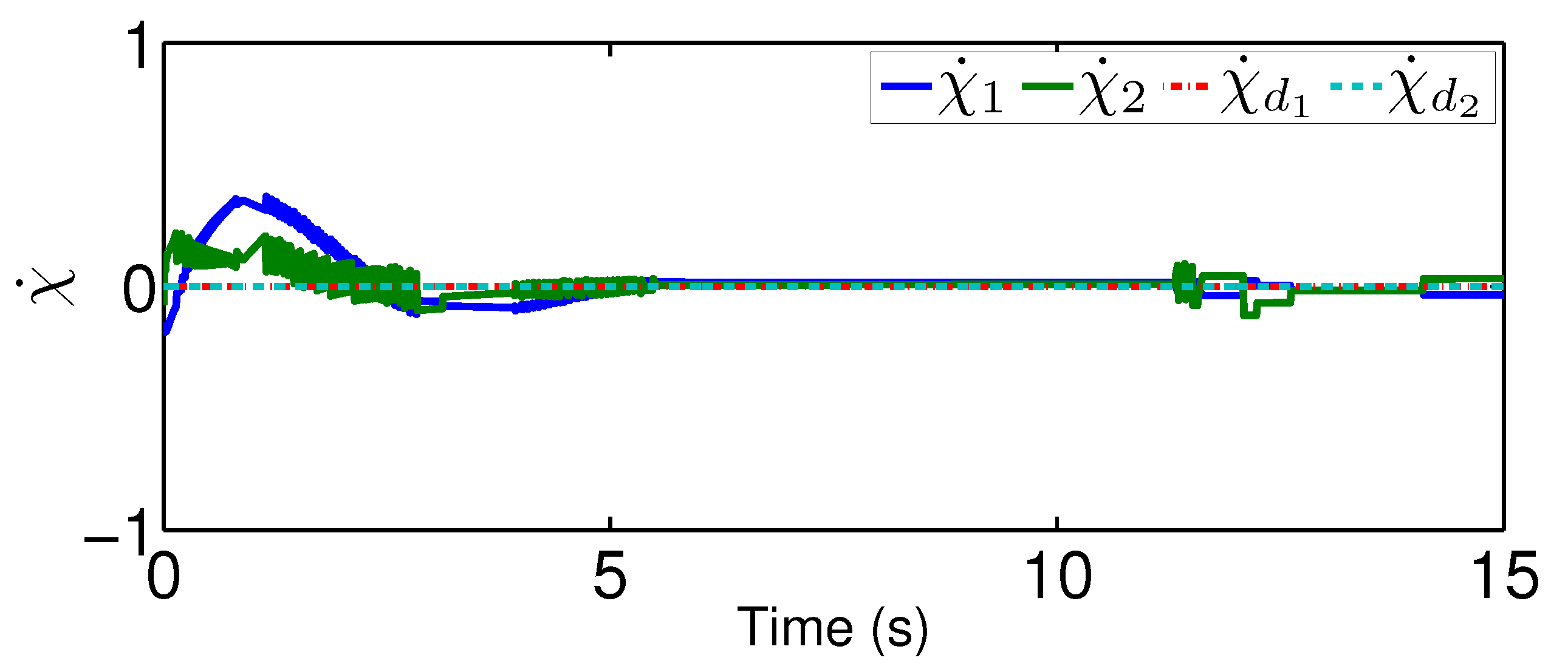
4. Simulation and Experiment
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- You, K.-Y.; Xie, L.-H. Survey of recent progress in networked control systems. Acta Autom. Sin. 2013, 39, 101–117. [Google Scholar] [CrossRef]
- Ishii, H.; Francis, B.A. Limited Data Rate in Control Systems With Networks; Springer Science & Business Media: Berlin, Germany, 2002; Volume 275. [Google Scholar]
- Tatikonda, S.; Mitter, S. Control under communication constraints. IEEE Trans. Autom. Control 2004, 49, 1056–1068. [Google Scholar] [CrossRef]
- Yan, J.; Zhang, X.; Luo, X.; Wang, Y.; Chen, C.; Guan, X. Asynchronous localization with mobility prediction for underwater acoustic sensor networks. IEEE Trans. Veh. Technol. 2017, 67, 2543–2556. [Google Scholar] [CrossRef]
- Elia, N.; Mitter, S.K. Stabilization of linear systems with limited information. IEEE Trans. Autom. Control 2001, 46, 1384–1400. [Google Scholar] [CrossRef]
- Liu, J.; Elia, N. Quantized feedback stabilization of non-linear affine systems. Int. J. Control 2004, 77, 239–249. [Google Scholar] [CrossRef]
- de Persis, C.; Isidori, A. Stabilizability by state feedback implies stabilizability by encoded state feedback. Syst. Control Lett. 2004, 53, 249–258. [Google Scholar] [CrossRef]
- Fu, M.; Xie, L. Finite-level quantized feedback control for linear systems. IEEE Trans. Autom. Control 2009, 54, 1165–1170. [Google Scholar]
- Hayakawa, T.; Ishii, H.; Tsumura, K. Adaptive quantized control for nonlinear uncertain systems. Syst. Control Lett. 2009, 58, 625–632. [Google Scholar] [CrossRef]
- Zhou, J.; Wen, C.; Yang, G. Adaptive backstepping stabilization of nonlinear uncertain systems with quantized input signal. IEEE Trans. Autom. Control 2014, 59, 460–464. [Google Scholar] [CrossRef]
- Xing, L.; Wen, C.; Zhu, Y.; Su, H.; Liu, Z. Output feedback control for uncertain nonlinear systems with input quantization. Automatica 2016, 65, 191–202. [Google Scholar] [CrossRef]
- Xie, K.; Chen, C.; Lewis, F.L.; Xie, S. Adaptive asymptotic neural network control of nonlinear systems with unknown actuator quantization. IEEE Trans. Neural Netw. Learn. Syst. 2018, 29, 6303–6312. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Wen, C.; Wang, W. Adaptive control of uncertain nonlinear systems with quantized input signal. Automatica 2018, 95, 152–162. [Google Scholar] [CrossRef]
- Yang, C.; Jiang, Y.; He, W.; Na, J.; Li, Z.; Xu, B. Adaptive parameter estimation and control design for robot manipulators with finite-time convergence. IEEE Trans. Ind. Electron. 2018, 65, 8112–8123. [Google Scholar] [CrossRef]
- Chen, C.; Wen, C.; Liu, Z.; Xie, K.; Zhang, Y.; Chen, C.L.P. Adaptive consensus of nonlinear multi-agent systems with non-identical partially unknown control directions and bounded modelling errors. IEEE Trans. Autom. Control 2017, 62, 4654–4659. [Google Scholar] [CrossRef]
- Jiang, Y.; Fan, J.; Chai, T.; Li, J.; Lewis, F.L. Data-driven flotation industrial process operational optimal control based on reinforcement learning. IEEE Trans. Ind. Inform. 2017, 14, 1974–1989. [Google Scholar] [CrossRef]
- Yang, C.; Jiang, Y.; Li, Z.; He, W.; Su, C. Neural control of bimanual robots with guaranteed global stability and motion precision. IEEE Trans. Ind. Inform. 2017, 13, 1162–1171. [Google Scholar] [CrossRef]
- Yan, J.; Li, X.; Luo, X.; Guan, X. Virtual-lattice based intrusion detection algorithm over actuator-assisted underwater wireless sensor networks. Sensors 2017, 17, 1168. [Google Scholar] [CrossRef]
- Li, X.; Wen, C.; Chen, C. Adaptive formation control of networked robotic systems with bearing-only measurements. IEEE Trans. Cybern. 2020. [Google Scholar] [CrossRef]
- Chen, C.; Lewis, F.L.; Xie, S.; Modares, H.; Liu, Z.; Zuo, S.; Davoudi, A. Resilient adaptive and H∞ controls of multi-agent systems under sensor and actuator faults. Automatica 2019, 102, 19–26. [Google Scholar] [CrossRef]
- Wang, H.; Xie, S.; Zhou, B.; Wang, W. Non-fragile robust h∞ filtering of takagi-sugeno fuzzy networked control systems with sensor failures. Sensors 2020, 20, 27. [Google Scholar] [CrossRef]
- Sánchez, J.R.G.; Tavera-Mosqueda, S.; Silva-Ortigoza, R.; Guzmán, V.M.H.; Sandoval-Gutierrez, J.; Marcelino-Aranda, M.; Taud, H.; Marciano-Melchor, M. Robust switched tracking control for wheeled mobile robots considering the actuators and drivers. Sensors 2018, 18, 4316. [Google Scholar] [CrossRef] [PubMed]
- Kim, G.; Hirata, K. Motion control of a two-degree-of-freedom linear resonant actuator without a mechanical spring. Sensors 2020, 20, 1954. [Google Scholar] [CrossRef] [PubMed]
- Tao, G.; Kokotovic, P.V. Adaptive Control of Systems With Actuator And Sensor Nonlinearities; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1996. [Google Scholar]
- Tao, G.; Chen, S.; Tang, X.; Joshi, S.M. Adaptive Control of Systems with Actuator Failures; Springer Science & Business Media: Berlin, Germany, 2013. [Google Scholar]
- Chen, C.; Wen, C.; Liu, Z.; Xie, K.; Zhang, Y.; Chen, C.P. Adaptive asymptotic control of multivariable systems based on a one-parameter estimation approach. Automatica 2017, 83, 124–132. [Google Scholar] [CrossRef]
- Ruderman, M.; Iwasaki, M. Sensorless torsion control of elastic-joint robots with hysteresis and friction. IEEE Trans. Ind. Electron. 2016, 63, 1889–1899. [Google Scholar] [CrossRef]
- Chen, C.; Xie, K.; Lewis, F.L.; Xie, S.; Davoudi, A. Fully distributed resilience for adaptive exponential synchronization of heterogeneous multi-agent systems against actuator faults. IEEE Trans. Autom. Control 2019, 64, 3347–3354. [Google Scholar] [CrossRef]
- Lin, C.; Wang, S.; Lin, C. Interval type-2 neural fuzzy controller-based navigation of cooperative load-carrying mobile robots in unknown environments. Sensors 2018, 18, 4181. [Google Scholar] [CrossRef] [PubMed]
- Xie, K.; Lai, Y.; Li, W. Computational efficiency-based adaptive tracking control for robotic manipulators with unknown input bouc-wen hysteresis. Sensors 2019, 19, 2776. [Google Scholar] [CrossRef]
- Nozaki, T.; Shimizu, S.; Murakami, T.; Oboe, R. Impedance field expression of bilateral control for reducing data traffic in haptic transmission. IEEE Trans. Ind. Electron. 2019, 66, 1142–1150. [Google Scholar] [CrossRef]
- Yan, J.; Gong, Y.; Chen, C.; Luo, X.; Guan, X. Auv-aided localization for internet of underwater things: A reinforcement learning-based method. IEEE Internet Things J. 2020. [Google Scholar] [CrossRef]
- Chen, C.; Xie, K.; Lewis, F.; Xie, S.; Fierro, R. Adaptive synchronization of multi-agent systems with resilience to communication link faults. Automatica 2020, 111, 108636. [Google Scholar] [CrossRef]
- Lewis, F.L.; Dawson, D.M.; Abdallah, C.T. Robot Manipulator Control: Theory and Practice; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Lai, G.; Liu, Z.; Zhang, Y.; Chen, C.P. Adaptive fuzzy quantized control of time-delayed nonlinear systems with communication constraint. Fuzzy Sets Syst. 2017, 314, 61–78. [Google Scholar] [CrossRef]
- Chen, C.; Modares, H.; Xie, K.; Lewis, F.L.; Wan, Y.; Xie, S. Reinforcement learning-based adaptive optimal exponential tracking control of linear systems with unknown dynamics. IEEE Trans. Autom. Control 2019, 64, 4423–4438. [Google Scholar] [CrossRef]
- Lu, X.; Kiumarsi, B.; Chai, T.; Jiang, Y.; Lewis, F.L. Operational control of mineral grinding processes using adaptive dynamic programming and reference governor. IEEE Trans. Ind. Inform. 2019, 15, 2210–2221. [Google Scholar] [CrossRef]



| The Proposed Method | The Existing Method | |
|---|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyu, Y.; Yang, Q.; Kolaric, P. Quantization-Mitigation-Based Trajectory Control for Euler-Lagrange Systems with Unknown Actuator Dynamics. Sensors 2020, 20, 3974. https://doi.org/10.3390/s20143974
Lyu Y, Yang Q, Kolaric P. Quantization-Mitigation-Based Trajectory Control for Euler-Lagrange Systems with Unknown Actuator Dynamics. Sensors. 2020; 20(14):3974. https://doi.org/10.3390/s20143974
Chicago/Turabian StyleLyu, Yi, Qiyu Yang, and Patrik Kolaric. 2020. "Quantization-Mitigation-Based Trajectory Control for Euler-Lagrange Systems with Unknown Actuator Dynamics" Sensors 20, no. 14: 3974. https://doi.org/10.3390/s20143974
APA StyleLyu, Y., Yang, Q., & Kolaric, P. (2020). Quantization-Mitigation-Based Trajectory Control for Euler-Lagrange Systems with Unknown Actuator Dynamics. Sensors, 20(14), 3974. https://doi.org/10.3390/s20143974




