Spatial Baseline Optimization for Spaceborne Multistatic SAR Tomography Systems
Abstract
1. Introduction
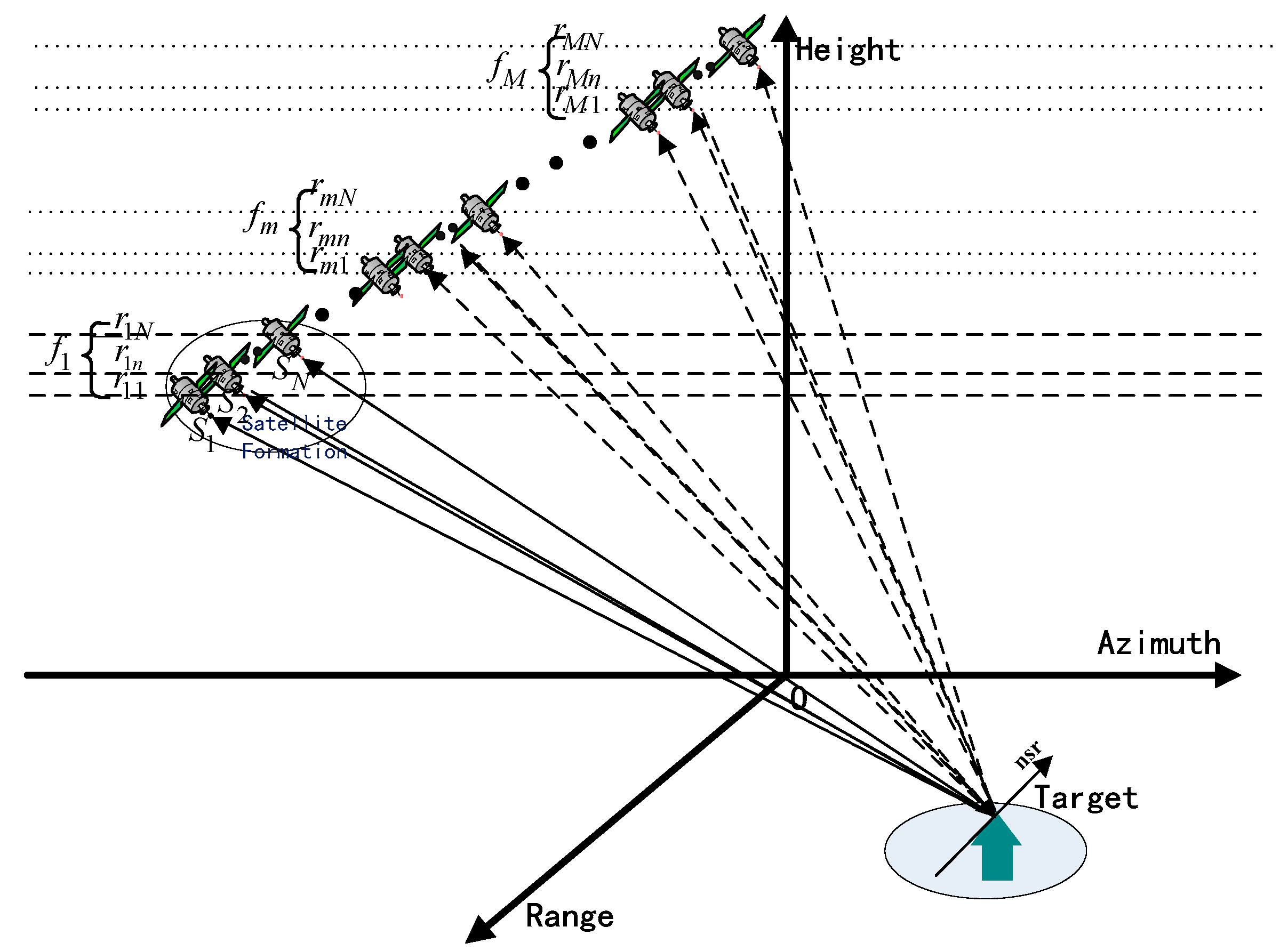
2. Baseline Equivalence Analysis of SMS-TomoSAR
3. Three-dimensional Imaging Analysis of an SMS-TomoSAR System
4. Spatial Baseline Optimization Method for SMS-TomoSAR systems
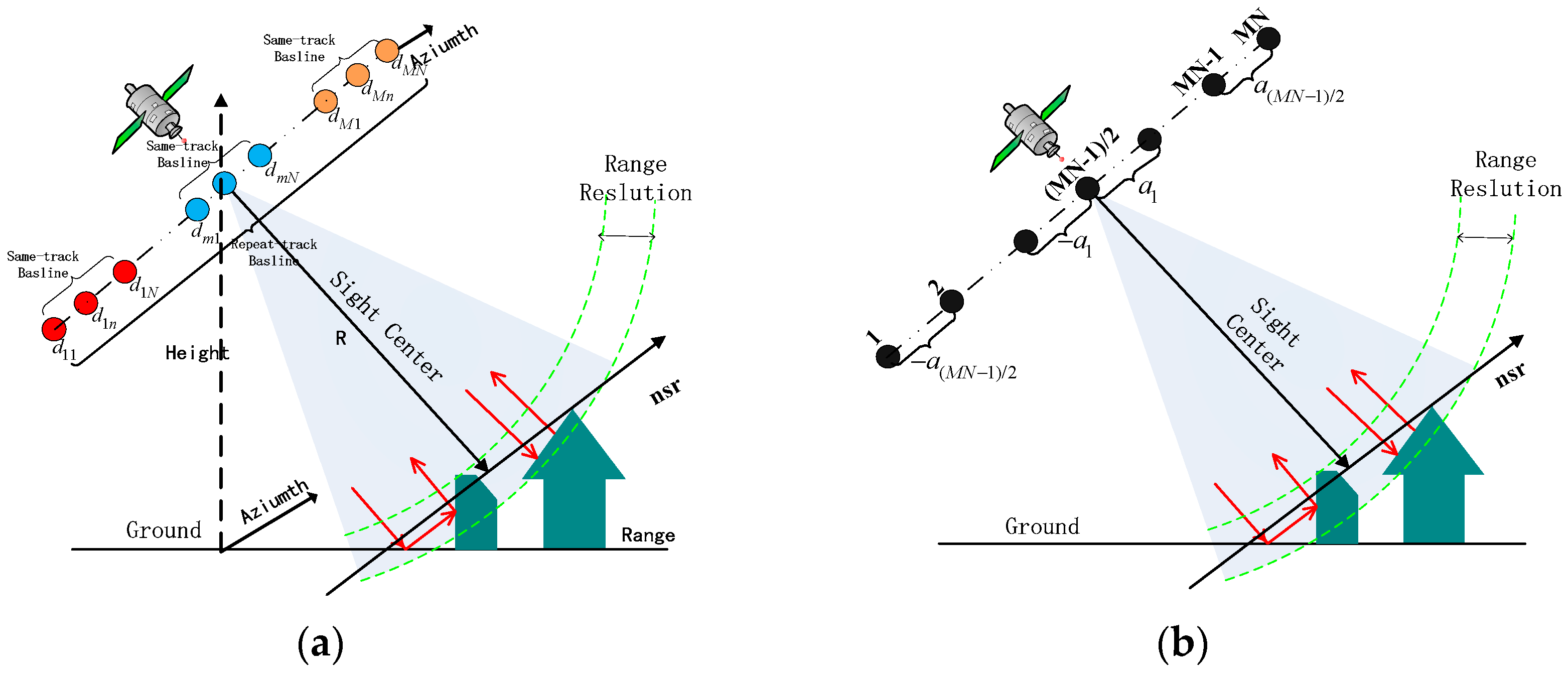
4.1. Baseline Model Construction
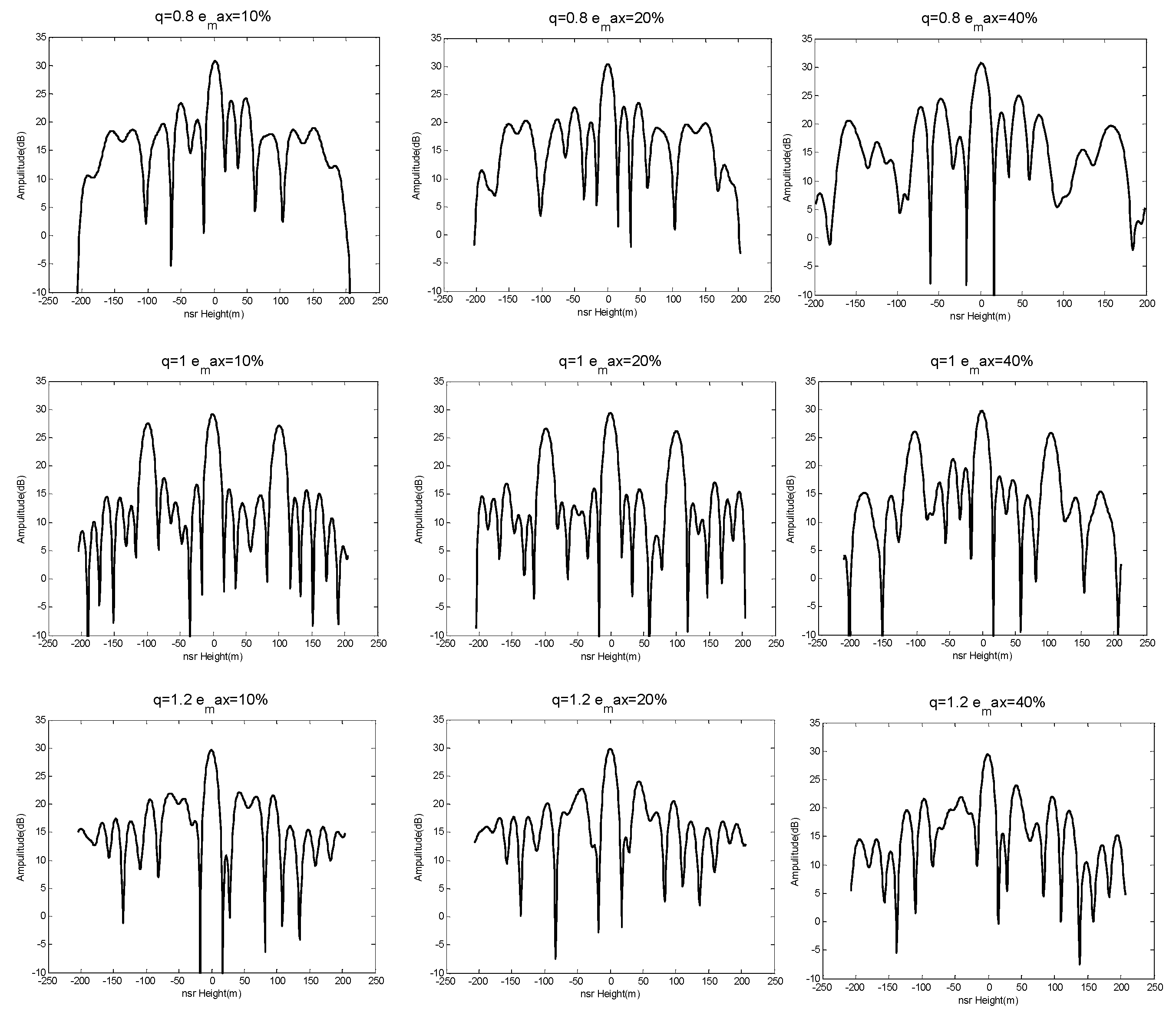
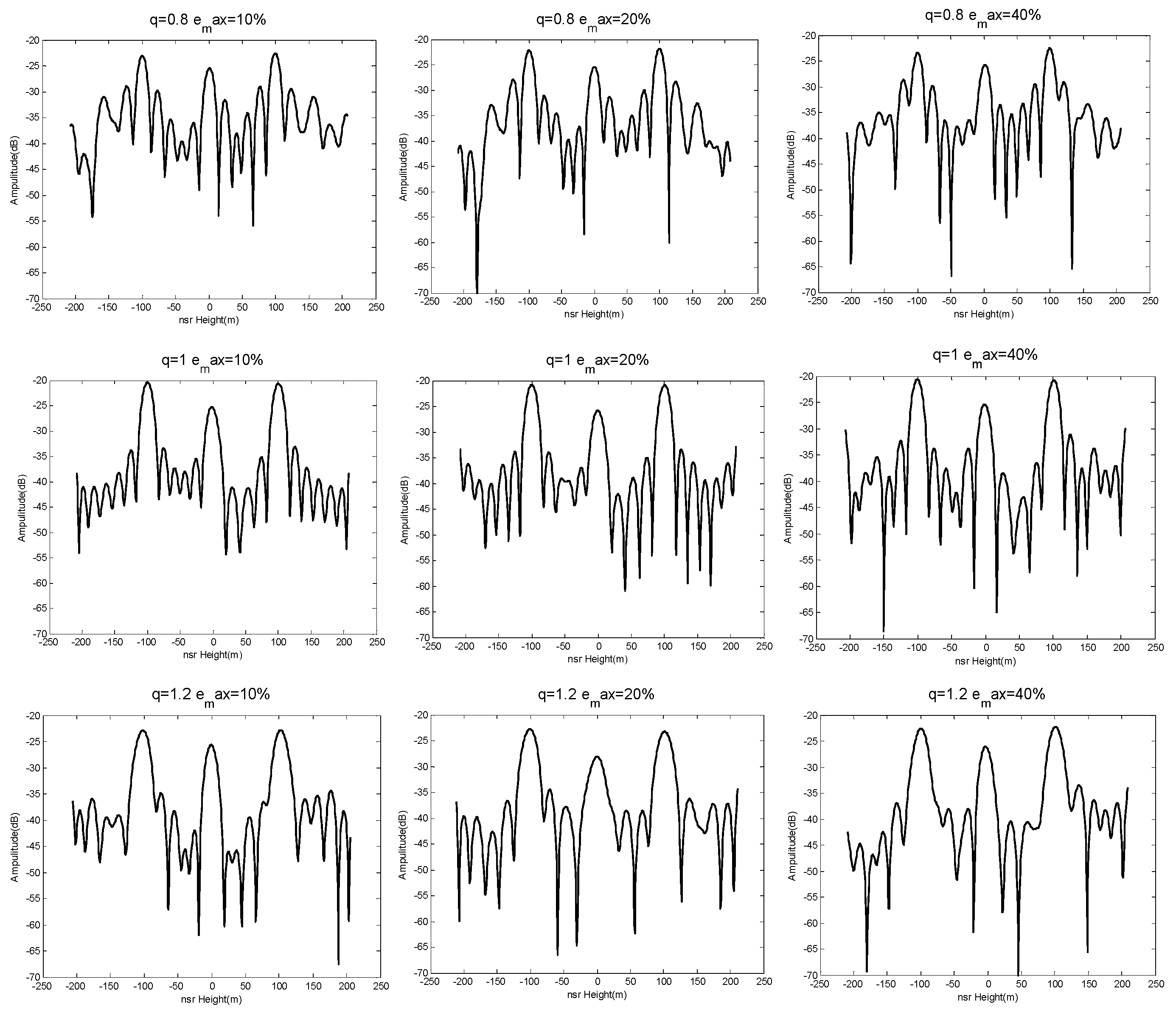
4.2. Maximum Perturbation Estimation Method
5. Experimental Verification
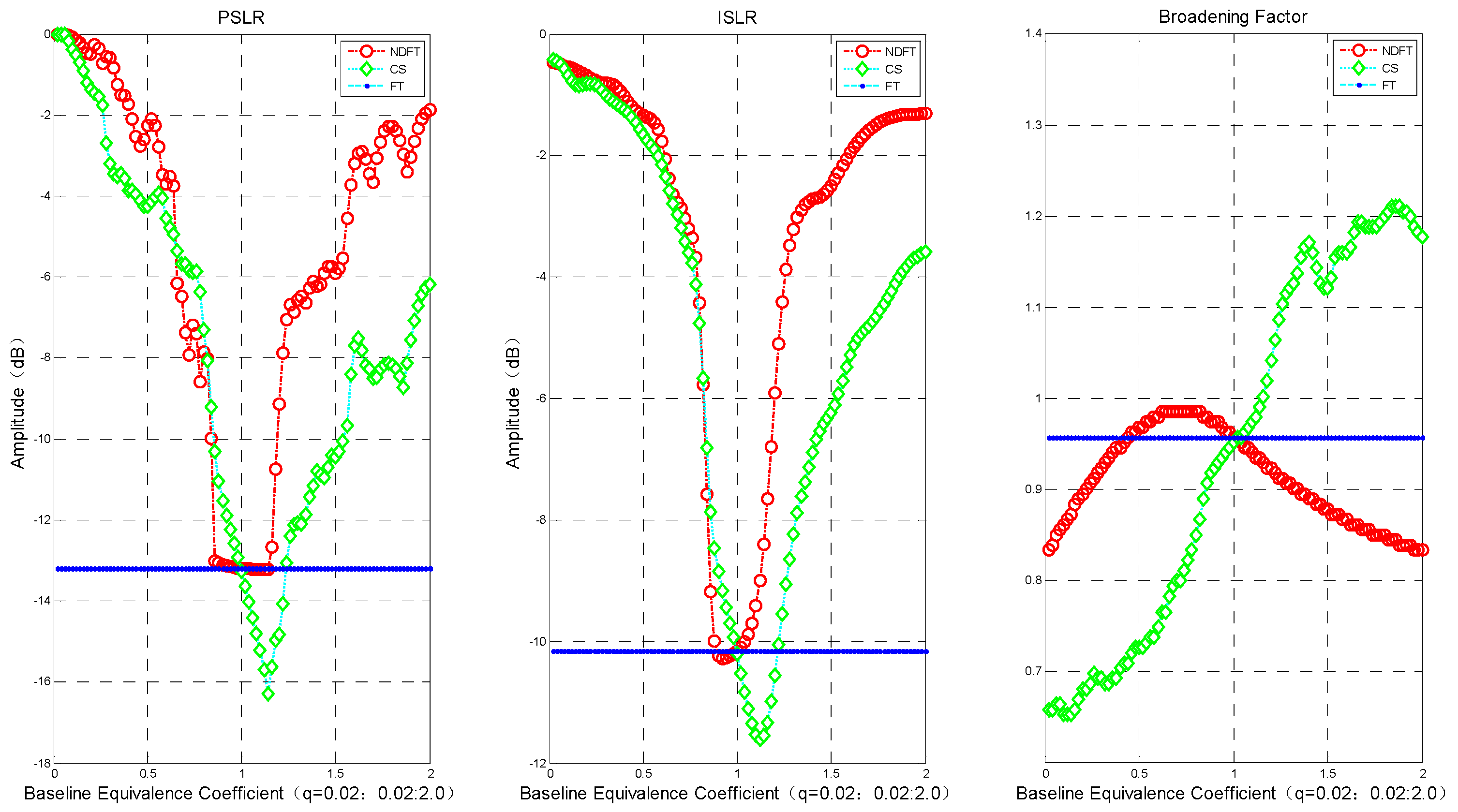
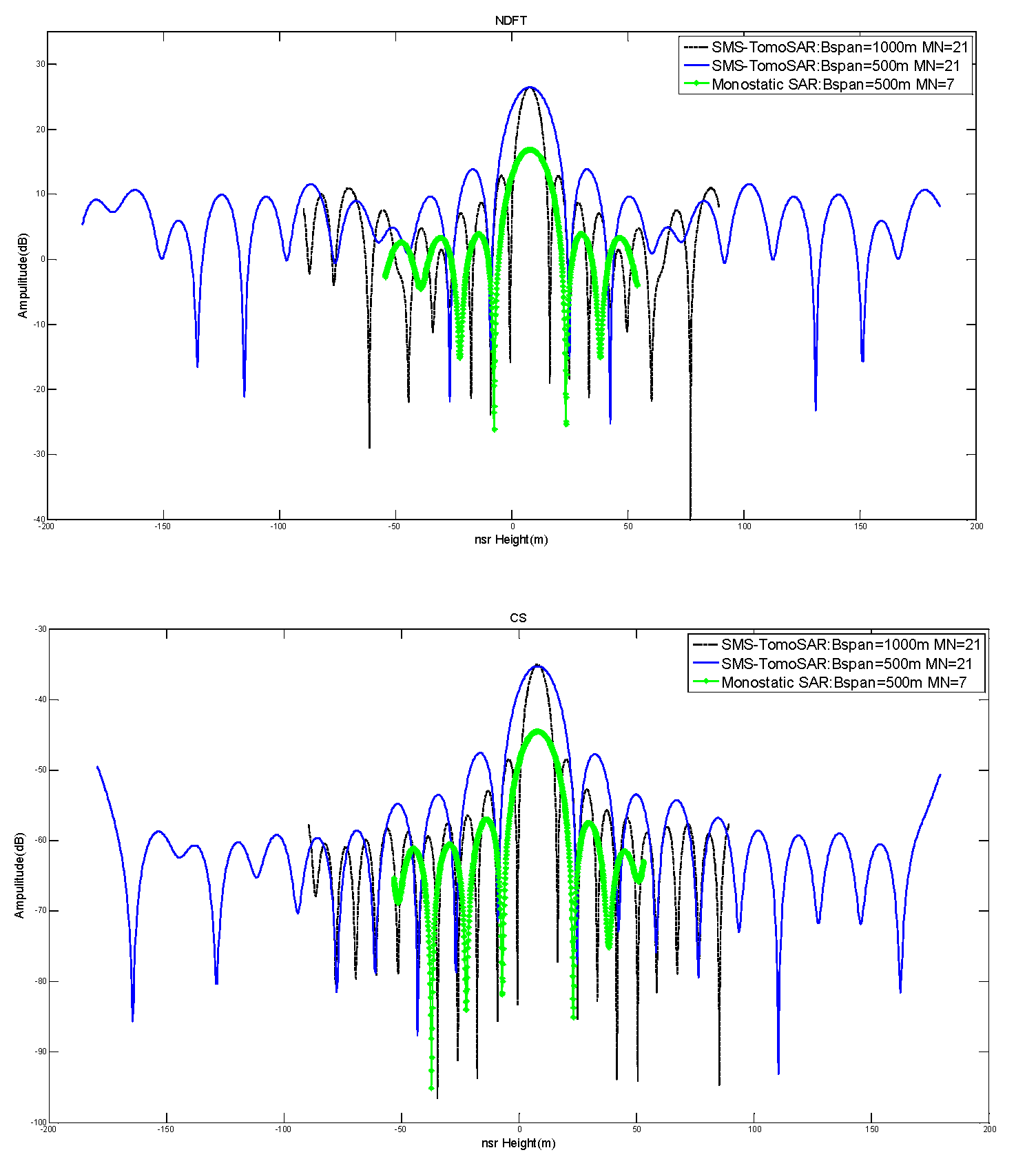
5.1. Analysis of Imaging Intensity Results
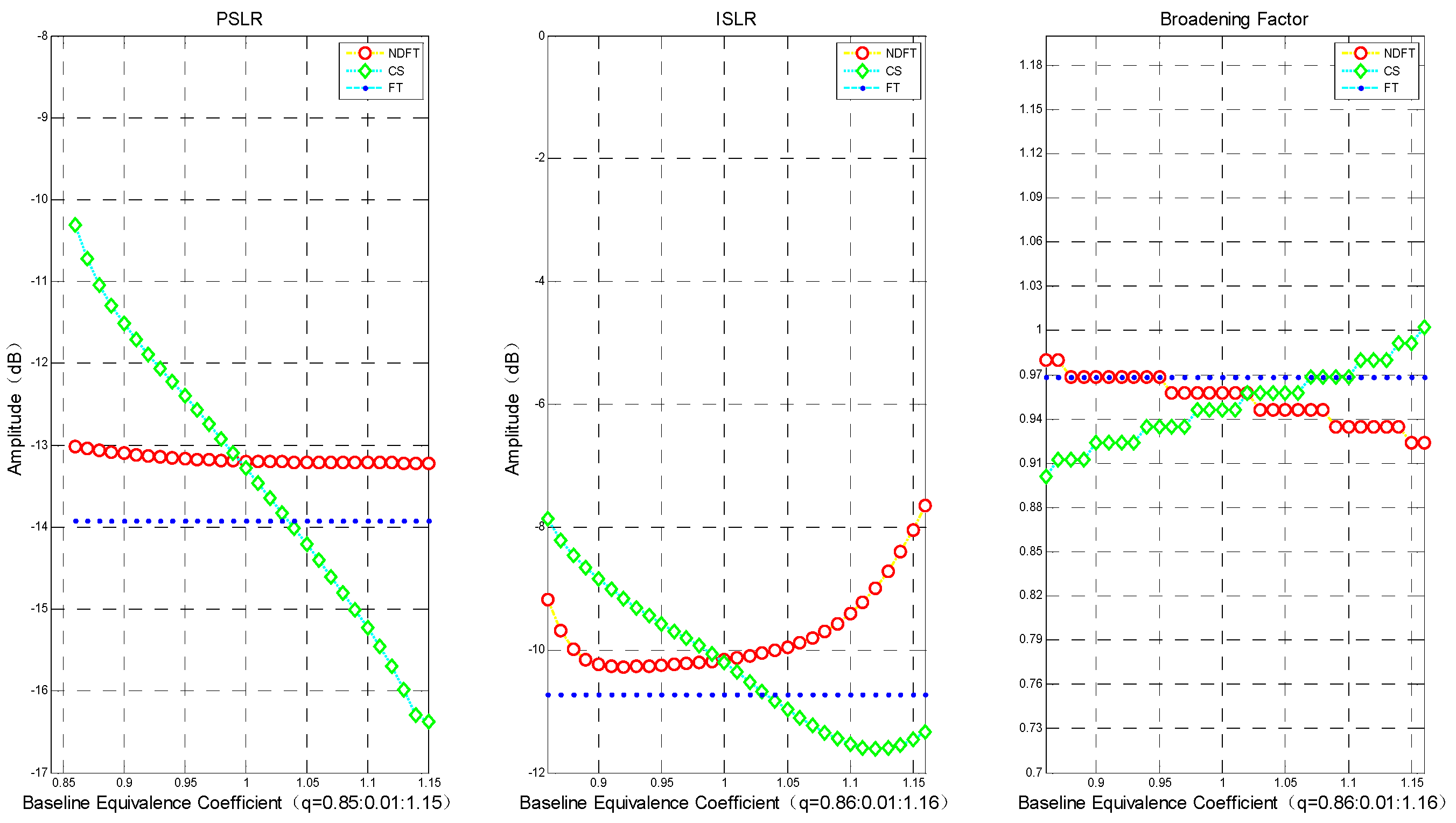
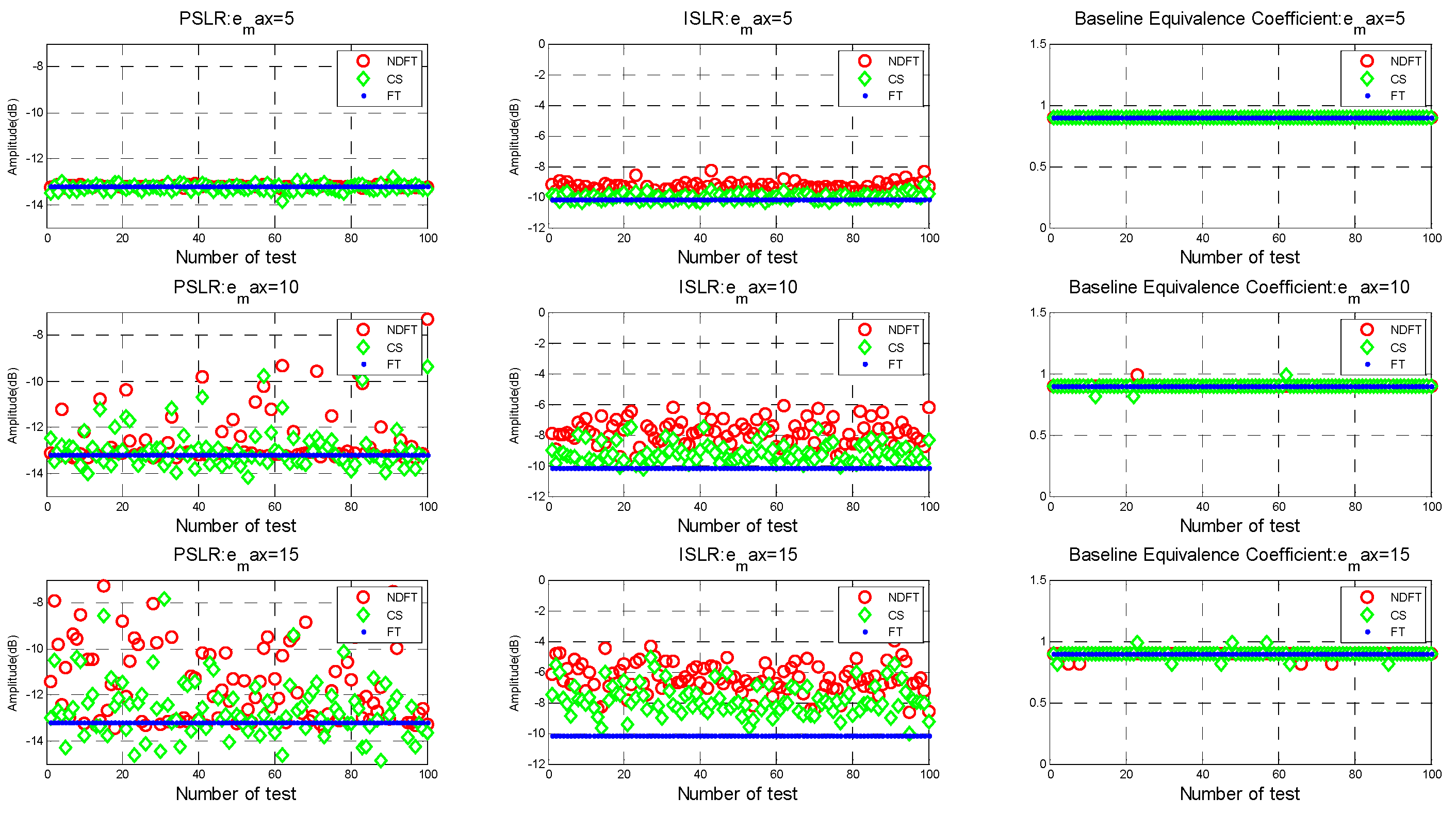
5.2. Baseline Perturbation Analysis
5.3. Baseline Optimization Method Verification
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Knaell, K. Three-dimensional SAR from curvilinear apertures. In Proceedings of the SPIE’s International Symposium on Optical Engineering and Photonics in Aerospace Sensing, Orlando, FL, USA, 4–8 April 1994; pp. 120–134. [Google Scholar]
- Knaell, K.; Cardillo, G.P. Radar tomography for the generation of three-dimensional images. IEE Proc. Radar Sonar Navig. 1995, 142, 54–60. [Google Scholar] [CrossRef]
- Graham, L.C. Synthetic Interferometer Radar for Topographic Mapping. Proc. IEEE 1974, 62, 763–768. [Google Scholar] [CrossRef]
- Lombardini, F. Differential tomography: A new framework for SAR interferometry. IEEE Trans. Geosci. Remote Sens. 2005, 43, 37–44. [Google Scholar] [CrossRef]
- Reigber, A.; Moreira, A. First demonstration of airborne SAR tomography using multibaseline L-band data. IEEE Trans. Geosci. Remote Sens. 2000, 38, 2142–2152. [Google Scholar] [CrossRef]
- Frey, O.; Morsdorf, F.; Meier, E. Tomographic imaging of a forested area by airborne multi-baseline P-band SAR. Sensors 2008, 8, 5884–5896. [Google Scholar] [CrossRef] [PubMed]
- Schmitt, M.; Shahzad, M.; Zhu, X.X. Reconstruction of individual trees from multi-aspect TomoSAR data. Remote Sens. Environ. 2015, 165, 175–185. [Google Scholar] [CrossRef]
- Zhu, X.X.; Bamler, R. Superresolving SAR tomography for multidimensional imaging of urban areas: Compressive sensing-based TomoSAR inversion. IEEE Signal Process. Mag. 2014, 31, 51–58. [Google Scholar] [CrossRef]
- Zhu, X.X.; Bamler, R. Demonstration of Super-Resolution for Tomographic SAR Imaging in Urban Environment. IEEE Trans. Geosci. Remote Sens. 2012, 50, 3150–3157. [Google Scholar] [CrossRef]
- Tebaldini, S.; Rocca, F.; D’Alessandro, M.M.; Ferro-Famil, L. Phase Calibration of Airborne Tomographic SAR Data via Phase Center Double Localization. IEEE Trans. Geosci. Remote Sens. 2016, 54, 1775–1792. [Google Scholar] [CrossRef]
- Zhu, X.; Dong, Z.; Yu, A.; Wu, M.; Li, D.; Zhang, Y. New Approaches for Robust and Efficient Detection of Persistent Scatters in SAR Tomography. Remote Sens. 2019, 11, 356. [Google Scholar] [CrossRef]
- She, Z.; Gray, D.; Bogner, R.; Homer, J. Three-dimensional SAR imaging via multiple pass processing. In Proceedings of the 1995 International Geoscience and Remote Sensing Symposium (IGARSS’95), Florance, Italy, 28 June–2 July 1995; Volume 5, pp. 2389–2391. [Google Scholar]
- Lombardini, F.; Reigber, A. Adaptive Spectral Estimation for Multi-baseline SAR Tomography with Airborne L-band Data. In Proceedings of the 2003 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Toulouse, France, 21–25 July 2003; Volume 3, pp. 2014–2016. [Google Scholar]
- Kumar, S.; Joshi, S.K.; Govil, H. Spaceborne PolSAR Tomography for Forest Height Retrieval. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2017, 10, 5175–5185. [Google Scholar] [CrossRef]
- Sauer, S.; Ferro-Famil, L.; Reigber, A.; Pottier, E. Three-dimensional imaging and scattering mechanism estimation over urban scenes using dual-baseline polarimetric InSAR observations at L-band. IEEE Trans. Geosci. Remote Sens. 2011, 49, 4616–4629. [Google Scholar] [CrossRef]
- Huang, Y.; Ferro-Famil, L. 3D characterization of buildings in a dense urban environment using L-band PolInSAR data with irregular baselines. In Proceedings of the 2009 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Cape Town, South Africa, 12–17 July 2009; Volume 3, pp. 29–32. [Google Scholar]
- Huang, Y.; Ferro-Famil, L.; Reigber, A. Under-foliage Object Imaging Using SAR Tomography and Polarimetric Spectral Estimators. IEEE Trans. Geosci. Remote Sens. 2012, 50, 2213–2225. [Google Scholar] [CrossRef]
- Candès, E.J.; Romberg, J.; Tao, T. Robust uncertainty principles: Exact signal reconstruction from highly incomplete frequency information. IEEE Trans. Inf. Theory 2006, 52, 489–509. [Google Scholar] [CrossRef]
- Budillon, A.; Evangelista, A.; Schirinzi, G. Three-Dimensional SAR Focusing from Multipass Signals Using Compressive Sampling. IEEE Trans. Geosci. Remote Sens. 2011, 49, 488–499. [Google Scholar] [CrossRef]
- Zhu, X.X.; Bamler, R. Tomographic SAR Inversion by L1-Norm Regularization—The Compressive Sensing Approach. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3839–3846. [Google Scholar] [CrossRef]
- Budillon, A.; Ferraioli, G.; Schirinzi, G. Localization Performance of Multiple Scatterers in Compressive Sampling SAR Tomography: Results on COSMO-SkyMed Data. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2014, 7, 2902–2910. [Google Scholar] [CrossRef]
- Bu, Y.C.; Liang, X.D.; Wang, Y. A Unified Algorithm for Channel Imbalance and Antenna Phase Center Position Calibration of a Single-Pass Multi-Baseline TomoSAR System. IEEE Trans. Geosci. Remote Sens. 2018, 10, 456. [Google Scholar] [CrossRef]
- Auterman, J.L. Phase stability requirements for bistatic SAR. In Proceedings of the IEEE National Radar Conference, Atlanta, GA, USA, 1984; pp. 45–52. [Google Scholar]
- Sun, X.L. Research on Differential Intereference Based on Bistatic SAR. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2007. [Google Scholar]
- Wang, M.; Sun, X.L.; Yu, A.X. Research on differential intereference based on bistatic SAR. J. Natl. Def. Sci. Technol. 2008, 30, 71–75. [Google Scholar]






| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Main image height (m) | Target height (m) | ||
| Number of formation satellites | Target complex scattering coefficient | ||
| Number of flights | Target height span (m) | ||
| Repeat-track vertical baseline length (m) | Same-track vertical baseline length (m) | ||
| Baseline span (m) | Unambiguity height (m) |
| DFT | NDFT | CS | |
|---|---|---|---|
| 0.42 m/45.0° | 0.42 m/45.0° | ||
| 0.42 m/45.0° | 0.33 m/45.0° | ||
| 0.42 m/45.0° | 0.29 m/45.0° | ||
| 0.42 m/45.0° | 0.42 m/45.0° | ||
| 0.42 m/45.0° | 0.42 m/45.0° | 0.42 m/45.0° | |
| 0.42 m/45.0° | 0.42 m/45.0° | ||
| 0.42 m/45.0° | 0.42 m/45.0° | ||
| 0.42 m/45.0° | 0.11 m/45.0° | ||
| 0.42 m/45.0° | 0.42 m/45.0° |
| Parameter | |||
|---|---|---|---|
| Parameter (NDFT/CS) | Monostatic SAR | SMS-TomoSAR | SMS-TomoSAR |
|---|---|---|---|
| Target height span (m) | |||
| Rayleigh resolution (m) | |||
| Imaging height error and phase | |||
| Unambiguity height (m) | |||
| PSLR (dB) | |||
| ISLR (dB) |
| Target | Complex Scattering Coefficient | Target nsr Height (m) |
|---|---|---|
| Target A | ||
| Target B | ||
| Target C complex scattering coefficient |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, J.; Yu, A.; Zhang, Y.; Zhu, X.; Dong, Z. Spatial Baseline Optimization for Spaceborne Multistatic SAR Tomography Systems. Sensors 2019, 19, 2106. https://doi.org/10.3390/s19092106
Zhao J, Yu A, Zhang Y, Zhu X, Dong Z. Spatial Baseline Optimization for Spaceborne Multistatic SAR Tomography Systems. Sensors. 2019; 19(9):2106. https://doi.org/10.3390/s19092106
Chicago/Turabian StyleZhao, Jiuchao, Anxi Yu, Yongsheng Zhang, Xiaoxiang Zhu, and Zhen Dong. 2019. "Spatial Baseline Optimization for Spaceborne Multistatic SAR Tomography Systems" Sensors 19, no. 9: 2106. https://doi.org/10.3390/s19092106
APA StyleZhao, J., Yu, A., Zhang, Y., Zhu, X., & Dong, Z. (2019). Spatial Baseline Optimization for Spaceborne Multistatic SAR Tomography Systems. Sensors, 19(9), 2106. https://doi.org/10.3390/s19092106




