Active Vibration Control of a Piezo-Bonded Laminated Composite in the Presence of Sensor Partial Debonding and Structural Delaminations
Abstract
1. Introduction
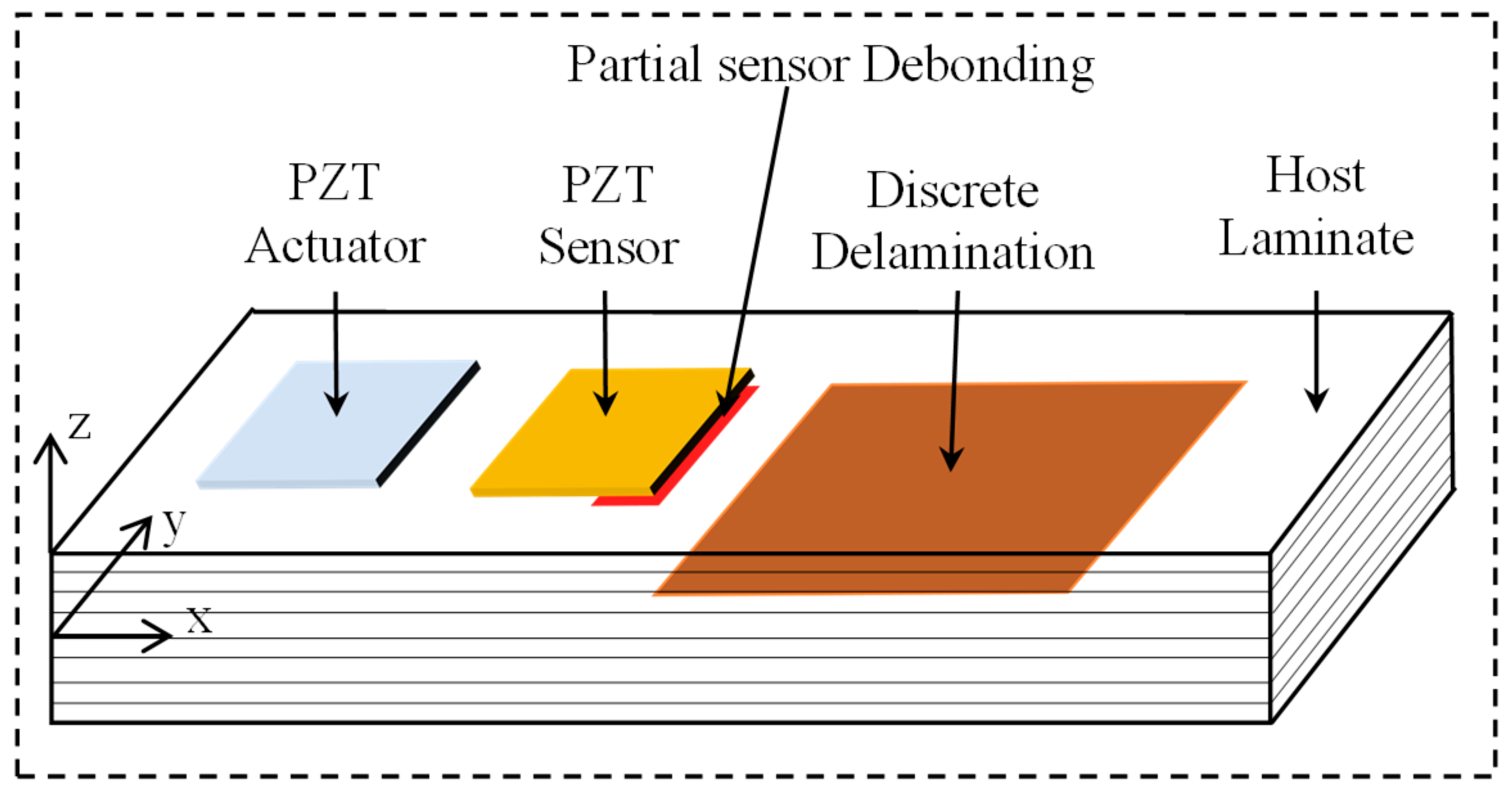
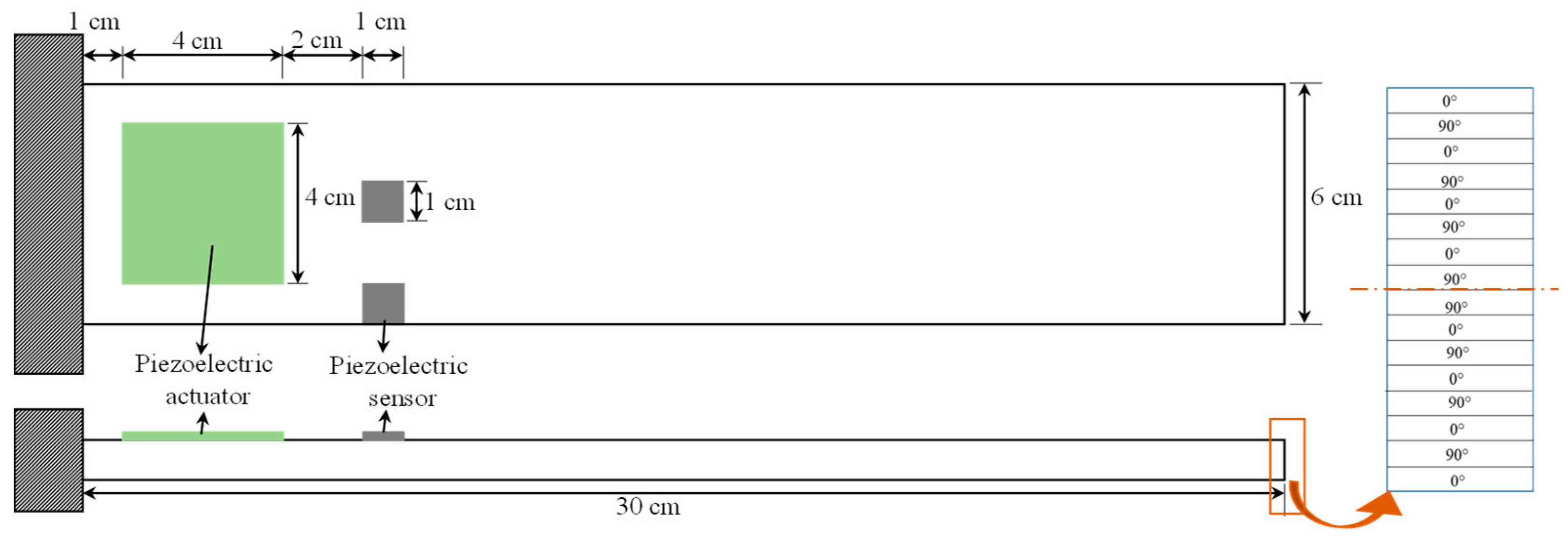
2. Theoretical Formulation
3. Numerical Results and Discussion
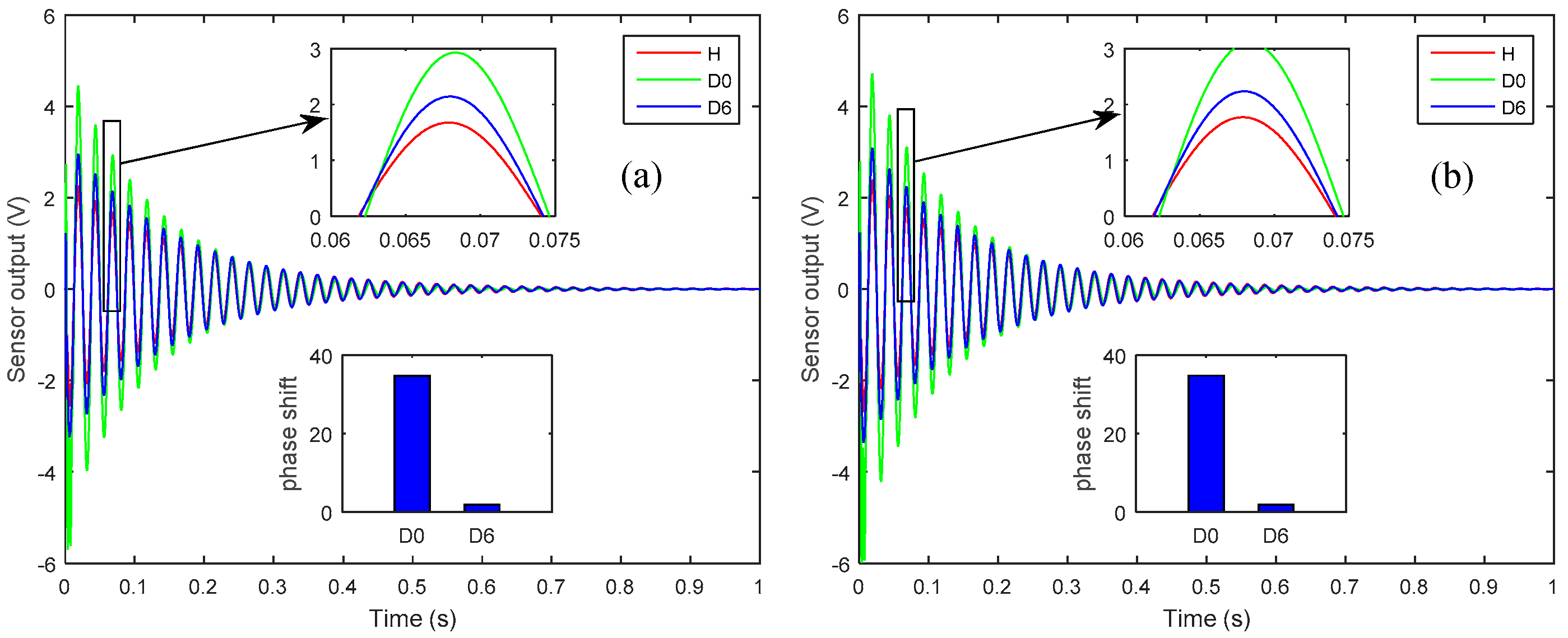
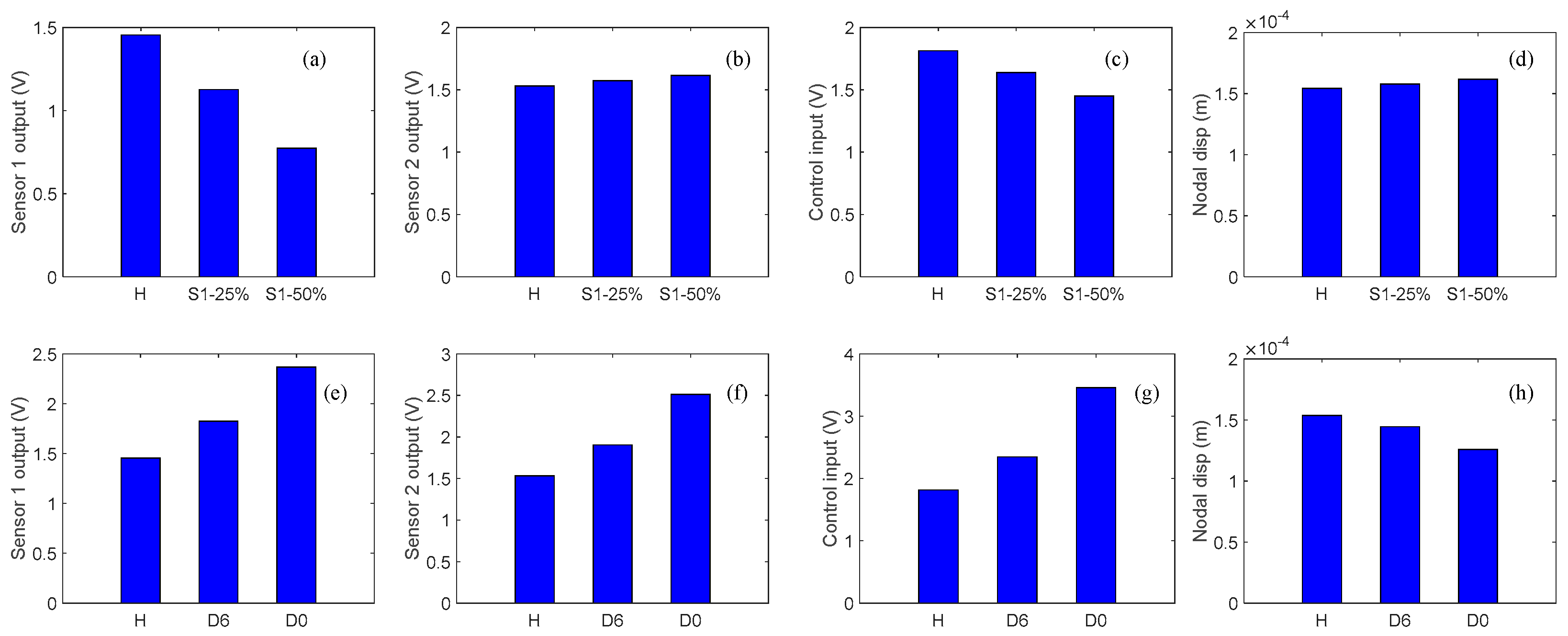
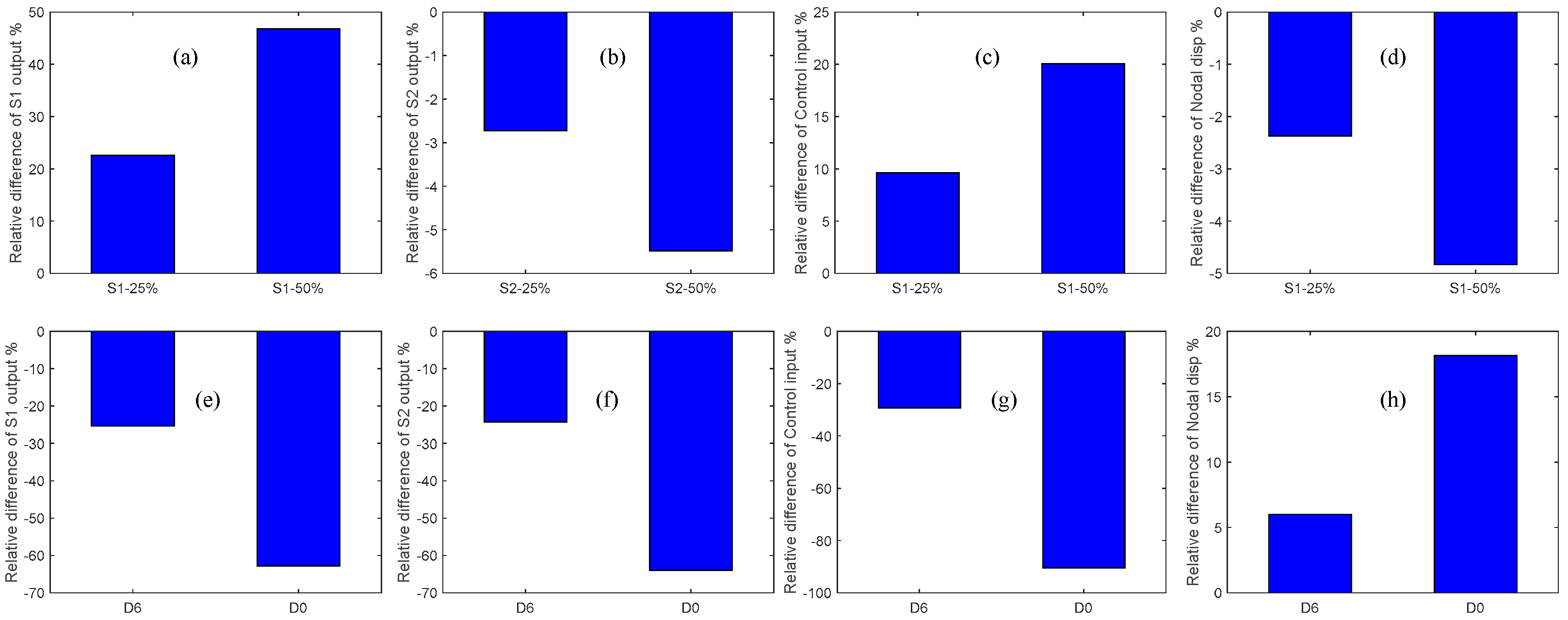
3.1. Effect of Partial Debonding of Sensor
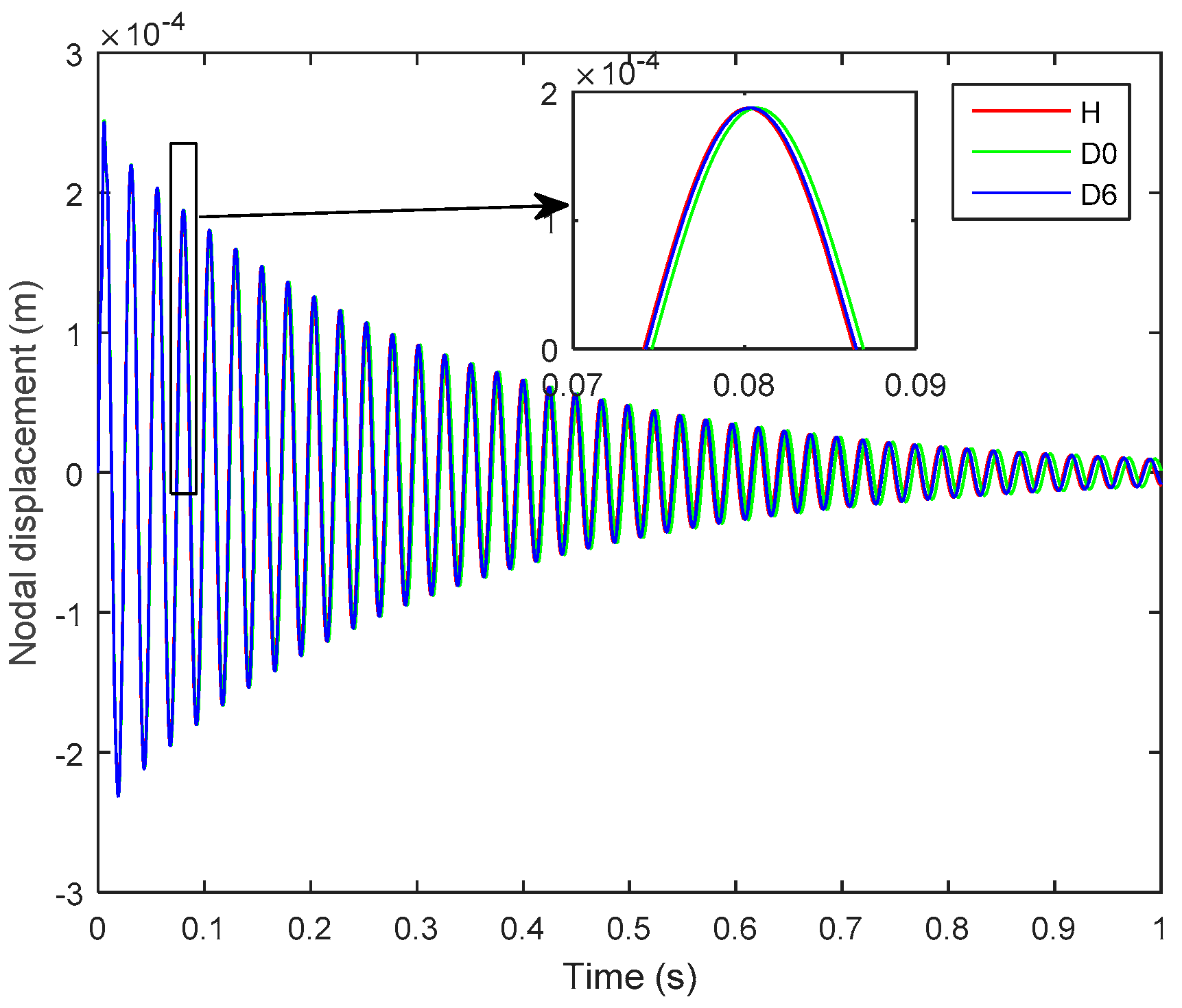
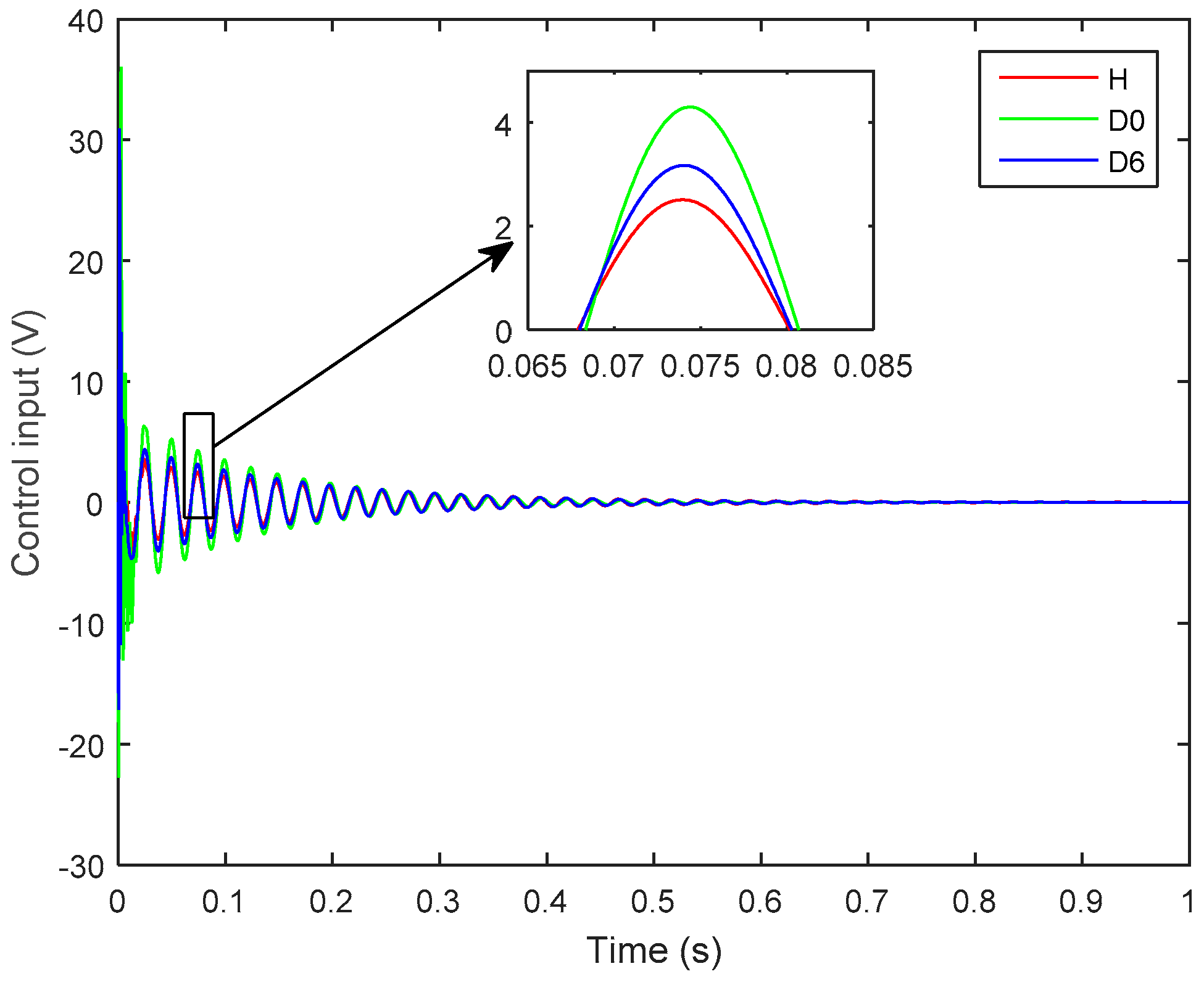
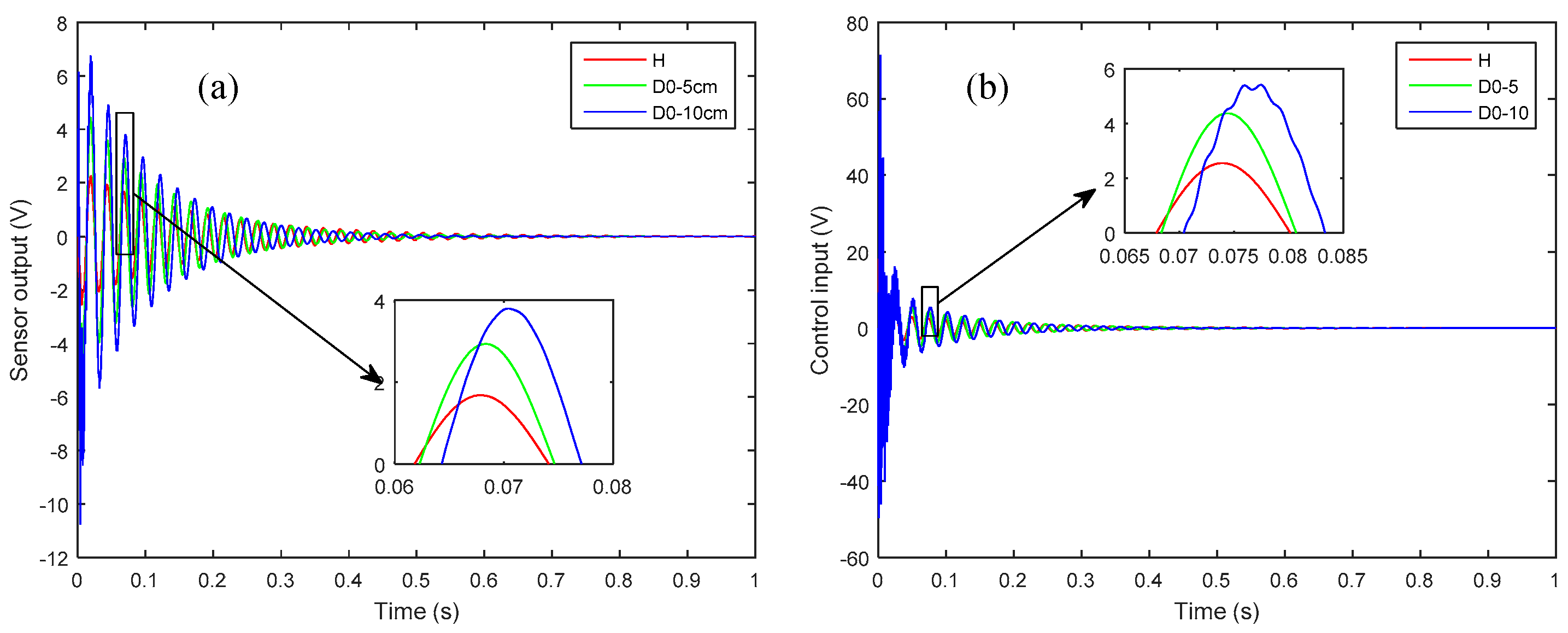
3.2. Effect of Structural Delaminations
3.3. Comparison of Sensor Partial Debonding and Structural Delamination
4. Conclusions
- (1)
- Sensor partial debonding and structural delamination show opposite trends in terms of the control input signals being applied to the actuator and the controlled nodal displacements.
- (2)
- The presence of partial debonding between the piezoelectric sensor and host laminate causes the control input signals to reduce in magnitude and the controlled nodal displacements to increase in magnitude, whereas the presence of delamination damage in the host laminate increases the control input signals and diminishes the controlled nodal displacements.
- (3)
- From a control standpoint, a partially debonded sensor may lead to an unstable controlled system because of the loss of control authority, whereas a delaminated structure may enable the controller to better suppress the vibrations. Also, it is worth noting that the presence of a large structural delamination may cause the smart structure to vibrate with an unboundedly larger amplitude vibration because of the larger amplitude of the control input signal.
- (4)
- Relative phase difference could be used as an index for assessing the severity of sensor partial debonding and structural delamination.
Author Contributions
Funding
Conflicts of Interest
References
- Murugan, S.; Friswell, M. Morphing wing flexible skins with curvilinear fiber composites. Compos. Struct. 2013, 99, 69–75. [Google Scholar] [CrossRef]
- Park, J.-S.; Kim, J.-H. Analytical development of single crystal macro fiber composite actuators for active twist rotor blades. Smart Mater. Struct. 2005, 14, 745. [Google Scholar] [CrossRef]
- Gay, D.; Hoa, S.V. Composite Materials: Design and Applications; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Feng, Q.; Ou, J. Self-sensing cfrp fabric for structural strengthening and damage detection of reinforced concrete structures. Sensors 2018, 18, 4137. [Google Scholar] [CrossRef] [PubMed]
- Feng, Q.; Kong, Q.; Jiang, J.; Liang, Y.; Song, G. Detection of interfacial debonding in a rubber–steel-layered structure using active sensing enabled by embedded piezoceramic transducers. Sensors 2017, 17, 2001. [Google Scholar] [CrossRef] [PubMed]
- Her, S.-C.; Lin, C.-S. Vibration analysis of composite laminate plate excited by piezoelectric actuators. Sensors 2013, 13, 2997–3013. [Google Scholar] [CrossRef] [PubMed]
- Khan, A.; Lee, H.S.; Kim, H.S. Analysis of sensor-debonding failure in active vibration control of smart composite plate. J. Intell. Mater. Syst. Struct. 2017, 28, 2603–2616. [Google Scholar] [CrossRef]
- Ren, L. A theoretical study on shape control of arbitrary lay-up laminates using piezoelectric actuators. Compos. Struct. 2008, 83, 110–118. [Google Scholar] [CrossRef]
- Shankar, G.; Mahato, P.K.; Keshava Kumar, S. Transient analysis and control of delaminated composite plates in hygrothermal environment using active fiber composite actuator. Mech. Adv. Mater. Struct. 2018, 1–21. [Google Scholar] [CrossRef]
- Raja, S.; Adya, H.P.; Viswanath, S. Analysis of piezoelectric composite beams and plates with multiple delaminations. Struct. Health Monit. 2006, 5, 255–266. [Google Scholar] [CrossRef]
- Kim, H.S.; Chattopadhyay, A.; Ghoshal, A. Dynamic analysis of composite laminates with multiple delamination using improved layerwise theory. AIAA J. 2003, 41, 1771–1779. [Google Scholar] [CrossRef]
- Li, B.; Ye, L.; Li, Z.; Ma, Z.; Kalhori, H. Quantitative identification of delamination at different interfaces using guided wave signals in composite laminates. J. Reinf. Plast. Compos. 2015, 34, 1506–1525. [Google Scholar] [CrossRef]
- Abrate, S. Matrix cracking in laminated composites: A review. Compos. Eng. 1991, 1, 337–353. [Google Scholar] [CrossRef]
- Le, M.; Bainier, H.; Néron, D.; Ha-Minh, C.; Ladevèze, P. On matrix cracking and splits modeling in laminated composites. Compos. Part A Appl.Sci. Manuf. 2018, 115, 294–301. [Google Scholar] [CrossRef]
- Jain, A.; Upadhyay, C.; Mohite, P. Fibre breaking damage model for unidirectional fibrous composites using micromechanics. In Proceedings of the 16th National Seminar on Aerospace Structures (NASAS), Mumbai, India, 19–20 November 2009. [Google Scholar]
- Wisnom, M. The role of delamination in failure of fibre-reinforced composites. Phil. Trans. R. Soc. A 2012, 370, 1850–1870. [Google Scholar] [CrossRef] [PubMed]
- Huang, B.; Kim, H.S. Free-edge interlaminar stress analysis of piezo-bonded composite laminates under symmetric electric excitation. Int. J. Solids Struct. 2014, 51, 1246–1252. [Google Scholar] [CrossRef]
- Mitchell, J.; Reddy, J. A refined hybrid plate theory for composite laminates with piezoelectric laminae. Int. J. Solids Struct. 1995, 32, 2345–2367. [Google Scholar] [CrossRef]
- Crawley, E.F.; De Luis, J. Use of piezoelectric actuators as elements of intelligent structures. AIAA J. 1987, 25, 1373–1385. [Google Scholar] [CrossRef]
- Ha, S.K.; Keilers, C.; Chang, F.-K. Finite element analysis of composite structures containing distributed piezoceramic sensors and actuators. AIAA J. 1992, 30, 772–780. [Google Scholar] [CrossRef]
- Gohari, S.; Sharifi, S.; Abadi, R.; Izadifar, M.; Burvill, C.; Vrcelj, Z. A quadratic piezoelectric multi-layer shell element for fe analysis of smart laminated composite plates induced by mfc actuators. Smart Mater. Struct. 2018, 27, 095004. [Google Scholar] [CrossRef]
- Chattopadhyay, A.; Seeley, C.E. A higher order theory for modeling composite laminates with induced strain actuators. Compos. Part B Eng. 1997, 28, 243–252. [Google Scholar] [CrossRef]
- Alibeigloo, A.; Madoliat, R. Static analysis of cross-ply laminated plates with integrated surface piezoelectric layers using differential quadrature. Compos. Struct. 2009, 88, 342–353. [Google Scholar] [CrossRef]
- Li, W.; Shen, H. A layerwise finite element formulation of laminated composite cylindrical shells with piezoelectric layers. J. Mech. Sci. Technol. 2018, 32, 731–741. [Google Scholar] [CrossRef]
- Peng, H.; Ye, L.; Meng, G.; Mustapha, S.; Li, F. Concise analysis of wave propagation using the spectral element method and identification of delamination in cf/ep composite beams. Smart Mater. Struct. 2010, 19, 085018. [Google Scholar] [CrossRef]
- Aoki, Y.; Gardonio, P.; Elliott, S. Modelling of a piezoceramic patch actuator for velocity feedback control. Smart Mater. Struct. 2008, 17, 015052. [Google Scholar] [CrossRef]
- Roy, T.; Chakraborty, D. Optimal vibration control of smart fiber reinforced composite shell structures using improved genetic algorithm. J. Sound Vib. 2009, 319, 15–40. [Google Scholar] [CrossRef]
- Fanson, J.; Caughey, T.K. Positive position feedback control for large space structures. AIAA J. 1990, 28, 717–724. [Google Scholar] [CrossRef]
- Proulx, B.; Cheng, L. Dynamic analysis of piezoceramic actuation effects on plate vibrations. Thin-Walled Struct. 2000, 37, 147–162. [Google Scholar] [CrossRef]
- Kapuria, S.; Yasin, M.Y.; Hagedorn, P. Active vibration control of piezolaminated composite plates considering strong electric field nonlinearity. AIAA J. 2014, 53, 603–616. [Google Scholar] [CrossRef]
- Bendine, K.; Boukhoulda, F.; Haddag, B.; Nouari, M. Active vibration control of composite plate with optimal placement of piezoelectric patches. Mech. Adv. Mater. Struct. 2017, 1–9. [Google Scholar] [CrossRef]
- Zorić, N.D.; Simonović, A.M.; Mitrović, Z.S.; Stupar, S.N. Optimal vibration control of smart composite beams with optimal size and location of piezoelectric sensing and actuation. J. Intell. Mater. Syst. Struct. 2013, 24, 499–526. [Google Scholar] [CrossRef]
- Phung-Van, P.; De Lorenzis, L.; Thai, C.H.; Abdel-Wahab, M.; Nguyen-Xuan, H. Analysis of laminated composite plates integrated with piezoelectric sensors and actuators using higher-order shear deformation theory and isogeometric finite elements. Comput. Mater. Sci. 2015, 96, 495–505. [Google Scholar] [CrossRef]
- Knauss, W.; Gonzalez, L. Global Failure Modes in Composite Structures; Technical Report; California Institute of Technology: Pasadena, CA, USA, 2001. [Google Scholar]
- Daniel, I. Failure of composite materials. Strain 2007, 43, 4–12. [Google Scholar] [CrossRef]
- Li, Z.; Koh, B.; Nagarajaiah, S. Detecting sensor failure via decoupled error function and inverse input–output model. J. Eng. Mech. 2007, 133, 1222–1228. [Google Scholar] [CrossRef]
- Kumar, D.N.; Raja, S.; Ikeda, T. Active vibration control of smart plates with partially debonded multilayered pzt actuators. Smart Mater. Struct. 2007, 16, 1584–1594. [Google Scholar] [CrossRef]
- Huang, B.; Kim, H.S.; Youn, B.D. Active vibration control of smart composite laminates with partial debonding of actuator. Int. J. Precis. Eng. Manuf. 2015, 16, 831–840. [Google Scholar] [CrossRef]
- Sun, D.; Tong, L.; Atluri, S.N. Effects of piezoelectric sensor/actuator debonding on vibration control of smart beams. Int. J. Solids Struct. 2001, 38, 9033–9051. [Google Scholar] [CrossRef]
- Kim, H.S.; Chattopadhyay, A.; Ghoshal, A. Characterization of delamination effect on composite laminates using a new generalized layerwise approach. Comput. Struct. 2003, 81, 1555–1566. [Google Scholar] [CrossRef]
- Kim, H.S.; Zhou, X.; Chattopadhyay, A. Interlaminar stress analysis of shell structures with piezoelectric patch including thermal loading. AIAA J. 2002, 40, 2517–2525. [Google Scholar] [CrossRef]
- Alnefaie, K. Finite element modeling of composite plates with internal delamination. Compos. Struct. 2009, 90, 21–27. [Google Scholar] [CrossRef]
- Munteanu, E.; Ursu, I. Piezo smart composite wing with LQG/LTR control. In Proceedings of the 2008 IEEE International Symposium on Industrial Electronics, Cambridge, UK, 30 June–2 July 2008; pp. 1160–1165. [Google Scholar]
- Chen, Y.; Viresh, W.; Zimcik, D. Development and verification of real-time controllers for the F/A-18 vertical fin buffet load alleviation. In Proceedings of the Smart Structures and Materials 2006: Smart Structures and Integrated Systems, San Diego, CA, USA, 5 April 2006. [Google Scholar] [CrossRef]




| Properties | Host Lamina | PZT-5H |
|---|---|---|
| Young’s modulus (GPa) | E1=372, E2 = E3 = 4.12 | 62 |
| Shear modulus (GPa) | G12 = G13 = 3.99, G23 = 3.6 | G = 23.67 |
| Poisson’s ration | ν12 = ν13 = 0.275, ν23 = 0.42 | ν = 0.31 |
| Density (kg/m3) | 1788.5 | 7500 |
| Piezoelectric constant (m/V) | - | d31 = d32 = −274 × 10−12 |
| - | - | d24 = d15 = 741 × 10−12 |
| Permittivity (nF/m) | - | b11 = b22 = b33 = 2.638 |
| Length (m) | 0.3 | 0.01 |
| Width (m) | 0.06 | 0.01 |
| Thickness (m) | 0.125×10−3 | 0.25 × 10−3 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, A.; Kim, H.S. Active Vibration Control of a Piezo-Bonded Laminated Composite in the Presence of Sensor Partial Debonding and Structural Delaminations. Sensors 2019, 19, 540. https://doi.org/10.3390/s19030540
Khan A, Kim HS. Active Vibration Control of a Piezo-Bonded Laminated Composite in the Presence of Sensor Partial Debonding and Structural Delaminations. Sensors. 2019; 19(3):540. https://doi.org/10.3390/s19030540
Chicago/Turabian StyleKhan, Asif, and Heung Soo Kim. 2019. "Active Vibration Control of a Piezo-Bonded Laminated Composite in the Presence of Sensor Partial Debonding and Structural Delaminations" Sensors 19, no. 3: 540. https://doi.org/10.3390/s19030540
APA StyleKhan, A., & Kim, H. S. (2019). Active Vibration Control of a Piezo-Bonded Laminated Composite in the Presence of Sensor Partial Debonding and Structural Delaminations. Sensors, 19(3), 540. https://doi.org/10.3390/s19030540






