Dynamics and Embedded Internet of Things Input Shaping Control for Overhead Cranes Transporting Multibody Payloads
Abstract
:1. Introduction
2. Contributions
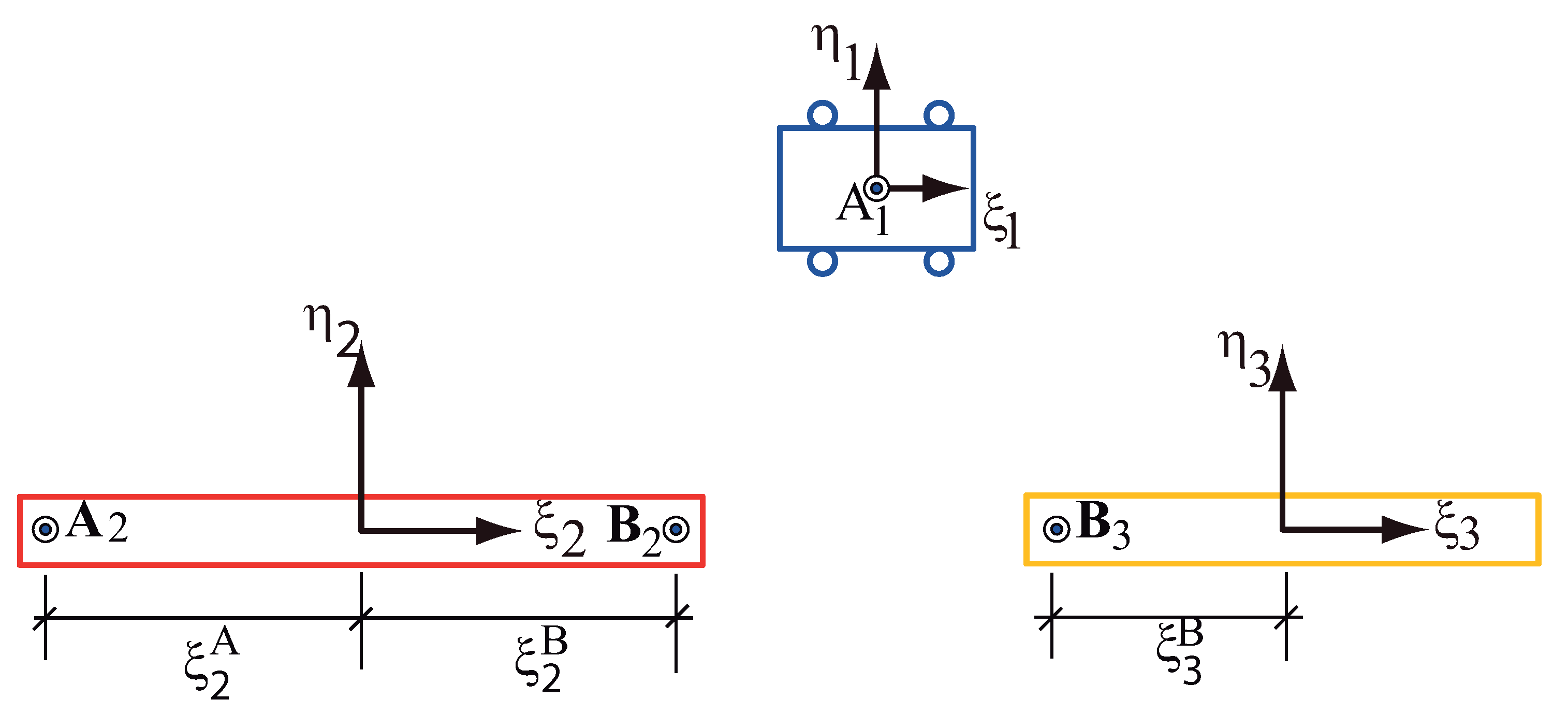
- First, the kinematic joints constraint equations of the multibody system model are written in compact form using generalized coordinates . Thus, deriving, the velocity constraints are obtained arising the corresponding Jacobian matrix of the multibody model DAEs .
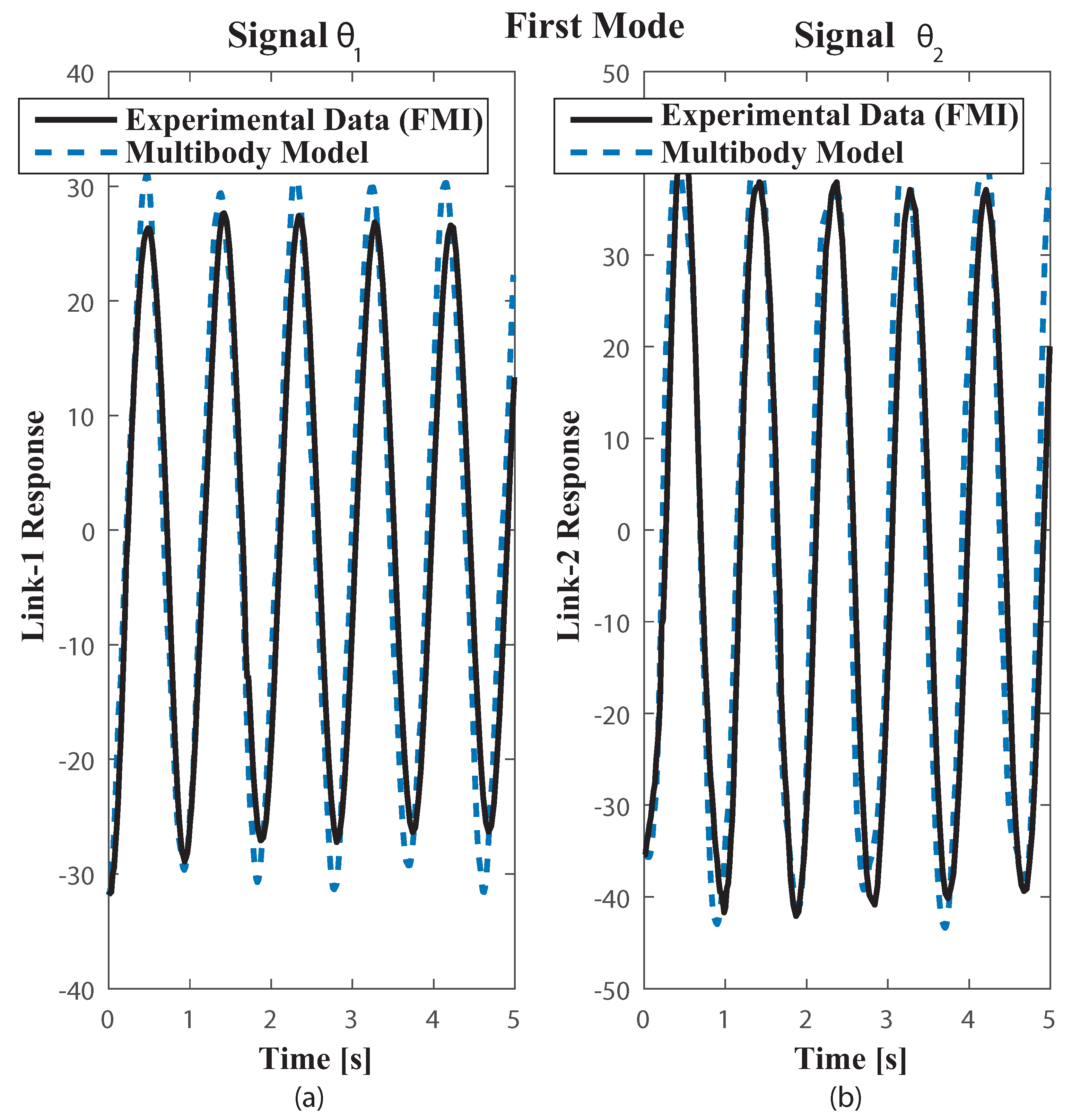
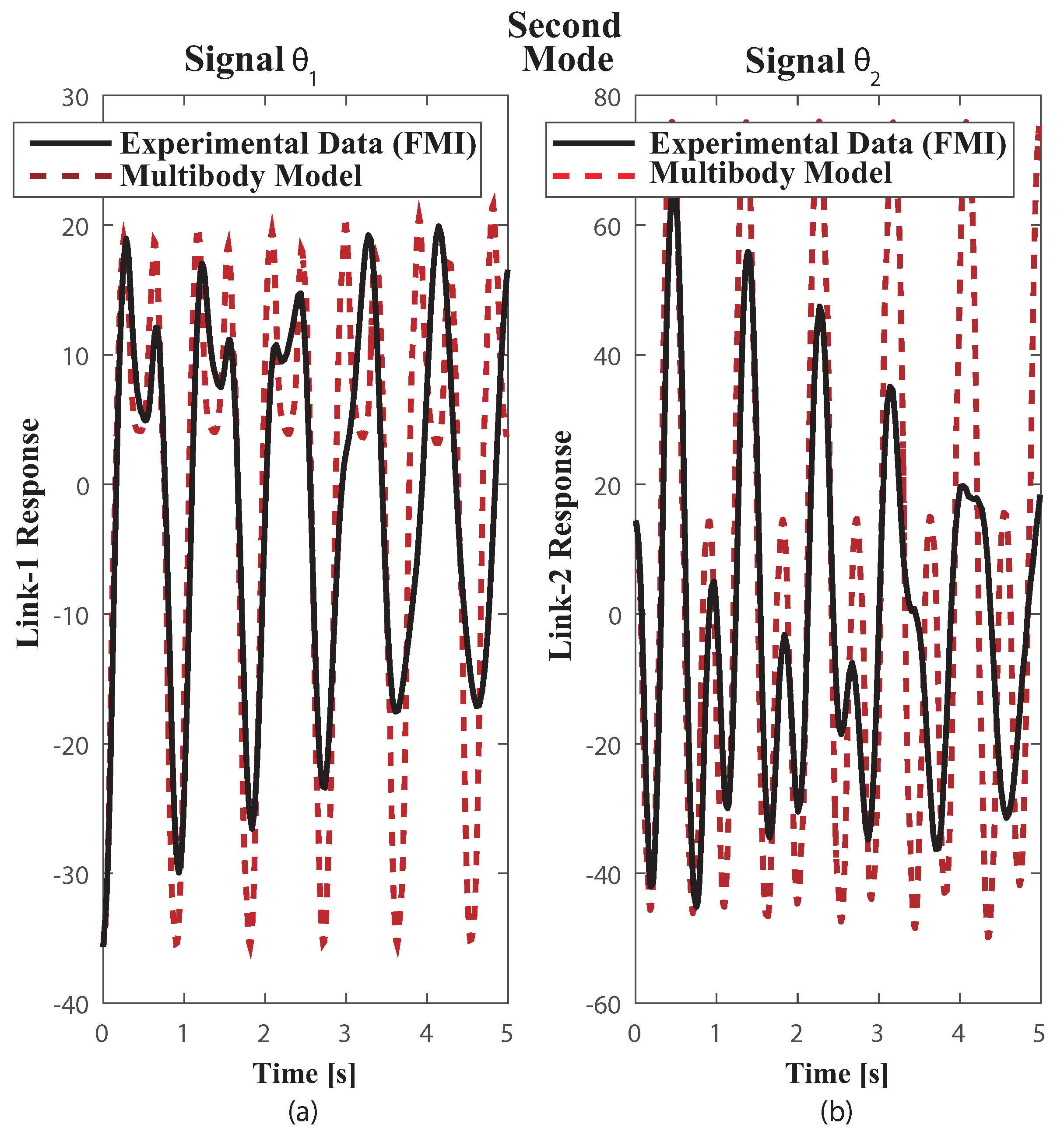
- The reliability of the multibody model is validated facing the simulated responses with the experimental signal of the real-world responses, extracted by video-tapping the functional mock up interface (FMI) motion.
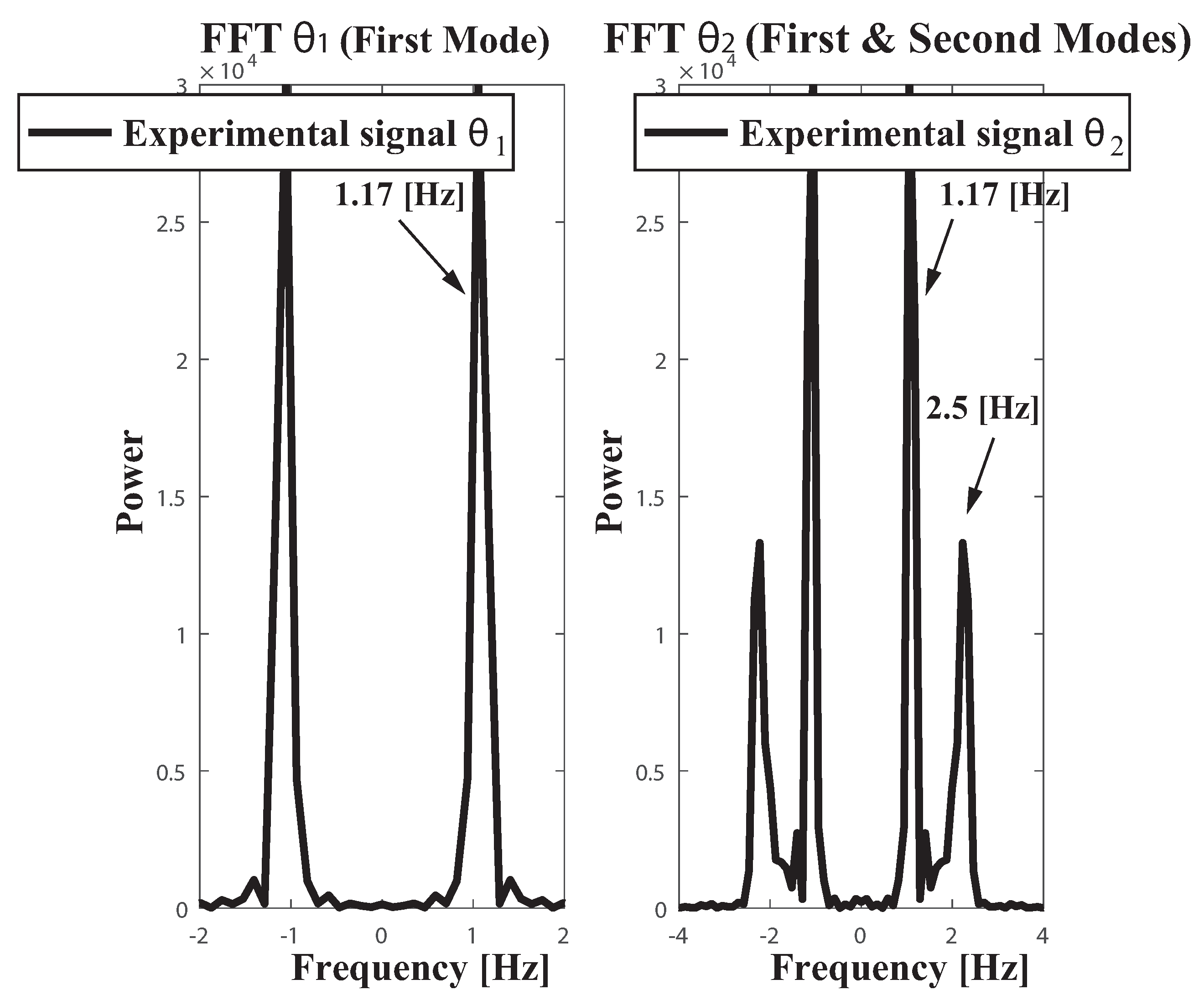
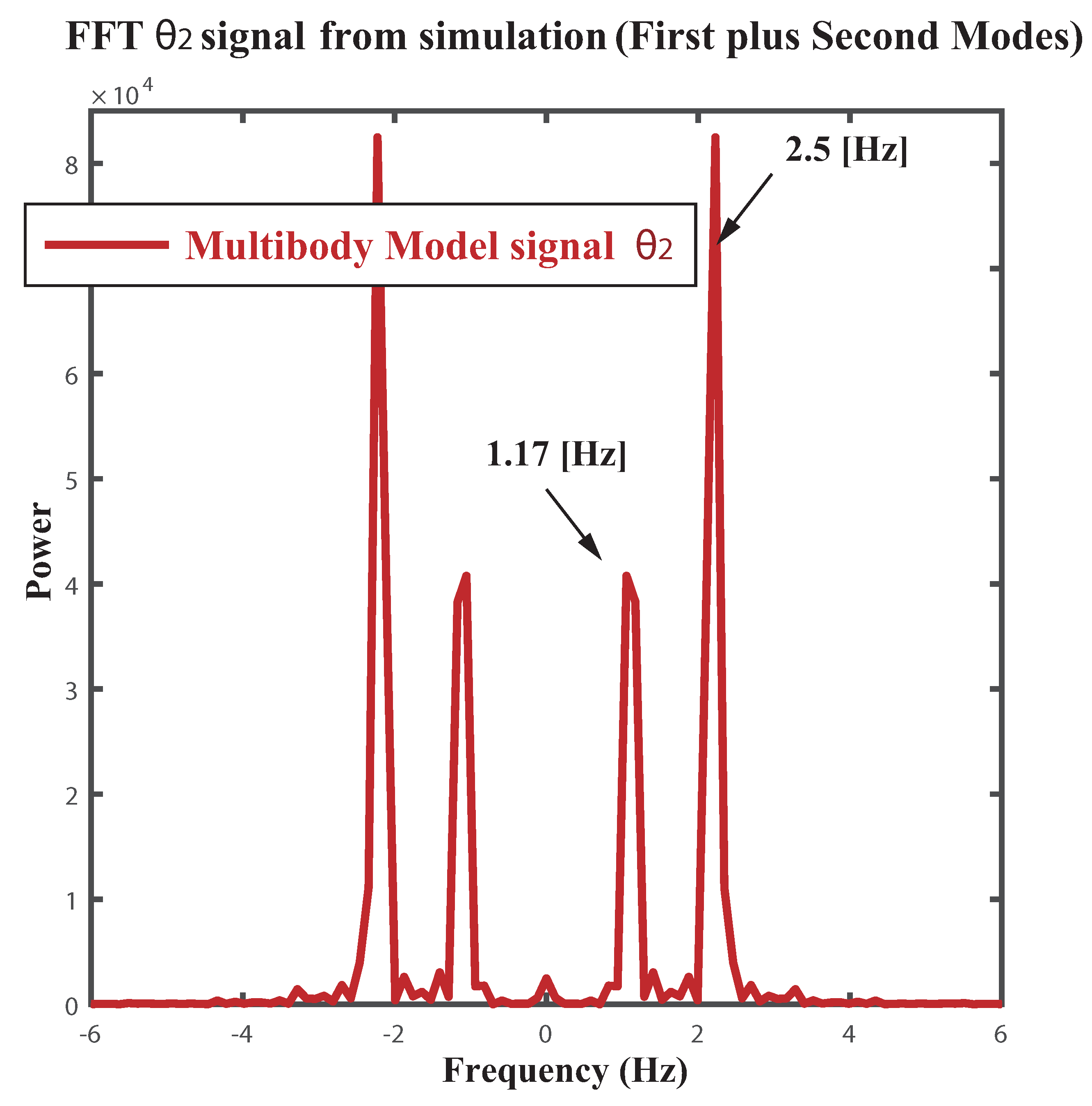
- The FFTs of the experimental and the simulated signals contain the same frequency harmonics only with somewhat different power due to some real world light damping in the joints. Nonetheless the high degree correspondence between the signal spectrums guarantees the reliable multibody system flexible modes of vibration identification.
- To give some insight into the multibody double pendulum dynamics by Lagrangian methods a somewhat simplified, using a composite revolute-revolute joint, two link benchmark multibody model, is proposed. This allows to develop a general study of this type of multibody payloads dynamics including its normal modes, modes ratios plus ranges of frequencies expected.
- A robust Direct Specified Insenstivity (DSI) shaper is synthesized for the frequency ranges to be cancelled with help of the pair GAMS-CONOPT a proven choice for highly nonlinear problems. The DSI shaper is robust plus optimal, regarding the number of the filter taps, to be implemented in an embedded microcontroller working as the system command generator that carry out the convolution sum in real-time.
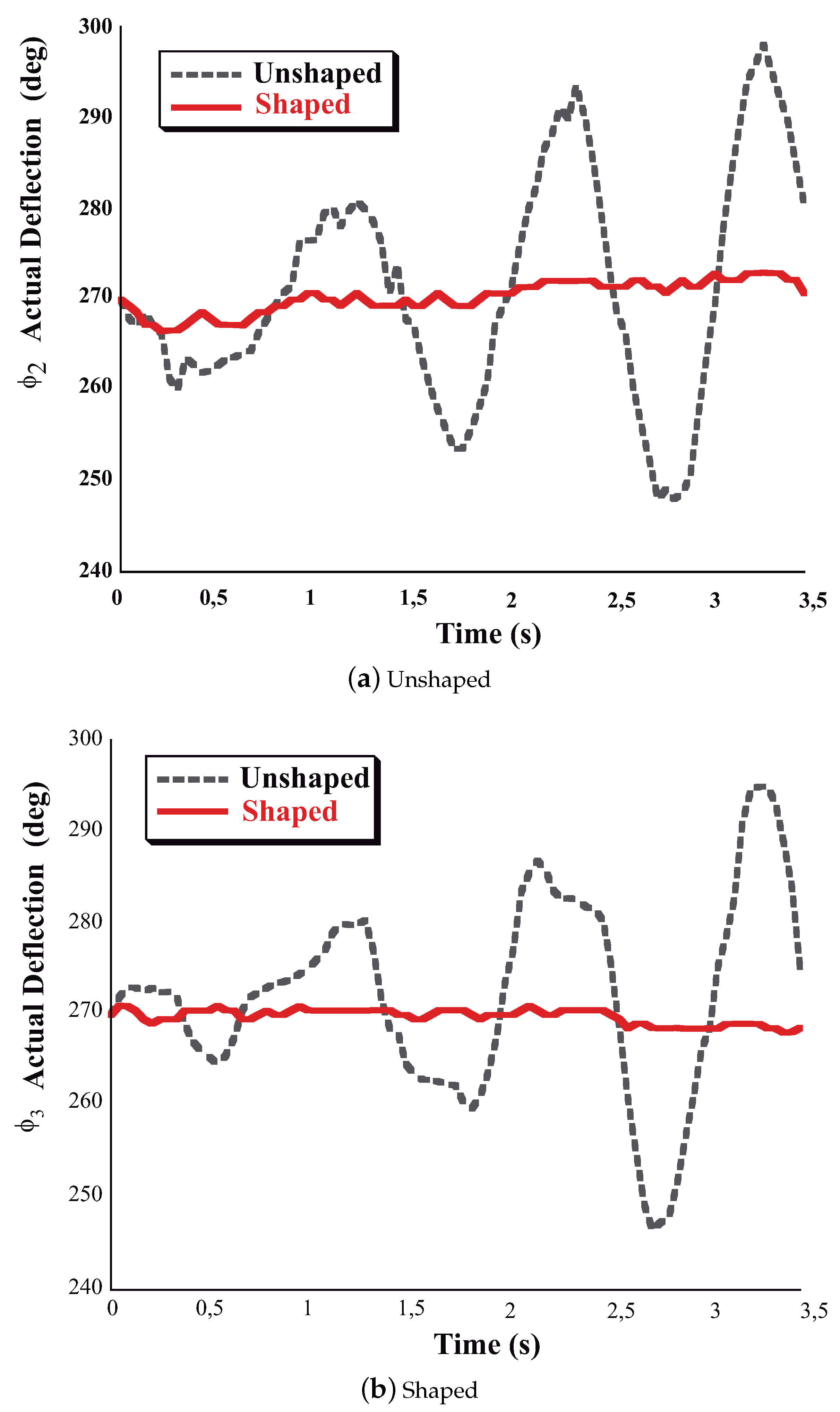
- The DSI shaper robustness performance is tested by simulation using the multibody model plus facing the DSI burned microcontroller working as the command generator of the real world FMI set up and the hydraulic crane.
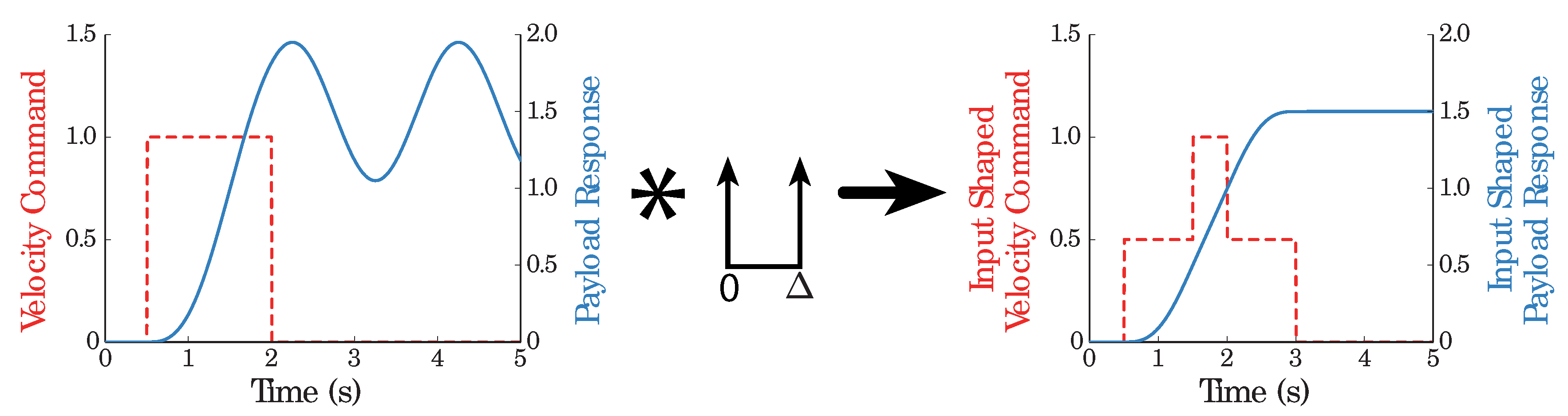
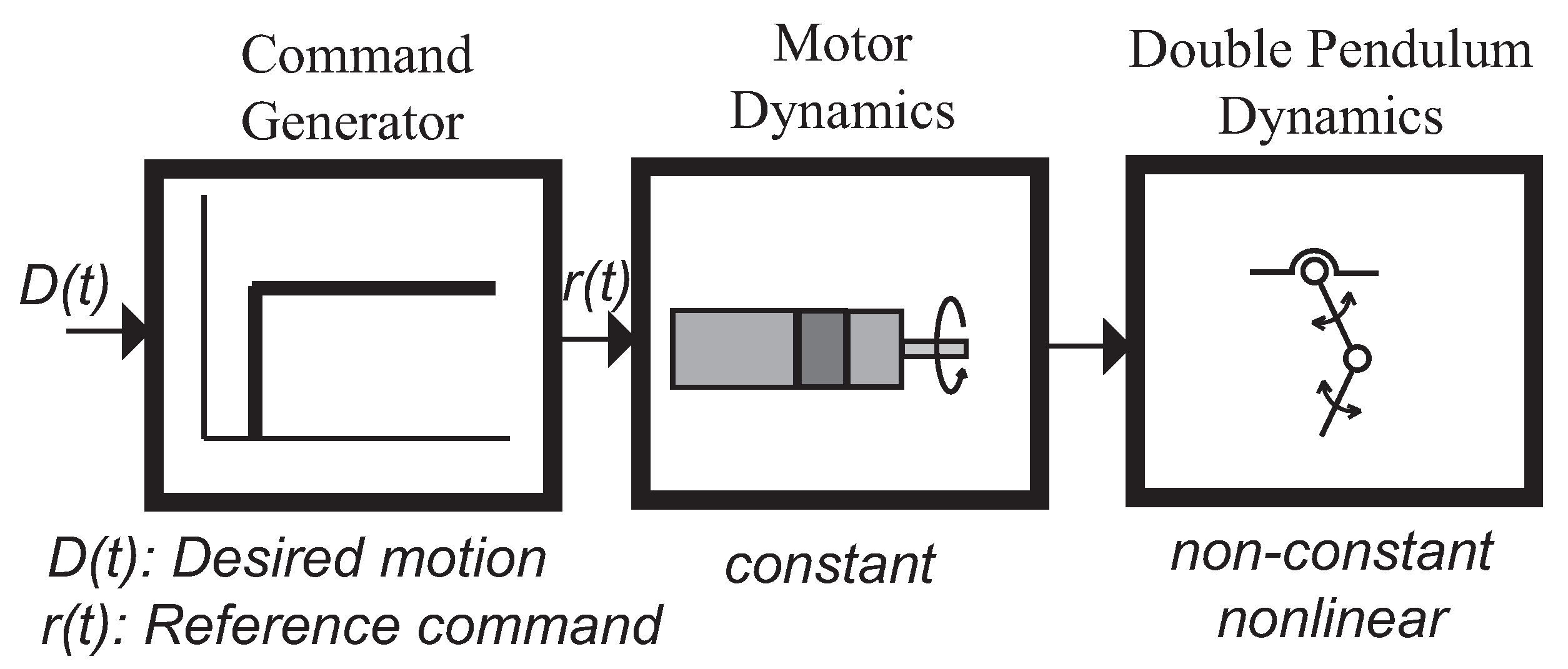
3. Conceptualization: Robust Input Shaping
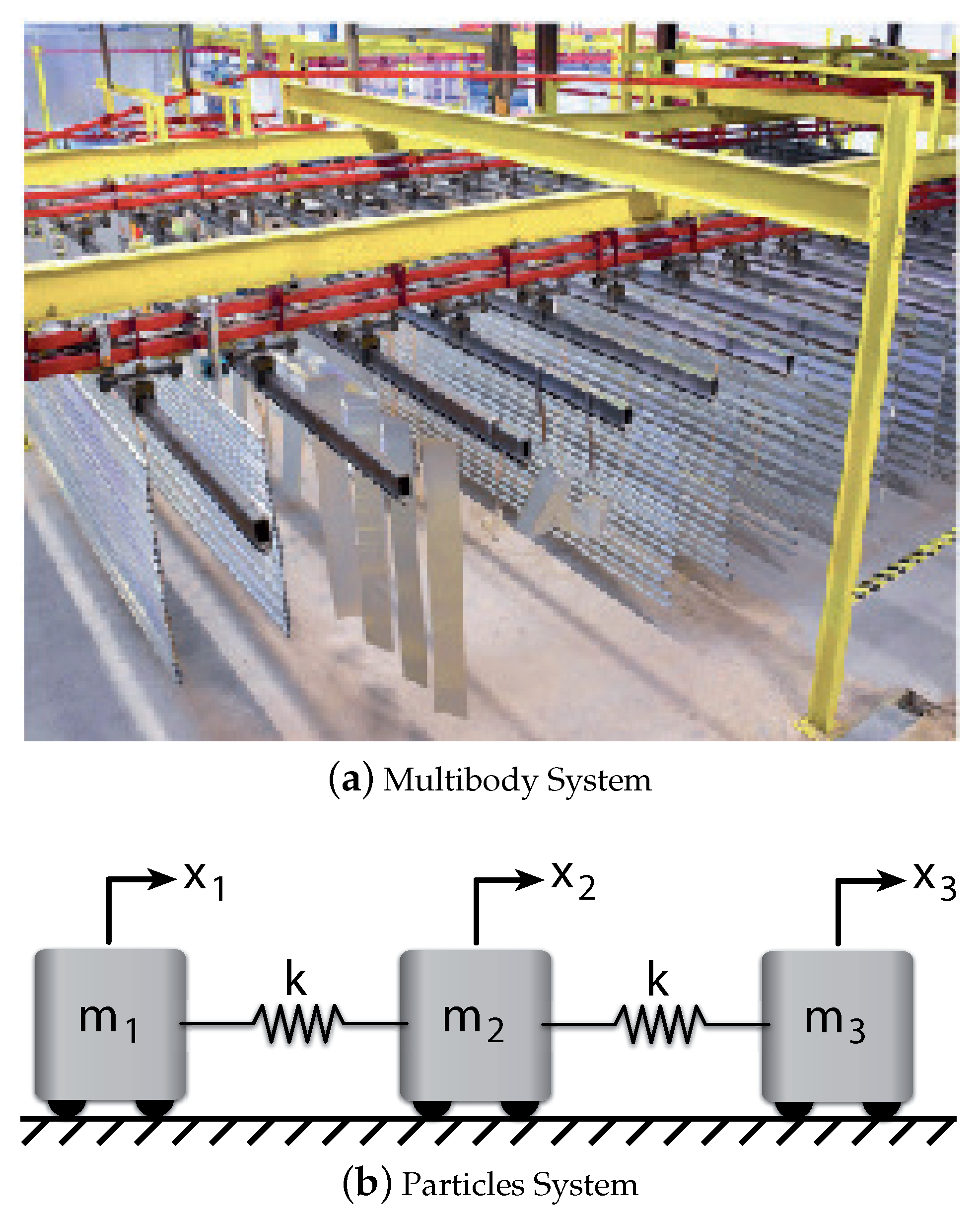
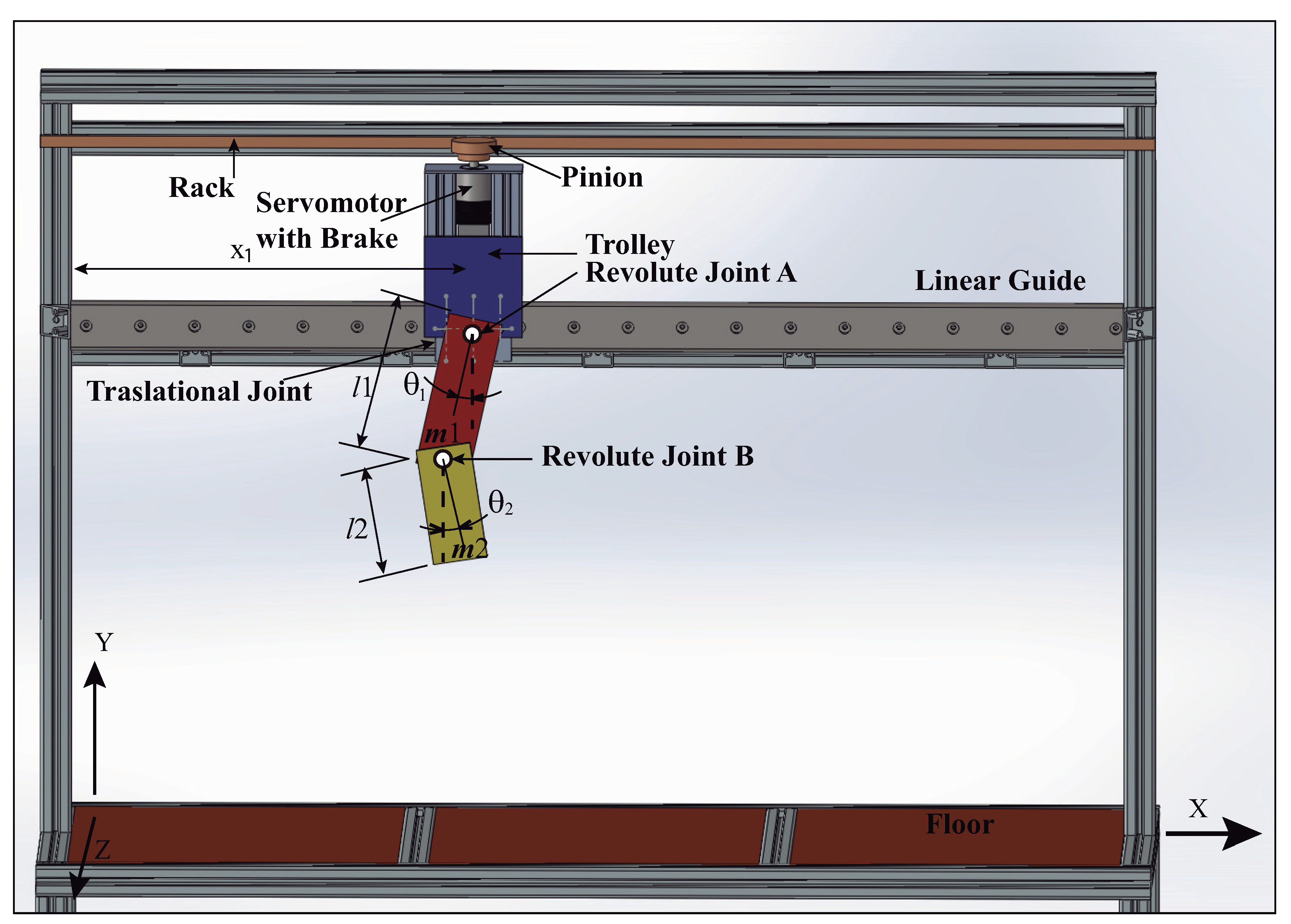
4. Overhead Crane Multibody Model
- A sliding joint between the body ①, the trolley, and the fixed frame.
- A pin joint between the body ②, the first link, and the trolley, body ①.
- A pin joint between the body ③, the second link, and the body ②, the first link.
5. Validation of the Model
5.1. Validation of the Model Simulation Results Face to Face with Experimental FMI Outcomes
5.2. Lagrangian Analysis
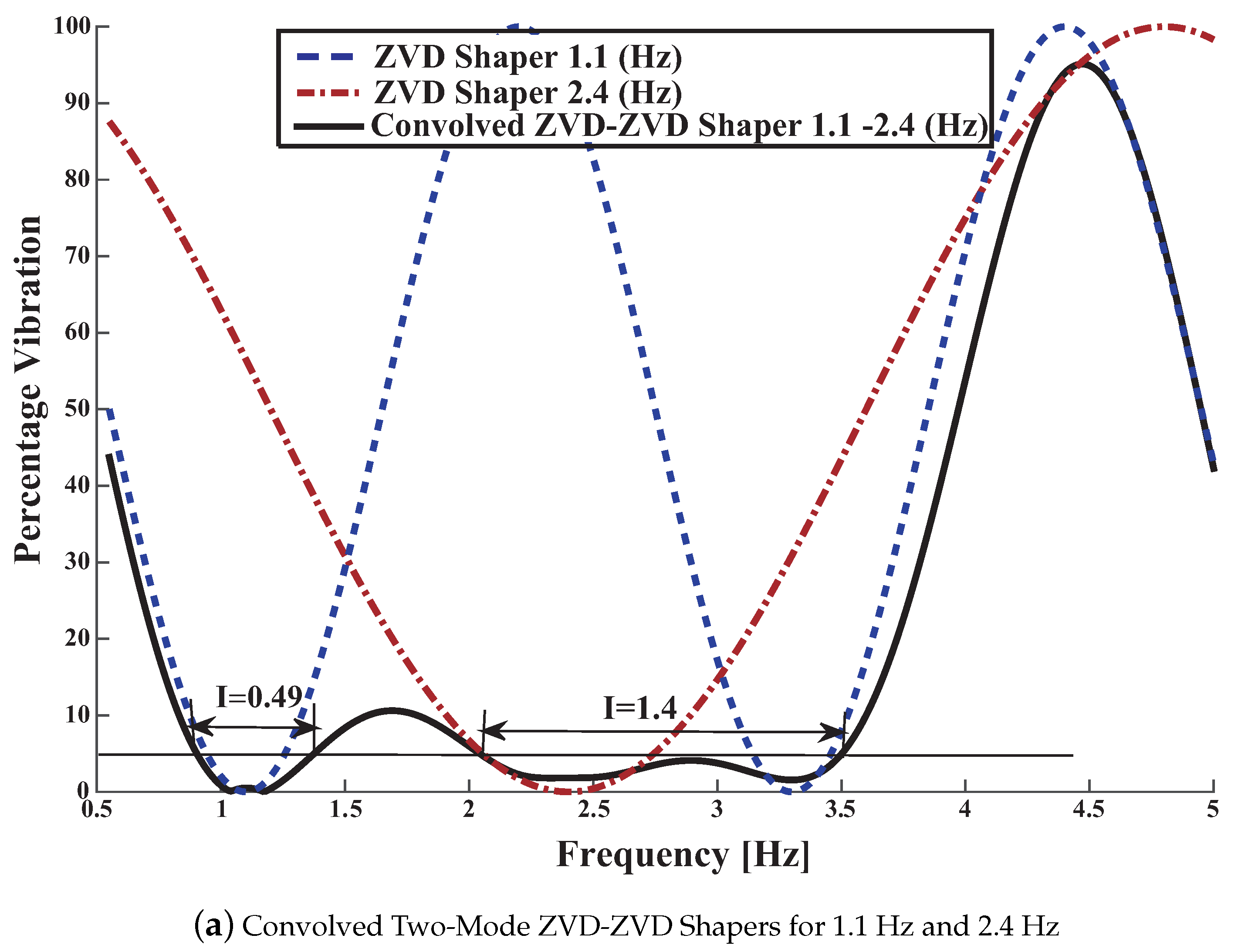
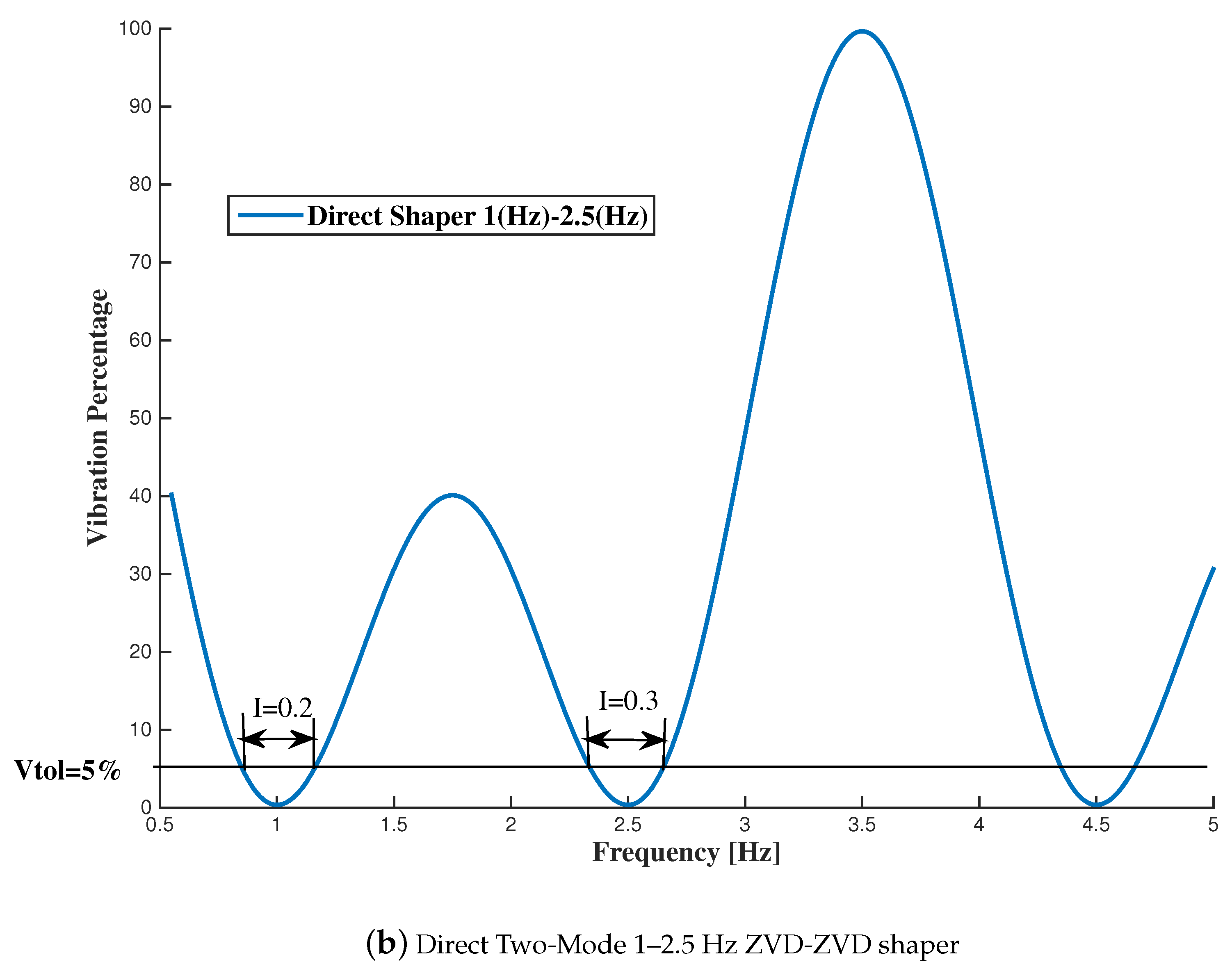
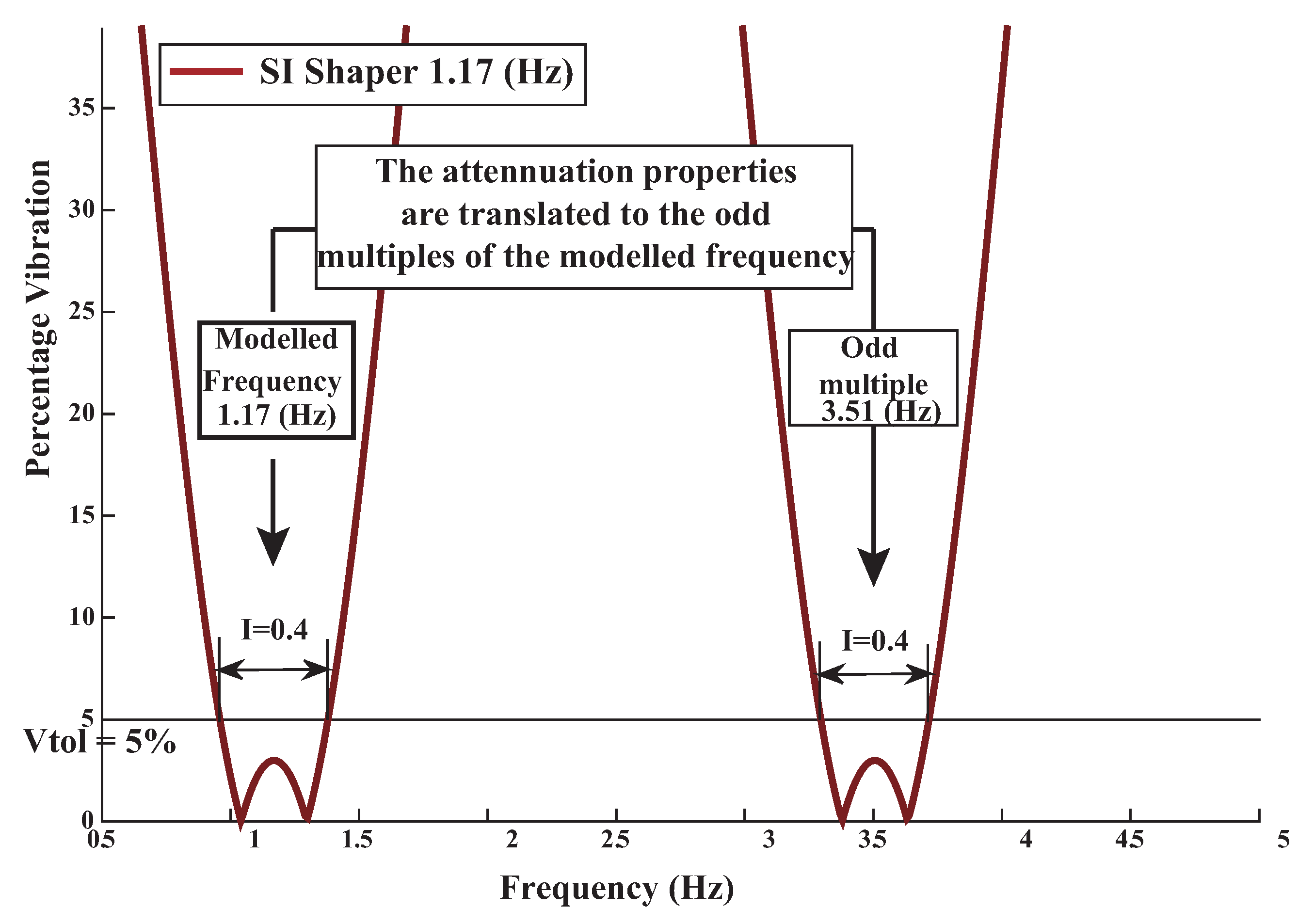
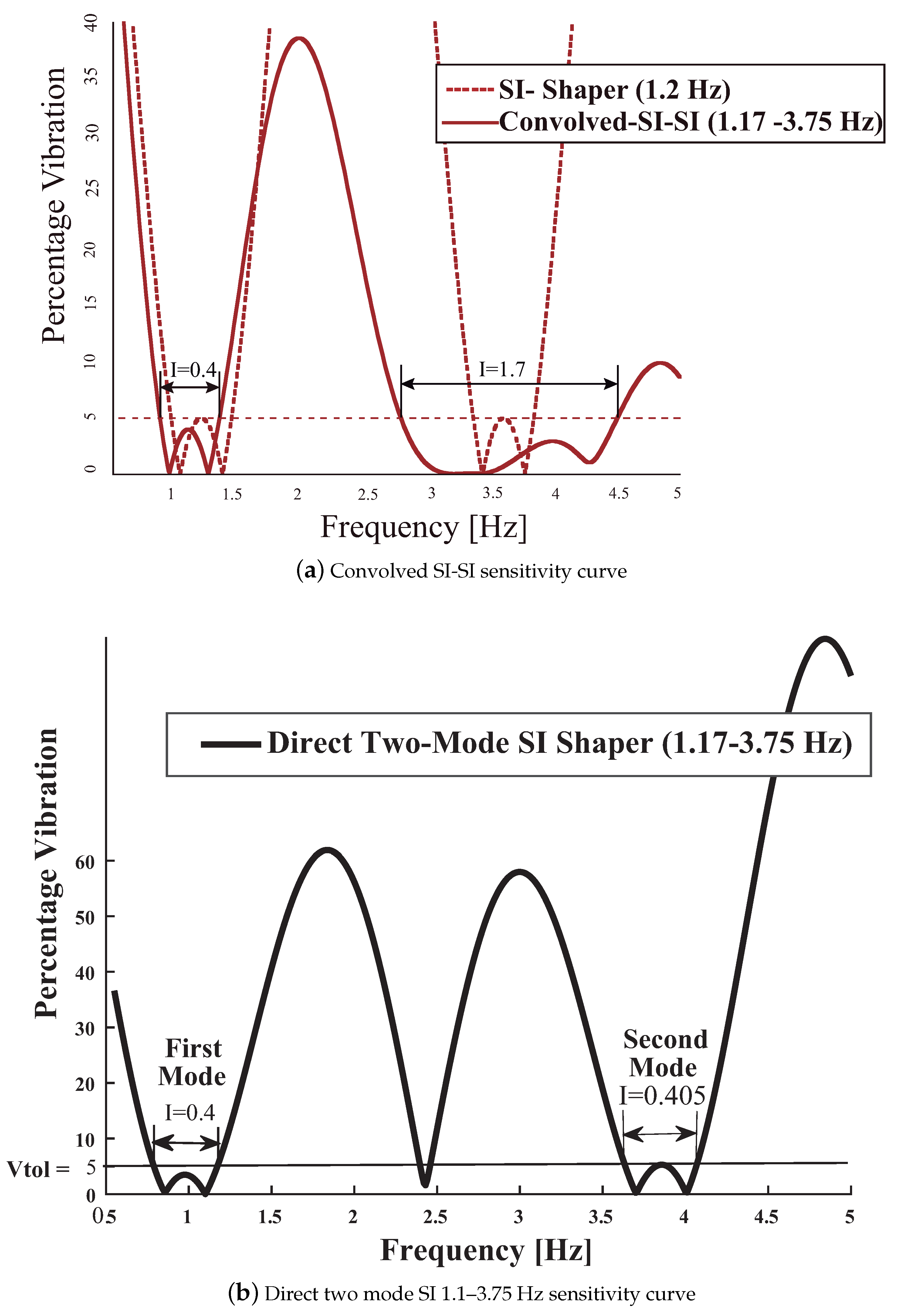
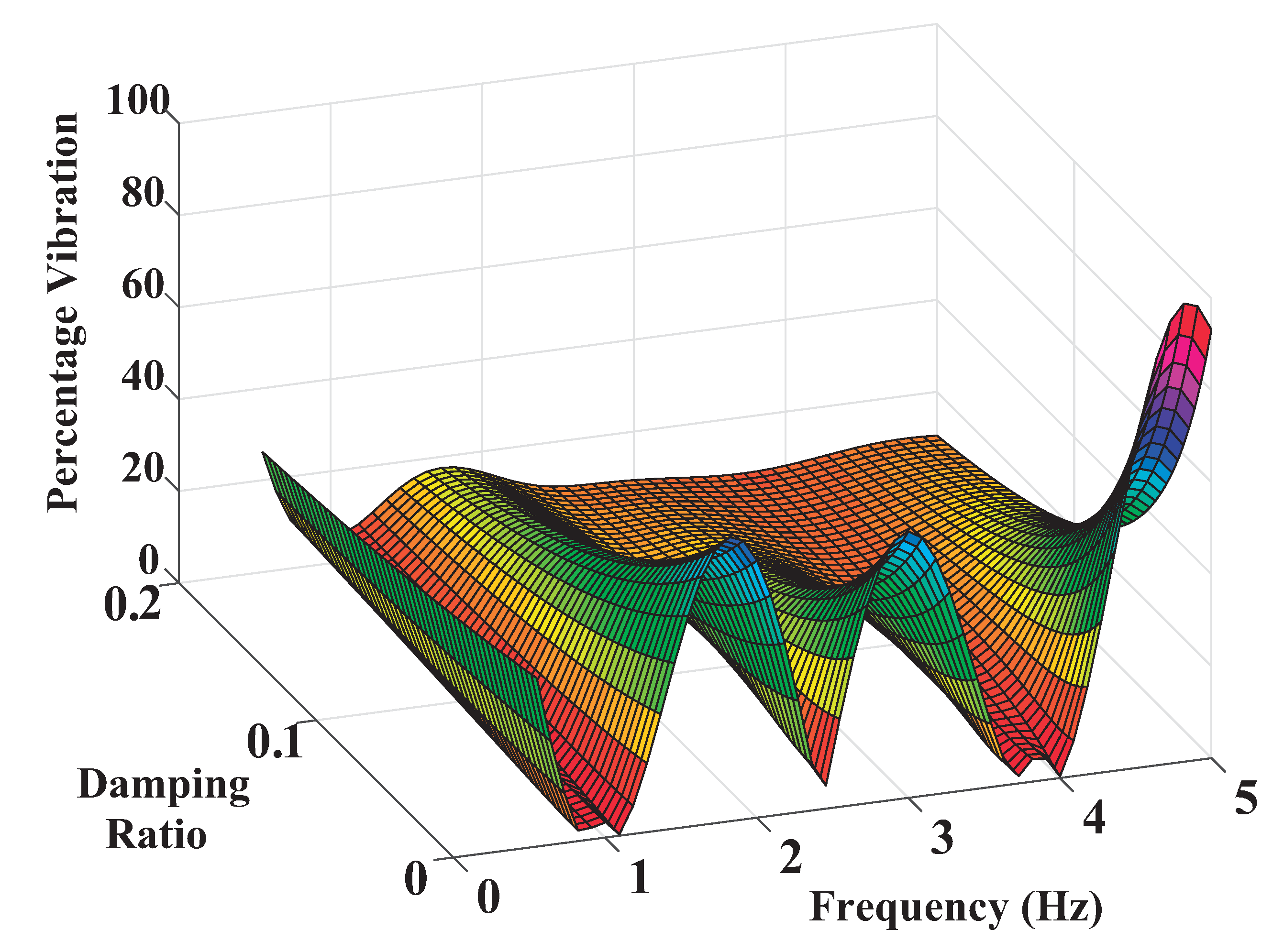
6. Multimode Specified Insensitivity Input Shapers for Multibody Systems
- Limit the residual vibration amplitude to below Vtol at the edges or guess limits of the frequency ranges to be cancelled [(1 − I/2) and (1 + I/2)] i = 1, 2.
- Set the residual vibration to Vtol at the unknown frequencies where the sensitivity curve reach one of the two local maximums.
- Set the slope of the sensitivity curve of such local maximums to zero.
- Force the residual vibration to zero at the four unknown touch frequencies twice for each associate hump of every mode.
- Solve the aforementioned constrain equations using a proven choice for highly nonlinear problems. At this point CONOPT’s efficient and reliable multi-method architecture handles a broad range of models. Specialized techniques achieve feasibility quickly, while powerful preprocessing tools reduce problem size and suggest formulation improvements.
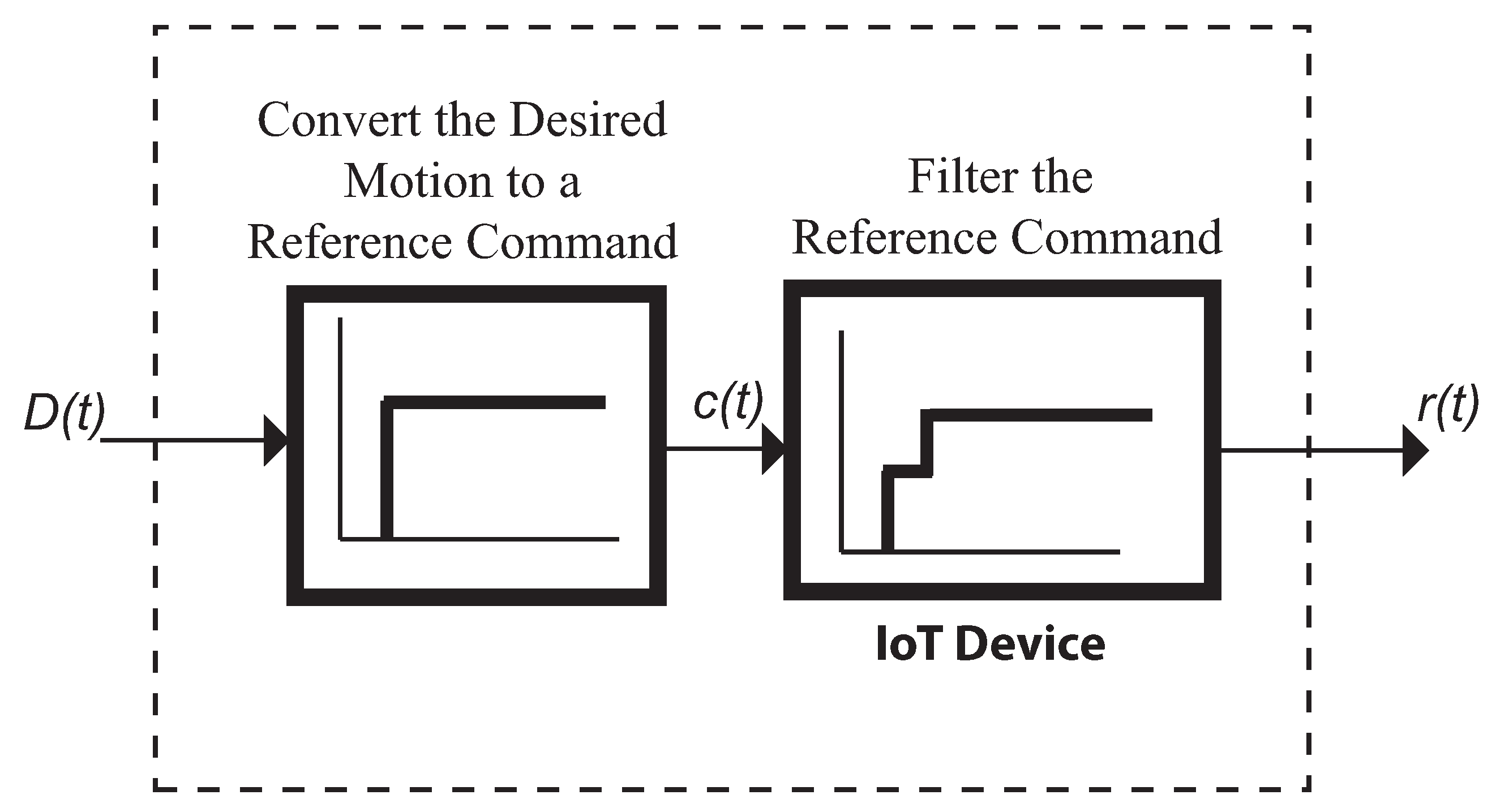
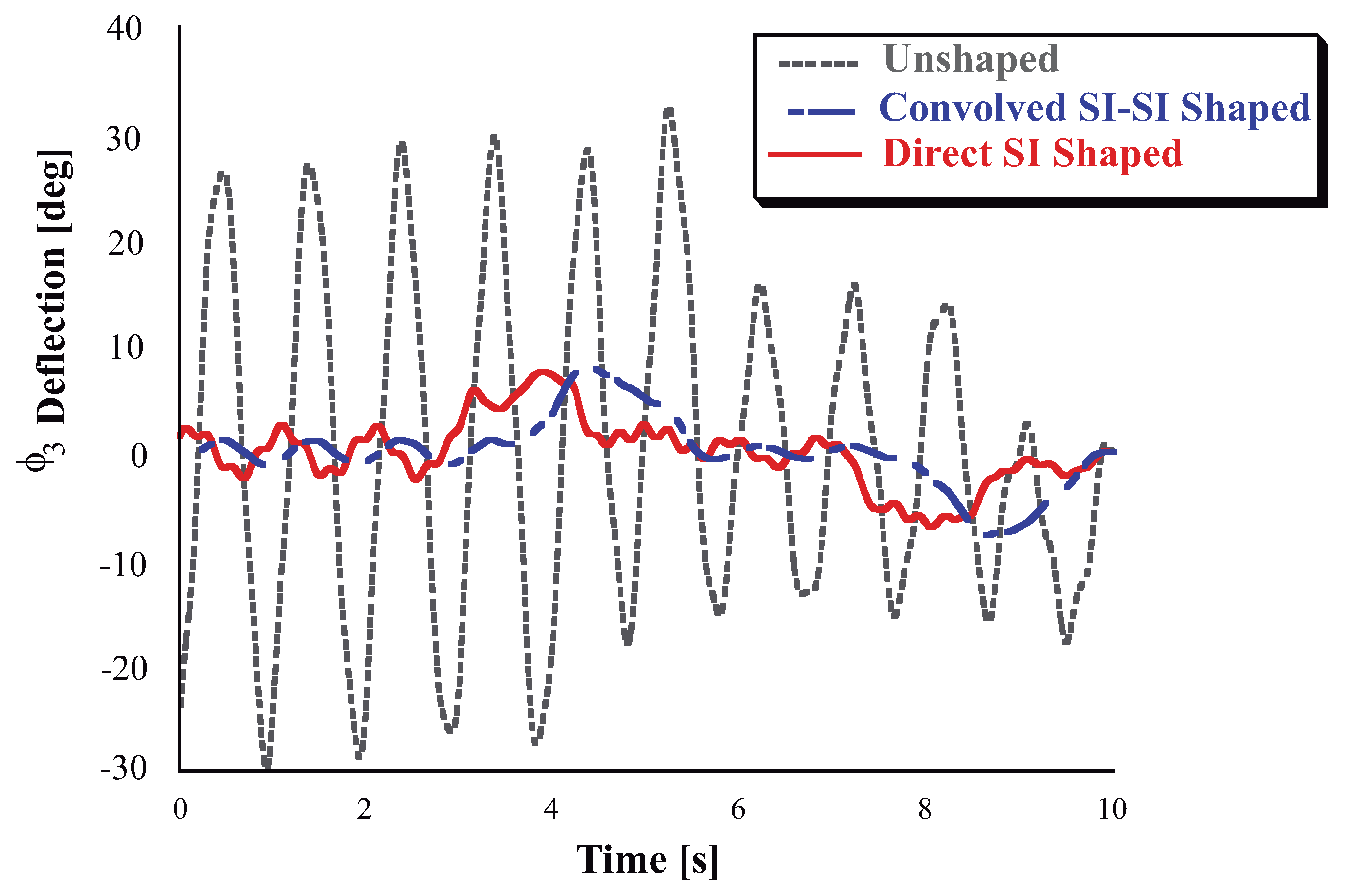
7. Embedded Input Shaping Design Methodology and Experimental Results
8. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| ODEs | ordinary differential equations |
| DAEs | differential algebraic equations |
| the vector since the origin of the local frames to the point | |
| ŭ | the vector u rotated counterclockwise 90° |
| local x-axis of body i at the mass centre | |
| local y-axis of body i at the mass centre | |
| rotational coordinate of body i | |
| X component of the mass centre of body i | |
| Y component of the mass centre of body i | |
| joints constraints equations | |
| velocity constraints equations | |
| D | Jacobian of the velocity constraints equations |
| the (right-hand side) of the acceleration constraints | |
| M | array of inertial properties of the bodies |
| h | external forces |
| Lagrange multipliers | |
| independent coordinate i | |
| A | State Space Jacobian matrix |
| Zero Vibration Shaper | |
| Zero Vibration and Derivative Shaper | |
| Specified Insensitivity Shaper | |
| Functional Mock-Up Interface |
References
- Haugh, E.J. Computer Aided Kinematics and Dynamics of Mechanical Systems; Allyn and Bacon Series in Engineering; Allyn and Bacon: Boston, MA, USA, 1989. [Google Scholar]
- García de Jalón, J.; Bayo, E. Kinematic and Dynamic Simulation of Multibody Systems: The Real-Time Challenge; Springer: New York, NY, USA, 1994. [Google Scholar]
- Nikravesh, P.E. Planar Multibody Dynamics: Formulation Programming and Applications; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Book, W.J. Controlled Motion in an Elastic World. J. Dyn. Syst. Meas. Control 1993, 115, 252–261. [Google Scholar] [CrossRef]
- Cook, G. Control of flexible structures via Posicast. In Proceedings of the Southeastern Symposium on System Theory, Knoxville, TN, USA, 7–8 April 1987; pp. 31–35. [Google Scholar]
- Junkins, J.L.; Kim, Y. Introduction to Dynamics and Control of Flexible Structures; American Institute of Aeronautics and Astronautics, Inc.: Washington, DC, USA, 1993. [Google Scholar]
- Banerjee, A.K.; Singhose, W.E. Minimum Time Fuel Efficient Maneuver of Flexible Spacecraft with Vibration Amplitude Constraint. In Proceedings of the AAS Astrodynamics Specialist Conference, Halifax, NS, Canada, 14–17 August 1995; Volume 95, pp. 318–323. [Google Scholar]
- Ben-Asher, J.; Burns, J.A.; Cliff, E.M. Time-Optimal Slewing of Flexible Spacecraft. J. Guid. Control Dyn. 1992, 15, 360–367. [Google Scholar] [CrossRef]
- Liu, Q.; Wie, B. Robust Time-Optimal Control of Uncertain Flexible Spacecraft. J. Guid. Control Dyn. 1992, 15, 597–604. [Google Scholar] [CrossRef]
- Pao, L.Y.; Singhose, W.E. A Comparison of Constant and Variable Amplitude Command Shaping Techniques for Vibration Reduction. In Proceedings of the IEEE Conference on Control Application Applications, Albany, NY, USA, 28–29 September 1995; pp. 875–881. [Google Scholar]
- Pao, L.Y. Characteristics of Time-Optimal Control of Flexible Structures with Damping. In Proceedings of the IEEE International Conference on Control and Applications, Glasgow, UK, 24–26 August 1994; pp. 1299–1304. [Google Scholar]
- Rogers, K.; Seering, W.P. Input Shaping for Limiting Loads and Vibration in Systems with On-Off Actuators. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, San Diego, CA, USA, 29–31 July 1996. [Google Scholar]
- Singhose, W.E.; Seering, W.P.; Banerjee, A.K. An Evaluation of Fuel-Efficient On-Off Input Shaping with Deflection Limiting. In Proceedings of the AIAA/AAS Astrodynamics Specialist Conference, San Diego, CA, USA, 29–31 July 1996. [Google Scholar]
- Singhose, W.E.; Seering, W.P.; Banerjee, A.K. Limiting Deflection During the Slewing of Flexible Spacecraft Using Deflection Sampling. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, San Diego, CA, USA, 29–31 July 1996. [Google Scholar]
- Blackburn, D.; Singhose, W.; Kitchen, J.; Patrangenaru, V.; Lawrence, J.; Kamoi, T.; Taura, A. Command Shaping for Nonlinear Crane Dynamics. J. Vib. Control 2010, 16, 477–501. [Google Scholar] [CrossRef]
- Singhose, W.E.; Singer, N.C. Input Shaping for Vibration Reduction with Specified Insensitivity to Modeling Errors. In Proceedings of the 1996 Japan-USA Symposium on Flexible Automation, Boston, MA, USA, 7–10 July 1996. [Google Scholar]
- Vaughan, J.; Kim, D.; Singhose, W.E. Control of Tower Cranes With Double-Pendulum Payload Dynamics. Trans. Control Syst. Tech. 2010, 18, 1345–1358. [Google Scholar] [CrossRef]
- Singhose, W.E.; Vaughan, J.; Peng, K.C.C.; Pridgen, B.; Glauser, U.; de Juanes Márquez, J.; Hong, S. Use of Cranes in Education an International Collaboration. J. Robot. Mechatron. 2011, 23, 881–892. [Google Scholar] [CrossRef]
- Singer, N.C.; Seering, W.P. An extension of Command Shaping Methods for Controlling Residual Vibration Using Frequency Sampling. In Proceedings of the IEEE International Conference on Robotics and Automation, Nice, France, 12–14 May 1992; Volume 1, pp. 800–805. [Google Scholar]
- Singhose, W.E.; Porter, L.; Tuttle, T.D.; Singer, N. Vibration reduction Using Multi-Hump Input Shapers. Trans. ASME J. Dyn. Syst. Meas. Control 1997, 119, 320–326. [Google Scholar] [CrossRef]
- Singh, T.; Valadi, S.R. Robust Time-Delay Control. ASME J. Dyn. Syst. Meas. Control 1993, 112, 303–306. [Google Scholar] [CrossRef]
- Singh, T. Minimax Design of Robust Controllers for Flexible Systems. J. Guid. Control Dyn. 2002, 25, 868–875. [Google Scholar] [CrossRef]
- Singh, T. Minimax Input Shaper Design Using Linear Programming. J. Dyn. Syst. Meas. Control 2008, 130, 9–18. [Google Scholar] [CrossRef]
- Singhose, W.E.; Potter, L.; Singer, N.C. Vibration Reduction Using Multi-Hump Extra Insensitive Input Shapers. In Proceedings of the American Control Conference, Seattle, WA, USA, 21–23 June 1995; pp. 3830–3834. [Google Scholar]
- Chatlatanagulchai, W.; Kijdech, D.; Benjalersyarnon, T. Quantitative Feedback Input Shaping for Flexible-Joint Robot Manipulator. J. Dyn. Syst. Meas. Control 2016, 138, 061006. [Google Scholar] [CrossRef]
- Sung, Y.-G.; Jang, W.-S.; Kim, J.-Y. Negative Input Shaped Commands for Unequal Acceleration and Braking Delays of Actuators. J. Dyn. Syst. Meas. Control 2018, 140, 094501. [Google Scholar] [CrossRef]
- Hung, J.Y. Posicast Control Past and Present. IEEE Multidiscip. Eng. Educ. Mag. 2007, 2, 7–11. [Google Scholar]
- Smith, O.J.M. Posicast control of damped oscillatory systems. Proc. IRE 1957, 45, 1249–1255. [Google Scholar] [CrossRef]
- Singh, T. Optimal Reference Shaping for Dynamical Systems: Theory and Applications; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Singhose, W.E.; Seering, W. Command Generation for Dynamic Systems; Lulu Press: Morrisville, NC, USA, 2012. [Google Scholar]
- Singhose, W.E.; Kivila, A.; Seering, W. Development of an Interactive Textbook for Command-Shaping Control Methods. In Proceedings of the ASME Dynamics Systems and Control Conference, Stanford, CA, USA, 21–23 October 2013. [Google Scholar]
- Yoon, S.; Howe, R.M.; Greenwood, D.T. Constraint Violation Stabilization Using Gradient feedback in Constrained Dynamics Simulation. J. Guid. Control Dyn. 1992, 15, 1467–1474. [Google Scholar] [CrossRef]
- Flores, P.; Seabra, E. Dynamics of Planar Multibody Systems: Concept, Formulation and Resolution; VDM Verlag Dr. Müller: Saarbrücken, Germany, 2010; ISBN-13: 978-3-8364-7746-8. [Google Scholar]
- Gómez, M.J.; Castejón, C.; García-Prada, J.C.; Ceccarelli, M. Analysis and Comparison of Motion Capture Systems for Human Walking. Exp. Tech. 2016, 40, 875–883. [Google Scholar] [CrossRef]
- Singhose, W.E. Command Generation for Flexible Systems. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 9 May 1997. [Google Scholar]
- Peláez, G.; Singhose, W. Implementation of Input Shaping on Flexible Machines with Integer Controllers. IFAC Proc. Vol. 2002, 35, 37–42. [Google Scholar] [CrossRef]
- Peláez, G.; Caparrini, N.; García-Prada, J.C. Two Impulse Sequence Input Shaper Design for Link Mechanism with Non-Linearities. In Proceedings of the American Control Conference, New York, NY, USA, 9–13 July 2007; pp. 822–827, ISBN 1-4244-0989-6. [Google Scholar]


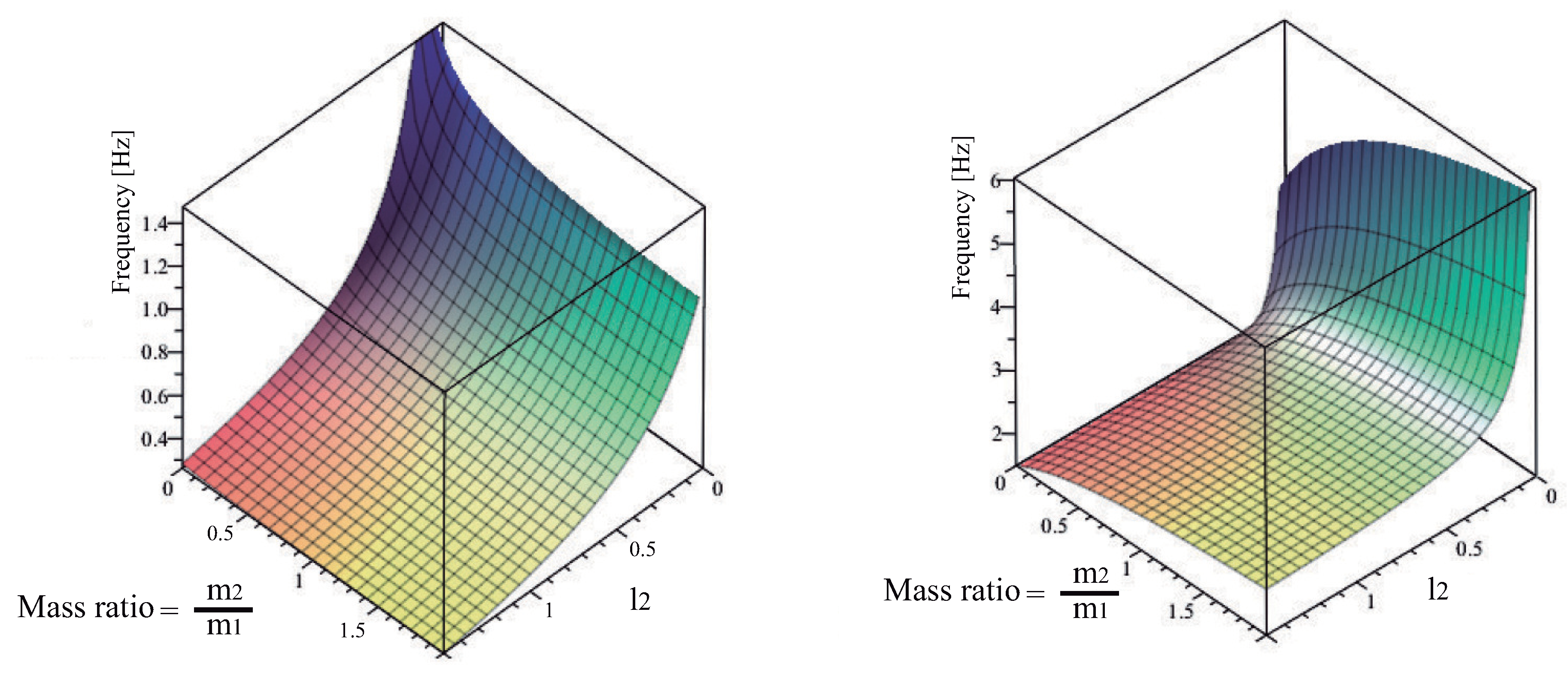
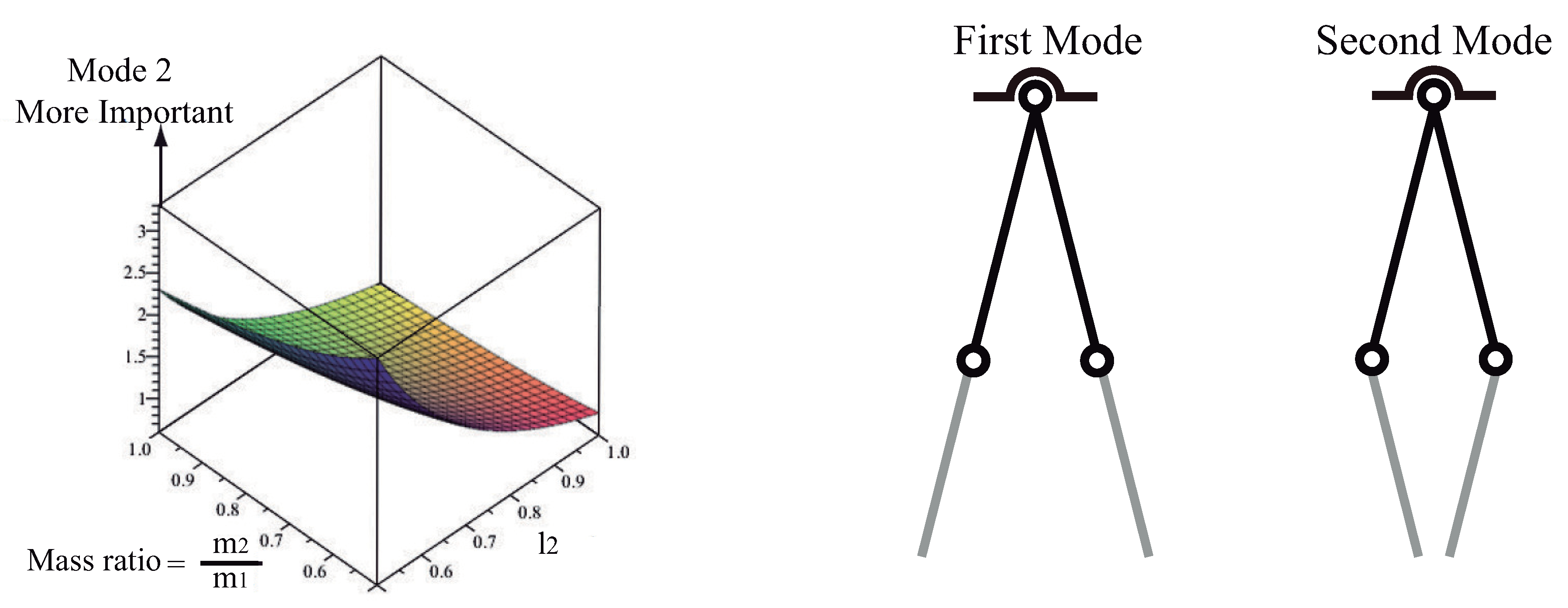
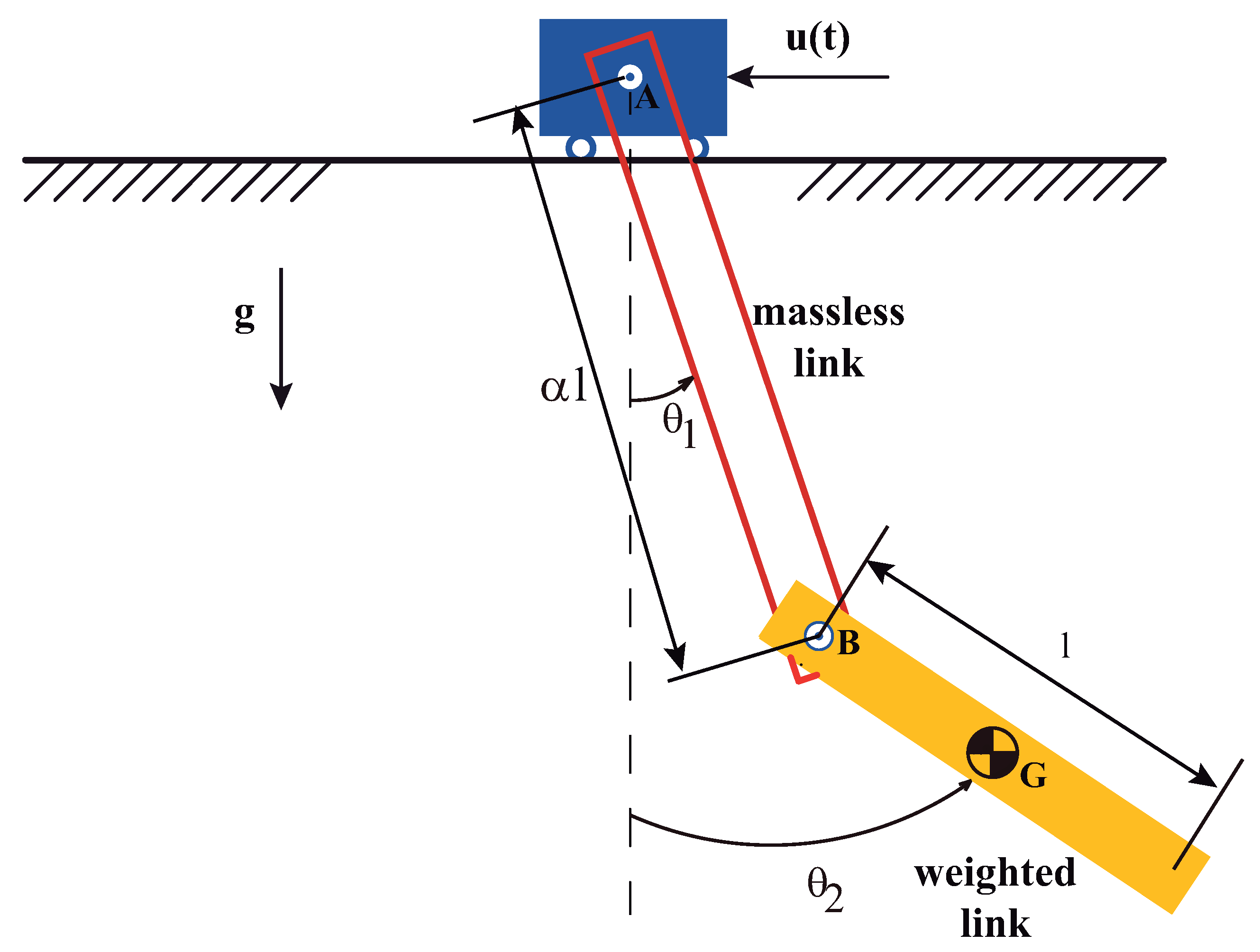
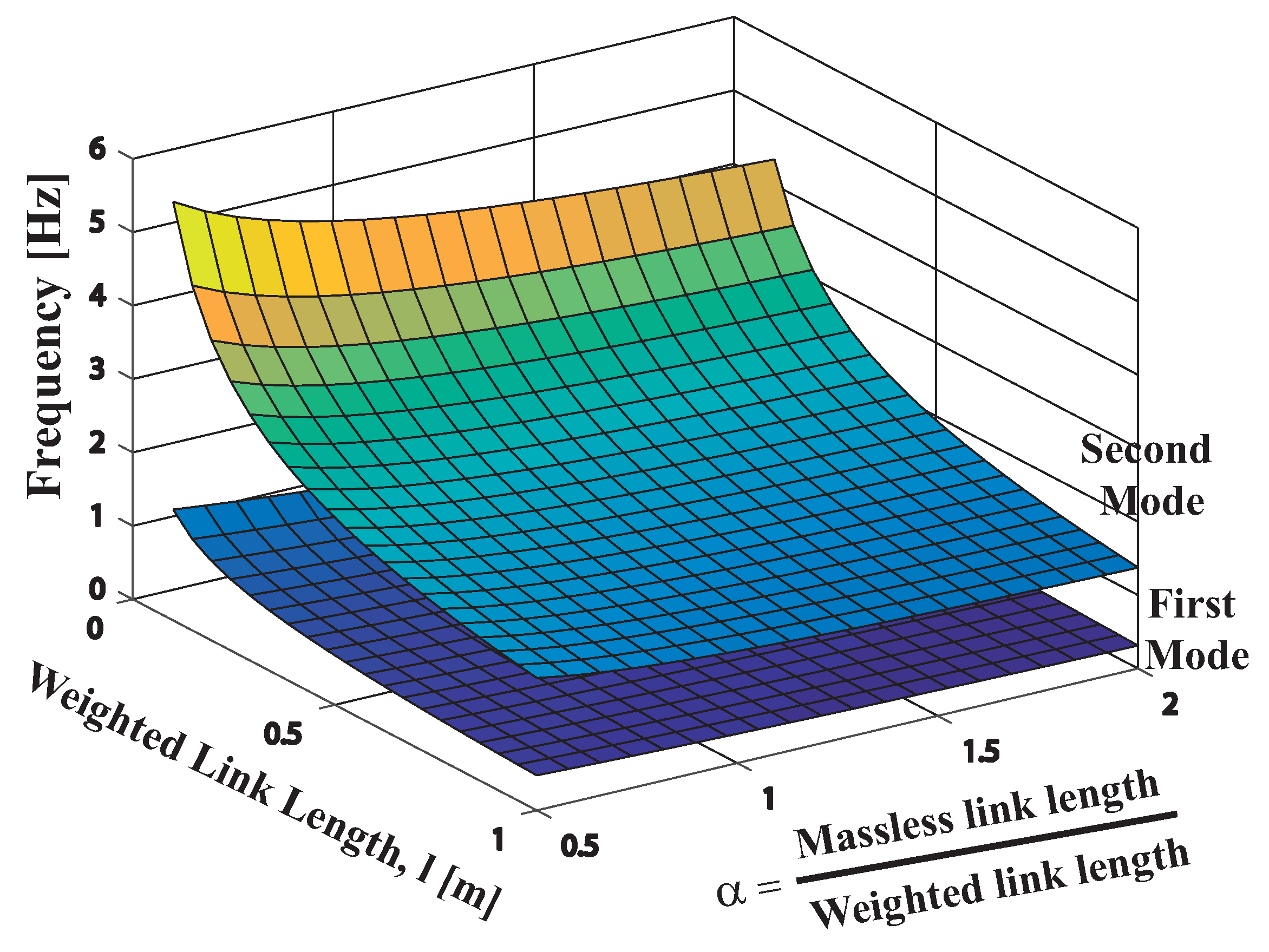




| Multibody Model Parameter | Value |
|---|---|
| −0.0825 (m) | |
| 0.0825 (m) | |
| −0.070 (m) | |
| 2.26875 × (Kgr·m) | |
| 1.21422 × (Kgr·m) | |
| 585 (gr) | |
| 99.12 (gr) | |
| 74.34 (gr) |
| Length [m] | • | • | • | • |
|---|---|---|---|---|
| l = 0.1 | 1.5097 | 1.2622 | 1.1491 | 0.9900 |
| l = 0.2 | 1.0675 | 0.8925 | 0.8125 | 0.7001 |
| l = 0.3 | 0.8716 | 0.7287 | 0.6634 | 0.5716 |
| l = 0.4 | 0.7548 | 0.6311 | 0.5745 | 0.4950 |
| l = 0.5 | 0.6751 | 0.5645 | 0.5139 | 0.4428 |
| • | ||||
|---|---|---|---|---|
| Length [m] | • | • | • | • |
| l = 0.1 | • | 4.8224 | 4.5873 | 4.3473 |
| l = 0.2 | • | 3.4100 | 3.2437 | 3.0740 |
| l = 0.3 | • | 2.7842 | 2.6485 | 2.5099 |
| l = 0.4 | • | 2.4112 | 2.2937 | 2.1737 |
| l = 0.5 | • | 2.1567 | 2.0515 | 1.9442 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peláez, G.; Vaugan, J.; Izquierdo, P.; Rubio, H.; García-Prada, J.C. Dynamics and Embedded Internet of Things Input Shaping Control for Overhead Cranes Transporting Multibody Payloads. Sensors 2018, 18, 1817. https://doi.org/10.3390/s18061817
Peláez G, Vaugan J, Izquierdo P, Rubio H, García-Prada JC. Dynamics and Embedded Internet of Things Input Shaping Control for Overhead Cranes Transporting Multibody Payloads. Sensors. 2018; 18(6):1817. https://doi.org/10.3390/s18061817
Chicago/Turabian StylePeláez, Gerardo, Joshua Vaugan, Pablo Izquierdo, Higinio Rubio, and Juan Carlos García-Prada. 2018. "Dynamics and Embedded Internet of Things Input Shaping Control for Overhead Cranes Transporting Multibody Payloads" Sensors 18, no. 6: 1817. https://doi.org/10.3390/s18061817
APA StylePeláez, G., Vaugan, J., Izquierdo, P., Rubio, H., & García-Prada, J. C. (2018). Dynamics and Embedded Internet of Things Input Shaping Control for Overhead Cranes Transporting Multibody Payloads. Sensors, 18(6), 1817. https://doi.org/10.3390/s18061817







